Investigation on Dynamic Mechanical Properties of Recycled Concrete Aggregate under Split-Hopkinson Pressure Bar Impact Test
Abstract
:1. Introduction
2. Materials and Methods
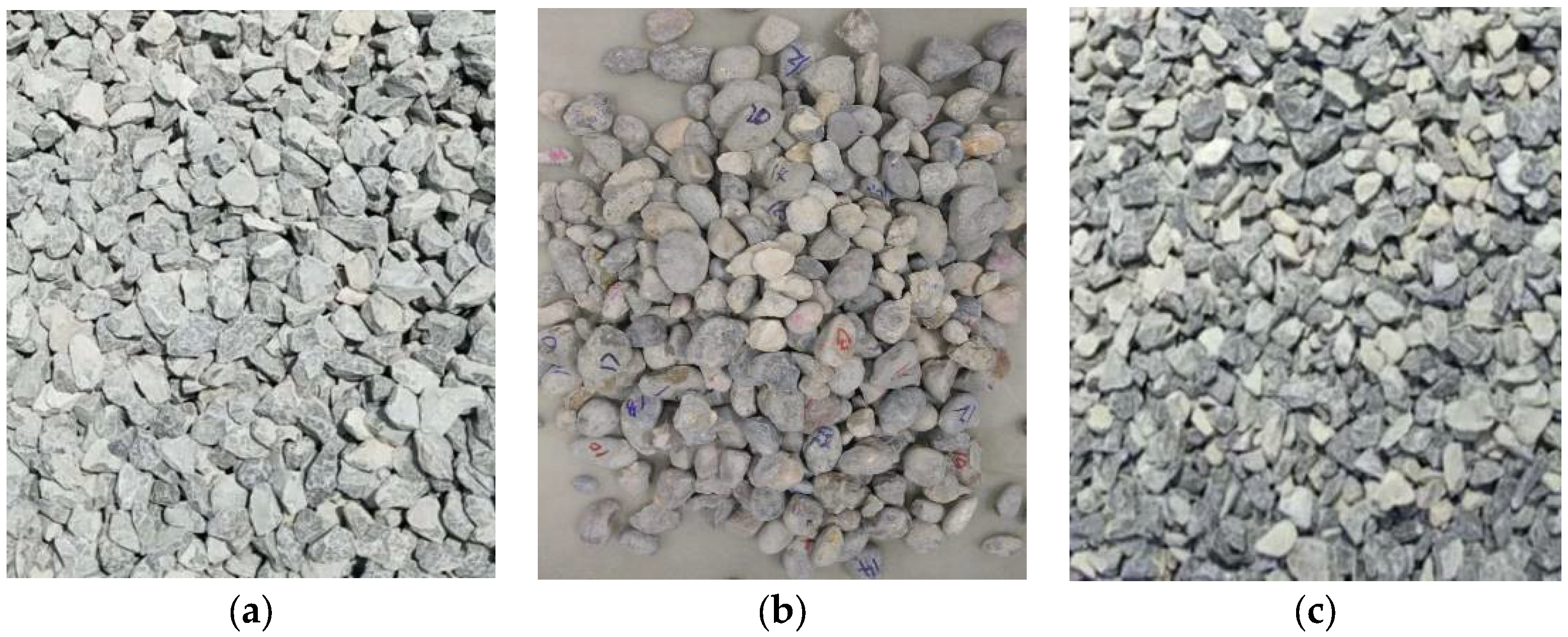
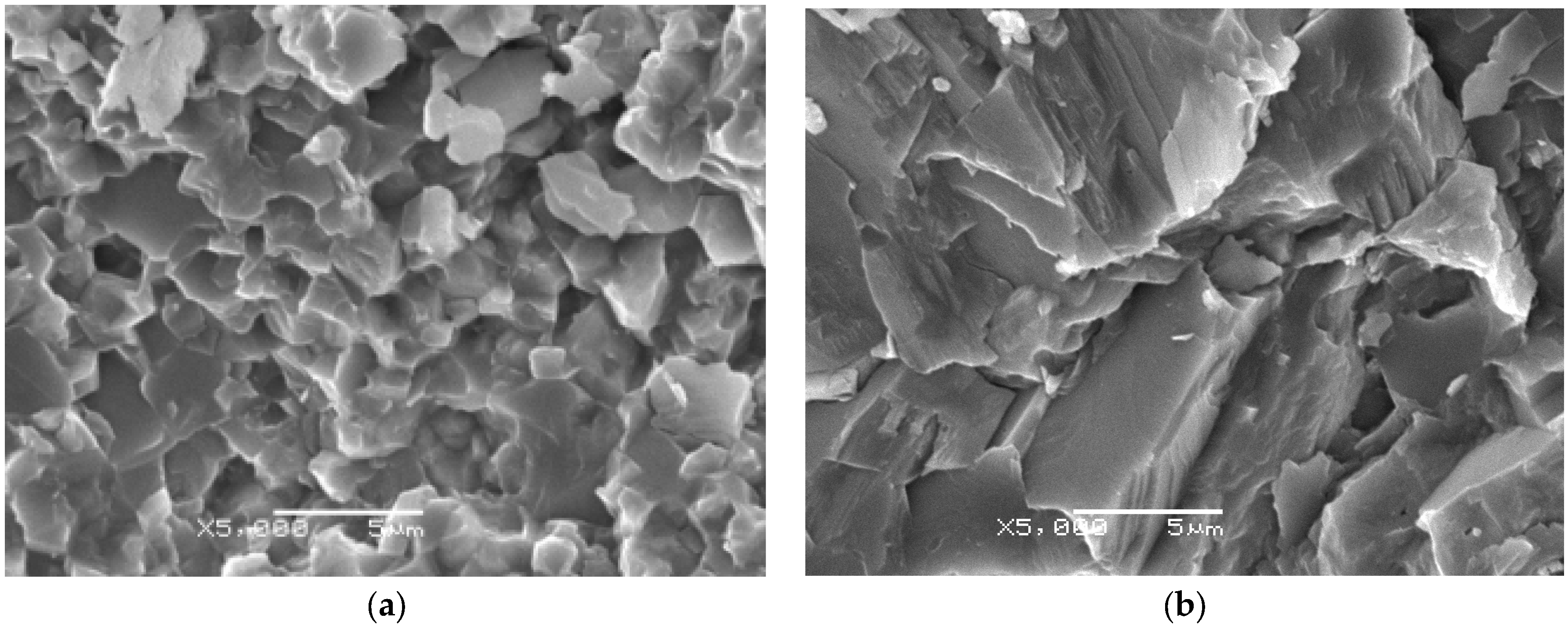
2.1. Materials and Specimens
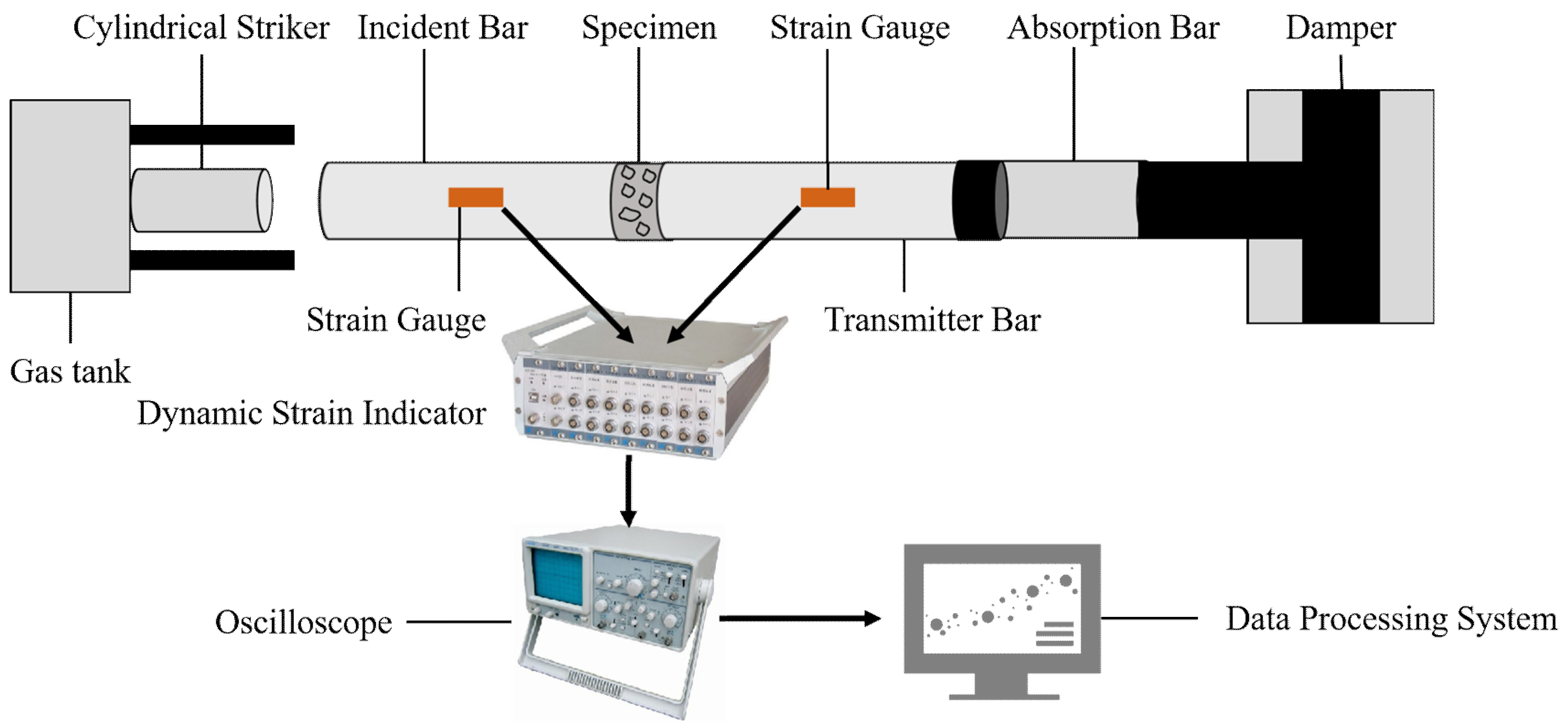
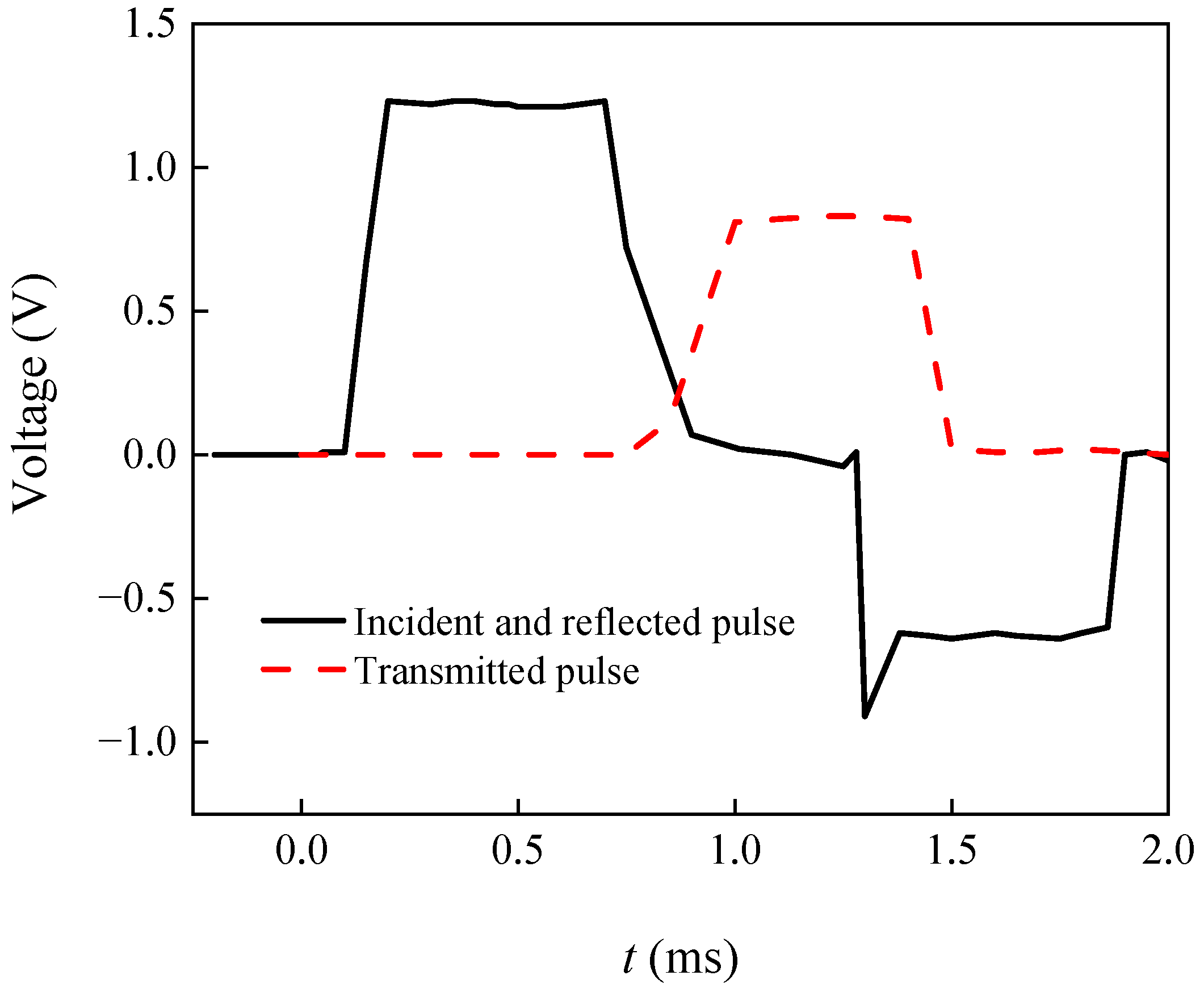
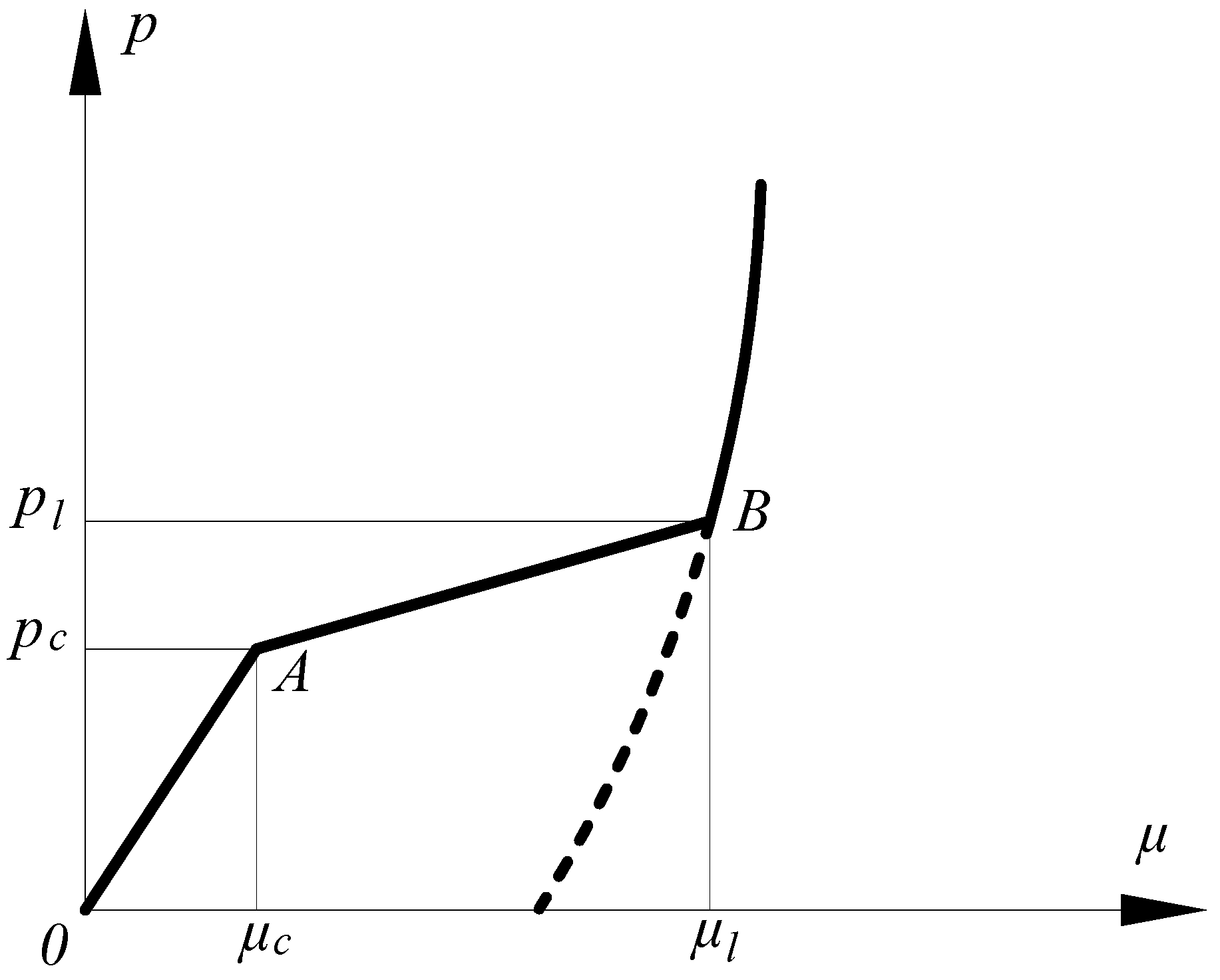
2.2. SHPB Test
3. Experimental Results
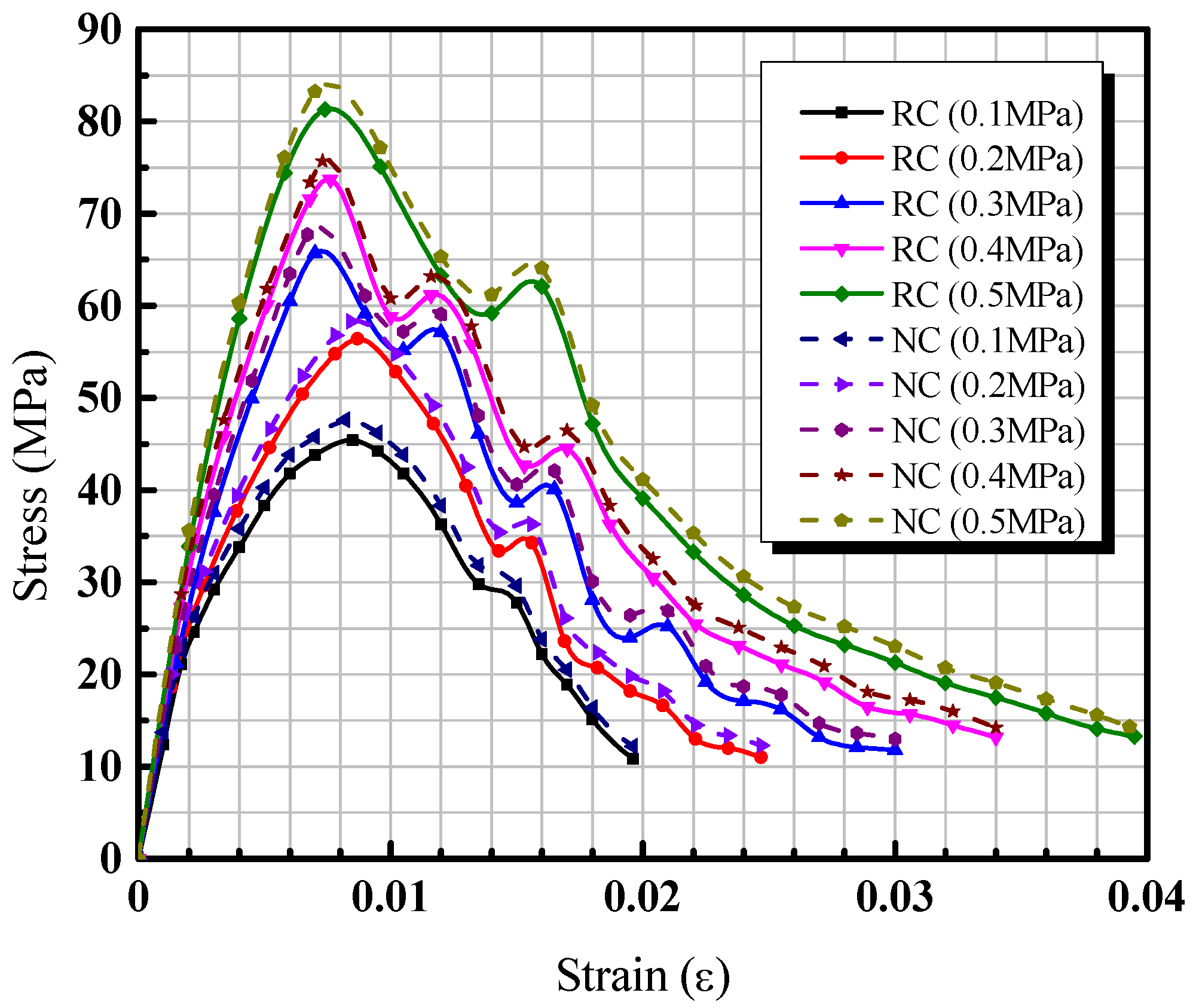
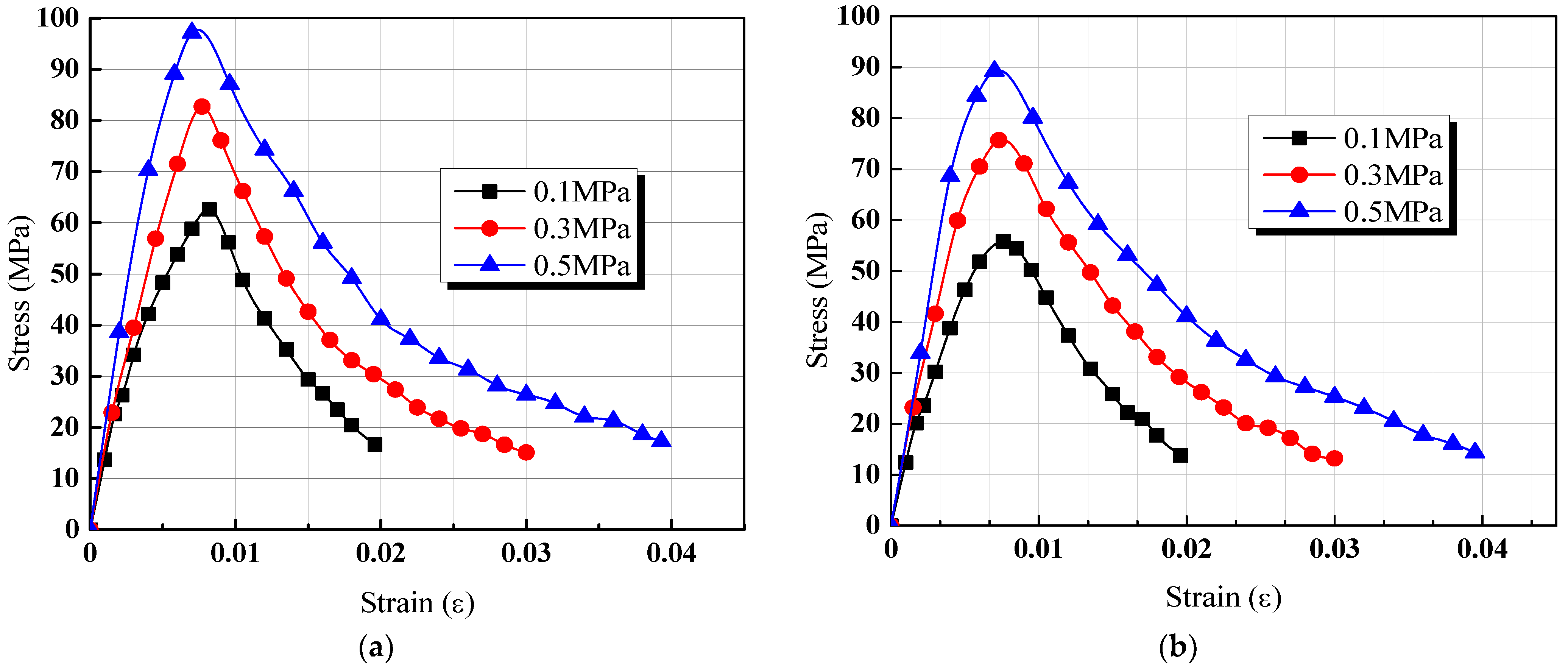
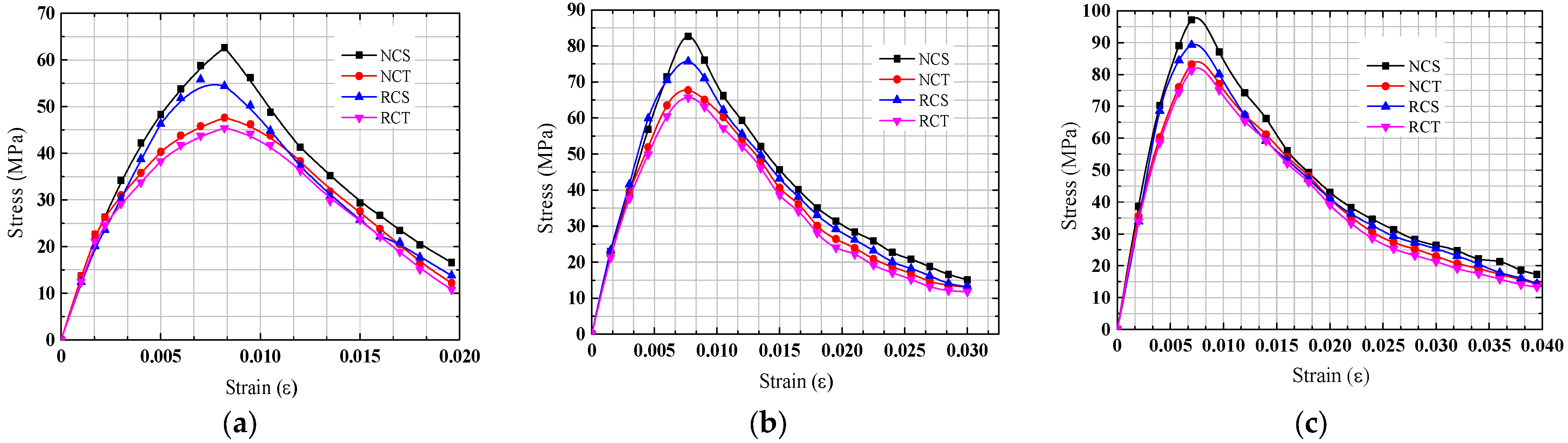
3.1. Stress–Strain Curves
3.2. Parameters Study
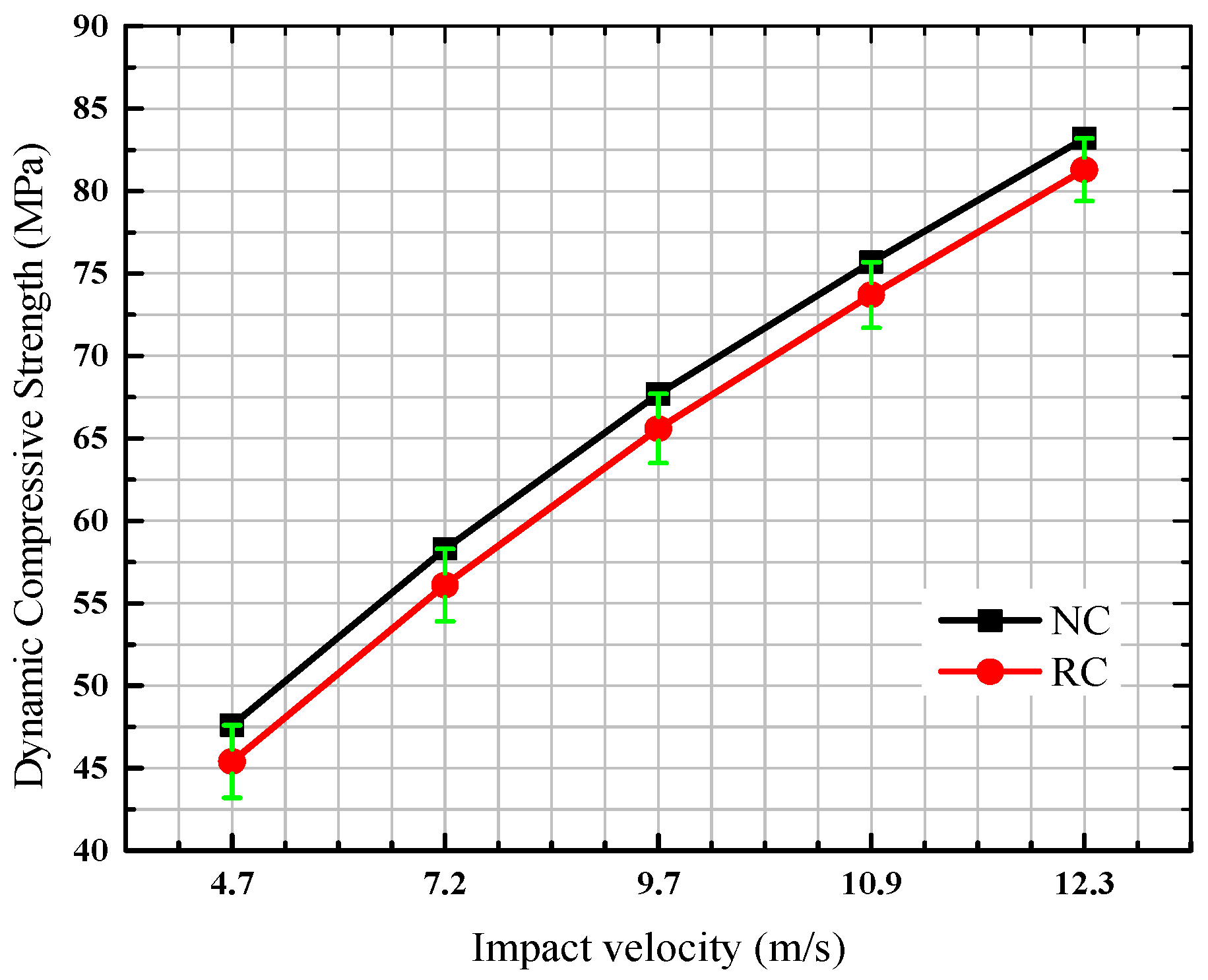
3.2.1. Dynamic Compressive Strength
3.2.2. Dynamic Increase Factor
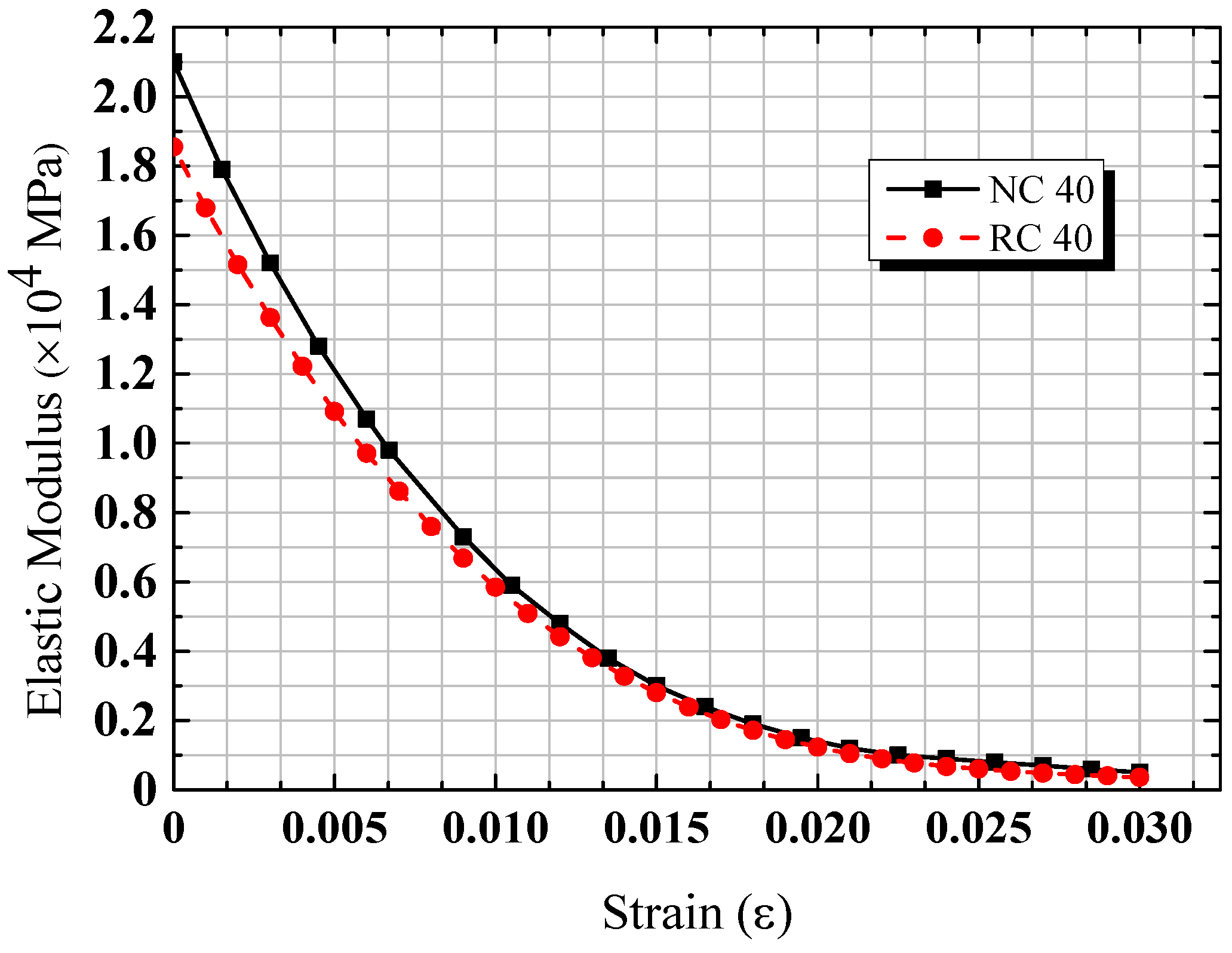
3.2.3. Initial Elastic Modulus
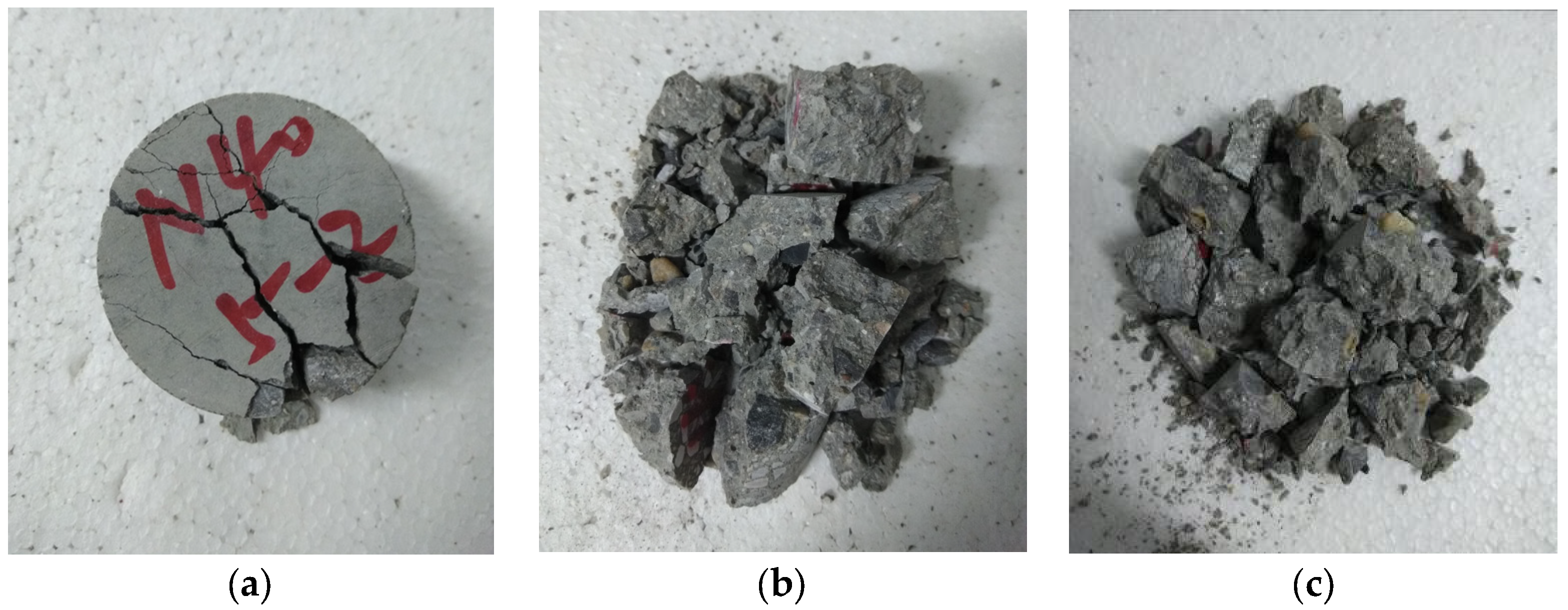
3.2.4. Failure Modes
4. Numerical Model Validation
4.1. Numerical FEM Model
4.2. Impact Stress–Strain Curves
4.3. Failure Modes
5. Conclusions
- (1)
- Quasi-static test results showed that the compressive strength of the NC was about 1.05 times higher than that of the RC. The dynamic results obtained suggest that the compressive strength of the NC was higher than that of the RC. With the increase in impact velocity, the compressive strength and the dynamic increase factor increased gradually. According to the impact tests, the logistic function expression of dynamic increase factor was obtained.
- (2)
- From the relationship between stress and the varying strain rates, it can be concluded that the stress–strain curves showed a linear behavior at the initial stage of loading. When the stress reached the maximum value, the curves presented a decreasing tendency. The concrete specimens presented the strain softening phenomenon.
- (3)
- With the increase in impact velocity, the growth rate of dynamic compressive strength became smaller. The expression of DIF gives an adequate estimate of the dynamic compression strength of the RC and NC within the strain rate range of 40–100 s−1. The proposed empirical model gives an adequate estimate of stress–strain curves of the RC and NC at the impact velocity of 9.6 m/s.
- (4)
- The initial elastic modulus showed a decreasing tendency with the increase in strain. The strain had a significant influence on the initial elastic modulus when the strain was less than 0.010, while they were in good agreement with each other when the strain was more than 0.010.
- (5)
- Failure modes can conclude that the damage degree of concrete developed from the edge of specimens. Then, the failure gradually extends to the center of the specimens. With the increase in impact time, many through-cracks originated at the center of specimens. Then, the main part of the specimens were completely separated and destroyed. The simulation results showed good agreement with the SHPB impact test. Therefore, this model was feasible for performing qualitative analysis of the SHPB impact test of the RC.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Islam, M.J.; Islam, K.; Shahjalal, M.; Khatun, E.; Islam, S.; Razzaque, A.B. Influence of different types of fibers on the mechanical properties of recycled waste aggregate concrete. Constr. Build. Mater. 2022, 337, 127577. [Google Scholar] [CrossRef]
- Huang, Y.J.; Wang, T.C.; Sun, H.L.; Li, C.X.; Yin, L.; Wang, Q. Mechanical properties of fibre reinforced seawater sea-sand recycled aggregate concrete under axial compression. Constr. Build. Mater. 2022, 331, 127338. [Google Scholar] [CrossRef]
- McGinnis, M.J.; Davis, M.; Rosa, A.D.L.; Weldon, B.D.; Kurama, Y.C. Strength and stiffness of concrete with recycled concrete aggregates. Constr. Build. Mater. 2017, 154, 258–269. [Google Scholar] [CrossRef]
- Akbarnezhad, A.; Ong, K.C.G.; Zhang, M.H.; Tam, C.T.; Foo, T.W.J. Microwave-assisted beneficiation of recycled concrete aggregates. Constr. Build. Mater. 2011, 25, 3469–3479. [Google Scholar] [CrossRef]
- Braga, A.M.; Silvestre, J.D.; Brito, J.D. Compared environmental and economic impact from cradle to gate of concrete with natural and recycled coarse aggregates. J. Clean. Prod. 2017, 162, 529–543. [Google Scholar] [CrossRef]
- Wang, C.Q.; Xiao, J.Z. Evaluation of the stress-strain behavior of confined recycled aggregate concrete under monotonic dynamic loadings. Cement. Concrete. Comp. 2018, 87, 149–163. [Google Scholar] [CrossRef]
- Xuan, D.X.; Zhan, B.J.; Poon, C.S. Durability of recycled aggregate concrete prepared with carbonated recycled concrete aggregates. Cement. Concrete. Comp. 2017, 84, 214–221. [Google Scholar] [CrossRef]
- Saravanakumar, P.; Abhiram, K.; Manoj, B. Properties of treated recycled aggregates and its influence on concrete strength characteristics. Constr. Build. Mater. 2016, 111, 611–617. [Google Scholar] [CrossRef]
- Song, I.H.; Ryou, J.S. Hybrid techniques for quality improvement of recycled fine aggregate. Constr. Build. Mater. 2014, 72, 56–64. [Google Scholar] [CrossRef]
- Du, W.P. Research on Beneficiation Technique of High Quality Recycled Concrete Aggregate; Xi’an University of Science and Technology: Xi’an, China, 2017. (In Chinese) [Google Scholar]
- Aslani, F.; Ma, G.W.; Wan, D.L.Y.; Muselin, G. Development of high-performance self-compacting concrete using waste recycled concrete aggregates and rubber granules. J. Clean. Prod. 2018, 182, 553–566. [Google Scholar] [CrossRef]
- Feng, W.H.; Tang, Y.C.; He, W.M.; Wei, W.B.; Yang, Y.M. Mode I dynamic fracture toughness of rubberised concrete using a drop hammer device and split Hopkinson pressure bar. J. Build. Eng. 2022, 48, 103995. [Google Scholar] [CrossRef]
- Bai, W.F.; Shen, J.X.; Guan, J.F.; Wang, J.Y.; Yuan, C.Y. Study on compressive mechanical properties of reycled aggregate concrete with silica fume at different strain rates. Mater. Today. Commun. 2022, 31, 103444. [Google Scholar] [CrossRef]
- Tang, Y.X.; Xiao, J.Z.; Zhang, H.H.; Duan, Z.H.; Xia, B. Mechanical properties and uniaxial compressive stress-strain behavior of fully recycled aggregate concrete. Constr. Build. Mater. 2022, 323, 126546. [Google Scholar] [CrossRef]
- Chen, A.J.; Han, X.Y.; Chen, M.; Wang, X.Y.; Wang, Z.H.; Guo, T.T. Mechanical and stress-strain behavior of basalt fiber reinforced rubberized recycled coarse aggregate concrete. Constr. Build. Mater. 2020, 260, 119888. [Google Scholar] [CrossRef]
- Xiao, J.Z.; Li, L.; Shen, L.M.; Poon, C.S. Compressive behaviour of recycled aggregate concrete under impact loading. Cement. Concrete. Res. 2015, 71, 46–55. [Google Scholar] [CrossRef]
- Xiao, J.Z.; Zhang, K.J.; Akbarnezhad, A. Variability of stress-strain relationship for recycled aggregate concrete under uniaxial compression loading. J. Clean. Prod. 2018, 181, 753–771. [Google Scholar] [CrossRef]
- Ramesh, K.T.; Hogan, J.D.; Kimberley, J.; Stickle, A. A review of mechanisms and models for dynamic failure, strength, and fragmentation. Planet. Space. Sci. 2015, 107, 10–23. [Google Scholar] [CrossRef]
- Rao, M.C.; Bhattacharyya, S.K.; Barai, S.V. Behaviour of recycled aggregate concrete under drop weight impact load. Constr. Build. Mater. 2011, 25, 69–80. [Google Scholar]
- Kong, X.Z.; Fang, Q.; Chen, L.; Wu, H. A new material model for concrete subjected to intense dynamic loadings. Int. J. Impact. Eng. 2018, 120, 60–78. [Google Scholar] [CrossRef]
- Guo, H.; Shi, C.J.; Guan, X.M.; Zhu, J.P.; Ding, Y.H.; Ling, T.C.; Zhang, H.B.; Wang, Y.L. Durability of recycled aggregate concrete—A review. Cement. Concrete. Comp. 2018, 89, 251–259. [Google Scholar] [CrossRef]
- Luccioni, B.; Isla, F.; Forni, D.; Cadoni, E. Modelling UHPFRC tension behavior under high strain rates. Cement. Concrete. Comp. 2018, 91, 209–220. [Google Scholar] [CrossRef]
- Jin, L.; Yu, W.X.; Du, X.L.; Zhang, S.; Li, D. Meso-scale modelling of the size effect on dynamic compressive failure of concrete under different strain rates. Int. J. Impact. Eng. 2019, 125, 1–12. [Google Scholar] [CrossRef]
- Li, W.G.; Luo, Z.Y.; Long, C.; Wu, C.Q.; Duan, W.H.; Shah, S.P. Effects of nanoparticle on the dynamic behaviors of recycled aggregate concrete under impact loading. Mater. Design. 2016, 112, 58–66. [Google Scholar] [CrossRef]
- Li, W.G.; Luo, Z.Y.; Wu, C.Q.; Tam, V.W.Y.; Duan, W.H.; Shah, S.P. Experimental and numerical studies on impact behaviors of recycled aggregate concrete-filled steel tube after exposure to elevated temperature. Mater. Design. 2017, 136, 103–118. [Google Scholar] [CrossRef]
- Li, W.G.; Luo, Z.Y.; Wu, C.Q.; Duan, W.H. Impact performances of steel tube-confined recycled aggregate concrete (STCRAC) after exposure to elevated temperatures. Cement. Concrete. Comp. 2018, 86, 87–97. [Google Scholar] [CrossRef]
- Li, L.J.; Tu, G.R.; Lan, C.; Liu, F. Mechanical characterization of waste-rubber-modified recycled-aggregate concrete. J. Clean. Prod. 2016, 124, 325–338. [Google Scholar] [CrossRef]
- Liu, F.; Chen, G.X.; Li, L.J.; Guo, Y.C. Study of impact performance of rubber reinforced concrete. Constr. Build. Mater. 2012, 36, 604–616. [Google Scholar] [CrossRef]
- Wijayasundara, M.; Mendis, P.; Crawford, R.H. Integrated assessment of the use of recycled concrete aggregate replacing natural aggregate in structural concrete. J. Clean. Prod. 2018, 174, 591–604. [Google Scholar] [CrossRef]
- Prince, M.J.R.; Gaurav, G.; Singh, B. Splice strength of steel reinforcement embedded in recycled aggregate concrete. Constr. Build. Mater. 2018, 160, 156–168. [Google Scholar] [CrossRef]
- Liu, F.; Feng, W.H.; Xiong, Z.; Tu, G.R.; Li, L.J. Static and impact behaviour of recycled aggregate concrete under daily temperature variations. J. Clean. Prod. 2018, 191, 283–296. [Google Scholar] [CrossRef]
- Bui, N.K.; Satomi, T.; Takahashi, H. Improvement of mechanical properties of recycled aggregate concrete basing on a new combination method between recycled aggregate and natural aggregate. Constr. Build. Mater. 2017, 148, 376–385. [Google Scholar] [CrossRef]







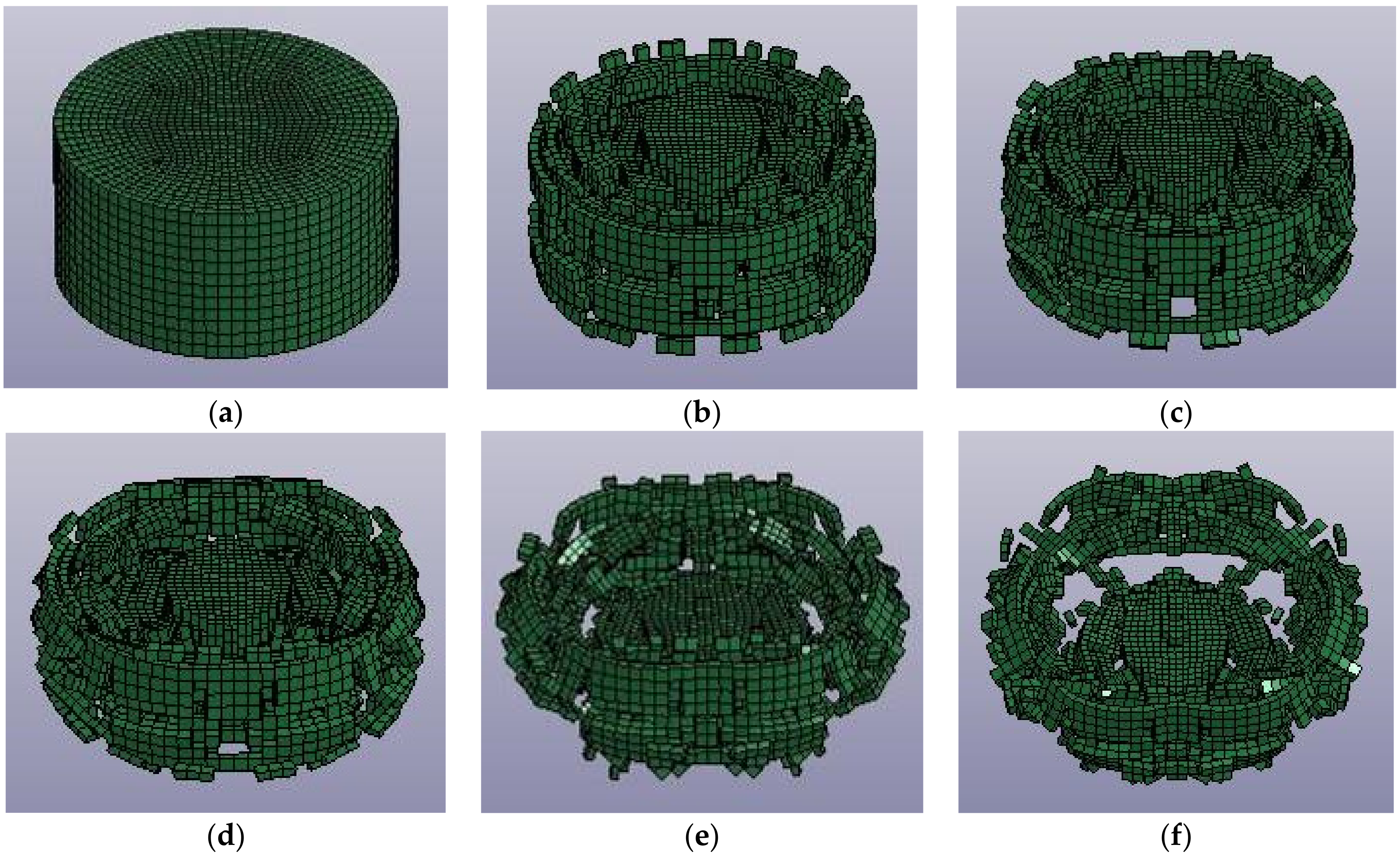
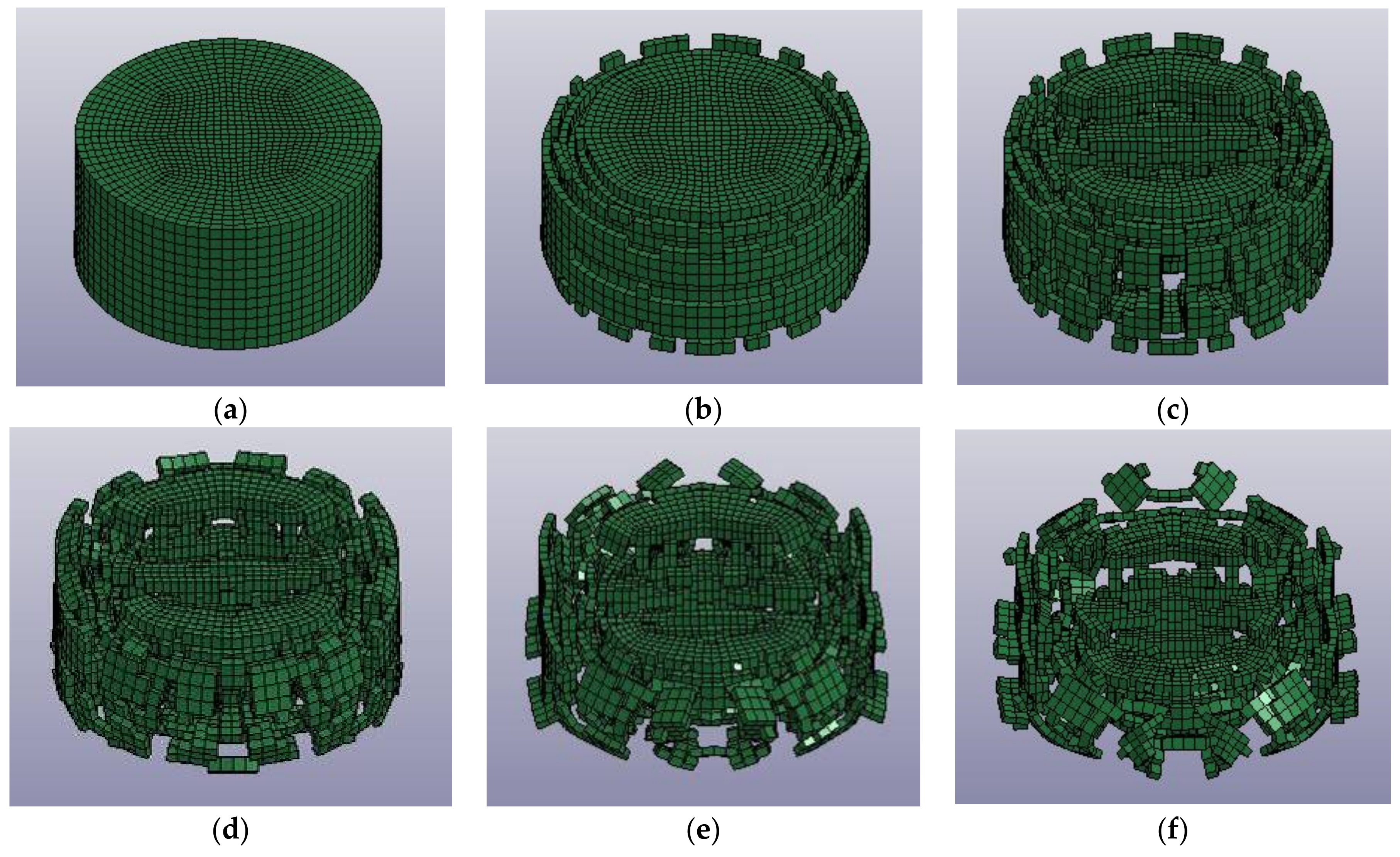
| Specimens | Load (kN) | Compressive Strength (MPa) | Average (MPa) |
|---|---|---|---|
| N40-1 | 929 | 41.3 | 41.52 |
| N40-2 | 918 | 40.8 | |
| N40-3 | 936 | 41.6 | |
| N40-4 | 947 | 42.1 | |
| N40-5 | 941 | 41.8 | |
| R40-1 | 880 | 39.1 | 39.44 |
| R40-2 | 884 | 39.3 | |
| R40-3 | 891 | 39.6 | |
| R40-4 | 887 | 39.4 | |
| R40-5 | 896 | 39.8 |
| Specimens | Impact Velocity (m/s) | Strain Rate (s−1) | Compressive Strength (MPa) | Specimens | Impact Velocity (m/s) | Strain Rate (s−1) | Compressive Strength (MPa) |
|---|---|---|---|---|---|---|---|
| NC-100-1 | 4.8 | 50 | 48.6 | RC-100-1 | 4.5 | 37 | 44.7 |
| NC-100-2 | 4.7 | 47 | 47.4 | RC-100-2 | 4.7 | 43 | 46.2 |
| NC-100-3 | 4.6 | 41 | 46.8 | RC-100-3 | 4.6 | 46 | 45.3 |
| NC-200-1 | 7.3 | 66 | 58.8 | RC-200-1 | 7.2 | 63 | 56.6 |
| NC-200-2 | 7.1 | 57 | 57.6 | RC-200-2 | 7.1 | 54 | 55.5 |
| NC-200-3 | 7.2 | 63 | 58.5 | RC-200-3 | 7.3 | 60 | 56.2 |
| NC-300-1 | 9.7 | 80 | 67.7 | RC-300-1 | 9.5 | 74 | 64.3 |
| NC-300-2 | 9.8 | 86 | 68.1 | RC-300-2 | 9.9 | 78 | 66.7 |
| NC-300-3 | 9.6 | 77 | 67.3 | RC-300-3 | 9.7 | 79 | 65.8 |
| NC-400-1 | 10.7 | 90 | 75.3 | RC-400-1 | 10.9 | 87 | 74.6 |
| NC-400-2 | 11.1 | 98 | 76.2 | RC-400-2 | 10.7 | 92 | 72.6 |
| NC-400-3 | 10.9 | 94 | 75.6 | RC-400-3 | 10.8 | 94 | 73.9 |
| NC-500-1 | 12.3 | 107 | 83.1 | RC-500-1 | 12.3 | 105 | 82.1 |
| NC-500-2 | 12.2 | 112 | 82.8 | RC-500-2 | 12.1 | 100 | 80.6 |
| NC-500-3 | 12.4 | 105 | 83.7 | RC-500-3 | 12.2 | 104 | 81.2 |
| Density (kg·m−3) | Shear Modulus (GPa) | Strength | Hardening Coefficient | Strain Rate Coefficient | Hardening Exponent | Compressive Strength (MPa) | Tensile Strength (MPa) | Strain Rate | Minimum Plastic Strain |
| 2400 | 14.86 | 0.79 | 1.6 | 0.007 | 0.61 | 48 | 4 | 1 × 10−6 | 0.01 |
| Normalize strength (MPa) | Compressive strain | Volume strain | Stress (GPa) | Strain rate (compacted) | Damage constant 1 | Damage constant 2 | Material constant 1 (MPa) | Material constant 2 (MPa) | Material constant 3 (MPa) |
| 7 | 16 | 1 × 10−3 | 0.80 | 0.1 | 0.04 | 1.0 | 85 | −171 | 208 |
| Density (kg·m−3) | Shear Modulus (GPa) | Strength | Hardening Coefficient | Strain rate Coefficient | Hardening Exponent | Compressive Strength (MPa) | Tensile Strength (MPa) | Strain Rate | Minimum Plastic Strain |
| 2450 | 18.47 | 0.79 | 1.8 | 0.007 | 0.99 | 45 | 4 | 1 × 10−6 | 0.01 |
| Normalize strength (MPa) | Compressive strain | Volume strain | Stress (GPa) | Strain rate (compacted) | Damage constant 1 | Damage constant 2 | Material constant 1 (MPa) | Material constant 2 (MPa) | Material constant 3 (MPa) |
| 7 | 15 | 8.0 × 10−4 | 1.3 | 0.07 | 0.04 | 1.0 | 85 | −171 | 208 |
| Density (g/mm3) | Elastic Modulus (MPa) | Poisson’s Ratio | Yield Strength (MPa) | Tangent Modulus (MPa) | Failure Strain |
|---|---|---|---|---|---|
| 7.8 × 10−3 | 2.1 × 105 | 0.3 | 500 | 6 × 102 | 0.28 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Du, W.; Yang, C.; De Backer, H.; Li, C.; Ming, K.; Zhang, H.; Pan, Y. Investigation on Dynamic Mechanical Properties of Recycled Concrete Aggregate under Split-Hopkinson Pressure Bar Impact Test. Buildings 2022, 12, 1055. https://doi.org/10.3390/buildings12071055
Du W, Yang C, De Backer H, Li C, Ming K, Zhang H, Pan Y. Investigation on Dynamic Mechanical Properties of Recycled Concrete Aggregate under Split-Hopkinson Pressure Bar Impact Test. Buildings. 2022; 12(7):1055. https://doi.org/10.3390/buildings12071055
Chicago/Turabian StyleDu, Wenping, Caiqian Yang, Hans De Backer, Chen Li, Kai Ming, Honglei Zhang, and Yong Pan. 2022. "Investigation on Dynamic Mechanical Properties of Recycled Concrete Aggregate under Split-Hopkinson Pressure Bar Impact Test" Buildings 12, no. 7: 1055. https://doi.org/10.3390/buildings12071055
APA StyleDu, W., Yang, C., De Backer, H., Li, C., Ming, K., Zhang, H., & Pan, Y. (2022). Investigation on Dynamic Mechanical Properties of Recycled Concrete Aggregate under Split-Hopkinson Pressure Bar Impact Test. Buildings, 12(7), 1055. https://doi.org/10.3390/buildings12071055






