Fatigue Behaviour of Aluminium Members with Different Notch Root Shapes
Abstract
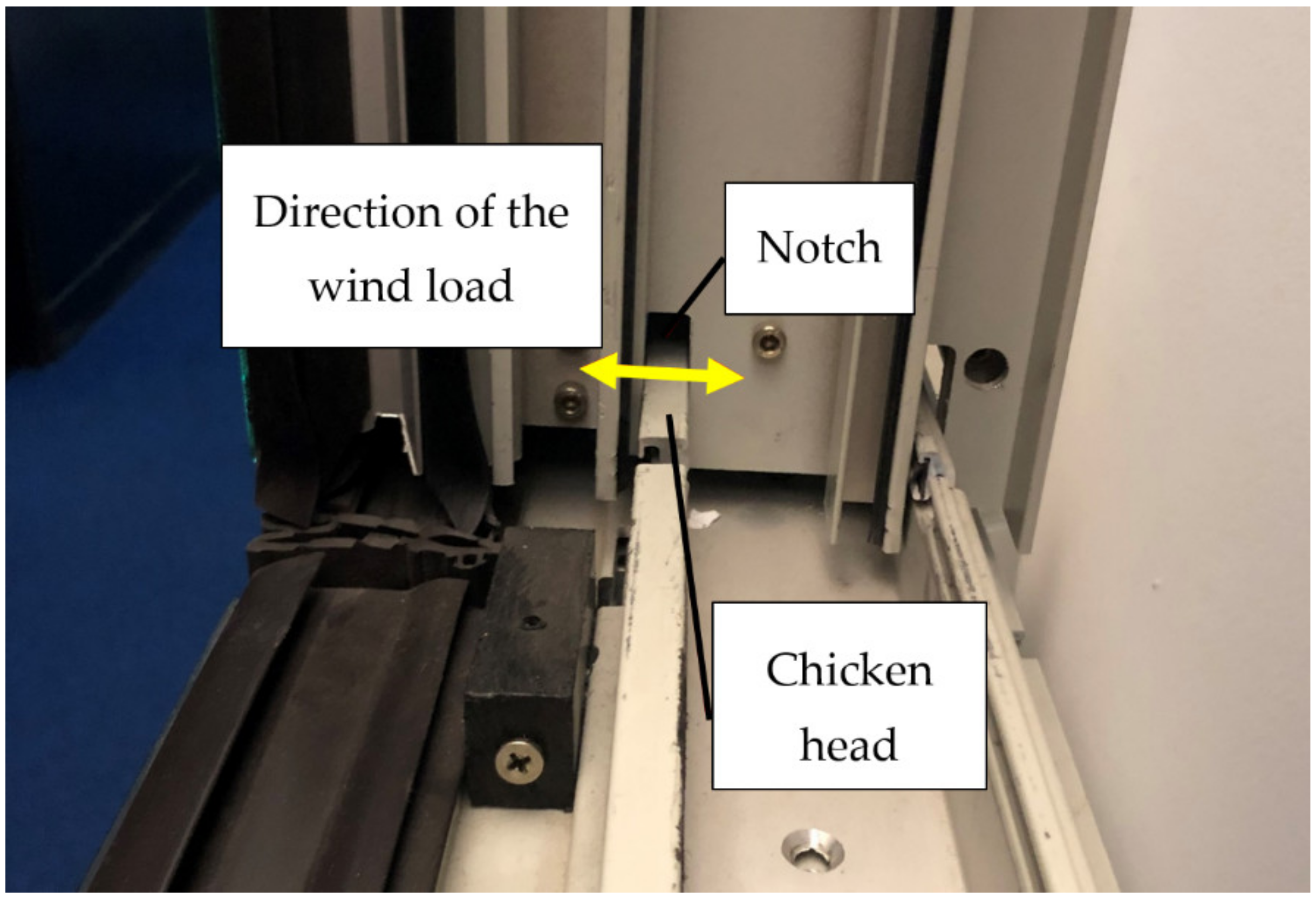
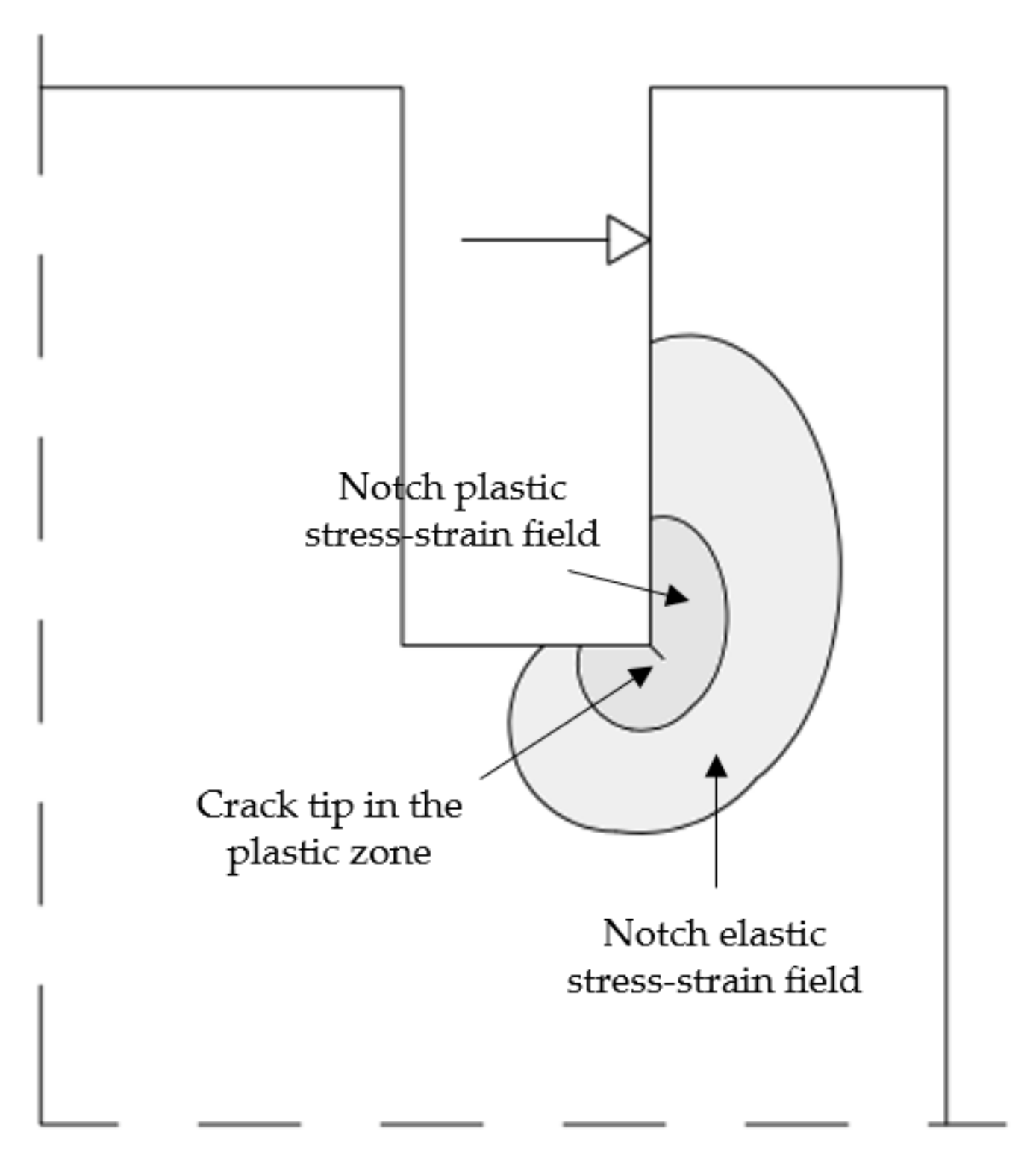
:1. Introduction
2. Experimental Study
2.1. General
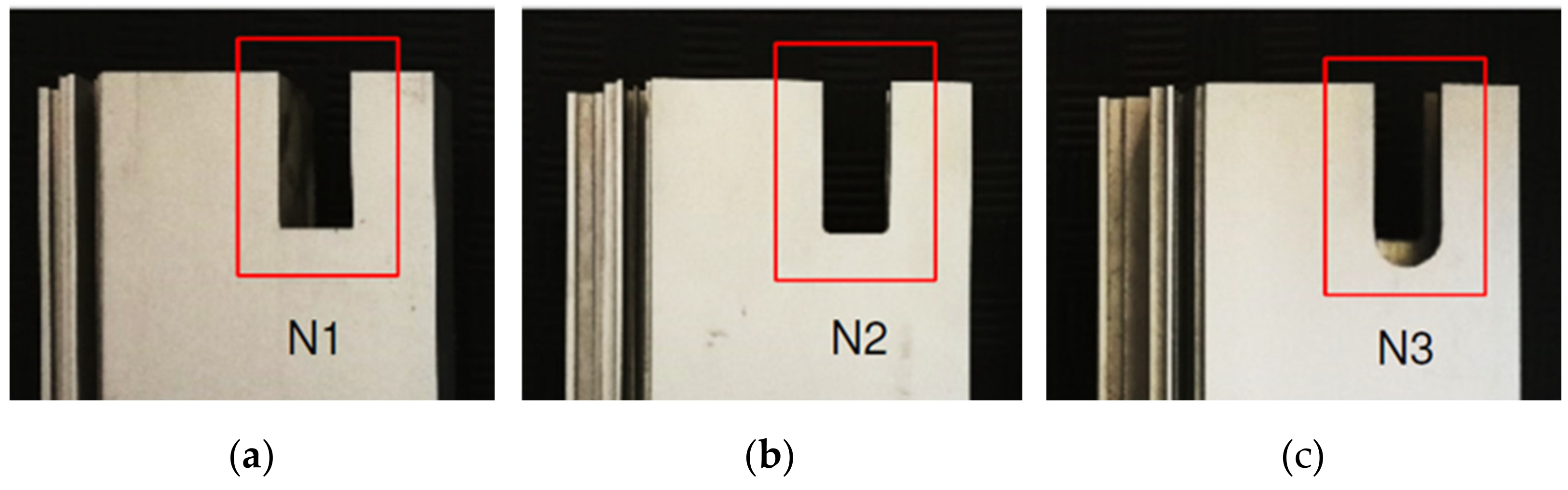
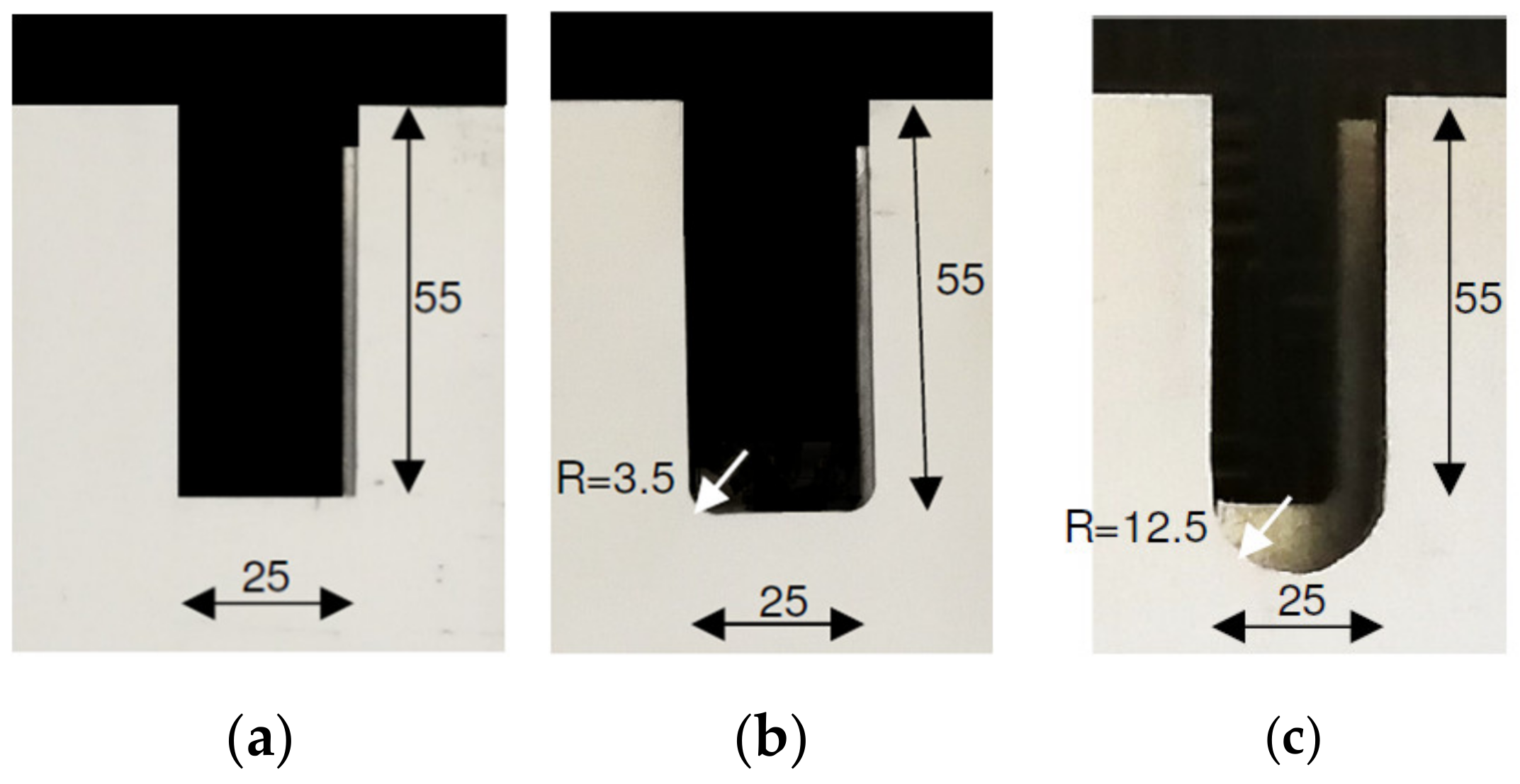
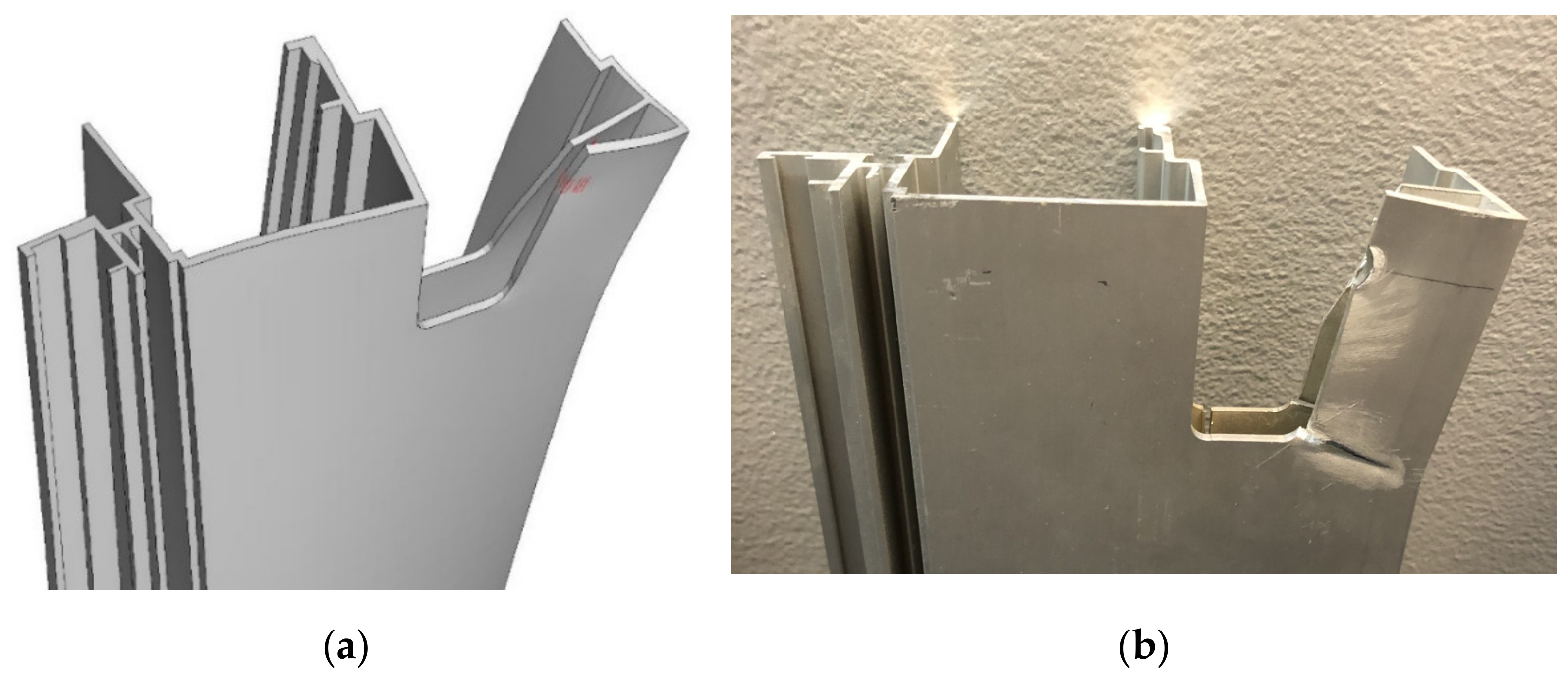
2.2. Material and Specimen Geometry
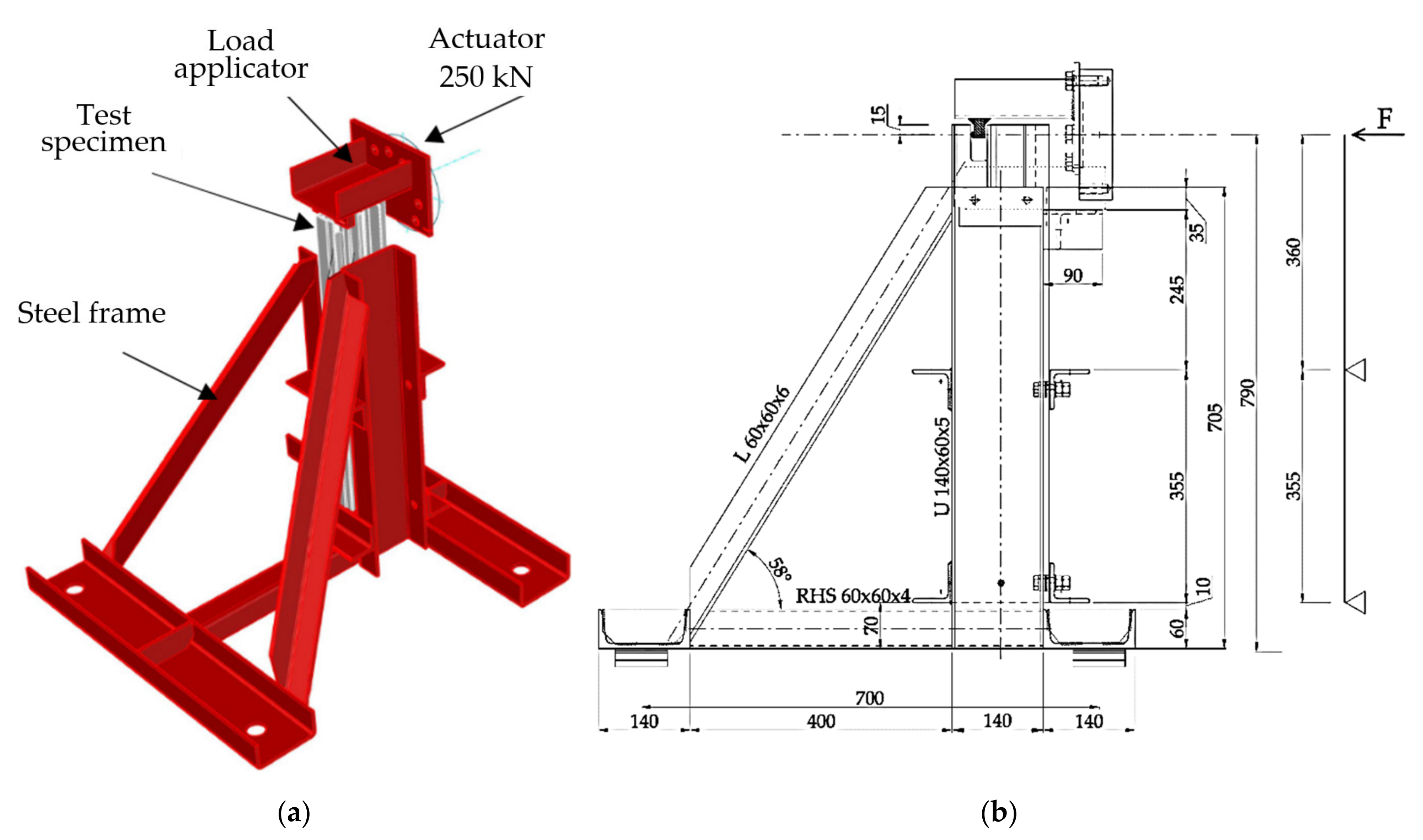
2.3. Monotonic Testing
- LVDT-1,2,3,4 were located at the level of the application of the load.
- LVDT-1—absolute displacement at the back of the profile behind the notch in the direction of the load.
- LVDT-2—absolute displacement at the tip of the specimen in the direction of the load
- The differential of LVDT-1 − LVDT-2 measures the opening of the notch under the load.
- LVDT-3—absolute displacement at the back of the profile behind the notch perpendicular to the direction of the load.
- LVDT-4—absolute displacement at the top end of the specimen perpendicular to the direction of the load.
- The differential of LVDT-3 − LVDT-4 measures the rotation of the tip of the specimen under load.
- LVDT-5—absolute displacement of the upper support of the specimen in the direction of the load (upper angle in the steel frame).
- LVDT-6—absolute displacement of the lower support of the specimen in the direction of the load (lower angle in the steel frame).
- The displacements of LVDT-5 and LVDT-6 are used to confirm the sufficient stiffness of the supporting steel frame.
- The differential of LVDT-5 − LVDT-6 determines the rotation of the supporting steel frame in order to distinguish between movement at the tip of the specimen due to the rotation of the support and movement due to the bending of the specimen.
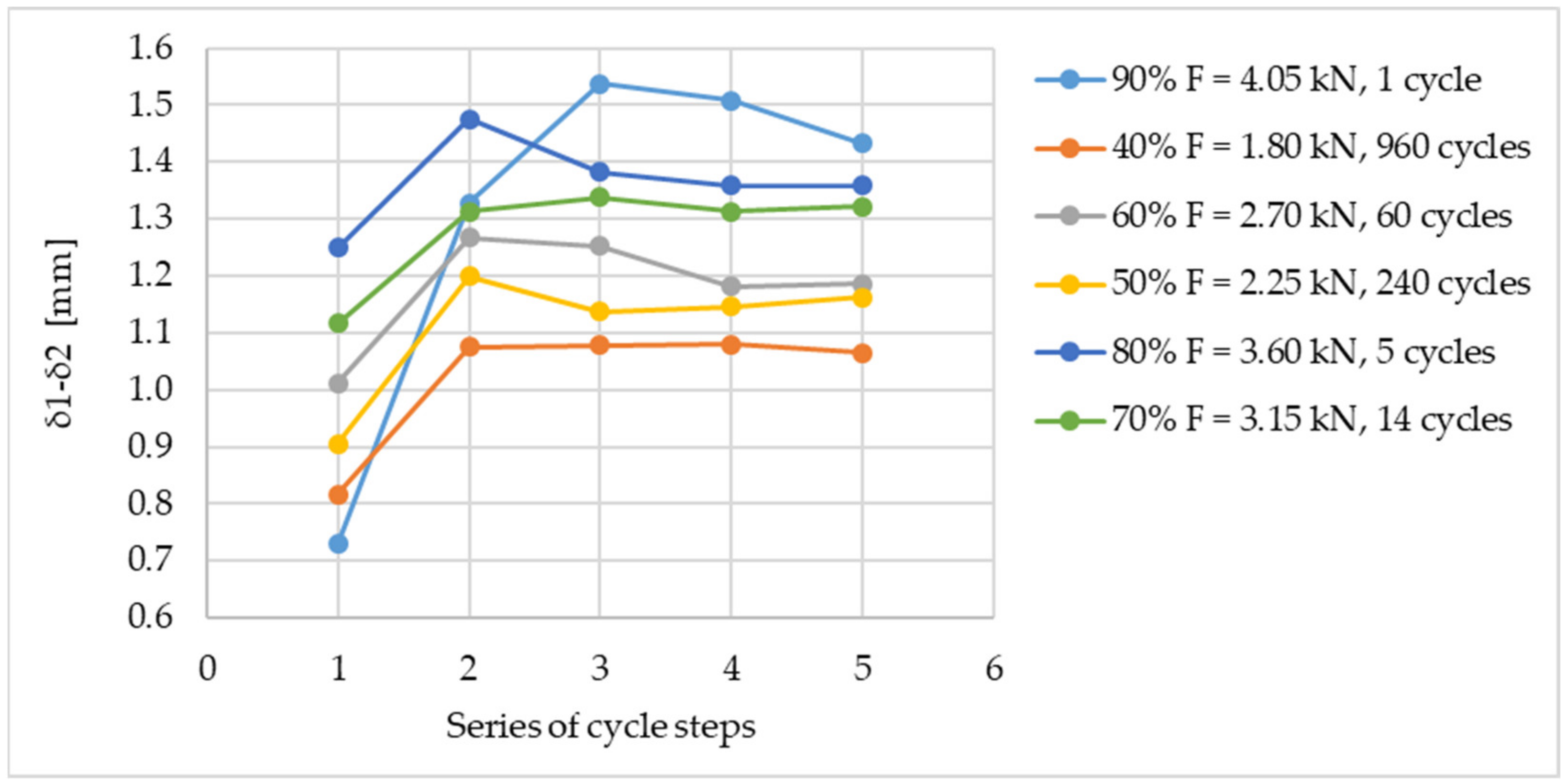
2.4. Cyclic Testing
2.4.1. Load History
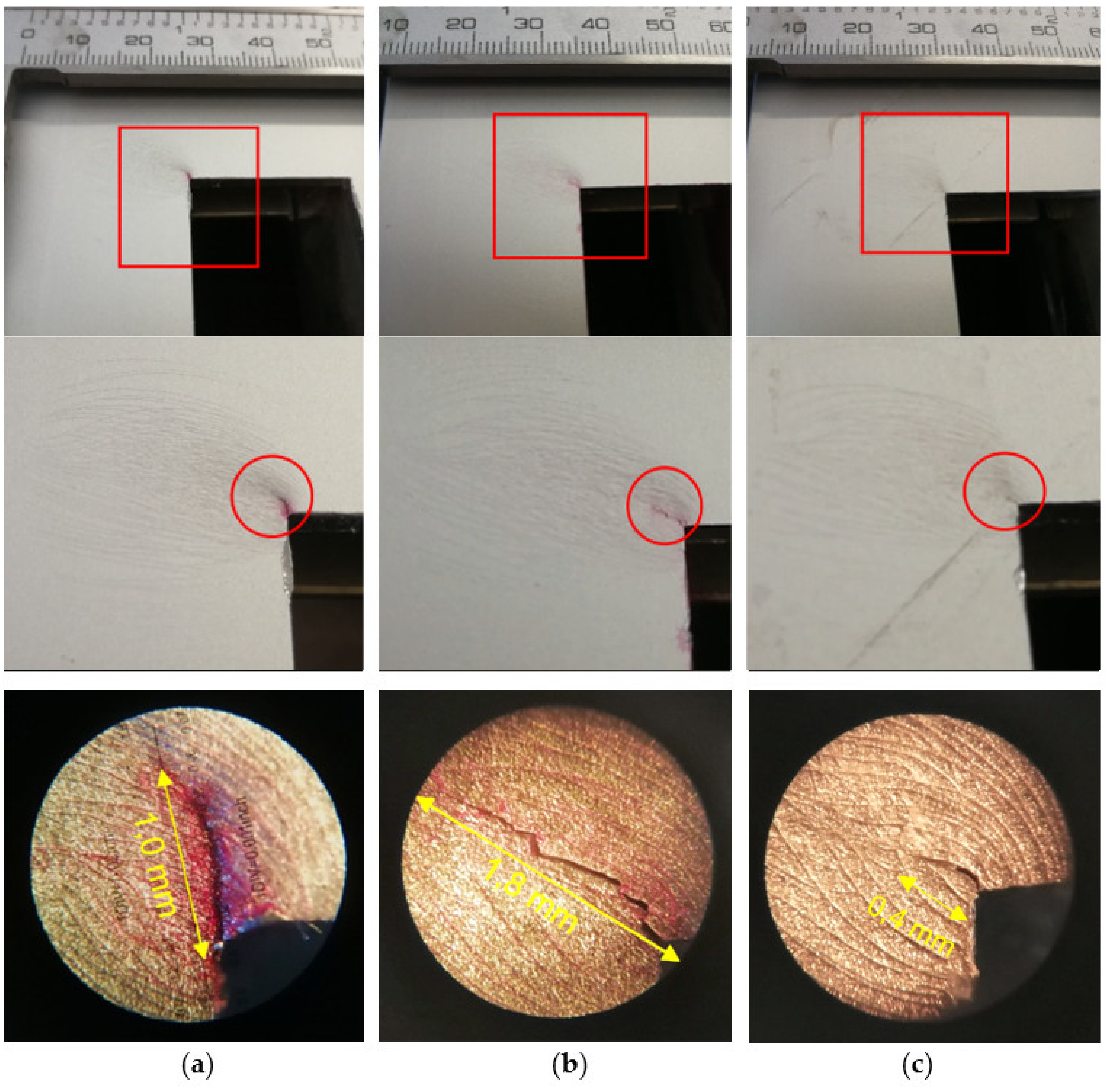
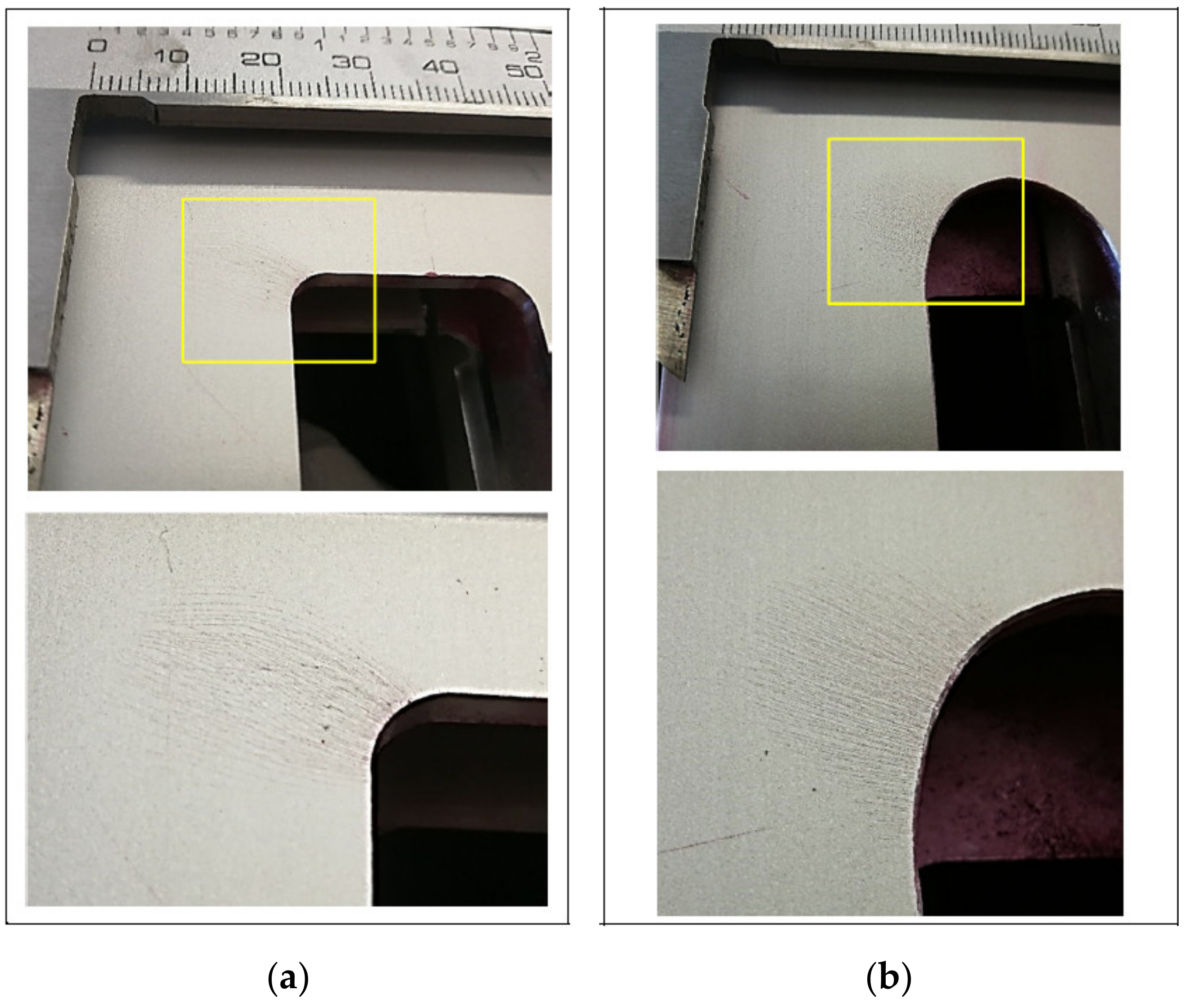
2.4.2. Cyclic Test Specimens
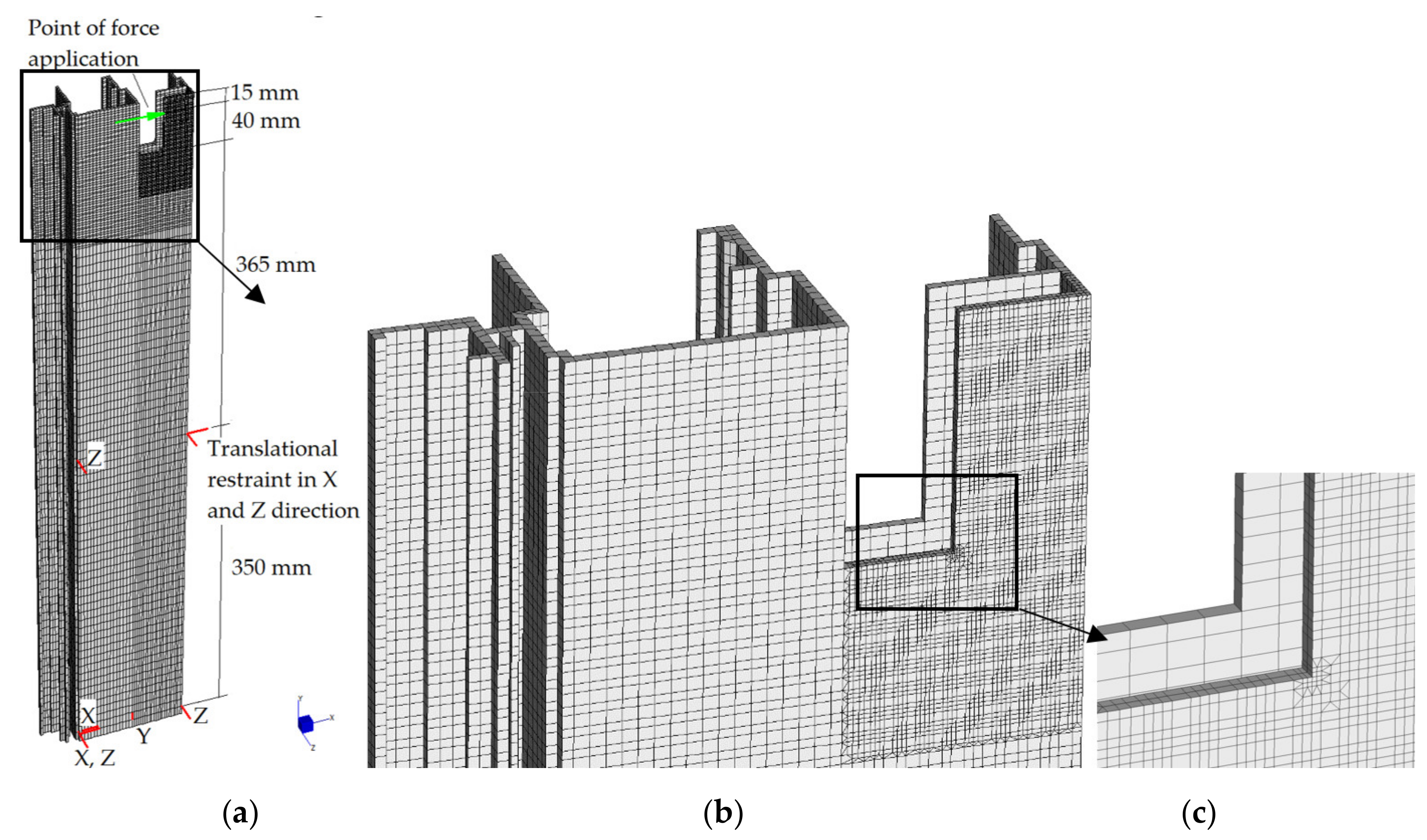
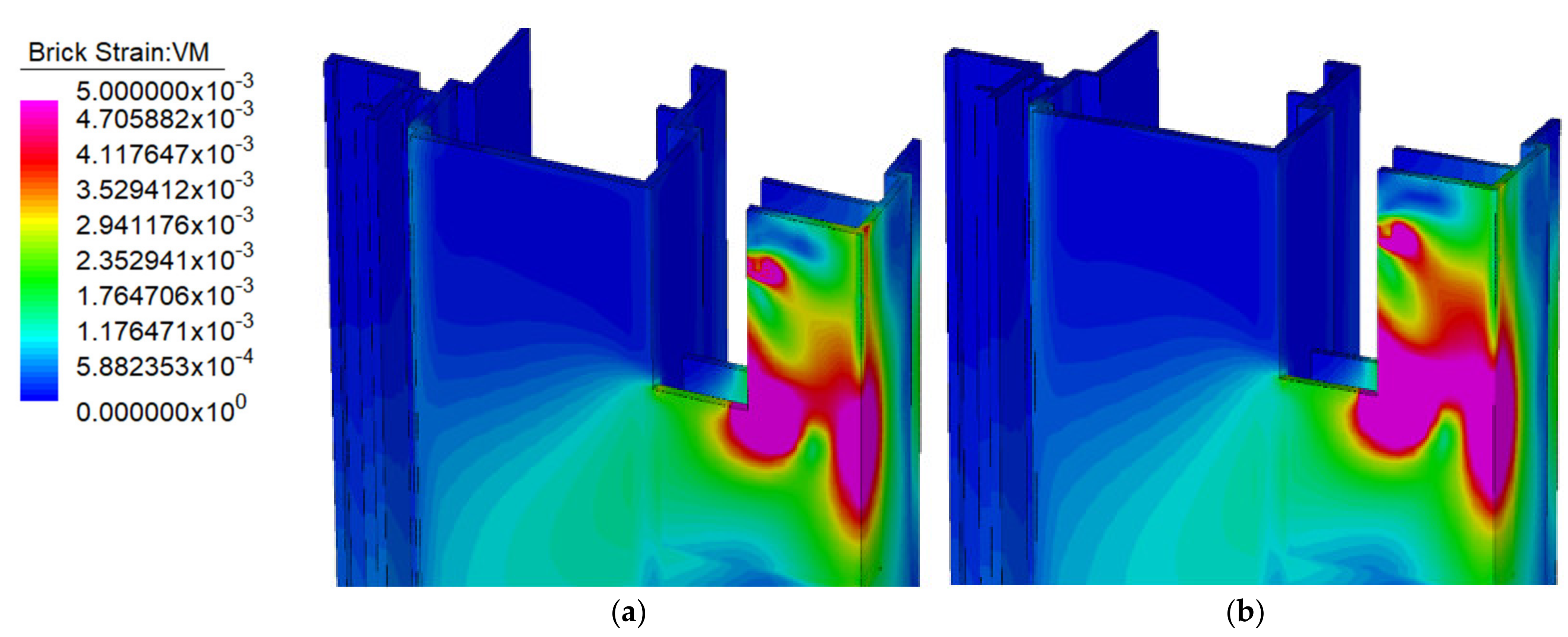
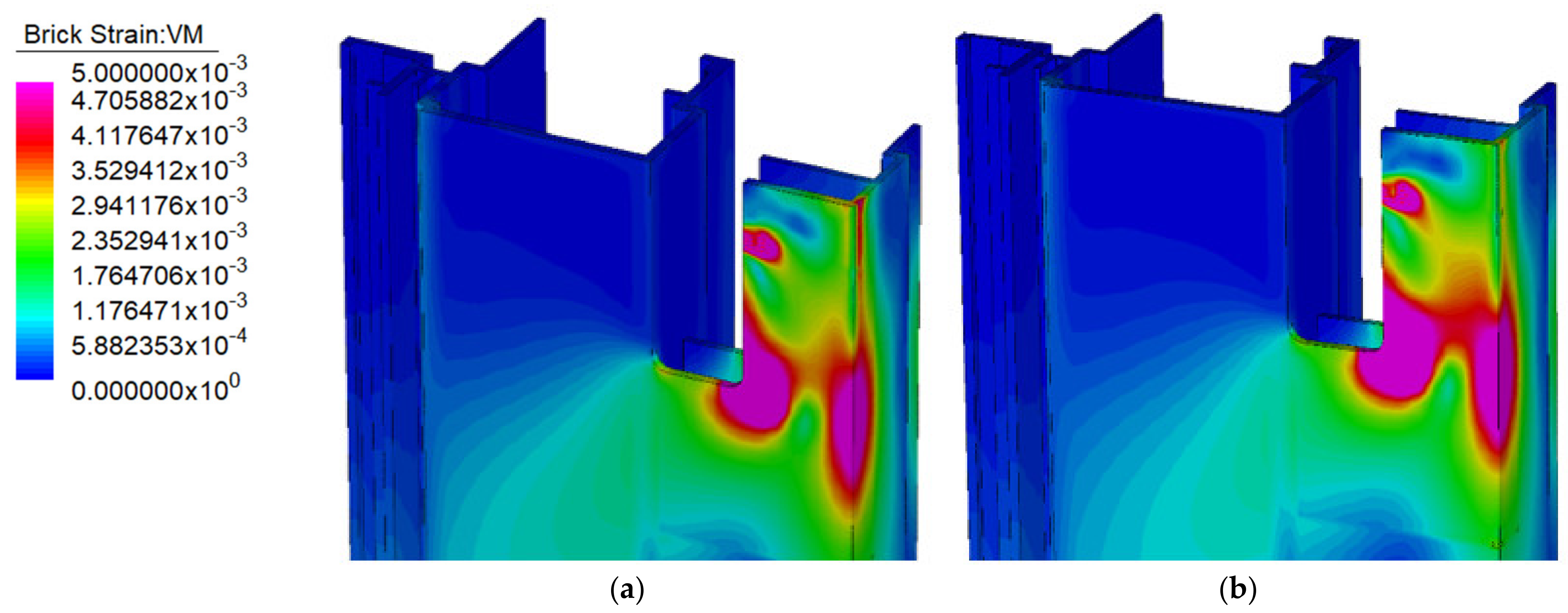
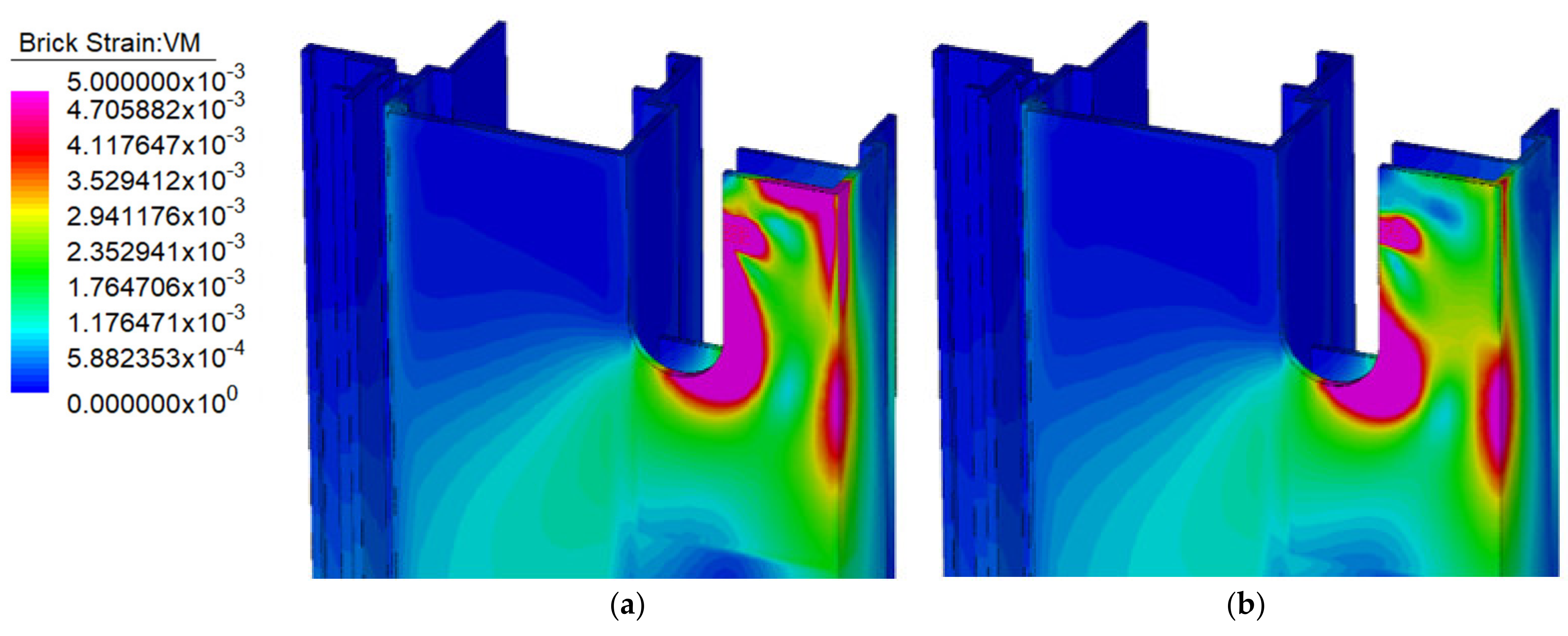
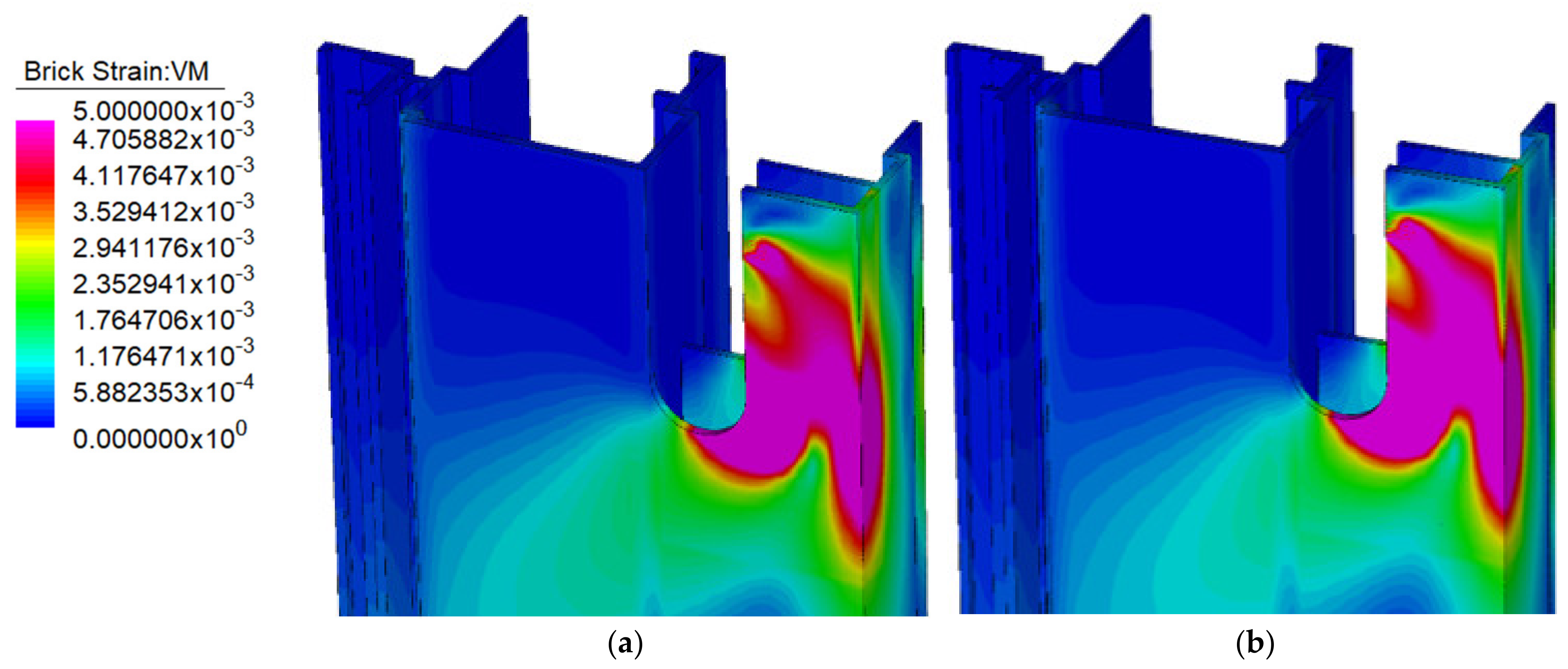
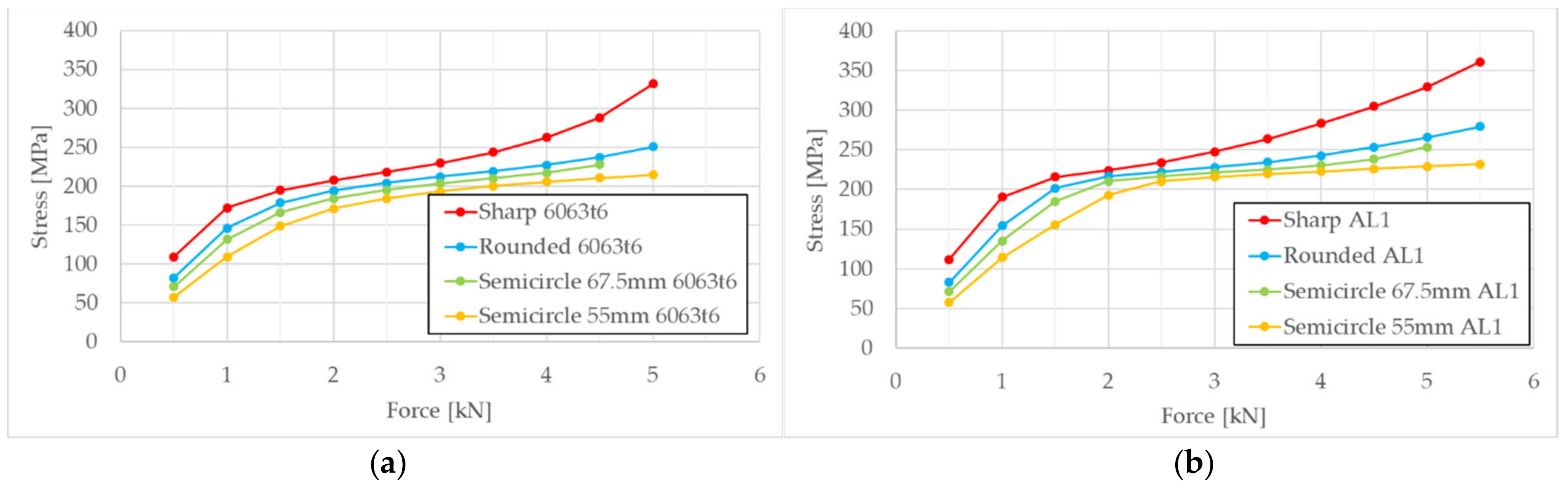
3. Numerical Analysis
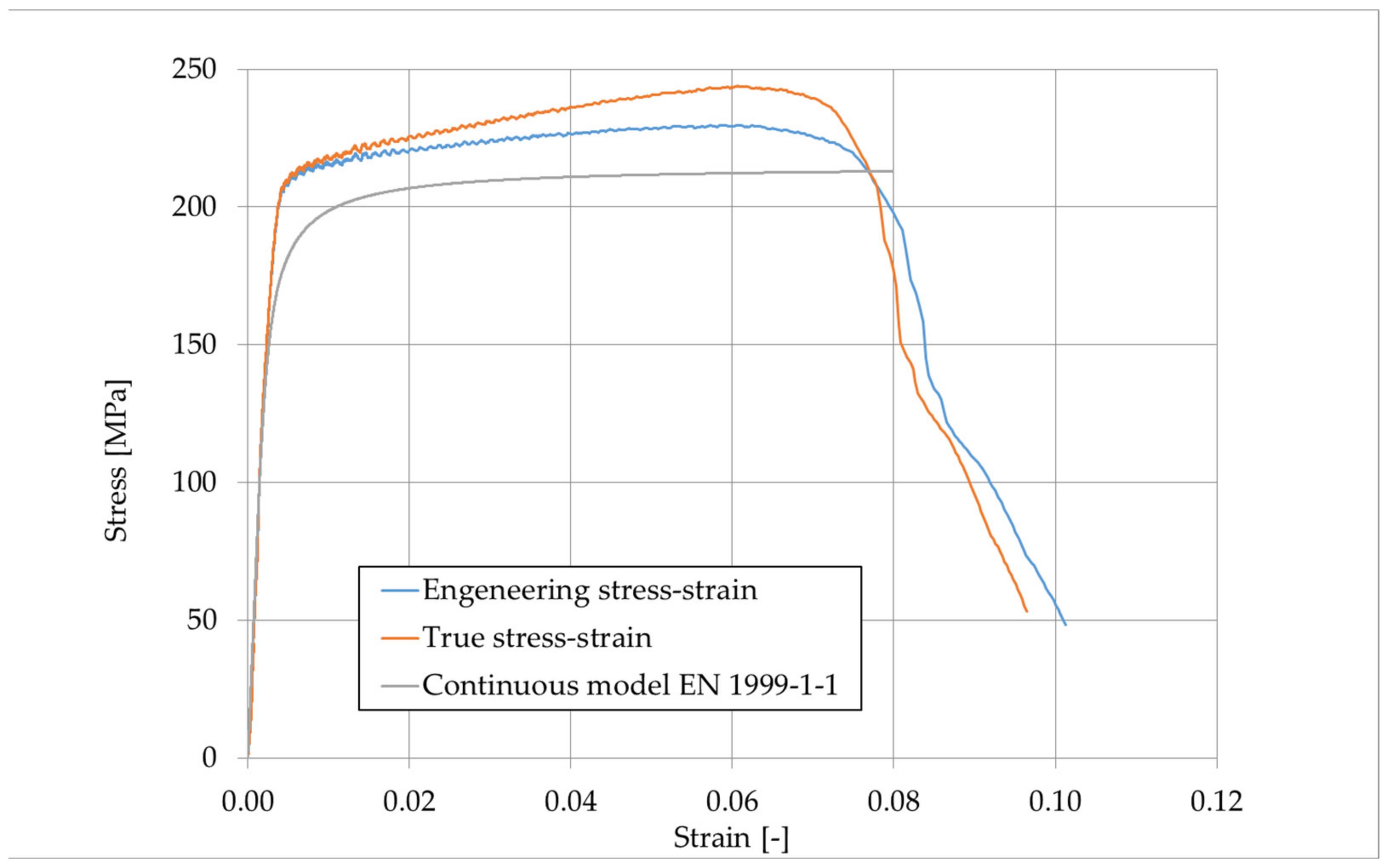
- Higher stress for the Al1 true curve, which had higher stress in the plastic zone.
- Higher strains for the standardised curve, which had lower proof stress, thus yielding earlier.
4. Fatigue Resistance According to Eurocode 9
- Δσi—stress range for the principal stresses at the detail, max hotspot stress range at the root of the notch in numerical models, [21]
- γFf = 1.0—partial factor for fatigue loads, [21]
- γMf = 1.0—partial factor for materials, [21]
- m0 = m1 = 7—inverse slope of logΔσ-logN curve at 103 ≤ N ≤ 105 cycles
- ni—number of cycles of stress range Δσi
- Ni—endurance under stress range Δσi
5. Discussion
6. Conclusions
- Specimens were first tested in the monotonic regime until failure in order to assess their maximal load capacity. As expected, specimens with a sharp root had a substantially lower failure load, roughly 20% lower than that of the other specimens.
- Specimens were then tested in the cyclic regime in order to assess their fatigue resistance. The applied variable load history is typical for a 50-year exposure to wind in the UK, which was the actual in-use condition of the original profiles. The selected magnitude of the load history was derived from the lowest monotonic failure load of the specimen with the sharp root, reduced by the partial factor for the wind load.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Georgantzia, E.; Gkantou, M.; Kamaris, G.S. Aluminium alloys as structural material: A review of research. Eng. Struct. 2021, 227, 111372. [Google Scholar] [CrossRef]
- Lotsberg, I. Fatigue Design of Marine Structures; Cambridge University Press: New York, NY, USA, 2016. [Google Scholar]
- Gurney, T.R. Fatigue of Welded Structures, 2nd ed.; Cambridge University Press: Cambridge, UK, 1979. [Google Scholar]
- Forrest, P.G. Fatigue of Metals; Pergamon Press: Oxford, UK, 1962. [Google Scholar]
- Maljaars, J.; Lukić, M.; Soetens, F. Comparison between the Eurocode for Fatigue of Steel Structures, EN 1993-1-9, and the Eurocode for Fatigue of Aluminium Structures, EN 1999-1-3. Procedia Eng. 2013, 66, 34–48. [Google Scholar] [CrossRef]
- Pilkey, W.D. Formulas for Stress, Strain, and Structural Matrices, 2nd ed.; Wiley & Sons, Inc.: Hoboken, NJ, USA, 2005. [Google Scholar]
- Fatemi, A. Notches and Their Effect; University of Toledo: Toledo, OH, USA, 2011. [Google Scholar]
- Cook, J.N. The Designer’s Guide to Wind Loading Structures; Part 2: Static Structures; BRE: London, UK, 1990. [Google Scholar]
- Krolo, P. Test Report 004/2020: Aluminium Extruded Profiles-Static Test; Faculty of Civil Engineering University of Rijeka: Rijeka, Croatia, 2020. [Google Scholar]
- EN 755-2:2016 Aluminium and Aluminium Alloys-Extruded Rod/Bar, Tube, and Profiles—Part 2: Mechanical Properties; European Committee for Standardization: Brussels, Belgium, 2016.
- Skejić, D.; Dokšanović, T.; Čudina, I.; Mazzolani, F.M. The Basis for Reliability-Based Mechanical Properties of Structural Aluminium Alloys. Appl. Sci. 2021, 11, 4485. [Google Scholar] [CrossRef]
- EN ISO 6892-1:2016: Metallic Materials–Tensile Testing—Part 1: Method of Test at Room Temperature; European Committee for Standardization: Brussels, Belgium, 2016.
- Gerhardt, H.J.; Kramer, C. Wind induced loading cycle and fatigue testing of light-weight roofing fixation. J. Wind. Eng. Ind. Aerodyn. 1986, 23, 237–247. [Google Scholar] [CrossRef]
- Xu, Y.L. Wind-induced fatigue loading on roof cladding of low-rise buildings. In Cyclone Structural Testing Station: Technical Report No 41; James Cook University of North Queensland: Townsville, Australia, 1993. [Google Scholar]
- EN 1991:2005 Eurocode 1: Actions on Structures—Part 1–4: General Actions—Wind Actions; European Committee for Standardization: Brussels, Belgium, 2005.
- EN 1990:2002+A1:2005+A1:2005/AC:2010 Eurocode 0: Basis of Structural Design; European Committee for Standardization: Brussels, Belgium, 2002.
- Krolo, P. Test Report 005/2020: Aluminium Extruded Profiles-Fatigue Test; Faculty of Civil Engineering University of Rijeka: Rijeka, Croatia, 2020. [Google Scholar]
- EN 1999-1-1:2007+A1:2009+A2:2013 Eurocode 9: Design of Aluminium Structures—Part 1-1: General Structural Rules; European Committee for Standardization: Brussels, Belgium, 2007.
- Strand7. Available online: https://www.strand7.com/html/docu_usingstrand7.htm (accessed on 20 November 2021).
- Joshi, A. Stress Concentration Factor Converts into Stress Intensity Factor using ANSYS. IJSER 2006, 7, 1662–1667. [Google Scholar]
- EN 1999-1-3:2007+A1:2011 Eurocode 9: Design of Aluminium Structures-Part 1–3: Structures Susceptible to Fatigue; European Committee for Standardization: Brussels, Belgium, 2007.
- Yen, C.S.; Dolan, T.J. A Critical Review of The Criteria for Notch Sensitivity in Fatigue of Metals; Bulletin Series No 398; University of Illinois Engineering Experiment Station: Urbana, IL, USA, 1952. [Google Scholar]
- Buljan, N. Fatigue Testing of the Notch in the Aluminium Profile; Faculty of Civil Engineering University of Rijeka: Rijeka, Croatia; Faculty of Civil Engineering University of Zagreb: Zagreb, Croatia, 2020. [Google Scholar]







| Coupon/Source | Rp0,2 | Rm | A |
|---|---|---|---|
| [MPa] | [MPa] | [%] | |
| Coupon Al1 | 207.7 | 229.8 | 10.1 |
| Coupon Al2 | 211.0 | 233.7 | 8.0 |
| Coupon Al3 | 222.1 | 241.9 | 9.7 |
| EN 755-2 [6] | 170.0 | 215.0 | 8.0 |
| Supplier’s certificate | 216.6 | 235.7 | 11.8 |
| Specimen | Shape of Notch N | Width of Notch (mm) | Depth of Notch (mm) | Radius at the Root of Notch (mm) | |
|---|---|---|---|---|---|
| Monotonic Test | Cyclic Test | ||||
| M_N1_1 | C_N1_1 | Sharp (N1) | 25 | 55 | 0 |
| M_N1_2 | C_N1_2 | ||||
| - | C_N1_3 | ||||
| M_N2_1 | C_N2_1 | Rounded (N2) | 55 | 3.5 | |
| M_N2_2 | C_N2_2 | ||||
| M_N3 | C_N3_1 | Semi-circular (N3) | 67.5 | 12.5 | |
| - | C_N3_2 | ||||
| Specimen | Ultimate Load | Opening of the Notch LVDT-1 − LVDT-2, Figure 8. |
|---|---|---|
| (kN) | (mm) | |
| M_N1_1 | 6.78 | 6.18 |
| M_N1_2 | 6.99 | 3.78 |
| M_N2_1 | 8.08 | 9.49 |
| M_N2_2 | 8.61 | 9.45 |
| M_N3 | 8.45 | 20.20 |
| Loading Step | Number of Cycles | Percentage of the Design Load F | Nominal Load Fi for Step i [kN] |
|---|---|---|---|
| 1 | 1 | 90% | 4.05 |
| 2 | 960 | 40% | 1.80 |
| 3 | 60 | 60% | 2.70 |
| 4 | 240 | 50% | 2.25 |
| 5 | 5 | 80% | 3.60 |
| 6 | 14 | 70% | 3.15 |
| 7 | 1 | 100% | 4.50 |
| Coupon | Engineering Stress Rm (MPa) | Corresponding True Stress σt (MPa) |
|---|---|---|
| Al1 | 229.8 | 243.9 |
| Al2 | 233.7 | 245.9 |
| Al3 | 241.9 | 258.0 |
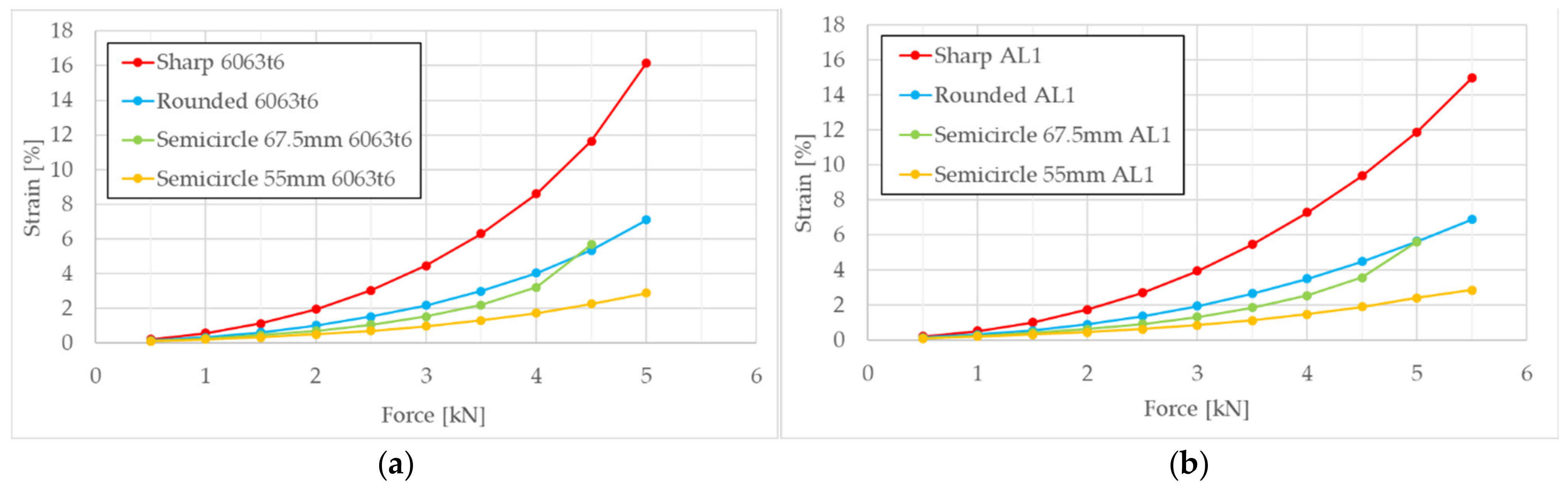
| Notch Shape | Strain [%] | |
|---|---|---|
| Nominal Stress–Strain Curve | True Stress–Strain Curve (Al1) | |
| Sharp | 16.17 | 11.88 |
| Rounded | 7.10 | 5.64 |
| Semi-circular (depth 55 mm) | 2.88 | 2.42 |
| Semi-circular (depth 67.5 mm) | 5.69 * | 5.62 |
| Detail Type | Detail Category Δσ-m1 | Product Forms Constructional Detail Initiation Site | Stress Orientation | Stress Analysis | Execution Requirements | |
|---|---|---|---|---|---|---|
| 1.6 | 100-7 | Notches, holes Surface irregularity | Parallel or normal to rolling or extrusion direction. | Account for stress concentration. | Surface free of sharp corners unless parallel to stress direction; edges free of stress raisers | No score marks transverse to stress orientation visual inspection |
| Cycles | Total of Cycles ni | Force (kN) |
|---|---|---|
| 1 | 5 | 4.05 |
| 960 | 4800 | 1.80 |
| 60 | 300 | 2.70 |
| 240 | 1200 | 2.25 |
| 5 | 25 | 3.60 |
| 14 | 70 | 3.15 |
| 1 | 1 | 4.50 |
| 6401 |
| Cycles | Force | Stress | Endurance | Damage |
|---|---|---|---|---|
| ni | [kN] | [MPa] | Ni | D |
| 5 | 4.05 | 285.1 | 4679 | 0.00107 |
| 4800 | 1.80 | 221.5 | 27,410 | 0.17512 |
| 300 | 2.70 | 238.6 | 16,292 | 0.01841 |
| 1200 | 2.25 | 228.6 | 21,947 | 0.05468 |
| 25 | 3.60 | 267.9 | 7235 | 0.00346 |
| 70 | 3.15 | 252.1 | 11,083 | 0.00632 |
| 1 | 4.50 | 304.1 | 2981 | 0.00034 |
| 6401 | 0.25939 |
| Notch Type | Total Damage DL | Expected Safe Life Ts [Years] |
|---|---|---|
| Sharp | 0.259 | 193 |
| Rounded | 0.192 | 260 |
| Semi-circular 55 mm | 0.076 | 660 |
| Semi-circular 67.5 mm | 0.144 | 348 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Buljan, N.; Skejić, D.; Krolo, P.; Lukić, M. Fatigue Behaviour of Aluminium Members with Different Notch Root Shapes. Buildings 2022, 12, 681. https://doi.org/10.3390/buildings12050681
Buljan N, Skejić D, Krolo P, Lukić M. Fatigue Behaviour of Aluminium Members with Different Notch Root Shapes. Buildings. 2022; 12(5):681. https://doi.org/10.3390/buildings12050681
Chicago/Turabian StyleBuljan, Nebojša, Davor Skejić, Paulina Krolo, and Mladen Lukić. 2022. "Fatigue Behaviour of Aluminium Members with Different Notch Root Shapes" Buildings 12, no. 5: 681. https://doi.org/10.3390/buildings12050681
APA StyleBuljan, N., Skejić, D., Krolo, P., & Lukić, M. (2022). Fatigue Behaviour of Aluminium Members with Different Notch Root Shapes. Buildings, 12(5), 681. https://doi.org/10.3390/buildings12050681








