The Investigation on Static Stability Analysis for Reticulated Shell with Initial Defect Value Using Stochastic Defect Mode Method
Abstract
:1. Introduction
- An accurate calculation method for the stable critical load of the single-layer reticulated shell is proposed.
- Put forward the calculation formula to evaluate the structural force state at stable critical state.
- An updated criterion to identify the structural force state at the stability critical state is developed.
- The initial geometric defect magnitude is determined by the structural force state and the defect coefficient.
2. Material and Methods
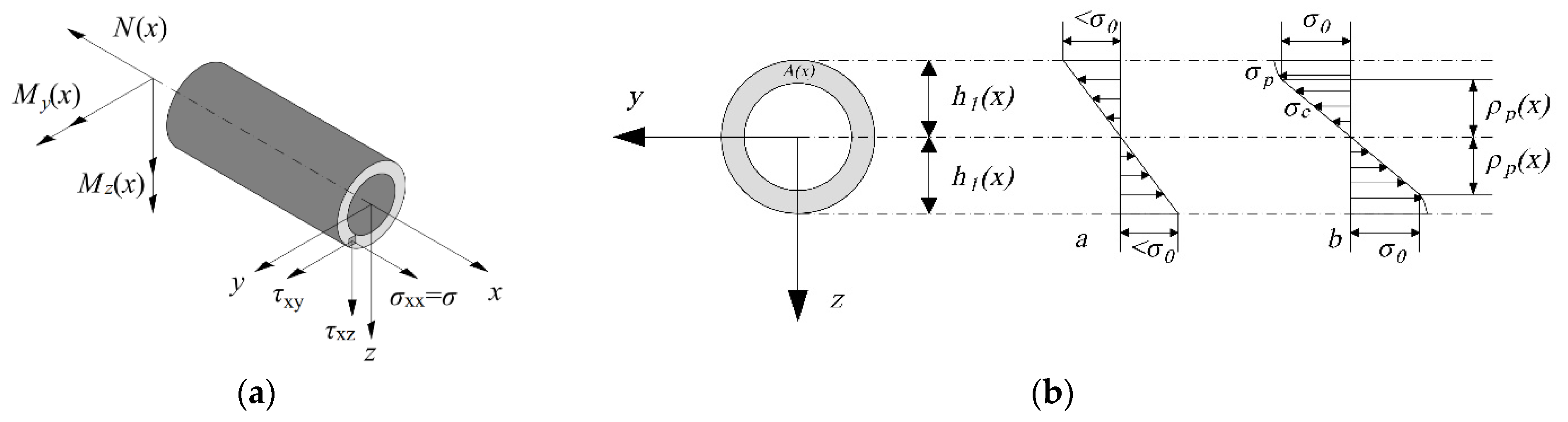
2.1. The Governing Equation and Constitutive Model
2.1.1. The Governing Equation
2.1.2. The Constitutive Model
2.2. Methodology
2.2.1. Analysis Model
- The installation deviation of each node in three directions of the coordinate axis conforms to the normal probability density function within the two-fold mean variance range, that is, the random variable of the installation deviation of each node is δX/2 (δ is the maximum allowable installation deviation, namely, the maximum calculated value of the initial geometric defect), and the random variable X obeys the standard normal distribution. The range of the error random variable is [−δ, δ];
- The random variable of each node installation error for the structure is mutually independent.
- The installation deviation with random variable RW/2 is introduced for each node of calculation model (R is the maximum allowable installation deviation, and W is a random variable, which obeys the standard normal distribution). For each nonlinear buckling analysis of the model, a SCL can be obtained.
- After repeated calculation for n times, n sample space for SCL is generated.
- The SCL sample space follows the standard normal distribution, and hence the SCL of each model is determined by the “3σ” principle [36].
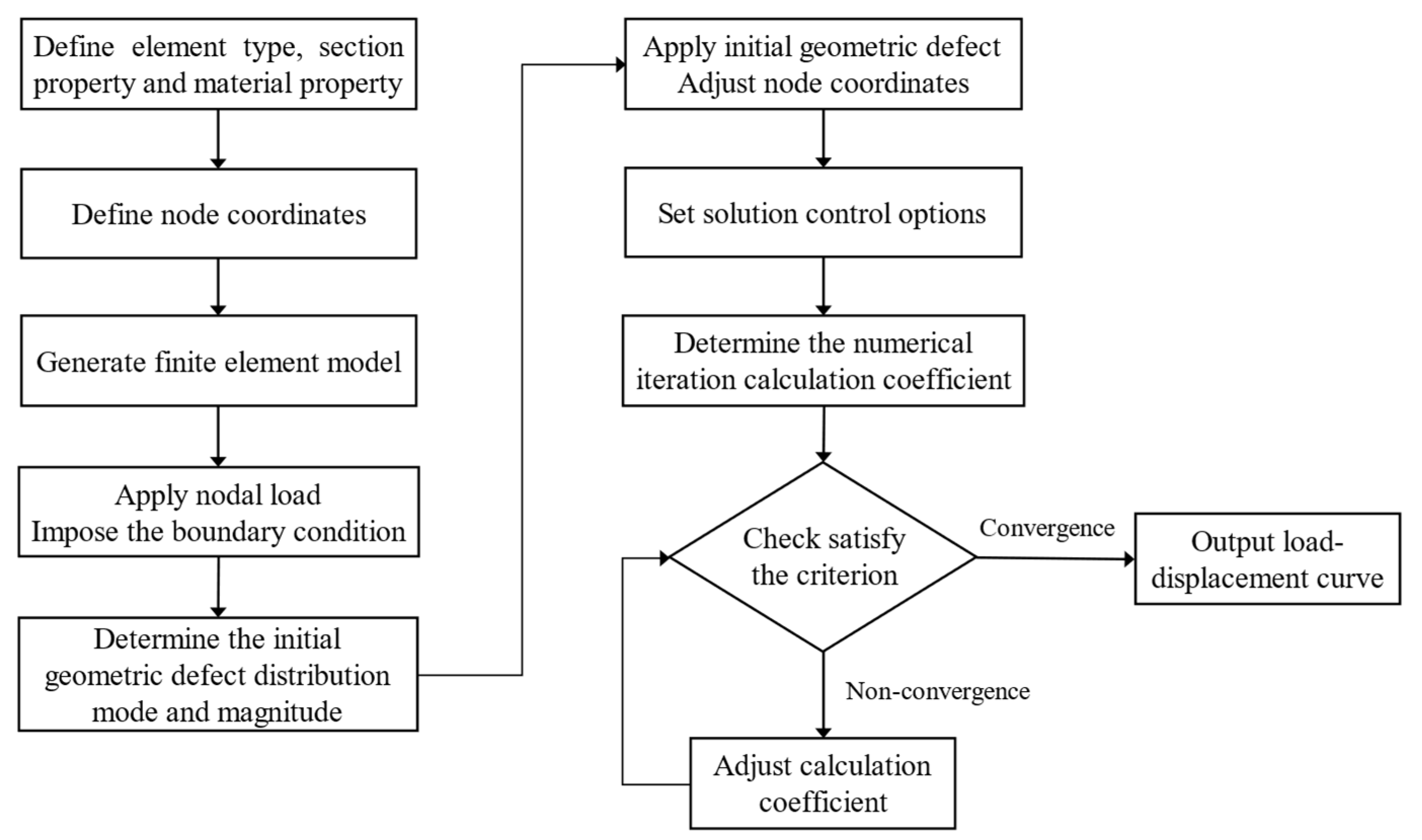
2.2.2. Calculation Model
- (1)
- Spherical arc-length method
- (2)
- Cylindrical arc-length methodwhere Δl is the increment of the arc length at each iteration.
2.2.3. Numerical Model
3. Results
4. Discussion
4.1. Stress Analysis
4.1.1. Analysis of the Member Force State
4.1.2. Analysis of the SFS
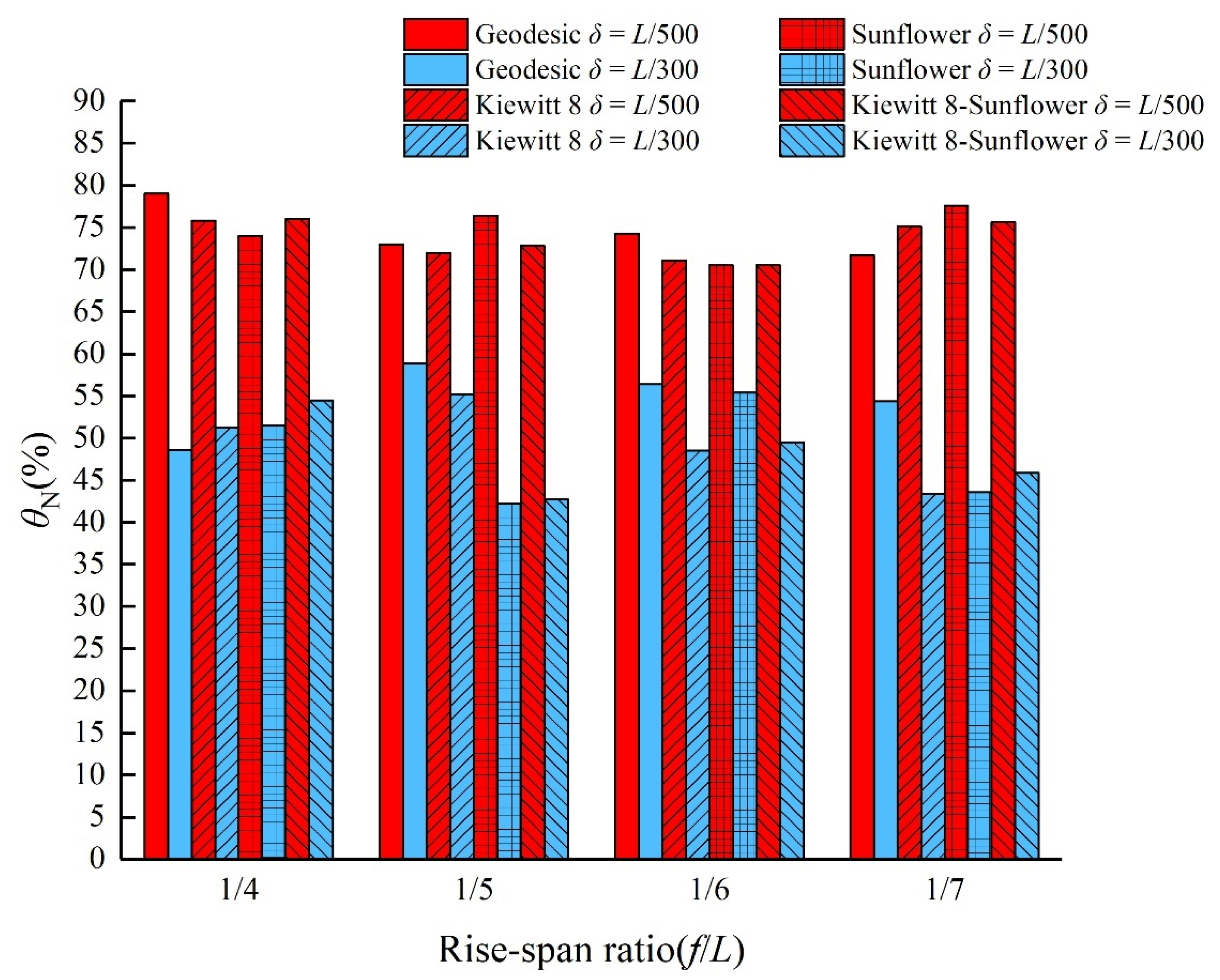
4.2. Selection of IGDM Based on the SFS
4.2.1. Determination Criteria for SFS
4.2.2. Determination of the IGDM
4.3. Selection of the IGDM Based on the Defect Coefficient
4.3.1. Choosing of the Defect Coefficient
4.3.2. Identification of the IGDM
5. Conclusions
- Increasing the initial geometric defect magnitude (IGDM) within a rational range always leads to a fall down of the stability critical load (SCL), and it does not perform a smooth or increase trend as did the earlier research [32].
- It is feasible to select 0.50 as the defect coefficient, which is better to describe the influence of the initial geometric defect (IGD) on the structural stability.
- The structure force state at the stability critical state (SCS) could be estimated by the ratio of normal stress produced by the bending moment and axial force. Briefly speaking, when the average of percentage of the member in which the force caused by the axial stress dominates (MAS) and is larger than 0.70 and meanwhile in these members, the average of the ratio of normal stress produced by the bending moment and axial force (RBA) is less than 0.25, the structural force state is dominated by the film internal force.
- When the initial geometric defect magnitude (IGDM) is adopted as L/300, the structure force state (SFS) is not the film internal force, and hence in this case, the initial geometric defect (IGD) on the structural stability is overestimated. Therefore, considering the structure force state (SFS) and the influence of the initial geometric defect (IGD) on the structural stability, it is more proper to select L/500 as the initial geometric defect magnitude (IGDM).
6. Recommendation for Future Research and Engineering Practice
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| IGD (Δ) | Initial Geometric Defect | R | Maximum allowable installation deviation |
| IGDM (δ) | Initial Geometric Defect Magnitude | W | Random variable |
| SFS | Structure Force State | Kt | Structural tangential stiffness matrix at time t |
| SCS | Stability Critical State | ΔU(i) | Iteration displacement increment at current time step |
| SCL | Stability Critical Load | F | Load vector |
| RBA (αi) | Ratio of normal stress produced by Bending moment and Axial force | Proportional coefficient of load at the i-th iteration | |
| MAS | Member in which the force caused by Axial Stress dominates | Δl | Increment of arc length at each iteration |
| MAB | Member with the force led by both Axial stress and Bending stress | N1 | Assumed optimal number of iterations at each step |
| L | Structural span | N2 | Number of iterations in the previous calculation step |
| A | Area of cross section of straight circular steel tube | E | Elastic modulus |
| My | Bending moment with y direction | ν | Poisson ratio |
| Mz | Bending moment with z direction | fy | Yield strength |
| N | Uniaxial force | f | Structural rise |
| l | Length of the tube | βδ | SCL ratio of the imperfect model to the perfect model |
| D | Outer diameter for cross section | σMi | Maximum bending stress |
| τxy | Shear stress with y direction | σNi | Axial stress of the i-th member |
| τxz | Shear stress with z direction | γN | Average of the RBA of the MAS |
| σxx | Normal stress | Sum of the RBA of the MAS | |
| σyield | Yield stress | j | Number of the MAS |
| Iy | Moment of inertia with respect to the y axis | ηN | Percentage of MAS to effective member |
| δe | Elastic zone with z direction | Mean value of the RBA of the MAB | |
| d | Inner diameter | Sum of the RBA of the MAB | |
| σp | Plastic stress | k | Number of the MAB |
| J2 | Second stress tensor invariant | Percentage of MAB to effective member | |
| k | Hardening coefficient | m | Number of effective members |
| Φ | Mises yield function | φN | Average of the MAS with RBA |
| Sij | Deviatoric stress tensor | θN | Average percentage of MAS to effective member |
| G | Shear modulus | Average of the MAB with RBA | |
| K | Bulk modulus | Average of percentage of MAB to effective member | |
| dλ | Plastic factor | Fδ | SCL of imperfected structure |
| σm | Average of principal stress | F0 | SCL of perfect structure |
| δσij | Variation of stress |
References
- Ma, J.; Fan, F.; Zhang, L.; Wu, C.; Zhi, X. Failure modes and failure mechanisms of single-layer reticulated domes subjected to interior blasts. Thin Wall Struct. 2018, 132, 208–216. [Google Scholar] [CrossRef]
- Fan, F.; Wang, D.; Zhi, X.; Shen, S. Failure modes of reticulated domes subjected to impact and the judgment. Thin Wall Struct. 2010, 48, 143–149. [Google Scholar] [CrossRef]
- Yu, P.; Yun, W.; Bordas, S.; He, S.; Zhou, Y. Static Stability Analysis of Single-Layer Reticulated Spherical Shell with Kiewitt-Sunflower Type. Int. J. Steel Struct. 2021, 21, 1859–1877. [Google Scholar] [CrossRef]
- He, S.; Wang, H.; Bordas, S.P.A.; Yu, P. A Developed Damage Constitutive Model for Circular Steel Tubes of Reticulated Shells. Int. J. Struct. Stab. Dyn. 2020, 20, 2050106. [Google Scholar] [CrossRef]
- He, S.; Nie, Y.; Bordas, S.P.A.; Yu, P. Damage-Plastic Constitutive Model of Thin-Walled Circular Steel Tubes for Space Structures. J. Eng. Mech. 2020, 146, 4020131. [Google Scholar] [CrossRef]
- Fan, F.; Yan, J.; Cao, Z. Elasto-plastic stability of single-layer reticulated domes with initial curvature of members. Thin Wall Struct. 2012, 60, 239–246. [Google Scholar] [CrossRef]
- Fan, F.; Yan, J.; Cao, Z. Stability of reticulated shells considering member buckling. J. Constr. Steel Res. 2012, 77, 32–42. [Google Scholar] [CrossRef]
- Zhao, Z.W.; Liu, H.Q.; Liang, B.; Yan, R.Z. Influence of random geometrical imperfection on the stability of single-layer reticulated domes with semi-rigid. Adv. Steel Constr. 2019, 15, 93–99. [Google Scholar] [CrossRef]
- Fan, F.; Cao, Z.; Shen, S. Elasto-plastic stability of single-layer reticulated shells. Thin Wall Struct. 2010, 48, 827–836. [Google Scholar] [CrossRef]
- Elishakoff, I. Uncertain buckling: Its past, present and future. Int. J. Solids Struct. 2000, 37, 6869–6889. [Google Scholar] [CrossRef]
- Bruno, L.; Sassone, M.; Venuti, F. Effects of the Equivalent Geometric Nodal Imperfections on the stability of single layer grid shells. Eng. Struct. 2016, 112, 184–199. [Google Scholar] [CrossRef] [Green Version]
- Chen, G.; Zhang, H.; Rasmussen, K.J.R.; Fan, F. Modeling geometric imperfections for reticulated shell structures using random field theory. Eng. Struct. 2016, 126, 481–489. [Google Scholar] [CrossRef]
- Kashani, M.; Croll, J.G.A. Lower bounds for overall buckling of spherical space domes. J. Eng. Mech. 1994, 120, 949–970. [Google Scholar] [CrossRef]
- Gioncu, V. Buckling of Reticulated Shells: State-of-the-Art. Int. J. Space Struct. 1995, 10, 1–46. [Google Scholar] [CrossRef]
- Kato, S.; Mutoh, I.; Shomura, M. Collapse of semi-rigidly jointed reticulated domes with initial geometric imperfections. J. Constr. Steel Res. 1998, 48, 145–168. [Google Scholar] [CrossRef]
- He, Y.; Zhou, X.; Liu, D. Research on stability of single-layer inverted catenary cylindrical reticulated shells. Thin Wall Struct. 2014, 82, 233–244. [Google Scholar] [CrossRef]
- Zhu, S.; Ohsaki, M.; Guo, X.; Zeng, Q. Shape optimization for non-linear buckling load of aluminum alloy reticulated shells with gusset joints. Thin Wall Struct. 2020, 154, 106830. [Google Scholar] [CrossRef]
- Shan, Z.; Ma, H.; Yu, Z.; Fan, F. Dynamic failure mechanism of single-layer reticulated (SLR) shells with bolt-column (BC) joint. J. Constr. Steel Res. 2020, 169, 106042. [Google Scholar] [CrossRef]
- Chen, X.; Shen, S.Z. Complete Load-Deflection Response and Initial Imperfection Analysis of Single-Layer Lattice Dome. Int. J. Space Struct. 1993, 8, 271–278. [Google Scholar] [CrossRef]
- Yamada, S.; Takeuchi, A.; Tada, Y.; Tsutsumi, K. Imperfection-sensitive overall buckling of single-layer lattice domes. J. Eng. Mech. 2001, 127, 382–386. [Google Scholar] [CrossRef]
- Zhao, J.; Zhang, Y.; Lin, Y. Study on mid-height horizontal bracing forces considering random initial geometric imperfections. J. Constr. Steel Res. 2014, 92, 55–66. [Google Scholar] [CrossRef]
- Liu, H.; Zhang, W.; Yuan, H. Structural stability analysis of single-layer reticulated shells with stochastic imperfections. Eng. Struct. 2016, 124, 473–479. [Google Scholar] [CrossRef]
- See, T.; McConnel, R.E. Large displacement elastic buckling of space structures. J. Struct. Eng. 1986, 112, 1052–1069. [Google Scholar] [CrossRef]
- Borri, C.; Spinelli, P. Buckling and post-buckling behaviour of single layer reticulated shells affected by random Buckling and post-buckling behaviour of single layer reticulated shells affected by random imperfections. Comput. Struct. 1988, 4, 937–943. [Google Scholar] [CrossRef]
- Morris, N.F. Effect of imperfections on lattice shells. J. Struct. Eng. 1991, 117, 1796–1814. [Google Scholar] [CrossRef]
- Ministry of Housing and Urban-Rural Development of the People’s Republic of China. Technical Specification for Space Frame Structure. (JGJ7–2010); China Architecture Industry Press: Beijing, China, 2010. [Google Scholar]
- Liu, H.; Ding, Y.; Chen, Z. Static stability behavior of aluminum alloy single-layer spherical latticed shell structure with Temcor joints. Thin Wall Struct. 2017, 120, 355–365. [Google Scholar] [CrossRef]
- Cui, X.Z.; Li, Y.G.; Hong, H.P. Effect of spatially correlated initial geometric imperfection on reliability of spherical latticed shell considering global instability. Struct. Saf. 2020, 82, 101895. [Google Scholar] [CrossRef]
- Xiong, Z.; Guo, X.; Luo, Y.; Zhu, S. Elasto-plastic stability of single-layer reticulated shells with aluminium alloy gusset joints. Thin Wall Struct. 2017, 115, 163–175. [Google Scholar] [CrossRef]
- Guo, J.M. Research on distribution and magnitude of initial geometrical imperfection affecting stability of suspen-dome. Adv. Steel Constr. 2011, 7, 344–358. [Google Scholar]
- Shu, Z.; Li, Z.; He, M.; Chen, F.; Hu, C.; Liang, F. Bolted joints for small and medium reticulated timber domes: Experimental study, numerical simulation, and design strength estimation. Arch. Civ. Mech. Eng. 2020, 20, 1–24. [Google Scholar] [CrossRef]
- Shen, S.Z.; Chen, X. Stability of Lattice Shell Structures; Science Press of China: Beijing, China, 1999. [Google Scholar]
- Štok, B.; Halilovič, M. Analytical solutions in elasto-plastic bending of beams with rectangular cross section. Appl. Math. Model. 2009, 33, 1749–1760. [Google Scholar] [CrossRef]
- Dai, H.H.; Huo, Y. Asymptotically approximate model equations for nonlinear dispersive waves in incompressible elastic rods. Acta Mech. 2002, 157, 97–112. [Google Scholar] [CrossRef]
- Chen, W.-F.; Han, D.-J. Plasticity for Structural Engineers; Springer Science & Business Media: Berlin, Germany, 2012. [Google Scholar]
- Rice, J.A. Mathematical Statistics and Data Analysis; Cengage Learning: Boston, MA, USA, 2006. [Google Scholar]
- Crisfield, M.A. A faster modified newton-raphson iteration. Comput. Methods Appl. Mech. Eng. 1979, 20, 267–278. [Google Scholar] [CrossRef]
- Crisfield, M.A. An arc-length method including line searches and accelerations. Int. J. Numer. Methods Eng. 1983, 19, 1269–1289. [Google Scholar] [CrossRef]
- Riks, E. An incremental approach to the solution of snapping and buckling problems. Int. J. Solids Struct. 1979, 15, 529–551. [Google Scholar] [CrossRef]
- Batoz, J.; Dhatt, G. Incremental displacement algorithms for nonlinear problems. Int. J. Numer. Methods Eng. 1979, 14, 1262–1267. [Google Scholar] [CrossRef]
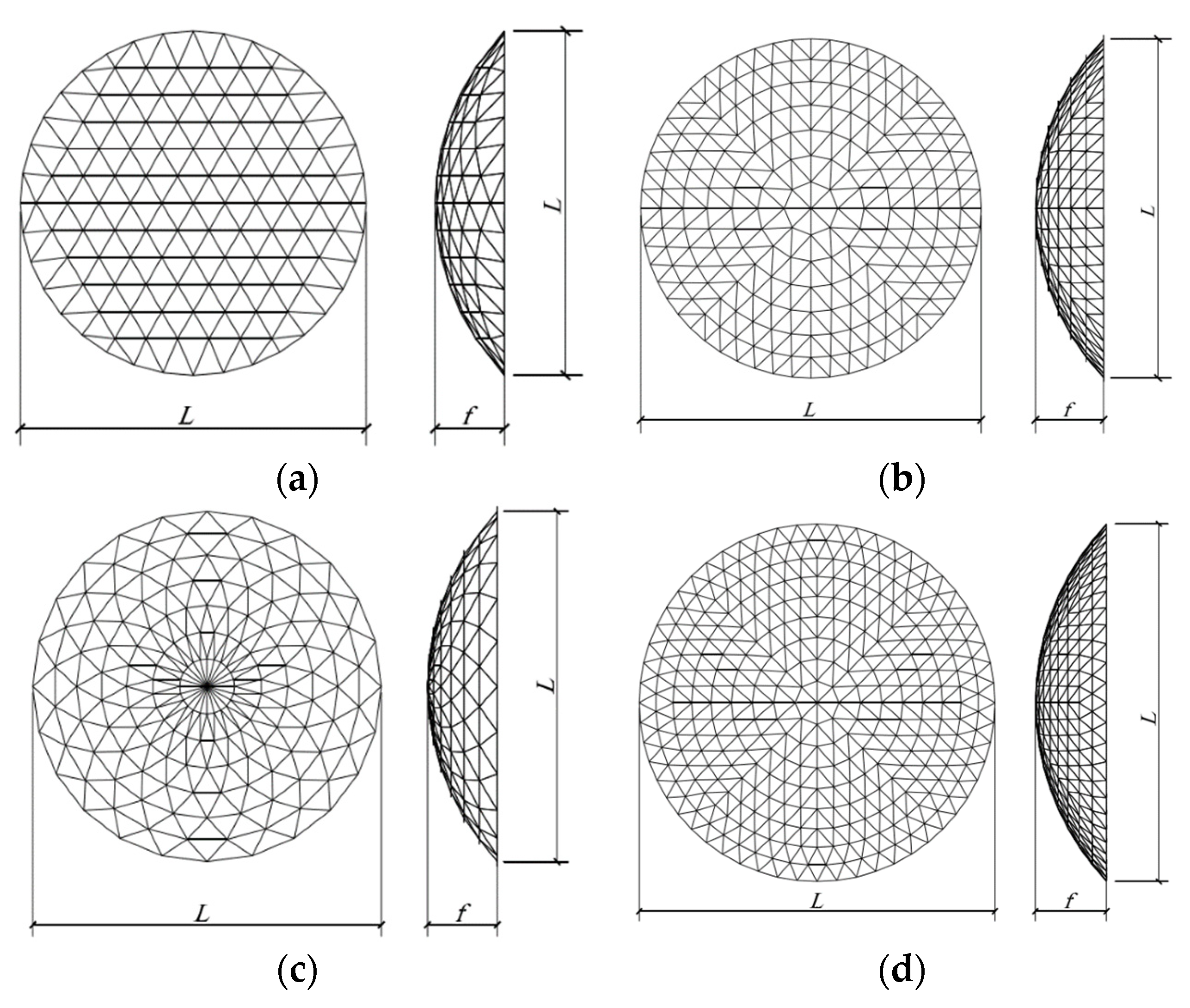

| Type | L(m) | f/L | Radial and Hoop Members | Oblique Members |
|---|---|---|---|---|
| Outer Diameter × Wall Thickness (mm) | ||||
| Geodesic | 40 | 1/7, 1/6, 1/5, 1/4 | Φ121 × 5 | Φ121 × 5 |
| Kiewitt 8 | 50 | Φ168 × 6 | Φ152 × 5 | |
| Sunflower | 60 | Φ180 × 6 | Φ168 × 5 | |
| Kiewitt 8-Sunflower | 70 | Φ219 × 7/Φ152 × 6 | Φ180 × 6/Φ203 × 6 | |
| δ | Geodesic (f/L = 1/7) | Kiewitt 8 (f/L = 1/5) | Sunflower (f/L = 1/6) | Kiewitt 8-Sunflower (f/L = 1/4) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Δ (mm) | Fδ (kN/m2) | βδ | Δ (mm) | Fδ (kN/m2) | βδ | Δ (mm) | Fδ (kN/m2) | βδ | Δ (mm) | Fδ (kN/m2) | βδ | |
| 0 | 0 | 5422 | — | 0 | 10.237 | — | 0 | 5158 | — | 0 | 9.810 | — |
| L/1000 | 40 | 3815 | 0.70 | 50 | 6953 | 0.68 | 60 | 3603 | 0.70 | 70 | 6918 | 0.71 |
| L/900 | 44 | 3498 | 0.65 | 56 | 6616 | 0.65 | 67 | 3387 | 0.66 | 78 | 6566 | 0.67 |
| L/800 | 50 | 3222 | 0.59 | 63 | 6255 | 0.61 | 75 | 3154 | 0.61 | 88 | 6123 | 0.62 |
| L/700 | 57 | 3007 | 0.55 | 71 | 5846 | 0.57 | 86 | 3001 | 0.58 | 100 | 5699 | 0.58 |
| L/600 | 67 | 2794 | 0.52 | 83 | 5482 | 0.54 | 100 | 2788 | 0.54 | 117 | 5284 | 0.54 |
| L/500 | 80 | 2540 | 0.47 | 100 | 4869 | 0.48 | 120 | 2497 | 0.48 | 140 | 4886 | 0.50 |
| L/400 | 100 | 2432 | 0.45 | 125 | 4206 | 0.41 | 150 | 2196 | 0.43 | 175 | 4412 | 0.45 |
| L/300 | 133 | 2262 | 0.42 | 167 | 3979 | 0.39 | 200 | 1998 | 0.39 | 233 | 3953 | 0.40 |
| L/200 | 200 | 1958 | 0.36 | 250 | 3532 | 0.35 | 300 | 1656 | 0.32 | 350 | 3217 | 0.33 |
| L/100 | 400 | 1488 | 0.27 | 500 | 2911 | 0.28 | 600 | 0963 | 0.19 | 700 | 2112 | 0.22 |
| δ | Geodesic (f/L = 1/7) | Kiewitt 8 (f/L = 1/5) | Sunflower (f/L = 1/6) | Kiewitt 8-Sunflower (f/L = 1/4) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Δ (mm) | φN | θN (%) | Δ (mm) | φN | θN (%) | Δ (mm) | φN | θN (%) | Δ (mm) | φN | θN (%) | |
| 0 | 0 | 0.169 | 82.8 | 0 | 0.141 | 92.9 | 0 | 0.175 | 89.0 | 0 | 0.183 | 89.3 |
| L/1000 | 40 | 0.186 | 82.1 | 50 | 0.165 | 83.8 | 60 | 0.198 | 80.2 | 70 | 0.195 | 83.6 |
| L/900 | 44 | 0.195 | 80.5 | 56 | 0.177 | 82.2 | 67 | 0.204 | 78.5 | 78 | 0.201 | 82.3 |
| L/800 | 50 | 0.206 | 79.5 | 63 | 0.190 | 79.9 | 75 | 0.215 | 77.2 | 88 | 0.211 | 81.1 |
| L/700 | 57 | 0.218 | 78.3 | 71 | 0.203 | 77.6 | 86 | 0.223 | 75.5 | 100 | 0.226 | 79.8 |
| L/600 | 67 | 0.224 | 75.0 | 83 | 0.225 | 75.3 | 100 | 0.234 | 73.4 | 117 | 0.235 | 78.3 |
| L/500 | 80 | 0.235 | 71.7 | 100 | 0.249 | 72.0 | 120 | 0.240 | 70.5 | 140 | 0.244 | 76.0 |
| L/400 | 100 | 0.281 | 65.3 | 125 | 0.279 | 63.9 | 150 | 0.282 | 65.0 | 175 | 0.293 | 68.5 |
| L/300 | 133 | 0.325 | 54.4 | 167 | 0.319 | 55.2 | 200 | 0.306 | 55.4 | 233 | 0.323 | 54.5 |
| L/200 | 200 | 0.411 | 42.9 | 250 | 0.402 | 41.0 | 300 | 0.376 | 40.2 | 350 | 0.409 | 40.0 |
| L/100 | 400 | 0.505 | 22.2 | 500 | 0.498 | 21.2 | 600 | 0.437 | 20.9 | 700 | 0.499 | 20.5 |
| Type | L(m) | f/L | δ = L/500 | δ = L/300 | ||
|---|---|---|---|---|---|---|
| φN | θN(%) | φN | θN(%) | |||
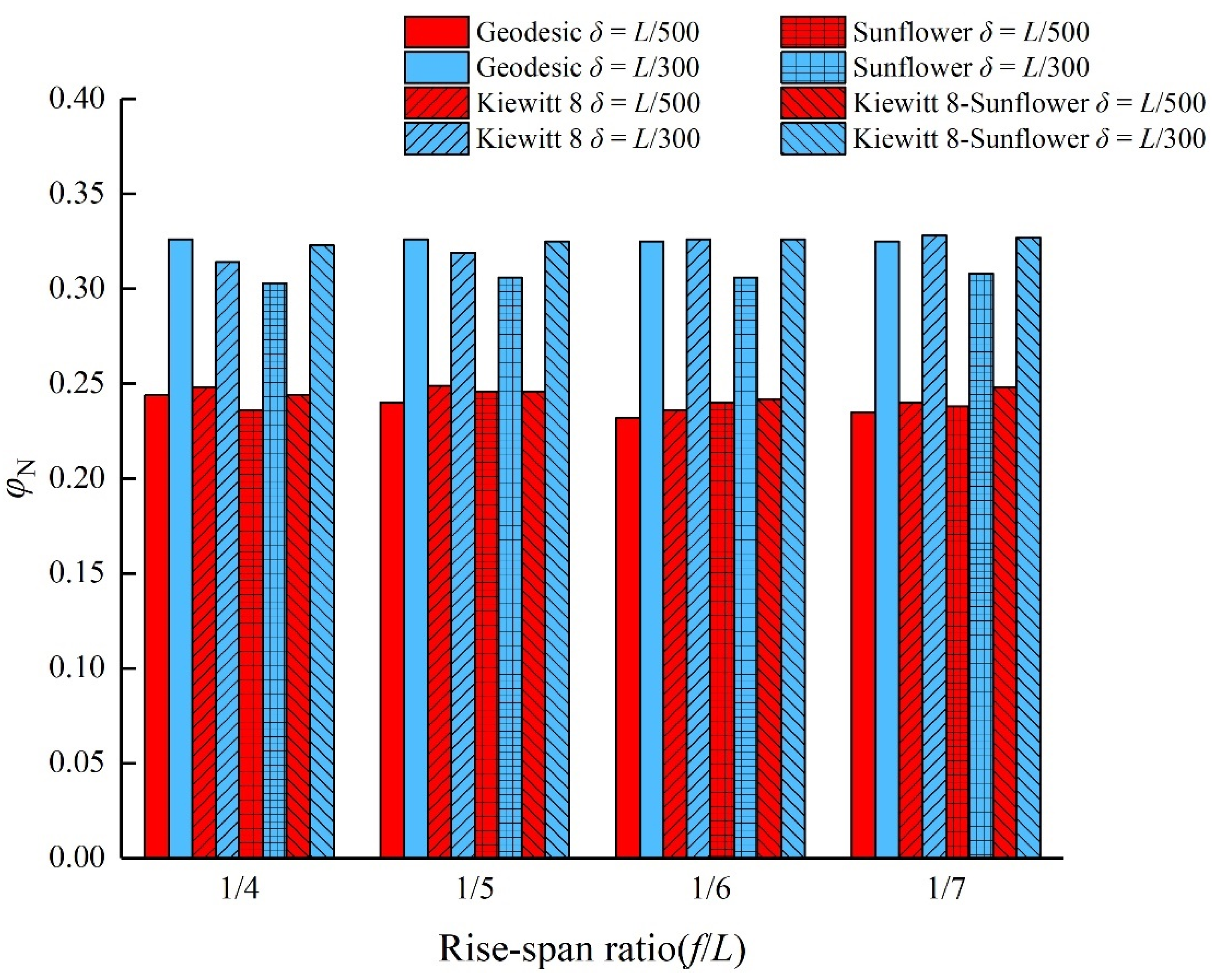
| Geodesic | 40 | 1/4 | 0.244 | 79.0 | 0.326 | 48.6 |
| 1/5 | 0.240 | 73.0 | 0.326 | 58.9 | ||
| 1/6 | 0.232 | 74.3 | 0.325 | 56.4 | ||
| 1/7 | 0.235 | 71.7 | 0.325 | 54.4 | ||
| Kiewitt 8 | 50 | 1/4 | 0.248 | 75.8 | 0.314 | 51.2 |
| 1/5 | 0.249 | 72.0 | 0.319 | 55.2 | ||
| 1/6 | 0.236 | 71.1 | 0.326 | 48.5 | ||
| 1/7 | 0.240 | 75.1 | 0.328 | 43.4 | ||
| Sunflower | 60 | 1/4 | 0.236 | 74.0 | 0.303 | 51.5 |
| 1/5 | 0.246 | 76.4 | 0.306 | 42.2 | ||
| 1/6 | 0.240 | 70.5 | 0.306 | 55.4 | ||
| 1/7 | 0.238 | 77.6 | 0.308 | 43.6 | ||
| Kiewitt 8-Sunflower | 70 | 1/4 | 0.244 | 76.0 | 0.323 | 54.5 |
| 1/5 | 0.246 | 72.8 | 0.325 | 42.7 | ||
| 1/6 | 0.242 | 70.5 | 0.326 | 49.5 | ||
| 1/7 | 0.248 | 75.6 | 0.327 | 45.9 | ||
| Type | L (m) | f/L | F0 (kN/m2) | δ = L/500 | δ = L/300 | ||
|---|---|---|---|---|---|---|---|
| Fδ (kN/m2) | βδ | Fδ (kN/m2) | βδ | ||||
| Geodesic | 40 | 1/4 | 9598 | 4650 | 0.48 | 3793 | 0.40 |
| 1/5 | 8829 | 4527 | 0.51 | 3314 | 0.38 | ||
| 1/6 | 6802 | 3396 | 0.50 | 2722 | 0.40 | ||
| 1/7 | 5422 | 2540 | 0.47 | 2262 | 0.42 | ||
| Kiewitt 8 | 50 | 1/4 | 12,077 | 5997 | 0.50 | 4795 | 0.40 |
| 1/5 | 10,237 | 4869 | 0.48 | 3979 | 0.39 | ||
| 1/6 | 9154 | 4438 | 0.48 | 3418 | 0.37 | ||
| 1/7 | 8163 | 3861 | 0.47 | 3059 | 0.37 | ||
| Sunflower | 60 | 1/4 | 8179 | 4012 | 0.49 | 3196 | 0.39 |
| 1/5 | 6050 | 2972 | 0.49 | 2419 | 0.40 | ||
| 1/6 | 5158 | 2497 | 0.48 | 1998 | 0.39 | ||
| 1/7 | 4545 | 1996 | 0.44 | 1817 | 0.40 | ||
| Kiewitt 8-Sunflower | 70 | 1/4 | 9810 | 4886 | 0.50 | 3953 | 0.40 |
| 1/5 | 8646 | 4229 | 0.49 | 3371 | 0.39 | ||
| 1/6 | 7538 | 3722 | 0.49 | 2963 | 0.39 | ||
| 1/7 | 5940 | 2780 | 0.47 | 2484 | 0.42 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, S.; Huang, X.; Yu, P.; Yun, W. The Investigation on Static Stability Analysis for Reticulated Shell with Initial Defect Value Using Stochastic Defect Mode Method. Buildings 2022, 12, 615. https://doi.org/10.3390/buildings12050615
He S, Huang X, Yu P, Yun W. The Investigation on Static Stability Analysis for Reticulated Shell with Initial Defect Value Using Stochastic Defect Mode Method. Buildings. 2022; 12(5):615. https://doi.org/10.3390/buildings12050615
Chicago/Turabian StyleHe, Sheng, Xinheng Huang, Peng Yu, and Weijing Yun. 2022. "The Investigation on Static Stability Analysis for Reticulated Shell with Initial Defect Value Using Stochastic Defect Mode Method" Buildings 12, no. 5: 615. https://doi.org/10.3390/buildings12050615
APA StyleHe, S., Huang, X., Yu, P., & Yun, W. (2022). The Investigation on Static Stability Analysis for Reticulated Shell with Initial Defect Value Using Stochastic Defect Mode Method. Buildings, 12(5), 615. https://doi.org/10.3390/buildings12050615






