Optimization of the Use Time of a Shake Table with Specimen Preparation outside the Table Surface
Abstract
:1. Introduction
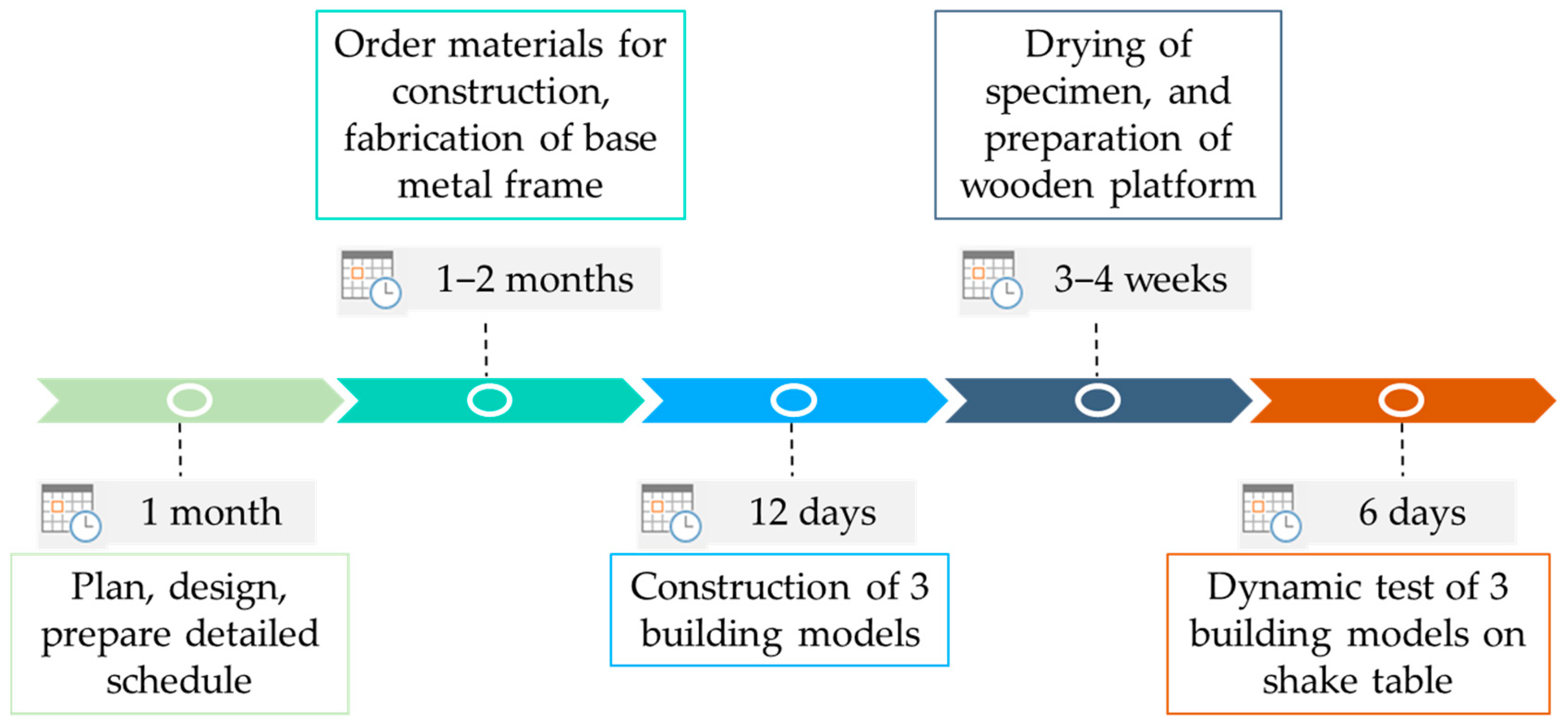
2. Need for and Scope of Work
3. Shake Table Specifications
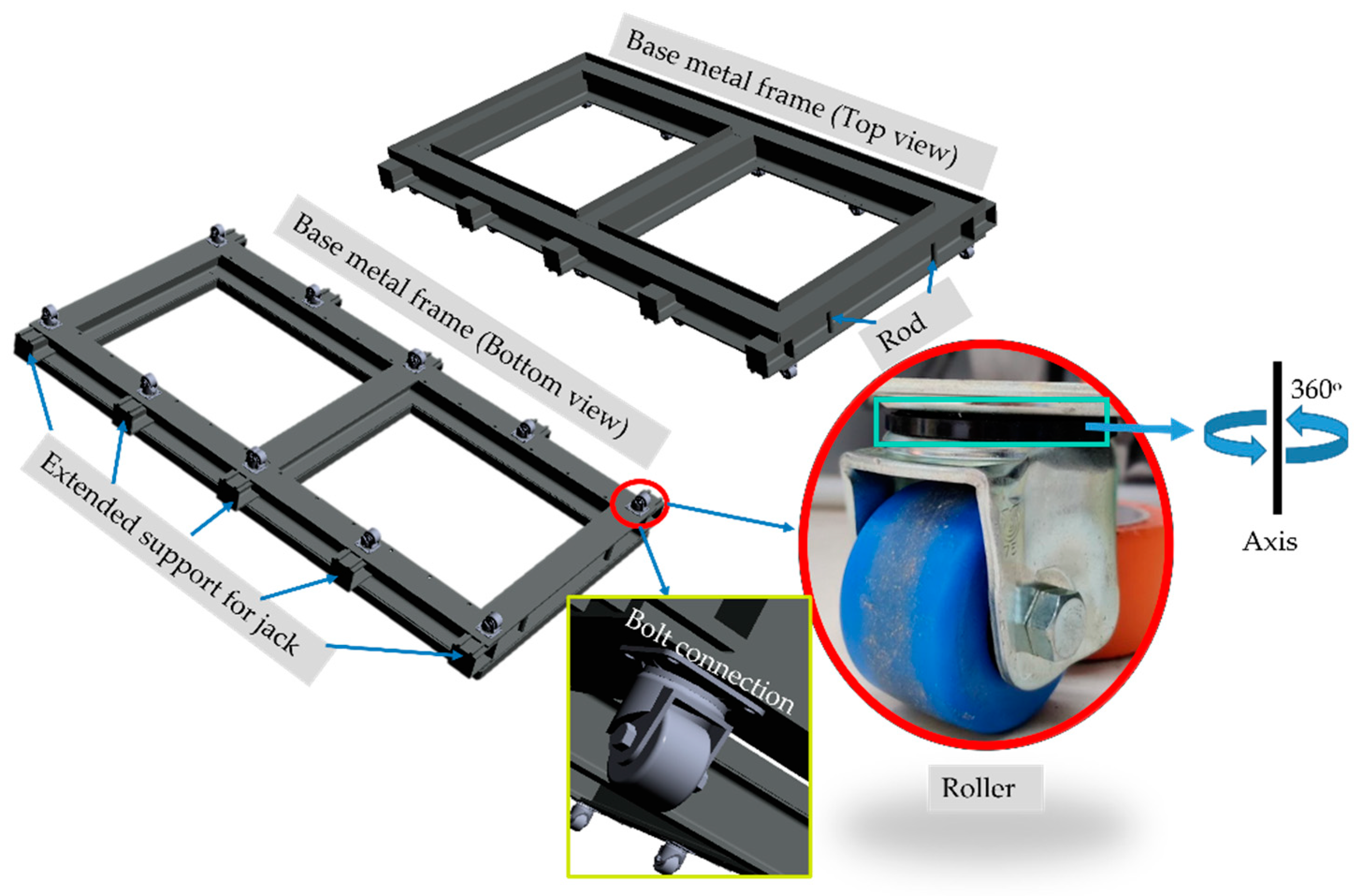
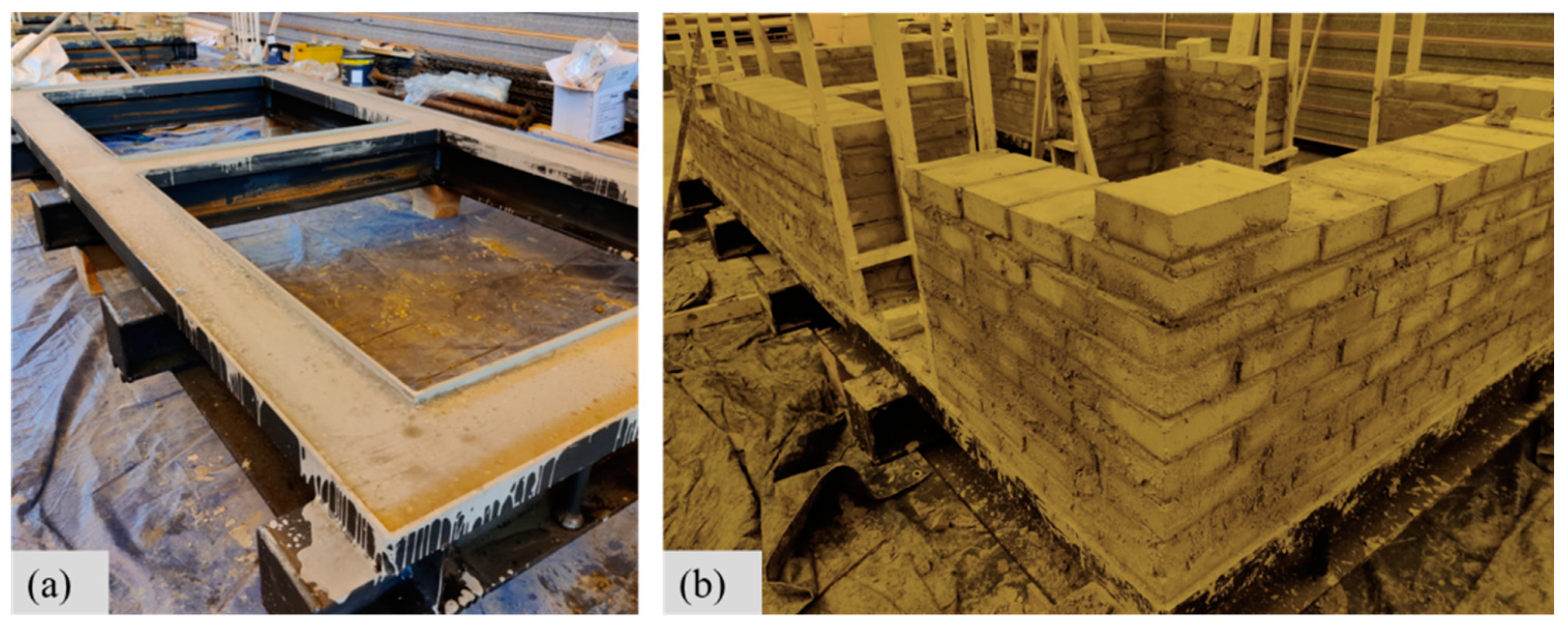
4. Metallic Base Frame
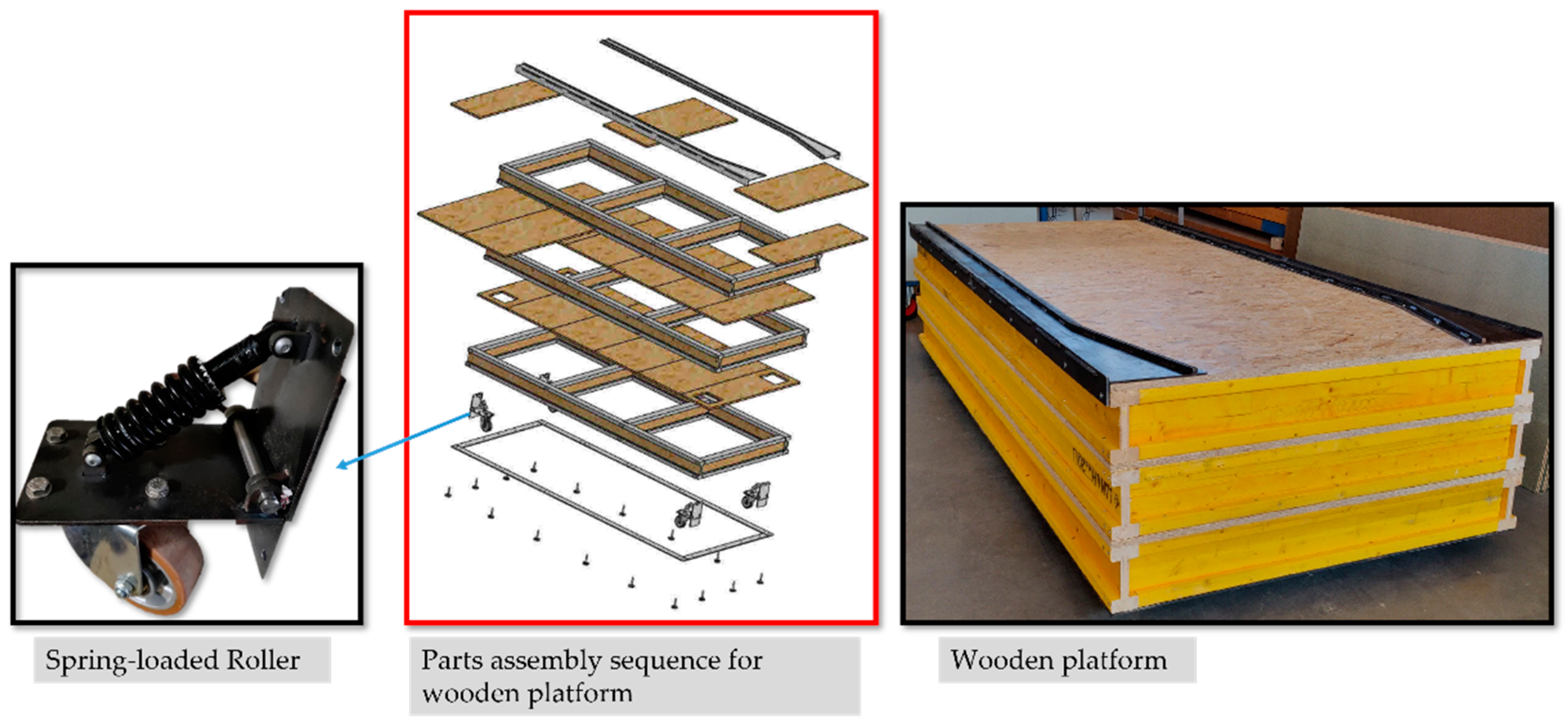
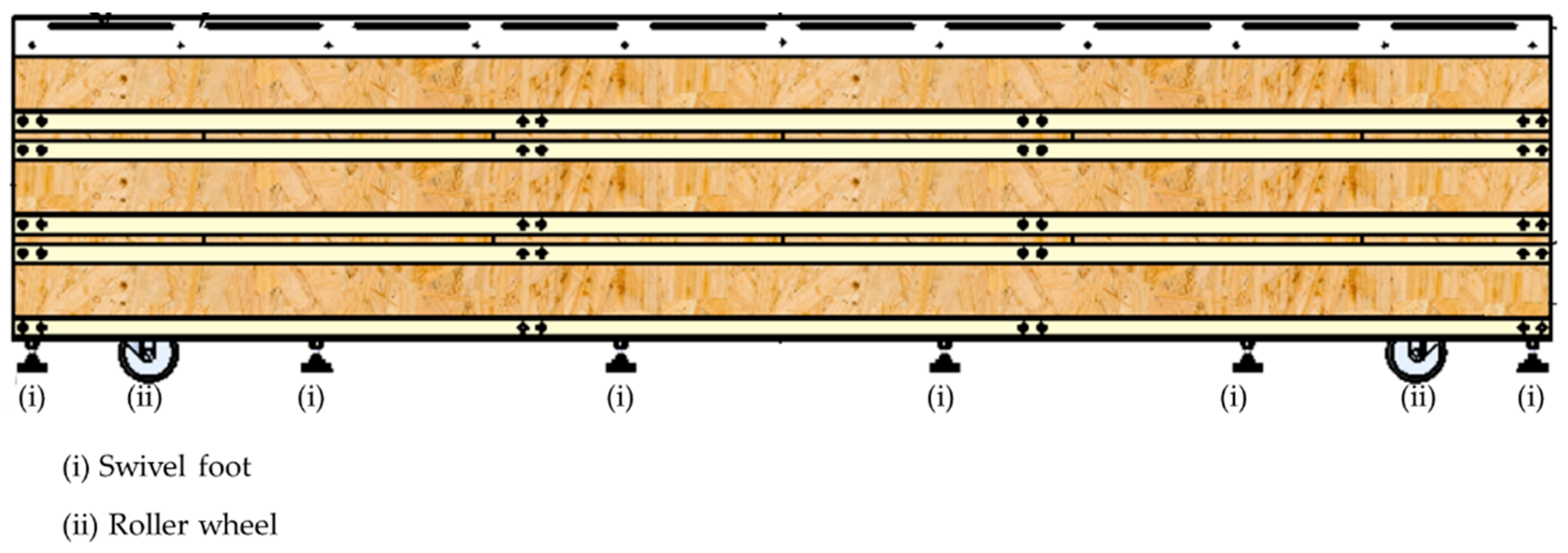
5. Wooden Platform
6. Building Displacement Sequence
- The metallic base frame was gently elevated from the floor to remove the supporting timber parts and to place the ten rollers (see Figure 2) at the bottom of the frame. To do that, four hydraulic jacks (see Figure 6) were used at each corner of the building simultaneously to prevent any damage to the structure;
- Once the model on its rollers was placed on the floor, a weight-lifting belt was passed through the metallic rod (shown in Figure 2) and connected to a forklift truck, as shown in Figure 6a. The static frictional force required to move the model was calculated assuming a frictional coefficient of 0.6 between the rubber and the concrete floor. For a maximum dead load of 4.8 tons, the force required to move the model is 2.88 tons. This calculated force was required when designing the rod parts in the metallic frame (see Figure 2) and in the selection of the weight-lifting belt. With the help of the forklift truck, the building model was moved close to the shake table and aligned parallel to it;
- The next step was to lift the model building vertically and to elevate it high enough to place the wooden platform under it. To elevate the building model, initially, four farm jacks, each with a maximum capacity of 3 tons, were used. However, this approach was not effective due to the lack of stability of the farm jacks for displacement intervals of 20 mm for each lift. Therefore, to improve this process, four hydraulic car jacks, each with a capacity of 2 tons, were used to elevate the building models vertically. The four hydraulic jacks were placed at the four external metal supports. The elevation operation was manually synchronized by counting the pumps (see Figure 6b). The building was elevated in steps of 3–4 cm on all sides simultaneously, and timber blocks were placed under the extended metallic support (see Figure 2) to hold the building in position before beginning each new elevation cycle. Timber blocks were used for security (if one jack had a problem, the building fell only a few centimeters) and because of the limited vertical displacement of the hydraulic car jacks;
- Once the building model was elevated enough to be slightly higher than the shake table site, the wooden platform was placed under the building model, as shown in Figure 6c. The rollers’ alignment was checked before releasing the hydraulic jacks and putting the building model on the wooden platform;
- The building model was finally rolled onto the shake table using the weight-lifting belt attached to the metallic rod on the base frame and pulled using two manual cable pulling sets (also known as come-alongs), as shown in Figure 6d;
- The position of the metallic frame was adjusted to fix the base frame on the table before removing the rollers. All the base rollers were removed using a similar approach as in Stage 1 (see Figure 7a). The metallic frame was fixed to the shake table using 12 bolts. To decrease the total mass during displacement, the roof was assembled on the building only after securely fixing the building to the shake table, as shown in Figure 7b.
7. Conclusions and Recommendation
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Acknowledgments
Conflicts of Interest
References
- Adhikari, R.K.; D’Ayala, D. Numerical Seismic Performance Assessment of Pre-Earthquake Stone Masonry Residential Building Typology in Light of 2015 Nepal Earthquake. In Proceedings of the Society for Earthquake and Civil Engineering Dynamics Conference (SECED), London, UK, 9–10 September 2019. [Google Scholar]
- Ortega, J.; Vasconcelos, G.; Rodrigues, H.; Correia, M. Assessment of the efficiency of traditional earthquake resistant techniques for vernacular architecture. Eng. Struct. 2018, 173, 1–27. [Google Scholar] [CrossRef]
- Ponte, M.; Milosevic, J.; Bento, R. Parametrical study of rubble stone masonry panels through numerical modelling of the in-plane behaviour. Bull. Earthq. Eng. 2019, 17, 1553–1574. [Google Scholar] [CrossRef]
- Damerji, H.; Yadav, S.; Sieffert, Y.; Vieux-champagne, F.; Malecot, Y. Damage investigation of adobe walls using numerical simulations. In Proceedings of the 7th ECCOMAS Thematic Conference on Computational Methods in Structural Dynamics and Earthquake Engineering, Crete, Greece, 24–26 June 2019. [Google Scholar]
- Reyes, J.C.; Smith-Pardo, J.P.; Yamin, L.E.; Galvis, F.A.; Sandoval, J.D.; Gonzalez, C.D.; Correal, J.F. In-plane seismic behavior of full-scale earthen walls with openings retrofitted with timber elements and vertical tensors. Bull. Earthq. Eng. 2019, 17, 4193–4215. [Google Scholar] [CrossRef]
- Howlader, M.K.; Masia, M.J.; Griffith, M.C. Numerical analysis and parametric study of unreinforced masonry walls with arch openings under lateral in-plane loading. Eng. Struct. 2020, 208, 110337. [Google Scholar] [CrossRef]
- Furukawa, A.; Kiyono, J.; Toki, K. Numerical Simulation of the Failure Propagation of Masonry Buildings during an Earthquake. J. Nat. Disaster Sci. 2012, 33, 11–36. [Google Scholar] [CrossRef] [Green Version]
- Vieux-Champagne, F.; Sieffert, Y.; Grange, S.; Polastri, A.; Ceccotti, A.; Daudeville, L. Experimental analysis of seismic resistance of timber-framed structures with stones and earth infill. Eng. Struct. 2014, 69, 102–115. [Google Scholar] [CrossRef]
- Vieux-Champagne, F.; Sieffert, Y.; Grange, S.; Belinga Nko’ol, C.; Bertrand, E.; Duccini, J.C.; Faye, C.; Daudeville, L. Experimental analysis of a shake table test of timber-Framed structures with stone and earth infill. Earthq. Spectra 2017, 33, 1075–1100. [Google Scholar] [CrossRef]
- Decret, D. Numerical Model for the Assessment of the Seismic Vulnerability of Traditional Masonry Structures with Seismic Bands. Ph.D. Thesis, Université Grenoble Alpes, Grenoble, France, 2021. [Google Scholar]
- Flora, A.; Perrone, G.; Cardone, D. Evaluating collapse fragility curves for existing buildings retrofitted using seismic isolation. Appl. Sci. 2020, 10, 2844. [Google Scholar] [CrossRef] [Green Version]
- Maharjan, A.; Parajuli, H.R. Seismic Performance Evaluation of Stone Masonry Houses Constructed with Reinforced Concrete Bands. Nepal J. Sci. Technol. 2020, 19, 204–214. [Google Scholar] [CrossRef]
- Saitta, F.; Clemente, P.; Buffarini, G.; Bongiovanni, G. Vulnerability Analysis and Seismic Retrofit of a Strategic Building. J. Perform. Constr. Facil. 2017, 31, 04016085. [Google Scholar] [CrossRef]
- Ponzo, F.C.; Di Cesare, A.; Telesca, A.; Pavese, A.; Furinghetti, M. Advanced modelling and risk analysis of RC buildings with sliding isolation systems designed by the Italian seismic code. Appl. Sci. 2021, 11, 1938. [Google Scholar] [CrossRef]
- Baran, T.; Tanrikulu, A.K.; Dundar, C.; Tanrikulu, A.H. Construction and performance test of a low-cost shake table. Exp. Tech. 2011, 35, 8–16. [Google Scholar] [CrossRef]
- Damerji, H.; Yadav, S.; Sieffert, Y.; Debove, L.; Vieux-champagne, F.; Malecot, Y. Design of a Shake Table with Moderate Cost. Exp. Tech. 2021. [Google Scholar] [CrossRef]
- Bothara, J.; Desai, R.; Ingham, J.; Dizhur, D. Seismic simulation tools and methods appropriate for developing countries. Structures 2021, 34, 314–326. [Google Scholar] [CrossRef]
- Calabrese, A.; Strano, S.; Terzo, M. Real-time hybrid simulations vs shaking table tests: Case study of a fibre-reinforced bearings isolated building under seismic loading. Struct. Control Health Monit. 2014, 22, 535–556. [Google Scholar] [CrossRef]
- Furinghetti, M.; Lanese, I.; Pavese, A. Experimental Assessment of the Seismic Response of a Base-Isolated Building Through a Hybrid Simulation Technique. Front. Built Environ. 2020, 6, 33. [Google Scholar] [CrossRef]
- Zhang, C.; Jiang, N. A shaking table real-time substructure experiment of an equipment–structure–soil interaction system. Adv. Mech. Eng. 2017, 9, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Mouzakis, C.; Adami, C.; Karapitta, L.; Vintzileou, E. Seismic behaviour of timber-laced stone masonry buildings before and after interventions: Shaking table tests on a two-storey masonry model. Bull. Earthq. Eng. 2018, 16, 803–829. [Google Scholar] [CrossRef]
- Wang, M.; Liu, K.; Guragain, R.; Shrestha, H.; Ma, X. Shake table tests on the two-storey dry-joint stone masonry structures reinforced with timber laces and steel wires. Bull. Earthq. Eng. 2018, 17, 2199–2218. [Google Scholar] [CrossRef]
- Khan, F.Z.; Ahmad, M.E.; Ahmad, N. Shake table testing of confined adobe masonry structures. Earthq. Struct. 2021, 20, 149–160. [Google Scholar] [CrossRef]
- Xie, Q.; Wang, L.; Zhang, L.; Hu, W.; Zhou, T. Seismic behaviour of a traditional timber structure: Shaking table tests, energy dissipation mechanism and damage assessment model. Bull. Earthq. Eng. 2018, 17, 1689–1714. [Google Scholar] [CrossRef]
- Rafi, M.M.; Lodi, S.H.; Qazi, S.A.; Kumar, A.; Verjee, F. Seismic response of reduced scale stone masonry building. Proc. Inst. Civil Eng. Struct. Build. 2018, 171, 528–541. [Google Scholar] [CrossRef]
- Sundqvist, E.; Backlund, F.; Chronéer, D. What is Project Efficiency and Effectiveness? Procedia Soc. Behav. Sci. 2014, 119, 278–287. [Google Scholar] [CrossRef] [Green Version]
- Yadav, S. Experimental Analysis of the Seismic Vulnerability of Earth Masonry Reinforced with Horizontal Bands. Ph.D. Thesis, Université Grenoble Alpes, Grenoble, France, 2021. [Google Scholar]
- DUDBC. Design Catalogue for Reconstruction of Earthquake Resistant Houses; Government of Nepal: Kathmandu, Nepal, 2015; Volume 1.
- Misumi Roulettes—Charge Très Lourde, Type Pivotant. Available online: https://fr.misumi-ec.com/vona2/detail/110300506920/ (accessed on 20 February 2022).
- Yadav, S. Timelapse Video Showing the Steps for Displacing the Specimen on the Shake Table. Available online: https://cloud.univ-grenoble-alpes.fr/index.php/s/bsNnDX4zpEyr4yK/ (accessed on 20 February 2022).


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yadav, S.; Sieffert, Y.; Vieux-Champagne, F.; Debove, L.; Decret, D.; Malecot, Y.; Garnier, P. Optimization of the Use Time of a Shake Table with Specimen Preparation outside the Table Surface. Buildings 2022, 12, 319. https://doi.org/10.3390/buildings12030319
Yadav S, Sieffert Y, Vieux-Champagne F, Debove L, Decret D, Malecot Y, Garnier P. Optimization of the Use Time of a Shake Table with Specimen Preparation outside the Table Surface. Buildings. 2022; 12(3):319. https://doi.org/10.3390/buildings12030319
Chicago/Turabian StyleYadav, Santosh, Yannick Sieffert, Florent Vieux-Champagne, Laurent Debove, Damien Decret, Yann Malecot, and Philippe Garnier. 2022. "Optimization of the Use Time of a Shake Table with Specimen Preparation outside the Table Surface" Buildings 12, no. 3: 319. https://doi.org/10.3390/buildings12030319
APA StyleYadav, S., Sieffert, Y., Vieux-Champagne, F., Debove, L., Decret, D., Malecot, Y., & Garnier, P. (2022). Optimization of the Use Time of a Shake Table with Specimen Preparation outside the Table Surface. Buildings, 12(3), 319. https://doi.org/10.3390/buildings12030319






