Modeling the Effect of Different Support Structures in Electron Beam Melting of Titanium Alloy Using Finite Element Models
Abstract
1. Introduction and Literature Review
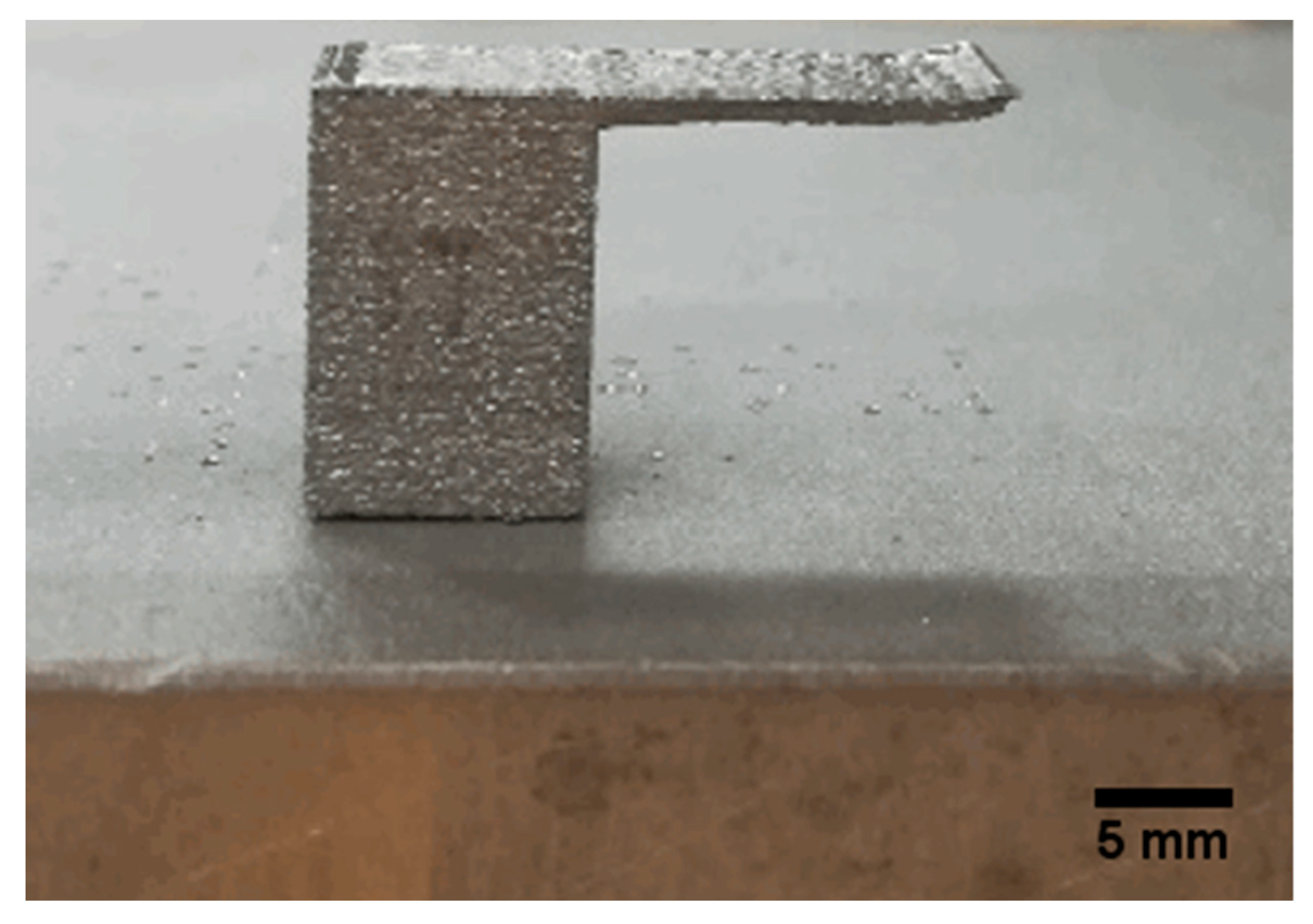
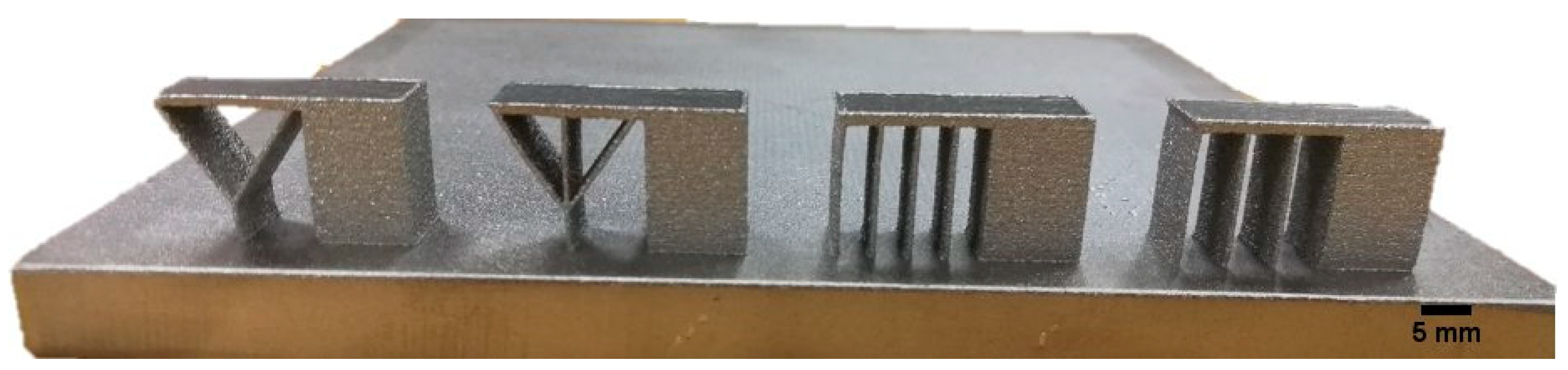
2. Experimental Setup
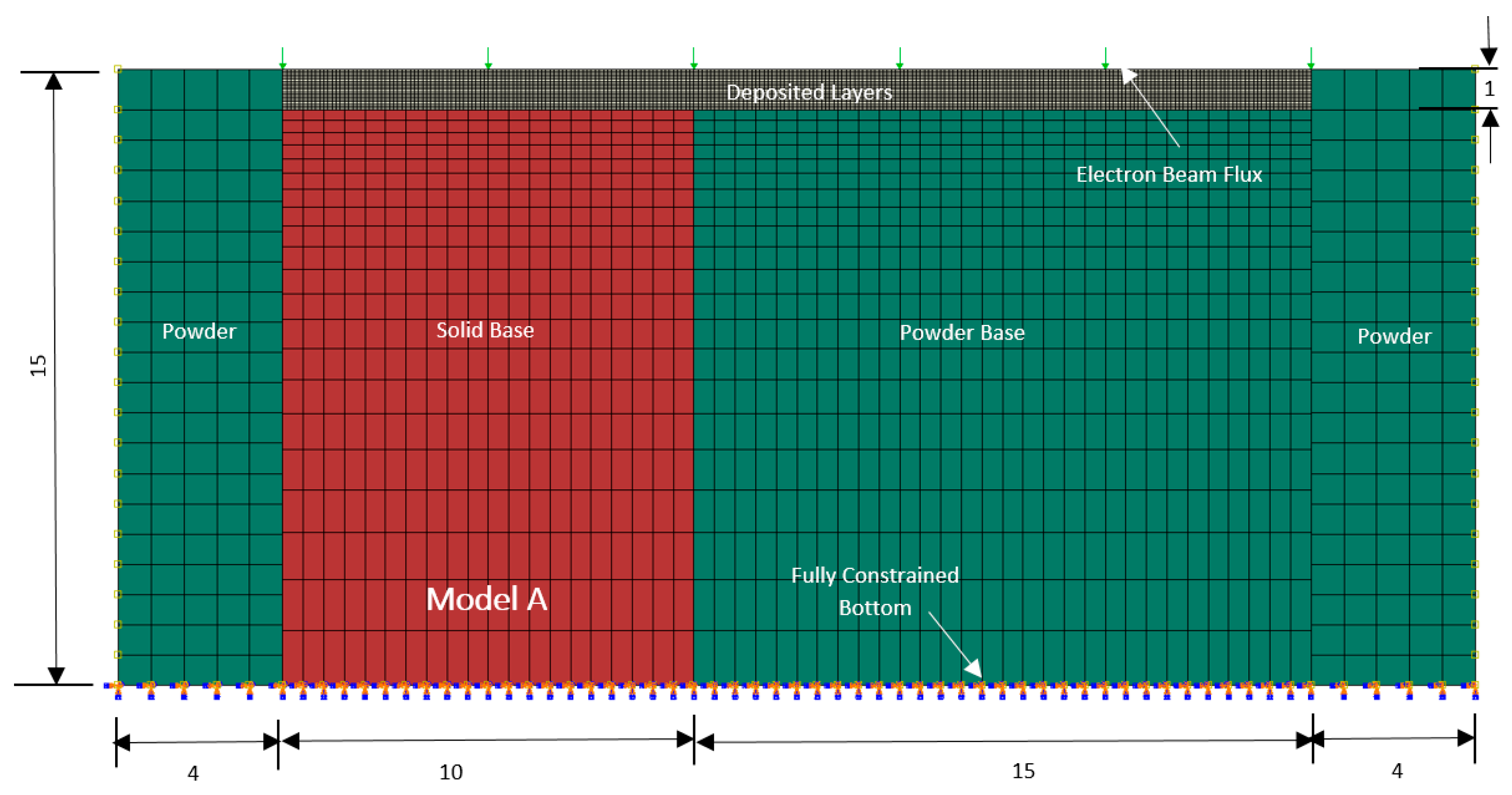
3. Finite Element Models
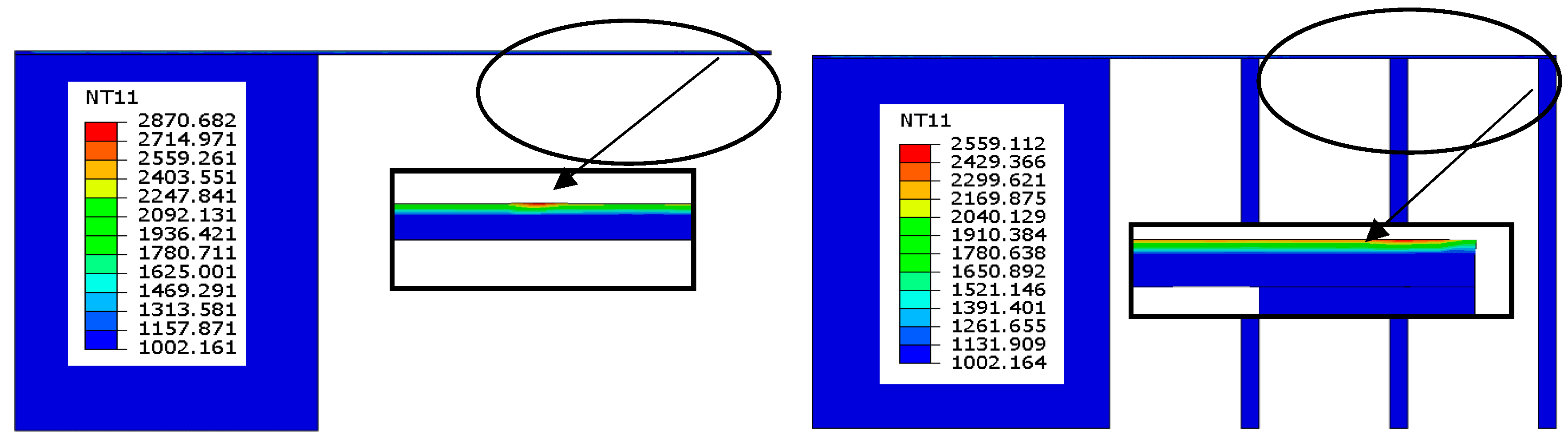
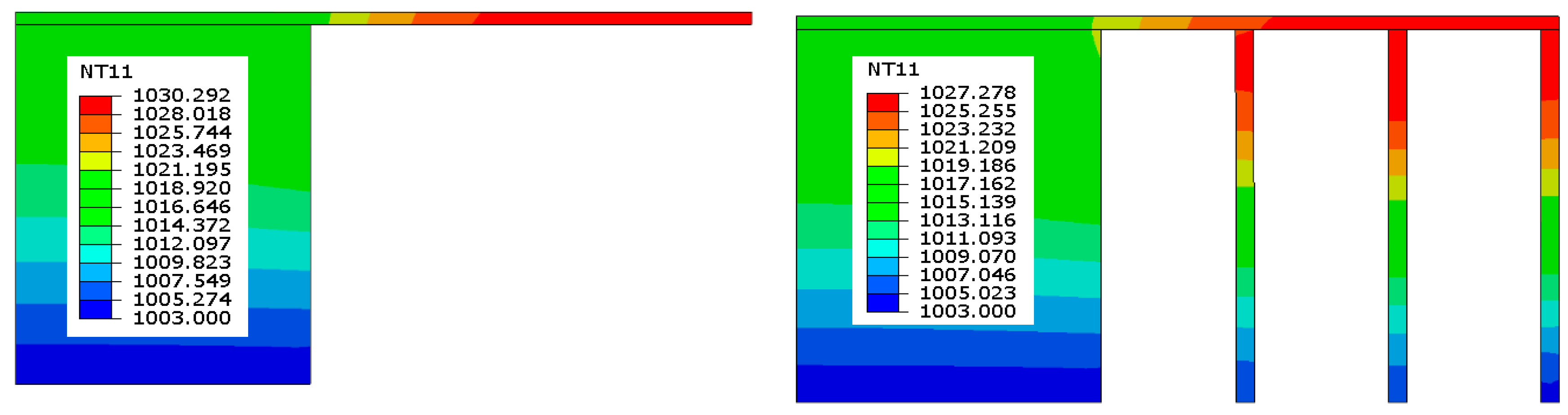
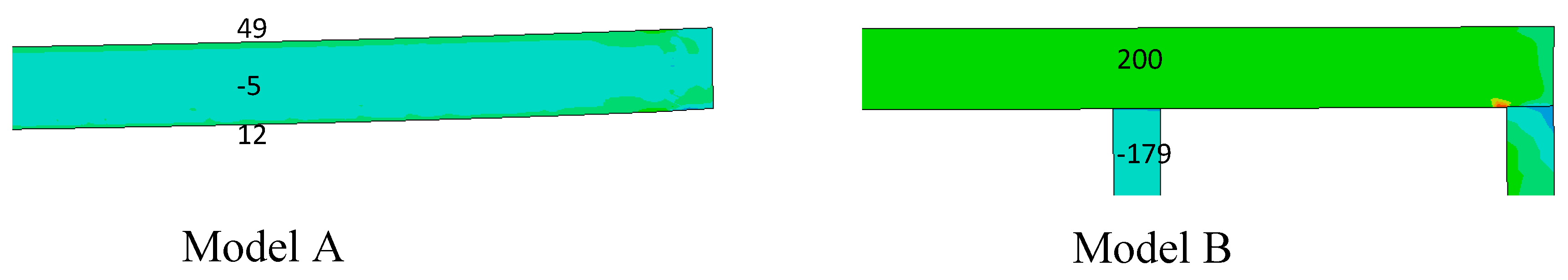
4. Results and Discussions
- Plane strain formulation was considered, i.e., lateral distortions can’t be predicted.
- Due to unavailability of data, mechanical properties of solid and powder alloys were considered the same.
- Simulation of a complete part was not considered due to the requirement of huge computational time. So, deformations present in the base part prior to the deposition of the top 20 layers was ignored.
5. Conclusions
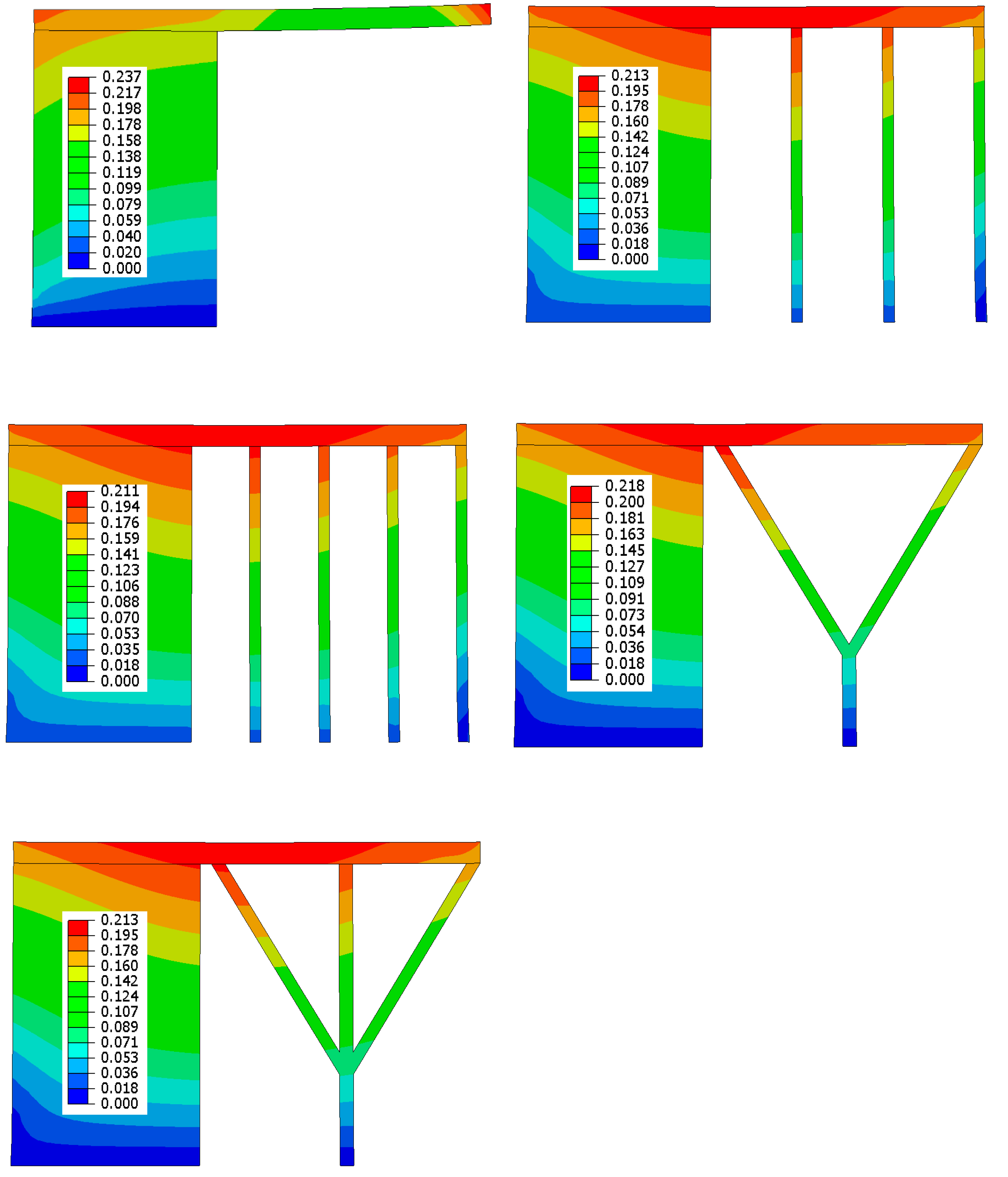
- From the above analysis, it can be concluded that 2D FE models based on plane strain formulations are useful in predicting the overhang deformations for EBM built parts.
- The FE models underestimate the deformations in all cases and the errors are around 22%. However, the models are able to predict the effects of different support structures on overhang warping up to a reasonable degree of accuracy.
- The temperature predictions during each layer build-up could be useful in determining the phase transformation from powder to liquid and liquid to solid alloy.
- These FE models are also useful in determining the size and duration of the melt pool during each layer build-up.
- Residual stress patterns for the deposited layers are found to be very much different from the un-supported model and the overhang areas show fluctuating stresses from tensile to compressive.
- The deposited layers for the models with support structures show uniform tensile stresses, which are balanced by the compressive stresses inside the support columns.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Poyraz, Ö.; Yasa, E.; Akbulut, G.; Orhangül, A.; Pilatin, S. Investigation of support structures for direct metal laser sintering (dmls) of in625 parts. In Proceedings of the Twenty-Sixth Annual International Solid Freeform Fabrication (SFF) Symposium—An Additive Manufacturing Conference, Austin, TX, USA, 10–12 August 2015; pp. 560–574. [Google Scholar]
- Vayre, B.; Vignat, F.; Villeneuve, F. Identification on some design key parameters for additive manufacturing: Application on electron beam melting. Procedia CIRP 2013, 7, 264–269. [Google Scholar] [CrossRef]
- Drescher, P.; Reimann, T.; Seitz, H. Investigation of powder removal of net–structured titanium parts made from electron beam melting. Int. J. Rapid Manuf. 2014, 4, 81–89. [Google Scholar] [CrossRef]
- Barriobero-Vila, P.; Gussone, J.; Haubrich, J.; Sandlöbes, S.; Da Silva, J.C.; Cloetens, P.; Schell, N.; Requena, G. Inducing stable α + β microstructures during selective laser melting of ti-6al-4v using intensified intrinsic heat treatments. Materials 2017, 10, 268. [Google Scholar] [CrossRef] [PubMed]
- Barriobero-Vila, P.; Gussone, J.; Stark, A.; Schell, N.; Haubrich, J.; Requena, G. Peritectic titanium alloys for 3d printing. Nat. Commun. 2018, 9, 3426. [Google Scholar] [CrossRef] [PubMed]
- Vora, P.; Derguti, F.; Mumtaz, K.A.; Todd, I.; Hopkinson, N. Investigating a semi-solid processing technique using metal powder bed additive manufacturing processes. In Proceedings of the Solid Freeform Fabrication Symposium, Austin, TX, USA, 12 August 2013; pp. 454–462. [Google Scholar]
- Tounsi, R.; Vignat, F. New concept of support structures in electron beam melting manufacturing to reduce geomtric defects. In Proceedings of the 15 Colloque National AIP-Priméca, Montalbert, France, 12–14 April 2017; pp. 1–6. [Google Scholar]
- Ali, H.; Ghadbeigi, H.; Hosseinzadeh, F.; Oliveira, J.; Mumtaz, K. Effect of pre-emptive in situ parameter modification on residual stress distributions within selective laser-melted ti6al4v components. Int. J. Adv. Manuf. Technol. 2019, 1–13. [Google Scholar] [CrossRef]
- Kuo, Y.-H.; Cheng, C.-C.; Lin, Y.-S.; San, C.-H. Support structure design in additive manufacturing based on topology optimization. Struct. Multidiscip. Optim. 2018, 57, 183–195. [Google Scholar] [CrossRef]
- Ameen, W.; Al-Ahmari, A.; Mohammed, M.K.; Mian, S.H. Manufacturability of overhanging holes using electron beam melting. Metals 2018, 8, 397. [Google Scholar] [CrossRef]
- Weißmann, V.; Drescher, P.; Bader, R.; Seitz, H.; Hansmann, H.; Laufer, N. Comparison of single ti6al4v struts made using selective laser melting and electron beam melting subject to part orientation. Metals 2017, 7, 91. [Google Scholar] [CrossRef]
- Zhang, K.; Fu, G.; Zhang, P.; Ma, Z.; Mao, Z.; Zhang, D.Z. Study on the geometric design of supports for overhanging structures fabricated by selective laser melting. Materials 2018, 12, 27. [Google Scholar] [CrossRef]
- Oter, Z.C.; Coskun, M.; Akca, Y.; Surmen, O.; Yilmaz, M.S.; Ozer, G.; Tarakci, G.; Khan, H.M.; Koc, E. Support optimization for overhanging parts in direct metal laser sintering. Optik 2019, 181, 575–581. [Google Scholar] [CrossRef]
- Shi, W.; Wang, P.; Liu, Y.; Han, G. Experiment of process strategy of selective laser melting forming metal nonhorizontal overhanging structure. Metals 2019, 9, 385. [Google Scholar] [CrossRef]
- Zäh, M.F.; Lutzmann, S. Modelling and simulation of electron beam melting. Prod. Eng. 2010, 4, 15–23. [Google Scholar] [CrossRef]
- Gusarov, A.V.; Yadroitsev, I.; Bertrand, P.; Smurov, I. Heat transfer modelling and stability analysis of selective laser melting. Appl. Surf. Sci. 2007, 254, 975–979. [Google Scholar] [CrossRef]
- Shen, N.; Chou, K. Simulations of thermo-mechanical characteristics in electron beam additive manufacturing. ASME Int. Mech. Eng. Congr. Expo. 2012, 3, 67–74. [Google Scholar]
- Shen, N.; Chou, K. Numerical thermal analysis in electron beam additive manufacturing with preheating effects. In Proceedings of the Twenty-Third Annual International Solid Freeform Fabrication (SFF) Symposium—An Additive Manufacturing Conference, University of Texas in Austin, Austin, TX, USA, 6 August 2012; pp. 774–784. [Google Scholar]
- Cheng, B.; Lu, P.; Chou, K. Thermomechanical investigation of overhang fabrications in electron beam additive manufacturing. In Proceedings of the International Manufacturing Science and Engineering Conference, Detroit, MI, USA, 22–26 June 2014; ASME: Detroit, MI, USA, 2014; p. V002T002A024. [Google Scholar]
- Cheng, B.; Chou, K. Geometric consideration of support structures in part overhang fabrications by electron beam additive manufacturing. Comput.-Aided Des. 2015, 69, 102–111. [Google Scholar] [CrossRef]
- Cheng, B.; Chou, Y.K. Overhang support structure design for electron beam additive manufacturing. In Proceedings of the ASME 2017 12th International Manufacturing Science and Engineering Conference MSEC2017, Los Angeles, CA, USA, 4–8 June 2017; ASME: Los Angeles, CA, USA, 2017; p. V002T001A018. [Google Scholar]
- Han, Q.; Gu, H.; Soe, S.; Setchi, R.; Lacan, F.; Hill, J. Manufacturability of alsi10mg overhang structures fabricated by laser powder bed fusion. Mater. Des. 2018, 160, 1080–1095. [Google Scholar] [CrossRef]
- Cheng, L.; Liang, X.; Bai, J.; Chen, Q.; Lemon, J.; To, A. On utilizing topology optimization to design support structure to prevent residual stress induced build failure in laser powder bed metal additive manufacturing. Addit. Manuf. 2019, 27, 290–304. [Google Scholar] [CrossRef]
- Lu, X.; Lin, X.; Chiumenti, M.; Cervera, M.; Li, J.; Ma, L.; Wei, L.; Hu, Y.; Huang, W. Finite element analysis and experimental validation of the thermomechanical behavior in laser solid forming of ti-6al-4v. Addit. Manuf. 2018, 21, 30–40. [Google Scholar] [CrossRef]
- Ameen, W.; Al-Ahmari, A.; Abdulhameed, O. Design for metal additive manufacturing: An investigation of key design application on electron beam melting. Int. J. Mech. Aerosp. Ind. Mechatron. Manuf. Eng. 2019, 13, 264–269. [Google Scholar]
- Yang, J.; Sun, S.; Brandt, M.; Yan, W. Experimental investigation and 3d finite element prediction of the heat affected zone during laser assisted machining of ti6al4v alloy. J. Mater. Process. Technol. 2010, 210, 2215–2222. [Google Scholar] [CrossRef]
- Rai, R. Modeling of Heat Transfer and Fluid Flow in Keyhole Mode Welding. Ph.D. Thesis, Penn State University, Centen, PA, USA, 2008. [Google Scholar]
- Mills, K.C. Recommended Values of Thermophysical Properties for Selected Commercial Alloys; Woodhead Publishing: Sawston, UK, 2002. [Google Scholar]
- Cheng, B.; Price, S.; Lydon, J.; Cooper, K.; Chou, K. On process temperature in powder-bed electron beam additive manufacturing: Model development and validation. J. Manuf. Sci. Eng. 2014, 136, 061018. [Google Scholar] [CrossRef]
- Liu, C.; Wu, B.; Zhang, J.X. Numerical investigation of residual stress in thick titanium alloy plate joined with electron beam welding. Metall. Mater. Trans. B 2010, 41, 1129–1138. [Google Scholar] [CrossRef]
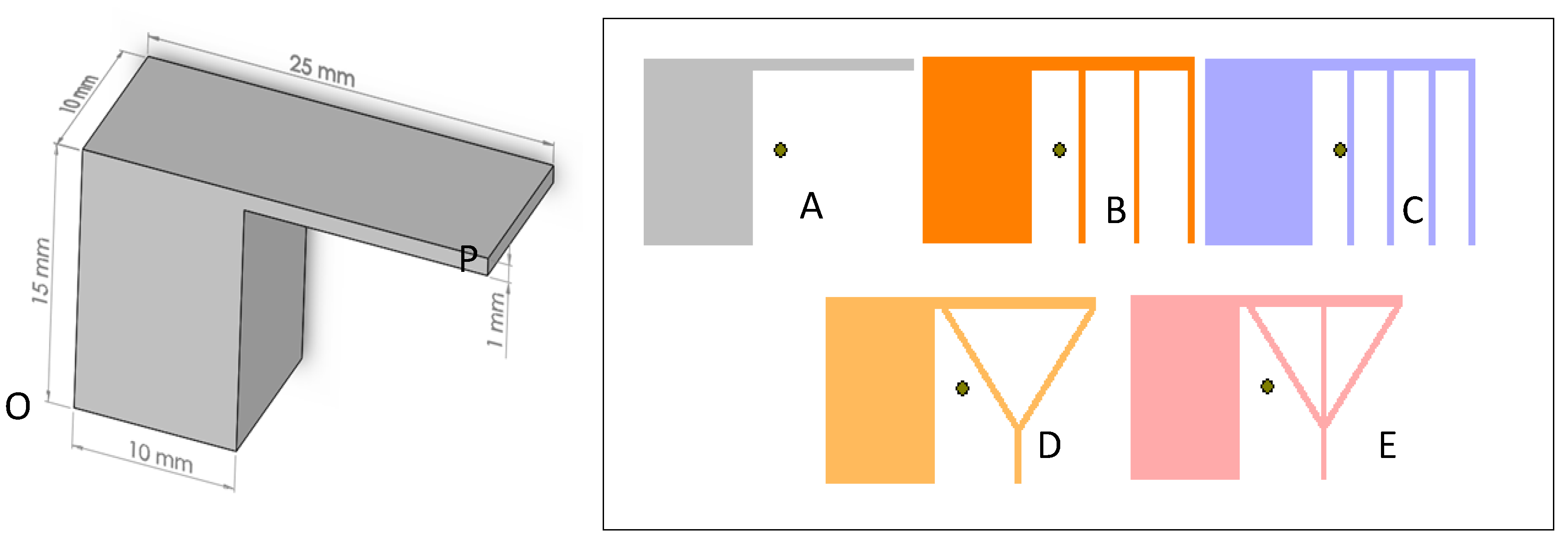
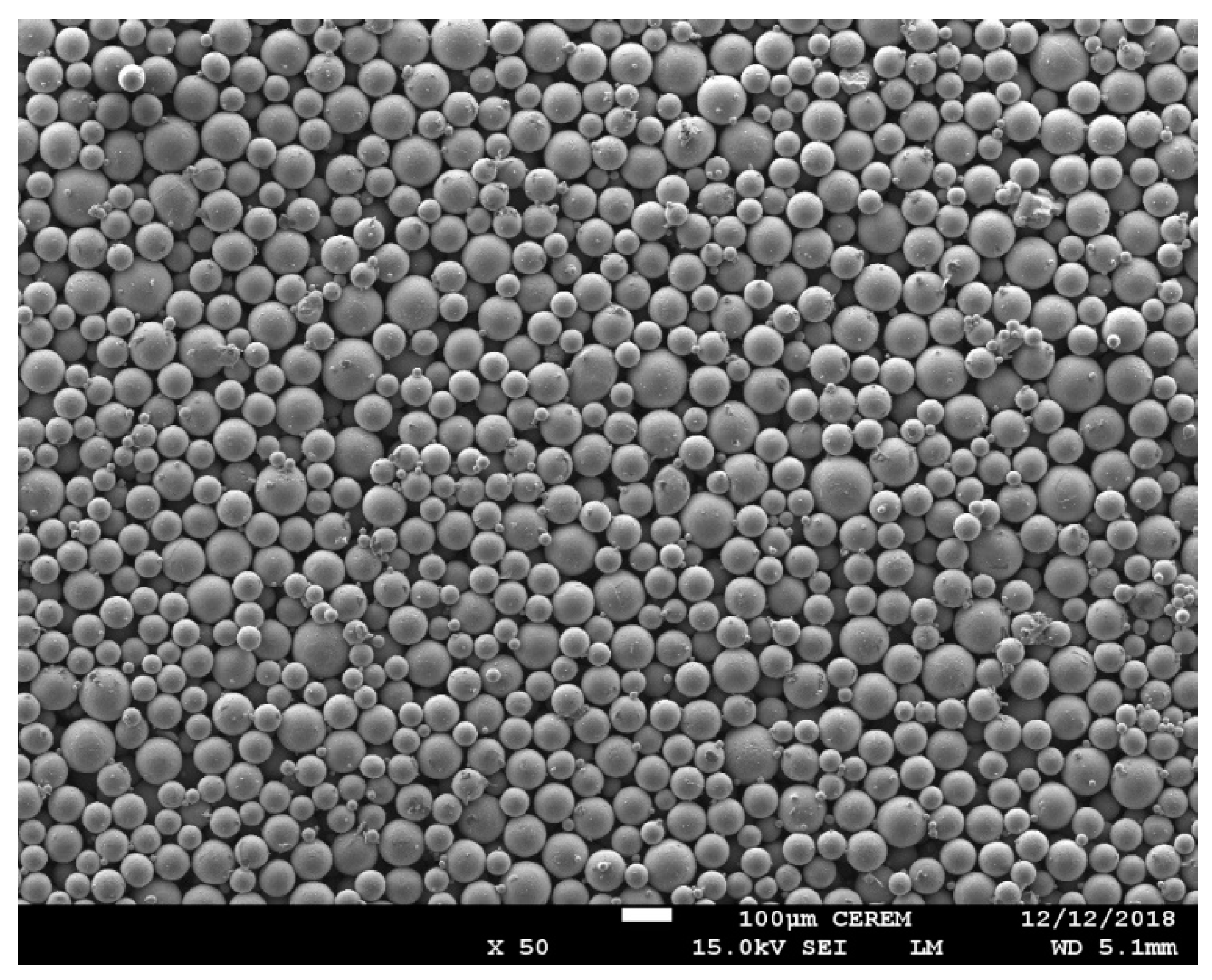
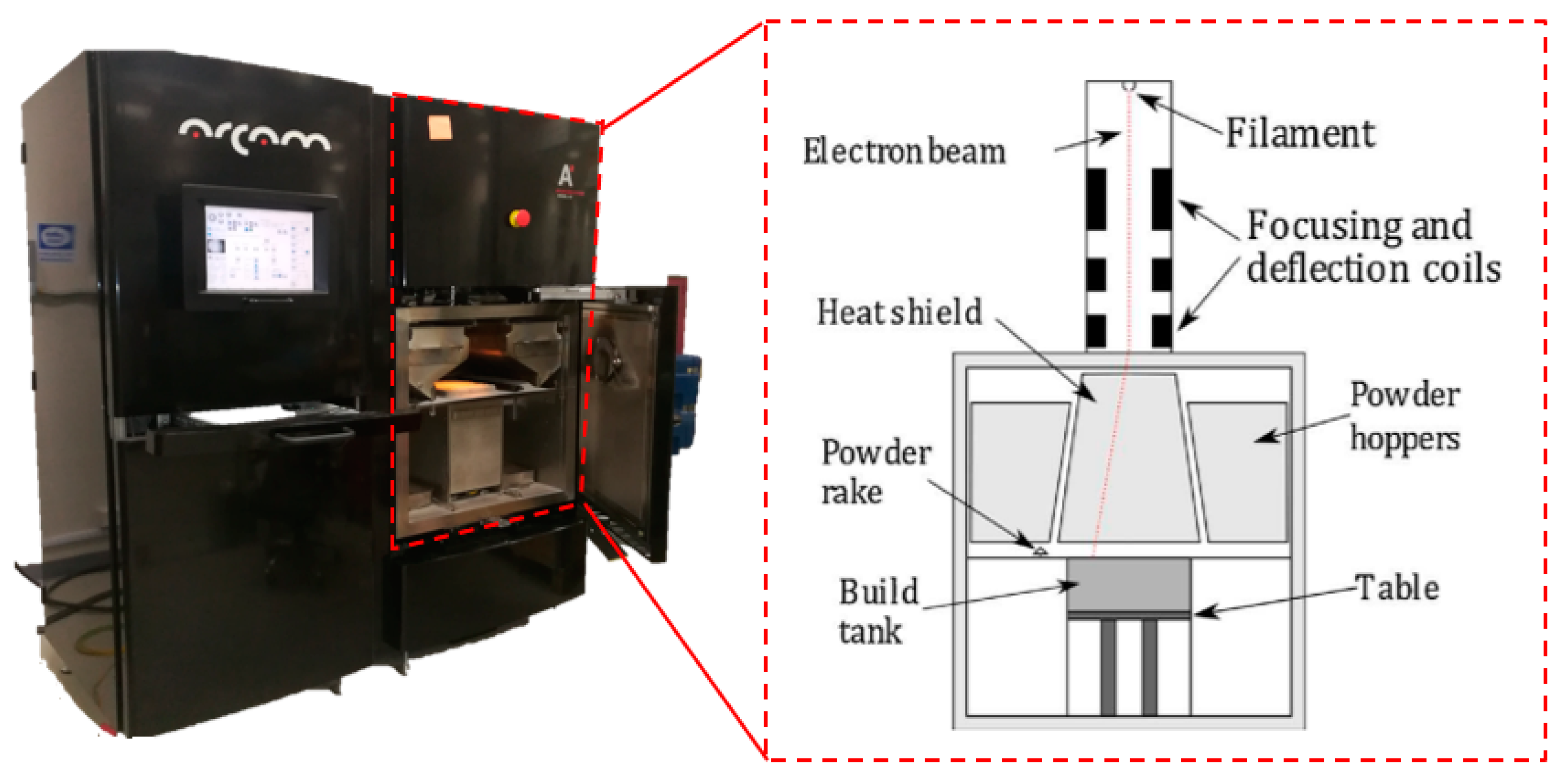
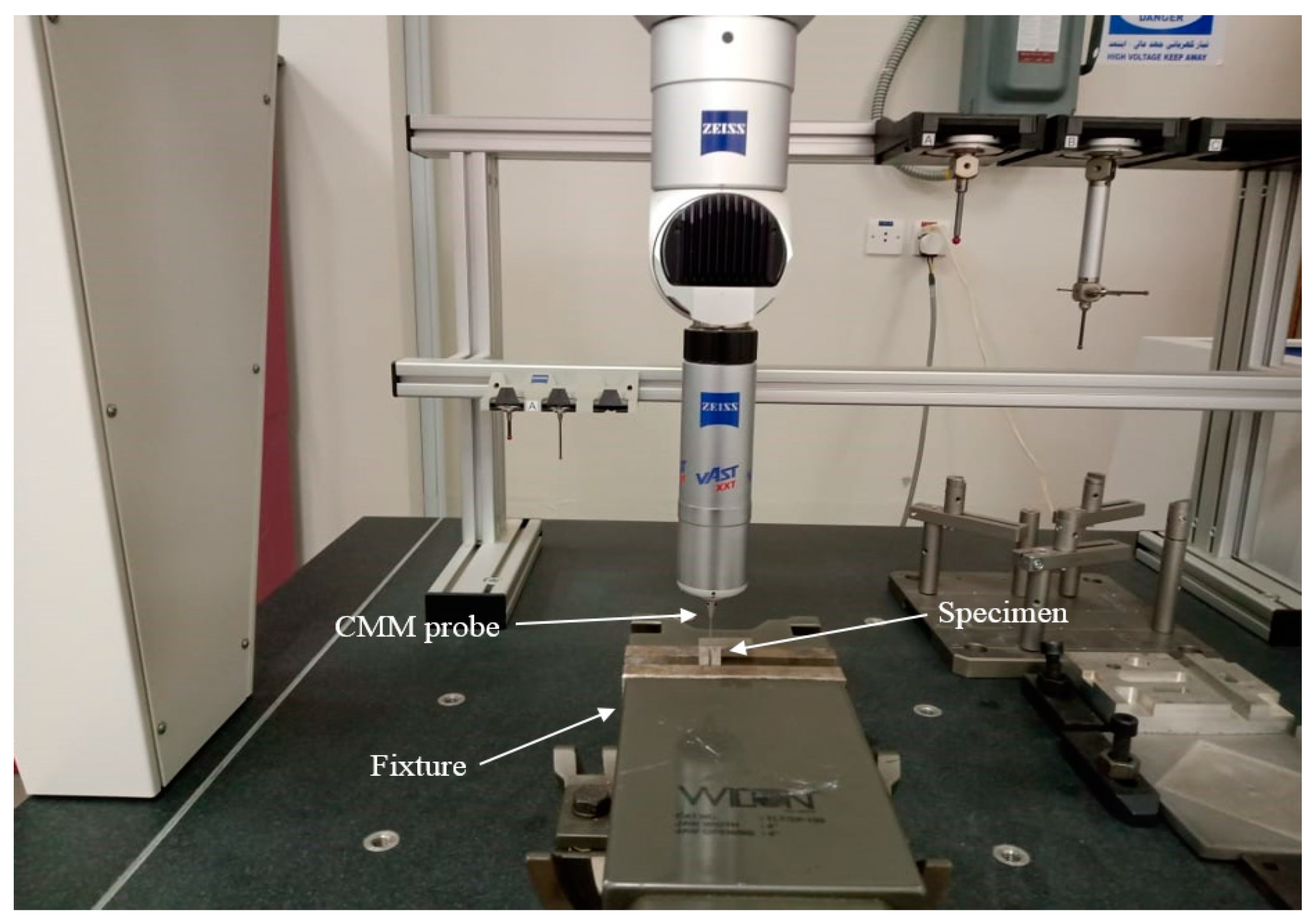



| Parameters | Values |
|---|---|
| Beam current, I (mA) | 15 |
| Scan speed, vs (mm/s) | 4530 |
| Line offset (mm) | 0.1 |
| Acceleration voltage, V (kV) | 60 |
| Electron beam diameter, d (µm) | 200 |
| Powder layer thickness (mm) | 0.05 |
| Beam penetration depth, D (mm) | 1.5 layer |
| Solidus temperature (K) | 1878 |
| Liquidus temperature (K) | 1928 |
| Preheat temperature, Tinitial (K) | 1003 |
| Temperature Range (°C) | Thermal Conductivity (W/m.K) | Specific Heat (J/Kg-k) | Density (Kg/m3) | Yield Stress (MPa) | Young′s Modulus (GPa) | Expansion Coefficient (10–6/C°) | |
|---|---|---|---|---|---|---|---|
| Solid | Powder | ||||||
| 0 | 6 | 0.5 | 547 | 4400 | 982 | 120 | 8.8 |
| 400 | 8.56 | 1.28 | 627 | 4349 | 694 | 112 | 9.8 |
| 800 | 10.91 | 1.76 | 718 | 4294 | 529 | 102 | 10.6 |
| 1200 | 12.85 | 2 | 680 | 4236 | 364 | 64 | 11.53 |
| 1600 | 15.38 | 2.36 | 748 | 4186 | 64 | 26 | 12.27 |
| 2000 | - | - | - | 4094 | - | - | - |
| 2400 | - | - | - | 4003 | - | - | - |
| 2800 | - | - | - | 3905 | - | - | - |
| 3200 | - | - | - | 3811 | - | - | - |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Umer, U.; Ameen, W.; Abidi, M.H.; Moiduddin, K.; Alkhalefah, H.; Alkahtani, M.; Al-Ahmari, A. Modeling the Effect of Different Support Structures in Electron Beam Melting of Titanium Alloy Using Finite Element Models. Metals 2019, 9, 806. https://doi.org/10.3390/met9070806
Umer U, Ameen W, Abidi MH, Moiduddin K, Alkhalefah H, Alkahtani M, Al-Ahmari A. Modeling the Effect of Different Support Structures in Electron Beam Melting of Titanium Alloy Using Finite Element Models. Metals. 2019; 9(7):806. https://doi.org/10.3390/met9070806
Chicago/Turabian StyleUmer, Usama, Wadea Ameen, Mustufa Haider Abidi, Khaja Moiduddin, Hisham Alkhalefah, Mohammed Alkahtani, and Abdulrahman Al-Ahmari. 2019. "Modeling the Effect of Different Support Structures in Electron Beam Melting of Titanium Alloy Using Finite Element Models" Metals 9, no. 7: 806. https://doi.org/10.3390/met9070806
APA StyleUmer, U., Ameen, W., Abidi, M. H., Moiduddin, K., Alkhalefah, H., Alkahtani, M., & Al-Ahmari, A. (2019). Modeling the Effect of Different Support Structures in Electron Beam Melting of Titanium Alloy Using Finite Element Models. Metals, 9(7), 806. https://doi.org/10.3390/met9070806







