Finite Fracture Mechanics Assessment in Moderate and Large Scale Yielding Regimes
Abstract
:1. Introduction
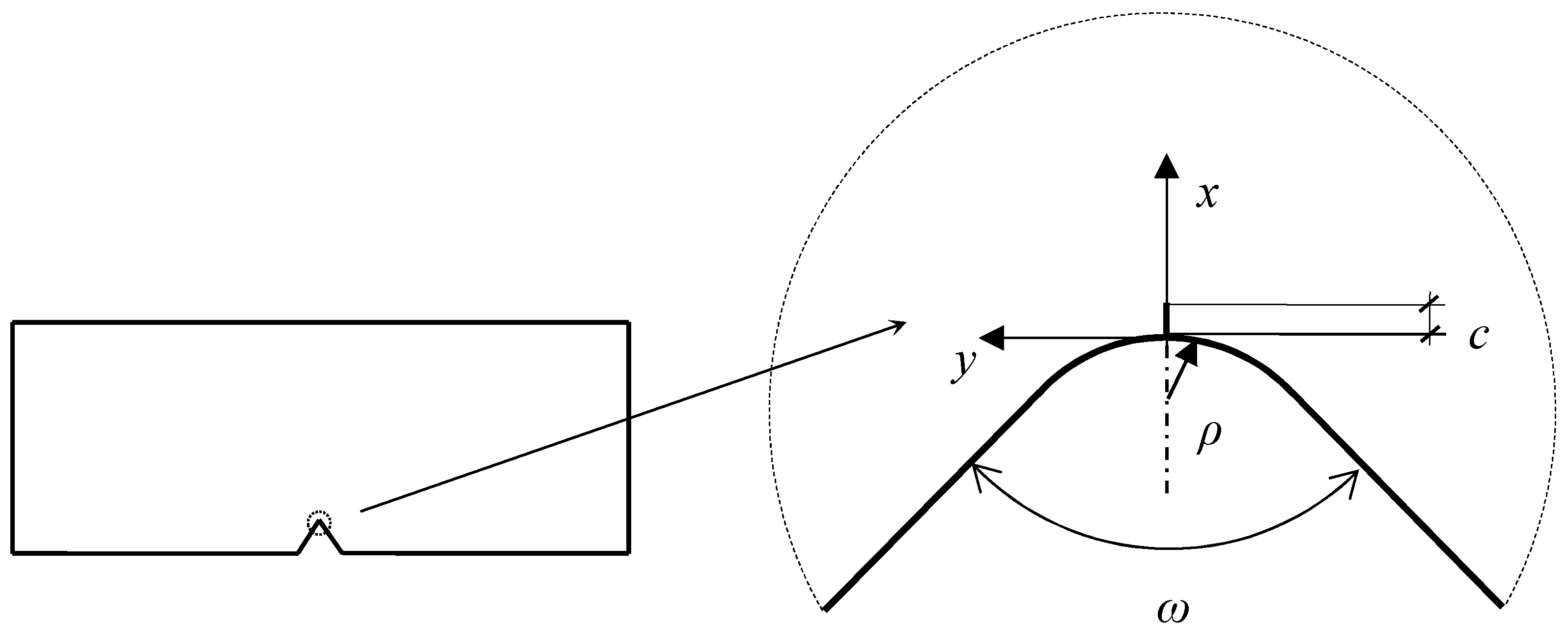
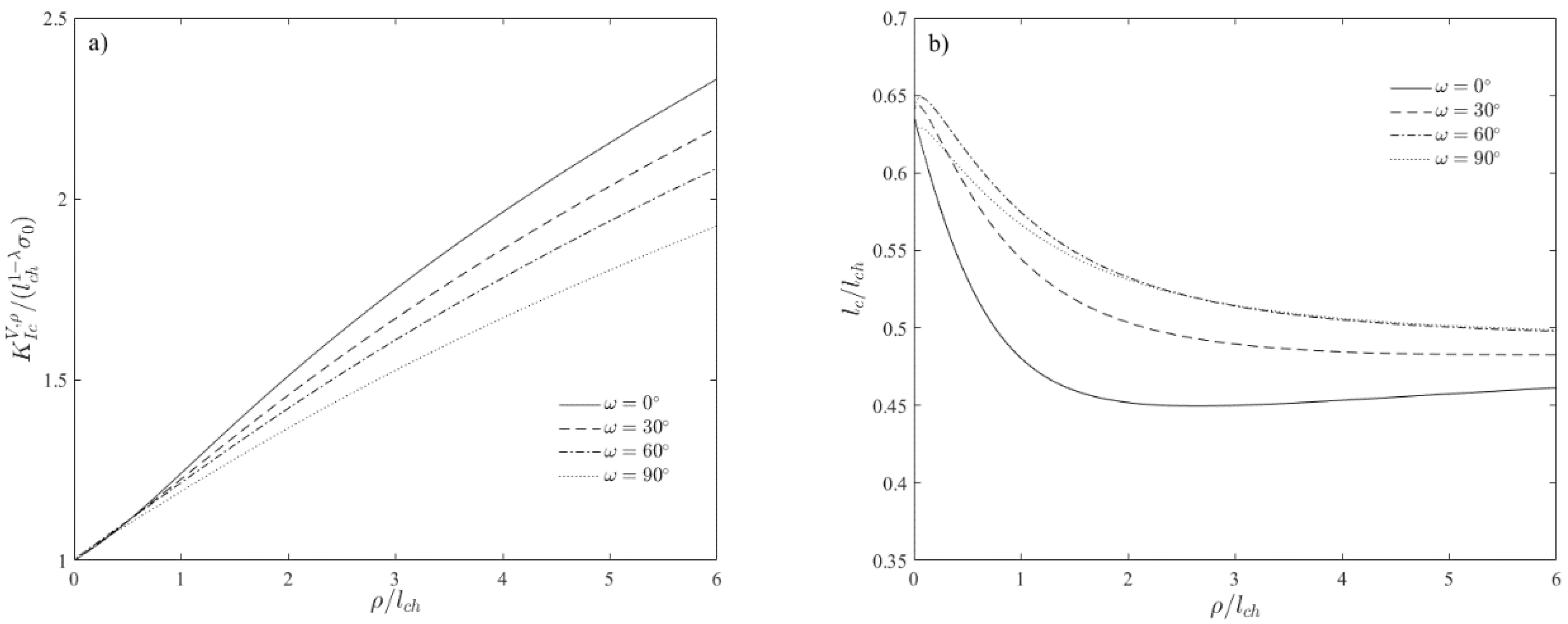
2. FFM Criteria
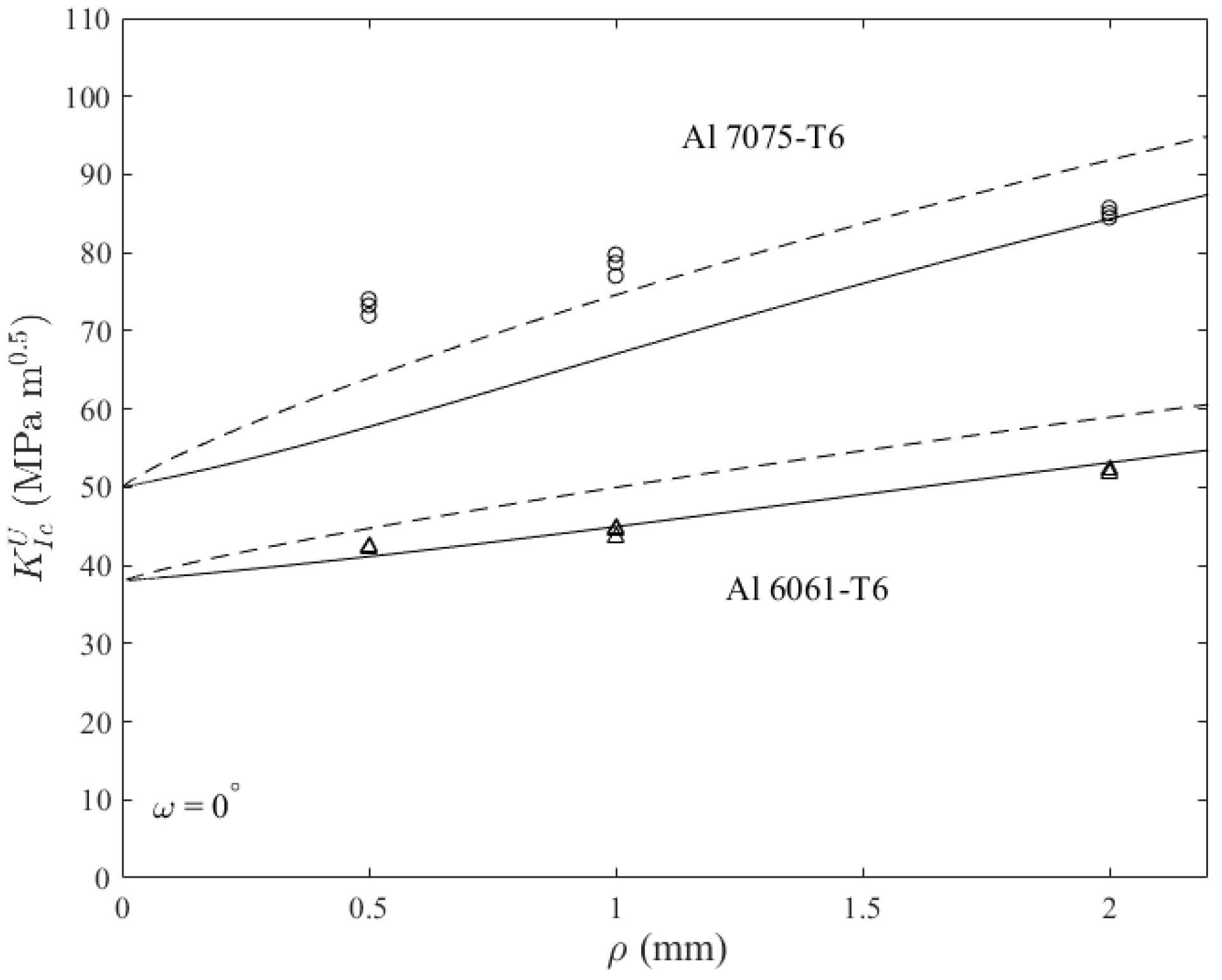
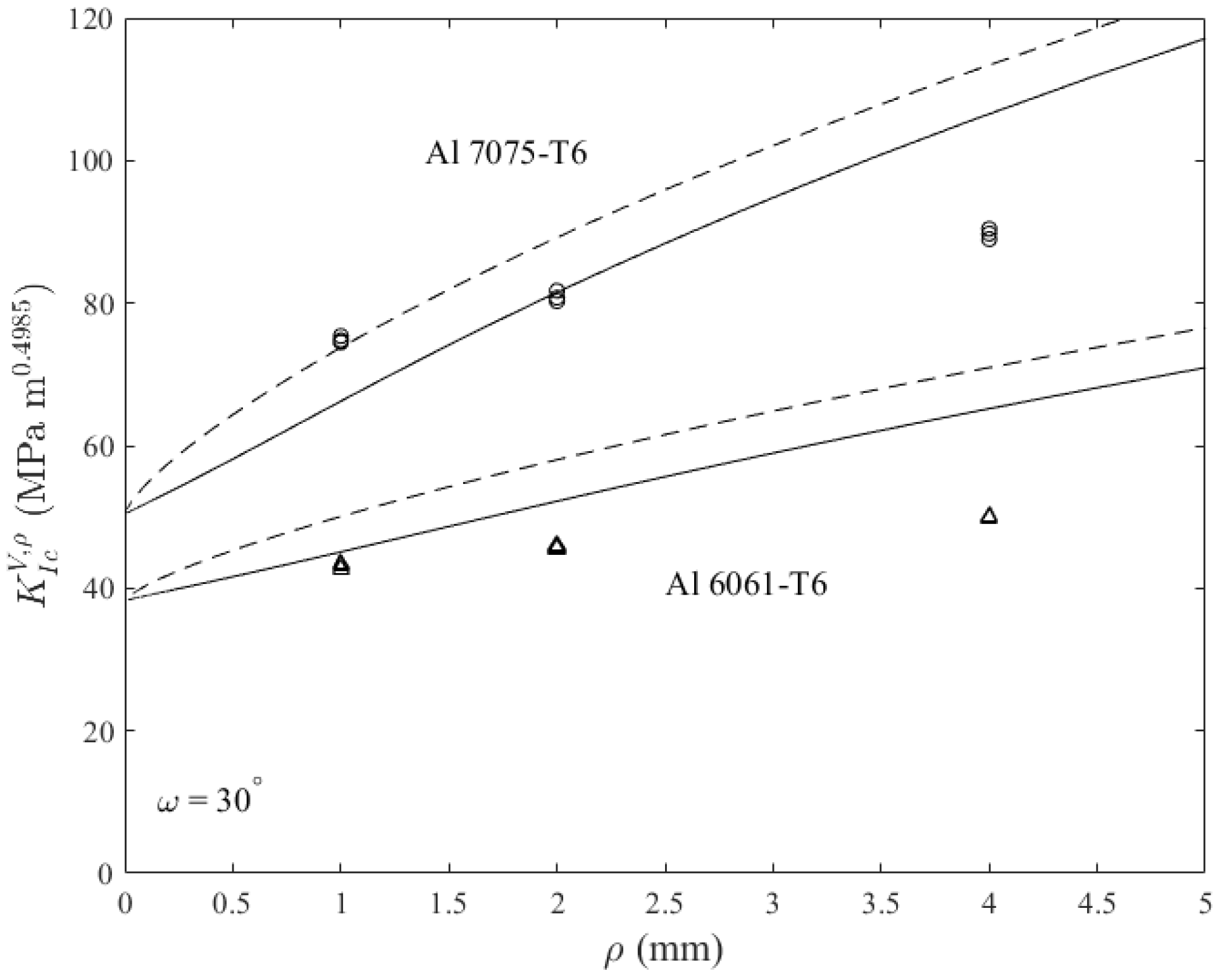
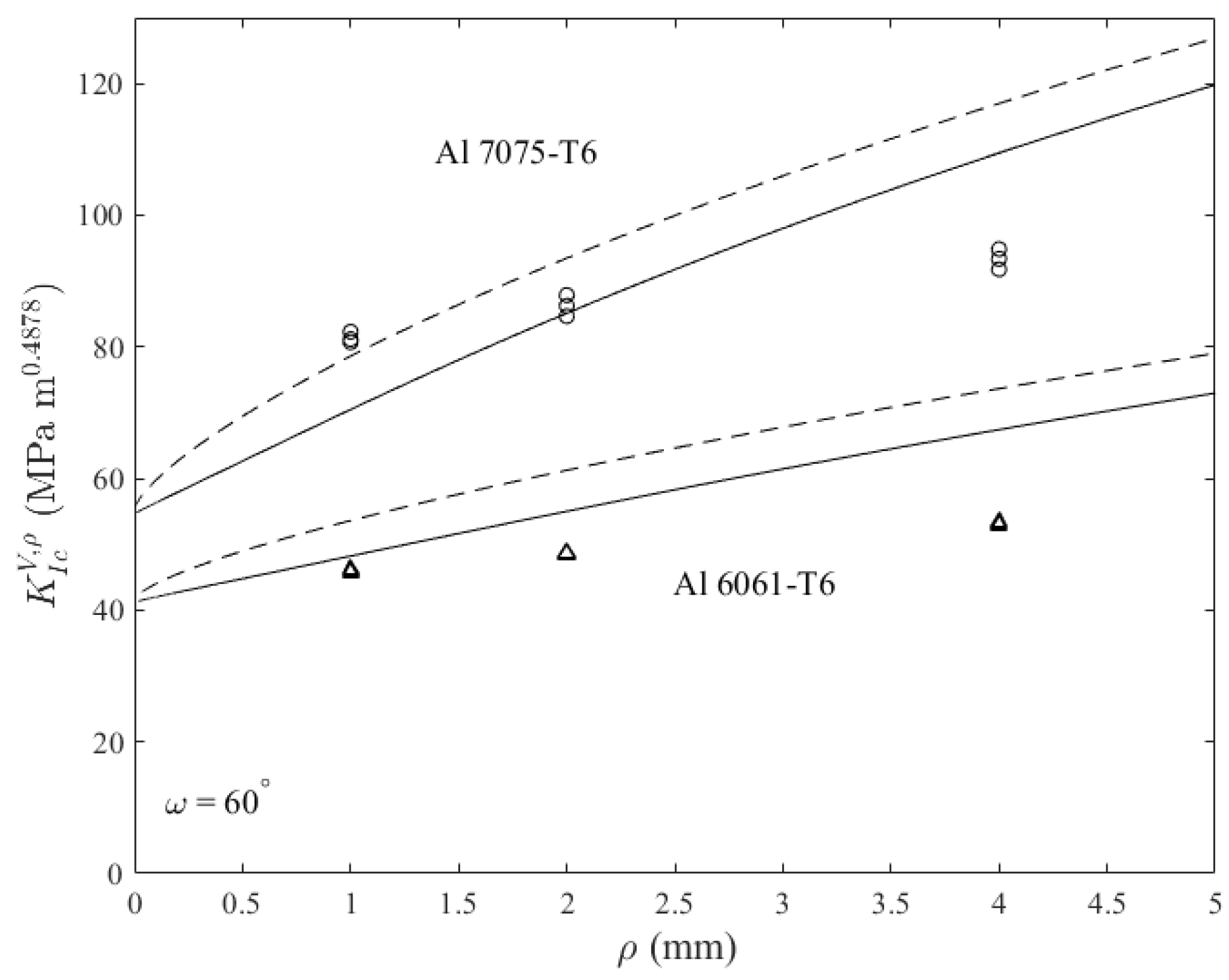
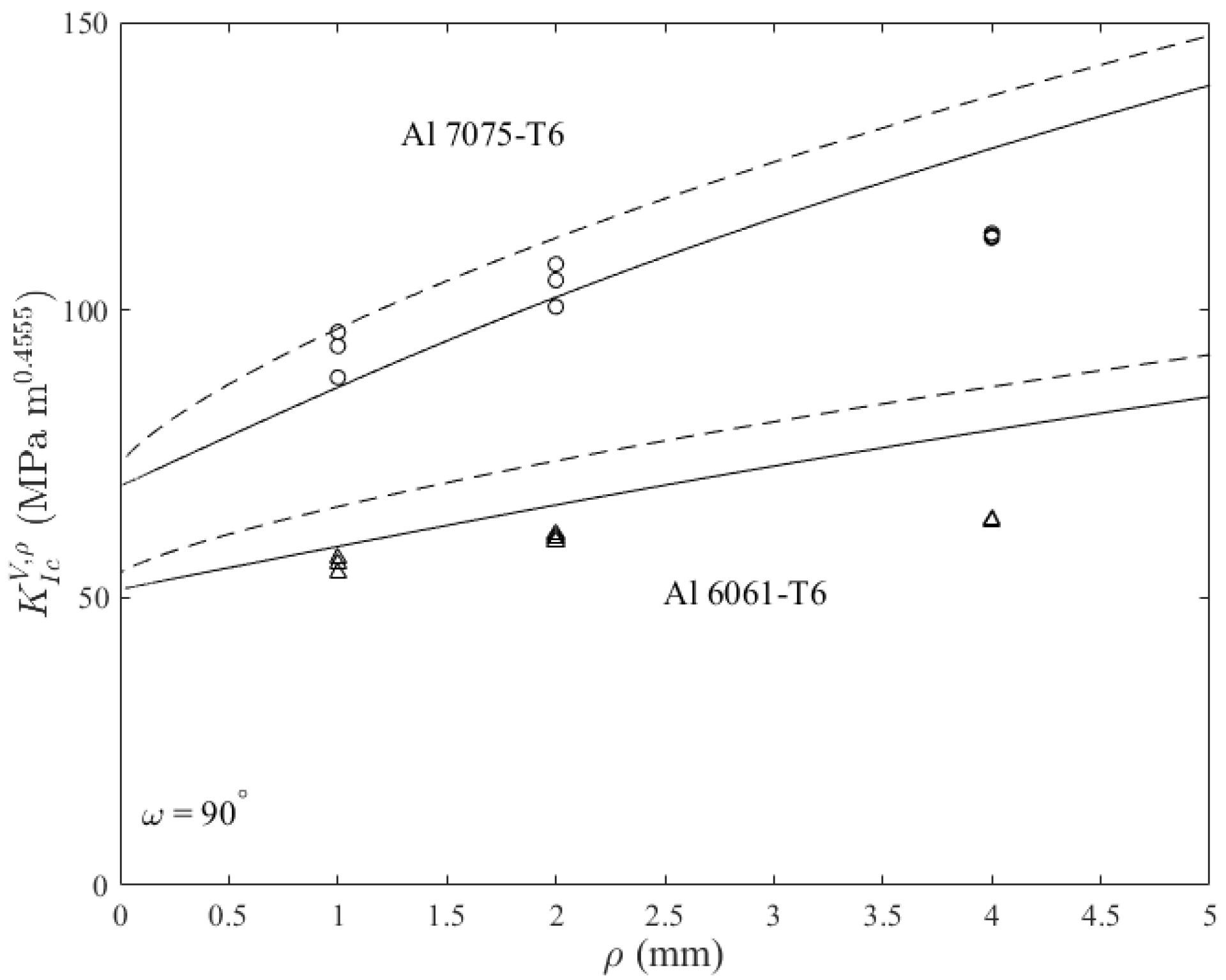
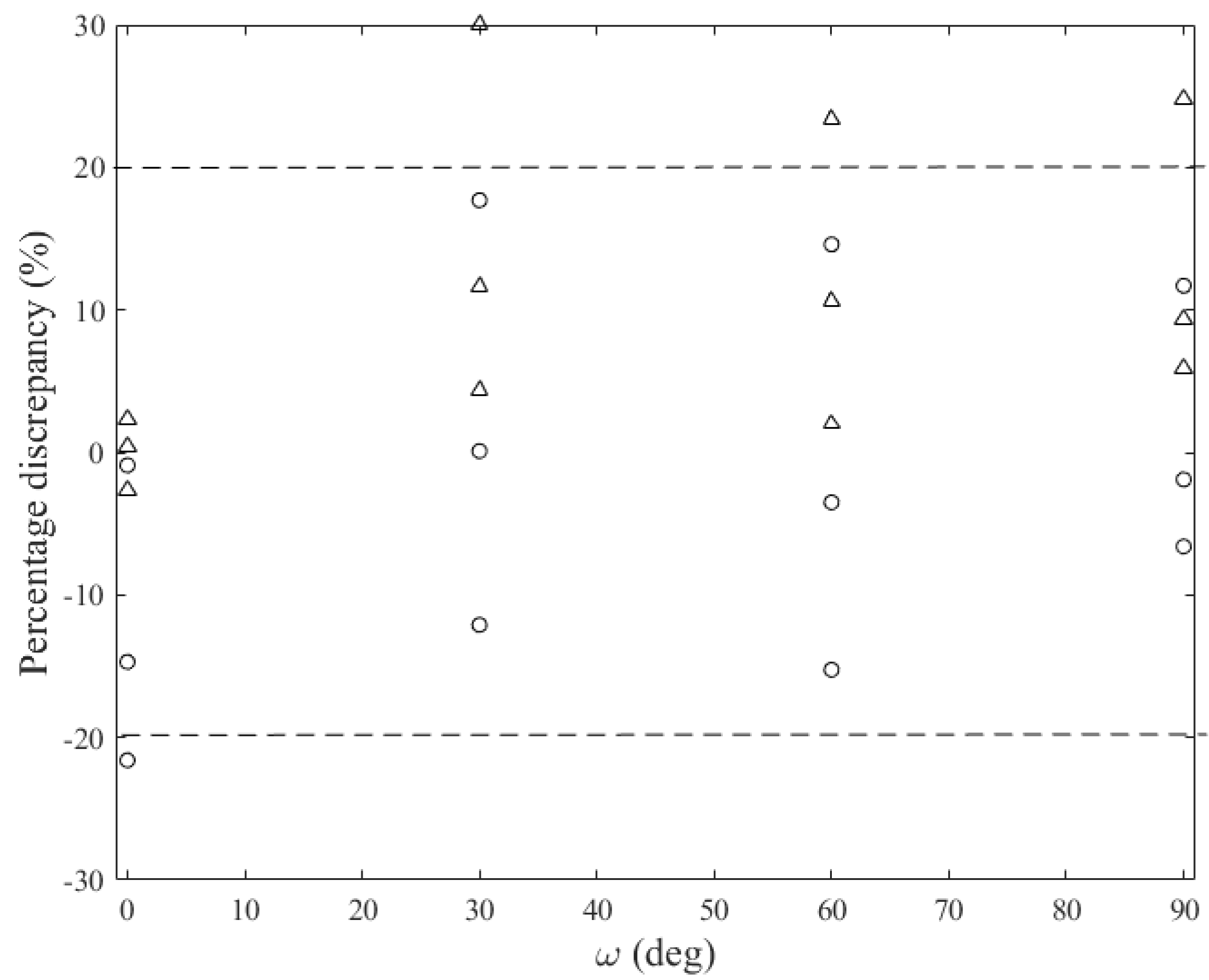
3. Comparison with Experimental Results
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Taylor, D. The Theory of Critical Distances: A New Perspective in Fracture Mechanics; Elsevier: Oxford, UK, 2006. [Google Scholar]
- Lazzarin, P.; Zambardi, R. A Finite-volume-energy based approach to predict the static and fatigue behaviour of components with sharp V-shaped notches. Int. J. Fract. 2002, 112, 275–298. [Google Scholar] [CrossRef]
- Seweryn, A.; Lukaszewicz, A. Verification of brittle fracture criteria for elements with V-shaped notches. Engng. Fract. Mech. 2002, 69, 1487–1510. [Google Scholar] [CrossRef]
- Seweryn, A. Brittle fracture criterion for structures with sharp notches. Eng. Fract. Mech. 1994, 47, 673–681. [Google Scholar] [CrossRef]
- Leguillon, D. Strength or toughness? A criterion for crack onset at a notch. Eur. J. Mech. A/Solids 2002, 21, 61–72. [Google Scholar] [CrossRef]
- Carpinteri, A.; Cornetti, P.; Pugno, N.; Sapora, A.; Taylor, D. Generalized fracture toughness for specimens with re-entrant corners: Experiments vs. theoretical predictions. Struct. Eng. Mech. 2009, 32, 609–620. [Google Scholar] [CrossRef]
- Sapora, A.; Firrao, D. Finite fracture mechanics predictions on the apparent fracture toughness of as-quenched Charpy V-type AISI 4340 steel specimens. Fatigue Fract. Eng. Mater. Struct. 2017, 40, 949–958. [Google Scholar] [CrossRef]
- Susmel, L.; Taylor, D. On the use of the theory of critical distances to predict static failures in ductile metallic materials containing different geometrical features. Eng. Fract. Mech. 2008, 75, 4410–4421. [Google Scholar] [CrossRef]
- Madrazo, V.; Cicero, S.; Carrascal, I.A. On the Point Method and the Line Method notch effect predictions in Al7075-T651. Eng. Fract. Mech. 2012, 79, 363–379. [Google Scholar] [CrossRef]
- Cicero, S.; Fuentes, J.D.; Procopio, I.; Madrazo, V.; González, P. Critical distance default values for structural steels and a simple formulation to estimate the apparent fracture toughness in U-notched conditions. Metals 2018, 8, 871. [Google Scholar] [CrossRef]
- Torabi, A.R.; Alaei, M. Mixed-mode ductile failure analysis of V-notched Al 7075-T6 thin sheets. Eng. Fract. Mech. 2015, 150, 70–95. [Google Scholar] [CrossRef]
- Torabi, A.R.; Habibi, R.; Hosseini, B.M. On the ability of the Equivalent Material Concept in predicting ductile failure of U-notches under moderate- and large-scale yielding conditions. Phys. Mesomech. 2015, 18, 337–347. [Google Scholar] [CrossRef]
- Torabi, A.R.; Alaei, M. Application of the equivalent material concept to ductile failure prediction of blunt V-notches encountering moderate-scale yielding. Int. J. Damage Mech. 2016, 25, 853–877. [Google Scholar] [CrossRef]
- Torabi, A.R.; Keshavarzian, M. Tensile crack initiation from a blunt V-notch border in ductile plates in the presence of large plasticity at the notch vicinity. Int. J. Terraspace Sci. Eng. 2016, 8, 93–101. [Google Scholar]
- Torabi, A.R. Estimation of tensile load-bearing capacity of ductile metallic materials weakened by a V-notch: The equivalent material concept. Mater. Sci. Eng. A 2012, 536, 249–255. [Google Scholar] [CrossRef]
- Torabi, A.R.; Berto, F.; Campagnolo, A. Elastic-plastic fracture analysis of notched Al 7075-T6 plates by means of the local energy combined with the equivalent material concept. Phys. Mesomech. 2016, 19, 204–214. [Google Scholar] [CrossRef]
- Fuentes, J.D.; Cicero, S.; Berto, F.; Torabi, A.R.; Madrazo, V.; Azizi, P. Estimation of Fracture Loads in AL7075-T651 Notched Specimens Using the Equivalent Material Concept Combined with the Strain Energy Density Criterion and with the Theory of Critical Distances. Metals 2018, 8, 87. [Google Scholar] [CrossRef]
- Muñoz-Reja, M.; Távara, L.; Mantič, V.; Cornetti, P. Crack onset and propagation at fibre–matrix elastic interfaces under biaxial loading using finite fracture mechanics. Compos. Part. A 2016, 82, 267–278. [Google Scholar] [CrossRef]
- Cornetti, P.; Sapora, A.; Carpinteri, A. Short cracks and V-notches: Finite Fracture Mechanics vs. Cohesive Crack Model. Eng. Fract. Mech. 2016, 168, 2–12. [Google Scholar] [CrossRef]
- Cornetti, P.; Muñoz-Reja, M.; Sapora, A.; Carpinteri, A. Finite fracture mechanics and cohesive crack model: Weight functions vs. cohesive laws. Int. J. Solid Struct. 2019, 156–157, 126–136. [Google Scholar] [CrossRef]
- Doitrand, A.; Estevez, R.; Leguillon, D. Comparison between cohesive zone and coupled criterion modeling of crack initiation in rhombus hole specimens under quasi-static compression. Theor. Appl. Fract. Mech. 2019, 99, 51–59. [Google Scholar] [CrossRef]
- Felger, J.; Stein, N.; Becker, W. Mixed-mode fracture in open-hole composite plates of finite-width: An asymptotic coupled stress and energy approach. Int. J. Solid. Struct. 2017, 122–123, 14–24. [Google Scholar] [CrossRef]
- Sapora, A.; Cornetti, P. Crack onset and propagation stability from a circular hole under biaxial loading. Int. J. Fract. 2019, 214, 97–104. [Google Scholar] [CrossRef]
- Doitrand, A.; Leguillon, D. 3D application of the coupled criterion to crack initiation prediction in epoxy/aluminum specimens under four point bending. Int. J. Solids Struct. 2018, 143, 175–182. [Google Scholar] [CrossRef] [Green Version]
- Stein, N.; Weißgraeber, P.; Becker, W. A model for brittle failure in adhesive lap joints of arbitrary joint configuration. Compos. Struct. 2015, 133, 707–718. [Google Scholar] [CrossRef]
- Felger, J.; Rosendahl, P.L.; Leguillon, D.; Becker, W. Predicting crack patterns at bi-material junctions: A coupled stress and energy approach. Int. J. Solids Struct. 2019, 164, 191–201. [Google Scholar] [CrossRef]
- Sapora, A.; Cornetti, P.; Carpinteri, A. Cracks at rounded V-notch tips: An analytical expression for the stress intensity factor. Int. J. Fract. 2014, 187, 285–291. [Google Scholar] [CrossRef]
- Carpinteri, A.; Cornetti, P.; Sapora, A. Brittle failures at rounded V-notches: A finite fracture mechanics approach. Int. J. Fract. 2011, 172, 1–8. [Google Scholar] [CrossRef]
- Filippi, S.; Lazzarin, P.; Tovo, R. Developments of some explicit formulas useful to describe elastic stress fields ahead of notches in plates. Int. J. Solid. Struct. 2002, 39, 4543–4565. [Google Scholar] [CrossRef]
| ω | λ | β | η | μ | m |
|---|---|---|---|---|---|
| 0° | 0.5000 | 1.000 | 1.000 | −0.5000 | 1.820 |
| 30° | 0.5015 | 1.005 | 1.034 | −0.4561 | 1.473 |
| 60° | 0.5122 | 1.017 | 0.9699 | −0.4057 | 1.338 |
| 90° | 0.5445 | 1.059 | 0.8101 | −0.3449 | 1.314 |
| Material | Al 7075-T6 | Al 6061-T6 |
|---|---|---|
| KIc (MPa √m) | 50 | 38 |
| σ0 (MPa) | 1845 | 1066 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Torabi, A.R.; Berto, F.; Sapora, A. Finite Fracture Mechanics Assessment in Moderate and Large Scale Yielding Regimes. Metals 2019, 9, 602. https://doi.org/10.3390/met9050602
Torabi AR, Berto F, Sapora A. Finite Fracture Mechanics Assessment in Moderate and Large Scale Yielding Regimes. Metals. 2019; 9(5):602. https://doi.org/10.3390/met9050602
Chicago/Turabian StyleTorabi, Ali Reza, Filippo Berto, and Alberto Sapora. 2019. "Finite Fracture Mechanics Assessment in Moderate and Large Scale Yielding Regimes" Metals 9, no. 5: 602. https://doi.org/10.3390/met9050602
APA StyleTorabi, A. R., Berto, F., & Sapora, A. (2019). Finite Fracture Mechanics Assessment in Moderate and Large Scale Yielding Regimes. Metals, 9(5), 602. https://doi.org/10.3390/met9050602







