3.2. Arrhenius Model
In the Arrhenius model shown in Equation (1), for a certain temperature, the values of
A,
Q,
R, and
T are constants. In order to evaluate the material parameters individually, sequential linear regressions are used to solve the parameters. The values of
n’ and
α can be determined by the following formulas:
where
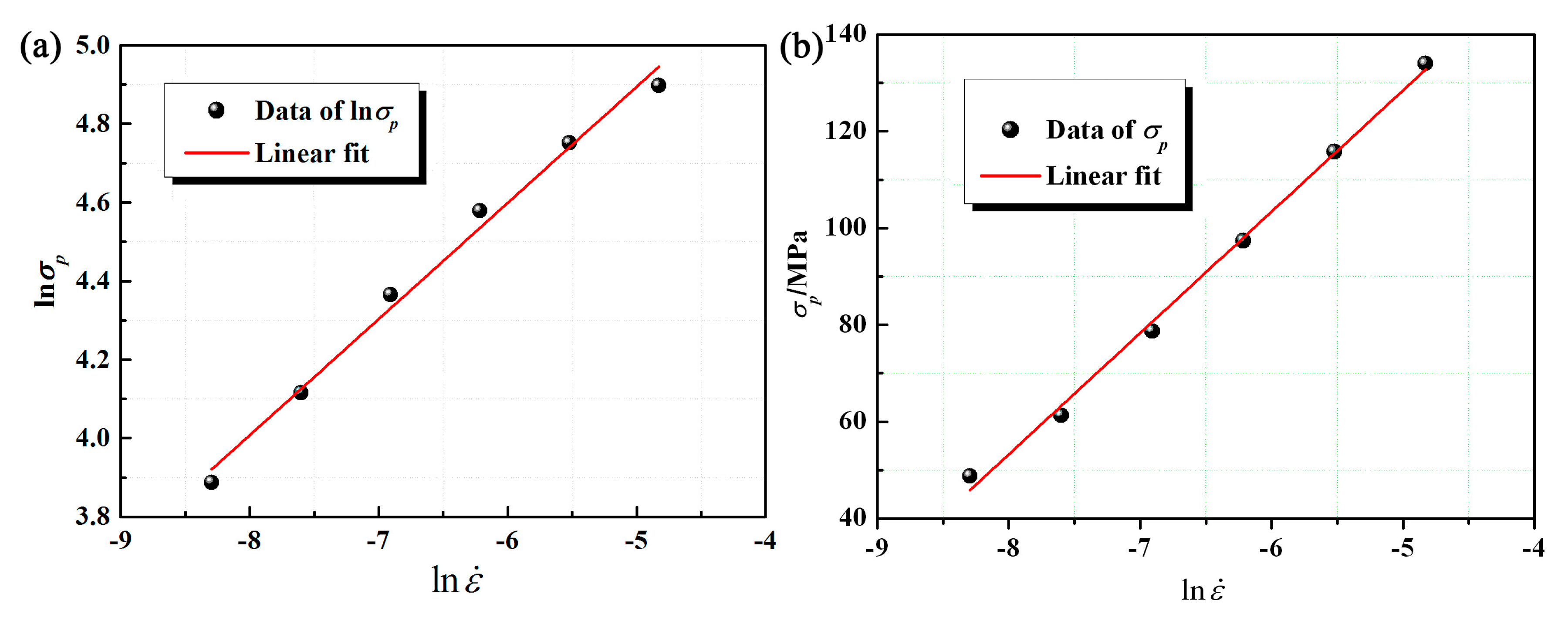
σ is the peak value
σp, the values of
n’ and
n’α are obtained by linear fitting between
and
, as shown in
Figure 2. The fitted values of
n(1/
m) and
α were equal to 3.383 and 0.0118, respectively. The values of
ασ varied from 0.58 to 1.58 with strain rates varying from 2.5 × 10
−4 s
−1 to 8 × 10
−3 s
−1 at 970 °C, indicating a moderate stress level. Therefore, it is reasonable to choose the third formula of Equation (3) as the constitutive model.
When the temperature changes, the values of
A,
α,
R, and
n’ are constants while the value of
Q varies with the temperature. The values of
Q and
A are calculated as follows:
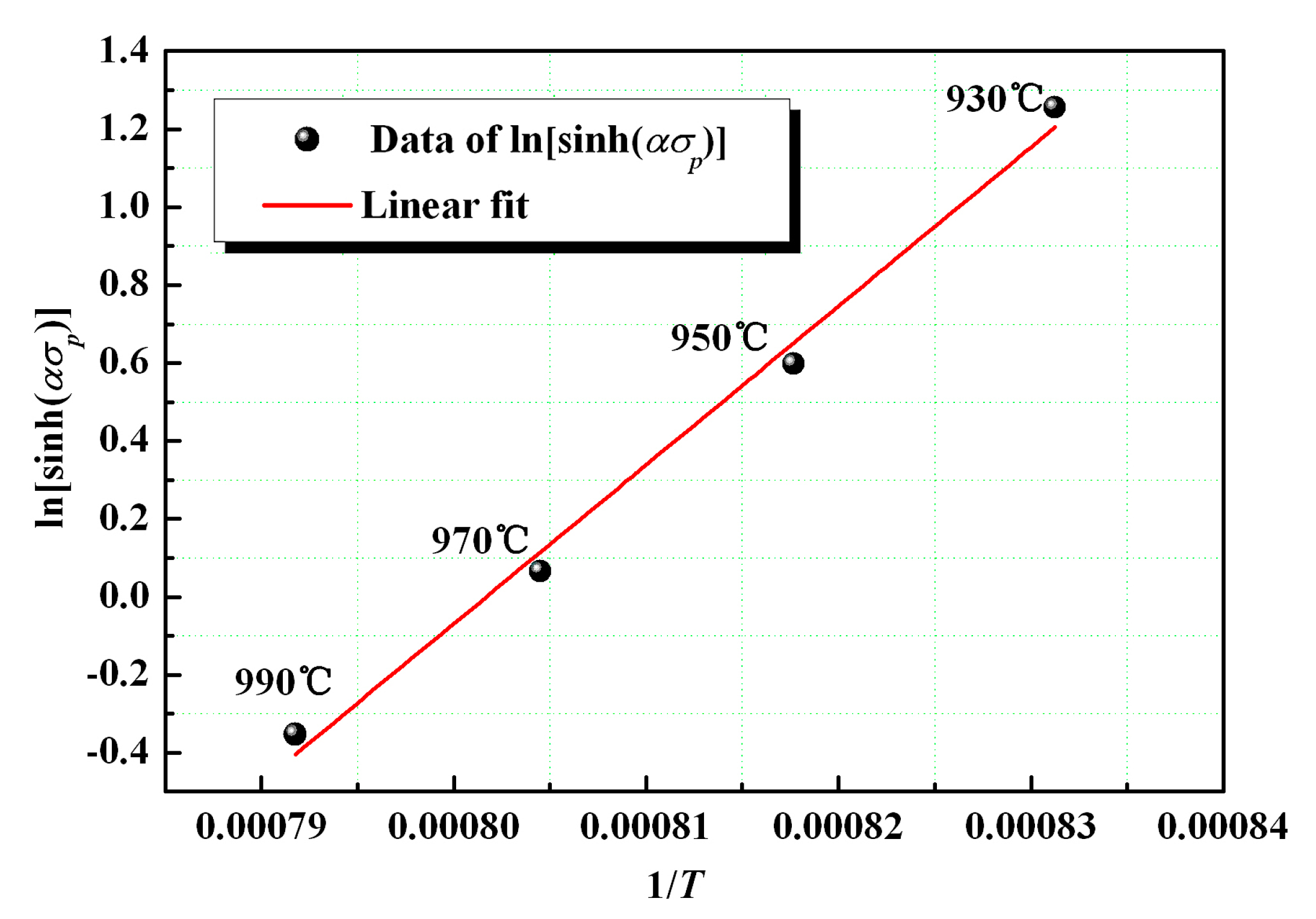
Substituting
α and
n’ values into Equation (12), the ln[sinh(ασ
p)] versus 1/
T scatter can be plotted under a deformation strain rate of
, as shown in
Figure 3.
By the linear fitting, the value of
is equal to the slope of the plot and the values of
Q (1146 kJ·mol
−1) and
A (1.175 × 10
45) are finally obtained. Hence, the Arrhenius constitutive equation of the Ti-22Al-25Nb alloy sheet can be described as:
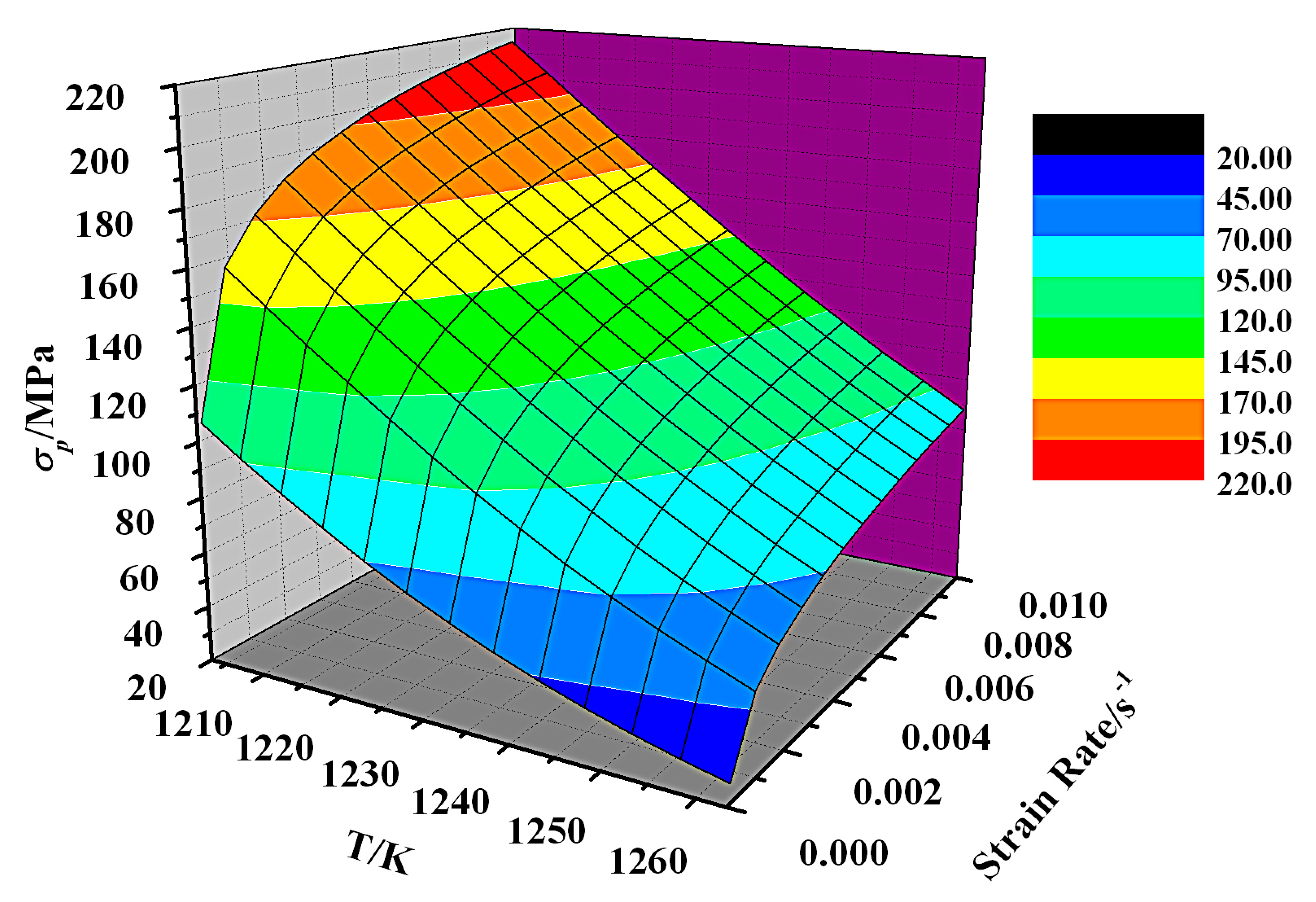
Since
σ is a binary function of
and
T with a curved surface graphically, as indicated in
Figure 4, the peak stress of
σp can be obtained from any point (
,
T).
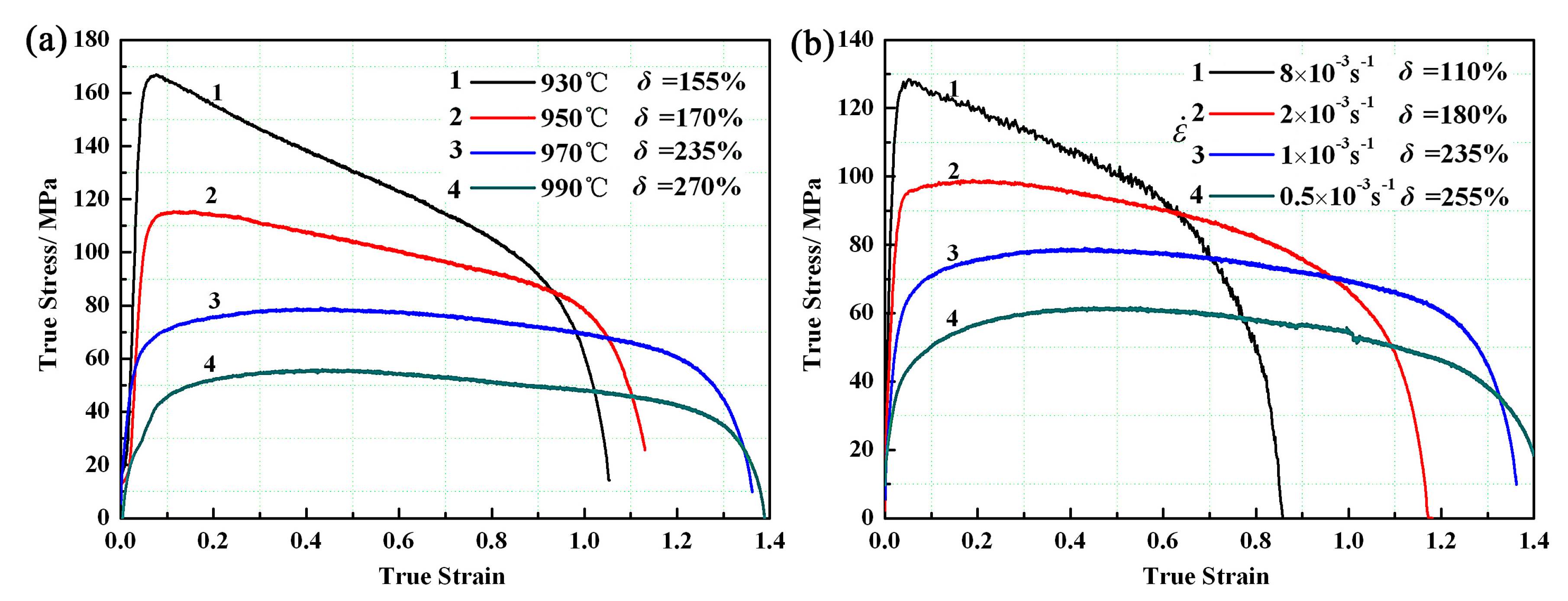
As shown in
Figure 1, the flow stress first achieved the peak and then decreased immediately, indicating that the alloy undergoes softening rather than hardening with increasing strain. However, when the deformation temperature is too high or the strain rate is too low, such as at
T ≥ 970 °C and
, the strain softening effect is no more prominent and the flow stress can be maintained at a constant level for a larger strain, even exhibiting a superplasticity. In this case, the deformation behavior can be described by the Backofen equation.
Equation (15) can be obtained by taking natural logarithms on both sides of the Backofen model in Equation (4):
The strain rate sensitivity index
m is the slope of
curves. Under the deformation condition of
T = 970 °C and
,
K and
m were equal to 585.81 and 0.2956, respectively. Then, the Backofen constitutive equation was indicated as below:
With the further simultaneous consideration of strain, strain rate, and temperature effect on the flow stress, Equation (6) can be used. Taking natural logarithm on both sides, it can be described as follows:
The value of
is the intercept of
curves (
Figure 2a) and the determined value of
K is 4.03 × 10
−46. Then, the Norton–Hoff constitutive equation with temperature variables is shown as below:
For materials with both strain and strain-rate hardening, the deformation can be described using the Norton–Hoff equation (Equation (6)). As shown in
Figure 1, the flow stress of Ti-22Al-25Nb alloy increased at the beginning and then decreased with straining, exhibiting both the hardening and softening effect. Since the strain softening is not considered in the Norton–Hoff equation, the model cannot be directly used for this alloy. In consideration of strain and thermal sensitivity based on Hensel–Spittel and Hirt laws [
17], the modified Norton–Hoff equation is obtained by introducing a softening factor
, as given in Equation (19):
where
b is the temperature-softening coefficient and
s is the strain-softening coefficient.
As known from the previous analysis, the hardening index n of the alloy at room temperature is equal to 0.11 and the strain-rate sensitivity index m is equal to 0.2956 at a temperature of 970 °C and strain rates ranging from 2.5 × 10−4 s−1 to 8 × 10−3 s−1.The parameters b and s are calculated in the following.
Taking natural logarithms on both sides of Equation (19):
Assuming the strain and strain rate are constants, the value of
is also a constant. The value of
b is the slope of the
curve shown in
Figure 5, which is equal to −0.0184.
Assuming the temperature and strain rate are constants, the value of
is a constant. For a homogeneous deformation at a certain temperature and strain rate, Equations (21) and (22) can be obtained if
,
and
,
:
Subtracting Equation (22) from Equation (21), the value of
s can be calculated as follows:
From the stress–strain curves, if
,
MPa and
,
MPa, the value of
s obtained from Equation (23) is equal to −0.3861. When
T = 970 °C and
, the peak stress is 78.7 MPa at a strain of 0.4. Substituting the peak stress
into Equation (19), the constant
K is obtained as 6.71 × 10
12. Finally, the modified Norton–Hoff equation considering the softening factor
is obtained as follows:
3.3. Johnson–Cook Model
For the Johnson–Cook model in Equation (7) and the definition of the relative temperature in Equation (9), the model can be used from the room temperature to the melting temperature. However, the material parameters A, α, n, and m are all tested at room temperature. For the deformation at elevated temperature, on one hand, dynamic recovery or recrystallization may occur, resulting in the reduced or even disappeared strain-hardening effect. Therefore, it is unreasonable to use the parameters A, B, and n tested at room temperature. On the other hand, for most metals, the strain-rate sensitivity varies with temperature or strain rate at elevated temperatures. Therefore, the strain-rate sensitivity exponent m tested at room temperature is not accurate to study the elevated temperature deformation.
For the Ti-22Al-25Nb alloy in the present study, strong strain softening occurs within the temperature range of 930~990 °C, and the strain-rate sensitivity is greatly dependent on the deformation temperature and strain rate. Therefore, it is not reasonable to use the strict format of the model to describe the elevated temperature deformation. In fact, the initial Johnson–Cook model essentially takes the yield stress at room temperature and a certain strain rate as the referential flow stress and represents the effects of the strain, strain rate, and temperature increment on the referential flow stress (yield stress). Therefore, for elevated temperature deformation, when taking the flow stress in the specified condition range as the referential flow stress, it is feasible to study the flow behavior in the specified condition, such as at a certain temperature and a certain strain rate.
Equation (7) can be transformed:
When the strain rate takes the referential one,
= 1, the flow stress at room temperature
and the flow stress at melting point
σm = 0. The relations between the flow stress and temperature can be understood as:
or
It is obvious that the relative increment of stress varies with temperature in power function. When the strain rate varies, the Johnson–Cook model can be described as:
where the reference temperature is the room temperature
Tr and the temperature range is [
Tr,
Tm]. In view of the format of Equation (28), if the temperature range is randomly selected as [
Tl,
Th], where
Tr represents the referential temperature, Equation (28) can be generalized as:
where
and
are the flow stress at
Tl and
Th, respectively. In this case, the applicable temperature range of the constitutive model (Equation (29)) can be randomly selected. This model format is an improvement on the initial J–C model, whose validity will be verified through the following experimental results.
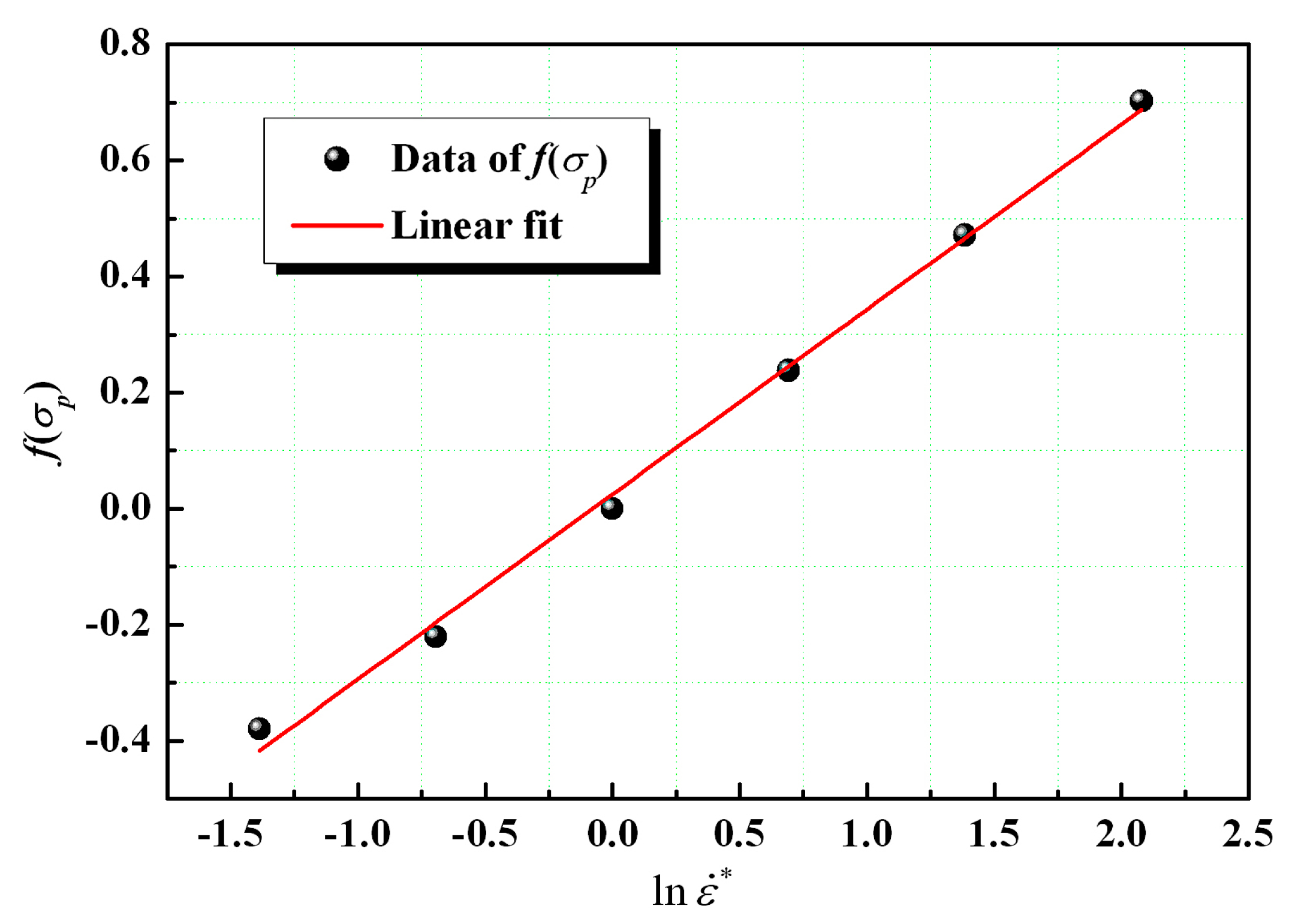
Here, taking the temperature
T = 930 °C (1203 K) as the referential temperature and the strain rate
as the referential strain rate, the J–C model of the Ti-22Al-25Nb alloy under the condition of
T = 930~990 °C and
is established as below:
Taking natural logarithms on both sides of Equation (26) as below:
Obviously, the value of m is the slope of Equation (31).
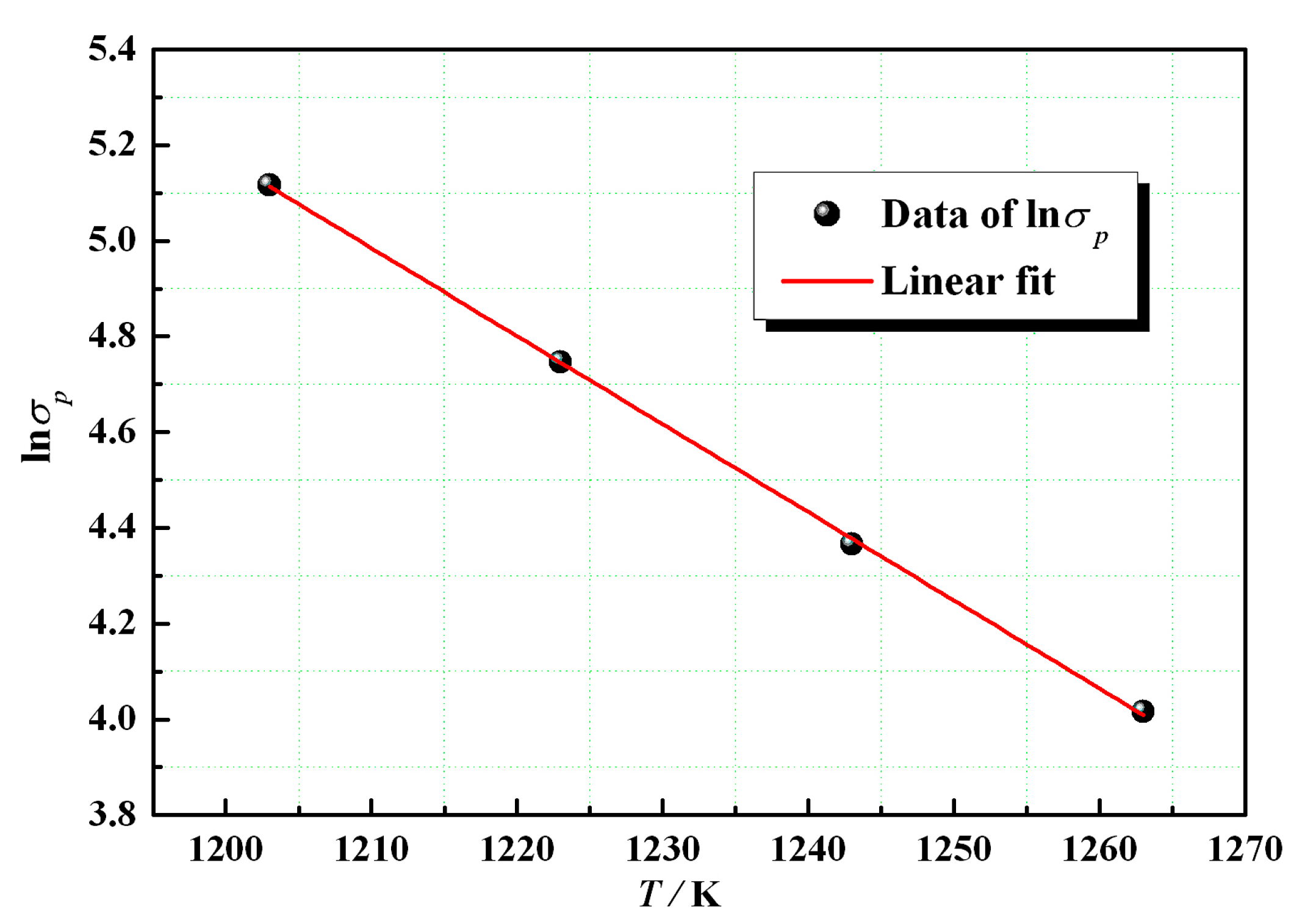
The stress–strain curves at
show that the peak stresses
σp1203 is 166.8 MPa at 930 °C (1203 K) and
σp1263 is 55.5 MPa at 990 °C (1263 K). Then, Equation (31) can be written as:
Taking the flow stress at various temperatures into Equation (32), Equation (33) is obtained. The
m value is the slope of
curve, as shown in
Figure 6. The value of
m is equal to 0.7537 through the linear fitting.
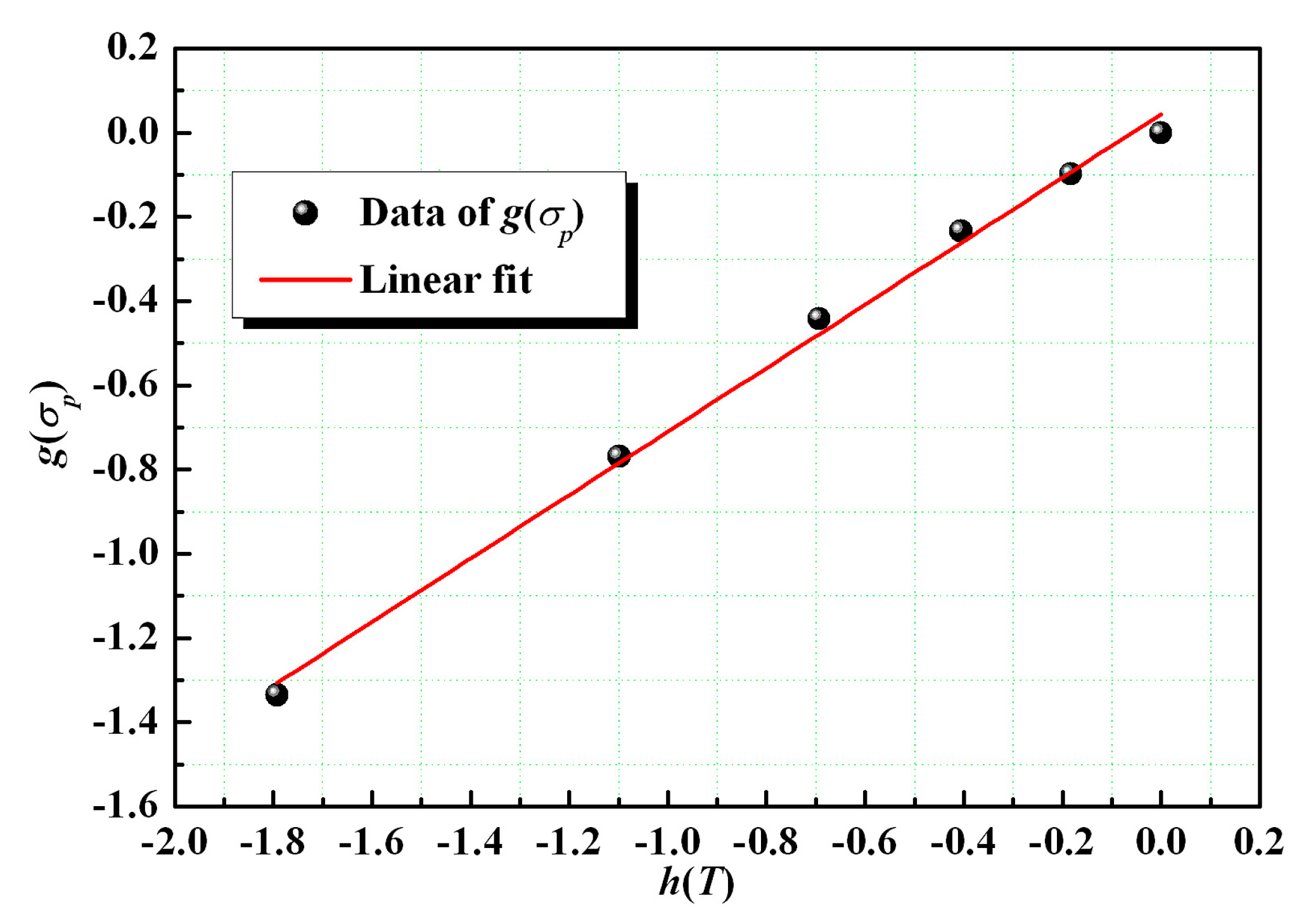
From the stress–strain curves in
Figure 1, the peak stress
σp is 166.8 MPa at
T = 930 °C (1203 K) and
. By taking the peak stress
σp into Equation (29), Equation (34) can be obtained as below:
The value of
C is the slope of
curve (
Figure 7) and equal to 0.3186 by the linear fitting.
Since the referential temperature is 930 °C (1203 K),
MPa and
MPa in Equation (29) and let
Th = 990 °C (1263 K) replace the melting temperature
Tm. Then, taking
C and
m values into Equation (29), the modified J–C model (Equation (35)) is obtained at temperature range
T = 930~990 °C and strain-rate range
with the referential temperature of 930 °C and the referential strain rate of
.
where
,
,
. The first bracket represents the softening effect of temperature on the flow stress and the second bracket represents the hardening effect of strain rate on the flow stress. The softening effect of strain on the flow stress can be characterized by introducing a softening factor, which will be introduced later.
If taking the material parameters into the original format of the J–C model in Equation (7), the constitutive equation can be rewritten as:
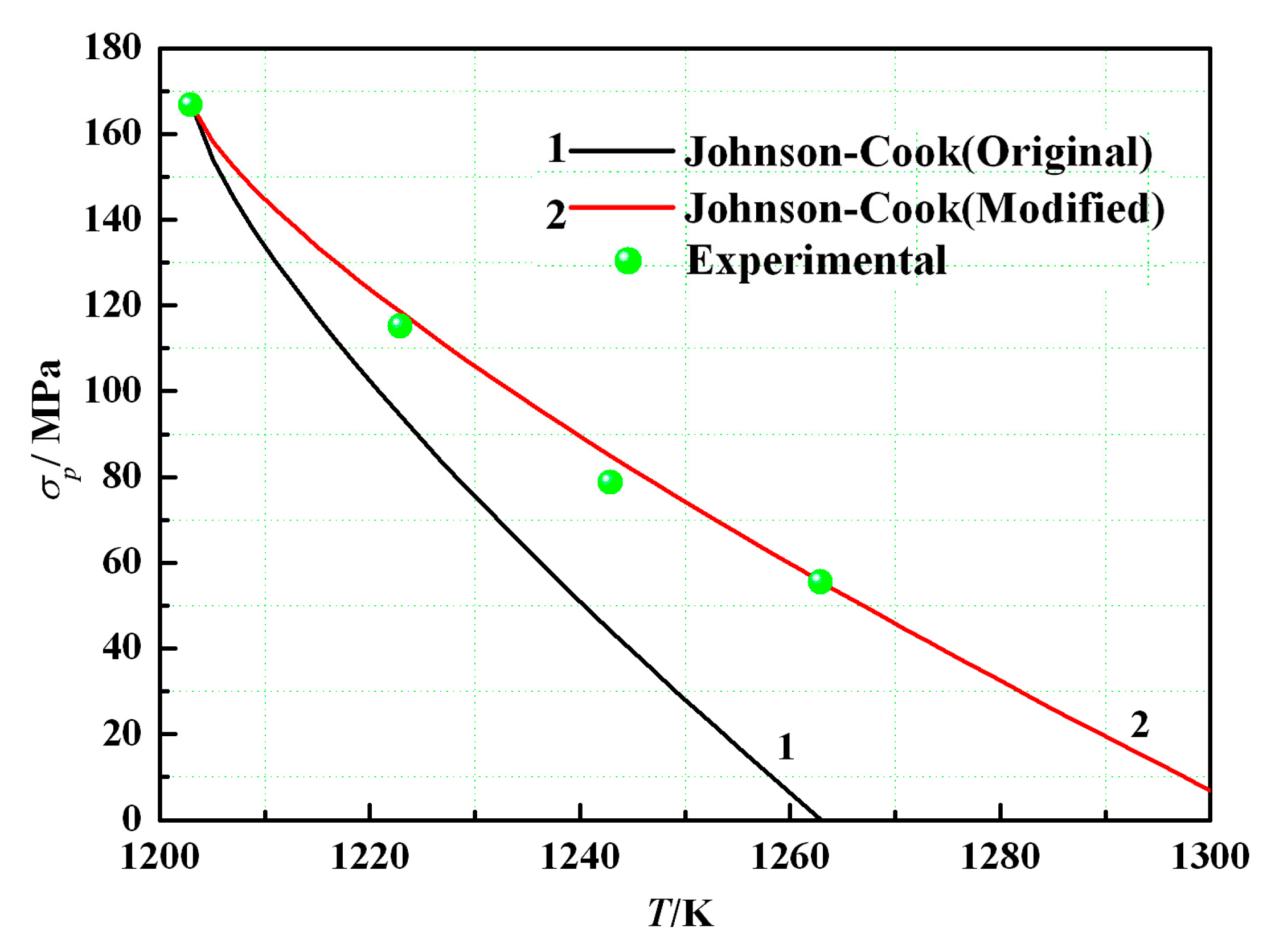
The dependence of peak stress
σp on temperature obtained from the J–C model in Equations (35) and (36) at a strain rate of 1.0 × 10
−3 s
−1 is shown in
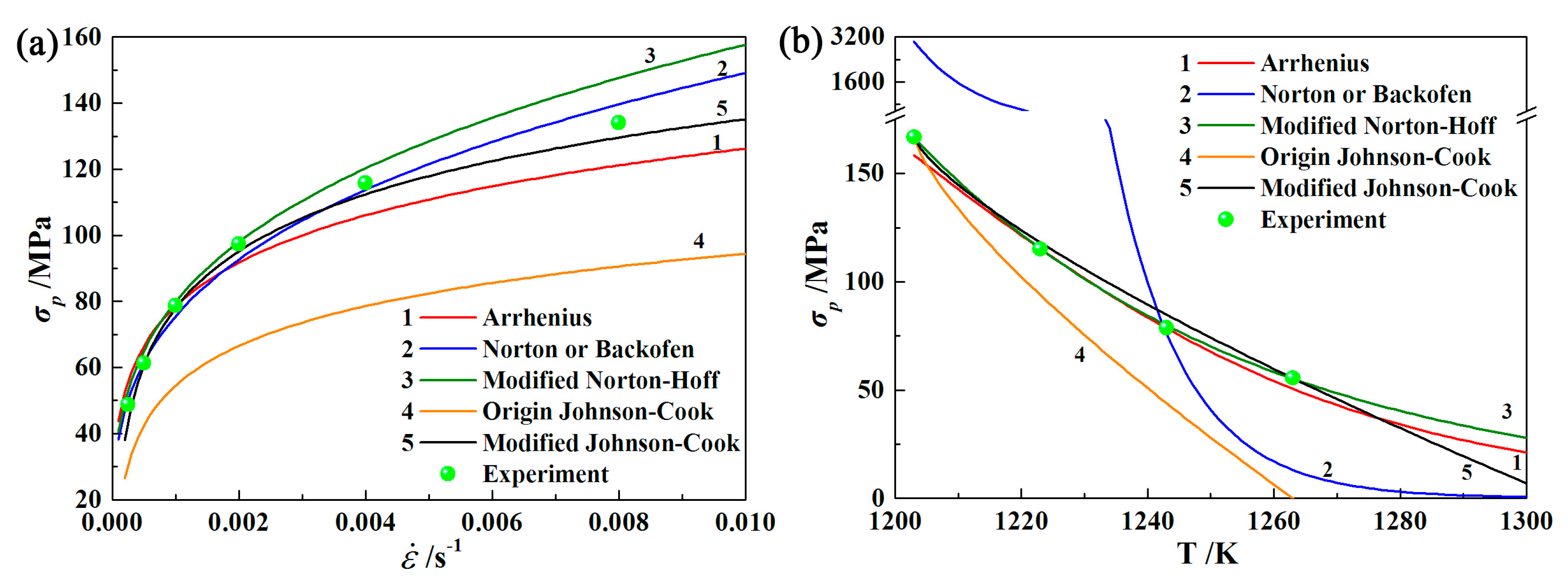
Figure 8. Obviously, the fitting of the constitutive equation in Equation (35) exhibits good agreement with the experimental data. In contrast, the constitutive equation depicted in Equation (36) shows a large deviation from the experimental data, indicating that using the improved J–C model to describe the mechanical behavior of some material at a specific temperature range is feasible. However, the original J–C model with the temperature range from the room temperature to melting temperature is not suitable for any deformation condition.
The modification of the J–C constitutive model is useful in investigating the deformation behavior of materials in a specific narrow temperature range, within which the deformation mechanism remains relatively stable. Otherwise, in terms of the original definition of the J–C model, it is unrealistic to use one equation to characterize the material mechanical behavior from the room to melting temperature due to the different deformation mechanism at various temperatures. Therefore, establishing a material constitutive model for various temperature ranges can accurately indicate the influence of temperature on the material deformation behavior. Meanwhile, in most cases, we also focus on the mechanical behavior of material for a certain temperature range. Then, it is extremely important and necessary to establish the material constitutive models in a specific temperature range.