Carbon Nano-Onions as Nanofillers for Enhancing the Damping Capacity of Titanium and Fiber-Reinforced Titanium: A Numerical Investigation
Abstract
1. Introduction
2. Materials and Methods
2.1. Numerical Treatment of Basic Phases
2.1.1. Alphα Titanium Alloy
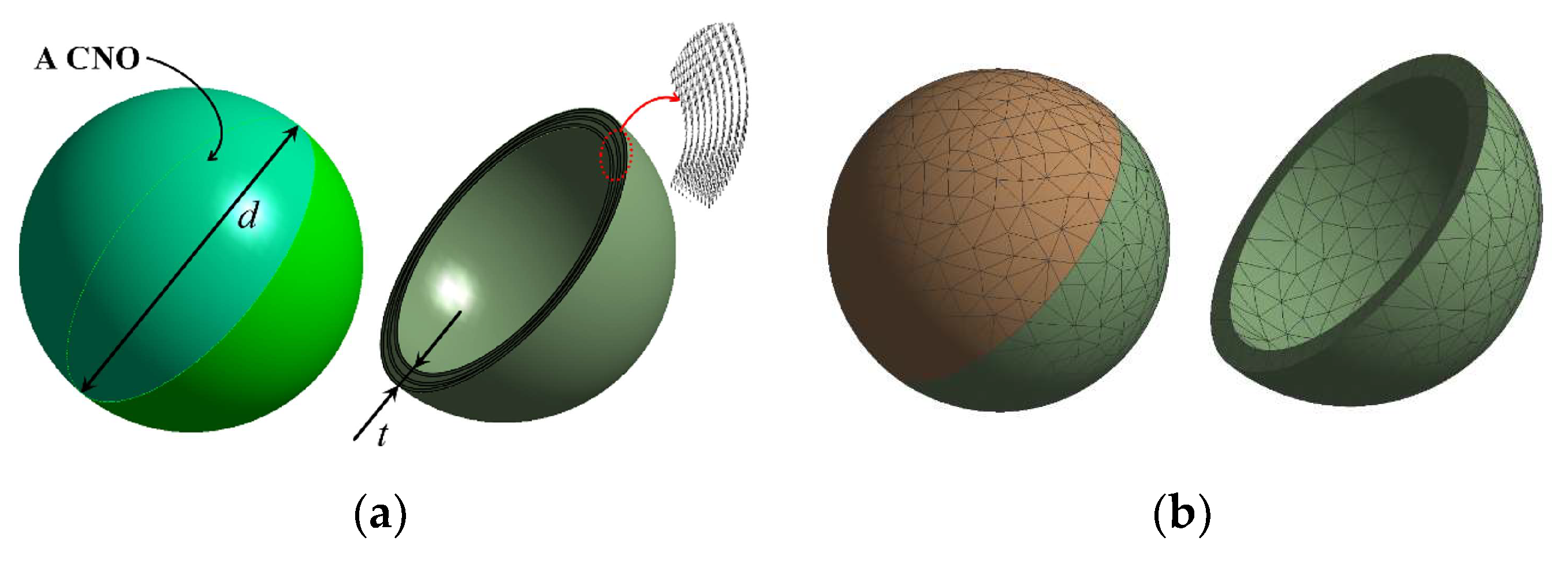
2.1.2. Carbon Nano-Onions
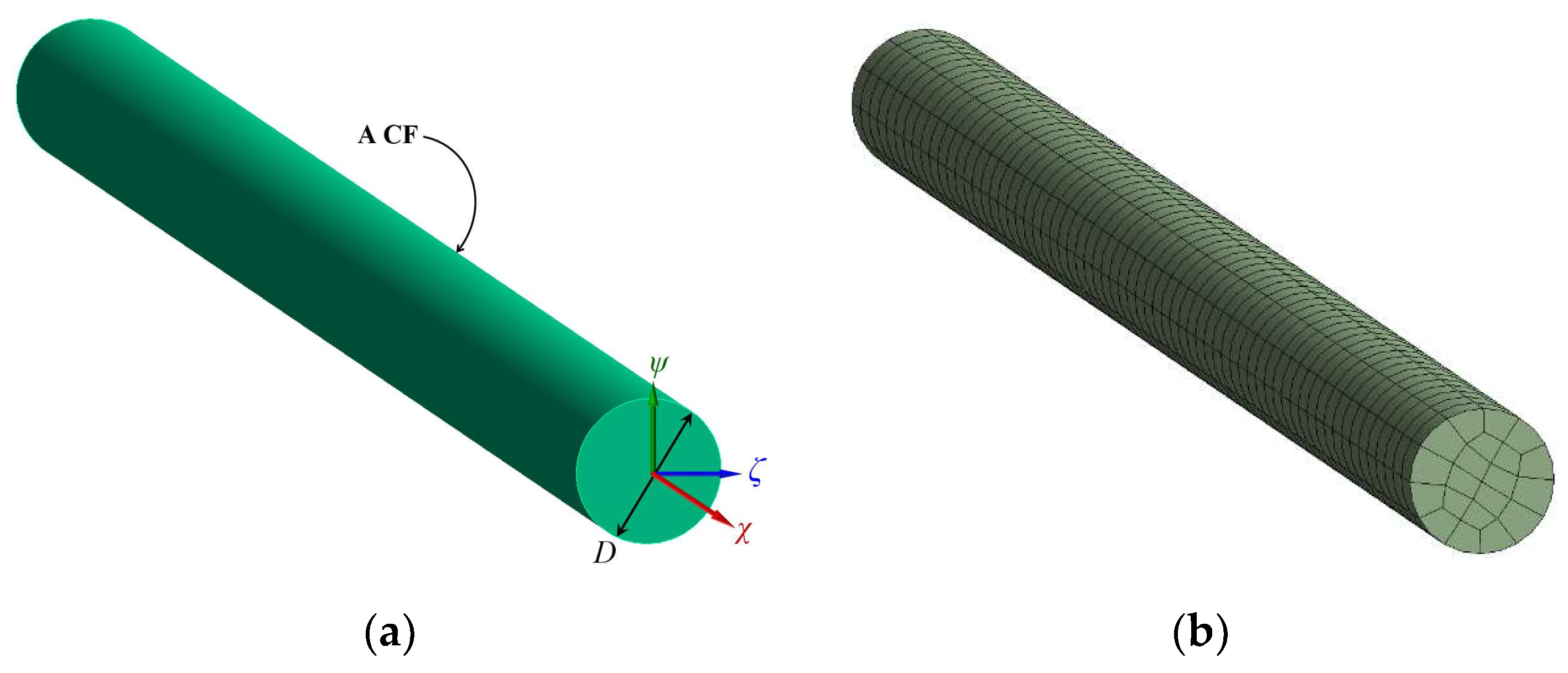
2.1.3. Carbon Fibers
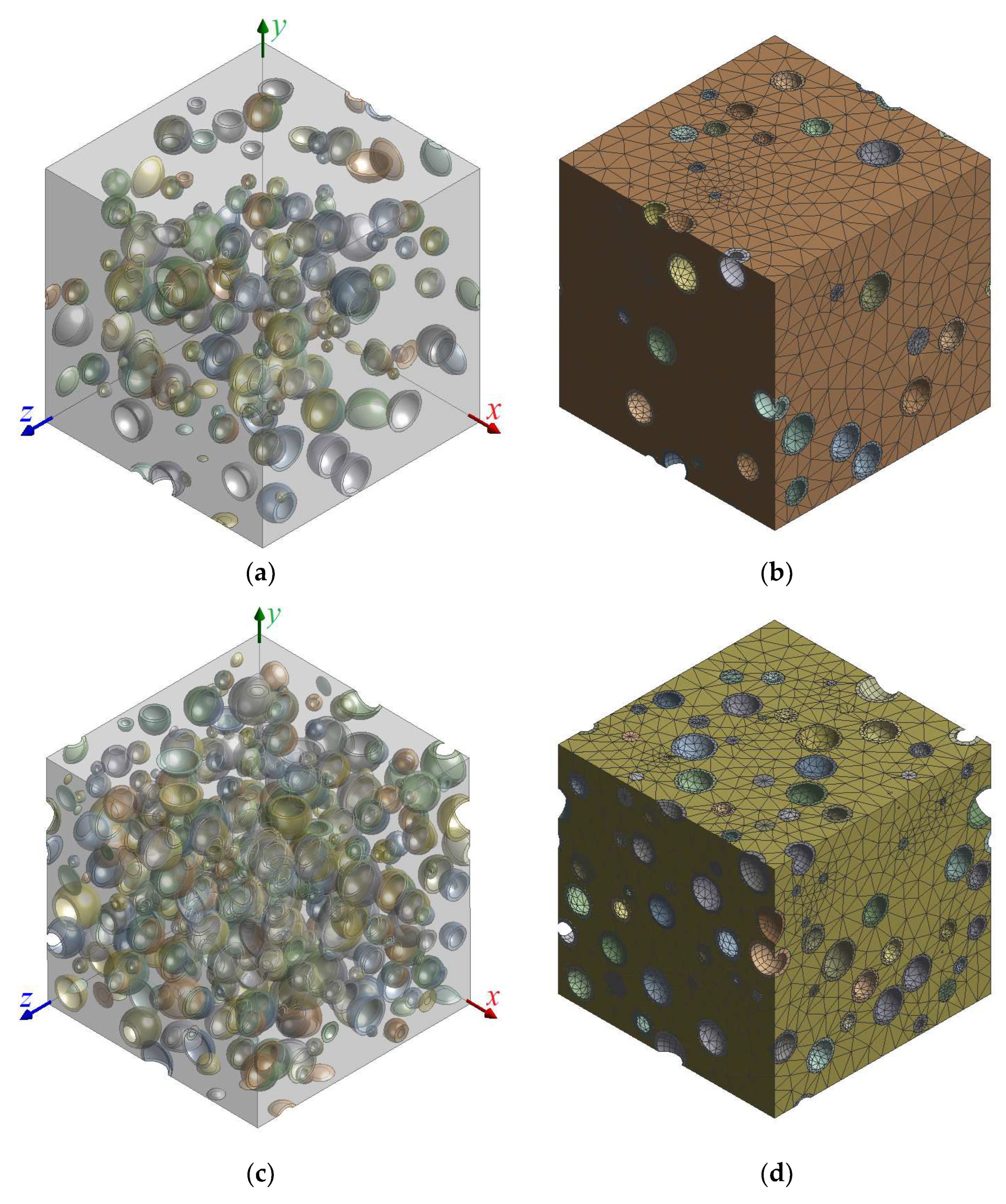
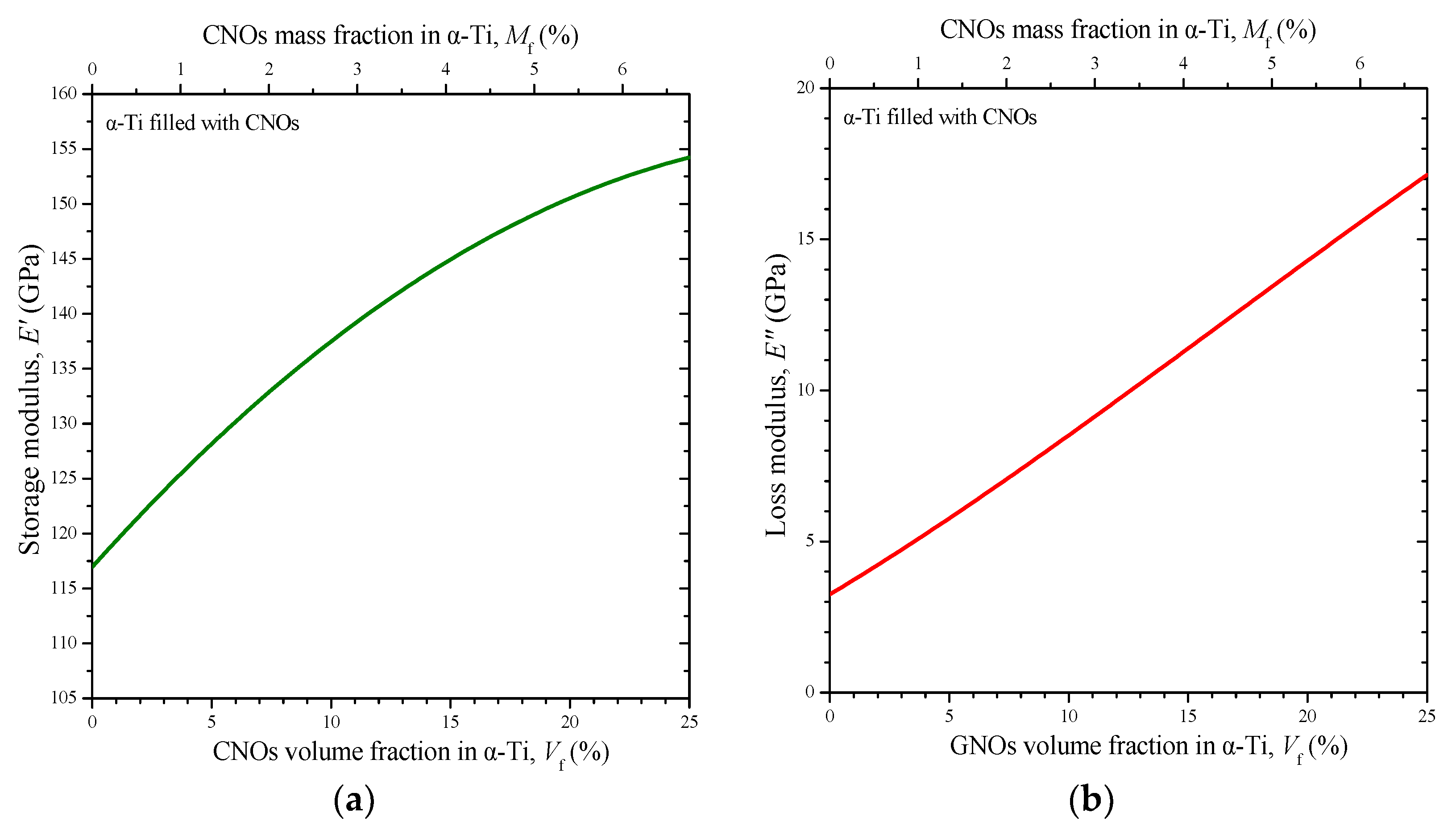
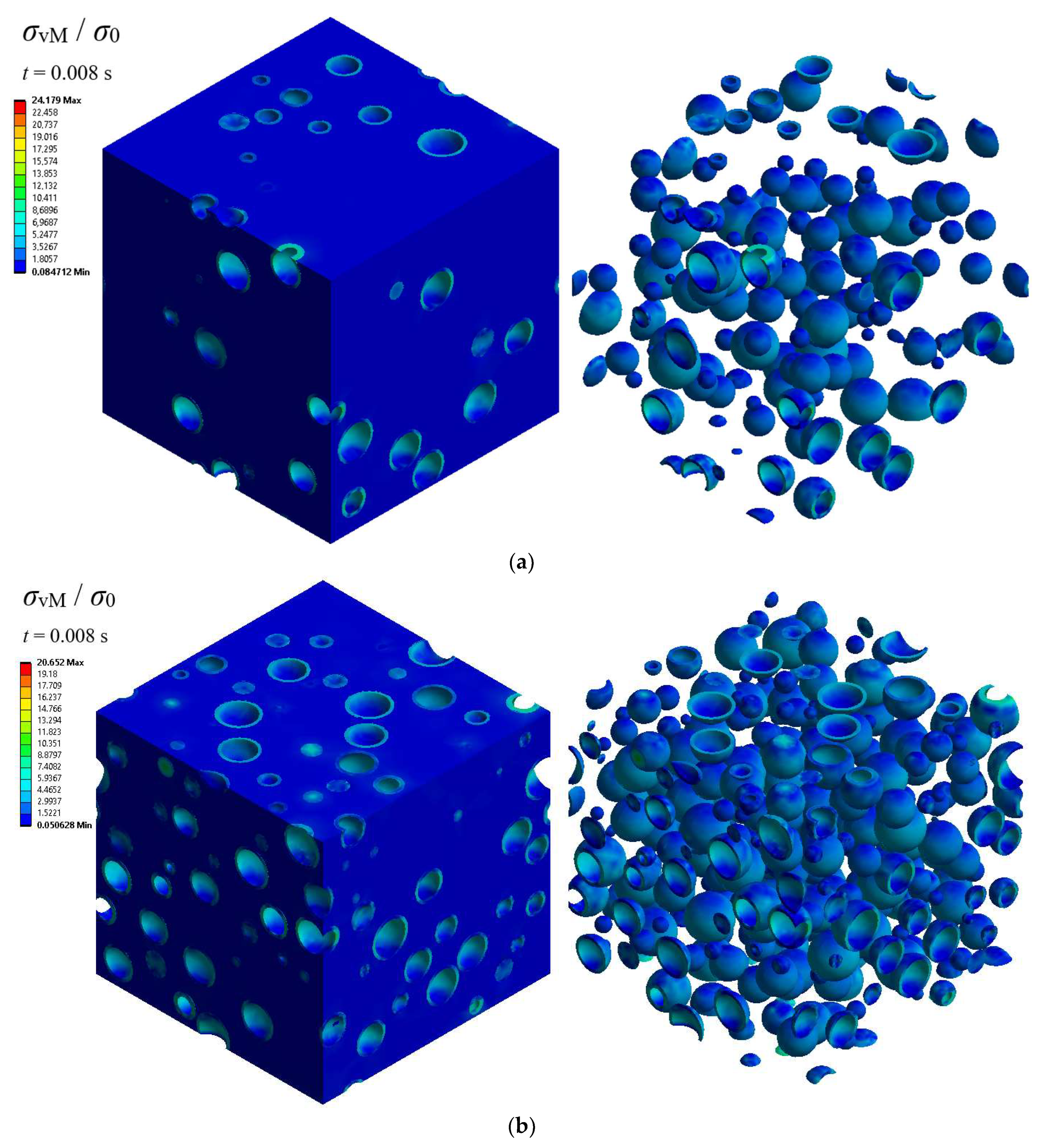
2.2. Numerical Treatment of α-Ti Filled with CNOs
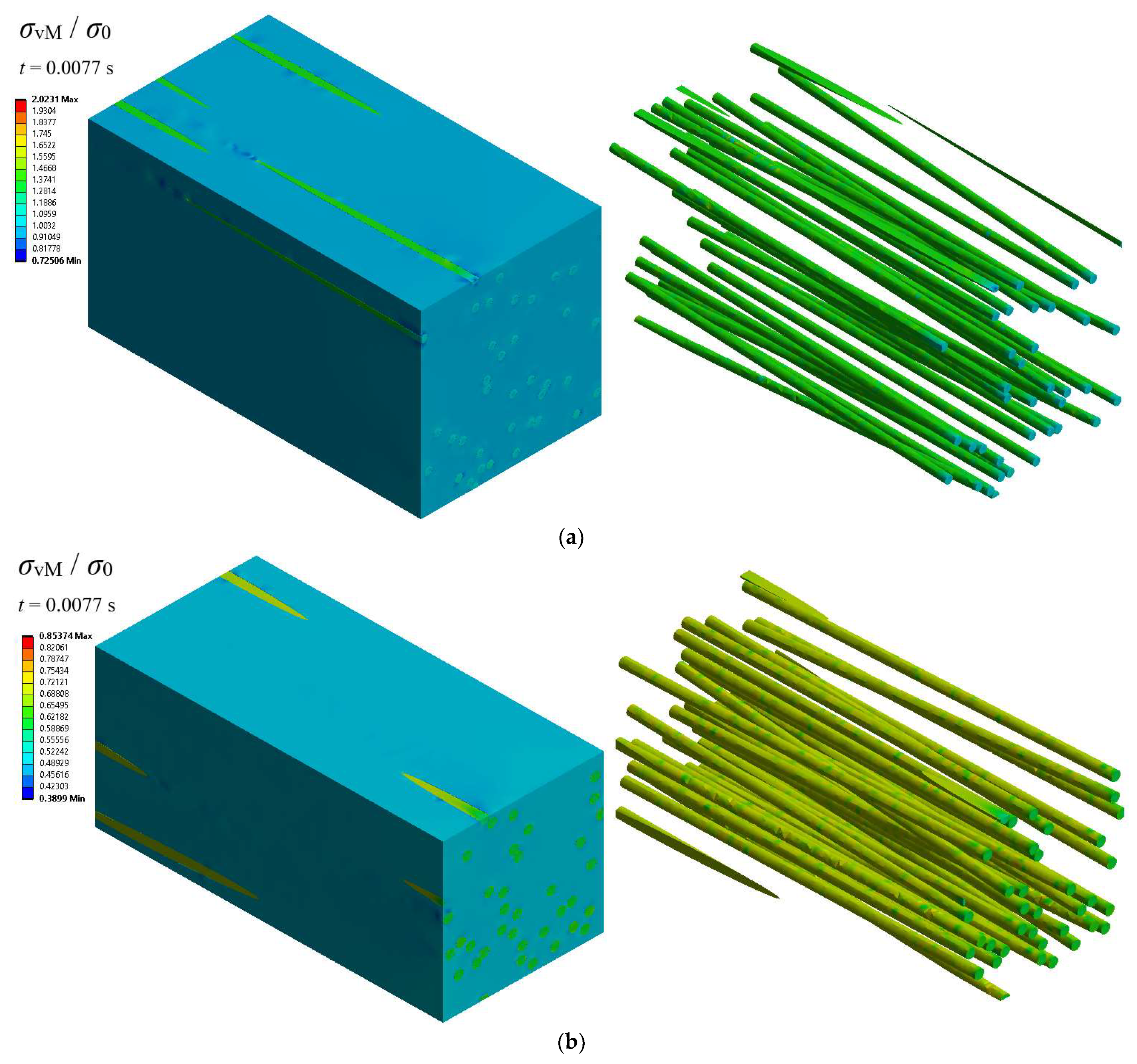
2.3. Numerical Treatment of CNOs/α-Ti Nanocomposite Reinforced with CFs
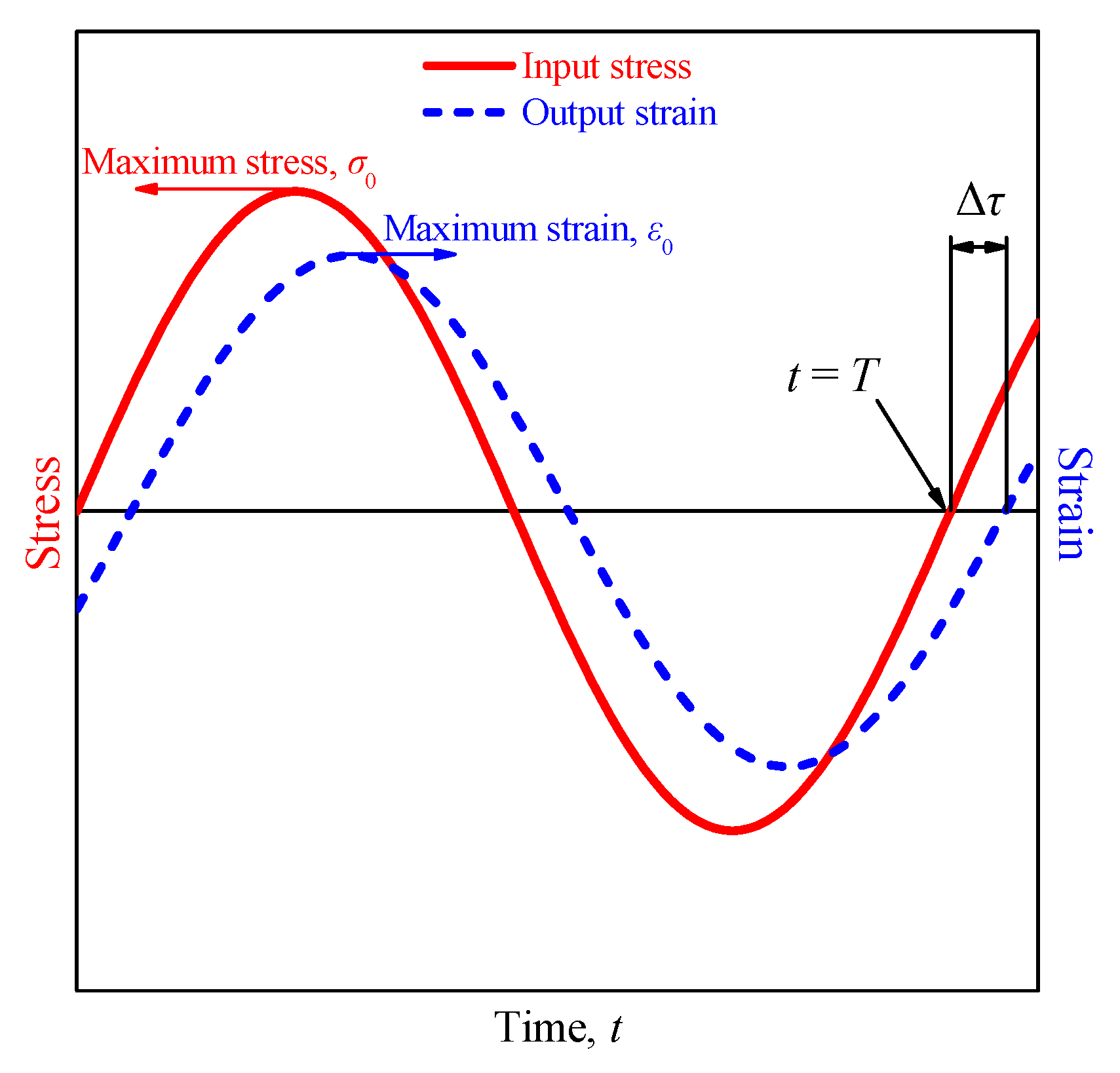
2.4. Computation of Dynamic Properties
3. Numerical Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Tjong, S.C. Recent progress in the development and properties of novel metal matrix nanocomposites reinforced with carbon nanotubes and graphene nanosheets. Mater. Sci. Eng. R Rep. 2013, 74, 281–350. [Google Scholar]
- Yoo, S.C.; Lee, D.; Ryu, S.W.; Kang, B.; Ryu, H.J.; Hong, S.H. Recent progress in low-dimensional nanomaterials filled multifunctional metal matrix nanocomposites. Prog. Mater. Sci. 2023, 132, 101034. [Google Scholar]
- Ghavanloo, E.; Rafii-Tabar, H.; Kausar, A.; Giannopoulos, G.I.; Fazelzadeh, S.A. Experimental and computational physics of fullerenes and their nanocomposites: Synthesis, thermo-mechanical characteristics and nanomedicine applications. Phys. Rep. 2023, 996, 1–116. [Google Scholar]
- Mykhailiv, O.; Zubyk, H.; Plonska-Brzezinska, M.E. Carbon nano-onions: Unique carbon nanostructures with fascinating properties and their potential applications. Inorganica Chim. Acta 2017, 468, 49–66. [Google Scholar]
- Ajay Kumar, P.; Vishnu Namboodiri, V.; Joshi, G.; Mehta, K.P. Fabrication and applications of fullerene-based metal nanocomposites: A review. J. Mater. Res. 2021, 36, 114–128. [Google Scholar]
- Zeiger, M.; Jäckel, N.; Mochalin, V.N.; Presser, V. Review: Carbon onions for electrochemical energy storage. J. Mater. Chem. A 2016, 4, 3172–3196. [Google Scholar]
- He, C.; Zhao, N.; Shi, C.; Du, X.; Li, J.; Cui, L. A practical method for the production of hollow carbon onion particles. J. Alloys Compd. 2006, 425, 329–333. [Google Scholar] [CrossRef]
- Klose, M.; Pinkert, K.; Zier, M.; Uhlemann, M.; Wolke, F.; Jaumann, T.; Jehnichen, P.; Wadewitz, D.; Oswald, S.; Eckert, J.; et al. Hollow carbon nano-onions with hierarchical porosity derived from commercial metal organic framework. Carbon 2014, 79, 302–309. [Google Scholar]
- Ruan, C.; Li, Z.; Zhang, D.; Yuan, X.; Liang, C.; Chang, Y.; Huang, H.; Xu, L.; Chen, M. A scalable chemical vapor deposition synthesis of high purity hollow carbon onions. Carbon 2020, 161, 622–628. [Google Scholar] [CrossRef]
- Fan, K.; Zhang, F.; Shang, C.; Saba, F.; Yu, J. Mechanical properties and strengthening mechanisms of titanium matrix nanocomposites reinforced with onion-like carbons. Compos. Part A Appl. Sci. Manuf. 2020, 132, 105834. [Google Scholar]
- Aissou, T.; Casteignau, F.; Braidy, N.; Veilleux, J. Synthesis and Growth of Onion-Like Polyhedral Graphitic Nanocapsules by Thermal Plasma. Plasma Chem. Plasma Process. 2023, 43, 413–427. [Google Scholar] [CrossRef]
- Baowan, D.; Thamwattana, N.; Hill, J.M. Continuum modelling of spherical and spheroidal carbon onions. Eur. Phys. J. D 2007, 44, 117–123. [Google Scholar] [CrossRef]
- Todt, M.; Bitsche, R.D.; Hartmann, M.A.; Fischer, F.D.; Rammerstorfer, F.G. Growth limit of carbon onions—A continuum mechanical study. Int. J. Solids Struct. 2014, 51, 706–715. [Google Scholar] [CrossRef][Green Version]
- Izadi, R.; Ghavanloo, E.; Nayebi, A. Elastic properties of polymer composites reinforced with C60 fullerene and carbon onion: Molecular dynamics simulation. Phys. B Condens. Matter 2019, 574, 311636. [Google Scholar] [CrossRef]
- Pereira Júnior, M.L.; da Cunha, W.F.; de Sousa Junior, R.T.; Amvame Nze, G.D.; Galvão, D.S.; Ribeiro Júnior, L.A. Dynamics and structural transformations of carbon onion-like structures under high-velocity impacts. Carbon 2022, 189, 422–429. [Google Scholar] [CrossRef]
- Zuo, Y.Q.; Smith, D.J.; Partridge, P.G. Damping capacity of laminates composed of layers of titanium alloy and titanium alloy MMC. Mater. Sci. Technol. 1998, 14, 518–521. [Google Scholar] [CrossRef]
- Kalyan Phani, M.; Kumar, A.; Arnold, W.; Samwer, K. Elastic stiffness and damping measurements in titanium alloys using atomic force acoustic microscopy. J. Alloys Compd. 2016, 676, 397–406. [Google Scholar] [CrossRef]
- Meng, X.; Zhao, Y.; Lu, J.; Huang, S.; Zhou, J.; Su, C. Improvement of damping property and its effects on the vibration fatigue in Ti6Al4V titanium alloy treated by warm laser shock peening. Metals 2019, 9, 746. [Google Scholar] [CrossRef]
- Liu, W.; Wang, N.; Chen, Y.; Hou, Z.; Zhao, Q.; Ouyang, W.; Kang, Y.; Wu, G.; Zhu, L.; Zhao, Y. Damping behavior of typical titanium alloys by varied frequency micro harmonic vibration at cryogenic temperatures. J. Mater. Res. Technol. 2022, 21, 3746–3755. [Google Scholar] [CrossRef]
- Lahiri, D.; Das, S.; Choi, W.; Agarwal, A. Unfolding the damping behavior of multilayer graphene membrane in the low-frequency regime. ACS Nano 2012, 6, 3992–4000. [Google Scholar] [CrossRef]
- Zhang, J.; Gungor, M.N.; Lavernia, E.J. The effect of porosity on the microstructural damping response of 6061 aluminium alloy. J. Mater. Sci. 1993, 28, 1515–1524. [Google Scholar] [CrossRef]
- Panteliou, S.D.; Dimarogonas, A.D. Damping associated with porosity and crack in solids. Theor. Appl. Fract. Mech. 2000, 34, 217–223. [Google Scholar] [CrossRef]
- Tabie, V.M.; Li, C.; Saifu, W.; Li, J.; Xu, X. Mechanical properties of near alpha titanium alloys for high-temperature applications–a review. Aircr. Eng. Aerosp. Technol. 2020, 92, 521–540. [Google Scholar]
- Klepikov, V.F.; Lonin, Y.F.; Ponomarev, A.G.; Startsev, O.A.; Uvarov, V.T. Physical and mechanical properties of titanium alloy VT1-0 after high-current electron beam irradiation. Probl. At. Sci. Technol. 2015, 96, 39–42. [Google Scholar]
- Konovalov, S.; Komissarova, I.; Ivanov, Y.; Gromov, V.; Kosinov, D. Structural and phase changes under electropulse treatment of fatigue-loaded titanium alloy VT1-0. J. Mater. Res. Technol. 2019, 8, 1300–1307. [Google Scholar] [CrossRef]
- Panin, A.V.; Kazachenok, M.S.; Kazantseva, L.A.; Martynov, S.A.; Panina, A.A.; Lobova, T.A. Microstructure and Phase Composition of VT1-0, VT6, and VT14 Titanium Alloys Produced by Wire-Feed Electron-Beam Additive Manufacturing. J. Surf. Investig. 2022, 16, 983–991. [Google Scholar] [CrossRef]
- Ansys, Workbench 2020 R2. Available online: https://www.ansys.com/products/ansys-workbench (accessed on 1 June 2023).
- Lee, H.-H. Finite Element Simulations with ANSYS Workbench 2020; SDC Publications: Mission, KS, USA, 2020. [Google Scholar]
- Ansari, R.; Ahmadi, M.; Rouhi, S. Impact resistance of short carbon fibre-carbon nanotube-polymer matrix hybrid composites: A stochastic multiscale approach. Proc. Inst. Mech. Eng. Part L J. Mater. Des. Appl. 2021, 235, 1925–1936. [Google Scholar] [CrossRef]
- Strozzi, M.; Giacomobono, R.; Rubini, R.; Cocconcelli, M. Preliminary orthotropic elastic model for the study of natural frequencies and mode shapes of a 3D printed Onyx thin circular cylindrical shell. Int. J. Mech. Control 2020, 21, 51–62. [Google Scholar]
- Shinde, V.V.; Wang, Y.; Salek, M.F.; Auad, M.L.; Beckingham, L.E.; Beckingham, B.S. Material Design for Enhancing Properties of 3D Printed Polymer Composites for Target Applications. Technologies 2022, 10, 45. [Google Scholar] [CrossRef]
- Zheng, Y.; Huang, X.; Chen, J.; Wu, K.; Wang, J.; Zhang, X. A review of conductive carbon materials for 3d printing: Materials, technologies, properties, and applications. Materials 2021, 14, 3911. [Google Scholar] [CrossRef]
- Mileiko, S. Carbon-Fibre/Metal-Matrix Composites: A Review. J. Compos. Sci. 2022, 6, 297. [Google Scholar] [CrossRef]
- Mileiko, S.T.; Rudnev, A.M.; Gelachov, M.V. Carbon-fibre/titanium silicide interphase/titanium-matrix composites: Fabrication, structure and mechanical properties. Compos. Sci. Technol. 1995, 55, 255–260. [Google Scholar] [CrossRef]
- Even, C.; Arvieu, C.; Quenisset, J.M. Powder route processing of carbon fibres reinforced titanium matrix composites. Compos. Sci. Technol. 2008, 68, 1273–1281. [Google Scholar] [CrossRef][Green Version]
- Lv, S.; Li, J.S.; Li, S.F.; Kang, N.; Chen, B. Effects of heat treatment on interfacial characteristics and mechanical properties of titanium matrix composites reinforced with discontinuous carbon fibers. J. Alloys Compd. 2021, 877, 160313. [Google Scholar]
- Mileiko, S.T.; Kolchin, A.A.; Krivtsov, D.I.; Galyshev, S.N.; Prokopenko, N.A.; Shakhlevich, O.F.; Petrova, O.V. Carbon-fibre/titanium-matrix composites of a hierarchical microstructure. Compos. Part A Appl. Sci. Manuf. 2022, 155, 106817. [Google Scholar] [CrossRef]
- Ng, S.-P.; Tse, P.-C.; Lau, K.-J. Numerical and experimental determination of in-plane elastic properties of 2/2 twill weave fabric composites. Compos. Part B Eng. 1998, 29, 735–744. [Google Scholar] [CrossRef]
- Lesieutre, G.A.; Eckel, A.J.; DiCarlo, J.A. Damping of bromine-intercalated P-100 graphite fibers. Carbon 1991, 29, 1025–1032. [Google Scholar] [CrossRef]
- Rahul, B.; Dharani, J.; Balaji, R. Optimal method for determination of rayleigh damping coefficients for different materials using modal analysis. Int. J. Veh. Struct. Syst. 2021, 13, 102–111. [Google Scholar]
- Meyers, M.; Chawla, K. Mechanical Behaviors of Materials, 2nd ed.; Cambridge University Press: New York, NY, USA, 2009; pp. 124–125. [Google Scholar]





| Material/Phase | Behavior | Density (kg/m3) | Elastic Constant (GPa) | Poisson’s Ratio (−) | Loss Factor (−) | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| ρ | Exx | Eyy | Ezz | Gxy | Gyz | Gxz | νxy | νyz | νxz | tanδ | ||
| α-Ti | Isotropic | 4505 | 117 | 117 | 177 | 43.985 | 43.985 | 43.985 | 0.33 | 0.33 | 0.33 | 0.0278 |
| CNOs | Isotropic | 2267 | 387 | 1050 | 1050 | 442.66 | 442.66 | 442.66 | 0.186 | 0.186 | 0.186 | 0.518 |
| CFs | Orthotropic | 2150 | 17.24 | 17.24 | 17.24 | 7.1 | 7.1 | 7.1 | 0.214 | 0.214 | 0.214 | 0.000159 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Giannopoulos, G.I.; Batsoulas, N.D. Carbon Nano-Onions as Nanofillers for Enhancing the Damping Capacity of Titanium and Fiber-Reinforced Titanium: A Numerical Investigation. Metals 2023, 13, 1577. https://doi.org/10.3390/met13091577
Giannopoulos GI, Batsoulas ND. Carbon Nano-Onions as Nanofillers for Enhancing the Damping Capacity of Titanium and Fiber-Reinforced Titanium: A Numerical Investigation. Metals. 2023; 13(9):1577. https://doi.org/10.3390/met13091577
Chicago/Turabian StyleGiannopoulos, Georgios I., and Nikolaos D. Batsoulas. 2023. "Carbon Nano-Onions as Nanofillers for Enhancing the Damping Capacity of Titanium and Fiber-Reinforced Titanium: A Numerical Investigation" Metals 13, no. 9: 1577. https://doi.org/10.3390/met13091577
APA StyleGiannopoulos, G. I., & Batsoulas, N. D. (2023). Carbon Nano-Onions as Nanofillers for Enhancing the Damping Capacity of Titanium and Fiber-Reinforced Titanium: A Numerical Investigation. Metals, 13(9), 1577. https://doi.org/10.3390/met13091577







