Using Portable Force Plates to Assess Vertical Jump Performance: A Metrological Appraisal
Abstract
:1. Introduction
2. Materials and Methods
2.1. Participants
2.2. Experimental Approach to the Problem
2.3. Data Analyses
2.4. Statistical Analyses
3. Results
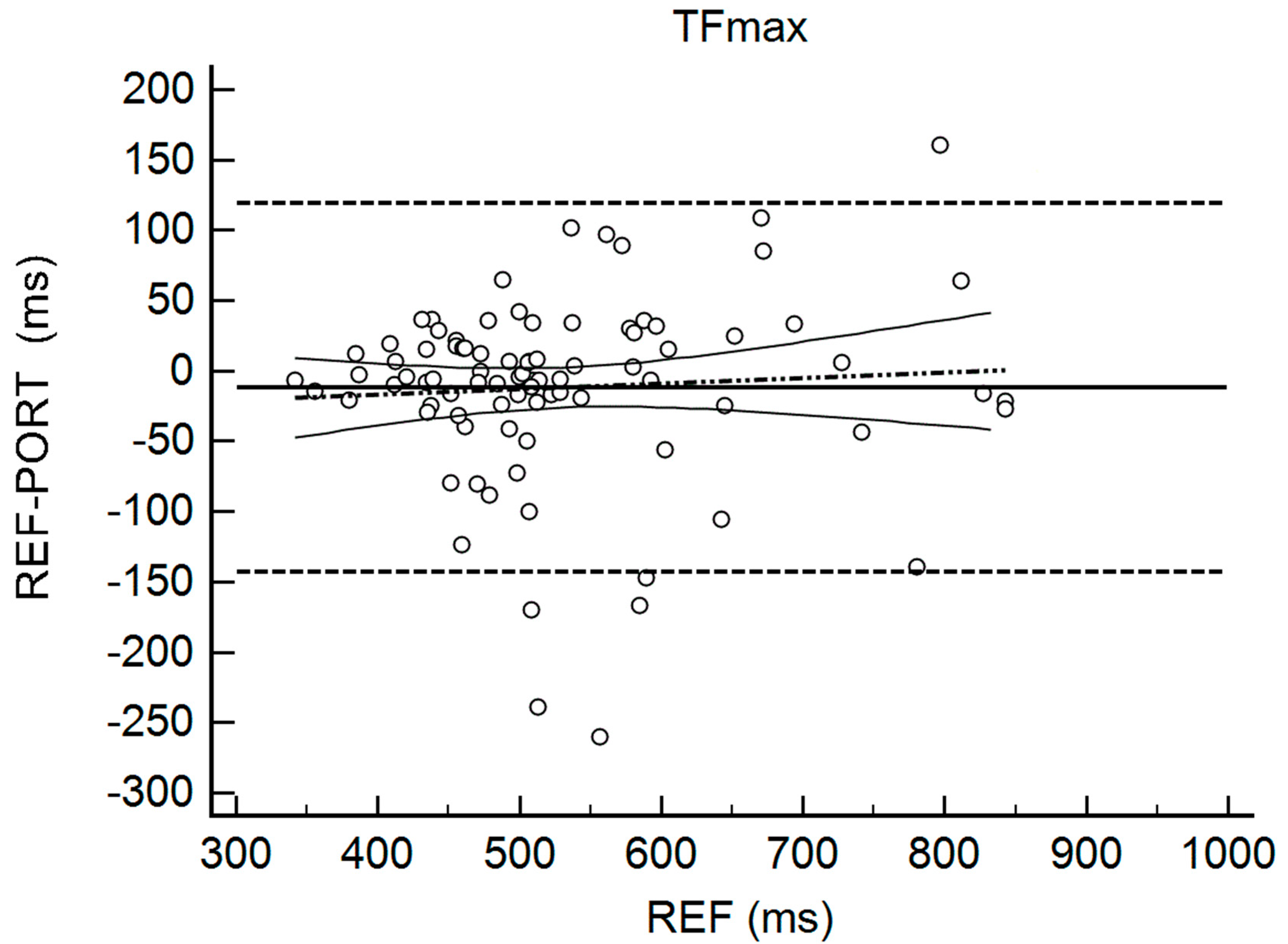
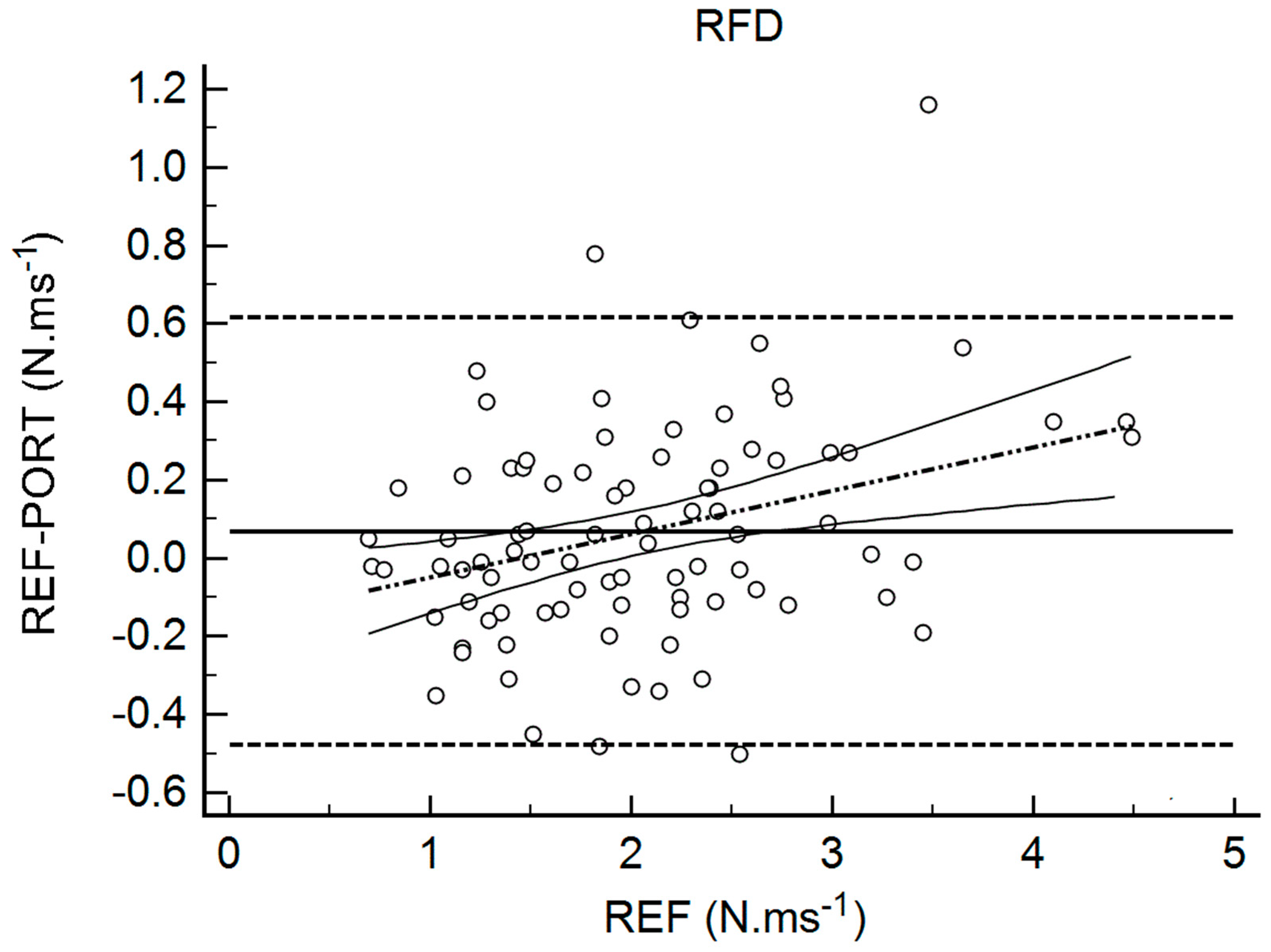
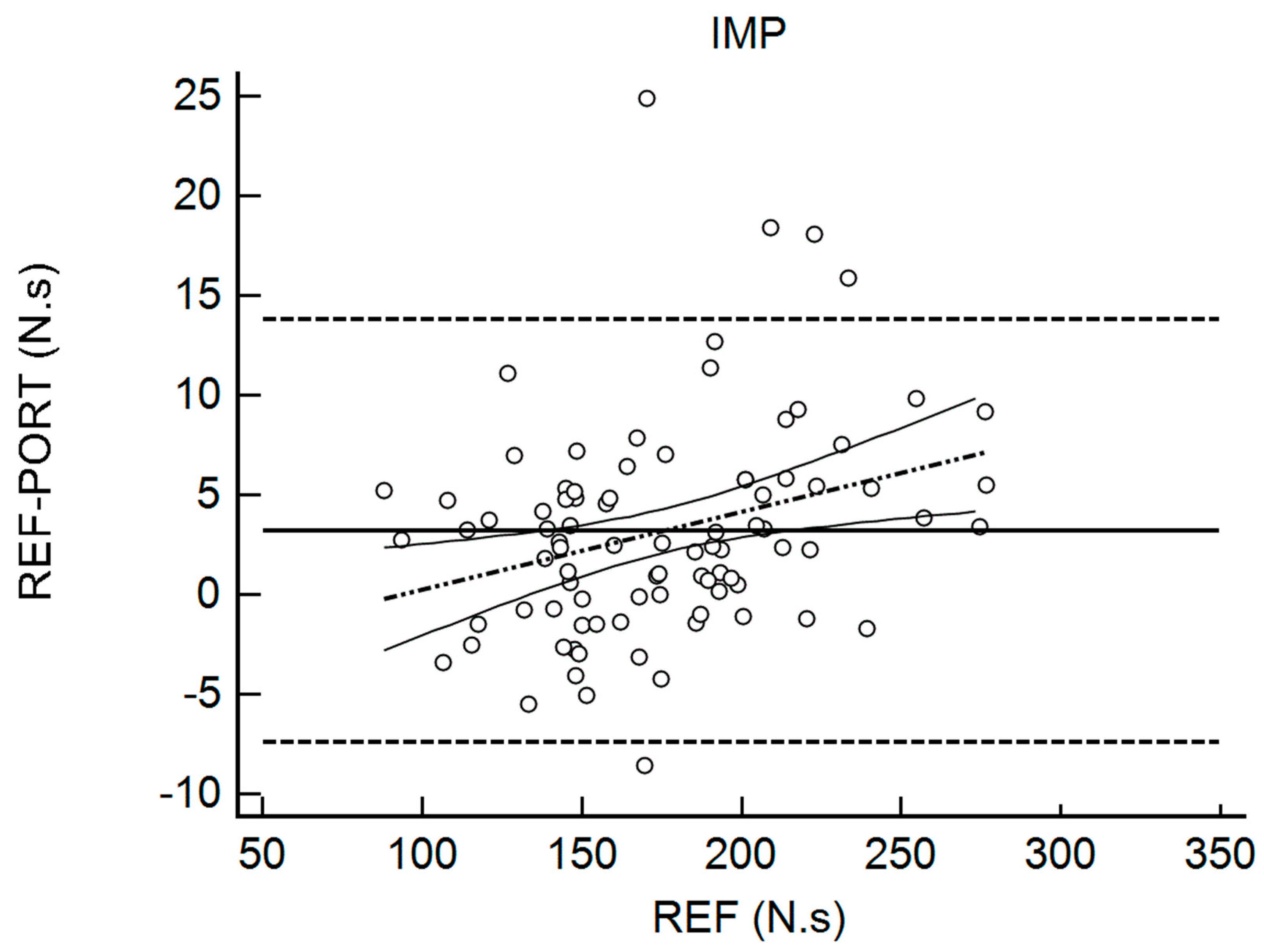
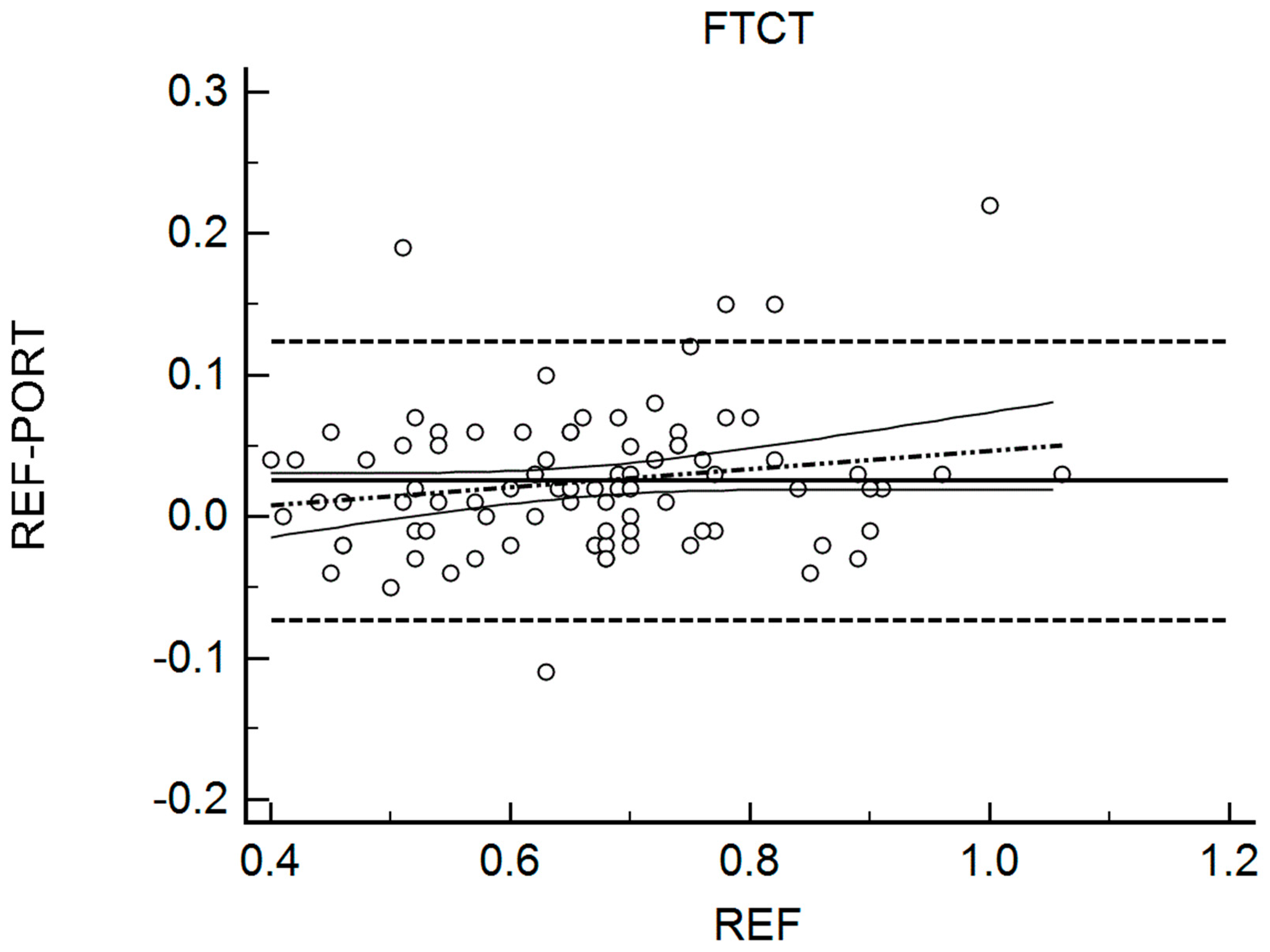
3.1. Interchangeability
3.2. Reliability
4. Discussion
5. Practical Applications
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Kreider, R.B.; Fry, A.C.; O’Toole, M.L. Overtraining in Sport; Human Kinetics: Champaign, IL, USA, 1998; 403p. [Google Scholar]
- Garber, C.E.; Blissmer, B.; Deschenes, M.R.; Franklin, B.A.; Lamonte, M.J.; Lee, I.M.; Nieman, D.C.; Swain, D.P.; American College of Sports Medicine. American College of Sports Medicine position stand. Quantity and quality of exercise for developing and maintaining cardiorespiratory, musculoskeletal, and neuromotor fitness in apparently healthy adults: Guidance for prescribing exercise. Med. Sci. Sports Exerc. 2011, 43, 1334–1359. [Google Scholar] [CrossRef] [PubMed]
- Kellmann, M.; Bertollo, M.; Bosquet, L.; Brink, M.; Coutts, A.J.; Duffield, R.; Erlacher, D.; Halson, S.L.; Hecksteden, A.; Heidari, J.; et al. Recovery and Performance in Sport: Consensus Statement. Int. J. Sports Physiol. Perform. 2018, 13, 240–245. [Google Scholar] [CrossRef] [PubMed]
- Buchheit, M. Monitoring training status with HR measures: Do all roads lead to Rome? Front. Physiol. 2014, 5, 73. [Google Scholar] [CrossRef] [PubMed]
- Gathercole, R.; Sporer, B.; Stellingwerff, T. Countermovement Jump Performance with Increased Training Loads in Elite Female Rugby Athletes. Int. J. Sports Med. 2015, 36, 722–728. [Google Scholar] [CrossRef] [PubMed]
- Gathercole, R.; Sporer, B.; Stellingwerff, T.; Sleivert, G. Alternative countermovement-jump analysis to quantify acute neuromuscular fatigue. Int. J. Sports Physiol. Perform. 2015, 10, 84–92. [Google Scholar] [CrossRef] [PubMed]
- Howatson, G.; Brandon, R.; Hunter, A.M. The Response to and Recovery from Maximum-Strength and -Power Training in Elite Track and Field Athletes. Int. J. Sports Physiol. Perform. 2016, 11, 356–362. [Google Scholar] [CrossRef] [PubMed]
- Jordan, M.J.; Aagaard, P.; Herzog, W. Lower limb asymmetry in mechanical muscle function: A comparison between ski racers with and without ACL reconstruction. Scand. J. Med. Sci. Sports 2015, 25, e301–e309. [Google Scholar] [CrossRef] [PubMed]
- Taylor, K.L.; Cronin, J.; Gill, N.D.; Chapman, D.W.; Sheppard, J. Sources of variability in iso-inertial jump assessments. Int. J. Sports Physiol. Perform. 2010, 5, 546–558. [Google Scholar] [PubMed]
- Cormie, P.; McBride, J.M.; McCaulley, G.O. Power-time, force-time, and velocity-time curve analysis of the countermovement jump: Impact of training. J. Strength Cond. Res. 2009, 23, 177–186. [Google Scholar] [CrossRef] [PubMed]
- Cormack, S.J.; Newton, R.U.; McGuigan, M.R. Neuromuscular and endocrine responses of elite players to an Australian rules football match. Int. J. Sports Physiol. Perform. 2008, 3, 359–374. [Google Scholar] [CrossRef] [PubMed]
- Robertson, S.; Bartlett, J.D.; Gastin, P.B. Red, Amber, or Green? Athlete Monitoring in Team Sport: The Need for Decision-Support Systems. Int. J. Sports Physiol. Perform. 2017, 12, S273–S279. [Google Scholar] [CrossRef] [PubMed]
- Lake, J.; Mundy, P.; Comfort, P.; McMahon, J.J.; Suchomel, T.J.; Carden, P. Concurrent Validity of a Portable Force Plate Using Vertical Jump Force-Time Characteristics. J. Appl. Biomech. 2018, 1–16. [Google Scholar] [CrossRef] [PubMed]
- Bland, J.M.; Altman, D.G. Statistical methods for assessing agreement between two methods of clinical measurement. Lancet 1986, 1, 307–310. [Google Scholar] [CrossRef]
- Kirby, T.J.; McBride, J.M.; Haines, T.L.; Dayne, A.M. Relative net vertical impulse determines jumping performance. J. Appl. Biomech. 2011, 27, 207–214. [Google Scholar] [CrossRef] [PubMed]
- Field, A.P. Discovering Statistics Using SPSS: (And Sex and Drugs and Rock ‘n’ Roll), 3rd ed.; SAGE Publications: Los Angeles, CA, USA; London, UK, 2009; 821p. [Google Scholar]
- Desjardins-Crepeau, L.; Berryman, N.; Vu, T.T.; Villalpando, J.M.; Kergoat, M.J.; Li, K.Z.; Bosquet, L.; Bherer, L. Physical functioning is associated with processing speed and executive functions in community-dwelling older adults. J. Gerontol. B Psychol. Sci. Soc. Sci. 2014, 69, 837–844. [Google Scholar] [CrossRef] [PubMed]
- Dupuy, O.; Lussier, M.; Fraser, S.; Bherer, L.; Audiffren, M.; Bosquet, L. Effect of overreaching on cognitive performance and related cardiac autonomic control. Scand. J. Med. Sci. Sports 2014, 24, 234–242. [Google Scholar] [CrossRef] [PubMed]
- Cohen, J. Statistical Power Analysis for the Behavioral Sciences, 2nd ed.; L. Erlbaum Associates: Hillsdale, MI, USA, 1988. [Google Scholar]
- Ludbrook, J. Confidence in Altman-Bland plots: A critical review of the method of differences. Clin. Exp. Pharmacol. Physiol. 2010, 37, 143–149. [Google Scholar] [CrossRef] [PubMed]
- Bosquet, L.; Berryman, N.; Dupuy, O. A comparison of 2 optical timing systems designed to measure flight time and contact time during jumping and hopping. J. Strength Cond. Res. 2009, 23, 2660–2665. [Google Scholar] [CrossRef] [PubMed]
- Weir, J.P. Quantifying test-retest reliability using the intraclass correlation coefficient and the SEM. J. Strength Cond. Res. 2005, 19, 231–240. [Google Scholar] [CrossRef] [PubMed]
- Dupuy, O.; Mekary, S.; Berryman, N.; Bherer, L.; Audiffren, M.; Bosquet, L. Reliability of heart rate measures used to assess post-exercise parasympathetic reactivation. Clin. Physiol. Funct. Imaging 2012, 32, 296–304. [Google Scholar] [CrossRef] [PubMed]
- Currell, K.; Jeukendrup, A.E. Validity, reliability and sensitivity of measures of sporting performance. Sports Med. 2008, 38, 297–316. [Google Scholar] [CrossRef] [PubMed]

| Variables | Interchangeability Analysis | Reliability Analysis |
|---|---|---|
| Cohort size | 88 | 84 |
| Sex | 38 M, 50 W | 37 M, 47 W |
| Testing sequence | 43 PORT-REF, 45 REF-PORT | 40 GL, 44 LG |
| Age a | 24.72 (6.52) | 24.50 (6.34) |
| Body mass b | 72.08 (12.47) | 72.67 (12.46) |
| Variables | Portable | Reference | p Value | ES | Bias (±95% LOA) | Bias (%REF) |
|---|---|---|---|---|---|---|
| FMax (N) | 1710.24 (370.31) | 1725.69 (381.93) | 0.060 | 0.04 | 15.46 (149.74) | 0.90 |
| TFMax (ms) | 541.87 (125.76) | 530.17 (110.85) | 0.327 | −0.10 | −11.69 (131.16) | −2.20 |
| RFD (N·ms−1) | 1.98 (0.77) | 2.05 (0.82) | 0.060 | 0.08 | 0.07 (0.55) | 3.41 |
| IMP * (N·s) | 172.11 (40.09) | 175.30 (41.37) | <0.001 | 0.08 | 3.19 (10.66) | 1.82 |
| FTCT * | 0.64 (0.14) | 0.66 (0.14) | <0.001 | 0.17 | 0.02 (0.10) | 3.03 |
| Variables | Lab | S&C | p Value | ES | ICC | SEM | CV | MD | MD% |
|---|---|---|---|---|---|---|---|---|---|
| FMax (N) | 1710.42 (377.66) | 1721.01 (404.81) | 0.152 | 0.02 | 0.99 | 47.50 | 3.91 | 131.65 | 7.65 |
| TFMax (ms) | 543.48 (124.07) | 542.56 (130.19) | 0.887 | −0.01 | 0.89 | 41.89 | 10.91 | 116.12 | 21.37 |
| RFD (N·ms−1) | 1.97 (0.81) | 2.00 (0.85) | 0.307 | 0.03 | 0.95 | 0.18 | 13.07 | 0.51 | 25.50 |
| IMP (N·s) | 173.44 (42.24) | 173.31 (42.81) | 0.782 | 0.00 | 1.00 | 3.01 | 2.45 | 8.33 | 4.80 |
| FTCT | 0.61 (0.17) | 0.62 (0.16) | 0.316 | 0.11 | 0.48 | 0.12 | 26.72 | 0.33 | 53.23 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Raymond, F.; Lussier, B.; Dugas, F.; Charbonneau, M.; Croteau, F.; Kennedy, C.; Berryman, N. Using Portable Force Plates to Assess Vertical Jump Performance: A Metrological Appraisal. Sports 2018, 6, 149. https://doi.org/10.3390/sports6040149
Raymond F, Lussier B, Dugas F, Charbonneau M, Croteau F, Kennedy C, Berryman N. Using Portable Force Plates to Assess Vertical Jump Performance: A Metrological Appraisal. Sports. 2018; 6(4):149. https://doi.org/10.3390/sports6040149
Chicago/Turabian StyleRaymond, François, Benoit Lussier, François Dugas, Mathieu Charbonneau, Félix Croteau, Cory Kennedy, and Nicolas Berryman. 2018. "Using Portable Force Plates to Assess Vertical Jump Performance: A Metrological Appraisal" Sports 6, no. 4: 149. https://doi.org/10.3390/sports6040149
APA StyleRaymond, F., Lussier, B., Dugas, F., Charbonneau, M., Croteau, F., Kennedy, C., & Berryman, N. (2018). Using Portable Force Plates to Assess Vertical Jump Performance: A Metrological Appraisal. Sports, 6(4), 149. https://doi.org/10.3390/sports6040149





