Reproducibility of Blood Lactate Concentration Rate under Isokinetic Force Loads
Abstract
:1. Introduction
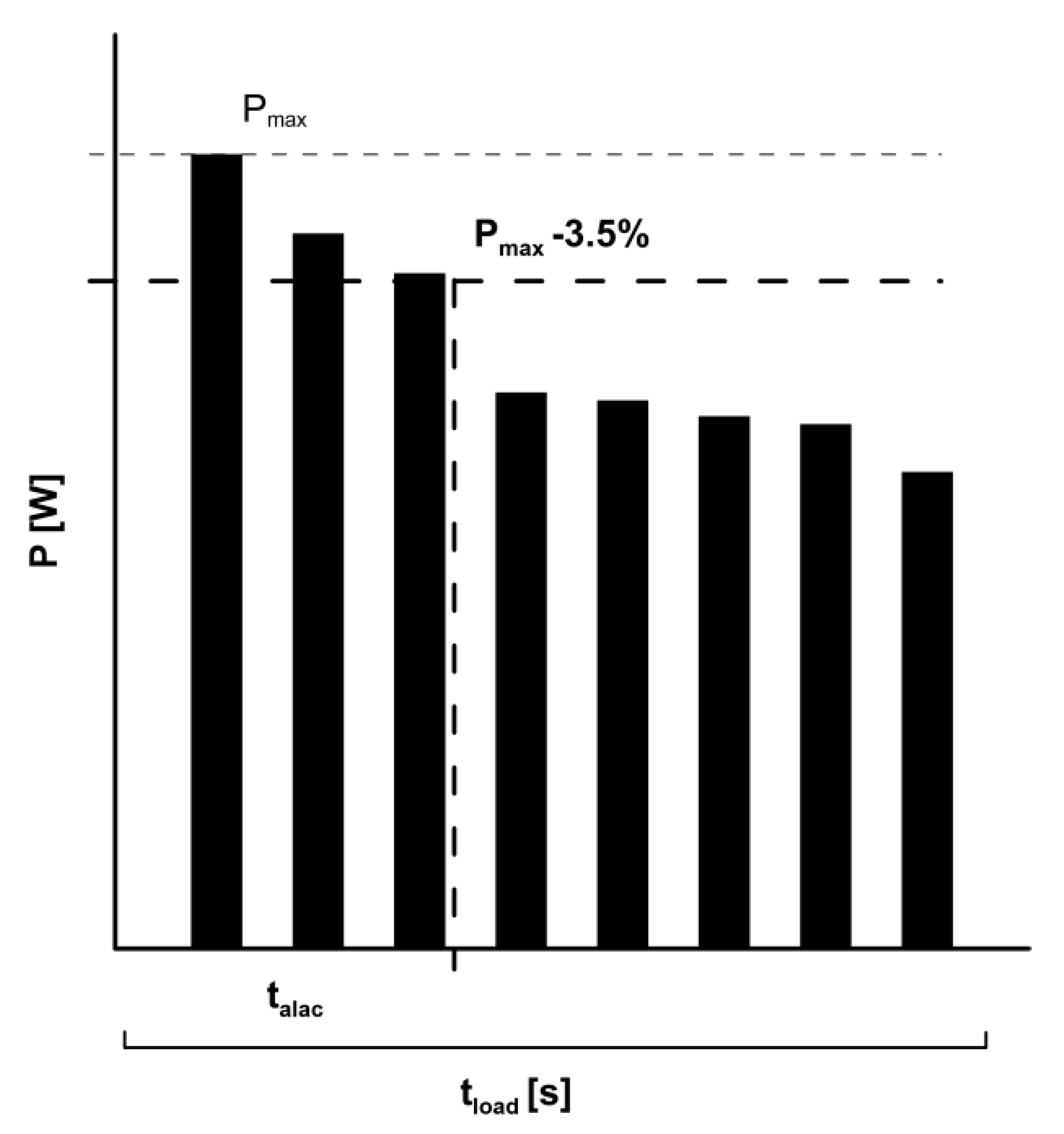
2. Materials and Methods
3. Results
3.1. T-Tests and Correlations
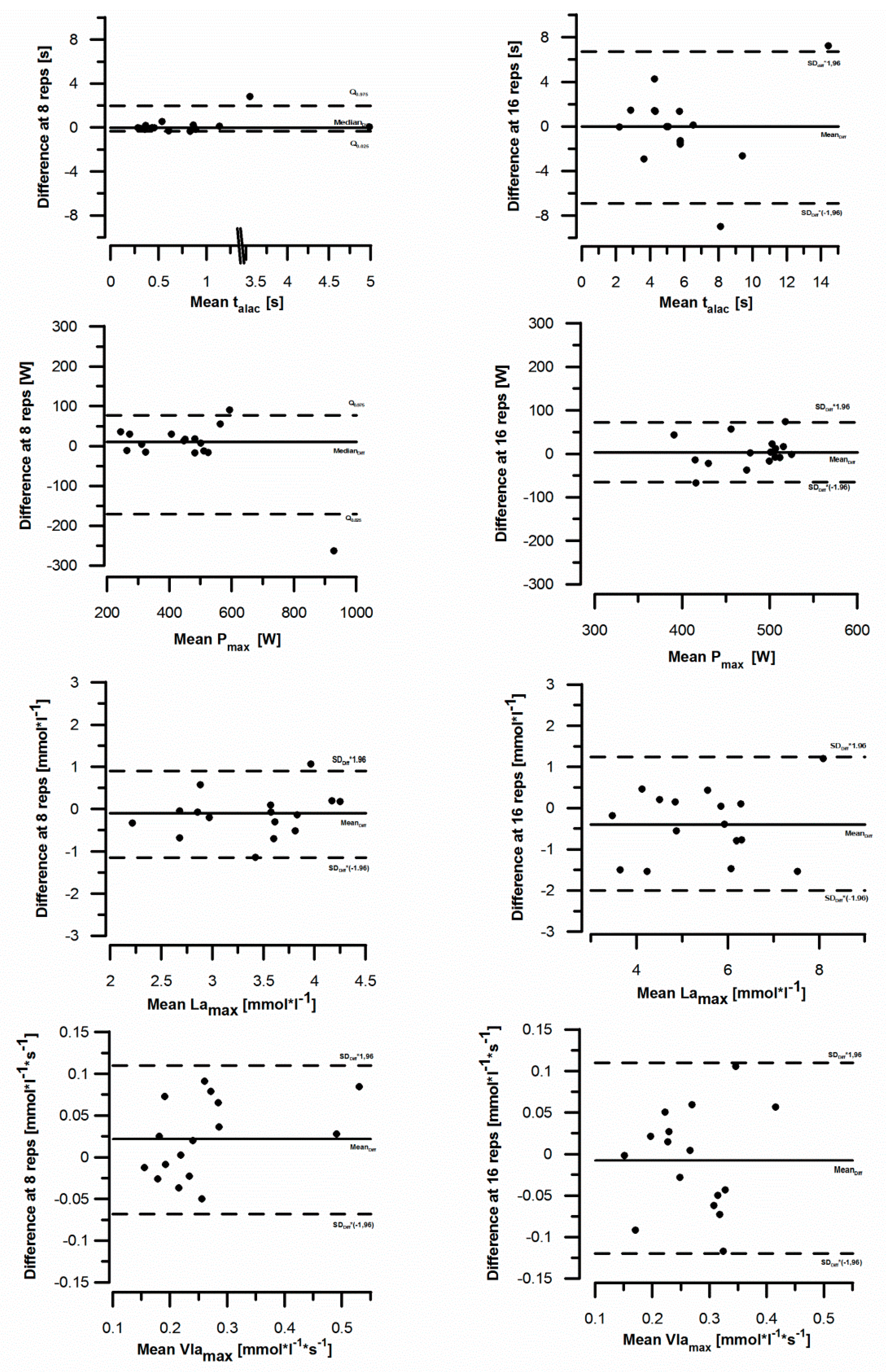
3.2. Variability of Measurement
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Beneke, R.; Wittekind, A.; Muhling, M.; Bleif, I.; Leithauser, R.M. Lactate response to short term exercise with elevated starting levels. Eur. J. Appl. Physiol. 2010, 110, 215–218. [Google Scholar] [CrossRef] [PubMed]
- Mader, A. Aussagekraft der Laktatleistungskurve in Kombination mit anaeroben Tests zur Bestimmung der Stoffwechselkapazität. In Stellenwert der Laktatbestimmung in der Leistungsdiagnostik: 32 Tabellen; Clasing, D., Ed.; G. Fischer: Stuttgart, Germany, 1994; pp. 133–152. [Google Scholar]
- Heck, H.; Schulz, H. Diagnostics of anaerobic power and capacity. Dtsch. Z. Sportmed. 2002, 53, 202–212. [Google Scholar] [CrossRef]
- Vandewalle, H.; Peres, G.; Monod, H. Standard anaerobic exercise tests. Sports Med. 1987, 4, 268–289. [Google Scholar] [CrossRef] [PubMed]
- Adam, J.; Ohmichen, M.; Ohmichen, E.; Rother, J.; Müller, U.M.; Hauser, T.; Schulz, H. Reliability of the calculated maximal lactate steady state in amateur cyclists. Biol. Sport 2015, 32, 97–102. [Google Scholar] [CrossRef] [PubMed]
- Burgess, K.; Holt, T.; Munro, S.; Swinton, P. Reliability and validity of the running anaerobic sprint test (RAST) in soccer players. J. Trainology 2016, 5, 24–29. [Google Scholar] [CrossRef] [Green Version]
- Hauser, T. Einfluss der Belastungsdauer bei Sprintbelastungen auf die Laktatbildungsrate. Dtsch. Z. Sportmed. 2009, 60, 177. [Google Scholar]
- Hislop, H.J.; Perrine, J.J. The isokinetic concept of exercise. Phys. Ther. 1967, 47, 114–117. [Google Scholar] [CrossRef] [PubMed]
- Nitzsche, N.; Baumgärtel, L.; Schulz, H. Comparison of maximum lactate formation rates in ergometer sprint and maximum strength loads. Dtsch. Z. Sportmed. 2018, 2018, 13–18. [Google Scholar] [CrossRef]
- Nitzsche, N.; Zschäbitz, D.; Baumgärtel, L.; Schulz, H. The effect of isokinetic resistance load on glycolysis rate. In Book of Abstracts, Proceedings of the 22nd Annual Congress of the European College of Sport Science, MetropolisRuhr, Germany, 5–8 July 2017; Ferrauti, A., Platen, P., Grimminger-Seidensticker, E., Jaitner, T., Bartmus, U., Becher, L., de Marées, M., Mühlbauer, T., Schauerte, A., Wiewelhove, T., et al., Eds.; Bochumer Universitätsverlag Westdeutscher Universitätsverlag: Bochum, Germany, 2017; Volume 558. [Google Scholar]
- Bosquet, L.; Maquet, D.; Forthomme, B.; Nowak, N.; Lehance, C.; Croisier, J.-L. Effect of the lengthening of the protocol on the reliability of muscle fatigue indicators. Int. J. Sports Med. 2010, 31, 82–88. [Google Scholar] [CrossRef] [PubMed]
- Guilhem, G.; Giroux, C.; Couturier, A.; Maffiuletti, N.A. Validity of trunk extensor and flexor torque measurements using isokinetic dynamometry. J. Electromyogr. Kinesiol. 2014, 24, 986–993. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gleeson, N.P.; Mercer, T.H. Reproducibility of isokinetic leg strength and endurance characteristics of adult men and women. Eur. J. Appl. Physiol. 1992, 65, 221–228. [Google Scholar] [CrossRef]
- Impellizzeri, F.; Bizzini, M.; Rampinini, E.; Cereda, F.; Maffiuletti, N. Reliability of isokinetic strength imbalance ratios measured using the Cybex NORM dynamometer. Clin. Physiol. Funct. Imaging 2008, 28, 113–119. [Google Scholar] [CrossRef] [PubMed]
- Maffiuletti, N.A.; Bizzini, M.; Desbrosses, K.; Babault, N.; Munzinger, U. Reliability of knee extension and flexion measurements using the Con-Trex isokinetic dynamometer. Clin. Physiol. Funct. Imaging 2007, 27, 346–353. [Google Scholar] [CrossRef] [PubMed]
- Bosquet, L.; Gouadec, K.; Berryman, N.; Duclos, C.; Gremeaux, V.; Croisier, J.L. The Total Work Measured During a High Intensity Isokinetic Fatigue Test Is Associated with Anaerobic Work Capacity. J. Sport Sci. Med. 2016, 15, 126–130. [Google Scholar]
- Nummela, A.; Alberts, M.; Rijntjes, R.P.; Luhtanen, P.; Rusko, H. Reliability and validity of the maximal anaerobic running test. Int. J. Sports Med. 1996, 17 (Suppl. 2), S97–S102. [Google Scholar] [CrossRef] [PubMed]
- Hauser, T.; Adam, J.; Schulz, H. Comparison of calculated and experimental power in maximal lactate-steady state during cycling. Theor. Biol. Med. Model. 2014, 11, 25. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cohen, L.; Manion, L.; Morrison, K.R.B. Research Methods in Education, 5th ed.; RoutledgeFalmer: London, UK, 2005. [Google Scholar]
- Bland, J.M.; Altman, D.G. Statistical methods for assessing agreement between two methods of clinical measurement. Lancet 1986, 1, 307–310. [Google Scholar] [CrossRef]
- Bland, J.M.; Altman, D.G. Measuring agreement in method comparison studies. Stat. Methods Med. Res. 1999, 8, 135–160. [Google Scholar] [CrossRef] [PubMed]
- Bland, J.M.; Altman, D.G. Measurement error. BMJ 1996, 312, 1654. [Google Scholar] [CrossRef] [PubMed]
- Di Prampero, P.E. Energetics of muscular exercise. Rev. Physiol. Biochem. Pharmacol. 1981, 89, 143–222. [Google Scholar] [PubMed]
- Wittekind, A.L.; Micklewright, D.; Beneke, R. Teleoanticipation in all-out short-duration cycling. Br. J. Sports Med. 2011, 45, 114–119. [Google Scholar] [CrossRef] [PubMed]
- Mikulski, T.; Ziemba, A.; Nazar, K. Influence of body carbohydrate store modification on catecholamine and lactate responses to graded exercise in sedentary and physically active subjects. J. Physiol. Pharmacol. 2008, 59, 603–616. [Google Scholar] [PubMed]
- Havemann, L.; West, S.J.; Goedecke, J.H.; Macdonald, I.A.; St Clair Gibson, A.; Noakes, T.D.; Lambert, E.V. Fat adaptation followed by carbohydrate loading compromises high-intensity sprint performance. J. Appl. Physiol. (1985) 2006, 100, 194–202. [Google Scholar] [CrossRef] [PubMed] [Green Version]
| Groups | Group 1 with 8 reps | Group 2 with 16 reps | ||||
|---|---|---|---|---|---|---|
| Parameter | Test 1 | Test 2 | r | Test 1 | Test 2 | r |
| RL (mmol L−1) | 0.86 ± 0.22 | 0.91 ± 0.35 | 0.83 ± 0.41 | 0.76 ± 0.18 | ||
| tload(s) | 12.1 ± 1.02 | 11.5 ± 1.03 | 23.1 ± 0.31 | 23.0 ± 0.28 | ||
| Mmax-mean (Nm) | 143.8 ± 43.3 | 145.5 ± 45.1 | 0.983 (0.000) | 143.8 ± 16.9 | 142.6 ± 18.9 | 0.840 (0.000) |
| Pmax-mean (Watt) | 424.6 ± 182.2 | 424.9 ± 142.6 | 0.946 (0.000) | 419.6 ± 40.2 | 419.9 ± 40.1 | 0.753 (0.001) |
| Pmax (Watt) | 457.9 ± 193.5 | 455.9 ± 146.9 | 0.938 (0.000) | 476.0 ± 42.8 | 479.7 ± 49.9 | 0.726 (0.001) |
| Lamax (mmol L−1) | 3.44 ± 0.59 | 3.31 ± 0.70 | 0.688 (0.002) | 5.66 ± 1.33 | 5.27 ± 1.44 | 0.821 (0.000) |
| Lamax (mmol L−1 s−1) | 0.25 ± 0.11 | 0.27 ± 0.11 | 0.721 (0.002) | 0.27 ± 0.07 | 0.26 ± 0.07 | 0.677 (0.004) |
| talac (s) | 0.97 ± 1.1 | 1.1 ± 1.5 | 0.665 (0.005) | 5.87 ± 3.1 | 5.76 ± 3.6 | 0.482 (0.059) |
| Parameter | Group 1 | Group 2 |
|---|---|---|
| Pmax (Watt) | 51.75 (11.33%) | 24.05 (5.03%) |
| Lamax (mmol L−1) | 0.37 (10.92%) | 0.63 (11.54%) |
| Lamax (mmol L−1 s−1) | 0.04 (13.47%) | 0.04 (15.78%) |
| talac (s) | 0.52 (49.28%) | 2.38 (40.9%) |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nitzsche, N.; Baumgärtel, L.; Maiwald, C.; Schulz, H. Reproducibility of Blood Lactate Concentration Rate under Isokinetic Force Loads. Sports 2018, 6, 150. https://doi.org/10.3390/sports6040150
Nitzsche N, Baumgärtel L, Maiwald C, Schulz H. Reproducibility of Blood Lactate Concentration Rate under Isokinetic Force Loads. Sports. 2018; 6(4):150. https://doi.org/10.3390/sports6040150
Chicago/Turabian StyleNitzsche, Nico, Lutz Baumgärtel, Christian Maiwald, and Henry Schulz. 2018. "Reproducibility of Blood Lactate Concentration Rate under Isokinetic Force Loads" Sports 6, no. 4: 150. https://doi.org/10.3390/sports6040150
APA StyleNitzsche, N., Baumgärtel, L., Maiwald, C., & Schulz, H. (2018). Reproducibility of Blood Lactate Concentration Rate under Isokinetic Force Loads. Sports, 6(4), 150. https://doi.org/10.3390/sports6040150





