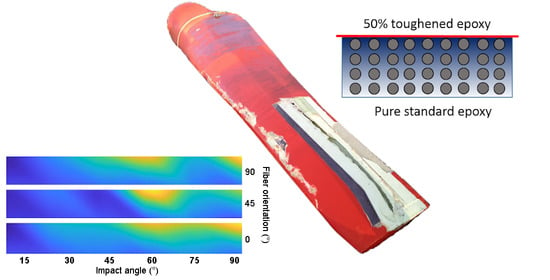
Erosion Mapping of Through-Thickness Toughened Powder Epoxy Gradient Glass-Fiber-Reinforced Polymer (GFRP) Plates for Tidal Turbine Blades
Abstract
1. Introduction
2. Materials and Methods
2.1. General Overview of the Different Experimental Procedures for Erosion and Mechanical Manufacturing and Testing
- First, a screening of mechanical properties was carried out to select the best experimental toughened epoxy for use as the composite matrix for the surface layer;
- Second, a mechanical compatibility study between the standard and toughened epoxies was carried out to determine the cohesive ability between the two resin systems;
- Third, an erosion study was completed using pure standard epoxy laminates and through-thickness gradient epoxy laminates.
2.2. Fibers
2.3. Epoxy Powders
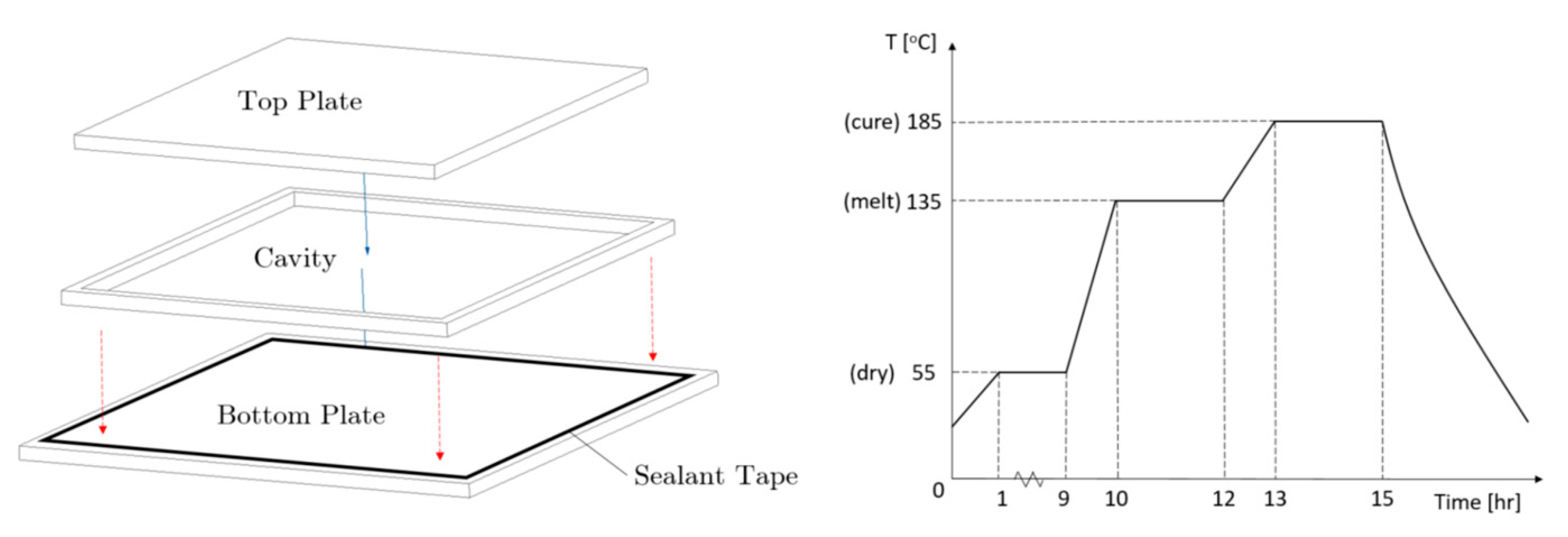
2.4. GFRP Manufacturing Procedure
2.5. Mechanical Test Procedure
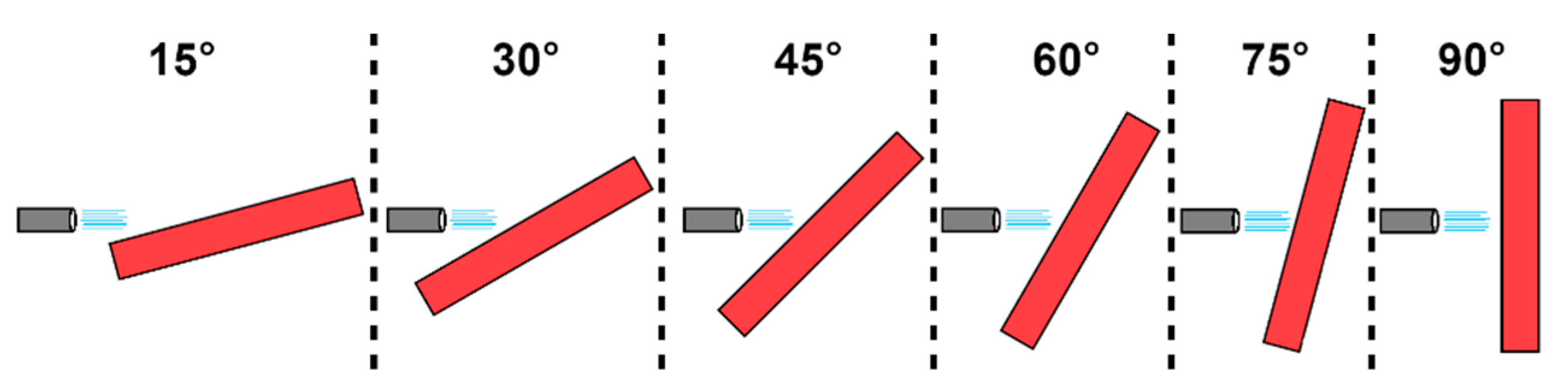
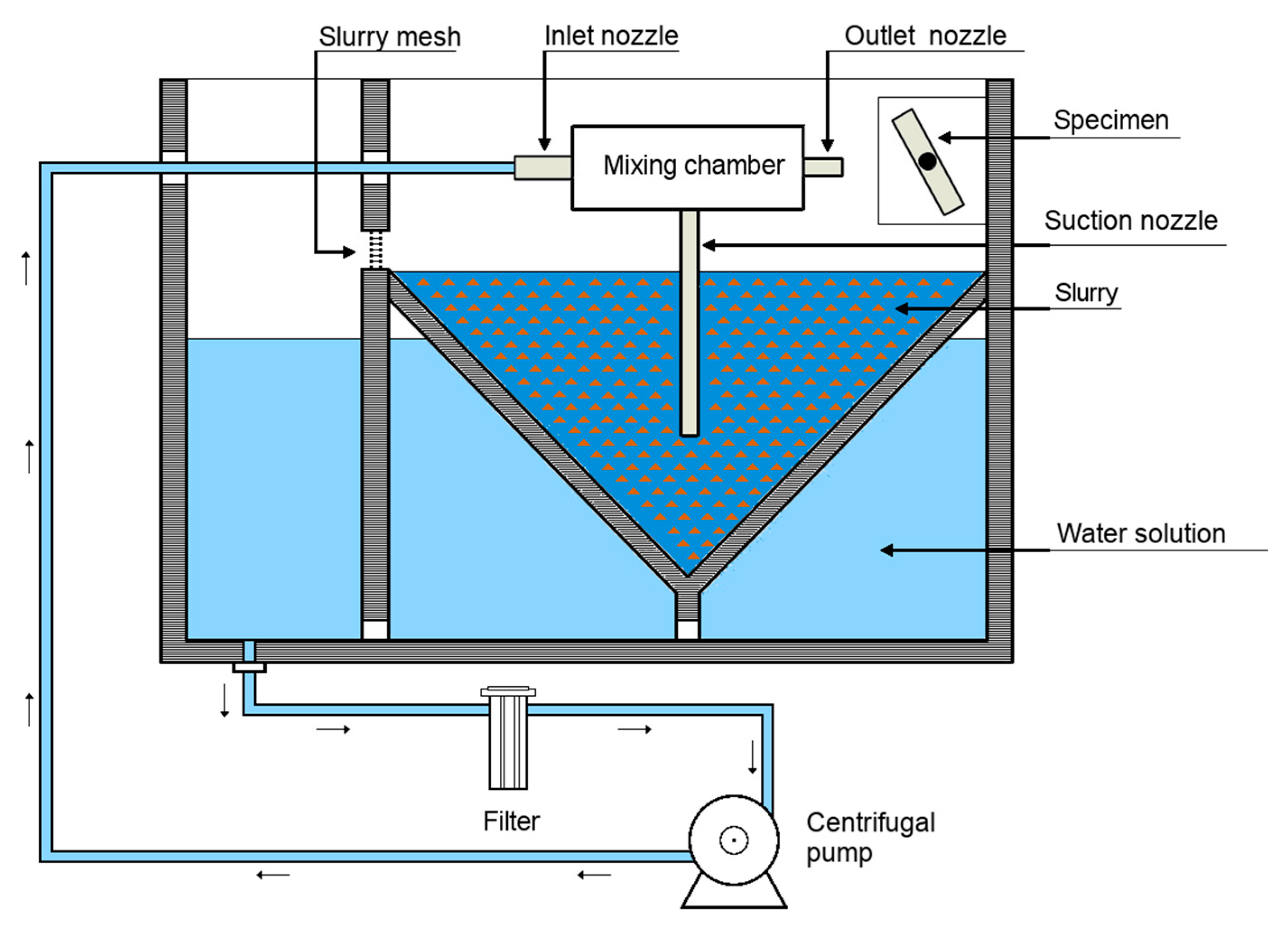
2.6. Erosion Test Protocol
2.7. Sand Analysis
3. Results and Discussion
3.1. Epoxy Powders Calorimetry and Fiber Volume Fraction Characterization
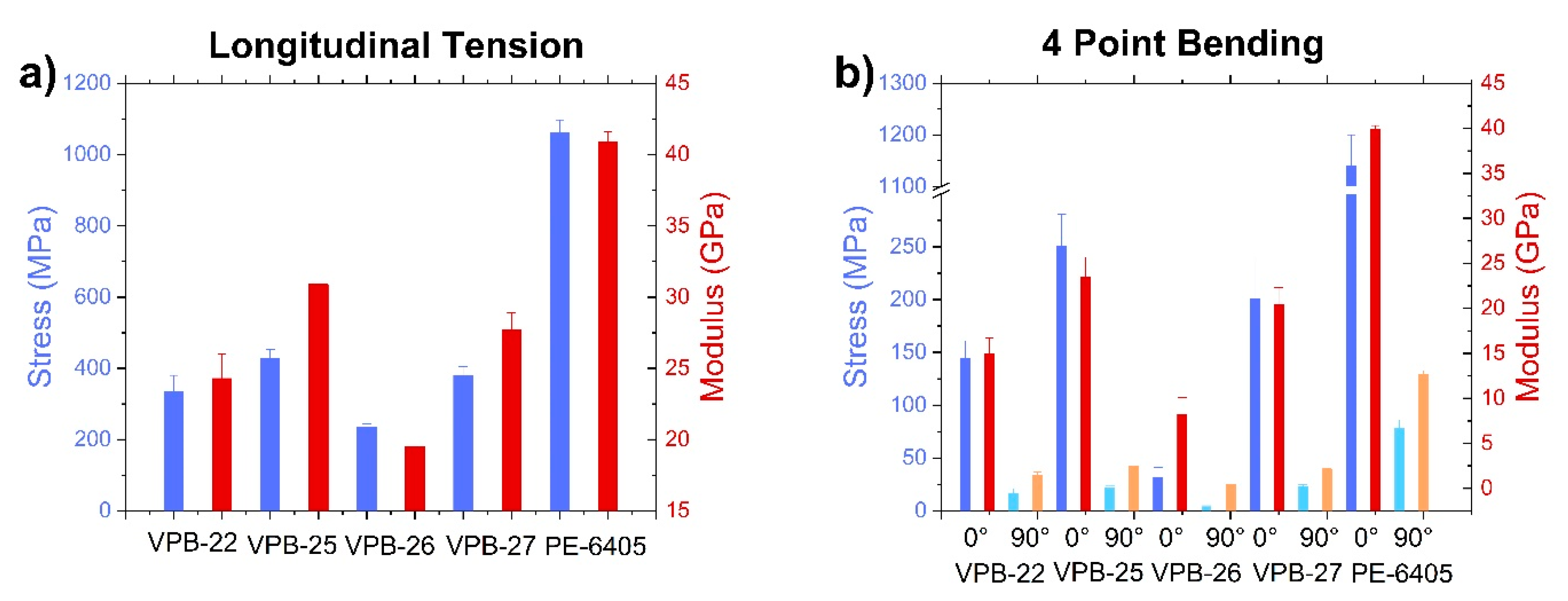
3.2. Initial Toughened Powder Screening
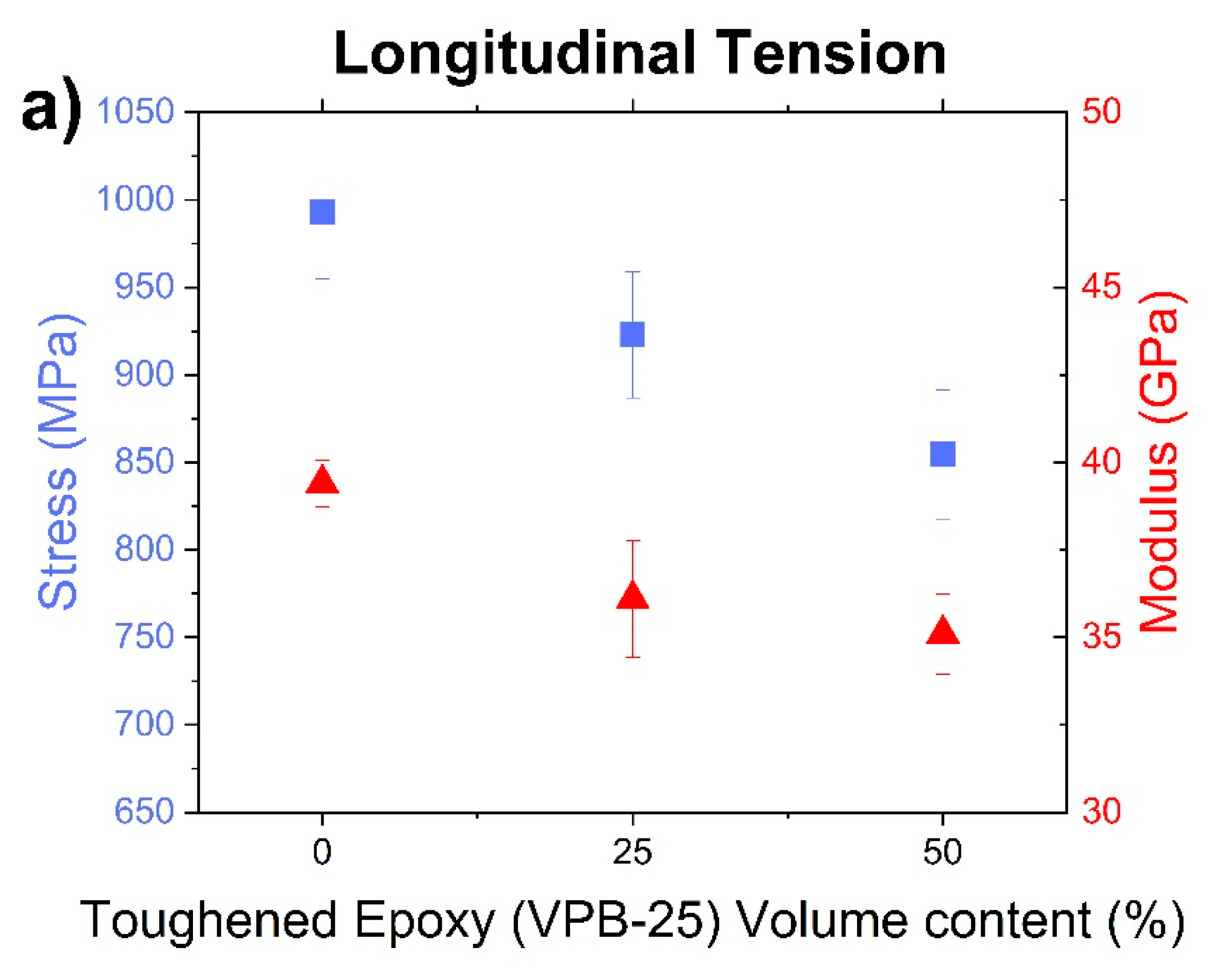
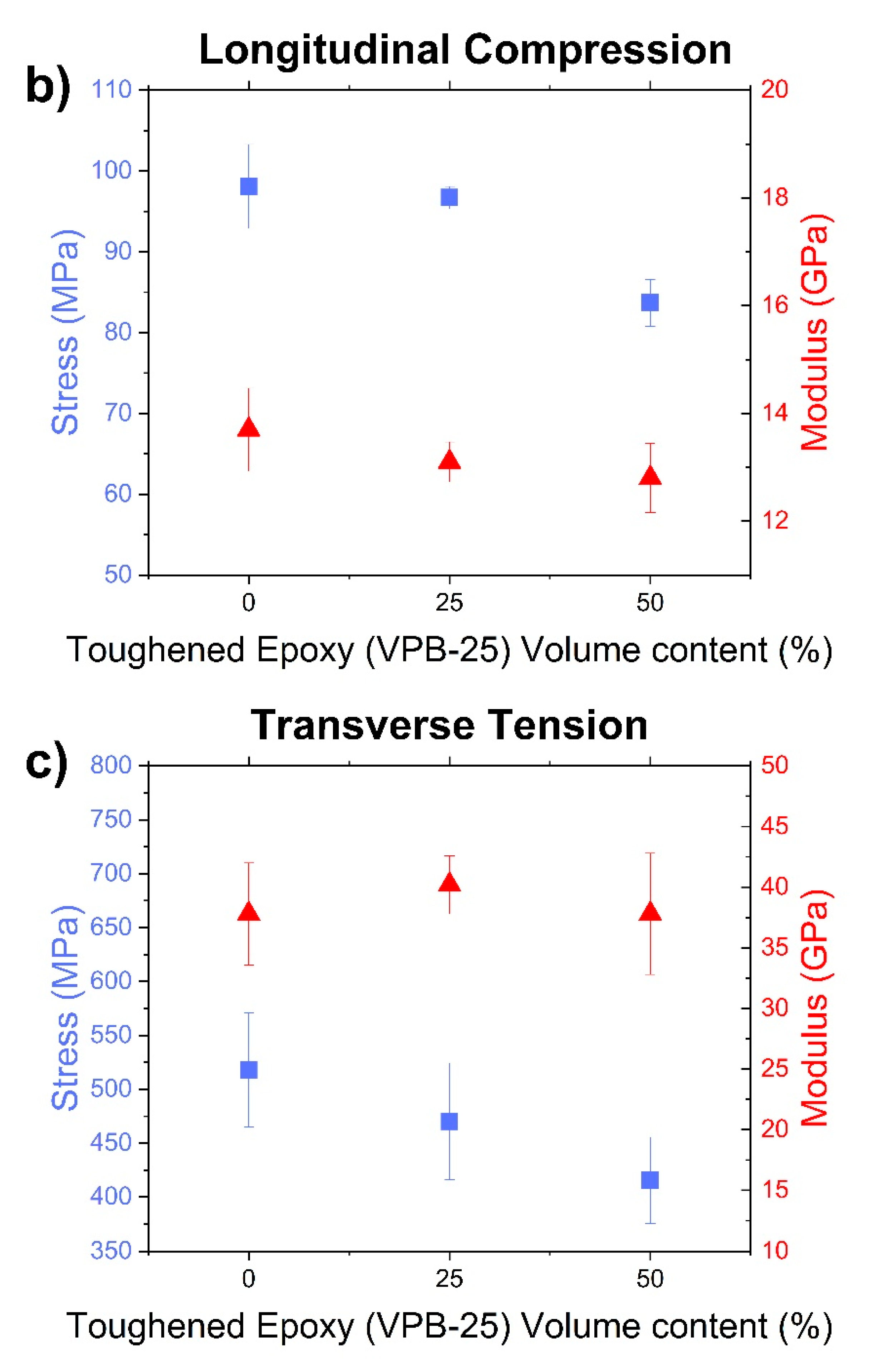
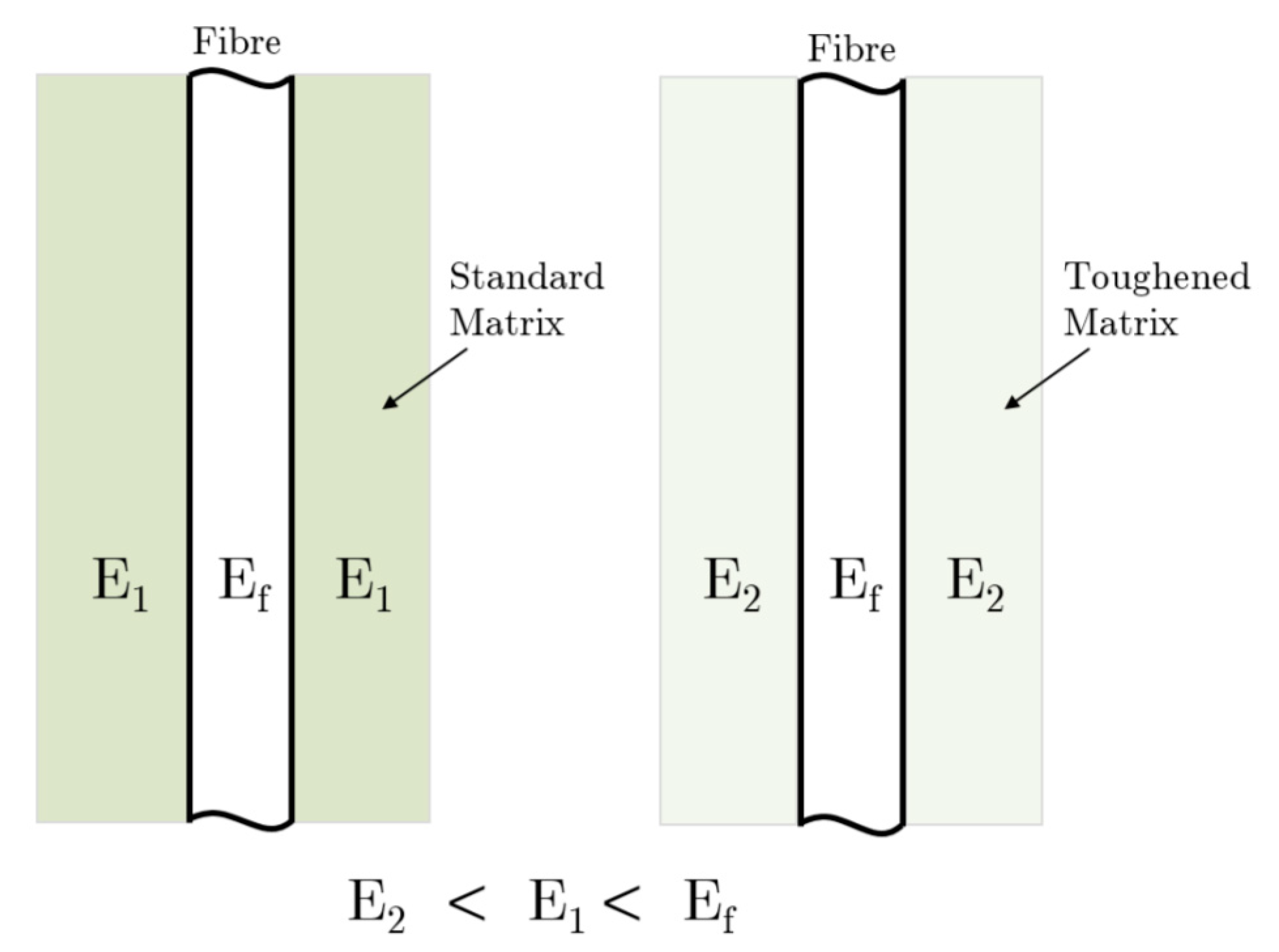
3.3. Toughened and Standard Epoxy Compatibility Study
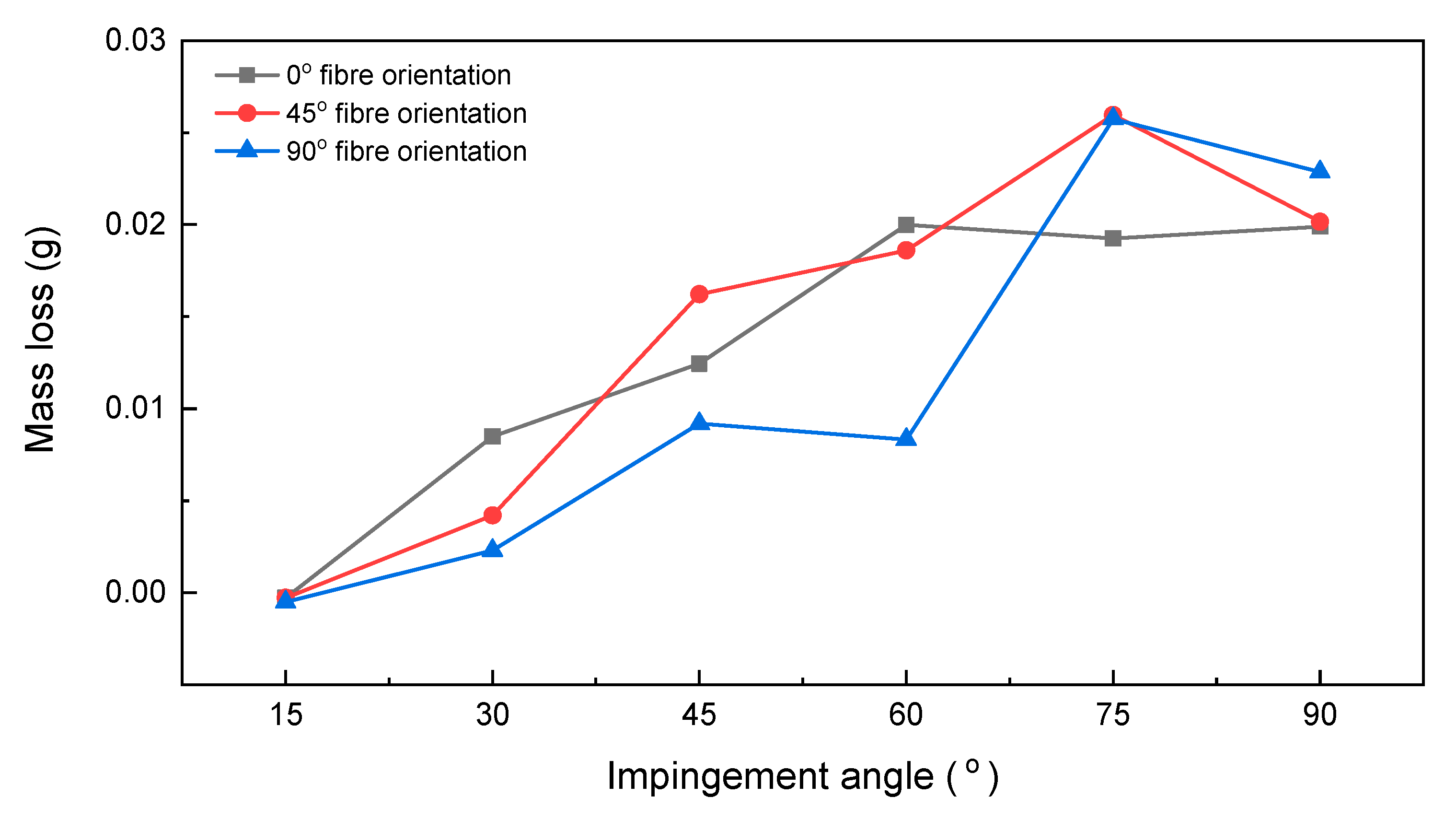
3.4. Slurry Erosion Tests
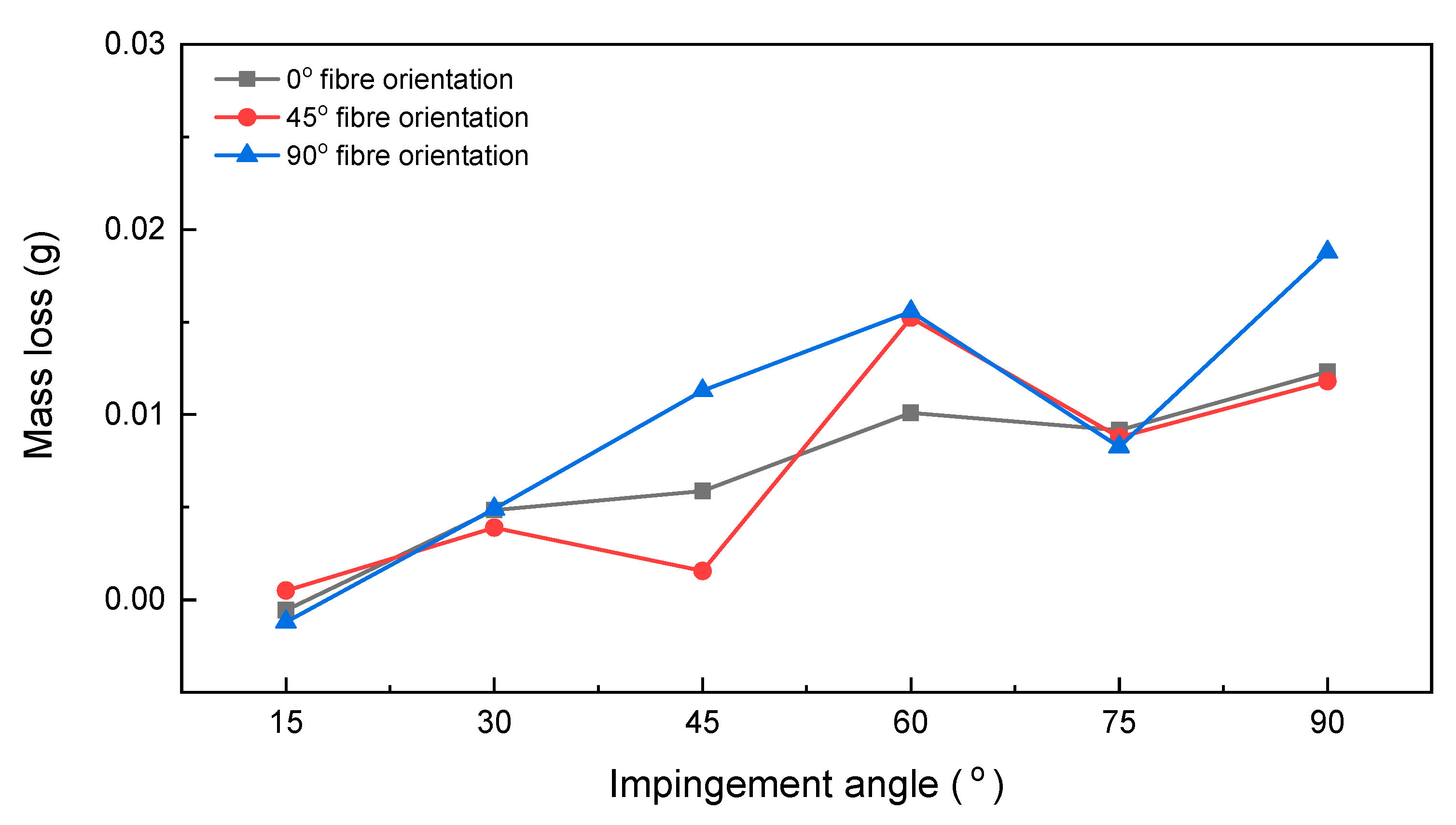
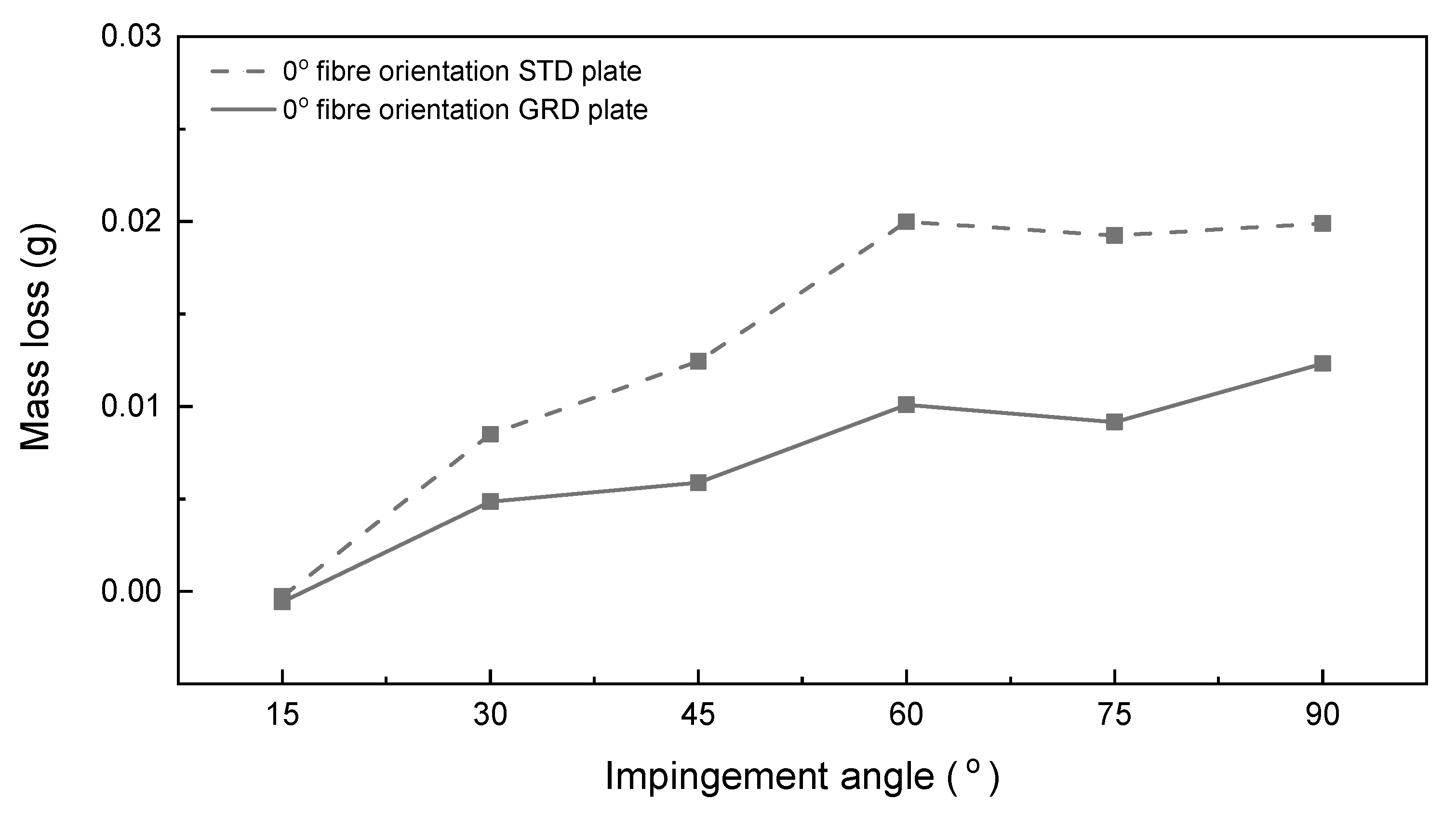
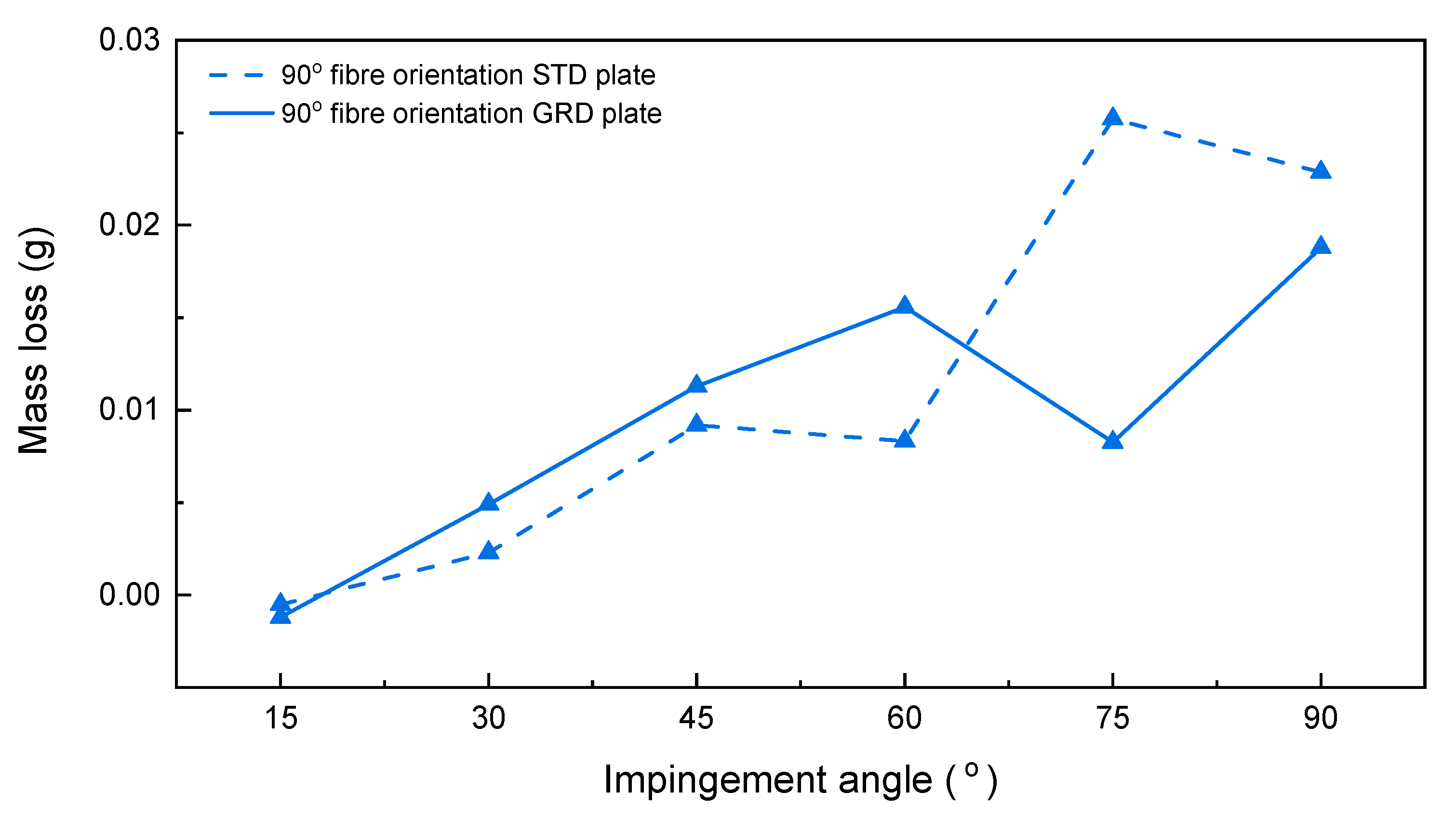
3.5. Standard versus Gradient Plate Comparison
3.6. Surface Optical Analysis
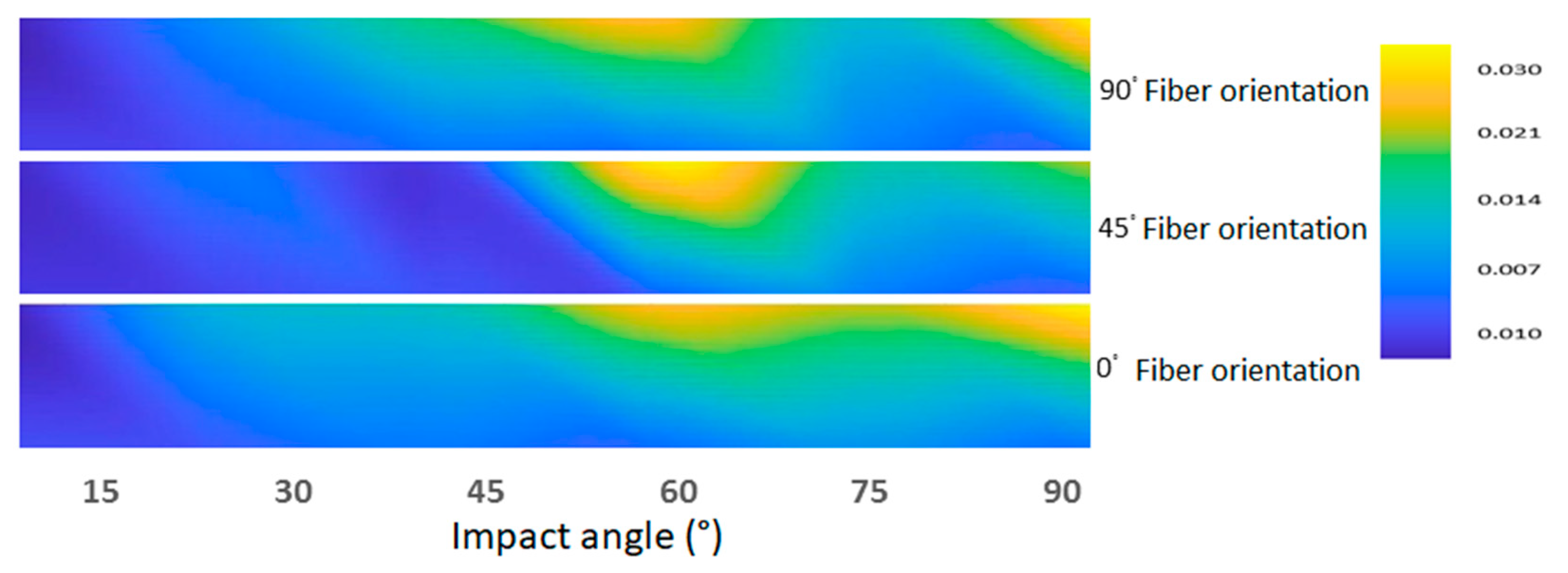
3.7. Erosion Wastage Maps
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- A Oceans of Energy—European Ocean Energy Roadmap 2010–2050. Available online: https://www.icoe-conference.com/publication/oceans_of_energy_european_ocean_energy_roadmap_2010_2050/ (accessed on 22 February 2021).
- Ocean Energy Europe. Powering Homes Today, Powering Nations Tomorrow. 2019. Available online: https://www.oceanenergy-europe.eu/wp-content/uploads/2019/04/ETIP-Ocean-Integrated-Strategy-2019-LR.pdf (accessed on 22 February 2021).
- Giorgi, S.; Ringwood, J.V. Can tidal current energy provide base load? Energies 2013, 6, 2840–2858. [Google Scholar] [CrossRef]
- Siemens Gamesa Renewable Energy. Available online: https://www.siemensgamesa.com/en-int/about-us/company-history (accessed on 22 February 2021).
- Orbital Marine Power. Available online: https://orbitalmarine.com/index.php (accessed on 22 February 2021).
- Alan Walker. The Future of Marine Renewables; The Royal Academy of Engineering: London, UK, 2011. [Google Scholar]
- Arany, L.; Bhattacharya, S.; Macdonald, J.; Hogan, S.J. Simplified critical mudline bending moment spectra of offshore wind turbine support structures. Wind Energy 2015, 18, 2171–2197. [Google Scholar] [CrossRef]
- Kennedy, C.R.; Leen, S.B.; Ó Brádaigh, C.M. A preliminary design methodology for fatigue life prediction of polymer composites for tidal turbine blades. Proc. Inst. Mech. Eng. Part L J. Mater. Des. Appl. 2012, 226, 203–218. [Google Scholar] [CrossRef]
- Grogan, D.M.; Leen, S.B.; Kennedy, C.R.; Ó Brádaigh, C.M. Design of composite tidal turbine blades. Renew. Energy 2013, 57, 151–162. [Google Scholar] [CrossRef]
- Kennedy, C.R.; Jaksic, V.; Leen, S.B.; Ó Brádaigh, C.M. Fatigue life of pitch-and stall-regulated composite tidal turbine blades. Renew. Energy 2018, 121, 688–699. [Google Scholar] [CrossRef]
- Fagan, E.M.; Leen, S.B.; Kennedy, C.R.; Goggins, J. Finite element based damage assessment of composite tidal turbine blades. In Proceedings of the Journal of Physics: Conference Series; IOP Publishing: Bristol, UK, 2015; Volume 628, p. 12106. [Google Scholar]
- Fagan, E.M.; Kennedy, C.R.; Leen, S.B.; Goggins, J. Damage mechanics based design methodology for tidal current turbine composite blades. Renew. Energy 2016, 97, 358–372. [Google Scholar] [CrossRef]
- Fagan, E.; Wallace, F.; Jiang, Y.; Kazemi, A.; Goggins, J. Design and testing of a full-scale 2 MW tidal turbine blade. Engrxiv 2020. [Google Scholar] [CrossRef]
- Alam, P.; Robert, C.; Ó Brádaigh, C.M. Tidal turbine blade composites-A review on the effects of hygrothermal aging on the properties of CFRP. Compos. Part B Eng. 2018, 149, 248–259. [Google Scholar] [CrossRef]
- Kennedy, C.R.; Leen, S.B.; Ó Brádaigh, C.M. Immersed fatigue performance of glass fibre-reinforced composites for tidal turbine blade applications. J. Bio Tribo Corros. 2016, 2, 12. [Google Scholar] [CrossRef]
- Tual, N.; Carrere, N.; Davies, P.; Bonnemains, T.; Lolive, E. Characterization of sea water ageing effects on mechanical properties of carbon/epoxy composites for tidal turbine blades. Compos. Part A Appl. Sci. Manuf. 2015, 78, 380–389. [Google Scholar] [CrossRef]
- Humeau, C.; Davies, P.; Jacquemin, F. An experimental study of water diffusion in carbon/epoxy composites under static tensile stress. Compos. Part A Appl. Sci. Manuf. 2018, 107, 94–104. [Google Scholar] [CrossRef]
- Davies, P. Towards More Representative Accelerated Aging of Marine Composites. In Advances in Thick Section Composite and Sandwich Structures; Springer: Berlin/Heidelberg, Germany, 2020; pp. 507–527. [Google Scholar]
- Guobys, R.; Rodríguez, Á.; Chernin, L. Cavitation erosion of glass fibre reinforced polymer composites with unidirectional layup. Compos. Part B Eng. 2019, 177, 107374. [Google Scholar] [CrossRef]
- Lawn, B.R.; Deng, Y.; Miranda, P.; Pajares, A.; Chai, H.; Kim, D.K. Overview: Damage in brittle layer structures from concentrated loads. J. Mater. Res. 2002, 17, 3019–3036. [Google Scholar] [CrossRef]
- Wang, A.G.; Hutchings, I.M. Wear of alumina fibre–aluminium metal matrix composites by two-body abrasion. Mater. Sci. Technol. 1989, 5, 71–76. [Google Scholar] [CrossRef]
- Jana, B.D.; Stack, M.M. A note on threshold velocity criteria for modelling the solid particle erosion of WC/Co MMCs. Wear 2011, 270, 439–445. [Google Scholar] [CrossRef][Green Version]
- Ahamed, R.A.R.; Johnstone, C.M.; Stack, M.M. Impact angle effects on erosion maps of GFRP: Applications to tidal turbines. J. Bio Tribo Corros. 2016, 2, 14. [Google Scholar] [CrossRef]
- Maguire, J.M.; Nayak, K.; Ó Brádaigh, C.M. Characterisation of epoxy powders for processing thick-section composite structures. Mater. Des. 2018, 139, 112–121. [Google Scholar] [CrossRef]
- Robert, C.; Pecur, T.; Maguire, J.M.; Lafferty, A.D.; McCarthy, E.D.; Ó Brádaigh, C.M. A novel powder-epoxy towpregging line for wind and tidal turbine blades. Compos. Part B Eng. 2020, 203, 108443. [Google Scholar] [CrossRef]
- Flanagan, T.; Maguire, J.; Ó Brádaigh, C.M.; Mayorga, P.; Doyle, A. Smart affordable composite blades for tidal energy. In Proceedings of the 11th European Wave and Tidal Energy Conference EWTEC2015, Nantes, France, 6–11 September 2015; pp. 6–11. [Google Scholar]
- Glennon, T.; Flanagan, T.; Doyle, A.; Kelly, G.; Ó Brádaigh, C.M.; Finnegan, W. Development of Novel Manufacturing Techniques for Composite Tidal Turbine Blades. In Proceedings of the SAMPE Europe Conference, Southampton, UK, 11–13 September 2018. [Google Scholar]
- Davies, P.; Arhant, M. Fatigue behaviour of acrylic matrix composites: Influence of seawater. Appl. Compos. Mater. 2019, 26, 507–518. [Google Scholar] [CrossRef]
- Sathishkumar, T.P.; Satheeshkumar, S.; Naveen, J. Glass fiber-reinforced polymer composites—A review. J. Reinf. Plast. Compos. 2014, 33, 1258–1275. [Google Scholar] [CrossRef]
- Rubino, F.; Nisticò, A.; Tucci, F.; Carlone, P. Marine Application of Fiber Reinforced Composites: A Review. J. Mar. Sci. Eng. 2020, 8, 26. [Google Scholar] [CrossRef]
- Rasool, G.; Johnstone, C.; Stack, M.M. Tribology of tidal turbine blades: Impact angle effects on erosion of polymeric coatings in sea water conditions. In Proceedings of the 3rd Asian Wave and Tidal Energy Conference (AWTEC 2016), Singapore, 24–28 October 2016; Volume 2, pp. 1016–1033. [Google Scholar]
- Bradbury, S.; Joy, D.C.; Ford, B.J. Scanning Electron Microscope. 2019. Available online: https://www.britannica.com/technology/scanning-electron-microscope (accessed on 22 February 2021).
- Zu, J.B.; Hutchings, I.M.; Burstein, G.T. Design of a slurry erosion test rig. Wear 1990, 140, 331–344. [Google Scholar] [CrossRef]
- Riley, N.A. Projection sphericity. J. Sediment. Res. 1941, 11, 94–95. [Google Scholar]
- MacLeod, N. Geometric morphometrics and geological shape-classification systems. Earth Sci. Rev. 2002, 59, 27–47. [Google Scholar] [CrossRef]
- Arabnejad, H.; Shirazi, S.A.; McLaury, B.S.; Subramani, H.J.; Rhyne, L.D. The effect of erodent particle hardness on the erosion of stainless steel. Wear 2015, 332–333, 1098–1103. [Google Scholar] [CrossRef]
- Levy, A.V.; Chik, P. The effects of erodent composition and shape on the erosion of steel. Wear 1983, 89, 151–162. [Google Scholar] [CrossRef]
- Tilly, G.P. A two stage mechanism of ductile erosion. Wear 1973, 23, 87–96. [Google Scholar] [CrossRef]
- Holmberg, K.; Laukkanen, A.; Ronkainen, H.; Wallin, K.; Varjus, S.; Koskinen, J. Tribological contact analysis of a rigid ball sliding on a hard coated surface: Part II: Material deformations, influence of coating thickness and Young’s modulus. Surf. Coat. Technol. 2006, 200, 3810–3823. [Google Scholar] [CrossRef]
- Reddy, M.K.; Babu, V.S.; Srinadh, K.V. Investigation on effect of fibre volume and fiber orientation on erosive wear behavior of carbon fiber reinforced epoxy (CFRP) composites. Mater. Sci. Forum 2019, 969, 134–139. [Google Scholar] [CrossRef]
- Liu, B.; Bao, L.; Xu, A. Effect of fabric orientation and impact angle on the erosion behavior of high-performance thermoplastic composites reinforced with ductile fabric. Wear 2016, 352, 24–30. [Google Scholar] [CrossRef]
- Fouad, Y.; El-Meniawi, M.; Afifi, A. Erosion behaviour of epoxy based unidirectionl (GFRP) composite materials. Alexandria Eng. J. 2011, 50, 29–34. [Google Scholar] [CrossRef]
- Boggarapu, V.; Gujjala, R.; Ojha, S. A critical review on erosion wear characteristics of polymer matrix composites. Mater. Res. Express 2020, 7, 22002. [Google Scholar] [CrossRef]
- Sharifi, S.; Johnstone, C.; Stack, M.M. Tribological challenges of scaling up tidal turbine blades. In Proceedings of the 11th European Wave and Tidal Energy Conference (EWTEC2015), Nantes, France, 6–11 September 2015. [Google Scholar]
- Clark, H.M.; Burmeister, L.C. The influence of the squeeze film on particle impact velocities in erosion. Int. J. Impact Eng. 1992, 12, 415–426. [Google Scholar] [CrossRef]
- Rasool, G.; Stack, M.M. Some views on the mapping of erosion of coated composites in tidal turbine simulated conditions. Tribol. Trans. 2019, 62, 512–523. [Google Scholar] [CrossRef]







| Test Description | Nominal Sample Length (mm) | Nominal Sample Width (mm) | Nominal Sample Thickness (mm) |
|---|---|---|---|
| Tensile 0° and 90° (ISO 527-4 and ISO 527-5) | 250 | 25 | 2 |
| Compression 0° and 90° (ASTM D6641) | 140 | 13 | 2 |
| 4 point bending 90° 0° and (ISO 14125-Class III) | 60 | 15 | 2 |
| Fiber volume fraction (ASTM D2734) | 10 | 10 | 2 |
| Specimen Codename | Description |
|---|---|
| Std0 | GFRP standard plate with 0° surface fiber orientation |
| Std45 | GFRP standard plate with 45° surface fiber orientation |
| Std90 | GFRP standard plate with 90° surface fiber orientation |
| Grd0 | GFRP gradient plate with 0° surface fiber orientation |
| Grd45 | GFRP gradient plate with 45° surface fiber orientation |
| Grd90 | GFRP gradient plate with 90° surface fiber orientation |
| Parameter | Value |
|---|---|
| Impingement angle | 15°, 30°, 45°, 60°, 75°, 90° |
| Solutions | Salt and Sand |
| Salinity (wt %) | 3.5 |
| Sand concentration (wt %) | 3 |
| Test duration (min/sample) | 30 |
| Sand particle size (µm) | 300–600 |
| Impact velocity (ms−1) | 9.04 |
| Sample | Mass of Composite (g) | Mass of Fibers (g) | Volume of Composites (cm3) | Volume of Fibers (cm3) | FVF (%) |
|---|---|---|---|---|---|
| 25% Toughened (1) | 1.060 | 0.688 | 0.572 | 0.265 | 46.3 |
| 25% Toughened (2) | 1.103 | 0.723 | 0.592 | 0.278 | 47.0 |
| 50% Toughened (3) | 1.062 | 0.664 | 0.587 | 0.255 | 43.5 |
| 50% Toughened (4) | 1.371 | 0.865 | 0.754 | 0.333 | 44.1 |
| Impingement Angle | Defect Type |
|---|---|
| STD 0° fiber orientation | |
| 15° | Exposed fiber, fiber fracture, particle embedment |
| 30° | Fiber fracture |
| 45° | Fiber fracture |
| 60° | Fiber cracking |
| 75° | Particle embedment, matrix cutting, fiber fracture |
| 90° | Fiber fragmentation, fiber cracks |
| STD 45° fiber orientation | |
| 15° | Particle embedment, matrix cutting |
| 30° | Fiber fracture, fiber cracking |
| 45° | Fiber fragmentation |
| 60° | Matrix cutting, fiber fragmentation |
| 75° | Fiber fragmentation |
| 90° | Fiber fracture, fiber cracking |
| STD 90° fiber orientation | |
| 15° | Particle embedment |
| 30° | Matrix cutting, fiber exposure |
| 45° | Matrix debonding, fiber exposure, fiber cracking |
| 60° | Fiber cracking |
| 75° | Matrix cutting, fiber fracture |
| 90° | Matrix cutting, fiber fragmentation |
| GRD 0° fiber orientation | |
| 15° | Fiber exposure, fiber cracking, particle embedment |
| 30° | NaCl deposition, fiber cracking, matrix debonding |
| 45° | Matrix cutting, fiber exposure |
| 60° | Fiber cracking, matric cutting |
| 75° | Fiber cracking |
| 90° | Fiber fracture, matrix cutting |
| GRD 45° fiber orientation | |
| 15° | Particle embedment |
| 30° | Matrix debonding |
| 45° | Matrix cutting, matrix debonding |
| 60° | Fiber fracture |
| 75° | Matrix debonding, fiber cracking |
| 90° | Particle embedment, matrix cutting, fiber fracture |
| GRD 90° fiber orientation | |
| 15° | Fiber exposure, particle embedment |
| 30° | Matrix cutting, NaCl deposition |
| 45° | Fiber exposure, matrix debonding, |
| 60° | Fiber cracking, matrix cutting |
| 75° | Fiber fragmentation |
| 90° | Matrix cutting, Matrix debonding, fiber fragmentation, fiber cracking |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hassan, E.; Zekos, I.; Jansson, P.; Pecur, T.; Floreani, C.; Robert, C.; Ó Brádaigh, C.M.; Stack, M.M. Erosion Mapping of Through-Thickness Toughened Powder Epoxy Gradient Glass-Fiber-Reinforced Polymer (GFRP) Plates for Tidal Turbine Blades. Lubricants 2021, 9, 22. https://doi.org/10.3390/lubricants9030022
Hassan E, Zekos I, Jansson P, Pecur T, Floreani C, Robert C, Ó Brádaigh CM, Stack MM. Erosion Mapping of Through-Thickness Toughened Powder Epoxy Gradient Glass-Fiber-Reinforced Polymer (GFRP) Plates for Tidal Turbine Blades. Lubricants. 2021; 9(3):22. https://doi.org/10.3390/lubricants9030022
Chicago/Turabian StyleHassan, Emadelddin, Iasonas Zekos, Philip Jansson, Toa Pecur, Christophe Floreani, Colin Robert, Conchúr M. Ó Brádaigh, and Margaret M. Stack. 2021. "Erosion Mapping of Through-Thickness Toughened Powder Epoxy Gradient Glass-Fiber-Reinforced Polymer (GFRP) Plates for Tidal Turbine Blades" Lubricants 9, no. 3: 22. https://doi.org/10.3390/lubricants9030022
APA StyleHassan, E., Zekos, I., Jansson, P., Pecur, T., Floreani, C., Robert, C., Ó Brádaigh, C. M., & Stack, M. M. (2021). Erosion Mapping of Through-Thickness Toughened Powder Epoxy Gradient Glass-Fiber-Reinforced Polymer (GFRP) Plates for Tidal Turbine Blades. Lubricants, 9(3), 22. https://doi.org/10.3390/lubricants9030022