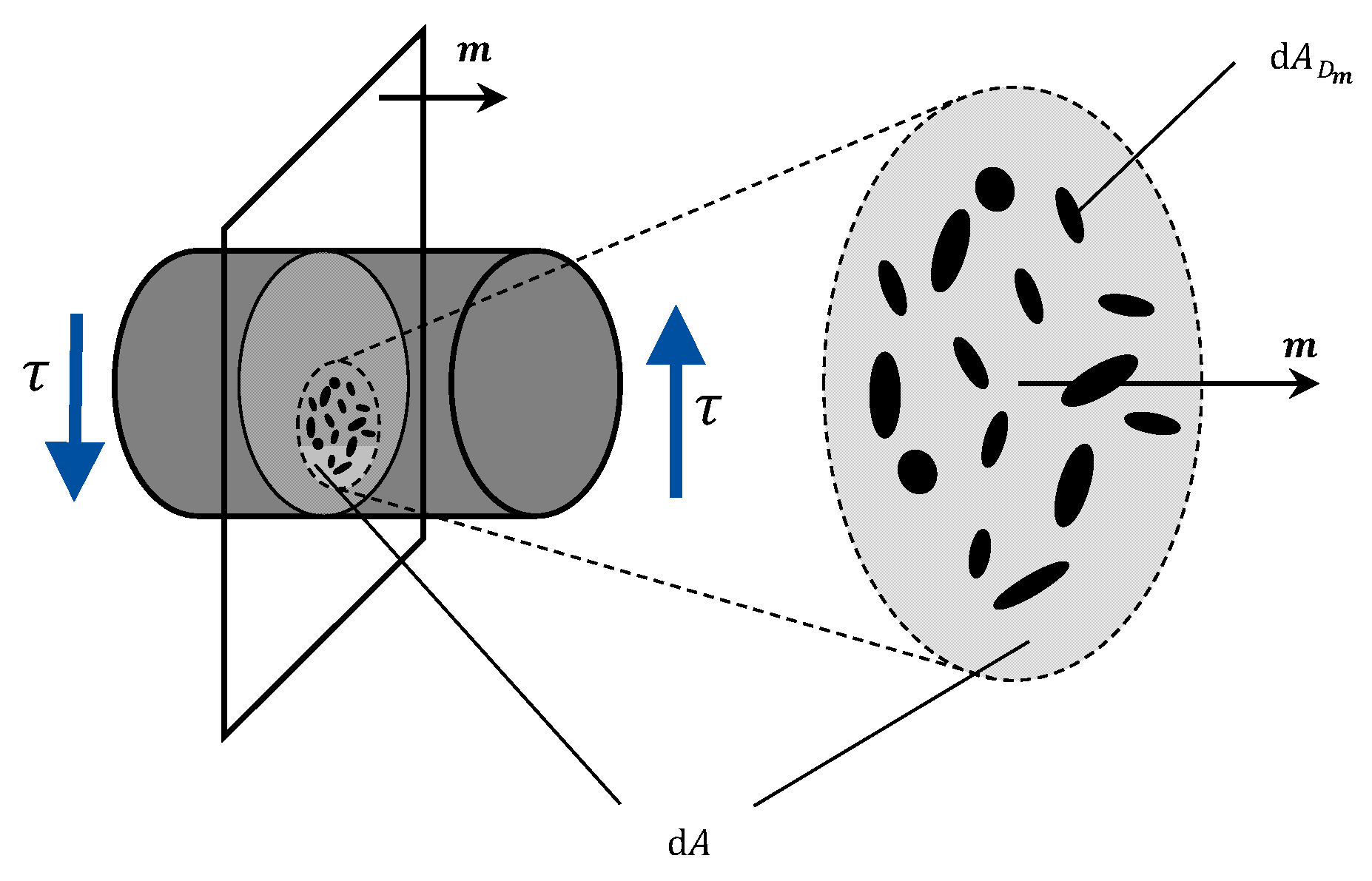
Energy-Based Modelling of Adhesive Wear in the Mixed Lubrication Regime
Abstract
:1. Introduction
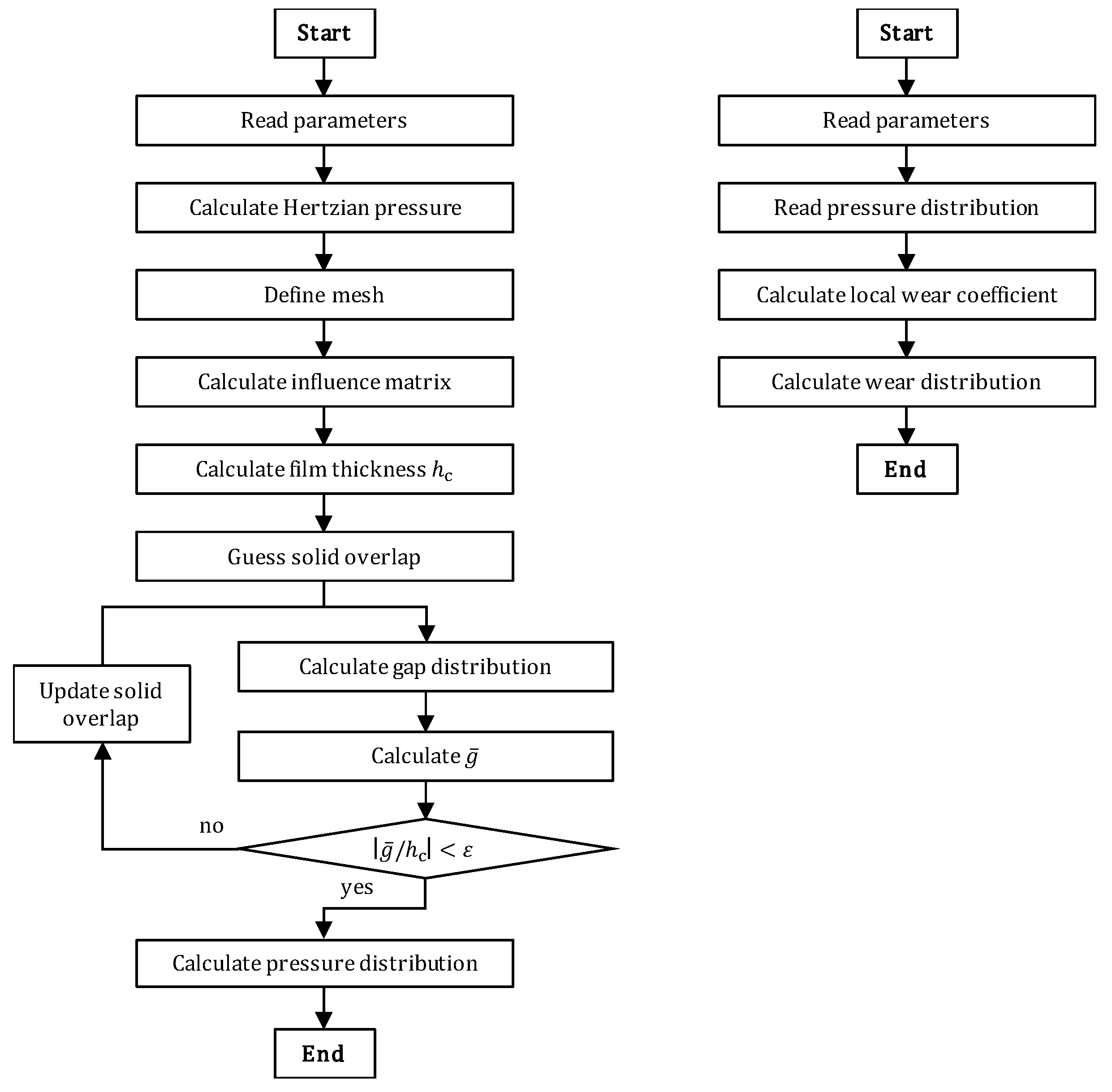
2. Calculation Method
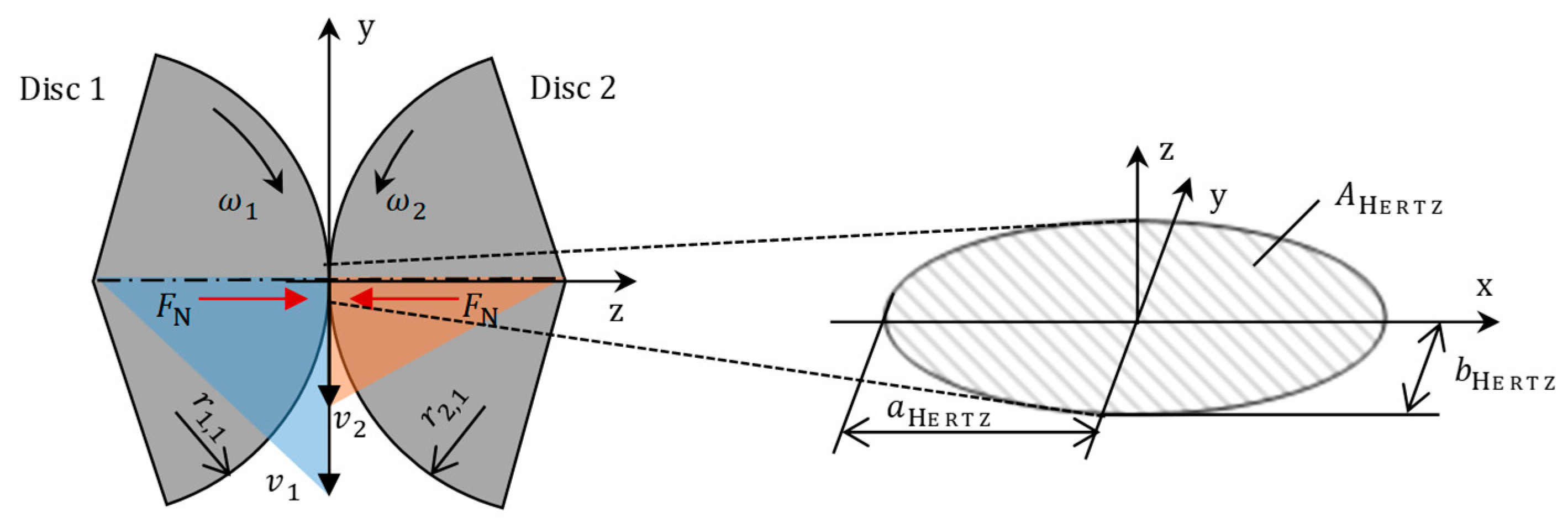
2.1. Numerical Contact Model
2.2. Numerical Wear Model
2.3. Calculation Algortihm
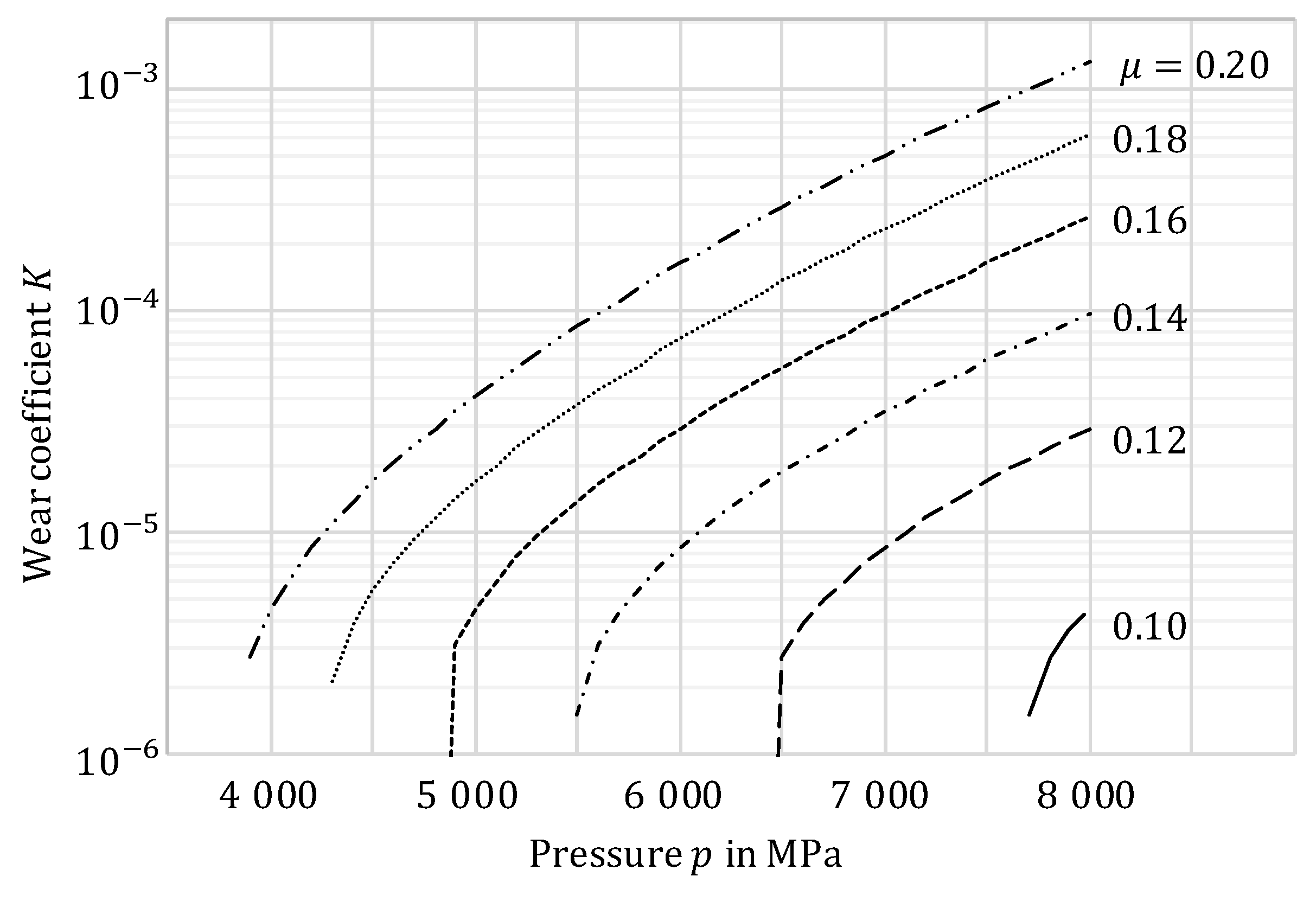
3. Simulation Results
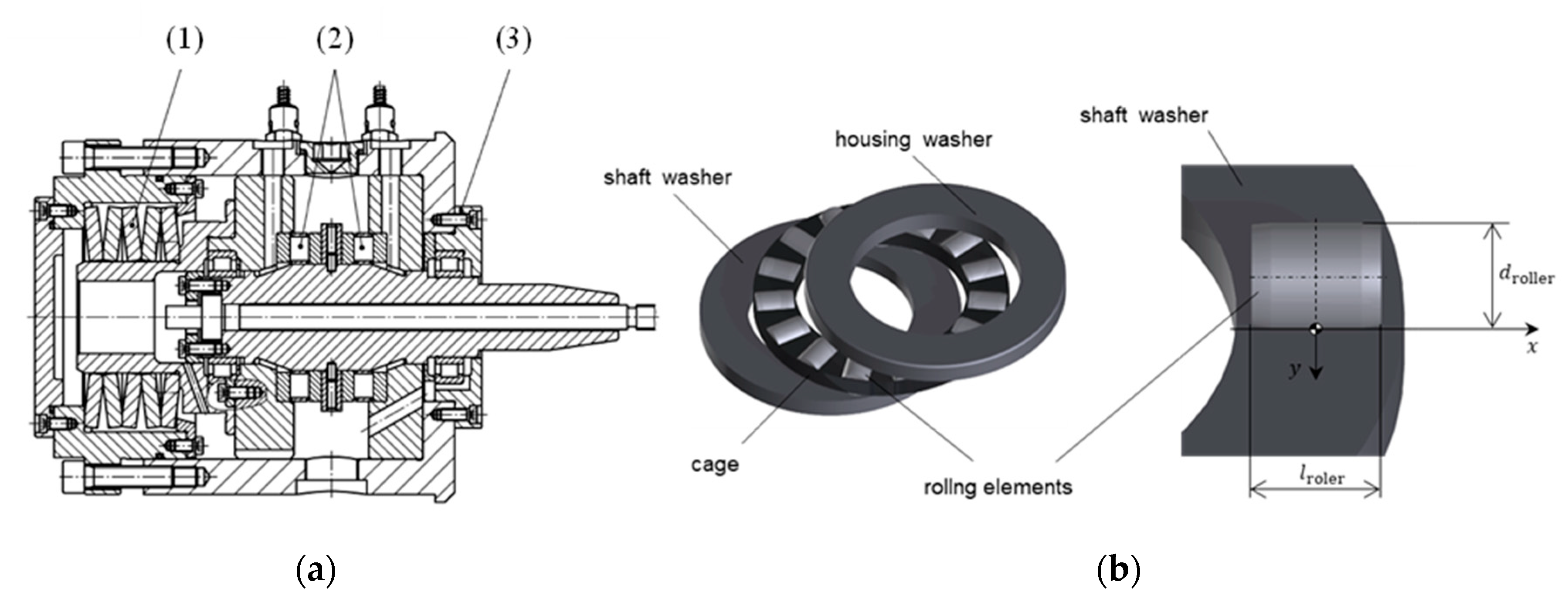
3.1. Model System
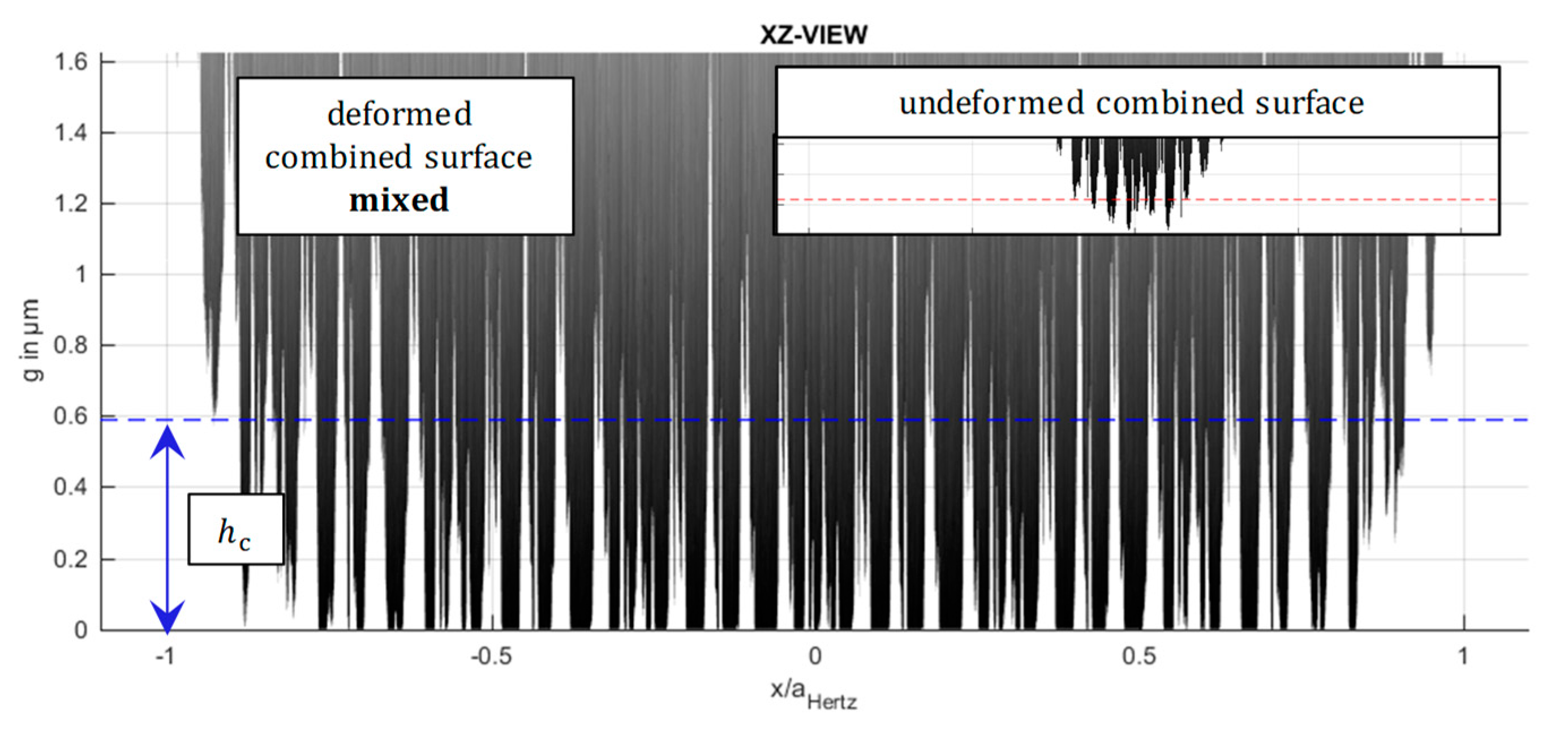
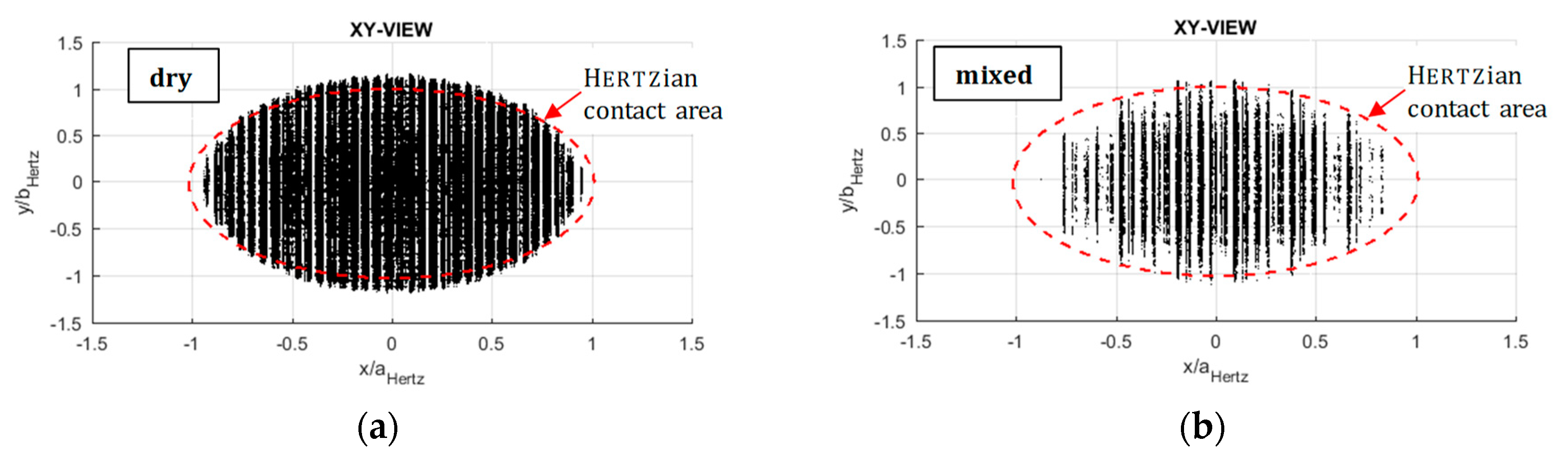
3.2. Simulation of Gap and Pressure Distributions
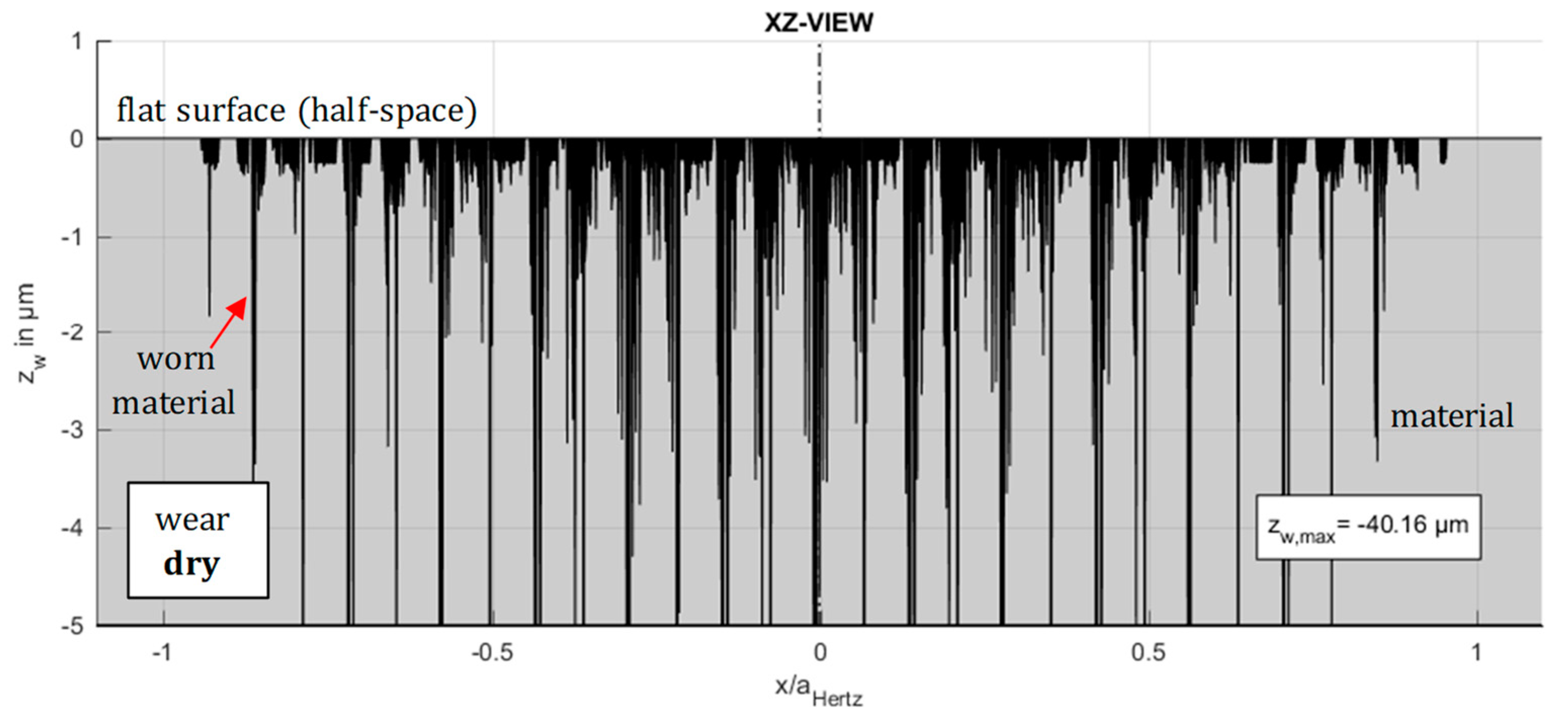
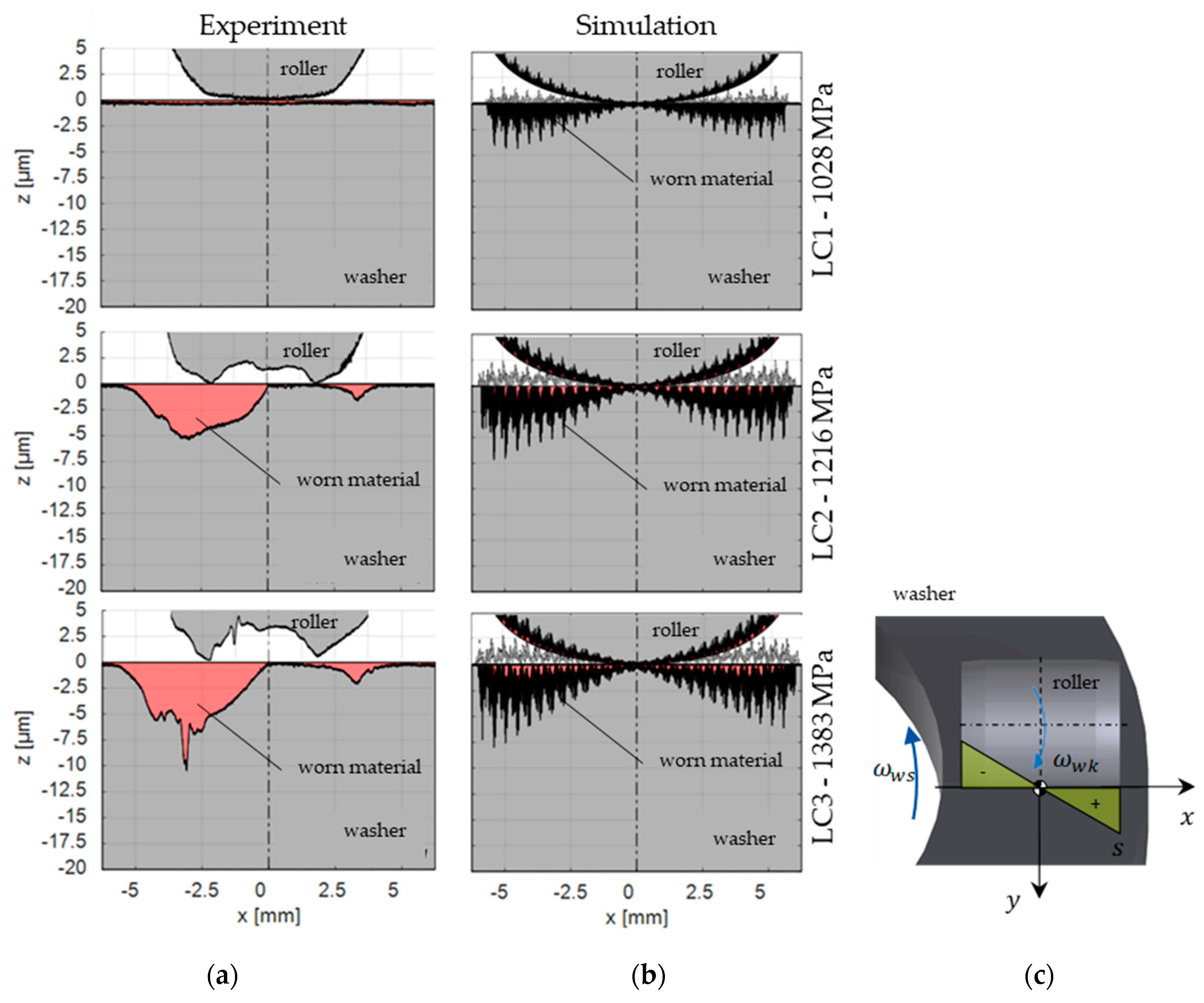
3.3. Simulation of Wear Distribution
4. Conclusions and Outlook
Author Contributions
Funding
Conflicts of Interest
List of Symbols
| Micro contact area at pos. | Archard Wear coefficient at pos. | ||
| Hertzian contact length | Normalized pressure | ||
| Parameter (film thickness) | Normalized flow pressure | ||
| Damage propagation after load cycles | Pressure | ||
| Hertzian contact width | Pressure at pos. | ||
| Influence matrix | Hertzian pressure | ||
| Damage variable | Pressure acc. to Hertz at pos. | ||
| Critical damage | Flow pressure | ||
| Damage variable after load cycles | Solid pressure at pos. | ||
| Element length | Geometry parameter | ||
| Cross section | RMS roughness | ||
| Young’s modulus | Asperity radius | ||
| App. frict. energy density | , | Radius disc 1, plane 1/2 | |
| Normal force at pos. | Radius disc 2, plane 1/2 | ||
| Solid force at pos. | Sliding path | ||
| EHL force at pos. | Sliding path vector | ||
| Normal force | Micro sliding path at pos. | ||
| Solid force | Duration | ||
| Solid force, dry contact | Velocity parameter | ||
| Solid force, mixed contact | Wear volume | ||
| EHL force | Micro wear volume at pos. | ||
| EHL force, dry contact | Entrainment velocity | ||
| EHL force, mixed contact | Circumferential velocity body 1 | ||
| Lubrication parameter | Circumferential velocity body 2 | ||
| Gap | Relative velocity | ||
| Gap at pos. | Deformation at pos. | ||
| Mean gap | W | Load parameter | |
| Surface hardness | Frictional work | ||
| Film thickness | Z | Number of asperities | |
| Wear intensity factor | Wear depth at pos. | ||
| Position | Wear depth vector | ||
| Hardening coefficient | Dynamic viscosity | ||
| Hardening exponent, | Specific film thickness | ||
| Number of elements in -direction | Ellipticity | ||
| Normal vector | Friction coefficient | ||
| N | Number of elements in -direction | ν | Poisson’s ratio |
| Critical number of load cycles | Shear stress | ||
| , | Number of load cycles | Endurance limit | |
| Number of non-contacting asperities | True failure stress | ||
| Number of contacting asperities | Shear stress at pos. | ||
| Archard Wear coefficient | Damaged area | ||
| Archard Wear coefficient vector | |||
| K1, k | Probability factor |
References
- Bowden, F.P.; Tabor, D. The Friction and Lubrication of Solids, 2nd ed.; Clarendon Press: Oxford, UK, 1954. [Google Scholar]
- Terwey, J.T.; Berninger, S.; Burghardt, G.; Jacobs, G.; Poll, G. Numerical Calculation of Local Adhesive Wear in Machine Elements under Boundary Lubrication Considering the Surface Roughness. In Proceedings of the 7th International Conference on Fracture Fatigue and Wear. (FFW 2018), Ghent, Belgium, 9–10 July 2018. [Google Scholar]
- Archard, J.F. Contact and Rubbing of Flat Surfaces. J. Appl. Phys. 1953, 24, 981–988. [Google Scholar] [CrossRef]
- Bartel, D. Berechnung von Festkörper-und Mischreibung bei Metallpaarungen; Shaker: Aachen, Germany, 2001. [Google Scholar]
- Wriggers, P. Computational Contact Mechanics, 2nd ed.; Springer: Berlin, Germany, 2006. [Google Scholar]
- Fleischer, G.; Gröger, H.; Thum, H. Verschleiß und Zuverlässigkeit; VEB Verlag Technik: Berlin, Germany, 1980. [Google Scholar]
- Love, A.E.H. The Stress Produced in a Semi-Infinite Solid by Pressure on Part of the Boundary. Philos. Trans. R. Soc. 1929, 228, 377–420. [Google Scholar]
- Brecher, C.; Renkens, D.; Löpenhaus, C. Method for Calculating Normal Pressure Distribution of High Resolution and Large Contact Area. J. Tribol. 2016, 138. [Google Scholar] [CrossRef]
- Hamrock, B.J.; Dowson, D. Ball Bearing Lubrication—The Elastohydrodynamics of Elliptical Contacts; John Wiley & Sons: New York, NY, USA, 1981. [Google Scholar]
- Hertz, H. Ueber die Berührung fester elastischer Körper. J. Reine Angew. Math. 1881, 92, 156–171. [Google Scholar]
- Harris, T.A.; Kotzalas, M.N. Rolling Bearing Analysis—Essential Concepts of Bearing Technology, 5th ed.; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
- Archard, J.F. Wear Theory and Mechanisms. In Wear Control Handbook; Peterson, M.B., Winer, W.O., Eds.; American Society of Mechanical Engineers: New York, NY, USA, 1980; pp. 35–80. [Google Scholar]
- Bhattcharya, B.; Ellingwood, B. A Damage Mechanics Based Approach to Structural Detoriation and Reliability; Oak Ridge National Laboratory: Baltimore, MD, USA, 1998. [Google Scholar]
- Bhattcharya, B.; Ellingwood, B. Continuum Damage Mechanics Analysis of Fatigue Crack Initiation. Int. J. Fatigue 1998, 20, 631–639. [Google Scholar] [CrossRef]
- Bhattcharya, B.; Ellingwood, B. A New CDM-Based Approach to Structural Deterioration. Int. J. Solids Struct. 1999, 36, 1757–1779. [Google Scholar] [CrossRef]
- Lemaitre, J.; Desmorat, R. Engineering Damage Mechanics; Springer: Berlin, Germany, 2005. [Google Scholar]
- Beheshti, A.; Khonsari, M.M. A Thermodynamic Approach for Prediction of Wear Coefficient Under Unlubricated Sliding Condition. Tribol. Lett. 2010, 38, 347–354. [Google Scholar] [CrossRef]
- Beheshti, A.; Khonsari, M.M. On the Prediction of Fatigue Crack Initiation in Rolling/Sliding Contacts with Provision for Loading Sequence Effects. Tribol. Int. 2011, 44, 1620–1628. [Google Scholar] [CrossRef]
- van de Sandt, N. Gebrauchsdauer von Axial Belasteten Wälzlagern bei Starker Mischreibung; Verlag Mainz: Aachen, Germany, 2004. [Google Scholar]
- DIN 51819. Prüfung von Schmierstoffen—Mechanisch-dynamische Prüfung auf dem Wälzlagerschmierstoff-Prüfgerät FE8; Beuth Verlag: Berlin, Germany, 2016. [Google Scholar]
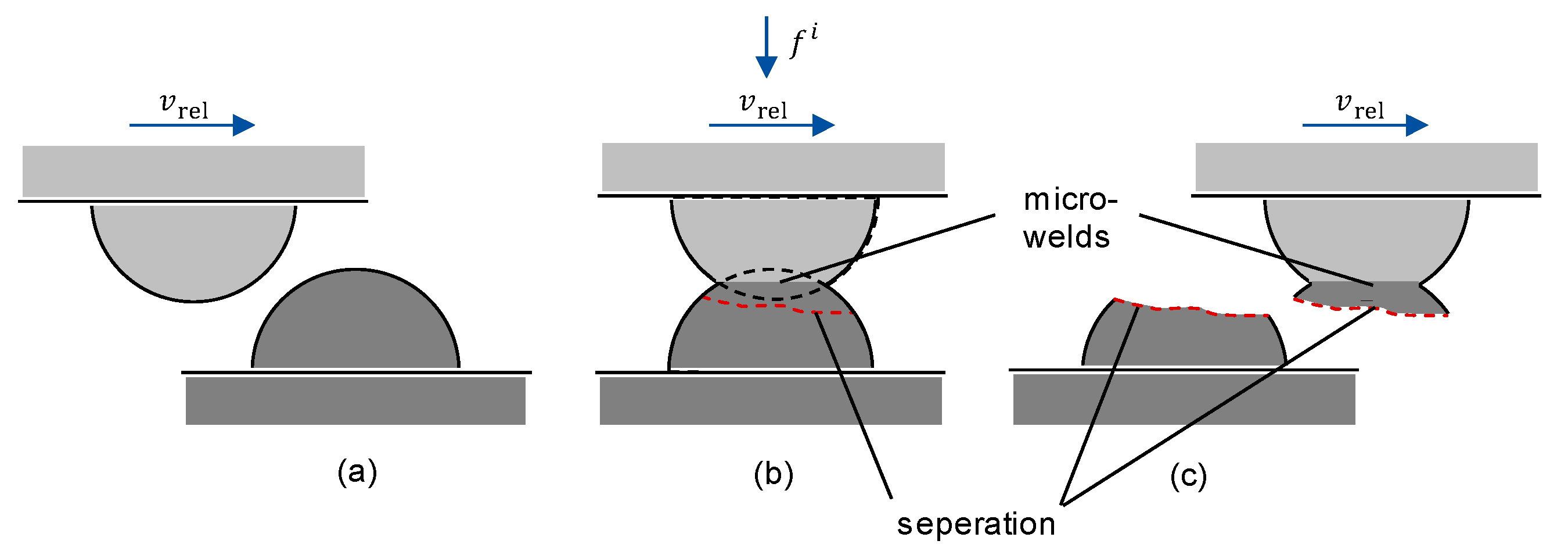
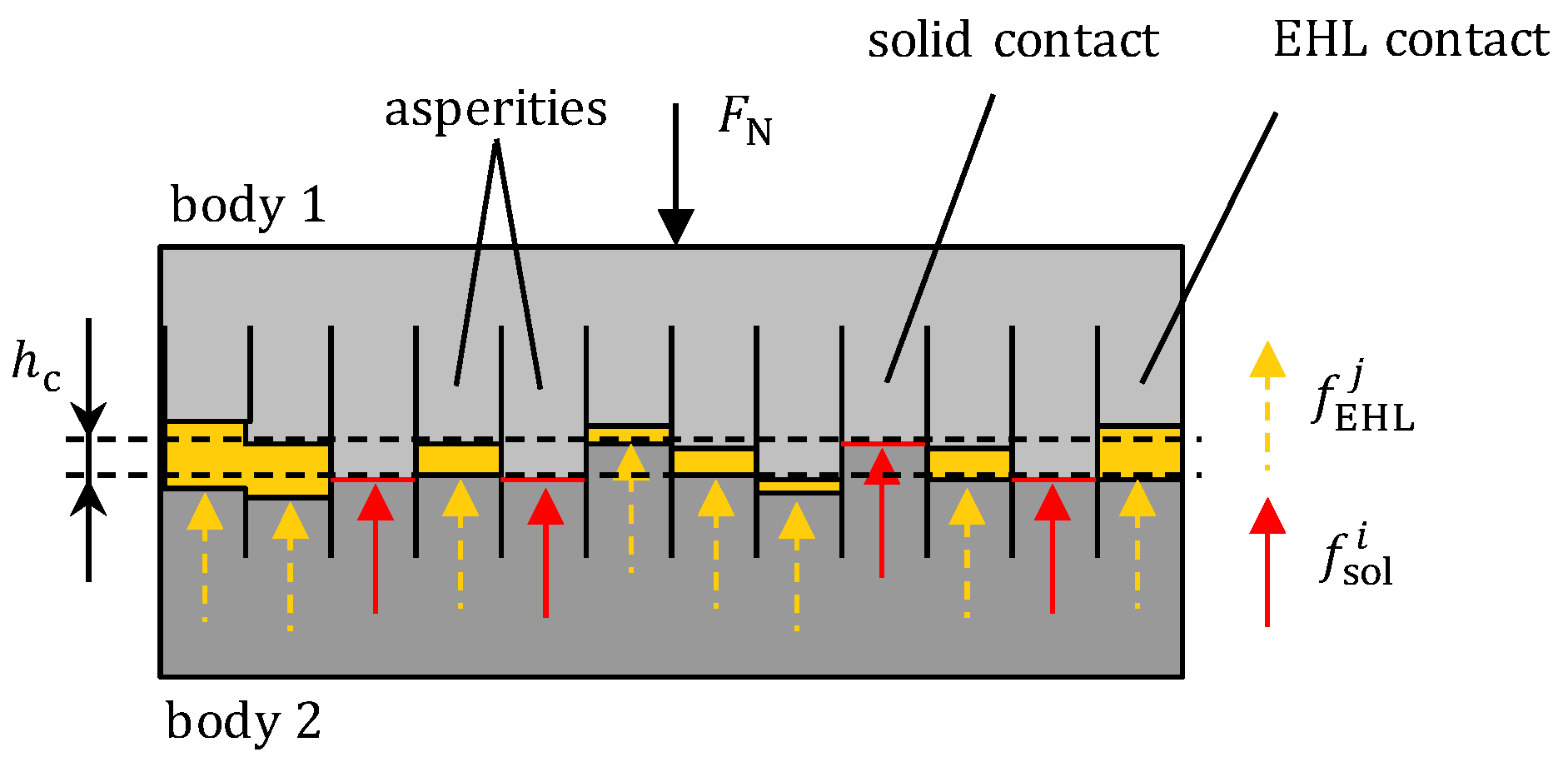

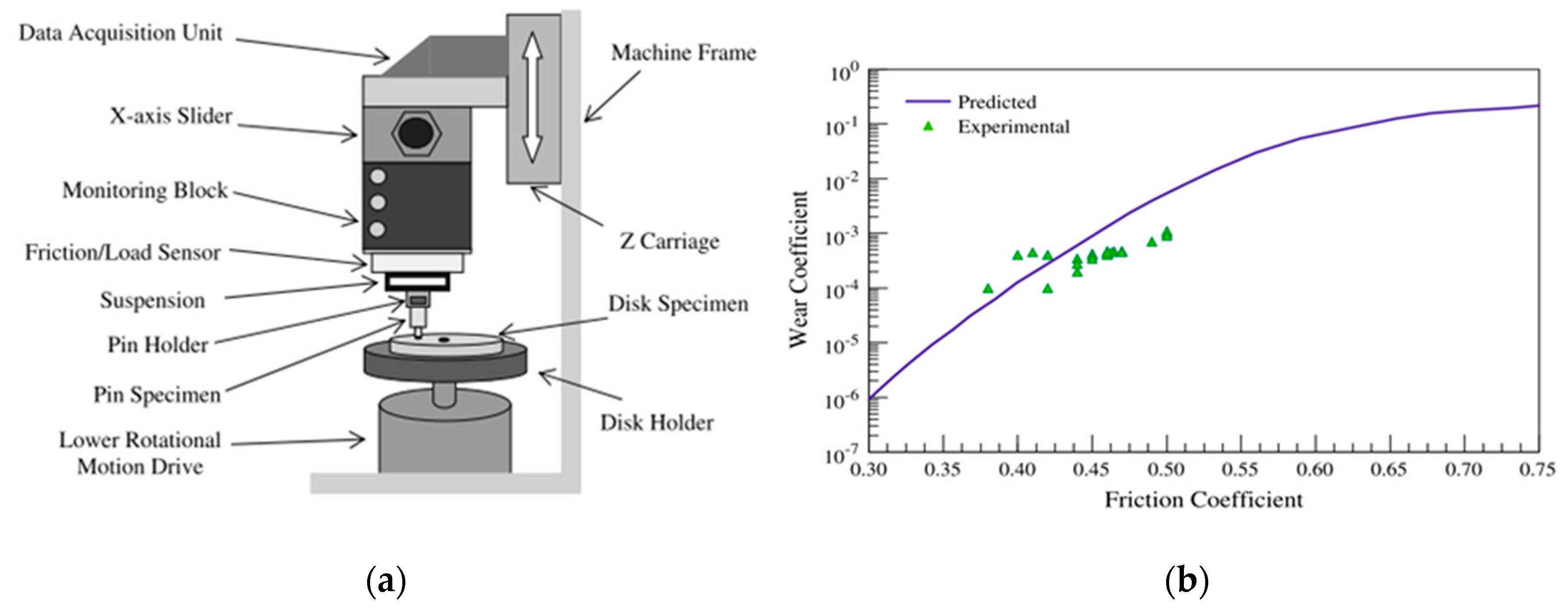




| Material Parameters | Crowning disc 2 | ||||
| Young’s Modulus | Root mean squared (RMS) roughness | ||||
| Poisson’s ratio | Simulation Parameters | ||||
| Flow pressure | Normal force | ||||
| Cycl. hard. exponent | Hertzian pressure | ||||
| Hard. modulus | Entrainment velocity | ||||
| True failure stress | Relative velocity | ||||
| Endurance limit | Duration | ||||
| Critical Damage | Film thickness | ||||
| Dyn. viscosity | Rel. film thickness | ||||
| Geometry Parameters | Friction coeff. (solid) | ||||
| Radius disc 1 | Element width | ||||
| Crowning disc 1 | Elements in -dir. | ||||
| Radius disc 2 | Elements in -dir. | ||||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Terwey, J.T.; Fourati, M.A.; Pape, F.; Poll, G. Energy-Based Modelling of Adhesive Wear in the Mixed Lubrication Regime. Lubricants 2020, 8, 16. https://doi.org/10.3390/lubricants8020016
Terwey JT, Fourati MA, Pape F, Poll G. Energy-Based Modelling of Adhesive Wear in the Mixed Lubrication Regime. Lubricants. 2020; 8(2):16. https://doi.org/10.3390/lubricants8020016
Chicago/Turabian StyleTerwey, J. Torben, Mohamed Ali Fourati, Florian Pape, and Gerhard Poll. 2020. "Energy-Based Modelling of Adhesive Wear in the Mixed Lubrication Regime" Lubricants 8, no. 2: 16. https://doi.org/10.3390/lubricants8020016
APA StyleTerwey, J. T., Fourati, M. A., Pape, F., & Poll, G. (2020). Energy-Based Modelling of Adhesive Wear in the Mixed Lubrication Regime. Lubricants, 8(2), 16. https://doi.org/10.3390/lubricants8020016