Bearing Power Losses with Water-Containing Gear Fluids
Abstract
:1. Introduction
2. Experimental Setups
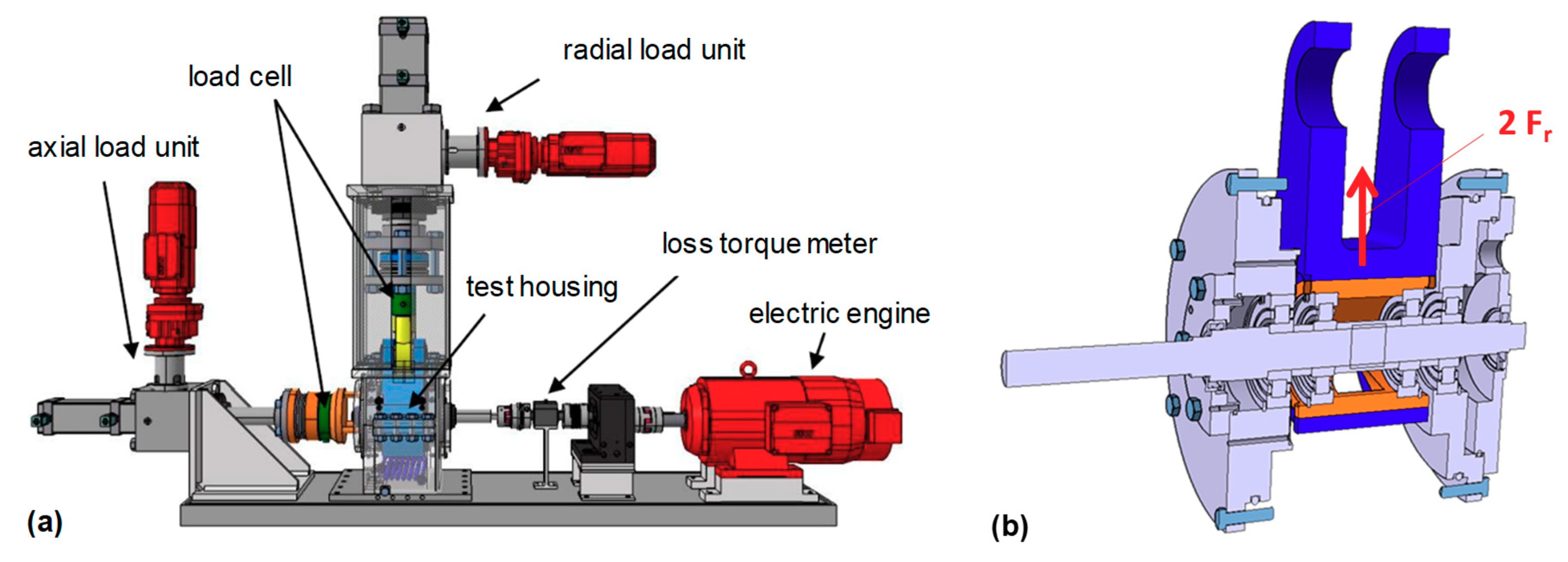
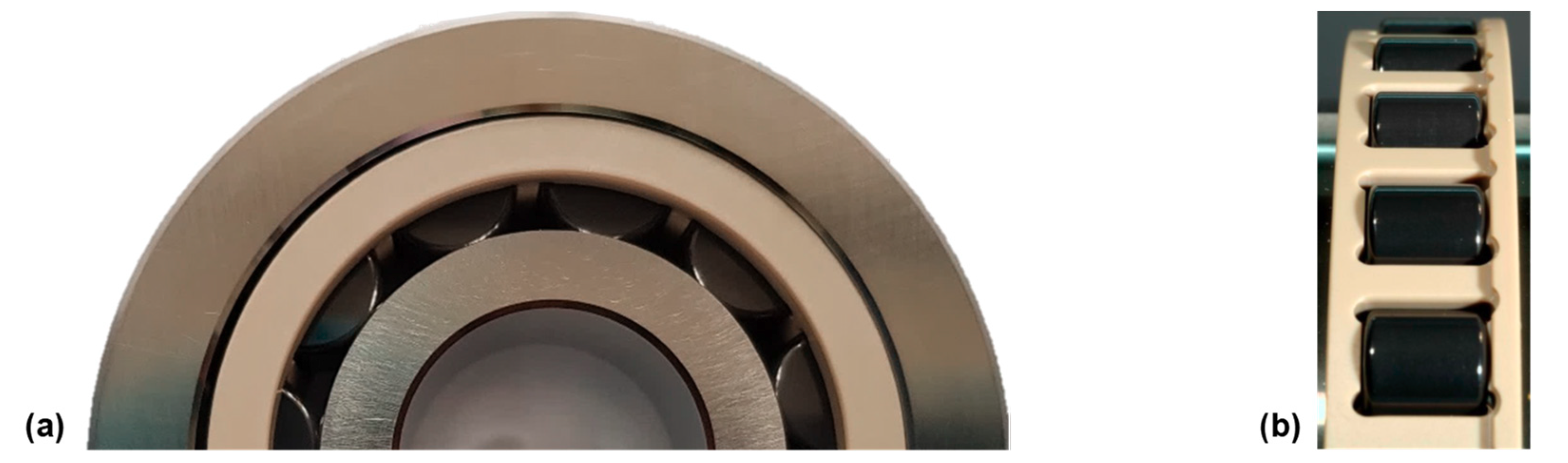
2.1. Bearing Power Loss Test Rig
2.2. Operating Conditions and Lubricants
2.3. Load-Dependent Bearing Losses for Derivation of Mean Gear Coefficients of Friction
3. Results and Discussion
3.1. Bearing Loss Torques
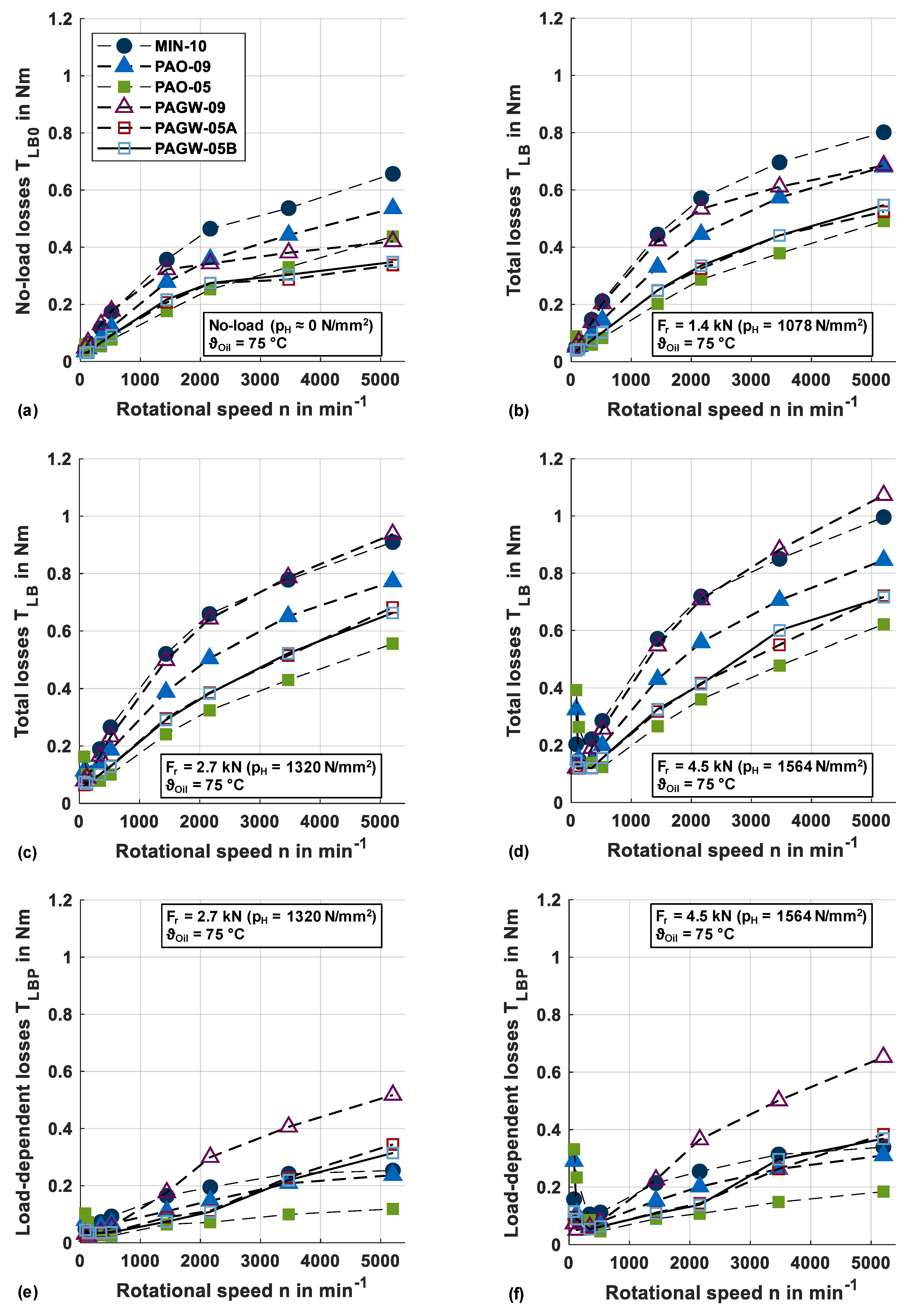
3.1.1. No-load Losses TLB0
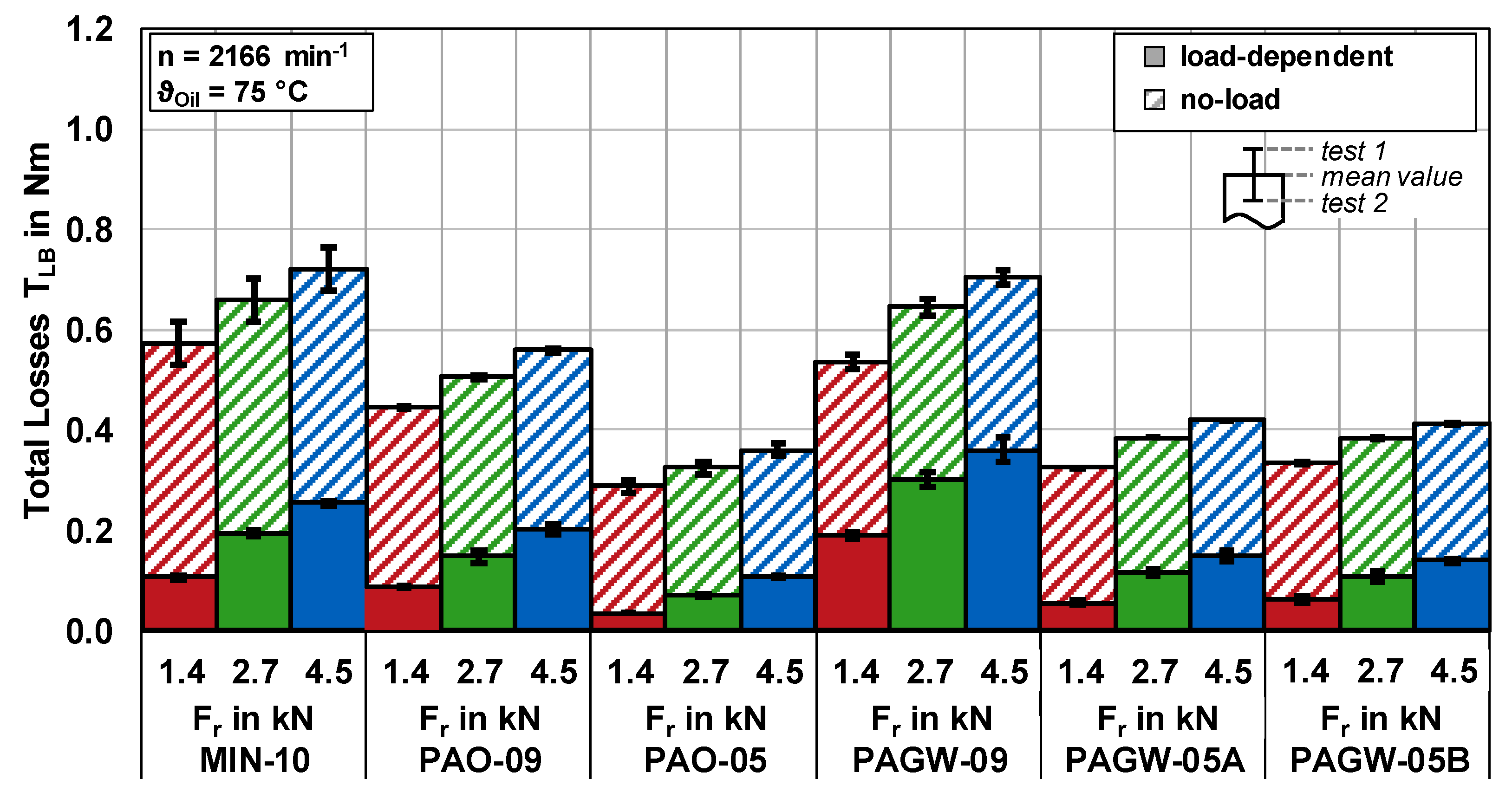
3.1.2. Total Losses TLB
3.1.3. Load-Dependent Losses TLBP
3.2. Comparison between Measured and Calculated Bearing Loss Torques
3.3. Derivation of Mean Gear Coefficients of Friction
4. Conclusion
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| B | Bearing width | mm |
| d | Bearing inner diameter | mm |
| D | Bearing outer diameter | mm |
| Fr | Radial force | kN |
| n | Rotational speed | min−1 |
| Hertzian pressure at the pitch point | N/mm2 | |
| Hertzian pressure | N/mm2 | |
| Time | s | |
| T | Torque | Nm |
| vt | Pitch line velocity | m/s |
| Greek symbols | ||
| Bulk temperature | °C | |
| Oil temperature | °C | |
| Oil kinematic viscosity | mm2/s | |
| µmz | Mean gear coefficient of friction | - |
| ρ | Oil density | kg/m3 |
| Indices | ||
| 0 | No-load | |
| B | Bearing | |
| G | Gear | |
| L | Loss | |
| P | Load-dependent | |
References
- Hinterstoißer, M.; Sedlmair, M.; Lohner, T.; Stahl, K. Minimizing Load-Dependent Gear Losses. Tribologie und Schmierungstechnik 2019, 3, 15–25. [Google Scholar]
- Hinterstoißer, M. Zur Optimierung des Wirkungsgrades von Stirnradgetrieben. On the Optimization of the Efficiency of Spur Gears. Ph.D. Dissertation, Technical University of Munich, Munich, Germany, 2014. [Google Scholar]
- Jurkschat, T.; Otto, M.; Stahl, K. Lebensdauer-Industriegetriebe-Wälzlager. Lifetime of Roller Bearings in Industrial Transmissions; FVA-Nr. 1145; Forschungsvereinigung Antriebstechnik e.V.: Frankfurt/Main, Germany, 2015. [Google Scholar]
- Aul, V.; Kiekbusch, T.; Marquart, M.; Sauer, B. Experimentelle und simulative Ermittlung von Reibmomenten in Wälzlagern. Experimental and simulative determination of friction torque of roller bearings. In Proceedings of the 51st German Tribology Conference, Göttingen, Germany, 27–29 September 2010. [Google Scholar]
- Talbot, D.; Stilwell, A.; Kahraman, A.; Singh, A.; Napau, I. Mechanical power losses of full-complement needle bearings of planetary gear sets. Proc. Inst. Mech. Eng. 2015, 230, 251–262. [Google Scholar]
- Balan, M.R.D.; Stamate, V.C.; Houpert, L.; Olaru, D.N. The influence of the lubricant viscosity on the rolling friction torque. Tribol. Int. 2014, 72, 1–12. [Google Scholar] [CrossRef]
- Koryciak, J. Einfluss der Ölmenge auf das Reibmoment von Wälzlagern mit Linienberührung. Influence of the Oil Amount on Friction Losses of Roller Bearings with Line Contact. Ph.D. Dissertation, Ruhr Universität Bochum, Bochum, Germany, 2007. [Google Scholar]
- Aul, V.; Kiekbusch, T.; Marquart, M.; Sauer, B. Experimentelle und Simulative Ermittlung von Reibmomenten in Wälzlagern bei Minimalmengenschmierung. Experimental and Simulative Determination of Friction Torque of Roller Bearings under Minimum Quantity Lubrication. In Proceedings of the 52nd German Tribology Conference, Göttingen, Germany, 26–28 September 2011. [Google Scholar]
- Jurkschat, T.; Otto, M.; Lohner, T.; Stahl, K. Bestimmung des Verlustverhaltens und der Wärmebilanz von Wälzlagern. Determination of the Loss and Thermal Behavior of Roller Bearings. Springer Forschung im Ingenieurwesen 2018, 82, 149–155. [Google Scholar] [CrossRef]
- SKF-Gruppe: SKF Hauptkatalog 2004. SKF main catalogue. Media-Print Informationstechnologie (2004). Available online: https://www.booklooker.de/B%C3%BCcher/SKF+Hauptkatalog-Das-W%C3%A4lzlager-Handbuch-f%C3%BCr-Studenten/id/A024NHes01ZZf (accessed on 28 December 2019).
- Wang, D. Berechnung der Wälzlagerreibung aufgrund weiterentwickelter rheologischer Fluidmodelle. Calculation of friction torque of roller bearings with developed rheological fluid models. Ph.D. Dissertation, Gottfried Wilhelm Leibniz Universität Hannover, Hannover, Germany, 2015. [Google Scholar]
- Wang, D.; Jurkschat, T.; Otto, M.; Poll, G.; Stahl, K. Erweiterung der Berechnung der Wälzlagerreibung in FVA-Software. Extension of Bearing Friction Calculation in FVA-Software; FVA-Nr. 1157; Forschungsvereinigung Antriebstechnik e.V.: Frankfurt/Main, Germany, 2015. [Google Scholar]
- Schleich, T. Zum Temperaturverhalten von Wälzlagern in Getrieben. Thermal Behavior of Roller Bearings in Transmissions. Ph.D. Dissertation, Technical University of Munich, Munich, Germany, 2013. [Google Scholar]
- Yilmaz, M.; Mirza, M.; Lohner, T.; Stahl, K. Superlubricity in EHL Contacts with Water-Containing Gear Fluid. Lubricants 2019, 7, 46. [Google Scholar] [CrossRef]
- Hirano, M.; Shinjo, K. Atomistic locking and friction. Phys. Rev. B 1990, 41, 11837–11851. [Google Scholar] [CrossRef]
- Yilmaz, M.; Lohner, T.; Michaelis, K.; Stahl, K. Minimizing Gear Friction with Water-Containing Gear Fluids. In Forschung im Ingenieurwesen; Springer: Berlin, Germany, 2019; Volume 83, pp. 327–337. [Google Scholar]
- Yilmaz, M.; Lohner, T.; Michaelis, K.; Stahl, K. Increasing Gearbox Efficiency by Water-Containing Fluids and Minimum Quantity Lubrication. In Proceedings of the 60th German Tribology Conference, Göttingen, Germany, 23–25 June 2019. [Google Scholar]
- Laukotka, E.M. Referenzöle Datensammlung. Reference Lubricants Data Collection; FVA-Nr. 660; Forschungsvereinigung Antriebstechnik e.V.: Frankfurt/Main, Germany, 2007. [Google Scholar]
- Thoma, F.; Otto, M.; Höhn, B.-R. Erweiterung Ritzelkorrekturprogramm (RIKOR) zur Bestimmung der Lastverteilung von Stirnradgetrieben. Extension of RIKOR to Determine the Load Distribution of Spur Gears; FVA-Nr. 914; Forschungsvereinigung Antriebstechnik e.V.: Frankfurt/Main, Germany, 2010. [Google Scholar]
- Schaeffler Technologies AG & Co. KG: BEARINX®—Online Easy Friction—Detaillierte Reibungsberechnung von Wälzlagern; Detailled friction calculation of roller bearings; Schaeffler Technologies AG & Co.: Herzogenaurach, Germany, 2011. [Google Scholar]




| Radial Force Fr in kN | Hertzian Pressure pH in N/mm2 | Rotational Speed n in min−1 | Time t in min | Oil Temperature ϑOil in °C |
|---|---|---|---|---|
| no-load | no-load | 87, 131, 348, 522, 1444, 2166, 3479, 5218 | 5 (for each rotational speed) | 75 |
| 1.4 | 1078 | |||
| 2.7 | 1320 | |||
| 4.5 | 1564 |
| Conventional Gear Oils | Water-Containing Gear Fluids | |||||
|---|---|---|---|---|---|---|
| MIN-10 | PAO-09 | PAO-05 | PAGW-09 | PAGW-05A | PAGW-05B | |
| ν (40 °C) in mm2/s | 94.1 | 50.2 | 20.4 | 45.7 | 23.6 | 22.9 |
| ν (60 °C) in mm2/s | 38.4 | 25.7 | 12.3 | 24.7 | 13.6 | 12.9 |
| ν (100 °C) in mm2/s | 10.6 | 9.0 | 5.0 | 9.2 | 5.3 | 4.8 |
| Viscosity index VI | 95 | 165 | 185 | 189 | 167 | 135 |
| ρ (15 °C) in kg/m3 | 884.5 | 850.0 | 840.0 | 1115.0 | 1109.0 | 1097.0 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yilmaz, M.; Lohner, T.; Michaelis, K.; Stahl, K. Bearing Power Losses with Water-Containing Gear Fluids. Lubricants 2020, 8, 5. https://doi.org/10.3390/lubricants8010005
Yilmaz M, Lohner T, Michaelis K, Stahl K. Bearing Power Losses with Water-Containing Gear Fluids. Lubricants. 2020; 8(1):5. https://doi.org/10.3390/lubricants8010005
Chicago/Turabian StyleYilmaz, Mustafa, Thomas Lohner, Klaus Michaelis, and Karsten Stahl. 2020. "Bearing Power Losses with Water-Containing Gear Fluids" Lubricants 8, no. 1: 5. https://doi.org/10.3390/lubricants8010005
APA StyleYilmaz, M., Lohner, T., Michaelis, K., & Stahl, K. (2020). Bearing Power Losses with Water-Containing Gear Fluids. Lubricants, 8(1), 5. https://doi.org/10.3390/lubricants8010005







