Research on the Correlation between Mechanical Seal Face Vibration and Stationary Ring Dynamic Behavior Characteristics
Abstract
1. Introduction
2. Mathematical Model
2.1. Viscous Shear Model
- 1.
- Calculation of bearing capacity components
- 2.
- Calculation of friction force components
- 3.
- Mathematical model of viscous shear
2.2. Micro-Convex Body Contact Model
2.2.1. Rough Surface Contact Deformation
2.2.2. Simulation of Contact Deformation of Asperities
- 1.
- Calculation of friction force of a single asperity
- 2.
- Calculation of contact vibration energy of a single asperity
- 3.
- Calculation of total vibration energy
- 4.
- Calculation of vibration energy generation rate
- 5.
- Calculation of vibration RMS
3. Experimental Validation
3.1. Construction of Mechanical Seal Test Rig
- The test device replicates common seal arrangements found in industrial applications, such as mechanical seal arrangements for pumps;
- Ensure sealing ring centering control during the design and installation process;
- The replacement of seals becomes simple and can be completed in only twenty minutes.
3.2. Tribological Behavior Change Test of Mechanical Seal
3.2.1. Mechanical Seal Start–Stop Experiment
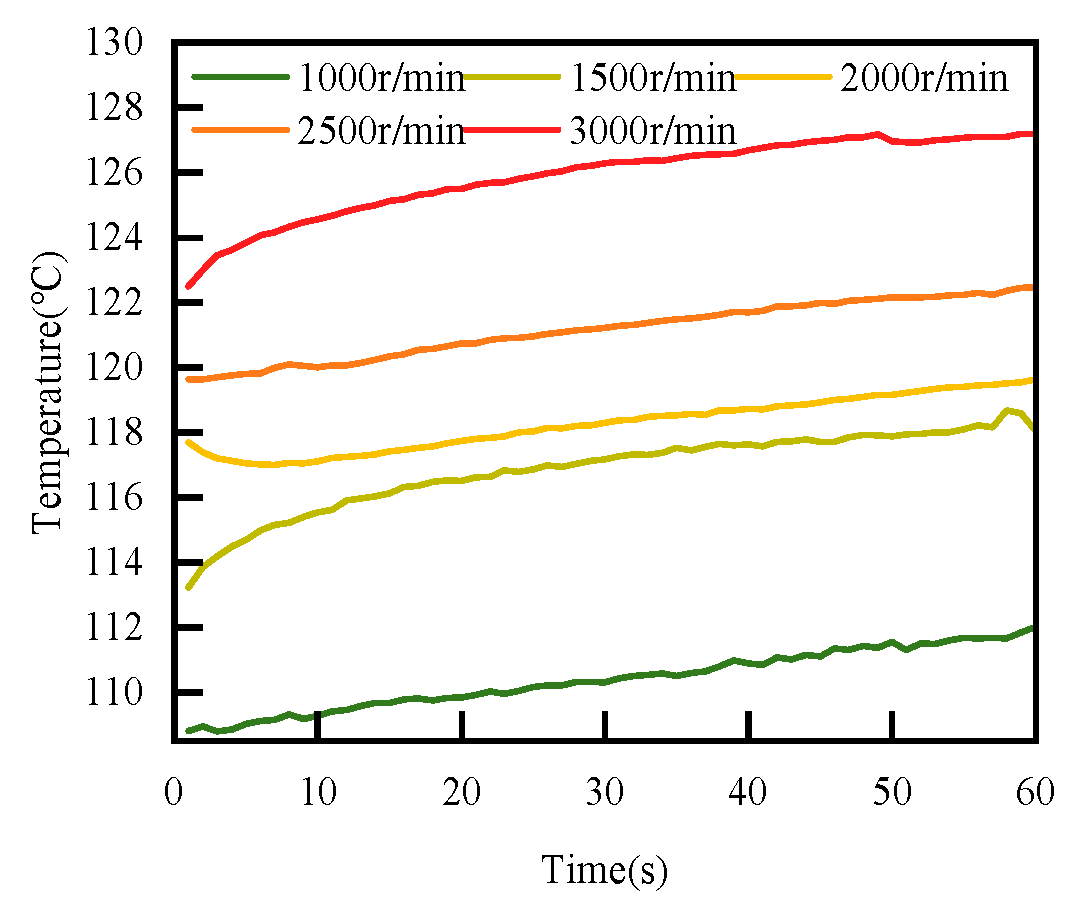
3.2.2. Mechanical Seal Rotation Speed Change Test
- Use the manual pressure pump replenishment method shown in Figure 10 to fill the mechanical seal tribology behavior test bench system with lubricating oil, and at the same time, let it reach the test set pressure of 1.6 MPa (which is the actual working pressure of the mechanical seal), and ensure that the lubricating oil viscosity is 32 pa∙s.
- Set the motor frequency converter frequency to 17 Hz. After ensuring safety, turn on the motor. At this time, ensure that the motor speed is 1000 r/min.
- Start data collection, and use the LabVIEW virtual instrument to set the face vibration acceleration and face temperature sampling frequency to 25,600 Hz, the sampling time to 1 s, and the number of sampling points to 25,600. At the same time, use the LabVIEW virtual instrument to save the face vibration acceleration data.
- Collect the face vibration acceleration signal and face temperature signal under the condition of 1000 r/min rotation speed, and ensure that the sampling duration is 1 min.
- Repeat steps 3 and 4 when the motor speed is 1500 r/min, 2000 r/min, 2500 r/min, and 3000 r/min.
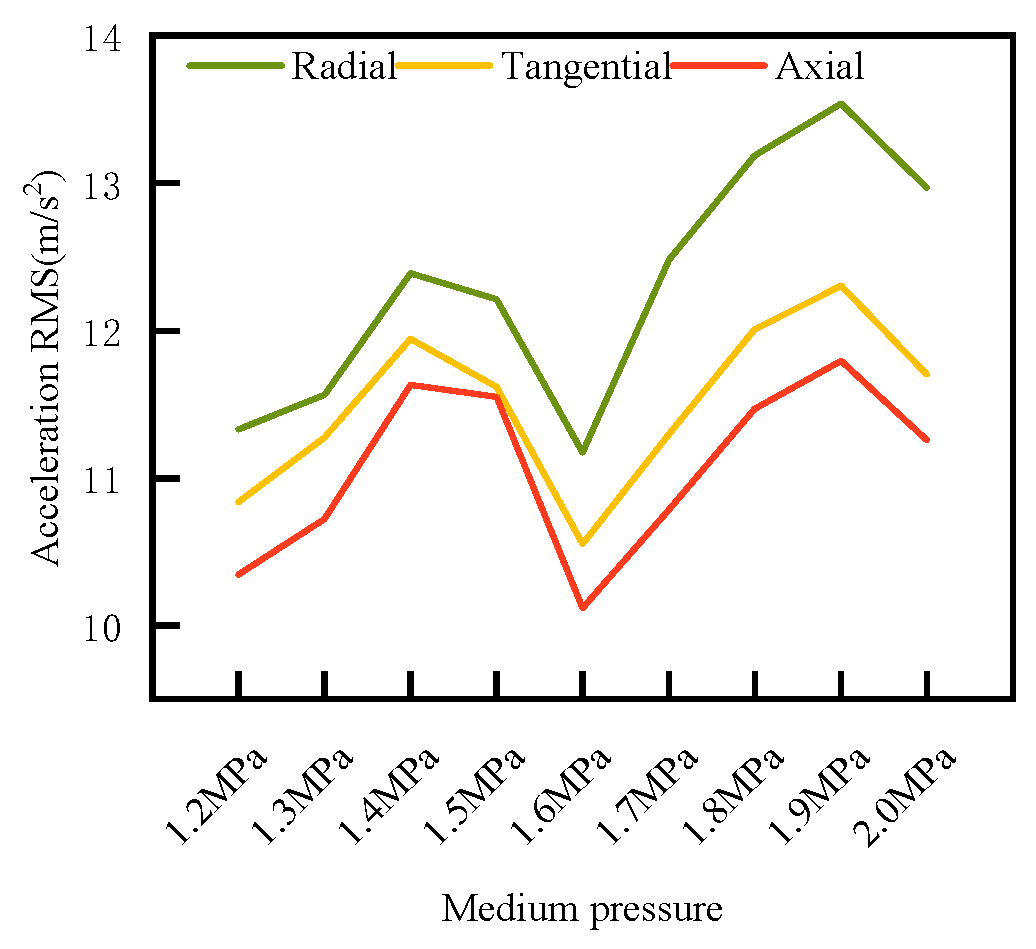
3.2.3. Mechanical Seal Medium Pressure Change Test
- Use the manual pressure pump replenishment method shown in Figure 10 to fill the mechanical seal tribology behavior test bench system with lubricating oil, and at the same time, let it reach the test set pressure of 1.2 MPa.
- Set the motor frequency converter frequency to 50 Hz and turn on the motor. At this time, ensure that the motor speed is 3000 r/min.
- Start data collection, and use the LabVIEW (version 2020) virtual instrument to set the face vibration acceleration and face temperature sampling frequency to 25,600 Hz, the sampling time to 1 s, and the number of sampling points to 25,600.
- Collect the face vibration acceleration signal and face temperature signal under the conditions of 3000 r/min rotation speed and medium pressure 1.2 MPa. Ensure that the sampling duration is 1 min.
- Use a hand pump to replenish fluid, and repeat steps 3 and 4 under the conditions of medium pressures of 1.3 MPa, 1.4 MPa, 1.5 MPa, 1.6 MPa, 1.7 MPa, 1.8 MPa, 1.9 MPa, and 2.0 MPa.
- Combine the face vibration acceleration data collected at each pressure gradient into time domain waveform data in three directions: axial, radial, and tangential.
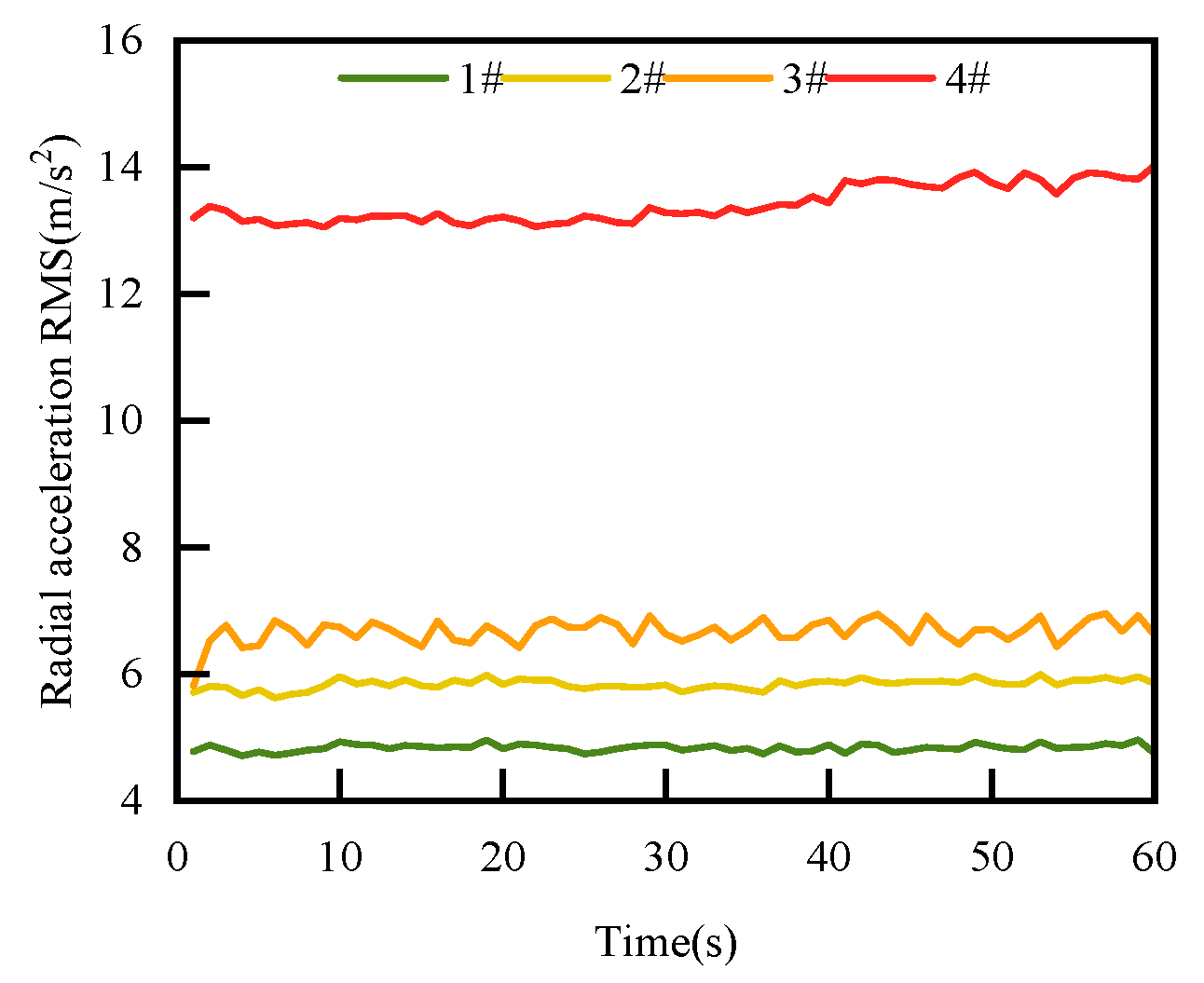
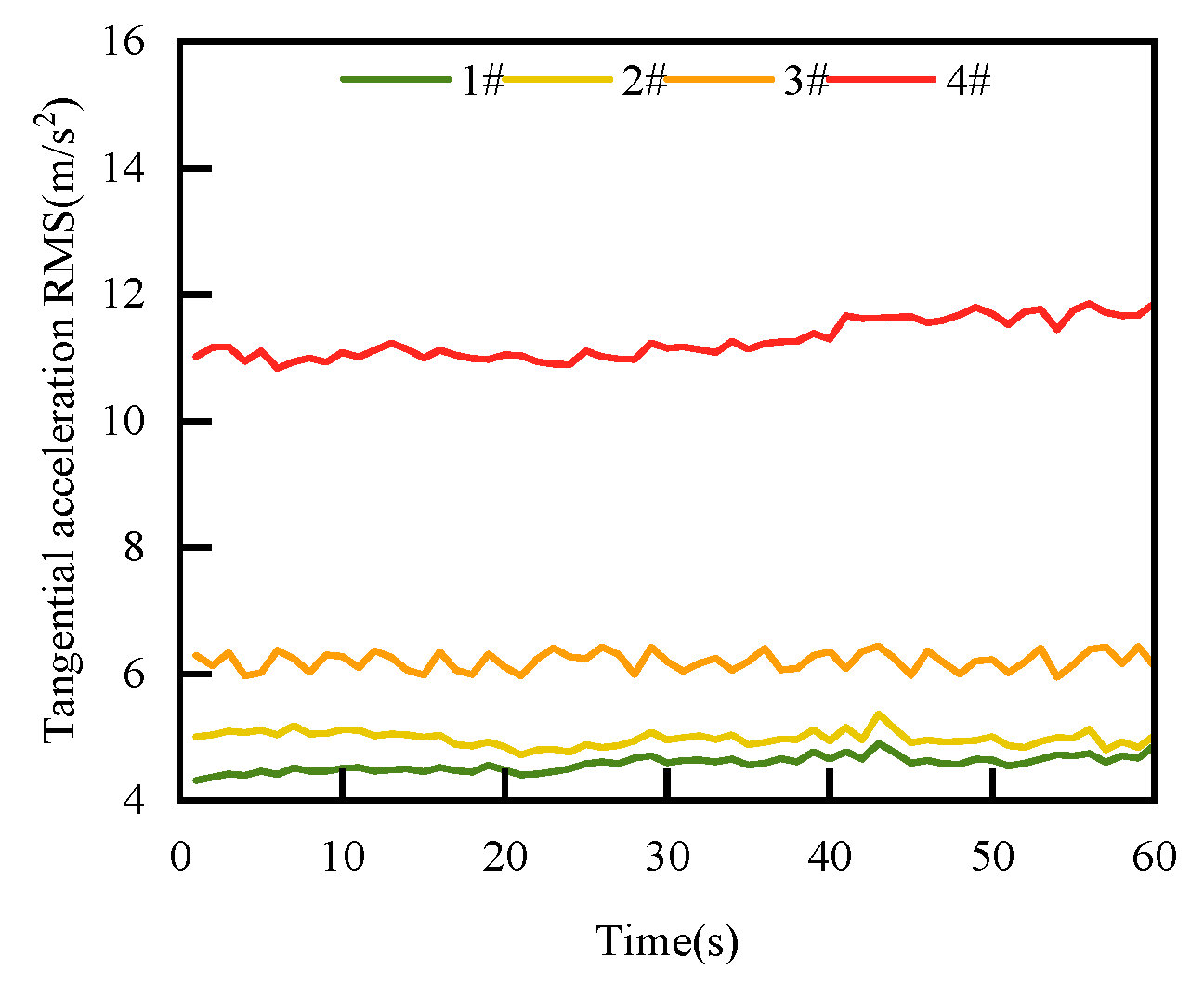
3.2.4. Mechanical Seal Surface Wear Change Test
- Install the 1# rotating ring, use the manual pressure pump to replenish fluid as shown in Figure 10, fill the mechanical seal tribology behavior test bench system with lubricating oil, and simultaneously let it reach the test set pressure of 1.6 MPa.
- Set the motor frequency converter frequency to 50 Hz and turn on the motor. At this time, ensure that the motor speed is 3000 r/min.
- Use the LabVIEW data acquisition virtual instrument, set the face vibration acceleration and face temperature sampling frequency to 25,600 Hz, the sampling time to 1 s, the number of sampling points to 25,600, and start data collection.
- Collect the face vibration acceleration signal and face temperature signal under the conditions of 3000 r/min rotation speed and medium pressure 1.6 MPa. Ensure that the sampling duration is 1 min.
- After the collection is completed, replace the 2#, 3#, and 4# rotating rings in sequence, and repeat steps 2 to 4 in sequence.
4. Results
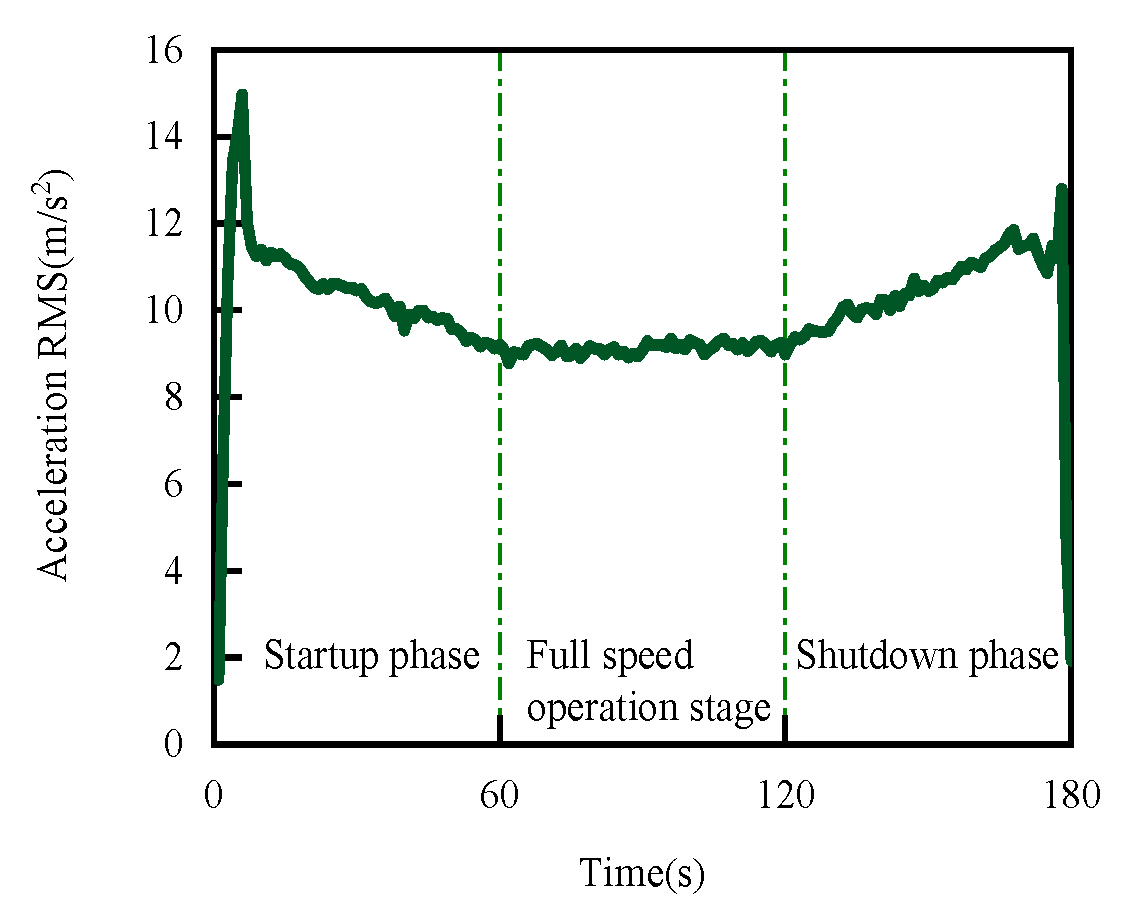
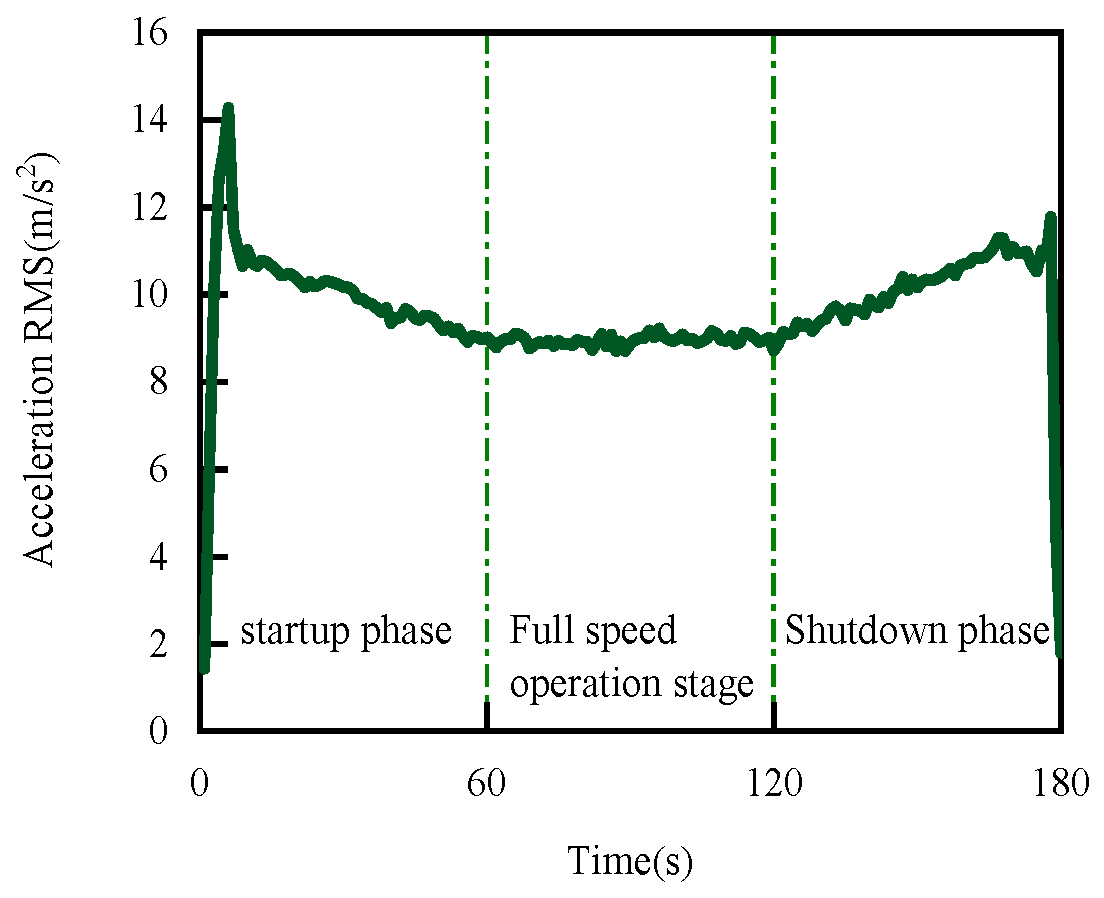
4.1. RMS of Face Vibration Acceleration Change Law during Starting and Stopping Process
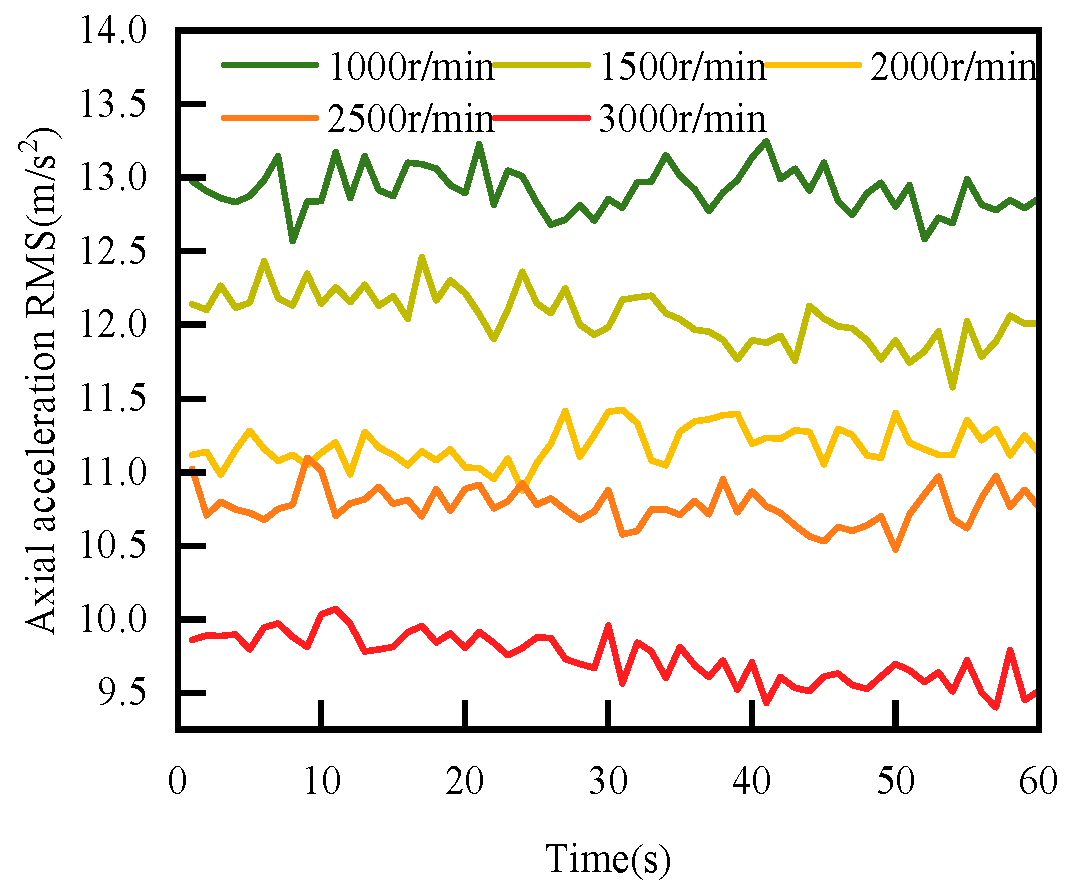
4.2. The RMS of the Face Vibration Acceleration Change Law with the Rotation Speed Law
4.3. The RMS of the Face Vibration Acceleration Change Law with the Sealing Medium Pressure
4.4. RMS of Face Vibration Acceleration Change Law with Surface Roughness
5. Conclusions
- A method for measuring the tribological behavior of mechanical seals by the face vibration acceleration is proposed and applied to the test rig.
- A tribological behavior model of mechanical seals based on micro-convex contact and viscous shear is established, and the relationship between face acceleration RMS and tribological behavior parameters is clarified, providing theoretical sources for the method.
- This experimental study shows that the face tribological regime changes during the start and stop stages of the mechanical seal, and the change in the RMS curve is consistent with the changing trend in the Stribeck curve, which further proves the effectiveness of the proposed method.
- According to the experimental study, an increase in speed will lead to a decrease in the RMS value, an increase in sealing medium pressure will lead to a nonlinear change in RMS, and an increase in surface roughness will lead to an increase in RMS.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| RMS | Root mean square |
| BL | Boundary lubrication |
| ML | Mixed lubrication |
| HL | Hydrodynamic lubrication |
| Friction force | |
| The shear stress in the asperity–asperity (a-a) interaction | |
| The friction force acting on the free face of each equivalent asperity | |
| The vibration energy | |
| The bending stress | |
| The bending moment | |
| The vibration energy leading to asperity–asperity (a-a) interaction | |
| The average energy leading to asperity–asperity (a-a) interaction | |
| The total vibration energy | |
| The total vibration energy | |
| The vibration energy generation rate | |
| The relationship between the RMS of vibration and vibration energy | |
| U1 | The first face’s x-direction velocity vector |
| V1 | The first face’s y-direction velocity vector |
| W1 | The first face’s z-direction velocity vector |
| U2 | The second face’s x-direction velocity vector |
| V2 | The second face’s y-direction velocity vector |
| W2 | The second face’s z-direction velocity vector |
| The velocity vector of fluid film in the x-direction | |
| The velocity vector of fluid film in the y-direction | |
| The velocity vector of fluid film in the z-direction | |
| The density of the fluid | |
| The dynamic viscosity of the fluid | |
| The total load-bearing capacity resulting from fluid pressure and contact pressure | |
| The contact pressure | |
| The fraction of the total area of the contact area | |
| The total circumferential friction force | |
| The tangential contact friction force | |
| The contact friction stress | |
| The total friction caused by viscous shear and contact friction | |
| The radial flow rate of the fluid | |
| The circumferential flow rate of the fluid | |
| The volume of fluid along the radial direction | |
| The volume of fluid along the circumferential direction | |
| The axial face acceleration | |
| The radial face acceleration | |
| The circumferential face acceleration |
References
- Meng, X.; Tu, Z.; Ma, Y.; Jiang, J.; Peng, X. Topology optimization of liquid lubricating zero-leakage mechanical face seals. Tribol. Int. 2022, 169, 107490. [Google Scholar] [CrossRef]
- Jamari, J. Running-In of Rolling Contacts. Ph.D. Thesis, University of Twente, Enschede, The Netherlands, 2006. [Google Scholar]
- Towsyfyan, H.; Gu, F.; Ball, A.D.; Liang, B. Modelling acoustic emissions generated by tribological behaviour of mechanical seals for condition monitoring and fault detection. Tribol. Int. 2018, 125, 46–58. [Google Scholar] [CrossRef]
- Lebeck, A.O. Principles and Design of Mechanical Face Seals; Wiley: Hoboken, NJ, USA, 1991. [Google Scholar]
- Towsyfyan, H. Investigation of the Nonlinear Tribological Behaviour of Mechanical Seals for Online Condition Monitoring. Doctoral Dissertation, University of Huddersfield, Huddersfield, UK, 2018. [Google Scholar]
- Gani, M.; Santos, I.F.; Arghir, M.; Grann, H. Model validation of mechanical face seals in two-phase flow conditions. Tribol. Int. 2022, 167, 107417. [Google Scholar] [CrossRef]
- Li, Q.-P.; Zhang, J.-Y.; Zhang, J.-X.; Zhang, Y.-X. Influence of pressure disturbance wave on dynamic response characteristics of liquid film seal for multiphase pump. Pet. Sci. 2024, 21, 2048–2065. [Google Scholar] [CrossRef]
- Yu, B.; Hao, M.; Xinhui, S.; Wang, Z.; Fuyu, L.; Yongfan, L. Analysis of dynamic characteristics of spiral groove liquid film seal under thermal–fluid–solid coupling. Ind. Lubr. Tribol. 2021, 73, 882–890. [Google Scholar] [CrossRef]
- Huang, W.; Liao, C.; Liu, X.; Suo, S.; Liu, Y.; Wang, Y. Thermal fluid-solid interaction model and experimental validation for hydrostatic mechanical face seals. Chin. J. Mech. Eng. 2014, 27, 949–957. [Google Scholar] [CrossRef]
- Liu, X.; Peng, X.D.; Meng, X.K. Thermo-elasto-hydrostatic effect analysis of the double tapered hydrostatic mechanical seals for reactor coolant pumps under the influence of flow regime. In Proceedings of the 2012 Asia-Pacific Power and Energy Engineering Conference, Shanghai, China, 27–29 March 2012; pp. 1–6. [Google Scholar]
- Zhou, X.; Chen, Z.; Gu, C.; Wang, J. Thermo-mechanical coupling analysis of the end faces for a mechanical seal under dry friction. Tribol. Int. 2021, 160, 107050. [Google Scholar] [CrossRef]
- Ni, X.; Sun, J.; Ma, C.; Zhang, Y. Wear Model of a Mechanical Seal Based on Piecewise Fractal Theory. Fractal Fract. 2023, 7, 251. [Google Scholar] [CrossRef]
- Li, X.; Yang, Z.; Xu, J.; Chen, R.; Yang, H. The fractal leakage model of contact mechanical seals considering wear and thermal deformation. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 521. [Google Scholar] [CrossRef]
- Ni, X.; Ma, C.; Sun, J.; Zhang, Y.; Yu, Q. A leakage model of contact mechanical seals based on the fractal theory of porous medium. Coatings 2021, 11, 20. [Google Scholar] [CrossRef]
- Zheng, W.; Sun, J.; Ma, C.; Yu, Q. Interface leakage theory of mechanical seals considering microscopic forces. Coatings 2023, 13, 1435. [Google Scholar] [CrossRef]
- Sinou, J.J.; Cayer-Barrioz, J.; Berro, H. Friction-induced vibration of a lubricated mechanical system. Tribol. Int. 2013, 61, 156–168. [Google Scholar] [CrossRef]
- Akay, A. Acoustics of friction. J. Acoust. Soc. Am. 2002, 111, 1525–1548. [Google Scholar] [CrossRef]
- Sharma, R.B.; Parey, A. Modelling of acoustic emission generated in rolling element bearing. Appl. Acoust. 2019, 144, 96–112. [Google Scholar] [CrossRef]
- Kaya, S.; Yilan, F.; Urtekin, L. Influences of Cr on the microstructural, wear and mechanical performance of high-chromium white cast iron grinding balls. SAE Int. J. Mater. Manuf. 2023, 1, 23–30. [Google Scholar] [CrossRef]
- Köse, R.; Urtekin, L.; Ceylan, A.; Salman, S.; Findik, F. Three types of ceramic coating applicability in automotive industry for wear resistance purpose. Ind. Lubr. Tribol. 2005, 57, 140–144. [Google Scholar] [CrossRef]
- Mousavi, S.B.; Heris, S.Z.; Estellé, P. Experimental comparison between ZnO and MoS2 nanoparticles as additives on performance of diesel oil-based nano lubricant. Sci. Rep. 2020, 10, 5813. [Google Scholar] [CrossRef]
- Mousavi, S.B.; Pourpasha, H.; Heris, S.Z. High-temperature lubricity and physicochemical behaviors of synthesized Cu/TiO2/MnO2-doped GO nanocomposite in high-viscosity index synthetic biodegradable PAO oil. Int. Commun. Heat Mass Transf. 2024, 156, 107642. [Google Scholar] [CrossRef]
- Mousavi, S.B.; Zeinali Heris, S.; Hosseini, M.G. Experimental investigation of MoS2/diesel oil nanofluid thermophysical and rheological properties. Int. Commun. Heat Mass Transf. 2019, 108, 104298. [Google Scholar] [CrossRef]
- Mousavi, S.B.; Zeinali Heris, S.; Estellé, P. Viscosity, tribological and physicochemical features of ZnO and MoS2 diesel oil-based nanofluids: An experimental study. Fuel 2021, 293, 120481. [Google Scholar] [CrossRef]
- Aghaei Sarvari, A.; Zeinali Heris, S.; Mohammadpourfard, M.; Mousavi, S.B.; Estellé, P. Numerical investigation of TiO2 and MWCNTs turbine meter oil nanofluids: Flow and hydrodynamic properties. Fuel 2022, 320, 123943. [Google Scholar] [CrossRef]
- Zhang, E.Q.; Fu, P.; Li, K.; Li, X.; Zhou, Z. Film thickness measurement of mechanical seal based on cascaded artificial neural network recognition model. Int. J. Smart Sens. Intell. Syst. 2014, 7, 1870–1889. [Google Scholar] [CrossRef]
- Huang, D.; Yan, X.; Larsson, R.; Almqvist, A. Numerical simulation of static seal contact mechanics including hydrostatic load at the contacting interface. Lubricants 2021, 9, 1. [Google Scholar] [CrossRef]
- Luo, Y.; Zhang, W.; Fan, Y.; Han, Y.; Li, W.; Acheaw, E. Analysis of vibration characteristics of centrifugal pump mechanical seal under wear and damage degree. Shock Vib. 2021, 2021, 6670741. [Google Scholar] [CrossRef]
- Wang, Q.; Song, Y.; Li, H.; Shu, Y.; Xiao, Y. Tribological behavior characterization and fault detection of mechanical seals based on face vibration acceleration measurements. Lubricants 2023, 11, 430. [Google Scholar] [CrossRef]
- Dowson, D. A generalized Reynolds equation for fluid-film lubrication. Int. J. Mech. Sci. 1962, 4, 159–170. [Google Scholar] [CrossRef]
- Wang, S.T.; Wu, O.Y.; Li, Z.; Wang, B. Load carrying capacity of a novel magnetic-liquid double suspension fixed pad thrust bearing. Ind. Lubr. Tribol. 2021, 73, 381–387. [Google Scholar] [CrossRef]
- Greenwood, J.; Williamson, J. Contact of nominally flat surfaces. Proc. R. Soc. Lond. (A) 1966, 295, 300–319. [Google Scholar] [CrossRef]
- Persson, B.N.J. Contact mechanics for randomly rough surfaces. Surf. Sci. Rep. 2006, 61, 201–227. [Google Scholar] [CrossRef]
- Lin, Z.; Sayer, P. Influence of water depth variation on the hydrodynamics of deep-water mooring characteristics. Ocean Eng. 2015, 109, 553–566. [Google Scholar] [CrossRef][Green Version]
- Gross, D.; Ehlers, W.; Wriggers, P.; Schröder, J.; Müller, R. Mechanics of Materials–Formulas and Problems; Springer: Berlin/Heidelberg, Germany, 2017. [Google Scholar]
- Hurtado, J.A.; Kim, K.S. Scale effects in friction of single–asperity contacts. II. Multiple–dislocation–cooperated slip. Proc. R. Soc. A Math. Phys. Eng. Sci. 1999, 455, 3363–3384. [Google Scholar] [CrossRef]
- Hibbeler, R. Mechanics of Materials; SI Edition; Pearson Schweiz: Amsterdam, The Netherlands, 2013. [Google Scholar]
- Archard, J.F. Contact and rubbing of flat surfaces. J. Appl. Phys. 1953, 24, 981–988. [Google Scholar] [CrossRef]
- Ibrahim, R.A. Friction-induced vibration, chatter, squeal, and chaos—Part I: Mechanics of contact and friction. Appl. Mech. Rev. 1994, 47, 227. [Google Scholar] [CrossRef]
- MIL-PRF-23699F; Performance Specification: Lubricating Oil, Aircraft Turbine Engine, Synthetic Base, Nato Code Number O-156. United States Military Standard: Washington, DC, USA, 1997.
- Liu, X.; Xia, J.; Zhou, M.; Deng, S.; Li, Z. A new formula for predicting movable bed roughness coefficient in the Middle Yangtze River. Progr. Phys. Geogr. Earth Environ. 2022, 46, 441–457. [Google Scholar] [CrossRef]
- JB/T 4127.1-2013; Mechanical Seals Part 1: Technical Conditions. Ministry of Industry and Information Technology: Beijing, China, 2013.
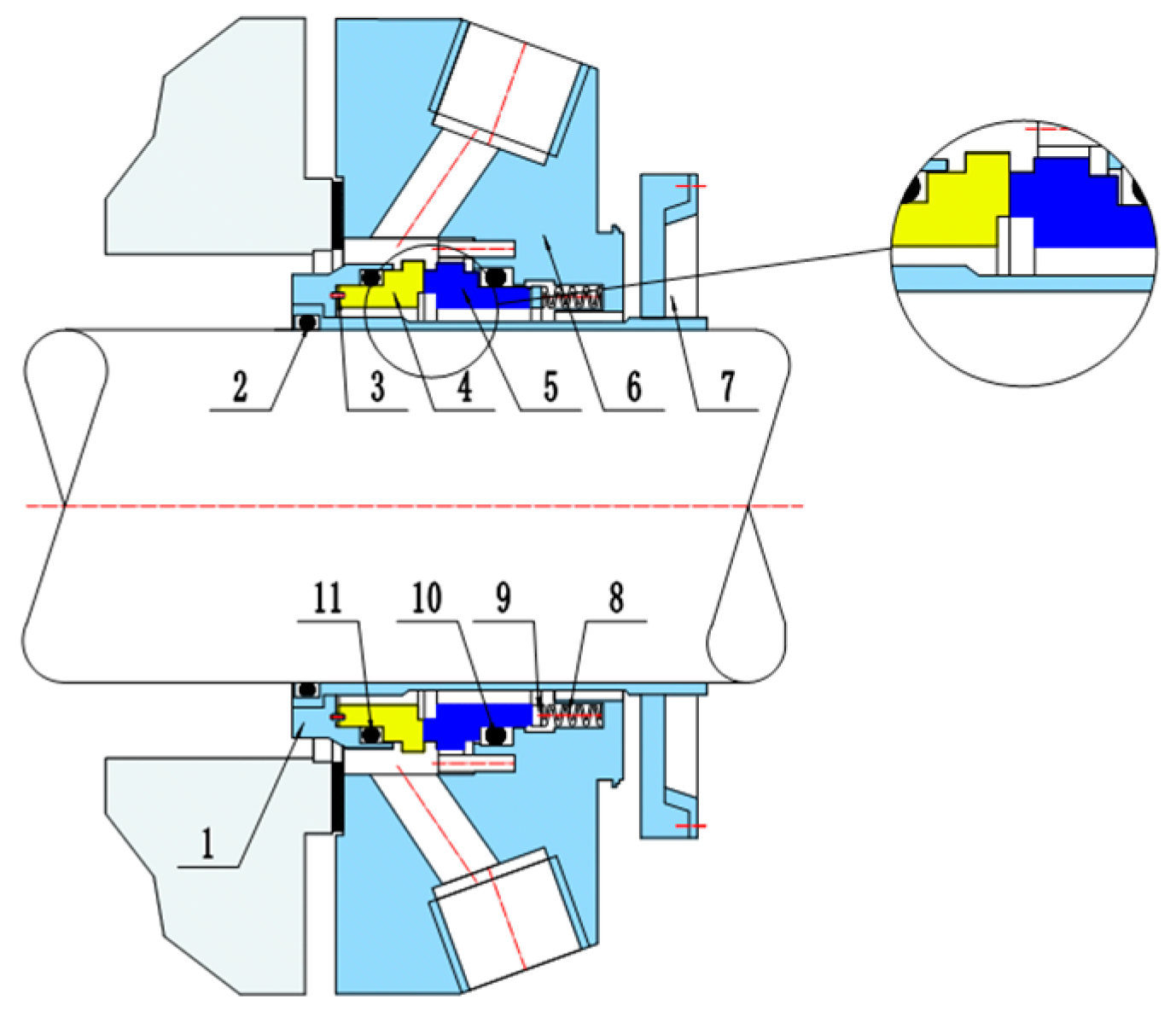
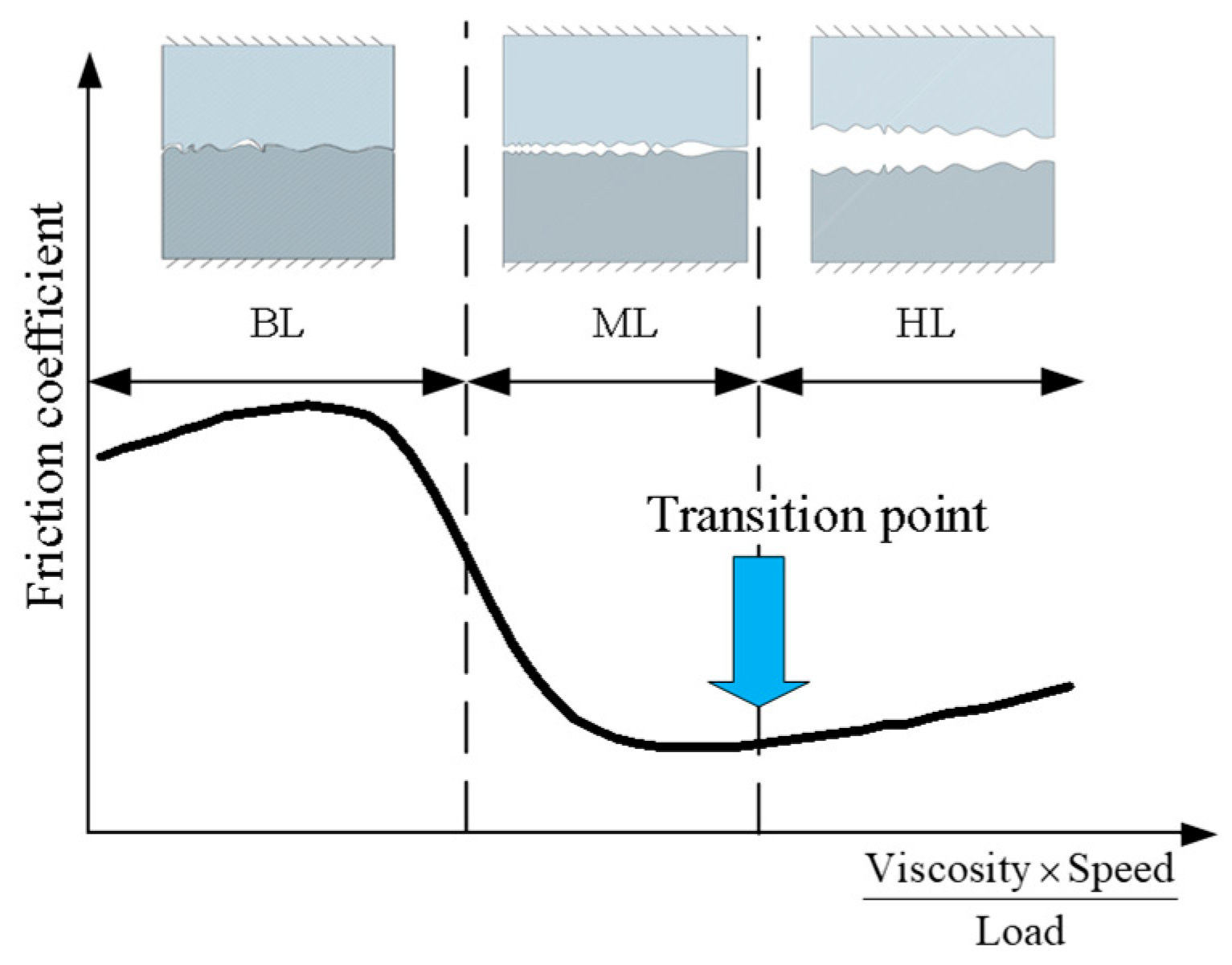
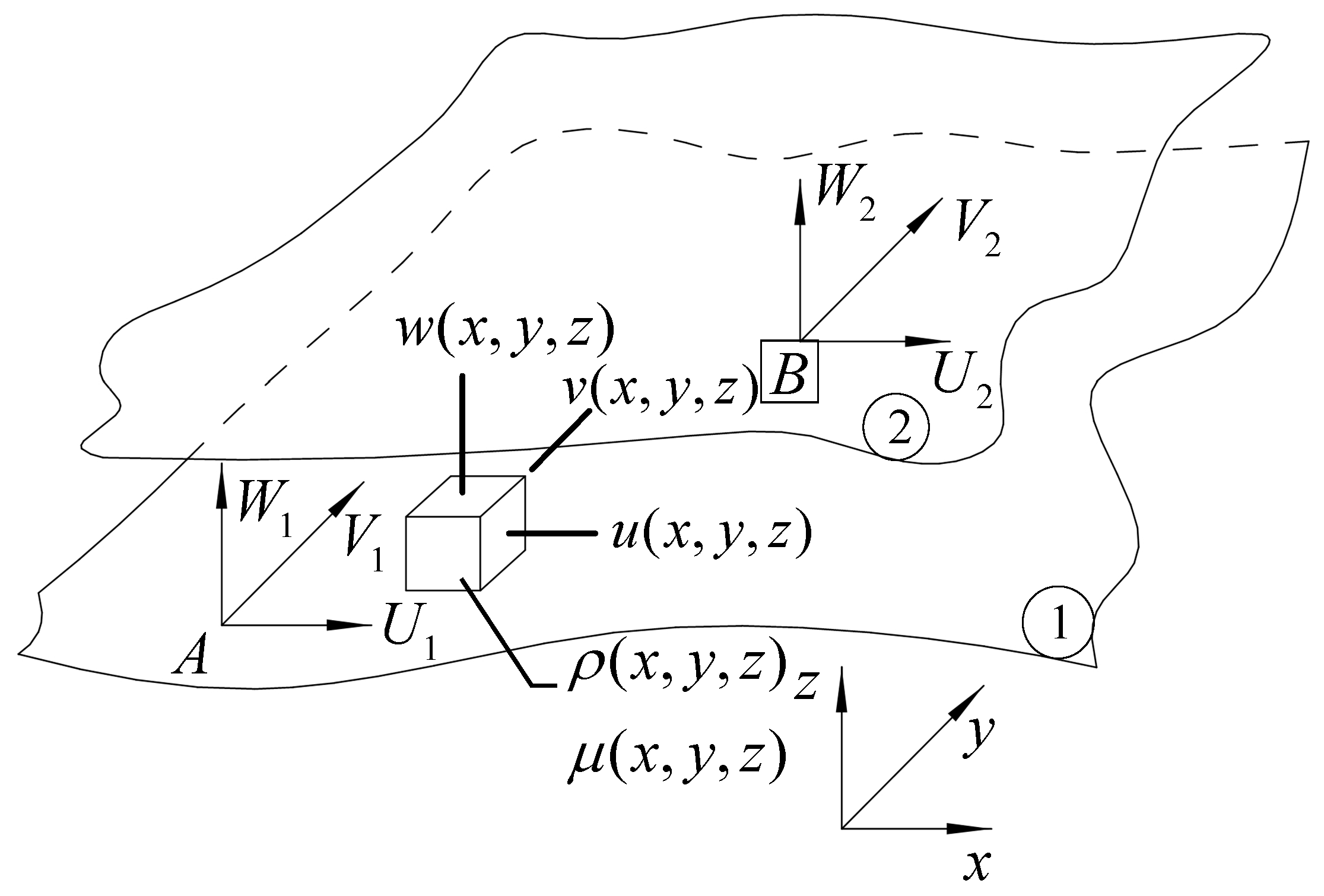
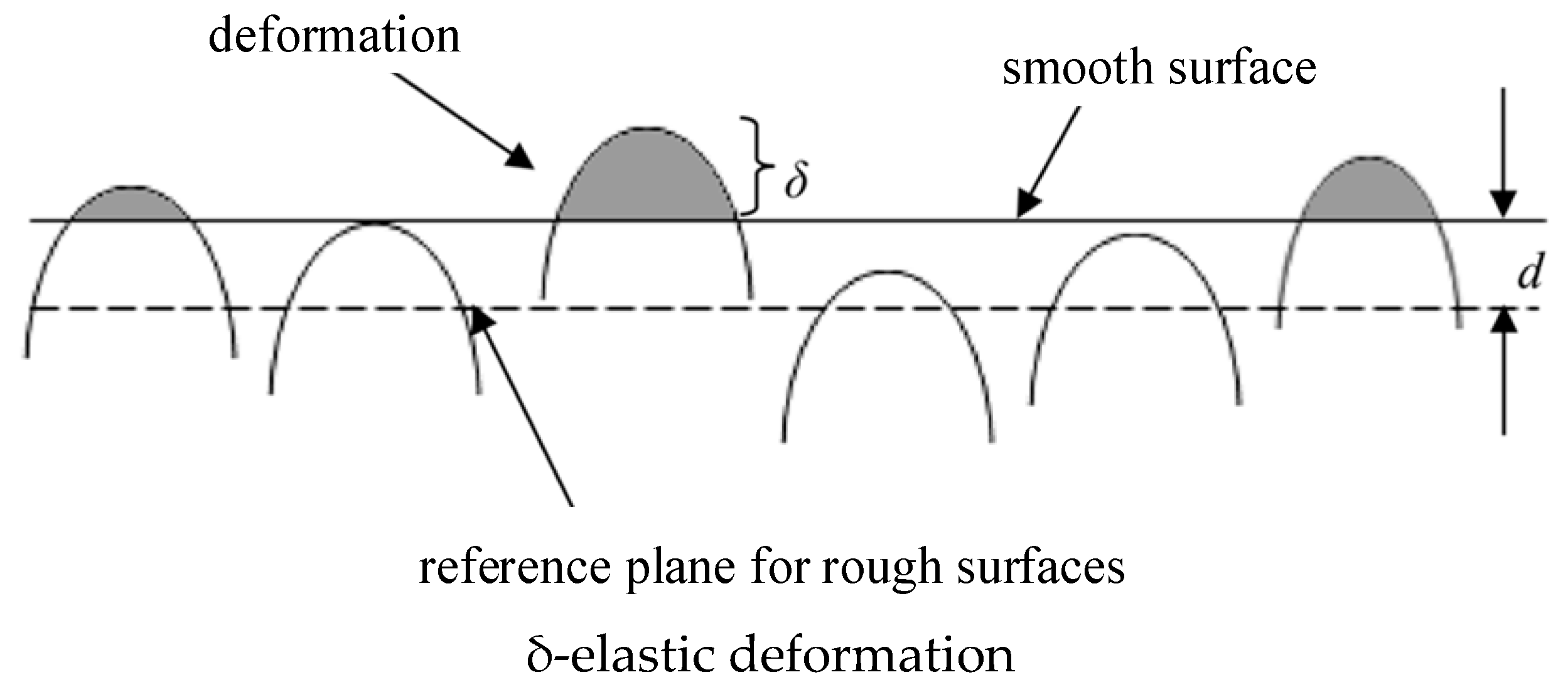
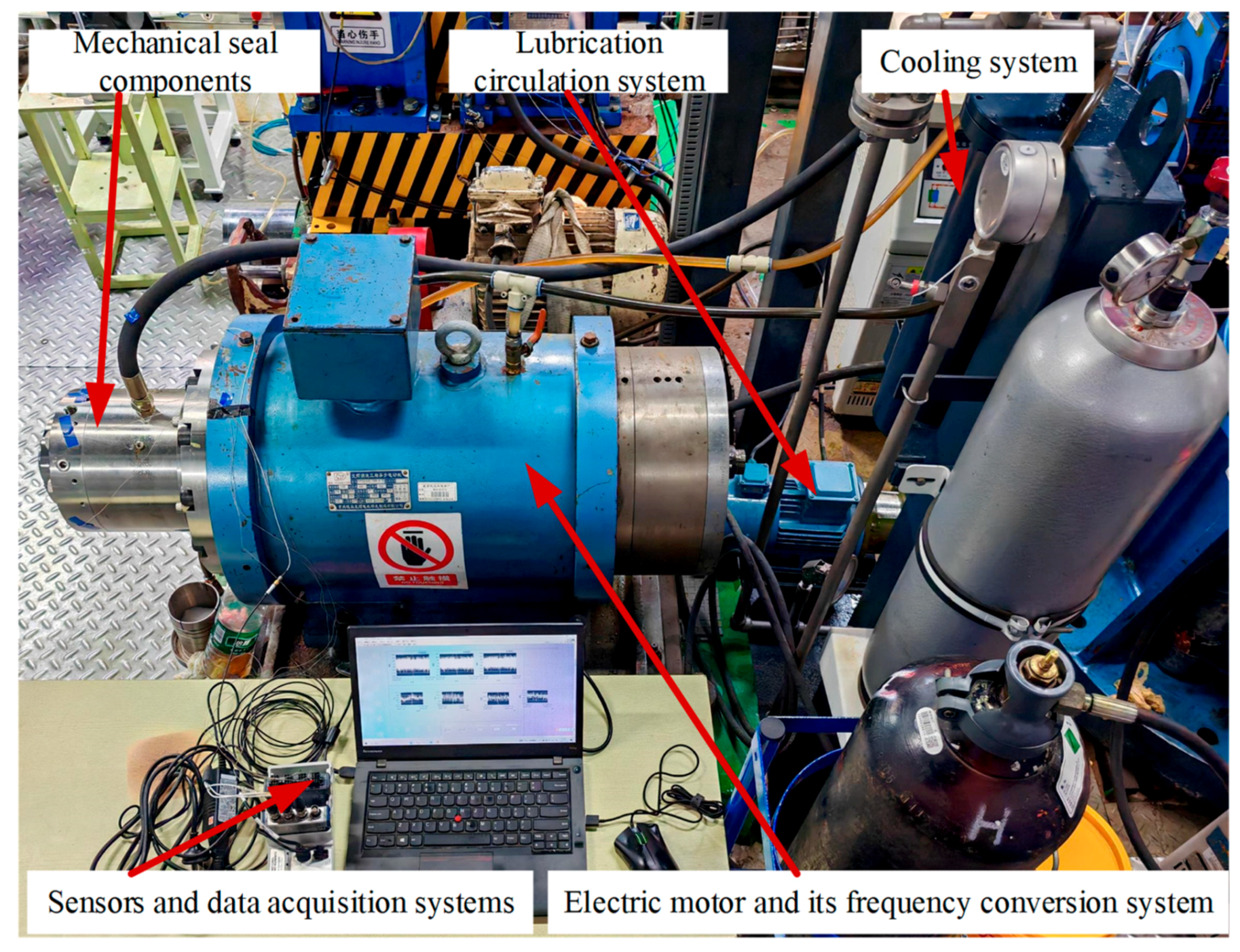
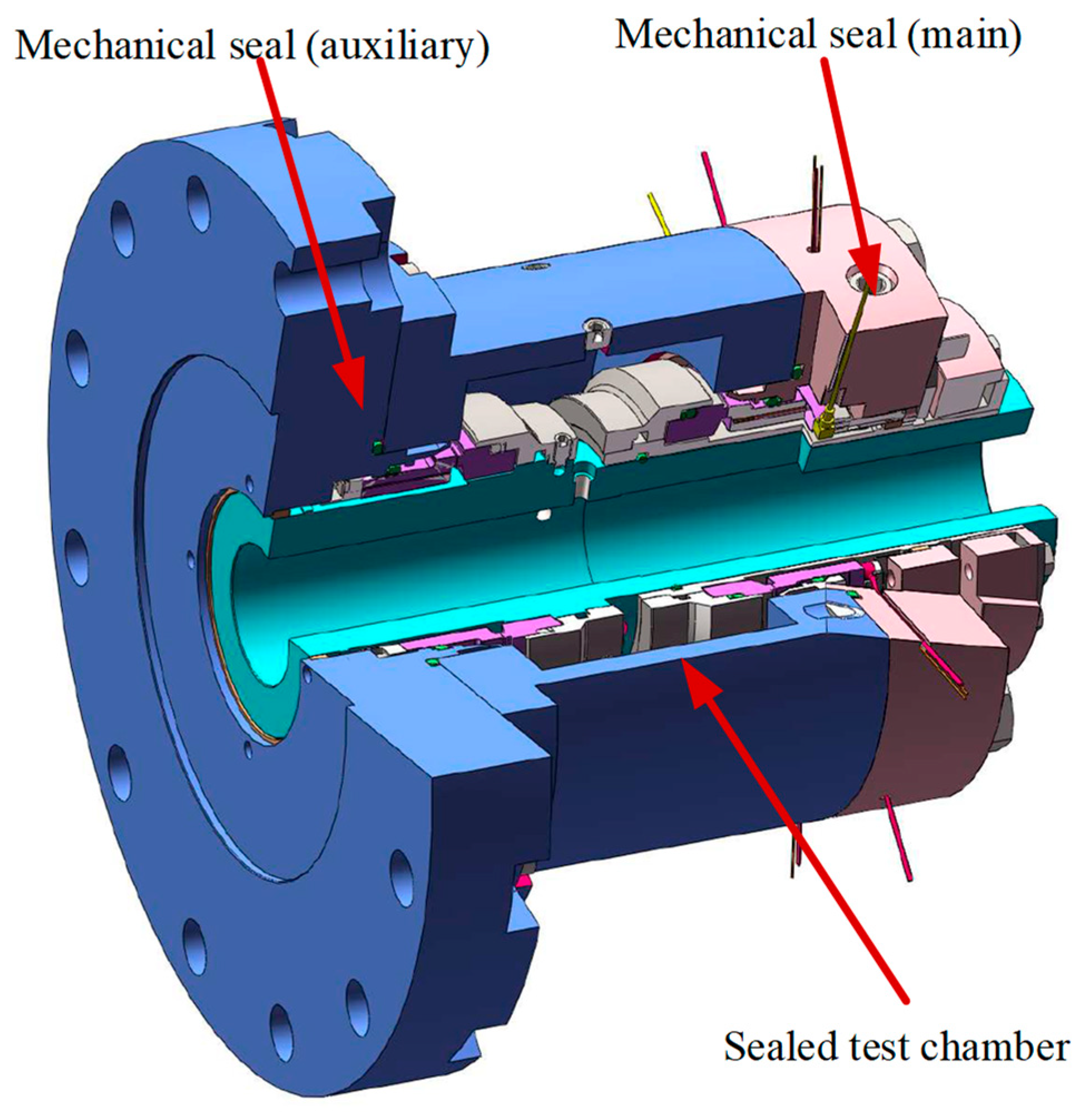
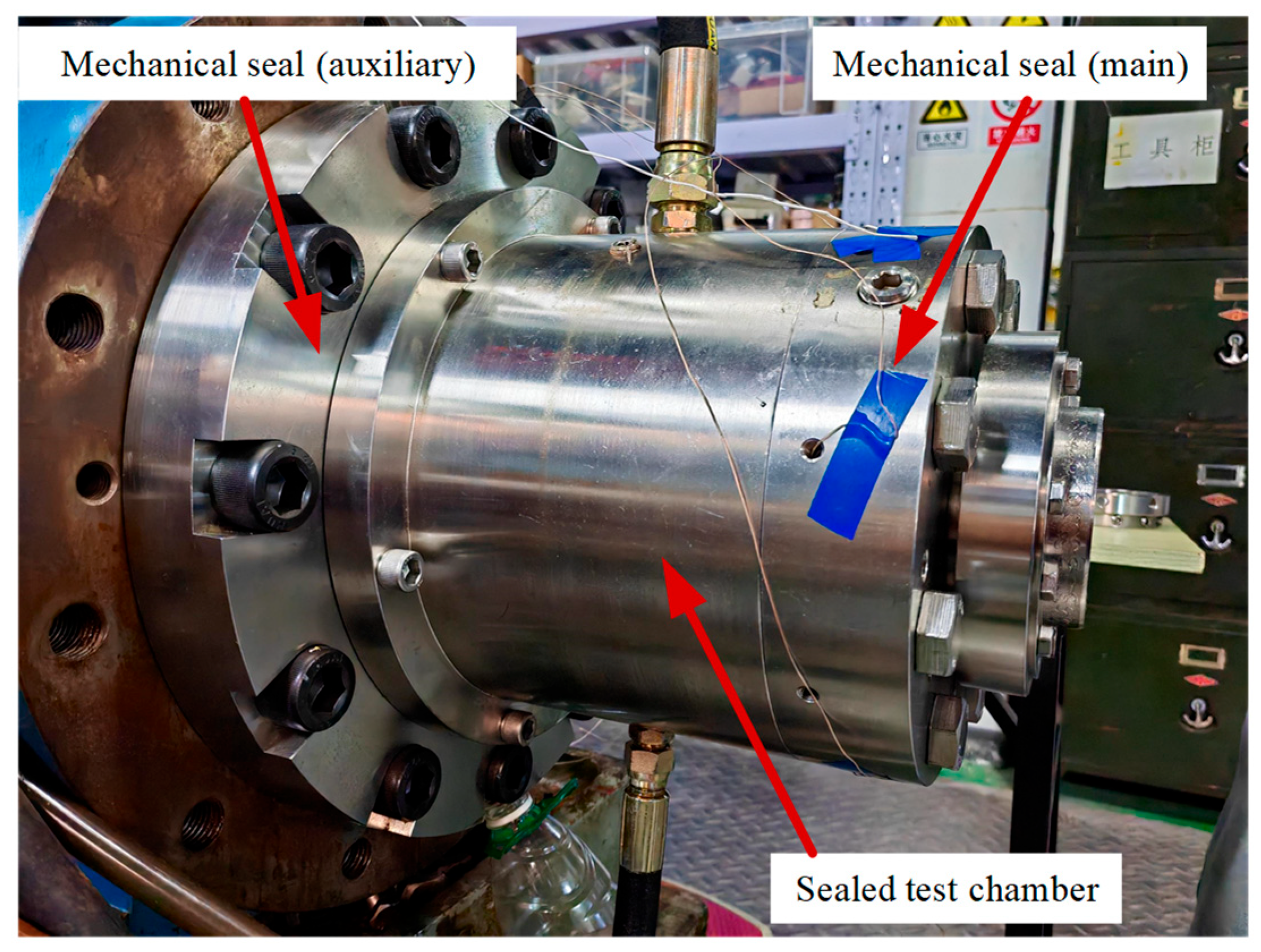
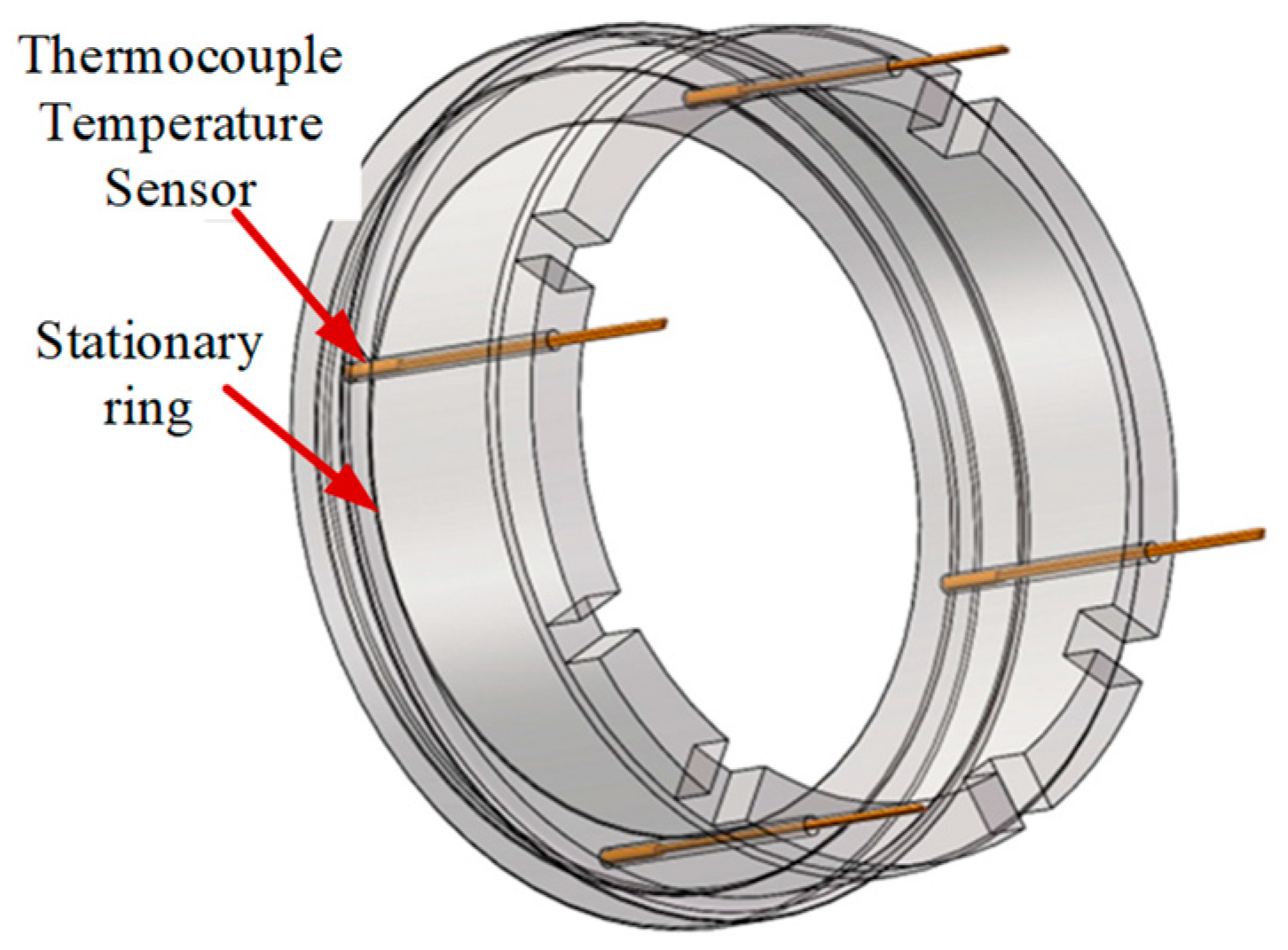
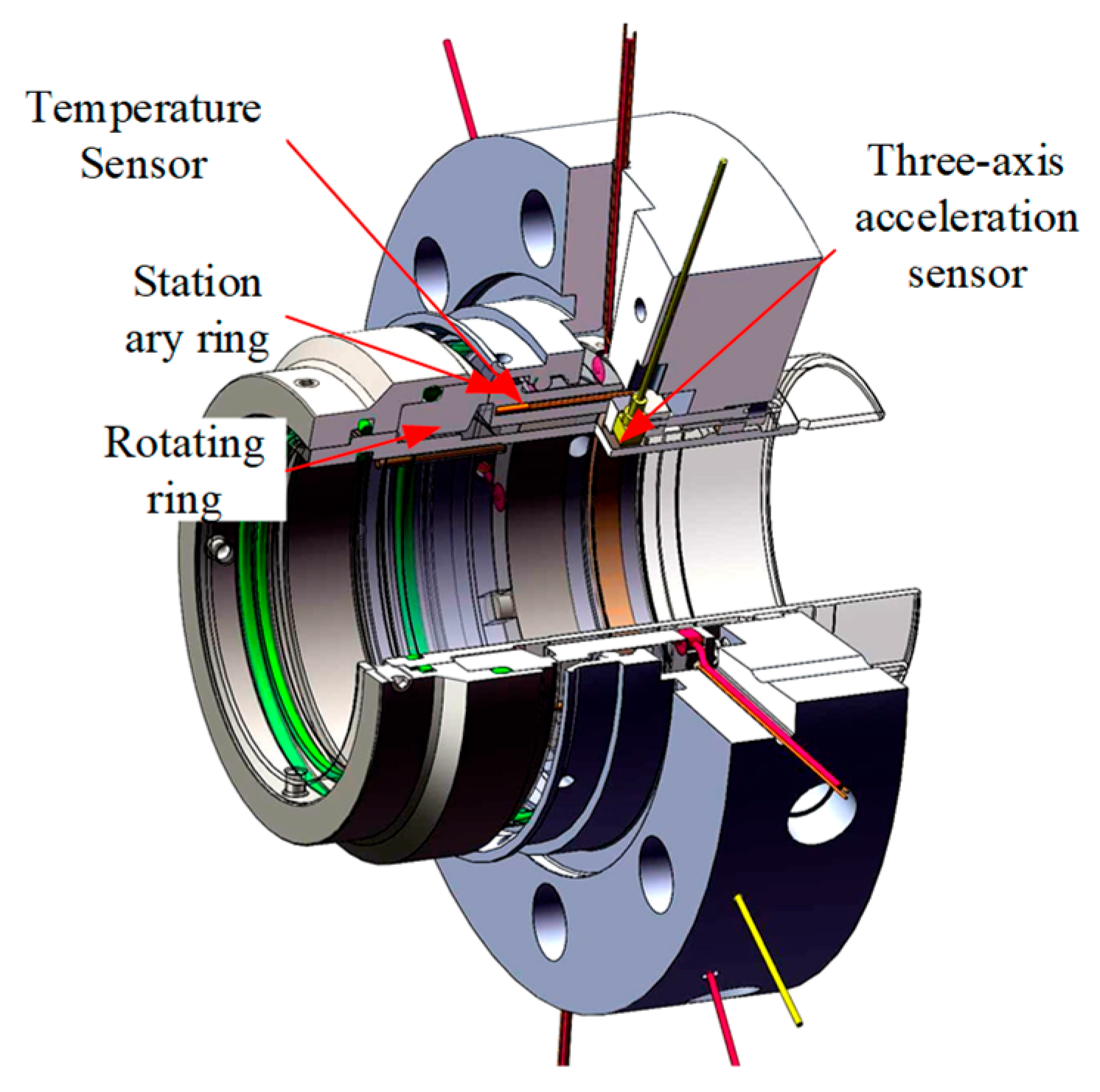












| Project | Material | Young’s Modulus | Poisson Ratio | Inside Diameter | Outside Diameter | Height |
|---|---|---|---|---|---|---|
| Stationary ring | SIC | 475 GPa | 0.142 | 126 mm | 137 mm | 42 mm |
| Rotating ring | 27 mm |
| Model | PCB 356a03 |
|---|---|
| Sensitivity | 10 mv/g |
| Range | ±500 g |
| Resolution | 0.003 g rms |
| Frequency response range | 2~5000 Hz |
| Installation method | Bonding |
| Size | 6.35 × 6.35 × 6.35 mm |
| Model | Pt100 |
|---|---|
| Range | −50 °C–400 °C |
| Precision | 0.3 °C |
| Type | Aero Oil |
|---|---|
| Implementation standards | MIL-PRF-23699 [40] |
| Component | Synthetic ester oil (pentaerythritol ester) |
| Kinematic viscosity at 100 °C | 5.0 mm2/s |
| Kinematic viscosity at 40 °C | 27.6 mm2/s |
| Kinematic viscosity at −40 °C | 11,000 mm2/s |
| Pour point | −59 °C |
| Flash point | 270 °C |
| Project | Condition 1 | Condition 2 | Condition 3 | Condition 4 | Condition 5 |
|---|---|---|---|---|---|
| Spindle speed | 1000 r/min | 1500 r/min | 2000 r/min | 2500 r/min | 3000 r/min |
| Inverter frequency | 17 Hz | 25 Hz | 33 Hz | 42 Hz | 50 Hz |
| Pressure | 1.6 MPa | 1.6 MPa | 1.6 MPa | 1.6 MPa | 1.6 MPa |
| Sampling time | 1 s | 1 s | 1 s | 1 s | 1 s |
| Sampling frequency | 25.6 kHz | 25.6 kHz | 25.6 kHz | 25.6 kHz | 25.6 kHz |
| Sampling points | 25,600 | 25,600 | 25,600 | 25,600 | 25,600 |
| Sampling duration | 1 min | 1 min | 1 min | 1 min | 1 min |
| Face vibration acceleration | Axial, radial, tangential | Axial, radial, tangential | Axial, radial, tangential | Axial, radial, tangential | Axial, radial, tangential |
| Project | Condition 1 | Condition 2 | Condition 3 | Condition 4 | Condition 5 | Condition 6 | Condition 7 | Condition 8 | Condition 9 |
|---|---|---|---|---|---|---|---|---|---|
| Pressure | 1.2 MPa | 1.3 MPa | 1.4 MPa | 1.5 MPa | 1.6 MPa | 1.7 MPa | 1.8 MPa | 1.9 MPa | 2.0 MPa |
| Spindle speed | 3000 r/min | 3000 r/min | 3000 r/min | 3000 r/min | 3000 r/min | 3000 r/min | 3000 r/min | 3000 r/min | 3000 r/min |
| Sampling time | 1 s | 1 s | 1 s | 1 s | 1 s | 1 s | 1 s | 1 s | 1 s |
| Sampling frequency | 25.6 kHz | 25.6 kHz | 25.6 kHz | 25.6 kHz | 25.6 kHz | 25.6 kHz | 25.6 kHz | 25.6 kHz | 25.6 kHz |
| Sampling points | 25,600 | 25,600 | 25,600 | 25,600 | 25,600 | 25,600 | 25,600 | 25,600 | 25,600 |
| Sampling duration | 1 min | 1 min | 1 min | 1 min | 1 min | 1 min | 1 min | 1 min | 1 min |
| Face vibration acceleration | Axial, radial, tangential | Axial, radial, tangential | Axial, radial, tangential | Axial, radial, tangential | Axial, radial, tangential | Axial, radial, tangential | Axial, radial, tangential | Axial, radial, tangential | Axial, radial, tangential |
| Rotating Ring Number | Surface Roughness Ra/μm |
|---|---|
| 1# | 0.07 |
| 2# | 0.09 |
| 3# | 0.20 |
| 4# | 0.32 |
| Project | Condition 1 | Condition 2 | Condition 3 | Condition 4 |
|---|---|---|---|---|
| Face roughness | 0.07 μm | 0.09 μm | 0.20 μm | 0.32 μm |
| Pressure | 1.6 MPa | 1.6 MPa | 1.6 MPa | 1.6 MPa |
| Spindle speed | 3000 r/min | 3000 r/min | 3000 r/min | 3000 r/min |
| Sampling time | 1 s | 1 s | 1 s | 1 s |
| Sampling frequency | 25.6 kHz | 25.6 kHz | 25.6 kHz | 25.6 kHz |
| Sampling points | 25,600 | 25,600 | 25,600 | 25,600 |
| Sampling duration | 1 min | 1 min | 1 min | 1 min |
| Face vibration acceleration | Axial, radial, tangential | Axial, radial, tangential | Axial, radial, tangential | Axial, radial, tangential |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, Y.; Li, H.; Xiao, W.; Li, S.; Wang, Q. Research on the Correlation between Mechanical Seal Face Vibration and Stationary Ring Dynamic Behavior Characteristics. Lubricants 2024, 12, 316. https://doi.org/10.3390/lubricants12090316
Song Y, Li H, Xiao W, Li S, Wang Q. Research on the Correlation between Mechanical Seal Face Vibration and Stationary Ring Dynamic Behavior Characteristics. Lubricants. 2024; 12(9):316. https://doi.org/10.3390/lubricants12090316
Chicago/Turabian StyleSong, Yunfeng, Hua Li, Wang Xiao, Shuangxi Li, and Qingfeng Wang. 2024. "Research on the Correlation between Mechanical Seal Face Vibration and Stationary Ring Dynamic Behavior Characteristics" Lubricants 12, no. 9: 316. https://doi.org/10.3390/lubricants12090316
APA StyleSong, Y., Li, H., Xiao, W., Li, S., & Wang, Q. (2024). Research on the Correlation between Mechanical Seal Face Vibration and Stationary Ring Dynamic Behavior Characteristics. Lubricants, 12(9), 316. https://doi.org/10.3390/lubricants12090316





