Calculation and Analysis of Equilibrium Position of Aerostatic Bearings Based on Bivariate Interpolation Method
Abstract
1. Introduction
2. Building and Calculation of the BIM
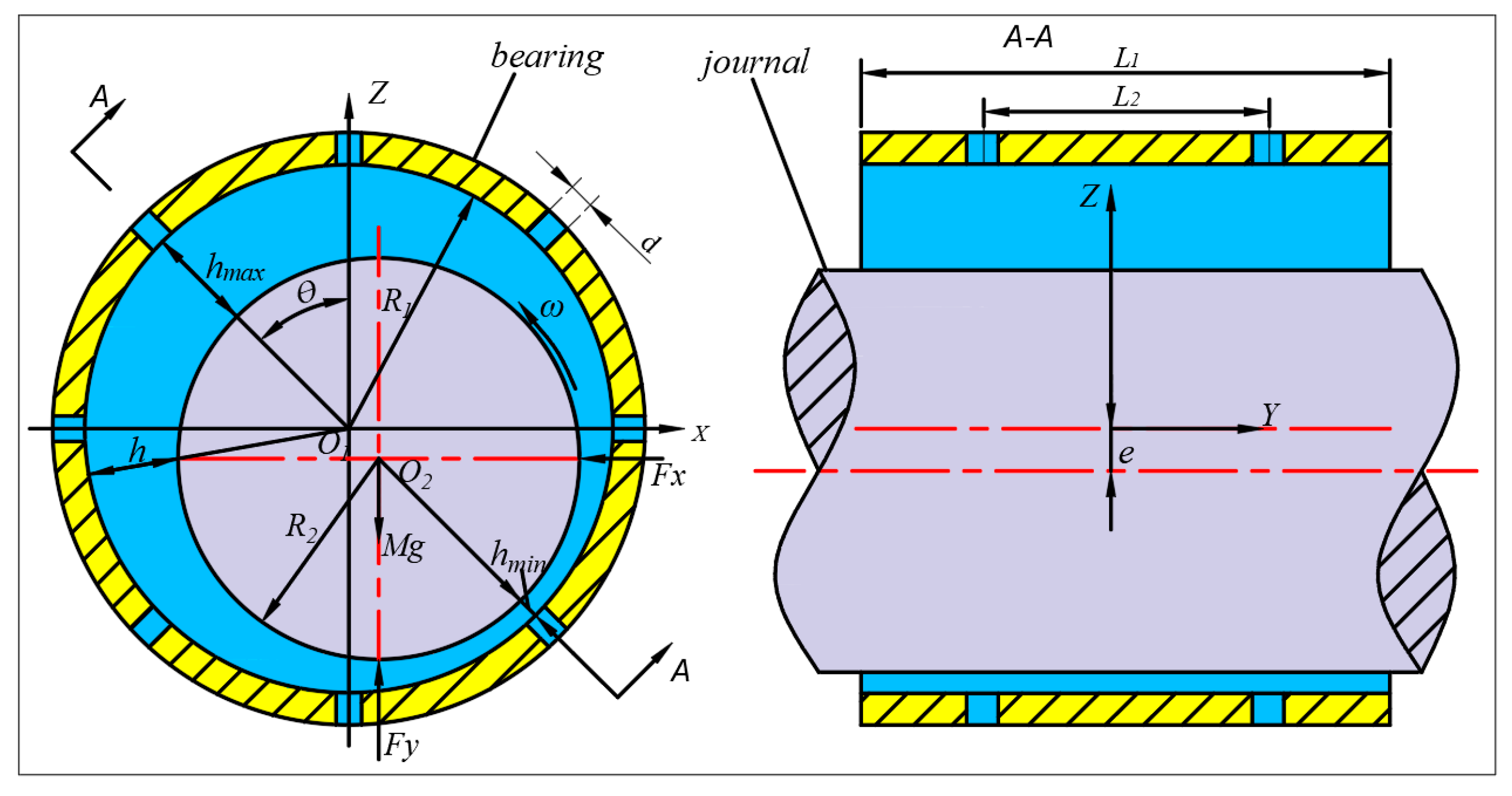
2.1. Establishment of Mathematical Model of Bearing
2.2. Mathematical Model of the BIM
- (1)
- After completing a calculation in the vertical direction, the horizontal bearing capacity fails to satisfy the convergence requirements, necessitating a recalculation of the abscissa. Therefore, until the ordinate satisfies the convergence requirements, each correction of the ordinate requires iterative adjustments of the abscissa. This process results in a reduction in computational efficiency.
- (2)
- In the iterative calculation process, it is essential to determine the influence factors. Influence factors are used to correct the coordinates. The influence factors vary depending on the rotor’s position. If the influence factors are excessively large, it can result in iterative divergence, whereas if they are excessively small, it can impact computational efficiency.
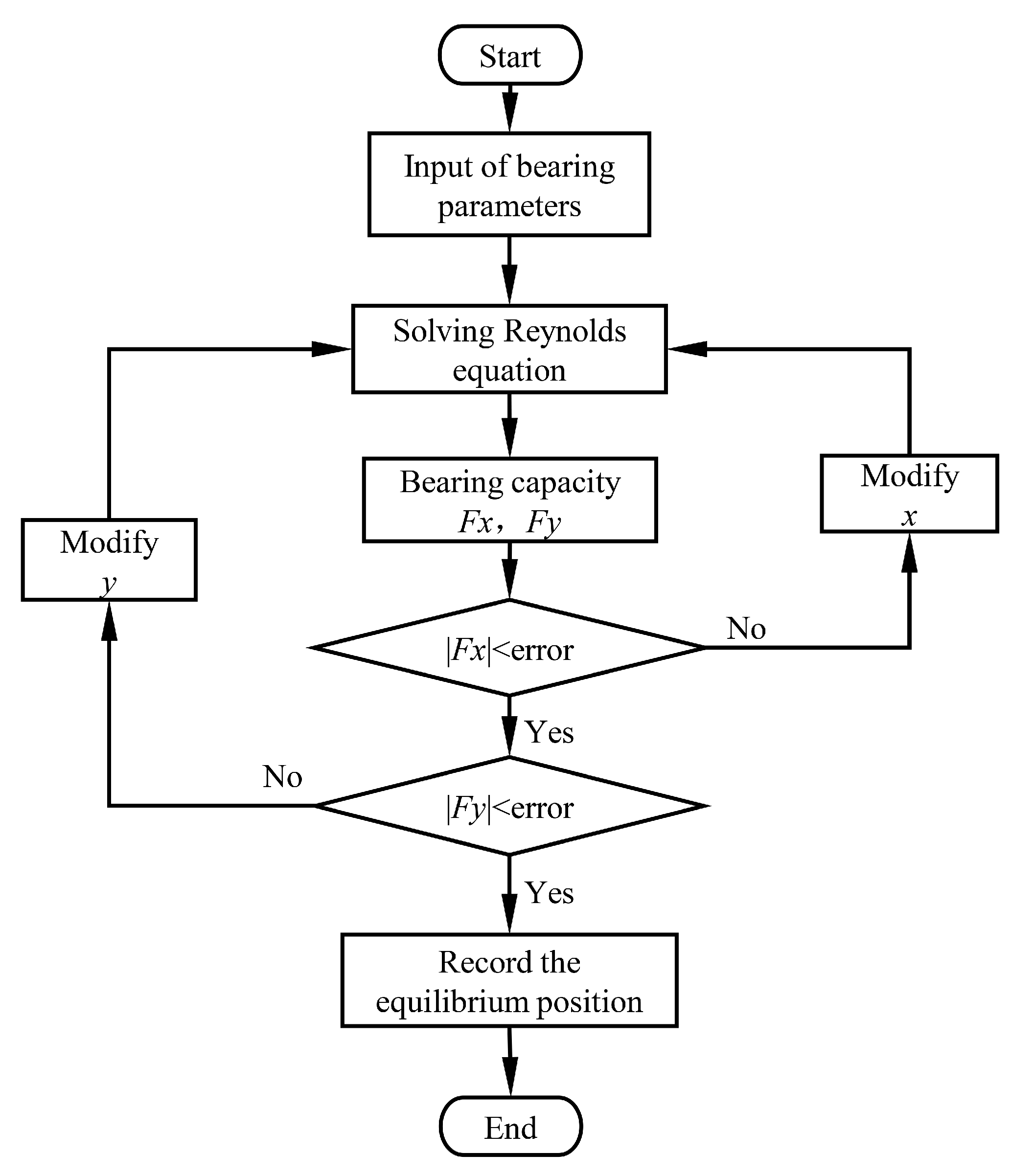
2.3. Calculation Process of the BIM
- (1)
- Given convergence criteria (resultant force N, N, 0.001 N force can be ignored).
- (2)
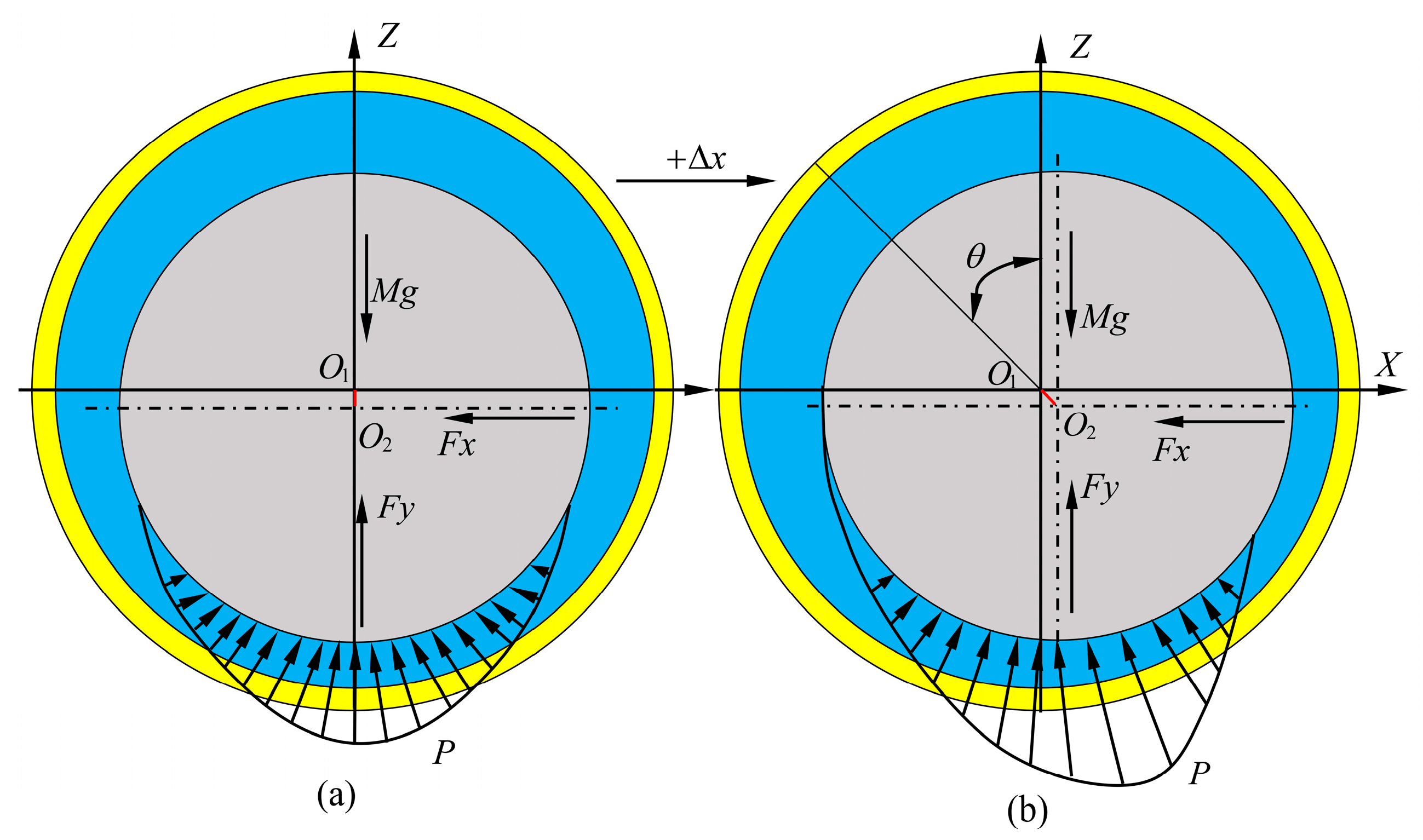
- According to Figure 4, four points , , , in the bearing range are selected, and are calculated.
- (3)
- The air film forces , , , at the corresponding positions were calculated by the Reynolds equation.
- (4)
- Through Equation (8), coordinate points are obtained after two consecutive interpolation calculations.
- (5)
- The air film forces at the position of the fourth step are obtained by the Reynolds equation and compared with the convergence condition. If it is satisfied, the iteration is terminated. If it is not satisfied, proceed to step 6.
- (6)
- Determine the size of the impact factors and and determine the coordinate value that has the greatest influence on the air film force. If > , modify ; otherwise, modify . Repeat steps 2 to 5 after updating the data.
3. Results and Discussion
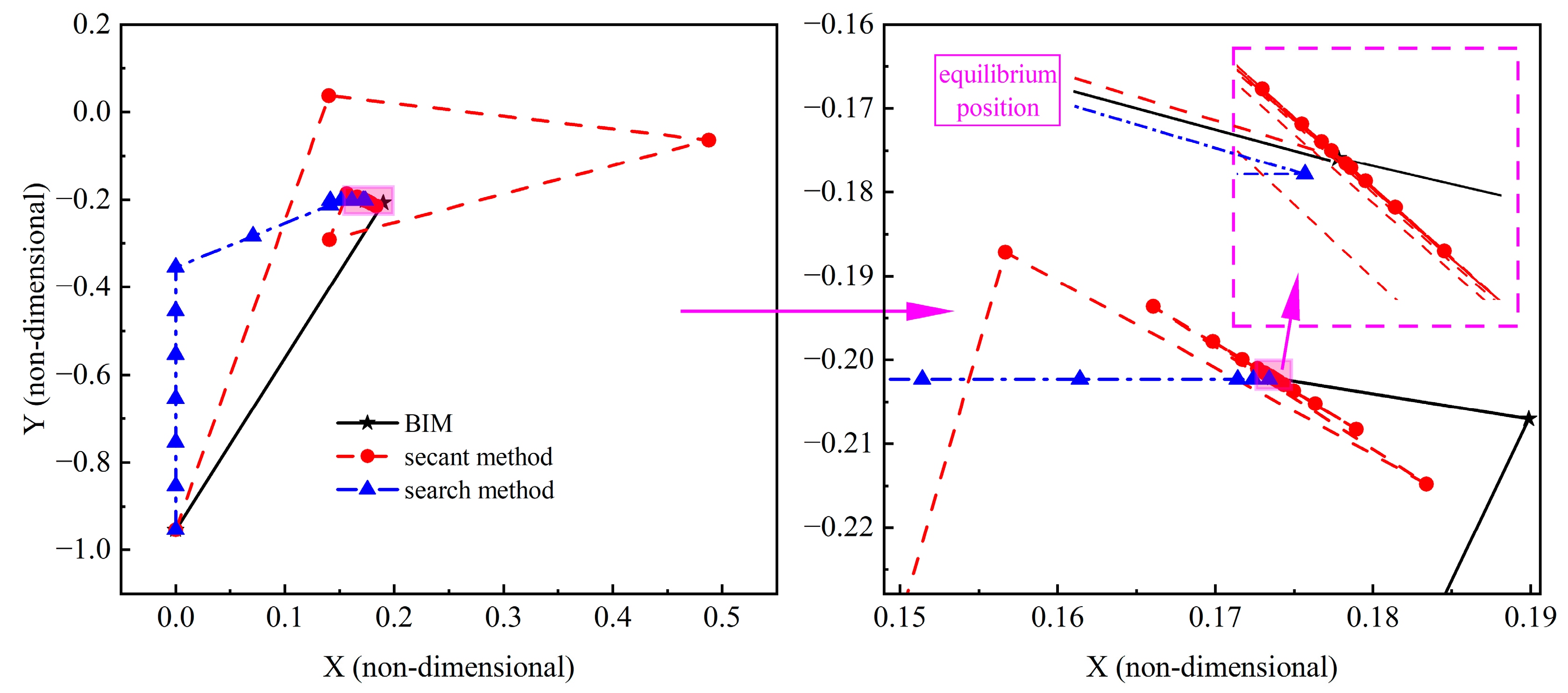
3.1. Comparison of Different Methods
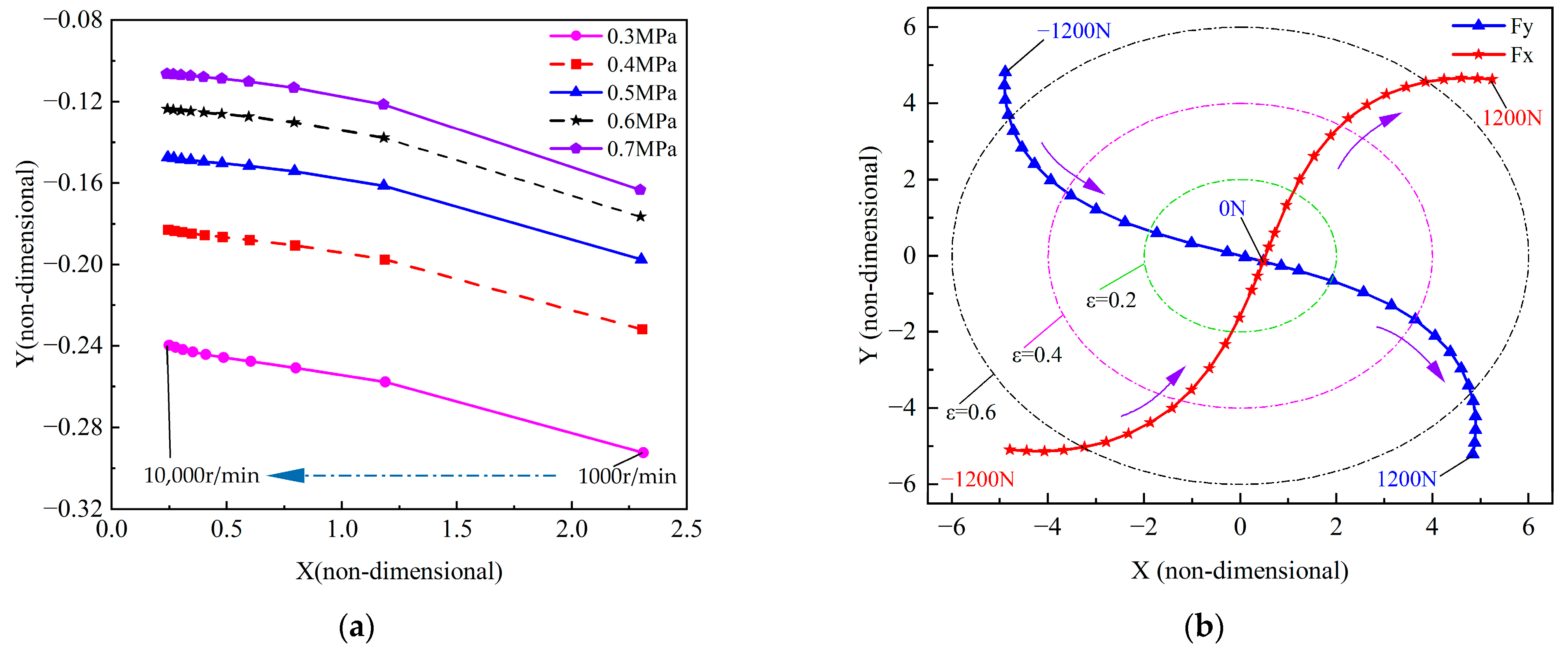
3.2. Calculation of Equilibrium Position Based on the BIM
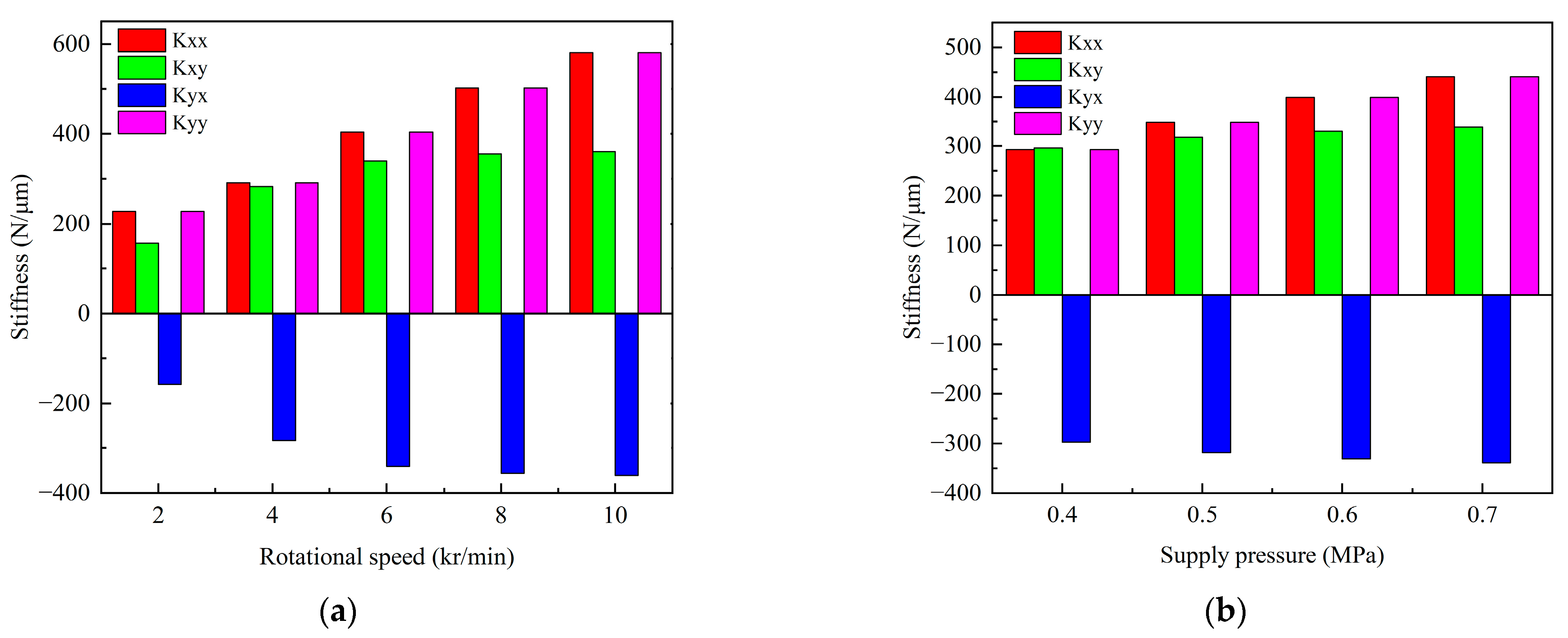
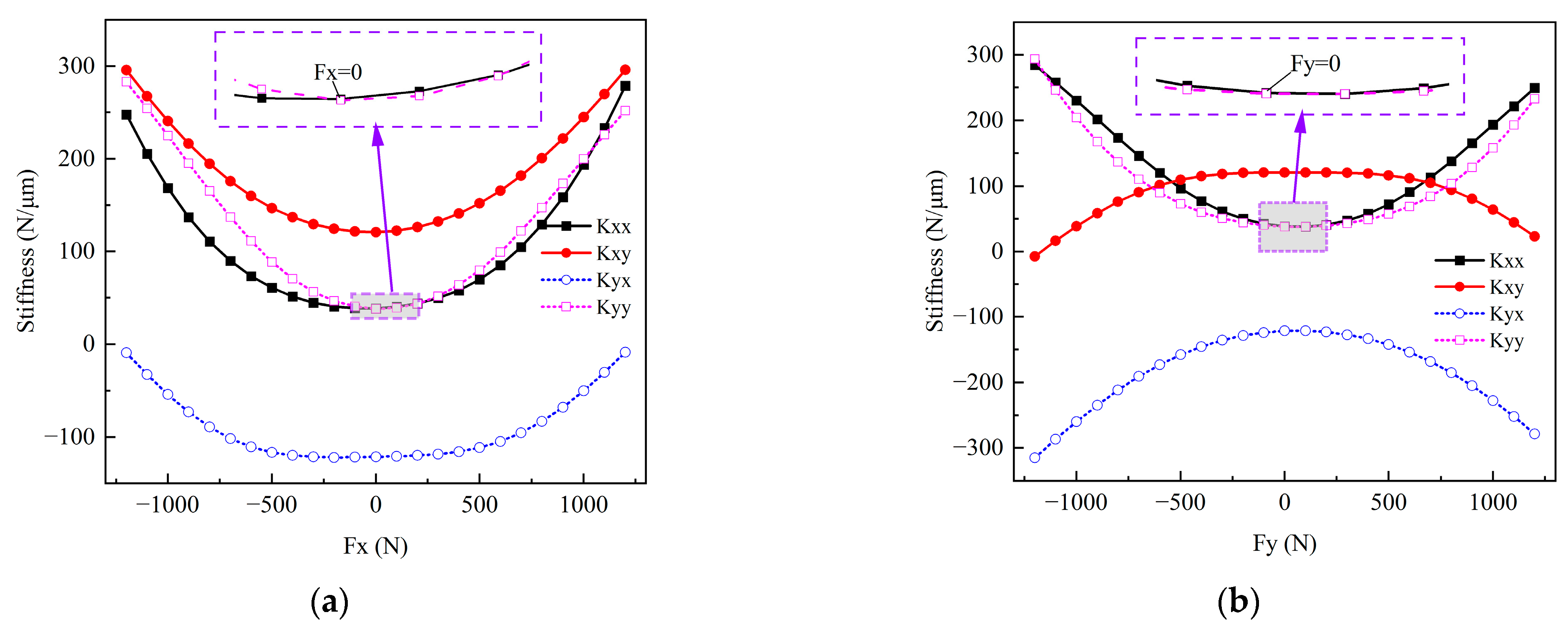
3.3. Calculation of Stiffness of Equilibrium Position Based on the BIM
4. Experiments and Comparison
4.1. Introduction of Experimental Platform
4.2. Experiment Process
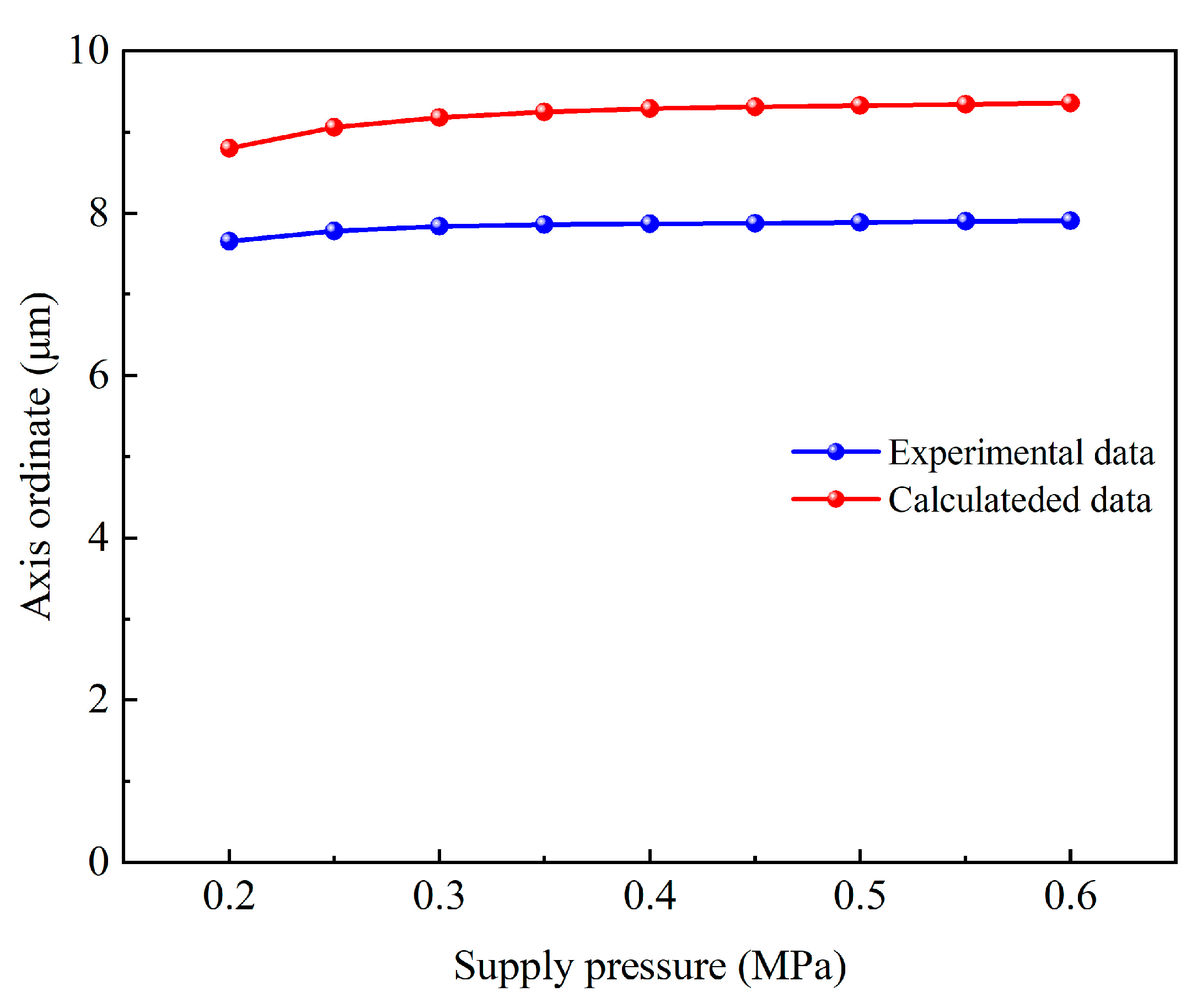
4.3. Discussion of Experimental Results
5. Conclusions
- (1)
- The BIM effectively solves the problem of iteration divergence caused by inappropriate initial value selection. Compared with the calculation results of the secant method and search method, the maximum error of the equilibrium position is 0.15%, the required iterative steps are only 1/4 of those of other methods, and the BIM convergence is better.
- (2)
- When the direction of external load on the rotor is unchanged and the amplitude continues to increase, the eccentricity increases nonlinearly. The equilibrium position initially moves away from the direction of the load and later moves closer to it. The main stiffness of the bearing increases with the increase in the external load, independent of the direction of the external load. When the horizontal external load increases gradually, the absolute value of the cross stiffness Kyx decreases, while the Kxy increases. Conversely, when the vertical external load gradually increases, the absolute value of the cross stiffness Kyx increases, while the Kxy decreases.
- (3)
- Without external load, the change in the equilibrium position and its stiffness with rotational speed and supply pressure is consistent with the conclusions of the references. And the reliability of the BIM is proved by the comparative analysis of the experiment and calculation, and the maximum error between them is 15.4%.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gao, Q.; Chen, W.; Lu, L.; Huo, D.; Cheng, K. Aerostatic bearings design and analysis with the application to precision engineering: State-of-the-art and future perspectives. Tribol. Int. 2019, 135, 1–17. [Google Scholar] [CrossRef]
- Hwang, J.; Park, C.H.; Kim, S.W. Estimation method for errors of an aerostatic planar XY stage based on measured profiles errors. Int. J. Adv. Manuf. Technol. 2010, 46, 877–883. [Google Scholar] [CrossRef]
- Abele, E.; Altintas, Y.; Brecher, C. Machine tool spindle units. CIRP Ann. 2010, 59, 781–802. [Google Scholar] [CrossRef]
- Tsai, M.H.; Hsu, T.Y.; Pai, K.R.; Shih, M.C. Precision position control of pneumatic servo table embedded with aerostatic bearing. J. Syst. Des. Dyn. 2008, 2, 940–949. [Google Scholar] [CrossRef]
- Pandey, N.P.; Tiwari, A. CFD Simulation of Air Bearing Material. Int. J. Sci. Res. Dev. 2010, 3, 126–129. [Google Scholar]
- Vainio, V.; Miettinen, M.; Majuri, J.; Theska, R.; Viitala, R. Manufacturing and static performance of porous aerostatic bearings. Precis. Eng. 2023, 84, 177–190. [Google Scholar] [CrossRef]
- Cui, W.; Li, S.; Zhu, B.; Yang, F.; Chen, B. Research on the influence of a micro-groove-orifice structure and its layout form on the static characteristics of aerostatic journal bearings under a high gas supply pressure. Adv. Mech. Eng. 2023, 15, 16878132231153263. [Google Scholar] [CrossRef]
- Zhang, J.; Deng, Z.; Zhang, K.; Jin, H.; Yuan, T.; Chen, C.; Su, Z.; Cao, Y.; Xie, Z.; Wu, D.; et al. The Influences of Different Parameters on the Static and Dynamic Performances of the Aerostatic Bearing. Lubricants 2023, 11, 130. [Google Scholar] [CrossRef]
- Ene, N.M.; Dimofte, F.; Keith, T.G. A stability analysis for a hydrodynamic three-wave journal bearing. Tribol. Int. 2008, 41, 434–442. [Google Scholar] [CrossRef]
- Li, Y.; Yu, M.; Ao, L.; Yue, Z. Thermohydrodynamic lubrication analysis on equilibrium position and dynamic coefficient of journal bearing. Trans. Nanjing Univ. Aeronaut. Astronaut. 2012, 29, 227–236. [Google Scholar] [CrossRef]
- Chakraborty, M.B.; Charraborti, D.P. Design and Development of Different Applications of PATB (Porous Aerostatic Thrust Bearing): A Review. Tribol.—Finn. J. Tribol. 2023, 40, 18–28. [Google Scholar] [CrossRef]
- Shuai, Q.; Wu, L.; Chen, Z. Solution of the three lobe’s journal center orbit under steady-state force. Mech. Eng. Autom. 2006, 2, 119–121. [Google Scholar]
- Qian, K.; Li, X. Lubrication simulation of three lobe journal bearing and solution for the equilibrium position problem. Lubr. Eng. 2008, 10, 52–54. [Google Scholar] [CrossRef]
- Wang, W.; Song, P.; Yu, H.; Zhang, G. Research on vibration amplitude of ultra-precision aerostatic motorized spindle under the combined action of rotor unbalance and hydrodynamic effect. Sensors 2023, 23, 496. [Google Scholar] [CrossRef] [PubMed]
- Yu, S.; Chen, S. Calculation of steady-state equilibrium position of tilting pad journal bearing in motorized spindle. China Mech. Eng. 2012, 23, 2492. [Google Scholar] [CrossRef]
- Wan, Z.; Wang, W.; Long, X.; Meng, G. On the calculation of journal static equilibrium position of oil film bearings. Lubr. Eng. 2019, 44, 11–16. [Google Scholar] [CrossRef]
- Zheng, T.; Hasebe, N. Calculation of equilibrium position and dynamic coefficients of a journal bearing using free boundary theory. J. Tribol. 2000, 122, 616–621. [Google Scholar] [CrossRef]
- Pokorný, J. An efficient method for establishing the static equilibrium position of the hydrodynamic tilting-pad journal bearings. Tribol. Int. 2021, 153, 106641. [Google Scholar] [CrossRef]
- Yang, M.; Xu, C. Twofold secant method of solving nonlinear equation. J. Henan Norm. Univ. 2010, 38, 14–16. [Google Scholar] [CrossRef]
- Zhou, W.; Wei, X.; Wang, L.; Wu, G. A super-linear iteration method for calculation of finite length journal bearing’s static equilibrium position. R. Soc. Open Sci. 2017, 4, 161059. [Google Scholar] [CrossRef]
- Deng, Z.; Cheng, W.; Cao, G. Solution of quiescent point in gas foil bearings. Bearing 2021, 9, 20–28. [Google Scholar] [CrossRef]
- Wen, B.; Gu, J.; Xia, S. Advanced Rotor Dynamics: Theory, Technology and Application; China Machine Press: Beijing, China, 1999; pp. 86–87. [Google Scholar]
- Jia, C.; Pang, H.; Ma, W.; Qiu, M. Analysis of dynamic characteristics and stability prediction of gas bearings. Ind. Lubr. Tribol. 2017, 69, 123–130. [Google Scholar] [CrossRef]
- Yang, L.; Li, H.; Yu, L. Dynamic stiffness and damping coefficients of aerodynamic tilting-pad journal bearings. Tribol. Int. 2007, 40, 1399–1410. [Google Scholar] [CrossRef]
- Wang, D.; Zhu, J. A finite element method for computing dynamic coefficient of hydrodynamic journal bearing. J. Aerosp. Power 1995, 69–71, 110. [Google Scholar] [CrossRef]
- Meng, S. Research on the rotary accuracy of the hydrostatic-dynamic spindle affected by journal geometric error and electromagnetic eccentricity. Ph.D. Thesis, Hunan University, Changsha, China, 2016. [Google Scholar]
- Qi, S.; Geng, H.; Lu, L. Dynamic Stiffness and Dynamic Damping Coefficients of Aerodynamic Bearings. J. Mech. Eng. 2007, 5, 91–98. [Google Scholar] [CrossRef]
- Li, Y.; Ao, L.; Li, L.; Yue, Z. Dynamic analysis method of dynamic character coefficient of hydrodynamic journal bearing. J. Mech. Eng. 2010, 46, 48–53. [Google Scholar] [CrossRef]
- Lo, C.; Wang, C.; Lee, Y. Performance analysis of high-speed spindle aerostatic bearings. Tribol. Int. 2005, 38, 5–14. [Google Scholar] [CrossRef]



| Parameter | Value |
|---|---|
| Journal length L1 (mm) | 160 |
| Journal radius R2 (mm) | 25 |
| Mean air film thickness c (μm) | 10 |
| Diameter of orifice d (mm) | 0.2 |
| Row number of Orifice on journal bearing | 2 |
| Orifice number of each row | 8 |
| Rotor quality M (kg) | 6.5 |
| Density of air ρ (kg·m−3) | 1.204 |
| Supply pressure Ps (MPa) | 0.5 |
| Environment pressure P (MPa) | 0.1 |
| Viscosity of air μ (N·s·m−2) | 1.8 × 10−5 |
| Solution Method | Condition of Convergence | Iteration Step | Equilibrium Position | Relative Error | |
|---|---|---|---|---|---|
| Secant method | 1 × 10−2 | 22 | X = 0.17369 | ▲ | \ |
| Y = −0.20219 | ▲ | \ | |||
| Search method | 5 × 10−2 | 15 | X = 0.17342 | \ | ▲ |
| Y = −0.20228 | \ | ▲ | |||
| BIM | 1 × 10−3 | 4 | X = 0.17364 | 0.03% | 0.15% |
| Y = −0.20215 | 0.02% | 0.06% | |||
| Journal Diameter (mm) | Bearing Diameter (mm) | Mean Air Film Thickness (μm) | |
|---|---|---|---|
| 1 | 49.997 | 50.021 | 12 |
| 2 | 49.998 | 50.023 | 12.5 |
| 3 | 49.996 | 50.020 | 12 |
| Average | \ | \ | 12.2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, S.; Huang, Y.; Yu, H.; Wang, W.; Zhang, G.; Kou, X.; Zhang, S.; Li, Y. Calculation and Analysis of Equilibrium Position of Aerostatic Bearings Based on Bivariate Interpolation Method. Lubricants 2024, 12, 85. https://doi.org/10.3390/lubricants12030085
Li S, Huang Y, Yu H, Wang W, Zhang G, Kou X, Zhang S, Li Y. Calculation and Analysis of Equilibrium Position of Aerostatic Bearings Based on Bivariate Interpolation Method. Lubricants. 2024; 12(3):85. https://doi.org/10.3390/lubricants12030085
Chicago/Turabian StyleLi, Shuai, Yafu Huang, Hechun Yu, Wenbo Wang, Guoqing Zhang, Xinjun Kou, Suxiang Zhang, and Youhua Li. 2024. "Calculation and Analysis of Equilibrium Position of Aerostatic Bearings Based on Bivariate Interpolation Method" Lubricants 12, no. 3: 85. https://doi.org/10.3390/lubricants12030085
APA StyleLi, S., Huang, Y., Yu, H., Wang, W., Zhang, G., Kou, X., Zhang, S., & Li, Y. (2024). Calculation and Analysis of Equilibrium Position of Aerostatic Bearings Based on Bivariate Interpolation Method. Lubricants, 12(3), 85. https://doi.org/10.3390/lubricants12030085





