Predictive Model for Scuffing Temperature Field Rise of Spiral Bevel Gears under Different Machining Conditions
Abstract
1. Introduction
2. Modeling of Spiral Bevel Gears with Different Surface Roughnesses
2.1. Simulation of Surface Profile Curves of Spiral Bevel Gears
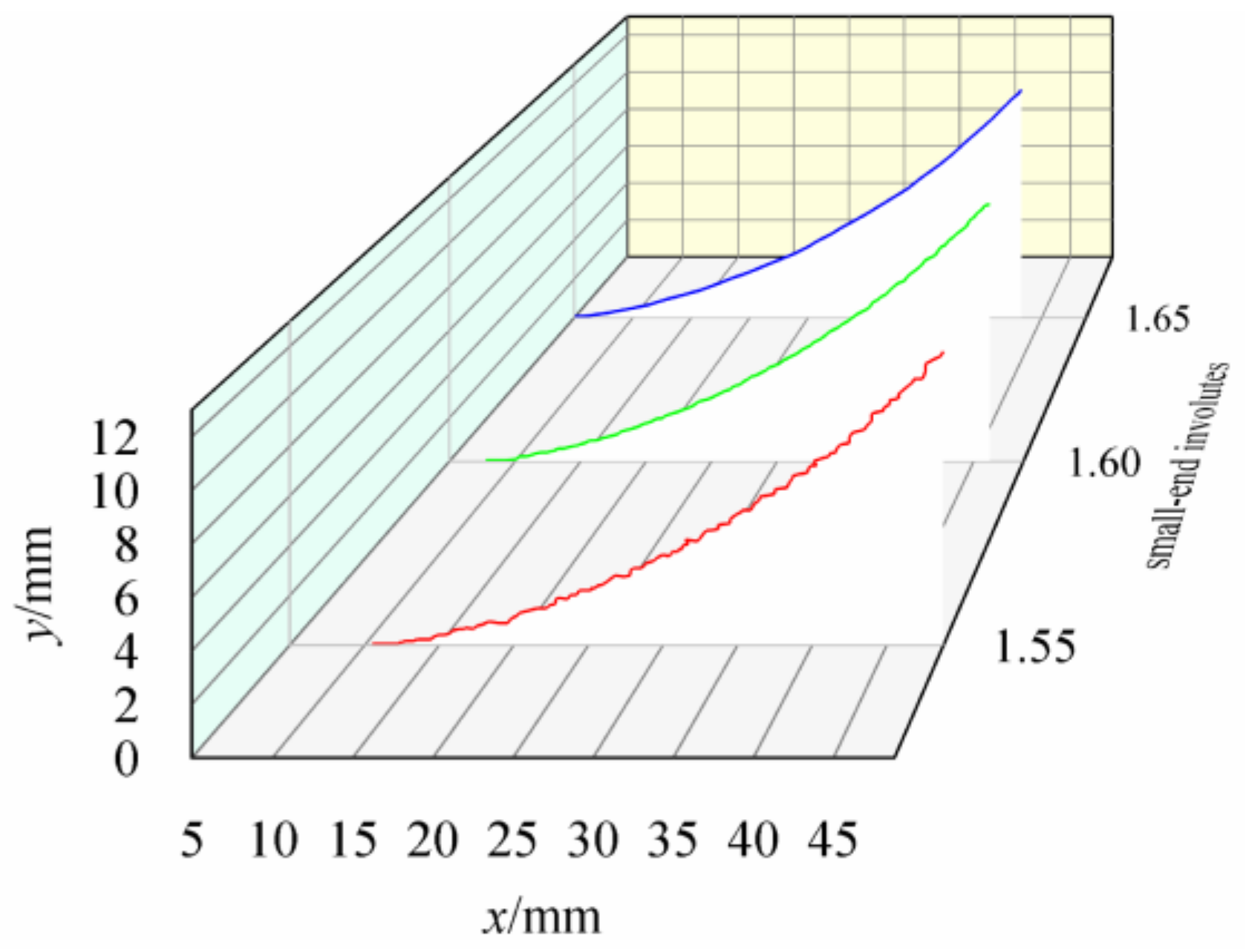
2.2. Representation of Gear Involutes
3. Thermal Analysis of Spiral Bevel Gears
3.1. Calculation of Heat Transfer Coefficient for Gear Contact
3.2. Heat Exchange Conditions under Different Lubrication Methods
3.3. Calculation of Frictional Heat
4. Temperature Field Simulation of Spiral Bevel Gears
4.1. Temperature Field in Steady State
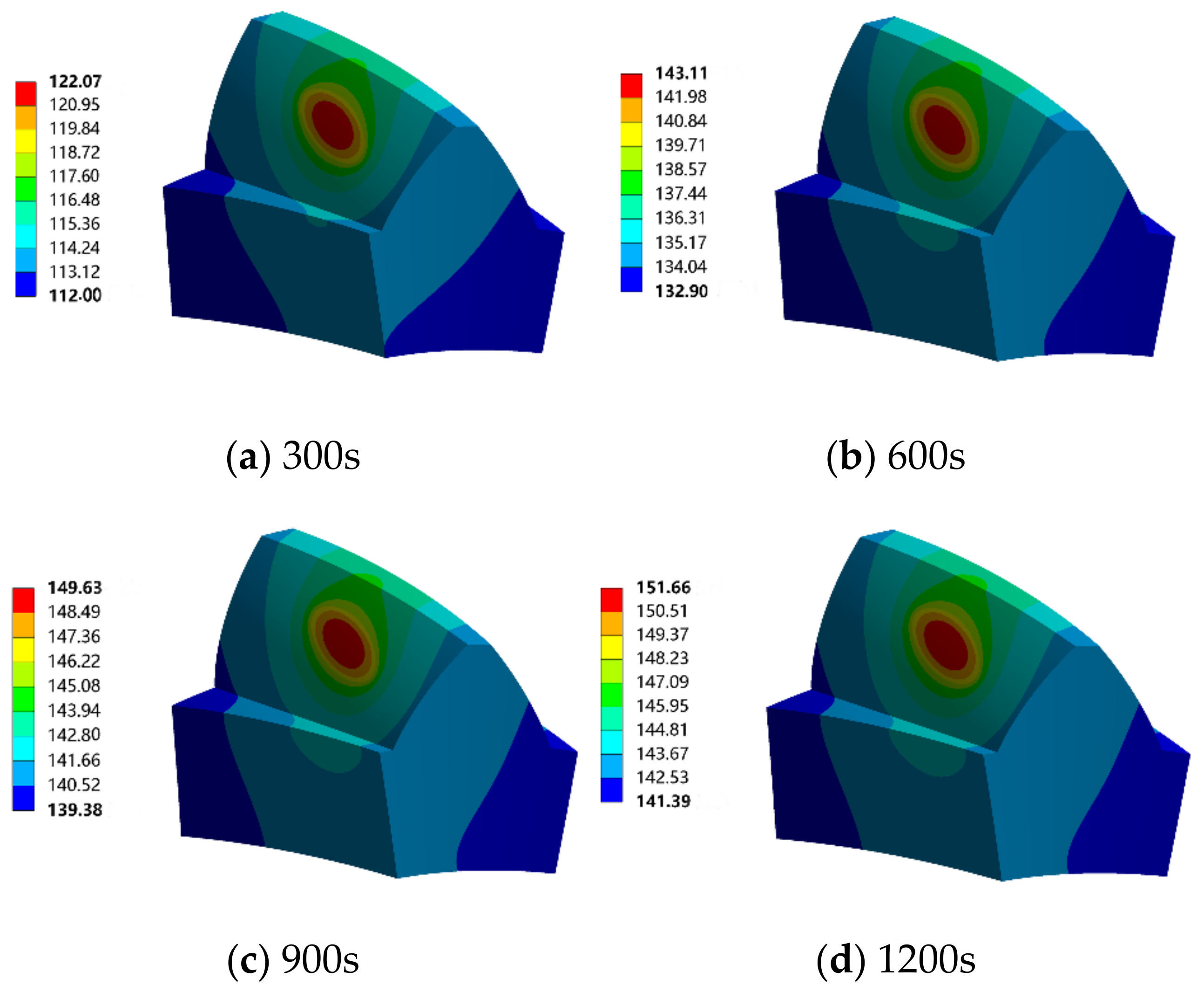
4.2. Temperature Field in Transient State
- (1)
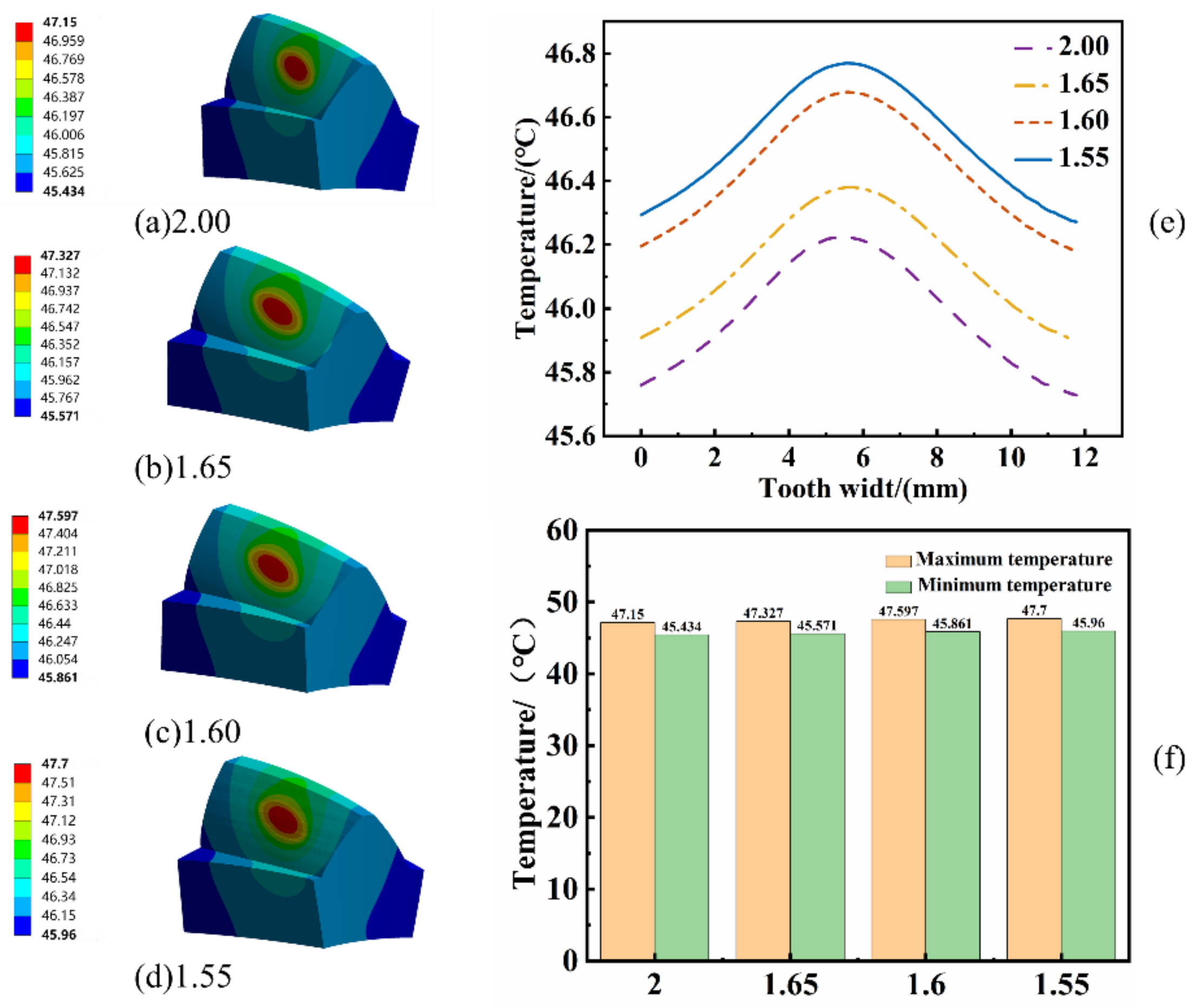
- The transient temperature field distribution of an individual tooth closely resembles that of the steady-state temperature field. Notably, the temperature at the end face of the single tooth is comparatively lower. The temperature within the body section of the tooth is higher than at the end face, but lower than on the meshing surface.
- (2)
- The maximum temperature on the meshing surface of the single tooth experiences a notable increase of 74.92 °C from 0 s to 1200 s.
- (3)
- Examining the temperature rise curve reveals distinct phases: initially, the maximum temperature of the single tooth rises sharply with increasing time, followed by a period where the rise becomes more gradual, and eventually stabilizes.
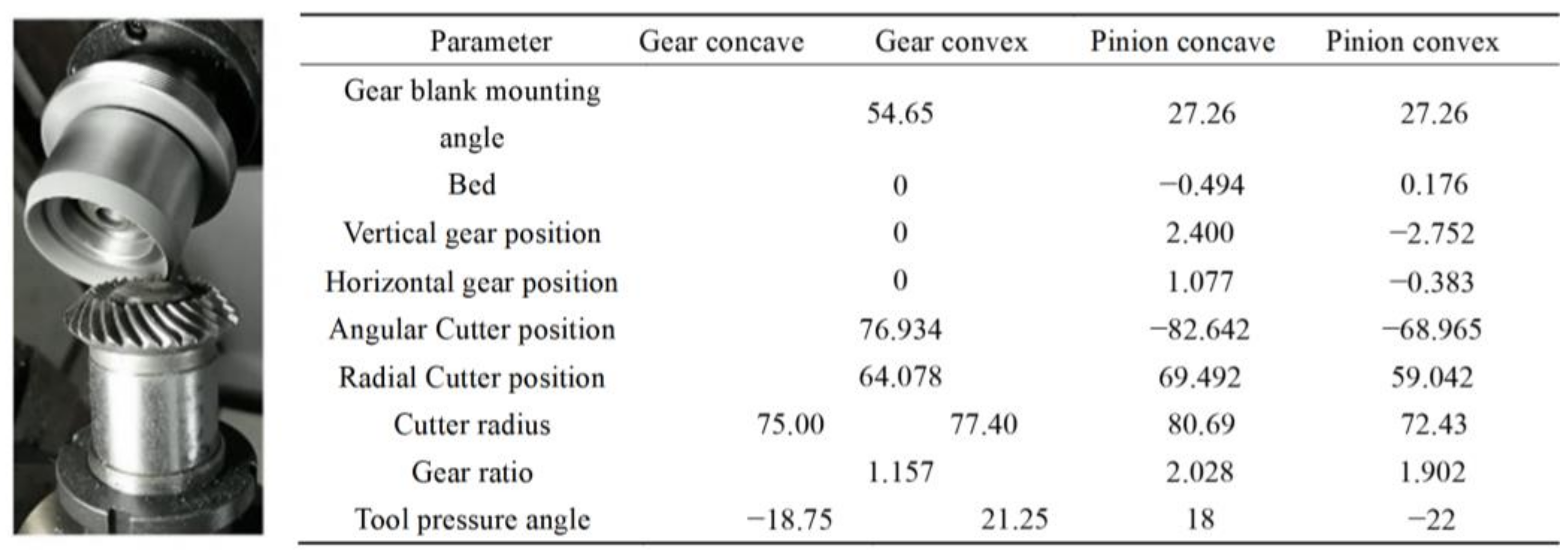
4.3. Experimental Validation
5. Conclusions
- Three-dimensional modeling of gears with roughness: A three-dimensional gear model, considering surface roughness, was established by combining the W-M function with the involute of spiral bevel gears. It was observed that reducing the fractal dimension increased surface fluctuation and roughness. Using these models, gears with varying roughness levels were generated.
- Temperature increase with roughness: The steady-state maximum tooth temperatures increased by 0.38%, 0.95%, and 1.16% at surface roughness values of 0.2 μm, 0.4 μm, and 0.8 μm, respectively. These results can help determine the initial oil temperature in scuffing tests.
- Validation with experiments: A comparison between simulation and experimental results showed a maximum error of 4.74%. Higher transient temperatures were observed with increasing fractal dimensions, confirming the model’s effectiveness in predicting.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhao, Z.; Yang, Y.; Han, H.; Ma, H.; Wang, H.; Li, Z. Meshing characteristics of spur gears considering three-dimensional fractal rough surface under elastohydrodynamic lubrication. Machines 2022, 10, 705. [Google Scholar] [CrossRef]
- Hu, X.; Jiang, Y.; Luo, C.; Feng, L.; Dai, Y. Churning power losses of a gearbox with spiral bevel geared transmission. Tribol. Int. 2019, 129, 398–406. [Google Scholar] [CrossRef]
- Li, W.; Pang, D.; Hao, W. Effects of the helix angle, the friction coefficient and mechanical errors on unsteady-state temperature field of helical gear and thermal sensitivity analysis. Int. J. Heat Mass Transf. 2019, 144, 118669. [Google Scholar] [CrossRef]
- Patir, N. A numerical procedure for random generation of rough surfaces. Wear 1978, 47, 263–277. [Google Scholar] [CrossRef]
- Ge, S.R. Study on fractal characterization and fractal representation of rough surfaces. J. Tribol. 1997, 1, 74–81. [Google Scholar]
- Meng, F.; Xia, H.; Zhang, X.; Wang, J.; Jin, Y. Study on nonlinear dynamic characteristics of gear system with 3D anisotropic rough tooth surface based on fractal theory. Int. J. Non-Linear Mech. 2023, 150, 104366. [Google Scholar] [CrossRef]
- Ding, H.; Li, Y.; Zhao, Q.; Lu, S.; Rong, K.; Sun, J.; Hu, Z. On micro flank geometric topography design for spiral bevel and hypoid gears. Mech. Mach. Theory 2023, 183, 105236. [Google Scholar] [CrossRef]
- Sutyagin, O.V.; Bolotov, A.N.; Rachishkin, A.A. Computer simulation of the contact of rough surfaces. J. Frict. Wear 2016, 37, 198–203. [Google Scholar] [CrossRef]
- Wang, H.; Zhou, C.; Wang, H.; Hu, B.; Liu, Z. A novel contact model for rough surfaces using piecewise linear interpolation and its application in gear wear. Wear 2021, 476, 203685. [Google Scholar] [CrossRef]
- Modaresahmadi, S.; Khalesi, J.; Li, K.; Bird, J.Z.; Williams, W.B. Convective heat transfer analysis of a laminated flux focusing magnetic gearbox. Therm. Sci. Eng. Prog. 2020, 18, 100552. [Google Scholar] [CrossRef]
- Handschuh, R.F.; Kicher, T.P. A Method for thermal analysis of spiral bevel gears. J. Mech. Des. 1996, 118, 580–585. [Google Scholar] [CrossRef]
- Liu, S.; Liu, Z.; Lyu, Y. Investigation Into the Temperature Field and Thermal Deformation of a Spiral Bevel Gear Pair at Different Rotational Speeds. In Turbo Expo: Power for Land, Sea, and Air; American Society of Mechanical Engineers: New York, NY, USA, 2018; Volume 51098, p. V05BT18A003. [Google Scholar]
- Wang, Z.; Zheng, P. Thermal Elastohydrodynamic Lubrication and Efficiency of Grease Lubricated Spiral Bevel Gears. Tribology 2023, 44, 323–334. [Google Scholar]
- Zhang, J.; Liu, S.; Fang, T. Determination of surface temperature rise with the coupled thermo-elasto-hydrodynamic analysis of spiral bevel gears. Appl. Therm. Eng. 2017, 124, 494–503. [Google Scholar] [CrossRef]
- Cao, W.; Pu, W.; Wang, J.; Xiao, K. Effect of contact path on the mixed lubrication performance, friction and contact fatigue in spiral bevel gears. Tribol. Int. 2018, 123, 359–371. [Google Scholar] [CrossRef]
- Lyu, B.; Meng, X.; Zhang, R.; Wen, C. A deterministic contact evolution and scuffing failure considering lubrication deterioration due to temperature rise under heavy loads. Eng. Fail. Anal. 2021, 123, 105276. [Google Scholar] [CrossRef]
- Zhang, J.; Li, W.; Wang, H.; Song, Q.; Lu, L.; Wang, W.; Liu, Z. A comparison of the effects of traditional shot peening and micro-shot peening on the scuffing resistance of carburized and quenched gear steel. Wear 2016, 368, 253–257. [Google Scholar] [CrossRef]
- Li, S.; Kahraman, A. A scuffing model for spur gear contacts. Mech. Mach. Theory 2021, 156, 104161. [Google Scholar] [CrossRef]
- Handschuh, M.J.; Kahraman, A.; Anderson, N.E. Development of a high-speed two-disc tribometer for evaluation of traction and scuffing of lubricated contacts. Tribol. Trans. 2020, 63, 509–518. [Google Scholar] [CrossRef]
- Chen, T.; Wei, P.; Zhu, C.; Zeng, P.; Li, D.; Parker, R.; Liu, H. Experimental Investigation of Gear Scuffing for Various Tooth Surface Treatments. Tribol. Trans. 2023, 66, 35–46. [Google Scholar] [CrossRef]
- Castro, J.; Seabra, J. Global and local analysis of gear scuffing tests using a mixed film lubrication model. Tribol. Int. 2008, 41, 244–255. [Google Scholar] [CrossRef]
- Simon, V. Influence of misalignments on EHD lubrication in hypoid gears. In Proceedings of the International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Las Vegas, NV, USA, 4–7 September 2007; Volume 48086, pp. 671–680. [Google Scholar]
- Simon, V. Minimization of the influence of misalignments on EHD lubrication in face-hobbed spiral bevel gears. In Proceedings of the International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Portland, OR, USA, 4–7 August 2013; American Society of Mechanical Engineers: New York, NY, USA, 2013; Volume 55928, p. V005T11A037. [Google Scholar]
- Liu, J.; Ma, C.; Wang, S.; Wang, S.; Yang, B. Thermal contact resistance between bearing inner ring and shaft journal. Int. J. Therm. Sci. 2019, 138, 521–535. [Google Scholar] [CrossRef]
- Zhu, H.; Ge, S.; Huang, X.; Zhang, D.; Liu, J. Experimental study on the characterization of worn surface topography with characteristic roughness parameter. Wear 2003, 255, 309–314. [Google Scholar] [CrossRef]
- Cai, Z.J.; Zheng, X.Q.; Lan, H.Q.; Wang, L.N.; Yang, S.W.; Sheng, R. Time-Varying Meshing Stiffness and Dynamic Parameter Model of Spiral Bevel Gears with Different Surface Roughness. Appl. Sci. 2024, 14, 1533. [Google Scholar] [CrossRef]
- Blok, H. The postulate about the constancy of scoring temperature. NASA Spec. Publ. 1970, 237, 153. [Google Scholar]
- Wang, T. Study on the Steady-State Temperature Field of Spiral Bevel Gears; Northwestern Polytechnical University: Xi’an, China, 1999. [Google Scholar]
- Luo, B.; Li, W. Influence factors on bulk temperature field of gear. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2017, 231, 953–964. [Google Scholar] [CrossRef]
- Yan, Y.; Sun, L. Transient thermal analysis of spiral bevel gears under loss of lubrication. Appl. Mech. Mater. 2010, 1021, 566–570. [Google Scholar] [CrossRef]
- Xue, J.H. The Research on Thermal Behavior and the Modification Design of High-Speed and Heavy Power Gear System; University of Science and Technology Beijing: Beijing, China, 2015. [Google Scholar]
- Hu, X.; Yuan, Y.; Chen, J. Study on Temperature Field Distribution of a High-Speed Double-Helical Gear Pair with Oil Injection Lubrication. Lubricants 2024, 12, 315. [Google Scholar] [CrossRef]
- Shao, S.; Zhang, K.; Yao, Y.; Liu, Y.; Yang, J.; Xin, Z.; He, K. A Study on the Lubrication Characteristics and Parameter Influence of a High-Speed Train Herringbone Gearbox. Lubricants 2024, 12, 270. [Google Scholar] [CrossRef]
- Hartnett, J.P.; Deland, E.C. The influence of Prandtl number on the heat transfer from rotating non-isothermal disks and cones. Trans. ASME J. Heat Transf. 1961, 83, 95–96. [Google Scholar] [CrossRef]
- Dorfman, L.A. Hydrodynamic Resistance and the Heat Loss of Rotating Solids; Oliver and Boyd: Edinburgh, UK, 1963; pp. 150–230. [Google Scholar]
- Gong, M.Y.; Chen, S.C.; Wang, Y.J. Strength Calculation and Structure Design of Involute Cylindrical Gear; China Machine Press: Beijing, China, 1986. [Google Scholar]
- ISO 6336-21:2017; Calculation of Load Capacity of Spur and Helical Gears. Calculation of Scuffing Load Capacity (also Applicable to Bevel and Hypoid Gears). ISO: Geneva, Switzerland, 2017.
- Chen, T.; Zhu, C.; Liu, H.; Wei, P.; Zhu, J.; Xu, Y. Simulation and experiment of carburized gear scuffing under oil jet lubrication. Eng. Fail. Anal. 2022, 139, 106406. [Google Scholar] [CrossRef]
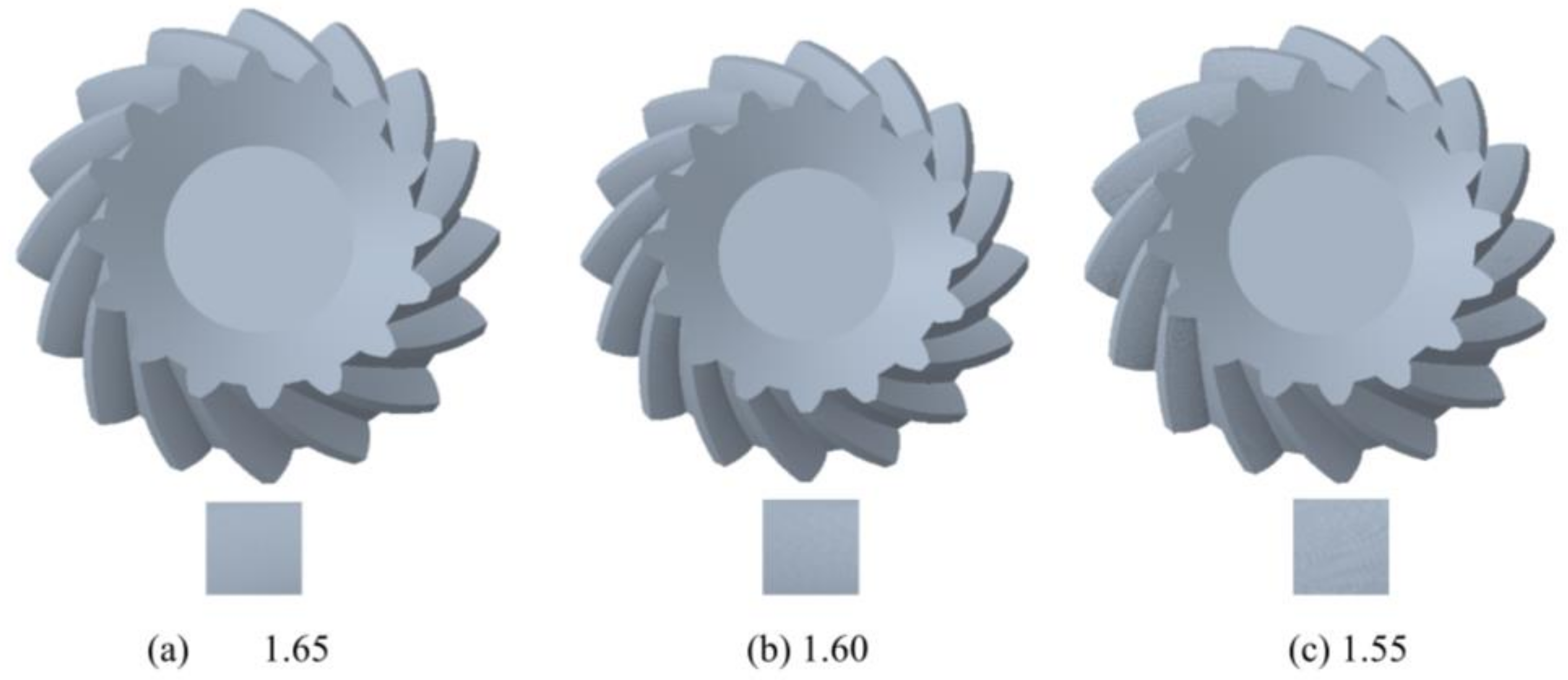
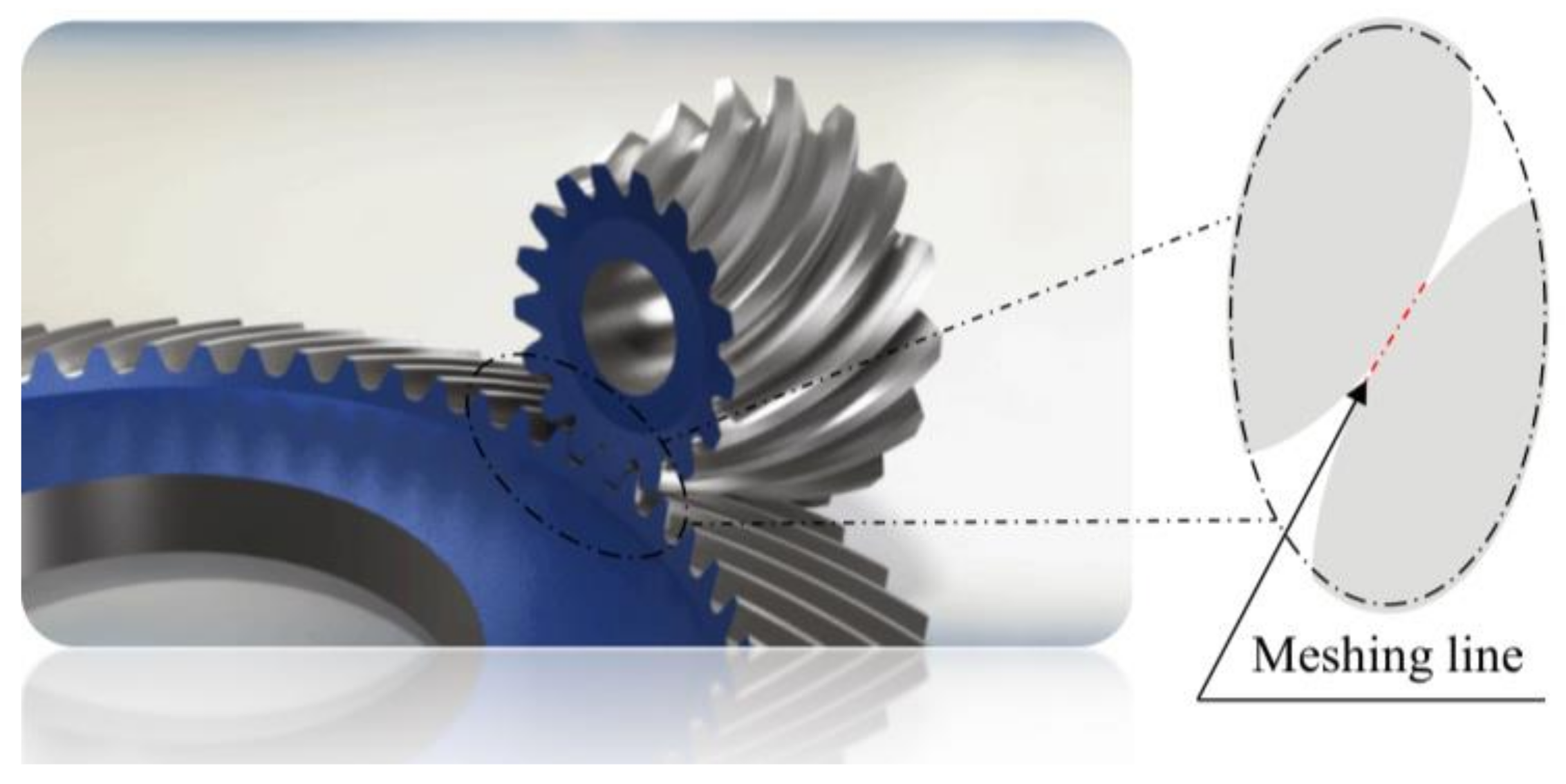
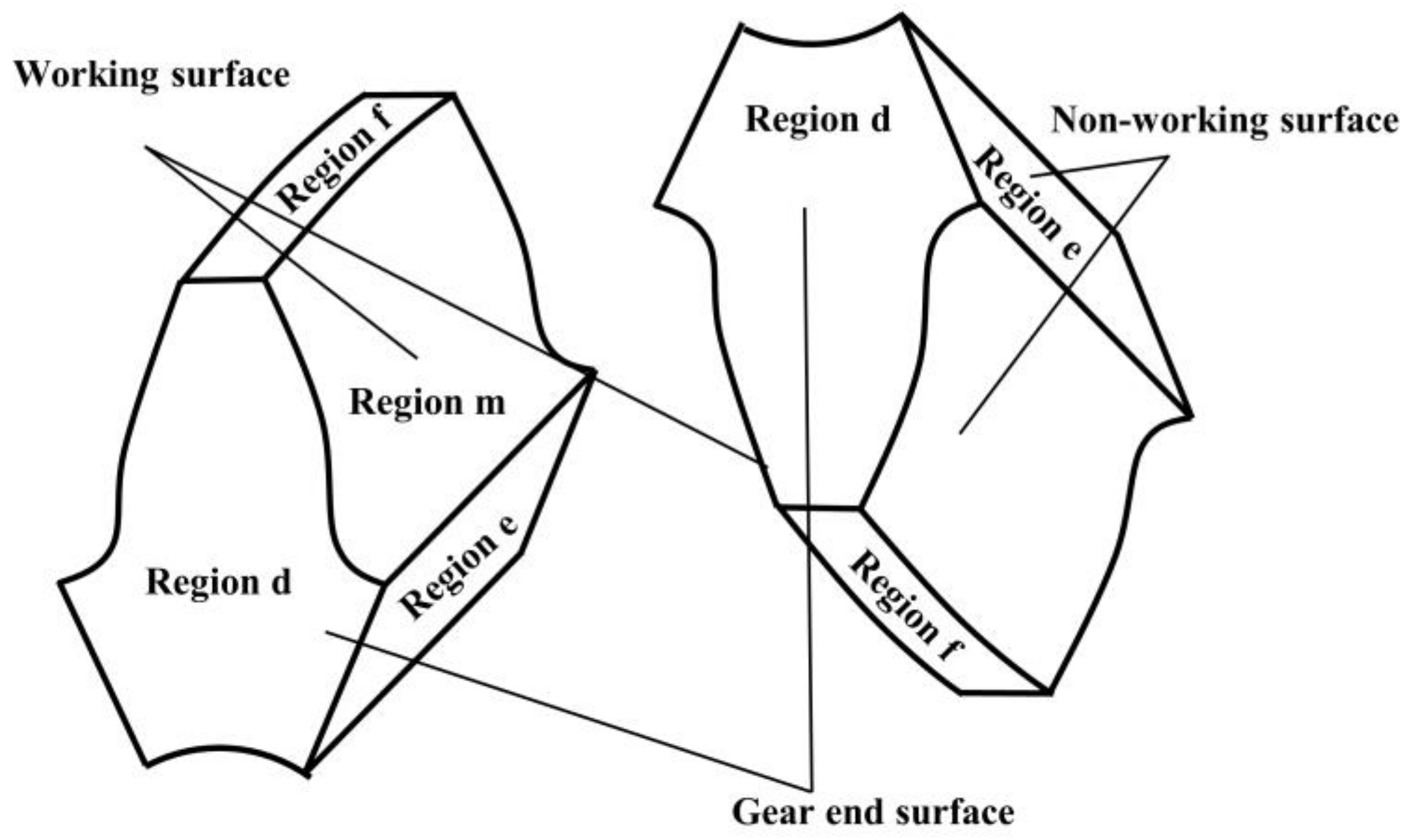

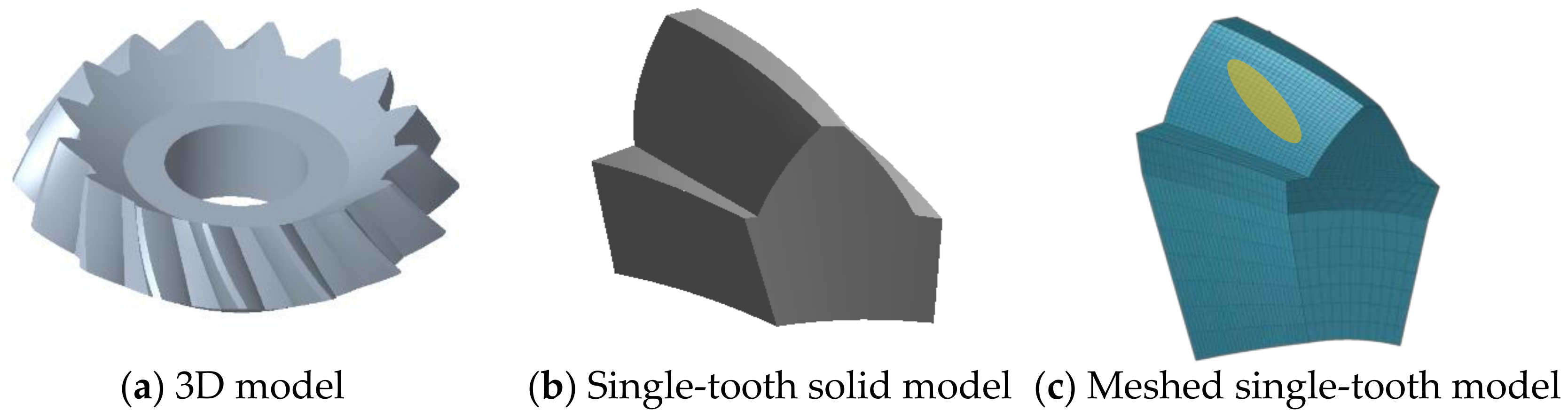
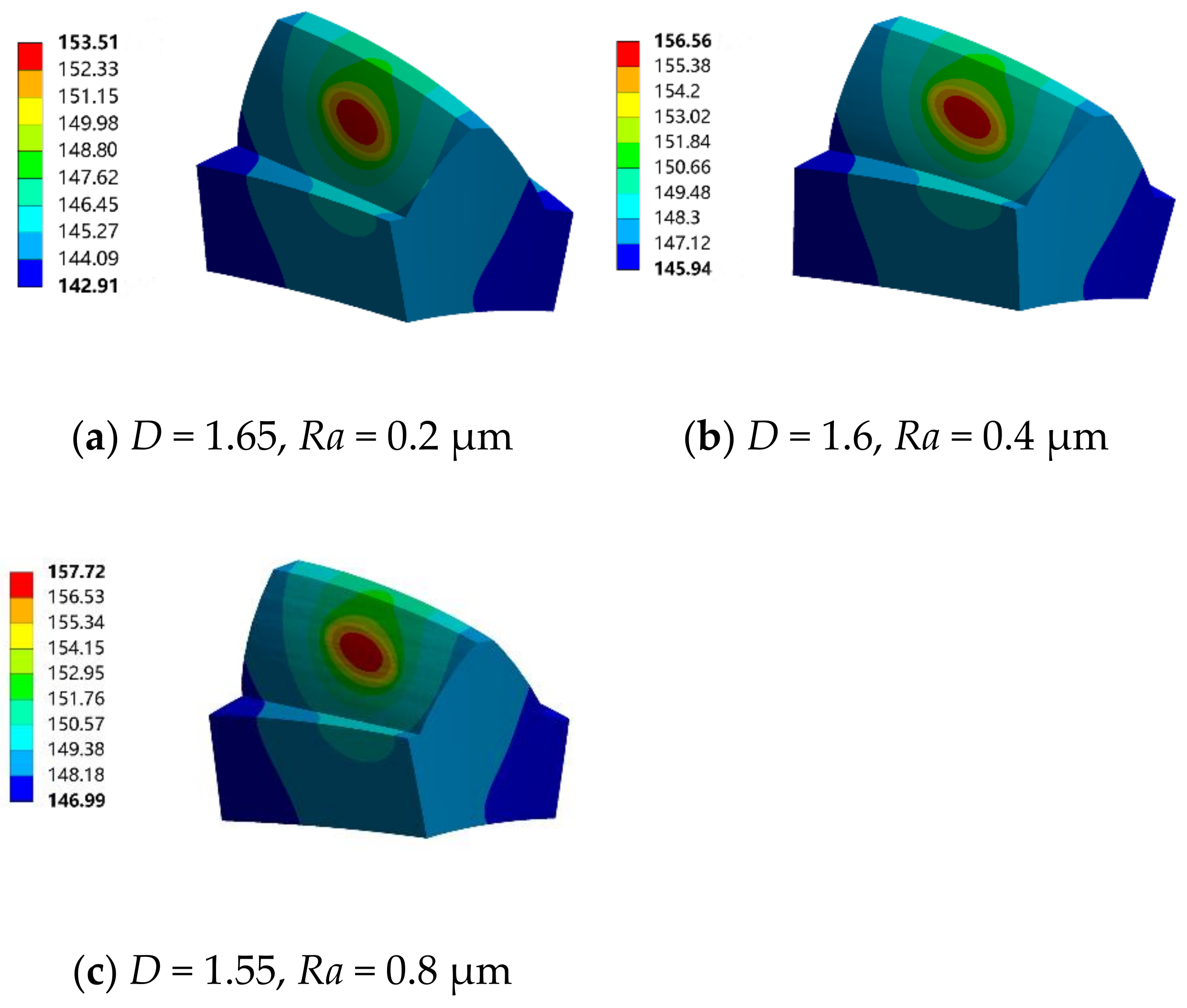


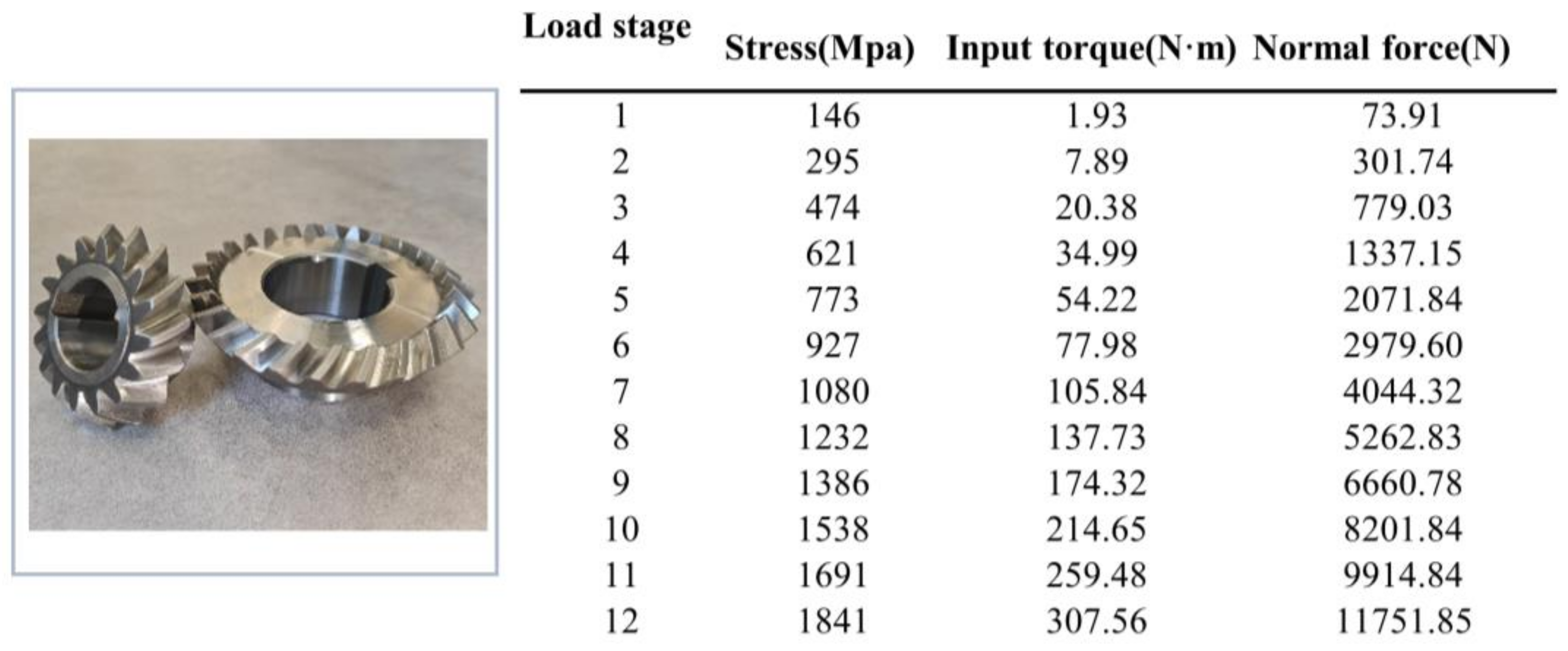

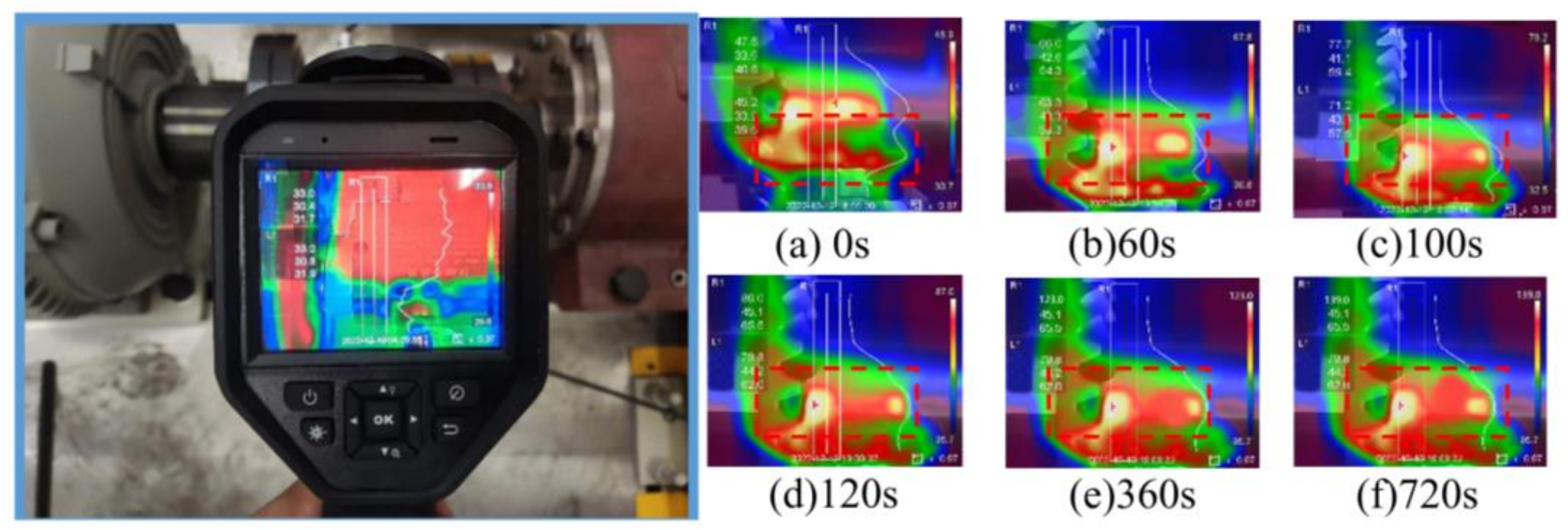
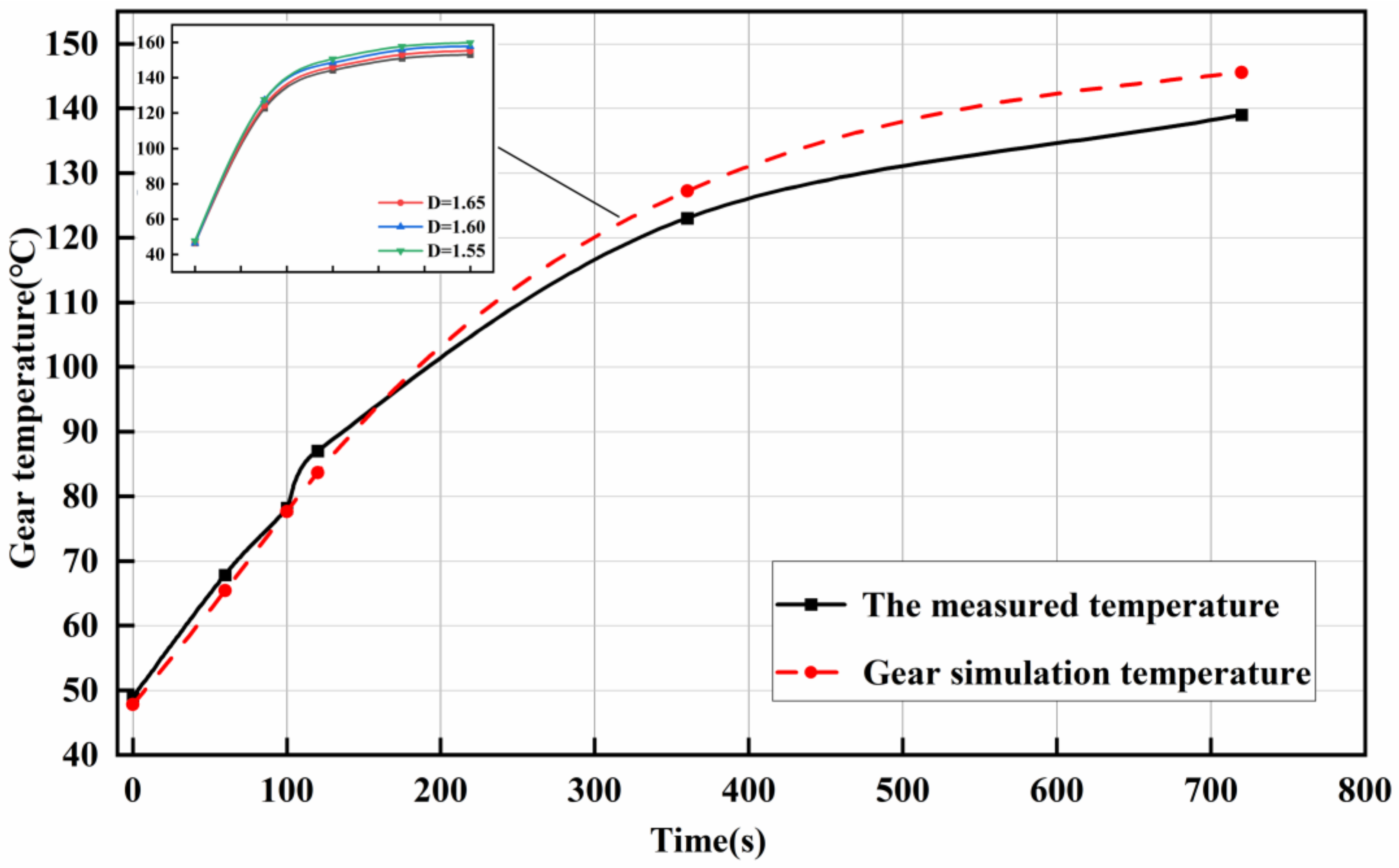
| Roughness/μm | Fractal Dimension | Characteristic Scale Factor |
|---|---|---|
| 0.8 | 1.55 | 5 × 10−6 |
| 0.4 | 1.60 | 3 × 10−6 |
| 0.2 | 1.65 | 2 × 10−6 |
| Parameter | Unit | Pinion | Gear |
|---|---|---|---|
| Number of teeth Z | — | 16 | 27 |
| Module m | mm | 4.25 | |
| Tooth width b | mm | 12 | |
| Normal pressure angle αn | ° | 20 | |
| Midpoint helix angle | ° | 30 | |
| Axis intersection angle β | ° | 90 | |
| Hand | — | left | right |
| Postprocessing Operation | Roughness/μm |
|---|---|
| unground machining | 0.8 |
| grinding machining | 0.4 |
| polishing | 0.2 |
| Density ρh kg/m3 | Thermal Conductivity W/(m·°C) | Specific heat Capacity c J/(kg·°C) | Coefficient of Linear Expansion α 10(−6)/°C | Modulus of Elasticity E GPa | Poisson’s Ratio μ |
|---|---|---|---|---|---|
| 7880 | 29 | 465 | 11.6 | 204 | 0.377 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cai, Z.-J.; Zheng, X.-Q.; Lan, H.-Q.; Wang, L.-N.; Yang, S.-W.; Shen, R. Predictive Model for Scuffing Temperature Field Rise of Spiral Bevel Gears under Different Machining Conditions. Lubricants 2024, 12, 354. https://doi.org/10.3390/lubricants12100354
Cai Z-J, Zheng X-Q, Lan H-Q, Wang L-N, Yang S-W, Shen R. Predictive Model for Scuffing Temperature Field Rise of Spiral Bevel Gears under Different Machining Conditions. Lubricants. 2024; 12(10):354. https://doi.org/10.3390/lubricants12100354
Chicago/Turabian StyleCai, Zhi-Jie, Xi-Qing Zheng, Hui-Qing Lan, Liu-Na Wang, Si-Wei Yang, and Rui Shen. 2024. "Predictive Model for Scuffing Temperature Field Rise of Spiral Bevel Gears under Different Machining Conditions" Lubricants 12, no. 10: 354. https://doi.org/10.3390/lubricants12100354
APA StyleCai, Z.-J., Zheng, X.-Q., Lan, H.-Q., Wang, L.-N., Yang, S.-W., & Shen, R. (2024). Predictive Model for Scuffing Temperature Field Rise of Spiral Bevel Gears under Different Machining Conditions. Lubricants, 12(10), 354. https://doi.org/10.3390/lubricants12100354





