Investigation of Programmable Friction with Ionic Liquid Mixtures at the Nano- and Macroscales
Abstract
:1. Introduction
| Reference | Geometry | Material | Lubricant | el. Potential | Change in COF * | |
|---|---|---|---|---|---|---|
| V | - | % | ||||
| Gat 2019 [35] | ball-on-3-pins | 100Cr6 | [P66614][DEHP]:[P66614][BTA] 1:3 | 3.0 | 0.028 | 26.7 |
| “ | −3.0 | −0.005 | −4.8 | |||
| Gat 2020 [16] | ball-on-3-pins | 100Cr6 | [P66614][Doc]:[P66614][BTA] 1:4 | 3.0 | 0.045 | 45.0 |
| “ | −3.0 | −0.004 | −4.3 | |||
| Kaw 2019 [45] | disk-on-disk | AISI 52100 + ta-C | [BMIM][FAB] | 4.0 | 0.027 | 40.9 |
| [BMIM][PF6] | −2.0 | −0.004 | −6.3 | |||
| Guo 2019 [46] | ball-on-disk | GCr15 | C10 NFs | 4.5 | −0.017 | −13.5 |
| Mic 2020 [47] | ball-on-disk | C45/C10 | [BMIM]PF6 | 1.0 | 0.023 | 69.2 |
| Li 2014 [26] | AFM cantilever tip on plate | Si/HOPG | [HMIm]FAP | −1.0 | 0.001 | 5.3 |
| “ | 1.5 | −0.018 | −94.7 | |||
| Yang 2014 [48] | ball-on-disk | ZrO2/steel 4340 | PC oil | 0.6 | 0.020 | 8.0 |
| [DMIm]PF6/PC (0.5 mM) | −0.6 | −0.120 | −46.2 | |||
| Zhang 2022 [24] | ball-on-disk | GCr15 | 1 wt.% [OMIm]PF6 + PAO50 | 3.0 | 0.100 | 125.0 |
| Liu 2021 [27] | ball-on-disk | ZrO2/Al2O3 | 1 mM SDS and 10 mM NaCl | −2.0 | 0.220 | 169.2 |
2. Materials and Methods
2.1. Materials and Used Ionic Liquids
2.2. Friction Force Microscopy Experiments
2.3. AFM-Based Imaging
2.4. Viscosity and Pressure–Viscosity Coefficient
2.5. Macroscopic Friction Tests
2.6. Nuclear Magnetic Resonance (NMR) Spectroscopy
2.7. Wear Analysis
3. Results
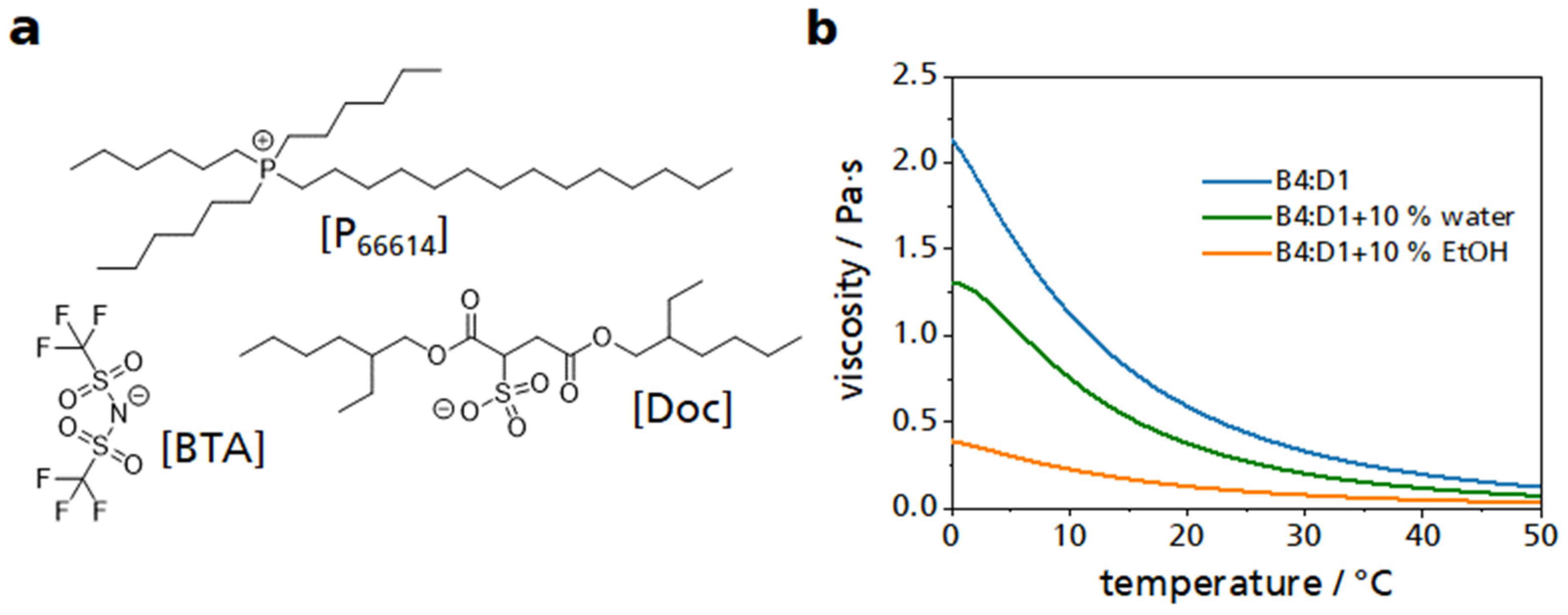
3.1. Viscosity and Pressure–Viscosity Coefficient
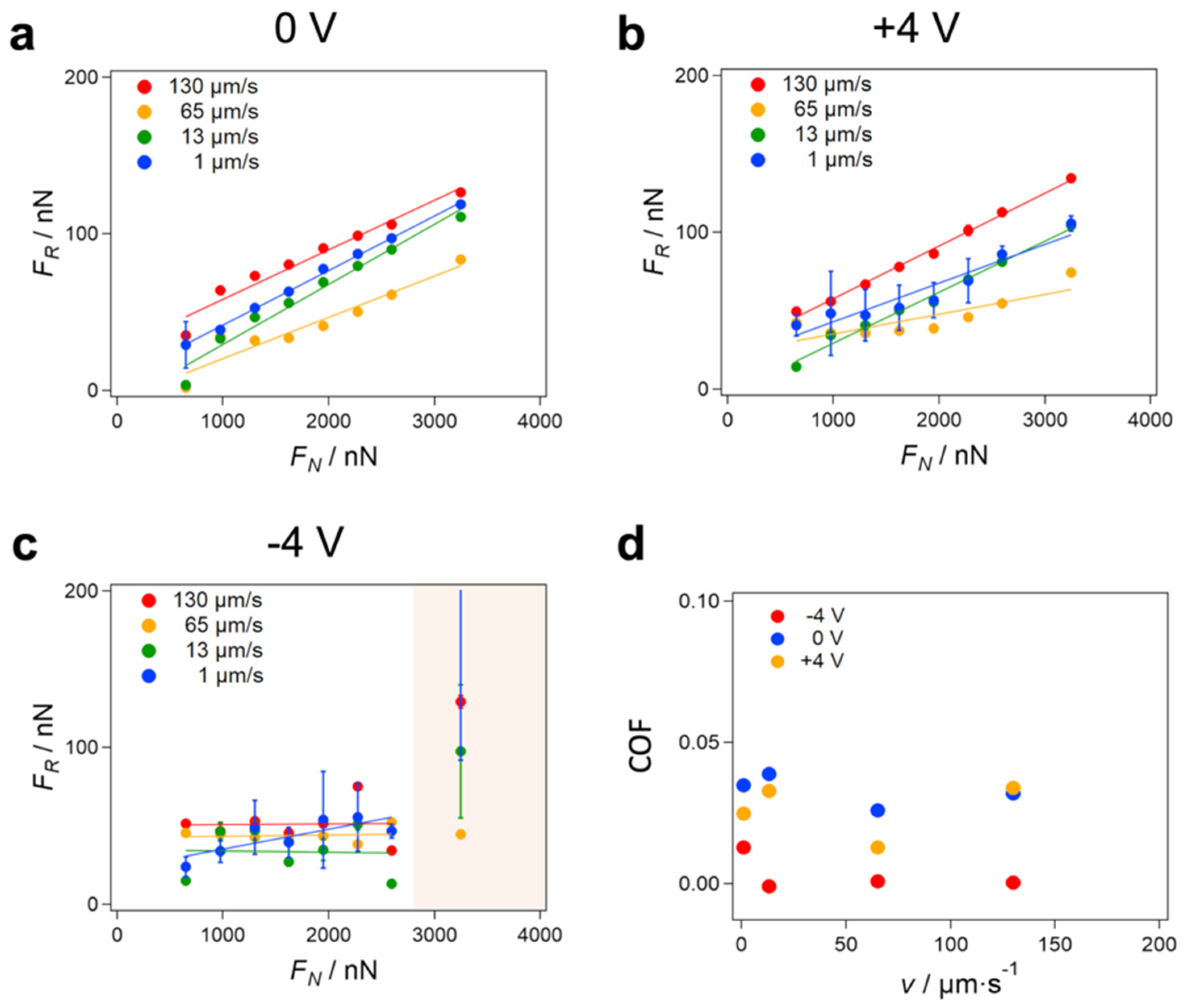
3.2. Nanotribology
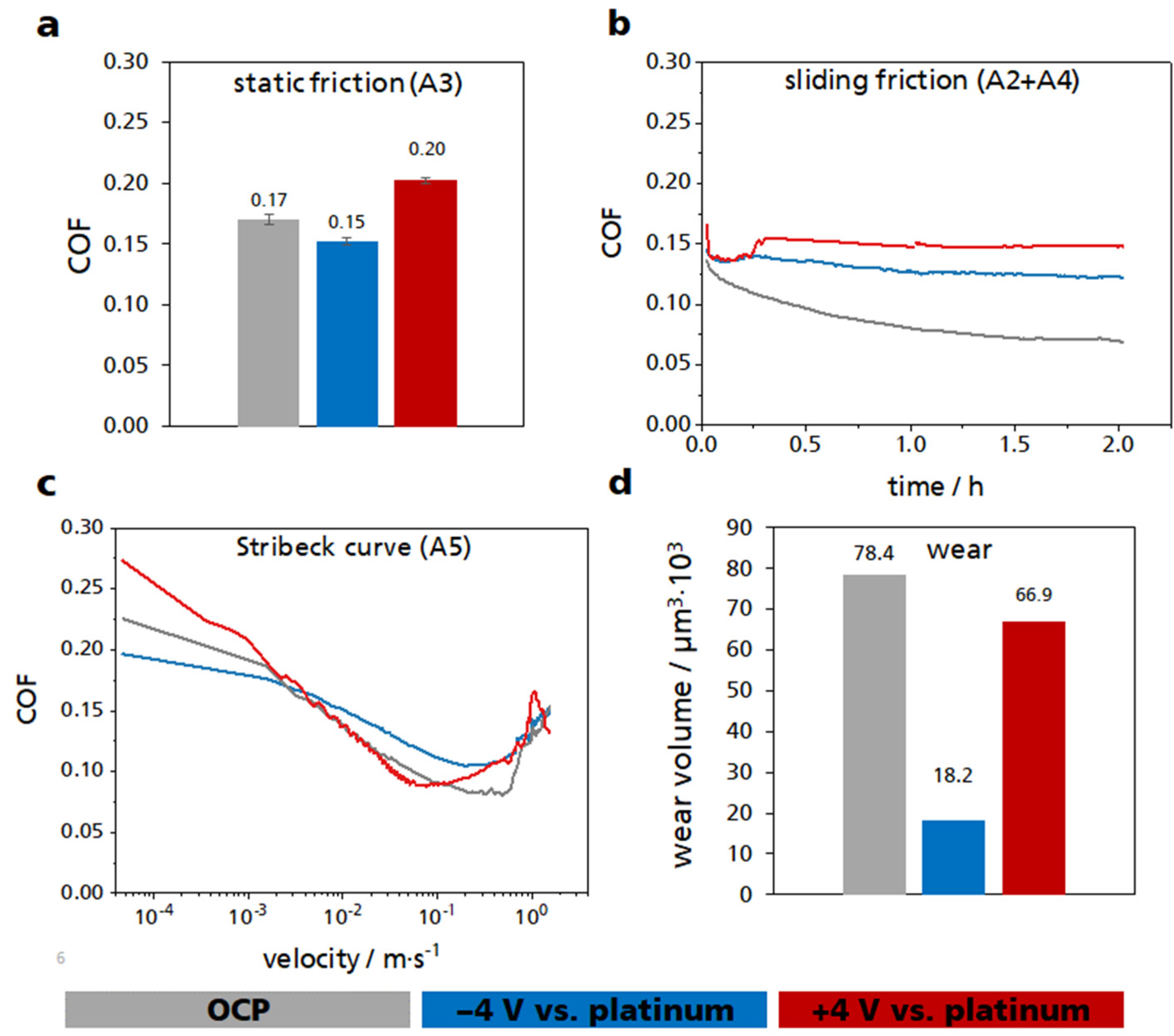
3.3. Macroscopic Tribology
3.4. Wear Analysis
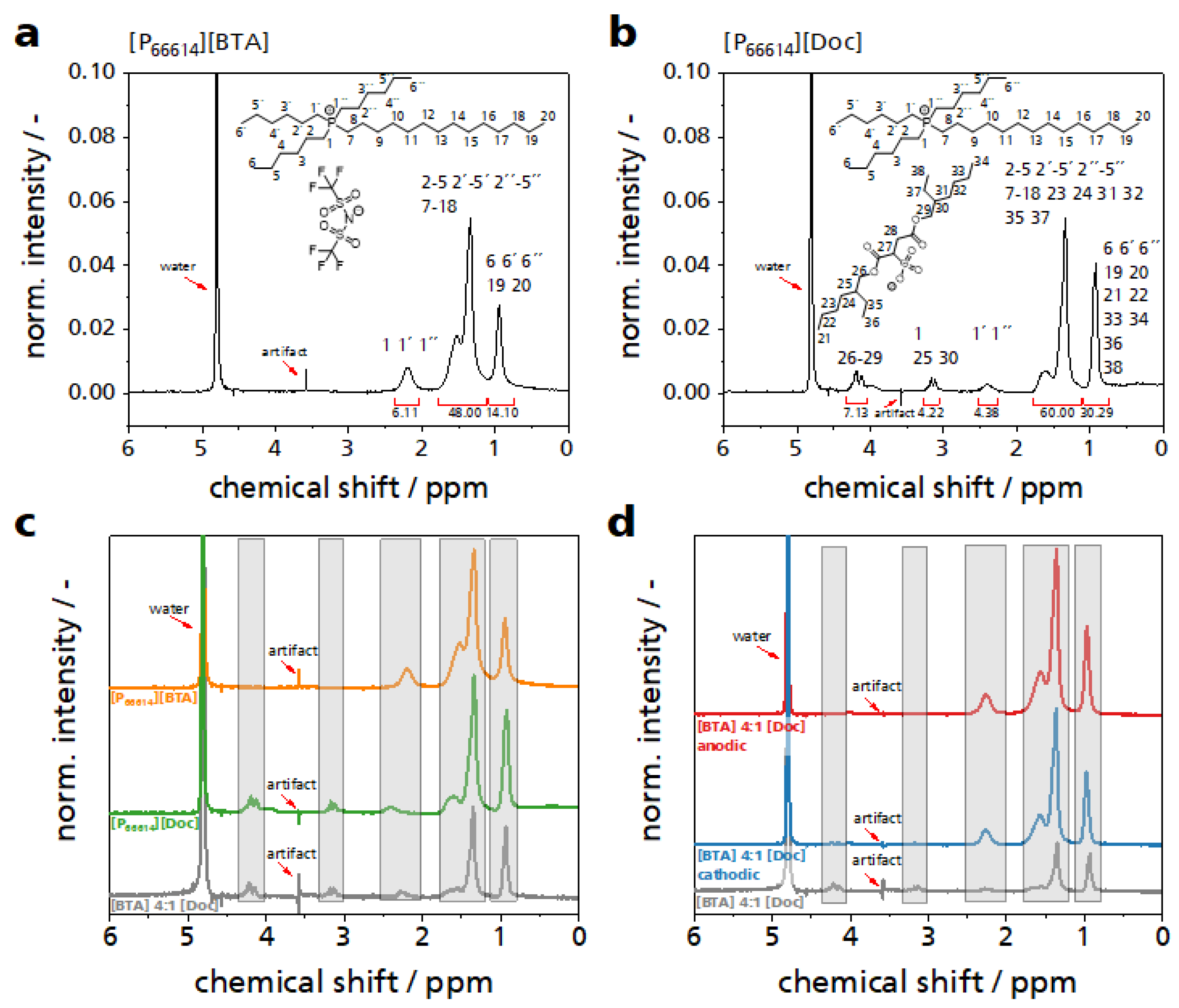
3.5. NMR Spectroscopy
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Holmberg, K.; Erdemir, A. The impact of tribology on energy use and CO2 emission globally and in combustion engine and electric cars. Tribol. Int. 2019, 135, 389–396. [Google Scholar] [CrossRef]
- Meng, Y.; Xu, J.; Ma, L.; Jin, Z.; Prakash, B.; Ma, T.; Wang, W. A review of advances in tribology in 2020–2021. Friction 2022, 10, 1443–1595. [Google Scholar] [CrossRef]
- Luo, J.; Liu, M.; Ma, L. Origin of friction and the new frictionless technology—Superlubricity: Advancements and future outlook. Nano Energy 2021, 86, 106092. [Google Scholar] [CrossRef]
- Cai, W.; Trefs, J.L.; Hugel, T.; Balzer, B.N. Anisotropy of π–π Stacking as Basis for Superlubricity. ACS Mater. Lett. 2023, 5, 172–179. [Google Scholar] [CrossRef]
- Adibnia, V.; Mirbagheri, M.; Faivre, J.; Robert, J.; Lee, J.; Matyjaszewski, K.; Lee, D.W.; Banquy, X. Bioinspired polymers for lubrication and wear resistance. Prog. Polym. Sci. 2020, 110, 101298. [Google Scholar] [CrossRef]
- Cecilia, J.A.; Ballesteros Plata, D.; Alves Saboya, R.M.; Tavares de Luna, F.M.; Cavalcante, C.L.; Rodríguez-Castellón, E. An Overview of the Biolubricant Production Process: Challenges and Future Perspectives. Processes 2020, 8, 257. [Google Scholar] [CrossRef]
- Shah, R.; Woydt, M.; Zhang, S. The Economic and Environmental Significance of Sustainable Lubricants. Lubricants 2021, 9, 21. [Google Scholar] [CrossRef]
- Amiril, S.; Rahim, E.A.; Syahrullail, S. A review on ionic liquids as sustainable lubricants in manufacturing and engineering: Recent research, performance, and applications. J. Clean. Prod. 2017, 168, 1571–1589. [Google Scholar] [CrossRef]
- Naveed, T.; Zahid, R.; Mufti, R.A.; Waqas, M.; Hanif, M.T. A review on tribological performance of ionic liquids as additives to bio lubricants. Proc. Inst. Mech. Eng. Part. J. J. Eng. Tribol. 2021, 235, 1782–1806. [Google Scholar] [CrossRef]
- Ma, L.; Zhang, C.; Liu, S. Progress in experimental study of aqueous lubrication. Chin. Sci. Bull. 2012, 57, 2062–2069. [Google Scholar] [CrossRef]
- Bresme, F.; Kornyshev, A.A.; Perkin, S.; Urbakh, M. Electrotunable friction with ionic liquid lubricants. Nat. Mater. 2022, 21, 848–858. [Google Scholar] [CrossRef]
- Sharma, B.K.; Biresaw, G. Environmentally Friendly and Biobased Lubricants; CRC Press: Boca Raton, FL, USA, 2016; ISBN 9781482232035. [Google Scholar]
- Tysoe, W.T.; Spencer, N.D. Rapid testing of tribotronic materials. Tribol. Lubr. Technol. 2021, 77, 76–77. [Google Scholar]
- He, S.; Meng, Y.; Tian, Y.; Zuo, Y. Response Characteristics of the Potential-Controlled Friction of ZrO2/Stainless Steel Tribopairs in Sodium Dodecyl Sulfate Aqueous Solutions. Tribol. Lett. 2010, 38, 169–178. [Google Scholar] [CrossRef]
- Glavatskih, S.; Höglund, E. Tribotronics—Towards active tribology. Tribol. Int. 2008, 41, 934–939. [Google Scholar] [CrossRef]
- Gatti, F.; Amann, T.; Kailer, A.; Baltes, N.; Rühe, J.; Gumbsch, P. Towards programmable friction: Control of lubrication with ionic liquid mixtures by automated electrical regulation. Sci. Rep. 2020, 10, 17634. [Google Scholar] [CrossRef] [PubMed]
- Krim, J. Controlling Friction With External Electric or Magnetic Fields: 25 Examples. Front. Mech. Eng. 2019, 5, 22. [Google Scholar] [CrossRef]
- Fajardo, O.Y.; Bresme, F.; Kornyshev, A.A.; Urbakh, M. Electrotunable Friction with Ionic Liquid Lubricants: How Important Is the Molecular Structure of the Ions? J. Phys. Chem. Lett. 2015, 6, 3998–4004. [Google Scholar] [CrossRef] [PubMed]
- Liu, C.; Li, X.; Li, X.; Li, W.; Tian, Y.; Meng, Y. On-Line Feedback Control of Sliding Friction of Metals Lubricated by Adsorbed Boundary SDS Films. Lubricants 2022, 10, 148. [Google Scholar] [CrossRef]
- David, A.; Fajardo, O.Y.; Kornyshev, A.A.; Urbakh, M.; Bresme, F. Electrotunable lubricity with ionic liquids: The influence of nanoscale roughness. Faraday Discuss. 2017, 199, 279–297. [Google Scholar] [CrossRef]
- Comtet, J.; Niguès, A.; Kaiser, V.; Coasne, B.; Bocquet, L.; Siria, A. Nanoscale capillary freezing of ionic liquids confined between metallic interfaces and the role of electronic screening. Nat. Mater. 2017, 16, 634–639. [Google Scholar] [CrossRef]
- Fedorov, M.V.; Kornyshev, A.A. Ionic liquids at electrified interfaces. Chem. Rev. 2014, 114, 2978–3036. [Google Scholar] [CrossRef] [PubMed]
- Raviv, U.; Klein, J. Fluidity of bound hydration layers. Science 2002, 297, 1540–1543. [Google Scholar] [CrossRef] [PubMed]
- Zhang, C.; Chen, J.; Liu, M.; Wang, F.; Zheng, Y.; Cheng, Y.; Liu, Z. Relationship between Viscosity and Resistance of Oil Film: A New Way to Investigate the Controllable Friction between Charged Interfaces Lubricated by Ionic Lubricating Oil. Adv. Mater. Interfaces 2022, 9, 2200229. [Google Scholar] [CrossRef]
- Reddy, A.B.; Pilkington, G.A.; Rutland, M.W.; Glavatskih, S. Tribotronic control of an ionic boundary layer in operando extends the limits of lubrication. Sci. Rep. 2022, 12, 20479. [Google Scholar] [CrossRef]
- Li, H.; Wood, R.J.; Rutland, M.W.; Atkin, R. An ionic liquid lubricant enables superlubricity to be “switched on” in situ using an electrical potential. Chem. Commun. 2014, 50, 4368–4370. [Google Scholar] [CrossRef] [PubMed]
- Liu, C.; Fang, J.; Wen, X.; Tian, Y.; Meng, Y. Active Control of Boundary Lubrication of Ceramic Tribo-Pairs in Sodium Dodecyl Sulfate Aqueous Solutions. Tribol. Lett. 2021, 69, 144. [Google Scholar] [CrossRef]
- Amann, T.; Waidele, M.; Kailer, A. Analysis of mechanical and chemical mechanisms on cavitation erosion-corrosion of steels in salt water using electrochemical methods. Tribol. Int. 2018, 124, 238–246. [Google Scholar] [CrossRef]
- Gatti, S.F.; Gatti, F.; Amann, T.; Kailer, A.; Moser, K.; Weiss, P.; Seidel, C.; Rühe, J. Tribological performance of electrically conductive and self-lubricating polypropylene-ionic-liquid composites. RSC Adv. 2023, 13, 8000–8014. [Google Scholar] [CrossRef]
- Amann, T.; Kailer, A.; Herrmann, M. Influence of Electrochemical Potentials on the Tribological Behavior of Silicon Carbide and Diamond-Coated Silicon Carbide. J. Bio Tribo Corros. 2015, 1, 30. [Google Scholar] [CrossRef]
- Kailer, A.; Amann, T.; Krummhauer, O.; Herrmann, M.; Sydow, U.; Schneider, M. Influence of electric potentials on the tribological behaviour of silicon carbide. Wear 2011, 271, 1922–1927. [Google Scholar] [CrossRef]
- Amann, T.; Gatti, F.; Oberle, N.; Kailer, A.; Rühe, J. Galvanically induced potentials to enable minimal tribochemical wear of stainless steel lubricated with sodium chloride and ionic liquid aqueous solution. Friction 2018, 6, 230–242. [Google Scholar] [CrossRef]
- Amann, T.; Dold, C.; Kailer, A. Potential Controlled Tribological Behavior of Water-Based Ionic Liquids. KEM 2016, 674, 250–256. [Google Scholar] [CrossRef]
- Dold, C.; Amann, T.; Kailer, A. Influence of electric potentials on friction of sliding contacts lubricated by an ionic liquid. Phys. Chem. Chem. Phys. 2015, 17, 10339–10342. [Google Scholar] [CrossRef]
- Gatti, F.; Amann, T.; Kailer, A.; Abicht, J.; Rabenecker, P.; Baltes, N.; Rühe, J. Makroskopische Reibwertsteuerung durch elektrochemische Potentiale mit ionischen Flüssigkeiten. Tribol. Und Schmier. 2019, 66, 51–57. [Google Scholar] [CrossRef]
- Capozza, R.; Benassi, A.; Vanossi, A.; Tosatti, E. Electrical charging effects on the sliding friction of a model nano-confined ionic liquid. J. Chem. Phys. 2015, 143, 144703. [Google Scholar] [CrossRef]
- Wang, Y.-L.; Golets, M.; Li, B.; Sarman, S.; Laaksonen, A. Interfacial Structures of Trihexyltetradecylphosphonium-bis(mandelato)borate Ionic Liquid Confined between Gold Electrodes. ACS Appl. Mater. Interfaces 2017, 9, 4976–4987. [Google Scholar] [CrossRef] [PubMed]
- Shah, F.U.; Holmgren, A.; Rutland, M.W.; Glavatskih, S.; Antzutkin, O.N. Interfacial Behavior of Orthoborate Ionic Liquids at Inorganic Oxide Surfaces Probed by NMR, IR, and Raman Spectroscopy. J. Phys. Chem. C 2018, 122, 19687–19698. [Google Scholar] [CrossRef]
- Pilkington, G.A.; Harris, K.; Bergendal, E.; Reddy, A.B.; Palsson, G.K.; Vorobiev, A.; Antzutkin, O.N.; Glavatskih, S.; Rutland, M.W. Electro-responsivity of ionic liquid boundary layers in a polar solvent revealed by neutron reflectance. J. Chem. Phys. 2018, 148, 193806. [Google Scholar] [CrossRef] [PubMed]
- Ghazipour, H.; Gutiérrez, A.; Alavianmehr, M.M.; Hosseini, S.M.; Aparicio, S. Tuning the properties of ionic liquids by mixing with organic solvents: The case of 1-butyl-3-methylimidazolium glutamate with alkanols. J. Mol. Liq. 2021, 347, 117953. [Google Scholar] [CrossRef]
- Lang, H.; Zou, K.; Chen, R.; Huang, Y.; Peng, Y. Role of Interfacial Water in the Tribological Behavior of Graphene in an Electric Field. Nano Lett. 2022, 22, 6055–6061. [Google Scholar] [CrossRef]
- Espinosa-Marzal, R.M.; Arcifa, A.; Rossi, A.; Spencer, N.D. Microslips to "Avalanches" in Confined, Molecular Layers of Ionic Liquids. J. Phys. Chem. Lett. 2014, 5, 179–184. [Google Scholar] [CrossRef] [PubMed]
- Espinosa-Marzal, R.M.; Arcifa, A.; Rossi, A.; Spencer, N.D. Ionic Liquids Confined in Hydrophilic Nanocontacts: Structure and Lubricity in the Presence of Water. J. Phys. Chem. C 2014, 118, 6491–6503. [Google Scholar] [CrossRef]
- Smith, A.M.; Parkes, M.A.; Perkin, S. Molecular Friction Mechanisms Across Nanofilms of a Bilayer-Forming Ionic Liquid. J. Phys. Chem. Lett. 2014, 5, 4032–4037. [Google Scholar] [CrossRef]
- Kawada, S.; Ogawa, S.; Sasaki, S.; Miyatake, M. Friction Control by Applying Electric Potential under Lubrication with Ionic Liquids. Tribol. Online 2019, 14, 71–77. [Google Scholar] [CrossRef]
- Guo, Y.; Liu, G.; Li, G.; Zhao, F.; Zhang, L.; Guo, F.; Zhang, G. Solvent-free ionic silica nanofluids: Smart lubrication materials exhibiting remarkable responsiveness to weak electrical stimuli. Chem. Eng. J. 2020, 383, 123202. [Google Scholar] [CrossRef]
- Michalec, M.; Svoboda, P.; Krupka, I.; Hartl, M.; Vencl, A. Investigation of the tribological performance of ionic liquids in non-conformal EHL contacts under electric field activation. Friction 2020, 8, 982–994. [Google Scholar] [CrossRef]
- Yang, X.; Meng, Y.; Tian, Y. Effect of Imidazolium Ionic Liquid Additives on Lubrication Performance of Propylene Carbonate under Different Electrical Potentials. Tribol. Lett. 2014, 56, 161–169. [Google Scholar] [CrossRef]
- Sader, J.E.; Chon, J.W.M.; Mulvaney, P. Calibration of rectangular atomic force microscope cantilevers. Rev. Sci. Instrum. 1999, 70, 3967–3969. [Google Scholar] [CrossRef]
- Li, Q.; Kim, K.-S.; Rydberg, A. Lateral force calibration of an atomic force microscope with a diamagnetic levitation spring system. Rev. Sci. Instrum. 2006, 77, 65105. [Google Scholar] [CrossRef]
- Filleter, T.; McChesney, J.L.; Bostwick, A.; Rotenberg, E.; Emtsev, K.V.; Seyller, T.; Horn, K.; Bennewitz, R. Friction and dissipation in epitaxial graphene films. Phys. Rev. Lett. 2009, 102, 86102. [Google Scholar] [CrossRef] [PubMed]
- Gosvami, N.N.; Ma, J.; Carpick, R.W. An In Situ Method for Simultaneous Friction Measurements and Imaging of Interfacial Tribochemical Film Growth in Lubricated Contacts. Tribol. Lett. 2018, 66, 154. [Google Scholar] [CrossRef]
- Ramakrishna, S.N.; Nalam, P.C.; Clasohm, L.Y.; Spencer, N.D. Study of adhesion and friction properties on a nanoparticle gradient surface: Transition from JKR to DMT contact mechanics. Langmuir 2013, 29, 175–182. [Google Scholar] [CrossRef] [PubMed]
- Nečas, D.; Klapetek, P. Gwyddion: An open-source software for SPM data analysis. Open Phys. 2012, 10, 181–188. [Google Scholar] [CrossRef]
- Gold, P.W.; Schmidt, A.; Dicke, H.; Loos, J.; Assmann, C. Viscosity–pressure–temperature behaviour of mineral and synthetic oils. J. Synth. Lubr. 2001, 18, 51–79. [Google Scholar] [CrossRef]
- Hamrock, B.J.; Dowson, D. Isothermal Elastohydrodynamic Lubrication of Point Contacts: Part III—Fully Flooded Results. J. Lubr. Tech. 1977, 99, 264–275. [Google Scholar] [CrossRef]
- Czichos, H.; Habig, K.-H. Tribologie-Handbuch: Tribometrie, Tribomaterialien, Tribotechnik, 4th ed.; Springer Vieweg: Wiesbaden, Germany, 2015. [Google Scholar]
- Bennewitz, R.; Gnecco, E.; Gyalog, T.; Meyer, E. Atomic friction studies on well-defined surfaces. Tribol. Lett. 2001, 10, 51–56. [Google Scholar] [CrossRef]
- Marchetto, D.; Held, C.; Hausen, F.; Wählisch, F.; Dienwiebel, M.; Bennewitz, R. Friction and Wear on Single-Layer Epitaxial Graphene in Multi-Asperity Contacts. Tribol. Lett. 2012, 48, 77–82. [Google Scholar] [CrossRef]
- Kwon, S.; Lee, K.E.; Lee, H.; Koh, S.J.; Ko, J.-H.; Kim, Y.-H.; Kim, S.O.; Park, J.Y. The Effect of Thickness and Chemical Reduction of Graphene Oxide on Nanoscale Friction. J. Phys. Chem. B 2018, 122, 543–547. [Google Scholar] [CrossRef]
- Chen, L.; Xiao, C.; He, X.; Yu, B.; Kim, S.H.; Qian, L. Friction and Tribochemical Wear Behaviors of Native Oxide Layer on Silicon at Nanoscale. Tribol. Lett. 2017, 65, 139. [Google Scholar] [CrossRef]
- Wang, K.; Zhang, J.; Ma, T.; Liu, Y.; Song, A.; Chen, X.; Hu, Y.; Carpick, R.W.; Luo, J. Unraveling the Friction Evolution Mechanism of Diamond-Like Carbon Film during Nanoscale Running-In Process toward Superlubricity. Small 2021, 17, e2005607. [Google Scholar] [CrossRef]
- Guo, D.; Li, J.; Xie, G.; Wang, Y.; Luo, J. Elastic properties of polystyrene nanospheres evaluated with atomic force microscopy: Size effect and error analysis. Langmuir 2014, 30, 7206–7212. [Google Scholar] [CrossRef] [PubMed]
- Montagne, A.; Tromas, C.; Audurier, V.; Woirgard, J. A new insight on reversible deformation and incipient plasticity during nanoindentation test in MgO. J. Mater. Res. 2009, 24, 883–889. [Google Scholar] [CrossRef]
- Mente, P.L.; Lewis, J.L. Elastic modulus of calcified cartilage is an order of magnitude less than that of subchondral bone. J. Orthop. Res. 1994, 12, 637–647. [Google Scholar] [CrossRef]
- Bertoldi, K.; Reis, P.M.; Willshaw, S.; Mullin, T. Negative Poisson’s ratio behavior induced by an elastic instability. Adv. Mater. 2010, 22, 361–366. [Google Scholar] [CrossRef] [PubMed]
- Young, M.L.; Almer, J.D.; Daymond, M.R.; Haeffner, D.R.; Dunand, D.C. Load partitioning between ferrite and cementite during elasto-plastic deformation of an ultrahigh-carbon steel. Acta Mater. 2007, 55, 1999–2011. [Google Scholar] [CrossRef]
- Deschamps, T.; Martinet, C.; Neuville, D.R.; de Ligny, D.; Coussa-Simon, C.; Champagnon, B. Silica under hydrostatic pressure: A non continuous medium behavior. J. Non-Cryst. Solids 2009, 355, 2422–2424. [Google Scholar] [CrossRef]
- Jacobs, T.D.B.; Pastewka, L.; Guest Editors. Surface topography as a material parameter. MRS Bull. 2022, 47, 1205–1210. [Google Scholar] [CrossRef]
- Lian, C.; Liu, K.; van Aken, K.L.; Gogotsi, Y.; Wesolowski, D.J.; Liu, H.L.; Jiang, D.E.; Wu, J.Z. Enhancing the Capacitive Performance of Electric Double-Layer Capacitors with Ionic Liquid Mixtures. ACS Energy Lett. 2016, 1, 21–26. [Google Scholar] [CrossRef]
- Osti, N.C.; Gallegos, A.; Dyatkin, B.; Wu, J.; Gogotsi, Y.; Mamontov, E. Mixed Ionic Liquid Improves Electrolyte Dynamics in Supercapacitors. J. Phys. Chem. C 2018, 122, 10476–10481. [Google Scholar] [CrossRef]
- Siimenson, C.; Siinor, L.; Lust, K.; Lust, E. Electrochemical Characterization of Iodide Ions Adsorption Kinetics at Bi(111) Electrode from Three-Component Ionic Liquids Mixtures. ECS Electrochem. Lett. 2015, 4, H62–H65. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gatti, F.J.; Cai, W.; Herzog, R.; Gharavian, A.; Kailer, A.; Baltes, N.; Rabenecker, P.; Mörchel, P.; Balzer, B.N.; Amann, T.; et al. Investigation of Programmable Friction with Ionic Liquid Mixtures at the Nano- and Macroscales. Lubricants 2023, 11, 376. https://doi.org/10.3390/lubricants11090376
Gatti FJ, Cai W, Herzog R, Gharavian A, Kailer A, Baltes N, Rabenecker P, Mörchel P, Balzer BN, Amann T, et al. Investigation of Programmable Friction with Ionic Liquid Mixtures at the Nano- and Macroscales. Lubricants. 2023; 11(9):376. https://doi.org/10.3390/lubricants11090376
Chicago/Turabian StyleGatti, Felix Joachim, Wanhao Cai, Richard Herzog, Amirmasoud Gharavian, Andreas Kailer, Norman Baltes, Peter Rabenecker, Philipp Mörchel, Bizan N. Balzer, Tobias Amann, and et al. 2023. "Investigation of Programmable Friction with Ionic Liquid Mixtures at the Nano- and Macroscales" Lubricants 11, no. 9: 376. https://doi.org/10.3390/lubricants11090376
APA StyleGatti, F. J., Cai, W., Herzog, R., Gharavian, A., Kailer, A., Baltes, N., Rabenecker, P., Mörchel, P., Balzer, B. N., Amann, T., & Rühe, J. (2023). Investigation of Programmable Friction with Ionic Liquid Mixtures at the Nano- and Macroscales. Lubricants, 11(9), 376. https://doi.org/10.3390/lubricants11090376






