Tribological Performance of a Composite Cold Spray for Coated Bores
Abstract
1. Introduction
- The use of materials/coatings with a low friction coefficient;
- Surface finish and/or texturing, especially by optimization using computer models and detailed topographic characterizations;
- Low-viscosity oils and improved friction-modifying additives.
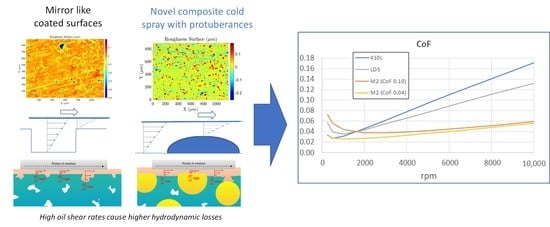
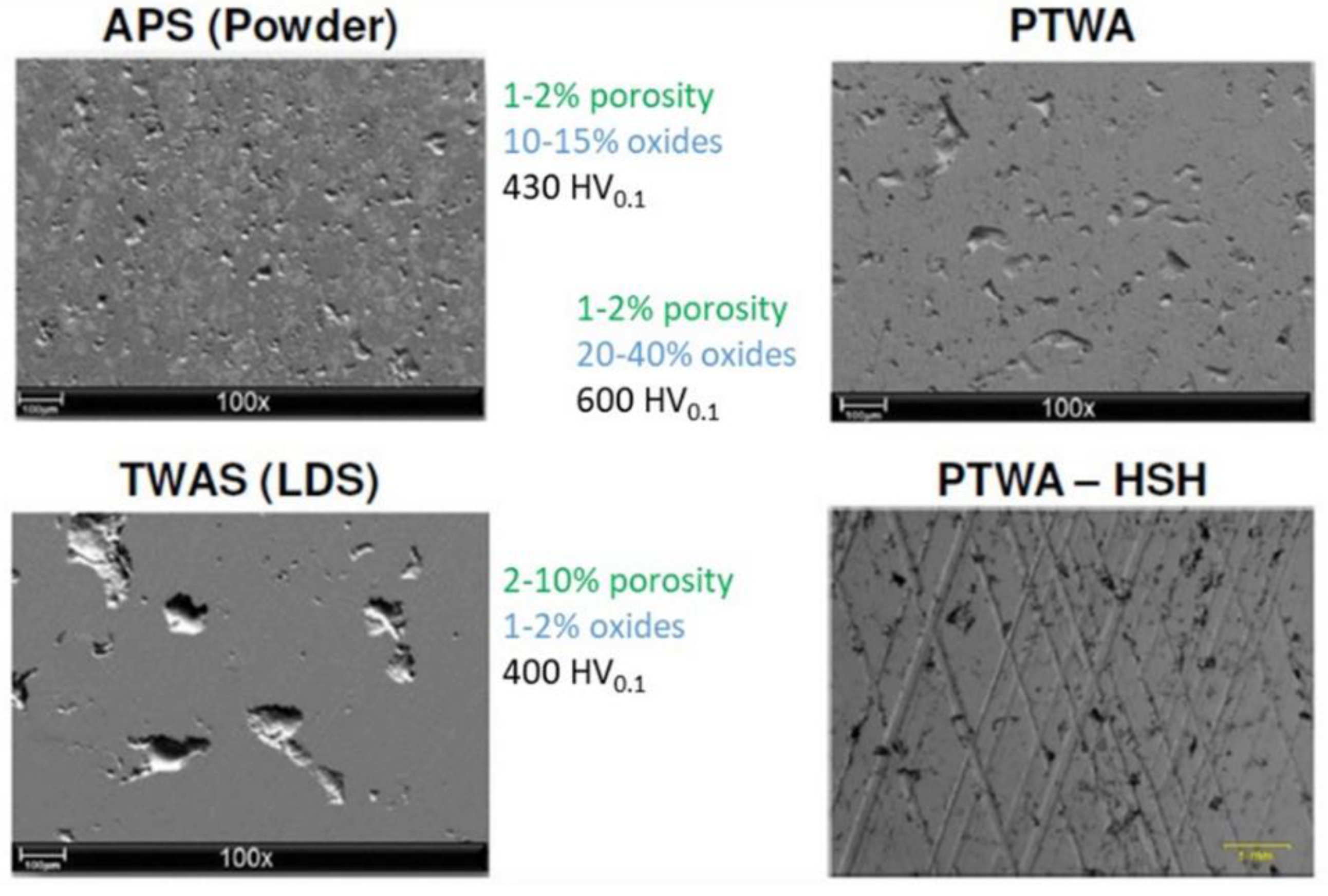
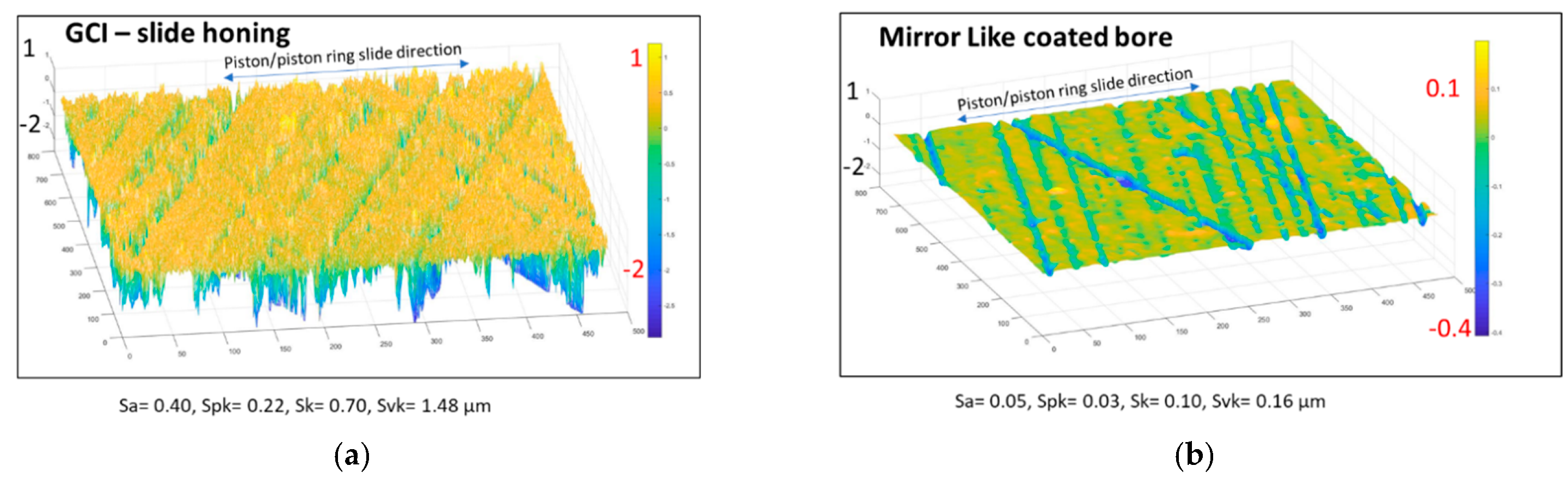
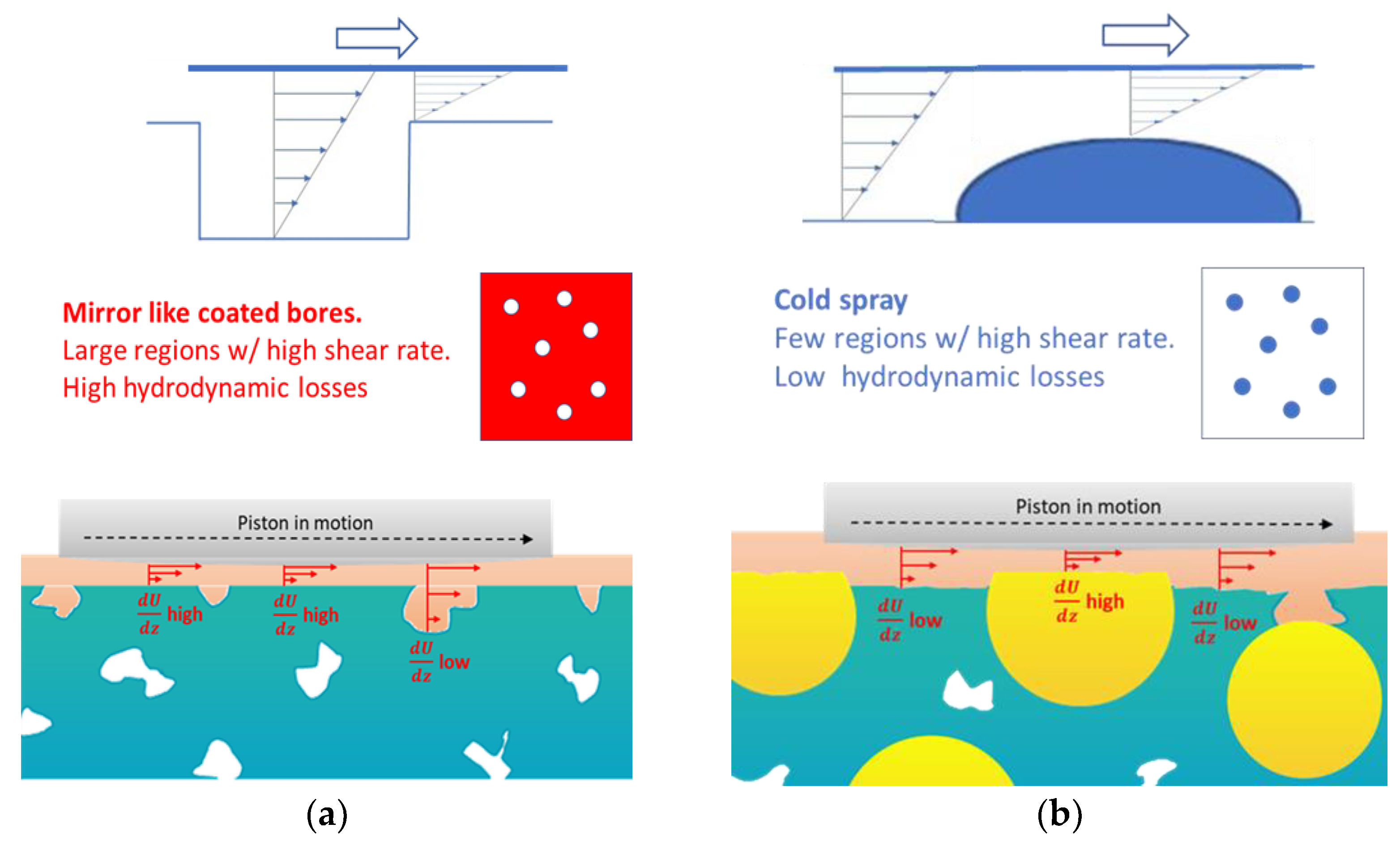
1.1. Mirror-like Coated Bores Produced by Thermal Spraying
1.2. Topographical Characterization of Textured Surfaces
1.3. Computer Models for Piston Ring and Cylinder Bores
- Classical Reynolds equation and stochastic asperity contact models: The hydrodynamic pressures are calculated using the Reynolds equation, considering the ring kinematics, the running profile and the physical properties of the lubricant. Under mixed lubrication conditions, where the oil film thickness is not enough to completely separate the surfaces, a statistically based rough contact model (usually the Greenwood–Williamson or Greenwood–Tripp model) is adopted to calculate the asperity contact pressures. This approach fails to account for the hydrodynamic pressures generated by the surface roughness, and it predicts zero hydrodynamic pressures for flat, parallel surfaces (e.g., oil control ring outer lands).
- Average Reynolds equation and flow factors: The previous approach can be improved for mixed lubrication analysis by incorporating the effect of surface roughness on lubrication through the adoption of averaging flow methods. In these cases, the influence of roughness (microscale) is considered by utilizing averaging parameters (flow factors) introduced in the Reynolds equation, which is then solved considering the macroscopic geometry of the contacting surfaces. The most common averaging method used for piston ring simulation is the Patir–Cheng average flow model, which provides a modified average Reynolds equation.
- Deterministic simulations: In this approach, the effect of surface topography is directly considered for the simulation of a mixed lubrication regime. The hydrodynamic and asperity contact problems are solved simultaneously in the same numerical framework. Due to the high CPU efforts, the most common approach is to segment the surface topography into small slices and then solve the coupled hydrodynamic and asperity contact problems in a quasistatic manner by imposing different separations between each slice and a rigid plane. Alternatively, fully deterministic simulations can be employed such that no segmentation is applied beforehand, and the surface separations are calculated based on the instantaneous load equilibrium.
2. Materials and Methods
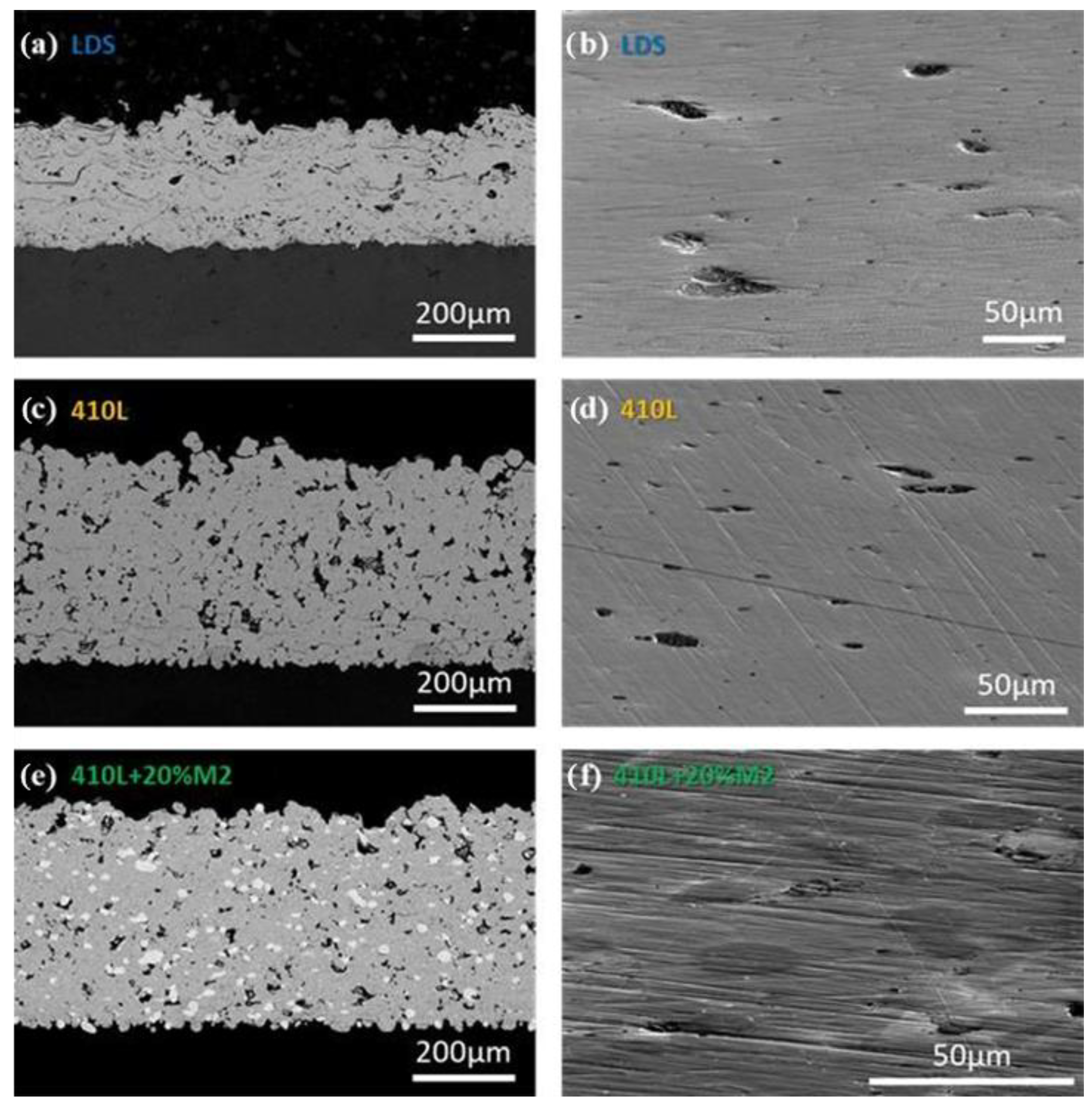
Coated Cylinder Bores
- LDS: already in production in recent engines; made with 13Mn6 steel and obtained by the LDS (Lichtbogen–Draht–Spritzen) process, also known as BSC (bore spray coating), TWA (twin wire arc) or TWAS (twin wire arc spray);
- 410L: obtained by a cold spray process using a 410L feed stock powder alone;
- 410L + 20% M2: obtained by cold spraying using the same 410L powder with the addition of 20% vol. M2 tool steel powder. The composite coating obtained by cold spraying the powder mixture consisted of a 410L stainless steel matrix with a hardness of 356 ± 39 Hv0.01. The 20% in volume of M2 tool steel in the powder stock resulted in about 8% area of particles with a hardness of 799 ± 81 Hv0.01.
3. Results
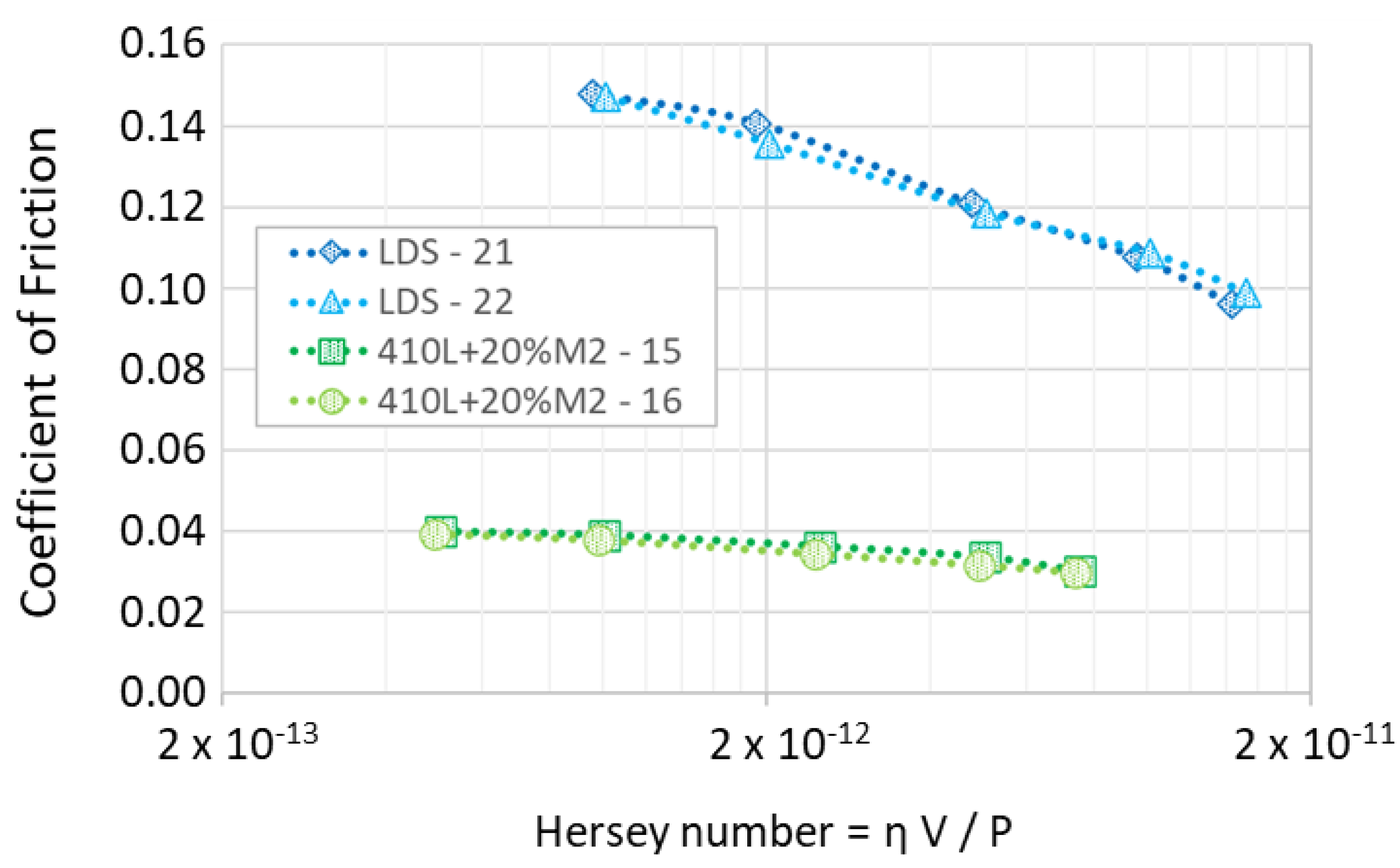
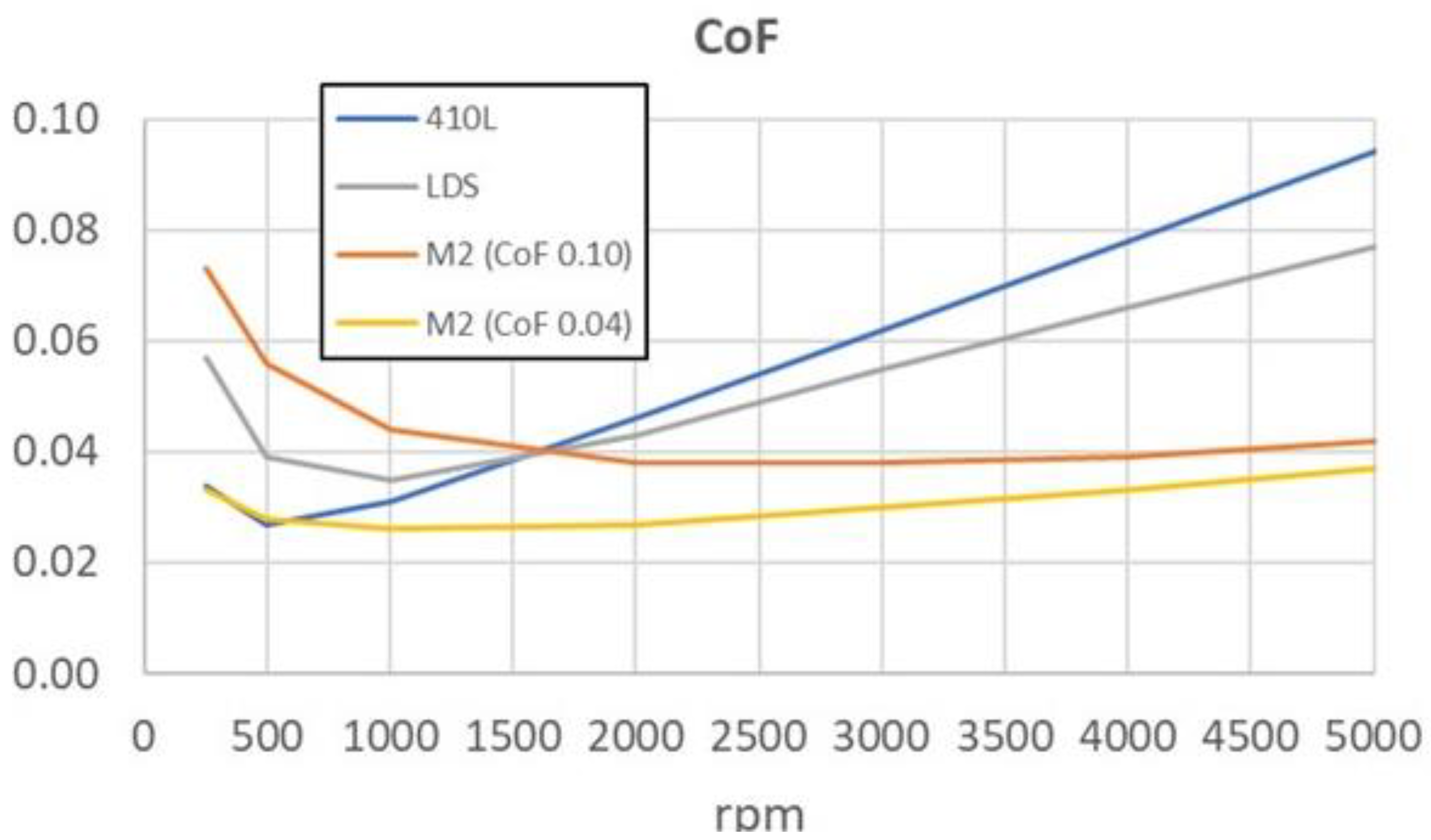
3.1. Tribological Tests
3.2. Surface Characterization
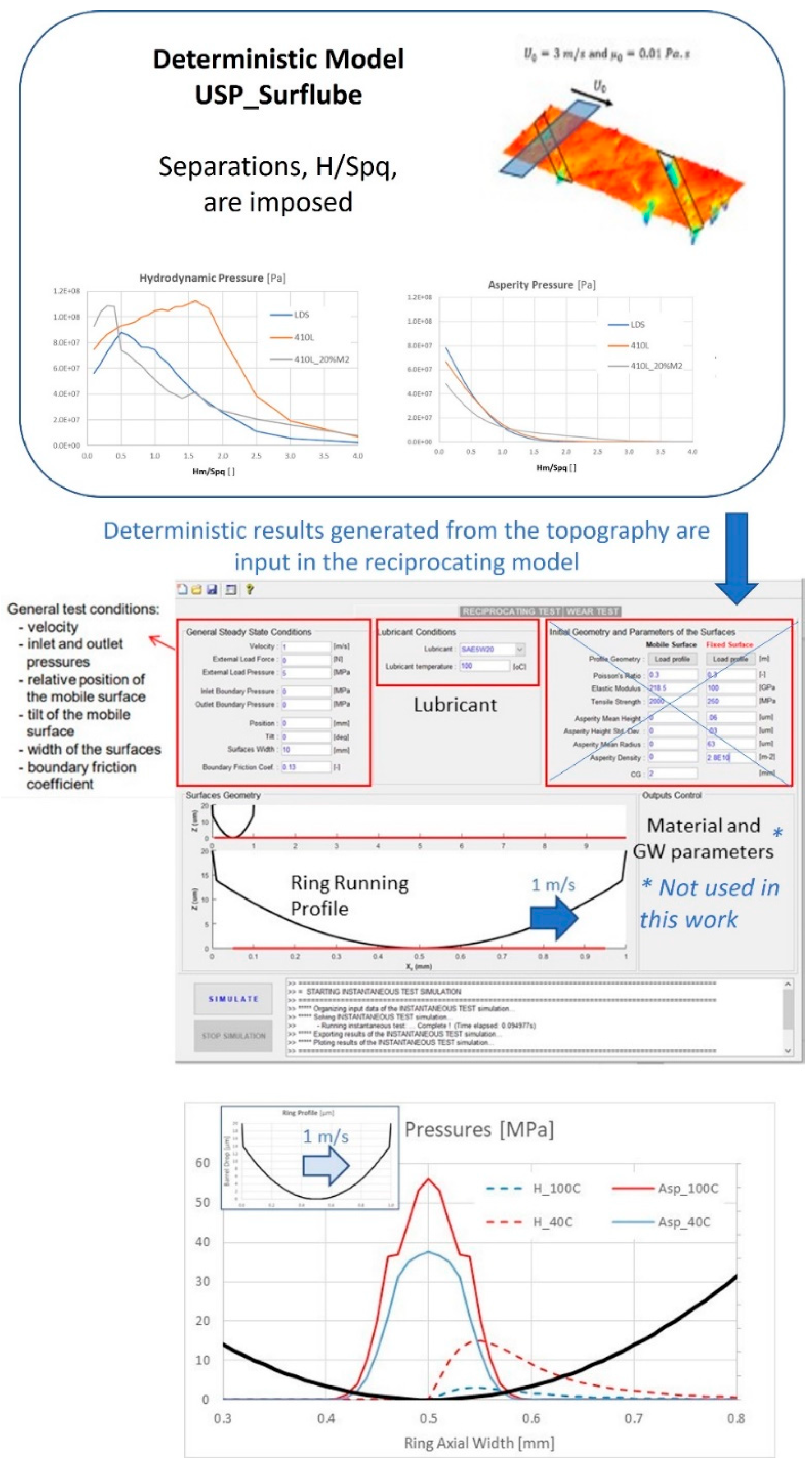
3.3. Computer Simulation
- Additional roughness filtering of surface form and waviness than can impact the contact. The measured topography is divided into 200 µm slices to minimize the CPU effort and to mimic the ring contact area;
- A deterministic model of the mixed regime in which surface separations, oil viscosity and speed are imposed to calculate the effect of the surface on oil flow, pressures and contact;
- Use of the averaged slice results, also known as “correlation factors”, from the deterministic model on a reciprocating ring/bore code with speeds, loads and oil viscosity values representative of the actual system to predict the instantaneous oil film thickness, friction losses, etc.
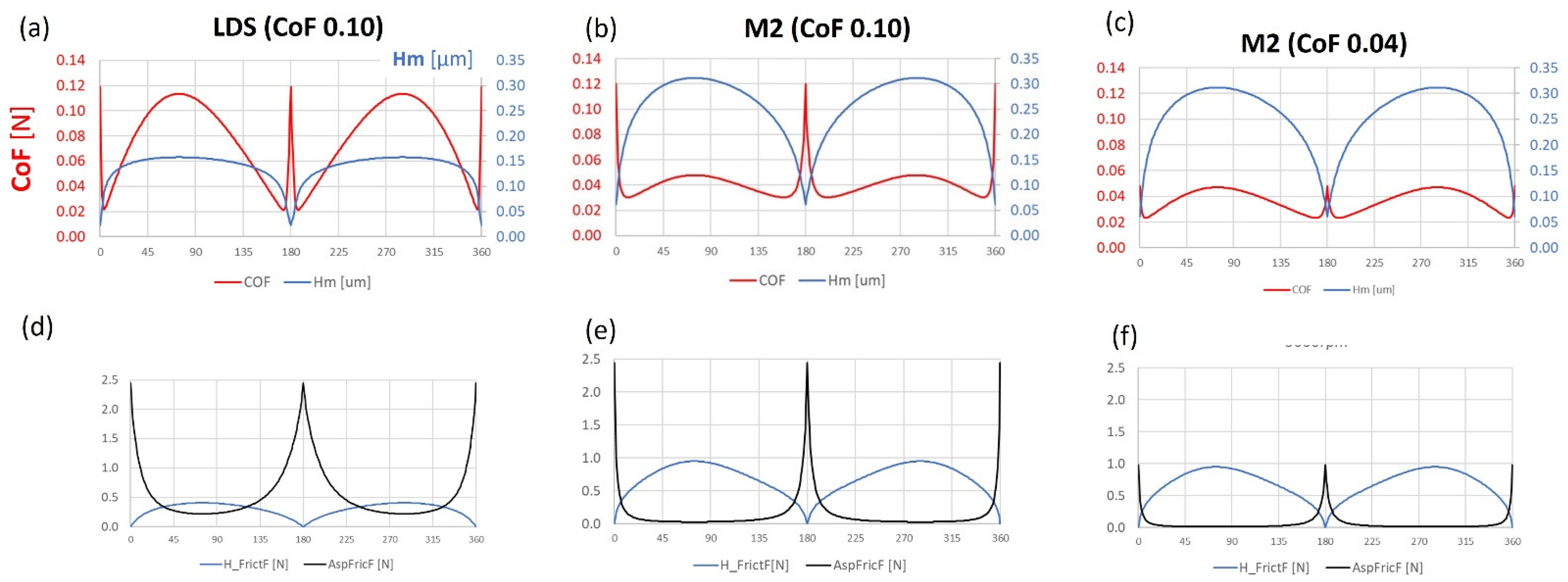
3.3.1. Deterministic Model
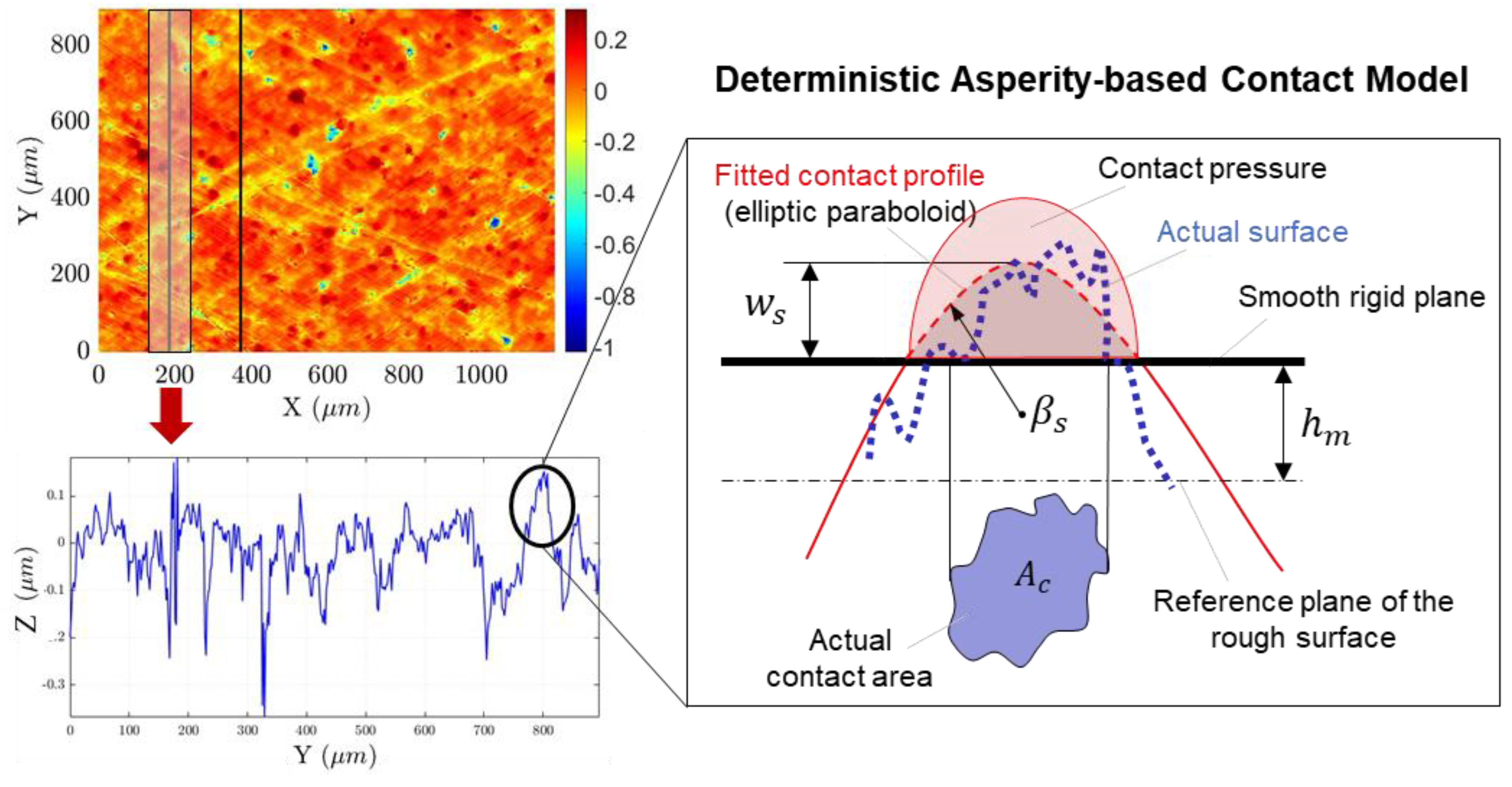
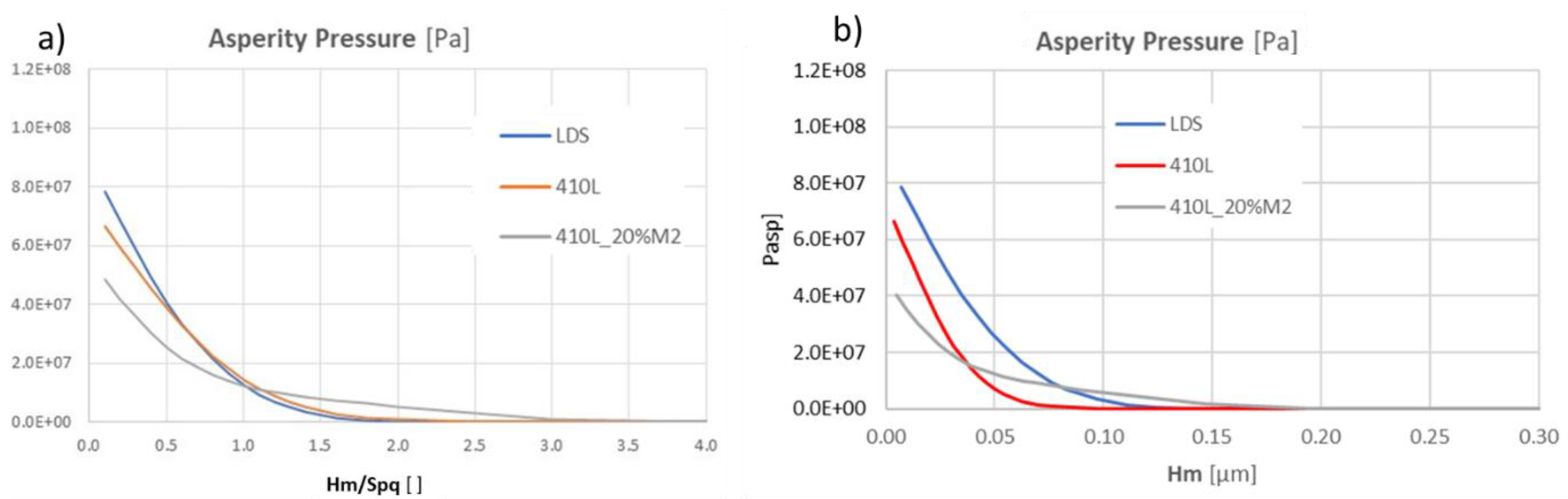
Asperity Contact Modeling
Hydrodynamic Friction Modeling
- Oil viscosity: 0.01 Pa·s;
- Oil flow speed: 3 m/s;
- Surface separations: 0.1 to 10× Spq.
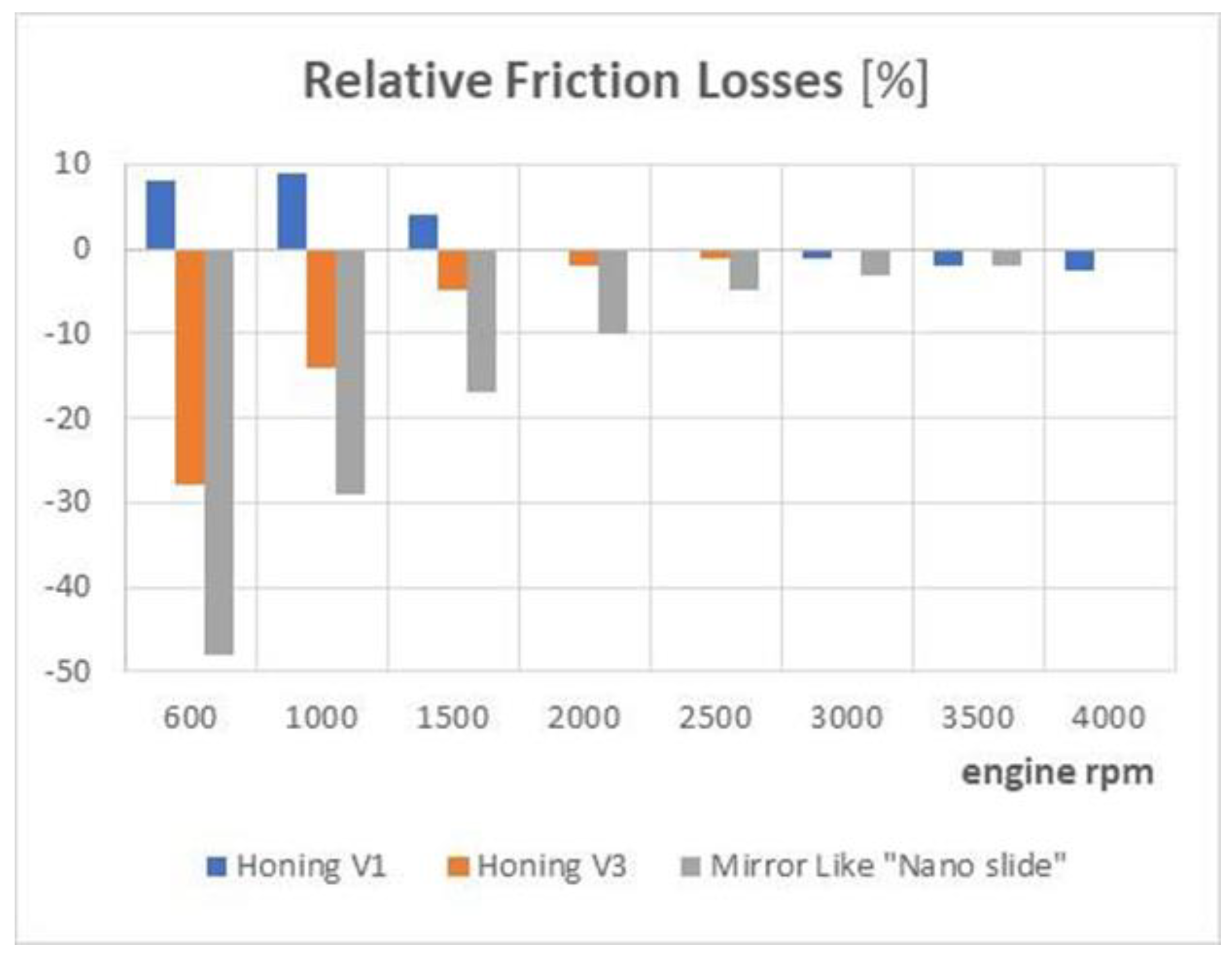
3.4. Piston Ring Reciprocating Simulation
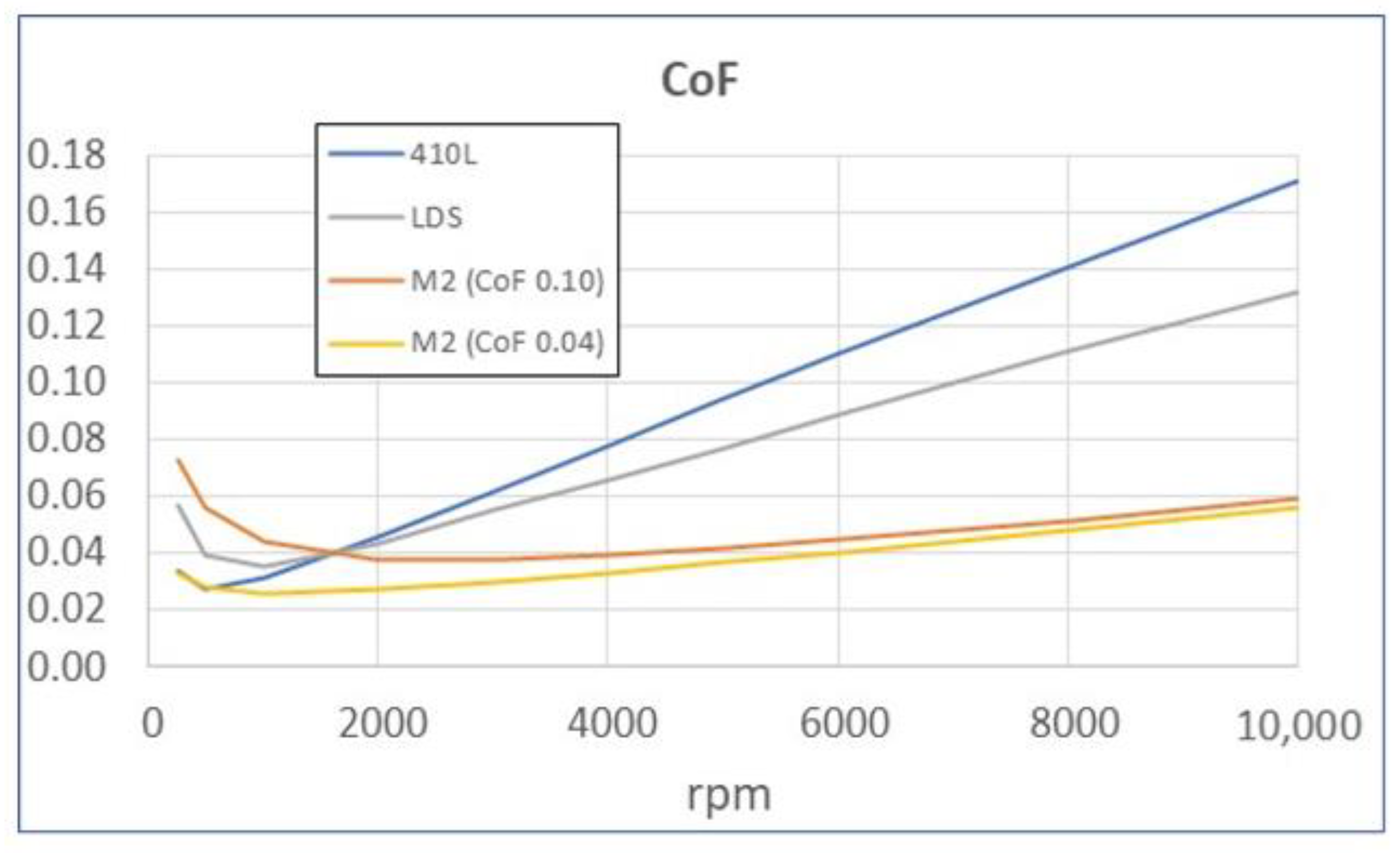
- M2 (CoF 0.10): data from the 410L + 20%, using the boundary CoF = 0.10 of the 410 and the LDS cases as input;
- M2 (CoF 0.04): same as before, with the asperity friction correlation factors multiplied by 0.40 to reproduce the experimental values when using oil FM, as presented in Figure 7.
4. Discussion
- -
- In boundary/mixed lubricant regimes, the protuberances help to create a MoS2 tribofilm when using friction modifiers (FMs) in the oils. The results and mechanisms were validated by experiments [20];
- -
- In the mixed/hydro regime, where the friction losses are dominated by the oil shear, the protuberances help to create a thicker oil film, reducing the shear rate, as illustrate in Figure 18.
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Surface Characterization and Function Orientated Analysis
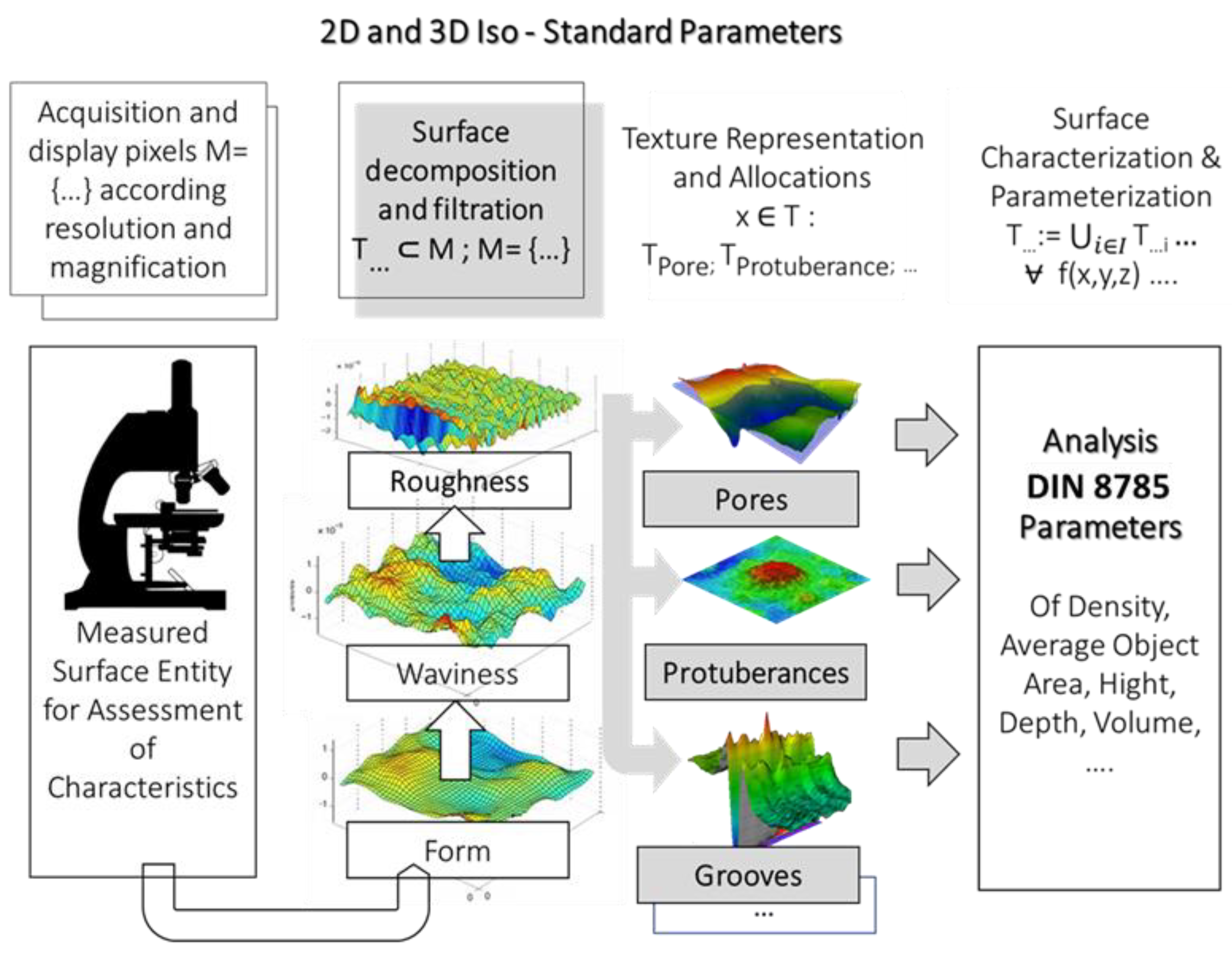
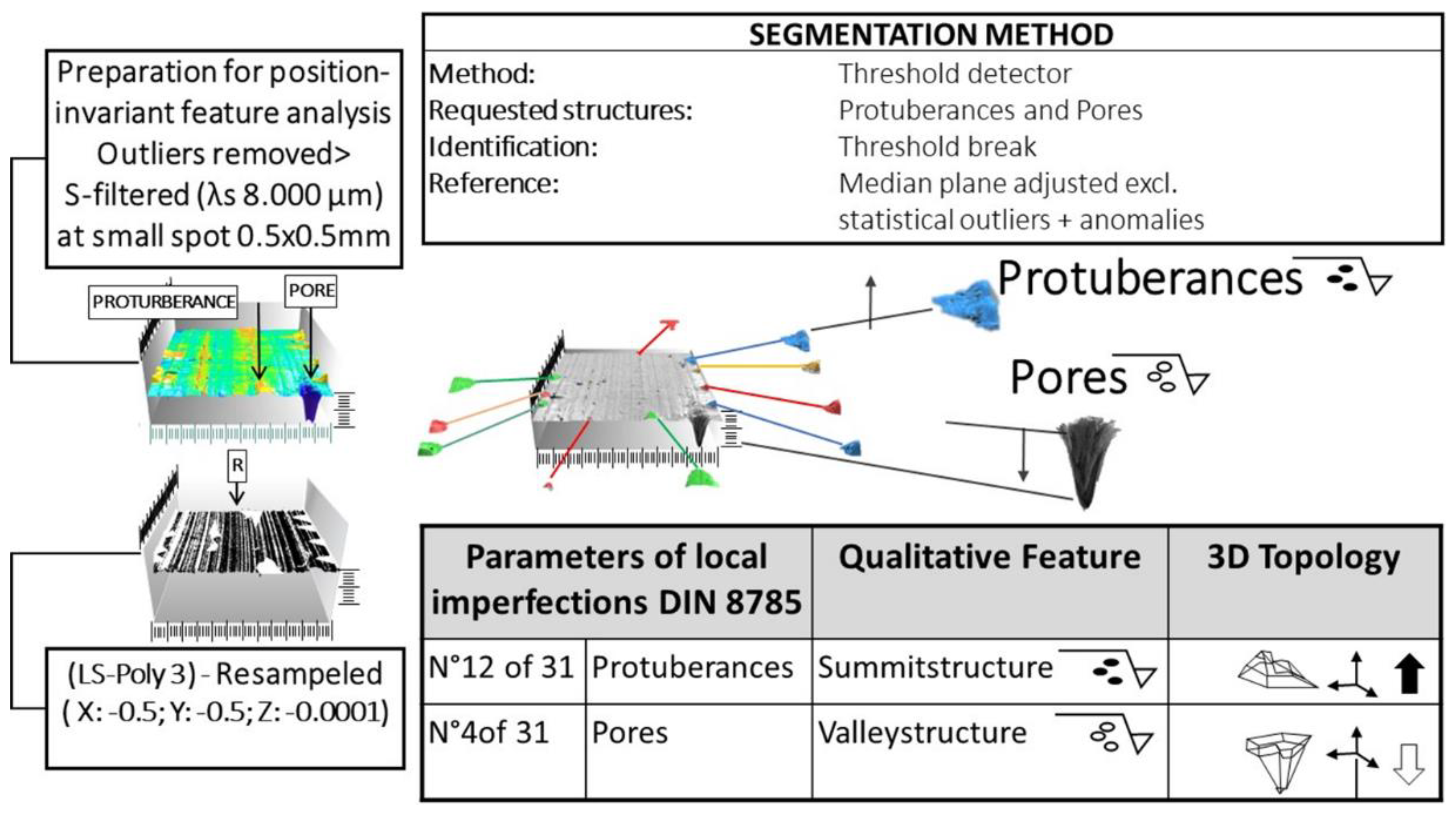
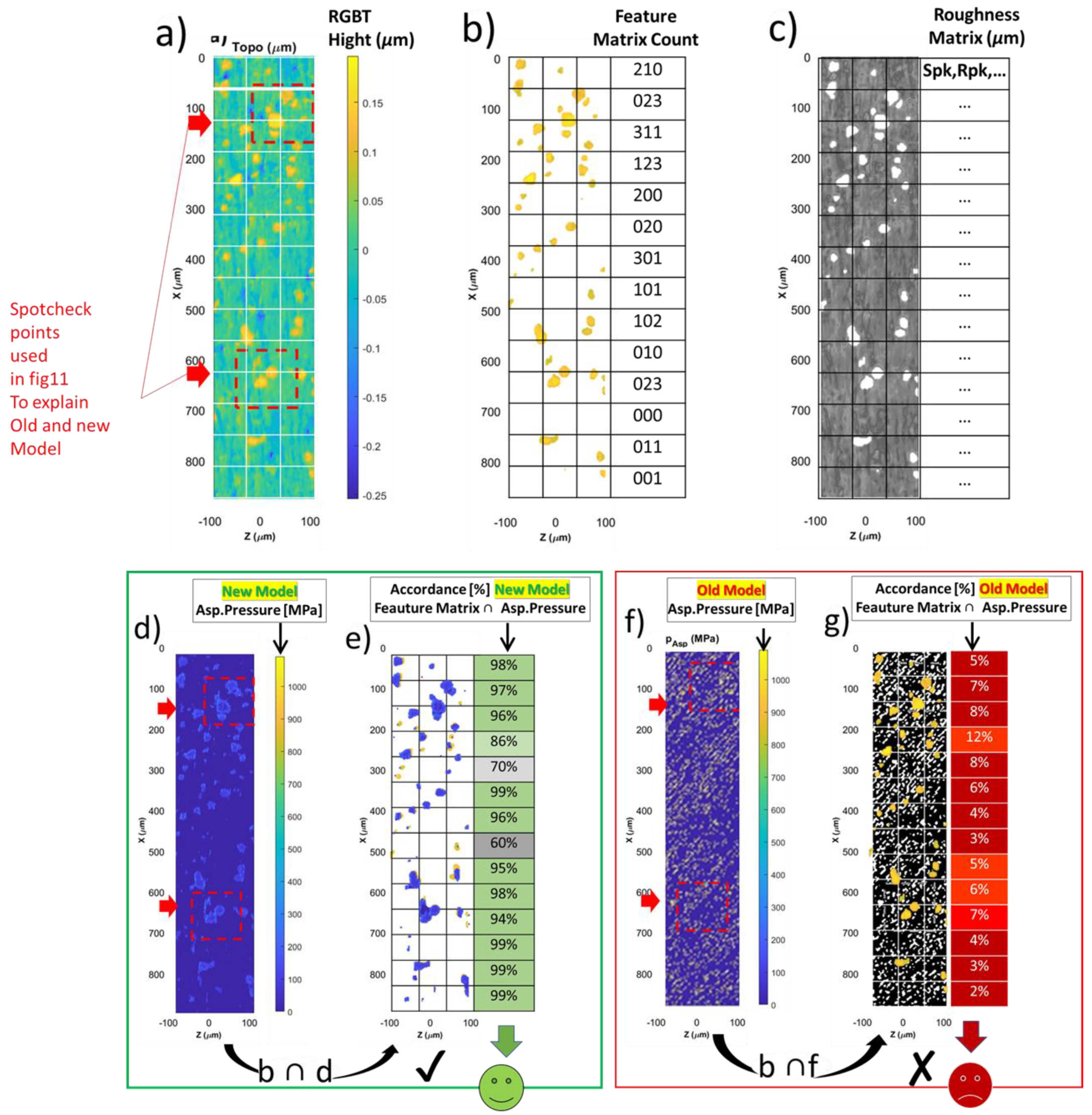
- Topography segmentation for feature extraction is applied (in this case protuberances), and the features are quantified. As an example, Figure A3b shows the protuberance density. As periodical features need to be excluded from standard roughness parameter calculation, the periodical portion of the surface can also be quantified as Figure A3c (which helps to validate each 200 µm slice of the remaining area without features);
- A joint feature plot is generated (Figure A3e, which contributed to better analysis of the objects for comparison with computer simulation of contact;
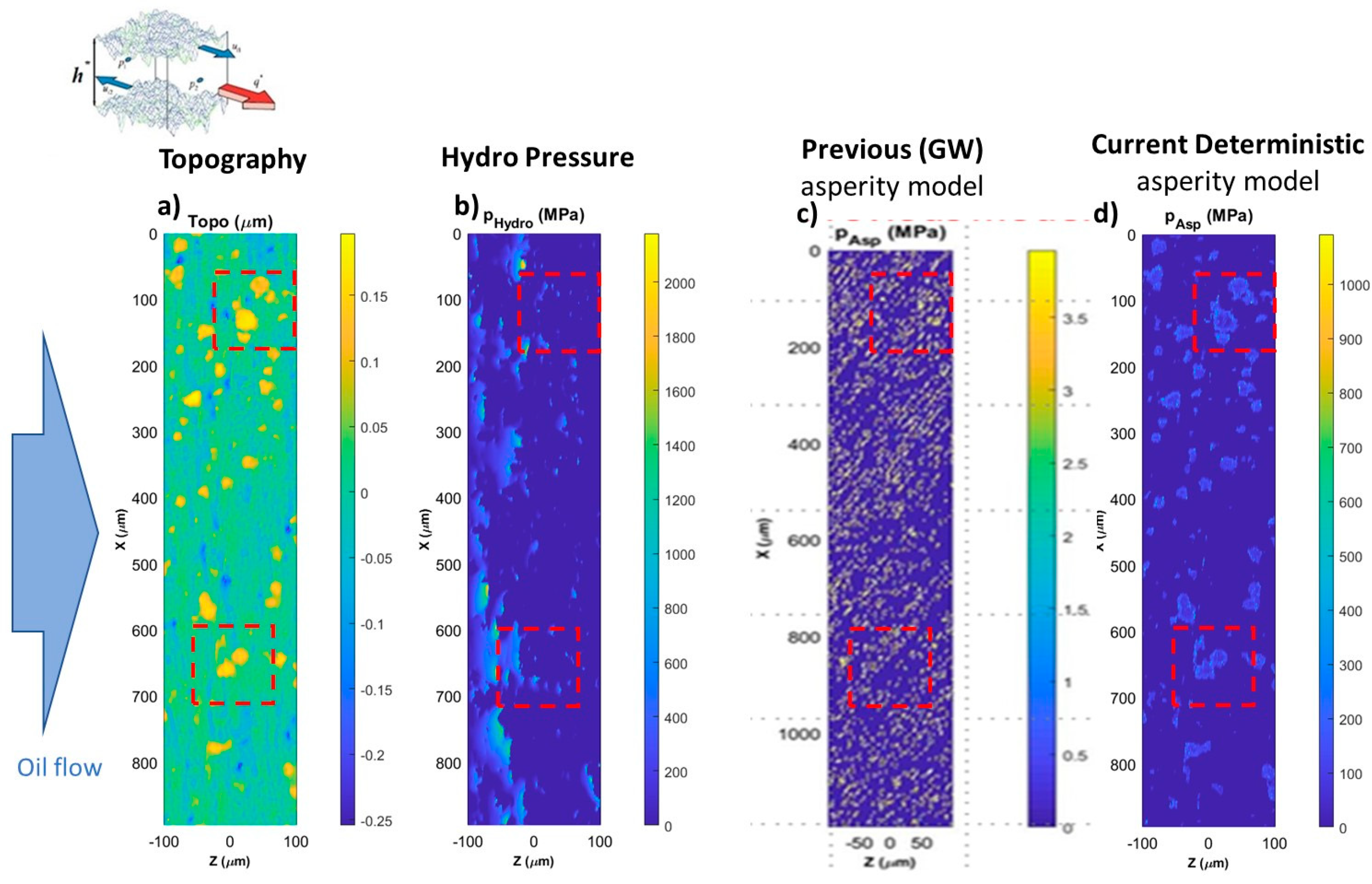
- The agreement between simulated data and theoretical knowledge/assumption can be evaluated. Figure A3d,f show asperity contact for two different simulation models. Figure A3e,g show that the surface segmentation can be used to validate the models. It is unlikely that the asperity contact does not match the segmented upstanding microplateaus. The predictions made with the new simulation model (Figure 10) are more realistic than those made with the previous model.
References
- Holmberg, K.; Andesson, P.; Erdemir, A. Global Energy consumption due to friction in passenger cars. Tribol. Int. 2012, 47, 221–234. [Google Scholar] [CrossRef]
- Holmberg, K.; Andersson, P.; Nylund, N.O.; Mäkelä, K.; Erdemir, A. Global Energy consumption due to friction in trucks and buses. Tribol. Int. 2014, 78, 94–114. [Google Scholar] [CrossRef]
- Tomanik, E.; Profito, F.; Sheets, B.; Souza, R. Combined Lubricant-Surface System Approach for Potential Passenger Car CO2 Reduction on Piston-ring cylinder bore assembly. Tribol. Int. 2020, 149, 105514. [Google Scholar] [CrossRef]
- Woydt, M.; Gradt, T.; Hosenfeldt, T.; Luther, R.; Rienäcker, A.; Wetzel, J.; Wincierz, C. Tribologie, frottement et émissions de CO2—Une étude de la Société allemande de tribology. Tech. l’ingénieur 2020, TRI140 v1, 23. [Google Scholar]
- Gachot, C.; Rosenkranz, A.; Hsu, S.M.; Costa, H.L. A critical assessment of surface texturing for friction and wear improvement. Wear 2017, 372–373, 21–41. [Google Scholar] [CrossRef]
- Zimmer, M.; Vlădescu, S.C.; Mattsson, L.; Fowell, M.; Reddyhoff, T. Shear-area variation: A mechanism that reduces hydrodynamic friction in macro-textured piston ring liner contacts. Tribol. Int. 2021, 161, 107067. [Google Scholar] [CrossRef]
- Zhmud, B.; Tomanik, E.; Jiménez, A.J.; Profito, F.; Tormos, B. Powertrain Friction Reduction by Synergistic Optimization of Cylinder Bore Surface and Lubricant—Part 2: Engine Tribology Simulations and Tests. SAE Int. 2021. [Google Scholar] [CrossRef]
- Spikes, H. The History and Mechanisms of ZDDP. Tribol. Lett. 2004, 17, 469–489. [Google Scholar] [CrossRef]
- Deshpande, P.; Minfray, C.; Dassenoy, F.; Thiebaut, B.; Le Mogne, T.; Vacher, B.; Jarnias, F. Tribological behaviour of TiO2 Atmospheric Plasma Spray (APS) coating under mixed and boundary lubrication conditions in presence of oil containing MoDTC. Tribol. Int. 2018, 118, 273–286. [Google Scholar] [CrossRef]
- Martin, J.; Grossiord, C.; Varlot, K.; Vacher, B.; Igarashi, J. Synergistic effects in binary systems of lubricant additives: A chemical hardness approach. Tribol. Lett. 2000, 8, 193–201. [Google Scholar] [CrossRef]
- Hriouech, A. Études de Surfaces Texturées Pour Chemise Moteur: Physico-Chimie des Tribofilms et Lois de Frottement Associées. Ph.D. Thesis, Université de Lyon, Lyon, France, 15 November 2021. [Google Scholar]
- Zheng, D.; Cai, Z.-B.; Shen, M.-X.; Li, Z.-Y.; Zhu, M.-H. Investigation of the tribology behaviour of the graphene nanosheets as oil additives on textured alloy cast iron surface. Appl. Surf. Sci. 2016, 387, 66–75. [Google Scholar] [CrossRef]
- Hoffmeister, H.-W.; Grosse, T.; Gerdes, A. Investigation of the influence of different process setting parameters on the surface formation at honing of thermally sprayed layers. Procedia CIRP 2012, 1, 371–376. [Google Scholar] [CrossRef]
- Klink, U.; Dellin M, B.; Kraushaar, S. Umweltbewusst und Kostengünstig Fertigen, 2nd ed.; Carl Hanser Verlag GmbH & Co. KG: Munich, Germany, 2022. [Google Scholar]
- Tomanik, E.; Grabon, W.; Profito, F.; Bussas, M. Effect of the coated bore pores on the piston ring tribology through deterministic simulation. Int. Colloq. Tribol. Essl. 2020, 28, 30. [Google Scholar]
- Schütz, M.; Döll, G.; Waltner, A.; Kemmler, R. The new Mercedes-Benz 3.0-l V6 DI gasoline engine with twin turbo. MTZ Worldw. 2013, 74, 18–24. [Google Scholar] [CrossRef]
- Tomanik, E.; Profito, F.J.; Tormos, B.; Jiménez, A.J.; Zhmud, B. Powertrain Friction Reduction by Synergistic Optimization of the Cylinder Bore Surface and Lubricant Part 1: Basic Modelling. SAE Tech. Pap. 2021. [Google Scholar] [CrossRef]
- Böhm, J.; Hartweg, M.; Hercke, T.; Izquierdo, P.; Michel, M.; Schweickert, S.; Rau, G. Thermisch beschichtetes Bauteil mit einer reibungsoptimierten Laufbahnoberfläche und Verfahren zur Bauteil-Beschichtungsoberflachensimulation eines thermisch beschichteten Bauteils. DE Patent 10 2012 002 766 B4, 22 May 2014. [Google Scholar]
- Simon, M. Topografiebasierte Reibungssimulation von Kolbenringen in Verbrennungsmotoren. Ph.D. Thesis, Ulm University, Ulm, Germany, 21 July 2021. [Google Scholar]
- Aubanel, L. Étude du Comportement Tribologique en Régime Lubrifié de Revêtements Cold Spray Composites à Base D’acier. Matériaux. Ph.D. Thesis, Université Paris Sciences et Lettres, Paris, France, 2 December 2022. [Google Scholar]
- Tomanik, E.; El Mansori, M.; Souza, R.; Profito, F. Effect of waviness and roughness on cylinder liner friction. Tribol. Int. 2018, 120, 547–555. [Google Scholar] [CrossRef]








| Parameter | Unit | LDS | 410L | 410L + 20% M2 |
|---|---|---|---|---|
| Sa | nm | 53 | 32 | 44 |
| Spk | nm | 64 | 30 | 102 |
| Sk | nm | 91 | 89 | 105 |
| Svk | nm | 271 | 72 | 87 |
| Pore surface ratio | % | 2.2 ± 0.6 | 0.7 ± 0.4 | 0.8 ± 0.4 |
| Density | 1/mm2 | 292 ± 117 | 511 ± 193 | 385 ± 99 |
| Avg. area | µm2 | 84 ± 34 | 14 ± 4 | 20 ± 7 |
| Avg. volume | µm3 | 64 ± 73 | 0.5 ± 0.3 | 1.5 ± 1.1 |
| Protuberance surface ratio | % | 0.79 ± 0.95 | 7.5 ± 0.5 | |
| Density | 1/mm2 | 1.40 ± 1.48 | 208 ± 11 | |
| Diameter | µm | 75.5 ± 36.1 | 18.8 ± 7.1 | |
| Avg. height | nm | 184 ± 93 | 119 ± 15 | |
| Max. height | nm | 338 ± 248 | 198 ± 22 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tomanik, E.; Aubanel, L.; Bussas, M.; Delloro, F.; Lampke, T. Tribological Performance of a Composite Cold Spray for Coated Bores. Lubricants 2023, 11, 127. https://doi.org/10.3390/lubricants11030127
Tomanik E, Aubanel L, Bussas M, Delloro F, Lampke T. Tribological Performance of a Composite Cold Spray for Coated Bores. Lubricants. 2023; 11(3):127. https://doi.org/10.3390/lubricants11030127
Chicago/Turabian StyleTomanik, Eduardo, Laurent Aubanel, Michael Bussas, Francesco Delloro, and Thomas Lampke. 2023. "Tribological Performance of a Composite Cold Spray for Coated Bores" Lubricants 11, no. 3: 127. https://doi.org/10.3390/lubricants11030127
APA StyleTomanik, E., Aubanel, L., Bussas, M., Delloro, F., & Lampke, T. (2023). Tribological Performance of a Composite Cold Spray for Coated Bores. Lubricants, 11(3), 127. https://doi.org/10.3390/lubricants11030127