Aerodynamic forces due to blade-tip clearance eccentricity are a known source mechanism for destabilizing rotating machinery with unshrouded impellers (turbines and compressors). While in operation, a static offset of a turbine or compressor rotor changes the blade-tip clearance and induces non-uniform flow and pressure fields acting on the blades around the rotor. Aerodynamic forces are typically load- and speed-dependent. In addition, for shrouded impellers, dynamic forces also arise due to changes in pressure within the small clearance between the compressor (or turbine) casing and its shroud. Lateral rotor motions induce this type of impeller–shroud excitation force, which can affect the stability of shrouded wheels.
3.1. Aerodynamic Forces in (Unshrouded) Axial Turbines and Axial Compressors
In 1958, Thomas [
4] postulated that destabilizing aerodynamic forces in axial (steam) turbines are the result of the non-uniform tip clearance induced by an eccentric rotor, resulting in the whirl of the rotor. In a turbine whose center is eccentric (
e), Thomas noted that variations in the clearance cause a change in blade efficiency, i.e., blades with a smaller tip clearance restrict leakage, and the force acting on the blade increases, see
Figure 6. The opposite effect occurs in the region of larger local tip clearance. As a result, a net tangential force (
Ft) is generated that tends to cause forward (destabilizing) whirl; i.e., in the direction of rotor spinning and with
Ft = (
k ×
e). The Thomas “clearance–excitation” force is proportional to the mass flow rate (
) through the turbine and the enthalpy drop (Δ
HD) across a stage, and inversely proportional to shaft speed (Ω). Thus, this force is load-dependent, a function of the applied torque
(T0). Recall that mechanical power equals (
T0 × Ω)~
.
In 1965, Alford [
5] advanced a similar model for an axial compressor. Alford reasoned that in a whirling rotor, blades with a smaller tip clearance operate more efficiently and the blades are more lightly loaded than those with a larger clearance. This imbalance of blade forces results in a net follower or tangential force that promotes forward rotor whirl,
Ft~(
k ×
e). Alford’s cross-coupled stiffness (
k) equals
where
To is the compressor stage torque, and
D and
hB are the mean tip diameter and blade length, respectively. Above,
β is “the change of thermodynamic efficiency per unit of rotor displacement, expressed as fraction of blade height.” Alford recommended
β ranging from 1.0 to 1.50. Most importantly, as argued by Alford, the aerodynamic force is forward (direction of shaft rotation) for both compressors and turbines. Hence, in Alford’s theory, there is no distinction between the physical operation of both types of machines.
For an axial turbine (unshrouded) stage, the experimental results from Urlichs [
22] verified Thomas’ clearance–excitation force model. Conducted with blades whose tips had variable axial and radial tip clearances, the measurements recorded both a tangential force and a radial force, as a function of the static rotor offset relative to its casing. In the measurements, the radial force was not a centering force and was a sizeable fraction of the tangential force. Later, in 2005, Kanki and Tanitsuji [
23] presented experimental results demonstrating that a turbine, operating under partial admission, has a larger destabilizing aerodynamic force that is greatly affected by the pattern of partial admission. Note that Alford’s Equation (9) is not explicitly a function of the tip clearance, whereas measurements demonstrate that enlarged clearances determine smaller forces (and less efficient stages); see the work of Ehrich [
6]. In addition, the model in Equation (9) fails to quantify the (measured) centering force with direct stiffness
K.
In 1984, Vance and Laudadio [
24] conducted measurements of aerodynamic forces in a small, high-speed, axial-flow fan with a moveable casing, to control the rotor offset. In the tests, the rotor deflection and speed and drive torque were measured. In spite of the poor test repeatability (typically ±20% for two successive measurements), the test data showed that Alford’s force is a function of speed as well as of torque. The aerodynamic force inducing forward whirl did not appear until a certain torque threshold was achieved. However, under specific test conditions, the aerodynamic force became negative; i.e., driving backward whirl (
k < 0).
In his textbook (1992), Ehrich [
21] advanced the argument above and questioned Alford’s physical arguments. Normalization of the test data from Vance and Laudadio [
24] showed that Alford’s force varied linearly with torque, as predicted, but evidencing a positive magnitude above a certain threshold torque. The magnitude of the tangential force increases as the shaft speed increases. Below the threshold torque, the force is negative and drives the backward whirl of the test rotor.
Based on the actuator disc model of Horlock and Greitzer [
25] (1983), Colding-Jorgensen [
26] (1992) introduced an analysis to determine the direction of the aerodynamic force in axial unshrouded compressors. The predictions showed that forward whirl prevails over the normal operating range of a compressor, although backward whirl can be present for extremely low flow rates (i.e., stall conditions). The predicted
β was ~2.0, a typical value assumed for compressors.
For axial compressors, the correct sign (positive or negative) of the aerodynamic force was a major unknown. Not until 1993 did Ehrich [
6] demonstrate that the aerodynamic (tangential) force in axial compressors destabilizes backward rotor whirl and stabilizes forward rotor whirl motions. That is
k < 0! Later in 2001, a comprehensive experimental effort [
27,
28] endorsed Ehrich’s assertion. Ehrich argued that since (axial) compressor blades with smaller tip clearances operate more efficiently, they are subject to a higher static pressure difference across their tips and are, in fact, more highly loaded than blades with a larger tip clearance (opposite to Alford’s argument). Hence, in an axial flow compressor, the net tangential force direction promotes backward rotor whirl.
Litang et al. [
29] (1994) conducted strain gauges measurements on a single stage axial compressor, to determine the direction of Alford’s force. The experimental results showed that Alford’s force acts in a direction opposite to the shaft rotation, thus promoting backward whirl. In addition, the non-uniformly distributed dynamic pressure around the compressor also generates a radial force component that acts in the direction opposite to rotor eccentricity, i.e., a centering stiffness (
K > 0).
In the late 1990s, Storace et al. [
27] and Ehrich et al. [
28] (2000), at the GE Low Speed Research Compressor Facility, conducted a large-scale research effort to resolve the apparent disparity in findings, by definitively determining the direction of whirl caused by Alford’s force in axial compressors. The test program used an axial flow compressor with several distinct blade configurations, although only one configuration with a static rotor offset (eccentricity). The measurements showed that
β < 0 over most of the compressor operating speed range, with some near-zero and small positive values occurring at high flow rates near the design point. The authors, based on their definitive measurements, stressed that backward-whirl-inducing forces are present in axial flow compressors. Incidentally, measurements of blade loading showed that blades with a smaller tip clearance are more highly loaded than those with a larger tip clearance; hence, validating Ehrich’s model of compressor whirl forces.
Implementing a “parallel compressor” model and using the data collected at the GE Low Speed Research Compressor facility (a four stage compressor and two tip clearances), Ehrich et al. [
28] reported
β ranging from +0.27 (forward whirl) to −0.71 (backward whirl) along a normal operating line;
β~0.08 to −1.21 in the vicinity of blade peak efficiency; and
β < 0 (−1.16 to −3.36) near the compressor stall condition. In their experiments, backward rotor whirl appeared over much of the compressor’s normal operating range, with strong backward rotor whirl near stall operating conditions.
In 2000, Spakovszky [
30] and Song and Cho [
31] developed computational physical models to more accurately predict
β. Spakovszky introduced an additional parameter (
βspool), known as the blade loading indicator, that denotes the effect of a non-axisymmetric pressure distribution on the rotor spool. This effect, first identified by Song and Martinez-Sanchez [
32] (1997), induces an additional tangential force in turbines and is suspected to play a role in compressors as well. Under a steady shaft offset displacement (eccentricity), Spakovszky predicted a strong tendency towards backward whirl for operation at low flow coefficients, whereas forward whirl appears for high flow coefficients. These findings are in agreement with Ehrich’s measurements [
6] (1993). Spakovszky found
βspool > 0 over most of the compressor operating range, i.e., inducing forward whirl, though its magnitude is typically ~50% of Alford’s
β parameter. However, under forced whirling conditions,
βspool is comparable in magnitude to Alford’s
β, but acting in the direction opposite of the whirl. The blade loading indicator (
βspool), a function of the stage geometry and mean flow, determines the direction of whirl due to tangential blade loading forces.
The model by Song and Cho [
31] also includes the effect of a non-axisymmetric pressure distribution and predicts the velocity and pressure fields induced by tip clearance asymmetry. Torque asymmetry determines a negative cross-coupled force, i.e., promoting backward whirl, while pressure asymmetry brings a larger positive cross force (
k > 0). Thus, the end effect induces a forward whirl force in axial turbines. The analysis also revealed a positive radial force, mainly due to pressure asymmetry. In a follow up paper, Song and Park [
33] (2001) presented a novel actuator–disk model to analyze flow redistribution as a result of rotor-tip clearance in axial compressors. Their predictions showed that the cross-coupled force is negative, thus promoting backward whirl. In brief, the tangential force induced by a negative (drag) torque is slightly larger than the positive force originating from pressure asymmetry.
In 2003, Al-Nahwi et al. [
34] presented a first principles-based model of the fluid-induced forces acting on an axial compressor and combined it with the Moore–Greitzer model [
35] of an axial compressor flow field. Myllerup and Keith [
36] (2004) [
24] further advanced the model by Al-Nahwi et al. for ready use in a rotordynamics predictive program. The model identifies three contributions to the overall value of the cross-coupled (tangential) aerodynamic force: nonuniform torque, nonuniform hydrostatic pressure, and unsteady momentum storage within the rotor blade passages. These three contributions are represented by four terms in the model: one torque term, two pressure terms, and one unsteady momentum term. The model shows that, since the terms may all be of comparable magnitude, all of the force contributions must be taken into account to accurately predict the whirl direction of the aerodynamic force. Thus, aerodynamic forces can induce forward or backward whirl, depending on the operating conditions of the compressor, namely the flow rate and whirl frequency.
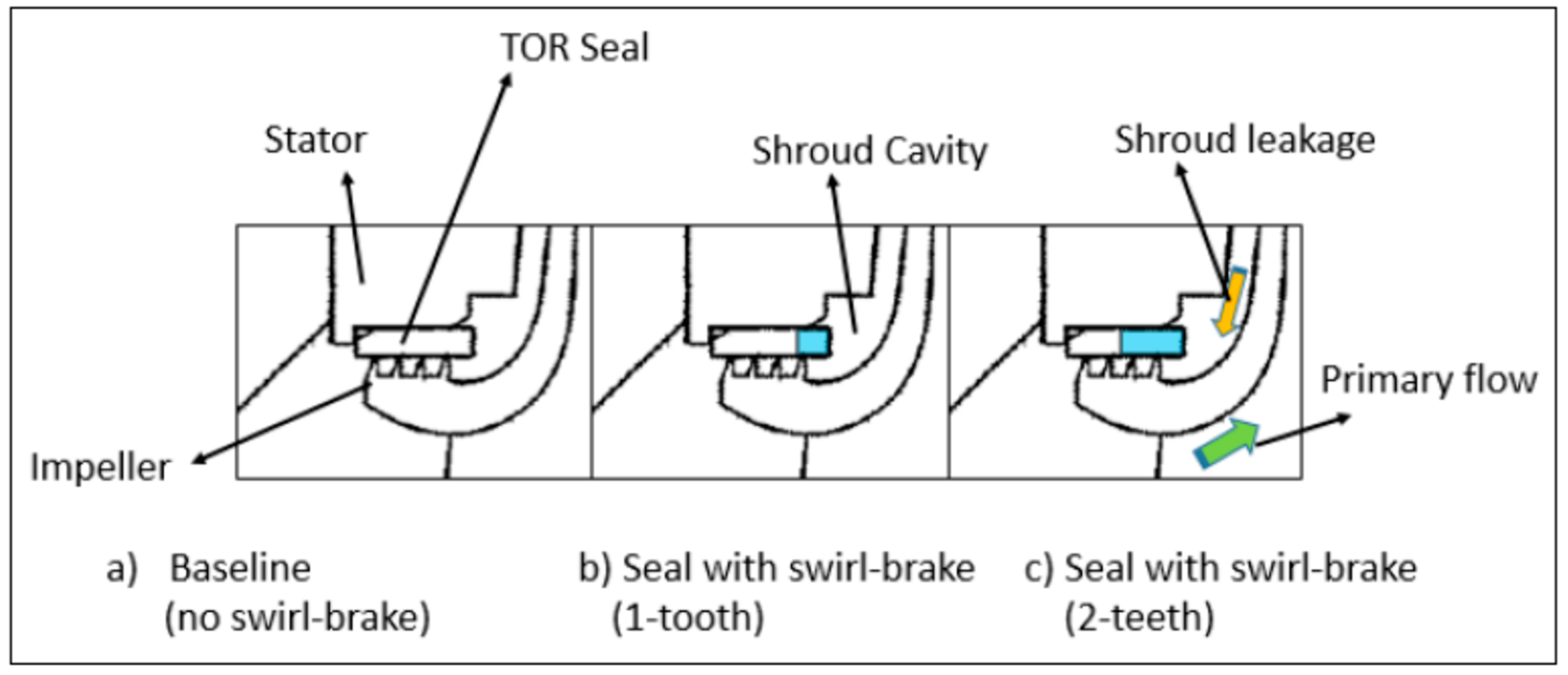
Based on a review of the published experimental data to 2013, Childs [
19] noted that shrouded turbines are more prone to subsynchronous whirl destabilizing forces than those without shrouds. That is, the magnitude of the aerodynamic destabilizing force can be two or more times as large as without blade shrouds. Apparently, stage seals are a destabilizing agent. Childs recommended the implementation of upstream swirl brakes (SBs) to reduce the inlet swirl velocity into stage seals; see Venkataraman et al. [
20] for an application in an unstable compressor that became stable after inserting Sbs upstream of the neck-ring stage labyrinth seals.
Most measurements conducted with axial compressors show aerodynamic forces inducing backward whirl over most of the operating range in axial compressors; forward whirl only occurs at high flow rates. In contrast, early numerical models (1990s), such as those from Alford [
5] and Colding-Jorgensen [
26], gave conflicting results, suggesting only forward whirl. However, more advanced models, in particular that of Myllerup and Keith [
36] stressed that there are other contributions to the aerodynamic force. In particular, the non-axisymmetric pressure distribution on the blades and the unsteady momentum storage within blade passages must be accounted for, to accurately predict the magnitude of the force and its direction of whirl. A compressor may display forward or backward whirl, depending on the operating conditions and compressor geometry. Importantly, in gas turbines for aircraft or power generation, the effect of the aerodynamic force from the turbine is more important than that of the compressor, due to the vast differences in power (torque).
As Spakovszky emphasized recently [
3] (2021), aerodynamic forces can induce either forward or backward whirl, depending on the operating conditions of the compressor or turbine, namely the flow rate and whirl frequency, as well as the stage geometry. One must also stress that, to date (2022), rotordynamic engineers in the O&G industry have indiscriminately applied Alford’s Eq., rather than results from more advanced analytical models [
30,
31,
36] and those based on computational fluid mechanics [
37,
38] published since 2018. These latter models predict both radial and tangential forces, i.e., direct and cross-coupled stiffnesses (
K,
k), and provide comparisons with the experimental data of Ulrichs [
22] and the blade geometry given in Ref. [
39]. These recent papers produce more complex models that account for rotor eccentricity and blade tip clearance.
3.2. Destabilizing Forces in (Shrouded and Unshrouded) Centrifugal Compressors
As demonstrated by Ehrich [
6] in 1993, Alford’s argument for the direction (forward whirl) of the aerodynamic force in compressors is incorrect. Nonetheless, Childs [
11,
19] stresses that shop and field operational experience with centrifugal compressors attests to severe rotordynamic instabilities, always inducing forward rotor whirl. In particular, units with shrouds are more prone to subsynchronous rotor whirl than those without shrouds. The magnitude of the destabilizing force can be two or more times larger [
40] than the force induced by an open impeller, i.e., one without a shroud. The source and magnitude of the destabilizing force has promoted much discussion and research.
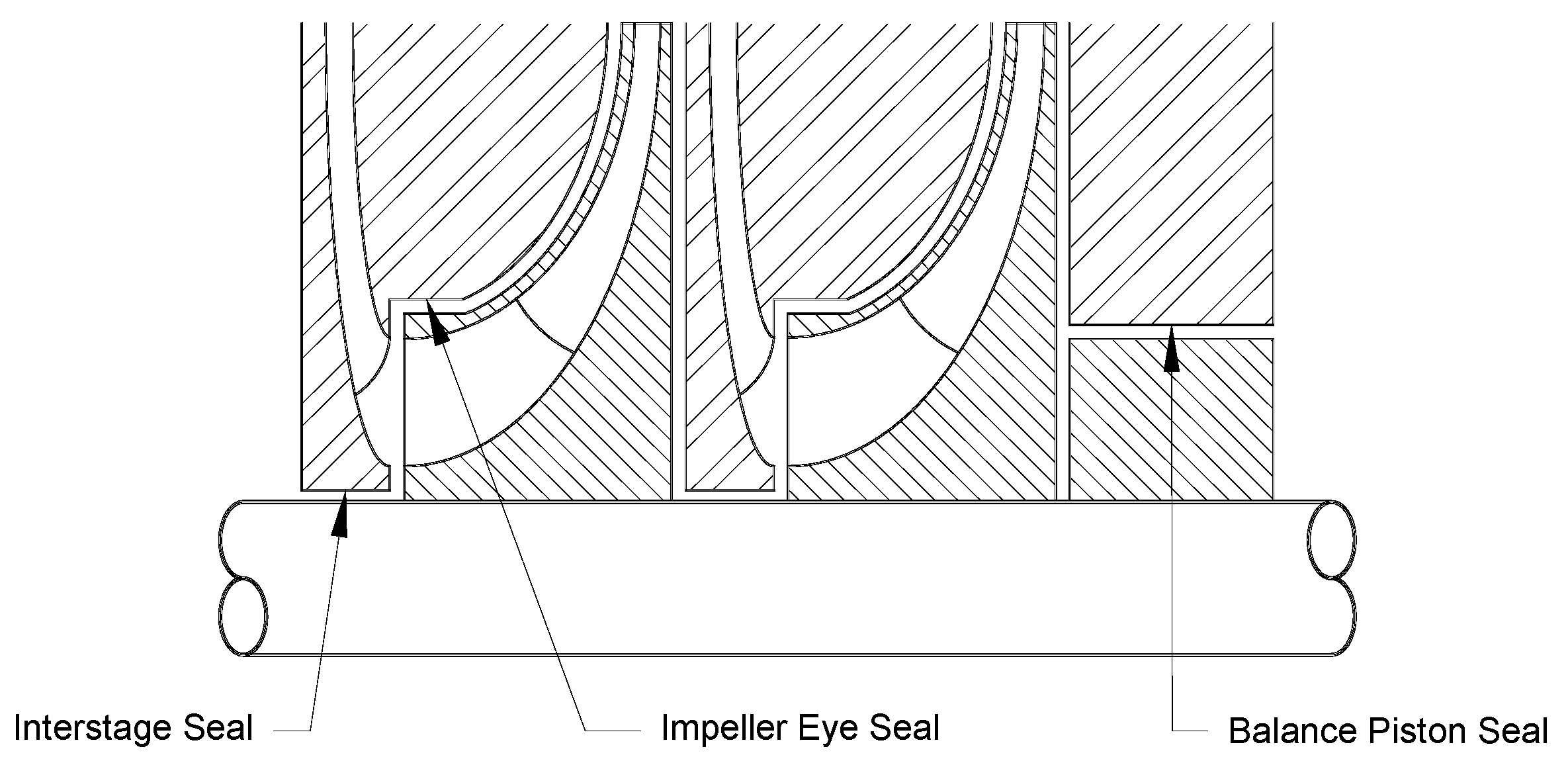
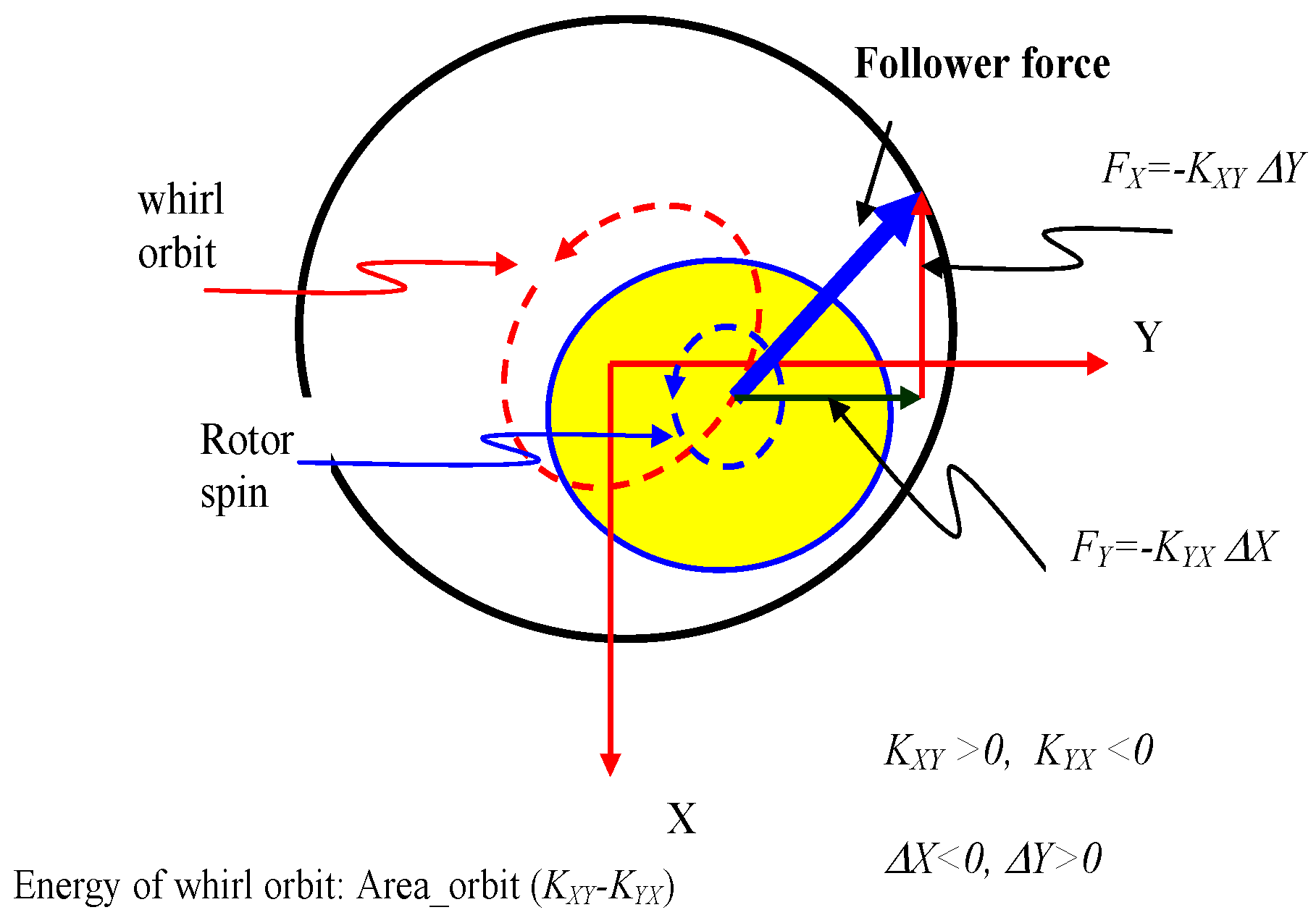
With reference to
Figure 1, as the rotor vibrates, the impeller moves and open/closes the secondary flow paths along the front shroud and back face of the impeller. The same fluid streams also flow through the impeller eye (or neck-ring) seal and through the inter stage shaft seal. The secondary flow, displacing radially inward through a shrouded gap, accelerates circumferentially and enters the neck ring seals with a large swirl, whose mean circumferential velocity exceeds 50% of the rotor surface speed. The large swirl generates large cross-coupled forces (
k > 0) that can induce a rotordynamic instability. This force is generally known as the rotor–impeller shroud interaction force, to distinguish it from the tip clearance excitation forces that are typical in axial compressors and turbines.
Childs [
19] notes that impeller–rotor interaction forces increase sharply (
k >> 0) with the decreasing clearance between the impeller shroud and the pump housing. Ehrich [
21] also argues that enlarged clearances in the exit (eye) seal due to rubbing, wear, or damage increase the (mean) circumferential flow velocity through the shroud, entering into the seal and thus increasing the cross-coupled forces.
Experimental data are scarce! Moreover, there has been no definitive analysis on impeller–rotor interaction forces applied to centrifugal pumps and compressor stages. For liquid pumps, predictive analysis relies on a handful of experimental results conducted at Cal Tech [
41] (1982) and Sulzer [
42] (1987), see inset graph taken from Childs’ textbook (
Figure 7) [
11].
To date, the most important question has been to pinpoint the exact source of the dynamic reaction forces (or moments). The impeller–rotor interaction force combines the contributions of dynamic forces arising from the front and back faces of the impeller, as well as those arising from the neck-ring (eye) seal and inter-stage seals.
Impeller lateral forces are typically modeled as (Childs [
11]):
This reduced model force representation assumes rotational symmetry and circular centered whirl motions. Experimental results are typically presented in dimensionless form as
with Ω as the rotor speed and
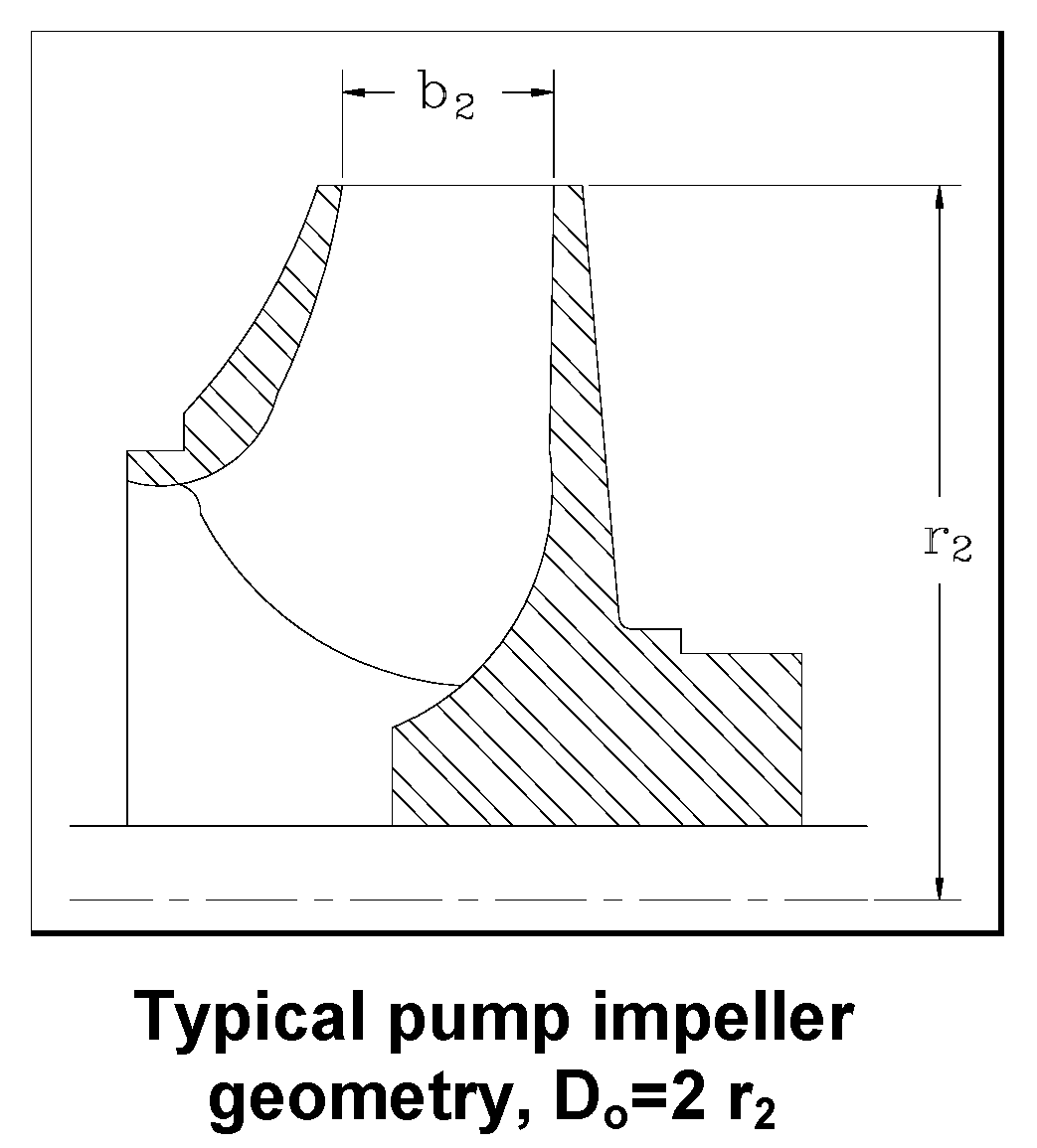
mref as a reference fluid mass (density x volume of solid disk with tip diameter
Do and thickness
b2). For
m~0, the whirl frequency ratio,
WFR = k/(
CΩ), relates the whirl frequency of unstable motions to shaft speed. A
WFR = 0.5 means that the compressor cannot stably operate at a shaft speed above two times (=1/
WFR) the system first natural frequency.
Figure 8 displays the four pump impellers tested at Sulzer [
42], and
Table 1 lists the dimensionless force coefficients obtained from the experiments. These tests are of particular importance, since the clearance between the impeller shroud and casing was small,
Cr = 0.067 mm to 0.081 mm, representative of actual applications.
From the experimental results, see
Table 1, the following conclusions can be derived:
Impellers have negative direct stiffness and large direct and cross-coupled inertia coefficients.
The cross coupled stiffnesses are much larger for tight clearance impellers, thus generating large destabilizing forces at rotor speeds below the rotor-bearing system critical speed, i.e., WFRs > 1.
The shroud generates most of the destabilizing forces in a pump impeller.
Enlarged clearances in neck ring seals aggravate the generation of cross-coupled forces, since the swirl flow into the seal increases. Enlarged clearances are the result of transient rubbing events in the operation of a pump.
At reduced flow rates (<QBEP), the impeller cross-coupled stiffnesses are smaller.
Impeller–volute interaction forces are small and benign.
In a radial flow impeller, the cross-coupled stiffnesses are small, i.e., the destabilizing force is negligible, since the projected axial area of the shroud is quite small.
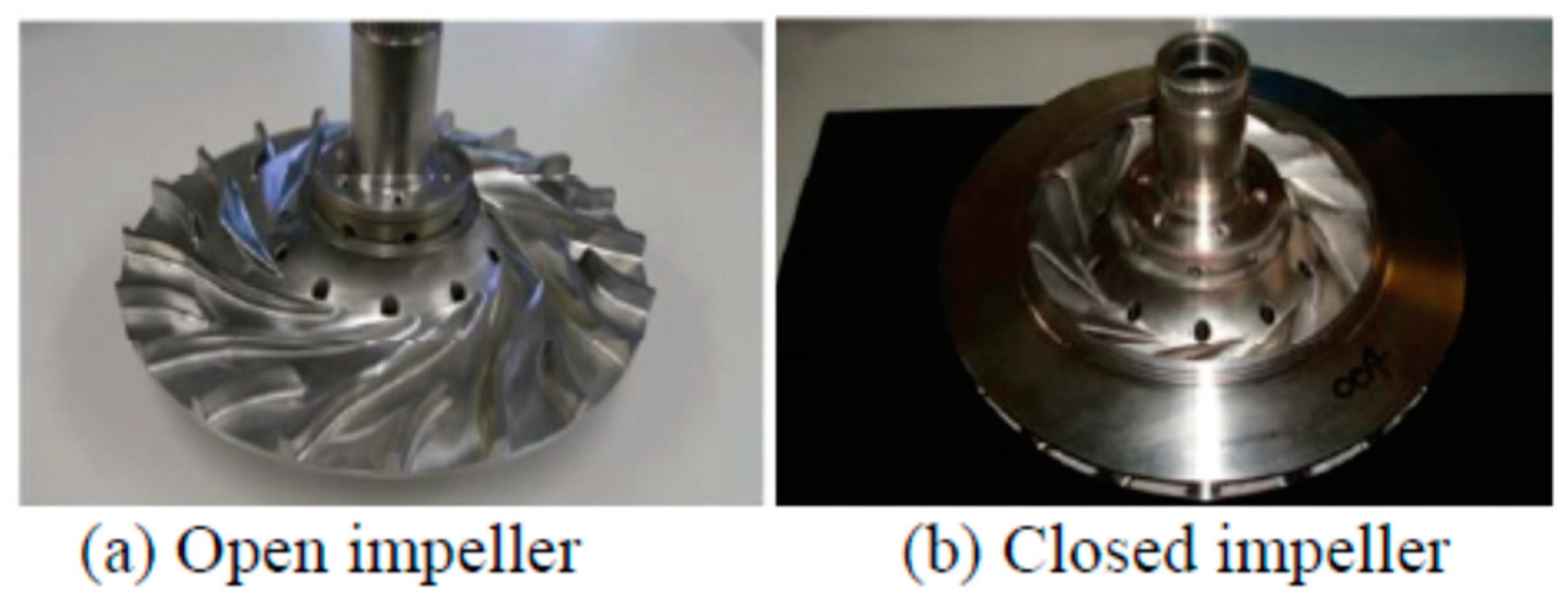
In 2012, Uchiami et al. [
43] presented the results of an extensive R&D program testing open and closed impellers for a cryogenic fluid turbopump, see
Figure 9 The test fluid was water, and the excitation frequency reached up to 1.5 times the shaft speed (max 1200 rpm [30 Hz]). The measured forces (radial and tangential) for the impeller were small compared to those of the closed impeller. The open impeller produced a positive centering stiffness (
K > 0) and added mass (
M > 0) coefficients, while the closed impeller showed
K < 0 and a lower
M < 0. As per the damping coefficients, the open impeller had direct and cross-coupled damping coefficients
C,
c > 0, whereas the closed face impeller produced
c >
C > 0. Both impeller types produced a cross-stiffness of similar magnitude; hence, the open impeller offered a larger speed stability range than the one with a shroud.
Recently (2021), Jolly et al. [
44] compared the force coefficients obtained from a pump impeller with an open face (unshrouded) with another with a closed end (front shroud). Both units had a vaned diffuser. The intended application uses a cryogenic liquid, whereas the tests were conducted with water and at a fraction of the rotor speed and pressure rise that a typical aerospace turbopump would see. The closed impeller configuration had an eye packing seal with one of three configurations: a smooth surface annular seal, a labyrinth seal, and a face seal. The front casing of the shrouded impeller was either a smooth surface or a textured one. Rotor speed reached 5 krpm, and water was supplied at 6 bar. The experimental results showed that the open impeller produced force coefficients with a lesser magnitude than the closed faces impellers; in particular, the direct stiffness for the closed impeller with annular seal was positive, whilst that for the open impeller was negative. The direct damping coefficients were insensitive to rotor speed with the closed impeller, having an annular seal producing the largest magnitude; typically twice as large as the coefficient for the open impeller. Cross-coupled stiffnesses and damping coefficients were similar in magnitude for all configurations, open and closed, and decreased as the rotor speed increased. The rather short paper does not give details on stability, albeit one can infer a
WFR = (
KC/Ω
CD) > 1 for low shaft speeds (<2 krpm), and
WFR→0.5 at the highest speed (5 krpm) for the closed impeller, whilst for the open impeller
WFR > 4 at low speed and WFR→1 at the highest speed.
Yoshida et al. [
45] (1999) presented the only rotordynamic force measurements conducted with an unshrouded 12 blade centrifugal compressor, albeit operating with water to amplify the magnitude of the aerodynamic forces. The impeller operated inside a vaneless diffuser and with an axisymmetric discharge collector to minimize the interactions with stationary parts. In the experiments, conducted for increasing whirl ratios (ω/Ω) with orbit radius at 60% of the clearance and increasing flow rates, aerodynamic forces were recorded using four-axis load sensors and also by integrating casing pressures. The tests showed destabilizing forces at a small positive whirl speed ratio (0 <
ω/Ω < 0.3) throughout the flow range of normal operation. At small flow rates (Φ~0.285) with inlet backflow, the magnitude of the fluid force changed suddenly at a whirl speed ratio
ω/Ω~0.8, resulting in even larger destabilizing rotordynamic forces. The test data showed that the radial and tangential forces did not fit the (linearized) rotordynamics force model in Equation (10).
In the cited reference [
45], the forces estimated from the pressure difference across the impeller blades did not agree with the direct measurements of force, and hence the authors noted that the destabilizing force was not just due to tip-clearance variations that changed the blade loading, but also due to a nonuniform pressure distribution acting on the blades. The analyses of Al-Nahwi et al. [
34] and Myllerup and Keith [
36] fully endorsed the rationale derived from the experimental data.
Table 2 lists the dimensionless test force coefficients obtained by Yoshida et al. for the compressor operating at its design flow rate. A comparison with the pump–impeller test data, see
Table 1 in particular the row labeled as CT-volute, shows that the compressor developed similar levels of cross-coupled stiffness
(=1.10
pump) and direct damping
(=3.14
pump) and a nearly identical whirl frequency ratio
(WFR~0.35
pump). The pump, on the other hand, evidenced large cross-coupled damping
~7.91
pump and cross-coupled inertia
~−0.58
pump. The most important difference relates to the compressor developing a positive direct stiffness
= 1.25, while = −2.5
pump.
To date, there have been no other comprehensive experimental programs conducted with centrifugal compressors, shrouded or unshrouded, and aiming to measure rotordynamic force coefficients and determine their stability characteristics.
3.3. Wachel’s Equation and Its Effect on Compressor Stability
Returning to the basic query of this section, there has been much experience with closed face (shrouded) centrifugal compressors (and turbo expanders) becoming rotordynamically unstable, always with forward whirl. Since the mid 1980s’, the Proceedings of the Turbomachinery Symposium have hosted many lectures and case studies showcasing these often costly instabilities, along with remedies to fix or resolve the issue. An exhaustive list of the mentioned papers is outside the scope of the current review.
Developed for shrouded compressors, Wachel’s Eq. (1981) below is routinely used for prediction of a destabilizing cross-coupled stiffness coefficient (
k > 0) [
46,
47].
where
To is the torque = (compressor power/shaft angular speed),
D is the impeller tip diameter, and
hW is the diffuser width. Above
MW is the gas molecular weight, and (
ρout/
ρin) is the ratio of gas densities discharged over the inlet. The parameter
β ranges from 2 to 5 for most high performance compressors. Note that Wachel’s equation, derived from correlation studies of field experiences with unstable compressors, is in actuality a modified form of Alford’s force model, as explained by Evans and Fulton [
7]. The ratio (
MW/10) appears arbitrary, and API [
48] sets
MW = 30 for hydrocarbon gas processing compressors. The API formula is known as the PACC #, i.e., the predicted aerodynamic cross-coupling number.
Routine rotordynamic analyses for shrouded wheels of centrifugal compressors implement Equation (12), with additional correction factors known to the manufacturer and based on experience. For example Dresser-Rand [
49] (currently Siemens Energy) multiply Equation (12) × 0.80 and call it the Modified PACC number (MPACC).
In 2005, Nicholas and Kocur [
50] and Li et al. [
51] argued there is a long-standing confusion regarding the application of Wachel’s formula to predict destabilizing forces and their ultimate impact on the stability of (shrouded) centrifugal compressors. Gas densities in Wachel’s formula, Equation (12), can relate to either the inlet and exit conditions in a single stage or the entire compressor train, for example. Furthermore, when assuming a single Wachel’s force to represent the entire machine, an open question is its actual (physical) location in the compressor train. The references try to make sense of API 617 requirements, albeit applying ad hoc procedures that integrate indiscriminately both labyrinth seal forces and Wachel’s formula.
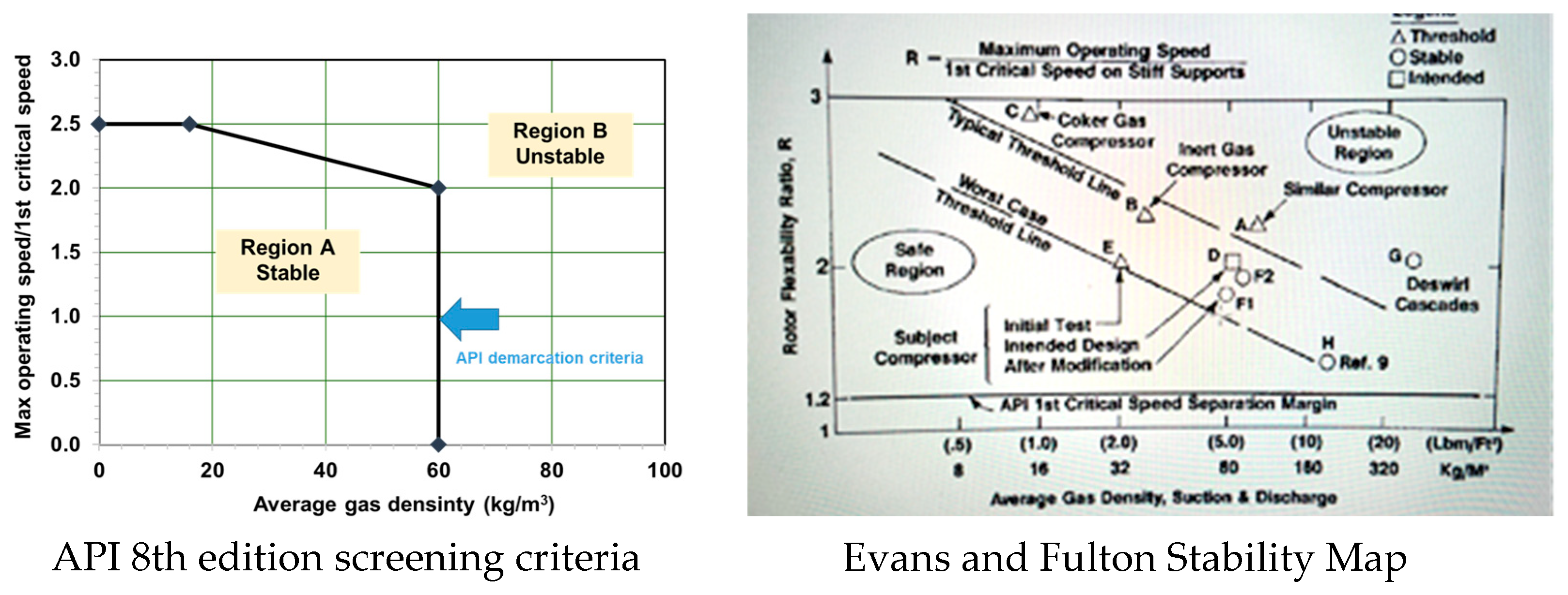
In 2010, Evans and Fulton [
7] provided a more useful insight into the origin of Wachel’s formula and fully explained its various forms, as applied to a rotordynamic analysis. The authors also elaborated the (so-called) Fulton–Sood stability map shown in
Figure 10. The map contains an informative collection of stable and unstable compressors defined by the ratio of operating speed/critical speed (>2.5) vs. average gas density (from suction and discharge). The authors stressed that Wachel’s formula estimates the force from the shroud cavity (front and back) and includes the seals, eye, and inter-stage. That is, Wachel’s equation applies to a whole stage and not to its various flow paths and elements.
In practice, engineering process calls for varying the cross-coupled stiffness (
k), to predict the margin of system modal damping and the threshold speed of instability in consideration of API 617 [
48] specifications, as per level I and level II analyses. This is a sensitivity analysis that focuses on quantifying the stability, or lack thereof, of the first forward damped mode with a frequency greater than the first undamped critical speed on rigid supports. The API norm makes a distinction between rotors fully supported on two bearings, overhung rotors, and double overhung rotors.
A level I analysis uses Wachel’s equation to approximate the destabilizing effect of the labyrinth seals and (any other) aerodynamic excitation (namely the impeller secondary flow paths) from each impeller and adds to a total anticipated . The overall anticipated cross-stiffness (Q) is varied to a maximum of 10 QA or until the log-dec δA = 0 obtained for an overall cross-coupled coefficient with magnitude Qo.
If the compressor fails the Level I specification, then a more sophisticated Level II analysis is required. Failing level I means predicting either
δA < 0.1, or
Qo/QA < 2, or
Qo/QA < 10, and the location in region B of the point is defined by the intersection of the critical speed ratio CSR (operating speed/undamped natural frequency) and the average density at the normal operating condition, see
Figure 9. The Level II analysis accounts for forces from all stages, including their labyrinth seals, damper seals, impeller/blade flow aerodynamic effects, and internal friction.
Published in 2022, the API 617 9th edition [
52] offers more insight (and details) into the screening criteria for level I analysis, with force coefficients for labyrinth seals calculated at the minimum and maximum seals’ operating clearances and using the operating clearance for damper seals, including any mechanical deformation. For double overhung machines, the norm explicitly calls for a stability quantification (log-dec estimation) of the first two forward modes.
Gupta and Childs [
8] emphatically note that Wachel’s formula, though based on certain empirical evidence, is neither supported by sound physical arguments, nor by analysis. The analysis of impeller–diffuser interaction forces should include the flows and dynamic pressures generated on the curved path in front of the shroud and on the back face of the impeller. Most importantly, the analysis must account for the sealing elements (labyrinth typically): neck ring or eye seal and inter stage seal, where large pressure gradients occur and are greatly influenced by the inlet fluid rotation or swirl condition. In Gupta and Childs analysis, the eye seal and the inter-stage seals account for 80% and 18% of the cross-coupled stiffness (
k), while the front shroud contributes just 2.2%. The effect of the back shroud is negligible, even for radial inflow impellers.
Since the early 2000s, Moore and co-workers at Southwest Research Institute have conducted CFD studies of centrifugal compressors and radial turbines (unshrouded single stage), to predict the aerodynamic destabilizing forces; see Refs. [
53,
54]. Moore and Ransom [
55,
56] have presented an alternative to Wachel’s equation, namely
where the dynamic head
at the exit plane of a compressor replaces the drive torque,
U is the wheel tip speed,
Lshroud is the axial length of the shroud, and (
Q/Qdesign) is the flow relative to the design condition.
Cmr, a constant for a given impeller design, ranges from 4.0 to 7.5 (when using SI units). If Equation (13) is to be applied to a turbine or a turbo expander, take the inlet plane as the reference for dynamic head.
In Lerche et al. [
53], for the particularly unstable turbo expander analyzed, the cross coupled stiffness (
k) predicted by various models differed significantly. The unit processed a refrigerant gas whose large
MW = 104 played a significant role in amplifying the unstable force. Using Alford’s Equation (9) with
β = 1.5, then
kAlford = 6355 N/m; whereas Wachel’s Equation (12) produces
kWachel = 11.13
kAlford, and using API’s formula with
MW = 30 predicts
kAPI = 3.27
kAlford. Similarly, using Moore and Ransom’s Equation (13) gives
kMoore-Ransom = 1.71
kAlford, while the prediction from an unsteady CFD study of the forces produced by the expander delivers
kCFD = 2.32
kAlford.
CFD analyses continue to evolve as computational resources are more readily available, along with faster speeds of execution. High fidelity 3D CFD models, constructed with many millions of nodes or cells, include both the main flow path and the secondary (leakage) flow paths in the front and back shrouds and through the seals. Transient or periodic shaft motions, along with deformable meshes predict the unsteady flow and pressure fields acting on the impeller surfaces to produce forces, from which the force coefficients are extracted.
These examples of CFD analysis have made palpable the importance of the shroud cavity in generating a significant fraction of the destabilizing force. Zhang et al. [
57] reported force coefficients in the front shroud cavity and eye seal of a stage in a centrifugal compressor. At one operating design condition,
kCFD = (
kLS +
kshroud) = (40% + 60%), i.e., the shroud cavity produced 50% more cross-stiffness than the eye seal. Predictions showed that the total
k (>
K) grew with the power two of the shaft speed. Later, Hoopes et al. [
58] reported, at one operating condition,
kCFD = 2.99 MN/m = (
kLS +
kshroud) = (34 % + 66%) = 1.53
kWachel = 2.35
kMoore-Ransom. The authors noted the generation of a non-uniform circumferential pressure field regulated by the LS varying clearance as the impeller whirled.
In 2021, Kim and Venkataraman [
59] modeled a multiple-stage compressor that was rotor-dynamically unstable in the field, and where installation of swirl brakes (SB) upstream of the eye seals fixed the issue, see Ref. [
20]. For the baseline condition, before SB installation, at the 2nd stage of the compressor,
kWachel = 439 kN/m,
kAPI = 1.07
kWachel,
kMoore-Ransom = 0.935
kWatchel, and
kCFD = 1.245
kWatchel. Similarly, for the 5th stage,
kWachel = 424 kN/m, and
kAPI = 1.07
kWachel,
kMoore-Ransom = 1.127
kWatchel, whereas
kCFD = 1.917
kWatchel. The CFD cross-coupled stiffnesses (
k’s) were larger, since they accounted for the asymmetric pressure field in the shroud that also exerted a force on the impeller. Note again the wide variation in predictions depending on the model used. The same reference produced a table (see below) that separated the individual contributions of the eye seal and shroud to the force coefficients,
k and
C. The tabulated results made evident the benefits of the SB upstream of the LS. Note that, for the baseline condition (one without SBs), the eye seal contributed 57% of the total
k. On the other hand, for the stage with the installed SB, the total
k magnitude was much lower (~38% of baseline), with the shroud cavity contributing 134% of the total
k; i.e.,
kLS < 0. With the SB, the eye seal produced a
k < 0.
In the work of Kim and Venkataraman, for the baseline case (no SB), the eye seal and the shroud contributed 57% and 43%, respectively, to the destabilizing coefficient
k. This distribution was markedly different to that in Gupta and Childs [
8], who reported that the front (and back) shroud cavity contributed little to the total force.
Table 3 from Ref. [
59] includes the direct damping coefficient and the whirl frequency ratio
WFR =
k/(Ω
C) for the whole stage and its two components, the LS, and the shroud. In short, the eye seal contributes most to the damping (an energy dissipative action), rather than the shroud.
Not that adding SBs upstream of the LS reduced the number of knives in the seal, hence incurring a penalty in leakage (about 10%) and a loss in stage efficiency. Rather than relying on a swirl brakes to decrease the impeller forces by reducing the inlet swirl, another way to fix an instability issue is by injecting gas in the direction opposite to shaft rotation. Anti-swirl injectors or shunt injection or swirl-cancellers draw gas from the discharge of the impeller, and after mechanical modifications in the compressor casing, route the additional gas upstream of the eye seal. For a recent case study, see the work of Osada et al. [
60].
In 2022, Wu and Kuzdal [
61] presented full-load and full-pressure testing of a 15.3 MW six-stage compressor with three open impellers in the first section and three closed impellers in the second section. The rotordynamic tests conducted with shaft speeds to 17.8 krpm demonstrated that the compressor was stable with a log dec
δ = 0.3 without load and
δ = 0.46 at full load (15.3 MW). The predictions, on the other hand, showed
δ as decreasing as the load increased. At 10,000 HP, both the predictions and experimental estimation of the log-dec coincided. The authors gave little details on the wheels’ dimensions (diffuser gaps) or the gas properties or the inlet/outlet pressure conditions for each stage. The paper listed predictions of the anticipated cross-coupled stiffness (
QA) based on Alford’s Eq., Wachel’s Eq., API 617 formula, their own MPACC, and a
Q derived (somehow) from a CFD study of one open stage.
Table 4 summarizes the results, which reveal the MPACC number and CFD based predictions produced the best correlation with the measured log-dec for full power conditions. The paper also calls to attention the lack of experimental results related to unshrouded stages.
To date there has only been one published experimental evaluation of the static force, with radial and tangential components, in a shrouded impeller of a compressor. Years in the making, Song and Song [
9] diligently worked on fully instrumenting the shroud cavity and eye (labyrinth) seal with multiple sensors, along the secondary flow path and around the circumference of the impeller casing, to measure the pressure field as the casing was displaced eccentrically. The measured forces were proportional to the static displacement, which reached 44% of the clearance in the labyrinth seal. The radial force, about an order of magnitude smaller than the tangential force, produced a negative direct stiffness (
K < 0). Most importantly, and contrary to common assumptions and many model predictions, the shroud cavity produced a cross-coupled stiffness that was 2 ½ × the
k for the labyrinth seal. That is, the shroud cavity contributed 71% of the total
k = (
kshroud +
kLS).
In a companion paper, Song and Song [
10] detailed a comprehensive analysis for predicting the non-axisymmetric flow fields induced by an off-center shroud in a shrouded centrifugal compressor stage. The model is divided into eight sections, the main and secondary flow paths, and particularly considers the boundary conditions at the respective interfaces. The model for the flow in the shroud cavity is similar to that of Gupta and Childs [
8] for the circumferentially non-uniform upstream (blades discharge) and downstream (labyrinth eye seals) conditions. Predictions from the model agreed well with the experimentally derived force coefficients, while verifying the pressure variations in the shroud cavity contributed most to the generation of the destabilizing force. Note that, in the model, the shroud cavity contributed 67% to the total
kmodel~1.14
kexp.
Shujan, Mortazavi, and Palazzolo [
62] reported a complex CFD model reproducing the impeller stage and shroud in Ref. [
9] and calculated steady and transient flow fields through the primary and secondary paths. CFD predictions best replicated the experimental results and performed better than the model results in Ref. [
10]. In the work of Mortazavi and Palazzolo,
kCFD~kexp for the shroud contribution. In addition, the authors noted
kAlford~0.25
kexp (shroud),
kWachel~0.59
kexp, and
kMoore-Ransom~0.28
kexp. Clearly, the conventional methods for predicting the cross-coupled (destabilizing) coefficients produce very different results from experimental results or a prediction from a full CFD model.
Other than pursuing a complex CFD approach, an engineering path to deliver accurate predictions of force predictions in a shrouded impeller requires a rational account for the multiple component interactions along the main and secondary (leakage) flow paths, see Ref. [
9]. Nonetheless, system-level CFD models are becoming common, as CFD of the primary flow path is already a part of engineering practice. The addition of the unsteady secondary flow paths through the front and back shroud cavities and end seals will become an integral part of the design of modern centrifugal compressors handling more dense fluids and operating at ever-increasing shaft speeds, well above the first and even a second critical speed. Units with larger pressure ratios are more prone to rotordynamic stability issues, as the destabilizing force (
k >> 0) is load dependent.
Difficulties in the estimation of impeller–rotor interaction forces extend to radial turbines and turbo expanders. Refs. [
63,
64] describe the costly rotordynamic instability issues found in a turbo expander supported on magnetic bearings. The cross-coupled stiffnesses based on Wachel’s equation had to be multiplied by 10, to produce a force large enough to induce the instability at the operating rotor speed. The radial inflow shrouded turbine had labyrinth seals in the front and back surface seals. Gas condensation played a significant role in the rotor instability. Similar behavior is expected for sCO
2 turbomachinery, with the added caveat of likely fluid condensation in turbines handing the supercritical fluid.