Methodological Approach in the Simulation of the Robustness Boundaries of Tribosystems under the Conditions of Boundary Lubrication
Abstract
1. Introduction
- Stable tribosystems, which, after being brought out of equilibrium by any external disturbance (change in load or sliding speed, or the short-term cessation of lubricant supply), after the removal of this disturbance, return to the original stable state, i.e., the established operating mode.
- Neutral tribosystems, which, after removing the disturbance, switch to a state of stable operation in a new mode, which is different from the original one.
- Unstable tribosystems, which, as they are brought out of equilibrium by any external disturbance, do not return to the original stable state after the removal of this disturbance, but switch to the mode of accelerated wear or to burr, i.e., cease operation.
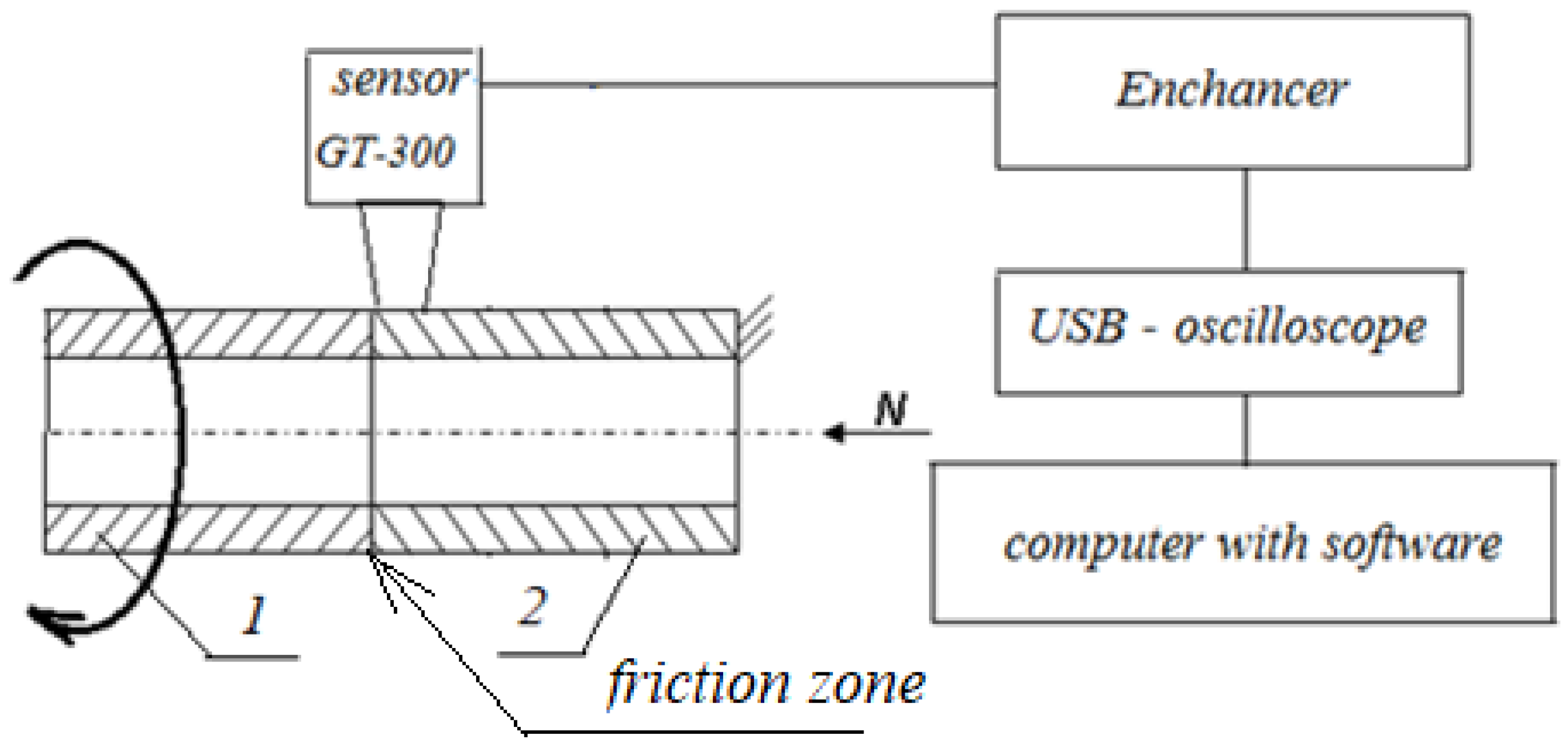
2. Materials and Methods
3. The Results of the Experimental Research
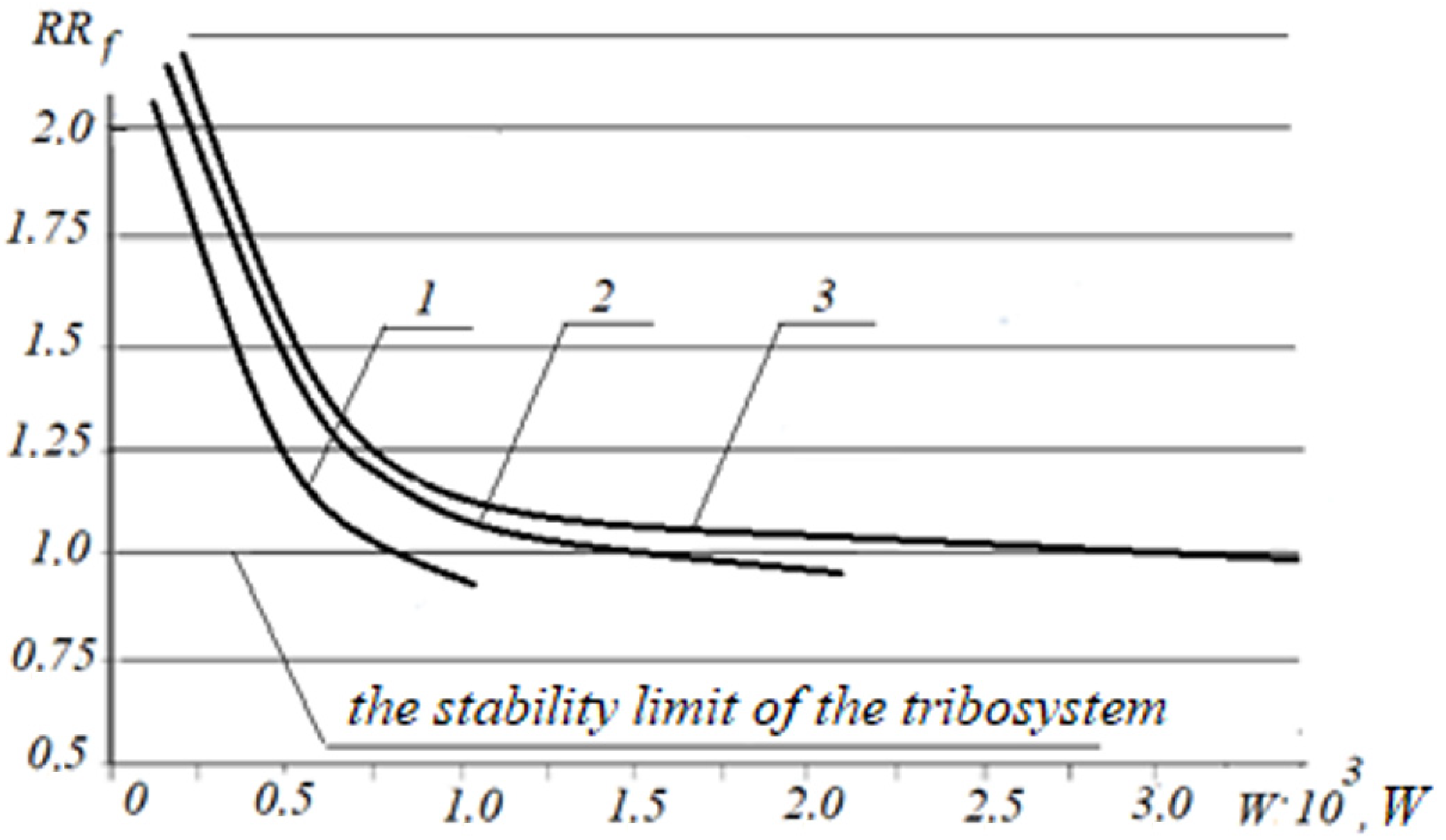
- Tribosystem №1: «steel 5140 + steel 5140», (RSTS(max) = 326.7 m−1); Kf = 6.25 m−1; lubricating medium Ey = 2.43·1014 J/m3, (HH, ISO-L-HL). The value of the Q–factor of the tribosystem Q0 = 1.12·1010 J/m3.
- Tribosystem №2: «steel 5140 + bronze C61900», (RSTS(max) = 436.0 m−1); Kf = 12.5 m−1; lubricating medium Ey = 3.6·1014 J/m3, (SAE 40, API CC). The value of the Q–factor of the tribosystem Q0 = 5.5·1010 J/m3.
- Tribosystem №3: «steel 5140 + brass CW723R», (RSTS(max) = 460.9 m−1); Kf = 14.5 m−1; lubricating medium Ey = 4.18·1014 J/m3, (SAE 120, API GL-4). The value of the Q–factor of the tribosystem Q0 = 7.69·1010 J/m3.
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| T1, T2, T3 | time constants, dimension s. |
| K1, K2, K3 | amplification factors, dimensionless values. |
| trun | tribosystem run-in time, dimension s. |
| Vrun | the given volume of materials of the moving and stationary triboelements of the tribosystem, dimension m3 |
| arun | given coefficient of thermal conductivity of materials of movable ap and fixed an triboelements, dimension m2/s. |
| dacs | diameter of the actual spot of contact, dimension m. |
| nacs | the number of contact spots on the friction surface |
| Vdef | given volume of deformable surface layers, dimension m3. |
| the magnitude of the rate of deformation of the surface layers of the materials of the movable and fixed triboelements, dimension s−1. | |
| Q0 and Qmax | the initial Q-factor value of the tribosystem and the Q-factor value after run-in, dimension J/m3. |
| WTR | speed of dissipation in the tribosystem, dimension J/s. |
| Kf | tribosystem form factor, dimension m−1. |
| RSTC(max) | the maximum value of the rheological properties of the combined materials in the tribosystem after the completion of running-in, dimension m−1. |
| N | load on the tribosystem, dimension N. |
| vsl | sliding speed, dimension m/s. |
| tl | load change time, dimension s. |
| kd(f) | coefficient of change of load speed according to the friction coefficient parameter, dimensionless value. |
| kd(I) | coefficient of change of the load speed according to the parameter of the wear rate, dimensionless value. |
| RRf | robustness of the tribosystem according to the friction coefficient, dimensionless value. |
| RRI | robustness of the tribosystem according to the rate of wear, dimensionless value. |
| Wb(i), Wb(aw) | the value of the magnitude of the external influence on the tribosystem at which occurs the loss of stability (burr or accelerated wear), according to the results of the experiment and according to the results of simulation, dimension J/s. |
| Ey | tribological properties of the lubricating medium, dimension J/m3. |
| SWb | root mean square deviation of values of external influence during experimental studies, dimension J/s. |
| vWb | coefficient of variation of measurements of external influence, at which the event of loss of stability of the tribosystem occurs, %. |
| eWb | relative error of modeling the robustness of tribosystems, %. |
| Ra | initial roughness of friction surfaces, dimension µm. |
References
- Voitov, A. Structural identification of the mathematical model of the functioning of tribosystems under conditions of boundary lubrication. Probl. Tribol. 2021, 26, 26–33. Available online: http://tribology.khnu.km.ua/index.php/ProbTrib/article/view/794/1269 (accessed on 8 November 2022). [CrossRef]
- Kondratiev, A.V.; Gaidachuk, V.E. Mathematical analysis of technological parameters for producing superfine prepregs by flattening carbon fibers. Mech. Compos. Mater. 2021, 57, 91–100. [Google Scholar] [CrossRef]
- Li, H.; Liu, Y.; Liao, H.; Liang, Z. Accelerated Wear Test Design Based on Dissipation Wear Model Entropy Analysis under Mixed Lubrication. Lubricants 2022, 10, 71. [Google Scholar] [CrossRef]
- Ye, L.; Hu, Y.; Deng, S.; Zhang, W.; Cui, Y.; Xu, J. A Novel Model for Evaluating the Operation Performance Status of Rolling Bearings Based on Hierarchical Maximum Entropy Bayesian Method. Lubricants 2022, 10, 97. [Google Scholar] [CrossRef]
- Al-Quraan, T.M.A.; Mikosyanchik, O.O.; Mnatsakanov, R.G. Temperature resistance of the boundary lubrication layers under rolling with slippage condition. Int. J. Mech. Eng. Appl. 2017, 5, 78–86. [Google Scholar] [CrossRef][Green Version]
- Gurt, A.; Khonsari, M. The Use of Entropy in Modeling the Mechanical Degradation of Grease. Lubricants 2019, 7, 82. [Google Scholar] [CrossRef]
- Bogdanovych, O.I.; Al-Quraan, T.M.A.; Tokaruk, V.V.; Haddad, J.S. Algorithm for tribokinetic modeling tests of triboconjuc-tion materials for industrial products. Tribol. Ind. 2020, 43, 159–166. [Google Scholar] [CrossRef]
- Karl, D. Mass and Energy Balance of a Three-Body Tribosystem. Lubricants 2022, 10, 95. [Google Scholar] [CrossRef]
- Kondratiev, A.; Píštěk, V.; Smovziuk, L.; Shevtsova, M.; Fomina, A.; Kučera, P. Stress–strain behaviour of reparable composite panel with step–variable thickness. Polymers 2021, 13, 3830. [Google Scholar] [CrossRef]
- Al-Quraan, T.M.A.; Mikosyanchik, O.O.; Mnatsakanov, R.G.; Zaporozhets, O.I. Structural-Energy characteristics of tribotechnical contact in unsteady operational modes. Mod. Mech. Eng. 2016, 6, 91–97. [Google Scholar] [CrossRef][Green Version]
- Rosenkranz, A.; Marian, M.; Profito, F.J.; Aragon, N.; Shah, R. The Use of Artificial Intelligence in Tribology—A Perspective. Lubricants 2021, 9, 2. [Google Scholar] [CrossRef]
- Miroshnuk, O.O.; Tymchuk, S.O. Uniform distribution of loads in the electric system 0.38/0.22 KV using genetic algorithms. Tech. Electrodyn. 2013, 4, 67–73. Available online: http://www.scopus.com/inward/record.url?eid=2-s2.0-84885913005&partnerID=MN8TOARS (accessed on 8 November 2022).
- Argatov, I. Artificial Neural Networks (ANNs) as a Novel Modeling Technique in Tribology. Front. Mech. Eng. 2019, 5, 1074. [Google Scholar] [CrossRef]
- Joerger, A.; Reichert, S.; Wittig, C.; Aghdam, D.S.; Albers, A. An Approach for the Transfer of Real Surfaces in Finite Element Simulations. Lubricants 2021, 9, 77. [Google Scholar] [CrossRef]
- Wang, C.; Schipper, D.J. On an Elastoplastic Sliding Model for a Coated Single Asperity. Lubricants 2018, 6, 96. [Google Scholar] [CrossRef]
- Dmitriev, A.I.; Österle, W.; Wetzel, B.; Zhang, G. Mesoscale modeling of the mechanical and tribological behavior of a poly-mer matrix composite based on epoxy and 6 vol.% silica nanoparticles. Comput. Mater. Sci. 2015, 110, 204–214. [Google Scholar] [CrossRef]
- Winkler, A.; Marian, M.; Tremmel, S.; Wartzack, S. Numerical Modeling of Wear in a Thrust Roller Bearing under Mixed Elastohydrodynamic Lubrication. Lubricants 2020, 8, 58. [Google Scholar] [CrossRef]
- Terwey, J.T.; Fourati, M.A.; Pape, F.; Poll, G. Energy-Based Modelling of Adhesive Wear in the Mixed Lubrication Regime. Lubricants 2020, 8, 16. [Google Scholar] [CrossRef]
- Jacq, C.; Nelias, D.; Lormand, G.; Girodin, D. Development of a three-dimensional semi-analytical elastic-plastic contact code. J. Tribol. 2002, 124, 653–667. [Google Scholar] [CrossRef]
- Delprete, C.; Gastaldi, C.; Giorio, L. A Minimal Input Engine Friction Model for Power Loss Prediction. Lubricants 2022, 10, 94. [Google Scholar] [CrossRef]
- Wang, Z.; Yu, H.; Wang, Q. Analytical solutions for elastic fields caused by eigenstrains in two joined and perfectly bonded half-spaces and related problems. Int. J. Plast. 2016, 76, 1–28. [Google Scholar] [CrossRef]
- Orgeldinger, C.; Tremmel, S. Understanding Friction in Cam–Tappet Contacts—An Application-Oriented Time-Dependent Simulation Approach Considering Surface Asperities and Edge Effects. Lubricants 2021, 9, 106. [Google Scholar] [CrossRef]
- Yang, L.; Wang, D.; Guo, Y.; Liu, S. Tribological behaviors of quartz sand particles for hydraulic fracturing. Tribol. Int. 2016, 102, 485–496. [Google Scholar] [CrossRef]
- Scherge, M. The Running-in of Lubricated Metal-Metal Contacts—A Review on Ultra-Low Wear Systems. Lubricants 2018, 6, 54. [Google Scholar] [CrossRef]
- Vojtov, V.A.; Biekirov, A.S.; Voitov, A.V.; Tsymbal, B.M. Running-in procedures and performance tests for tribosystems. J. Frict. Wear 2019, 40, 376–383. [Google Scholar] [CrossRef]
- Kondratiev, A.; Píštěk, V.; Smovziuk, L.; Shevtsova, M.; Fomina, A.; Kučera, P.; Prokop, A. Effects of the Temperature–Time Regime of Curing of Composite Patch on Repair Process Efficiency. Polymers 2021, 13, 4342. [Google Scholar] [CrossRef]
- Wojciechowski, Ł.; Mathia, T.G. Proposal of invariant precursors for boundary lubricated scuffing. Wear 2015, 340–341, 53–62. [Google Scholar] [CrossRef]
- Wojciechowski, Ł.; Wieczorowski, M.; Mathia, T.G. Transition from the boundary lubrication to scuffing—The role of metallic surfaces morphology. Wear 2017, 392–393, 39–49. [Google Scholar] [CrossRef]
- Pusterhofer, M.; Summer, F.; Gódor, I.; Grün, F. Cumulative damage assessment of tribological durability limits. Wear 2020, 456–457, 203318. [Google Scholar] [CrossRef]
- Savolainen, M.; Lehtovaara, A. An experimental approach for investigating scuffing initiation due to overload cycles with a twin-disc test device. Tribol. Int. 2017, 109, 311–318. [Google Scholar] [CrossRef]
- Hershberger, J.; Ajayi, O.O.; Zhang, J.; Yoon, H.; Fenske, G.R. Evidence of scuffing initiation by adiabatic shear instability. Wear 2005, 258, 1471–1478. [Google Scholar] [CrossRef]
- Zhang, C.; Peng, B.; Gu, L.; Wang, T.; Wang, L. A scuffing criterion of steels based on the friction-induced adiabatic shear instability. Tribol. Int. 2020, 148, 120–134. [Google Scholar] [CrossRef]
- Wojciechowski, L.; Mathia, T.G. Focus on the concept of pressure-velocity-time (pVt) limits for boundary lubricated scuffing. Wear 2018, 402–403, 179–186. [Google Scholar] [CrossRef]
- Castro, J.; Sottomayor, A.; Seabra, J. Experimental and analystical scuffing criteria for FZG gears. Tribol. Ser. 2003, 43, 651–661. [Google Scholar] [CrossRef]
- Xue, J.-h.; Li, W.; Qin, C. The scuffing load capacity of involute spur gear systems based on dynamic loads and transient thermal elastohydrodynamic lubrication. Tribol. Int. 2014, 79, 74–83. [Google Scholar] [CrossRef]
- Im, K.; Avouac, J.-P. Linear stability analysis of the condition for vibration during frictional slip. J. Mech. Phys. Solids 2022, 167, 104993. [Google Scholar] [CrossRef]
- Sui, X.; Ding, Q. Bifurcation and stability analyses for a pad-on-disc frictional system. Int. J. Non-Linear Mech. 2018, 107, 112–125. [Google Scholar] [CrossRef]
- Onishchenko, V. Investigation of tooth wears from scuffing of heavy duty machine spur gears. Mech. Mach. Theory 2015, 83, 38–55. [Google Scholar] [CrossRef]
- Parmar, A.; Ramkumar, P.; Shankar, K. Macro geometry multi-objective optimization of planetary gearbox considering scuffing constraint. Mech. Mach. Theory 2020, 154, 104045. [Google Scholar] [CrossRef]
- Voitov, A.V. Parametric identification of the mathematical model of the functioning of tribosystems in the conditions of boundary lubrication. Probl. Tribol. 2021, 27, 6–14. [Google Scholar] [CrossRef]
- Vojtov, V.; Biekirov, A.; Voitov, A. The quality of the tribosystem as a factor of wear resistance. Int. J. Eng. Technol. 2018, 7, 25–29. [Google Scholar] [CrossRef]
- Voitov, A.; Fenenko, K.; Fenenko, O. Simulation of change in rheological properties of structure of combined materials in tribosystem. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1021, 012052. [Google Scholar] [CrossRef]
- Havrylenko, Y.; Kholodniak, Y.; Halko, S.; Vershkov, O.; Bondarenko, L.; Suprun, O.; Miroshnyk, O.; Shchur, T.; Śrutek, M.; Gackowska, M. Interpolation with Specified Error of a Point Series Belonging to a Monotone Curve. Entropy 2021, 23, 493. [Google Scholar] [CrossRef] [PubMed]
- Iegorov, O.; Iegorova, O.; Miroshnyk, O.; Savchenko, O. Improving the accuracy of determining the parameters of induction motors in transient starting modes. Energetika 2020, 66, 15–23. [Google Scholar] [CrossRef]
- Havrylenko, Y.; Kholodniak, Y.; Halko, S.; Vershkov, O.; Miroshnyk, O.; Suprun, O.; Dereza, O.; Shchur, T.; Śrutek, M. Representation of a Monotone Curve by a Contour with Regular Change in Curvature. Entropy 2021, 23, 923. [Google Scholar] [CrossRef]
- Wei, Y.; Zhao, X.; Wei, Y.; Chen, Y.Q. Lyapunov stability criteria in terms of class K functions for Riemann–Liouville nabla fractional order systems. ISA Trans. 2022, 131, 137–145. [Google Scholar] [CrossRef]
- Gokul, P.; Rakkiyappan, R. New finite-time stability for fractional-order time-varying time-delay linear systems: A Lyapunov approach. J. Frankl. Inst. 2022, 359, 7620–7631. [Google Scholar] [CrossRef]
- Mondié, S.; Egorov, A.; Gomez, M.A. Lyapunov stability tests for linear time-delay systems. Annu. Rev. Control. 2022, 54, 68–80. [Google Scholar] [CrossRef]
- Shen, C.; Li, Y.; Zhu, X.; Duan, W. Improved stability criteria for linear systems with two additive time-varying delays via a novel Lyapunov functional. J. Comput. Appl. Math. 2020, 363, 312–324. [Google Scholar] [CrossRef]
- Zhan, X.; Hu, Y. On the relation between Hurwitz stability of matrix polynomials and matrix-valued Stieltjes functions. J. Comput. Appl. Math. 2023, 417, 114614. [Google Scholar] [CrossRef]
- Zhan, X.; Dyachenko, A. On generalization of classical Hurwitz stability criteria for matrix polynomials. J. Comput. Appl. Math. 2021, 383, 113113. [Google Scholar] [CrossRef]
- El-Marhomy, A.A.; Abdel-Sattar, N.E. Stability analysis of rotor-bearing systems via Routh-Hurwitz criterion. Appl. Energy 2004, 77, 287–308. [Google Scholar] [CrossRef]
- Jin, X.-C.; Lu, J.-G. Delay-dependent criteria for robust stability and stabilization of fractional-order time-varying delay systems. Eur. J. Control. 2022, 67, 100704. [Google Scholar] [CrossRef]
- Vojtov, V.; Fenenko, K.; Voitov, A.; Hrynkiv, A.; Lyashuk, O.; Vovk, Y. Methodical Approach to Using Acoustic Emission Method for Tribosystem Monitoring. Tribol. Ind. 2022, 44, 470–481. [Google Scholar] [CrossRef]

| Construction of the Tribosystem | Wb(s), N·m/s | Wb(exp), N·m/s | SWb, N·m/s | vWb, % | eWb, % |
|---|---|---|---|---|---|
| Steel 5140 + bronze C61900, motor oil SAE 40, APICC, Ey = 3.6·1014 J/m3, Kf = 6.25 m−1 | 1300 (I) * | 1200 (I) * | 200 | 16.6 | 8.3 |
| Steel 5140 + bronze C61900, motor oil SAE 40, APICC, Ey = 3.6·1014 J/m3, Kf = 12.5 m−1 | 1900 (I, f) * | 1700 (I, f) * | 300 | 17.6 | 11.7 |
| Steel 5140 + bronze C61900, motor oil SAE 40, APICC, Ey = 3.6·1014 J/m3, Kf = 22.6 m−1 | 2300 (f) * | 2000 (f) * | 400 | 20.0 | 15.0 |
| Steel 5140 + bronze C61900, Kf = 12.5 m−1, hydraulic oil HH, ISO-L-HL, (Ey = 2.43·1014 J/m3) | 850 (f) * | 1000 (f) * | 200 | 20.0 | 15.0 |
| Steel 5140 + bronze C61900, Kf = 12.5 m−1, motor oil SAE 40, APICC, (Ey = 3.6·1014 J/m3) | 1900 (I, f) * | 1700 (I, f) * | 300 | 17.6 | 11.7 |
| Steel 5140 + bronze C61900, Kf = 12.5 m−1, transmission oil SAE 120, APIGL-4, (Ey = 4.18·1014 J/m3) | 2600 (I) * | 2350 (I) * | 350 | 14.8 | 10.6 |
| Steel 5140 + steel5140, (RSTS(max) = 326.7 m−1), Kf = 12.5 m−1, motor oil SAE 40, APICC, Ey = 3.6·1014 J/m3 | 1300 (f) * | 1150 (f) * | 250 | 21.7 | 13.0 |
| Steel 5140 + bronze C61900, (RSTS(max) = 436.0 m−1), Kf = 12.5 m−1, motor oil SAE 40, APICC, Ey = 3.6·1014 J/m3 | 1900 (I, f) * | 1700 (I, f) * | 300 | 17.6 | 11.7 |
| Steel 5140 +brass CW723R, (RSTS(max) = 460.9 m−1), Kf = 12.5 m−1, motor oil SAE 40, APICC, Ey = 3.6·1014 J/m3 | 1950 (I, f) * | 1800 (I, f) * | 300 | 16.6 | 8.3 |
| Tribosystem No. 1: Steel 5140 + Steel 5140, (RSTS(max) = 326.7 m−1), Kf = 6.25 m−1, hydraulic oil HH, ISO-L-HL, (Ey = 2.43·1014 J/m3), Q0 = 1.12·1010 J/m3 | 650 (f) * | 800 (f) * | 150 | 18.7 | 18.7 |
| Tribosystem No. 2: Steel 5140 + bronze C61900, (RSTS(max) = 436.0 m−1), Kf = 12.5 m−1, motor oil SAE 40, APICC, Ey = 3.6·1014 J/m3, Q0 = 5.5·1010 J/m3 | 2000 (I, f) * | 1700 (I, f) * | 300 | 17.6 | 17.6 |
| Tribosystem No. 3: Steel 5140 + brass CW723R, (RSTS(max) = 460.9 m−1), Kf = 14.5 m−1,transmission oil SAE 120, APIGL-4, Ey = 4.18·1014 J/m3, Q0 = 7.69·1010 J/m3 | 3100 (I) * | 2700 (I) * | 350 | 12.9 | 14.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al-Quraan, T.M.A.; Alfaqs, F.; Alrefo, I.F.S.; Vojtov, V.; Voitov, A.; Kravtsov, A.; Miroshnyk, O.; Kondratiev, A.; Kučera, P.; Píštěk, V. Methodological Approach in the Simulation of the Robustness Boundaries of Tribosystems under the Conditions of Boundary Lubrication. Lubricants 2023, 11, 17. https://doi.org/10.3390/lubricants11010017
Al-Quraan TMA, Alfaqs F, Alrefo IFS, Vojtov V, Voitov A, Kravtsov A, Miroshnyk O, Kondratiev A, Kučera P, Píštěk V. Methodological Approach in the Simulation of the Robustness Boundaries of Tribosystems under the Conditions of Boundary Lubrication. Lubricants. 2023; 11(1):17. https://doi.org/10.3390/lubricants11010017
Chicago/Turabian StyleAl-Quraan, Tareq M. A., Fadi Alfaqs, Ibrahim F. S. Alrefo, Viktor Vojtov, Anton Voitov, Andrey Kravtsov, Oleksandr Miroshnyk, Andrii Kondratiev, Pavel Kučera, and Václav Píštěk. 2023. "Methodological Approach in the Simulation of the Robustness Boundaries of Tribosystems under the Conditions of Boundary Lubrication" Lubricants 11, no. 1: 17. https://doi.org/10.3390/lubricants11010017
APA StyleAl-Quraan, T. M. A., Alfaqs, F., Alrefo, I. F. S., Vojtov, V., Voitov, A., Kravtsov, A., Miroshnyk, O., Kondratiev, A., Kučera, P., & Píštěk, V. (2023). Methodological Approach in the Simulation of the Robustness Boundaries of Tribosystems under the Conditions of Boundary Lubrication. Lubricants, 11(1), 17. https://doi.org/10.3390/lubricants11010017








