Imprint of Pressure on Characteristic Dark Matter Profiles: The Case of ESO0140040
Abstract
1. Introduction
2. DM Distribution in Spirals
- 1.
- The ISO profile [14]:where r is the radial coordinate/distance. The model depends upon the two constants and .
- 2.
- The model depends on the same constants as in the ISO profile.
- 3.
- The model depends upon the same constants as above and overcomes the issue related to the cusp in galaxies. Despite its experimental triumph, the Burkert profile is not theoretically motivated and remains a phenomenological approach to face the DM problem.
- 4.
- The NFW profile [17]which was originally found as consequence of simulated halo formation in which the cosmological model is fixed.
- 5.
- Moore profile [18]:which is motivated by the fact that several studies claimed that simulated DM halos show significantly steeper cusps than NFW profile. This leads to a phenomenological reconstruction of DM profile, alternative to the NFW profile.
- 6.
- Einasto profile [19]:where is the Einasto free parameter. This extra parameter determines the degree of curvature (shape) of the profile. If are identified with cuspy halos, while for presents a cored-like behavior. The Einasto profile is quite different from the other models, whose slope is a power-law function. The larger , the more rapidly the slope varies with radius [20].
2.1. Galaxy Eso140040 within Newtonian Gravity
2.2. Perturbations and Optical Properties
2.3. The Role of the Dm Equation of State
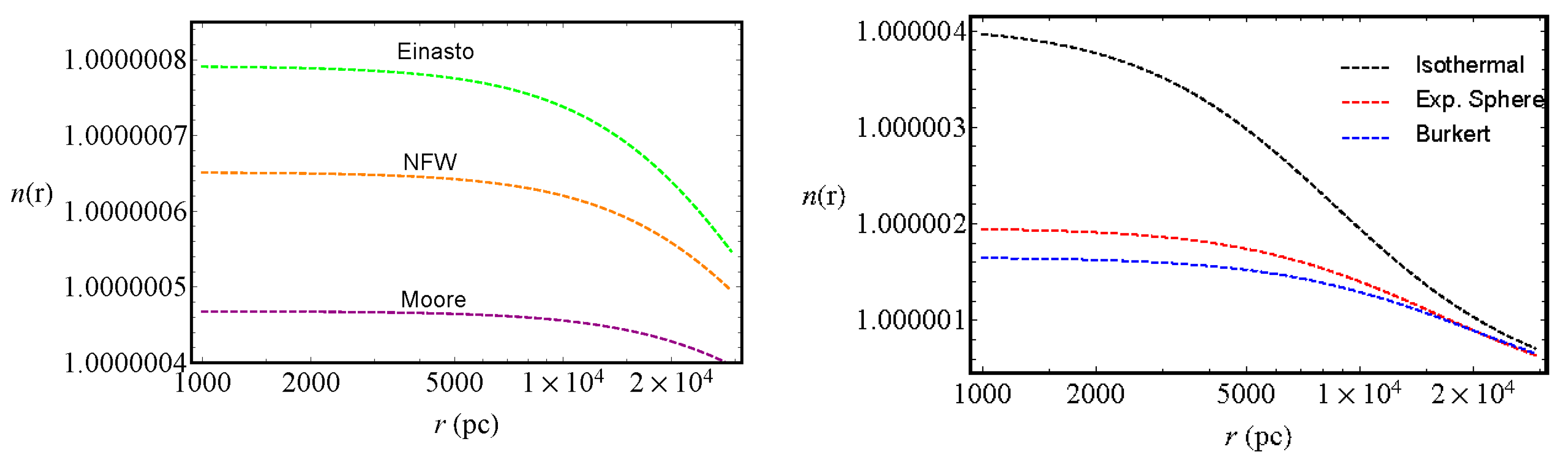
3. Methods and Analyses
3.1. Numerical Results
4. Final Outlooks and Perspectives
Author Contributions
Funding
Conflicts of Interest
References
- Ade, P.A.R.; Aghanim, N.; Alves, M.I.R.; Armitage-Caplan, C.; Arnaud, M.; Ashdown, M.; Atrio-Barandela, F.; Aumont, J.; Aussel, H.; Baccigalupi, C.; et al. Planck 2013 results. I. Overview of products and scientific results. Astron. Astrophys. 2014, 571, A1. [Google Scholar] [CrossRef]
- Bertone, G.; Hooper, D.; Silk, J. Particle dark matter: Evidence, candidates and constraints. Phys. Rep. 2005, 405, 279–390. [Google Scholar] [CrossRef]
- Schumann, M. Direct detection of WIMP dark matter: Concepts and status. J. Phys. Nucl. Part. Phys. 2019, 46, 103003. [Google Scholar] [CrossRef]
- Tam, S.I.; Jauzac, M.; Massey, R.; Harvey, D.; Eckert, D.; Ebeling, H.; Ellis, R.S.; Ghirardini, V.; Klein, B.; Kneib, J.P.; et al. The distribution of dark matter and gas spanning 6 Mpc around the post-merger galaxy cluster MS 0451–03. Mon. Not. R. Astron. Soc. 2020, 496, 4032–4050. [Google Scholar] [CrossRef]
- Ablimit, I.; Zhao, G.; Flynn, C.; Bird, S.A. The Rotation Curve, Mass Distribution, and Dark Matter Content of the Milky Way from Classical Cepheids. Astrophys. J. Lett. 2020, 895, L12. [Google Scholar] [CrossRef]
- Epinat, B.; Adamczyk, P.; Bounissou, S.; Amram, P.; Neichel, B. Dark matter distribution in distant galaxies with HARMONI. In Proceedings of the SF2A-2019: Proceedings of the Annual meeting of the French Society of Astronomy and Astrophysics, Paris, Nice, France, 14–17 May 2019. [Google Scholar]
- Jungman, G.; Kamionkowski, M.; Griest, K. Supersymmetric dark matter. Phys. Rep. 1996, 267, 195–373. [Google Scholar] [CrossRef]
- Einasto, J.; Kaasik, A.; Saar, E. Dynamic evidence on massive coronas of galaxies. Nature 1974, 250, 309–310. [Google Scholar] [CrossRef]
- Rubin, V.C.; Burstein, D.; Ford, W.K.J.; Thonnard, N. Rotation velocities of 16 SA galaxies and a comparison of Sa, SB and SC rotation properties. Astrophys. J. 1985, 289, 81–104. [Google Scholar] [CrossRef]
- Davis, M.; Efstathiou, G.; Frenk, C.S.; White, S.D.M. The evolution of large-scale structure in a universe dominated by cold dark matter. Astrophys. J. 1985, 292, 371–394. [Google Scholar] [CrossRef]
- Bode, P.; Bahcall, N.A.; Ford, E.B.; Ostriker, J.P. Evolution of the Cluster Mass Function: GPC3 Dark Matter Simulations. Astrophys. J. 2001, 551, 15–22. [Google Scholar] [CrossRef]
- Boshkayev, K.; Zhumakhanova, G.; Mutalipova, K.; Muccino, M. Investigation of different dark matter profiles. News Natl. Acad. Sci. Repub. Kazakhstan. Phys. Math. Ser. 2019, 6, 25–33. [Google Scholar] [CrossRef]
- Chechin, L.M.; Konysbayev, T.K. Searching the Parameters of Dark Matter Halos on the Basis of Dwarf Galaxies’ Dynamics. J. Mod. Phys. 2016, 7, 982–988. [Google Scholar] [CrossRef]
- Jimenez, R.; Verde, L.; Oh, S.P. Dark halo properties from rotation curves. Mon. Not. R. Astron. Soc. 2003, 339, 243–259. [Google Scholar] [CrossRef]
- Sofue, Y. Rotation Curve and Mass Distribution in the Galactic Center - From Black Hole to Entire Galaxy. Publ. Astron. Soc. Jpn. 2013, 65, 118. [Google Scholar] [CrossRef]
- Burkert, A. The Structure of Dark Matter Halos in Dwarf Galaxies. Astrophys. J. Lett. 1995, 447, L25–L28. [Google Scholar] [CrossRef]
- Navarro, J.F.; Frenk, C.S.; White, S.D.M. The Structure of Cold Dark Matter Halos. Astrophys. J. 1996, 462, 563. [Google Scholar] [CrossRef]
- Moore, B.; Governato, F.; Quinn, T.; Stadel, J.; Lake, G. Resolving the Structure of Cold Dark Matter Halos. Astrophys. J. Lett. 1998, 499, L5–L8. [Google Scholar] [CrossRef]
- Merritt, D.; Graham, A.W.; Moore, B.; Diemand, J.; Terzić, B. Empirical Models for Dark Matter Halos. I. Nonparametric Construction of Density Profiles and Comparison with Parametric Models. Astron. J. 2006, 132, 2685–2700. [Google Scholar] [CrossRef]
- Einasto, J. On the Construction of a Composite Model for the Galaxy and on the Determination of the System of Galactic Parameters. Trudy Astrofiz. Inst.-Alma-Ata 1965, 5, 87–100. [Google Scholar]
- Barranco, J.; Bernal, A.; Núñez, D. Dark matter equation of state from rotational curves of galaxies. Mon. Not. R. Astron. Soc. 2015, 449, 403–413. [Google Scholar] [CrossRef]
- de Blok, W.J.G.; McGaugh, S.S.; Rubin, V.C. High-Resolution Rotation Curves of Low Surface Brightness Galaxies. II. Mass Models. Astron. J. 2001, 122, 2396–2427. [Google Scholar] [CrossRef]
- Shapiro, S.L.; Teukolsky, S.A. Black Holes, White Dwarfs and Neutron Stars: The Physics of Compact Objects; Wiley-VCH: Weinheim, Germany, 1986. [Google Scholar]
- Misner, C.W.; Thorne, K.S.; Wheeler, J.A. Gravitation Volume 2; W. H. Freeman & Company: San Francisco, CA, USA, 1977; Volume 2. [Google Scholar]
- Capozziello, S.; D’Agostino, R.; Luongo, O. Extended gravity cosmography. Int. J. Mod. Phys. D 2019, 28, 1930016. [Google Scholar] [CrossRef]
- Del Popolo, A. Dark matter, density perturbations, and structure formation. Astron. Rep. 2007, 51, 169–196. [Google Scholar] [CrossRef]
- Perlick, V. Gravitational Lensing from a Spacetime Perspective. Living Rev. Relativ. 2004, 7, 9. [Google Scholar] [CrossRef]
- Chechin, L.M.; Kurmanov, E.B.; Konysbaev, T.K. Geometrical Optics in a Universe with Dominance of Dark Matter. Russ. Phys. J. 2020, 63, 58–63. [Google Scholar] [CrossRef]
- Capozziello, S.; De Laurentis, M.; Luongo, O.; Ruggeri, A. Cosmographic Constraints and Cosmic Fluids. Galaxies 2013, 1, 216–260. [Google Scholar] [CrossRef]
- Dunsby, P.K.S.; Luongo, O. On the theory and applications of modern cosmography. Int. J. Geom. Methods Mod. Phys. 2016, 13, 1630002–1630606. [Google Scholar] [CrossRef]
- Aviles, A.; Gruber, C.; Luongo, O.; Quevedo, H. Cosmography and constraints on the equation of state of the Universe in various parametrizations. Phys. Rev. D 2012, 86, 123516. [Google Scholar] [CrossRef]
- Salucci, P. Dark Matter in Galaxies: Evidences and Challenges. Found. Phys. 2018, 48, 1517–1537. [Google Scholar] [CrossRef]
- Copeland, E.J.; Sami, M.; Tsujikawa, S. Dynamics of Dark Energy. Int. J. Mod. Phys. D 2006, 15, 1753–1935. [Google Scholar] [CrossRef]
- Faber, T.; Visser, M. Combining rotation curves and gravitational lensing: How to measure the equation of state of dark matter in the galactic halo. Mon. Not. R. Astron. Soc. 2006, 372, 136–142. [Google Scholar] [CrossRef]
- Luongo, O.; Muccino, M. Speeding up the Universe using dust with pressure. Phys. Rev. D 2018, 98, 103520. [Google Scholar] [CrossRef]
- Campos, J.P.; Fabris, J.C.; Perez, R.; Piattella, O.F.; Velten, H. Does Chaplygin gas have salvation? Eur. Phys. J. C 2013, 73, 2357. [Google Scholar] [CrossRef]
- Will, C.M. The Confrontation between General Relativity and Experiment. Living Rev. Relativ. 2014, 17, 4. [Google Scholar] [CrossRef]
- Yegorova, I.A.; Babic, A.; Salucci, P.; Spekkens, K.; Pizzella, A. Rotation curves of luminous spiral galaxies. Astron. Astrophys. Trans. 2012, 27, 335–338. [Google Scholar] [CrossRef]
- Levenberg, K. A method for the solution of certain non-linear problems in least squares. Q. Appl. Math. 1944, 2, 164–168. [Google Scholar] [CrossRef]
- Marquardt, D. An algorithm for least-squares estimation of nonlinear parameters. J. Appl. Math. 1963, 11, 431–441. [Google Scholar] [CrossRef]
- Garcia-Aspeitia, M.A.; Lopez-Dominguez, J.C.; Ortiz, C.; Hinojosa-Ruiz, S.; Rodriguez-Meza, M.A. Energy density profile inspired by noncommutativity. arXiv 2015, arXiv:1511.06740. [Google Scholar]
- Akaike, H. A new look at the statistical model identification. IEEE Trans. Autom. Control. 1974, 19, 716–723. [Google Scholar] [CrossRef]
- Kunz, M.; Trotta, R.; Parkinson, D.R. Measuring the effective complexity of cosmological models. Phys. Rev. D 2006, 74, 023503. [Google Scholar] [CrossRef]
- Schwarz, G.D. Estimating the Dimension of a Model. Ann. Stat. 1963, 6, 461–464. [Google Scholar] [CrossRef]
- Siutsou, I.; Argüelles, C.R.; Ruffini, R. Dark matter massive fermions and Einasto profiles in galactic haloes. Astron. Rep. 2015, 59, 656–666. [Google Scholar] [CrossRef]
- Salucci, P.; Lapi, A.; Tonini, C.; Gentile, G.; Yegorova, I.; Klein, U. The universal rotation curve of spiral galaxies—II. The dark matter distribution out to the virial radius. Mon. Not. R. Astron. Soc. 2007, 378, 41–47. [Google Scholar] [CrossRef]




| Profiles | [] | [kpc] | [] | [] | BIC | |
|---|---|---|---|---|---|---|
| Burkert | 14 | 3.3 | ||||
| NFW | 3 | 1 | ||||
| ISO | 5 | 1 | ||||
| Moore | 7 | 1.4 | ||||
| Einasto | - | 0.4 | ||||
| Exp. Sphere | 18 | 5.5 |
| 1. | One is forced to exclude dark baryons since the numerical simulations provide radically different large-scale structure of the Universe. |
| 2. | As morphological and clustering properties of galaxies, abundances of rich clusters, halo masses degenerate if we consider cold, warm or collisional DM. We therefore seek tests of DM’s nature that could be sensitive to its presumed microphysics and/or to its interaction properties and we can assume to consider density profiles only without taking care at this stage of other velocity corrections. |
| 3. | In general, all model parameters can be estimated by analyzing galaxies, albeit with a strong unvoidable degeneracy [12]. |
| 4. | With increasing interests, models that aim to unify DM with dark energy are always more studied. A final goal could be to unify the dark Universe under the same standards. |
| 5. | For a review of galaxy morphology and properties of DM see [38]. |
| 6. | The Levenberg-Marquardt algorithm is an iterative technique that locates the minimum of a function that is expressed as the sum of squares of nonlinear functions. It consists of a combination of the Gauss–Newton algorithm and the method of the steepest descent gradient. |
| 7. | BIC is a selection criterion among a finite set of models, conceived to solve the overfitting issue when increasing the number of parameters in the fitting function. For the sake of completeness, please notice that other selection criteria could be used, e.g., the Akaike information and/or DIC criteria [42,43]. |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Boshkayev, K.; Konysbayev, T.; Kurmanov, E.; Luongo, O.; Muccino, M. Imprint of Pressure on Characteristic Dark Matter Profiles: The Case of ESO0140040. Galaxies 2020, 8, 74. https://doi.org/10.3390/galaxies8040074
Boshkayev K, Konysbayev T, Kurmanov E, Luongo O, Muccino M. Imprint of Pressure on Characteristic Dark Matter Profiles: The Case of ESO0140040. Galaxies. 2020; 8(4):74. https://doi.org/10.3390/galaxies8040074
Chicago/Turabian StyleBoshkayev, Kuantay, Talgar Konysbayev, Ergali Kurmanov, Orlando Luongo, and Marco Muccino. 2020. "Imprint of Pressure on Characteristic Dark Matter Profiles: The Case of ESO0140040" Galaxies 8, no. 4: 74. https://doi.org/10.3390/galaxies8040074
APA StyleBoshkayev, K., Konysbayev, T., Kurmanov, E., Luongo, O., & Muccino, M. (2020). Imprint of Pressure on Characteristic Dark Matter Profiles: The Case of ESO0140040. Galaxies, 8(4), 74. https://doi.org/10.3390/galaxies8040074








