Blazar Optical Polarimetry: Current Progress in Observations and Theories

Abstract

Polarimetry has been a standard tool to probe the active galactic nucleus (AGN) jet magnetic field. In recent years, several optical polarization monitoring programs have been carried out, bringing in many exciting new results and insights into jet dynamics and emission. This article discusses current progress in blazar optical polarimetry. The main focus is the variability of polarization signatures, which has spurred a lot of theoretical studies. These novel developments have provided unique constraints on the blazar flares and emphasized the role of the magnetic field in jet evolution. Optical polarimetry will continue to act as an essential component in the multi-messenger study of AGN jets, in particular with the upcoming high-energy polarimetry. Comparing to first-principle numerical simulations, future multi-wavelength polarimetry can shed light on jet dynamics, particle acceleration, and radiation processes.

1. Introduction

Relativistic jets from active galactic nuclei (AGNs) are powered by strong accretion onto central supermassive black holes. These plasma jets can stay highly collimated during their propagation through space, extending to kilo-parsec (kpc) or even up to mega-parsec (Mpc) scales (see, e.g., [1,2]). They are the most powerful astrophysical systems in our universe, showing bright emission from radio up to TeV $\gamma$-rays (e.g., [3,4,5,6]). Jet emission is non-thermal-dominated and highly variable. Blazars are a subclass of AGNs whose jets point very close to our line of sight. Due to relativistic beaming, their apparent luminosity is strongly boosted, making them the most numerous extragalactic $\gamma$-ray sources [7,8]. Blazars consist of flat spectrum radio quasars (FSRQs) and BL Lac objects (BL Lacs). The difference is that BLLacs generally do not show strong emission lines in the optical band. For recent reviews on blazars, see, for example, [9,10].

Blazars exhibit a broadband nonthermal spectrum extending from radio to $\gamma$-rays. This spectrum consists of a low-energy component and a high-energy component. The low-energy component usually goes from radio to infrared/optical, and in some cases it can extend to soft X-rays. The high-energy component extends from X-rays to $\gamma$-rays. The low-energy component is dominated by the synchrotron emission of relativistic electrons. This is evident by the high linear polarization degree in the radio to optical bands (e.g., [11,12]). The high-energy component may be explained by either leptonic or hadronic scenarios. The leptonic scenario assumes that the same electrons that make the low-energy synchrotron component can inverse Compton scatter low-energy photons to high energies. The source of the low-energy photons can come from either the synchrotron emission itself (synchrotron-self Compton, SSC, [13,14]) or external sources such as the thermal emission from the broad line region and dusty torus (external Compton, EC, [15,16,17]). Spectral fitting with leptonic models usually assumes a relatively low magnetic field, typically from mG to G levels [18,19]. On the other hand, the hadronic models suggest that the protons may be accelerated to very high energies, then produce the high-energy component via proton synchrotron and/or photomeson processes [20,21,22]. Depending on the model parameters, the SSC from electrons can contribute to the high-energy component in the hadronic model as well [18]. Hadronic models generally predict the production of neutrinos in jets. In the case of proton synchrotron models, the required magnetic field is usually strong (≳10 G), and the acceleration of ultra high energy cosmic rays (UHECRs) in the blazar jet is often necessary [18,23]. Both scenarios can reproduce the typical blazar spectra and light curves (see, e.g., [24,25,26,27,28]). Recently, the IceCube-170922A event showed the first potential association of a very high energy neutrino detection with the flaring blazar TXS 0506+056, opening a multi-messenger window for the study of AGN jets, and IceCube has discovered 13 neutrino events from the same direction in the 2014–2015 period [29,30]. Nonetheless, so far, theoretical studies find it very controversial how the blazar jets accelerate protons to very high energy and produce the neutrino and blazar flare events (refer to, e.g., [31,32,33,34,35]).

Clearly, additional information beyond spectra and light curves are necessary to better understand jet physics. Polarization signatures can probe the magnetic field structure and evolution in AGN jets. Radio and optical polarimetry have been successfully run for decades, providing direct constraints on the jet magnetic field (e.g., [36,37,38,39]). Recent optical polarization monitoring programs have made significant efforts in collecting both spectral and temporal optical polarization data. These observations have shown that polarization signatures can be highly variable, especially during blazar flaring activities [40,41,42,43]. Very interestingly, optical polarization angle swings have been reported in correlation with multi-wavelength flares, indicating active magnetic field evolution during flares [44,45,46]. These results are consistent with predictions of recent first-principle numerical simulations, which have also demonstrated that the magnetic field in jets can play a crucial role in jet dynamics and particle acceleration (e.g., [47,48,49,50]). Furthermore, predictions on multi-wavelength polarimetry have suggested that future X-ray and $\gamma$-ray polarimetry alongside optical polarimetry can be powerful diagnostics of proton acceleration and neutrino emission in AGN jets [51,52].

This article reviews recent progress in the optical polarimetry of blazar jets. Section 2 covers results from the optical polarization monitoring programs, focusing on the time-dependent signatures. Section 3 summarizes current theoretical interpretations of these phenomena, especially the polarization angle swings. Section 4 discusses future prospects of optical polarimetry in the multi-messenger era. Although the goal of this article is to provide a balanced overview of optical polarimetry studies, it may be biased by the relevance to the above topics and the author’s research interests.

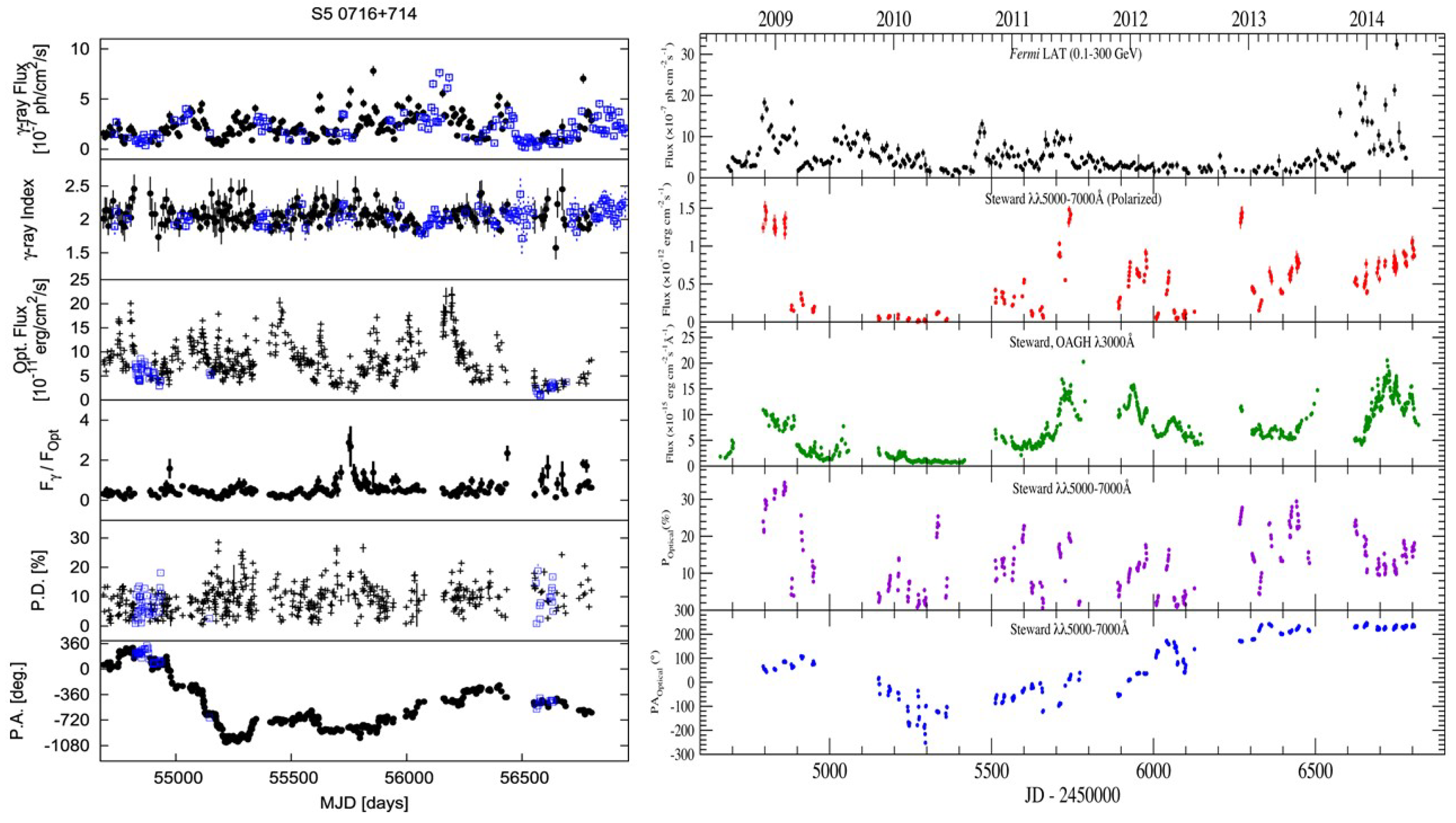
2. Progress in Observations

Blazar optical emission can be linearly polarized up to ∼50% [11,53,54]. Circular polarization is hardly detected in blazars (but see [55] for potential detection in quasars). Therefore, the term polarization usually refers to linear polarization in the optical bands. Both polarization degree and angle are commonly reported in the literature. In some cases, the polarized flux, a part of total flux that is equivalent to 100% linear polarization, is reported along with the polarization degree [56]. This number can help to identify whether there is a newly emerging polarized/unpolarized emission component in the jet. In this section, we highlight some recent discoveries in the optical polarization measurement of blazar jets, with an emphasis on the very interesting polarization angle rotations.

2.1. Overview

Since the launch of the Fermi $\gamma$-ray telescope, the optical spectropolarimetric measurements of blazars have been performed by several programs, such as the Steward Observatory blazar monitoring program1 and the Boston University blazar program,2 as a complement to the multi-wavelength spectra and light curves [40,46,57,58,59,60]. The optical polarization signatures can be different from the radio counterparts because the radio emission usually comes from a much larger portion of the jet. The optical polarization degree is typically at ∼10%, but sometimes it can reach significantly higher values, especially during flares [45,53,61]. The polarization angle apparently does not always align with the radio jet direction (e.g., [62,63]). The polarization signatures between different optical bands are generally consistent.

Long-term optical polarization monitoring reveals that the overall temporal polarization signatures appear erratic ([57,58,64,65], and see Figure 1 for examples). However, particularly during active states, both the polarization degree and angle can exhibit systematic changes, with reported variability time scales ranging from months down to hours [57,65,66,67]. Several patterns are frequently reported in the literature. One is that the polarization degree can increase during the flare [56,68,69]. This may originate from a shock that compresses the initially turbulent magnetic field [70] or the emergence of a new, strongly polarized emission zone [56]. In some cases, the polarization signatures are very erratic during flares, which may be attributed to the turbulent magnetic field structure [71]. A rare but very interesting behavior is the polarization angle rotation. These rotations generally happen together with blazar flares [44,72,73], indicating considerable change in the jet structure and/or the magnetic field morphology.

The RoboPol3 program makes the first attempt to study the statistical patterns of optical polarization signatures (see Figure 2 for some trends that they have found). They have gathered several years of polarization measurements for tens of $\gamma$-ray -oud and $\gamma$-ray-quiet blazars, with no preference for whether or not the target blazar is in active state, so as to perform a systematic study of the polarization variability. Based on their sample,

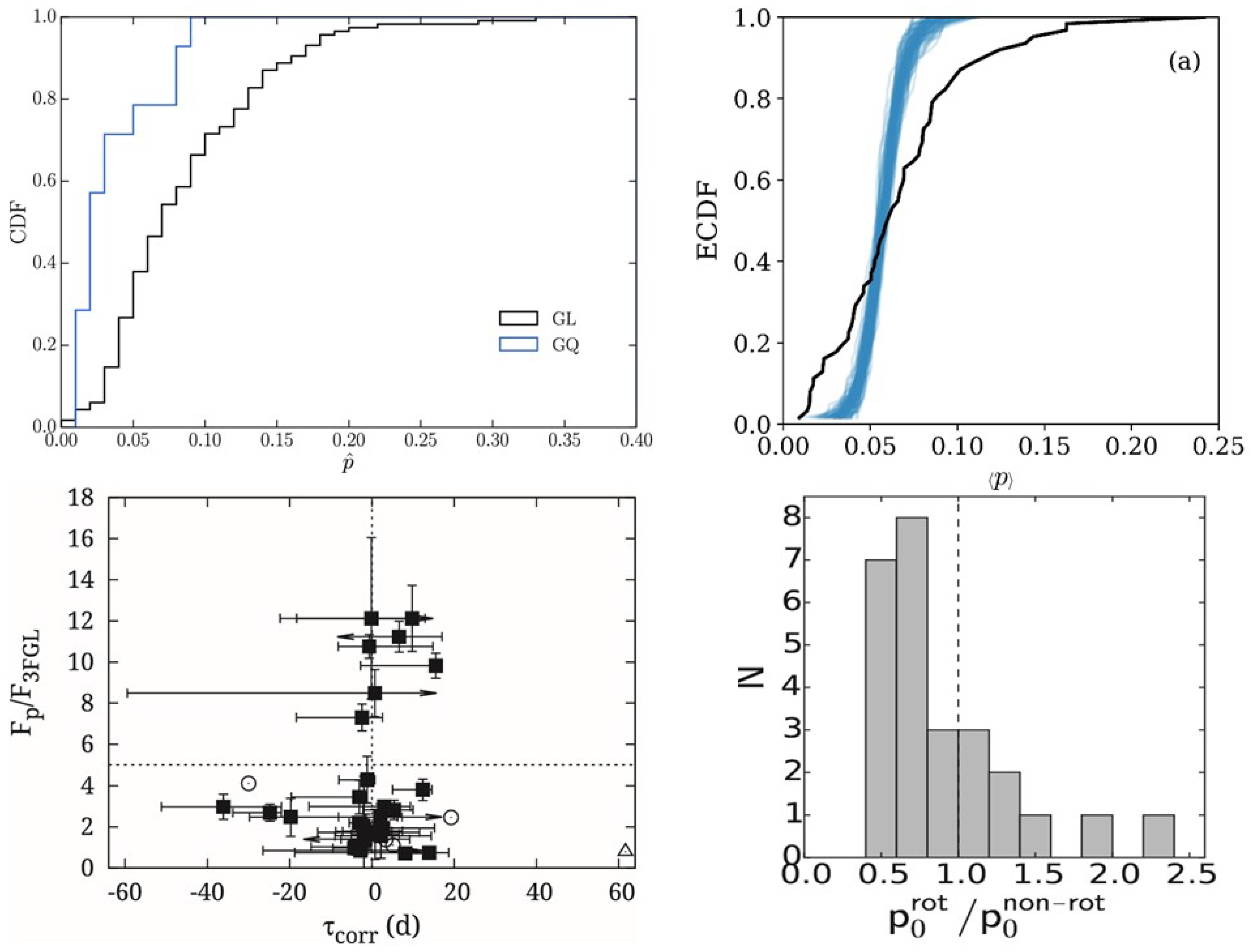
• The average polarization degree of $\gamma$-ray-loud blazars appears systematically higher than $\gamma$-ray-quiet ones [74].
• The overall polarization temporal behaviors are similar regardless of whether the blazar is detected in the TeV band [75].
• The average polarization degree seems to decrease with a higher peak location of the synchrotron spectral component [74] (this trend has been found in previous works [76,77]).
• The polarization degree variability cannot be completely reproduced by random walk simulations [78].

These trends hint at the potential connection of the overall polarization signatures to the “blazar sequence” [79]. Additional observational data can examine the robustness of these trends, and if confirmed, what the physical causes are.

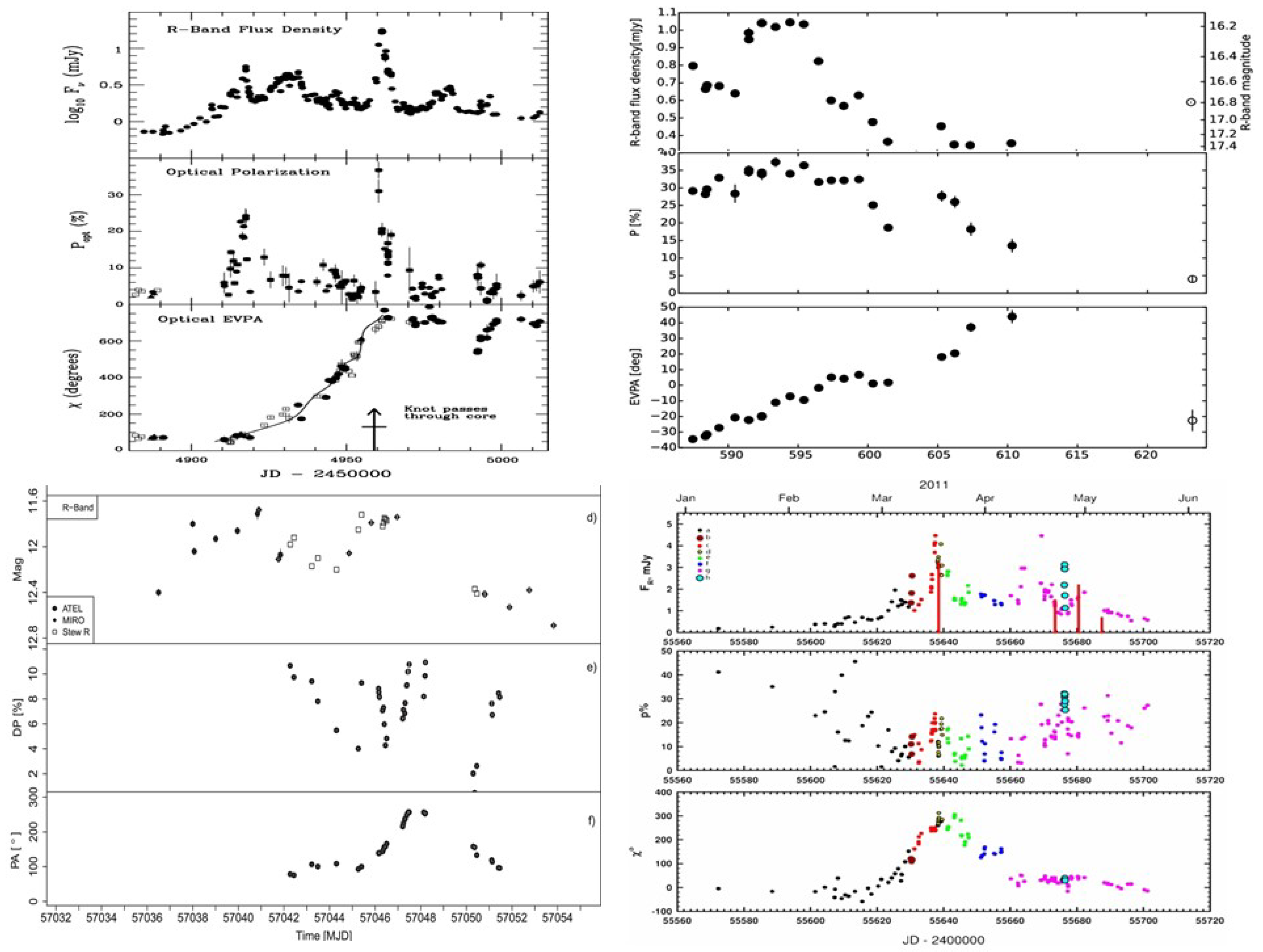
2.2. Polarization Angle Rotation

The polarization angle usually shows small erratic fluctuations. Sometimes it can continuously rotate in one direction, and in very rare cases, it can swing ∼180${}^{\circ }$ or even beyond that. Such events were first discovered simultaneously with multi-wavelength blazar flares in ([44,81], see Figure 3), indicating major changes in the magnetic field during flares. Notice that [81] does not include Steward Observatory data in the analysis. By including those data, the polarization angle swing is not present. Since the polarization angle has the ${180}^{\circ }$ ambiguity, it requires close sampling of data points to establish a polarization angle swing. Recent optical polarization monitoring programs have collected a large number of polarization angle data and demonstrated that such swings can be present in a number of blazars (see, e.g., [46,58,65,75]).

Although large polarization angle rotations (≳90${}^{\circ }$ rotation) are very rare events, they have been reported in both FSRQs and BL Lacs [80]. Here we highlight some interesting features in the observations (see Figure 4 for some examples). Many large polarization angle swings rotate ∼180${}^{\circ }$ [46,74]. So far, the largest polarization angle swing is ∼${720}^{\circ }$ [57]. Almost all of these large rotations are reported to be simultaneous with multi-wavelength blazar flares [73]. Given the limited samples, this may be a selection bias, since optical polarimetry is often performed when there are flaring activities (but see RoboPol data for unbiased observations). In most cases, the polarization degree is strongly varying along with the swings [45,57]. The timescale of these angle swings are typically in days to weeks [46,57,58]. With the increasing reports of polarization angle swings, it is now recognized that these swings can happen in either direction in the same source [53,72].

The RoboPol program has significantly boosted the number of observed polarization angle swings. They have attempted statistical study based on their data sample, in which they have found several interesting trends:

• Polarization angle swings are associated with $\gamma$-ray flares, with little time lag [73].
• Polarization angle swings do not happen in all blazars, but they can happen in both FSRQs and BL Lacs [80].
• Blazars that have shown rotations generally have brighter and more variable $\gamma$-ray emission [80].
• The polarization degree generally decreases during angle rotations [80].
• It is unlikely that all rotations are consistent with random walks [78].

Given the small sample of angle swings, additional observations are essential to examine the robustness of these trends. Nevertheless, these trends strongly suggest that the polarization angle rotations may reflect major changes in the jet magnetic field morphology.

3. Theoretical Models

As previously mentioned, the long-term polarization signatures appear erratic, which is likely due to instabilities and/or turbulence in the blazar jets. Several works have shown that the observed polarization degree and angle suggest a helical magnetic field structure [82,83]. In some cases, radio observations have shown that blazar activities, including optical polarization variations, are correlated to the emergence of a new radio emission blob [44,62]. However, a detailed theoretical study of such events generally requires a comprehensive modeling of large-scale jet dynamics with particle transport and radiation transfer, which is not mature at present. For the short-term polarization signatures, the frequently observed increasing polarization degree during flares is often interpreted as a shock compressing the initially turbulent magnetic field and accelerating particles [70,71]. Recently, the polarization angle swings have triggered a large number of theoretical studies. They can be generally categorized into geometrical and physical models. In this section, we give a general review of both scenarios.

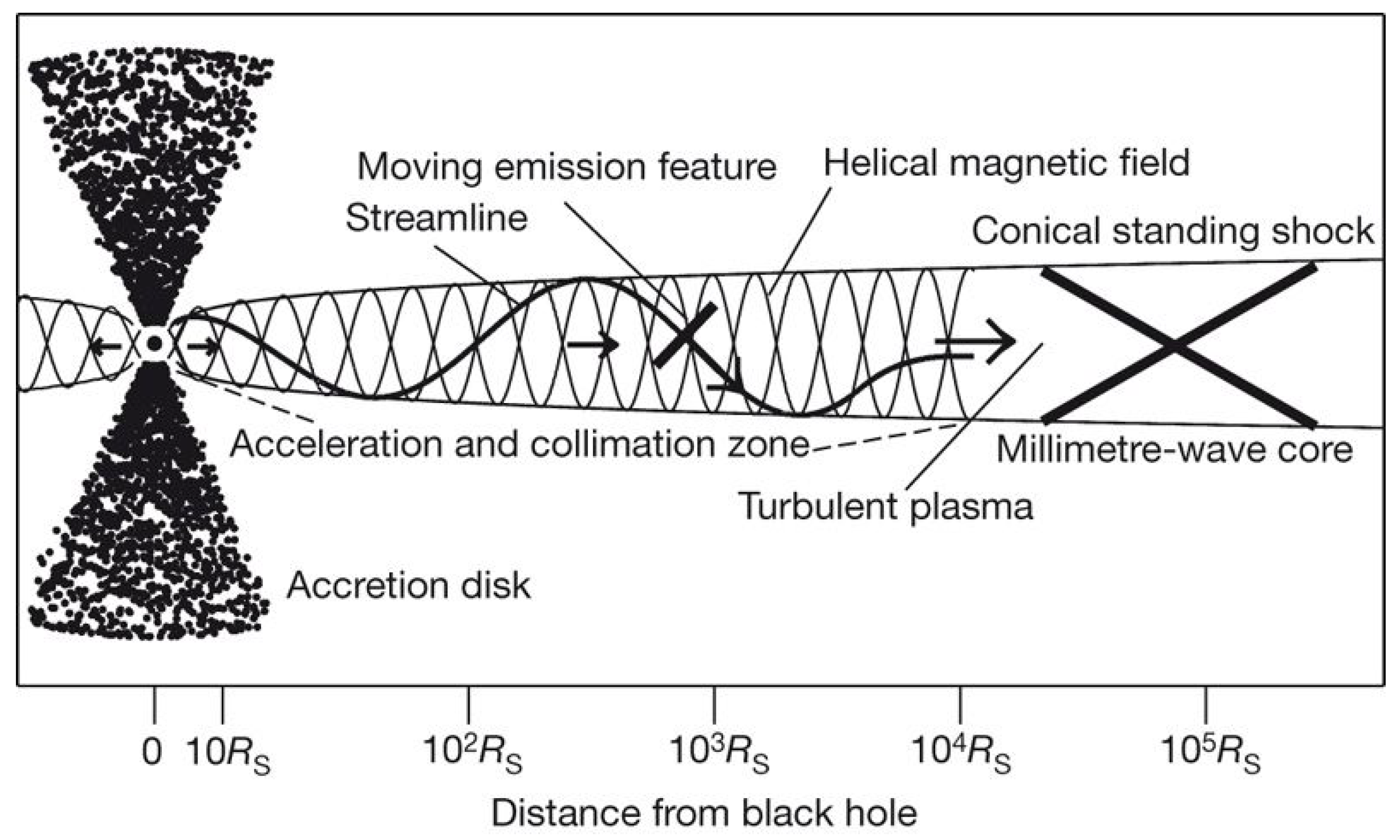
3.1. Geometrical Models

A bending jet may explain the ∼${180}^{\circ }$ polarization angle swing. The idea is that if the jet curvature is confined to a plane inclined to the line of sight within an angle ${\theta }_{\mathrm{obs}}<1/{\Gamma }_{\mathrm{jet}}$, where ${\Gamma }_{\mathrm{jet}}$ is the jet bulk Lorentz factor, then the curvature alters the projection of the jet magnetic field on the plane of the sky, giving rise to a polarization angle rotation [45]. Such jet configurations are frequently observed in radio observations [84,85]. Due to the change in the Doppler boosting, the bending jet model predicts that the angle rotation should always accompany a blazar flare, which is consistent with current observations. However, the bending jet model can only generate rotation up to ${180}^{\circ }$. Furthermore, the jet curvature assumed in the model intrinsically links to large-scale jet structure, requiring that all angle rotations in a given source be in the same direction, which contradicts some observations [53,72].

Reference [44] has suggested a spiral motion model where a compact emission blob propagates in a spiral trajectory in a jet pervaded by a helical magnetic field (see Figure 5). The emission blob then lights up the magnetic field structure at its location. Since the magnetic field has a helical shape, this gives rise to the rotating polarization angle. In principle, the amplitude of the rotation can be arbitrary, depending on the helical magnetic field structure and the propagation of the emission blob. The model assumes that the compact emission blob is driven by internal shocks, thus the polarization angle rotation should accompany blazar flares. This model has been successfully applied to several observations [44,45]. However, the direction of the rotation is determined by the helicity of the jet magnetic field, which is unlikely to change in the same blazar. In addition, the propagation of internal shocks in jets should alter the magnetic field structure, which may affect the resulting polarization signatures.

Recently, there have been a couple of new geometric models proposed. Reference [86] has considered the ballistic motion of emission blobs in a jet with a helical magnetic field. Their results have shown that the polarization angle rotations are accompanied by a highly variable polarization degree, which may reach zero during very fast angle rotations. Additionally, the flux is usually at the maximum during fast angle rotations. Reference [87] has proposed a relativistic polarization angle rotation model, where multiple emission blobs in a partially ordered magnetic field may generate angle rotation due to different Doppler boosting. Both models emphasize the effect of the viewing angle, and their results are qualitatively consistent with current observations.

3.2. Physical Models

Given that the observed optical polarization degree is typically below 20%–30%, while the theoretical synchrotron polarization degree in an ordered magnetic field should reach ≳$70\%$, it is very likely that the blazar emission region has a considerable level of turbulence. Reference [71] has put forward a turbulent extreme multi-zone (TEMZ) model to explain the polarization signatures (see Figure 6). The model splits the emission region into a large number of turbulent cells with different magnetic field orientations. The summed synchrotron radiation from these cells can result in a low residual polarization degree with erratic fluctuations in both degree and angle. During active states, shock or magnetic reconnection may inject nonthermal particles and change the magnetic field in some cells, leading to flares and polarization variations. This model has successfully reproduced the typical blazar flares and stochastic polarization signatures. In rare cases, the polarization angle may exhibit large swings. The swings can happen in either direction, with arbitrary amplitude. Due to this stochastic nature, the polarization signatures all appear to be random walks, thus the larger rotations are rarer. Recently, reference [88] has performed particle-in-cell (PIC) simulations of the relaxation of unstable force-free magnetostatic equilibria. The resulting very turbulent magnetic field morphology leads to stochastic polarization signatures, consistent with the predictions of the TEMZ model. However, due to the stochastic nature of this model, the polarization angle swings should be statistically unrelated to the blazar flares, contradicting the RoboPol results [73]. In addition, the RoboPol results do not suggest that all polarization angle swings are random walks [78].

Shock and kink instabilities can efficiently dissipate jet bulk energy to accelerate particles [90,91,92,93]. A side effect is that both mechanisms can considerably alter the magnetic field morphology. Reference [94] developed the 3DPol code to study the radiation and polarization signatures of shock and kink instabilities. They have shown that both mechanisms can produce ∼${180}^{\circ }$ polarization angle swings along with multi-wavelength emission ([95,96,97], and see Figure 7). Both mechanisms predict smooth polarization angle rotations on time-scales of days to weeks. Numerical simulations have shown that the angle rotations are in the unit of ∼${90}^{\circ }$ for both mechanisms, but neither can go much beyond ${180}^{\circ }$ [97,98,99,100]. Another drawback is that the direction of the rotation depends on the helicity of the magnetic field in the emission region, thus the polarization angle prefers to rotate in the same direction in one active epoch. Although this model has successfully reproduced the multi-wavelength radiation and polarization signatures for a couple of events [67,72,96], they cannot account for all of the polarization angle rotations.

Magnetic reconnection can efficiently dissipate magnetic energy to accelerate particles [49,50,101]. In recent years, it has been well studied, both analytically and numerically, that magnetic reconnection can explain the blazar spectra and light curves [88,102,103,104,105]. Recently, [106] has combined PIC with polarized radiation transfer simulations to study the polarization signatures during reconnection. Very interestingly, magnetic reconnection can make strong polarization angle swings (see Figure 8). The idea is that the large plasmoids generated in the reconnection layer can merge into each other and trigger secondary reconnection. This leads to additional particle acceleration where the accelerated particles can stream along the magnetic field lines in the plasmoids, giving rise to polarization angle swings. The rotating angle appears to be in the unit of ∼${90}^{\circ }$, and it can extend well beyond ${180}^{\circ }$. Additionally, the polarization angle can rotate in either direction. During the swings, the polarization degree usually drops, and there are always flares due to the particle acceleration. This model shows promising features consistent with current observations, but it still needs further examination to test its robustness.

4. Future Prospects

The study of AGN jets will become multi-messenger in the next decade. Optical polarimetry will be an important component of multi-messenger studies for two reasons. First, since the correlation of electromagnetic and neutrino signals, and especially their variability, is essential to multi-messenger study, optical polarization variability can provide an additional dimension of observables, providing invaluable constraints for the physical processes in AGN jets. Second, the high-energy polarimetry of AGN jets will become available in the next decade. Synergizing with optical polarimetry, multi-wavelength polarimetry will unveil the high-energy radiation mechanisms and the extreme particle acceleration in AGN jets.

4.1. Polarization Variability

Polarization variability is a very promising research field that can boost our understanding of magnetic field evolution in AGN jets. Recent years of optical polarization monitoring have already made significant breakthroughs in jet dynamics. An important yet not well explored aspect is the statistical trends in the polarization signatures, due to the limited observational data. Continual efforts in polarization monitoring will lead to a better understanding of these trends and the diagnosis of whether the polarization variability is of geometrical or physical origins. Questions to be answered include whether the temporal polarization patterns are different between different optical bands, and when available, X-ray and $\gamma$-ray bands; whether polarization angle rotations can be categorized into stochastic and deterministic, and what differences they may have, such as the time scales, polarization degrees, and smoothness; and what makes the blazars with polarization angle rotations different from other blazars. Although some of these questions already have some preliminary answers [58,65,74,80], current results are not fully consistent between different research groups.

In order to better understand the polarization variability, theoretical models need to have a consistent picture of jet dynamics, particle acceleration, and radiation processes. However, the very dynamical and complex nature of AGN jets prevents the use of simple analytical models. Recent first-principle numerical simulations have attempted to consistently combine plasma dynamics and radiation processes to directly compare with observations [88,98,104,105,107], which have shown interesting predictions in polarization variability. With the development of CPU and GPU computing in the next decade, this multi-physics approach will revolutionize our understanding of jet dynamics.

4.2. Multi-Wavelength Polarimetry

High-energy polarimetry will open a new window to study AGN jets. The Imaging X-ray Polarimetry Explorer (IXPE) mission4 has been selected by NASA for launch in the early 2020s and will explore the X-ray polarization signatures from bright blazars. The All-Sky Medium Energy Gamma-Ray Observatory (AMEGO) mission,5 if selected, will be capable of detecting polarization from bright blazars in MeV $\gamma$-rays.

The X-ray to $\gamma$-ray blazar emissions can be polarized [51,108]. Since the optical and high-energy emissions are likely from the same emission region, they should share the same magnetic field morphology. In this situation, [52,107] have shown that the high-energy polarization degree may be detectable with high-energy polarimeters, which can distinguish the leptonic and hadronic models (see Figure 9 left). [109] has studied the temporal behavior of multi-wavelength polarization signatures. Their results have shown that the optical polarization variability can be very different from the high-energy counterparts, which may be used to identify the particle acceleration processes (see Figure 9 (right) for an example). Simultaneous optical and high-energy polarization measurements can examine these predictions.

5. Summary

Recent optical polarization monitoring programs have resulted in a rich understanding of polarization variability. In particular, the rare and extreme optical polarization angle swings accompanied by multi-wavelength flares have attracted a lot of attention in the AGN jet community. A number of geometric and physical models have been proposed to explain these phenomena, involving very different jet dynamics and physical processes. Better knowledge of the statistical trends of polarization variability will be essential to distinguish these models in future. Future optical polarimetry will play an essential part in the multi-messenger study of AGN jets. High-energy polarimetry will synergize with optical polarimetry to unveil the radiation and particle acceleration mechanisms in the most violent jet environment.