BCD Spectrophotometry and Rotation of Active B-Type Stars: Theory and Observations
Abstract
1. Introduction
2. B-Type Stars Observed with the BCD Spectrophotometric System
2.1. Brief Historical Account
2.2. Basics of the BCD system
2.3. Relation between the BCD Quantities and the Physical Parameters of Stars
2.4. The BCD System Today
2.5. Be Stars Observed with the BCD System
2.5.1. Main Characteristics of the Balmer Discontinuity in Be stars
2.5.2. The Apparent HR Diagram of Be Stars
2.5.3. Correlations of the BCD Parameters with the Emission Characteristics of Be Stars
2.5.4. Disc-Shaped Envelopes in Be Stars, Just a Few Reminders
2.5.5. What Can BCD-like Data Contribute to the Study of Be Star CDs?
2.6. BCD Parameters of B and Be Stars in Clusters
2.7. Bn Stars Observed with the BCD System
2.8. He-Weak and He-Strong Stars Observed with the BCD System
2.9. B[e] Stars Observed with the BCD System
3. Rotation of B-Type Stars: Theory and Observations
3.1. Introduction
3.2. Rigid against Differential Rotation; Some Theoretical Principles
3.2.1. Rigid Rotation of Homogeneous Bodies
3.2.2. Rigid Rotation of Polytropes with Index
3.2.3. Differential Rotation of Polytropes with Index
3.2.4. Barotropes and Baroclines
3.3. Geometry of Rotating Stars
3.4. On the Critical Rotation Rates
3.5. Notes on the Stellar Effective Mass, Total Energy Production and Age
3.6. Effects of the Rotation on the Emitted Radiation Field
3.7. ‘Apparent’ and ‘Parent Non-Rotating Counterpart’ Parameters
4. CNO Abundances and Stellar Rotation
5. Measurement of the Rotational Velocity in Early-Type Stars
5.1. Rotational Broadening in Gaia DR3
5.2. Caveats on the FT and ‘Spun-Up’-Based Methods
5.3. Uncertainties on the Related to the Non-Consideration of Velocity Fields in the Calculations of Radiation Transfer in Spectral Lines
5.4. Uncertainty on the Related with the Gravity Darkening
5.5. Effects on the Related with the Structure of Rotating Stellar Atmospheres
5.5.1. The Thermal Structure in Depth of Rotating Stellar Atmospheres
5.5.2. The Line Source Functions in a Gravity Darkened Atmosphere
5.6. Effects of the Microturbulence and the Macroturbulence on the Determination the Vsin i
5.7. Effects Carried by the Differential Rotation on the Value of Vsin i
5.8. Line Profiles Produced by Near-Critical Rigid Rotators
5.9. Double Valued Vsini
5.10. Asymmetrical Rotational Broadening Functions: Expanding Layers and tidal Interactions
6. Interferometry of Rotating Stars
7. The Origin of the Stellar Angular Momentum
7.1. First Findings and Discussions
7.2. Stellar Axial Rotation of Field and Cluster B and Be Stars
7.3. What about Be Stars?
7.4. Distribution of the Rotational Velocities of B and Be Stars
7.5. Rotational Velocities of B and Be Stars as a Function of the Metallicity
7.6. Two Different Visions on the Origin of the Stellar Angular Momentum
8. Understanding the Evolution of Rotational Velocities in Single Early-Type Stars
8.1. Models of Stellar Evolution with Rotation
8.2. Rigid or Differential Rotation: First Observational Guesses
8.3. Rigid or Differential Rotation: Extreme Behaviors
8.4. Rigid or Differential Rotation: Comparison of Models with Observations
8.5. Rigid or Differential Rotation: New Observational Inferences
9. Be and Bn Stars, Rapid Rotation Siblings?
9.1. Evolutionary Status of Be Stars
9.2. Distribution of Rotational Velocities of Bn Stars
9.3. Evolutionary Status of Bn Stars
9.4. Rotational Velocities of Be and Bn Stars in the ZAMS
10. Rotation of Magnetic B-Type Stars
11. Rotation of B[e] Stars
12. Concluding Remarks
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Chalonge, D. Étude du spectre continu de quelques étoiles entre 3100 et 4600 Å. J. Obs. 1936, 19, 149. [Google Scholar]
- Arnulf, A.; Barbier, D.; Chalonge, D.; Safir, H. Étude du rayonnement continu de quelques étoiles entre 3100 et 4600 Å (I). Annales d’Astrophysique 1938, 1, 293. [Google Scholar]
- Guérin, P. Réalisation et étude d’une lampe luminiscente étalon secondarie de répartition spectrale. In Contributions de l’Institut d’Astrophysique de Paris; CNRS; 1954; Volume 162, p. 1. [Google Scholar]
- Baillet, A.; Chalonge, D.; Divan, L. Quartz spectograph with oscillating plateholder for stellar spectrophotometry. Review of some applications. Nouvelle Revue d’Optique 1973, 4, 151–158. [Google Scholar] [CrossRef]
- Divan, L. List of Spectrophotometric Standards in Use at the Institut d’ASTROPHYSIQUE of Paris. In Spectral Classification and Multicolour Photometry: Proceedings of the IAU Symposium No. 24, Saltsjöbaden, Sweden, 17–21 August 1964; Loden, K., Loden, L.O., Sinnerstad, U., Eds.; Academic Press: London, UK, 1966; Volume 24, p. 311. [Google Scholar]
- Divan, L. Discussion: Notes on the definition of the Balmer discontinuity in the BCD system. In Proceedings of the Problems of Calibration of Absolute Magnitudes and Temperature of Stars, Geneva, Switzerland, 12–15 September 1972; Reidel: Dordrecht, The Netherlands; Boston, MA, USA, 1973; Volume 54, p. 267. [Google Scholar]
- Divan, L. Recherches sur la loi d’absorption de la poussière interstellaire et sur le spectre continu des étoiles O et B. Annales d’Astrophysique 1954, 17, 456. [Google Scholar]
- Chalonge, D.; Divan, L. La classification stellaire BCD: Paramètre caractéristique du type spectral calibration en magnitudes absolues. Astron. Astrophys. 1973, 23, 69. [Google Scholar]
- Barbier, D.; Chalonge, D. On the Continuous Spectrum of Stars with Extended Atmospheres. Astrophys. J. 1939, 90, 627–629. [Google Scholar] [CrossRef]
- Aidelman, Y.; Cidale, L.S.; Zorec, J.; Arias, M.L. Open clusters. I. Fundamental parameters of B stars in NGC 3766 and NGC 4755. Astron. Astrophys. 2012, 544, A64. [Google Scholar] [CrossRef]
- Allen, C.W. Astrophysical Quantities, 4th ed.; Springer: Berlin/Heidelberg, Germany, 2002. [Google Scholar]
- Barbier, D.; Chalonge, D. Étude du rayonnement continu de quelques étoiles entre 3 100 et 4 600 Å (4e Partie-discussion générale). Annales d’Astrophysique 1941, 4, 30. [Google Scholar]
- Chalonge, D.; Divan, L. Recherches sur les spectres continus stellaires. V. Etude du spectre continu de 150 etoiles entre 3150 et 4600 A. Annales d’Astrophysique 1952, 15, 201. [Google Scholar]
- Barbier, D. Introduction to the stellar classification based on the Balmer discontinuity. In Proceedings of the Frinciples of Stellar Classification, Paris, France, 29 June–4 July 1953; CNRS. 1955; p. 47. [Google Scholar]
- Chalonge, D. Classification using two or three parameters of early type stars. In Proceedings of the Frinciples of Stellar Classification, Paris, France, 29 June–4 July 1953; CNRS. 1955; p. 55. [Google Scholar]
- Keenan, P.C.; Morgan, W.W. Classification of Stellar Spectra. In Proceedings of the 50th Anniversary of the Yerkes Observatory and Half a Century of Progress in Astrophysics; Hynek, J.A., Ed.; McGraw-Hill: New York, NY, USA, 1951; p. 12. [Google Scholar]
- Underhill, A.B.; Divan, L.; Prevot-Burnichon, M.L.; Doazan, V. Effective temperatures, angular diameters, distances and linear radii for 160 O and B stars. Mon. Not. R. Astron. Soc. 1979, 189, 601–605. [Google Scholar] [CrossRef]
- Zorec, J.; Briot, D. Absolute magnitudes of B emission line stars: Correlation between the luminosity excess and the effective temperature. Astron. Astrophys. 1991, 245, 150. [Google Scholar]
- Zorec, J.; Cidale, L.; Arias, M.L.; Frémat, Y.; Muratore, M.F.; Torres, A.F.; Martayan, C. Fundamental parameters of B supergiants from the BCD system. I. Calibration of the (λ1, D) parameters into Teff. Astron. Astrophys. 2009, 501, 297–320. [Google Scholar] [CrossRef]
- Zorec, J. Thèse d’État, Structure et Rotation difféRentielle dans les Étoiles B avec et sans Émission; Université Paris VII: Paris, France, 1986. [Google Scholar]
- Husser, T.O.; Wende-von Berg, S.; Dreizler, S.; Homeier, D.; Reiners, A.; Barman, T.; Hauschildt, P.H. A new extensive library of PHOENIX stellar atmospheres and synthetic spectra. Astron. Astrophys. 2013, 553, A6. [Google Scholar] [CrossRef]
- Divan, L. Quantitative Spectral Classsification in the BCD System for LMC Supergiants. In Proceedings of the Spectral Classification and Multicolour Photometry, Villa Carlos Paz, Argentina, 18–24 October 1971; Reidel: Dordrecht, The Netherlands; Boston, MA, USA, 1973; Volume 50, p. 27. [Google Scholar]
- Divan, L. Calibration en Magnitudes Absolute de la Classification BCD. Application à la DéTERMINATION du Module de Distance du Grand Nuage de Magellan. In Proceedings of the Problems of Calibration of Absolute Magnitudes and Temperature of Stars, Geneva, Switzerland, 12–15 September 1972; Reidel: Dordrecht, The Netherlands; Boston, MA, USA, 1973; Volume 54, p. 78. [Google Scholar]
- Chalonge, D.; Divan, L. BCD classification: Relation between the spectral type and the effective temperature. Astron. Astrophys. 1977, 55, 121–124. [Google Scholar]
- Chalonge, D.; Divan, L. BCD system of stellar classification and chemical composition. Astron. Astrophys. 1977, 55, 117–120. [Google Scholar]
- Gkouvelis, L.; Fabregat, J.; Zorec, J.; Steeghs, D.; Drew, J.E.; Raddi, R.; Wright, N.J.; Drake, J.J. Physical parameters of IPHAS-selected classical Be stars. I. Determination procedure and evaluation of the results. Astron. Astrophys. 2016, 591, A140. [Google Scholar] [CrossRef]
- Divan, L.; Zorec, J. Absolute magnitudes and other basic parameters of O and B stars. In Proceedings of the The Scientific Aspects of the Hipparcos Space Astrometry Mission; Perryman, M.A.C., Guyenne, T.D., Høg, E., Jaschek, C., Lacroute, P., Eds.; ESA: Paris, France, 1982; Volume 177, pp. 101–104. [Google Scholar]
- Cidale, L.; Zorec, J.; Tringaniello, L. BCD spectrophotometry of stars with the B[e] phenomenon. I. Fundamental parameters. Astron. Astrophys. 2001, 368, 160–174. [Google Scholar] [CrossRef]
- Mennickent, R.E.; Cidale, L.; Díaz, M.; Pietrzyński, G.; Gieren, W.; Sabogal, B. Revealing the nature of double-periodic blue variables in the Magellanic Clouds. Mon. Not. R. Astron. Soc. 2005, 357, 1219–1230. [Google Scholar] [CrossRef]
- Zebrun, K.; Soszynski, I.; Wozniak, P.R.; Udalski, A.; Kubiak, M.; Szymanski, M.; Pietrzynski, G.; Szewczyk, O.; Wyrzykowski, L. The Optical Gravitational Lensing Experiment. Difference Image Analysis of LMC and SMC Data. The Catalog. Acta Astron. 2001, 51, 317–329. [Google Scholar]
- Witham, A.R.; Knigge, C.; Drew, J.E.; Greimel, R.; Steeghs, D.; Gänsicke, B.T.; Groot, P.J.; Mampaso, A. The IPHAS catalogue of Hα emission-line sources in the northern Galactic plane. Mon. Not. R. Astron. Soc. 2008, 384, 1277–1288. [Google Scholar] [CrossRef]
- Fabricant, D.; Cheimets, P.; Caldwell, N.; Geary, J. The FAST Spectrograph for the Tillinghast Telescope. Publ. Astron. Soc. Pac. 1998, 110, 79–85. [Google Scholar] [CrossRef]
- Shokry, A.; Rivinius, T.; Mehner, A.; Martayan, C.; Hummel, W.; Townsend, R.H.D.; Mérand, A.; Mota, B.; Faes, D.M.; Hamdy, M.A.; et al. Stellar parameters of Be stars observed with X-shooter. Astron. Astrophys. 2018, 609, A108. [Google Scholar] [CrossRef]
- Vernet, J.; Dekker, H.; D’Odorico, S.; Kaper, L.; Kjaergaard, P.; Hammer, F.; Randich, S.; Zerbi, F.; Groot, P.J.; Hjorth, J.; et al. X-shooter, the new wide band intermediate resolution spectrograph at the ESO Very Large Telescope. Astron. Astrophys. 2011, 536, A105. [Google Scholar] [CrossRef]
- Freudling, W.; Romaniello, M.; Bramich, D.M.; Ballester, P.; Forchi, V.; García-Dabló, C.E.; Moehler, S.; Neeser, M.J. Automated data reduction workflows for astronomy. The ESO Reflex environment. Astron. Astrophys. 2013, 559, A96. [Google Scholar] [CrossRef]
- Shokry, A.; Nouh, M.I.; Saad, S.M.; Helmy, I. Fundamental parameters of some B-type stars using NOAO Indo-U.S. Library. New Astron. 2022, 93, 101780. [Google Scholar] [CrossRef]
- Valdes, F.; Gupta, R.; Rose, J.A.; Singh, H.P.; Bell, D.J. The Indo-US Library of Coudé Feed Stellar Spectra. Astrophys. J. Suppl. Ser. 2004, 152, 251–259. [Google Scholar] [CrossRef]
- Jaschek, M.; Slettebak, A.; Jaschek, C. Be star terminology. Be Star Newsl. 1981, 4, 9–11. [Google Scholar]
- Collins, G.W., I. The Use of Terms and Definitions in the Study of be Stars (review Paper). In Proceedings of the 92nd Colloquium of the International Astronomical Union, Boulder, CO, USA, 18–22 August 1986; Cambridge University Press: Cambridge, UK, 1987; p. 3. [Google Scholar]
- Rivinius, T.; Carciofi, A.C.; Martayan, C. Classical Be stars. Rapidly rotating B stars with viscous Keplerian decretion disks. Astron. Astrophys. Rev. 2013, 21, 69. [Google Scholar] [CrossRef]
- Barbier, D.; Chalonge, D. Remarques préliminaires sur quelques propriétés de la discontinuité de Balmer dans les spectres stellaires. Annales d’Astrophysique 1939, 2, 254. [Google Scholar]
- Jaschek, M.; Groth, H.G. (Eds.) IAU Symp. 89: Be Stars; 1982; Volume 89. [Google Scholar]
- Divan, L.; Zorec, J. Behavior of the energy distribution of 59 CYG in the far-ultraviolet and in the visible. In Proceedings of the Third European IUE Conference, Madrid, Spain, 10–13 May 1982; European Space Agency: Paris, France, 1982; Volume 176, pp. 291–293. [Google Scholar]
- Hubert-Delplace, A.M.; Hubert, H. The recent peculiar Behaviour of the be star, HD 200120, 59 Cyg. Astron. Astrophys. 1981, 44, 109–113. [Google Scholar]
- Divan, L.; Zorec, J. BCD spectrophotometry of the Be-shell star 88 Her. In Proceedings of the Be Stars; Jaschek, M., Groth, H.G., Eds.; Cambridge University Press: Cambridge, UK, 1982; Volume 98, pp. 61–63. [Google Scholar]
- Doazan, V.; Marlborough, J.M.; Morossi, C.; Peters, G.J.; Rusconi, L.; Sedmak, G.; Stalio, R.; Thomas, R.N.; Willis, A. Ultraviolet and visual variability of theta CrB during a normal B-phase following a shell phase (1980-1985). Astron. Astrophys. 1986, 158, 1–13. [Google Scholar]
- Doazan, V.; Franco, M.; Rusconi, L.; Sedmak, G.; Stalio, R. The long-term variations of γ Cas in the visual. Astron. Astrophys. 1983, 128, 171–180. [Google Scholar]
- Hirata, R.; Kogure, T. The spectral variation of Pleione in 1969–1975. Publ. Astron. Soc. Jpn. 1976, 28, 509–515. [Google Scholar]
- Kogure, T.; Hirata, R. The Be star phenomena. I. General properties. Bull. Astron. Soc. India 1982, 10, 281–309. [Google Scholar]
- de Loore, C.; Altamore, A.; Baratta, G.B.; Bunner, A.N.; Divan, L.; Doazan, V.; Hensberge, H.; Sterken, C.; Viotti, R. First coordinated campaign of X-ray and ground-based observations of X-Persei = 3U 0352+30. Astron. Astrophys. 1979, 78, 287–291. [Google Scholar]
- Divan, L.; Zorec, J.; Andrillat, Y. A Be type variation in an O star. Astron. Astrophys. 1983, 126, L8–L10. [Google Scholar]
- Barbier, D. Le spectre continu de Gamma Cassiopeiae. Annales d’Astrophysique 1948, 11, 13. [Google Scholar]
- Barbier, D.; Chalonge, D.; Vassy, E. In Proceedings of the Journal de Physique. Société Française de Physique; 1935; Volume 6, p. 137. [Google Scholar]
- Chalonge, D.; Safir, H. Proceedings of the C.R. Acad. Sci. Paris; Elsevier of behalf of the French Academy of Sciences: Issy-les-Moulineaux, France, 1936; Volume 203, p. 1329.
- Barbier, D.; Chalonge, D. Sur le spectre continu de Nova Herculis 1934 dans la région de courtes longueurs d’onde. Annales d’Astrophysique 1940, 3, 26. [Google Scholar]
- Barbier, D.; Chalonge, D.; Canavaggia, R. Étude du rayonnement continu de quelques étoiles entre 3 100 et 4 600 Å-V: Nouvelles mesures de D et λ1. Annales d’Astrophysique 1947, 10, 195. [Google Scholar]
- Zorec, J.; Divan, L.; Hoeflich, P. Phase variations of 88 Herculis: Do the UV observations confirm a connection between these variations and the changes of the phospheric parameters of the underlying star? Astron. Astrophys. 1989, 210, 279–283. [Google Scholar]
- Divan, L. Quantitative Spectral Classification of STARS Stars on Low Dispersion Spectra. In Proceedings of the IAU Colloq. 47: Spectral Classification of the Future, Vatican City, 11–15 July 1978; Vatican Observatory: Albano laziale, Italy, 1979; Volume 9, p. 247. [Google Scholar]
- Mermilliod, J.C. Stellar content of young open clusters. II. Be stars. Astron. Astrophys. 1982, 109, 48–65. [Google Scholar]
- Schmidt-Kaler, T. Die galaktischen Emissions-B-Sterne: (Spectralklassifikation, Photometrie, Entwicklung und Verteilung in der Milchstraszenebene). Veroeffentlichungen Des Astron. Inst. Der Univ. Bonn 1964, 70, 1. [Google Scholar]
- Zorec, J.; Frémat, Y.; Cidale, L. On the evolutionary status of Be stars. I. Field Be stars near the Sun. Astron. Astrophys. 2005, 441, 235–248. [Google Scholar] [CrossRef]
- Hubert, A.M. Variability in the Circumstellar Envelope of Be Stars. In Proceedings of the Pulsation; Rotation; and Mass Loss in Early-Type Stars; Balona, L.A., Henrichs, H.F., Le Contel, J.M., Eds.; Kluwer Academic Publishers: New York, NY, USA, 1994; Volume 162, p. 341. [Google Scholar]
- Hubert, A.M.; Floquet, M.; Gomez, A.E.; Aletti, V. Photometric Variability of B and Be Stars. In Proceedings of the ESA Symposium ‘Hipparcos-Venice 97’, Venice, Italy, 13–16 May 1997; Volume 402, pp. 315–318. [Google Scholar]
- Moujtahid, A.; Hubert, A.M.; Zorec, J.; Ballereau, D.; Chauville, J.; Floquet, M.; Mon, M. Correlated Spectroscopic and Spectrophotometric Behaviours of Be Stars. In Proceedings of the IAU Colloq. 175: The Be Phenomenon in Early-Type Stars, Alicantem, Spain, 28 June–2 July 1999; The University of Chicago Press: Chicago, NY, USA, 2000; Volume 214, p. 514. [Google Scholar]
- Moujtahid, A.; Zorec, J.; Hubert, A.M. Long-term visual spectrophotometric behaviour of Be stars. II. Correlations with fundamental stellar parameters and interpretation. Astron. Astrophys. 1999, 349, 151–168. [Google Scholar]
- Aubourg, E.; Bareyre, P.; Brehin, S.; Gros, M.; de Kat, J.; Lachieze-Rey, M.; Laurent, B.; Lesquoy, E.; Magneville, C.; Milsztajn, A.; et al. Search for very low-mass objects in the Galactic Halo. Astron. Astrophys. 1995, 301, 1. [Google Scholar]
- de Wit, W.J.; Lamers, H.J.G.L.M.; Marquette, J.B.; Beaulieu, J.P. The remarkable light and colour variability of Small Magellanic Cloud Be stars. Astron. Astrophys. 2006, 456, 1027–1035. [Google Scholar] [CrossRef]
- Hubert, A.M.; Floquet, M. Investigation of the variability of bright Be stars using HIPPARCOS photometry. Astron. Astrophys. 1998, 335, 565–572. [Google Scholar]
- Mennickent, R.E.; Pietrzyński, G.; Gieren, W.; Szewczyk, O. On Be star candidates and possible blue pre-main sequence objects in the Small Magellanic Cloud. Astron. Astrophys. 2002, 393, 887–896. [Google Scholar] [CrossRef]
- Alcock, C.; Akerlof, C.W.; Allsman, R.A.; Axelrod, T.S.; Bennett, D.P.; Chan, S.; Cook, K.H.; Freeman, K.C.; Griest, K.; Marshall, S.L.; et al. Possible gravitational microlensing of a star in the Large Magellanic Cloud. Nature 1993, 365, 621–623. [Google Scholar] [CrossRef]
- Keller, S.C.; Bessell, M.S.; Cook, K.H.; Geha, M.; Syphers, D. Blue Variable Stars from the MACHO Database. I. Photometry and Spectroscopy of the Large Magellanic Cloud Sample. Astron. J. 2002, 124, 2039–2044. [Google Scholar] [CrossRef]
- Udalski, A.; Kubiak, M.; Szymanski, M. Optical Gravitational Lensing Experiment. OGLE-2—The Second Phase of the OGLE Project. Acta Astron. 1997, 47, 319–344. [Google Scholar]
- Limber, D.N.; Marlborough, J.M. The Support of the Envelopes of be Stars. Astrophys. J. 1968, 152, 181. [Google Scholar] [CrossRef]
- Limber, D.N. Circumstellar Envelopes Formed Through Rotationally Forced Ejection. II. Astrophys. J. 1967, 148, 141. [Google Scholar] [CrossRef]
- Marlborough, J.M. Models for the Envelopes of be Stars. Astrophys. J. 1969, 156, 135. [Google Scholar] [CrossRef]
- Poeckert, R.; Marlborough, J.M. Intrinsic linear polarization of Be stars as a function of V sini. Astrophys. J. 1976, 206, 182–195. [Google Scholar] [CrossRef]
- Poeckert, R.; Marlborough, J.M. Linear polarization of Halpha in the Be star Gamma Cassiopeiae. Astrophys. J. 1977, 218, 220–226. [Google Scholar] [CrossRef]
- Poeckert, R.; Marlborough, J.M. A model for gamma Cassiopeiae. Astrophys. J. 1978, 220, 940–961. [Google Scholar] [CrossRef]
- Poeckert, R.; Marlborough, J.M. Be star models: Observable effects of model parameters. Astrophys. J. Suppl. Ser. 1978, 38, 229–252. [Google Scholar] [CrossRef]
- Marlborough, J.M. Models for the Circumstellar Envelopes of be Stars (review Paper). In Proceedings of the Be and Shell Stars; Slettebak, A., Ed.; Reidel Pub. Co.: Dordrecht, The Netherlands; Boston, MA, USA, 1976; Volume 70, p. 335. [Google Scholar]
- Poeckert, R. Model atmospheres of Be stars. In Proceedings of the Be Stars; Jaschek, M., Groth, H.G., Eds.; Dordrecht, D. Reidel Publishing Co.: Dordrecht, The Netherlands; Boston, MA, USA, 1982; Volume 98, pp. 453–477. [Google Scholar]
- Hirata, R.; Kogure, T. The Be star phenomena. II—Spectral formation and structure of envelopes. Bull. Astron. Soc. India 1984, 12, 109–151. [Google Scholar]
- Slettebak, A.; Snow, T.P. Physics of Be stars. In Proceedings of the 92nd Colloquium of the International Astronomical Union, Boulder, CO, USA, 18–22 August 1986; University Press: Cambridge, UK, 1987. [Google Scholar]
- Waters, L.B.F.M.; Marlborough, J.M. The Structure of the Circumstellar Material in Be Stars. In Proceedings of the Pulsation; Rotation; and Mass Loss in Early-Type Stars; Balona, L.A., Henrichs, H.F., Le Contel, J.M., Eds.; Kluwer Academic Publishers: New York, NY, USA, 1994; Volume 162, p. 399. [Google Scholar]
- Doazan, V.; Stalio, R.; Thomas, R.N. Empirical atmospheric velocity patterns from combined IUE and visual observations: The Be-similar stars. In Proceedings of the Four Years of IUE Research, Conference Held in NASA, Goddard Space Flight Center, Greenbelt, MD, USA, 30 March–1 April 1982; NASA: Washington, DC, USA, 1982; Volume 2238, pp. 584–588. [Google Scholar]
- Underhill, A.B.; Doazan, V.; Lesh, J.R.; Aizenman, M.L.; Thomas, R.N. B Stars with and without Emission Lines. Monograph Series on Nonthermal Phenomena in Stellar Atmospheres; NASA: Washington, DC, USA, 1982; Volume 456. [Google Scholar]
- Thomas, R.N. Stellar Atmospheric Structural Patterns; NASA: Washington, DC, USA, 1983; Volume 471. [Google Scholar]
- Catala, C.; Kunasz, P.B.; Praderie, F. Line formation in the wind of AB Aur. Astron. Astrophys. 1984, 134, 402–413. [Google Scholar]
- Catala, C. Line formation in the winds of Herbig Ae/Be stars. The C IV resonancelines. Astron. Astrophys. 1988, 193, 222–228. [Google Scholar]
- Cidale, L.S.; Ringuelet, A.E. Rigorous Treatment of the Radiative Transfer Problem in Stellar Winds: Significance of the Velocity Law and the Chromosphere in the H alpha Profile. Astrophys. J. 1993, 411, 874. [Google Scholar] [CrossRef]
- Vazquez, A.C.; Cidale, L.S.; Ringuelet, A.E. Expanding Atmospheric Model Including a Chromosphere. I. Study of the Infrared Excess in Be Stars. Astrophys. J. 1993, 419, 286. [Google Scholar] [CrossRef]
- Cidale, L.S.; Vazquez, A.C. Expanding Atmospheric Model Including a Chromosphere. II. Be Stars: Center-to-Limb Variation of the Emergent Intensity. Astrophys. J. 1995, 453, 393. [Google Scholar] [CrossRef]
- Boehm, T.; Catala, C.; Donati, J.F.; Welty, A.; Baudrand, J.; Butler, C.J.; Carter, B.; Collier-Cameron, A.; Czarny, J.; Foing, B.; et al. Azimuthal structures in the wind and chromosphere of the Herbig AE star AB Aurigae. Results from the MUSICOS 1992 campaign. Astron. Astrophys. 1996, 120, 431–450. [Google Scholar] [CrossRef]
- Cidale, L.S. Diagnosis of Stellar Winds and Temperature Structures in Be Stars through the Analysis of Mg II Lines. Astrophys. J. 1998, 502, 824–832. [Google Scholar] [CrossRef]
- Bouret, J.C.; Catala, C. NLTE calculations of neutral helium lines in the wind of the Herbig Ae star AB Aurigae. Astron. Astrophys. 2000, 359, 1011–1024. [Google Scholar]
- Zorec, J.; Arias, M.L.; Cidale, L.; Ringuelet, A.E. Be star disc characteristics near the central object. Astron. Astrophys. 2007, 470, 239–247. [Google Scholar] [CrossRef]
- Hanuschik, R.W. Stellar V/sin/i and Optical Emission Linewidths in Be-Stars. Astrophys. Space Sci. 1989, 161, 61–73. [Google Scholar] [CrossRef]
- Ballereau, D.; Chauville, J.; Zorec, J. Some Fe II emission-line profiles of nine southern Be stars. Astron. Astrophys. 1995, 111, 457. [Google Scholar]
- Lee, U.; Osaki, Y.; Saio, H. Viscous excretion discs around Be stars. Mon. Not. R. Astron. Soc. 1991, 250, 432–437. [Google Scholar] [CrossRef]
- Shakura, N.I.; Sunyaev, R.A. Black holes in binary systems. Observational appearance. Astron. Astrophys. 1973, 24, 337–355. [Google Scholar]
- Pringle, J.E. Accretion discs in astrophysics. Annu. Rev. Astron. Astrophys. 1981, 19, 137–162. [Google Scholar] [CrossRef]
- Pringle, J.E. The properties of external accretion discs. Mon. Not. R. Astron. Soc. 1991, 248, 754. [Google Scholar] [CrossRef]
- Narita, S.; Kiguchi, M.; Hayashi, C. The Structure and Evolution of Thin Viscous Disks. I. Non-steady Accretion and Excretion. Publ. Astron. Soc. Jpn. 1994, 46, 575–587. [Google Scholar]
- Okazaki, A.T. Viscous Transonic Outflow in Equatorial Discs of Be Stars. Commun. Konkoly Obs. Hung. 1997, 100, 407–412. [Google Scholar]
- Okazaki, A.T. Viscous Transonic Decretion in Disks of Be Stars. Publ. Astron. Soc. Jpn. 2001, 53, 119–125. [Google Scholar] [CrossRef]
- Haubois, X.; Carciofi, A.C.; Rivinius, T.; Okazaki, A.T.; Bjorkman, J.E. Dynamical Evolution of Viscous Disks around Be Stars. I. Photometry. Astrophys. J. 2012, 756, 156. [Google Scholar] [CrossRef]
- Ghoreyshi, M.R.; Carciofi, A.C.; Jones, C.E.; Faes, D.M.; Baade, D.; Rivinius, T. A Multi-Observing Technique Study of the Dynamical Evolution of the Viscous Disk around the Be Star ω CMa. Astrophys. J. 2021, 909, 149. [Google Scholar] [CrossRef]
- Kurfürst, P.; Feldmeier, A.; Krtička, J. Two-dimensional modeling of density and thermal structure of dense circumstellar outflowing disks. Astron. Astrophys. 2018, 613, A75. [Google Scholar] [CrossRef]
- Carciofi, A.C.; Bjorkman, J.E. Non-LTE Monte Carlo Radiative Transfer. II. Nonisothermal Solutions for Viscous Keplerian Disks. Astrophys. J. 2008, 684, 1374–1383. [Google Scholar] [CrossRef]
- Millar, C.E.; Marlborough, J.M. Rates of Energy Gain and Loss in the Circumstellar Envelopes of Be Stars: The Poeckert-Marlborough Model. Astrophys. J. 1998, 494, 715–723. [Google Scholar] [CrossRef]
- Millar, C.E.; Marlborough, J.M. Rates of Energy Gain and Loss in the Circumstellar Envelopes of BE Stars: Diffuse Radiation. Astrophys. J. 1999, 516, 276–279. [Google Scholar] [CrossRef]
- Carciofi, A.C.; Bjorkman, J.E. Non-LTE Monte Carlo Radiative Transfer. I. The Thermal Properties of Keplerian Disks around Classical Be Stars. Astrophys. J. 2006, 639, 1081–1094. [Google Scholar] [CrossRef]
- Sigut, T.A.A.; Jones, C.E. The Thermal Structure of the Circumstellar Disk Surrounding the Classical Be Star γ Cassiopeiae. Astrophys. J. 2007, 668, 481–491. [Google Scholar] [CrossRef]
- McGill, M.A.; Sigut, T.A.A.; Jones, C.E. The Effect of Density on the Thermal Structure of Gravitationally Darkened Be Star Disks. Astrophys. J. Suppl. Ser. 2013, 204, 2. [Google Scholar] [CrossRef]
- Thomas, R.N. The Source Function in a Non-Equilibrium Atmosphere. I. The Resonance Lines. Astrophys. J. 1957, 125, 260. [Google Scholar] [CrossRef]
- Thomas, R.N. Some Aspects of Non-Equilibrium Thermodynamics in the Presence of a Radiation Field; University of Colorado Press: Boulder, CO, USA, 1965. [Google Scholar]
- Jefferies, J.T. Spectral Line Formation; Blaisdell: Waltham, MA, USA, 1968. [Google Scholar]
- Mihalas, D. Stellar Atmospheres; W.H. Freeman: San Francisco, CA, USA, 1978. [Google Scholar]
- Hubeny, I.; Mihalas, D. Theory of Stellar Atmospheres; Princeton University Press: Princeton, NJ, USA, 2014. [Google Scholar]
- Viotti, R. Forbidden and permitted emission lines of singly ionized iron as a diagnostic in the investigation of stellar emission-line spectra. Astrophys. J. 1976, 204, 293–300. [Google Scholar] [CrossRef]
- Curé, M. The Influence of Rotation in Radiation-driven Wind from Hot Stars: New Solutions and Disk Formation in Be Stars. Astrophys. J. 2004, 614, 929–941. [Google Scholar] [CrossRef]
- Hartquist, T.W.; Dyson, J.E.; Pettini, M.; Smith, L.J. Mass-loaded astronomical flows—I. General principles and their application to RCW 58. Mon. Not. R. Astron. Soc. 1986, 221, 715–726. [Google Scholar] [CrossRef]
- Dyson, J.E.; Hartquist, T.W. Astronomical Bubbles with Clumpy Cores and Accelerating Haloes. Astrophys. Lett. Commun. 1992, 28, 301. [Google Scholar]
- Arthur, S.J.; Dyson, J.E.; Hartquist, T.W. Mass-loaded flows—VII. Transonic flows from the cores of planetary nebulae. Mon. Not. R. Astron. Soc. 1994, 269, 1117–1122. [Google Scholar] [CrossRef][Green Version]
- Kroll, P.; Hanuschik, R.W. Dynamics of Self-Accreting Disks in Be Stars. In Proceedings of the IAU Colloq. 163: Accretion Phenomena and Related Outflows, Port Douglas, Queensland, Australia, 15–19 July 1996; Astronomical Society of the Pacific: San Francisco, CA, USA, 1997; Volume 121, p. 494. [Google Scholar]
- Kee, N.D.; Owocki, S.; Townsend, R.; Müller, H.R. Pulsational Mass Ejection in Be Star Disks. In Proceedings of the Bright Emissaries: Be Stars as Messengers of Star-Disk Physics; Sigut, T.A.A., Jones, C.E., Eds.; Astronomical Society of the Pacific: San Francisco, CA, USA, 2016; Volume 506, p. 47. [Google Scholar]
- Zorec, J.; Frémat, Y.; Domiciano de Souza, A.; Royer, F.; Cidale, L.; Hubert, A.M.; Semaan, T.; Martayan, C.; Cochetti, Y.R.; Arias, M.L.; et al. Critical study of the distribution of rotational velocities of Be stars. I. Deconvolution methods, effects due to gravity darkening, macroturbulence, and binarity. Astron. Astrophys. 2016, 595, A132. [Google Scholar] [CrossRef]
- Krtička, J.; Owocki, S.P.; Meynet, G. Mass and angular momentum loss via decretion disks. Astron. Astrophys. 2011, 527, A84. [Google Scholar] [CrossRef]
- Granada, A.; Ekström, S.; Georgy, C.; Krtička, J.; Owocki, S.; Meynet, G.; Maeder, A. Populations of rotating stars. II. Rapid rotators and their link to Be-type stars. Astron. Astrophys. 2013, 553, A25. [Google Scholar] [CrossRef]
- Wade, G.A.; Neiner, C.; Alecian, E.; Grunhut, J.H.; Petit, V.; Batz, B.d.; Bohlender, D.A.; Cohen, D.H.; Henrichs, H.F.; Kochukhov, O.; et al. The MiMeS survey of magnetism in massive stars: Introduction and overview. Mon. Not. R. Astron. Soc. 2016, 456, 2–22. [Google Scholar] [CrossRef]
- Wade, G.A.; Petit, V.; Grunhut, J.H.; Neiner, C.; MiMeS Collaboration. Magnetic Fields of Be Stars: Preliminary Results from a Hybrid Analysis of the MiMeS Sample. In Proceedings of the Bright Emissaries: Be Stars as Messengers of Star-Disk Physics, Astronomical Society of the Pacific: Proceedings of a Meeting held at The University of Western Ontario, London, ON, Canada, 11–13 August 2014; Astronomical Society of the Pacific Conference Series; Sigut, T.A.A., Jones, C.E., Eds.; 2016; Volume 506, p. 207. [Google Scholar]
- Kochukhov, O.; Sudnik, N. Detectability of small-scale magnetic fields in early-type stars. Astron. Astrophys. 2013, 554, A93. [Google Scholar] [CrossRef]
- Cantiello, M.; Langer, N.; Brott, I.; de Koter, A.; Shore, S.N.; Vink, J.S.; Voegler, A.; Lennon, D.J.; Yoon, S.C. Sub-surface convection zones in hot massive stars and their observable consequences. Astron. Astrophys. 2009, 499, 279–290. [Google Scholar] [CrossRef]
- Cantiello, M.; Braithwaite, J. Magnetic spots on hot massive stars. Astron. Astrophys. 2011, 534, A140. [Google Scholar] [CrossRef]
- Cantiello, M.; Braithwaite, J. Envelope Convection, Surface Magnetism, and Spots in A and Late B-type Stars. Astrophys. J. 2019, 883, 106. [Google Scholar] [CrossRef]
- Maeder, A.; Georgy, C.; Meynet, G. Convective envelopes in rotating OB stars. Astron. Astrophys. 2008, 479, L37–L40. [Google Scholar] [CrossRef]
- Clement, M.J. On the equilibrium and secular instability of rapidly rotating stars. Astrophys. J. 1979, 230, 230–242. [Google Scholar] [CrossRef]
- Smith, M.A.; Lopes de Oliveira, R.; Motch, C. A Census of the Class of X-ray Active γ Cas Stars. In Proceedings of the Bright Emissaries: Be Stars as Messengers of Star-Disk Physics, Astronomical Society of the Pacific: Proceedings of a Meeting held at The University of Western Ontario, London, ON, Canada, 11–13 August 2014; Astronomical Society of the Pacific Conference Series; Sigut, T.A.A., Jones, C.E., Eds.; 2016; Volume 506, p. 299. [Google Scholar]
- Smith, M.A.; Lopes de Oliveira, R.; Motch, C. The X-ray emission of the γ Cassiopeiae stars. Adv. Space Res. 2016, 58, 782–808. [Google Scholar] [CrossRef]
- Owocki, S.P.; Castor, J.I.; Rybicki, G.B. Time-dependent Models of Radiatively Driven Stellar Winds. I. Nonlinear Evolution of Instabilities for a Pure Absorption Model. Astrophys. J. 1988, 335, 914. [Google Scholar] [CrossRef]
- Zorec, J.; Frémat, Y.; Domiciano de Souza, A.; Delaa, O.; Stee, P.; Mourard, D.; Cidale, L.; Martayan, C.; Georgy, C.; Ekström, S. Differential rotation in rapidly rotating early-type stars. I. Motivations for combined spectroscopic and interferometric studies. Astron. Astrophys. 2011, 526, A87. [Google Scholar] [CrossRef]
- Apparao, K.M.V.; Antia, H.M.; Chitre, S.M. Rapidly rotating stars and the Be star phenomenon. Astron. Astrophys. 1987, 177, 198–200. [Google Scholar]
- Aidelman, Y.; Cidale, L.S.; Zorec, J.; Panei, J.A. Open clusters. II. Fundamental parameters of B stars in Collinder 223, Hogg 16, NGC 2645, NGC 3114, and NGC 6025. Astron. Astrophys. 2015, 577, A45. [Google Scholar] [CrossRef]
- Aidelman, Y.; Cidale, L.S.; Zorec, J.; Panei, J.A. Open clusters. III. Fundamental parameters of B stars in NGC 6087, NGC 6250, NGC 6383, and NGC 6530 B-type stars with circumstellar envelopes. Astron. Astrophys. 2018, 610, A30. [Google Scholar] [CrossRef]
- Ekström, S.; Georgy, C.; Eggenberger, P.; Meynet, G.; Mowlavi, N.; Wyttenbach, A.; Granada, A.; Decressin, T.; Hirschi, R.; Frischknecht, U.; et al. Grids of stellar models with rotation. I. Models from 0.8 to 120 M⊙ at solar metallicity (Z = 0.014). Astron. Astrophys. 2012, 537, A146. [Google Scholar] [CrossRef]
- Cochetti, Y.R.; Zorec, J.; Cidale, L.S.; Arias, M.L.; Aidelman, Y.; Torres, A.F.; Frémat, Y.; Granada, A. Be and Bn stars: Balmer discontinuity and stellar-class relationship. Astron. Astrophys. 2020, 634, A18. [Google Scholar] [CrossRef]
- Hoffleit, D.; Jaschek, C. The Bright Star Catalogue. Fourth Revised Edition. (Containing Data Compiled through 1979); Yale University Observatory: New Haven, CT, USA, 1982. [Google Scholar]
- Hoffleit, D.; Saladyga, M.; Wlasuk, P. A Supplement to the Bright Star Catalogue. Containing Data Compiled through 1981 for Stars 7.10 V and Brighter That Are Not in the Bright Star Catalogue; Yale University Observatory: New Haven, CT, USA, 1983. [Google Scholar]
- Zorec, J. On the Initial Mass Function of Be Stars and the Missing Be Stars of Late Spectral Types. In Proceedings of the IAU Colloq. 175: The Be Phenomenon in Early-Type Stars, Alicante, Spain, 28 June–2 July 1999; The University of Chicago Press: Chicago, NY, USA, 2000; Volume 214, p. 51. [Google Scholar]
- Scalo, J.M. The Stellar Initial Mass Function. Fund. Cosmic Phys. 1986, 11, 1–278. [Google Scholar]
- Rana, N.C. Mass function of stars in the solar neighbourhood. Astron. Astrophys. 1987, 184, 104–118. [Google Scholar]
- Zorec, J.; Briot, D. Critical study of the frequency of Be stars taking into account their outstanding characteristics. Astron. Astrophys. 1997, 318, 443–460. [Google Scholar]
- Zorec, J. Rotation and Properties of Be Stars (Invited Review). In Proceedings of the Stellar Rotation; Maeder, A., Eenens, P., Eds.; Cambridge University Press: Cambridge, UK, 2004; Volume 215, p. 73. [Google Scholar]
- Maeder, A.; Meynet, G. The Evolution of Rotating Stars. Annu. Rev. Astron. Astrophys. 2000, 38, 143–190. [Google Scholar] [CrossRef]
- Cote, J.; van Kerkwijk, M.H. New bright Be stars and the Be star frequency. Astron. Astrophys. 1993, 274, 870–876. [Google Scholar]
- Ghosh, K.K.; Apparao, K.M.V.; Pukalenthi, S. Observations of BN and AN stars: New Be stars. Astron. Astrophys. 1999, 134, 359–364. [Google Scholar] [CrossRef][Green Version]
- De Marco, O.; Lanz, T.; Ouellette, J.A.; Zurek, D.; Shara, M.M. First Evidence of Circumstellar Disks around Blue Straggler Stars. Astrophys. J. Lett. 2004, 606, L151–L154. [Google Scholar] [CrossRef][Green Version]
- Porter, J.M.; Townsend, R.H.D. On the Evidence of Disks around Blue Straggler Stars. Astrophys. J. Lett. 2005, 623, L129–L132. [Google Scholar] [CrossRef][Green Version]
- Jaschek, C.; Jaschek, M. The Classification of Stars; Cambridge University Press: Cambridge, UK, 1987. [Google Scholar]
- Osmer, P.S.; Peterson, D.M. The composition and evolutionary status of the helium-rich stars. Astrophys. J. 1974, 187, 117–129. [Google Scholar] [CrossRef]
- Cidale, L.S.; Arias, M.L.; Torres, A.F.; Zorec, J.; Frémat, Y.; Cruzado, A. Fundamental parameters of He-weak and He-strong stars. Astron. Astrophys. 2007, 468, 263–272. [Google Scholar] [CrossRef]
- Hubeny, I.; Lanz, T. Non-LTE Line-blanketed Model Atmospheres of Hot Stars. I. Hybrid Complete Linearization/Accelerated Lambda Iteration Method. Astrophys. J. 1995, 439, 875. [Google Scholar] [CrossRef]
- Dimitrijevic, M.S.; Sahal-Brechot, S. Stark broadening of neutral helium lines. J. Quant. Spectrosc. Radiat. Transf. 1984, 31, 301–313. [Google Scholar] [CrossRef]
- Dimitrijevic, M.S.; Sahal-Brechot, S. Stark broadening of He I lines. Astron. Astrophys. 1990, 82, 519–529. [Google Scholar]
- Freudenstein, S.A.; Cooper, J. A simple formula for estimating Stark widths of neutral lines. Astrophys. J. 1978, 224, 1079–1084. [Google Scholar] [CrossRef]
- Allen, D.A.; Swings, J.P. Infrared Excesses and Forbidden Emission Lines in Early-Type Stars. Astrophys. Lett. 1972, 10, 83. [Google Scholar]
- Allen, D.A.; Swings, J.P. The spectra of peculiar Be star with infrared excesses. Astron. Astrophys. 1976, 47, 293–302. [Google Scholar]
- Zickgraf, F.J. Current Definition of B[e] Stars. In Proceedings of the B[e] Stars; Hubert, A.M., Jaschek, C., Eds.; Kluwer Academic Publishers: Dordrecht, The Netherlands; Boston, MA, USA, 1998; Volume 233, p. 1. [Google Scholar] [CrossRef]
- Lamers, H.J.G.L.M.; Zickgraf, F.J.; de Winter, D.; Houziaux, L.; Zorec, J. An improved classification of B[e]-type stars. Astron. Astrophys. 1998, 340, 117–128. [Google Scholar]
- Zickgraf, F.J.; Wolf, B.; Stahl, O.; Leitherer, C.; Klare, G. The hybrid spectrum of the LMC hypergiant R 126. Astron. Astrophys. 1985, 143, 421–430. [Google Scholar]
- Zickgraf, F.J.; Wolf, B.; Stahl, O.; Leitherer, C.; Appenzeller, I. B(e)-supergiants of the Magellanic Clouds. Astron. Astrophys. 1986, 163, 119–134. [Google Scholar]
- Zickgraf, F.J.; Wolf, B.; Stahl, O.; Humphreys, R.M. S 18: A new B(e) supergiant in the Small Magellanic Cloud with evidence for an excretion disk. Astron. Astrophys. 1989, 220, 206–214. [Google Scholar]
- Zickgraf, F.J.; Stahl, O.; Wolf, B. IR survey of OB emission-line stars in the SMC: Detection of a new B E supergiant, AV 172. Astron. Astrophys. 1992, 260, 205–212. [Google Scholar]
- Zickgraf, F.J.; Kovacs, J.; Wolf, B.; Stahl, O.; Kaufer, A.; Appenzeller, I. R4 in the Small Magellanic Cloud: A spectroscopic binary with a B[e]/LBV-type component. Astron. Astrophys. 1996, 309, 505–514. [Google Scholar]
- Zickgraf, F.J.; Humphreys, R.M.; Lamers, H.J.G.L.M.; Smolinski, J.; Wolf, B.; Stahl, O. Spectroscopic study of the outflowing disk winds of B[e] supergiants in the Magellanic Clouds. Astron. Astrophys. 1996, 315, 510–520. [Google Scholar]
- Gummersbach, C.A.; Zickgraf, F.J.; Wolf, B. B[e] phenomenon extending to lower luminosities in the Magellanic Clouds. Astron. Astrophys. 1995, 302, 409. [Google Scholar]
- Wolf, B.; Stahl, O. The absorption spectrum of the Be star MWC 300. Astron. Astrophys. 1985, 148, 412–416. [Google Scholar]
- McGregor, P.J.; Hyland, A.R.; Hillier, D.J. Atomic and Molecular Line Emission from Early-Type High-Luminosity Stars. Astrophys. J. 1988, 324, 1071. [Google Scholar] [CrossRef]
- Winkler, H.; Wolf, B. An analysis of high resolution spectra of the Be -stars CPD -52 9243and MWC 300. Astron. Astrophys. 1989, 219, 151–157. [Google Scholar]
- Kraus, M. A Census of B[e] Supergiants. Galaxies 2019, 7, 83. [Google Scholar] [CrossRef]
- Palla, F.; Stahler, S.W. The Pre-Main-Sequence Evolution of Intermediate-Mass Stars. Astrophys. J. 1993, 418, 414. [Google Scholar] [CrossRef]
- Bibo, E.A.; The, P.S. The type of variability of Herbig Ae/Be stars. Astron. Astrophys. 1991, 89, 319. [Google Scholar]
- Ciatti, F.; D’Odorico, S.; Mammano, A. Properties and evolution of BQ[] stars. Astron. Astrophys. 1974, 34, 181–186. [Google Scholar]
- Kenyon, S.J. The Symbiotic Stars; Cambridge University Press: Cambridge, UK, 1986. [Google Scholar]
- Miroshnichenko, A.S. Toward Understanding the B[e] Phenomenon. I. Definition of the Galactic FS CMa Stars. Astrophys. J. 2007, 667, 497–504. [Google Scholar] [CrossRef][Green Version]
- Miroshnichenko, A.S.; Manset, N.; Kusakin, A.V.; Chentsov, E.L.; Klochkova, V.G.; Zharikov, S.V.; Gray, R.O.; Grankin, K.N.; Gandet, T.L.; Bjorkman, K.S.; et al. Toward Understanding the B[e] Phenomenon. II. New Galactic FS CMa Stars. Astrophys. J. 2007, 671, 828–841. [Google Scholar] [CrossRef]
- Kraus, M. The pre- versus post-main sequence evolutionary phase of B[e] stars. Constraints from 13CO band emission. Astron. Astrophys. 2009, 494, 253–262. [Google Scholar] [CrossRef]
- Kraus, M.; Liimets, T.; Moiseev, A.; Sánchez Arias, J.P.; Nickeler, D.H.; Cidale, L.S.; Jones, D. Resolving the Circumstellar Environment of the Galactic B[e] Supergiant Star MWC 137.II. Nebular Kinematics and Stellar Variability. Astron. J. 2021, 162, 150. [Google Scholar] [CrossRef]
- Barsukova, E.A.; Burenkov, A.N.; Goranskij, V.P. Sudden strengthening of He II emission line in the spectrum of B[e] star CI Cam. Astron. Telegr. 2021, 14362, 1. [Google Scholar]
- Smith, D.; Remillard, R.; Swank, J.; Takeshima, T.; Smith, E. XTE J0421+560. IAU Circ. 1998, 6855, 1. [Google Scholar]
- Korčáková, D.; Sestito, F.; Manset, N.; Kroupa, P.; Votruba, V.; Šlechta, M.; Danford, S.; Dvořáková, N.; Raj, A.; Chojnowski, S.D.; et al. First detection of a magnetic field in low-luminosity B[e] stars. New scenarios for the nature and evolutionary stages of FS CMa stars. Astron. Astrophys. 2022, 659, A35. [Google Scholar] [CrossRef]
- Aidelman, Y.; Cidale, L.S.; Kraus, M. Aias, M.L.; Zorec, J. Fundamental parameters of B[e] stars. Astron. Astrophys. 2022. submitted. [Google Scholar]
- Arias, M.L.; Cidale, L.S.; Kraus, M.; Torres, A.F.; Aidelman, Y.; Zorec, J.; Granada, A. Near-infrared Spectra of a Sample of Galactic Unclassified B[e] Stars. Publ. Astron. Soc. Pac. 2018, 130, 114201. [Google Scholar] [CrossRef]
- Burnichon, M.L.; Chalonge, D.; Divan, L.; Swings, L. Etude de l’etoile Be HD 45677. J. Obs. 1967, 50, 391. [Google Scholar]
- Merrill, P.W. Bright iron lines in the spectrum of HD 45677. Astrophys. J. 1928, 67, 405–408. [Google Scholar] [CrossRef]
- Swings, P.; Struve, O. Spectrographic Observations of Peculiar Stars. Astrophys. J. 1940, 91, 546. [Google Scholar] [CrossRef]
- Swings, P.; Struve, O. Spectrographic Observations of Peculiar Stars.VI. Astrophys. J. 1943, 98, 91. [Google Scholar] [CrossRef]
- Zorec, J.; Moujtahid, A.; Ballereau, D.; Chauville, J. Fundamental Parameters of Two B[e] Stars: HD 45677 and HD 50138. In Proceedings of the B[e] Stars; Hubert, A.M., Jaschek, C., Eds.; Kluwer Academic Publishers: Dordrecht, The Netherlands; Boston, MA, USA, 1998; Volume 233, p. 55. [Google Scholar] [CrossRef]
- Zorec, J. Distances, Kinematics and Distribution of B[e] Stars in Our Galaxy. In Proceedings of the B[e] Stars; Hubert, A.M., Jaschek, C., Eds.; Kluwer Academic Publishers: Dordrecht, The Netherlands; Boston, MA, USA, 1998; Volume 233, p. 27. [Google Scholar] [CrossRef]
- Moujtahid, A.; Zorec, J.; Hubert, A.M. Ultraviolet Extinction of Circumstellar Dust in the B[e] Star HD 45677. In Proceedings of the Ultraviolet Astrophysics Beyond the IUE Final Archive; Wamsteker, W., Gonzalez Riestra, R., Harris, B., Eds.; ESA Publications Division: Noordwijk, The Netherlands, 1998; Volume 413, p. 261. [Google Scholar]
- Grinin, V.P.; Kiselev, N.N.; Minikulov, N.K. Observations of “zodiacal light” of the isolated Herbig Ae-star BF Ori. Pisma v Astronomicheskii Zhurnal 1989, 15, 1028–1038. [Google Scholar]
- Grinin, V.P.; Kiselev, N.N.; Minikulov, N.K.; Chernova, G.P.; Voshchinnikov, N.V. The investigations of ‘zodiacal light’ of isolated AE-Herbig stars with non-periodic Algol-type minima. Astrophys. Space Sci. 1991, 186, 283–298. [Google Scholar] [CrossRef]
- Jeřábková, T.; Korčáková, D.; Miroshnichenko, A.; Danford, S.; Zharikov, S.V.; Kříček, R.; Zasche, P.; Votruba, V.; Šlechta, M.; Škoda, P.; et al. Time-dependent spectral-feature variations of stars displaying the B[e] phenomenon. III. HD 50138. Astron. Astrophys. 2016, 586, A116. [Google Scholar] [CrossRef][Green Version]
- Kluska, J.; Benisty, M.; Soulez, F.; Berger, J.P.; Le Bouquin, J.B.; Malbet, F.; Lazareff, B.; Thiébaut, E. A disk asymmetry in motion around the B[e] star MWC158. Astron. Astrophys. 2016, 591, A82. [Google Scholar] [CrossRef]
- Miroshnichenko, A.S.; Zharikov, S.V.; Danford, S.; Manset, N.; Korčáková, D.; Kříček, R.; Šlechta, M.; Omarov, C.T.; Kusakin, A.V.; Kuratov, K.S.; et al. Toward Understanding the B[e] Phenomenon. V. Nature and Spectral Variations of the MWC 728 Binary System. Astrophys. J. 2015, 809, 129. [Google Scholar] [CrossRef]
- Miroshnichenko, A.S.; Bernabei, S.; Polcaro, V.F.; Viotti, R.F.; Norci, L.; Manset, N.; Klochkova, V.G.; Rudy, R.J.; Lynch, D.K.; Venturini, C.C.; et al. Optical and Near-IR Observations of the B[e] Star AS 119. In Proceedings of the Stars with the B[e] Phenomenon; Kraus, M., Miroshnichenko, A.S., Eds.; University of Chicago Press: Vlieland, The Netherlands, 2006; Volume 355, p. 347. [Google Scholar]
- Polster, J.; Korčáková, D.; Manset, N. Time-dependent spectral-feature variations of stars displaying the B[e] phenomenon. IV. V2028 Cygni: Modelling of Hα bisector variability. Astron. Astrophys. 2018, 617, A79. [Google Scholar] [CrossRef]
- Zickgraf, F.J.; Schulte-Ladbeck, R.E. Polarization characteristics of galactic Be stars. Astron. Astrophys. 1989, 214, 274–284. [Google Scholar]
- Condori, C.A.H.; Borges Fernandes, M.; Kraus, M.; Panoglou, D.; Guerrero, C.A. The study of unclassified B[e] stars and candidates in the Galaxy and Magellanic Clouds†. Mon. Not. R. Astron. Soc. 2019, 488, 1090–1110. [Google Scholar] [CrossRef]
- Langer, N.; Kudritzki, R.P. The spectroscopic Hertzsprung-Russell diagram. Astron. Astrophys. 2014, 564, A52. [Google Scholar] [CrossRef]
- Chentsov, E.L.; Klochkova, V.G.; Miroshnichenko, A.S. Spectral variability of the peculiar A-type supergiant 3Pup. Astrophys. Bull. 2010, 65, 150–163. [Google Scholar] [CrossRef]
- Miroshnichenko, A.S.; Danford, S.; Zharikov, S.V.; Klochkova, V.G.; Chentsov, E.L.; Vanbeveren, D.; Zakhozhay, O.V.; Manset, N.; Pogodin, M.A.; Omarov, C.T.; et al. Properties of Galactic B[e] Supergiants. V. 3 Pup-Constraining the Orbital Parameters and Modeling the Circumstellar Environments. Astrophys. J. 2020, 897, 48. [Google Scholar] [CrossRef]
- Langer, N.; Heger, A. B[e] Supergiants: What is Their Evolutionary Status? In Proceedings of the B[e] Stars; Hubert, A.M., Jaschek, C., Eds.; Kluwer Academic Publishers: Dordrecht, The Netherlands; Boston, MA, USA, 1998; Volume 233, p. 235. [Google Scholar]
- Podsiadlowski, P.; Morris, T.S.; Ivanova, N. Massive Binary Mergers: A Unique Scenario for the sgB[e] Phenomenon? In Proceedings of the Stars with the B[e] Phenomenon; Kraus, M., Miroshnichenko, A.S., Eds.; University of Chicago Press: Vlieland, The Netherlands, 2006; Volume 355, p. 259. [Google Scholar]
- de Mink, S.E.; Langer, N.; Izzard, R.G.; Sana, H.; de Koter, A. The Rotation Rates of Massive Stars: The Role of Binary Interaction through Tides, Mass Transfer, and Mergers. Astrophys. J. 2013, 764, 166. [Google Scholar] [CrossRef]
- de Mink, S.E.; Sana, H.; Langer, N.; Izzard, R.G.; Schneider, F.R.N. The Incidence of Stellar Mergers and Mass Gainers among Massive Stars. Astrophys. J. 2014, 782, 7. [Google Scholar] [CrossRef]
- Zickgraf, F.J. Discussion Session: Is there an Evolutionary Link between B[e] Supergiants and LBVs? In Proceedings of the Stars with the B[e] Phenomenon; Kraus, M., Miroshnichenko, A.S., Eds.; University of Chicago Press: Vlieland, The Netherlands, 2006; Volume 355, p. 211. [Google Scholar]
- Meynet, G.; Maeder, A. Single Massive Stars at the Critical Rotational Velocity: Possible Links with Be and B[e] Stars. In Proceedings of the Stars with the B[e] Phenomenon; Kraus, M., Miroshnichenko, A.S., Eds.; University of Chicago Press: Vlieland, The Netherlands, 2006; Volume 355, p. 27. [Google Scholar]
- Georgy, C.; Saio, H.; Ekström, S.; Meynet, G. The Advanced Stages of Stellar Evolution: Impact of Mass Loss, Rotation, and Link With B[e] Stars. In Proceedings of the The B[e] Phenomenon: Forty Years of Studies; Miroshnichenko, A., Zharikov, S., Korčáková, D., Wolf, M., Eds.; Insititut of Physics Publishing: Prague, Czech Republic, 2017; Volume 508, p. 99. [Google Scholar]
- Cox, J.P.; Giuli, R.T. Principles of Stellar Structure; Gordon and Breach: New York, NY, USA, 1968. [Google Scholar]
- Sweet, P.A. The importance of rotation in stellar evolution. Mon. Not. R. Astron. Soc. 1950, 110, 548. [Google Scholar] [CrossRef]
- Zahn, J.P. Instability and Mixing Processes in Upper Main Sequence Stars. In Proceedings of the Saas-Fee Advanced Course 13: Astrophysical Processes in Upper Main Sequence Stars; Cox, A.N., Vauclair, S., Zahn, J.P., Eds.; Geneva Observatory: Sauverny, France, 1983; p. 253. [Google Scholar]
- Zahn, J.P. Circulation and turbulence in rotating stars. Astron. Astrophys. 1992, 265, 115–132. [Google Scholar]
- Ferraro, V.C.A. The non-uniform rotation of the Sun and its magnetic field. Mon. Not. R. Astron. Soc. 1937, 97, 458. [Google Scholar] [CrossRef]
- Ferraro, V.C.A.; Plumpton, C. An Introduction to Magneto-Fluid Mechanics. J. Plasma Phys. 1967, 1, 499. [Google Scholar] [CrossRef]
- Spruit, H.C. Differential rotation and magnetic fields in stellar interiors. Astron. Astrophys. 1999, 349, 189–202. [Google Scholar]
- Spruit, H.C. Dynamo action by differential rotation in a stably stratified stellar interior. Astron. Astrophys. 2002, 381, 923–932. [Google Scholar] [CrossRef]
- Spruit, H.C. Angular Momentum Transport and Mixing by Magnetic Fields (Invited Review). In Proceedings of the Stellar Rotation; Maeder, A., Eenens, P., Eds.; 2004; Volume 215, p. 356. [Google Scholar]
- Maeder, A. Physics, Formation and Evolution of Rotating Stars; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar] [CrossRef]
- Fujimoto, M.Y. Dynamical stability of differentially rotating bodies to non-axisymmetric perturbations. Astron. Astrophys. 1987, 176, 53–58. [Google Scholar]
- Tassoul, J.L. Theory of Rotating Stars; University Press: Princeton, NJ, USA, 1978. [Google Scholar]
- Chandrasekhar, S. Ellipsoidal Figures of Equilibrium; New Haven Yale University Press: New Haven, CT, USA, 1969. [Google Scholar]
- Kippenhahn, R.; Meyer-Hofmeister, E.; Thomas, H.C. Rotation in Evolving Stars. Astron. Astrophys. 1970, 5, 155. [Google Scholar]
- Mark, J.W.K. Rapidly Rotating Stars. III. Massive Main-Sequence Stars. Astrophys. J. 1968, 154, 627. [Google Scholar] [CrossRef]
- Bodenheimer, P. Rapidly Rotating Stars. VII. Effects of Angular Momentum on Upper-Main Models. Astrophys. J. 1971, 167, 153. [Google Scholar] [CrossRef]
- Smith, R.C.; Collins, G.W., I. Differential rotation and polar hollows. Mon. Not. R. Astron. Soc. 1992, 257, 340–352. [Google Scholar] [CrossRef][Green Version]
- Ostriker, J.P.; Mark, J.W.K. Rapidly rotating stars. I. The self-consistent-field method. Astrophys. J. 1968, 151, 1075–1088. [Google Scholar] [CrossRef]
- Ostriker, J.P.; Bodenheimer, P. Rapidly Rotating Stars. II. Massive White Dwarfs. Astrophys. J. 1968, 151, 1089. [Google Scholar] [CrossRef]
- Bodenheimer, P.; Ostriker, J.P. Rapidly Rotating Stars.VI. Pre-Main—Evolution of Massive Stars. Astrophys. J. 1970, 161, 1101. [Google Scholar] [CrossRef]
- Jackson, S. Rapidly Rotating Stars. V. The Coupling of the Henyey and the Self-Consistent Methods. Astrophys. J. 1970, 161, 579. [Google Scholar] [CrossRef]
- Bodenheimer, P.; Ostriker, J.P. Rapidly Rotating Stars. VIII. Zero-Viscosity Polytropic Sequences. Astrophys. J. 1973, 180, 159–170. [Google Scholar] [CrossRef]
- Bisnovatyi-Kogan, G.S.; Blinnikov, S.I. Static Criteria for Stability of Arbitrarily Rotating Stars. Astron. Astrophys. 1974, 31, 391. [Google Scholar]
- Blinnikov, S.I. Self-consistent field method in the theory of rotating stars. Soviet Ast. 1975, 19, 151–156. [Google Scholar]
- Jackson, S.; MacGregor, K.B.; Skumanich, A. On the Use of the Self-consistent-Field Method in the Construction of Models for Rapidly Rotating Main-Sequence Stars. Astrophys. J. Suppl. Ser. 2005, 156, 245–264. [Google Scholar] [CrossRef][Green Version]
- Endal, A.S.; Sofia, S. The evolution of rotating stars. I. Method and exploratory calculations for a 7 M sun star. Astrophys. J. 1976, 210, 184–198. [Google Scholar] [CrossRef]
- Eriguchi, Y.; Mueller, E. A general computational method for obtaining equilibria of self-gravitating and rotating gases. Astron. Astrophys. 1985, 146, 260–268. [Google Scholar]
- Hachisu, I. A Versatile Method for Obtaining Structures of Rapidly Rotating Stars. Astrophys. J. Suppl. Ser. 1986, 61, 479. [Google Scholar] [CrossRef]
- Eriguchi, Y.; Mueller, E. Structure of rapidly rotating axisymmetric stars. I—A numerical method for stellar structure and meridional circulation. Astron. Astrophys. 1991, 248, 435–447. [Google Scholar]
- Clement, M.J. On the solution of Poisson’s equation for rapidly rotating stars. Astrophys. J. 1974, 194, 709–714. [Google Scholar] [CrossRef]
- Uryu, K.; Eriguchi, Y. Structures of Rapidly Rotating Baroclinic Stars-Part One—A Numerical Method for the Angular Velocity Distribution. Mon. Not. R. Astron. Soc. 1994, 269, 24. [Google Scholar] [CrossRef][Green Version]
- Uryu, K.; Eriguchi, Y. Structures of rapidly rotating baroclinic stars—II. an extended numerical method for realistic stellar models. Mon. Not. R. Astron. Soc. 1995, 277, 1411–1429. [Google Scholar] [CrossRef]
- Fujisawa, K. A versatile numerical method for obtaining structures of rapidly rotating baroclinic stars: Self-consistent and systematic solutions with shellular-type rotation. Mon. Not. R. Astron. Soc. 2015, 454, 3060–3072. [Google Scholar] [CrossRef]
- Meynet, G.; Maeder, A. Stellar evolution with rotation. I. The computational method and the inhibiting effect of the μ-gradient. Astron. Astrophys. 1997, 321, 465–476. [Google Scholar]
- Meynet, G.; Maeder, A. Stellar evolution with rotation. V. Changes in all the outputs of massive star models. Astron. Astrophys. 2000, 361, 101–120. [Google Scholar]
- Heger, A.; Langer, N.; Woosley, S.E. Presupernova Evolution of Rotating Massive Stars. I. Numerical Method and Evolution of the Internal Stellar Structure. Astrophys. J. 2000, 528, 368–396. [Google Scholar] [CrossRef]
- Hubbard, W.B.; Slattery, W.L.; Devito, C.L. High zonal harmonics of rapidly rotating planets. Astrophys. J. 1975, 199, 504–516. [Google Scholar] [CrossRef]
- Collins, G.W., I. Continuum Emission from Rotating Non-Gray Stellar Atmospheres. II. Astrophys. J. 1966, 146, 914. [Google Scholar] [CrossRef]
- Collins, G.W., II; Smith, R.C. The photometric effect of rotation in the A stars. Mon. Not. R. Astron. Soc. 1985, 213, 519–552. [Google Scholar] [CrossRef][Green Version]
- Frémat, Y.; Zorec, J.; Hubert, A.M.; Floquet, M. Effects of gravitational darkening on the determination of fundamental parameters in fast-rotating B-type stars. Astron. Astrophys. 2005, 440, 305–320. [Google Scholar] [CrossRef]
- Zorec, J.; Rieutord, M.; Espinosa Lara, F.; Frémat, Y.; Domiciano de Souza, A.; Royer, F. Gravity darkening in stars with surface differential rotation. Astron. Astrophys. 2017, 606, A32. [Google Scholar] [CrossRef]
- Milne, E.A. The equilibrium of a rotating star. Mon. Not. R. Astron. Soc. 1923, 83, 118–147. [Google Scholar] [CrossRef][Green Version]
- Sackmann, I.J. Rapid Uniform Rotation Along the Main Sequence II. Astron. Astrophys. 1970, 8, 76. [Google Scholar]
- Deupree, R.G. Stellar Evolution with Arbitrary Rotation Laws. IV. Survey of Zero-Age Main-Sequence Models. Astrophys. J. 2001, 552, 268–277. [Google Scholar] [CrossRef]
- Gillich, A.; Deupree, R.G.; Lovekin, C.C.; Short, C.I.; Toqué, N. Determination of Effective Temperatures and Luminosities for Rotating Stars. Astrophys. J. 2008, 683, 441–448. [Google Scholar] [CrossRef][Green Version]
- Higgins, E.R.; Vink, J.S. Massive star evolution: Rotation, winds, and overshooting vectors in the mass-luminosity plane. I. A calibrated grid of rotating single star models. Astron. Astrophys. 2019, 622, A50. [Google Scholar] [CrossRef]
- von Zeipel, H. The radiative equilibrium of a rotating system of gaseous masses. Mon. Not. R. Astron. Soc. 1924, 84, 665–683. [Google Scholar] [CrossRef]
- Roxburgh, I.W. On stellar rotation, III. Thermally generated magnetic fields. Mon. Not. R. Astron. Soc. 1966, 132, 201. [Google Scholar] [CrossRef][Green Version]
- Lucy, L.B. Gravity-Darkening for Stars with Convective Envelopes. Zeitschrift für Astrophysik 1967, 65, 89. [Google Scholar]
- Rieutord, M. Physical Processes Leading to Surface Inhomogeneities: The Case of Rotation. In Lecture Notes in Physics: Cartograpy of the Sun and the Stars; Rozelot, J.P., Neiner, C., Eds.; Springer International Publishing Switzerland: Cham, Switzerland, 2016; Volume 914, p. 101. [Google Scholar] [CrossRef]
- Smith, R.C.; Worley, R. Gravity-darkening in stars for general rotation laws. Mon. Not. R. Astron. Soc. 1974, 167, 199–214. [Google Scholar] [CrossRef][Green Version]
- Monnier, J.D.; Zhao, M.; Pedretti, E.; Thureau, N.; Ireland, M.; Muirhead, P.; Berger, J.P.; Millan-Gabet, R.; Van Belle, G.; ten Brummelaar, T.; et al. Imaging the Surface of Altair. Science 2007, 317, 342. [Google Scholar] [CrossRef] [PubMed]
- Monnier, J.D.; Che, X.; Zhao, M.; Ekström, S.; Maestro, V.; Aufdenberg, J.; Baron, F.; Georgy, C.; Kraus, S.; McAlister, H.; et al. Resolving Vega and the Inclination Controversy with CHARA/MIRC. Astrophys. J. Lett. 2012, 761, L3. [Google Scholar] [CrossRef]
- Zhao, M.; Monnier, J.D.; Pedretti, E.; Thureau, N.; Mérand, A.; ten Brummelaar, T.; McAlister, H.; Ridgway, S.T.; Turner, N.; Sturmann, J.; et al. Imaging and Modeling Rapidly Rotating Stars: α Cephei and α Ophiuchi. Astrophys. J. 2009, 701, 209–224. [Google Scholar] [CrossRef]
- Che, X.; Monnier, J.D.; Zhao, M.; Pedretti, E.; Thureau, N.; Mérand, A.; ten Brummelaar, T.; McAlister, H.; Ridgway, S.T.; Turner, N.; et al. Colder and Hotter: Interferometric Imaging of β Cassiopeiae and α Leonis. Astrophys. J. 2011, 732, 68. [Google Scholar] [CrossRef]
- Domiciano de Souza, A.; Kervella, P.; Moser Faes, D.; Dalla Vedova, G.; Mérand, A.; Le Bouquin, J.B.; Espinosa Lara, F.; Rieutord, M.; Bendjoya, P.; Carciofi, A.C.; et al. The environment of the fast rotating star Achernar. III. Photospheric parameters revealed by the VLTI. Astron. Astrophys. 2014, 569, A10. [Google Scholar] [CrossRef]
- Domiciano de Souza, A.; Bouchaud, K.; Rieutord, M.; Espinosa Lara, F.; Putigny, B. The evolved fast rotator Sargas. Stellar parameters and evolutionary status from VLTI/PIONIER and VLT/UVES. Astron. Astrophys. 2018, 619, A167. [Google Scholar] [CrossRef]
- Kippenhahn, R. Rotational darkening—Rotational brightening. Astron. Astrophys. 1977, 58, 267–271. [Google Scholar]
- Maeder, A. Stellar evolution with rotation IV: Von Zeipel’s theorem and anisotropic losses of mass and angular momentum. Astron. Astrophys. 1999, 347, 185–193. [Google Scholar]
- Maunder, E.W.; Maunder, A.S.D. Sun, rotation period of the, from Greenwich sun-spot measures, 1879–1901. Mon. Not. R. Astron. Soc. 1905, 65, 813–825. [Google Scholar] [CrossRef]
- Espinosa Lara, F.; Rieutord, M. Gravity darkening in rotating stars. Astron. Astrophys. 2011, 533, A43. [Google Scholar] [CrossRef]
- Maeder, A.; Peytremann, E. Stellar Rotation. Astron. Astrophys. 1970, 7, 120. [Google Scholar]
- Collins, G.W., I. Further Note on Terminology—Specific Luminosity. Astron. Astrophys. 1973, 26, 315. [Google Scholar]
- Moss, D.; Smith, R.C. REVIEW ARTICLE: Stellar rotation and magnetic stars. Reports on Progress in Physics 1981, 44, 831–891. [Google Scholar] [CrossRef]
- Zorec, J. L’effet de la rotation sur la magnitude absolue des étoiles. In Proceedings of the Ecole de Printemps d’Astrophysique de Goutelas: HIPPARCOS; 1993; pp. 407–425. [Google Scholar]
- Maeder, A.; Peytremann, E. Uniformly Rotating Stars with Hydrogen- and Metallic-Line Blanketed Model Atmospheres. Astron. Astrophys. 1972, 21, 279. [Google Scholar]
- Collins, G.W., I; Sonneborn, G.H. Some effects of rotation on the spectra of upper-main-sequence stars. Astrophys. J. Suppl. Ser. 1977, 34, 41–94. [Google Scholar] [CrossRef]
- Slettebak, A.; Kuzma, T.J.; Collins, G.W., I. Effects of stellar rotation on spectral classification. Astrophys. J. 1980, 242, 171–187. [Google Scholar] [CrossRef]
- Collins, G.W., I. Continuum Emission from a Rotating Non-Gray Stellar Atmosphere. Astrophys. J. 1965, 142, 265. [Google Scholar] [CrossRef]
- Zorec, J.; Royer, F. Rotational velocities of A-type stars. IV. Evolution of rotational velocities. Astron. Astrophys. 2012, 537, A120. [Google Scholar] [CrossRef]
- Georgy, C.; Ekström, S.; Eggenberger, P.; Meynet, G.; Haemmerlé, L.; Maeder, A.; Granada, A.; Groh, J.H.; Hirschi, R.; Mowlavi, N.; et al. Grids of stellar models with rotation. III. Models from 0.8 to 120 M⊙ at a metallicity Z = 0.002. Astron. Astrophys. 2013, 558, A103. [Google Scholar] [CrossRef]
- Sigut, T.A.A.; Ghafourian, N.R. Comparing Be Star Inclination Angles Determined from Hα Fitting and Gravitational Darkening. arXiv 2022, arXiv:2209.06885. [Google Scholar]
- Morel, T.; Blazère, A.; Semaan, T.; Gosset, E.; Zorec, J.; Frémat, Y.; Blomme, R.; Daflon, S.; Lobel, A.; Nieva, M.F.; et al. The Gaia-ESO survey: A spectroscopic study of the young open cluster NGC 3293. Astron. Astrophys. 2022, 665, A108. [Google Scholar] [CrossRef]
- Grant, L.P.; Peraiah, A. Spectral line formation in extended stellar atmo-spheres. Mon. Not. R. Astron. Soc. 1972, 160, 239. [Google Scholar] [CrossRef]
- Simonneau, E. Radiative transfer in atmospheres with spherical symmetry. J. Quant. Spectrosc. Radiat. Transf. 1976, 16, 741–753. [Google Scholar] [CrossRef]
- Simonneau, E. Radiative transfer in atmospheres with spherical symmetry—IV. The non-conservative problem. J. Quant. Spectrosc. Radiat. Transf. 1980, 23, 73–81. [Google Scholar] [CrossRef]
- Lopez, R.; Simonneau, E.; Isern, J. Model atmospheres for type I supernovae—Curvature effects. Astron. Astrophys. 1987, 184, 249–255. [Google Scholar]
- Korčáková, D.; Kubát, J. Emergent line profiles from rapidly rotating stars. Memorie della Societa Astronomica Italiana Supplementi 2005, 7, 130. [Google Scholar]
- Frémat, Y.; Zorec, J.; Levenhagen, R.; Leister, N.; Hubert, A.M.; Floquet, M.; Neiner, C. Chemical Composition of Early Type Be Stars. In Proceedings of the CNO in the Universe; Charbonnel, C., Schaerer, D., Meynet, G., Eds.; University of Chicago Press: Chicago, NY, USA, 2003; Volume 304, p. 57. [Google Scholar]
- Frémat, Y.; Zorec, J.; Hubert, A.M. Chemical Composition of the Pole-On Be Star HD 120991. In Proceedings of the Stellar Rotation; Maeder, A., Eenens, P., Eds.; Cambridge University Press: Cambridge, UK, 2004; Volume 215, p. 224. [Google Scholar]
- Frémat, Y.; Zorec, J.; Levenhagen, R.; Leister, N.; Hubert, A.M.; Floquet, M.; Neiner, C. CNO abundances in Early Type Be Stars. In Proceedings of the Stellar Rotation; Maeder, A., Eenens, P., Eds.; Cambridge University Press: Cambridge, UK, 2004; Volume 215, p. 222. [Google Scholar]
- Lennon, D.J.; Lee, J.K.; Dufton, P.L.; Ryans, R.S.I. A Be star with a low nitrogen abundance in the SMC cluster NGC 330. Astron. Astrophys. 2005, 438, 265–271. [Google Scholar] [CrossRef]
- Hunter, I.; Dufton, P.L.; Smartt, S.J.; Ryans, R.S.I.; Evans, C.J.; Lennon, D.J.; Trundle, C.; Hubeny, I.; Lanz, T. The VLT-FLAMES survey of massive stars: Surface chemical compositions of B-type stars in the Magellanic Clouds. Astron. Astrophys. 2007, 466, 277–300. [Google Scholar] [CrossRef]
- Hunter, I.; Brott, I.; Lennon, D.J.; Langer, N.; Dufton, P.L.; Trundle, C.; Smartt, S.J.; de Koter, A.; Evans, C.J.; Ryans, R.S.I. The VLT FLAMES Survey of Massive Stars: Rotation and Nitrogen Enrichment as the Key to Understanding Massive Star Evolution. Astrophys. J. Lett. 2008, 676, L29. [Google Scholar] [CrossRef]
- Hunter, I.; Brott, I.; Langer, N.; Lennon, D.J.; Dufton, P.L.; Howarth, I.D.; Ryans, R.S.I.; Trundle, C.; Evans, C.J.; de Koter, A.; et al. The VLT-FLAMES survey of massive stars: Constraints on stellar evolution from the chemical compositions of rapidly rotating Galactic and Magellanic Cloud B-type stars. Astron. Astrophys. 2009, 496, 841–853. [Google Scholar] [CrossRef]
- Trundle, C.; Dufton, P.L.; Hunter, I.; Evans, C.J.; Lennon, D.J.; Smartt, S.J.; Ryans, R.S.I. The VLT-FLAMES survey of massive stars: Evolution of surface N abundances and effective temperature scales in the Galaxy and Magellanic Clouds. Astron. Astrophys. 2007, 471, 625–643. [Google Scholar] [CrossRef]
- Dunstall, P.R.; Brott, I.; Dufton, P.L.; Lennon, D.J.; Evans, C.J.; Smartt, S.J.; Hunter, I. The VLT-FLAMES survey of massive stars: Nitrogen abundances for Be-type stars in the Magellanic Clouds. Astron. Astrophys. 2011, 536, A65. [Google Scholar] [CrossRef]
- Potter, A.T.; Tout, C.A.; Brott, I. Towards a unified model of stellar rotation—II. Model-dependent characteristics of stellar populations. Mon. Not. R. Astron. Soc. 2012, 423, 1221–1233. [Google Scholar] [CrossRef][Green Version]
- Brott, I.; Evans, C.J.; Hunter, I.; de Koter, A.; Langer, N.; Dufton, P.L.; Cantiello, M.; Trundle, C.; Lennon, D.J.; de Mink, S.E.; et al. Rotating massive main-sequence stars. II. Simulating a population of LMC early B-type stars as a test of rotational mixing. Astron. Astrophys. 2011, 530, A116. [Google Scholar] [CrossRef]
- Porter, J.M. On the possibility that rotation causes latitudinal abundance variations in stars. Astron. Astrophys. 1999, 341, 560–566. [Google Scholar]
- Langer, N.; Cantiello, M.; Yoon, S.C.; Hunter, I.; Brott, I.; Lennon, D.; de Mink, S.; Verheijdt, M. Rotation and Massive Close Binary Evolution. In Proceedings of the Massive Stars as Cosmic Engines; Bresolin, F., Crowther, P.A., Puls, J., Eds.; 2008; Volume 250, pp. 167–178. [Google Scholar] [CrossRef][Green Version]
- Shajn, G.; Struve, O. On the rotation of the stars. Mon. Not. R. Astron. Soc. 1929, 89, 222–239. [Google Scholar] [CrossRef]
- Struve, O. Axial rotation as a major factor in stellar spectroscopy. Observatory 1931, 54, 80–84. [Google Scholar]
- Elvey, C.T. The rotation of stars and the contours of Mg+ 4481. Astrophys. J. 1930, 71, 221–230. [Google Scholar] [CrossRef]
- Carroll, J.A. The spectroscopic determination of stellar rotation and its effect on line profiles. Mon. Not. R. Astron. Soc. 1933, 93, 478–507. [Google Scholar] [CrossRef]
- Slettebak, A. On the Axial Rotation of the Brighter O and B Stars. Astrophys. J. 1949, 110, 498. [Google Scholar] [CrossRef]
- Howarth, I.D. Rotation and Line Broadening in OBA Stars (Invited Review). In Proceedings of the Stellar Rotation; Maeder, A., Eenens, P., Eds.; Cambridge University Press: Cambridge, UK, 2004; Volume 215, p. 33. [Google Scholar]
- Slettebak, A.; Collins, G.W., I; Boyce, P.B.; White, N.M.; Parkinson, T.D. A system of standard stars for rotational velocity determinations. Astrophys. J. Suppl. Ser. 1975, 29, 137–159. [Google Scholar] [CrossRef]
- Dufton, P.L.; Smartt, S.J.; Lee, J.K.; Ryans, R.S.I.; Hunter, I.; Evans, C.J.; Herrero, A.; Trundle, C.; Lennon, D.J.; Irwin, M.J.; et al. The VLT-FLAMES survey of massive stars: Stellar parameters and rotational velocities in NGC 3293, NGC 4755 and NGC 6611. Astron. Astrophys. 2006, 457, 265–280. [Google Scholar] [CrossRef]
- Díaz, C.G.; González, J.F.; Levato, H.; Grosso, M. Accurate stellar rotational velocities using the Fourier transform of the cross correlation maximum. Astron. Astrophys. 2011, 531, A143. [Google Scholar] [CrossRef]
- Howarth, I.D.; Siebert, K.W.; Hussain, G.A.J.; Prinja, R.K. Cross-correlation characteristics of OB stars from IUE spectroscopy. Mon. Not. R. Astron. Soc. 1997, 284, 265–285. [Google Scholar] [CrossRef]
- Daflon, S.; Cunha, K.; de Araújo, F.X.; Wolff, S.; Przybilla, N. The Projected Rotational Velocity Distribution of a Sample of OB stars from a Calibration Based on Synthetic He I Lines. Astron. J. 2007, 134, 1570–1578. [Google Scholar] [CrossRef][Green Version]
- Stoeckley, T.; Mihalas, D. Limb Darkening and Rotation Broadening of Neutral Helium and Ionized Magnesium Line Profiles in Early-Type Stars; NCAR: Boulder, CO, USA, 1973; Volume NCAR. [Google Scholar]
- Huang, W.; Gies, D.R. Stellar Rotation in Young Clusters. I. Evolution of Projected Rotational Velocity Distributions. Astrophys. J. 2006, 648, 580–590. [Google Scholar] [CrossRef][Green Version]
- Balona, L.A. Equivalent widths and rotational velocities of southern early-type stars. Mem. R. Astron. Soc. 1975, 78, 51. [Google Scholar]
- Conti, P.S.; Ebbets, D. Spectroscopic studies of O-type stars. VII. Rotational velocities V sin i and evidence for macroturbulent motions. Astrophys. J. 1977, 213, 438–447. [Google Scholar] [CrossRef]
- Slettebak, A. Spectral types and rotational velocities of the brighter Be stars and A-F type shell stars. Astrophys. J. Suppl. Ser. 1982, 50, 55–83. [Google Scholar] [CrossRef]
- Wolff, S.C.; Edwards, S.; Preston, G.W. The origin of stellar angular momentum. Astrophys. J. 1982, 252, 322–336. [Google Scholar] [CrossRef]
- Abt, H.A.; Morrell, N.I. The Relation between Rotational Velocities and Spectral Peculiarities among A-Type Stars. Astrophys. J. Suppl. Ser. 1995, 99, 135. [Google Scholar] [CrossRef]
- Halbedel, E.M. Rotational Velocity Determinations for 164 Be and B Stars. Publ. Astron. Soc. Pac. 1996, 108, 833. [Google Scholar] [CrossRef]
- Penny, L.R. Projected Rotational Velocities of O-Type Stars. Astrophys. J. 1996, 463, 737. [Google Scholar] [CrossRef]
- Brown, A.G.A.; Verschueren, W. High S/N Echelle spectroscopy in young stellar groups. II. Rotational velocities of early-type stars in SCO OB2. Astron. Astrophys. 1997, 319, 811–838. [Google Scholar]
- Steele, I.A.; Negueruela, I.; Clark, J.S. A representative sample of Be stars . I. Sample selection, spectral classification and rotational velocities. Astron. Astrophys. 1999, 137, 147–156. [Google Scholar] [CrossRef]
- Chauville, J.; Zorec, J.; Ballereau, D.; Morrell, N.; Cidale, L.; Garcia, A. High and intermediate-resolution spectroscopy of Be stars 4481 lines. Astron. Astrophys. 2001, 378, 861–882. [Google Scholar] [CrossRef]
- Abt, H.A.; Levato, H.; Grosso, M. Rotational Velocities of B Stars. Astrophys. J. 2002, 573, 359–365. [Google Scholar] [CrossRef]
- Royer, F.; Gerbaldi, M.; Faraggiana, R.; Gómez, A.E. Rotational velocities of A-type stars. I. Measurement of v sin i in the southern hemisphere. Astron. Astrophys. 2002, 381, 105–121. [Google Scholar] [CrossRef]
- Royer, F.; Grenier, S.; Baylac, M.O.; Gómez, A.E.; Zorec, J. Rotational velocities of A-type stars in the northern hemisphere. II. Measurement of v sin i. Astron. Astrophys. 2002, 393, 897–911. [Google Scholar] [CrossRef]
- Keller, S.C. Rotation of Early B-type Stars in the Large Magellanic Cloud: The Role of Evolution and Metallicity. Publ. Astron. Soc. Aust. 2004, 21, 310–317. [Google Scholar] [CrossRef][Green Version]
- Penny, L.R.; Sprague, A.J.; Seago, G.; Gies, D.R. Effects of Metallicity on the Rotational Velocities of Massive Stars. Astrophys. J. 2004, 617, 1316–1322. [Google Scholar] [CrossRef]
- Glebocki, R.; Gnacinski, P. VizieR Online Data Catalog: Catalog of Stellar Rotational Velocities (Glebocki+ 2005); VizieR Online Data Catalog; 2005; p. III/244. [Google Scholar]
- Strom, S.E.; Wolff, S.C.; Dror, D.H.A. B Star Rotational Velocities in h and χ Persei: A Probe of Initial Conditions during the Star Formation Epoch? Astron. J. 2005, 129, 809–828. [Google Scholar] [CrossRef]
- Wolff, S.C.; Strom, S.E.; Dror, D.; Lanz, L.; Venn, K. Stellar Rotation: A Clue to the Origin of High-Mass Stars? Astron. J. 2006, 132, 749–755. [Google Scholar] [CrossRef]
- Frémat, Y.; Neiner, C.; Hubert, A.M.; Floquet, M.; Zorec, J.; Janot-Pacheco, E.; Renan de Medeiros, J. Fundamental parameters of Be stars located in the seismology fields of COROT. Astron. Astrophys. 2006, 451, 1053–1063. [Google Scholar] [CrossRef]
- Mokiem, M.R.; de Koter, A.; Evans, C.J.; Puls, J.; Smartt, S.J.; Crowther, P.A.; Herrero, A.; Langer, N.; Lennon, D.J.; Najarro, F.; et al. The VLT-FLAMES survey of massive stars: Mass loss and rotation of early-type stars in the SMC. Astron. Astrophys. 2006, 456, 1131–1151. [Google Scholar] [CrossRef]
- Levenhagen, R.S.; Leister, N.V. Spectroscopic analysis of southern B and Be stars. Mon. Not. R. Astron. Soc. 2006, 371, 252–262. [Google Scholar] [CrossRef][Green Version]
- Martayan, C.; Frémat, Y.; Hubert, A.M.; Floquet, M.; Zorec, J.; Neiner, C. Effects of metallicity, star-formation conditions, and evolution in B and Be stars. I. Large Magellanic Cloud, field of NGC 2004. Astron. Astrophys. 2006, 452, 273–284. [Google Scholar] [CrossRef]
- Martayan, C.; Frémat, Y.; Hubert, A.M.; Floquet, M.; Zorec, J.; Neiner, C. Effects of metallicity, star-formation conditions, and evolution in B and Be stars. II. Small Magellanic Cloud, field of NGC 330. Astron. Astrophys. 2007, 462, 683–694. [Google Scholar] [CrossRef]
- Wolff, S.C.; Strom, S.E.; Dror, D.; Venn, K. Rotational Velocities for B0-B3 Stars in Seven Young Clusters: Further Study of the Relationship between Rotation Speed and Density in Star-Forming Regions. Astron. J. 2007, 133, 1092–1103. [Google Scholar] [CrossRef]
- Wolff, S.C.; Strom, S.E.; Cunha, K.; Daflon, S.; Olsen, K.; Dror, D. Rotational Velocities for Early-Type Stars in the Young Large Magellanic Cloud Cluster R136: Further Study of the Relationship Between Rotation Speed and Density in Star-Forming Regions. Astron. J. 2008, 136, 1049–1060. [Google Scholar] [CrossRef][Green Version]
- Huang, W.; Gies, D.R. Stellar Rotation in Field and Cluster B Stars. Astrophys. J. 2008, 683, 1045–1051. [Google Scholar] [CrossRef][Green Version]
- Hunter, I.; Lennon, D.J.; Dufton, P.L.; Trundle, C.; Simón-Díaz, S.; Smartt, S.J.; Ryans, R.S.I.; Evans, C.J. The VLT-FLAMES survey of massive stars: Atmospheric parameters and rotational velocity distributions for B-type stars in the Magellanic Clouds. Astron. Astrophys. 2008, 479, 541–555. [Google Scholar] [CrossRef]
- Penny, L.R.; Gies, D.R. A FUSE Survey of the Rotation Rates of Very Massive Stars in the Small and Large Magellanic Clouds. Astrophys. J. 2009, 700, 844–858. [Google Scholar] [CrossRef]
- Fraser, M.; Dufton, P.L.; Hunter, I.; Ryans, R.S.I. Atmospheric parameters and rotational velocities for a sample of Galactic B-type supergiants. Mon. Not. R. Astron. Soc. 2010, 404, 1306–1320. [Google Scholar] [CrossRef]
- Huang, W.; Gies, D.R.; McSwain, M.V. A Stellar Rotation Census of B Stars: From ZAMS to TAMS. Astrophys. J. 2010, 722, 605–619. [Google Scholar] [CrossRef]
- Marsh Boyer, A.N.; McSwain, M.V.; Aragona, C.; Ou-Yang, B. Physical Properties of the B and Be Star Populations of h and χ Persei. Astron. J. 2012, 144, 158. [Google Scholar] [CrossRef][Green Version]
- Bragança, G.A.; Daflon, S.; Cunha, K.; Bensby, T.; Oey, M.S.; Walth, G. Projected Rotational Velocities and Stellar Characterization of 350 B Stars in the Nearby Galactic Disk. Astron. J. 2012, 144, 130. [Google Scholar] [CrossRef]
- Dufton, P.L.; Langer, N.; Dunstall, P.R.; Evans, C.J.; Brott, I.; de Mink, S.E.; Howarth, I.D.; Kennedy, M.; McEvoy, C.; Potter, A.T.; et al. The VLT-FLAMES Tarantula Survey. X. Evidence for a bimodal distribution of rotational velocities for the single early B-type stars. Astron. Astrophys. 2013, 550, A109. [Google Scholar] [CrossRef]
- Ramírez-Agudelo, O.H.; Simón-Díaz, S.; Sana, H.; de Koter, A.; Sabín-Sanjulían, C.; de Mink, S.E.; Dufton, P.L.; Gräfener, G.; Evans, C.J.; Herrero, A.; et al. The VLT-FLAMES Tarantula Survey. XII. Rotational velocities of the single O-type stars. Astron. Astrophys. 2013, 560, A29. [Google Scholar] [CrossRef]
- Simón-Díaz, S.; Herrero, A. The IACOB project. I. Rotational velocities in northern Galactic O- and early B-type stars revisited. The impact of other sources of line-broadening. Astron. Astrophys. 2014, 562, A135. [Google Scholar] [CrossRef]
- Garmany, C.D.; Glaspey, J.W.; Bragança, G.A.; Daflon, S.; Borges Fernandes, M.; Oey, M.S.; Bensby, T.; Cunha, K. Projected Rotational Velocities of 136 Early B-type Stars in the Outer Galactic Disk. Astron. J. 2015, 150, 41. [Google Scholar] [CrossRef]
- Holgado, G.; Simón-Díaz, S.; Herrero, A.; Barbá, R.H. The IACOB project. VII. The rotational properties of Galactic massive O-type stars revisited. Astron. Astrophys. 2022, 665, A150. [Google Scholar] [CrossRef]
- Solar, M.; Arcos, C.; Curé, M.; Levenhagen, R.S.; Araya, I. Automatic algorithm to obtain v sin i values via Fourier transform in the BeSOS database. Mon. Not. R. Astron. Soc. 2022, 511, 4404–4416. [Google Scholar] [CrossRef]
- Xiang, M.; Rix, H.W.; Ting, Y.S.; Kudritzki, R.P.; Conroy, C.; Zari, E.; Shi, J.R.; Przybilla, N.; Ramirez-Tannus, M.; Tkachenko, A.; et al. Stellar labels for hot stars from low-resolution spectra. I. The HotPayne method and results for 330 000 stars from LAMOST DR6. Astron. Astrophys. 2022, 662, A66. [Google Scholar] [CrossRef]
- Vallenari, A.; Brown, A.G.A.; Prusti, T.; de Bruijne, J.H.J.; Arenou, F.; Babusiaux, C.; Biermann, M.; Creevey, O.L.; Ducourant, C.; et al.; Gaia Collaboration Gaia Data Release 3: Summary of the content and survey properties. arXiv 2022, arXiv:arXiv:2208.00211. [Google Scholar]
- Sartoretti, P.; Blomme, R.; David, M.; Seabroke, G. Gaia DR3 documentation Chapter 6: Spectroscopy. Gaia DR3 documentation, European Space Agency; Gaia Data Processing and Analysis Consortium. 2022. Available online: https://gea.esac.esa.int/archive/documentation/GDR3/index.html (accessed on 1 February 2022).
- Creevey, O.L.; Sordo, R.; Pailler, F.; Frémat, Y.; Heiter, U.; Thévenin, F.; Andrae, R.; Fouesneau, M.; Lobel, A.; Bailer-Jones, C.A.L.; et al. Gaia Data Release 3: Astrophysical parameters inference system (Apsis) I—Methods and content overview. arXiv 2022, arXiv:2206.05864. [Google Scholar] [CrossRef]
- Frémat, Y.; Royer, F.; Marchal, O.; Blomme, R.; Sartoretti, P.; Guerrier, A.; Panuzzo, P.; Katz, D.; Seabroke, G.M.; Thévenin, F.; et al. Gaia Data Release 3: Properties of the line broadening parameter derived with the Radial Velocity Spectrometer (RVS). arXiv 2022, arXiv:2206.10986. [Google Scholar] [CrossRef]
- Blomme, R.; Frémat, Y.; Sartoretti, P.; Guerrier, A.; Panuzzo, P.; Katz, D.; Seabroke, G.M.; Thevenin, F.; Cropper, M.; Benson, K.; et al. Gaia Data Release 3: Hot-star radial velocities. arXiv 2022, arXiv:2206.05486. [Google Scholar] [CrossRef]
- Katz, D.; Sartoretti, P.; Guerrier, A.; Panuzzo, P.; Seabroke, G.M.; Thévenin, F.; Cropper, M.; Benson, K.; Blomme, R.; Haigron, R.; et al. Gaia Data Release 3 Properties and validation of the radial velocities. arXiv 2022, arXiv:2206.05902. [Google Scholar] [CrossRef]
- Gaia Collaboration; Creevey, O.L.; Sarro, L.M.; Lobel, A.; Pancino, E.; Andrae, R.; Smart, R.L.; Clementini, G.; Heiter, U.; Korn, A.J.; et al. Gaia Data Release 3: A Golden Sample of Astrophysical Parameters. arXiv 2022, arXiv:arXiv:2206.05870. [Google Scholar] [CrossRef]
- Ulla, A.; Creevey, O.L.; Álvarez, M.A.; Andrae, R.; Bailer-Jones, C.A.L.; Bellas-Velidis, I.; Brugaletta, E.; Carballo, R.; Dafonte, C.; Delchambre, L.; et al. Gaia DR3 Documentation Chapter 11: Astrophysical Parameters. Gaia DR3 Documentation, European Space Agency; Gaia Data Processing and Analysis Consortium. 2022. Available online: https://gea.esac.esa.int/archive/documentation/GDR3/index.html (accessed on 1 February 2022).
- Fouesneau, M.; Frémat, Y.; Andrae, R.; Korn, A.J.; Soubiran, C.; Kordopatis, G.; Vallenari, A.; Heiter, U.; Creevey, O.L.; Sarro, L.M.; et al. Gaia Data Release 3: Apsis II – Stellar Parameters. arXiv 2022, arXiv:2206.05992. [Google Scholar] [CrossRef]
- Shridharan, B.; Mathew, B.; Bhattacharyya, S.; Robin, T.; Arun, R.; Kartha, S.S.; Manoj, P.; Nidhi, S.; Maheshwar, G.; Paul, K.T.; et al. Emission line star catalogues post-Gaia DR3: A validation of Gaia DR3 data using LAMOST OBA emission catalogue. arXiv 2022, arXiv:2209.13221. [Google Scholar] [CrossRef]
- Plaskett, H.H. The formation of the magnesium b lines in the solar atmosphere. Mon. Not. R. Astron. Soc. 1931, 91, 870. [Google Scholar] [CrossRef][Green Version]
- Gray, D.F. The Observation and Analysis of Stellar Photospheres; Cambridge University Press: Cambridge, UK, 2008. [Google Scholar]
- Levenhagen, R.S. A Fourier Transform Method for Vsin i Estimations under Nonlinear Limb-darkening Laws. Astrophys. J. 2014, 797, 29. [Google Scholar] [CrossRef]
- Collins, G.W., I; Truax, R.J. Classical Rotational Broadening of Spectral Lines. Astrophys. J. 1995, 439, 860. [Google Scholar] [CrossRef]
- Korčáková, D.; Kubát, J. Radiative transfer in moving media. I. Discontinuous finite element method for one-dimensional atmospheres. Astron. Astrophys. 2003, 401, 419–428. [Google Scholar] [CrossRef][Green Version]
- Korčáková, D.; Kubát, J. Radiative transfer in moving media. II. Solution of the radiative transfer equation in axial symmetry. Astron. Astrophys. 2005, 440, 715–725. [Google Scholar] [CrossRef][Green Version]
- Hillier, D.J.; Lanz, T. CMFGEN: A non-LTE Line-Blanketed Radiative Transfer Code for Modeling Hot Stars with Stellar Winds. In Proceedings of the Spectroscopic Challenges of Photoionized Plasmas; Ferland, G., Savin, D.W., Eds.; University of Chicago Press: Chicago, NY, USA, 2001; Volume 247, p. 343. [Google Scholar]
- Hillier, D.J. The atomic physics underlying the spectroscopic analysis of massive stars and supernovae. Astrophys. Space Sci. 2011, 336, 87–93. [Google Scholar] [CrossRef]
- Hillier, D.J. Hot Stars with Winds: The CMFGEN Code. In Proceedings of the From Interacting Binaries to Exoplanets: Essential Modeling Tools; Richards, M.T., Hubeny, I., Eds.; Cambridge Unversity Press: Cambridge, UK, 2012; Volume 282, pp. 229–234. [Google Scholar] [CrossRef][Green Version]
- Stoeckley, T.R. Distribution of rotational velocities in Be stars. Mon. Not. R. Astron. Soc. 1968, 140, 141. [Google Scholar] [CrossRef]
- Stoeckley, T.R. Absorption line strengths in rotating stars. Mon. Not. R. Astron. Soc. 1968, 140, 149. [Google Scholar] [CrossRef]
- Townsend, R.H.D.; Owocki, S.P.; Howarth, I.D. Be-star rotation: How close to critical? Mon. Not. R. Astron. Soc. 2004, 350, 189–195. [Google Scholar] [CrossRef]
- Cranmer, S.R. A Statistical Study of Threshold Rotation Rates for the Formation of Disks around Be Stars. Astrophys. J. 2005, 634, 585–601. [Google Scholar] [CrossRef]
- Heap, S.R. Ultraviolet Observations of be Stars (review Paper). In Proceedings of the Be and Shell Stars; Slettebak, A., Ed.; 1976; Volume 70, p. 165. [Google Scholar]
- Heap, S.R. Apparent wavelength dependence of ν sin i for Zeta Tauri. Astrophys. J. Lett. 1977, 218, L17–L19. [Google Scholar] [CrossRef]
- Hutchings, J.B. V sin i Values in the Far Ultraviolet. Publ. Astron. Soc. Pac. 1976, 88, 5. [Google Scholar] [CrossRef]
- Hutchings, J.B.; Stoeckley, T.R. V and i in rotating stars from Copernicus UV data. Publ. Astron. Soc. Pac. 1977, 89, 19–22. [Google Scholar] [CrossRef]
- Sonneborn, G.H.; Collins, G.W., I. On the wavelength dependence of rotational line broadening. Astrophys. J. 1977, 213, 787–790. [Google Scholar] [CrossRef]
- Carpenter, K.G.; Slettebak, A.; Sonneborn, G. Rotational velocities of later B type and A type stars as determined from ultraviolet versus visual line profiles. Astrophys. J. 1984, 286, 741–746. [Google Scholar] [CrossRef]
- Osaki, Y. On the Atmosphere of a Rotating Star. Publ. Astron. Soc. Jpn. 1966, 18, 7. [Google Scholar]
- Pustyl’Nik, I. Radiative transfer in the atmospheres of rotating stars. Izv. Akad. Nauk Ehstonskoj SSR 1970, 19, 428–435. [Google Scholar] [CrossRef]
- Hadrava, P. Radiative transfer in rotating stars. Astron. Astrophys. 1992, 256, 519–524. [Google Scholar]
- Unsöld, A.; Struve, O. Curves of Growth and Line Contours. Astrophys. J. 1949, 110, 455. [Google Scholar] [CrossRef]
- Huang, S.S.; Struve, O. A Study of Line Profiles: The Spectrum of Rho Leonis. Astrophys. J. 1953, 118, 463. [Google Scholar] [CrossRef]
- Underhill, A.B. On the Effect of Radiation Pressure in the Atmospheres of Early-Type Stars. Mon. Not. R. Astron. Soc. 1949, 109, 562. [Google Scholar] [CrossRef][Green Version]
- Underhill, A.B. Numerical experiments concerning the rotational broadening of spectral lines. Bull. Astron. Institutes Neth. 1968, 19, 526. [Google Scholar]
- Rosendhal, J.D. Evolutionary Effects in the Rotation of Supergiants. Astrophys. J. 1970, 159, 107. [Google Scholar] [CrossRef]
- Grassitelli, L.; Fossati, L.; Simón-Diáz, S.; Langer, N.; Castro, N.; Sanyal, D. Observational Consequences of Turbulent Pressure in the Envelopes of Massive Stars. Astrophys. J. Lett. 2015, 808, L31. [Google Scholar] [CrossRef]
- Aerts, C.; Puls, J.; Godart, M.; Dupret, M.A. Collective pulsational velocity broadening due to gravity modes as a physical explanation for macroturbulence in hot massive stars. Astron. Astrophys. 2009, 508, 409–419. [Google Scholar] [CrossRef]
- Aerts, C.; Simón-Díaz, S.; Groot, P.J.; Degroote, P. On the use of the Fourier transform to determine the projected rotational velocity of line-profile variable B stars. Astron. Astrophys. 2014, 569, A118. [Google Scholar] [CrossRef]
- Simón-Díaz, S. Asteroseismology of OB stars with hundreds of single snapshot spectra (and a few time-series of selected targets). In Proceedings of the IAU Symposium; Meynet, G., Georgy, C., Groh, J., Stee, P., Eds.; 2015; Volume 307, pp. 194–199. [Google Scholar] [CrossRef]
- Ryans, R.S.I.; Dufton, P.L.; Rolleston, W.R.J.; Lennon, D.J.; Keenan, F.P.; Smoker, J.V.; Lambert, D.L. Macroturbulent and rotational broadening in the spectra of B-type supergiants. Mon. Not. R. Astron. Soc. 2002, 336, 577–586. [Google Scholar] [CrossRef]
- Gray, D.F. Atmospheric turbulence measured in stars above the main sequence. Astrophys. J. 1975, 202, 148–164. [Google Scholar] [CrossRef]
- Gray, D.F. The Observation and Analysis of Stellar Photospheres; Cambridge Astrophysics, Series; Cambridge University Press: Cambridge, UK, 1992. [Google Scholar]
- Simón-Díaz, S.; Herrero, A. Fourier method of determining the rotational velocities in OB stars. Astron. Astrophys. 2007, 468, 1063–1073. [Google Scholar] [CrossRef]
- Dufton, P.L.; Ryans, R.S.I.; Simón-Díaz, S.; Trundle, C.; Lennon, D.J. B-type supergiants in the Small Magellanic Cloud: Rotational velocities and implications for evolutionary models. Astron. Astrophys. 2006, 451, 603–611. [Google Scholar] [CrossRef][Green Version]
- Sundqvist, J.O.; Simón-Díaz, S.; Puls, J.; Markova, N. The rotation rates of massive stars. How slow are the slow ones? Astron. Astrophys. 2013, 559, L10. [Google Scholar] [CrossRef]
- Stoeckley, T.R. Determination of aspect and degree of differential rotation, from line profiles in rapidly rotating stars. Mon. Not. R. Astron. Soc. 1968, 140, 121. [Google Scholar] [CrossRef]
- Zorec, J.; Divan, L.; Mochkovitch, R.; Garcia, A. Differential rotation in B and Be stars. In Proceedings of the IAU Colloq. 92: Physics of Be Stars; Slettebak, A., Snow, T.P., Eds.; Cambridge University Press: Cambridge, UK, 1987; pp. 68–70. [Google Scholar]
- Stoeckley, T.R.; Buscombe, W. Axial inclination and differential rotation for 19 rapidly rotating stars. Mon. Not. R. Astron. Soc. 1987, 227, 801–813. [Google Scholar] [CrossRef]
- Zorec, J.; Mochkovitch, R.A.; Garcia, A. The Angular Momentum Loss and the Differential Rotation in B-Stars and Be-Stars. In Proceedings of the NATO ASIC Proc. 316: Angular Momentum and Mass Loss for Hot Stars; Willson, L.A., Stalio, R., Eds.; Springer: Berlin/Heidelberg, Germany, 1990; p. 239. [Google Scholar]
- Cranmer, S.R.; Collins, G.W., II. The effects of zonal atmospheric currents on the spectra of rotating early-type stars. Astrophys. J. 1993, 412, 720–730. [Google Scholar] [CrossRef]
- Rieutord, M. The dynamics of the radiative envelope of rapidly rotating stars. I. A spherical Boussinesq model. Astron. Astrophys. 2006, 451, 1025–1036. [Google Scholar] [CrossRef]
- Espinosa Lara, F.; Rieutord, M. The dynamics of a fully radiative rapidly rotating star enclosed within a spherical box. Astron. Astrophys. 2007, 470, 1013–1022. [Google Scholar] [CrossRef]
- Schou, J.; Antia, H.M.; Basu, S.; Bogart, R.S.; Bush, R.I.; Chitre, S.M.; Christensen-Dalsgaard, J.; Di Mauro, M.P.; Dziembowski, W.A.; Eff-Darwich, A.; et al. Helioseismic Studies of Differential Rotation in the Solar Envelope by the Solar Oscillations Investigation Using the Michelson Doppler Imager. Astrophys. J. 1998, 505, 390–417. [Google Scholar] [CrossRef]
- Espinosa Lara, F.; Rieutord, M. Self-consistent 2D models of fast-rotating early-type stars. Astron. Astrophys. 2013, 552, A35. [Google Scholar] [CrossRef]
- Rieutord, M.; Espinosa Lara, F.; Putigny, B. An algorithm for computing the 2D structure of fast rotating stars. J. Comput. Phys. 2016, 318, 277–304. [Google Scholar] [CrossRef]
- Gagnier, D.; Rieutord, M.; Charbonnel, C.; Putigny, B.; Espinosa Lara, F. Evolution of rotation in rapidly rotating early-type stars during the main sequence with 2D models. Astron. Astrophys. 2019, 625, A89. [Google Scholar] [CrossRef]
- Jeffery, C.S. Quasi-emission lines in rotating B stars. Mon. Not. R. Astron. Soc. 1991, 249, 327. [Google Scholar] [CrossRef][Green Version]
- Takeda, Y.; Kawanomoto, S.; Ohishi, N. High-Resolution and High-S/N Spectrum Atlas of Vega. Publ. Astron. Soc. Jpn. 2007, 59, 245–261. [Google Scholar] [CrossRef]
- Takeda, Y.; Kawanomoto, S.; Ohishi, N. Rotational Feature of Vega Revealed from Spectral Line Profiles. Astrophys. J. 2008, 678, 446–462. [Google Scholar] [CrossRef][Green Version]
- Zorec, J. Emission-like feature due to a latitudinal differential rotation. In Proceedings of the Pulsation; Rotation; and Mass Loss in Early-Type Stars; Balona, L.A., Henrichs, H.F., Le Contel, J.M., Eds.; Kluwer Academic Publishers: New York, NY, USA, 1994; Volume 162, pp. 257–258. [Google Scholar]
- Reiners, A.; Schmitt, J.H.M.M. Rotation and differential rotation in field F- and G-type stars. Astron. Astrophys. 2003, 398, 647–661. [Google Scholar] [CrossRef]
- Reiners, A.; Schmitt, J.H.M.M. Differential rotation in rapidly rotating F-stars. Astron. Astrophys. 2003, 412, 813–819. [Google Scholar] [CrossRef]
- Sobolev, V.V. Moving Envelopes of Stars; Harvard University Press: Cambridge, UK, 1960. [Google Scholar] [CrossRef]
- Kalkofen, W. Methods in Radiative Transfer; Cambridge University Press: Cambridge, UK, 1984. [Google Scholar]
- Mihalas, D.; Mihalas, B.W. Foundations of radiation hydrodynamics; New York: Oxford University Press, 1984. [Google Scholar]
- Kunasz, P.B. The theory of line transfer in expanding atmospheres. In Progress in Stellar Spectral Line Formation Theory; Beckman, J.E., Crivellari, L., Eds.; Springer Science & Business Media: Berlin, Germany, 1985; Volume 152, pp. 319–333. [Google Scholar]
- Kalkofen, W. Numerical Radiative Transfer; Cambridge University Press: Cambridge, UK, 1987. [Google Scholar]
- Conti, P.S.; Underhill, A.B.; Jordan, S.; Thomas, R.N.; Goldberg, L.; Pecker, J.C.; Baade, D.; Divan, L.; Garmany, C.D.; Henrichs, H.F.; et al. O Stars and Wolf-Rayet Stars; NASA: Washington, DC, USA, 1988; Volume 497. [Google Scholar]
- Sen, K.K.; Wilson, S.J. Radiative Transfer in Moving Media: Basic Mathematical Methods for Radiative Transfer In Spherically Symmetrical Moving Media; Springer: Berlin/Heidelberg, Germany, 1998. [Google Scholar]
- Stee, P. (Ed.) Radiative Transfer and Hydrodynamics in Astrophysics; EAS Publications Series; EDP Sciences: Les Ulis, France, 2002; Volume 5. [Google Scholar]
- Ivan Hubeny, D.M.; Werner, K. (Eds.) Stellar Atmosphere Modeling; Astronomical Society of the Pacific Conference Series; University Chicago Press: Chicago, NY, USA, 2003; Volume 288. [Google Scholar]
- Cannon, C.J. The Transfer of Spectral Line Radiation; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Furenlid, I.; Young, A. Mass loss and rotation in early-main-sequence B stars. Astrophys. J. Lett. 1980, 240, L59–L61. [Google Scholar] [CrossRef]
- Cook, K.H.; Alcock, C.; Allsman, H.A.; Axelrod, T.S.; Freeman, K.C.; Peterson, B.A.; Quinn, P.J.; Rodgers, A.W.; Bennett, D.P.; Reimann, J.; et al. Variable Stars in the MACHO Collaboration Database. In Proceedings of the IAU Colloq. 155: Astrophysical Applications of Stellar Pulsation; Stobie, R.S., Whitelock, P.A., Eds.; Cambridge University Press: Cambridge, UK, 1995; Volume 83, p. 221. [Google Scholar]
- Duval, P.; Karp, A.H. The combined effects of expansion and rotation on spectral line shapes. Astrophys. J. 1978, 222, 220–225. [Google Scholar] [CrossRef]
- Chelli, A.; Petrov, R.G. Model fitting and error analysis for differential interferometry. I. General formalism. Astron. Astrophys. 1995, 109, 389–399. [Google Scholar]
- Chelli, A.; Petrov, R.G. Model fitting and error analysis for differential interferometry. II. Application to rotating stars and binary systems. Astron. Astrophys. 1995, 109, 401–415. [Google Scholar]
- Vakili, F.; Mourard, D.; Bonneau, D.; Morand, F.; Stee, P. Subtle structures in the wind of P Cygni. Astron. Astrophys. 1997, 323, 183–188. [Google Scholar]
- Jankov, S.; Vakili, F.; Domiciano de Souza, A., Jr.; Janot-Pacheco, E. Interferometric-Doppler imaging of stellar surface structure. Astron. Astrophys. 2001, 377, 721–734. [Google Scholar] [CrossRef]
- ten Brummelaar, T.A.; McAlister, H.A.; Ridgway, S.T.; Bagnuolo, W.G., Jr.; Turner, N.H.; Sturmann, L.; Sturmann, J.; Berger, D.H.; Ogden, C.E.; Cadman, R.; et al. First Results from the CHARA Array. II. A Description of the Instrument. Astrophys. J. 2005, 628, 453–465. [Google Scholar] [CrossRef]
- Armstrong, J.T.; Mozurkewich, D.; Rickard, L.J.; Hutter, D.J.; Benson, J.A.; Bowers, P.F.; Elias, N.M., I; Hummel, C.A.; Johnston, K.J.; Buscher, D.F.; et al. The Navy Prototype Optical Interferometer. Astrophys. J. 1998, 496, 550–571. [Google Scholar] [CrossRef]
- Colavita, M.M.; Wallace, J.K.; Hines, B.E.; Gursel, Y.; Malbet, F.; Palmer, D.L.; Pan, X.P.; Shao, M.; Yu, J.W.; Boden, A.F.; et al. The Palomar Testbed Interferometer. Astrophys. J. 1999, 510, 505–521. [Google Scholar] [CrossRef]
- Glindemann, A.; Albertsen, M.; Andolfato, L.; Avila, G.; Ballester, P.; Bauvir, B.; Delplancke, F.; Derie, F.; Dimmler, M.; Duhoux, P.; et al. VLTI technical advances: Present and future. In Proceedings of the New Frontiers in Stellar Interferometry; Traub, W.A., Ed.; SPIE: Washington, DC, USA, 2004; Volume 5491, p. 447. [Google Scholar] [CrossRef]
- Petrov, R.G.; Malbet, F.; Weigelt, G.; Antonelli, P.; Beckmann, U.; Bresson, Y.; Chelli, A.; Dugué, M.; Duvert, G.; Gennari, S.; et al. AMBER, the near-infrared spectro-interferometric three-telescope VLTI instrument. Astron. Astrophys. 2007, 464, 1–12. [Google Scholar] [CrossRef]
- Domiciano de Souza, A. Long Baseline Interferometry of Rotating Stars across the HR Diagram: Flattening, Gravity Darkening, Differential Rotation. In Lecture Notes on Physics: The Rotation of Sun and Stars; Rozelot, J.P., Neiner, C., Eds.; Springer: Berlin/Heidelberg, Germany, 2009; Volume 765, pp. 171–194. [Google Scholar] [CrossRef]
- Domiciano de Souza, A. Interferometric Surface Mapping of Rapidly Rotating Stars: Application to the Be star Achernar. In Lecture Notes in Physics; Rozelot, J.P., Neiner, C., Eds.; Springer: Berlin/Heidelberg, Germany, 2016; Volume 914, p. 159. [Google Scholar] [CrossRef]
- Brown, R.H. The Intensity Interferometer; Its Application to Astronomy; Taylor and Francis LTD London and Halsted Press: London, UK, 1974. [Google Scholar]
- Domiciano de Souza, A.; Kervella, P.; Jankov, S.; Abe, L.; Vakili, F.; di Folco, E.; Paresce, F. The spinning-top Be star Achernar from VLTI-VINCI. Astron. Astrophys. 2003, 407, L47–L50. [Google Scholar] [CrossRef]
- Vinicius, M.M.F.; Zorec, J.; Leister, N.V.; Levenhagen, R.S. α Eridani: Rotational distortion, stellar and circumstellar activity. Astron. Astrophys. 2006, 446, 643–660. [Google Scholar] [CrossRef]
- Kanaan, S.; Meilland, A.; Stee, P.; Zorec, J.; Domiciano de Souza, A.; Frémat, Y.; Briot, D. Disk and wind evolution of Achernar: The breaking of the fellowship. Astron. Astrophys. 2008, 486, 785–798. [Google Scholar] [CrossRef]
- Kervella, P.; Domiciano de Souza, A. The polar wind of the fast rotating Be star Achernar. VINCI/VLTI interferometric observations of an elongated polar envelope. Astron. Astrophys. 2006, 453, 1059–1066. [Google Scholar] [CrossRef]
- van Belle, G.T. Interferometric observations of rapidly rotating stars. Astron. Astrophys. Rev. 2012, 20, 51. [Google Scholar] [CrossRef]
- Hadjara, M.; Petrov, R.G.; Jankov, S.; Cruzalèbes, P.; Spang, A.; Lagarde, S. Differential interferometry of the rapid rotator Regulus. Mon. Not. R. Astron. Soc. 2018, 480, 1263–1277. [Google Scholar] [CrossRef]
- Gray, R.O. The spectroscopic and photometric effects of rotation in the A-type stars. J. R. Astron. Soc. Can. 1988, 82, 336–348. [Google Scholar]
- McAlister, H.A.; ten Brummelaar, T.A.; Gies, D.R.; Huang, W.; Bagnuolo, W.G., Jr.; Shure, M.A.; Sturmann, J.; Sturmann, L.; Turner, N.H.; Taylor, S.F.; et al. First Results from the CHARA Array. I. An Interferometric and Spectroscopic Study of the Fast Rotator α Leonis (Regulus). Astrophys. J. 2005, 628, 439–452. [Google Scholar] [CrossRef]
- Aufdenberg, J.P.; Mérand, A.; Coudé du Foresto, V.; Absil, O.; Di Folco, E.; Kervella, P.; Ridgway, S.T.; Berger, D.H.; ten Brummelaar, T.A.; McAlister, H.A.; et al. First Results from the CHARA Array. VII. Long-Baseline Interferometric Measurements of Vega Consistent with a Pole-On, Rapidly Rotating Star. Astrophys. J. 2006, 645, 664–675. [Google Scholar] [CrossRef]
- Peterson, D.M.; Hummel, C.A.; Pauls, T.A.; Armstrong, J.T.; Benson, J.A.; Gilbreath, G.C.; Hindsley, R.B.; Hutter, D.J.; Johnston, K.J.; Mozurkewich, D.; et al. Vega is a rapidly rotating star. Nature 2006, 440, 896–899. [Google Scholar] [CrossRef]
- Peterson, D.M.; Hummel, C.A.; Pauls, T.A.; Armstrong, J.T.; Benson, J.A.; Gilbreath, G.C.; Hindsley, R.B.; Hutter, D.J.; Johnston, K.J.; Mozurkewich, D.; et al. Resolving the Effects of Rotation in Altair with Long-Baseline Interferometry. Astrophys. J. 2006, 636, 1087–1097. [Google Scholar] [CrossRef][Green Version]
- Challouf, M.; Nardetto, N.; Domiciano de Souza, A.; Mourard, D.; Tallon-Bosc, I.; Aroui, H.; Farrington, C.; Ligi, R.; Meilland, A.; Mouelhi, M. Flattening and surface-brightness of the fast-rotating star δ Persei with the visible VEGA/CHARA interferometer. Astron. Astrophys. 2017, 604, A51. [Google Scholar] [CrossRef][Green Version]
- Domiciano de Souza, A.; Vakili, F.; Jankov, S.; Janot-Pacheco, E.; Abe, L. Modelling rapid rotators for stellar interferometry. Astron. Astrophys. 2002, 393, 345–357. [Google Scholar] [CrossRef]
- Domiciano de Souza, A.; Zorec, J.; Vakili, F. CHARRON: Code for High Angular Resolution of Rotating Objects in Nature. In Proceedings of the SF2A-2012: Proceedings of the Annual meeting of the French Society of Astronomy and Astrophysics; Boissier, S., de Laverny, P., Nardetto, N., Samadi, R., Valls-Gabaud, D., Wozniak, H., Eds.; EDP Sciences: Les Ulis, France, 2012; pp. 321–324. [Google Scholar]
- Domiciano de Souza, A.; Zorec, J.; Jankov, S.; Vakili, F.; Abe, L.; Janot-Pacheco, E. Stellar differential rotation and inclination angle from spectro-interferometry. Astron. Astrophys. 2004, 418, 781–794. [Google Scholar] [CrossRef]
- Delaa, O.; Zorec, J.; Domiciano de Souza, A.; Mourard, D.; Perraut, K.; Stee, P.; Frémat, Y.; Monnier, J.; Kraus, S.; Che, X.; et al. Spectrally resolved interferometric observations of α Cephei and physical modeling of fast rotating stars. Astron. Astrophys. 2013, 555, A100. [Google Scholar] [CrossRef]
- Stee, P.; Allard, F.; Benisty, M.; Bigot, L.; Blind, N.; Boffin, H.; Borges Fernandes, M.; Carciofi, A.; Chiavassa, A.; Creevey, O.; et al. Science cases for a visible interferometer. arXiv 2017, arXiv:1703.02395. [Google Scholar]
- Meilland, A.; Millour, F.; Stee, P.; Domiciano de Souza, A.; Petrov, R.G.; Mourard, D.; Jankov, S.; Robbe-Dubois, S.; Spang, A.; Aristidi, E.; et al. An asymmetry detected in the disk of κ Canis Majoris with AMBER/VLTI. Astron. Astrophys. 2007, 464, 73–79. [Google Scholar] [CrossRef]
- Meilland, A.; Millour, F.; Stee, P.; Spang, A.; Petrov, R.; Bonneau, D.; Perraut, K.; Massi, F. δ Centauri: A new binary Be star detected by VLTI/AMBER spectro-interferometry. Astron. Astrophys. 2008, 488, L67–L70. [Google Scholar] [CrossRef]
- Meilland, A.; Stee, P.; Chesneau, O.; Jones, C. VLTI/MIDI observations of 7 classical Be stars. Astron. Astrophys. 2009, 505, 687–693. [Google Scholar] [CrossRef]
- Meilland, A.; Delaa, O.; Stee, P.; Kanaan, S.; Millour, F.; Mourard, D.; Bonneau, D.; Petrov, R.; Nardetto, N.; Marcotto, A.; et al. The binary Be star δ Scorpii at high spectral and spatial resolution. I. Disk geometry and kinematics before the 2011 periastron. Astron. Astrophys. 2011, 532, A80. [Google Scholar] [CrossRef]
- Meilland, A.; Millour, F.; Kanaan, S.; Stee, P.; Petrov, R.; Hofmann, K.H.; Natta, A.; Perraut, K. First spectro-interferometric survey of Be stars. I. Observations and constraints on the disk geometry and kinematics. Astron. Astrophys. 2012, 538, A110. [Google Scholar] [CrossRef]
- Meilland, A.; Stee, P.; Spang, A.; Malbet, F.; Massi, F.; Schertl, D. The binary Be star δ Scorpii at high spectral and spatial resolution. II. The circumstellar disk evolution after the periastron. Astron. Astrophys. 2013, 550, L5. [Google Scholar] [CrossRef]
- Delaa, O.; Stee, P.; Meilland, A.; Zorec, J.; Mourard, D.; Bério, P.; Bonneau, D.; Chesneau, O.; Clausse, J.M.; Cruzalebes, P.; et al. Kinematics and geometrical study of the Be stars 48 Persei and ψ Persei with the VEGA/CHARA interferometer. Astron. Astrophys. 2011, 529, A87. [Google Scholar] [CrossRef]
- Stee, P.; Delaa, O.; Monnier, J.D.; Meilland, A.; Perraut, K.; Mourard, D.; Che, X.; Schaefer, G.H.; Pedretti, E.; Smith, M.A.; et al. The relationship between γ Cassiopeiae’s X-ray emission and its circumstellar environment. II. Geometry and kinematics of the disk from MIRC and VEGA instruments on the CHARA Array. Astron. Astrophys. 2012, 545, A59. [Google Scholar] [CrossRef]
- Stee, P.; Meilland, A.; Stee, P.; Meilland, A. VLTI, CHARA and NPOI Observations of Be Stars. In Proceedings of the Circumstellar Dynamics at High Resolution, Proceedings on the ESO/IAG/USPWorkshop, Foz do Iguaçu, Brazil, 27 February–2 March 2012; Astronomical Society of the Pacific Conference Series; Carciofi, A.C., Rivinius, T., Eds.; ASP; 2012; Volume 464, p. 167. [Google Scholar]
- Cochetti, Y.R.; Arcos, C.; Kanaan, S.; Meilland, A.; Cidale, L.S.; Curé, M. Spectro-interferometric observations of a sample of Be stars. Setting limits to the geometry and kinematics of stable Be disks. Astron. Astrophys. 2019, 621, A123. [Google Scholar] [CrossRef]
- van Belle, G.T.; Ciardi, D.R.; ten Brummelaar, T.; McAlister, H.A.; Ridgway, S.T.; Berger, D.H.; Goldfinger, P.J.; Sturmann, J.; Sturmann, L.; Turner, N.; et al. First Results from the CHARA Array. III. Oblateness, Rotational Velocity, and Gravity Darkening of Alderamin. Astrophys. J. 2006, 637, 494–505. [Google Scholar] [CrossRef][Green Version]
- Pelletier, G.; Pudritz, R.E. Hydromagnetic Disk Winds in Young Stellar Objects and Active Galactic Nuclei. Astrophys. J. 1992, 394, 117. [Google Scholar] [CrossRef]
- Bodenheimer, P. Angular Momentum Evolution of Young Stars and Disks. Annu. Rev. Astron. Astrophys. 1995, 33, 199–238. [Google Scholar] [CrossRef]
- Ray, T. Losing spin: The angular momentum problem. Astron. Geophys. 2012, 53, 5–19. [Google Scholar] [CrossRef][Green Version]
- Larson, R.B. Processes in Collapsing Interstellar Clouds. Annu. Rev. Astron. Astrophys. 1973, 11, 219. [Google Scholar] [CrossRef]
- Burki, G.; Maeder, A. Observational tests on star formation I: Size variation of very young clusters through the Galaxy. Astron. Astrophys. 1976, 51, 247–254. [Google Scholar]
- Guthrie, B.N.G. The rotation of early-type stars and the problem of star formation. Mon. Not. R. Astron. Soc. 1984, 210, 159–171. [Google Scholar] [CrossRef][Green Version]
- Bodenheimer, P. Evolution of rotating interstellar clouds. III. On the formation of multiple star systems. Astrophys. J. 1978, 224, 488–496. [Google Scholar] [CrossRef]
- Deutsch, A.J. Maxwellian Distributions for Stellar Rotations. In Proceedings of the IAU Colloq. 4: Stellar Rotation; Slettebak, A., Ed.; Gordon and Breach Science Publishers: Philadelphia, PA, USA, 1970; p. 207. [Google Scholar]
- Maeder, A.; Grebel, E.K.; Mermilliod, J.C. Differences in the fractions of Be stars in galaxies. Astron. Astrophys. 1999, 346, 459–464. [Google Scholar]
- Huang, W.; Gies, D.R. Stellar Rotation in Young Clusters. II. Evolution of Stellar Rotation and Surface Helium Abundance. Astrophys. J. 2006, 648, 591–606. [Google Scholar] [CrossRef]
- Fabregat, J.; Torrejón, J.M. On the evolutionary status of Be stars. Astron. Astrophys. 2000, 357, 451–459. [Google Scholar]
- Keller, S.C.; Grebel, E.K.; Miller, G.J.; Yoss, K.M. UBVI and Hα Photometry of the h and χ Persei Cluster. Astron. J. 2001, 122, 248–256. [Google Scholar] [CrossRef]
- McSwain, M.V.; Gies, D.R. The Evolutionary Status of Be Stars: Results from a Photometric Study of Southern Open Clusters. Astrophys. J. Suppl. Ser. 2005, 161, 118–146. [Google Scholar] [CrossRef]
- Maeder, A. Influence of axial stellar rotation of age estimates of open star clusters. Astron. Astrophys. 1971, 10, 354–361. [Google Scholar]
- Packet, W. On the spin-up of the mass accreting component in a close binary system. Astron. Astrophys. 1981, 102, 17–19. [Google Scholar]
- Pols, O.R.; Cote, J.; Waters, L.B.F.M.; Heise, J. The formation of Be stars through close binary evolution. Astron. Astrophys. 1991, 241, 419. [Google Scholar]
- Klement, R.; Carciofi, A.C.; Rivinius, T.; Ignace, R.; Matthews, L.D.; Torstensson, K.; Gies, D.; Vieira, R.G.; Richardson, N.D.; Domiciano de Souza, A.; et al. Prevalence of SED Turndown among Classical Be Stars: Are All Be Stars Close Binaries? Astrophys. J. 2019, 885, 147. [Google Scholar] [CrossRef]
- Huang, S.S. A Statistical Study of the Rotation of the Stars. Astrophys. J. 1953, 118, 285. [Google Scholar] [CrossRef]
- van Dien, E. Axial Rotation of the Brighter Stars in the Pleiades Cluster. J. R. Astron. Soc. Can. 1948, 42, 249. [Google Scholar]
- Slettebak, A. The Spectra and Rotational Velocities of the Bright Stars of Draper Types B8-A2. Astrophys. J. 1954, 119, 146. [Google Scholar] [CrossRef]
- Slettebak, A.; Howard, R.F. Axial Rotation in the Brighter Stars of Draper Types B2-B5. Astrophys. J. 1955, 121, 102. [Google Scholar] [CrossRef]
- Kuiper, G.P. Problems of Double-Star Astronomy. I. Publ. Astron. Soc. Pac. 1935, 47, 15. [Google Scholar] [CrossRef]
- Chandrasekhar, S.; Münch, G. On the Integral Equation Governing the Distribution of the True and the Apparent Rotational Velocities of Stars. Astrophys. J. 1950, 111, 142. [Google Scholar] [CrossRef]
- Eddington, A.S. On a formula for correcting statistics for the effects of a known error of observation. Mon. Not. R. Astron. Soc. 1913, 73, 359–360. [Google Scholar] [CrossRef]
- Lucy, L.B. An iterative technique for the rectification of observed distributions. Astron. J. 1974, 79, 745. [Google Scholar] [CrossRef]
- Richardson, W.H. Bayesian-Based Iterative Method of Image Restoration. J. Opt. Soc. Am. 1972, 62, 55. [Google Scholar] [CrossRef]
- Yudin, R.V. Statistical analysis of intrinsic polarization, IR excess and projected rotational velocity distributions of classical Be stars. Astron. Astrophys. 2001, 368, 912–931. [Google Scholar] [CrossRef]
- Curé, M.; Rial, D.F.; Christen, A.; Cassetti, J. A method to deconvolve stellar rotational velocities. Astron. Astrophys. 2014, 565, A85. [Google Scholar] [CrossRef]
- Christen, A.; Escarate, P.; Curé, M.; Rial, D.F.; Cassetti, J. A method to deconvolve stellar rotational velocities II. The probability distribution function via Tikhonov regularization. Astron. Astrophys. 2016, 595, A50. [Google Scholar] [CrossRef]
- Orellana, R.; Escárate, P.; Curé, M.; Christen, A.; Carvajal, R.; Agüero, J.C. A method to deconvolve stellar rotational velocities. III. The probability distribution function via maximum likelihood utilizing finite distribution mixtures. Astron. Astrophys. 2019, 623, A138. [Google Scholar] [CrossRef]
- Yoon, S.C.; Langer, N.; Norman, C. Single star progenitors of long gamma-ray bursts. I. Model grids and redshift dependent GRB rate. Astron. Astrophys. 2006, 460, 199–208. [Google Scholar] [CrossRef]
- Martayan, C.; Zorec, J.; Frémat, Y.; Ekström, S. Can massive Be/Oe stars be progenitors of long gamma ray bursts? Astron. Astrophys. 2010, 516, A103. [Google Scholar] [CrossRef]
- Ramachandran, V.; Hamann, W.R.; Oskinova, L.M.; Gallagher, J.S.; Hainich, R.; Shenar, T.; Sander, A.A.C.; Todt, H.; Fulmer, L. Testing massive star evolution, star formation history, and feedback at low metallicity. Spectroscopic analysis of OB stars in the SMC Wing. Astron. Astrophys. 2019, 625, A104. [Google Scholar] [CrossRef]
- Ramachandran, V.; Hamann, W.R.; Hainich, R.; Oskinova, L.M.; Shenar, T.; Sander, A.A.C.; Todt, H.; Gallagher, J.S. Stellar population of the superbubble N 206 in the LMC. II. Parameters of the OB and WR stars, and the total massive star feedback. Astron. Astrophys. 2018, 615, A40. [Google Scholar] [CrossRef]
- Puls, J.; Vink, J.S.; Najarro, F. Mass loss from hot massive stars. Astron. Astrophys. Rev. 2008, 16, 209–325. [Google Scholar] [CrossRef]
- Krumholz, M.R. Massive Star Formation: A Tale of Two Theories. In Proceedings of the New Horizons in Astronomy: Frank N. Bash Symposium, Proceedings, Frank N Bash Symp. No 2005: New Horizons in Astronomy, Austin, TX, USA, 16–18 October 2005; Kannappan, S.J., Redfield, S., Kessler-Silacci, J.E., Landriau, M., Drory, N., Eds.; University of Chicago Press: Chicago, NY, USA, 2006; Volume 352, p. 31. [Google Scholar]
- Bonnell, I.A.; Vine, S.G.; Bate, M.R. Massive star formation: Nurture, not nature. Mon. Not. R. Astron. Soc. 2004, 349, 735–741. [Google Scholar] [CrossRef]
- Bally, J.; Zinnecker, H. The Birth of High-Mass Stars: Accretion and/or Mergers? Astron. J. 2005, 129, 2281–2293. [Google Scholar] [CrossRef][Green Version]
- Endal, A.S.; Sofia, S. Rotation in solar-type stars. I—Evolutionary models for the spin-down of the sun. Astrophys. J. 1981, 243, 625–640. [Google Scholar] [CrossRef]
- Potter, A.T.; Tout, C.A.; Eldridge, J.J. Towards a unified model of stellar rotation. Mon. Not. R. Astron. Soc. 2012, 419, 748–759. [Google Scholar] [CrossRef]
- Potter, A.T.; Chitre, S.M.; Tout, C.A. Stellar evolution of massive stars with a radiative α-Ω dynamo. Mon. Not. R. Astron. Soc. 2012, 424, 2358–2370. [Google Scholar] [CrossRef]
- Denissenkov, P.A.; Ivanova, N.S.; Weiss, A. Main-sequence stars of 10 and 30 M_sun: Approaching the steady-state rotation. Astron. Astrophys. 1999, 341, 181–189. [Google Scholar]
- Wolff, S.C.; Strom, S.E.; Hillenbrand, L.A. The Angular Momentum Evolution of 0.1-10 Msolar Stars from the Birth Line to the Main Sequence. Astrophys. J. 2004, 601, 979–999. [Google Scholar] [CrossRef]
- Tayler, R.J. Convection in rotating stars. Mon. Not. R. Astron. Soc. 1973, 165, 39. [Google Scholar] [CrossRef][Green Version]
- Deupree, R.G. Stellar Evolution with Arbitrary Rotation Laws. III. Convective Core Overshoot and Angular Momentum Distribution. Astrophys. J. 1998, 499, 340–347. [Google Scholar] [CrossRef]
- Deupree, R.G. Two-dimensional Hydrodynamic Simulations of Zero-Age Main-Sequence Convective Cores. Astrophys. J. 2000, 543, 395–405. [Google Scholar] [CrossRef]
- Kichatinov, L.L.; Rüdiger, G. Differential rotation in stellar convective envelopes. Astron. Lett. 1997, 23, 731–734. [Google Scholar]
- Browning, M.K.; Brun, A.S.; Toomre, J. Simulations of Core Convection in Rotating A-Type Stars: Differential Rotation and Overshooting. Astrophys. J. 2004, 601, 512–529. [Google Scholar] [CrossRef]
- Arnett, W.D.; Meakin, C. Turbulent Mixing in Stars: Theoretical Hurdles. In Proceedings of the Chemical Abundances in the Universe: Connecting First Stars to Planets; Cunha, K., Spite, M., Barbuy, B., Eds.; 2010; Volume 265, pp. 106–110. [Google Scholar] [CrossRef]
- Augustson, K.C.; Brun, A.S.; Toomre, J. The Magnetic Furnace: Intense Core Dynamos in B Stars. Astrophys. J. 2016, 829, 92. [Google Scholar] [CrossRef]
- Rosen, A.L.; Krumholz, M.R.; Ramirez-Ruiz, E. What Sets the Initial Rotation Rates of Massive Stars? Astrophys. J. 2012, 748, 97. [Google Scholar] [CrossRef]
- Haemmerlé, L.; Eggenberger, P.; Meynet, G.; Maeder, A.; Charbonnel, C. Star formation with disc accretion and rotation. I. Stars between 2 and 22 M⊙ at solar metallicity. Astron. Astrophys. 2013, 557, A112. [Google Scholar] [CrossRef][Green Version]
- Haemmerlé, L.; Eggenberger, P.; Meynet, G.; Maeder, A.; Charbonnel, C.; Klessen, R.S. Massive star formation by accretion. II. Rotation: How to circumvent the angular momentum barrier? Astron. Astrophys. 2017, 602, A17. [Google Scholar] [CrossRef][Green Version]
- Strittmatter, P.A. Stellar Rotation and Stellar Luminosity in Praesepe. Astrophys. J. 1966, 144, 430. [Google Scholar] [CrossRef]
- Strittmatter, P.A.; Sargent, W.L.W. Stellar Rotation and the Position of the Metallic-Line Stars in the Color-Magnitude Diagram. Astrophys. J. 1966, 145, 130. [Google Scholar] [CrossRef]
- Maeder, A. Stellar Rotation. Publ. Obs. Geneva 1968, 75, 125. [Google Scholar]
- Smith, R.C. Effects of rotation in the colour-magnitude diagrams of Praesepe and the Hyades. Mon. Not. R. Astron. Soc. 1971, 151, 463. [Google Scholar] [CrossRef][Green Version]
- Cotton, A.; Smith, R.C. The theoretical spread of the main sequence due to stellar rotation. Observatory 1983, 103, 8–12. [Google Scholar]
- Oke, J.B.; Greenstein, J.L. The Rotational Velocities of - - and G-Type Giant Stars. Astrophys. J. 1954, 120, 384. [Google Scholar] [CrossRef]
- Sandage, A.R. Axial Rotation and Stellar Evolution. Astrophys. J. 1955, 122, 263. [Google Scholar] [CrossRef]
- Danziger, I.J.; Faber, S.M. Rotation of evolving A and F stars. Astron. Astrophys. 1972, 18, 428. [Google Scholar]
- Ekström, S.; Meynet, G.; Maeder, A.; Barblan, F. Evolution towards the critical limit and the origin of Be stars. Astron. Astrophys. 2008, 478, 467–485. [Google Scholar] [CrossRef]
- Yang, W.; Bi, S.; Meng, X.; Tian, Z. Evolution of Rotational Velocities of A-type Stars. Astrophys. J. Lett. 2013, 765, L36. [Google Scholar] [CrossRef][Green Version]
- Sun, W.; Duan, X.W.; Deng, L.; de Grijs, R. Exploring the Stellar Rotation of Early-type Stars in the LAMOST Medium-resolution Survey. II. Statistics. Astrophys. J. 2021, 921, 145. [Google Scholar] [CrossRef]
- Sun, W.; Duan, X.W.; Deng, L.; de Grijs, R.; Zhang, B.; Liu, C. Exploring the Stellar Rotation of Early-type Stars in the LAMOST Medium-resolution Survey. I. Catalog. Astrophys. J. Suppl. Ser. 2021, 257, 22. [Google Scholar] [CrossRef]
- Rhodes, E.J., Jr.; Deubner, F.L.; Ulrich, R.K. A new technique for measuring solar rotation. Astrophys. J. 1979, 227, 629–637. [Google Scholar] [CrossRef]
- Deubner, F.L.; Ulrich, R.K.; Rhodes, E.J., Jr. Solar p-mode oscillations as a tracer of radial differential rotation. Astron. Astrophys. 1979, 72, 177–185. [Google Scholar]
- Ando, H. A New Method for Determining the Internal Rotational Angular Velocity of the Stars. Astrophys. Space Sci. 1980, 73, 159–174. [Google Scholar] [CrossRef]
- Reese, D.R. Internal rapid rotation and its implications for stellar structure and pulsations. Eur. Phys. J. Conf. 2015, 101, 05007. [Google Scholar] [CrossRef]
- Aerts, C.; Thoul, A.; Daszyńska, J.; Scuflaire, R.; Waelkens, C.; Dupret, M.A.; Niemczura, E.; Noels, A. Asteroseismology of HD 129929: Core Overshooting and Nonrigid Rotation. Science 2003, 300, 1926–1928. [Google Scholar] [CrossRef]
- Pamyatnykh, A.A.; Handler, G.; Dziembowski, W.A. Asteroseismology of the β Cephei star ν Eridani: Interpretation and applications of the oscillation spectrum. Mon. Not. R. Astron. Soc. 2004, 350, 1022–1028. [Google Scholar] [CrossRef]
- Briquet, M.; Morel, T.; Thoul, A.; Scuflaire, R.; Miglio, A.; Montalbán, J.; Dupret, M.A.; Aerts, C. An asteroseismic study of the β Cephei star θ Ophiuchi: Constraints on global stellar parameters and core overshooting. Mon. Not. R. Astron. Soc. 2007, 381, 1482–1488. [Google Scholar] [CrossRef]
- Dziembowski, W.A.; Pamyatnykh, A.A. The two hybrid B-type pulsators: ν Eridani and 12 Lacertae. Mon. Not. R. Astron. Soc. 2008, 385, 2061–2068. [Google Scholar] [CrossRef]
- Aerts, C.; Mathis, S.; Rogers, T.M. Angular Momentum Transport in Stellar Interiors. Annu. Rev. Astron. Astrophys. 2019, 57, 35–78. [Google Scholar] [CrossRef]
- Pedersen, M.G. Internal rotation and inclinations of slowly pulsating B stars: Evidence of interior angular momentum transport. arXiv 2022, arXiv:2208.14497. [Google Scholar] [CrossRef]
- Hatta, Y.; Sekii, T.; Takata, M.; Kurtz, D.W. The Two-dimensional Internal Rotation of KIC 11145123. Astrophys. J. 2019, 871, 135. [Google Scholar] [CrossRef]
- Burssens, S.; Bowman, D.M.; Michielsen, M.; Simón-Díaz, S.; Aerts, C. Internal rotation and mixing in the massive star HD192575. In Proceedings of the Posters from the TESS Science Conference II (TSC2), Virtually, 2–6 August 2021; p. 75. [Google Scholar] [CrossRef]
- Salmon, S.J.A.J.; Moyano, F.D.; Eggenberger, P.; Haemmerlé, L.; Buldgen, G. Backtracing the internal rotation history of the β Cep star HD 129929. Astron. Astrophys. 2022, 664, L1. [Google Scholar] [CrossRef]
- Salmon, S.J.A.J.; Montalbán, J.; Reese, D.R.; Dupret, M.A.; Eggenberger, P. The puzzling new class of variable stars in NGC 3766: Old friend pulsators? Astron. Astrophys. 2014, 569, A18. [Google Scholar] [CrossRef]
- Pedersen, M.G.; Aerts, C.; Pápics, P.I.; Michielsen, M.; Gebruers, S.; Rogers, T.M.; Molenberghs, G.; Burssens, S.; Garcia, S.; Bowman, D.M. Internal mixing of rotating stars inferred from dipole gravity modes. Nat. Astron. 2021, 5, 715–722. [Google Scholar] [CrossRef]
- Moravveji, E.; Townsend, R.H.D.; Aerts, C.; Mathis, S. Sub-inertial Gravity Modes in the B8V Star KIC 7760680 Reveal Moderate Core Overshooting and Low Vertical Diffusive Mixing. Astrophys. J. 2016, 823, 130. [Google Scholar] [CrossRef]
- Stoeckley, T.R.; Morris, C.S. Rotational distortion of stellar absorption lines. I. Parameters from photographic spectra. Astrophys. J. 1974, 188, 579–594. [Google Scholar] [CrossRef]
- Buscombe, W.; Stoeckley, T.R. Absorption Line Profiles and Rotational Velocities for 59 Stars. Astrophys. Space Sci. 1975, 37, 197–220. [Google Scholar] [CrossRef]
- Stoeckley, T.R.; Carroll, R.W.; Miller, R.D. Absorption line profiles for 39 rapidly rotating stars. Mon. Not. R. Astron. Soc. 1984, 208, 459. [Google Scholar] [CrossRef]
- Elste, G.H. Line Shapes of Rotating Stars with Application to Alpha Lyrae. Astrophys. J. 1992, 384, 284. [Google Scholar] [CrossRef]
- de Jager, C.; Nieuwenhuijzen, H.; van der Hucht, K.A. Mass loss rates in the Hertzsprung-Russell diagram. Astron. Astrophys. 1988, 72, 259–289. [Google Scholar]
- Kudritzki, R.P.; Puls, J. Winds from Hot Stars. Annu. Rev. Astron. Astrophys. 2000, 38, 613–666. [Google Scholar] [CrossRef]
- Vink, J.S.; de Koter, A.; Lamers, H.J.G.L.M. New theoretical mass-loss rates of O and B stars. Astron. Astrophys. 2000, 362, 295–309. [Google Scholar]
- Keszthelyi, Z.; de Koter, A.; Götberg, Y.; Meynet, G.; Brands, S.A.; Petit, V.; Carrington, M.; David-Uraz, A.; Geen, S.T.; Georgy, C.; et al. The effects of surface fossil magnetic fields on massive star evolution: IV. Grids of models at Solar, LMC, and SMC metallicities. Mon. Not. R. Astron. Soc. 2022, 517, 2028–2055. [Google Scholar] [CrossRef]
- Donati, J.F.; Semel, M.; Carter, B.D.; Rees, D.E.; Collier Cameron, A. Spectropolarimetric observations of active stars. Mon. Not. R. Astron. Soc. 1997, 291, 658–682. [Google Scholar] [CrossRef]
- Kochukhov, O.; Makaganiuk, V.; Piskunov, N. Least-squares deconvolution of the stellar intensity and polarization spectra. Astron. Astrophys. 2010, 524, A5. [Google Scholar] [CrossRef]
- Oksala, M.E.; Wade, G.A.; Townsend, R.H.D.; Owocki, S.P.; Kochukhov, O.; Neiner, C.; Alecian, E.; Grunhut, J. Revisiting the Rigidly Rotating Magnetosphere model for σ Ori E—I. Observations and data analysis. Mon. Not. R. Astron. Soc. 2012, 419, 959–970. [Google Scholar] [CrossRef]
- Grunhut, J.H.; Wade, G.A.; MiMeS Collaboration. The Incidence of Magnetic Fields in Massive Stars: An Overview of the MiMeS Survey Component. AIP Conf. Proc. 2012, 1429, 67. [Google Scholar]
- Babel, J.; Montmerle, T. On the Periodic X-Ray Emission from the O7 V Star theta 1 Orionis C. Astrophys. J. Lett. 1997, 485, L29–L32. [Google Scholar] [CrossRef]
- ud-Doula, A.; Owocki, S.P. Dynamical Simulations of Magnetically Channeled Line-driven Stellar Winds. I. Isothermal, Nonrotating, Radially Driven Flow. Astrophys. J. 2002, 576, 413–428. [Google Scholar] [CrossRef]
- Petit, V.; Owocki, S.P.; Wade, G.A.; Cohen, D.H.; Sundqvist, J.O.; Gagné, M.; Maíz Apellániz, J.; Oksala, M.E.; Bohlender, D.A.; Rivinius, T.; et al. A magnetic confinement versus rotation classification of massive-star magnetospheres. Mon. Not. R. Astron. Soc. 2013, 429, 398–422. [Google Scholar] [CrossRef]
- Townsend, R.H.D.; Owocki, S.P. A rigidly rotating magnetosphere model for circumstellar emission from magnetic OB stars. Mon. Not. R. Astron. Soc. 2005, 357, 251–264. [Google Scholar] [CrossRef]
- Ud-Doula, A.; Owocki, S.P.; Townsend, R.H.D. Dynamical simulations of magnetically channelled line-driven stellar winds—II. The effects of field-aligned rotation. Mon. Not. R. Astron. Soc. 2008, 385, 97–108. [Google Scholar] [CrossRef]
- Shultz, M.; Wade, G.; Rivinius, T.; Neiner, C.; Alecian, E.; Petit, V.; Grunhut, J.; MiMeS Collaboration; BinaMIcS Collaboration. What can magnetic early B-type stars tell us about early B-type stars in general? In Proceedings of the Lives and Death-Throes of Massive Stars; Eldridge, J.J., Bray, J.C., McClelland, L.A.S., Xiao, L., Eds.; Cambridge University Press: Cambridge, UK, 2017; Volume 329, pp. 126–130. [Google Scholar] [CrossRef]
- Shultz, M.E.; Owocki, S.; Rivinius, T.; Wade, G.A.; Neiner, C.; Alecian, E.; Kochukhov, O.; Bohlender, D.; ud-Doula, A.; Landstreet, J.D.; et al. The magnetic early B-type stars—IV. Breakout or leakage? H α emission as a diagnostic of plasma transport in centrifugal magnetospheres. Mon. Not. R. Astron. Soc. 2020, 499, 5379–5395. [Google Scholar] [CrossRef]
- Shultz, M.E.; Owocki, S.P.; ud-Doula, A.; Biswas, A.; Bohlender, D.; Chandra, P.; Das, B.; David-Uraz, A.; Khalack, V.; Kochukhov, O.; et al. MOBSTER—VI. The crucial influence of rotation on the radio magnetospheres of hot stars. Mon. Not. R. Astron. Soc. 2022, 513, 1429–1448. [Google Scholar] [CrossRef]
- Shultz, M.E.; Wade, G.A.; Rivinius, T.; Alecian, E.; Neiner, C.; Petit, V.; Owocki, S.; ud-Doula, A.; Kochukhov, O.; Bohlender, D.; et al. The magnetic early B-type stars—III. A main-sequence magnetic, rotational, and magnetospheric biography. Mon. Not. R. Astron. Soc. 2019, 490, 274–295. [Google Scholar] [CrossRef]
- Weber, E.J.; Davis, L.J. The Angular Momentum of the Solar Wind. Astrophys. J. 1967, 148, 217–227. [Google Scholar] [CrossRef]
- Ud-Doula, A.; Owocki, S.P.; Townsend, R.H.D. Dynamical simulations of magnetically channelled line-driven stellar winds—III. Angular momentum loss and rotational spin-down. Mon. Not. R. Astron. Soc. 2009, 392, 1022–1033. [Google Scholar] [CrossRef]
- Shultz, M.E.; Wade, G.A.; Rivinius, T.; Neiner, C.; Alecian, E.; Bohlender, D.; Monin, D.; Sikora, J.; MiMeS Collaboration; BinaMIcS Collaboration. The magnetic early B-type stars I: Magnetometry and rotation. Mon. Not. R. Astron. Soc. 2018, 475, 5144–5178. [Google Scholar] [CrossRef]
- Shultz, M.E.; Wade, G.A.; Rivinius, T.; Alecian, E.; Neiner, C.; Petit, V.; Wisniewski, J.P.; MiMeS Collaboration; BinaMIcS Collaboration. The magnetic early B-type Stars II: Stellar atmospheric parameters in the era of Gaia. Mon. Not. R. Astron. Soc. 2019, 485, 1508–1527. [Google Scholar] [CrossRef]
- Shultz, M.; Rivinius, T.; Das, B.; Wade, G.A.; Chandra, P. The accelerating rotation of the magnetic He-weak star HD 142990. Mon. Not. R. Astron. Soc. 2019, 486, 5558–5566. [Google Scholar] [CrossRef]
- Grunhut, J.H.; Wade, G.A.; Neiner, C.; Oksala, M.E.; Petit, V.; Alecian, E.; Bohlender, D.A.; Bouret, J.C.; Henrichs, H.F.; Hussain, G.A.J.; et al. The MiMeS survey of Magnetism in Massive Stars: Magnetic analysis of the O-type stars. Mon. Not. R. Astron. Soc. 2017, 465, 2432–2470. [Google Scholar] [CrossRef]
- Fossati, L.; Castro, N.; Schöller, M.; Hubrig, S.; Langer, N.; Morel, T.; Briquet, M.; Herrero, A.; Przybilla, N.; Sana, H.; et al. B fields in OB stars (BOB): Low-resolution FORS2 spectropolarimetry of the first sample of 50 massive stars. Astron. Astrophys. 2015, 582, A45. [Google Scholar] [CrossRef]
- Alecian, E.; Neiner, C.; Wade, G.A.; Mathis, S.; Bohlender, D.; Cébron, D.; Folsom, C.; Grunhut, J.; Le Bouquin, J.B.; Petit, V.; et al. The BinaMIcS project: Understanding the origin of magnetic fields in massive stars through close binary systems. In Proceedings of the New Windows on Massive Stars; Meynet, G., Georgy, C., Groh, J., Stee, P., Eds.; Cambridge University Press: Cambridge, UK, 2015; Volume 307, pp. 330–335. [Google Scholar] [CrossRef]
- Castro, N.; Fossati, L.; Hubrig, S.; Simón-Díaz, S.; Schöller, M.; Ilyin, I.; Carrol, T.A.; Langer, N.; Morel, T.; Schneider, F.R.N.; et al. B fields in OB stars (BOB). Detection of a strong magnetic field in the O9.7 V star HD 54879. Astron. Astrophys. 2015, 581, A81. [Google Scholar] [CrossRef][Green Version]
- Fossati, L.; Castro, N.; Morel, T.; Langer, N.; Briquet, M.; Carroll, T.A.; Hubrig, S.; Nieva, M.F.; Oskinova, L.M.; Przybilla, N.; et al. B fields in OB stars (BOB): On the detection of weak magnetic fields in the two early B-type stars beta CMa and epsilon CMa. Possible lack of a “magnetic desert” in massive stars. Astron. Astrophys. 2015, 574, A20. [Google Scholar] [CrossRef]
- Hubrig, S.; Schöller, M.; Fossati, L.; Morel, T.; Castro, N.; Oskinova, L.M.; Przybilla, N.; Eikenberry, S.S.; Nieva, M.F.; Langer, N. B fields in OB stars (BOB): FORS 2 spectropolarimetric follow-up of the two rare rigidly rotating magnetosphere stars HD 23478 and HD 345439. Astron. Astrophys. 2015, 578, L3. [Google Scholar] [CrossRef]
- Morel, T.; Castro, N.; Fossati, L.; Hubrig, S.; Langer, N.; Przybilla, N.; Schöller, M.; Carroll, T.; Ilyin, I.; Irrgang, A.; et al. The B Fields in OB Stars (BOB) Survey. In Proceedings of the New Windows on Massive Stars; Meynet, G., Georgy, C., Groh, J., Stee, P., Eds.; Cambridge University Press: Cambridge, UK, 2015; Volume 307, pp. 342–347. [Google Scholar] [CrossRef]
- Przybilla, N.; Fossati, L.; Hubrig, S.; Nieva, M.F.; Järvinen, S.P.; Castro, N.; Schöller, M.; Ilyin, I.; Butler, K.; Schneider, F.R.N.; et al. B fields in OB stars (BOB): Detection of a magnetic field in the He-strong star CPD-57° 3509. Astron. Astrophys. 2016, 587, A7. [Google Scholar] [CrossRef]
- Schöller, M.; Hubrig, S.; Fossati, L.; Carroll, T.A.; Briquet, M.; Oskinova, L.M.; Järvinen, S.; Ilyin, I.; Castro, N.; Morel, T.; et al. B fields in OB stars (BOB): Concluding the FORS 2 observing campaign. Astron. Astrophys. 2017, 599, A66. [Google Scholar] [CrossRef]
- Oksala, M.E.; Neiner, C.; Georgy, C.; Przybilla, N.; Keszthelyi, Z.; Wade, G.; Mathis, S.; Blazère, A.; Buysschaert, B. The evolution of magnetic fields in hot stars. In Proceedings of the The Lives and Death-Throes of Massive Stars; Eldridge, J.J., Bray, J.C., McClelland, L.A.S., Xiao, L., Eds.; 2017; Volume 329, pp. 141–145. [Google Scholar] [CrossRef]
- Neiner, C.; Oksala, M.E.; Georgy, C.; Przybilla, N.; Mathis, S.; Wade, G.; Kondrak, M.; Fossati, L.; Blazère, A.; Buysschaert, B.; et al. Discovery of magnetic A supergiants: The descendants of magnetic main-sequence B stars. Mon. Not. R. Astron. Soc. 2017, 471, 1926–1935. [Google Scholar] [CrossRef]
- Kobzar, O.; Khalack, V.; Bohlender, D.; Mathys, G.; Shultz, M.E.; Bowman, D.M.; Paunzen, E.; Lovekin, C.; David-Uraz, A.; Sikora, J.; et al. Analysis of eight magnetic chemically peculiar stars with rotational modulation. Mon. Not. R. Astron. Soc. 2022. [Google Scholar] [CrossRef]
- Shultz, M.; Wade, G.A.; Neiner, C.; Kochukhov, O. Magnetic Stars Observed by BRITE. In Proceedings of the 3rd BRITE Science Conference; Wade, G.A., Baade, D., Guzik, J.A., Smolec, R., Eds.; Polish Astronomical Society: Warsawm, Poland, 2018; Volume 8, pp. 146–153. [Google Scholar]
- Schneider, F.R.N.; Ohlmann, S.T.; Podsiadlowski, P.; Röpke, F.K.; Balbus, S.A.; Pakmor, R.; Springel, V. Stellar mergers as the origin of magnetic massive stars. Nature 2019, 574, 211–214. [Google Scholar] [CrossRef] [PubMed]
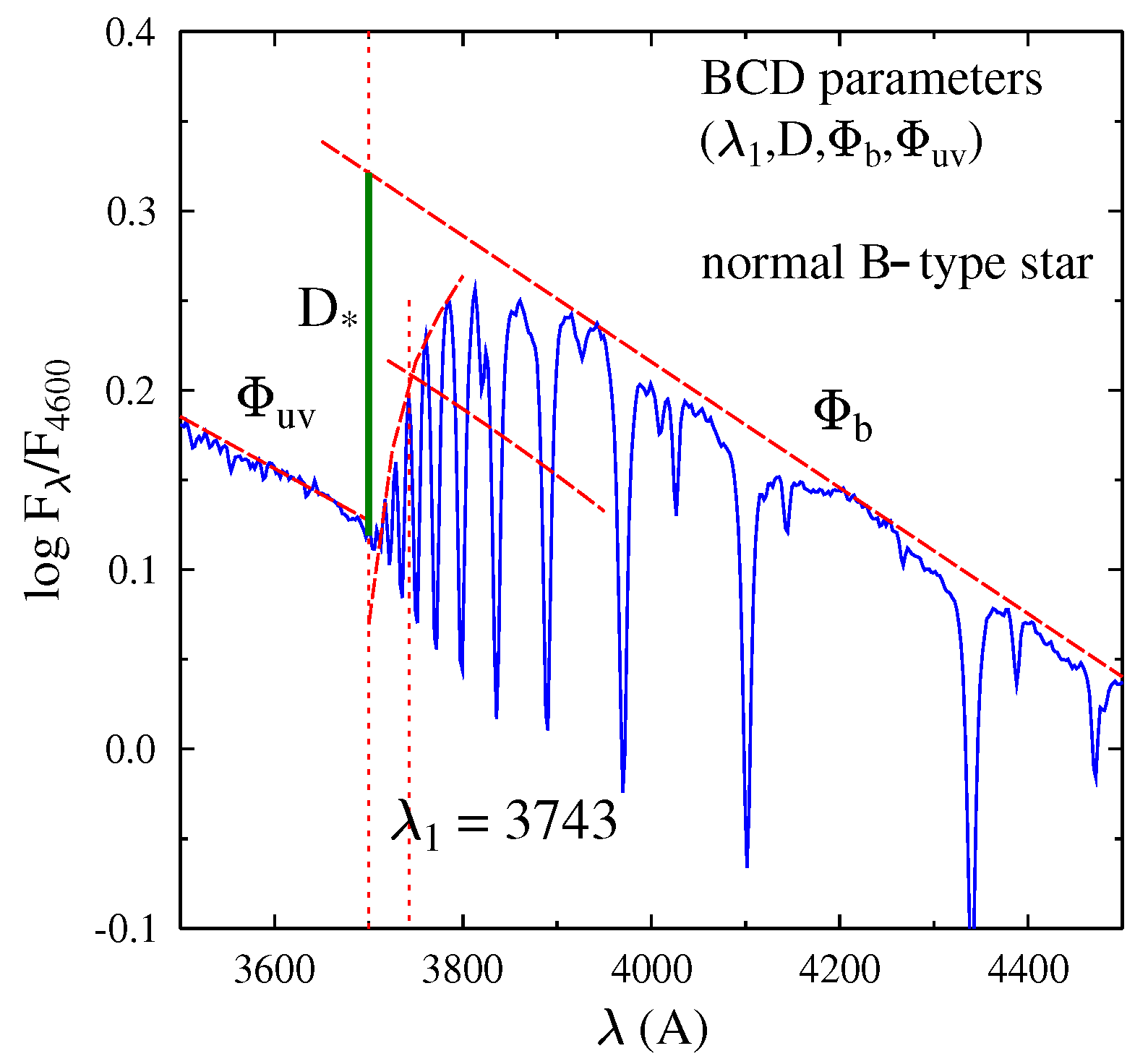
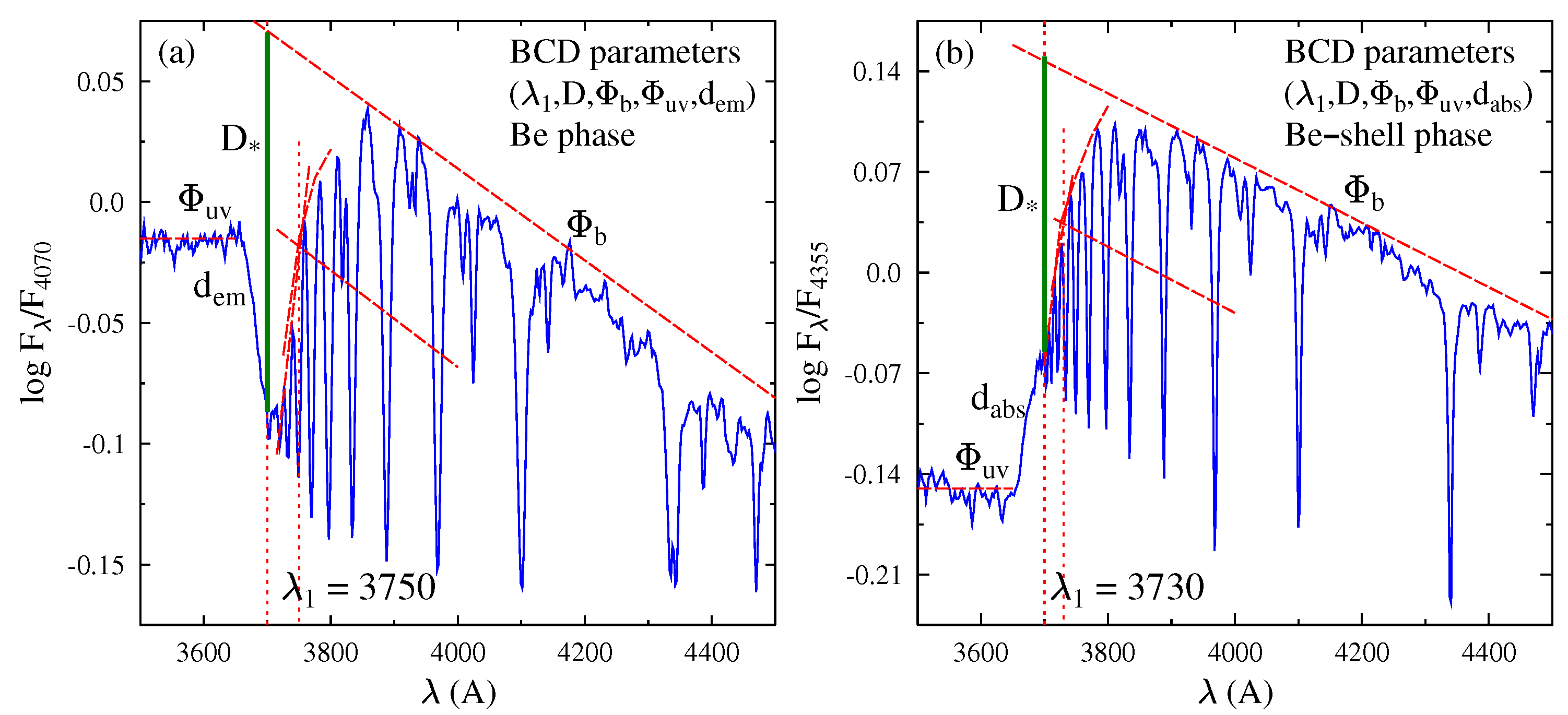
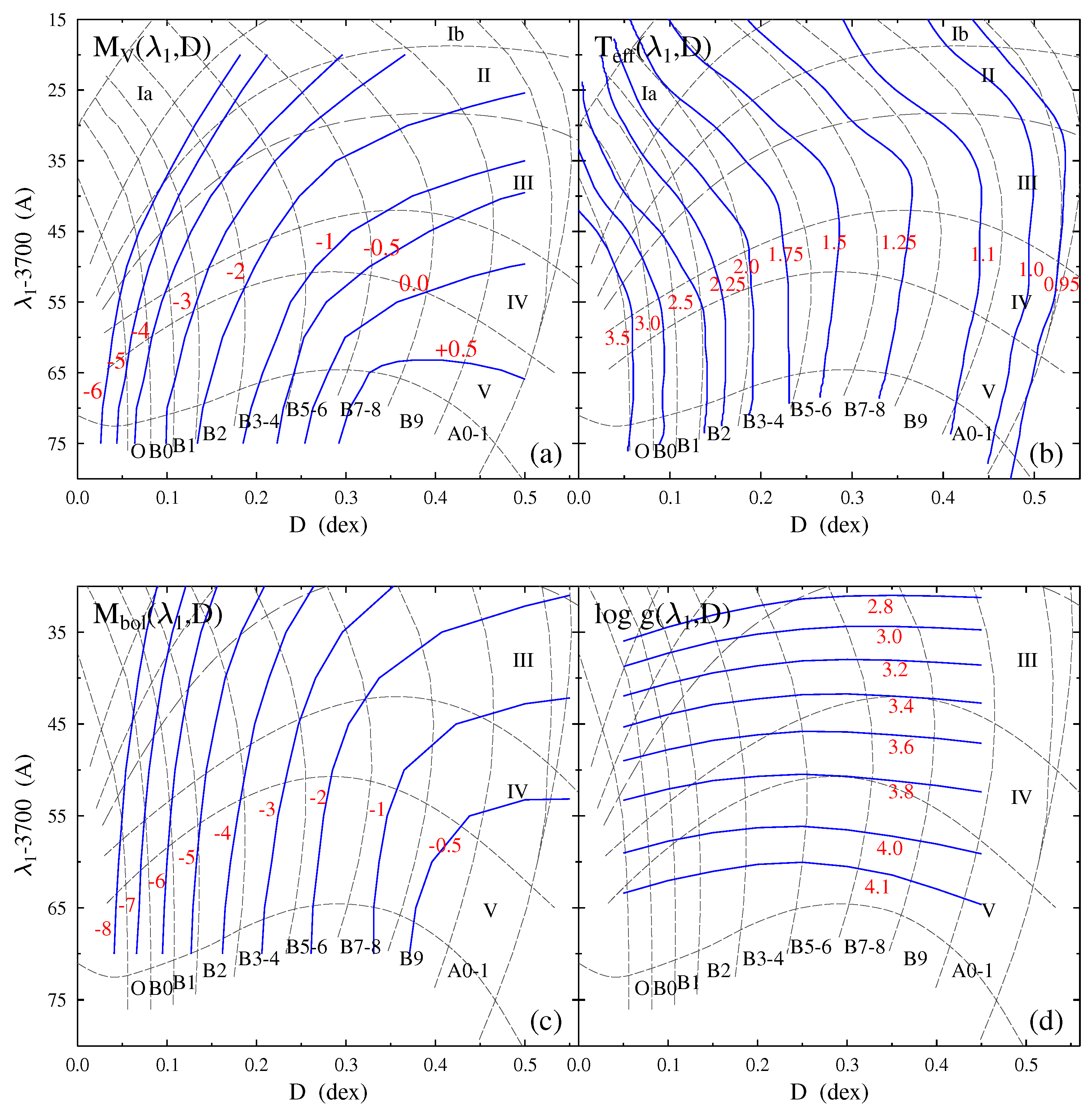
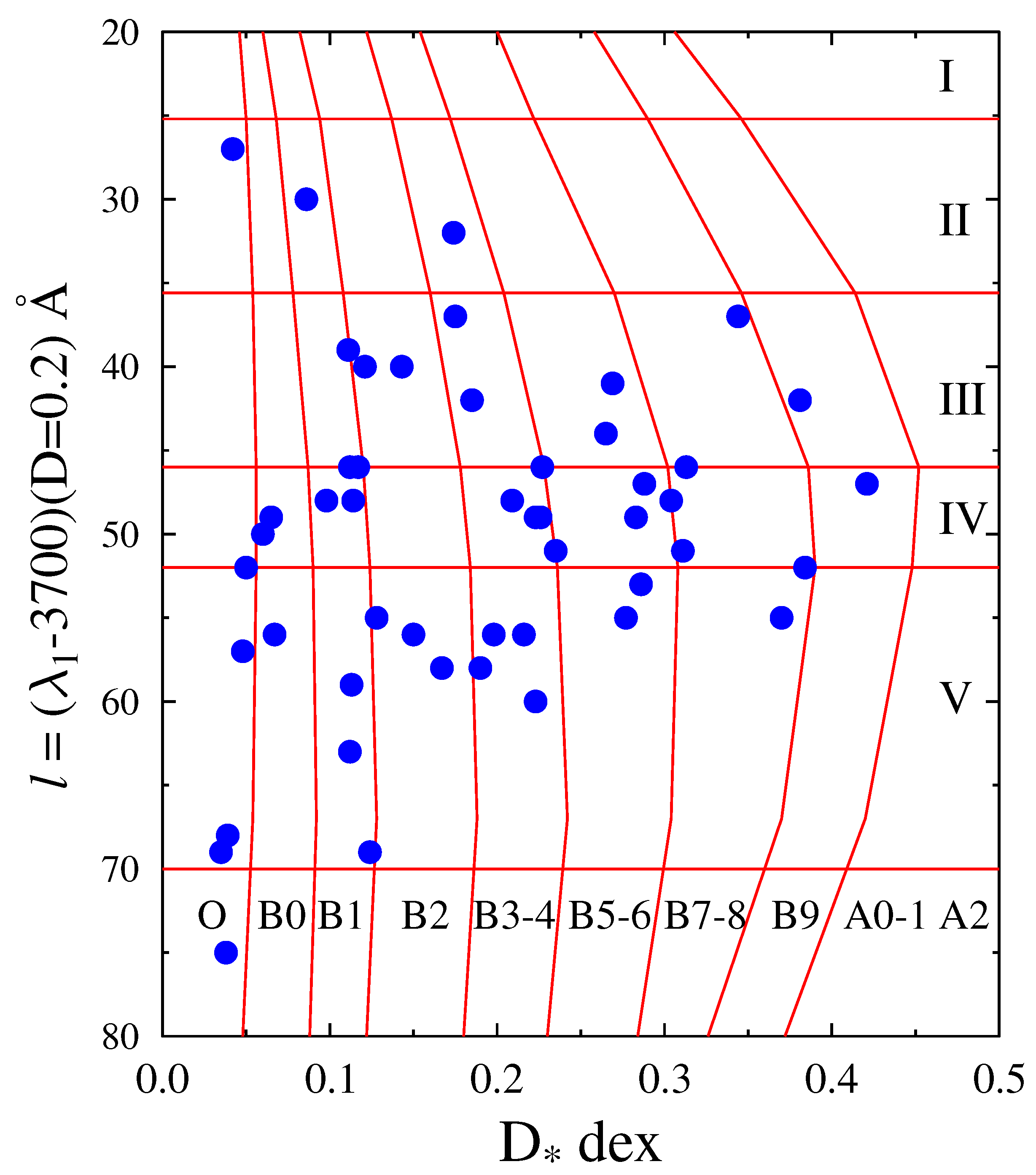

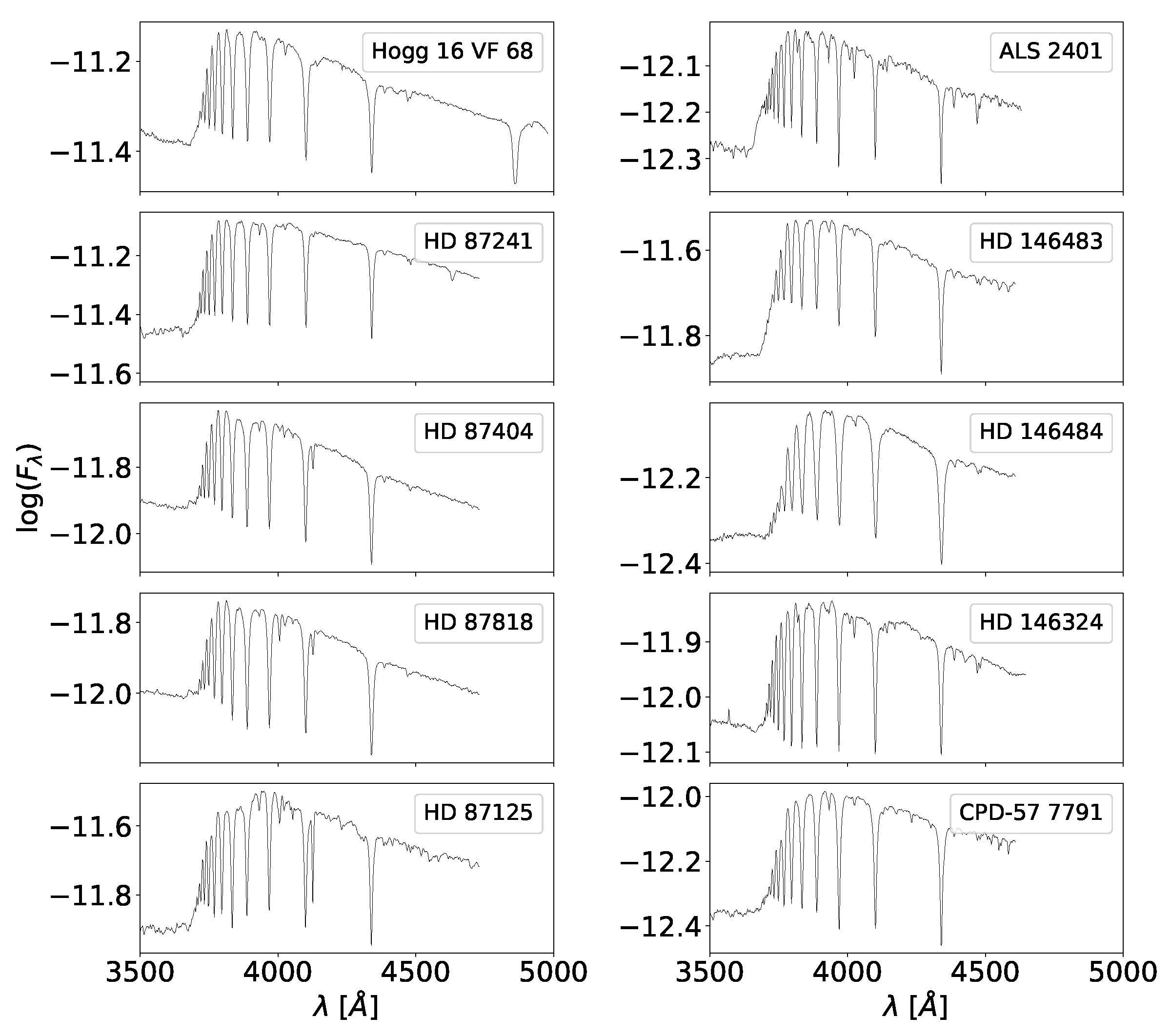

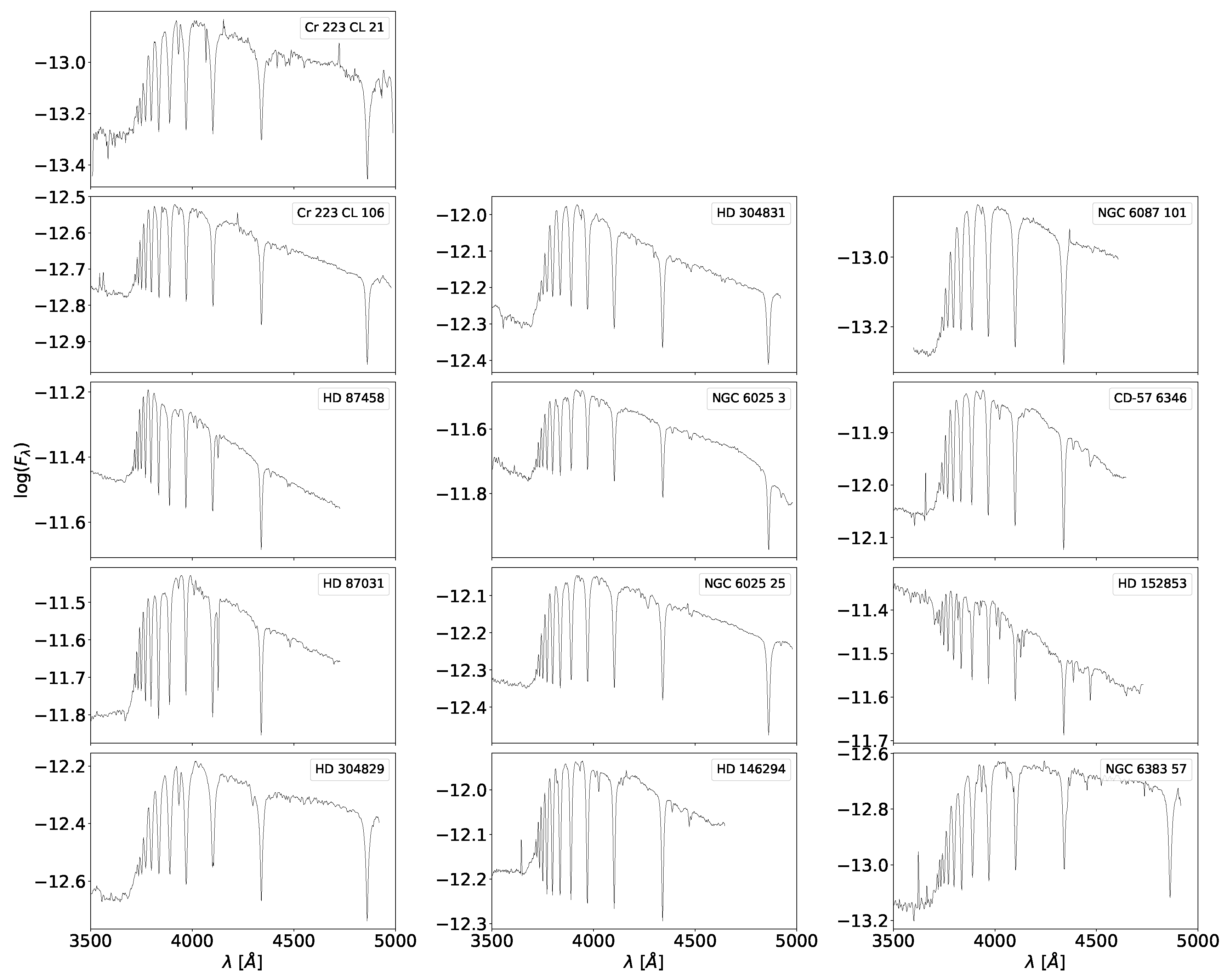
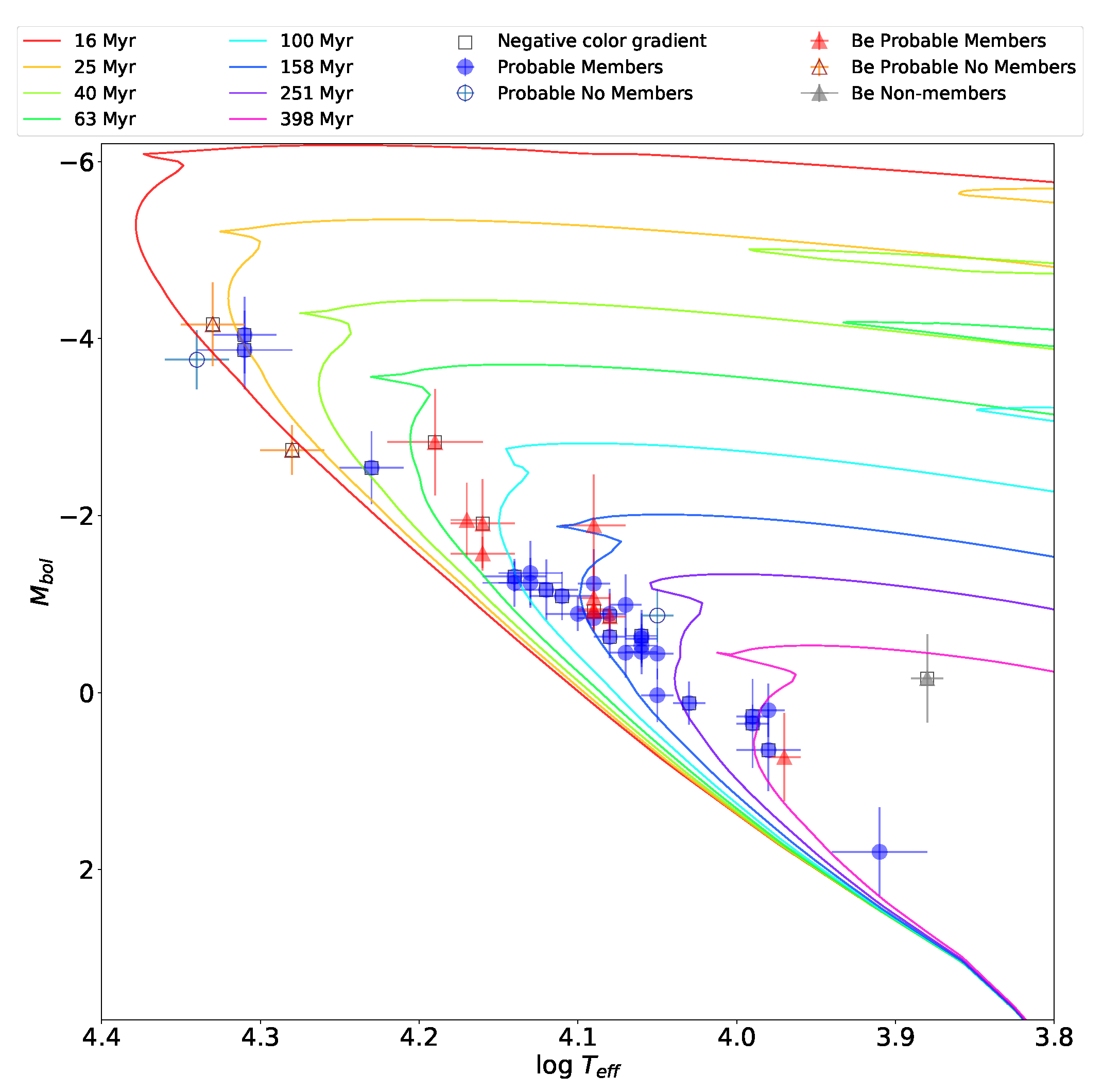
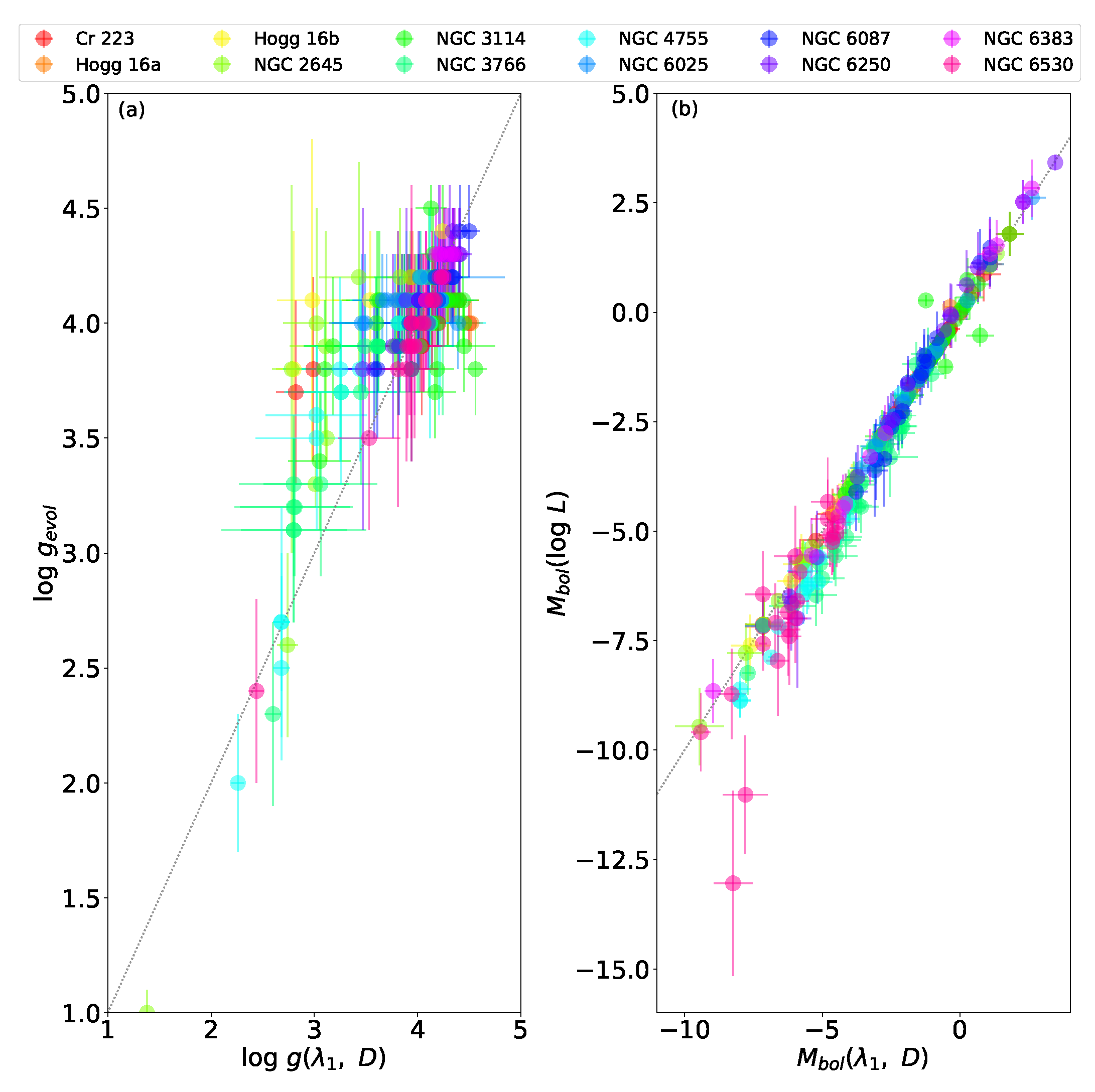


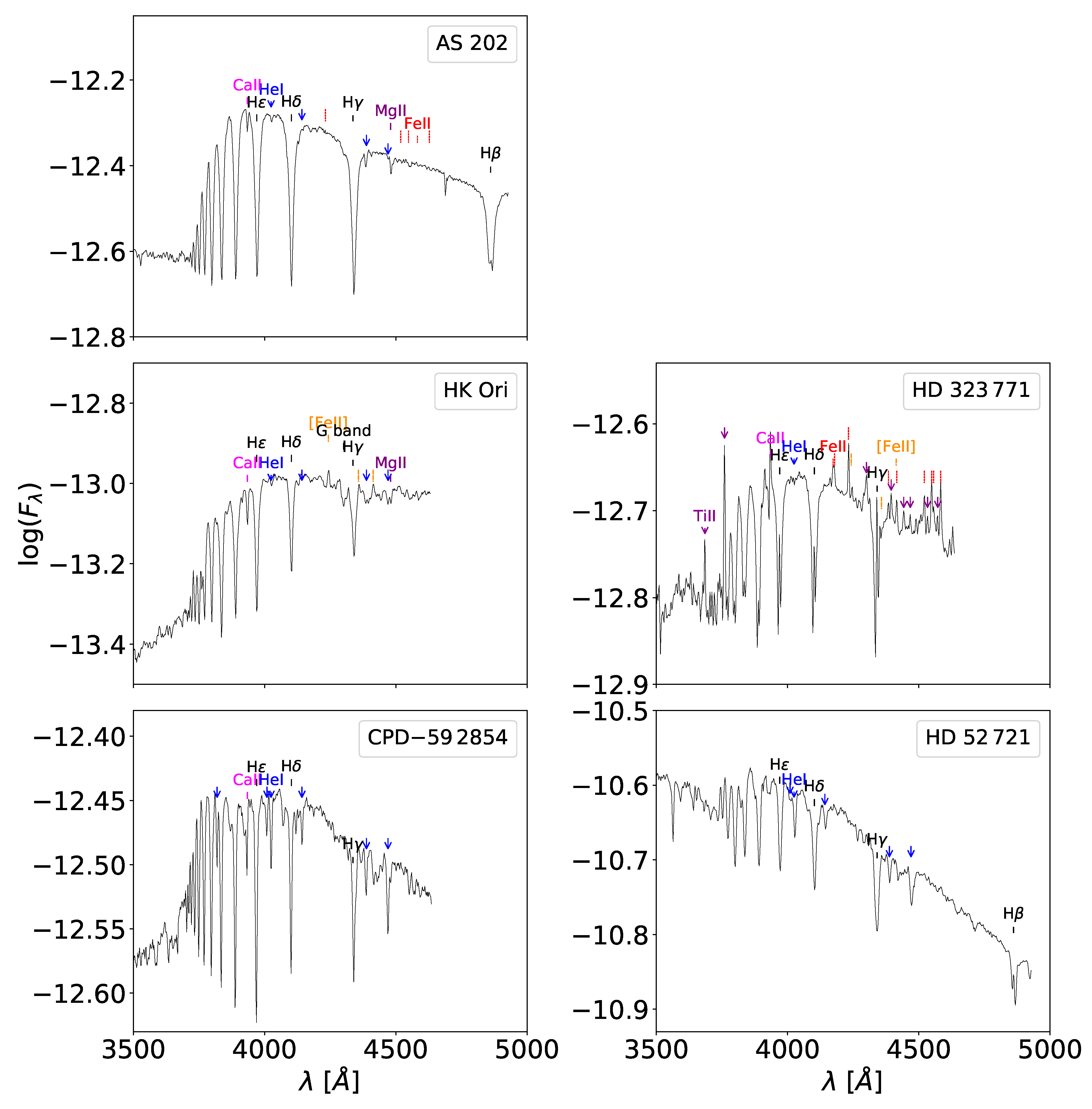
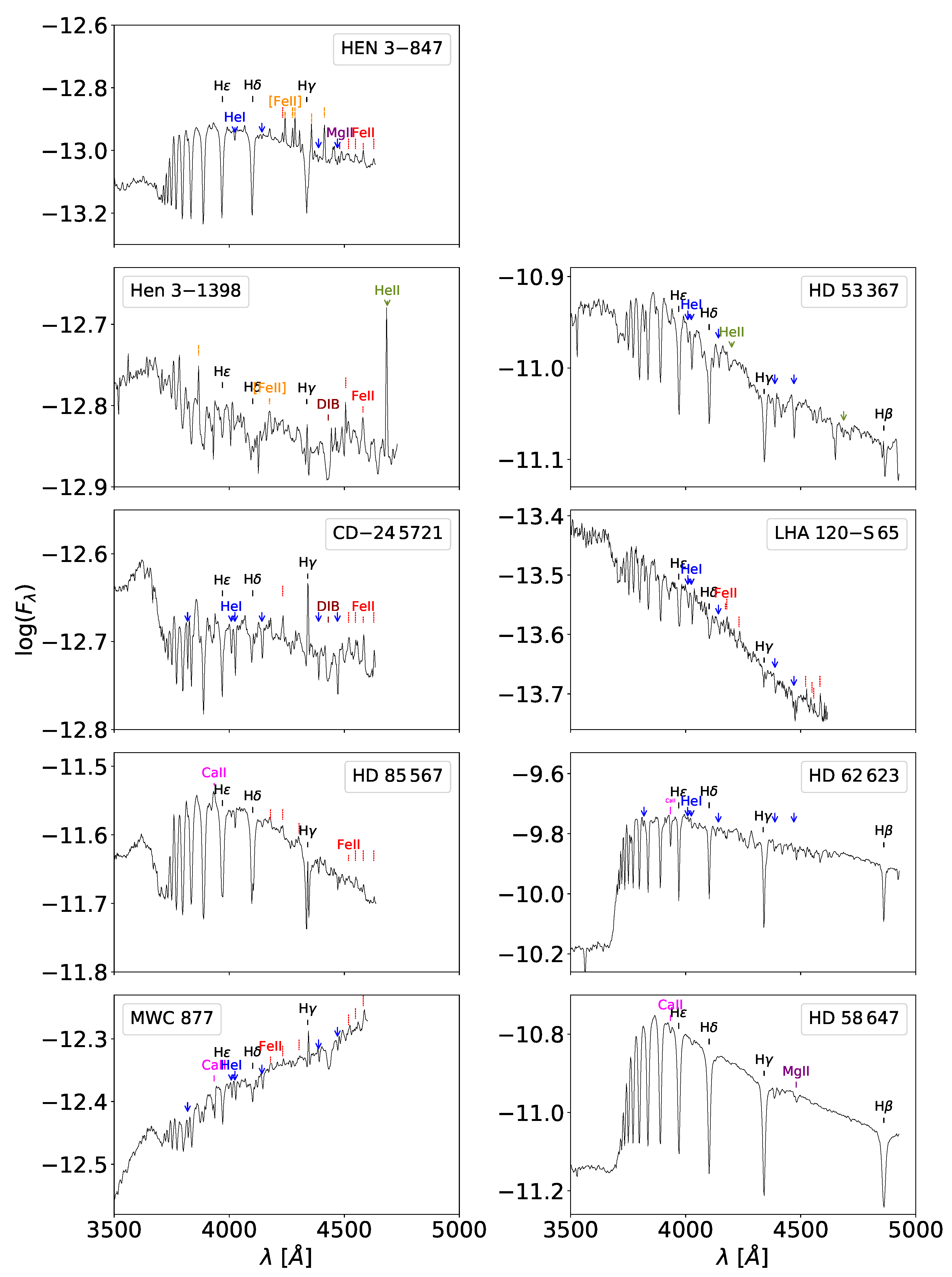
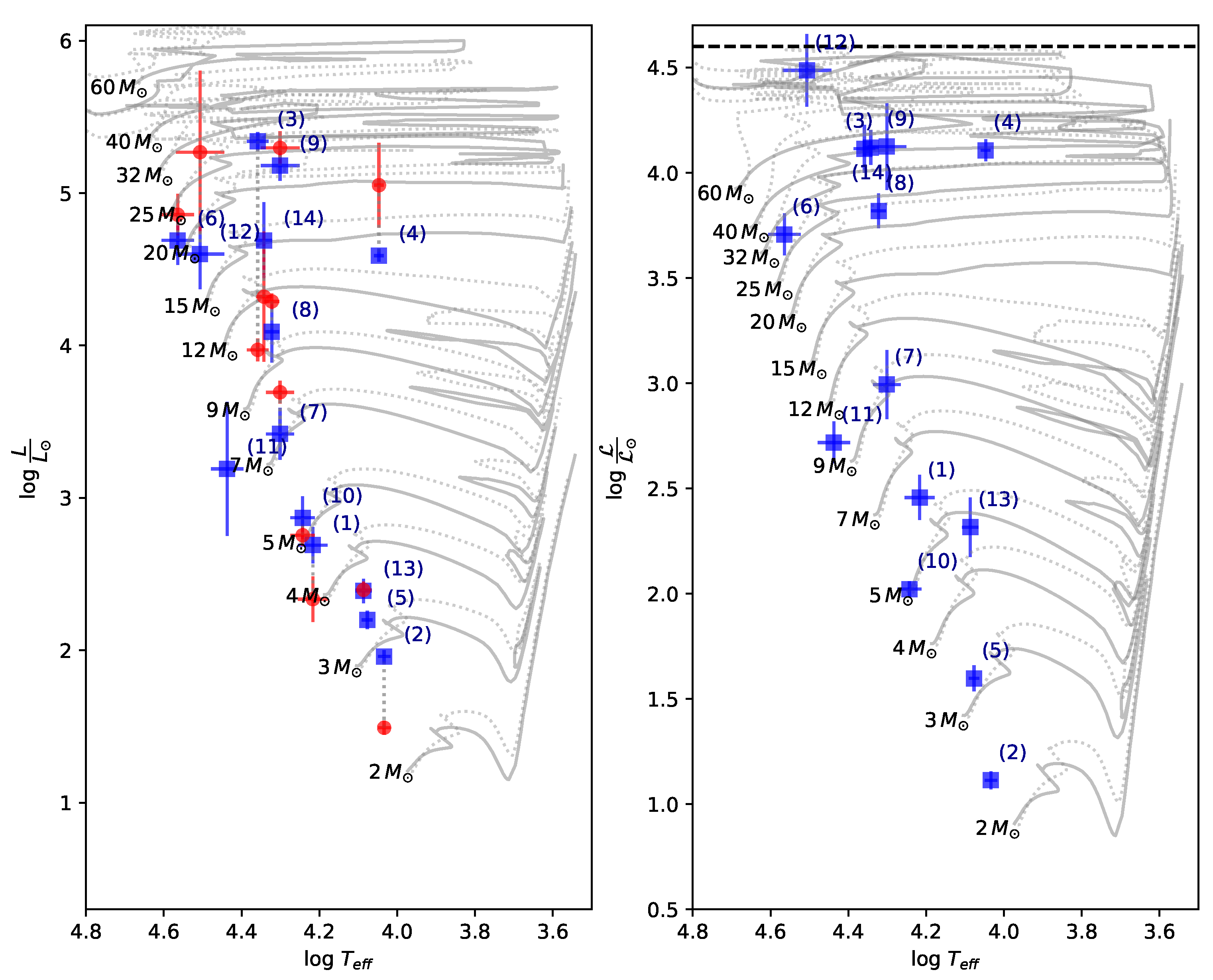
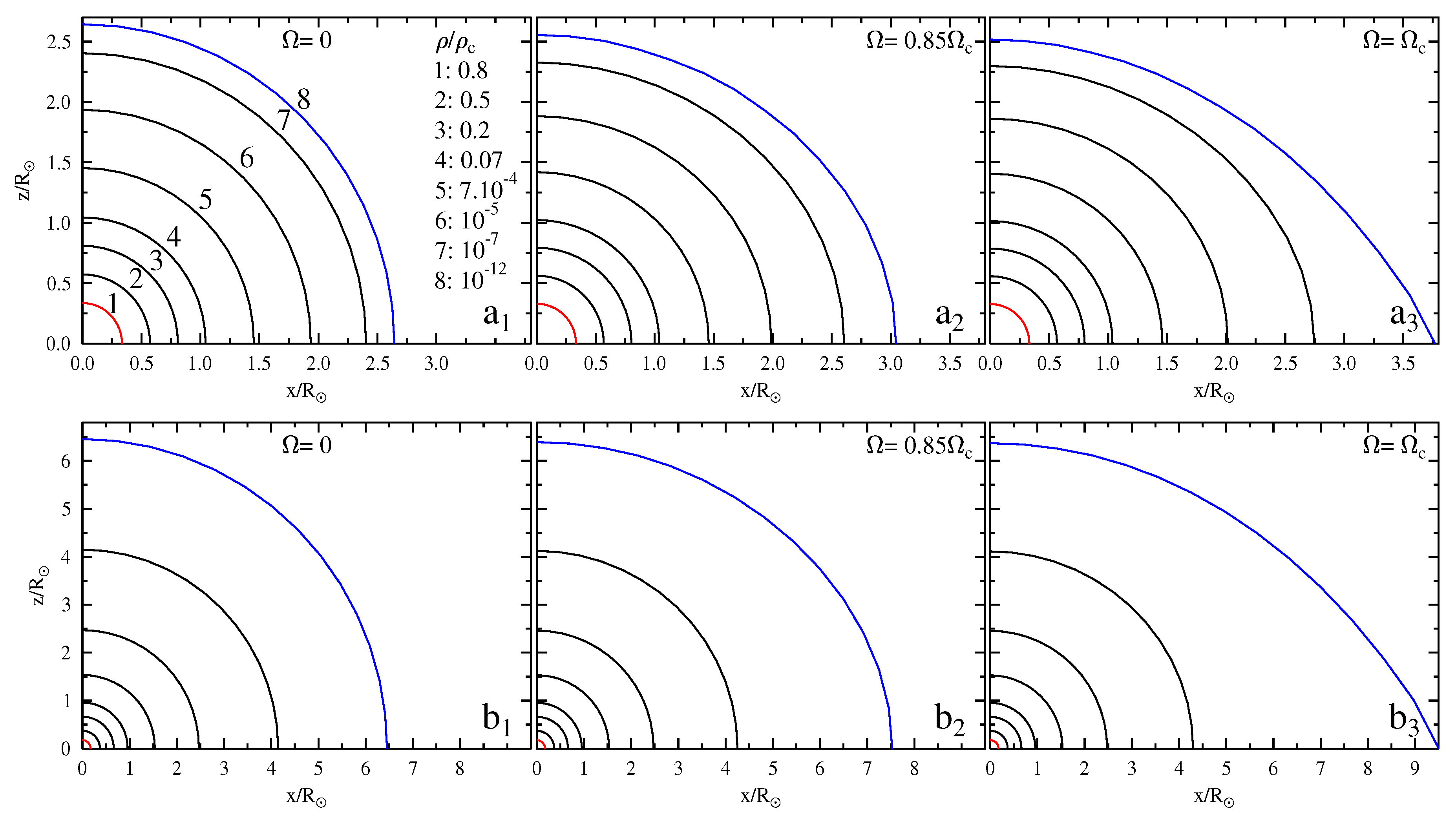

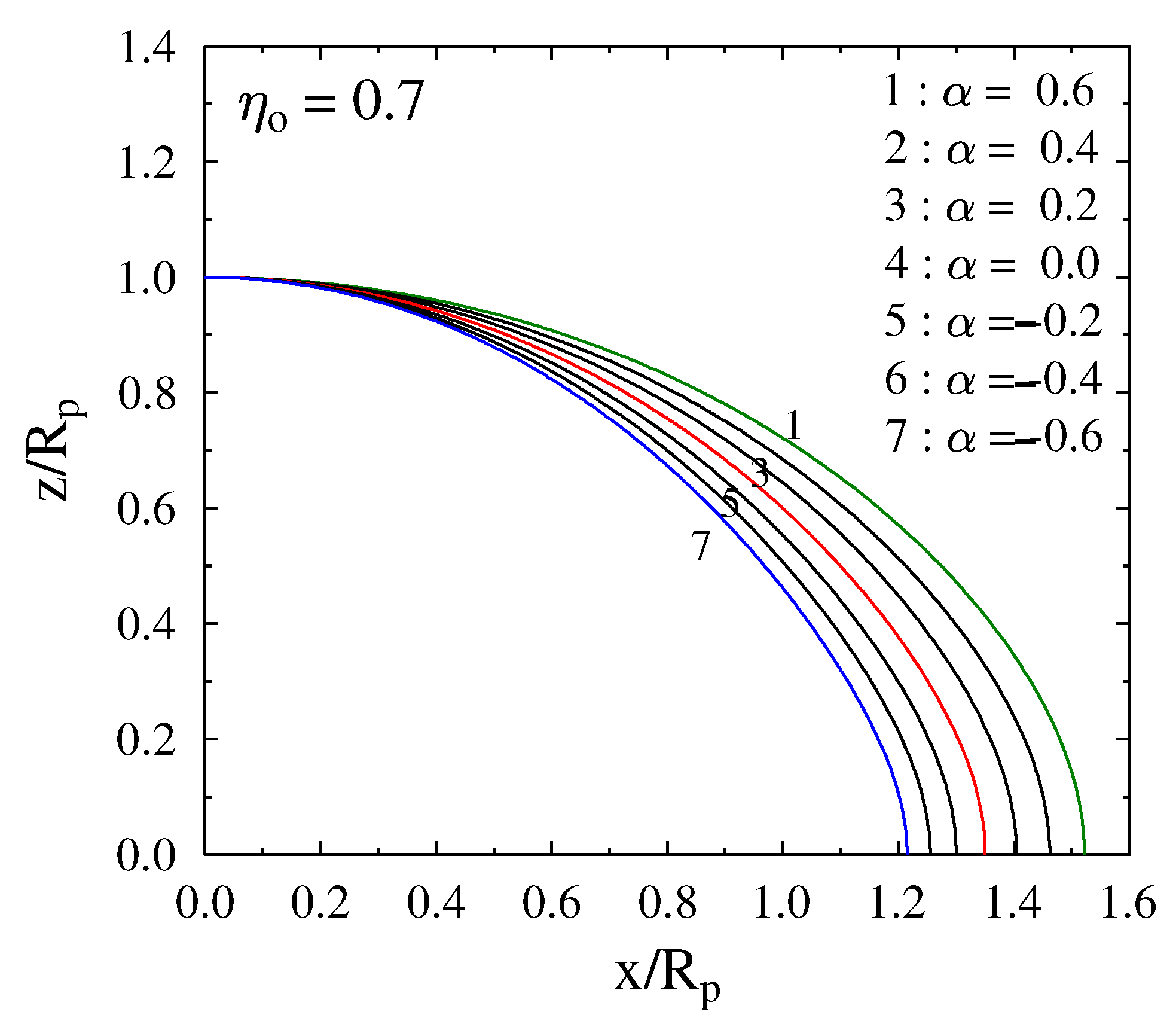
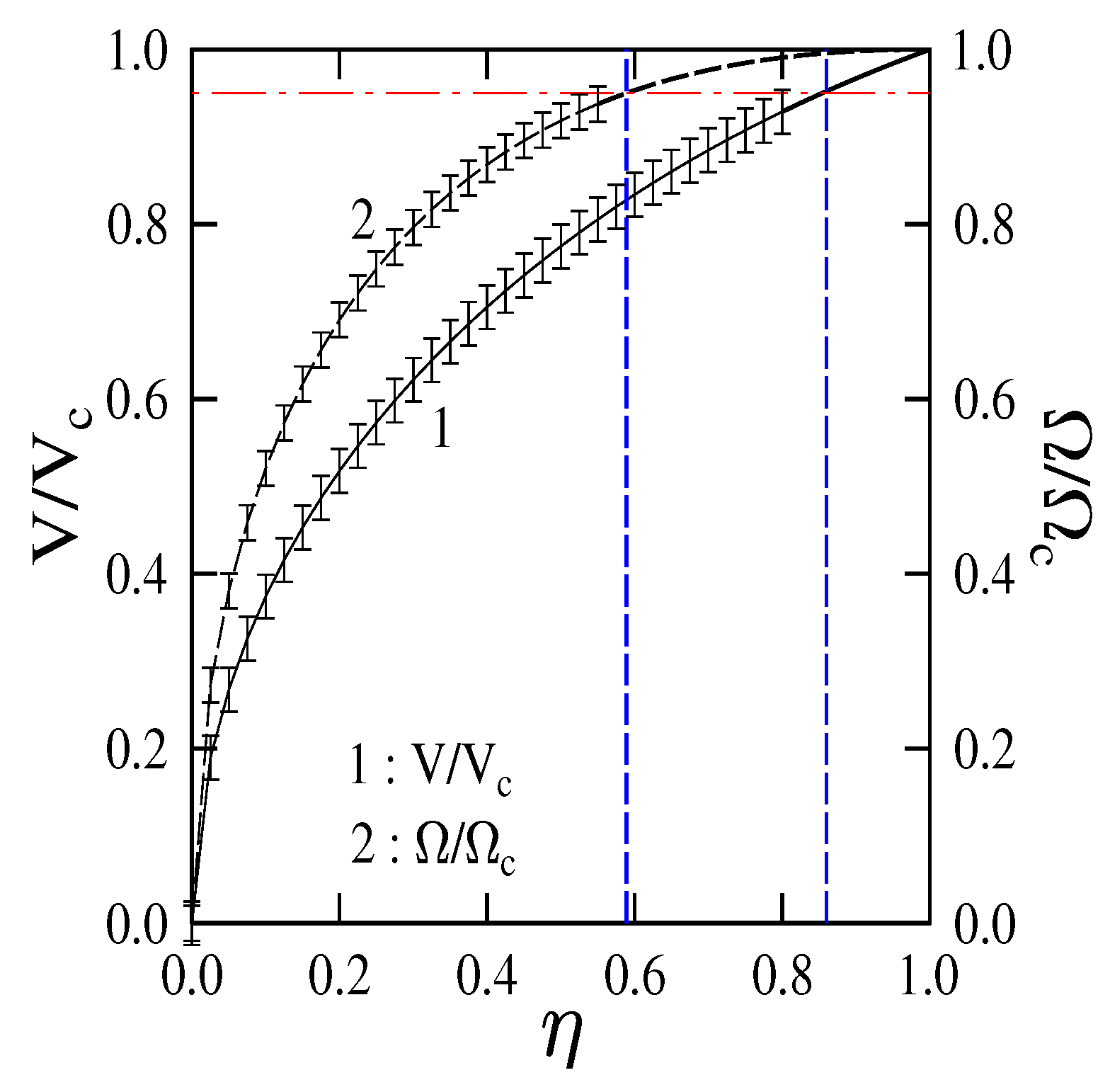

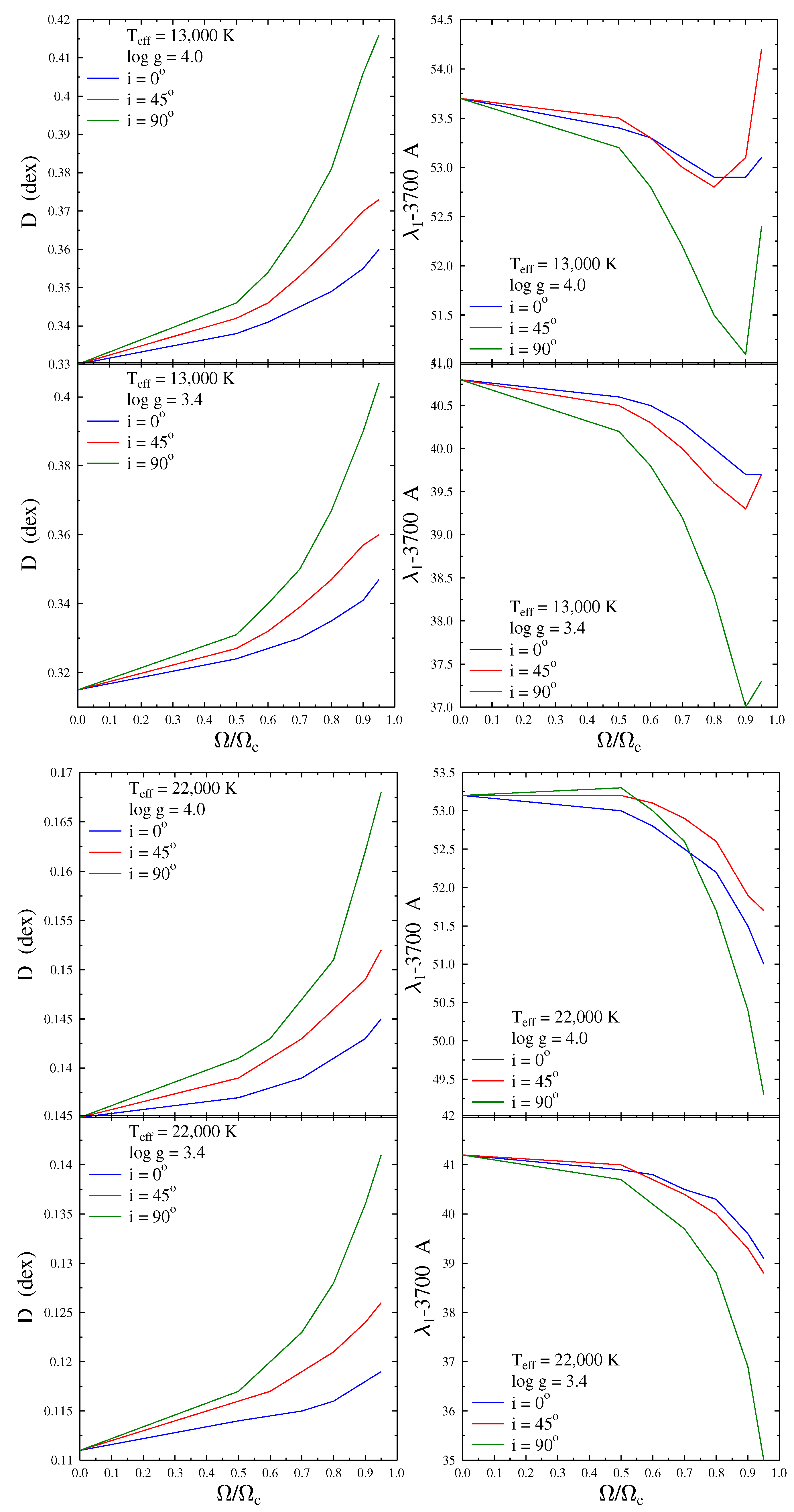
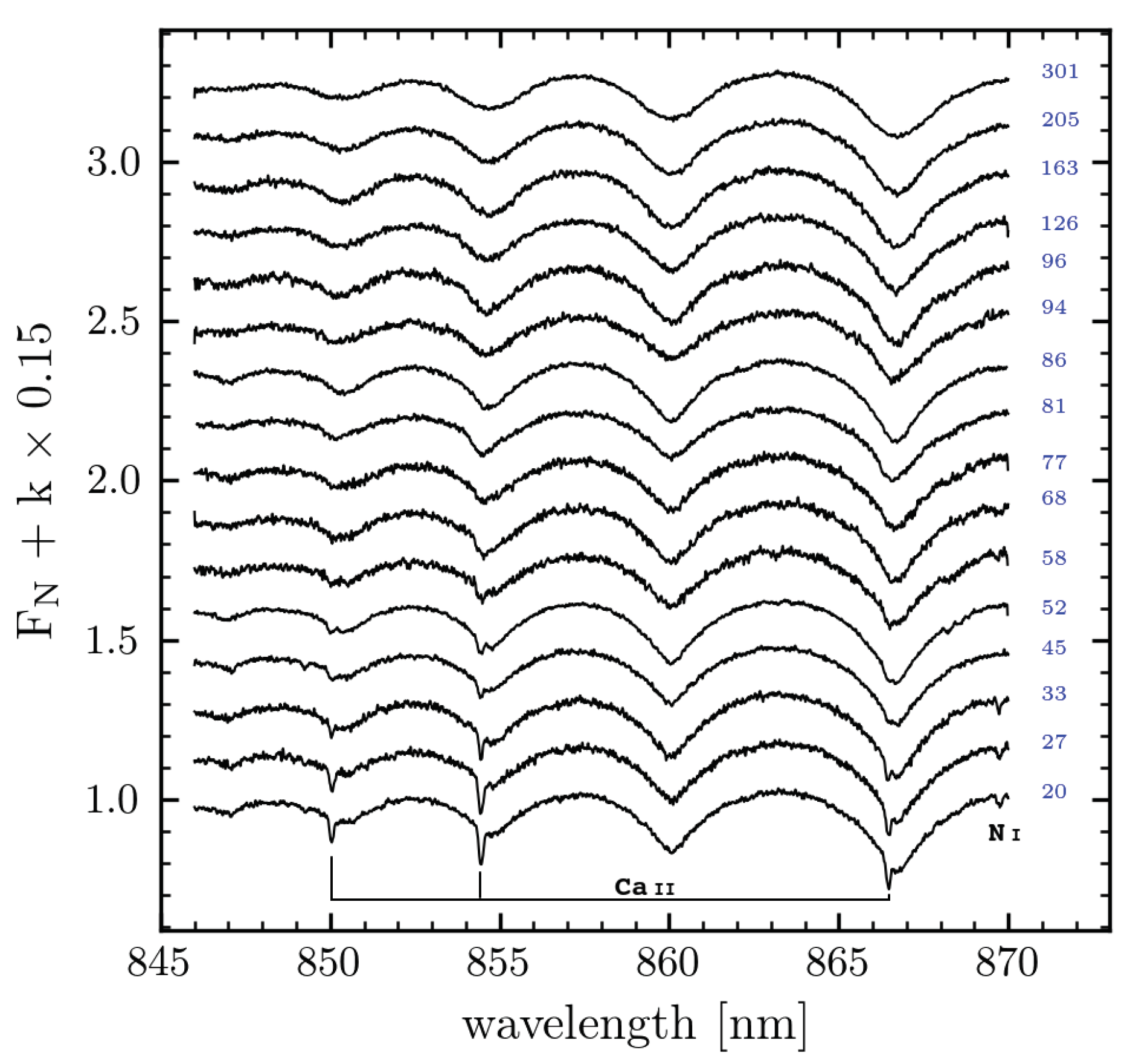


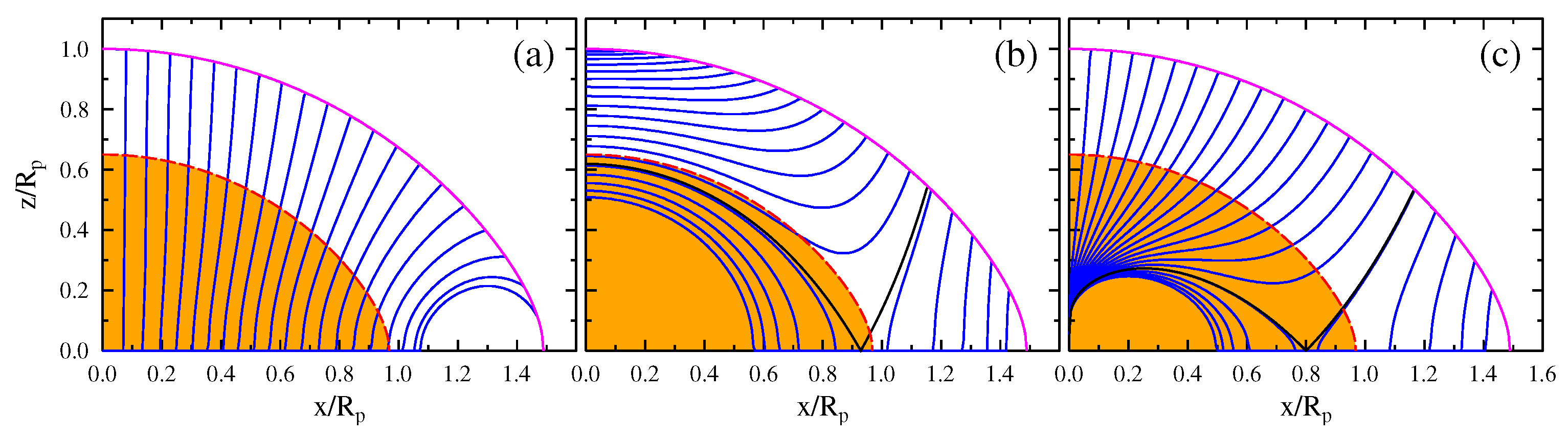
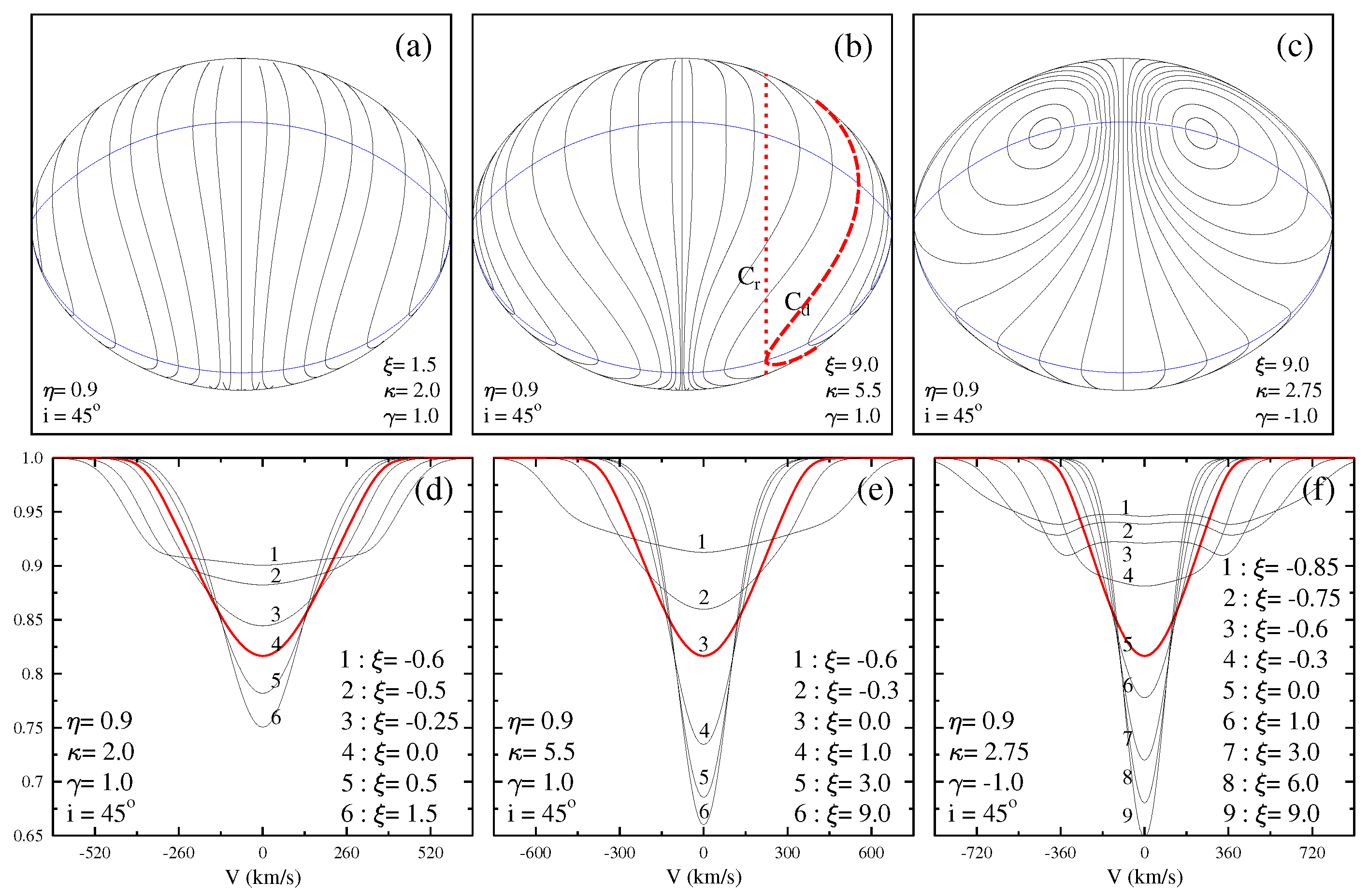

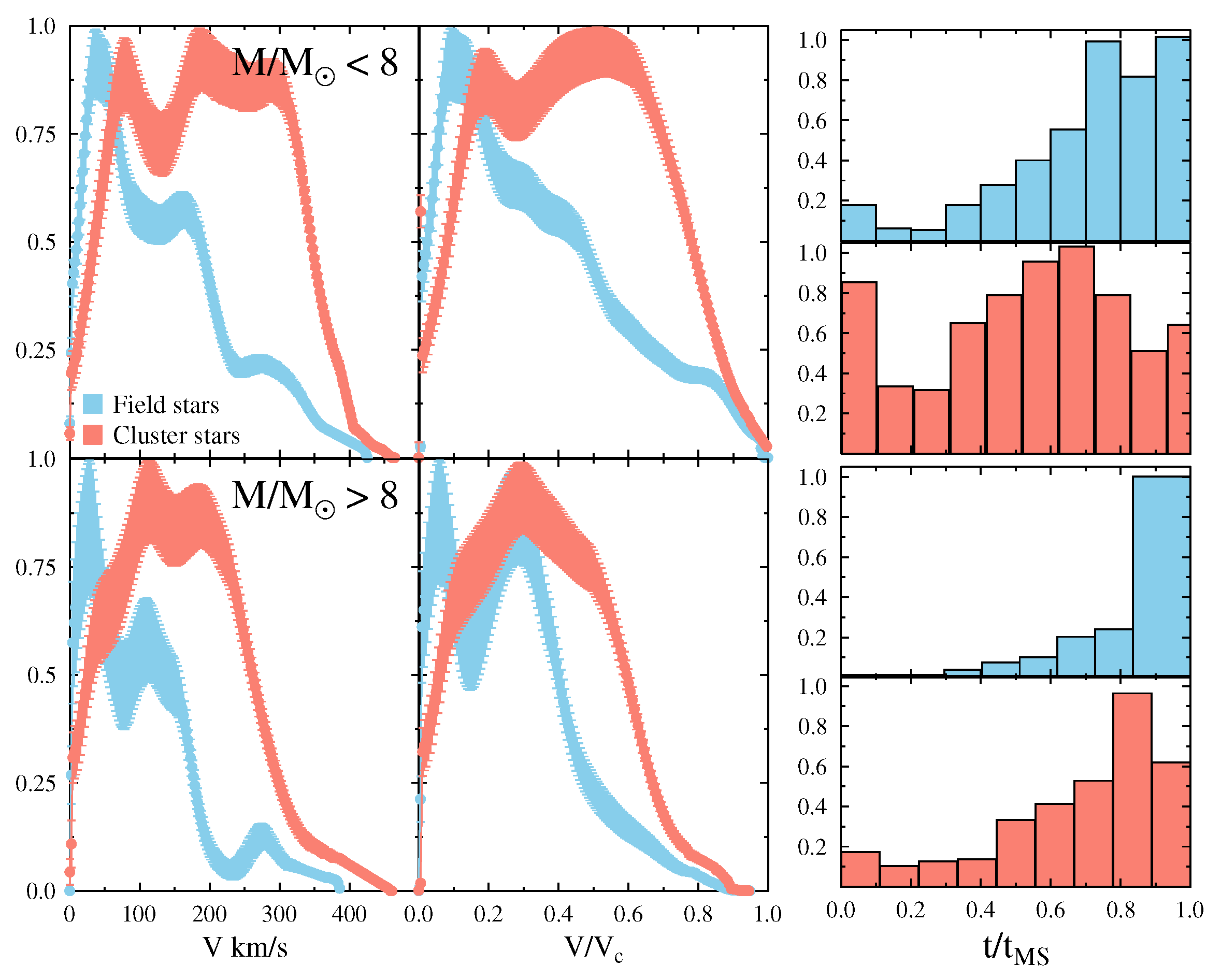

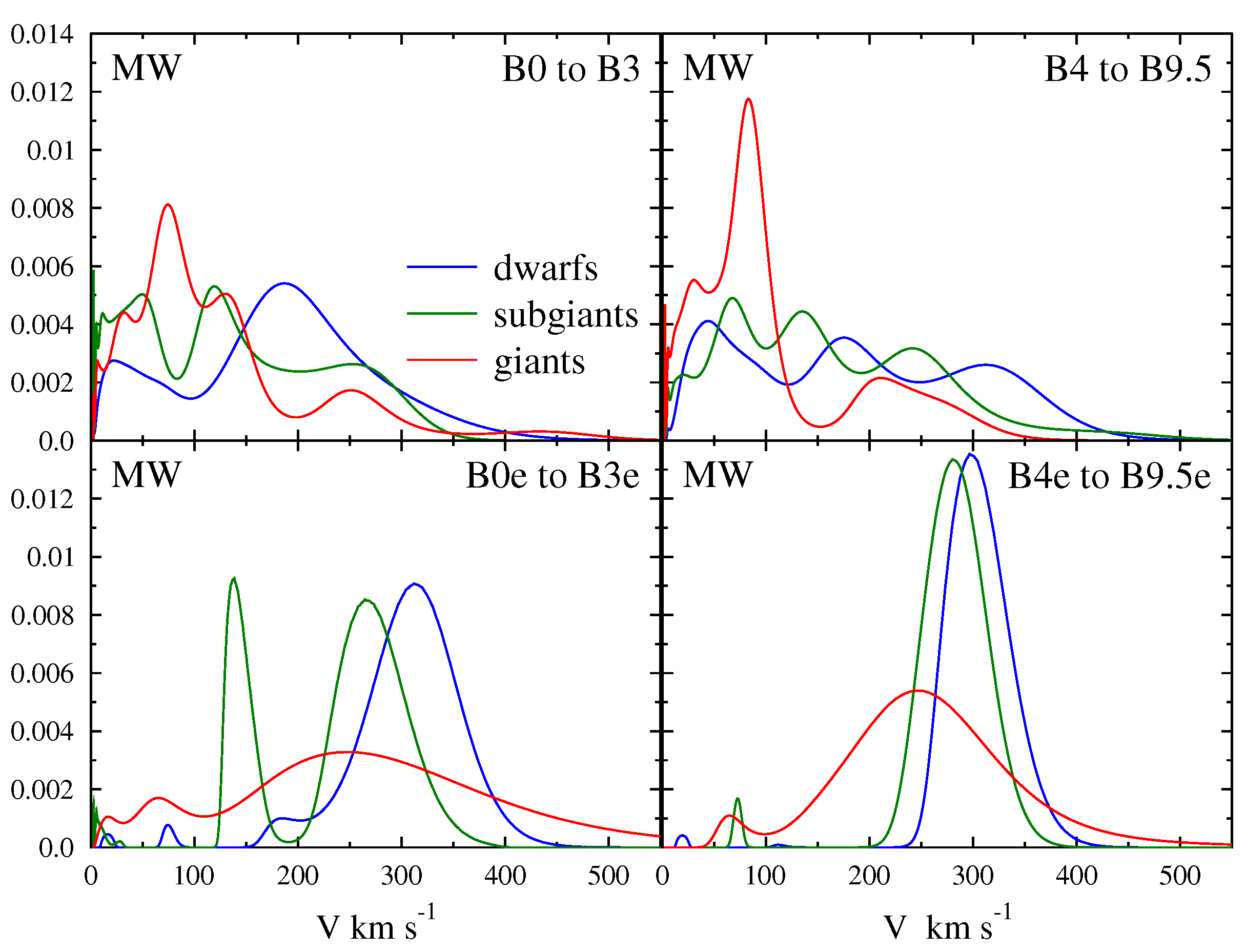


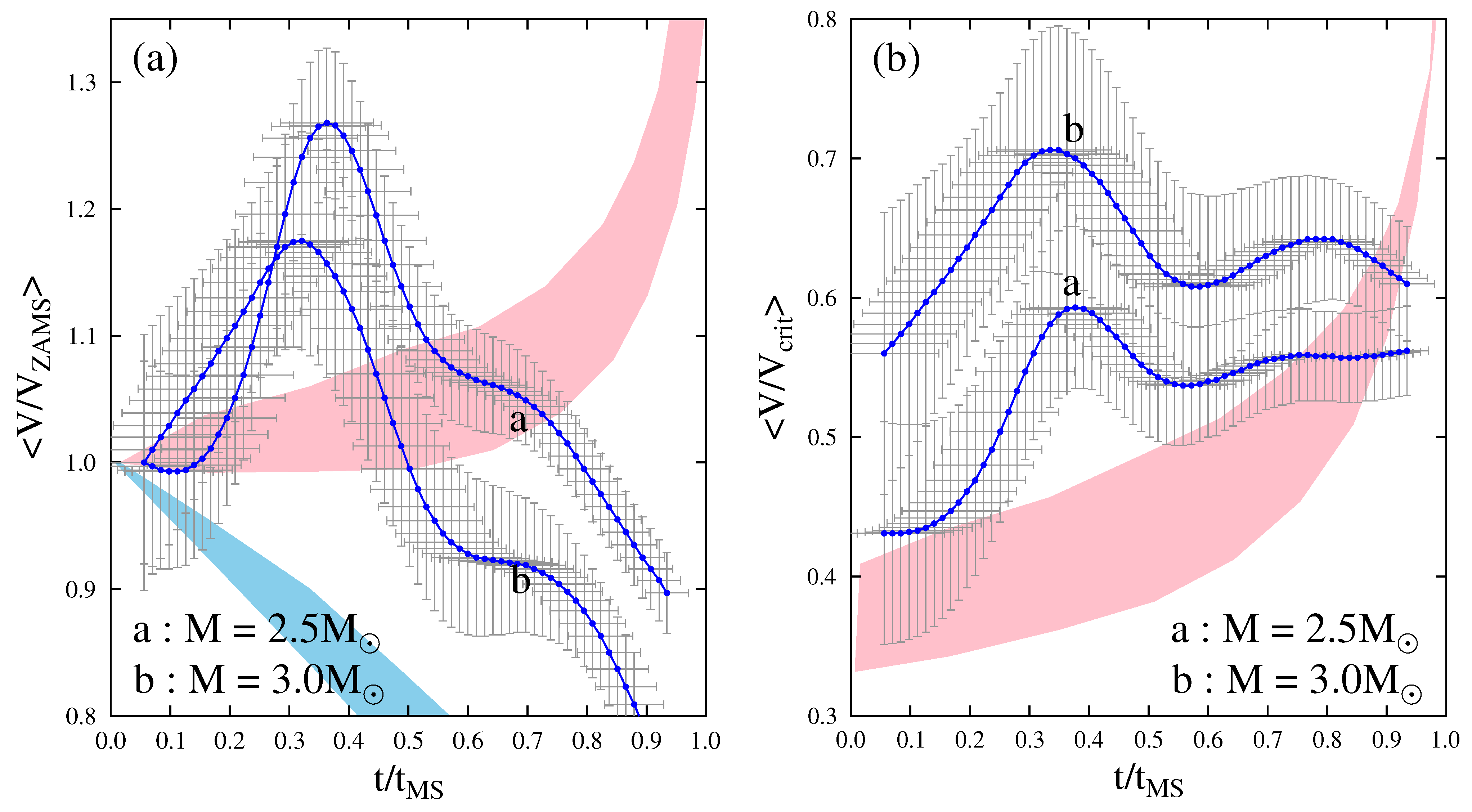
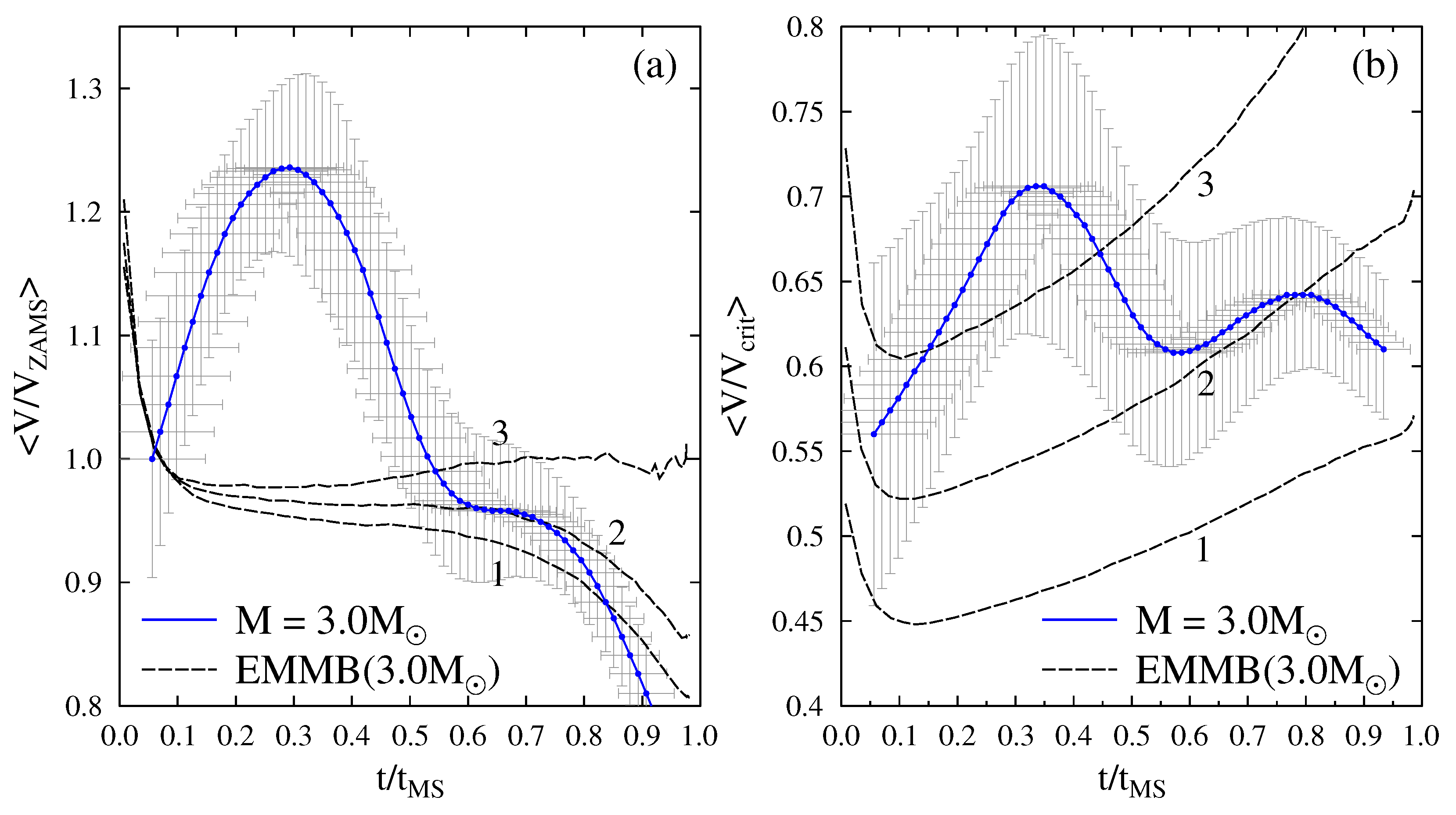
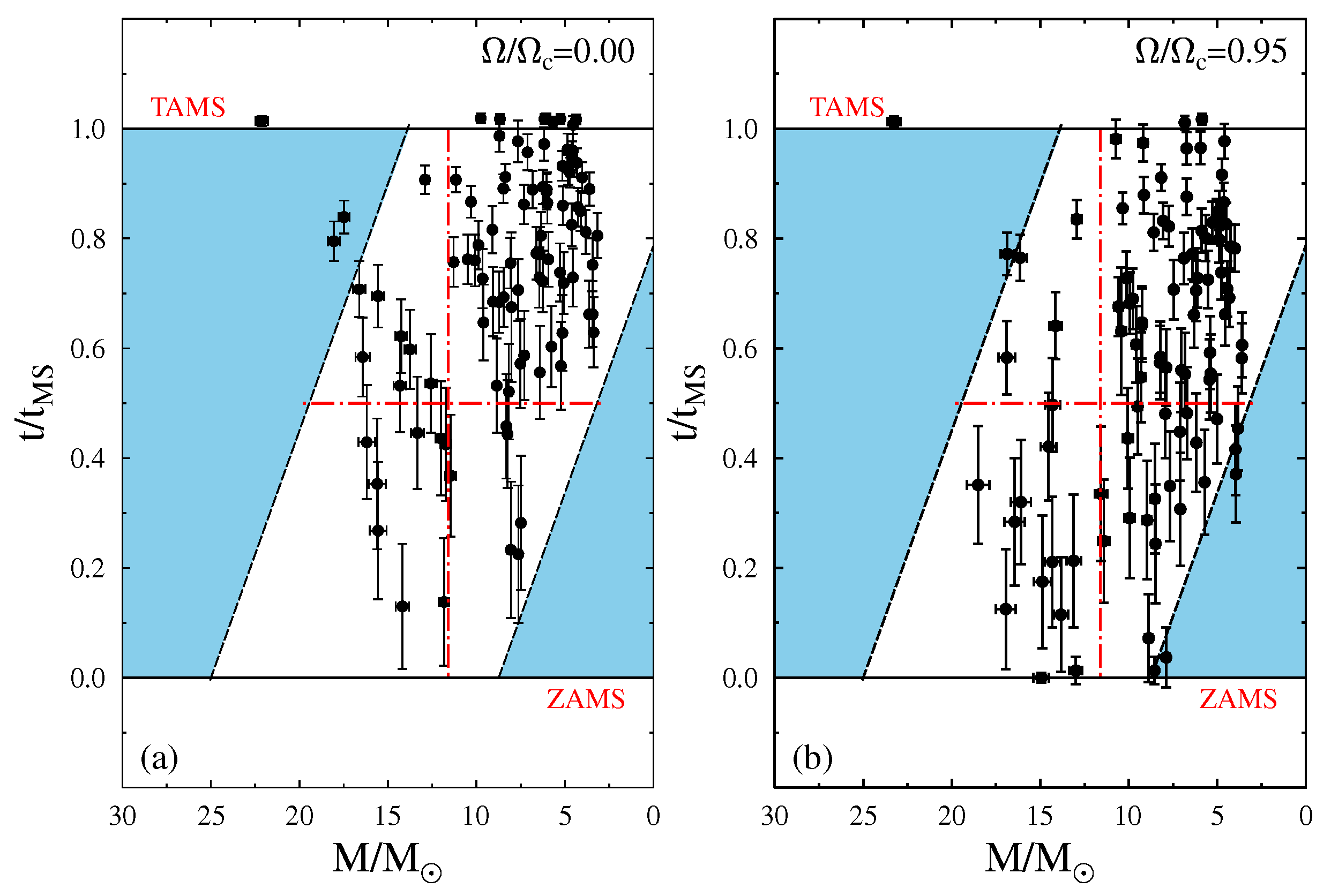
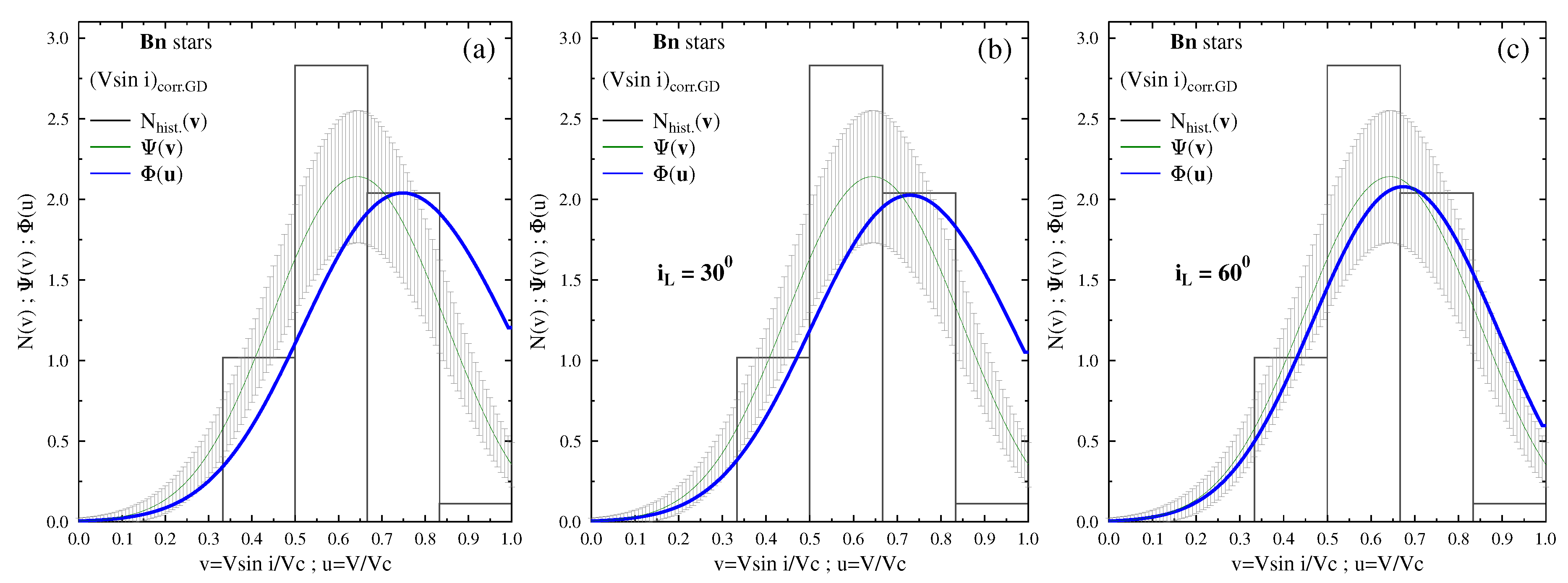
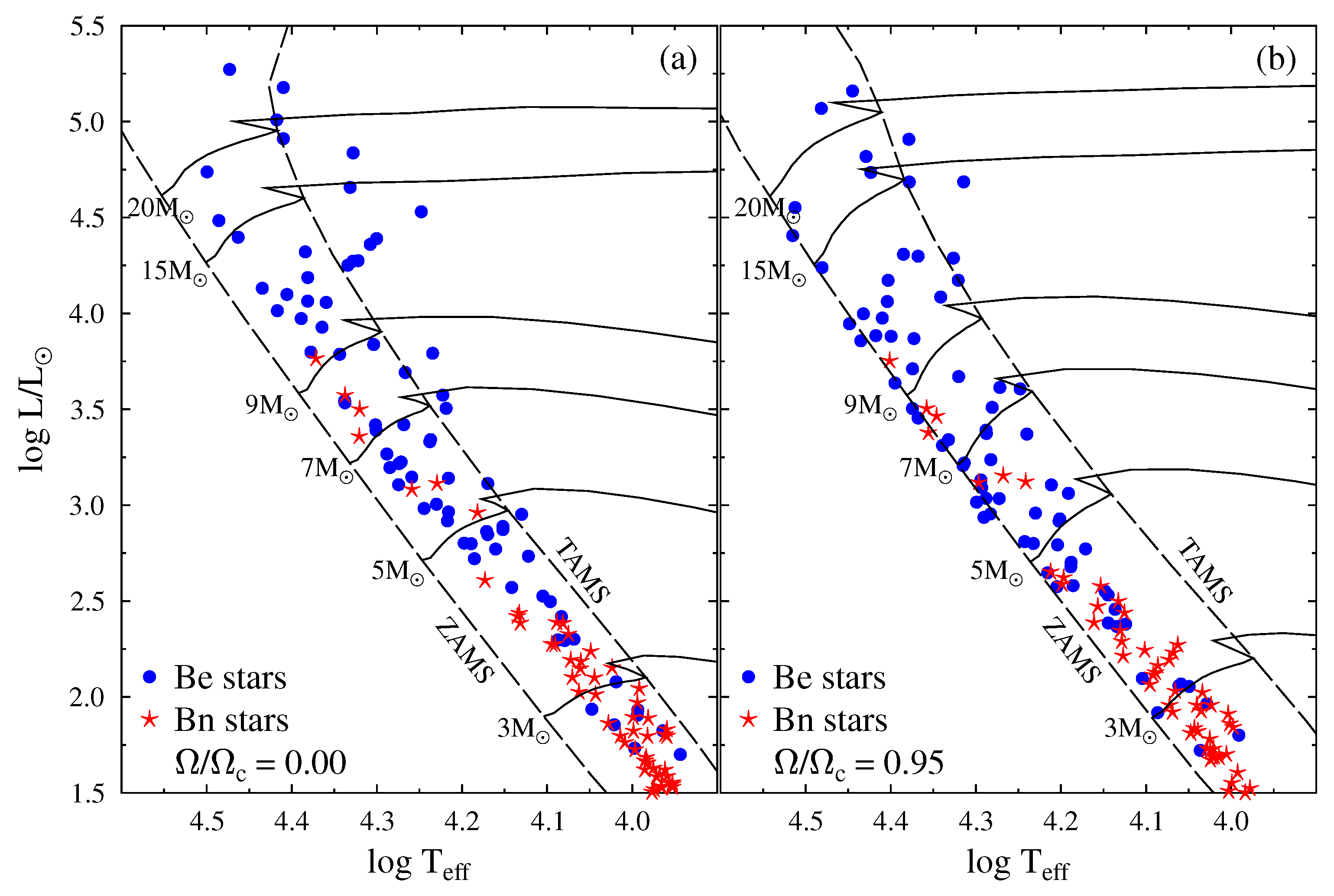
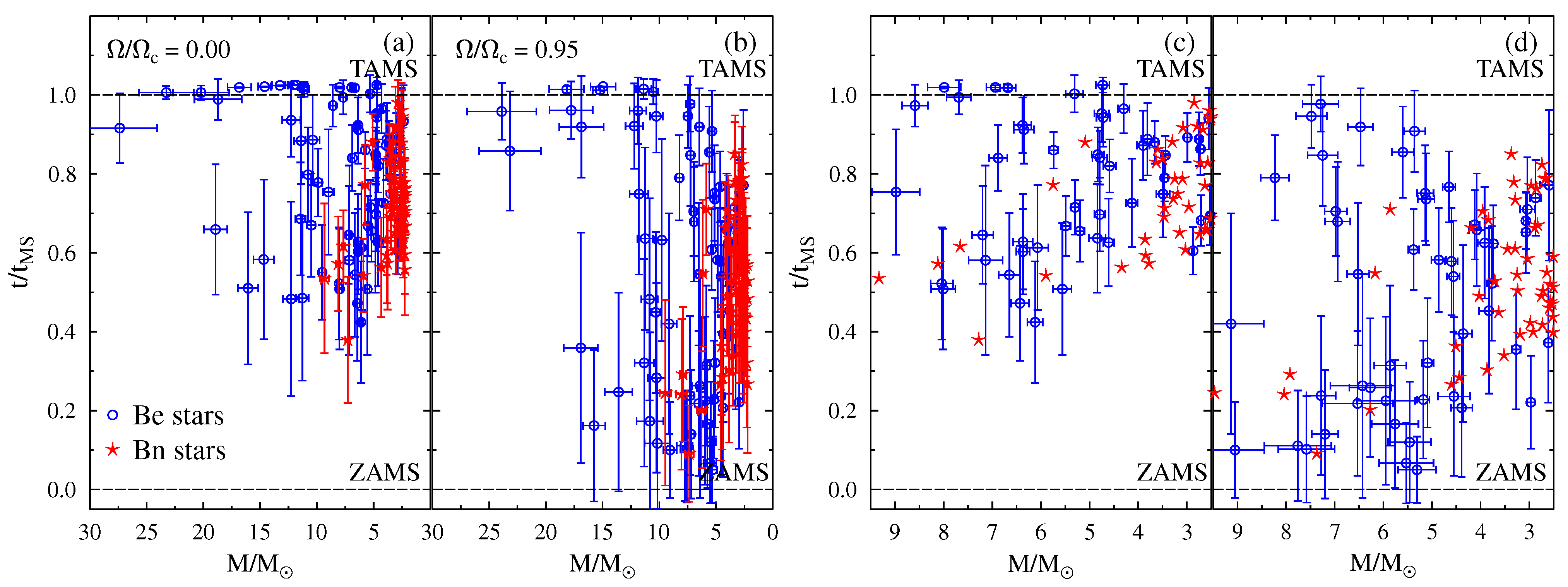


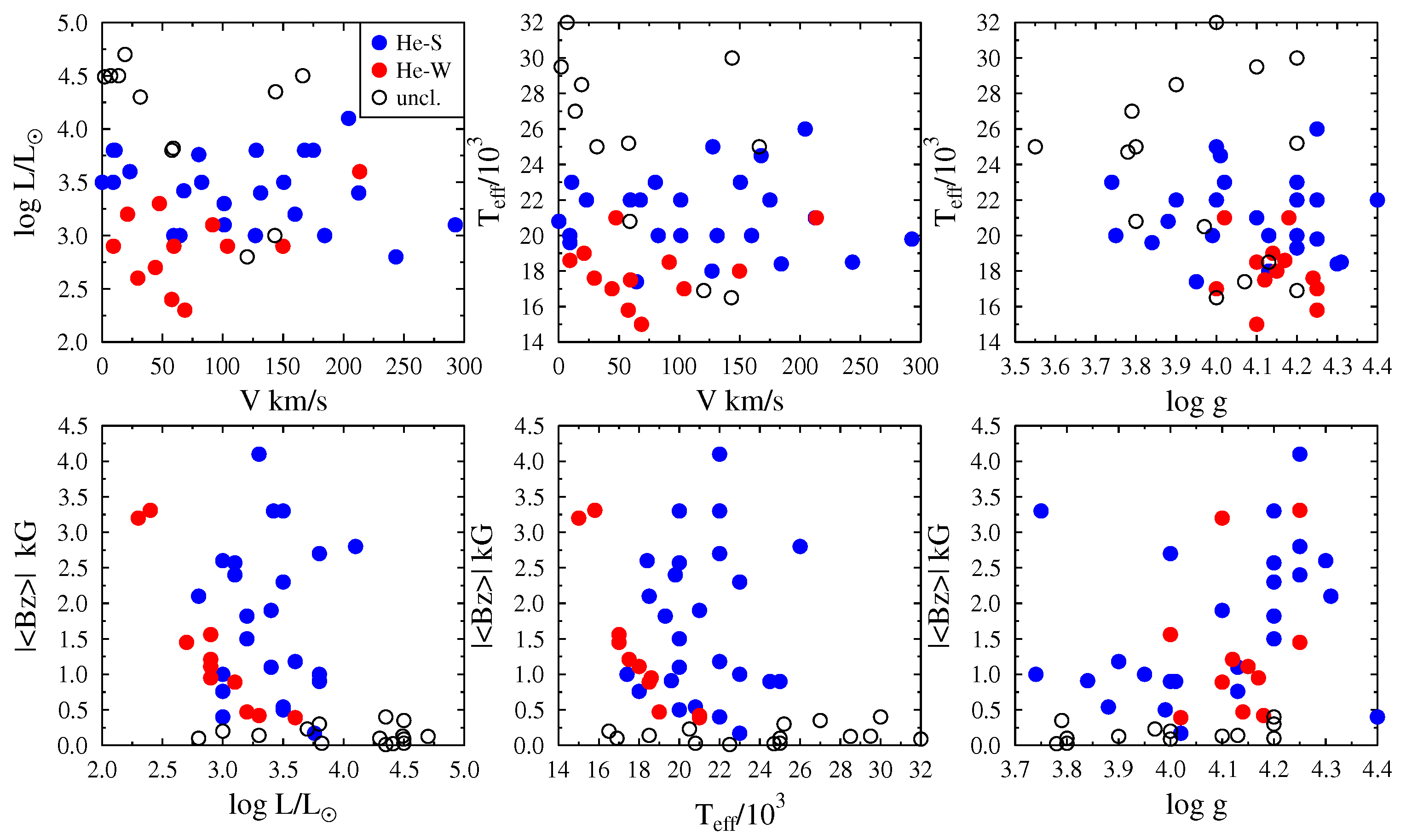
| Source | Environment | Stars | Method | Number |
|---|---|---|---|---|
| Balona [324] | MW | O, Oe, B, Be | 1 | 585 |
| Conti and Ebbets [325] | MW | O, Oe | 3 | 205 |
| Slettebak [326] | MW | Oe, Be, (A, F)shell | 1 | 183 |
| Wolff et al. [327] | Mw | B, Be | 1 | 306 |
| Abt and Morrell [328] | MW | A, Ashell, (B, Be) | 1 | 1700 |
| Halbedel [329] | MW | B.Be | 1 | 164 |
| Penny [330] | MW | O, On, Oe | 3 | 177 |
| Brown and Verschueren [331] | MW, assoc. | B, Be | 1, 3 | 156 |
| Howarth et al. [320] | MW | O, B, (Be) | 3 | 373 |
| Steele et al. [332] | MW | Be | 1 | 58 |
| Chauville et al. [333] | MW | O, B, A, Be | 4 | 233 |
| Abt et al. [334] | MW | B, Be | 1 | 1092 |
| Royer et al. [335] | MW | B, A | 2 | 525 |
| Royer et al. [336] | MW | B, A | 2 | 249 |
| Keller [337] | LMC | B | 3 | 100 |
| Penny et al. [338] | MW, SMC, LMC | O | 3 | 56 |
| Frémat et al. [259] | MW | B, Be | 1 | 233 |
| Glebocki and Gnacinski [339] | MW | all, (B, Be) | (1) | 28,179 |
| Strom et al. [340] | MW, cluster, field | B, Be | 1 | 216 |
| Dufton et al. [318] | MW, cluster | O, B, Be | 3 | 234 |
| Wolff et al. [341] | MW, cluster | O, Oe | 1 | 44 |
| Frémat et al. [342] | MW | Be | 5 | 64 |
| Mokiem et al. [343] | SMC | 0, B | 3 | 31 |
| Levenhagen and Leister [344] | MW | Be | 2 | 141 |
| Huang and Gies [323] | MW, cluster | B, Be | 5 | 496 |
| Martayan et al. [345] | LMC, cluster, field | B, Be | 5 | 202 |
| Hunter et al. [302] | SMC, LMC | B | 3 | 50 |
| Martayan et al. [346] | SMC, cluster, field | B, Be, (O, A) | 5 | 346 |
| Trundle et al. [305] | MW, SMC, LMC | B | 3 | 61 |
| Wolff et al. [347] | MW, cluster | B, Be | 1 | 168 |
| Wolff et al. [348] | LMC, cluster | OB | 3 | 34 |
| Huang and Gies [349] | MW, field, cluster | B, Be | 5 | 108 |
| Hunter et al. [350] | SMC, LMC | B, Be | 3 | 407 |
| Penny and Gies [351] | MW, SMC, LMC | O, B | 3 | 258 |
| Fraser et al. [352] | MW, supergiant | B | 2 | 57 |
| Huang et al. [353] | MW, field, cluster | B, Be | 5 | 634 |
| Díaz et al. [319] | MW | A | 2 | 251 |
| Marsh Boyer et al. [354] | MW, cluster | B, Be | 3 | 104 |
| Bragança et al. [355] | MW | B, Be | 3 | 350 |
| Dufton et al. [356] | LMC | O, B, Be | 2, 3 | 334 |
| Ramírez-Agudelo et al. [357] | LMC | O | 2, 3 | 216 |
| Simón-Díaz and Herrero [358] | MW | O, B | 2, 3 | 203 |
| Garmany et al. [359] | MW | B, Be | 3 | 130 |
| Zorec et al. [127] | MW | Be | 4 | 233 |
| Holgado et al. [360] | MW | O | 2, 3 | 285 |
| Solar et al. [361] | MW | Be | 2 | 57 |
| Xiang et al. [362] | MW | O, B, A | 3 | 132, 548 |
| Gaia DR31, vbroad | MW | late-B to early-M | 3 | 33, 812, 183 |
| Gaia DR31, vsini_esphs | MW | O, B, A | 3 | 780, 461 |
| V | ||||
|---|---|---|---|---|
| km s | (cm s) | km s | (cm s) | |
| 0.07 | 182 | 1.086 | 422 | 1.974 |
| 0.36 | 231 | 1.270 | 391 | 1.842 |
| 0.62 | 195 | 1.154 | 361 | 1.652 |
| 0.90 | 167 | 0.875 | 299 | 1.201 |
| 0.07 | 249 | 1.558 | 439 | 2.389 |
| 0.29 | 290 | 1.725 | 410 | 2.243 |
| 0.62 | 229 | 1.419 | 376 | 2.017 |
| 0.90 | 192 | 1.157 | 310 | 1.431 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zorec, J. BCD Spectrophotometry and Rotation of Active B-Type Stars: Theory and Observations. Galaxies 2023, 11, 54. https://doi.org/10.3390/galaxies11020054
Zorec J. BCD Spectrophotometry and Rotation of Active B-Type Stars: Theory and Observations. Galaxies. 2023; 11(2):54. https://doi.org/10.3390/galaxies11020054
Chicago/Turabian StyleZorec, Juan. 2023. "BCD Spectrophotometry and Rotation of Active B-Type Stars: Theory and Observations" Galaxies 11, no. 2: 54. https://doi.org/10.3390/galaxies11020054
APA StyleZorec, J. (2023). BCD Spectrophotometry and Rotation of Active B-Type Stars: Theory and Observations. Galaxies, 11(2), 54. https://doi.org/10.3390/galaxies11020054






