Thermodynamic Performance Analysis of Solar Based Organic Rankine Cycle Coupled with Thermal Storage for a Semi-Arid Climate
Abstract
1. Introduction
- Temperature profile variation during the charging and discharging mode is indicated and compared under the hottest and coldest month of weather conditions on an hourly basis.
- The thermodynamic performance of the system is based on the variation in the system efficiencies and net power output.
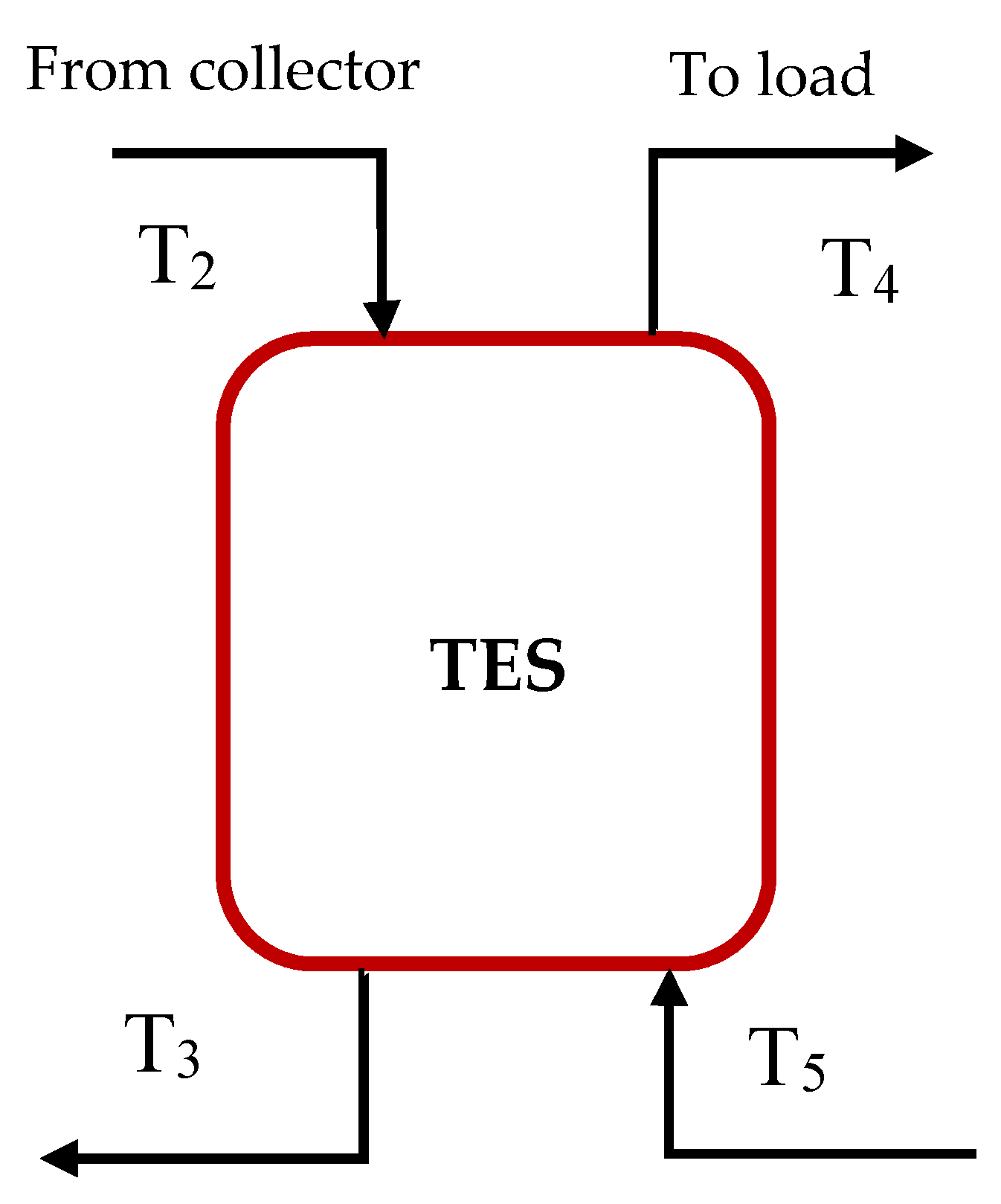
2. System Description
3. Thermodynamic Modeling
3.1. Solar Collectors
3.2. Thermal Energy Storage Tank
3.3. Organic Rankine Cycle
- Pressure drops in the heat exchanger, condenser, and connecting pipes are neglected;
- The system is assumed to be in steady-state condition;
- The isentropic efficiency of the pump and expander are chosen, respectively.
4. Results and Discussion
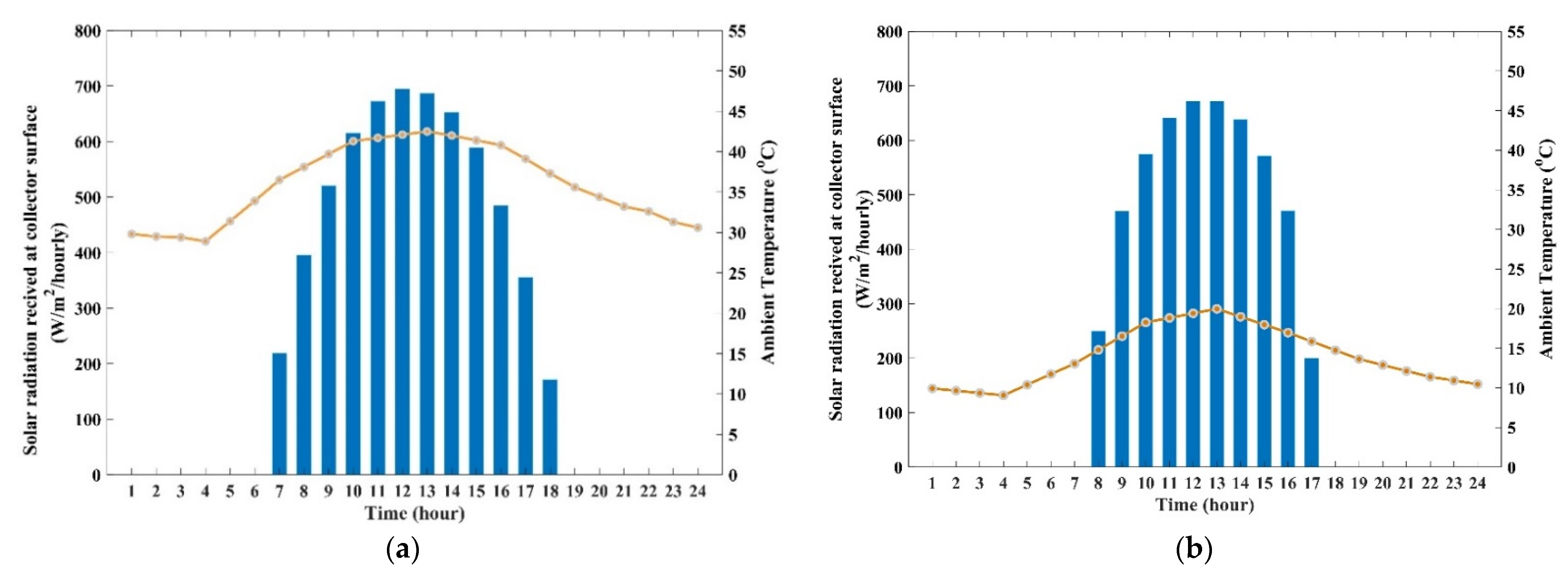
4.1. Performance of July (Hottest) Month on an Hourly Basis
4.1.1. Variation in the Tank Temperature Profiles during Charging Mode
4.1.2. Variation in the Tank Temperature Profiles during Discharging Mode
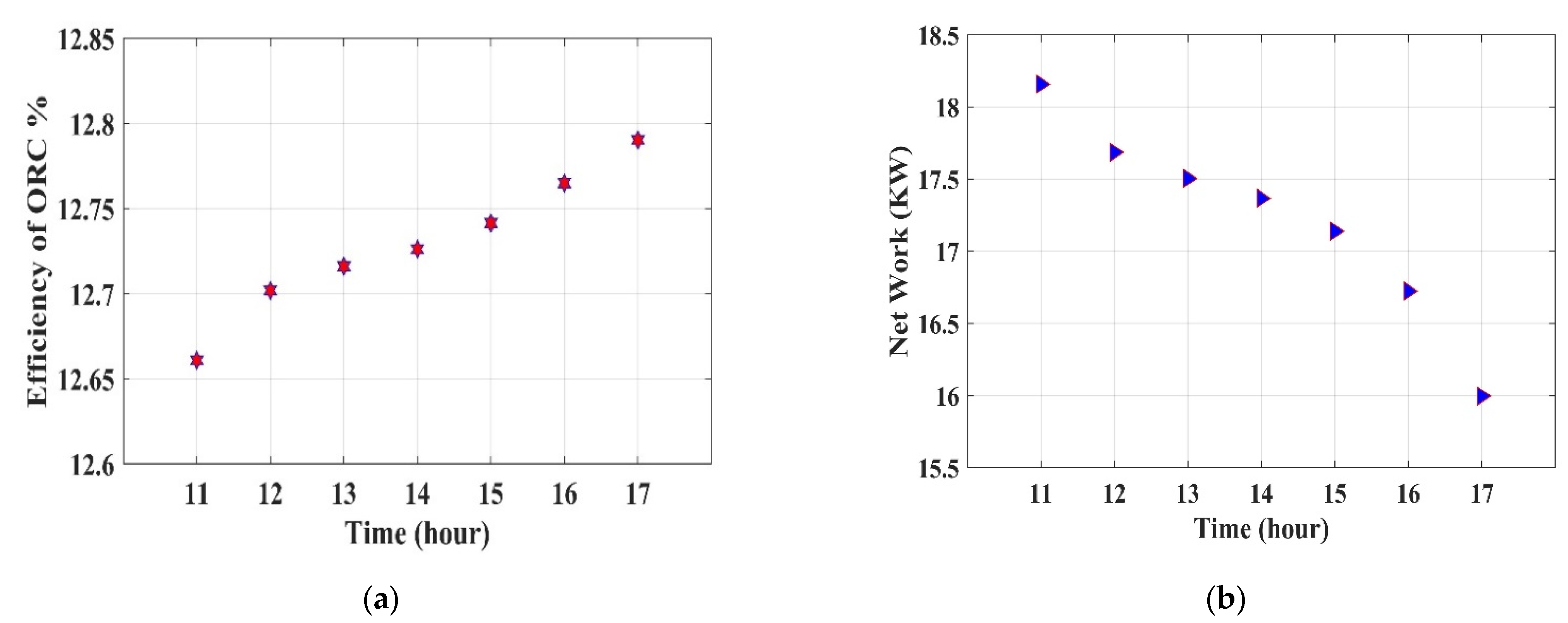
4.1.3. Variation in the System Efficiencies and Net Power Output
4.2. Performance of January (Coldest) Month on an Hourly Basis
4.2.1. Variation in the Tank Temperature Profiles during Charging Mode
4.2.2. Variation in the Tank Temperature Profiles during Discharging Mode
4.2.3. Variation in the System Efficiencies and Net Power Output
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| area () | |
| number of collectors | |
| T | temperature (°C) |
| efficiency (%) | |
| heat rate (W) | |
| mass flow (Kg-s) | |
| time | |
| density (Kg/) | |
| volume | |
| loss coefficient (W/m2K) | |
| specific enthalpy (J/kg) | |
| s | specific entropy (J/kg·K) |
| Abbreviations | |
| CPC | compound parabolic collector |
| TES | thermal energy storage |
| SORC | solar organic Rankine cycle |
| ORC | organic Rankine cycle |
| DNI | direct normal irradiation (Wh/ |
| Subscripts | |
| ambient temperature (°C) | |
| collector | |
| cond | condenser |
| specific heat capacity (J/kg·K) | |
| outside tank temperature (°C) | |
| exp | expander |
| hx | heat exchanger |
| P | pump |
| thermal energy storge |
References
- Pachauri, R.K.; Allen, M.R.; Barros, V.R.; Broome, J.; Cramer, W.; Christ, R.; Church, J.A.; Clarke, L.; Dahe, Q.; van Ypserle, J.P.; et al. IPCC, 2014: Climate Change 2014: Synthesis Report. Contribution of Working Groups I, II and III to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change; IPCC: Geneva, Switzerland, 2014. [Google Scholar]
- King Abdullah City for Atomic and Renewable Energy © 2020. Available online: https://www.energy.gov.sa/ar/FutureEnergy/RenewableEnergy/Pages/default.aspx (accessed on 6 November 2020).
- Boisgibault, L.; Al Kabbani, F. Energy Transition in Metropolises, Rural Areas and Deserts; John Wiley & Sons: Hoboken, NJ, USA, 2020. [Google Scholar]
- Tchanche, B.F.; Lambrinos, G.; Frangoudakis, A.; Papadakis, G. Low-grade heat conversion into power using organic Rankine cycles—A review of various applications. Renew. Sustain. Energy Rev. 2011, 15, 3963–3979. [Google Scholar] [CrossRef]
- Vetter, C.; Wiemer, H.J.; Kuhn, D. Comparison of sub- and supercritical Organic Rankine Cycles for power generation from low-temperature/low-enthalpy geothermal wells, considering specific net power output and efficiency. Appl. Therm. Eng. 2013, 51, 871–879. [Google Scholar] [CrossRef]
- Nafey, A.S.; Sharaf, M.A. Combined solar organic Rankine cycle with reverse osmosis desalination process: Energy, exergy, and cost evaluations. Renew. Energy 2010, 35, 2571–2580. [Google Scholar] [CrossRef]
- Pei, G.; Li, J.; Ji, J. Analysis of low temperature solar thermal electric generation using regenerative Organic Rankine Cycle. Appl. Therm. Eng. 2010, 30, 998–1004. [Google Scholar] [CrossRef]
- Khatoon, S.; Kim, M.H. Potential improvement and comparative assessment of supercritical Brayton cycles for arid climate. Energy Convers. Manag. 2019, 200, 112082. [Google Scholar] [CrossRef]
- Liu, Q.; Bai, Z.; Wang, X.; Lei, J.; Jin, H. Investigation of thermodynamic performances for two solar-biomass hybrid combined cycle power generation systems. Energy Convers. Manag. 2016, 122, 252–262. [Google Scholar] [CrossRef]
- Zhang, H.L.; Baeyens, J.; Degrève, J.; Cacères, G. Concentrated solar power plants: Review and design methodology. Renew. Sustain. Energy Rev. 2013, 22, 466–481. [Google Scholar] [CrossRef]
- Mills, D. Advances in solar thermal electricity technology. Sol. Energy 2004, 76, 19–31. [Google Scholar] [CrossRef]
- Gang, P.; Jing, L.; Jie, J. Design and analysis of a novel low-temperature solar thermal electric system with two-stage collectors and heat storage units. Renew. Energy 2011, 36, 2324–2333. [Google Scholar] [CrossRef]
- Jing, L.; Gang, P.; Jie, J. Optimization of low temperature solar thermal electric generation with Organic Rankine Cycle in different areas. Appl. Energy 2010, 87, 3355–3365. [Google Scholar] [CrossRef]
- Saitoh, T.S.; Kato, J.; Yamada, N. Advanced 3-D CPC solar collector for thermal electric system. Heat Transf. Asian Res. 2006, 35, 323–335. [Google Scholar] [CrossRef]
- Pereira, M.C. Design and Performance of a Novel Non-Evacuated 1. 2X Cpc Type Concentrator. Int. Sol. Energy Soc. 1986, 2, 1199–1204. [Google Scholar]
- Rabl, A.; O’Gallagher, J.; Winston, R. Design and test of non-evacuated solar collectors with compound parabolic concentrators. Sol. Energy 1980, 25, 335–351. [Google Scholar] [CrossRef]
- Saitoh, T.S.; Hoshi, A. Proposed solar Rankine cycle system with phase change steam accumulator and CPC solar collector. Proc. Intersoc. Energy Convers. Eng. Conf. 2002, 20150, 725–730. [Google Scholar]
- Colonna, P.; Casati, E.; Trapp, C.; Mathijssen, T. Organic Rankine cycle power systems: A review. In Proceedings of the International Conference on Power Engineering (ICOPE); The Japan Society of Mechanical Engineers: Tokyo, Japan, 2015. [Google Scholar]
- Kelly, B.; Kearney, D. Thermal Storage Commercial Plant Design Study for a 2-Tank Indirect Molten Salt System: Final Report, 13 May 2002–31 December 2004; National Renewable Energy Lab (NREL): Golden, CO, USA, 2002.
- Decker, T.; Burkhardt, J. Life Cycle Assessment of Thermal Energy Storage: Two-Tank Indirect and Thermocline; Energy Sustainability: San Francisco, CA, USA, 2017; pp. 1–2. [Google Scholar]
- Hoffmann, J.F.; Fasquelle, T.; Goetz, V.; Py, X. A thermocline thermal energy storage system with filler materials for concentrated solar power plants: Experimental data and numerical model sensitivity to different experimental tank scales. Appl. Therm. Eng. 2016, 100, 753–761. [Google Scholar] [CrossRef]
- Freeman, J.; Guarracino, I.; Kalogirou, S.A.; Markides, C.N. A small-scale solar organic Rankine cycle combined heat and power system with integrated thermal energy storage. Appl. Therm. Eng. 2017, 127, 1543–1554. [Google Scholar] [CrossRef]
- Alvi, J.Z.; Feng, Y.; Wang, Q.; Imran, M.; Alvi, J. Modelling, simulation and comparison of phase change material storage based direct and indirect solar organic Rankine cycle systems. Appl. Therm. Eng. 2020, 170, 114780. [Google Scholar] [CrossRef]
- Khatoon, S.; Almefreji, N.M.A.; Kim, M.-H. Thermodynamic Study of a Combined Power and Refrigeration System for Low-Grade Heat Energy Source. Energies 2021, 14, 410. [Google Scholar] [CrossRef]
- Wang, J.; Yan, Z.; Zhao, P.; Dai, Y. Off-design performance analysis of a solar-powered organic Rankine cycle. Energy Convers. Manag. 2014, 80, 150–157. [Google Scholar] [CrossRef]
- Wang, M.; Wang, J.; Zhao, Y.; Zhao, P.; Dai, Y. Thermodynamic analysis and optimization of a solar-driven regenerative organic Rankine cycle (ORC) based on flat-plate solar collectors. Appl. Therm. Eng. 2013, 50, 816–825. [Google Scholar] [CrossRef]
- Li, S.; Ma, H.; Li, W. Dynamic performance analysis of solar organic Rankine cycle with thermal energy storage. Appl. Therm. Eng. 2018, 129, 155–164. [Google Scholar] [CrossRef]
- Freeman, J.; Hellgardt, K.; Markides, C.N. An assessment of solar-powered organic Rankine cycle systems for combined heating and power in UK domestic applications. Appl. Energy 2015, 138, 605–620. [Google Scholar] [CrossRef]
- Global Solar Atlas. Available online: https://globalsolaratlas.info/map (accessed on 3 February 2021).
- European Commission. Available online: https://ec.europa.eu/jrc/en/PVGIS/docs/faq (accessed on 3 February 2021).
- Wang, J.; Dai, Y.; Gao, L.; Ma, S. A new combined cooling, heating and power system driven by solar energy. Renew. Energy 2009, 34, 2780–2788. [Google Scholar] [CrossRef]
- MATLAB Math Works—R2015a (Version 9.2). 2015. Available online: https://ch.mathworks.com/products/matlab/whatsnew.html (accessed on 3 February 2021).
- Lemmon, E.; Mc Linden, M.; Huber, M. NIST Reference Fluid Thermodynamic and Transport Properties Database: REFPROP Version 10. NIST Stand. Ref. Database 2018, 23, v7. [Google Scholar]




| Efficiency Coefficient | First Heat Loss Coefficient | Second Heat Loss Coefficient |
|---|---|---|
| 0.6831 | 0.2125 | 0.001672 |
| Property | Value |
|---|---|
| Molar mass (Kg/kmol) | 134.05 |
| Critical temperature (°C) | 154.01 |
| Critical pressure (MPa) | 3.651 |
| ODP | 0 |
| GWP | 820 |
| Parameter | Value | Unit |
|---|---|---|
| Number of CPC | 75 | - |
| Size of CPC | 2 | |
| Height of TES | 2 | |
| The diameter of TES | 1 | |
| Thickness | 0.006 | |
| Expander efficiency | 0.85 | % |
| Pump efficiency | 0.80 | % |
| Pinch point temperature in the evaporator | 5 | °C |
| Pressure inlet to the expander | 1300 | kPa |
| Pressure outlet from the expander | 180 | kPa |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Almefreji, N.M.A.; Khan, B.; Kim, M.-H. Thermodynamic Performance Analysis of Solar Based Organic Rankine Cycle Coupled with Thermal Storage for a Semi-Arid Climate. Machines 2021, 9, 88. https://doi.org/10.3390/machines9050088
Almefreji NMA, Khan B, Kim M-H. Thermodynamic Performance Analysis of Solar Based Organic Rankine Cycle Coupled with Thermal Storage for a Semi-Arid Climate. Machines. 2021; 9(5):88. https://doi.org/10.3390/machines9050088
Chicago/Turabian StyleAlmefreji, Nasser Mohammed A., Babras Khan, and Man-Hoe Kim. 2021. "Thermodynamic Performance Analysis of Solar Based Organic Rankine Cycle Coupled with Thermal Storage for a Semi-Arid Climate" Machines 9, no. 5: 88. https://doi.org/10.3390/machines9050088
APA StyleAlmefreji, N. M. A., Khan, B., & Kim, M.-H. (2021). Thermodynamic Performance Analysis of Solar Based Organic Rankine Cycle Coupled with Thermal Storage for a Semi-Arid Climate. Machines, 9(5), 88. https://doi.org/10.3390/machines9050088







