Sources of Excitation and Models for Cylindrical Gear Dynamics: A Review
Abstract
1. Introduction
2. Sources of Excitation
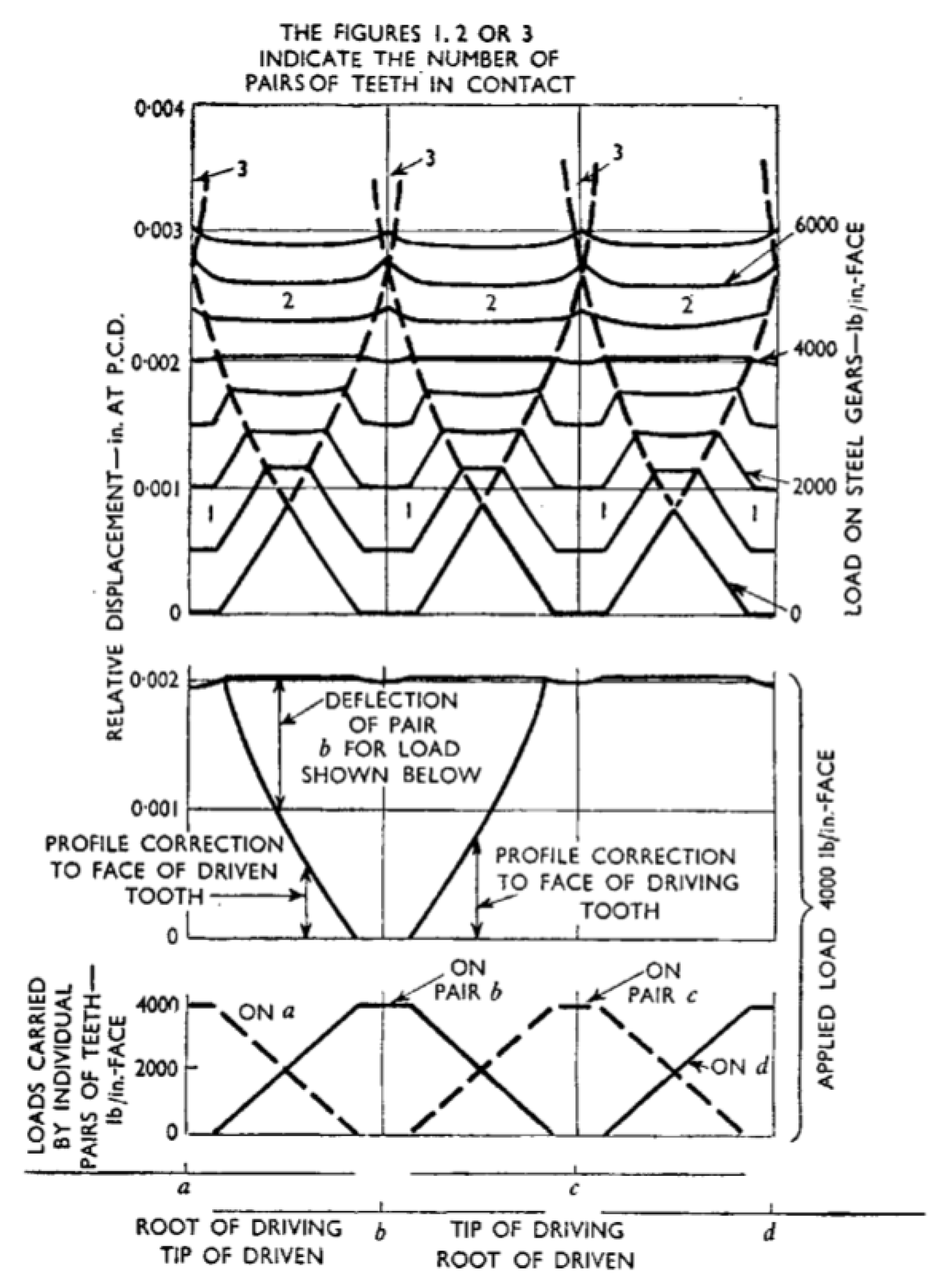
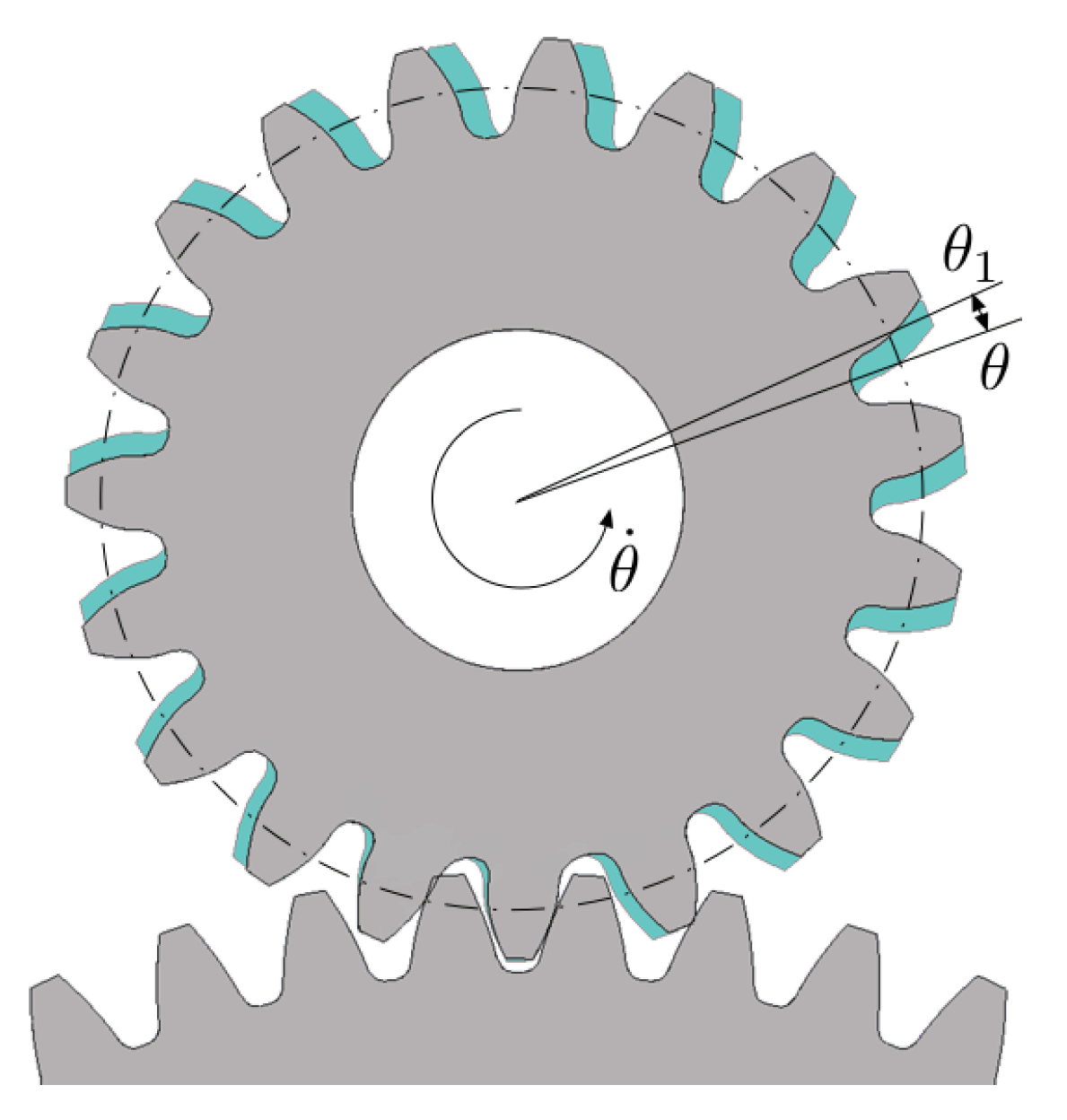
2.1. Transmission Error
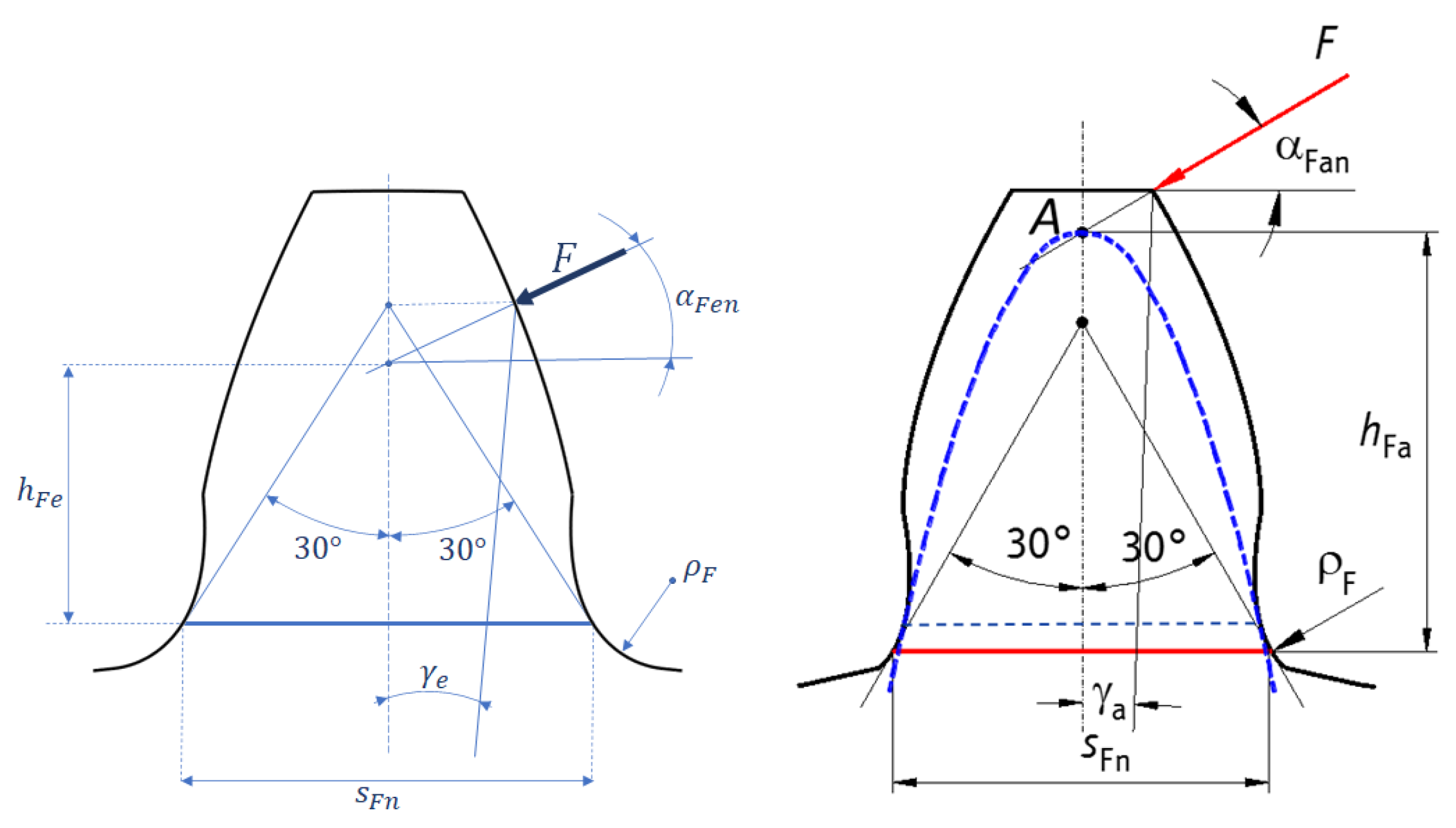
2.2. Other Sources
3. Dynamic Models
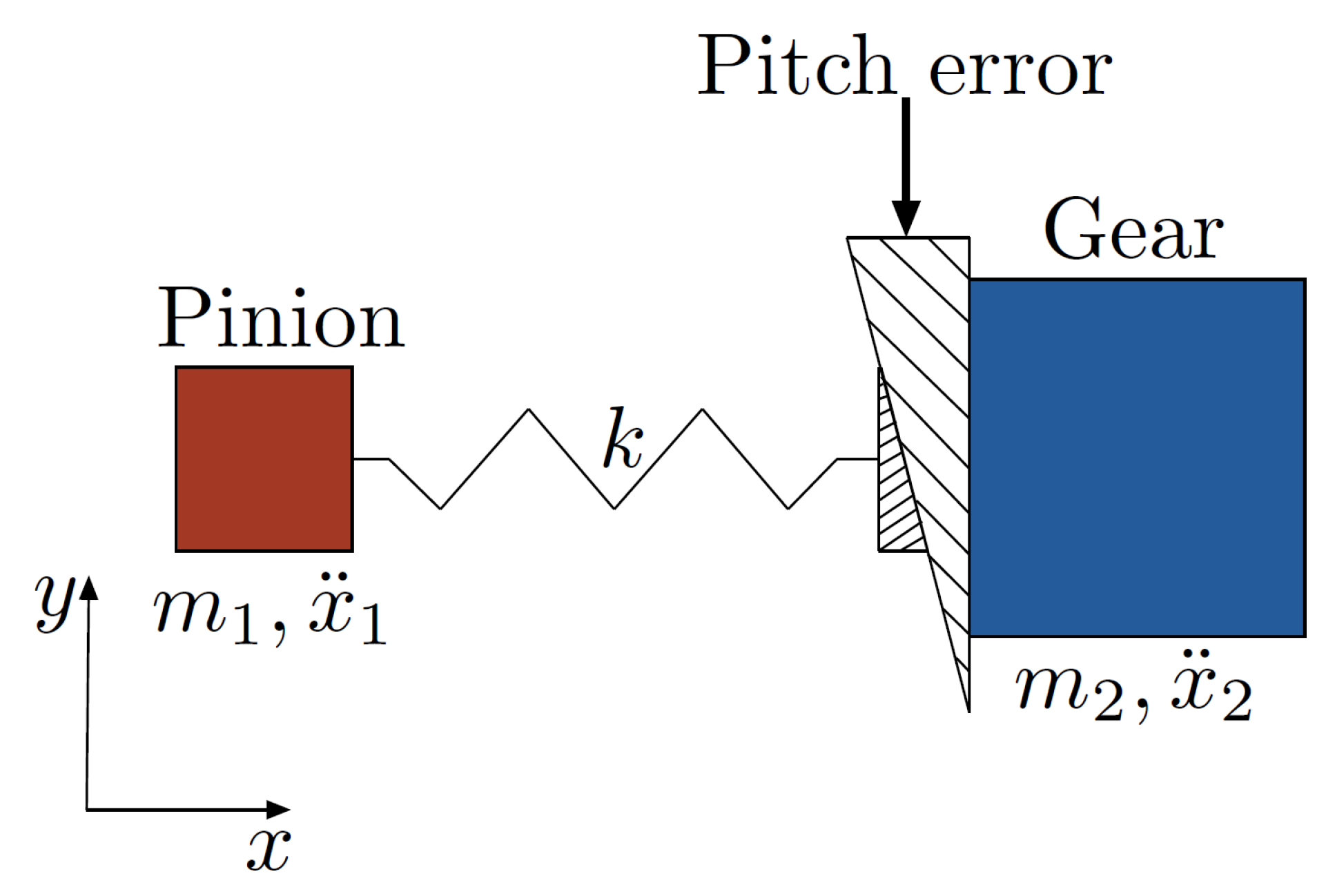
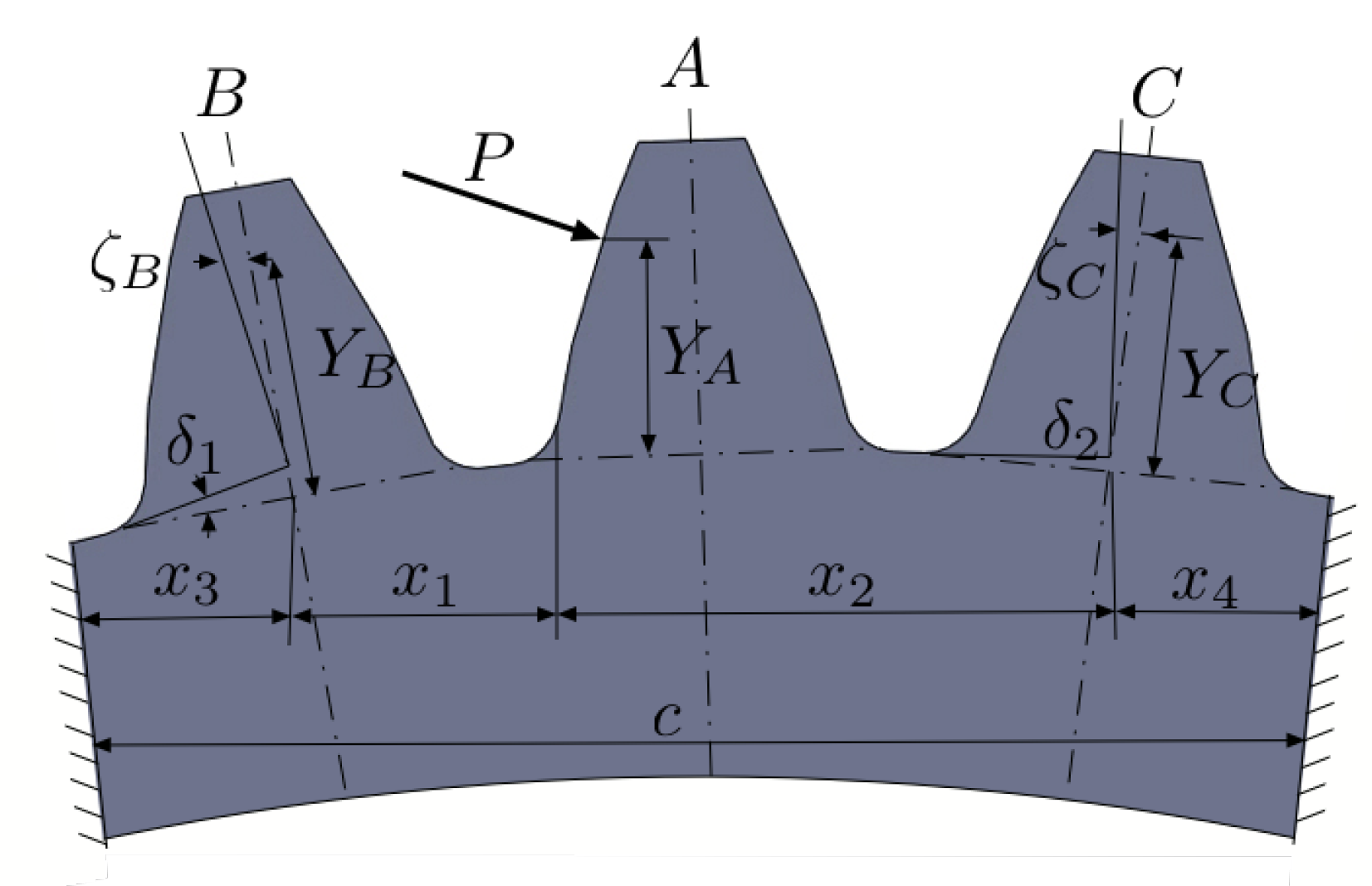
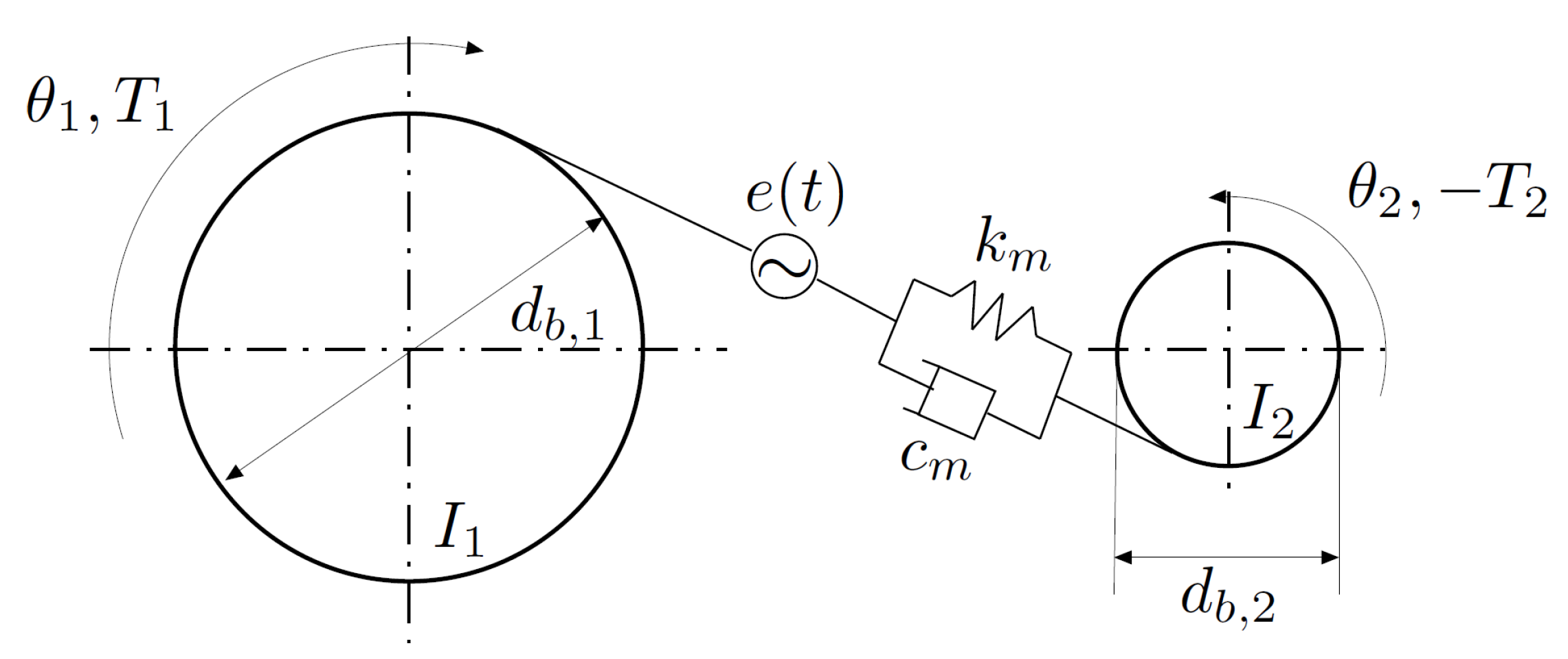
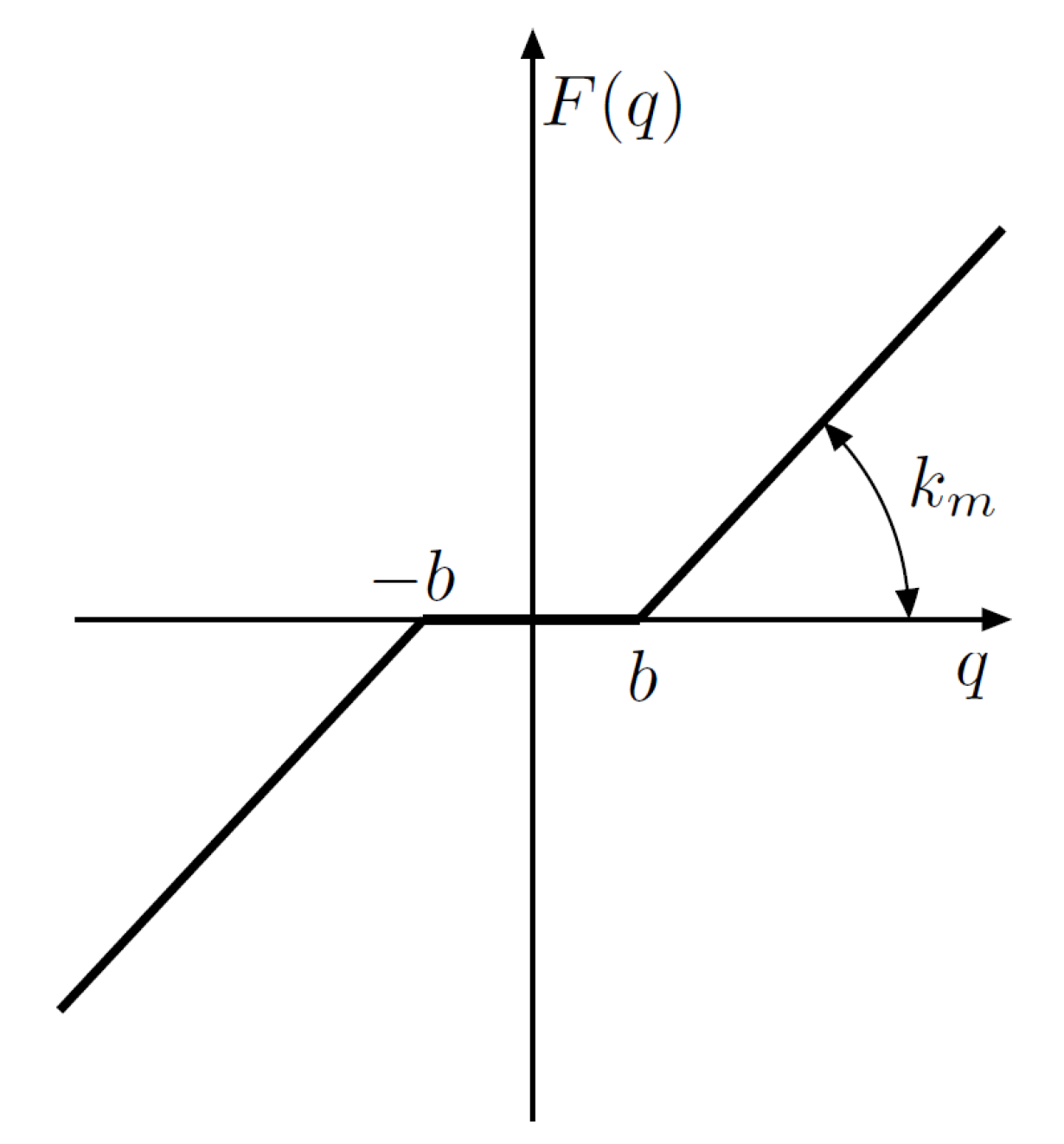
3.1. 1D Models
3.2. Advanced Models
4. Future Perspectives and Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| FE | Finite Element |
| DF | Dynamic Factor |
| TE | Transmission Error |
| (L)STE | (Loaded) Static Transmission Error |
| MTE | Manufacturing Transmission Error |
| NLTE | No Load Transmission Error |
| DTE | Dynamic Transmission Error |
| TVMS | Time-Varying Mesh Stiffness |
| BTVS | Bearing Time-Varying Stiffness |
References
- Burrows, M.; Sutton, G. Interacting Gears Synchronize Propulsive Leg Movements in a Jumping Insect. Science 2013, 341, 1254–1256. [Google Scholar] [CrossRef]
- Ozguven, H.N.; Houser, D.R. Mathematical models used in gear dynamics—A review. J. Sound Vib. 1988, 121, 383–411. [Google Scholar] [CrossRef]
- Abersek, B.; Flasker, J.; Glodez, S. Review of mathematical and experimental models for determination of service life of gears. Eng. Fract. Mech. 2004, 71, 439–453. [Google Scholar] [CrossRef]
- Prasil, L.; Mackerle, J. Finite element analyses and simulations of gears and gear drives a bibliography 1997–2006. Int. J. Comput. Aided Eng. Softw. 2008, 25, 196–219. [Google Scholar] [CrossRef]
- Fundamental Rating Factors and Calculation Methods for Involute Spur and Helical Gear Teeth; AGMA: Virginia, VA, USA, 2001.
- Calculation of Load Capacity of Spur and Helical Gears; ISO: Geneva, Switzerland, 2006.
- Fisher, A. Factors in Calculating the Load Carrying Capacity of Helical. Machinery 1961, 98, 545–552. [Google Scholar]
- Ross, A.A. Tooth Pressures for High-Speed Gears. Machinery 1927, 34, 110–112. [Google Scholar]
- Tuplin, W.A. Gear tooth stresses at high speed. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 1950, 163, 162–167. [Google Scholar] [CrossRef]
- Harris, S.L. Dynamic loads on teeth of spur gears. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 1958, 172, 87–112. [Google Scholar] [CrossRef]
- Velex, P.; Ajmi, M. On the modelling of excitations in geared systems by transmission errors. J. Sound Vib. 2006, 290, 882–909. [Google Scholar] [CrossRef]
- Velex, P.; Ajmi, M. Dynamic tooth loads and quasi-static transmission errors in helical gears- Approximate dynamic factor formulae. Mech. Mach. Theory 2007, 42, 1512–1526. [Google Scholar] [CrossRef]
- Velex, P.; Chapron, M.; Fakhfakh, H.; Bruyère, J.; Becquerelle, S. On transmission errors and profile modifications minimising dynamic tooth load in multi-mesh gears. J. Sound Vib. 2016, 379, 28–52. [Google Scholar] [CrossRef]
- Chi, C.W.; Howard, I.; Wang, J.D. An Experimental Investigation of the Static Transmission Error and Torsional Mesh Stiffness of Nylon Gears. In Proceedings of the ASME 2007 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Las Vegas, NV, USA, 4–7 September 2007; Volume 7, pp. 207–216. [Google Scholar]
- Raghuwanshi, N.K.; Parey, A. Experimental measurement of gear mesh stiffness of cracked spur gear by strain gauge technique. Measurement 2016, 86, 266–275. [Google Scholar] [CrossRef]
- Raghuwanshi, N.K.; Parey, A. Experimental measurement of mesh stiffness by laser displacement sensor technique. Measurement 2018, 128, 63–70. [Google Scholar] [CrossRef]
- Chaari, F.; Fakhfakh, T.; Haddar, M. Analytical modelling of spur gear tooth crack and influence on gearmesh stiffness. Eur. J. Mech. A/Solids 2009, 28, 461–468. [Google Scholar] [CrossRef]
- Ma, H.; Song, R.Z.; Wen, B.C. Time-varying mesh stiffness calculation of cracked spur gears. Eng. Fail. Anal. 2014, 44, 179–194. [Google Scholar] [CrossRef]
- Chen, Z.; Zhou, Z.; Zhai, W.; Wang, K. Improved analytical calculation model of spur gear mesh excitations with tooth profile deviations. Mech. Mach. Theory 2020, 149, 103838. [Google Scholar] [CrossRef]
- Ma, H.; Pang, X.; Feng, R.J.; Zeng, J.; Wen, B.C. Improved time-varying mesh stiffness model of cracked spur gears. Eng. Fail. Anal. 2015, 55, 271–287. [Google Scholar] [CrossRef]
- Hu, Z.H.; Tang, J.Y.; Zhong, J.; Chen, S.Y.; Yan, H.Y. Effects of tooth profile modification on dynamic responses of a high speed gear-rotor-bearing system. Mech. Syst. Signal Process. 2016, 76–77, 294–318. [Google Scholar] [CrossRef]
- Ye, S.; Tsai, S.J. A computerized method for loaded tooth contact analysis of high-contact-ratio spur gears with or without flank modification considering tip corner contact and shaft misalignment. Mech. Mach. Theory 2016, 97, 190–214. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, Y.; Tang, W.; Liu, P. Parametric finite element modeling and tooth contact analysis of spur and helical gears including profile and lead modifications. Eng. Comput. 2017, 34, 2877–2898. [Google Scholar] [CrossRef]
- Munro, R.G. Effect of Geometrical Errors on the Transmission of Motion between Gears. Inst. Mech. Eng. 1969, 184, 79–84. [Google Scholar] [CrossRef]
- Li, S. Effects of machining errors, assembly errors and tooth modifications on loading capacity, load-sharing ratio and transmission error of a pair of spur gears. Mech. Mach. Theory 2007, 42, 698–726. [Google Scholar] [CrossRef]
- Wei, J.; Sun, W.; Wang, L. Effect of flank deviation on load distributions for helical gear. J. Mech. Sci. Technol. 2011, 25, 1781–1789. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, Q.; Ma, H.; Huang, J.; Zhao, C. Dynamic analysis of three-dimensional helical geared rotor system with geometric eccentricity. J. Mech. Sci. Technol. 2013, 27, 3231–3242. [Google Scholar] [CrossRef]
- Inalpolat, M.; Handschuh, M.; Kahraman, A. Influence of indexing errors on dynamic response of spur gear pairs. Mech. Syst. Signal Process. 2015, 60–61, 391–405. [Google Scholar] [CrossRef]
- Wang, Q.; Zhang, Y. A model for analyzing stiffness and stress in a helical gear pair with tooth profile errors. J. Vib. Control 2017, 23, 272–289. [Google Scholar] [CrossRef]
- Li, H.; Chen, S.; Tang, J.; Chen, W.; Ouyang, H. A novel approach for calculating no-load static transmission error based on measured discrete tooth surfaces. Mech. Mach. Theory 2019, 138, 112–123. [Google Scholar] [CrossRef]
- Sato, T.; Umezawa, K.; Ishikawa, J. Effects of contact ratio and profile correction on gear rotational vibration. Bull. Jpn. Soc. Mech. Eng. 1983, 26, 2010–2016. [Google Scholar] [CrossRef]
- Umezawa, K.; Sato, T.; Ishikawa, J. Simulation on rotational vibration of spur gears. Bull. Jpn. Soc. Mech. Eng. 1984, 27, 102–109. [Google Scholar] [CrossRef][Green Version]
- Umezawa, K.; Sato, T.; Kohno, K. Influence of gear error on rotational vibration of power transmission spur gears (1st report, pressure angle error and normal pitch error). Bull. Jpn. Soc. Mech. Eng. 1984, 27, 569–575. [Google Scholar] [CrossRef]
- Umezawa, K.; Sato, T. Influence of gear error on rotational vibration of power transmission spur gears (2nd report, waved form error). Bull. Jpn. Soc. Mech. Eng. 1985, 28, 2143–2148. [Google Scholar] [CrossRef]
- Umezawa, K.; Ajima, T.; Houhoh, H. Vibration of three axes gear system (in Japanese). Bull. Jpn. Soc. Mech. Eng. 1986, 29, 950–957. [Google Scholar] [CrossRef]
- Kubo, A.; Kiyono, S.; Fujino, M. On analysis and prediction of machine vibration caused by gear meshing (1st report, nature of gear vibration and the total vibrational excitation). Bull. Jpn. Soc. Mech. Eng. 1986, 29, 4424–4429. [Google Scholar] [CrossRef]
- Yang, D.C.H.; Lin, J.Y. Hertzian damping, tooth friction and bending elasticity in gear impact dynamics. Trans. Am. Soc. Mech. Eng. J. Mech. Transm. Autom. Des. 1986, 109, 189–196. [Google Scholar] [CrossRef]
- Weber, C. The Deformation of Load Gears and the Effect on Their Load-Carrying Capacity; Technical Report n.3; British Department of Scientific and Industrial Research: London, UK, 1949. [Google Scholar]
- Weber, C.; Banaschek, K. Formänderung und Profilrücknahme bei Gerad-und Schragverzahnten Antriebstechnik; Vieweg Verlag: Brunswick, Germany, 1953. [Google Scholar]
- Hertz, H.R. On contact between elastic bodies. Collect. Works 1895, 1, 155–173. [Google Scholar]
- Lundberg, G. Elastische Berührung zweier Halbräume. Forsch. Ingenieurwesen 1939, 10, 201–211. [Google Scholar] [CrossRef]
- Ishikawa, J. Fundamental Investigations on the Design of Spur Gears. Bull. T.I.T. 1957, 3, 55–62. [Google Scholar]
- Attia, A.Y. Deflection of spur gear teeth cut in thin rims. J. Eng. Ind. 1964, 86, 333–341. [Google Scholar] [CrossRef]
- Cornell, R.W.; Westervelt, W.W. Dynamic tooth loads and stressing for high contact ratio spur gears. J. Mech. Des. 1978, 100, 69–76. [Google Scholar] [CrossRef]
- Cornell, R.W. Compliance and stress sensitivity of spur gear teeth. J. Mech. Des. 1981, 103, 447–459. [Google Scholar] [CrossRef]
- O’Donnel, W.J. Stress and deflection of built-in beams. J. Eng. Ind. 1963, 85, 265–272. [Google Scholar] [CrossRef]
- Sainsot, P.; Velex, P.; Duverger, O. Contribution of gear body to tooth deflections - A new bidimensional analytical formula. J. Mech. Des. 2004, 126, 748–752. [Google Scholar] [CrossRef]
- Cai, Y.; Hayashi, T. The optimum modification of tooth profile of power transmission spur gears to make the rotational vibration equal zero. Trans. Jpn. Soc. Mech. Eng. 1991, 57, 3957–3963. [Google Scholar] [CrossRef][Green Version]
- Cai, Y.; Hayashi, T. The linear approximated equation of vibration of a pair of spur gears. J. Mech. Des. 1994, 116, 558–564. [Google Scholar] [CrossRef]
- Umezawa, K. The meshing test on helical gears under tooth load transmission (3rd report, the static behaviours of driven gear). Bull. JSME 1974, 17, 1348–1355. [Google Scholar] [CrossRef]
- Umezawa, K.; Suzuki, T.; Sato, T. Vibration of power transmission helical gears: Approximate equation of tooth stiffness. Bull. JSME 1986, 29, 1605–1611. [Google Scholar] [CrossRef]
- Cai, Y. Simulation on the rotational vibration of helical gears in consideration of the tooth separation phenomenon: A new stiffness function of helical involute tooth pair. J. Mech. Des. 1995, 117, 460–469. [Google Scholar] [CrossRef]
- Wang, K.L.; Cheng, H.S. A numerical solution to the dynamic load, film thickness and surface temperatures in spur gears, Part I: Analysis. J. Mech. Des. 1981, 103, 177–187. [Google Scholar] [CrossRef]
- Steward, J.H. The compliance of solid, wide-faced spur gears. J. Mech. Des. 1990, 112, 590–595. [Google Scholar] [CrossRef]
- Deng, G.; Nakanishi, T.; Inoue, K. Bending load capacity enhancement using an asymmetric tooth profile. JSME Int. J. Ser. C 2003, 46, 1171–1177. [Google Scholar] [CrossRef][Green Version]
- Lin, T.; Ou, H.; Li, R. A finite element method for 3D static and dynamic contact/impact analysis of gear drives. Comput. Methods Appl. Mech. Eng. 2007, 196, 1716–1728. [Google Scholar] [CrossRef]
- Pedersen, N.L.; Jorgenses, M.F. On gear tooth stiffness evaluation. Comput. Struct. 2014, 135, 109–117. [Google Scholar] [CrossRef]
- Arafa, M.H.; Megahed, M.M. Evaluation of spur gear mesh compliance usign the finite element method. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 1999, 213, 569–579. [Google Scholar] [CrossRef]
- Hu, W.; Chen, Z. A multi-mesh mpm for simulating the meshing process of spur gears. Comput. Struct. 2003, 81, 1991–2002. [Google Scholar] [CrossRef]
- Wang, J.D.; Howard, I.M. Error analysis of finite element modeling of involute spur gears. J. Mech. Des. 2006, 128, 90–97. [Google Scholar] [CrossRef]
- He, S.; Gunda, R.; Singh, R. Effect of sliding friction on the dynamics of spur gear pair with realistic time-varying stiffness. J. Sound Vib. 2007, 301, 927–949. [Google Scholar] [CrossRef]
- Li, S. Finite element analyses for contact strenght and bending strenght of a pair of spur gears with machining errors, assembly errors and tooth modifications. Mech. Mach. Theory 2007, 42, 88–114. [Google Scholar] [CrossRef]
- Tesfahuneng, Y.A.; Rosa, F.; Gorca, C. The effects of the shape of tooth profile modifications on the transmission error, bending and contact stress of spur gears. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2010, 224, 1749–1758. [Google Scholar] [CrossRef]
- Nikolic, V.; Dolicanin, C.; Dimitrijevic, D. Dynamic model for the stress and strain state analysis of a spur gear transmission. J. Mech. Eng. 2012, 58, 56–67. [Google Scholar] [CrossRef]
- Parker, R.G.; Vijayakar, S.M.; Imajo, T. Non-linear dynamic response of a spur gear pair: Modelling and experimental comparisons. J. Sound Vib. 2000, 237, 435–455. [Google Scholar] [CrossRef]
- Ajmi, M.; Velex, P. A model for simulating the quasi-static and dynamic behaviour of solid wide-faced spur and helical gears. Mech. Mach. Theory 2005, 40, 173–190. [Google Scholar] [CrossRef]
- Guilbault, R.; Gosseling, C.; Cloutier, L. Helical gears, effect of tooth deviations and tooth modifications on load sharing and fillet stresses. J. Mech. Des. 2005, 128, 444–456. [Google Scholar] [CrossRef]
- Houser, D.R.; Oswald, F.; Valco, M. Comparison of transmission error predictions with noise measurements for several spur and helical gears. In Proceedings of the AIAA/ASME/SAE/ASEE 30th Joint Propulsion Conference, Indianapolias, IN, USA, 27–29 June 1994. [Google Scholar]
- Munro, R.G.; Morrish, L.; Palmer, D. Gear transmission error outside the normal path of contact due to corner and top contact. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 1999, 213, 389–400. [Google Scholar] [CrossRef]
- Bonori, G.; Barbieri, M.; Pellicano, F. Optimum profile modifications of spur gears by means of genetic algorithms. J. Sound Vib. 2008, 313, 603–616. [Google Scholar] [CrossRef]
- Faggioni, M.; Samani, F.S.; Bertacchi, G.; Pellicano, F. Dynamic optimization of spur gears. Mech. Mach. Theory 2011, 46, 544–557. [Google Scholar] [CrossRef]
- Conry, T.F.; Seireg, A. A mathematical programming method for design of elastic bodies in contact. J. Appl. Mech. 1971, 38, 387–392. [Google Scholar] [CrossRef]
- Conry, T.F.; Seireg, A. A mathematical programming technique for the evaluation of load distribution and optimal modifications of gear systems. J. Eng. Ind. 1973, 95, 1115–1122. [Google Scholar] [CrossRef]
- Maatar, M.; Velex, P. Quasi-static and dynamic analysis of narrow-faced helical gears with profile and lead modifications. J. Mech. Des. 1997, 119, 474–480. [Google Scholar] [CrossRef]
- Bruyere, J.; Velex, P. Derivation of optimum profile modifications in narrow-faced spur and helical gears using a perturbation method. J. Mech. Des. 2013, 135, 071009. [Google Scholar] [CrossRef]
- Bruyere, J.; Velex, P. A simplified multi-objective analysis of optimum profil modification in spur and helical gears. Mech. Mach. Theory 2014, 80, 70–83. [Google Scholar] [CrossRef]
- Bruyere, J.; Gu, X.; Velex, P. On the analytical definition of profile modifications minimising transmission error variation in narrow-faced spur helical gears. Mech. Mach. Theory 2015, 92, 257–272. [Google Scholar] [CrossRef]
- Wang, C.C. Rotational vibration with backlash: Part 1. J. Mech. Des. 1978, 100, 363–373. [Google Scholar] [CrossRef]
- Wang, C.C. Rotational vibration with backlash: Part 2. J. Mech. Des. 1981, 103, 387–397. [Google Scholar] [CrossRef]
- Bahgat, B.M.; Osman, M.O.M.; Sankar, T.S. On the spur-gear dynamic tooth-load under consideration of system elasticity and tooth involute profile. J. Mech. Transm. Autom. Des. 1983, 105, 302–309. [Google Scholar] [CrossRef]
- Kadmiri, Y.; Perret-Liaudet, J.; Rigaud, E.; Le Bot, A.; Vary, L. Influence ofmultiharmonics excitation on rattle noise in automotive gearboxes. Adv. Acoust. Vib. 2011, 2011, 659797. [Google Scholar]
- Brancati, R.; Rocca, E.; Siano, D.; Viscardi, M. Experimental vibro-acoustic analysis of the gear rattle induced by multi-harmonic excitation. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2018, 232, 785–796. [Google Scholar] [CrossRef]
- Bel Mabrouk, I.; El Hami, A.; Walha, L.; Zghal, B. Dynamic vibrations in wind energy systems: Application to vertical axis wind turbine. Mech. Syst. Signal Process. 2017, 85, 396–414. [Google Scholar] [CrossRef]
- Garambois, P.; Donnard, G.; Rigaud, E.; Perret-Liaudet, J. Multiphysics coupling between periodic gear mesh excitation and input/output fluctuating torques: Application to a roots vacuum pump. J. Sound Vib. 2017, 405, 158–174. [Google Scholar] [CrossRef]
- Liu, F.; Zhang, L.; Jiang, H.; Zhang, J. Nonlinear dynamic analysis of two external excitations for the gear system using an original computational algorithm. Mech. Syst. Signal Process. 2020, 144, 106823. [Google Scholar] [CrossRef]
- Jiang, H.; Liu, F. Dynamic modeling and analysis of spur gears considering friction–vibration interactions. J. Braz. Soc. Mech. Sci. Eng. 2017, 39, 4911–4920. [Google Scholar] [CrossRef]
- Han, L.; Xu, L.; Qi, H. Influences of friction and mesh misalignment on time-varying mesh stiffness of helical gears. J. Mech. Sci. Technol. 2017, 31, 3121–3130. [Google Scholar] [CrossRef]
- Jiang, H. Analysis of time-varying friction excitations in helical gears with refined general formulation. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2015, 229, 2467–2483. [Google Scholar] [CrossRef]
- Hou, S.; Wei, J.; Zhang, A.; Lim, T.C.; Zhang, C. Study of dynamic model of helical/herringbone planetary gear system with friction excitation. J. Comput. Nonlinear Dyn. 2018, 13, 121007. [Google Scholar] [CrossRef]
- Hedlund, J.; Lehtovaara, A. Testing method for the evaluation of parametric excitation of cylindrical gears. Nondestruct. Test. Eval. 2008, 23, 285–299. [Google Scholar] [CrossRef]
- Guo, F.; Fang, Z. The statistical analysis of the dynamic performance of a gear system considering random manufacturing errors under different levels of machining precision. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2020, 234, 3–18. [Google Scholar] [CrossRef]
- Inavolu, N.; Kamani, S.N.; Jaganmohan, R.M. Driveline Noise Source Identification and Reduction in Commercial Vehicles; SAE Technical Paper 2018-01-1474; SAE: Warrendale, PA, USA, 2018. [Google Scholar]
- Teja, R.; Milind, T.R.; Glover, R.; Sonawane, S. Dynamic Analysis of Helical Gear Pair Due to te and Shuttling Moment Excitations; SAE Technical Paper 2017-01-1818; SAE: Warrendale, PA, USA, 2017. [Google Scholar]
- Fang, Y.; Zhang, T. Modeling and Analysis of Electric Powertrain nvh under Multi-Source Dynamic Excitation; SAE Technical Paper 2014-01-2870; SAE: Warrendale, PA, USA, 2014. [Google Scholar]
- Ozguven, H.N.; Houser, D.R. Dynamic analysis of high speed gears by using the loaded static transmission error. J. Sound Vib. 1988, 125, 71–83. [Google Scholar] [CrossRef]
- Kubo, A.; Yamada, K.; Aida, T.; Sato, S. Research on ultra high speed gear devices. Bull. JSME 1972, 38, 2692–2715. [Google Scholar] [CrossRef]
- Kahraman, A.; Singh, R. Non-linear dynamics of a spur gear pair. J. Sound Vib. 1990, 142, 45–79. [Google Scholar] [CrossRef]
- Kahraman, A.; Singh, R. Interactions between time-varying mesh stiffness and clearance non-linearities in a geared system. J. Sound Vib. 1991, 146, 135–156. [Google Scholar] [CrossRef]
- Blankenship, G.W.; Kahraman, A. Steady state forced response of a mechanical oscillator with combined parametric excitation and clearance type non-linearity. J. Sound Vib. 1995, 185, 743–765. [Google Scholar] [CrossRef]
- Kasuba, R.; Evans, J.W. An extended model for determining dynamic loads in spur gearing. Trans. Am. Soc. Mech. Eng. J. Mech. Des. 1981, 103, 398–409. [Google Scholar] [CrossRef]
- Yang, D.C.H.; Sun, Z.S. A rotary model for spur gears dynamics. Trans. Am. Soc. Mech. Eng. J. Mech. Transm. Autom. Des. 1985, 107, 529–535. [Google Scholar] [CrossRef]
- Velex, P.; Maatar, M. A mathematical model for analyzing the influence of shape deviations and mounting errors on gear dynamic behaviour. J. Sound Vib. 1996, 191, 629–660. [Google Scholar] [CrossRef]
- Munro, R.G. The D.C. component of gear transmission error. In Proceedings of the 1989 International Power Transmission and Gearing Conference, Chicago, IL, USA, 25–28 April 1989; pp. 467–470. [Google Scholar]
- Amabili, M.; Rivola, A. Dynamic analysis of spur gear pairs: Steady-state response and stability of the sdof model with time-varying meshing damping. Mech. Syst. Signal Process. 1997, 11, 375–390. [Google Scholar] [CrossRef]
- Amabili, M.; Fregolent, A. A method to identify modal parameters and gear errors by vibrations of a spur gear pair. J. Sound Vib. 1998, 214, 339–357. [Google Scholar] [CrossRef]
- Bonori, G.; Pellicano, F. Non-smooth dynamics of spur gears with manufacturing errors. J. Sound Vib. 2007, 306, 271–283. [Google Scholar] [CrossRef]
- Lin, H.H.; Liou, C.H. A Parametric Study of Gear Dynamics; Techincal Report NASA/CR-1998-206598; NASA: Linthicum Heights, MD, USA, 1998. [Google Scholar]
- Kahraman, A.; Blankenship, G.W. Effect of involute tip relief on dynamic reponse of spur gear pairs. J. Mech. Des. Trans. ASME 1999, 121, 313–315. [Google Scholar] [CrossRef]
- Kahraman, A.; Blankenship, G.W. Effect of involute contact ratio on spur gear dynamics. J. Mech. Des. Trans. ASME 1999, 121, 112–118. [Google Scholar] [CrossRef]
- Vedmar, L.; Henriksson, B. A general approach for determining dynamic forces in spur gears. J. Mech. Des. 1998, 120, 593–598. [Google Scholar] [CrossRef]
- Vaishya, M.; Singh, R. Sliding friction-induced non-linearity and parametric effects in gears. J. Sound Vib. 2001, 248, 671–694. [Google Scholar] [CrossRef]
- Vaishya, M.; Singh, R. Strategies for modeling friction in gear dynamics. J. Mech. Des. 2003, 125, 383–393. [Google Scholar] [CrossRef]
- Maliha, R.; Dogruer, C.U.; Ozguven, N.H. Nonlinear dynamic modeling of gear-shaft-disk-bearing systems using finite elements and describing functions. J. Mech. Des. 2004, 126, 534–541. [Google Scholar] [CrossRef]
- Theodossiades, S.; Natsiavas, S. Non-linear dynamics of gear-pair systems with periodic stiffness and backlash. J. Sound Vib. 2000, 229, 287–310. [Google Scholar] [CrossRef]
- Theodossiades, S.; Natsiavas, S. On geared rotordynamic systems with oil journal bearings. J. Sound Vib. 2001, 243, 721–745. [Google Scholar] [CrossRef]
- Theodossiades, S.; Natsiavas, S. Periodic and chaotic dynamics of motor-driven gear-pair systems with backlash. Chaos Solitons Fractals 2001, 12, 2427–2440. [Google Scholar] [CrossRef]
- Bollinger, J.G.; Harker, R.J. Instability potential of high speed gearing. Ind. Math. 1967, 17, 39–55. [Google Scholar]
- Benton, M.; Seireg, A. Simulation of resonances and instability conditions in pinion-gear systems. Trans. Am. Soc. Mech. Eng. J. Mech. Des. 1978, 100, 26–32. [Google Scholar] [CrossRef]
- Kumar, A.S.; Sankar, T.S.; Osman, M.O.M. On dynamic tooth load and stability of a spur-gear system using the state-space approach. J. Mech. Transm. Autom. Des. 1985, 107, 54–60. [Google Scholar] [CrossRef]
- Dai, X.; Cooley, C.G.; Parker, R.G. Dynamic tooth root strains and experimental correlations in spur gear pairs. Mech. Mach. Theory 2016, 101, 60–74. [Google Scholar] [CrossRef]
- Cooley, C.G.; Parker, R.G.; Vijayakar, S.M. A frequency domain finite element approach for three-dimensional gear dynamics. J. Vib. Acoust. 2011, 133, 041004. [Google Scholar] [CrossRef]
- Parker, R.G.; Agashe, V.; Vijayakar, S.M. Dynamic response of a planetary gear system using a finite element/contact mechanics model. J. Mech. Des. 2000, 122, 304–310. [Google Scholar] [CrossRef]
- Ericson, T.M.; Parker, R.G. Planetary gear modal vibration experiments and correlation against lumped-parameter and finite element models. J. Sound Vib. 2013, 332, 2350–2375. [Google Scholar] [CrossRef]
- Kahraman, A.; Vijayakar, S.M. Effect of internal gear flexibility on the quasi-static behavior of a planetary gear set. J. Mech. Des. 2001, 123, 408–415. [Google Scholar] [CrossRef]
- Singh, A.; Kahraman, A.; Ligata, H. Internal gear strains and load sharing in planetary transmissions. J. Mech. Des. 2008, 130, 072602. [Google Scholar] [CrossRef]
- Kahraman, A.; Ligata, H.; Singh, A. Influence of ring gear rim thickness on planetary gear set behaviour. J. Mech. Des. 2010, 132, 1–8. [Google Scholar] [CrossRef]
- Liu, G.; Hong, J.; Parker, R.G. Influence of simultaneous time-varying bearing and tooth mesh stiffness fluctuations on spur gear pair vibration. Nonlinear Dyn. 2019, 97, 1403–1424. [Google Scholar] [CrossRef]
- Eritnel, T.; Parker, R.G. Three-dimensional nonlinear vibration of gear pairs. J. Sound Vib. 2012, 331, 3628–3648. [Google Scholar] [CrossRef]
- Eritnel, T.; Parker, R.G. An investigation of tooth mesh nonlinearity and partial contact loss in gear pairs using a lumped-parameter model. Mech. Mach. Theory 2012, 56, 28–51. [Google Scholar] [CrossRef]
- Eritnel, T.; Parker, R.G. Nonlinear vibration of gears with tooth surface modifications. J. Vib. Acoust. 2013, 135, 051005. [Google Scholar] [CrossRef]
- Dai, X.; Cooley, C.G.; Parker, R.G. An efficient hybrid analytical-computational method for nonlinear vibration of spur gear pairs. J. Vib. Acoust. 2018, 141, 011006. [Google Scholar] [CrossRef]
- Cooley, C.G.; Parker, R.G. Vibration of high-speed rotating rings coupled to spatial space-fixed stiffness. J. Sound Vib. 2014, 333, 2631–2648. [Google Scholar] [CrossRef]
- Cooley, C.G.; Parker, R.G. Limitations of an inextensible model for the vibration of high-speed rotating elastic rings with attached space-fixed discrete stiffness. Eur. J. Mech. A/Solids 2015, 54, 187–197. [Google Scholar] [CrossRef]
- Liu, C.; Cooley, C.G.; Parker, R.G. Parametric instability of spinning elastic rings excited by fluctuating space-fixed stiffnesses. J. Sound Vib. 2017, 400, 533–549. [Google Scholar] [CrossRef]
- Cooley, C.G.; Liu, C.; Dai, X.; Parker, R.G. Techniques for the calculation of gear pair mesh stiffness. In Proceedings of the International Conference on Power Transmissions 2016 (ICPT 2016), Chongqing, China, 27–30 October 2016; Volume 1, pp. 161–166. [Google Scholar]
- Hotait, M.A.; Kahraman, A. Experiments on the relationship between the dynamic transmission error and the dynamic stress factor of spur gear pairs. Mech. Mach. Theory 2013, 70, 116–128. [Google Scholar] [CrossRef]
- Palermo, A.; Mundo, D.; Hadjit, R.; Desmet, W. Multibody element for spur and helical transmission error and the dynamic stress factor of spur gear pairs. Mech. Mach. Theory 2013, 62, 13–30. [Google Scholar] [CrossRef]
- Lim, T.; Singh, R. A Review of Gear Housing Dynamics and Acoustics Literature; NASA Technical Report CR-185148; NASA: Columbus, OH, USA, 1989. [Google Scholar]
- Lim, T.; Singh, R. Vibration transmission through rolling element bearings, part II: System studies. J. Sound Vib. 1990, 139, 201–225. [Google Scholar] [CrossRef]
- Lim, T.; Singh, R. Vibration transmission through rolling element bearings, part III: Geared rotor system studies. J. Sound Vib. 1991, 151, 31–54. [Google Scholar] [CrossRef]
- Rigaud, E.; Sabot, J. Effect of Elasticity of Shafts, Bearings, Casing and Couplings on the Critical Rotational Speeds of a Gearbox; VDI Berichte: Düsseldorf, Germany, 1996; pp. 833–845. [Google Scholar]
- Zhou, J.; Sun, W.; Tao, Q. Gearbox low-noise design method based on panel acoustic contribution. Math. Probl. Eng. 2014, 2014, 850549. [Google Scholar] [CrossRef]
- Guo, Y.; Eritnel, T.; Ericson, T.M.; Parker, R.G. Vibro-acoustic propagation of gear dynamics in a gear-bearing-housing system. J. Sound Vib. 2014, 333, 5762–5785. [Google Scholar] [CrossRef]
- Qiao, Z.; Zhou, J.; Sun, W.; Zhang, X. A Coupling Dynamics Analysis Method for Two-Stage Spur Gear under Multisource Time-Varying Excitation. Shock Vib. 2019, 2019, 7350701. [Google Scholar] [CrossRef]
- Zhang, A.; Wei, J.; Shi, L.; Qin, D.; Lim, T. Modeling and dynamic response of parallel shaft gear transmission in non-inertial system. Nonlinear Dyn. 2019, 98, 997–1017. [Google Scholar] [CrossRef]
- Liu, C.; Fang, Z.; Wang, F. An improved model for dynamic analysis of a double-helical gear reduction unit by hybrid user-defined elements: Experimental and numerical validation. Mech. Mach. Theory 2018, 127, 96–111. [Google Scholar] [CrossRef]
- Huang, K.; Yi, Y.; Cheng, Z.; Chen, H. Nonlinear Dyn. analysis of high contact ratio gears system with multiple clearances. J. Braz. Soc. Mech. Sci. Eng. 2020, 42, 98. [Google Scholar] [CrossRef]
- Cao, Z.; Chen, Z.; Jiang, H. Nonlinear Dyn. of a spur gear pair with force-dependent mesh stiffness. Nonlinear Dyn. 2020, 99, 1227–1241. [Google Scholar] [CrossRef]
- Xiao, Z.; Zhou, C.; Chen, S.; Li, Z. Effects of oil film stiffness and damping on spur gear dynamics. Nonlinear Dyn. 2019, 96, 145–159. [Google Scholar] [CrossRef]
- Han, L.; Qi, H. Dynamic response analysis of helical gear pair considering the interaction between friction and mesh stiffness. Meccanica 2019, 54, 2325–2337. [Google Scholar] [CrossRef]
- Cirelli, M.; Valentini, P.; Pennestrì, E. A study of the non-linear dynamic response of spur gear using a multibody contact based model with flexible teeth. J. Sound Vib. 2019, 445, 148–167. [Google Scholar] [CrossRef]
- Cappellini, N.; Tamarozzi, T.; Blockmans, B.; Fiszer, J.; Cosco, F.; Desmet, W. Semi-analytic contact technique in a non-linear parametric model order reduction method for gear simulations. Meccanica 2018, 53, 49–75. [Google Scholar] [CrossRef]
- Liu, J.; Liu, J.; Shu, X.; Mikkola, A.; Ren, G. An efficient multibody dynamic model of three-dimensional meshing contacts in helical gear-shaft system and its solution. Mech. Mach. Theory 2019, 142, 103607. [Google Scholar] [CrossRef]
- Bruyere, J.; Velex, P.; Guilbert, B.; Houser, D. An analytical study on the combination of profile relief and lead crown minimizing transmission error in narrow-faced helical gears. Mech. Mach. Theory 2019, 136, 224–243. [Google Scholar] [CrossRef]
- Marafona, J.; Marques, P.; Martins, R.; Seabra, J. Towards constant mesh stiffness helical gears: The influence of integer overlap ratios. Mech. Mach. Theory 2019, 136, 141–161. [Google Scholar] [CrossRef]
- Atanasiu, V.; Buium, F.; Leohchi, D. Effects of Hob Tip Radius and Addendum Modification Coefficients on Tooth Bending Stress of Spur Gears under Dynamic Conditions. IOP Conf. Ser. Mater. Sci. Eng. 2018, 444, 052004. [Google Scholar] [CrossRef]
- Rosso, C.; Bonisoli, E. An Unified Framework for Studying Gear Dynamics Through Model Reduction Techniques. In Special Topics in Structural Dynamics, Proceedings of the 34th IMAC, A Conference and Exposition on Structural Dynamics, Orlando, FL, USA, 25–28 January 2016; Springer: Berlin, Germany, 2016; Volume 6, pp. 233–242. [Google Scholar]
- Olivieri, L.; Rosso, C.; Zucca, S. Influence of Actual Static Transmission Error and Contact Ratio on Gear Engagement Dynamics. In Nonlinear Dynamics, Proceedings of the 35th IMAC, A Conference and Exposition on Structural Dynamics, Orange County, CA, USA, 31 January–2 February 2017; Springer: Berlin, Germany, 2017; Volume 1, pp. 143–154. [Google Scholar]
- Bruzzone, F.; Maggi, T.; Marcellini, C.; Rosso, C.; Delprete, C. Proposal of a novel approach for 3D tooth contact analysis and calculation of the static transmission error in loaded gears. Procedia Struct. Integr. 2019, 24, 178–189. [Google Scholar] [CrossRef]
- Rosso, C.; Bruzzone, F.; Maggi, T.; Marcellini, C. Influence of Micro Geometry Modification on Gear Dynamics; SAE Technical Paper 2020-01-1323; SAE: Warrendale, PA, USA, 2020. [Google Scholar]


© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bruzzone, F.; Rosso, C. Sources of Excitation and Models for Cylindrical Gear Dynamics: A Review. Machines 2020, 8, 37. https://doi.org/10.3390/machines8030037
Bruzzone F, Rosso C. Sources of Excitation and Models for Cylindrical Gear Dynamics: A Review. Machines. 2020; 8(3):37. https://doi.org/10.3390/machines8030037
Chicago/Turabian StyleBruzzone, Fabio, and Carlo Rosso. 2020. "Sources of Excitation and Models for Cylindrical Gear Dynamics: A Review" Machines 8, no. 3: 37. https://doi.org/10.3390/machines8030037
APA StyleBruzzone, F., & Rosso, C. (2020). Sources of Excitation and Models for Cylindrical Gear Dynamics: A Review. Machines, 8(3), 37. https://doi.org/10.3390/machines8030037





