Experimental Study on Vibration and Noise Reduction of Gear Transmission System Based on ISFD
Abstract
1. Introduction
2. Materials and Methods
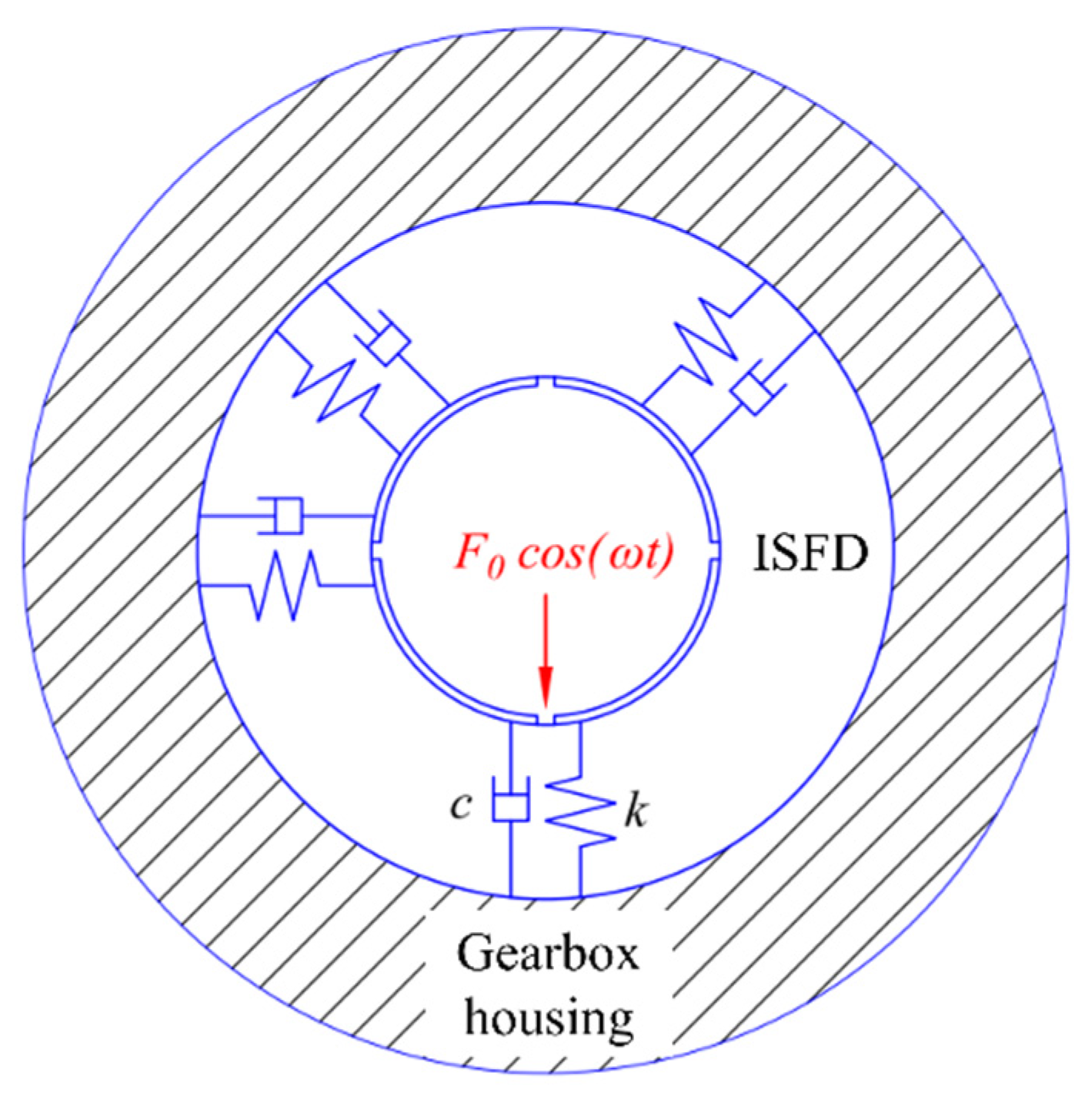
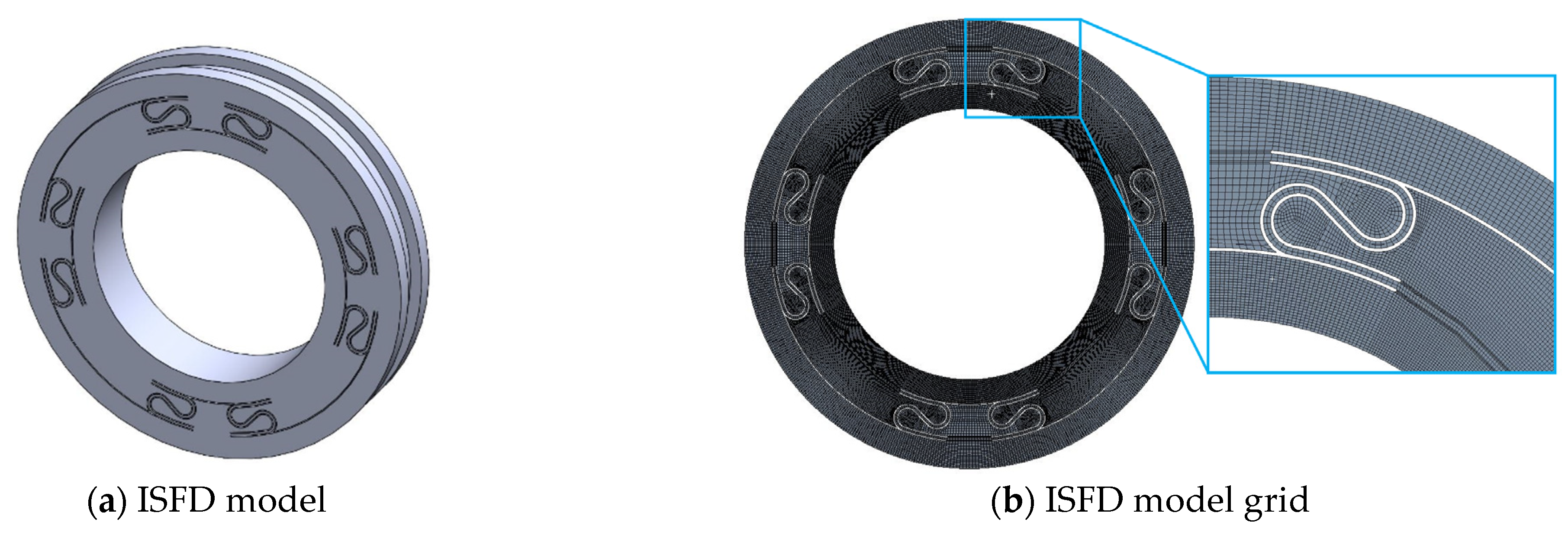
2.1. Vibration Isolation Modeling of the ISFD
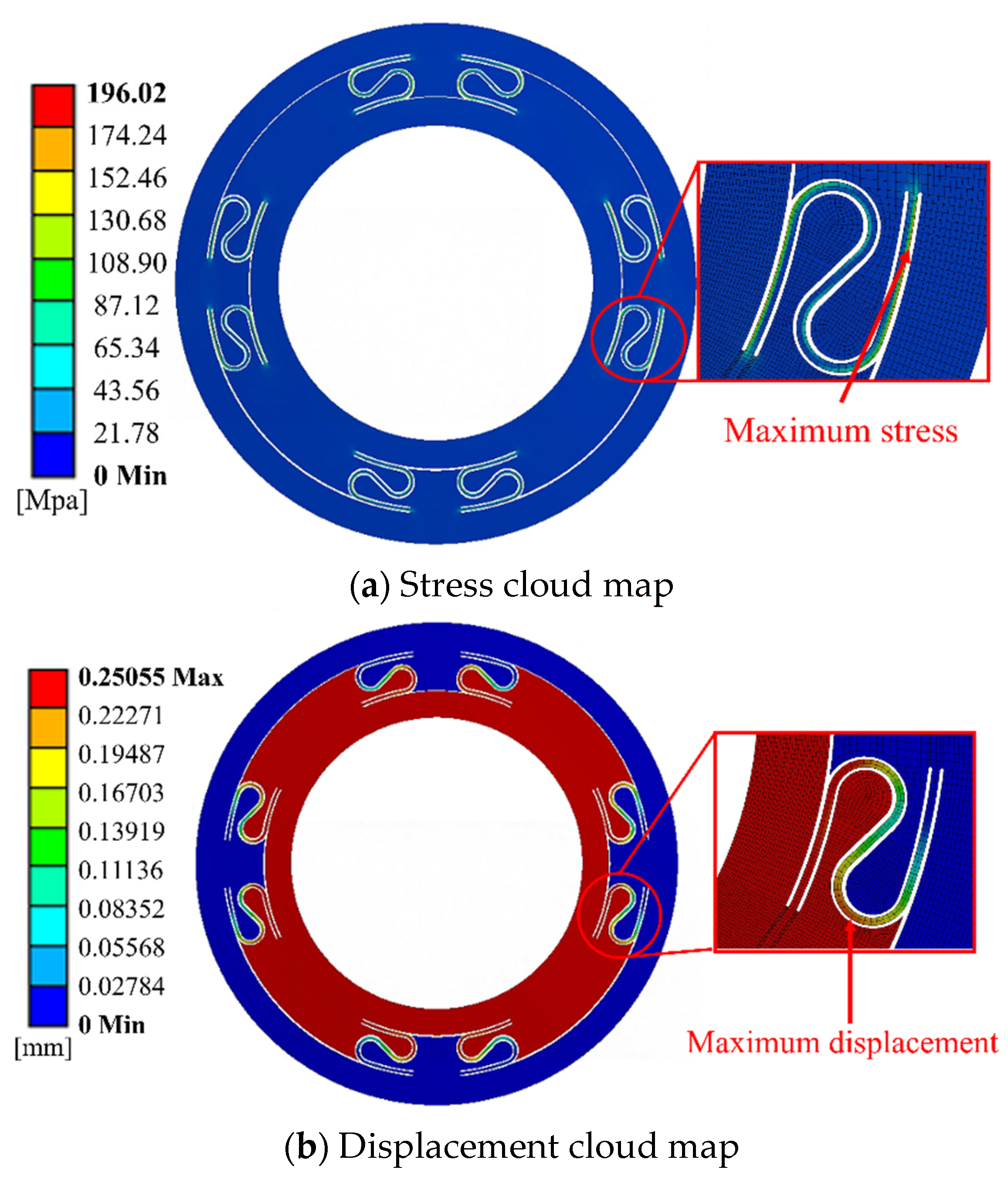
2.2. Statics Analysis of ISFD
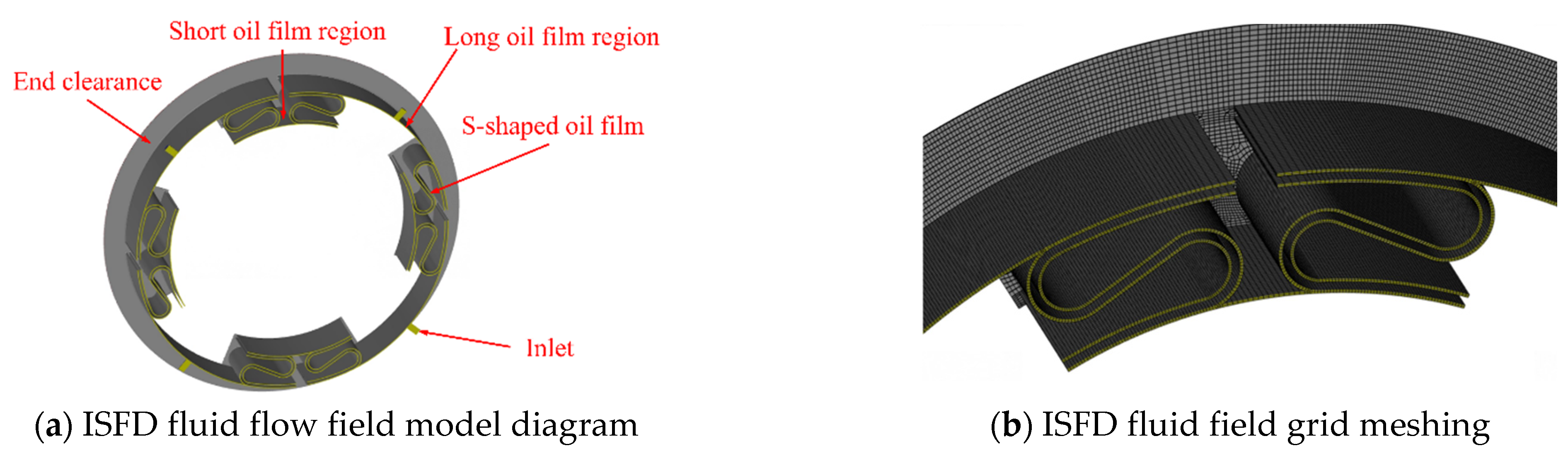
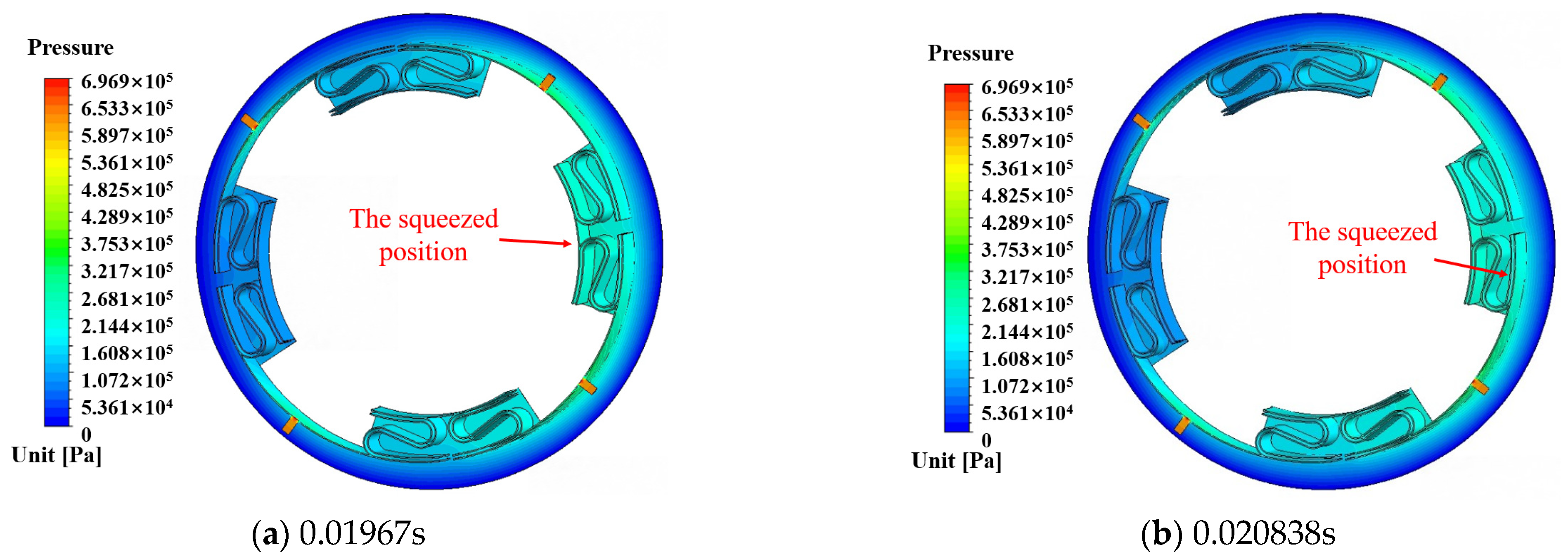
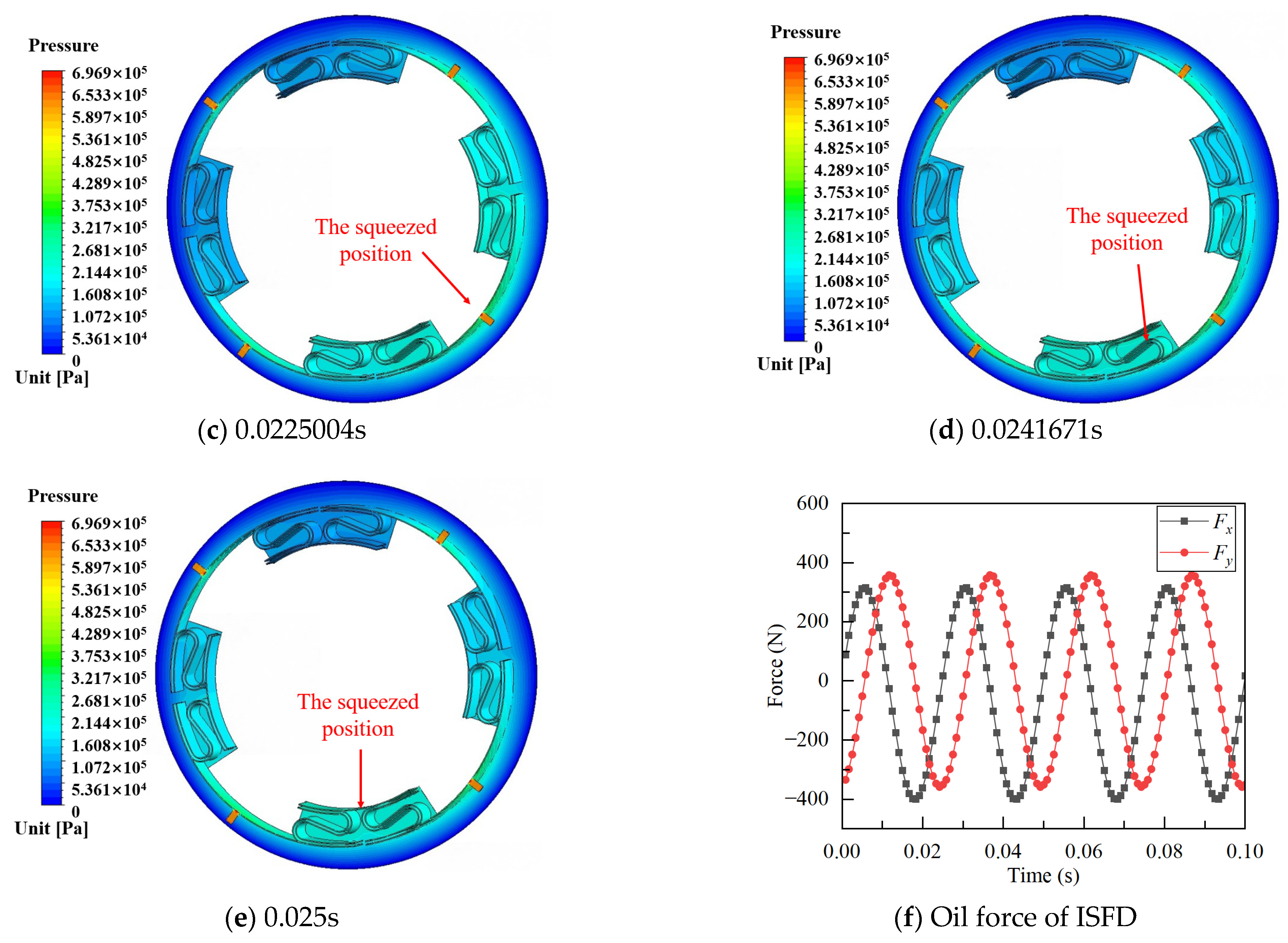
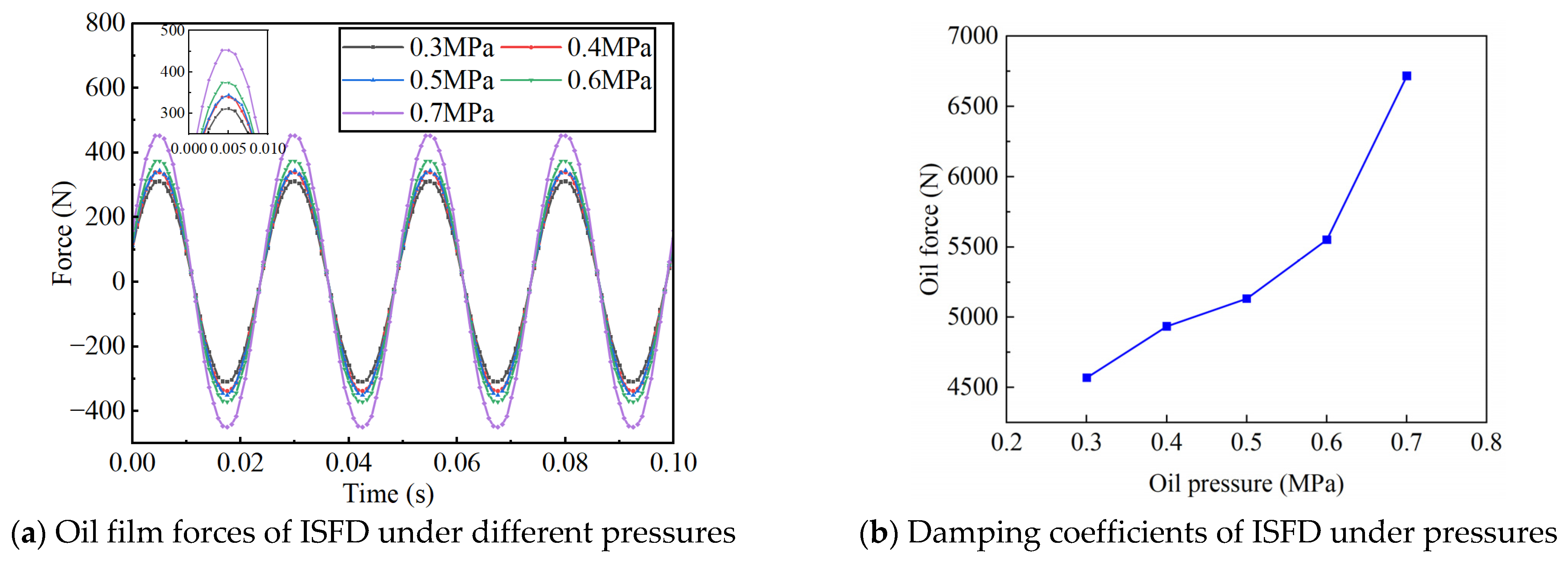
2.3. CFD Analysis of the ISFD
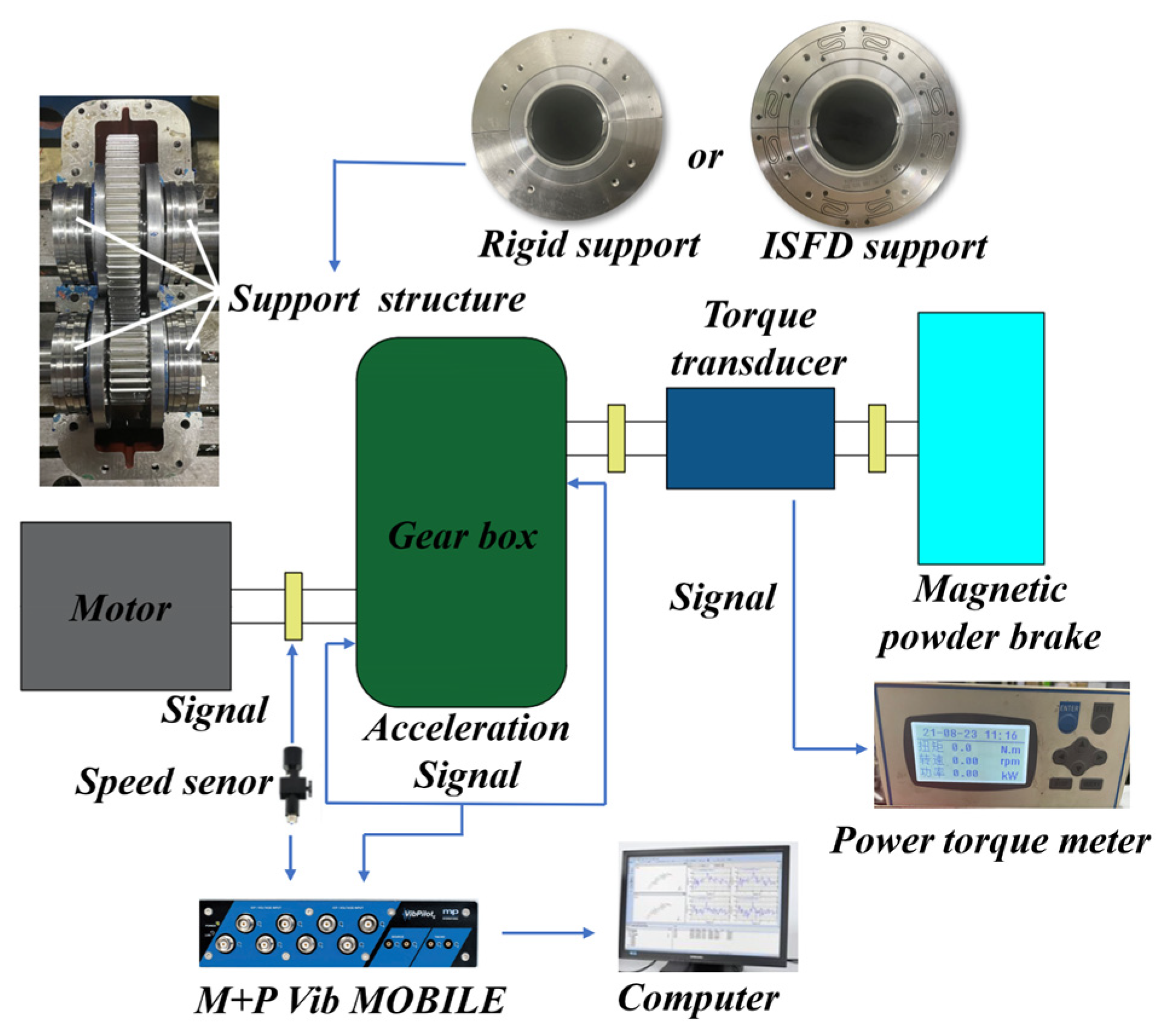
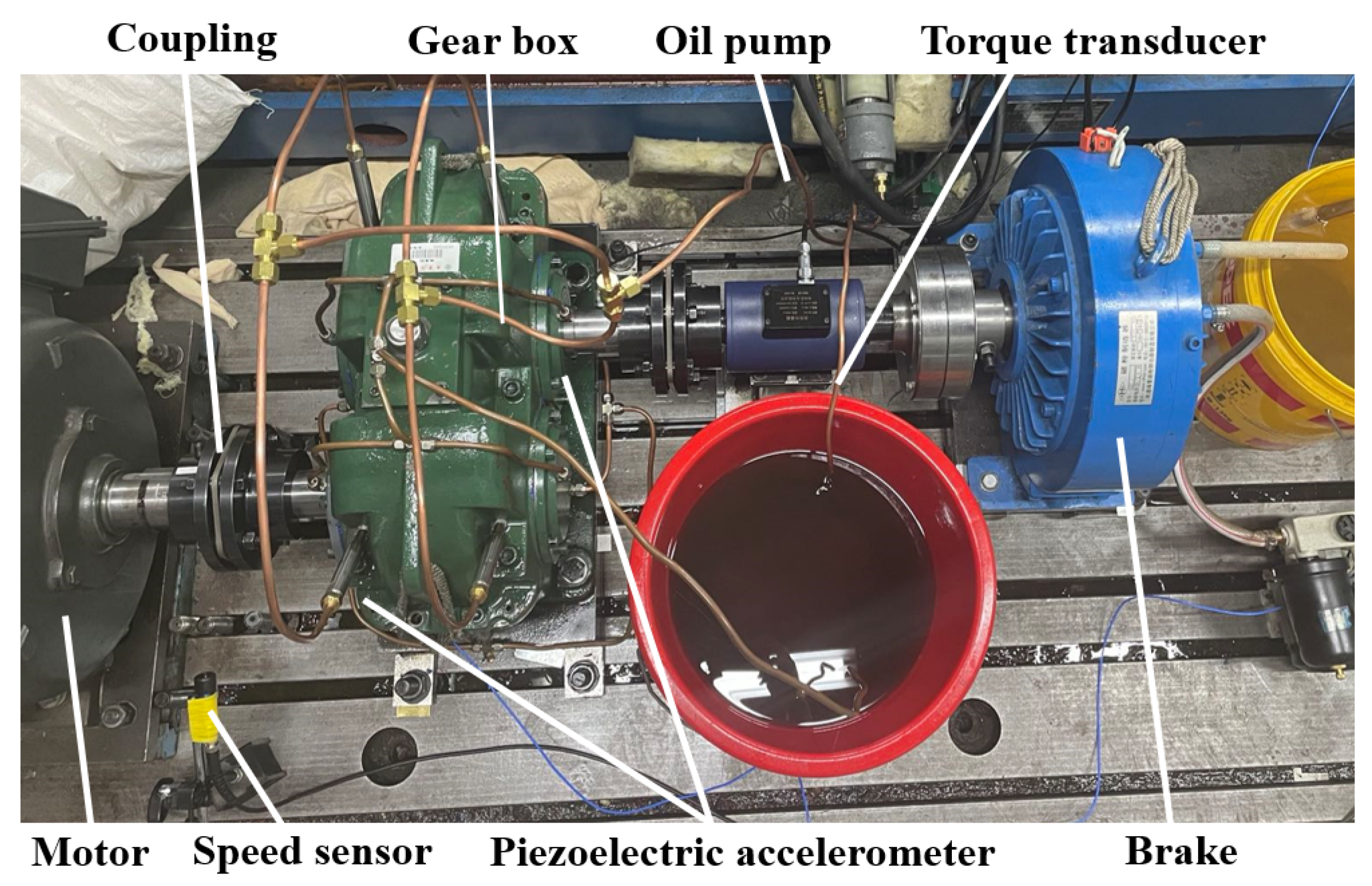
3. Experimental Setup
4. Results and Discussion
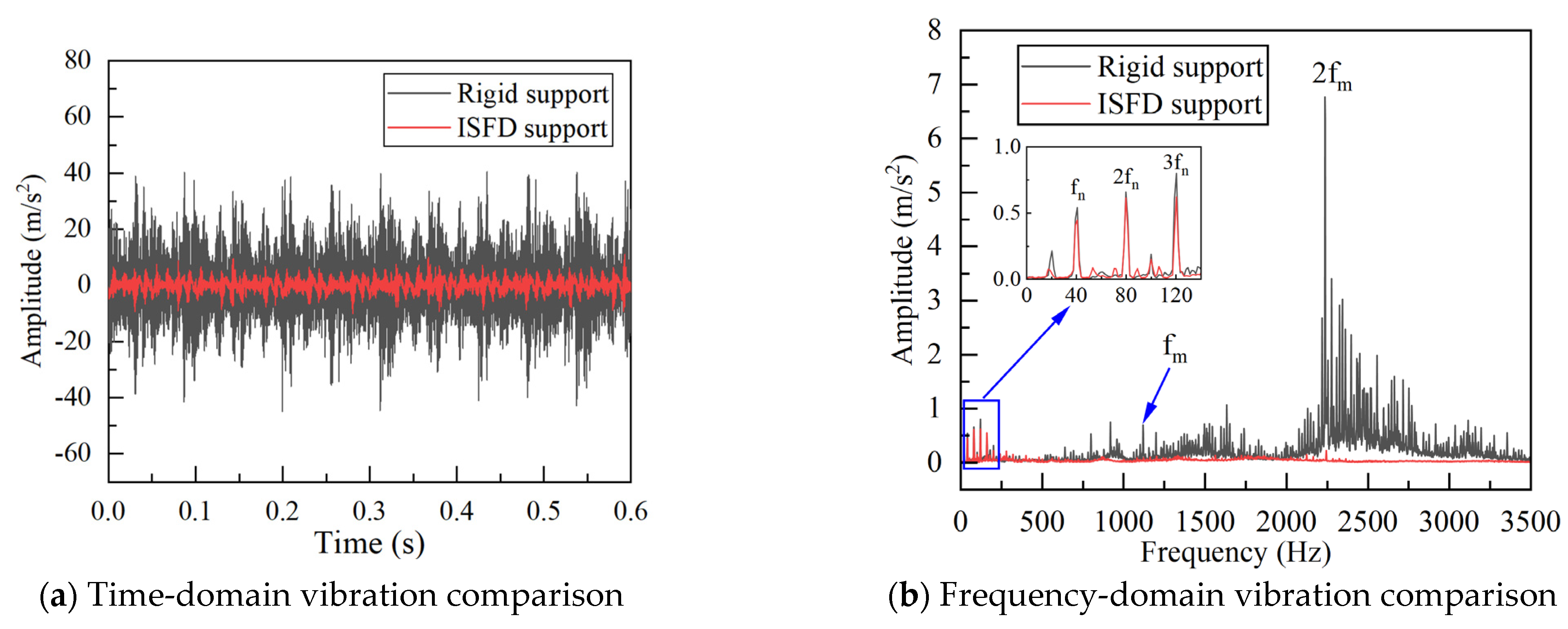
4.1. Experimental Analysis of Vibration Reduction in the Gear Transmission System Using the ISFD
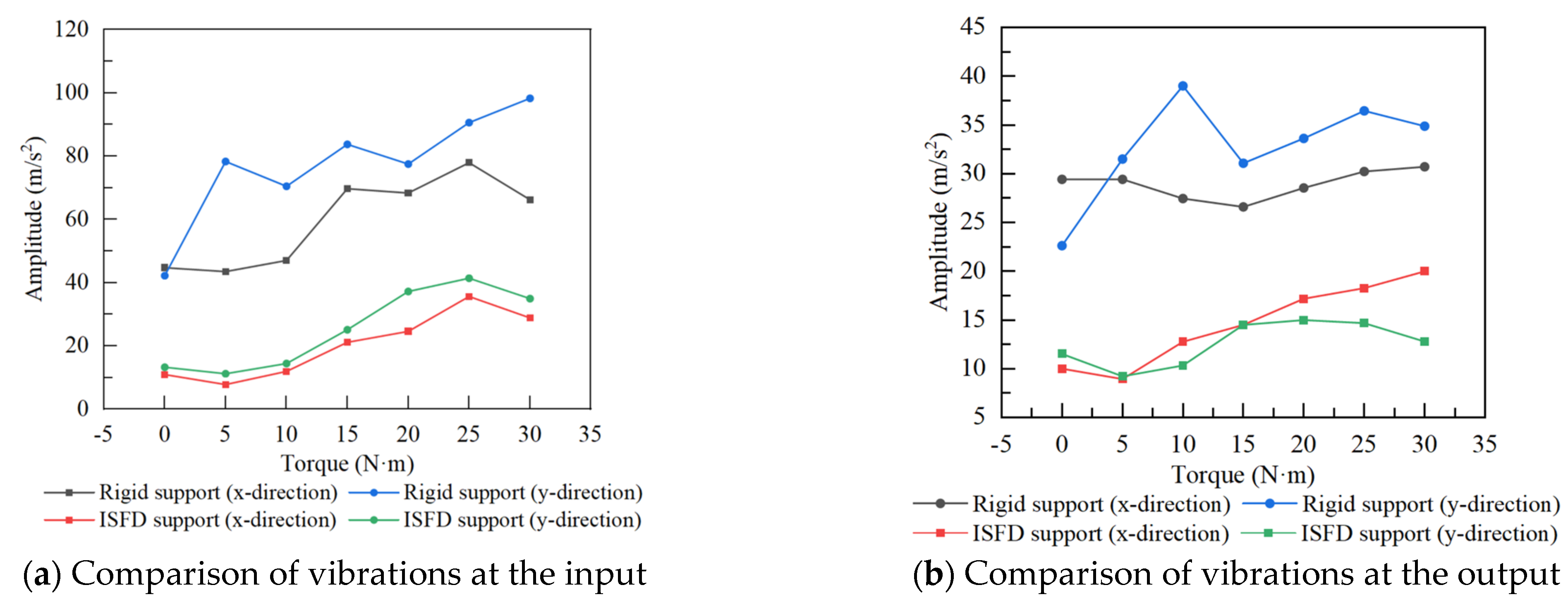
4.2. The Effect of Different Torque Loads on the Vibration Control of the ISFD
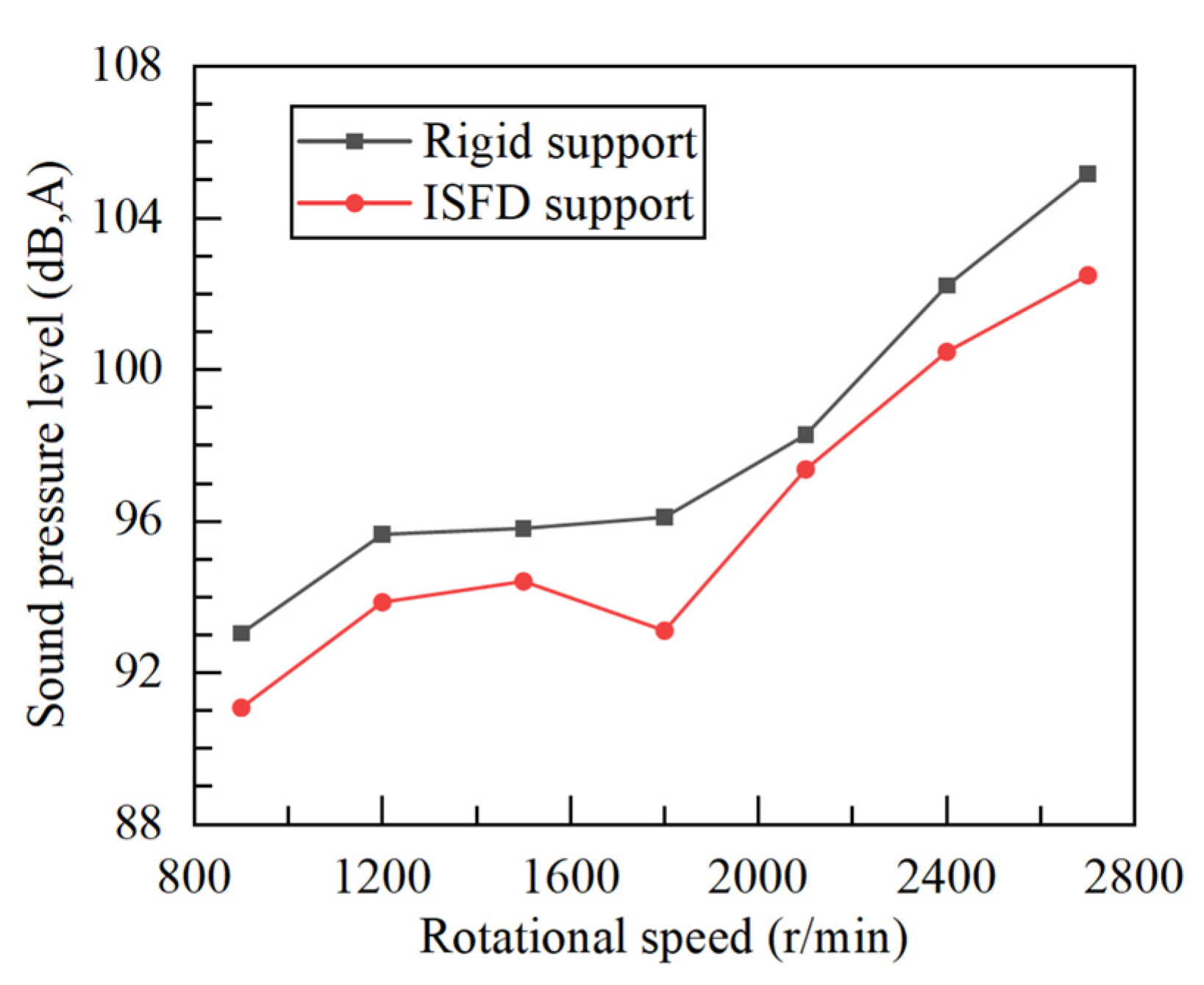
4.3. Experimental Study of the ISFD’s Reduction in Gear Transmission System Noise
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Geng, Z.; Li, J.; Xiao, K.; Wang, J. Analysis on the vibration reduction for a new rigid-flexible gear transmission system. J. Vib. Control 2022, 28, 2212–2225. [Google Scholar] [CrossRef]
- Xiao, W.Q.; Xu, Z.H.; Wang, S.; Yu, S.W.; Qin, K.; Hu, D.K. Research on vibration reduction characteristics of continuum and noncontinuum system on coupling for high-power gear transmission based on particle damping materials. Shock Vib. 2021, 2021, 8845526. [Google Scholar] [CrossRef]
- Wang, S.; Han, F.; Sun, B.; Li, H. Squeeze-film air damping of a five-axis electrostatic bearing for rotary micromotors. Sensors 2017, 17, 1119. [Google Scholar] [CrossRef]
- Chen, W.; Chen, S.; Hu, Z.; Tang, J.Y.; Li, H.N. A novel dynamic model for the spiral bevel gear drive with elastic ring squeeze film dampers. Nonlinear Dyn. 2019, 98, 1081–1105. [Google Scholar] [CrossRef]
- Jian, B.L.; Chu, L.M.; Chang, Y.P.; Chang, J.; Cai, W. Nonlinear dynamic analysis of gear-rotor-bearing system equipped with HSFD under hydraulic actuator active control. J. Low Freq. Noise Vib. Act. Control 2019, 40, 146134841988621. [Google Scholar] [CrossRef]
- Dong, H.; He, L.; Jia, X. Using integral squeeze film damper to suppress vibration of gas turbine. J. Vib. Eng. Technol. 2023, 11, 3163–3170. [Google Scholar] [CrossRef]
- Chen, W.; Chen, S.; Tang, J.; Li, H. Stability and bifurcation analysis of a bevel gear system supported by finite-length squeeze film dampers. Nonlinear Dyn. 2020, 100, 3321–3345. [Google Scholar] [CrossRef]
- Chang, J.; Cai, W.; Yau, H.T. Non-linear dynamic analysis of hybrid squeeze-film damper-mounted gear-bearing system and hydraulic active control. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2010, 224, 249–259. [Google Scholar]
- Chang, J.; Cai, W. Gear dynamics analysis with turbulent journal bearings mounted hybrid squeeze film damper—Chaos and active control analysis. ASME J. Comput. Nonlinear Dyn. 2015, 10, 011011. [Google Scholar] [CrossRef]
- Zapoměl, J.; Ferfecki, P.; Molčan, M. Optimization of control parameters of magnetorheological squeeze film dampers to minimize the vibration amplitude of rotors passing the critical speed. Appl. Sci. 2023, 13, 6905. [Google Scholar] [CrossRef]
- Andrés, L.S.; Jeung, S. Experimental performance of an open ends, centrally grooved, squeeze film damper operating with large amplitude orbital motions. ASME. J. Eng. Gas. Turbines Power 2015, 137, 032508. [Google Scholar] [CrossRef]
- Adiletta, G.; Pietra, L.D. The squeeze film damper over four decades of investigations. Part II: Rotordynamic analyses with rigid and flexible rotor. Shock Vib. Dig. 2002, 34, 97–126. [Google Scholar]
- Pietra, L.D.; Adiletta, G. The squeeze film damper over four decades of investigations. Part I: Characteristics and operating features. Shock Vib. Dig. 2002, 34, 3–26. [Google Scholar]
- Wang, W.; He, L.; Sun, Y.; Wang, Z.; Zhang, H.; Bao, Q. Research on new integral squeeze film damper vibration control of micro turbojet engine at high speed. J. Vib. Eng. Technol. 2023, 40, s451–s462. [Google Scholar] [CrossRef]
- Ferfecki, P.; Zapoměl, J.; Gebauer, M.; Polerich, V.; Křenek, J. A computational fluid dynamics investigation of the segmented integral squeeze film damper. MATEC Web Conf. 2019, 254, 8005. [Google Scholar] [CrossRef]
- Lu, X.L.; Andres, L.S.; Koo, B.; Tran, S. On the effect of the gap of end seals on force coefficients of a test integral squeeze film damper: Experiments and predictions. J. Eng. Gas. Turb. Power 2020, 143, 011014. [Google Scholar] [CrossRef]
- Yan, W.; He, L.; Zhu, G.; Wang, S.; Deng, Z. Experimental study on vibration suppression in a rotor system under base excitation using an integral squeeze film damper. High Technol. Lett. 2020, 26, 11. [Google Scholar]
- Yan, W.; He, L.; Zhu, G.; Jia, X. Effect of G-type integral squeeze film damper on the dynamic characteristics in rotor system. Int. J. Turbo Jet. Eng. 2023, 40, s195–s205. [Google Scholar] [CrossRef]
- Zhang, Y.; He, L.; Yang, J.; Zhu, G.; Jia, X.; Yan, W. Multi-objective optimization design of a novel integral squeeze film bearing damper. Machines 2021, 9, 206. [Google Scholar] [CrossRef]
- Ertas, B.; Cerny, V.; Kim, J.; Polreich, V. Stabilizing a 46 MW multistage utility steam turbine using integral squeeze film bearing support dampers. J. Eng. Gas. Turb. Power 2015, 137, 052506. [Google Scholar] [CrossRef]
- Ertas, B.; Delgado, A.; Moore, J. Dynamic characterization of an integral squeeze film bearing support damper for a supercritical CO2 expander. J. Eng. Gas. Turb Power 2018, 140, 052501. [Google Scholar] [CrossRef]
- Ertas, B. Compliant hybrid gas bearing using integral hermetically sealed squeeze film dampers. J. Eng. Gas. Turb. Power 2019, 141, 101020. [Google Scholar] [CrossRef]
- Yan, W.; Lu, J.; Pan, J.; Liu, J.; Fu, Y.C.; Ye, D. Research on dynamic characteristic coefficients of integral squeeze film damper. Machines 2024, 12, 274. [Google Scholar] [CrossRef]


| Parameters | Value |
|---|---|
| Inner diameter (mm) | 80 |
| Distribution angle of elastomers (°) | 40 |
| Radial position of elastomers (mm) | 46 |
| Radial thickness of elastomers (mm) | 10 |
| Axial length (mm) | 22 |
| Outside diameter (mm) | 125 |
| Parameters | Value |
|---|---|
| Inlet oil pressure (MPa) | 0.3 |
| Outlet oil pressure (MPa) | 0 |
| Whirling frequency (Hz) | 40 |
| Whirling amplitude (μm) | 20 |
| Whirling velocity (rad/s) | 251.32 |
| Oil film gap (mm) | 0.5 |
| End-face oil gap (mm) | 0.1 |
| Lubricating oil viscosity (kg/(m·s)) | 0.038 |
| Lubricating oil density (kg/m3) | 871 |
| Parameters | cxx | cyy |
|---|---|---|
| Value | 4.57 × 104 N·m/s | 4.54 × 104 N·m/s |
| Parameters | Value |
|---|---|
| Number of driving gear teeth | 28 |
| Number of driven gear teeth | 63 |
| Driving gear thickness (mm) | 35 |
| Driven gear thickness (mm) | 44 |
| Gear module (mm) | 3.5 |
| Transmission ratio | 2.25 |
| Frequency (Hz) | Rigid Support (m/s2) | ISFD Support (m/s2) | Vibration Reduction (%) |
|---|---|---|---|
| 1120 | 0.69 | 0.09 | 86.96 |
| 2240 | 6.40 | 0.14 | 97.81 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, G.; He, L.; Jia, X.; Tan, Z.; Qin, Q. Experimental Study on Vibration and Noise Reduction of Gear Transmission System Based on ISFD. Machines 2024, 12, 531. https://doi.org/10.3390/machines12080531
Zhu G, He L, Jia X, Tan Z, Qin Q. Experimental Study on Vibration and Noise Reduction of Gear Transmission System Based on ISFD. Machines. 2024; 12(8):531. https://doi.org/10.3390/machines12080531
Chicago/Turabian StyleZhu, Gang, Lidong He, Xingyun Jia, Zhifu Tan, and Qingwang Qin. 2024. "Experimental Study on Vibration and Noise Reduction of Gear Transmission System Based on ISFD" Machines 12, no. 8: 531. https://doi.org/10.3390/machines12080531
APA StyleZhu, G., He, L., Jia, X., Tan, Z., & Qin, Q. (2024). Experimental Study on Vibration and Noise Reduction of Gear Transmission System Based on ISFD. Machines, 12(8), 531. https://doi.org/10.3390/machines12080531





