Consensus Tracking Control of Multiple Unmanned Aerial Vehicles Subject to Distinct Unknown Delays
Abstract
1. Introduction
2. System Dynamic Model
3. Control Strategy
3.1. Altitude and Attitude Control
3.2. Translation Subsystem
3.3. Translation Control
- Leader agent control
- Follower agent control
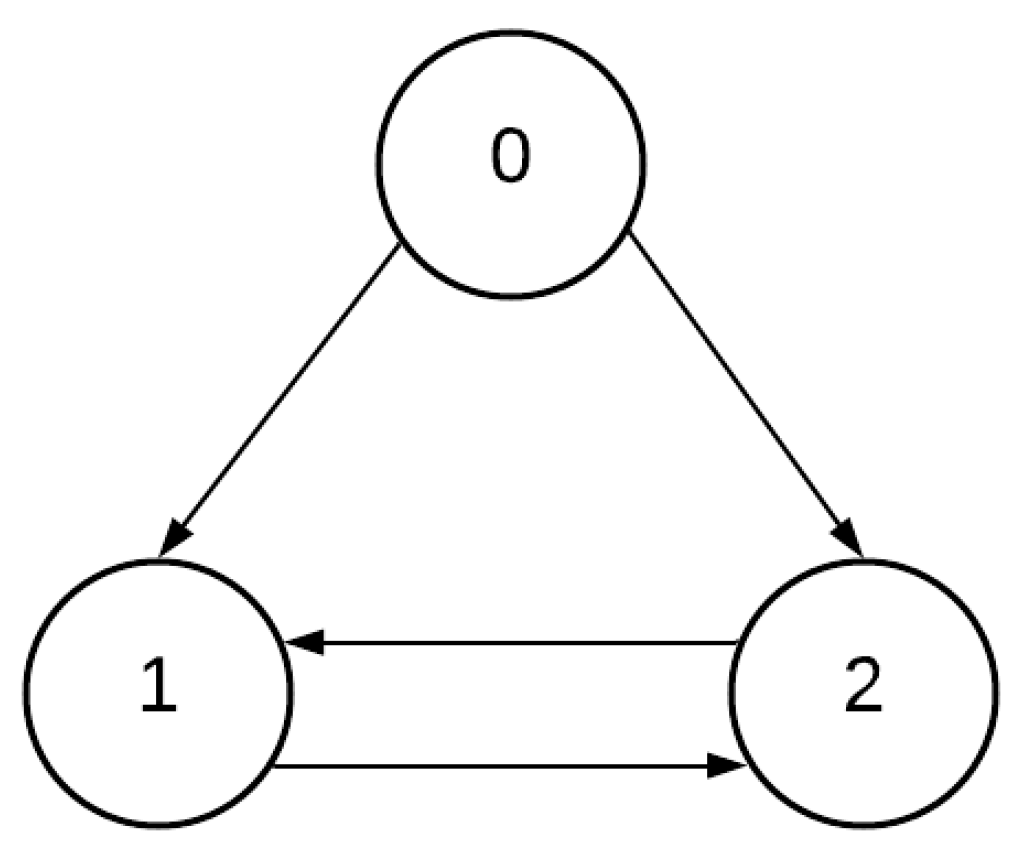
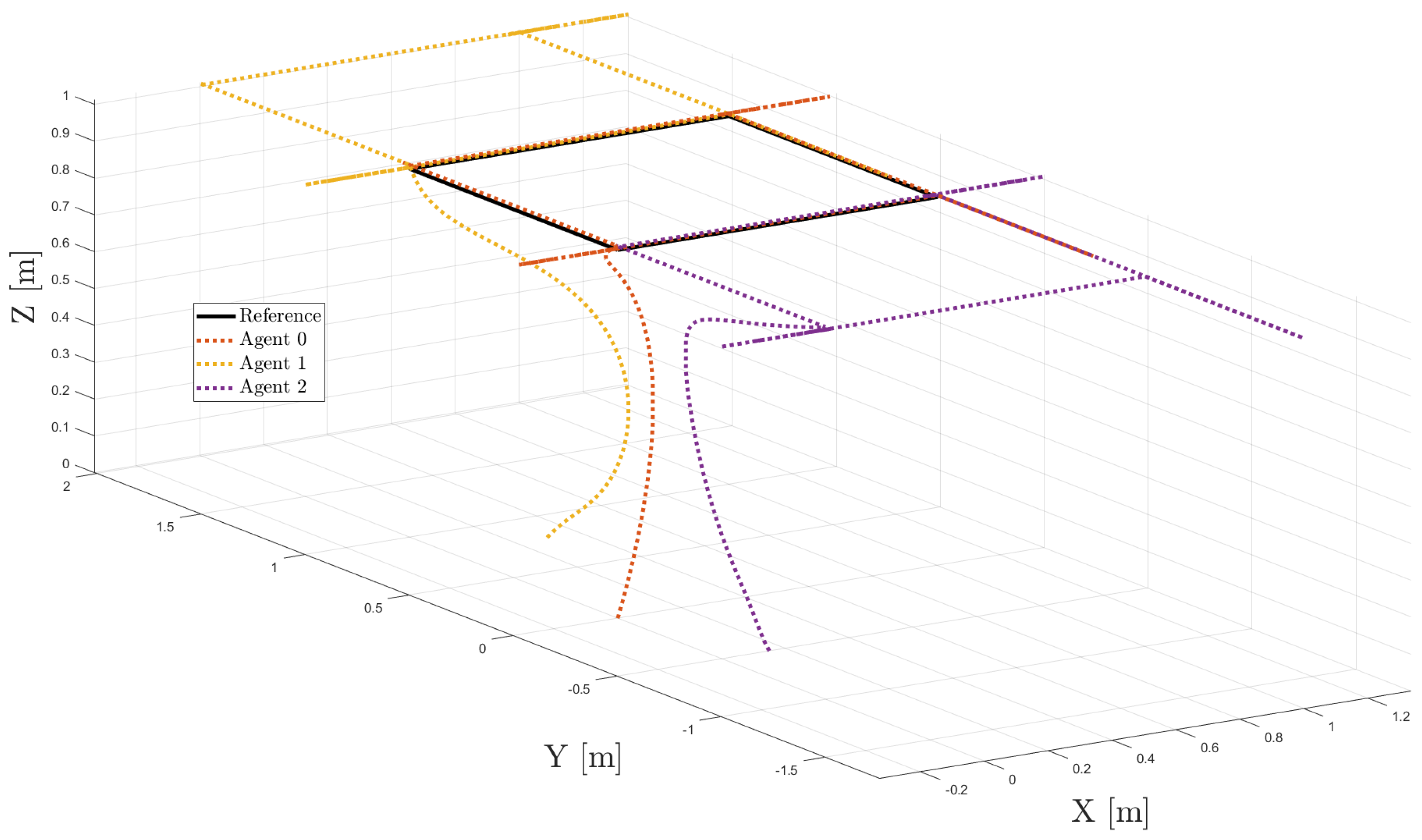
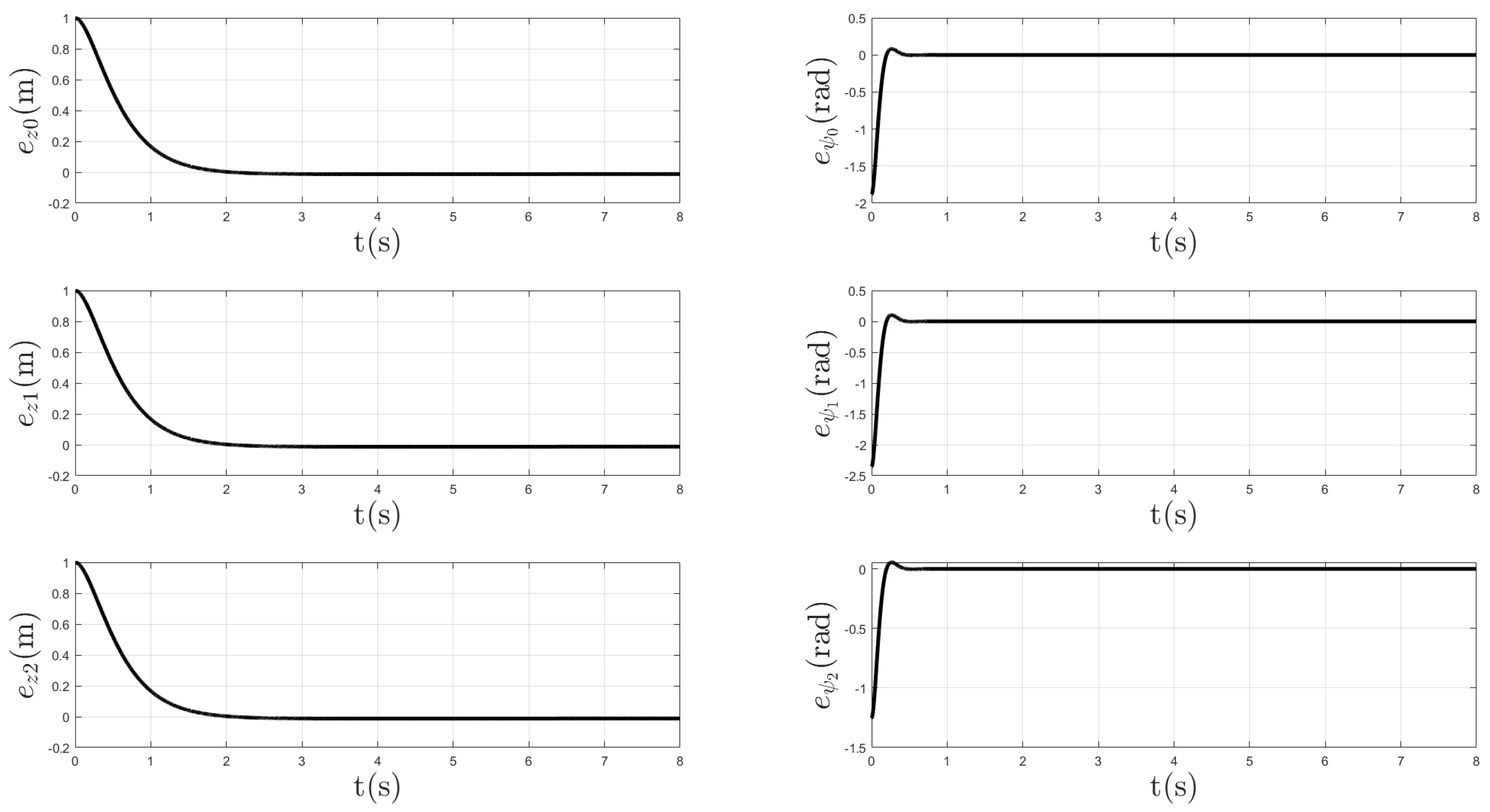
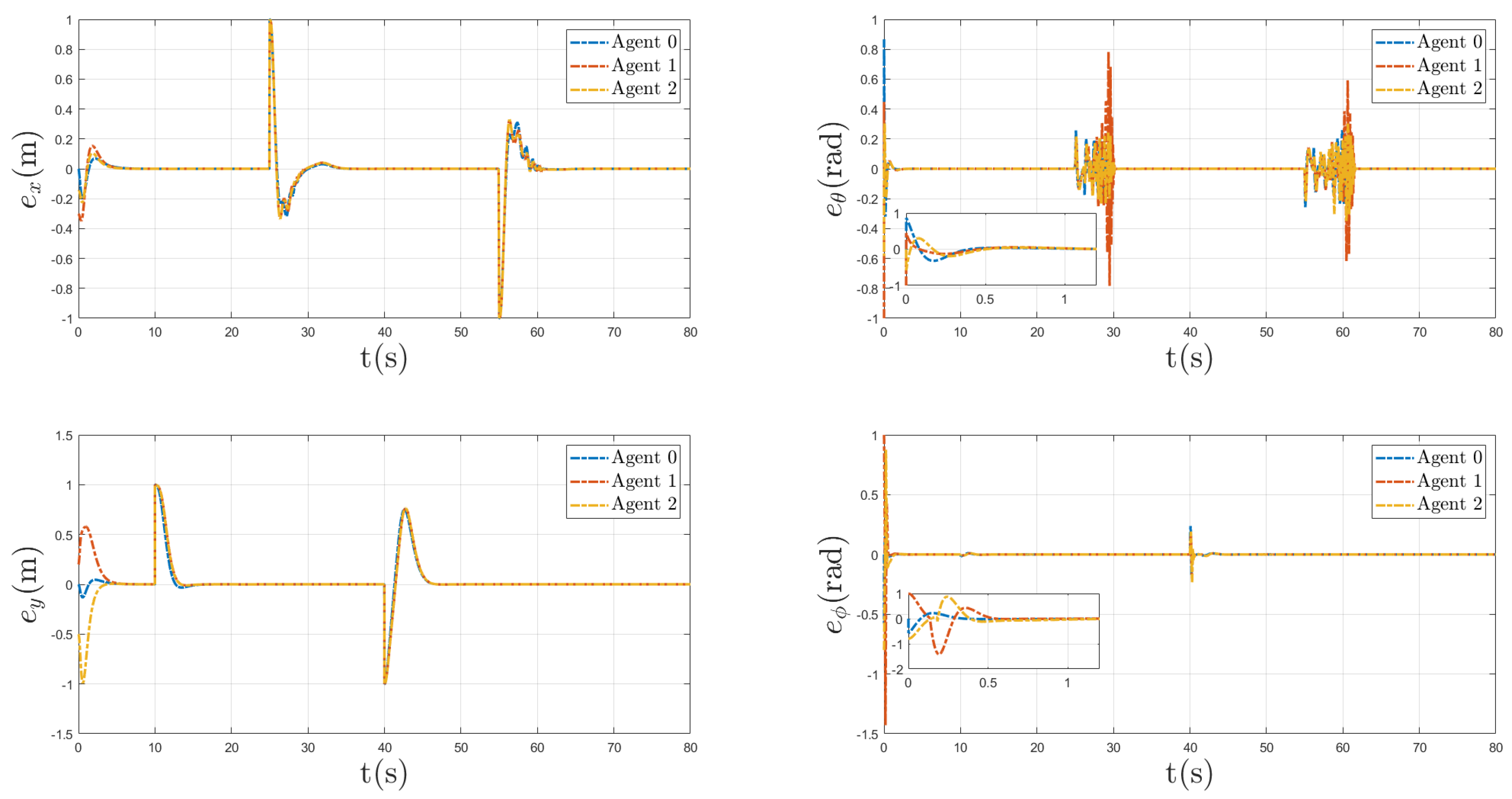
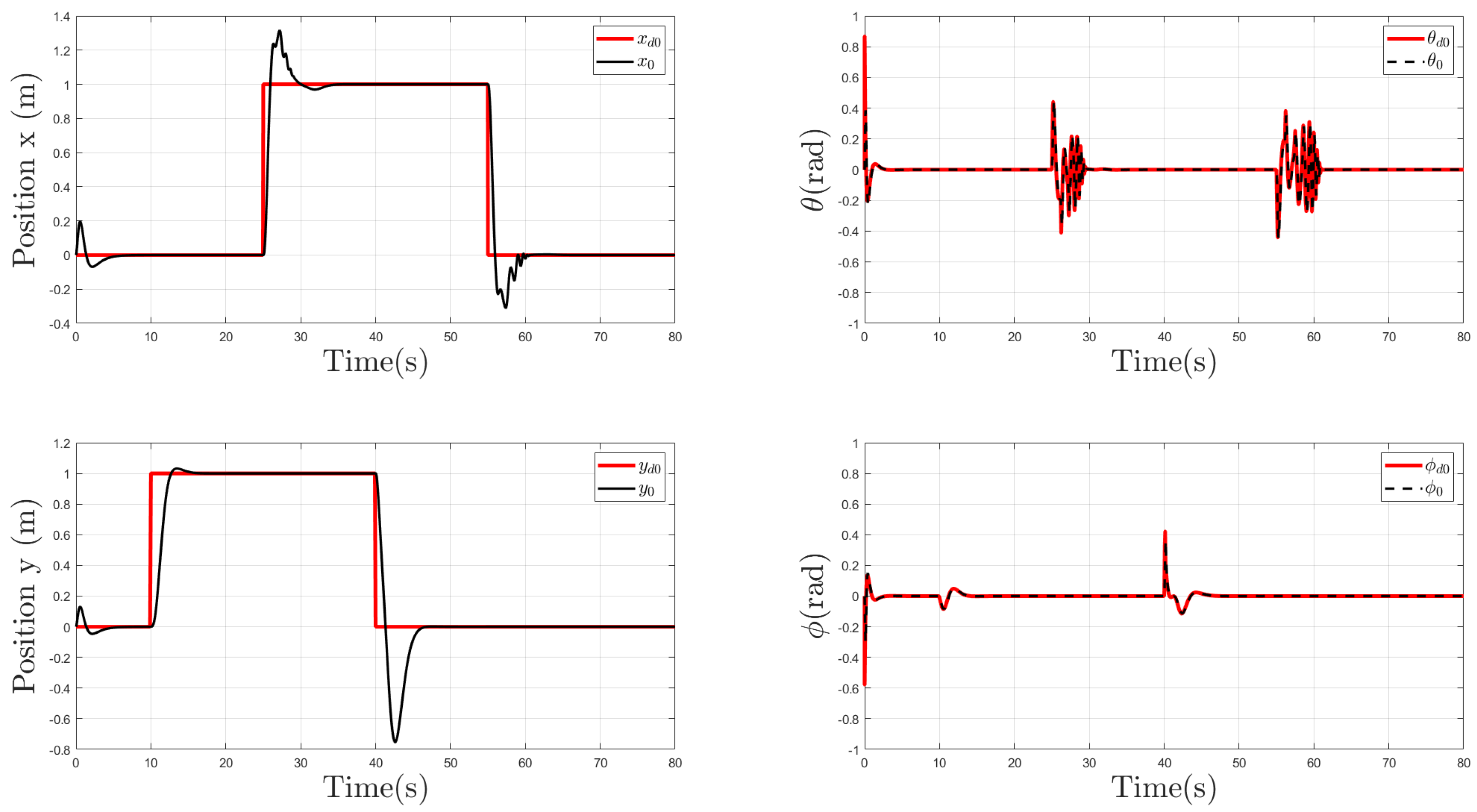
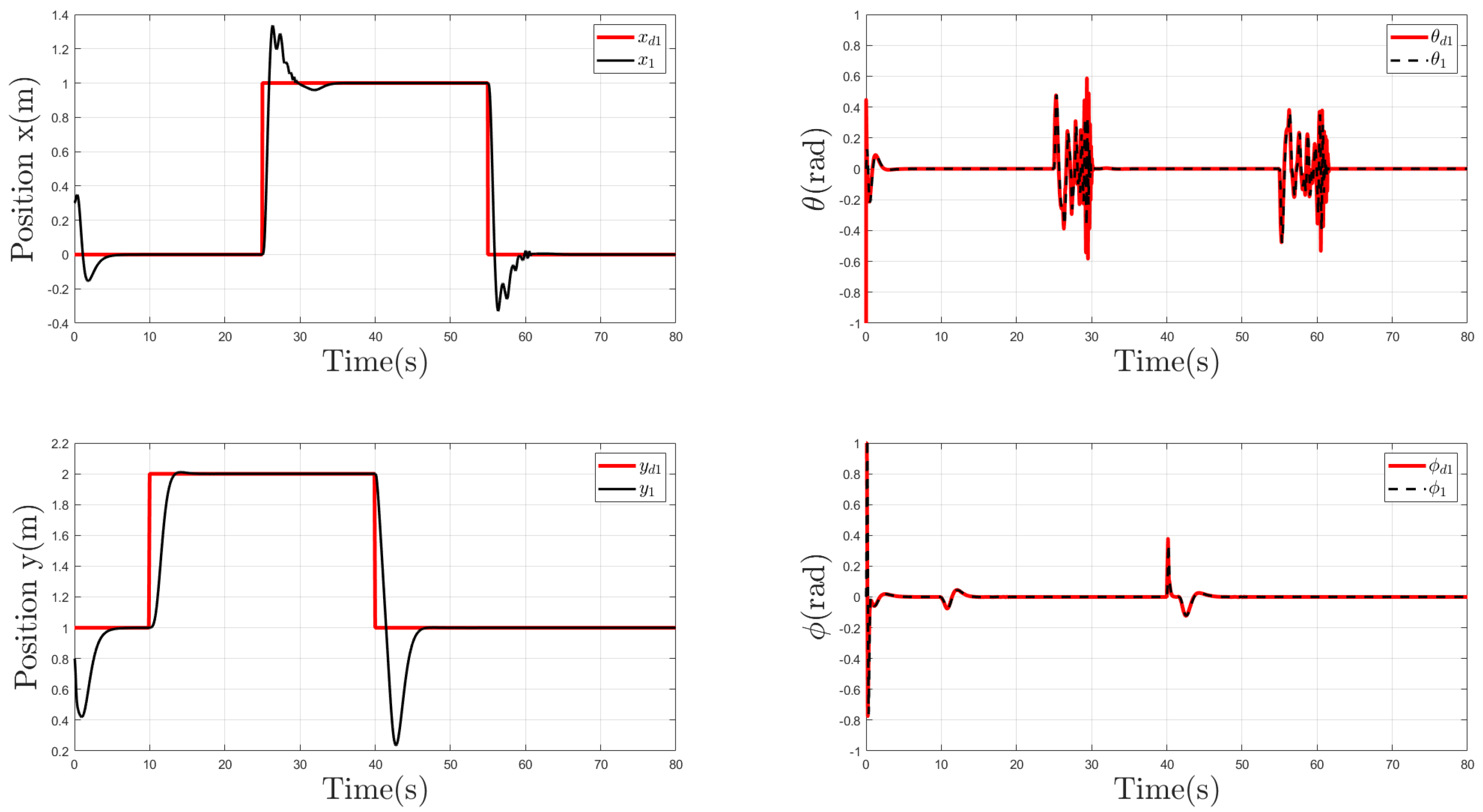
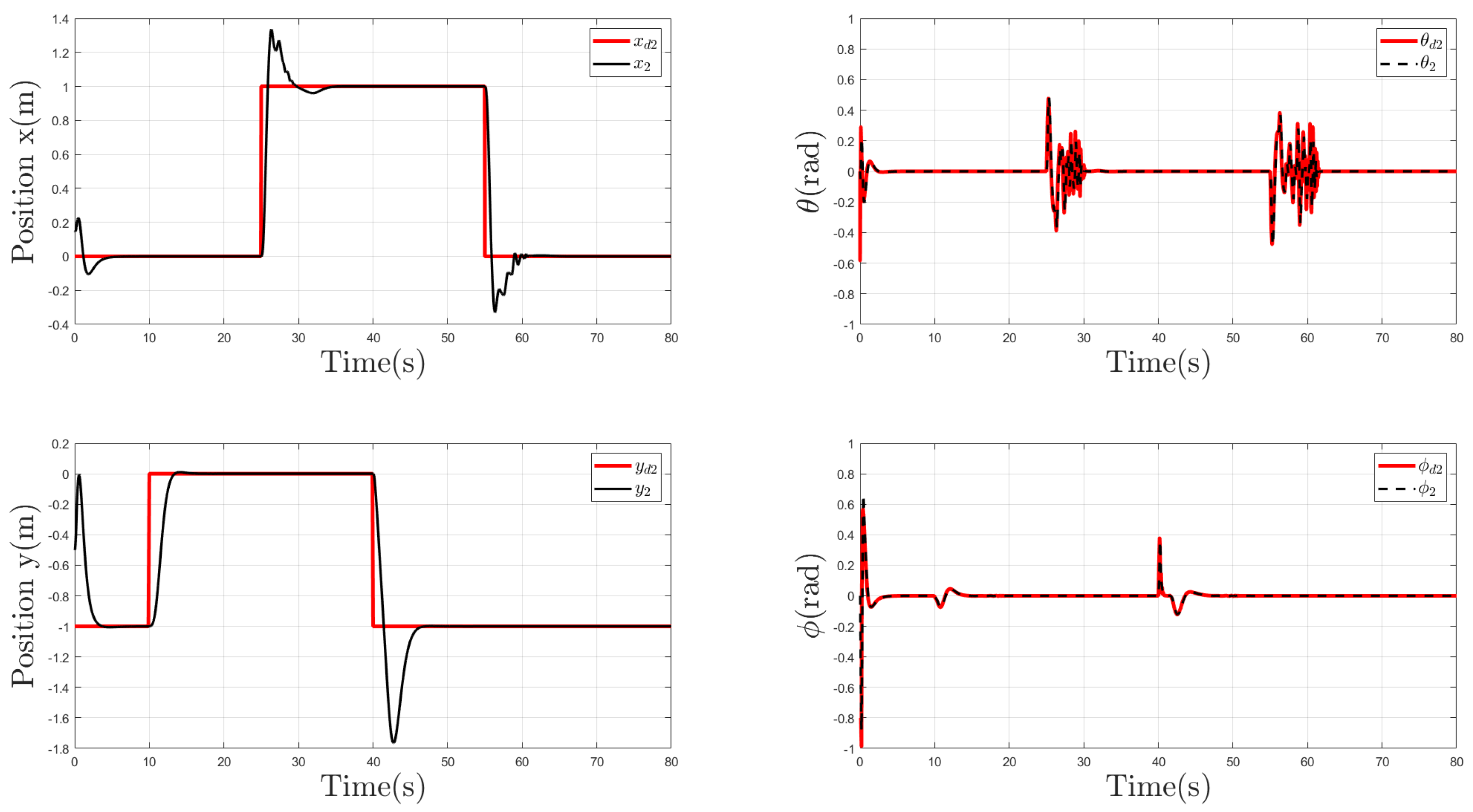
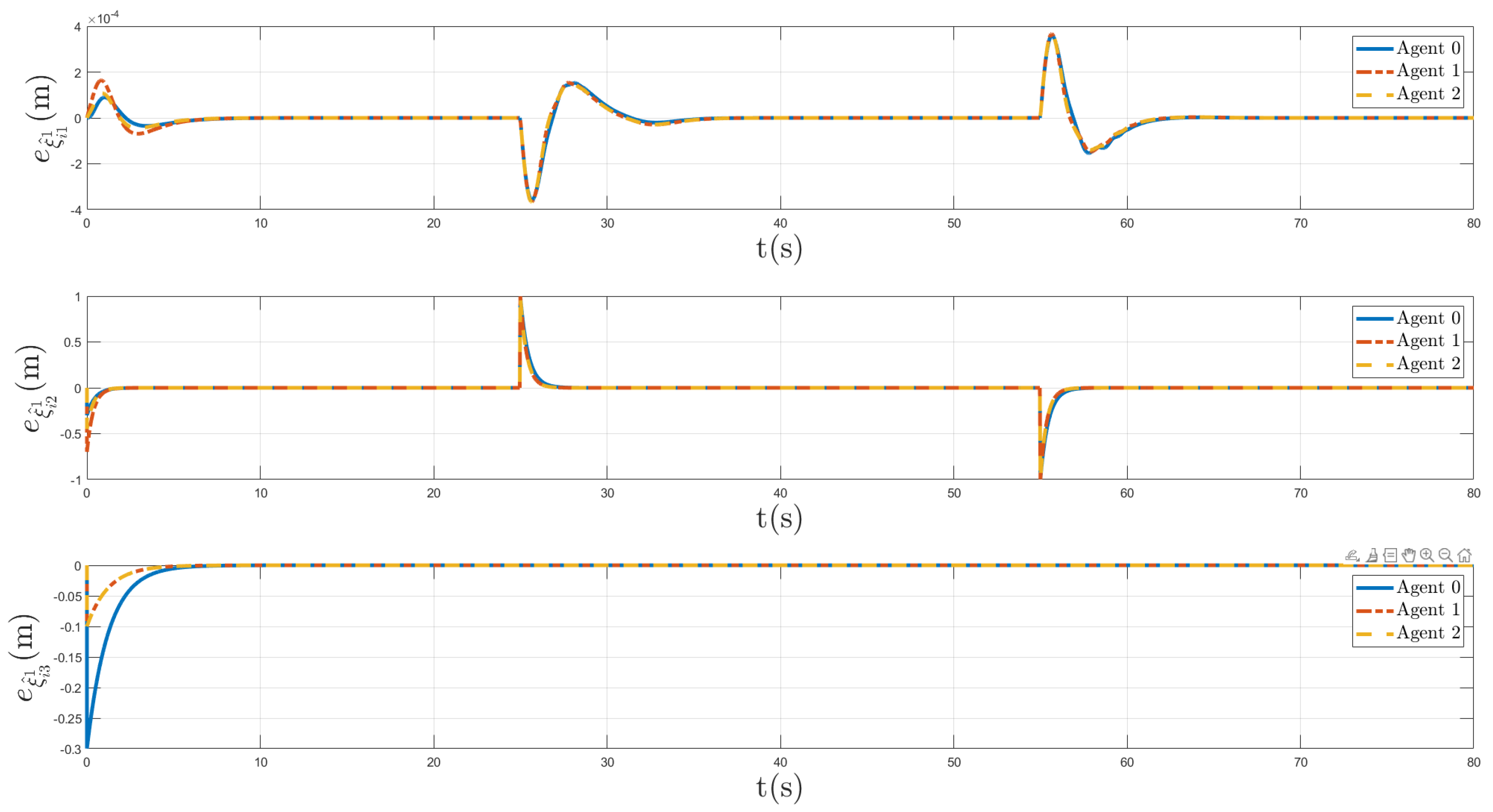
4. Simulation Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hou, Z.; Wang, W.; Zhang, G.; Han, C. A survey on the formation control of multiple quadrotors. In Proceedings of the 2017 14th International Conference on Ubiquitous Robots and Ambient Intelligence (URAI), Jeju, Republic of Korea, 28 June–1 July 2017; pp. 219–225. [Google Scholar]
- Mishra, B.; Garg, D.; Narang, P.; Mishra, V. Drone-surveillance for search and rescue in natural disaster. Comput. Commun. 2020, 156, 1–10. [Google Scholar] [CrossRef]
- Farrera, B.; López-Estrada, F.R.; Chadli, M.; Valencia-Palomo, G.; Gómez-Peñate, S. Distributed fault estimation of multi–agent systems using a proportional–integral observer: A leader–following application. Int. J. Appl. Math. Comput. Sci. 2020, 30, 551–560. [Google Scholar]
- Trejo, J.A.V.; Ponsart, J.C.; Adam-Medina, M.; Valencia-Palomo, G.; Theilliol, D. Distributed Observer-based Leader-following Consensus Control for LPV Multi-agent Systems: Application to multiple VTOL-UAVs Formation Control. In Proceedings of the 2023 International Conference on Unmanned Aircraft Systems (ICUAS), Warsaw, Poland, 6–9 June 2023; IEEE: Piscataway, NJ, USA, 2023; pp. 1316–1323. [Google Scholar]
- Hernández-González, O.; Targui, B.; Valencia-Palomo, G.; Guerrero-Sánchez, M. Robust cascade observer for a disturbance unmanned aerial vehicle carrying a load under multiple time-varying delays and uncertainties. Int. J. Syst. Sci. 2024, 55, 1056–1072. [Google Scholar] [CrossRef]
- Chen, Y.Q.; Wang, Z. Formation control: A review and a new consideration. In Proceedings of the 2005 IEEE/RSJ International Conference on Intelligent Robots and Systems, Edmonton, AB, Canada, 2–6 August 2005; pp. 3181–3186. [Google Scholar]
- Dong, X.; Yu, B.; Shi, Z.; Zhong, Y. Time-Varying Formation Control for Unmanned Aerial Vehicles: Theories and Applications. IEEE Trans. Control Syst. Technol. 2015, 23, 340–348. [Google Scholar] [CrossRef]
- Chen, Q.; Wang, Y.; Jin, Y.; Wang, T.; Nie, X.; Yan, T. A Survey of An Intelligent Multi-Agent Formation Control. Appl. Sci. 2023, 13, 5934. [Google Scholar] [CrossRef]
- Li, Z.; Duan, Z.; Huang, L. Leader-follower consensus of multi-agent systems. In Proceedings of the 2009 American Control Conference, St. Louis, MO, USA, 10–12 June 2009; pp. 3256–3261. [Google Scholar]
- Gao, Y.; Wang, L. Sampled-Data Based Consensus of Continuous-Time Multi-Agent Systems with Time-Varying Topology. IEEE Trans. Autom. Control 2011, 56, 1226–1231. [Google Scholar] [CrossRef]
- Liu, W.; Huang, J. Leader-Following Consensus for Linear Multiagent Systems via Asynchronous Sampled-Data Control. IEEE Trans. Autom. Control 2020, 65, 3215–3222. [Google Scholar] [CrossRef]
- Ning, B.; Han, Q.L.; Zuo, Z.; Ding, L.; Lu, Q.; Ge, X. Fixed-Time and Prescribed-Time Consensus Control of Multiagent Systems and Its Applications: A Survey of Recent Trends and Methodologies. IEEE Trans. Ind. Inform. 2023, 19, 1121–1135. [Google Scholar] [CrossRef]
- Ren, W. On Consensus Algorithms for Double-Integrator Dynamics. IEEE Trans. Autom. Control 2008, 53, 1503–1509. [Google Scholar] [CrossRef]
- Li, Z.; Ren, W.; Liu, X.; Fu, M. Consensus of Multi-Agent Systems with General Linear and Lipschitz Nonlinear Dynamics Using Distributed Adaptive Protocols. arXiv 2011, arXiv:1109.3799. [Google Scholar] [CrossRef]
- Cheng, L.; Hou, Z.G.; Tan, M.; Wang, X. Necessary and Sufficient Conditions for Consensus of Double-Integrator Multi-Agent Systems with Measurement Noises. IEEE Trans. Autom. Control 2011, 56, 1958–1963. [Google Scholar] [CrossRef]
- Altafini, C. Consensus Problems on Networks with Antagonistic Interactions. IEEE Trans. Autom. Control 2013, 58, 935–946. [Google Scholar] [CrossRef]
- Li, Z.; Wen, G.; Duan, Z.; Ren, W. Designing Fully Distributed Consensus Protocols for Linear Multi-Agent Systems with Directed Graphs. IEEE Trans. Autom. Control 2015, 60, 1152–1157. [Google Scholar] [CrossRef]
- Meng, D.; Jia, Y.; Du, J. Finite-Time Consensus for Multiagent Systems with Cooperative and Antagonistic Interactions. IEEE Trans. Neural Netw. Learn. Syst. 2016, 27, 762–770. [Google Scholar] [CrossRef] [PubMed]
- Meng, D.; Zou, Z. Signed-average consensus for networks of agents: A nonlinear fixed-time convergence protocol. Nonlinear Dyn. 2016, 85, 155–165. [Google Scholar] [CrossRef]
- Li, H.; Chen, G.; Liao, X.; Huang, T. Leader-Following Consensus of Discrete-Time Multiagent Systems with Encoding–Decoding. IEEE Trans. Circuits Syst. II Express Briefs 2016, 63, 401–405. [Google Scholar] [CrossRef]
- Li, Y.; Tang, K.; Yan, F. A Consensus Control Algorithm of High-order Multi-agent Systems with Time Delay. In Proceedings of the 2019 3rd International Symposium on Autonomous Systems (ISAS), Shanghai, China, 29–31 May 2019; pp. 502–507. [Google Scholar]
- Geng, H.; Wu, H.; Miao, J.; Hou, S.; Chen, Z. Consensus of Heterogeneous Multi-Agent Systems Under Directed Topology. IEEE Access 2022, 10, 5936–5943. [Google Scholar] [CrossRef]
- Xiao, F.; Chen, T.; Gao, H. Synchronous Hybrid Event- and Time-Driven Consensus in Multiagent Networks with Time Delays. IEEE Trans. Cybern. 2016, 46, 1165–1174. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, K.; Xie, W.C. Consensus of multi-agent systems via hybrid impulsive protocols with time-delay. Nonlinear Anal. Hybrid Syst. 2018, 30, 134–146. [Google Scholar] [CrossRef]
- Qi, T.; Lu, R.; Chen, J. Consensus of Continuous-Time Multiagent Systems via Delayed Output Feedback: Delay Versus Connectivity. IEEE Trans. Autom. Control 2021, 66, 1329–1336. [Google Scholar] [CrossRef]
- Cao, L.; Liu, G.P.; Zhang, D.W. A leader-follower consensus control of networked multi-agent systems under communication delays and switching topologies. In Proceedings of the 2022 41st Chinese Control Conference (CCC), Hefei, China, 25–27 July 2022; pp. 4378–4383. [Google Scholar]
- Tan, C.; Dong, X.; Li, Y.; Liu, G.P. Leader–following consensus problem of networked multi-agent systems under switching topologies and communication constraints. IET Control Theory Appl. 2020, 14, 3686–3696. [Google Scholar] [CrossRef]
- Jiang, W.; Charalambous, T. Distributed Consensus Tracking of Multi-Agent Systems with Time-varying Input/Output Delays and Mismatched Disturbances. IFAC-PapersOnLine 2021, 54, 787–794. [Google Scholar] [CrossRef]
- Cao, W.; Zhang, J.; Ren, W. Leader–follower consensus of linear multi-agent systems with unknown external disturbances. Syst. Control Lett. 2015, 82, 64–70. [Google Scholar] [CrossRef]
- Zhu, J.W.; Yang, G.H.; Zhang, W.A.; Yu, L. Cooperative Fault Tolerant Tracking Control for Multiagent Systems: An Intermediate Estimator-Based Approach. IEEE Trans. Cybern. 2018, 48, 2972–2980. [Google Scholar] [CrossRef]
- Zhao, S.; Yu, J.; Wang, Z.; Gao, D. Unknown input observer based distributed fault detection for nonlinear multi-agent systems with probabilistic time delay. J. Frankl. Inst. 2023, 360, 1058–1076. [Google Scholar] [CrossRef]
- Nemati, F.; Safavi Hamami, S.; Zemouche, A. A nonlinear observer-based approach to fault detection, isolation and estimation for satellite formation flight application. Automatica 2019, 107, 474–482. [Google Scholar] [CrossRef]
- Zhu, F.; Tan, C. Consensus Control of Linear Parameter-Varying Multi-Agent Systems with Unknown Inputs. Sensors 2023, 23, 5125. [Google Scholar] [CrossRef]
- Liu, C.; Shi, Y.; Meng, Y.; Wang, Y. Leader-following consensus of multi-agent systems with connectivity-mixed attacks and actuator/sensor faults. J. Frankl. Inst. 2023, 360, 3592–3617. [Google Scholar] [CrossRef]
- Li, P.; Qin, K.; Pu, H. Distributed robust time-varying formation control for multiple unmanned aerial vehicles systems with time-delay. In Proceedings of the 2017 29th Chinese Control And Decision Conference (CCDC), Chongqing, China, 28–30 May 2017; pp. 1539–1544. [Google Scholar] [CrossRef]
- Tang, Y.; Xue, Z.; Liu, X.; Han, Q.; Tuo, X. Leader-Following Consensus Control for Multiple Fixed-Wing UAVs’ Attitude System with Time Delays and External Disturbances. IEEE Access 2019, 7, 169773–169781. [Google Scholar] [CrossRef]
- Li, S.; Duan, N.; Xu, Z.; Liu, X.Y. Tracking Control of Quadrotor UAV with Input Delay. In Proceedings of the 2020 39th Chinese Control Conference (CCC), Shenyang, China, 27–29 July 2020; pp. 646–649. [Google Scholar] [CrossRef]
- Pedro, C.; Rogelio, L.; Alejandro, D. Modelling and Control of Mini-Flying Machines; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Nguyen, C.M.; Tan, C.P.; Trinh, H. State and delay reconstruction for nonlinear systems with input delays. Appl. Math. Comput. 2021, 390, 125609. [Google Scholar] [CrossRef]
- Nguyen, C.M.; Zemouche, A.; Trinh, H. Observer-Based Control Design for Nonlinear Systems with Unknown Delays. IEEE Trans. Circuits Syst. II Express Briefs 2022, 69, 1327–1331. [Google Scholar] [CrossRef]




| Model | Control | |||||
|---|---|---|---|---|---|---|
| Parameter | Value | Parameter | Value | Parameter | Value | |
| m [kg] | 0.4 | 0.09 | 50.88 | |||
| g [m/s2] | 9.807 | 4.29 | 3.97 | |||
| [kg·m2] | 0.177 | 2.69 | 98.33 | |||
| [kg·m2] | 0.177 | 65.18 | 8.27 | |||
| [kg·m2] | 0.354 | 3.99 | ||||
| Time-Varying Delays | |
|---|---|
| t | [10 s, 80 s) |
| 0.6 + 0.6 sin(0.6 t) | |
| 0.8 + 0.8 sin(0.6 t) | |
| 1 + sin(0.6 t) | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Campos-Martínez, S.-N.; Hernández-González, O.; Guerrero-Sánchez, M.-E.; Valencia-Palomo, G.; Targui, B.; López-Estrada, F.-R. Consensus Tracking Control of Multiple Unmanned Aerial Vehicles Subject to Distinct Unknown Delays. Machines 2024, 12, 337. https://doi.org/10.3390/machines12050337
Campos-Martínez S-N, Hernández-González O, Guerrero-Sánchez M-E, Valencia-Palomo G, Targui B, López-Estrada F-R. Consensus Tracking Control of Multiple Unmanned Aerial Vehicles Subject to Distinct Unknown Delays. Machines. 2024; 12(5):337. https://doi.org/10.3390/machines12050337
Chicago/Turabian StyleCampos-Martínez, Sandy-Natalie, Omar Hernández-González, María-Eusebia Guerrero-Sánchez, Guillermo Valencia-Palomo, Boubekeur Targui, and Francisco-Ronay López-Estrada. 2024. "Consensus Tracking Control of Multiple Unmanned Aerial Vehicles Subject to Distinct Unknown Delays" Machines 12, no. 5: 337. https://doi.org/10.3390/machines12050337
APA StyleCampos-Martínez, S.-N., Hernández-González, O., Guerrero-Sánchez, M.-E., Valencia-Palomo, G., Targui, B., & López-Estrada, F.-R. (2024). Consensus Tracking Control of Multiple Unmanned Aerial Vehicles Subject to Distinct Unknown Delays. Machines, 12(5), 337. https://doi.org/10.3390/machines12050337










