Adaptive Control of M3C-Based Variable Speed Drive for Multiple Permanent-Magnet-Synchronous-Motor-Driven Centrifugal Pumps
Abstract
:1. Introduction
1.1. Motivations
1.2. Background
- Controlling the average capacitor voltage (ACV) in cascade with the input currents amplitude control through the required input voltage .
- Keeping zero imbalance of the cluster capacitor voltage (CCV) in cascade with the circulating current control via the needed cluster voltage . It considers reducing the inter-CCV imbalances (CCV imbalance among clusters of different sub-converters) and the intra-CCV imbalances (CCV imbalance among clusters inside the same sub-converters) to zero.
- Controlling a required output variable by adjusting the output voltage amplitude and frequency.
1.3. Related Works
1.4. Contributions
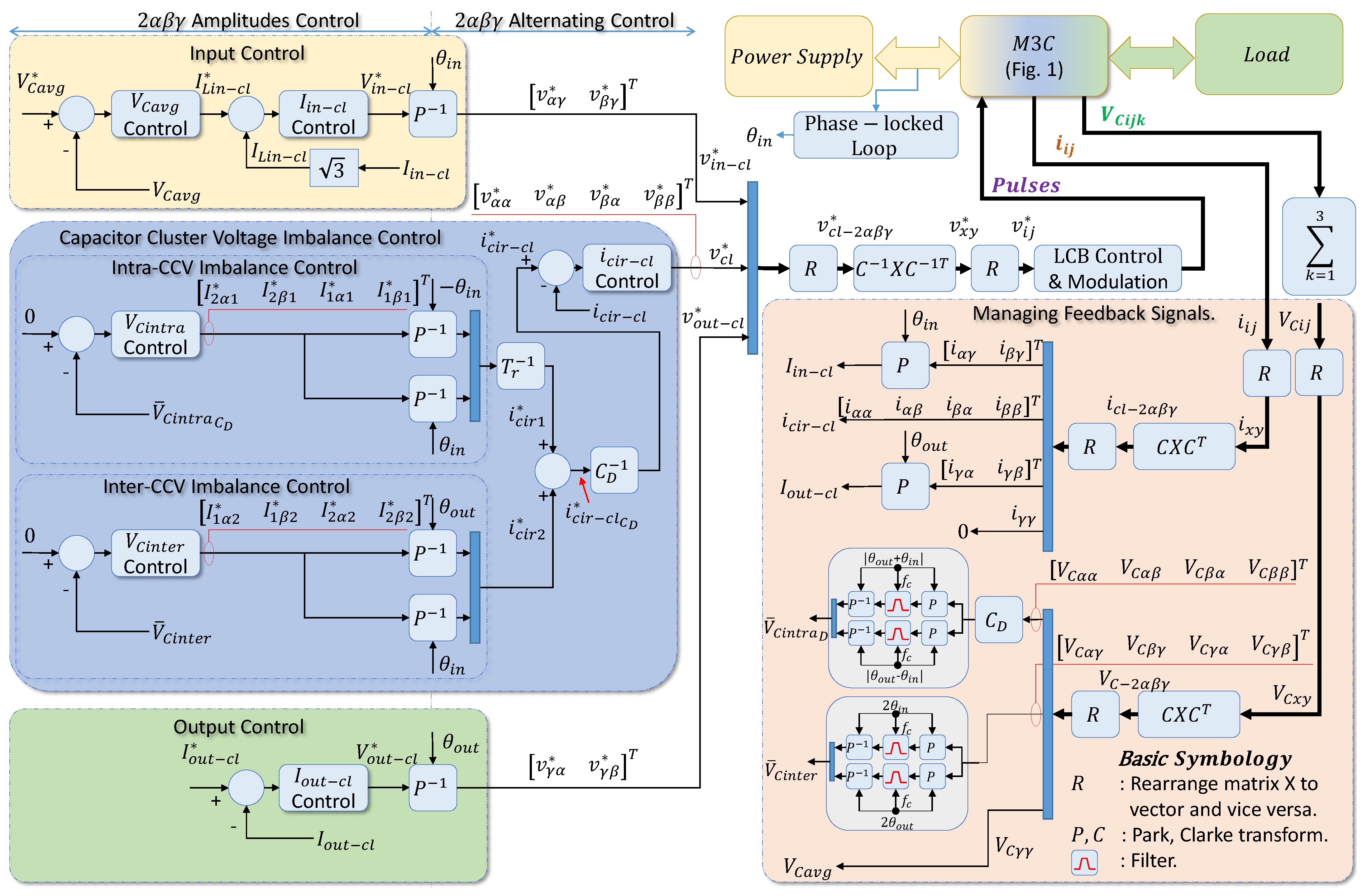
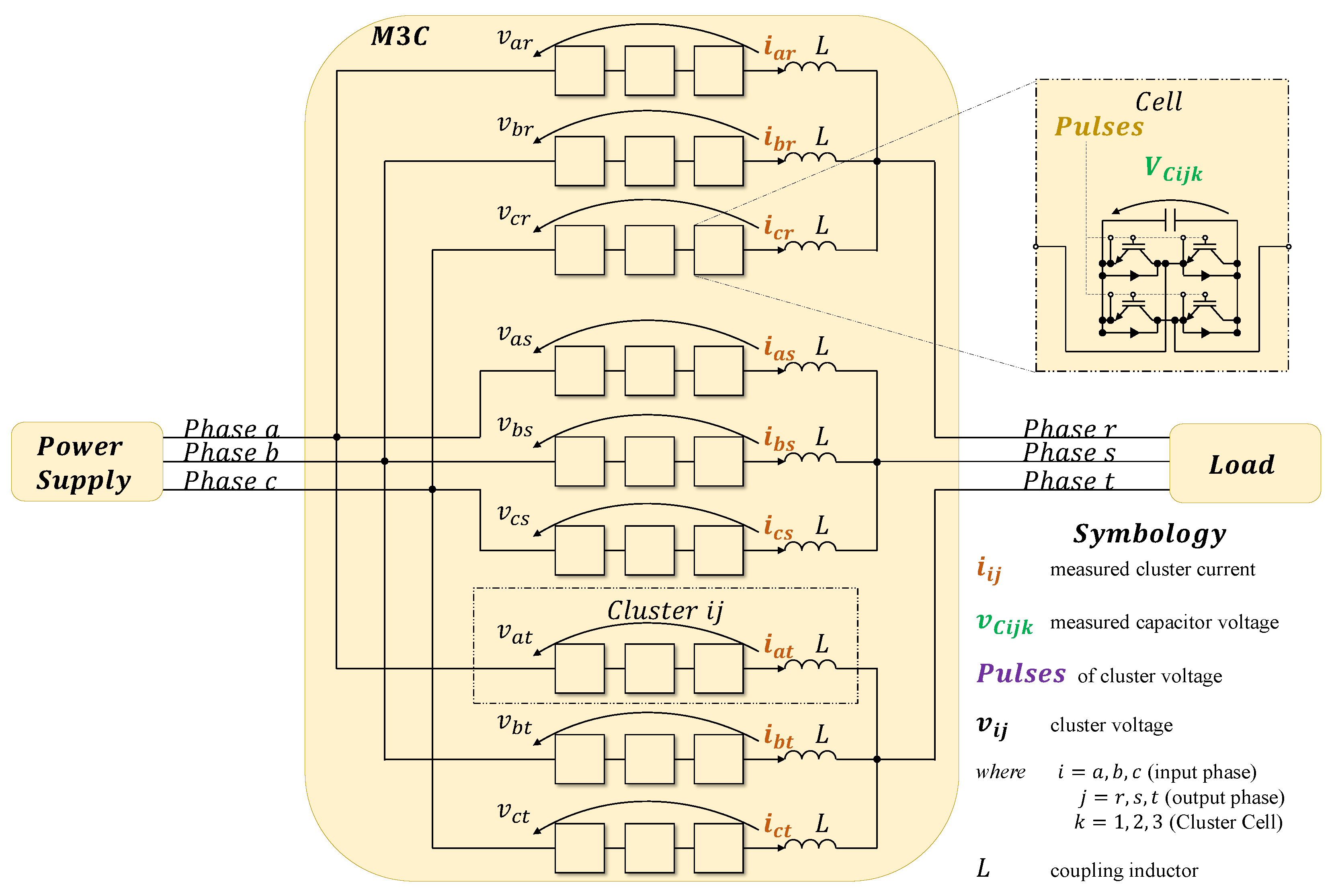
- Obtaining the multivariable M3C state-space model for control. It is an MIMO dynamical system with a currents inner loop, a voltages outer loop, and an inner–outer interface. Appendix A of this manuscript details the model obtained, which complements, describes, rearranges, and summarizes elements taken from [15,26,28]. In contrast to [15,26,28], herein, we give details for control implementation, such as the matrix and vector operations (please see, for instance, the managing feedback signals details given in Figure 2), and identify the state-space model form with inner and outer loops.
- Using MIMO adaptive controllers instead of non-adaptive SISO controllers [19,31,34]. We show that it is a viable and more straightforward solution. The proposal gains the benefits discussed in [34] of reducing the number of controllers by using an MIMO approach for an MMCC but herein for the M3C. In contrast to the works [19,31,34], tuning adaptive controllers does not require an initial estimation of the plant parameters, decreasing the commissioning time. Moreover, they adapt to plant changes without compromising their effectiveness.
- Proposing a passivity-based hybrid MRAC called PBMRAC. In contrast to [5,6,9], it uses the MRAC as a low-pass filter for the noisy reference input signals. Moreover, PBMRAC introduces to MRAC a term of an adaptive passivity-based controller (APBC) [11] to attend to the closed-loop system response time. M3C control particularly needs it after having inner reference input noise periods more than sixty times distant from the M3C inner time constant.
- Presenting APBC in cascade with PBMRAC. It expands the cascade MRAC [12] and the cascade APBC [11]. The first uses an outer SISO controller, whereas the M3C outer loop requires an MIMO controller. Moreover, as Figure 2 shows, the M3C has zero or constant outer references, eliminating the need for the outer reference model; therefore, an outer APBC [11] ensures a faster outer loop’s time response.
2. Preliminaries
2.1. M3C State-Space Model
2.2. Basic Control Based PI Controllers
2.3. Cascade Adaptive Control Background
3. Proposal
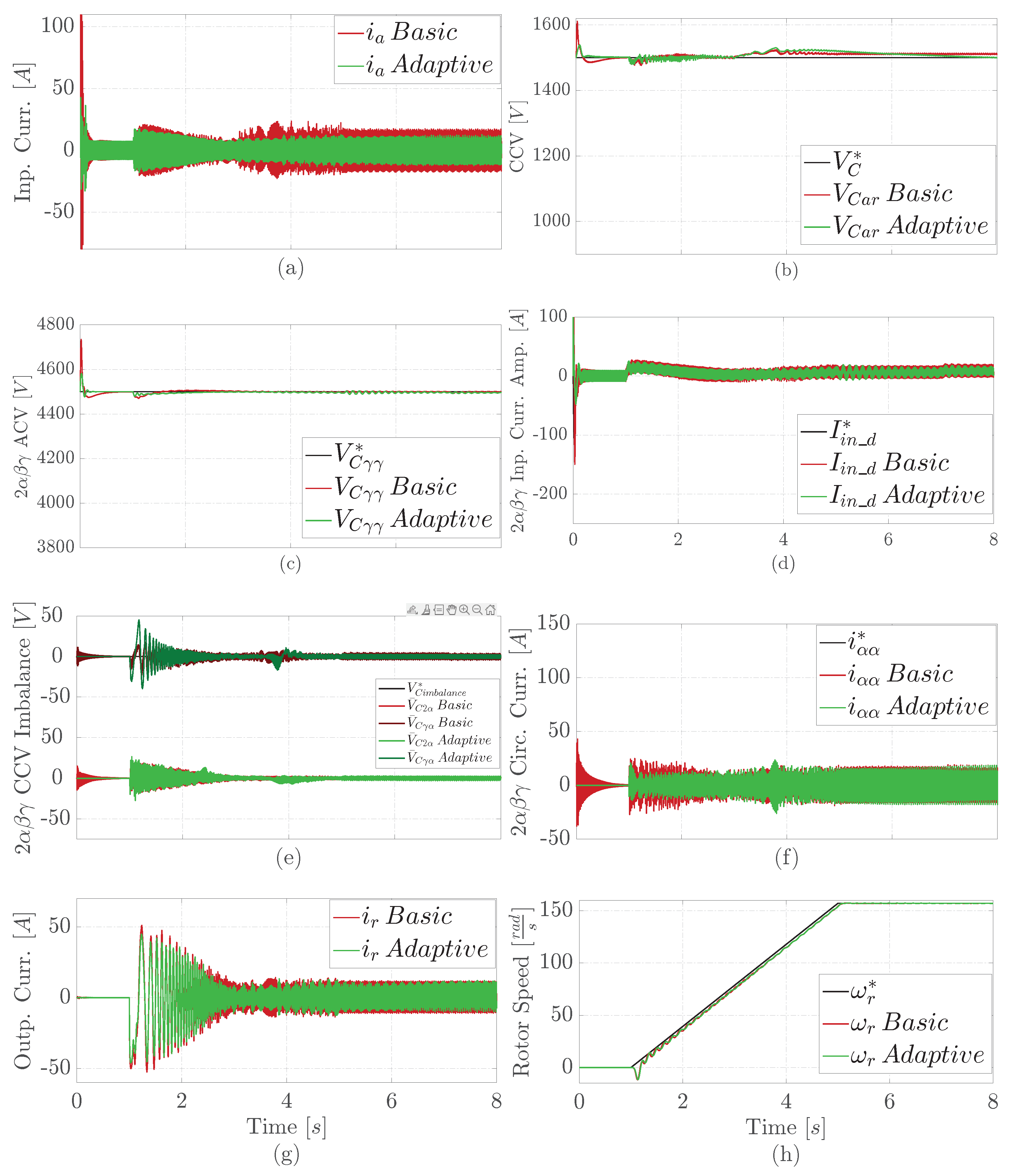
4. Simulation Results
4.1. Applied Controllers
4.1.1. Basic Control System [37]
- Input Control:
- -
- One (1) PI for the ACV control:where the constant cluster voltage amplitude is . The output of the ACV controller is the input cluster line current amplitude direct component reference . Here, the input cluster line current amplitude reference is and is controlled by the following controllers:
- -
- Two (2) PIs for the input cluster line current amplitude direct and quadrature components:
- CCV Imbalance Control.
- -
- Four (4) PIs for the intra-CCV imbalance control ([37], outer controller of Figure 3):
- -
- Four (4) PIs for the inter-CCV imbalance control ([37], Figure 4):with the constant voltage . Both of these controllers are in cascade with the following controller:
- -
- Four (4) PIs controllers for the circulating current, considering only a P action ([37], inner controller of Figure 3):
- Output control.
4.1.2. Adaptive Control System
- Input Control.
- -
- One (1) APBC (13) for the ACV control, with:The output of this ACV controller is the input cluster line current amplitude direct component reference . Therefore, the inner loop input cluster line current amplitude reference is , having the following controller:
- -
- One (1) PBMRAC (15) for the input cluster line current, and filtering a 2 KHz reference input noise:
- CCV imbalance control.
- Output control.
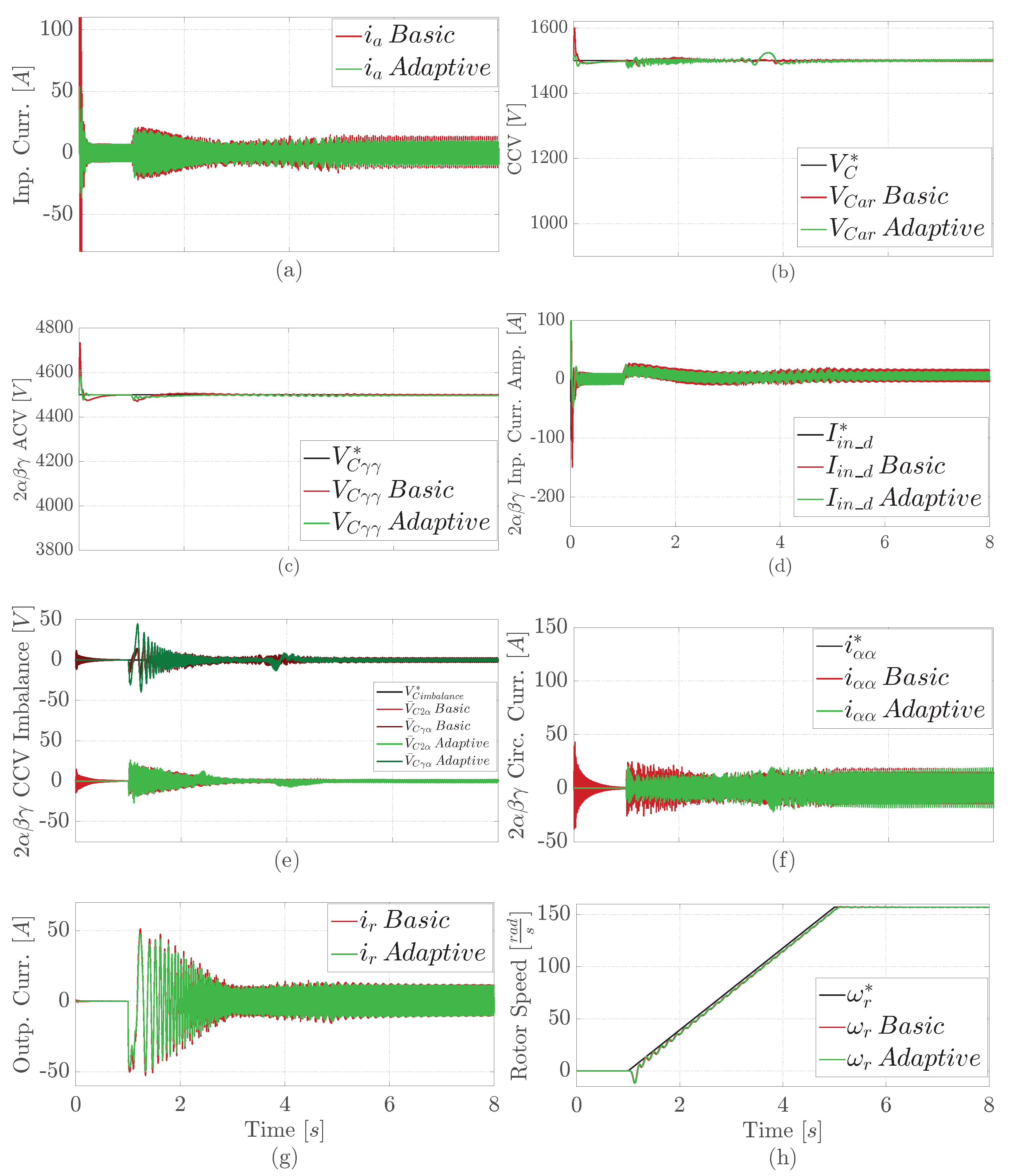
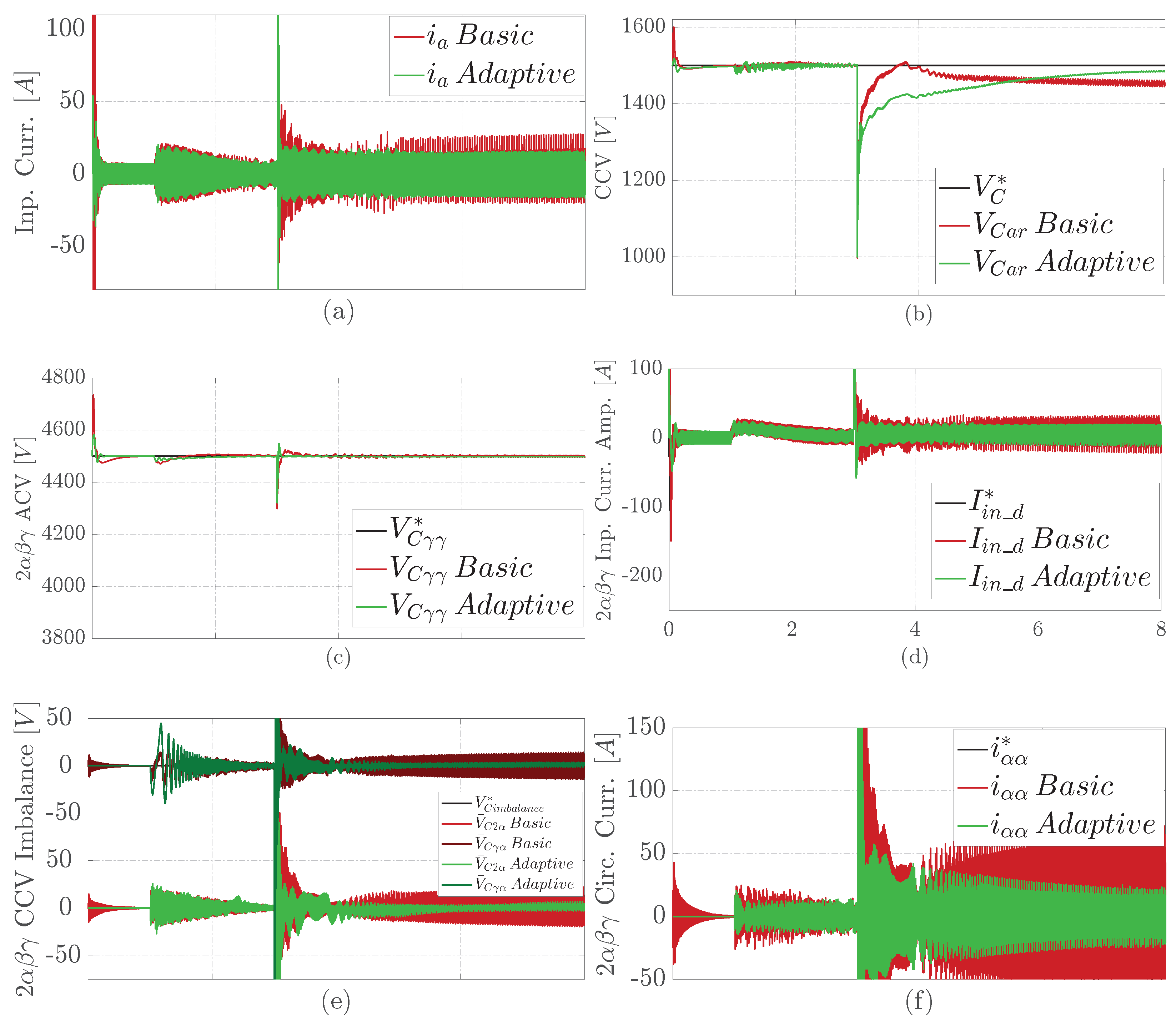
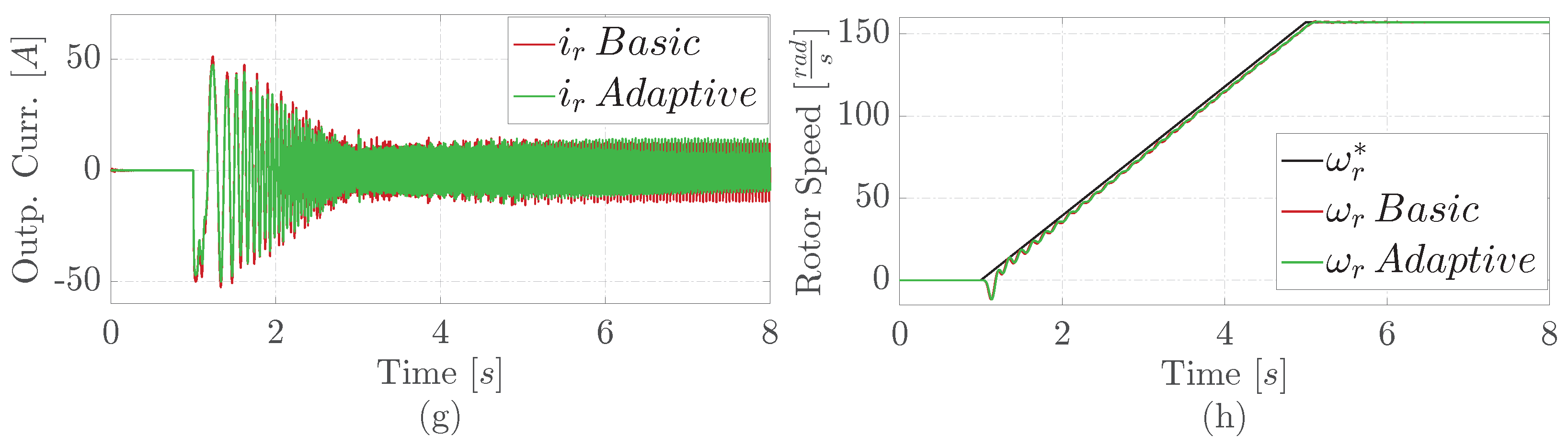
4.2. Results under a Normal Operation
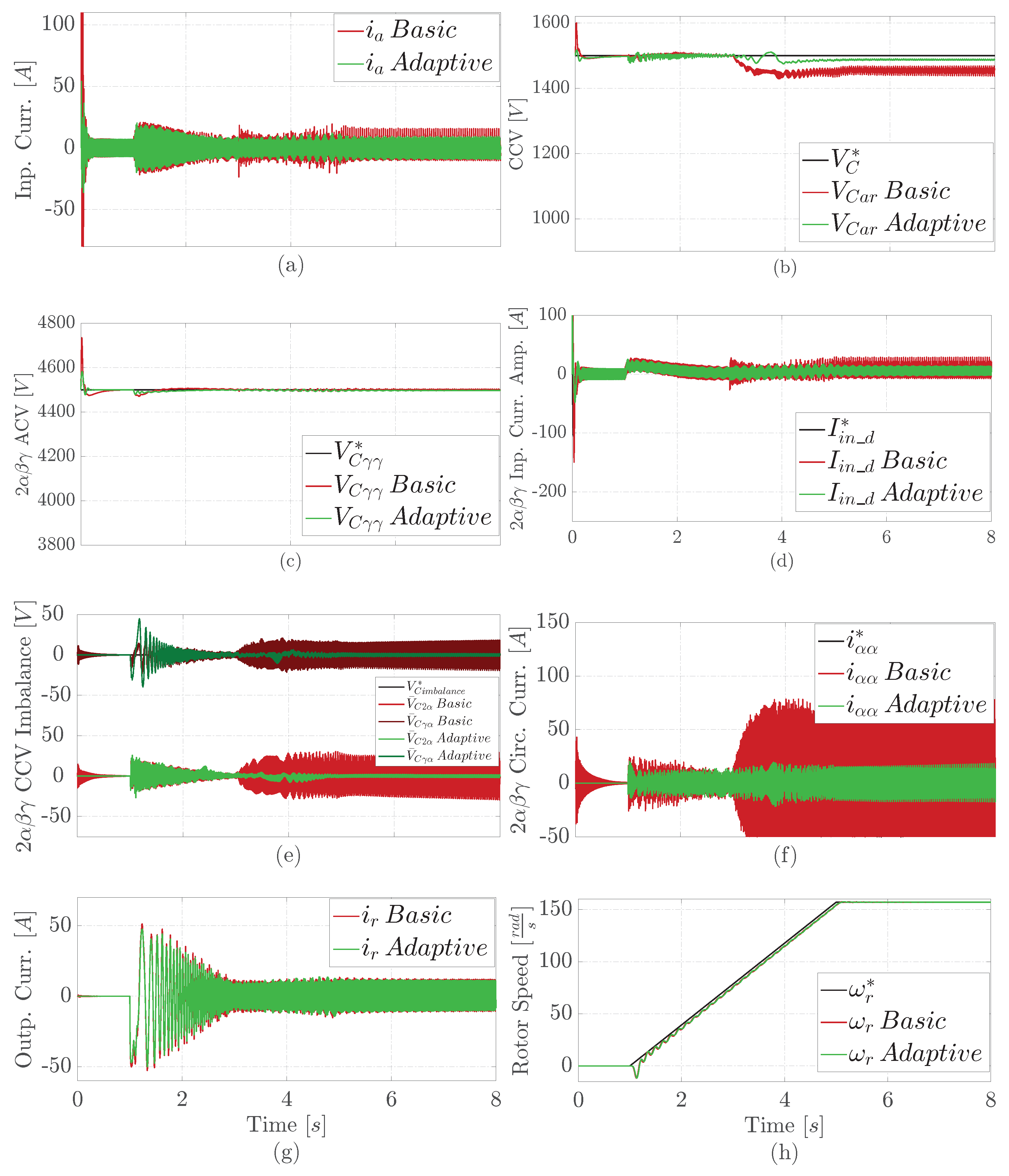
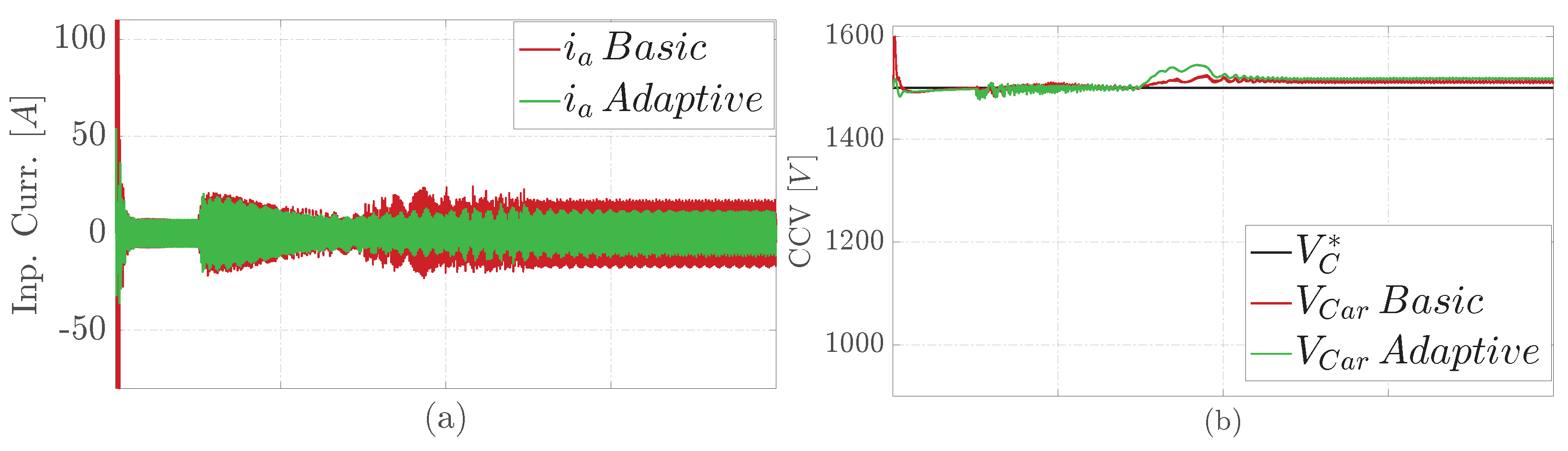
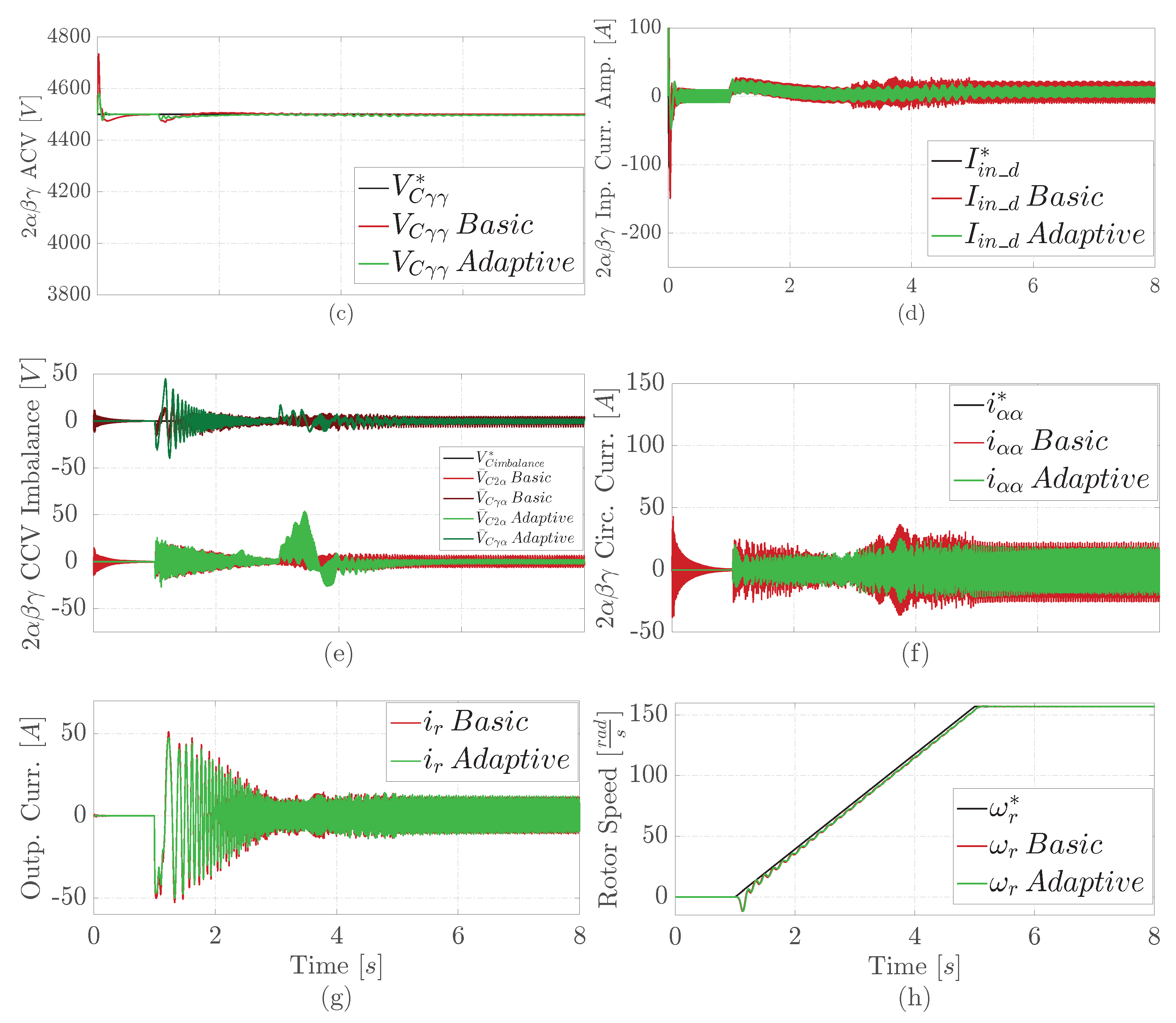
4.3. Results under an Input Phase Imbalance
4.4. Results under a Cluster Cell Short Circuit
4.5. Results under an Opened Cluster Cell
4.6. Results under Parameters Changes of the Motor–Pump Set
5. Conclusions
- It reduces the number of non-adaptive PI controllers from sixteen (16) to five (5) MIMO adaptive controllers.
- It is a more straightforward solution that does not require previous estimation of the plant parameters, reducing the commissioning time.
- The proposed adaptive control has fewer overshoots than the basic solution.
- Additionally, it shows a more stable CCV response (less noisy), which is as expected due to the APBC-PBMRAC design.
- Finally, the basic solution tends to remain degraded after a fault, while the adaptive approach tends to recover quickly from any studied fault.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
Appendix A.1. Inner Control Loop M3C Dynamical Model
Appendix A.1.1. x-y Voltage–Current Model ([15], Equation (9))
Appendix A.1.2. Double-αβγ Voltage–Current Model ([15], Equation (18))
Appendix A.1.3. Double-αβγ State-Space Model of Instantaneous Voltage–Current ([15], Equations (19)–(21))
Appendix A.1.4. Double-αβγ Voltage–Current State-Space Model
Appendix A.2. Outer Control Loop M3C Dynamical Model
Appendix A.2.1. x-y Cluster Capacitor Voltage–Power State-Space Model
Appendix A.2.2. Double-αβγ State-Space Model of Instantaneous Voltage–Power
Appendix A.2.3. Double-αβγ Voltage–Power State-Space Model
Appendix A.3. Vector and Matrix Transformation Details
References
- Lin, Y.; Li, X.; Zhu, Z.; Wang, X.; Lin, T.; Cao, H. An energy consumption improvement method for centrifugal pump based on bionic optimization of blade trailing edge. Energy 2022, 246, 123323. [Google Scholar] [CrossRef]
- Beck, M.; Sperlich, A.; Blank, R.; Meyer, E.; Binz, R.; Ernst, M. Increasing energy efficiency in water collection systems by submersible PMSM well pumps. Water 2018, 10, 1310. [Google Scholar] [CrossRef]
- Candelo-Zuluaga, C.; Riba, J.R.; Espinosa, A.G.; Blanch, P.T. Customized PMSM design and optimization methodology for water pumping applications. IEEE Trans. Energy Convers. 2021, 37, 454–465. [Google Scholar] [CrossRef]
- Hebala, A.; Nuzzo, S.; Connor, P.H.; Volpe, G.; Gerada, C.; Galea, M. Analysis and Mitigation of AC Losses in High Performance Propulsion Motors. Machines 2022, 10, 780. [Google Scholar] [CrossRef]
- Kashif, M.; Singh, B. Reduced-Sensor-Based Multistage Model Reference Adaptive Control of PV-Fed PMSM Drive for Water Pump. IEEE Trans. Ind. Electron. 2022, 70, 3782–3792. [Google Scholar] [CrossRef]
- Kashif, M.; Singh, B. Modified Active-Power MRAS Based Adaptive Control with Reduced Sensors for PMSM Operated Solar Water Pump. IEEE Trans. Energy Convers. 2022, 38, 38–52. [Google Scholar] [CrossRef]
- Dini, P.; Saponara, S. Review on model based design of advanced control algorithms for cogging torque reduction in power drive systems. Energies 2022, 15, 8990. [Google Scholar] [CrossRef]
- He, R.; Han, Q. Dynamics and stability of permanent-magnet synchronous motor. Math. Probl. Eng. 2017, 2017, 4923987. [Google Scholar] [CrossRef]
- Annaswamy, A.M.; Narendra, K.S. Stable Adaptive Systems; Prentice Hall: Hoboken, NJ, USA, 1989. [Google Scholar]
- Eugene, L.; Kevin, W.; Howe, D. Robust and Adaptive Control with Aerospace Applications; Springer: London, UK, 2013. [Google Scholar]
- Travieso-Torres, J.C.; Duarte-Mermoud, M.A.; Estrada, J.L. Tracking control of cascade systems based on passivity: The non-adaptive and adaptive cases. ISA Trans. 2006, 45, 435–445. [Google Scholar] [CrossRef]
- Travieso-Torres, J.C.; Ricaldi-Morales, A.; Véliz-Tejo, A.; Leiva-Silva, F. Robust Cascade MRAC for a Hybrid Grid-Connected Renewable Energy System. Processes 2023, 11, 1774. [Google Scholar] [CrossRef]
- Gili, L.C.; Dias, J.C.; Lazzarin, T.B. Review, Challenges and Potential of AC/AC Matrix Converters CMC, MMMC, and M3C. Energies 2022, 15, 9421. [Google Scholar] [CrossRef]
- Pires, V.F.; Cordeiro, A.; Foito, D.; Pires, A.J. Fault-tolerant multilevel converter to feed a switched reluctance machine. Machines 2022, 10, 35. [Google Scholar] [CrossRef]
- Diaz, M.; Cardenas, R.; Ibaceta, E.; Mora, A.; Urrutia, M.; Espinoza, M.; Rojas, F.; Wheeler, P. An Overview of Modelling Techniques and Control Strategies for Modular Multilevel Matrix Converters. Energies 2020, 13, 4678. [Google Scholar] [CrossRef]
- Kucka, J.; Karwatzki, D.; Mertens, A. AC/AC modular multilevel converters in wind energy applications: Design considerations. In Proceedings of the 2016 18th European Conference on Power Electronics and Applications (EPE’16 ECCE Europe), Karlsruhe, Germany, 5–9 September 2016; pp. 1–10. [Google Scholar]
- Miura, Y.; Mizutani, T.; Ito, M.; Ise, T. Modular multilevel matrix converter for low frequency AC transmission. In Proceedings of the 2013 IEEE 10th International Conference on Power Electronics and Drive Systems (PEDS), Kitakyushu, Japan, 22–25 April 2013; pp. 1079–1084. [Google Scholar]
- Bontemps, P.; Milovanovic, S.; Dujic, D. Performance analysis of energy balancing methods for matrix modular multilevel converters. IEEE Trans. Power Electron. 2022, 38, 2910–2924. [Google Scholar] [CrossRef]
- Fan, B.; Wang, K.; Wheeler, P.; Gu, C.; Li, Y. An optimal full frequency control strategy for the modular multilevel matrix converter based on predictive control. IEEE Trans. Power Electron. 2017, 33, 6608–6621. [Google Scholar] [CrossRef]
- Fan, B.; Wang, K.; Wheeler, P.; Gu, C.; Li, Y. A branch current reallocation based energy balancing strategy for the modular multilevel matrix converter operating around equal frequency. IEEE Trans. Power Electron. 2017, 33, 1105–1117. [Google Scholar] [CrossRef]
- Kawamura, W.; Chen, K.L.; Hagiwara, M.; Akagi, H. A low-speed, high-torque motor drive using a modular multilevel cascade converter based on triple-star bridge cells (MMCC-TSBC). IEEE Trans. Ind. Appl. 2015, 51, 3965–3974. [Google Scholar] [CrossRef]
- Wang, C.; Zheng, Z.; Wang, K.; Li, Y. Fault detection and tolerant control of IGBT open-circuit failures in modular multilevel matrix converters. IEEE J. Emerg. Sel. Top. Power Electron. 2022, 10, 6714–6727. [Google Scholar] [CrossRef]
- Wang, C.; Zheng, Z.; Wang, K.; Yang, B.; Zhou, P.; Li, Y. Analysis and control of modular multilevel matrix converters under branch fault conditions. IEEE Trans. Power Electron. 2021, 37, 1682–1699. [Google Scholar] [CrossRef]
- Wang, C.; Zheng, Z.; Wang, K.; Li, Y. Submodule Fault-Tolerant Control of Modular Multilevel Matrix Converters With Adaptive Optimum Common-Mode Voltage Injection. IEEE Trans. Power Electron. 2022, 37, 7548–7554. [Google Scholar] [CrossRef]
- Guo, F.; Yu, J.; Ni, Q.; Zhang, Z.; Meng, J.; Wang, Y. Grid-forming control strategy for PMSG wind turbines connected to the low-frequency AC transmission system. Energy Rep. 2023, 9, 1464–1472. [Google Scholar] [CrossRef]
- Kawamura, W.; Hagiwara, M.; Akagi, H. Control and experiment of a modular multilevel cascade converter based on triple-star bridge cells. IEEE Trans. Ind. Appl. 2014, 50, 3536–3548. [Google Scholar] [CrossRef]
- Erickson, R.W.; Al-Naseem, O.A. A new family of matrix converters. In Proceedings of the IECON’01. 27th Annual Conference of the IEEE Industrial Electronics Society (Cat. No.37243), Denver, CO, USA, 29 November–2 December 2001; Volume 2, pp. 1515–1520. [Google Scholar]
- Zhang, Z.; Jin, Y.; Xu, Z. Modeling and Control of Modular Multilevel Matrix Converter for Low-Frequency AC Transmission. Energies 2023, 16, 3474. [Google Scholar] [CrossRef]
- Bravo, P.; Pereda, J.; Merlin, M.M.; Neira, S.; Green, T.C.; Rojas, F. Modular Multilevel Matrix Converter as Solid State Transformer for Medium and High Voltage AC Substations. IEEE Trans. Power Deliv. 2022, 37, 5033–5043. [Google Scholar] [CrossRef]
- Park, R.H. Two-reaction theory of synchronous machines generalized method of analysis-part I. Trans. Am. Inst. Electr. Eng. 1929, 48, 716–727. [Google Scholar] [CrossRef]
- Kammerer, F.; Gommeringer, M.; Kolb, J.; Braun, M. Energy balancing of the modular multilevel matrix converter based on a new transformed arm power analysis. In Proceedings of the 2014 IEEE 16th European Conference on Power Electronics and Applications, Lappeenranta, Finland, 26–28 August 2014; pp. 1–10. [Google Scholar]
- Kawamura, W.; Chiba, Y.; Akagi, H. A broad range of speed control of a permanent magnet synchronous motor driven by a modular multilevel TSBC converter. IEEE Trans. Ind. Appl. 2017, 53, 3821–3830. [Google Scholar] [CrossRef]
- Kawamura, W.; Hagiwara, M.; Akagi, H.; Tsukakoshi, M.; Nakamura, R.; Kodama, S. AC-Inductors design for a modular multilevel TSBC converter, and performance of a low-speed high-torque motor drive using the converter. IEEE Trans. Ind. Appl. 2017, 53, 4718–4729. [Google Scholar] [CrossRef]
- Arias-Esquivel, Y.; Cardenas, R.; Urrutia, M.; Diaz, M.; Tarisciotti, L.; Clare, J.C. Continuous control set model predictive control of a modular multilevel converter for drive applications. IEEE Trans. Ind. Electron. 2022, 70, 8723–8733. [Google Scholar] [CrossRef]
- Travieso-Torres, J.C.; Vilaragut-Llanes, M.; Costa-Montiel, Á.; Duarte-Mermoud, M.A.; Aguila-Camacho, N.; Contreras-Jara, C.; Álvarez-Gracia, A. New adaptive high starting torque scalar control scheme for induction motors based on passivity. Energies 2020, 13, 1276. [Google Scholar] [CrossRef]
- Ogata, K. Modern Control Engineering; Prentice Hall: Upper Saddle River, NJ, USA, 2010; Volume 5. [Google Scholar]
- Diaz, M.; Cardenas, R.; Espinoza, M.; Hackl, C.M.; Rojas, F.; Clare, J.C.; Wheeler, P. Vector control of a modular multilevel matrix converter operating over the full output-frequency range. IEEE Trans. Ind. Electron. 2018, 66, 5102–5114. [Google Scholar] [CrossRef]
- Véliz-Tejo, A.; Travieso-Torres, J.C.; Peters, A.A.; Mora, A.; Leiva-Silva, F. Normalized-Model Reference System for Parameter Estimation of Induction Motors. Energies 2022, 15, 4542. [Google Scholar] [CrossRef]
- O’Rourke, C.J.; Qasim, M.M.; Overlin, M.R.; Kirtley, J.L. A geometric interpretation of reference frames and transformations: dq0, clarke, and park. IEEE Trans. Energy Convers. 2019, 34, 2070–2083. [Google Scholar] [CrossRef]
- Clarke, E. Circuit Analysis of AC Power Systems: Symmetrical and Related Components; Wiley: Hoboken, NJ, USA, 1943; Volume 1. [Google Scholar]
- Maity, A.; Höcht, L.; Holzapfel, F. Time-varying parameter model reference adaptive control and its application to aircraft. Eur. J. Control 2019, 50, 161–175. [Google Scholar] [CrossRef]
- Arias-Esquivel, Y.; Cárdenas, R.; Tarisciotti, L.; Díaz, M.; Mora, A. A Two-Step Continuous-Control-Set MPC for Modular Multilevel Converters Operating with Variable Output Voltage and Frequency. IEEE Trans. Power Electron. 2023, 1–12. [Google Scholar] [CrossRef]
- Dini, P.; Ariaudo, G.; Botto, G.; Greca, F.L.; Saponara, S. Real-time electro-thermal modelling & predictive control design of resonant power converter in full electric vehicle applications. IET Power Electron. 2023. [Google Scholar] [CrossRef]
| Controller | b | PI Quantity | |
|---|---|---|---|
| Input Current Amplitude Control | 2 | ||
| ACV Control d | (1 Hz) | 1 | |
| Intra-CCV Imbalance Control | (5 Hz) | 4 | |
| Inter-CCV Imbalance Control | (5 Hz) | 1 | |
| Inter-CCV Imbalance Control | (5 Hz) | 1 | |
| Inter-CCV Imbalance Control | (5 Hz) | 2 |
| Parameter | Value | Parameter | Value |
|---|---|---|---|
| 644 [W] | 4.1 [N-m] | ||
| 165 [V] | 2.65 [A] | ||
| 75 [Hz] | 0.95 | ||
| P | 3 | 0.305 [Wb] | |
| 157.08 [rad/s] | 0.0036 [Nms] | ||
| 6.2 [] | 0.0108 [Nms] | ||
| 25.025 [mH] | 93.053 · 10 [Kg m] | ||
| 40.17 [mH] | 0.41 [N-m] |
| Parameter | Value | Parameter | Value |
|---|---|---|---|
| 644 [W] | 1500 [V] | ||
| 220 [V] | 165 [V] | ||
| 50 [Hz] | 75 [Hz] | ||
| 1.5 [mH] | L | 1.0 [mH] | |
| 10 [KHz] | C | 3.3 [mF] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mendoza-Becker, R.; Travieso-Torres, J.C.; Díaz, M. Adaptive Control of M3C-Based Variable Speed Drive for Multiple Permanent-Magnet-Synchronous-Motor-Driven Centrifugal Pumps. Machines 2023, 11, 884. https://doi.org/10.3390/machines11090884
Mendoza-Becker R, Travieso-Torres JC, Díaz M. Adaptive Control of M3C-Based Variable Speed Drive for Multiple Permanent-Magnet-Synchronous-Motor-Driven Centrifugal Pumps. Machines. 2023; 11(9):884. https://doi.org/10.3390/machines11090884
Chicago/Turabian StyleMendoza-Becker, Rodrigo, Juan Carlos Travieso-Torres, and Matías Díaz. 2023. "Adaptive Control of M3C-Based Variable Speed Drive for Multiple Permanent-Magnet-Synchronous-Motor-Driven Centrifugal Pumps" Machines 11, no. 9: 884. https://doi.org/10.3390/machines11090884
APA StyleMendoza-Becker, R., Travieso-Torres, J. C., & Díaz, M. (2023). Adaptive Control of M3C-Based Variable Speed Drive for Multiple Permanent-Magnet-Synchronous-Motor-Driven Centrifugal Pumps. Machines, 11(9), 884. https://doi.org/10.3390/machines11090884







