Repeated Collision of a Planar Robotic Arm with a Surface Using Generalized Active Forces
Abstract
1. Introduction
2. Mathematical Model
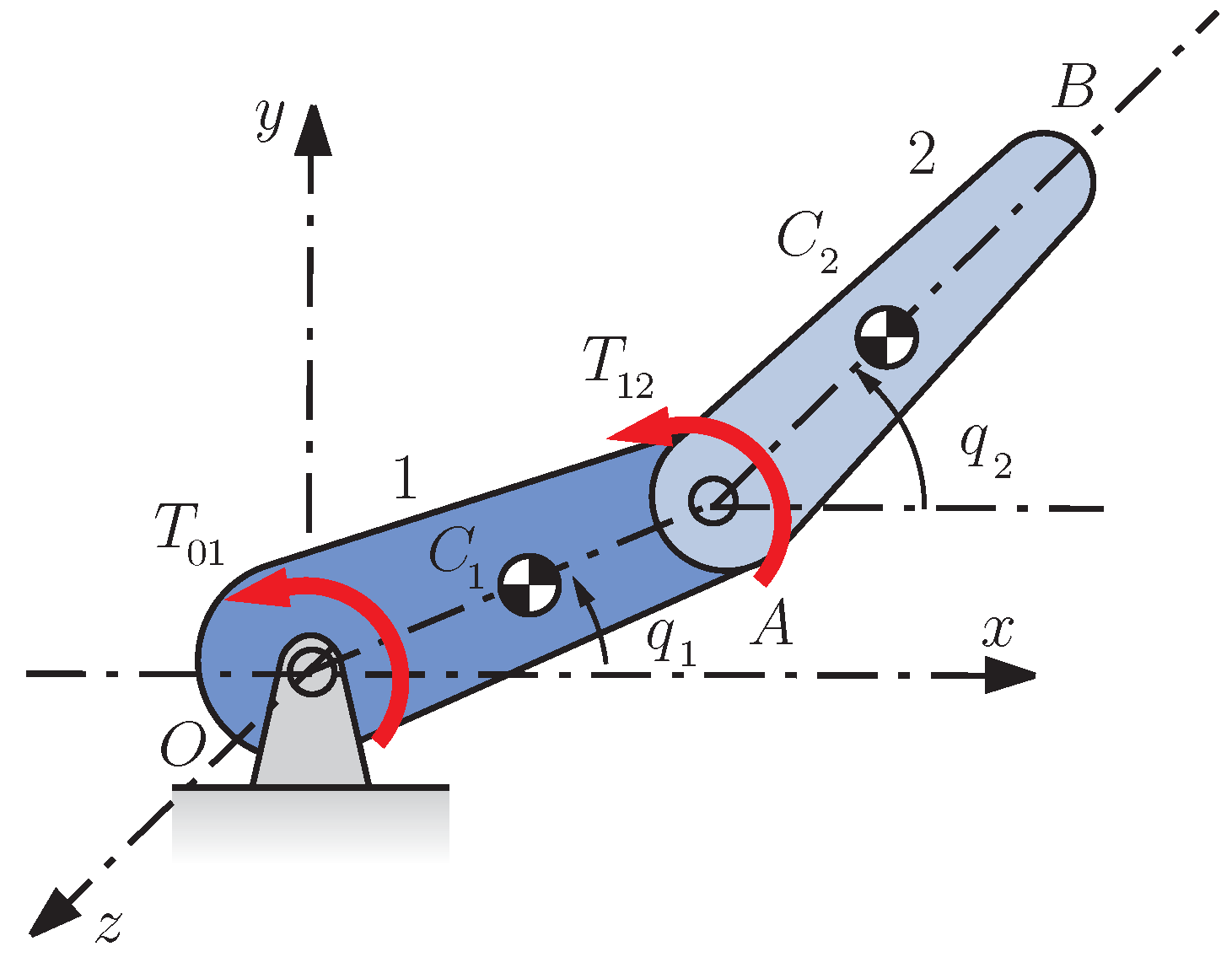
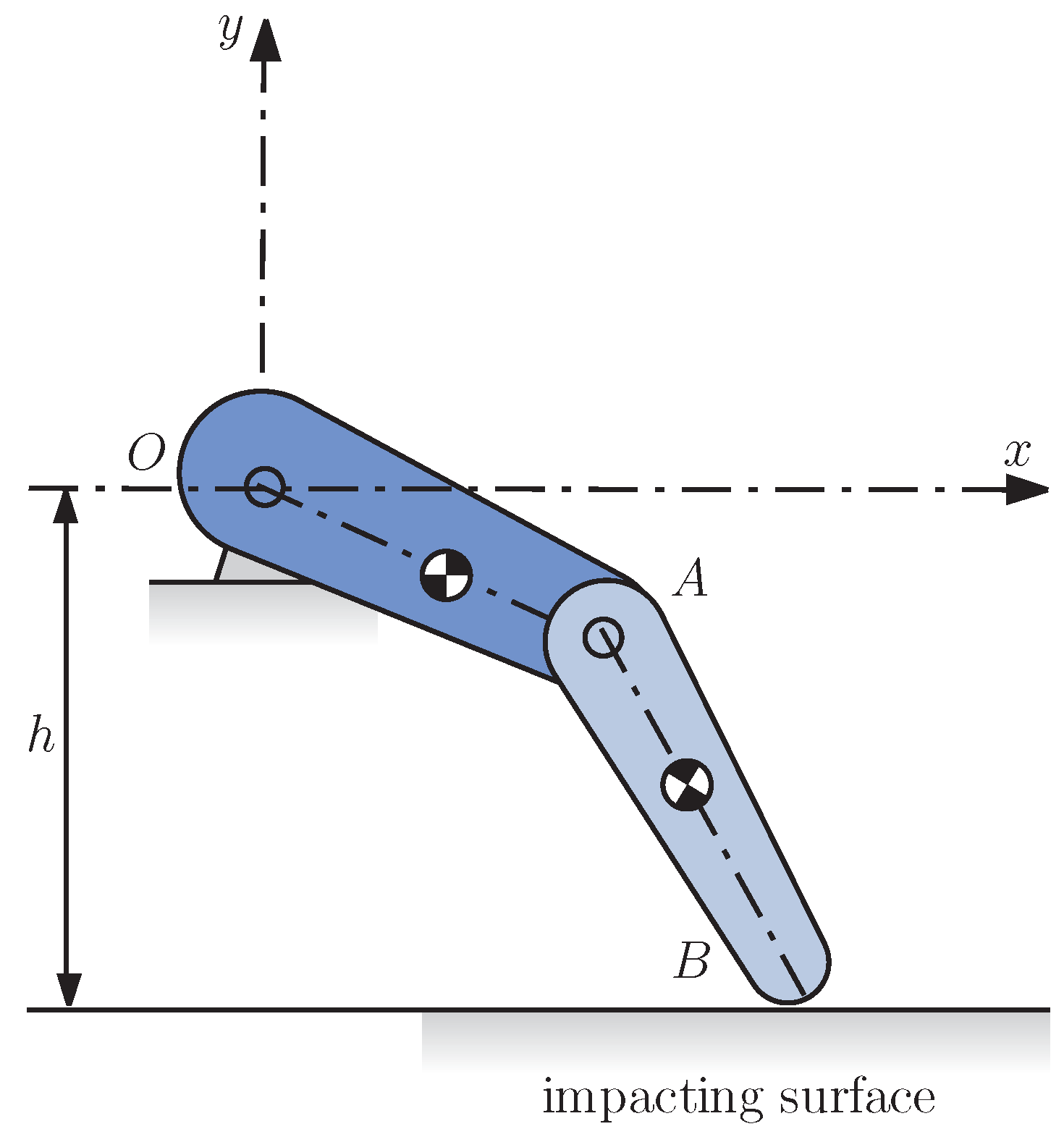
2.1. Configuration of the Robotic Arm
2.2. Equations of Motion
2.3. Control Strategy
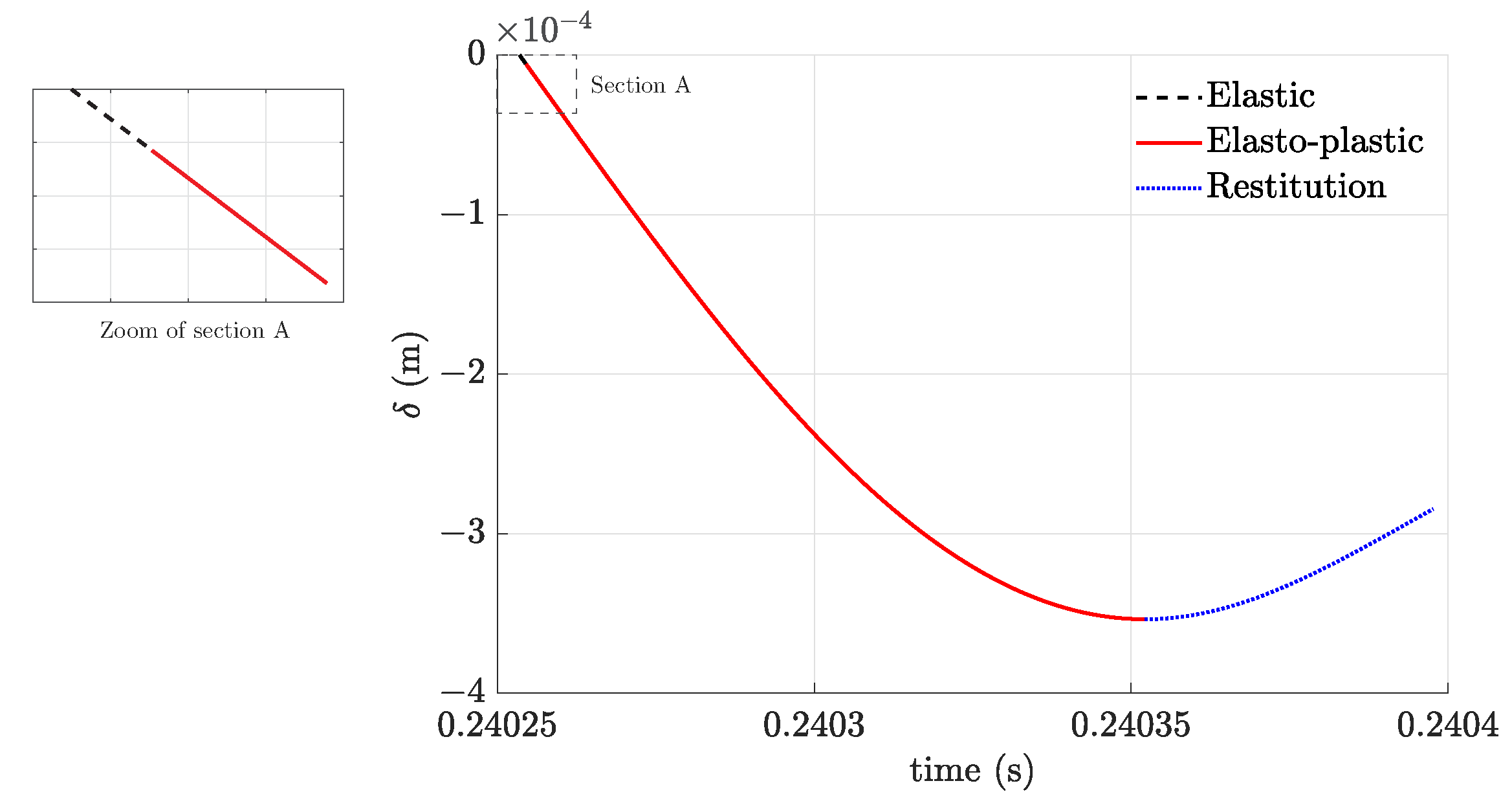
2.4. Contact Force during Impact
2.4.1. The Normal Impact Force
2.4.2. The Friction Force
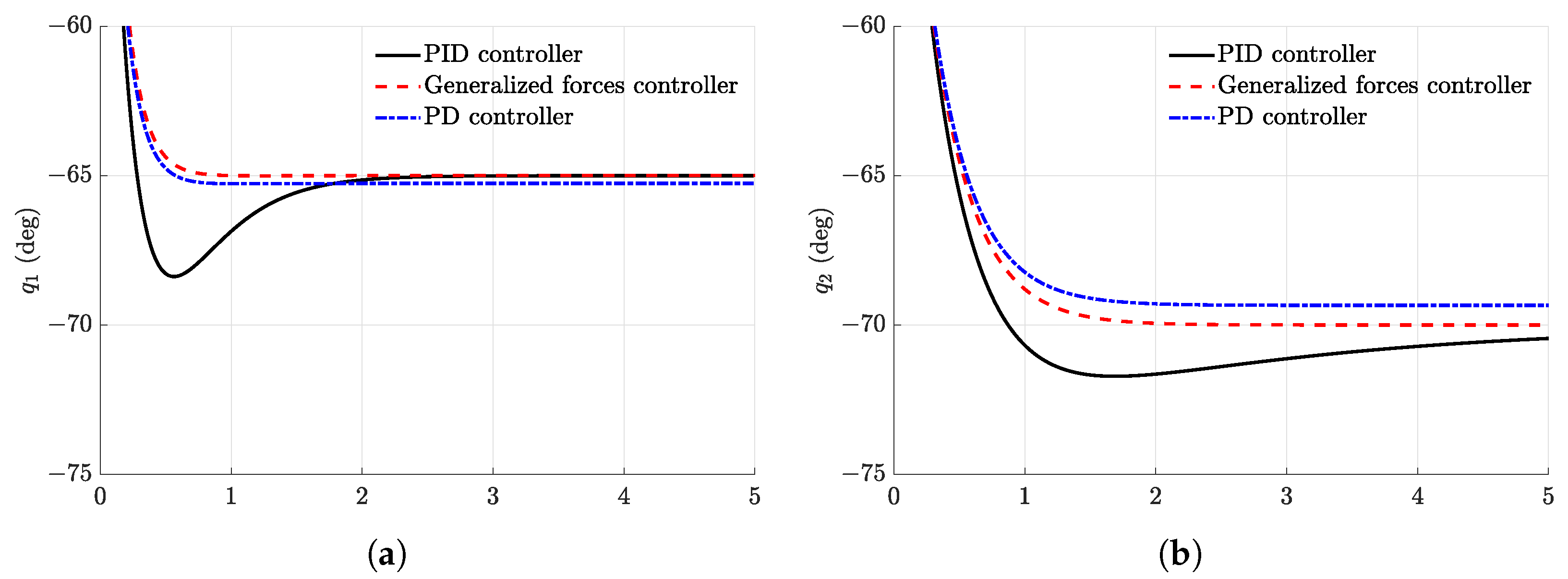
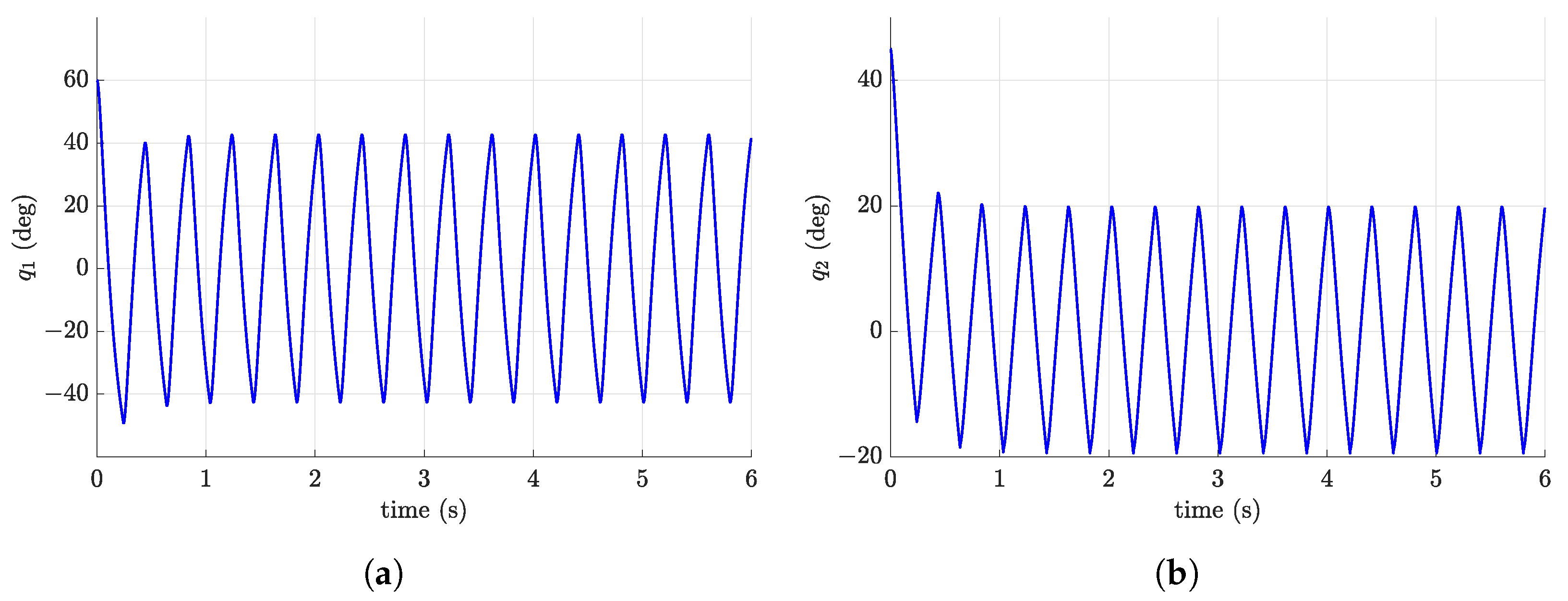
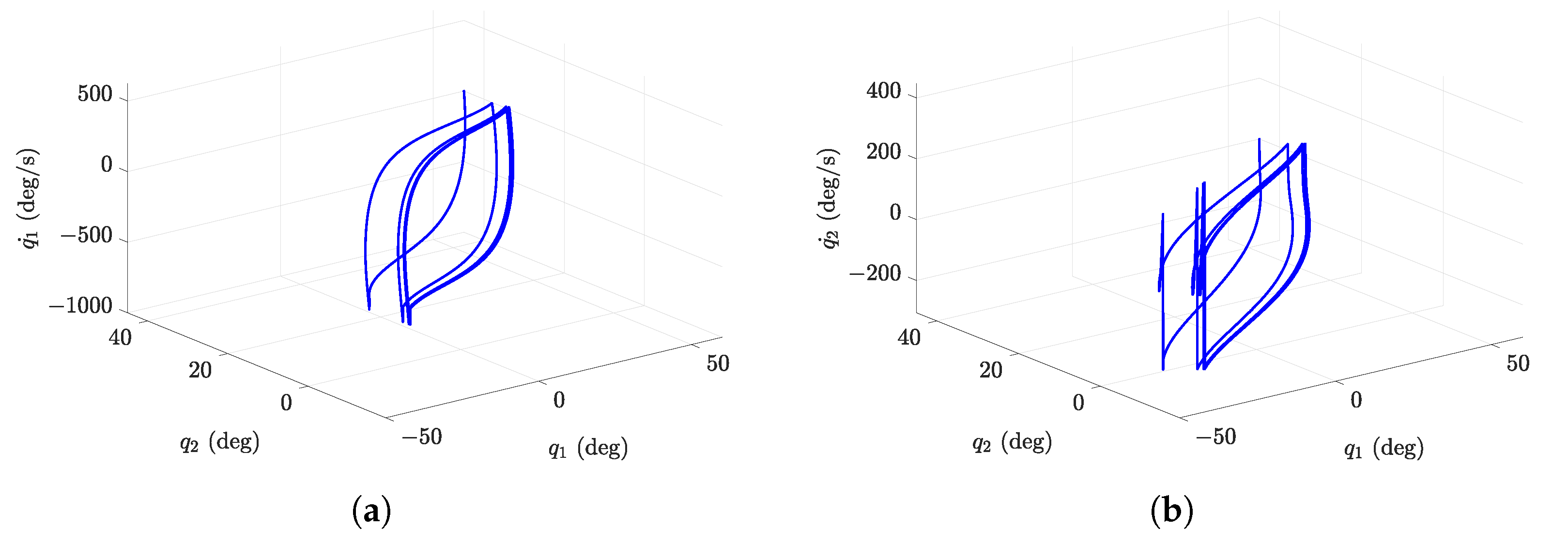
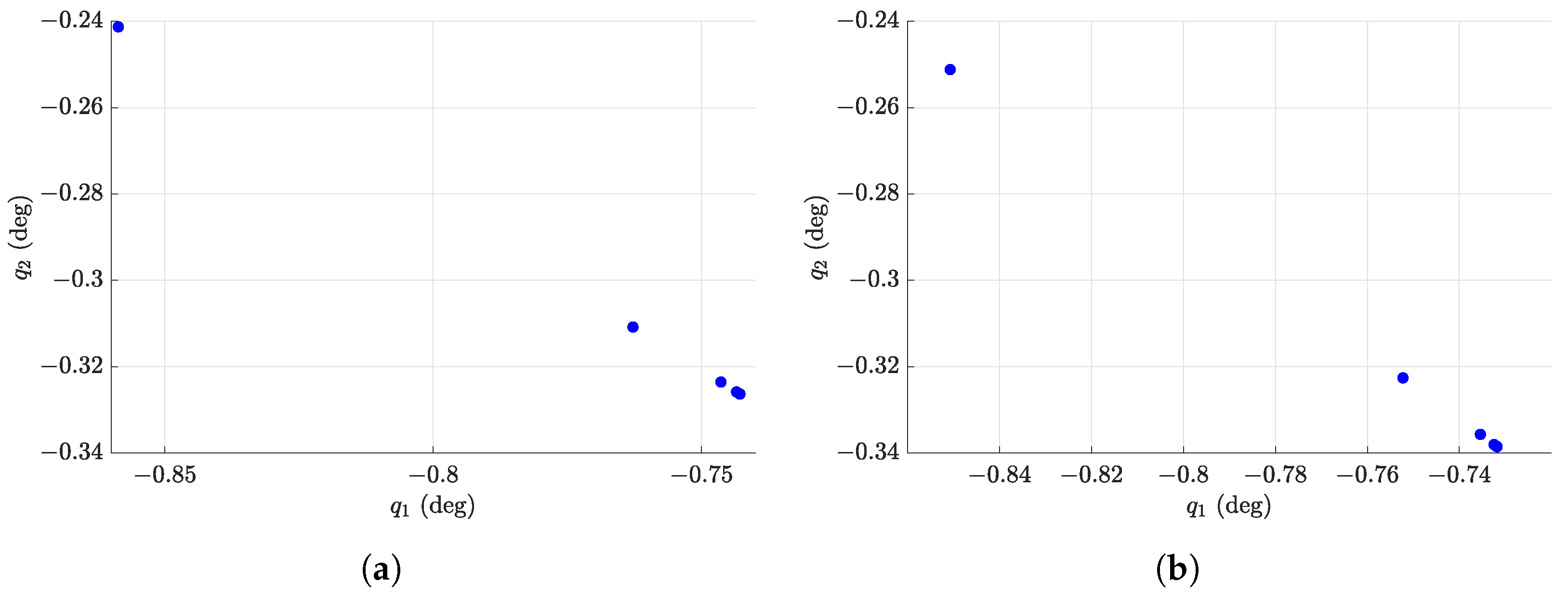
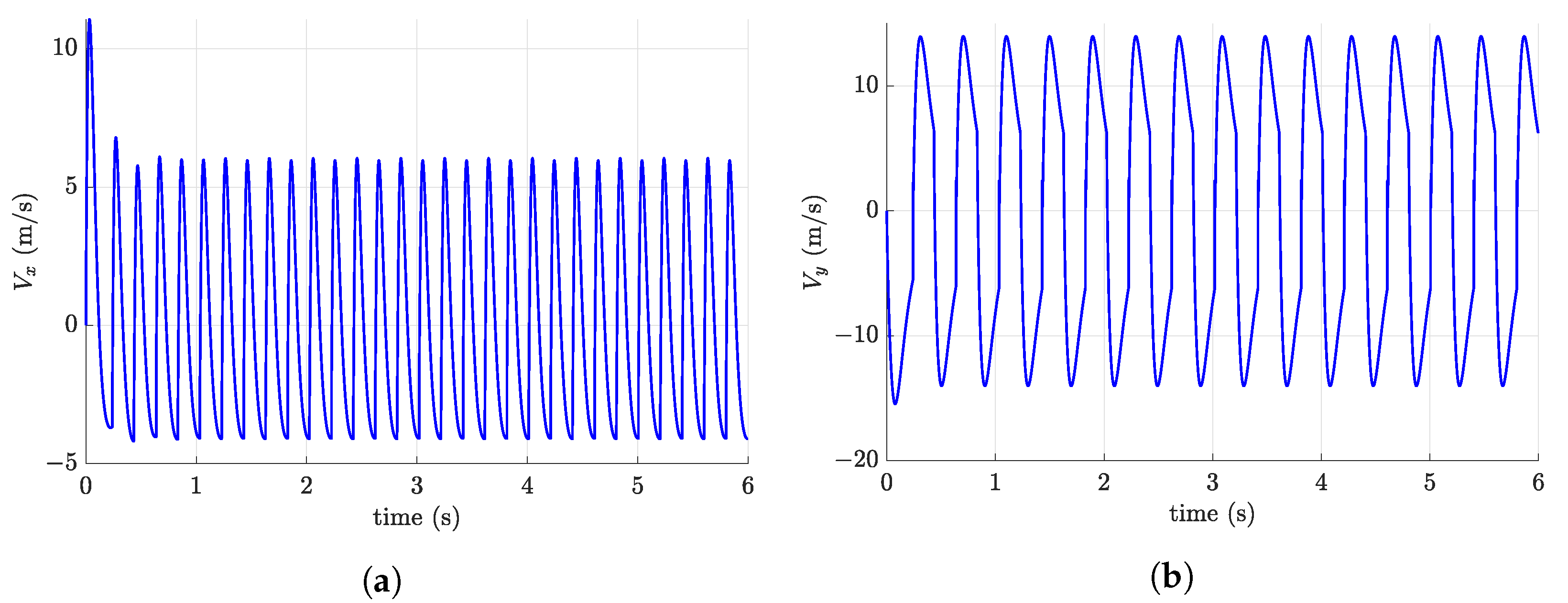
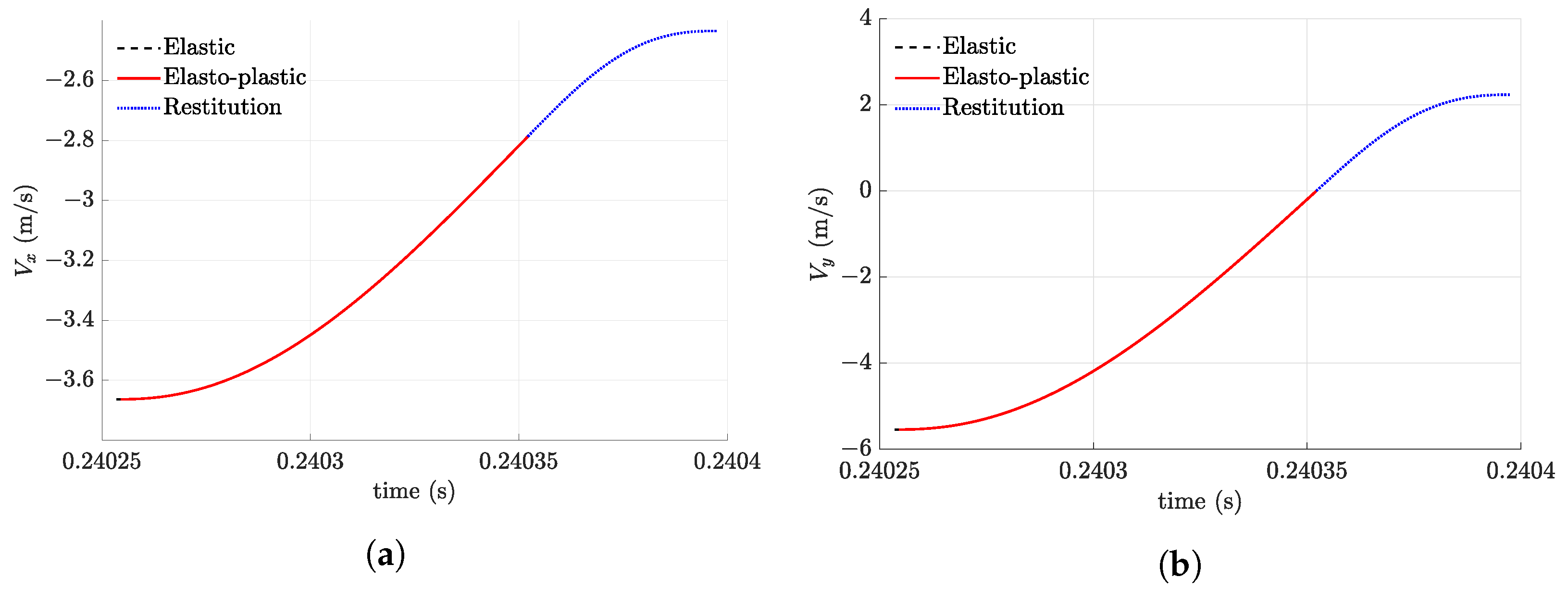
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Newton, I. Philosophiae naturalis principia mathematica; Londini, Jussu Societatis Regiæ ac Typis Josephi Streater; Prostat apud plures Bibliopolas: Washington, DC, USA, 1687. [Google Scholar]
- Poisson, S. Mechanics; Trans. HH Harte: London, UK, 1817; Volume II. [Google Scholar]
- Stronge, W. Unraveling paradoxical theories for rigid body collisions. J. Appl. Mech. 1991, 58, 1049–1055. [Google Scholar] [CrossRef]
- Blazejczyk-Okolewska, B.; Kapitaniak, T. Dynamics of impact oscillator with dry friction. Chaos Solitons Fract. 1996, 7, 1455–1459. [Google Scholar] [CrossRef]
- Chatterjee, A.; Ghaednia, H.; Bowling, A.; Brake, M. Estimation of impact forces during multi-point collisions involving small deformations. Multibody Syst. Dyn. 2021, 51, 45–90. [Google Scholar] [CrossRef]
- Green, I. The prediction of the coefficient of restitution between impacting spheres and finite thickness plates undergoing elastoplastic deformations and wave propagation. Nonlinear Dyn. 2022, 109, 2443–2458. [Google Scholar] [CrossRef]
- Jackson, R.L.; Green, I. A finite element study of elasto-plastic hemispherical contact against a rigid flat. J. Trib. 2005, 127, 343–354. [Google Scholar] [CrossRef]
- Zener, C. The intrinsic inelasticity of large plates. Phys. Rev. 1941, 59, 669. [Google Scholar] [CrossRef]
- Ghaednia, H.; Wang, X.; Saha, S.; Xu, Y.; Sharma, A.; Jackson, R.L. A review of elastic–plastic contact mechanics. Appl. Mech. Rev. 2017, 69, 060804. [Google Scholar] [CrossRef]
- Chen, W.; Jin, M.; Lawal, I.; Brake, M.R.; Song, H. Measurement of slip and separation in jointed structures with non-flat interfaces. Mech. Syst. Signal Process. 2019, 134, 106325. [Google Scholar] [CrossRef]
- Corral, E.; Moreno, R.G.; García, M.G.; Castejón, C. Nonlinear phenomena of contact in multibody systems dynamics: A review. Nonlinear Dyn. 2021, 104, 1269–1295. [Google Scholar] [CrossRef]
- Khulief, Y. Modeling of impact in multibody systems: An overview. J. Comput. Nonlinear Dyn. 2013, 8, 021012. [Google Scholar] [CrossRef]
- Velez-Lopez, G.C.; Vazquez-Leal, H.; Hernandez-Martinez, L.; Sarmiento-Reyes, A.; Diaz-Arango, G.; Huerta-Chua, J.; Rico-Aniles, H.D.; Jimenez-Fernandez, V.M. A Novel Collision-Free Homotopy Path Planning for Planar Robotic Arms. Sensors 2022, 22, 4022. [Google Scholar] [CrossRef]
- Gottschlich, S.N.; Kak, A.C. A dynamic approach to high-precision parts mating. In Proceedings of the 1988 IEEE International Conference on Robotics and Automation, Philadelphia, PA, USA, 24–29 April 1988; pp. 1246–1253. [Google Scholar]
- Walker, I.D. Impact configurations and measures for kinematically redundant and multiple armed robot systems. IEEE Trans. Robot. Autom. 1994, 10, 670–683. [Google Scholar] [CrossRef]
- Aghili, F. Control of redundant mechanical systems under equality and inequality constraints on both input and constraint forces. J. Comput. Nonlinear Dyn. 2011, 6, 031013. [Google Scholar] [CrossRef]
- Marhefka, D.W.; Orin, D.E. A compliant contact model with nonlinear damping for simulation of robotic systems. IEEE Trans. Syst. Man Cybern.-Part A Syst. Hum. 1999, 29, 566–572. [Google Scholar] [CrossRef]
- Mu, X.; Wu, Q. On impact dynamics and contact events for biped robots via impact effects. IEEE Trans. Syst. Man Cybern. Part B (Cybern.) 2006, 36, 1364–1372. [Google Scholar] [CrossRef]
- Konno, A.; Myojin, T.; Matsumoto, T.; Tsujita, T.; Uchiyama, M. An impact dynamics model and sequential optimization to generate impact motions for a humanoid robot. Int. J. Robot. Res. 2011, 30, 1596–1608. [Google Scholar] [CrossRef]
- Nenchev, D.N.; Yoshida, K. Impact analysis and post-impact motion control issues of a free-floating space robot subject to a force impulse. IEEE Trans. Robot. Autom. 1999, 15, 548–557. [Google Scholar] [CrossRef]
- Flores, P. Contact mechanics for dynamical systems: A comprehensive review. Multibody Syst. Dyn. 2022, 54, 127–177. [Google Scholar] [CrossRef]
- Tornambe, A. Modeling and control of impact in mechanical systems: Theory and experimental results. IEEE Trans. Autom. Control 1999, 44, 294–309. [Google Scholar] [CrossRef]
- Lee, E.; Park, J.; Loparo, K.A.; Schrader, C.B.; Chang, P.H. Bang-bang impact control using hybrid impedance/time-delay control. IEEE/ASME Trans. Mechatron. 2003, 8, 272–277. [Google Scholar]
- Pagilla, P.R.; Yu, B. A stable transition controller for constrained robots. IEEE/ASME Trans. Mechatron. 2001, 6, 65–74. [Google Scholar] [CrossRef]
- Hurmuzlu, Y. Complementarity Relationships and Critical Configurations in Rigid-Body Collisions of Planar Kinematic Chains With Smooth External Contacts. J. Appl. Mech. 2020, 87, 121004. [Google Scholar] [CrossRef]
- Aouaj, I.; Padois, V.; Saccon, A. Predicting the post-impact velocity of a robotic arm via rigid multibody models: An experimental study. In Proceedings of the 2021 IEEE International Conference on Robotics and Automation (ICRA), Xi’an, China, 30 May–5 June 2021; pp. 2264–2271. [Google Scholar]
- Yoshida, K.; Mavroidis, C.; Dubowsky, S. Impact dynamics of space long reach manipulators. In Proceedings of the IEEE International Conference on Robotics and Automation, Minneapolis, MN, USA, 22–28 April 1996; Volume 2, pp. 1909–1916. [Google Scholar]
- Bhasin, S.; Dupree, K.; Dixon, W. Control of Robotic Systems Undergoing a Non-Contact to Contact Transition. In Robot Manipulators; IntechOpen: London, UK, 2008. [Google Scholar]
- Liang, C.H.; Bhasin, S.; Dupree, K.; Dixon, W.E. A force limiting adaptive controller for a robotic system undergoing a noncontact-to-contact transition. IEEE Trans. Control Syst. Technol. 2009, 17, 1330–1341. [Google Scholar] [CrossRef]
- Cox, A.; Razzaghi, P.; Hurmuzlu, Y. Feedback linearization of inertially actuated jumping robots. Actuators 2021, 10, 114. [Google Scholar] [CrossRef]
- Saunders, B.E.; Vasconcellos, R.; Kuether, R.; Abdelkefi, A. Characterization and interaction of geometric and contact/impact nonlinearities in dynamical systems. Mech. Syst. Signal Process. 2022, 167, 108481. [Google Scholar] [CrossRef]
- Shinbrot, T.; Grebogi, C.; Wisdom, J.; Yorke, J.A. Chaos in a double pendulum. Am. J. Phys. 1992, 60, 491–499. [Google Scholar] [CrossRef]
- Lenci, S.; Rega, G. Numerical control of impact dynamics of inverted pendulum through optimal feedback strategies. J. Sound Vib. 2000, 236, 505–527. [Google Scholar] [CrossRef]
- Pagilla, P.R.; Yu, B. An experimental study of planar impact of a robot manipulator. IEEE/ASME Trans. Mechatron. 2004, 9, 123–128. [Google Scholar] [CrossRef]
- Marghitu, D.B.; Zhao, J. Impact of a multiple pendulum with a non-linear contact force. Mathematics 2020, 8, 1202. [Google Scholar] [CrossRef]
- Lankarani, H.M.; Nikravesh, P.E. A contact force model with hysteresis damping for impact analysis of multibody systems. In Proceedings of the International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Los Angeles, CA, USA, 6–10 February 1989; American Society of Mechanical Engineers: New York, NY, USA, 1989; Volume 3691, pp. 45–51. [Google Scholar]
- Modarres Najafabadi, S.A.; Kövecses, J.; Angeles, J. Impacts in multibody systems: Modeling and experiments. Multibody Syst. Dyn. 2008, 20, 163–176. [Google Scholar] [CrossRef]
- Gheadnia, H.; Cermik, O.; Marghitu, D.B. Experimental and theoretical analysis of the elasto-plastic oblique impact of a rod with a flat. Int. J. Impact Eng. 2015, 86, 307–317. [Google Scholar] [CrossRef]
- Jackson, R.L.; Green, I.; Marghitu, D.B. Predicting the coefficient of restitution of impacting elastic-perfectly plastic spheres. Nonlinear Dyn. 2010, 60, 217–229. [Google Scholar] [CrossRef]
- Pennestrì, E.; Rossi, V.; Salvini, P.; Valentini, P.P. Review and comparison of dry friction force models. Nonlinear Dyn. 2016, 83, 1785–1801. [Google Scholar] [CrossRef]
- Kim, T.; Rook, T.; Singh, R. Effect of smoothening functions on the frequency response of an oscillator with clearance non-linearity. J. Sound Vib. 2003, 263, 665–678. [Google Scholar] [CrossRef]
- Mostaghel, N. A non-standard analysis approach to systems involving friction. J. Sound Vib. 2005, 284, 583–595. [Google Scholar] [CrossRef]
- Akhan, A.F.; Cojocaru, D.; Marghitu, D.B. Kinetic energy for the frictional impact of two link chain. Int. J. Impact Eng. 2023, 179, 104655. [Google Scholar] [CrossRef]
- Najafabadi, S.A.M.; Kövecses, J.; Angeles, J. Generalization of the energetic coefficient of restitution for contacts in multibody systems. J. Comput. Nonlinear Dyn. 2008, 3, 041008. [Google Scholar] [CrossRef]


| Robotic Arm | Fixed Surface | ||
|---|---|---|---|
| 7800 (kg/m) | 7800 (kg/m) | ||
| E | 210 (GPa) | E | 210 (GPa) |
| 0.29 | 0.29 | ||
| 1.12 (GPa) | 1.12 (GPa) | ||
| 0.2 | 0.2 | ||
| L | 1 (m) | ||
| R | 0.005 (m) | ||
| m | 1 (kg) | ||
| Control Gains | Initial Position | Target Position | |||
|---|---|---|---|---|---|
| 600 (N m/rad) | |||||
| 300 (N m/rad) | |||||
| 100 (N m/rad) | 0 | ||||
| 100 (N m/rad) | 0 | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Akhan, A.F.; Zhao, J.; Tarnita, D.; Marghitu, D.B. Repeated Collision of a Planar Robotic Arm with a Surface Using Generalized Active Forces. Machines 2023, 11, 773. https://doi.org/10.3390/machines11080773
Akhan AF, Zhao J, Tarnita D, Marghitu DB. Repeated Collision of a Planar Robotic Arm with a Surface Using Generalized Active Forces. Machines. 2023; 11(8):773. https://doi.org/10.3390/machines11080773
Chicago/Turabian StyleAkhan, Ahmet Faruk, Jing Zhao, Daniela Tarnita, and Dan B. Marghitu. 2023. "Repeated Collision of a Planar Robotic Arm with a Surface Using Generalized Active Forces" Machines 11, no. 8: 773. https://doi.org/10.3390/machines11080773
APA StyleAkhan, A. F., Zhao, J., Tarnita, D., & Marghitu, D. B. (2023). Repeated Collision of a Planar Robotic Arm with a Surface Using Generalized Active Forces. Machines, 11(8), 773. https://doi.org/10.3390/machines11080773









