Dynamics-Based Thermal Analysis of High-Speed Angular Contact Ball Bearings with Under-Race Lubrication

Abstract

The paper mainly studied the temperature rise characteristics of under-race lubricated high-speed angular contact ball bearings under operational conditions from the perspective of dynamics. The steady-state calculation model of the bearing was established using a thermal network method in consideration of the influence factor of friction power consumption in bearing components based on a dynamic model. Following this, the steady-state change characteristics of the bearing were obtained by solving a thermal balance equation. Through this process, the influence laws of bearing rotation speed, oil supply, and environmental temperature on the friction temperature rise of the bearing were analyzed. Finally, the finite element analysis software ANSYS was employed to provide comparative verification. The results showed that the bearing temperature nonlinearly increased with the increase in inner ring rotation speed, and when it approached a certain critical value, the outer ring temperature exceeded the inner ring temperature. It had an obvious effect on controlling the temperature rise of the bearing inner ring by way of increasing the quantity and reducing the temperature of the lubricating oil supply. Comparative verification showed that the speed–temperature variation tendency from the dynamics-based thermal analysis well agrees with that of the finite element analysis.

1. Introduction

The big thrust-to-weight ratio and high power output of contemporary advanced aero engines are the current design and development trends. High-speed spindles present new issues and higher requirements for spindle bearing lubrication. The bearing produces a lot of heat when it operates regularly, including heat from friction caused by different friction pairs and heat from the viscous dissipation of lubricants. Some academics have proposed the use of under-race lubrication, which uses less oil and has positive effects on lowering the temperature in the bearing cavity, to optimize the heat dissipation of bearings at a high velocity of rotation. Therefore, it is important to research the under-race lubricated angular contact ball bearings’ characteristics of temperature rise.

Gupta et al. [1] proposed a dynamic system based on ordinary differential equations to study the motion of bearing rolling elements. They studied the interaction between rolling element cages and raceway cages in theory and experiments and considered the cage imbalance and the dynamic pressure of the oil film between the guide ring and the cage. Han et al. [2] established a series of nonlinear dynamic models to study the slip behavior of angular contact bearings and roller bearings and the vibration characteristics of the rotor-bearing system. Through a combination of dynamic analysis and thermal elastohydrodynamic lubrication studies, Wang et al. [3] investigated the effects of rotational speed and external load on oil film thickness, temperature, and power loss. Palmgren et al. [4] carried out friction torque tests using different kinds and sizes of bearings and obtained the empirical formula of bearing friction torque by regression analysis and fitting the collected test data. On the basis of Palmgren’s method, Harris et al. [5] adjusted the general calculation method of bearing friction power consumption using an experiment and presented a local heat generation calculation method taking the six elements of rolling bearing friction into account. Wang et al. [6] obtained the temperature rise distribution of the bearing by calculating the heat generation and heat transfer of the bearing and carrying out finite element simulation. Sun et al. [7] analyzed the influence of speed on bearing temperature and studied the relationship between oil–air lubricated oil inlet speed and bearing heat generation. Yan et al. [8,9] focused on the friction loss and temperature rise of the bearing system. Bian et al. [10] studied the influence of bearing temperature on bearing axial stiffness. Using the finite element method, Tarawneh et al. [11] investigated the temperature distribution of the bearing components, friction heat generation, and surface temperature of the bearing box. Gentle et al. [12] considered the sliding state of high-speed ball bearings under the lubrication conditions of rich oil and poor oil. The test results show that a proper degree of poor oil can improve the overall sliding degree of bearings, which may be due to the reduction in lubricating oil drag resistance. Nicolas et al. [13] used the thermal network method in the temperature field analysis of sliding bearings. Jeng et al. [14] studied the effect of preload, oil quantity, air flow rate, and rotational speed on bearing temperature rise based on the oil–gas lubrication experiment of high-speed ball bearings. Flouros et al. [15] obtained the correlation between bearing heat generation and outer ring temperature after testing ball bearings and bearing chambers of a turbine engine and considering the influence of oil inlet direction and axial load direction. According to the heat transfer experiment of an aero engine bearing box and by simulating the oil condition in a typical high-temperature bearing of a turboshaft engine, Yuan [16] obtained the heat transfer law in the bearing box and the influence of the heat transfer coefficient on the bearing DN value (DN > 3 × 10⁶, bore diameter (mm) × rotating speed (rpm)), lubricating oil flow, and oil supply temperature. Taking into account the effect of lubricating oil and thermal expansion, Zheng et al. [17,18] established a thermal grid analysis model of bearings and analyzed the factors that caused the temperature rise of bearings. Liu et al. [19] used the finite element software ANSYS simulation and verified the influence of load and speed on bearing temperature rise. Hu et al. [20] completed the temperature field analysis of angular contact ball bearings by finite element method and carried out experimental verification. It was concluded that the ball temperature among the bearing components was the highest, followed by the inner ring temperature, and the outer ring temperature was the lowest. Qin et al. [21] analyzed the main forms of heat transfer of rolling bearings and gave the heat generation calculation formulas of each element of rolling bearings. Chen et al. [22] analyzed the generation of each heat source of ball bearing and concluded that the rotational speed, axial load, and curvature radius coefficient of the inner ring groove had the main effect on the heat generation. Liang et al. [23] established the heat transfer model of hot nodes of rolling bearings and calculated the temperature of each node in consideration of the effect of bearing speed, load, centrifugal force, and spin on temperature rise. The results show that the heat generation of angular contact ball bearings is positively correlated with bearing rotation, load, centrifugal force, and spin. Ma et al. [24] established the friction torque model and the heat generation model of bearing, and then studied the influence of thread structure parameters and working condition parameters on friction heat generation. Zhang et al. [25] established the total needle bearing equation of a rocker arm on the basis of a dynamic analysis of rolling bearings and then studied the influence of working conditions on friction power consumption and the lubricant convection coefficient in consideration of the traction coefficient of the FVA-M reference lubricant.

In the above references, research on the temperature distribution of under-race lubrication bearing is rare, and there is a lack of research on the temperature distribution through dynamic analysis. In order to study the temperature characteristics of under-race lubrication bearing, this paper solves the dynamic equations and adopts the variable step integral algorithm (gear stiff) to carry out dynamic simulation analysis, thus obtaining the basic friction power consumption for thermal analysis, in which the influence of oil supply temperature, oil supply amount, and bearing speed change on bearing temperature is also considered. It provides a theoretical basis for controlling the temperature of angular contact ball bearings under the ring.

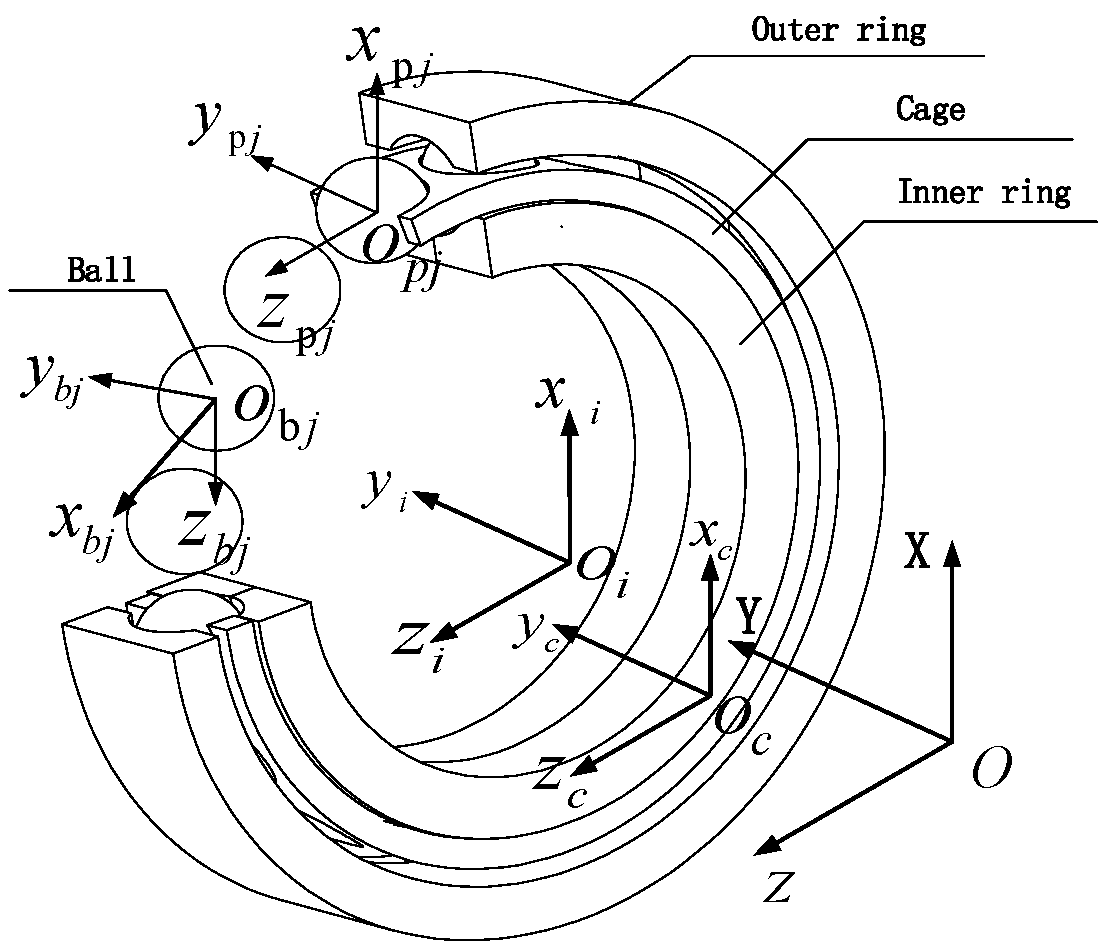
2. Bearing Coordinate System

The research object is an angular contact ball bearing. In the dynamic analysis, it is assumed that the outer ring is connected with the ground, and the cage is guided by the inner ring. The center of mass of each part coincides with the center of shape, and the surface of each part is the ideal surface. The coordinate system can be established, which is shown in Figure 1.

(1) $\left\{O;X;Y;\left.Z\right\}\right.$ is a coordinate system fixed in space, in which the $Y$ axis of the coordinate system coincides with the center line of the bearing, and the $XZ$ plane of the static coordinate system is parallel to the radial plane passing through the center of the bearing.

(2) $\left\{O_{bj};X_{bj};Y_{bj};Z_{bj}\right\}$ is the coordinate system of the center of mass of the rolling body, and J represents the jth rolling body. $O_{bj}$ coincides with the center of the rolling body; $Y_{bj}$ is finally parallel to the Y axis of the static coordinate system; $Z_{bj}$ is along the radial direction of the bearing; $X_{bj}$ is along the circumferential direction of the bearing, and the coordinate system moves along with the steel ball’s center.

(3) $\left\{O_i;X_i;Y_i;Z_i\right\}$ is the coordinate system of the center of mass of the inner ring, $O_i$ coincides with the geometric center of the outer ring, $Y_i$ is along the rotation axis of the inner ring; and the $XZ$ plane is parallel to the radial plane of the center of mass of the inner ring.

(4) $\left\{O_c;X_c;Y_c;Z_c\right\}$ is the centroid coordinate system of the outer ring, $Y_c$ coincides with the rotation axis of the cage, the origin coincides with its centroid. The coordinate system moves along with the centroid of the cage.

(5) $\left\{O_{pj};X_{pj};Y_{pj};Z_{pj}\right\}$ is the coordinate system of the jth pocket of the cage, which is fixed on the cage. $Q_{pj}$ is located in the geometric center of the pocket of the cage. $X_{pj}$ coincides with the radial direction of the bearing, and $Z_{pj}$ is along the circumferential direction of the bearing.

2.1. Differential Equations for Bearing Dynamics

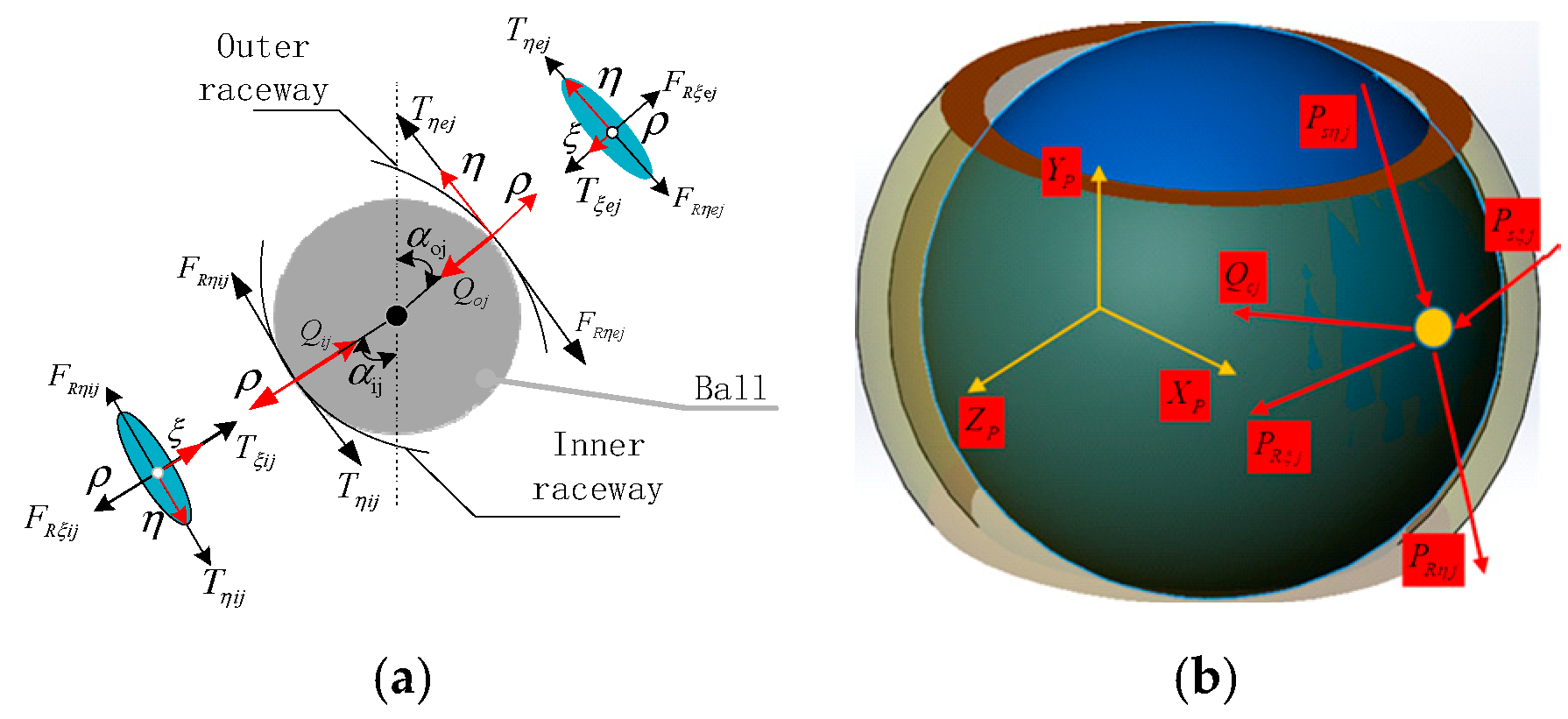
2.1.1. Differential Equations for the Dynamics of Steel Balls

Figure 2 shows the force of the jth steel ball. All calculation methods in the figure can be seen in [26]. The dynamic differential equation of steel balls based on Newton’s law and classic Euler equations is as follows:

$$\begin{array}{l}{Q}_{ej}\sin {\alpha }_{ej}-Q_{ij}\sin {\alpha }_{ij}+T_{\eta ej}\cos {\alpha }_{ej}-T_{\eta ij}\cos {\alpha }_{ij}-F_{R\eta ej}\cos {\alpha }_{ej}\\ +F_{R\eta ij}\cos {\alpha }_{ij}+F_{H\eta ej}\cos {\alpha }_{ej}-F_{H\eta ij}\cos {\alpha }_{ij}+P_{S\xi j}+P_{R\xi j}=m_b{\ddot{x}}_b\end{array}$$

(1)

$$Q_{ej}\cos {\alpha }_{ej}-Q_{ij}\cos {\alpha }_{ij}-T_{\eta ej}\sin {\alpha }_{ej}+T_{\eta ij}\sin {\alpha }_{ij}+F_{R\eta ej}\sin {\alpha }_{ej}-F_{R\eta ij}\sin {\alpha }_{ij}-F_{H\eta ej}\sin {\alpha }_{ej}+F_{H\eta ij}\sin {\alpha }_{ij}-F_{\eta j}-P_{S\eta j}-P_{R\eta j}=m_b{\ddot{y}}_b$$

(2)

$$T_{\xi ij}-T_{\xi ej}-F_{R\xi ij}+F_{R\xi ej}+F_{H\xi ij}-F_{H\xi ej}+Q_{cj}-F_{Dj}-F_{\tau j}=m_b{\ddot{z}}_b$$

(3)

$$(T_{\xi ij}-F_{R\xi ij})\frac{D_w}{2}\cos {\alpha }_{ij}+(T_{\xi ej}-F_{R\xi ej})\frac{D_w}{2}\cos {\alpha }_{ej}-(P_{S\eta j}+P_{R\eta j})\frac{D_w}{2}=I_b{\dot{\omega }}_{bx}+J_x{\dot{\omega }}_{xj}$$

(4)

$$(F_{R\xi ij}-T_{\xi ij})\frac{D_w}{2}\sin {\alpha }_{ij}+(F_{R\xi ej}-T_{\xi ej})\frac{D_w}{2}\sin {\alpha }_{ej}-G_{yj}-(P_{S\xi }{}_j+P_{R\xi j})\frac{D_w}{2}-J_y{\dot{\omega }}_{yj}=I_b{\dot{\omega }}_{by}-I_b{\omega }_{bjz}{\dot{\theta }}_{bj}$$

(5)

$$(T_{\eta ij}-F_{R\eta ij})\frac{D_w}{2}+(T_{\eta ej}-F_{R\eta ej})\frac{D_w}{2}-G_{zj}-J_z{\dot{\omega }}_{zj}=I_b{\dot{\omega }}_{bz}+I_b{\omega }_{by}{\dot{\theta }}_b$$

(6)

In the formula, the subscripts $\eta$ and $\xi$, respectively, represent the short and long semi-axes of the ellipse where the steel ball contacts the raceway; $m_b$ is the mass of the steel ball; ${\mathrm{T}}_{\eta ej}$, $T_{\eta ij}$, $T_{\xi ej}$, and $T_{\xi ij}$ are the drag force in the direction of the long and short axis at the contact between the steel ball and the inner and outer raceways; $F_{r\eta ej}$, $F_{r\eta ij}$, $F_{r\xi ej}$, and $F_{r\xi ij}$ are the hydrodynamic friction forces in the contact entrance area between the steel ball and the raceway; $F_{h\eta ej}$, $F_{h\eta ij}$, $F_{h\xi ej}$, and $F_{h\xi ij}$ are all horizontal components of hydrodynamic force acting on the center of the steel ball; $P_{s\eta j}$ and $P_{s\xi j}$ are the sliding friction forces on the surface of steel balls; $P_{r\eta j}$ and $P_{r\xi j}$ are the rolling friction forces on the steel ball surface; $F_{\mathrm{d}j}$ is the aerodynamic resistance of the oil–gas mixture to a single steel ball; $Q_{cj}$ is the collision force between the steel ball and the cage; $F_{nj}$ and $F_{\tau j}$ are the inertial force component during the movement of the steel ball; $G_{yj}$ and $G_{zj}$ are the components of the moment of inertia during the motion of the steel ball in the inertial coordinate system; $J_x$, $J_y$, and $J_z$ are the components of the moment of inertia of the steel ball in the inertial coordinate system; $I_b$ is the moment of inertia of the steel ball; ${\omega }_{bjx}$, ${\omega }_{bjy}$, and ${\omega }_{bjz}$ are the components of angular velocity of the steel ball in the inertial coordinate system, respectively; ${\dot{\omega }}_{bjx}$,${\dot{\omega }}_{bjy}$, and ${\dot{\omega }}_{bjz}$ are the components of angular acceleration of the steel ball in the inertial coordinate system, respectively; ${\ddot{x}}_{bj}$, ${\ddot{y}}_{bj}$, and ${\ddot{z}}_{bj}$ are the components of the acceleration of the steel ball centroid in the inertial coordinate system; and $\dot{\theta }$ is the rotation speed of the steel ball.

2.1.2. Dynamic Differential Equation of Cage

In the formulas, $m_c$ is the mass of the cage; $Q_{cxj}$, $Q_{cyj}$, and $Q_{czj}$ are the components of the collision force between the steel ball and the cage in the $X$, $Y$, and $Z$ directions in the inertial coordinate system, respectively; ${\phi }_j$ is the position angle of the jth steel ball; $d_m$ is the diameter of the bearing pitch; ${\ddot{x}}_c$, ${\ddot{y}}_c$, and ${\ddot{z}}_c$ are the displacement accelerations of the cage in the inertial coordinate system; $I_{cx}$, $I_{cy}$, and $I_{cz}$ are the moments of inertia of the cage in the inertial coordinate system; ${\omega }_{cx}$, ${\omega }_{cy}$, and ${\omega }_{cz}$ are the angular velocities of the cage in the inertial coordinate system; and ${\dot{\omega }}_{cx}$, ${\dot{\omega }}_{cy}$, and ${\dot{\omega }}_{cz}$ are the angular accelerations of the cage in the inertial coordinate system.

$${\displaystyle \sum _{j=1}^Z(P_{S\eta j}+P_{R\eta j}+Q_{cxj})}=m_c{\ddot{x}}_c$$

(7)

$${\displaystyle \sum _{j=1}^Z\left[(P_{S\xi j}+P_{R\xi j})\cos {\phi }_j+Q_{cyj}\right]}=m_c{\ddot{y}}_c$$

(8)

$${\displaystyle \sum _{j=1}^Z\left[(P_{S\xi j}+P_{R\xi j})\sin {\phi }_j-Q_{cz}{}_j\right]}=m_c{\ddot{z}}_c$$

(9)

$${\displaystyle \sum _{j=1}^Z\left[(P_{S\xi j}+P_{R\xi j})\frac{D_w}{2}-Q_c{}_j\frac{d_m}{2}\right]}=I_{cx}{\dot{\omega }}_{cx}-(I_{cy}-I_{cz}){\omega }_{cy}{\omega }_{cz}$$

(10)

$${\displaystyle \sum _{j=1}^Z(P_{S\eta j}+P_{R\eta j})\frac{d_m}{2}}\sin {\phi }_j=I_{cy}{\dot{\omega }}_{cy}-(I_{cz}-I_{cx}){\omega }_{cz}{\omega }_{cx}$$

(11)

$${\displaystyle \sum _{j=1}^Z(P_{S\eta j}+P_{R\eta j})\frac{d_m}{2}}\cos {\phi }_j=I_{cz}{\dot{\omega }}_{cz}-(I_{cx}-I_{cy}){\omega }_{cx}{\omega }_{cy}$$

(12)

2.1.3. Differential Equation of Inner Ring Dynamics

The bearing inner ring is mainly affected by the normal contact force, drag force, and hydrodynamic friction force of the contact inlet area of the steel ball to the inner ring.

$$F_x+{\displaystyle \sum _{j=1}^Z\left(Q_{ij}\sin {\alpha }_{ij}-F_{R\eta ij}\cos {\alpha }_{ij}\right)}=m_i{\ddot{x}}_i$$

(13)

$$F_y+{\displaystyle \sum _{j=1}^Z\left[(Q_{ij}\cos {\alpha }_{ij}+F_{R\eta ij}\sin {\alpha }_{ij})\cos {\phi }_j+(T_{\xi ij}-F_{R\xi ij})\sin {\phi }_j\right]}=m_i{\ddot{y}}_i$$

(14)

$$F_z-{\displaystyle \sum _{j=1}^Z\left[(Q_{ij}\cos {\alpha }_{ij}+F_{R\eta ij}\sin {\alpha }_{ij})\sin {\phi }_j+(T_{\xi ij}-F_{R\xi ij})\cos {\phi }_j\right]}=m_i{\ddot{z}}_i$$

(15)

$$M_y+{\displaystyle \sum _{j=1}^Z\left[r_j(Q_{ij}\sin {\alpha }_{ij}-F_{R\eta ij}\cos {\alpha }_{ij})\sin {\phi }_j+\frac{D_W}{2}{f}_i{T}_{\xi ij}\sin {\alpha }_{ij}\cos {\phi }_j\right]}=I_{iy}{\dot{\omega }}_{iy}-(I_{iz}-I_{ix}){\omega }_{iz}{\omega }_{ix}$$

(16)

$$M_z+{\displaystyle \sum _{j=1}^Z\left[r_j(Q_{ij}\sin {\alpha }_{ij}-F_{R\eta ij}\cos {\alpha }_{ij})\cos {\phi }_j-\frac{D_W}{2}{f}_i{T}_{\xi ij}\sin {\alpha }_{ij}\sin {\phi }_j\right]}=I_{iz}{\dot{\omega }}_{iz}-(I_{ix}-I_{iy}){\omega }_{ix}{\omega }_{iy}$$

(17)

where $m_i$ is the mass of the inner ring; ${\ddot{x}}_i$, ${\ddot{y}}_i$, and ${\ddot{z}}_i$ are the inner ring accelerations; $I_{ix}$, $I_{iy}$, and $I_{iz}$ are the main moments of inertia of the inner ring ${\omega }_{ix}$, ${\omega }_{iy}$, and ${\omega }_{iz}$ are the angular velocities of the inner ring; ${\dot{\omega }}_{ix}$, ${\dot{\omega }}_{iy}$, and ${\dot{\omega }}_{iz}$ are the angular accelerations of inner ring; $F_x$, $F_y$, $F_z$, $M_y$, and $M_z$ are the supporting forces and supporting moments acting on the inner ring, respectively; $r_j$ is the radial distance from the contact point between the steel ball and the inner ring raceway to the center of mass of the inner ring; $r_j=0.5d_m-0.5D_w{f}_i\cos {\alpha }_{ij}$. $f_i$ is inner ring raceway curvature radius coefficient. $D_w$ is the diameter of the steel ball. $F_{R\eta ij}$ and $F_{R\xi ij}$ refer to the rolling friction force between the steel ball and the inner ring.

The drag force formula is $T_{\xi ij}=\mu Q_{ij}$, $T_{\xi ij}$ is the drag force between the steel ball and the rolling contact surface, and $Q_{ij}$ is the contact force between the steel ball and the inner ring. The oil film drag coefficient $\mu$ is a variable, which can be obtained by experimentation. In this paper, FVA-M lubricating oil provided by Schaeffler Company in Germany is used, and the formula for calculating the drag coefficient of oil film is fitted from the experimental data according to the Gupta four-parameter model. The FVA-M lubricating oil drag coefficient measured on the tester in reference [27] can be expressed as the following formula:

$$\mu =(A+BS)e^{-CS}+D$$

(18)

$$\left\{\begin{array}{l}A=-0.5164{\overline{W}}^{0.7102}{\overline{U}}^{0.5353}{\overline{T}}^{-0.4159}\\ B=4.6747\times {10}^4{\overline{W}}^{-0.0725}{\overline{U}}^{0.2831}{\overline{T}}^{0.079635}\\ C=3.4129\times {10}^{-6}{\overline{W}}^{-0.021}{\overline{U}}^{0.274}{\overline{T}}^{-0.4796}\\ D=0.5164{\overline{W}}^{0.7102}{\overline{U}}^{0.5353}{\overline{T}}^{-0.4159}\end{array}\right.$$

(19)

where: $\overline{W}$ is a dimensionless load parameter; $\overline{U}$ is a dimensionless velocity parameter; $\overline{T}$ is a dimensionless temperature parameter; and $S$ is the slip–roll ratio at the contact between bearing elements.

3. Power Consumption Analysis Model of Bearing Assembly

Bearing Frictional Power Consumption

This paper uses the local method for calculation. For the local method, on the basis of Palmgren’s work, Harris et al. [5] modified the overall algorithm of bearing friction power consumption and proposed a local heat generation calculation method in consideration of the six factors of ball bearing friction power consumption. The friction power consumption of the angular contact ball bearing is comprised of ball-to-race interaction, ball-to-cage contact, cage-to-race contact, elastic hysteresis in the steel ball in a raceway, and churning loss and drag loss.

Friction Power Consumption Caused by Elastic Lag between Steel Ball and Raceway:

$$H_{Ei(e)}={\displaystyle \sum _{j=1}^Z{M}_{Ei(e)j}{\omega }_{bj}}$$

(20)

$$M_{Ei(e)j}=\frac{D_m}{4}(1-{\gamma }_0^2){\phi }_{i(e)j}\beta$$

(21)

$${\gamma }_0=D_w\cos {\alpha }_0/d_m$$

(22)

where ${\alpha }_0$ is the original contact angle; $\beta$ is the elastic hysteresis coefficient and is equal to 0.007; ${\omega }_{bj}$ is the rotation angular velocity of the jth steel ball; and $Z$ is the number of steel balls. M_i(j) is the friction torque generated by the bearing, and H_i(j) is the friction power consumption generated by the bearing.

$${\phi }_{i\left(e\right)j}=\frac{9}{128}\times {\left(\frac{2k}{\pi \mu }\right)}_{i\left(e\right)}\times \frac{1}{V_{i\left(e\right)}}\times {\left(\frac{8}{3}{\displaystyle \sum {\rho }_{i\left(e\right)}}\right)}^{2/3}\times {E^{\prime }}^{1/3}{F}_{i\left(e\right)j}^{4/3}$$

(23)

$${\left(\frac{2k}{\pi \mu }\right)}_{i\left(e\right)}=\frac{2K_{i\left(e\right)}\left(e\right)}{\pi }{\left(\frac{\pi }{2k_{i\left(e\right)}^2{L}_{i\left(e\right)}\left(e\right)}\right)}^{1/3}$$

(24)

$$V_{i\left(e\right)}={\left(\frac{2L_{i\left(e\right)}\left(e\right)}{\pi k_{i\left(e\right)}}\right)}^{1/3}$$

(25)

$$k_{i\left(e\right)}=\frac{a_{i\left(e\right)}}{b_{i\left(e\right)}}$$

(26)

$$E^{\prime }=\frac{4{\left(1-{\mu }_b\right)}^2}{E_b}+\frac{4{\left(1-{\mu }_{i\left(e\right)}\right)}^2}{E_{i\left(e\right)}}$$

(27)

where $F_{ij}$ and $F_{ej}$ are the normal contact forces between the jth steel ball and the inner and outer ring raceways, respectively; $K_i{}_{\left(e\right)}\left(e\right)$ and $L_i{}_{\left(e\right)}\left(e\right)$ are elliptic integrals of the first and second kinds, respectively; ${\mu }_b$ is the Poisson’s ratio of steel balls; ${\mu }_i{}_{\left(e\right)}$ is the Poisson’s ratio of the inner and outer rings of the bearing; $a_i{}_{\left(e\right)}$ is the major semi-axis of the ellipse between the steel ball and the inner and outer rings; $b_i{}_{\left(e\right)}$ is the short semi-axis of the ellipse in contact between the steel ball and the inner and outer rings; $E_b$ is the elastic modulus of the steel ball; $E_i{}_{\left(e\right)}$ is the elastic modulus of the inner and outer rings of the bearing; and $\sum {\rho }_i{}_{(e)}$ is the sum of the main curvature of the contact point between the steel ball and the inner and outer rings.

Friction Power Consumption Caused by Differential Sliding between Steel Ball and Raceway [28]:

$$H_{Di(e)}={\displaystyle \sum _{j=1}^Z{M}_{Di(e)j}{\omega }_{bj}}$$

(28)

$$M_{Di(e)j}=\frac{D_m(1-{\gamma }^2)}{2D_w}{\mathrm{\Psi }}_{Di(e)j}{f}_s$$

(29)

$${\mathrm{\Psi }}_{Di(e)j}=0.04025\times \frac{U_{i(e)}}{r_{ci(e)}}\times {(\frac{3E\prime }{8{\displaystyle \sum {\rho }_{i(e)}}})}^{2/3}\times F_{i(e)j}$$

(30)

$$U_{i\left(e\right)}={\left(\frac{2k_{i\left(e\right)}^2{L}_{i\left(e\right)}}{\pi }\right)}^{1/3}$$

(31)

$$r_{ci(e)}=\frac{f_{i(e)}{D}_w}{0.5+f_{i(e)}}$$

(32)

$$r_{ci(e)}=\frac{f_{i(e)}{D}_w}{0.5+f_{i(e)}}$$

(33)

where $f_i{}_{\left(e\right)}$ is the radius coefficient of curvature of the groove of the inner and outer rings of the bearing and $f_s$ is the sliding coefficient of friction.

Friction Power Consumption Caused by Steel Ball Spin Sliding:

$$H_{Si(e)}={\displaystyle \sum _{j=1}^Z{M}_{Si(e)j}}{\omega }_{si(e)j}$$

(34)

$$M_{Si\left(e\right)j}=\frac{3}{8}\times f_s\times {\displaystyle \sum _{}^{}\left(L_{i\left(e\right)}\left(e\right)a_{i\left(e\right)j}{F}_{i\left(e\right)j}\sin {\alpha }_{i\left(e\right)j}\right)}$$

(35)

where ${\alpha }_{i\left(e\right)j}$ is the actual working contact angle between the jth steel ball and the inner and outer ring raceways and ${\omega }_{si(e)j}$ is the jth steel ball spin angular velocity.

Friction Power Consumption Caused by Contact between Cage and Guide Surface:

$$H_{cr}=1.38\times {10}^{-7}W{f}_c{n}_{i(e)c}\epsilon D_{2i(e)}{(1-r)}^2\times {10}^{-3}{\omega }_c$$

(36)

where $n_i{}_{\left(e\right)c}$ is the rotational speed of the ferrule relative to the cage; ${\omega }_c$ is the cage rotation angular velocity; $\epsilon$ offset of the cage center to the bearing center; $D_{2i\left(e\right)}$ is the diameter of the guide ferrule flange; $W$ is the cage weight; $r=D_w\cos {\alpha }_0/d_m$, where ${\alpha }_0$ is the original contact angle.

Friction Power Consumption between Steel Ball and Cage:

$$H_{cb}=\frac{d_m}{4}(1-r^2)\sin \left[{\alpha }_0+arctg\left(\frac{D_w\sin {\alpha }_0}{r_1}\right)\right]W{f}_c{\omega }_b$$

(37)

where $r_1=\frac{d_m}{2}(1-r)$; $W$ is the weight of the cage; and $f_c$ is the coefficient of sliding friction.

Friction Power Consumption Caused by Viscous Loss of Oil Film:

$$H_{oil}=6.35{\alpha }_{oil}{S}_n\frac{d_m}{2}{\displaystyle \sum _{Z=1}^Z\left[\left(\frac{H_e+H_i}{2}\right)(a_e+a_i)\right]}{\omega }_c$$

(38)

where $H_i{}_{\left(e\right)}$ is the thickness of the oil film; ${\alpha }_{oil}$ is the viscosity coefficient; $S_n$ is the sufficient lubrication coefficient. The oil film lubrication coefficient can be taken as $a_i{}_{\left(e\right)}$ which is the contact ellipse major axis.

Total Friction Power Consumption:

The total energy power consumption of angular contact ball bearings caused by friction during operation is:

$$H=H_{Ei(o)}+H_{Di(o)}+H_{Si(o)}+H_{cr}+H_{cb}+H_{oil}$$

(39)

4. Thermal Network Method

During this study, through adopting the under-race lubrication method, the lubricating oil was transported to the inner race through the centrifugal force generated by the high-speed rotation of the shaft system. The friction power consumption caused by the bearing will eventually be dissipated by heat conduction and convection heat exchange when thermal radiation is being ignored. When the thermal network method is used to analyze the steady-state temperature field of the bearing system, the appropriate thermal nodes are selected first, then the heat transfer relationship of each component is determined. The thermal network relationship diagram is then established, and finally the thermal network linear equation system is established. The steady-state temperature values of each node are obtained by solving linear equations.

Table 1. Basic structural parameters of bearings.

Parameters	Symbol	Value
Outer ring diameter	D	62 mm
Inner ring diameter	d	30 mm
Bearing width	B	16 mm
Pitch diameter	dm	46 mm
Contact angle	$\mathrm{\alpha }$	30 °C
Steel ball diameter	Dw	9.525 mm
Number of steel balls	Z	11

shows the basic structural parameters of bearings.

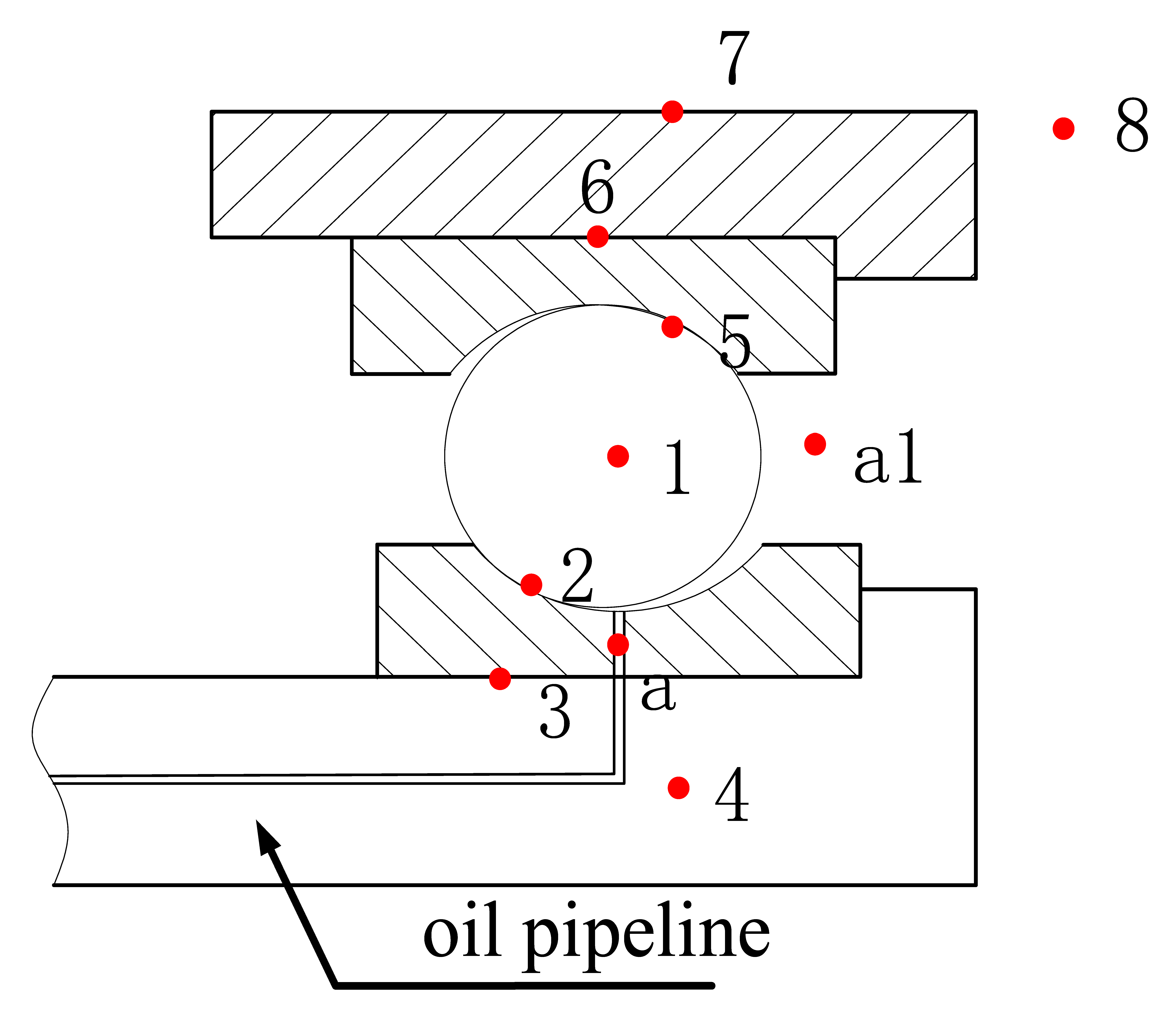
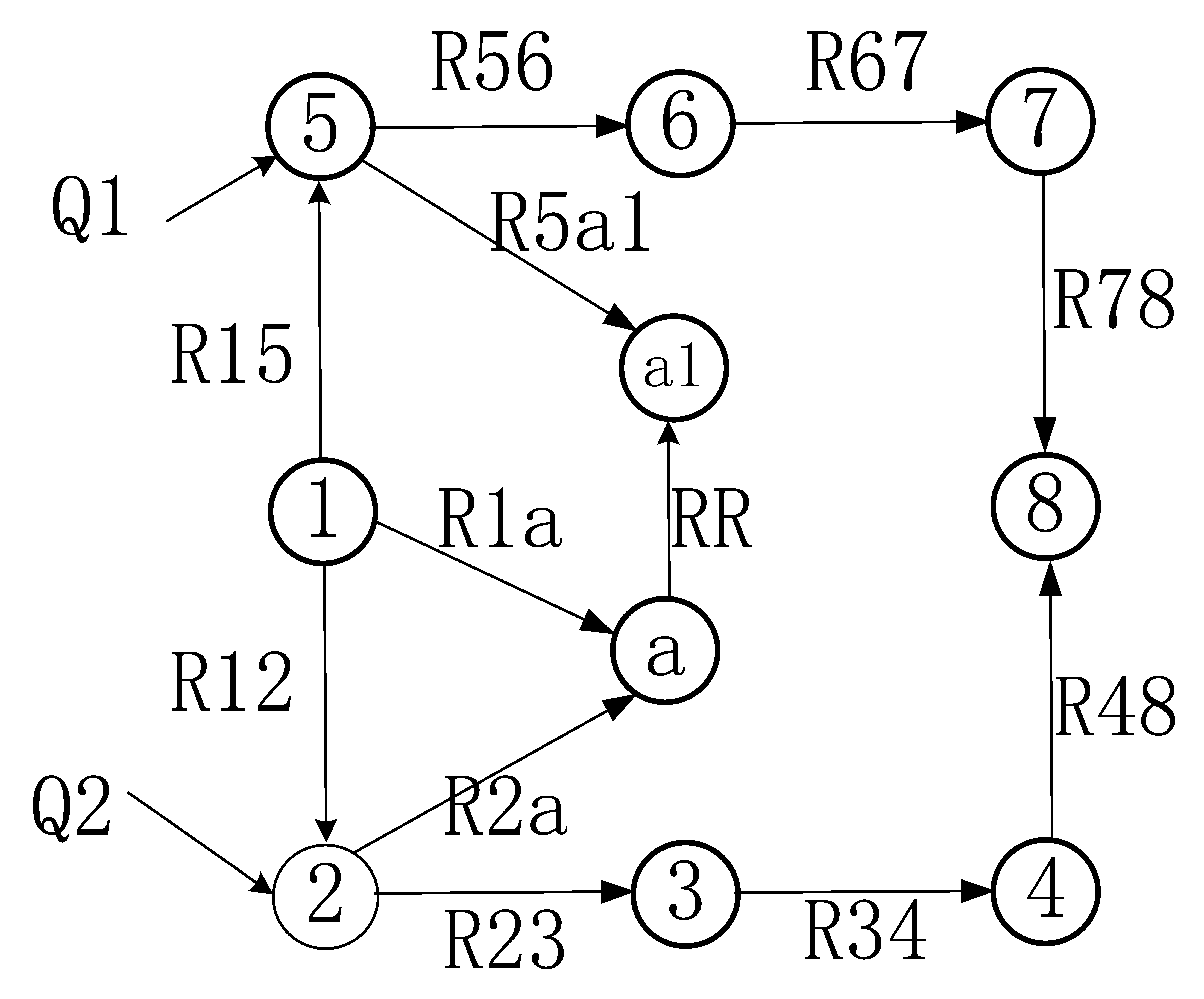
4.1. Node Division of Thermal Network

As shown in Figure 3, 10 thermal nodes are selected in the angular contact ball bearing system, in which node T1 is the steel ball temperature; node T2 is the bearing inner ring raceway contact point temperature; node T3 is the bearing inner ring temperature; node T4 is the spindle temperature; node T5 is the bearing outer ring raceway contact point temperature; node T6 is the bearing housing temperature; and node T7 is the bearing housing temperature. In addition, node T8 and node Ta1, respectively, represent ambient temperature and lubricant temperature.

4.2. Thermal Resistance Calculation

According to the selection of nodes in Figure 3 and the heat transfer relationship between nodes, the thermal network analysis diagram can be obtained as shown in Figure 4.

4.2.1. Thermal Conductivity and Resistance

For the bearing inner ring, outer ring, and bearing block, it can be regarded as circular ring heat conduction, and the calculation formula is as follows [29]:

$$R=\frac{\ln \left(d_o/d_i\right)}{2\pi kl}$$

(40)

where $d_o$ is the outer diameter of the ring, $d_i$ is the inner diameter of the ring, $k$ is the thermal conductivity of the material, and $l$ is the characteristic length. Contact thermal resistance between the steel ball and raceway is [30]:

$$R=\frac{1}{\pi }\left(\frac{a}{b}\right)\frac{1}{ka\sqrt{pe}}$$

(41)

where $a$ and $b$ are steel balls and long and short semi-axes rolling to the contact area, respectively; $k$ is the thermal conductivity; $pe=a\rho V_c{C}_p/k$ is Peclet number, where $V_c$ is characteristic rotation speed and $C_p$ is the specific heat capacity of lubrication.

4.2.2. Convective Thermal Resistance

Convective thermal resistance of a solid surface is usually expressed by the following formula:

$$R=\frac{1}{h_v{S}_d}$$

(42)

where $h_v$ is the surface convective heat transfer coefficient and $S_d$ is the convective heat transfer area. For the solution of $h_v$, please refer to [30].

For the lubricating oil to enter the bearing cavity to absorb energy, the calculation method is as follows [30]:

$$H=(T_{a1}-T_a){\rho }_{oil}{L}_{oil}{C}_p$$

(43)

where $T_{a1}$ is the oil outlet temperature, $T_a$ is the oil inlet temperature, ${\rho }_{oil}$ is the lubricating oil density, and $L_{oil}$ is the lubricating oil flow rate.

The thermal grid method is based on the theory of heat transfer and takes the energy conservation theorem as the core. For establishing the heat balance equation, it is assumed that there is no heat exchange between 2 parts that are not in direct contact [19].

Based on the generalized ohm theorem, when calculating the steady-state heat transfer, the inflow heat of each node is equal to the outflow heat [30].

$$H_f+H_V+H_d=0$$

(44)

where $H_f$ is the bearing heat production; $H_V$ is the heat exchanged by thermal convection; and $H_d$ is the heat exchanged by heat conduction.

The heat balance equation between nodes is [30]:

$${\displaystyle \sum \frac{T_X-T_y}{R_{xy}}}=H_f$$

(45)

where $T_X$ is the temperature between nodes and $R_{xy}$ is the thermal resistance between nodes.

4.3. Establishment of Heat Balance Equations

The heat balance equations can be listed according to the thermal network analysis diagram, in which $Q1$ is the heat generation between the steel ball and the outer raceway and $Q2$ is the heat generated between the steel ball and the inner raceway. Since the heat generated at the contact point of the inner and outer raceways accounts for most of the heat generated by the bearing, the viewpoint put forward by Burton and Steph can make the heat generated at the contact point flow into the ring and the steel ball according to the ratio of 1:1 and at the same time simplify the heat source as the heat generated between the steel ball and the inner and outer raceways. Q1 and Q2 are calculated as follows:

$$\begin{array}{l}Q1={\displaystyle \sum _{j=1}^Z{H}_{rej}}+{\displaystyle \sum _{j=1}^Z{H}_{dej}}+{\displaystyle \sum _{j=1}^Z{H}_{sej}}+0.5(H_{oil}+H_{cL}+H_c)\\ Q2={\displaystyle \sum _{j=1}^Z{H}_{rij}}+{\displaystyle \sum _{j=1}^Z{H}_{dij}}+{\displaystyle \sum _{j=1}^Z{H}_{sij}}+0.5(H_{oil}+H_{cL}+H_c)\end{array}$$

(46)

The linear equations for heat source, thermal resistance, and node temperature are established above, in which the ambient temperature node 8 and the oil supply node Ta1 are known. The Gauss–Seidel iterative method is used to solve the equations and obtain 8 unknown node temperatures.

5. Result

5.1. Calculation and Analysis of Bearing Power Consumption

5.1.1. Influence of Bearing Rotation Speed on Friction Power Consumption

Figure 5 and Figure 6 show the change laws of various types of friction power consumption inside the bearing with the variation in bearing rotation speed. The working conditions of the bearing include an axial load of Fa = 3000 N and a radial load of Fr = 780 N. Figure 5 shows the effect of bearing rotation speed on friction power consumption.

As the rotation speed increases, the various friction power consumptions of the bearing also increase. However, the increase resulting from the rolling element’s spin sliding along the raceway is more noticeable than the increase in other friction power consumptions. The rolling element’s spin-sliding friction is the main cause of the increase in friction power consumption, which is much greater than the friction caused by the elastic hysteresis rolling friction, differential sliding friction, friction between the rolling element and cage, and viscosity loss of oil film.

As shown in Figure 6, the friction power consumption between the inner ring and the balls is greater than that between the outer ring and the balls, and the total friction power consumption of the bearing shows an obvious upward trend with the increase in bearing rotation speed, which is mainly due to the friction of all parts of the bearing increasing with the increase in bearing rotation speed.

5.1.2. Effect of Axial Load on Friction Power Consumption

In order to analyze the influence of different axial loads on friction power consumption, the value of bearing rotation speed is set to 10,000 rpm while the radial load is fixed to the constant 780 N, and the axial load is increased from 500 to 9000 N. The results are shown in Figure 7 and Figure 8.

As can be seen from Figure 7, the greater the axial load, the more the friction power consumption of the bearing increases. Among them, the power consumption caused by the spin–sliding friction of the rolling elements is the largest, accounting for more than 50%, followed by the friction power consumption caused by elastic hysteresis, while the friction power consumption between the cage and the steel ball and between the cage and the guide surface are basically unchanged and account for a small proportion. The reason is that with the increase in axial load, the contact angle increases, which leads to the increase in the spin–sliding of the rolling elements, while the power consumption of other frictions increases slightly.

From Figure 8, it can be seen that on the whole, the friction power consumption between the inner ring and the balls is greater than that between the outer ring and the balls. With the increase in axial load, the total friction power consumption of the bearing is also increased.

5.1.3. Influence of Radial Load on Friction Power Consumption

In order to analyze the influence of different radial loads on friction power consumption, the value of bearing rotation speed is set to 10,000 rpm while the axial load is fixed to the constant 3000 N, and the radial load is increased from 100 to 3100 N. The results are shown in Figure 9 and Figure 10.

From Figure 9, when the radial load increases, the friction power consumption of each part in the bearing does not change obviously. Among them, the friction caused by spin–sliding only increased slightly and accounts for a large proportion, followed by the friction power consumption caused by steel balls and cages, followed by elastic hysteresis and friction power consumption caused by cage guide surfaces. Differential sliding and oil film agitation account for the smallest proportion. The friction power consumption of other parts remained in a relatively stable state.

As can be seen from Figure 10, as the radial load increases, the friction power consumption of the bearing inner and outer rings changes very little, from which it can be concluded that the radial load has little effect on the frictional power consumption of this bearing.

5.2. Temperature Simulation Analysis

For obtaining the relationship between the outer ring temperature and the rotation speed, the initial operating parameters are given as follows: the radial load 780 N, the axial load 3000 N, and the ambient temperature 20 °C. The changing trend of the bearing outer ring temperature is observed.

As can be seen from Figure 11, before the bearing rotation speed reaches the certain critical value at approximately 11,000 rpm, the outer ring temperature does not increase obviously. When the bearing rotation speed increases, the bearing temperature increases more obviously. As this bearing has under-race lubrication, when the temperature of the inner and outer rings rises gradually beyond the oil temperature, the lubricating oil temperature will reduce the temperature of the inner ring. Therefore, when the bearing rotation speed reaches the critical rotation speed, the temperature of the inner and outer rings of the bearing rise sharply, and the temperature of the outer ring exceeds the inner ring temperature.

The operational conditions of the bearing at various ambient temperatures are shown in Figure 12. As can be seen, each bearing node’s steady-state temperature rises as the ambient temperature rises under the conditions of the radial load at 780 N and the axial load at 3000 N.

Figure 13 illustrates the temperature variation trend of the outer ring of the bearing at different lubricating oil temperatures. The radial load of the bearing is fixed at 780 N and the axial load is 3000 N. The bearing rotational speed is from 9000 to 17,000 rpm. The figure shows that the temperature of the bearing outer ring increases with the increase in bearing speed. On the other hand, when the rotational speed is a certain value, the higher the oil supply temperature, the higher the temperature the outer ring will be.

According to Figure 14, while the bearing is operating with a radial load of 780 N and an axial load of 3000 N, the rotation speed change is 9000–17,000 rpm, and the flow rate of oil is 1–10 L/min, allowing us to observe how the bearing’s temperature changes. As can be seen from the figure, when the flow rate of oil is less than 2 L/min, the temperature of the bearing outer ring has an obvious upward trend. It is worth noting that when the oil flow rate allows, the temperature of the bearing outer ring shows a relatively linear change in the range of 2 L/min to 10 L/min of oil quantity. On the other hand, when the bearing rotation speed is fixed, the temperature of the bearing outer ring will not change obviously with the increase in the flow rate of oil.

6. Comparative Verification of the Dynamics-Based Thermal Analysis Results

6.1. Finite Element Comparative Analysis

Based on the finite element analysis theory, the thermal analysis model of the angular contact ball bearing was established using ANSYS software, and the power consumption calculation result was applied to the contact area to obtain the bearing temperature. The solution process is shown in Figure 15.

6.1.1. Establishment of Finite Element Model of Angular Contact Ball Bearing

The angular contact ball bearing’s transition fillet and chamfer are omitted in modeling since they have little impact on the internal stress distribution and deformation. A cuboid is divided in the contact area between the ball and raceway of bearings, and its length and width are roughly equivalent to the long and short axes of the contact ellipse obtained by solving the Hertz contact problem in order to impose boundary conditions in the thermal analysis of bearings and avoid taking the whole raceway or the entire spherical surface as the target surface or contact surface. The model creates a total of 22 contact pairs when each ball develops contact pairs with the inner and outer rings, respectively. In order to reduce the calculation scale and ensure calculation accuracy, local mesh refinement is carried out in the contact area, as shown in the figure. The finite element model adopts eight-node solid 70 elements.

In order to facilitate the analysis, the following assumptions and simplifications were made for the model:

(1) The friction coefficient between contact surfaces, the linear expansion coefficient of materials, thermal conductivity, and other parameters are constant in the whole analysis process.

(2) As the thermal conductivity of the nylon cage is worse than that of bearing steel, its influence is ignored in the model.

(3) Burton and Staph [31] proposed that frictional heat is distributed 1:1 between the bearing ball and the ring, that is, half of the frictional heat enters the ball, and the other half enters the ring.

6.1.2. Influence of Rotation Speed on Bearing Temperature

Using a local heat generation model, friction heat generation model, and the convection coefficient obtained from Equation (42) as boundary conditions for temperature field calculation, bearing temperatures at different rotation speeds can be calculated. Figure 16 shows the distribution of bearing temperature.

Table 2. The comparative results of outer ring temperature from dynamics-based thermal analysis (1) and finite element analysis (2).

Rotation Speed/rpm	Friction Power Consumption of Inner Ring/W	Friction Power Consumption of Outer Ring/W	Outer Ring Temperature (1)/°C	Outer Ring Temperature (2)/°C	Error (%)
9000	33.38	21.19	58.18	63.1	8.73
10,000	35.305	23.65	59.92	64.5	9.04
11,000	39.3	24.1	63.02	68.02	7.93
12,000	43.42	27.65	66.14	71	7.77
13,000	46.57	31.65	69.38	72	10.63
14,000	50.86	34.505	72.97	77.1	8.04
15,000	57.23	38.445	76.34	81.9	5.81
16,000	64.1	40.125	80.5	85	6.83
17,000	69.34	42.125	85.09	90	5.98

shows the comparative analysis of the bearing inner ring temperature resulting from dynamics-based thermal analysis and from finite element simulation.

The comparative analysis of the bearing outer ring temperature under various operating conditions is illustrated in Figure 17. It can be seen that there is good agreement between the results from the finite element simulation and from dynamics-based thermal analysis, with a relative error of 10.63% at the highest and 5.81% at the lowest.

The comparative verifications confirm the accuracy of the dynamics-based thermal analysis results presented in this paper.

7. Conclusions

The angular contact ball bearing was used as the research object in this paper, and under-race lubrication was the chosen as the lubrication method. According to the bearing dynamics calculation, the bearing heat generation was calculated using the integral method. The steady-state temperature field was established using the thermal network method, and the temperature rise of each node under various operating conditions was forecasted and compared. Finite element analysis comparative verifications were carried out to evaluate the correctness of dynamics-based thermal analysis results.

• The results showed the bearing temperature nonlinearly increased with the increase in inner ring rotation speed, and when it approached the certain critical value (approximately 11,000 rpm) the outer ring temperature exceeded the inner ring temperature. It had an obvious effect on controlling the temperature rise of the bearing inner ring by way of increasing the quantity and reducing the temperature of the lubricating oil supply.
• The bearing temperature rise is analyzed by the bearing dynamics theory, and the friction power consumption between the bearing components is calculated more accurately. The friction power consumption of the bearing increases with the change in bearing rotation speed and axial load. On the other hand, the influence of radial load on friction power consumption is not obvious.
• During the high-speed rotation of the angular contact ball bearing with the under-race lubrication, according to the temperature distribution of the outer ring, it is recommended that the flow rate of oil is 2–10 L/min because the temperature variation in the outer ring of the bearing is relatively average, which is more conducive to running with the bearing.
• The temperature of the bearing node was obtained through a dynamic simulation. After comparative analysis, it was concluded that the difference between dynamic simulation analysis and finite element analysis results is less than 11%. The accuracy of this study was verified.