1. Introduction
Permanent magnet synchronous motors (PMSM) are widely used in various industrial fields due to their small size, high efficiency, convenient control, and ample speed regulation. In actual operations, however, its moment of inertia often changes, making the motor control parameters set before the servo system run out of sync with the controlled object during system operation. This leads to a deterioration in both the stability and rapidity of the speed of the servo control system. To maintain a fast and stable response to the system speed, the servo control system must obtain the information on inertia in real time and accordingly adjust the motor control parameters following the inertia parameter information to adapt to the changes in the moment of inertia.
Currently, numerous researchers have proposed equally numerous methods in anti-inertia disturbance, such as PI control [
1,
2,
3,
4], adaptive control [
5,
6,
7,
8], intelligent control [
9,
10,
11,
12], fuzzy control [
13,
14,
15], predictive control [
16,
17,
18], and sliding mode control [
19,
20,
21,
22,
23,
24], among others. Their methods are mainly divided into two categories: added observers and no observers.
There exist numerous studies on the anti-inertia disturbance of adding observers, all of which forward varying methods that effectively improve real-time tracking accuracy. Most studies in the literature [
1,
2] generally use the gradient algorithm to identify the moment of inertia online and make real-time adjustments of the PI parameters of the velocity ring according to the principle of pole configuration. Although the effectiveness of the method can be verified by experiments, simple PI parameter adjustments cannot meet the complex parameter changes of multivariate systems. Other studies [
3,
4] also identify and compensate for the disturbance inertia using the inertia disturbance observer established by the Kalman filter. Said compensation system has good anti-inertia disturbance performance and robustness, but its adjustment of PI parameters still cannot meet the complex parameter change requirements. Some studies [
5,
6] follow the traditional disturbance observer as improved by the variable gain algorithm and are combined with the model reference adaptive algorithm. By then, the identified moment of inertia is updated for the improved disturbance observer, thus effectively improving the system’s anti-disturbance performance. Other studies [
7,
8] use the forgetting factor recursive least squares algorithm to observe the moment of inertia and subsequently design the moment of inertia’s adaptive controller to eliminate the influence of identification error on speed performance. There are also studies [
5,
6,
7,
8] showing good anti-inertia disturbance performance, but all have high hardware requirements and are difficult to implement in engineering.
There are also many studies on anti-inertia disturbances without adding observers; such methods make up for the lack of tracking accuracy by selecting control strategies. Some [
9,
10] propose an anti-inertia velocity control method for adaptive radial basis function networks that achieves high speed tracking accuracy (without current measurement or control) by directly acting on the voltage of the machine. Others [
11,
12] apply neural networks to PMSM control systems with inertia disturbances, simplify the control structure and parameter dependence, and ultimately achieve high servo accuracy under parameter changes. There also exists [
13,
14,
15] various designs wherein a fuzzy inference module was used to apply to the adaptive current error integral-backward-step controller, thereby solving the problem of the influence of inertia disturbance on the system and improving the dynamic response performance of the system. Improved predictive control has also been applied to the PMSM current loop [
16,
17,
18], effectively reducing the sensitivity of the servo system to motor inductance parameters and improving the high dynamic performance of the system. A non-singular fast terminal sliding mode control method has also been adopted [
19] to suppress the influence of moment of inertia, thus ensuring that the speed tracking error converges quickly in a limited time and effectively weakens the speed fluctuation. Global fast terminal sliding mode control was also used [
20] to quickly converge the system state to the equilibrium point, improve the response speed of the system, and enhance the robustness of the system. The note [
21] proposes a robust integral sliding mode (RISM) manifold and a corresponding design method for stabilization control for uncertain systems with control input time delays. An improved sliding mode control, which is delay-dependent and suitable for small input time delays, keeps the system in the neighborhood of the RISM surface in finite time, improving the system’s stability. The authors of [
22] studied fault-tolerant control (FTC) designs based on nonsingular terminal sliding-mode control and nonsingular fast terminal sliding-mode control (NFTSMC). The proposed active FTC laws are shown to be able to achieve fault-tolerant objectives and maintain stabilization performance even when some of the actuators fail to operate. In order to achieve robust tracking performance, a novel sliding-mode control law [
23] is designed in the SMC unit, and the adaptive unit is put forward to deal with uncertain gains. The article [
24] proposes a continuous nonsingular terminal sliding-mode control with integral-type sliding surfaces (CNTSMC-ISS) framework for disturbed systems. Compared with the existing sliding-mode controllers, the noteworthy contributions are the alleviation of the chattering phenomenon, the fast finite-time stability, and the singularity-free and ease-of-implementation characteristics. These methods have also achieved satisfactory results in the experiment, although the lack of real-time observation of inertia cannot meet the requirements of the system for high tracking accuracy.
In order to improve the tracking accuracy of the system to the motor speed, this paper improves the extended state observer to a new inertia observer. By adding a time-varying function, the gain increases slowly, reducing the impact on the motor speed while observing the inertia. To improve the stability and rapidity of the motor speed when the inertia changes, this paper proposes an integral time-varying fast terminal sliding mode control (ITFTSMC) method. In order to shorten the duration of the approaching motion stage and ensure the dynamic quality of the approaching motion, the author improves the exponential approaching law and proposes a new type of approaching law. In order to improve the stability and fast performance of the motor speed, the author designed a new type of integral time-varying module and fast terminal module to improve the conventional sliding mode controller. The integral time-varying module can improve the stability of the motor speed and keep the speed overshoot within a small error range, and the fast terminal module can improve the speed performance of the motor speed so that the speed can recover to stability in a short time. Finally, experiments were designed to verify the effectiveness of the method.
2. Mathematical Model of the Moment of Inertia of PMSM
To solve the influence of the disturbance of the moment of inertia on the speed of the motor operation, the current study starts with the mechanical motion equation of the permanent magnet synchronous motor in the d-q coordinate system. It also obtains the correlation between the moment of inertia and the motor speed through the analysis of this equation.
The mechanical equation of motion of PMSM in the
d-
q coordinate system is shown in Formula (1) below:
where
is the moment of inertia;
is the mechanical angular velocity;
is the electromagnetic torque;
is load torque;
is the damping coefficient.
In the moment of inertia identification algorithm, the sampling frequency of the actual speed of the motor is relatively high; hence, the damping coefficient of the system can be ignored. Therefore, the above formula can be simplified into Formula (2) below:
The logical relationship between motor speed and mechanical angular velocity is shown in Equation (3) below:
where
is the motor speed.
From Equations (2) and (3), the moment of inertia is clearly inversely proportional to the rate of change of the mechanical angular velocity, and the mechanical angular velocity is proportional to the rotational speed; hence, the rate of change of the moment of inertia and the speed are also inversely proportional. As shown in Equation (4):
Obviously, when the load torque remains constant, the moment of inertia becomes a disturbance factor that affects the changes in motor speed. In order to quickly restore stability to the speed, this article identifies the moment of inertia and updates the identification value in real-time to the speed loop, which improves the fast performance and stability of the speed.
3. Inertia Identification Based on Extended State Observer
For the speed to quickly return to a stable state when the moment of inertia changes, accurate and rapid real-time monitoring of the inertia change is required. In this paper, an extended state observer (ESO) is used to identify the inertia online.
The motor angular velocity expression shown in Equation (2) is transformed into:
in the formula,
is the torque constant,
;
is the polar logarithm;
is the permanent magnet flux chain;
is the reference value of inertia;
is the reference current;
is the actual current; and
is the disturbance of the speed loop.
For the motor angular velocity equation shown in Equation (5), the extended state observer is designed as follows:
in the equation,
is the motor reference mechanical angular velocity, is usually a constant, and
is the actual mechanical angular velocity;
,
,
and
are the parameters of the observer, where
,
, and
. The function
is represented as:
where
is the interval length of the linear segment;
is a nonlinear factor; and
is a sign function.
In the observer, the high gain of
can improve the accuracy and bandwidth of the observer. However, when the initial value of the observer is inconsistent with the actual speed of the motor, the high gain of the observer can cause the speed to overshoot, which has a negative impact on speed control. To reduce the impact of speed overshoot, this article utilizes the saturation characteristics of the sgn function and replaces
with a time-varying function
, so that the observer adopts a smaller gain during operation. As time increases, the observer’s gain gradually increases until the time-varying function saturates. The expression of the extended state observer based on time-varying parameters is:
in the formula,
and
are both gain coefficients, where
,
, and
is the set time constant.
This article sets the load torque to remain constant with only changes in inertia, so the speed loop disturbance is caused by changes in inertia. As shown in the following equation, the rate of change of
can also be written as:
where
is the inertia identification value. Equation (10) can be obtained by combining Equations (8) and (9).
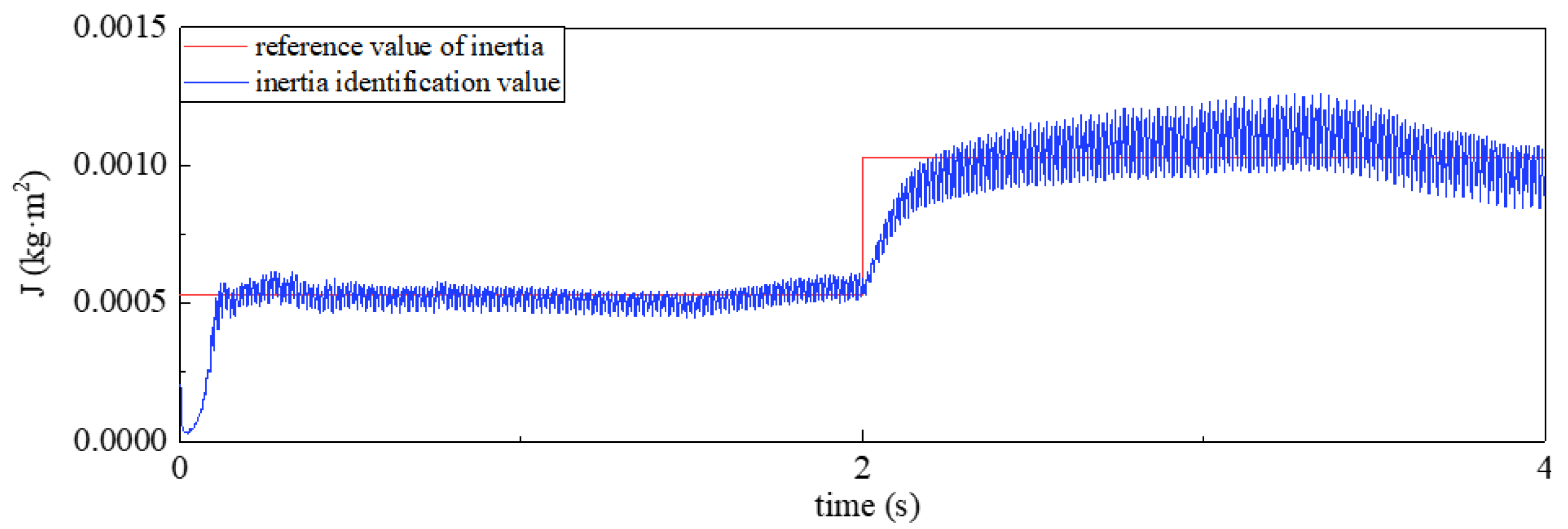
Figure 1 shows the simulation diagram of inertia identification.
As shown in the figure, good observation results can be obtained by using the extended state observer to observe the moment of inertia.
4. Speed Controller Design
To improve the dynamic performance of the speed at the time of inertia change, an integral time-varying fast terminal sliding mode control (ITFTSMC) is designed to be applied to the speed loop.
Figure 2 shows the system control block diagram.
The input of the system was the speed error; the output was the given value of the current; the speed signal of PMSM was fed back through the optical encoder; the current loop adopted PI control; the q-axis current was the velocity ring output value; and the d-axis current was taken as 0. The adjustment and update of ITFTSMC control law parameters was carried out by feeding back to the inertia recognition value of the speed controller, which was derived from the online identification of the improved Inertia observer. The system modulation method adopts the spatial vector pulse width modulation (SVPWM) scheme.
4.1. ITFTSMC Analytical Model
Define the status variables of the PMSM system as:
This is obtained according to Equations (5) and (11):
In order to improve the fast and stable performance of the rotating speed of the motor, a new sliding mode surface is defined in this paper by combining the integral time-varying module and the fast terminal module. The function of the sliding mode surface is shown in Equation (13):
where
are constants,
are Positive odd numbers, and,
,
,
, and
.
The derivation of Formula (13) can be obtained:
To achieve the global robustness of sliding mode control, the system trajectory needs to be on the time-varying sliding mode surface at the initial moment, so that
yields:
In order to shorten the time of approaching motion and improve the dynamic quality of approaching motion, this paper improves the exponential approach law and designs a new approach law, as shown in Equation (16):
where
,
.
From Equations (12), (14), and (16), it can be obtained that the actual current of the q-axis is as follows:
By substituting the rotational inertia identification value
into Equation (17), the reference current of the q-axis can be obtained as follows:
4.2. ITFTSMC Submodule Analysis
To improve the dynamic performance of the speed, this paper improves the traditional sliding mode controller and adds both the integral module, the time-varying module, and the fast terminal module one by one based on this. The sliding surface of the conventional sliding mode controller is , the sliding surface of the integral sliding mode controller is , the sliding surface of the integral time-varying sliding mode controller is , and the sliding surface of the integral time-varying fast terminal sliding mode controller is . The reaching laws of the four sliding mode controllers are .
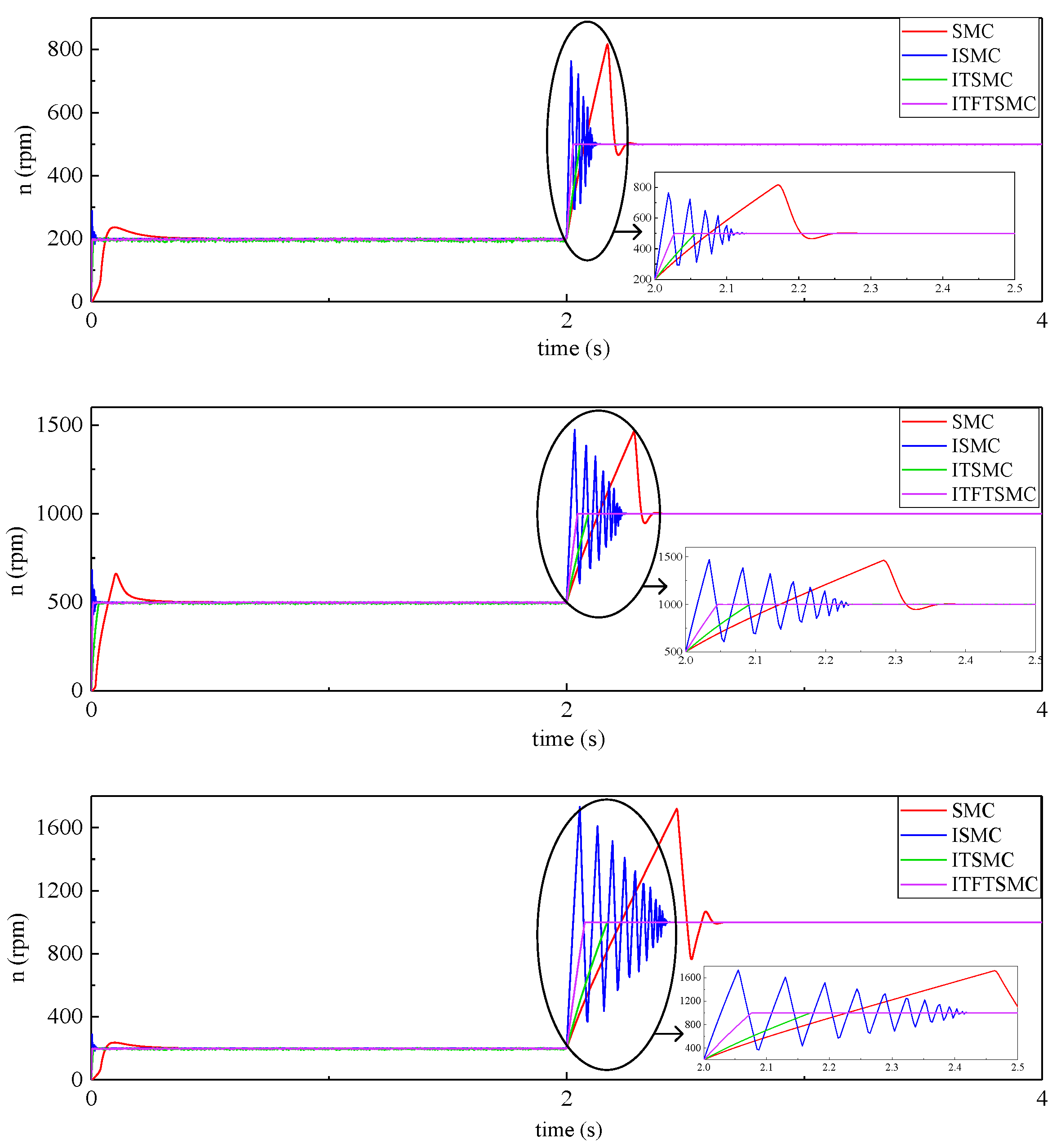
Figure 3 shows the rotational speed comparison waveform brought about by conventional sliding mode control and integral sliding mode control (ISMC).
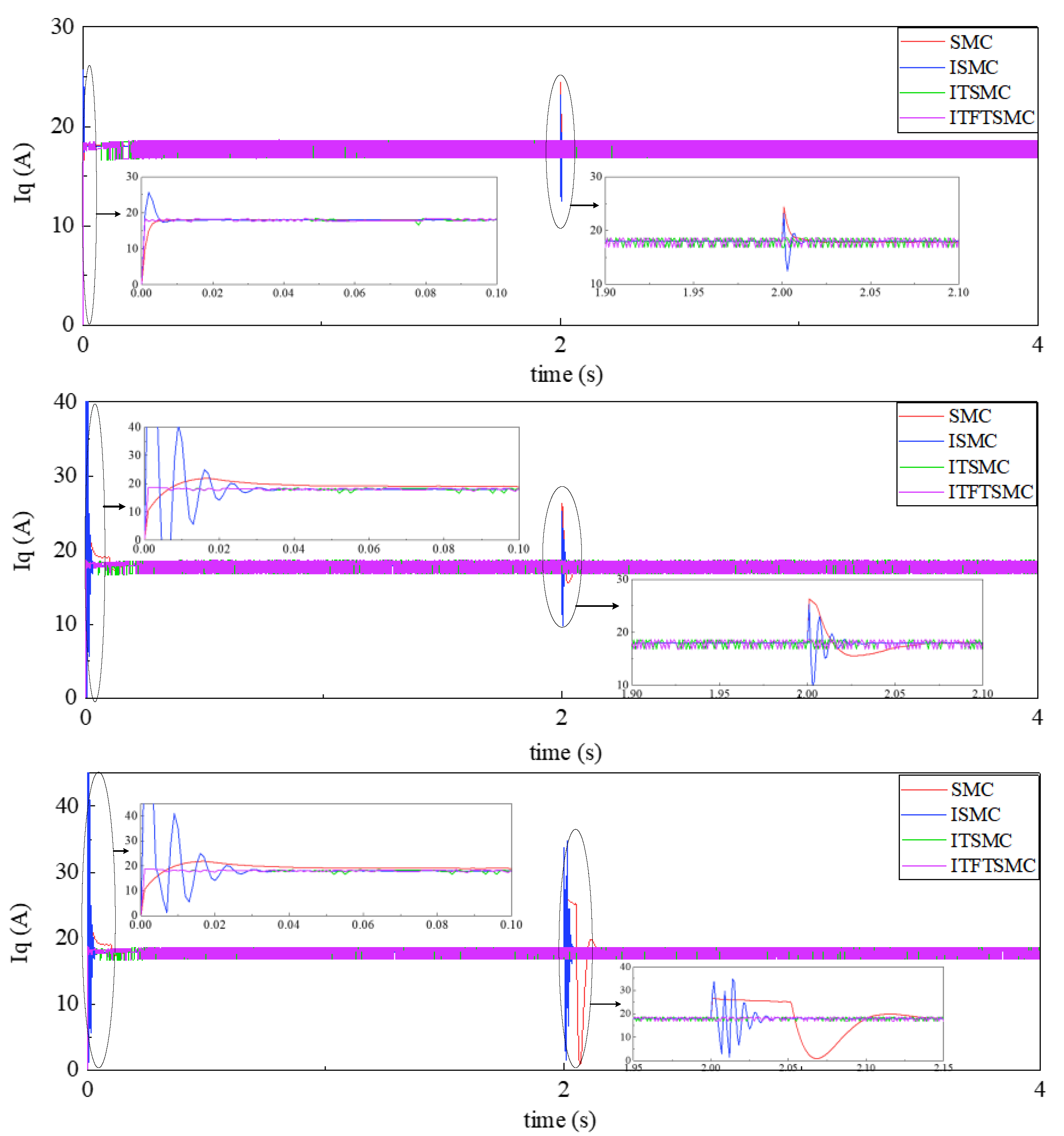
Figure 4 shows the comparison waveform of the q-axis current caused by conventional sliding mode control and integral sliding mode control.
As shown in
Figure 3, the speed of SMC has a large overshoot and long response time when the motor starts and the inertia changes, and the speed waveform obtained by the sliding mode controller after adding the integral module has a great improvement in response time. However, the overshoot remains large and needs further improvement. As shown in
Figure 4, the fluctuation trend of the q-axis current and the fluctuation trend of the speed are roughly the same, but the current fluctuation amplitude of ISMC is extremely large, which is not conducive to the operation of the motor and needs further improvement.
To reduce the high overshoot, the current study adds a time-varying module based on ISMC to obtain integrated time-varying sliding mode control (ITSMC).
Figure 5 shows the speed comparison waveform under SMC, ISMC, and ITSMC.
Figure 6 shows the q-axis current comparison waveform under SMC, ISMC, and ITSMC.
Following
Figure 5, the speed of ISMC has a large overshoot when the motor is started, and the speed waveform obtained by the sliding mode controller after adding the time-varying module has greatly improved the overshoot. As shown in
Figure 6, the overall current fluctuation of ITSMC slightly increases, but the overshoot is much smaller than that of ISMC, and there is still room for improvement.
To reduce the large response time, the fast terminal module was added to the ITSMC to obtain the integrated time-varying sliding mode control (ITFTSMC).
Figure 7 shows the speed comparison waveform under SMC, ISMC, ITSMC, ITFTSMC, and ITFTSMC.
Figure 8 shows the q-axis current comparison waveform under SMC, ISMC, ITSMC, and ITFTSMC.
As shown in
Figure 7, after the introduction of the fast terminal module in ITSMC, the response time of the motor during startup is significantly reduced, and there is almost no overshoot. Compared to the other three sliding mode controllers, ITFTSMC provides a faster and more stable response speed during motor startup. When the moment of inertia changes, both SMC and ISMC have larger overshoots and longer response times, while both ITSMC and ITFTSMC have smaller response times and almost no overshoots. Overall, when the moment of inertia suddenly changes, the rotational speed of the ITFTSMC has the fastest and most stable performance. Additionally, as shown in
Figure 8, the q-axis current of ITFTSMC also has the same characteristics, with the best response time and overshoot, which meet the design requirements.
4.3. Stability Analysis
To verify the stability of the integral time-varying fast terminal sliding mode controller, the Lyapunov function was selected, which is expressed as: .
The derivation of this function yields .
Substituting Equations (12) and (14) into the above equation, the following equation is obtained:
Substitute Equation (17) into Equation (19) to obtain:
Obviously, the sliding mode controller designed in this paper satisfies the Lyapunov stability theorem: . The system is asymptotically stable.