Simulation and Experimental Study on Jetting Projectile Charge Penetrating Multi-Layer Spaced Targets with Large Spacing
Abstract
:1. Introduction
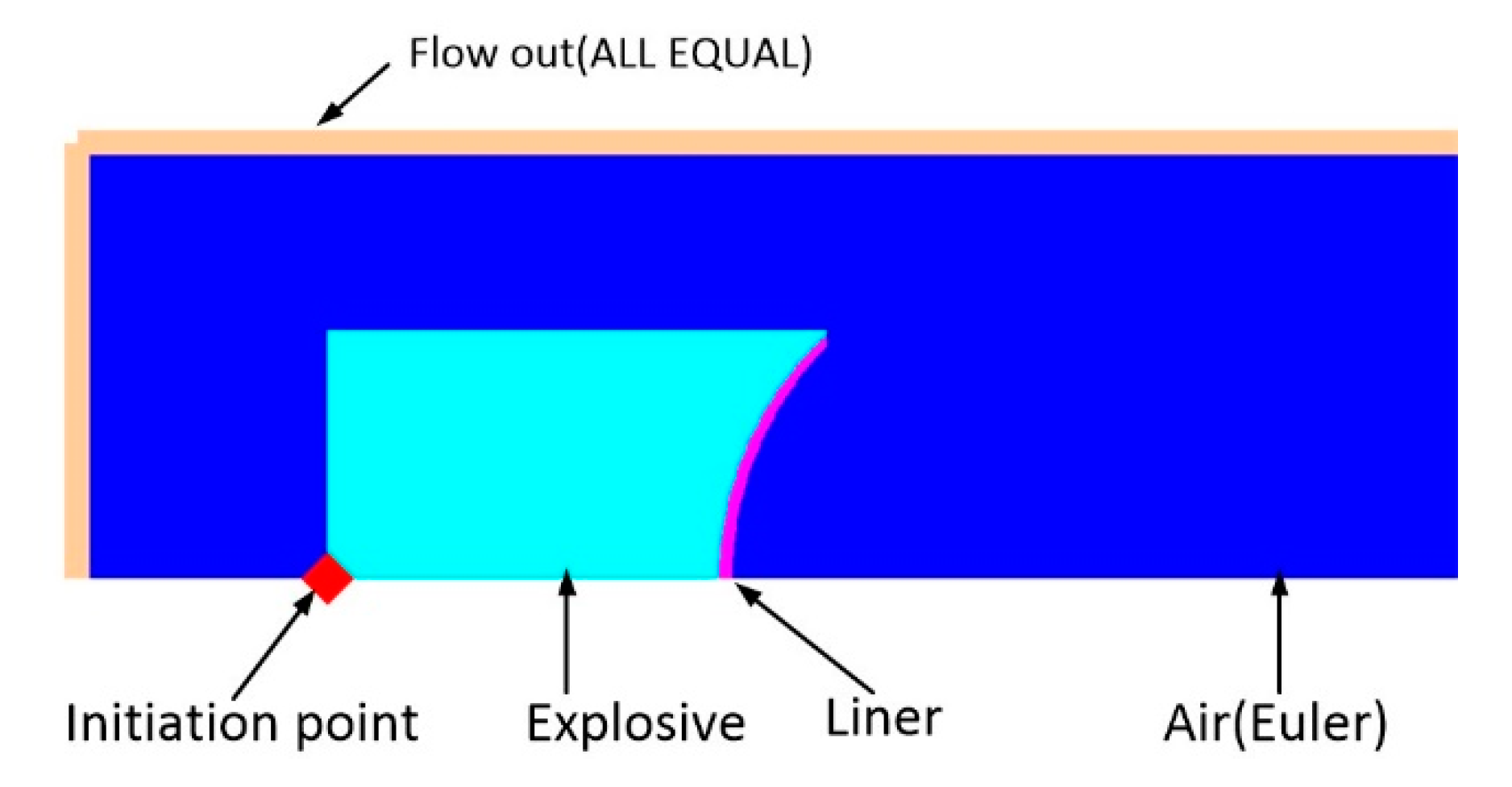
2. Numerical Simulation Model
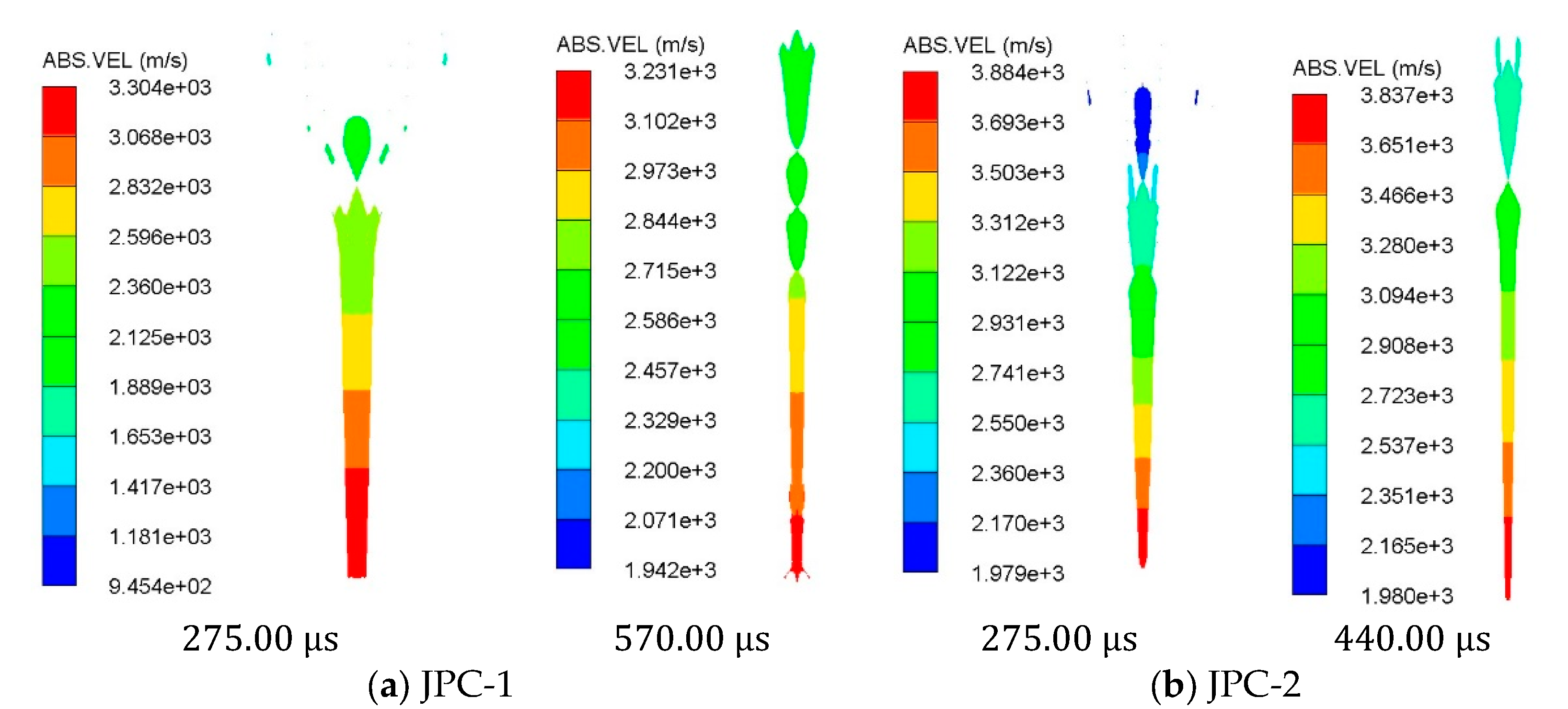
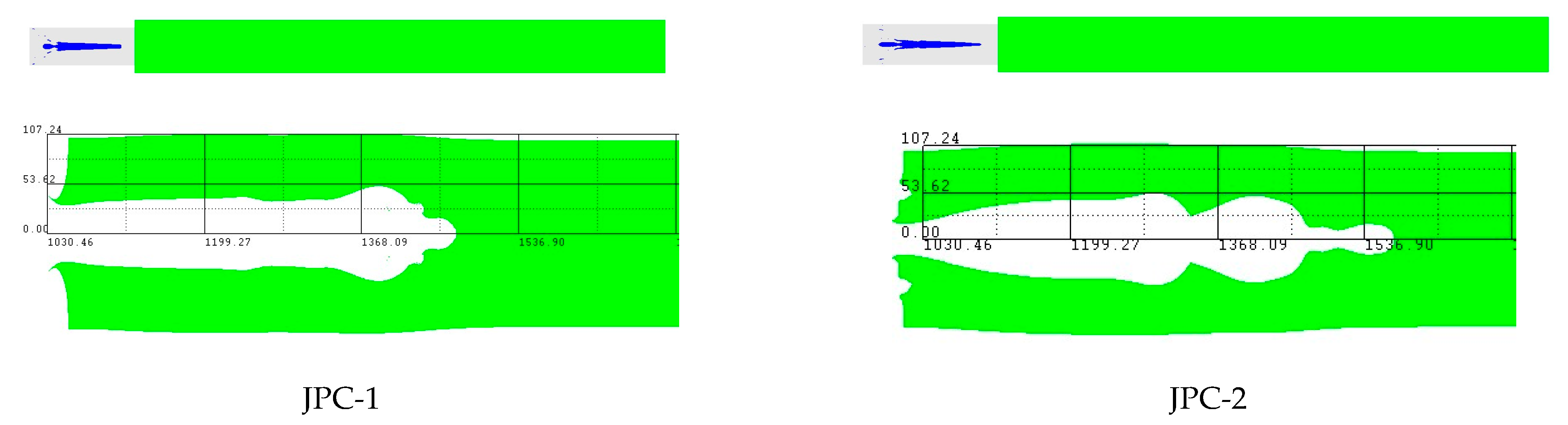
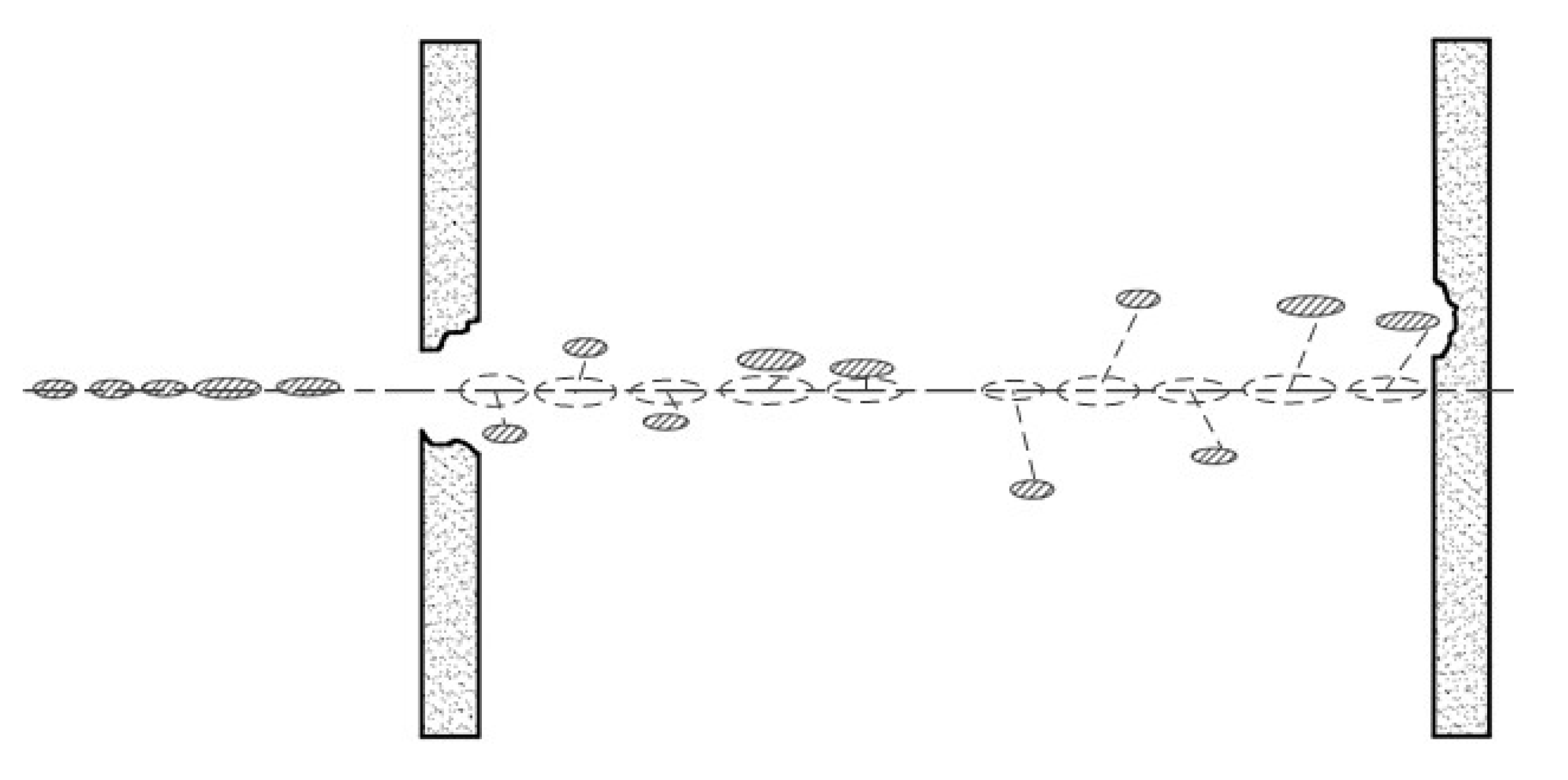
2.1. JPC Formation and Stretching
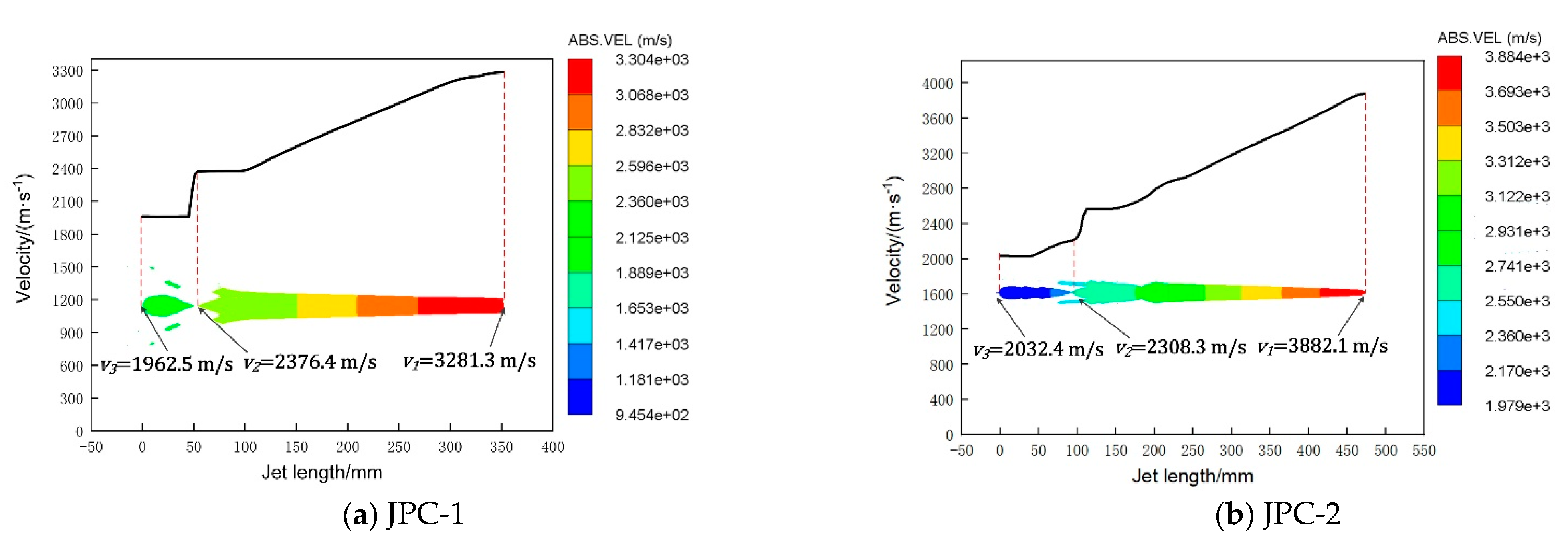
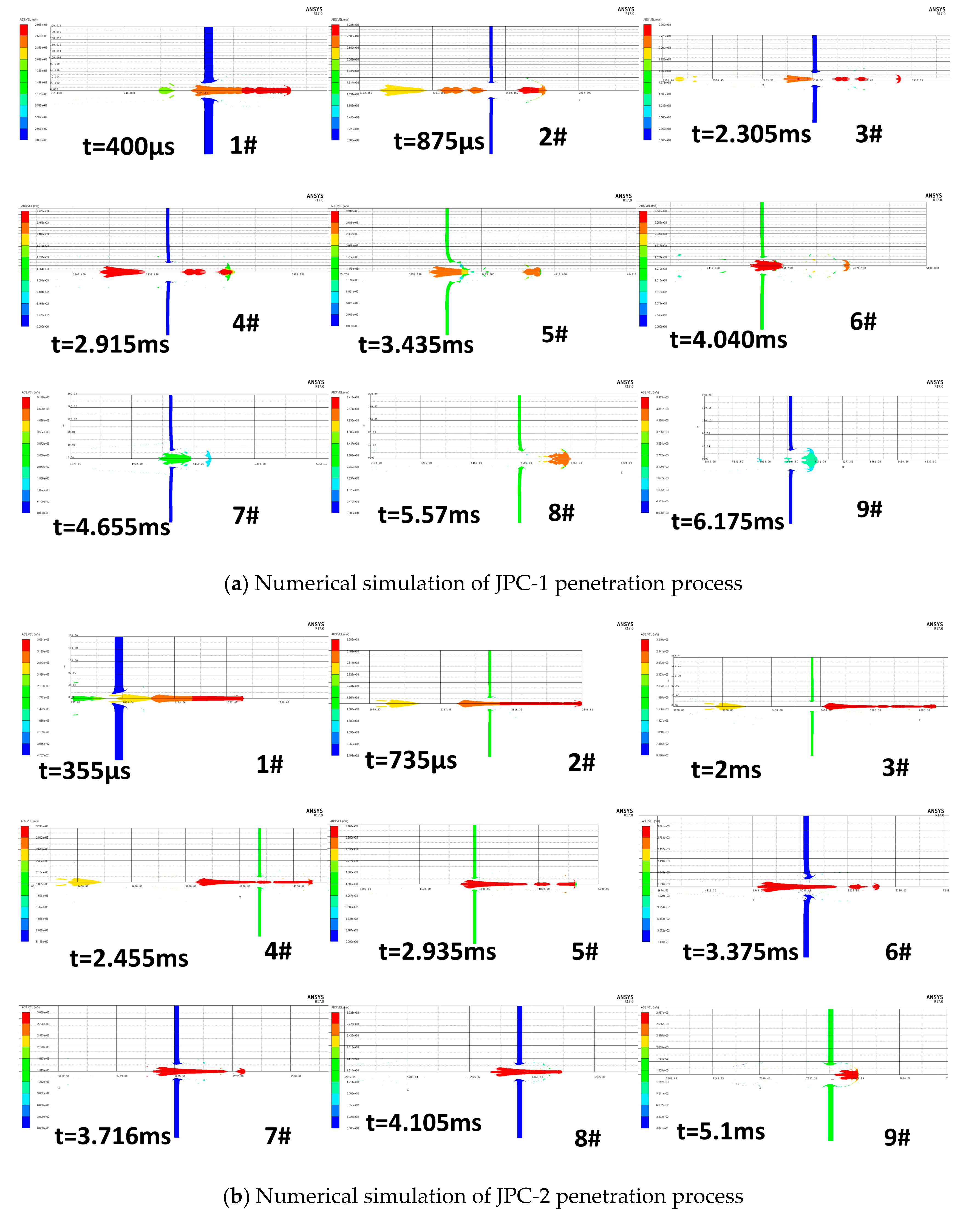
2.2. Numerical Simulation Analysis of the Penetration of JPCs into Spaced Targets
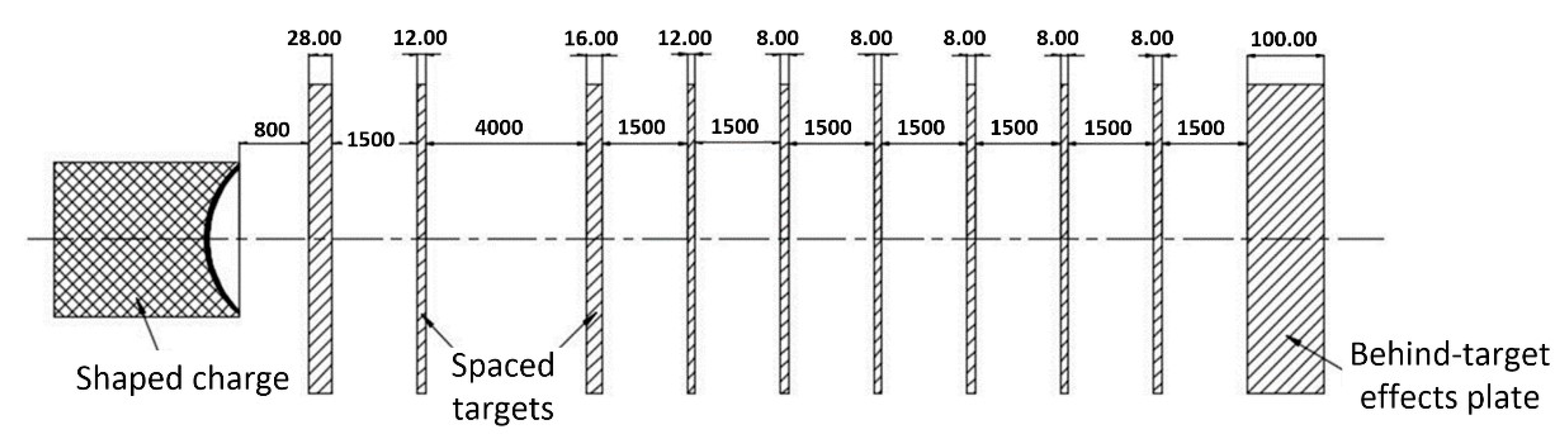
3. Experimental Studies
3.1. Test Setup
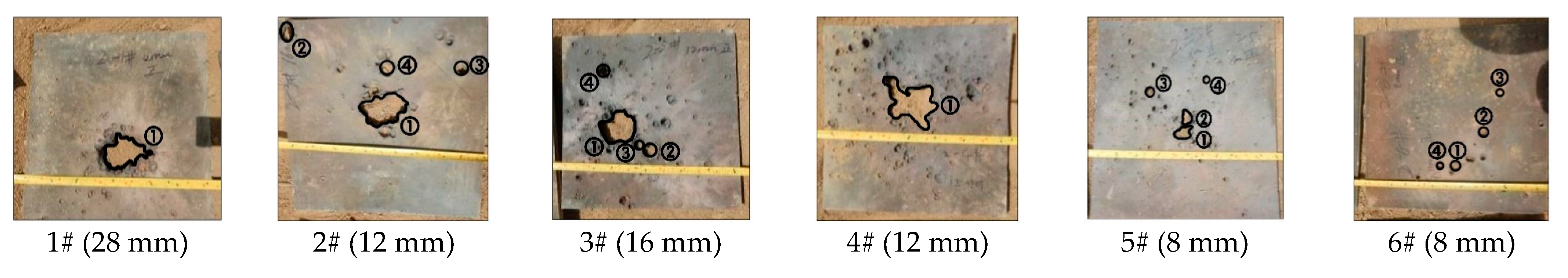
3.2. Experimental Results
4. Discussion
4.1. Influence of Spaced Target Effect on Penetration Power
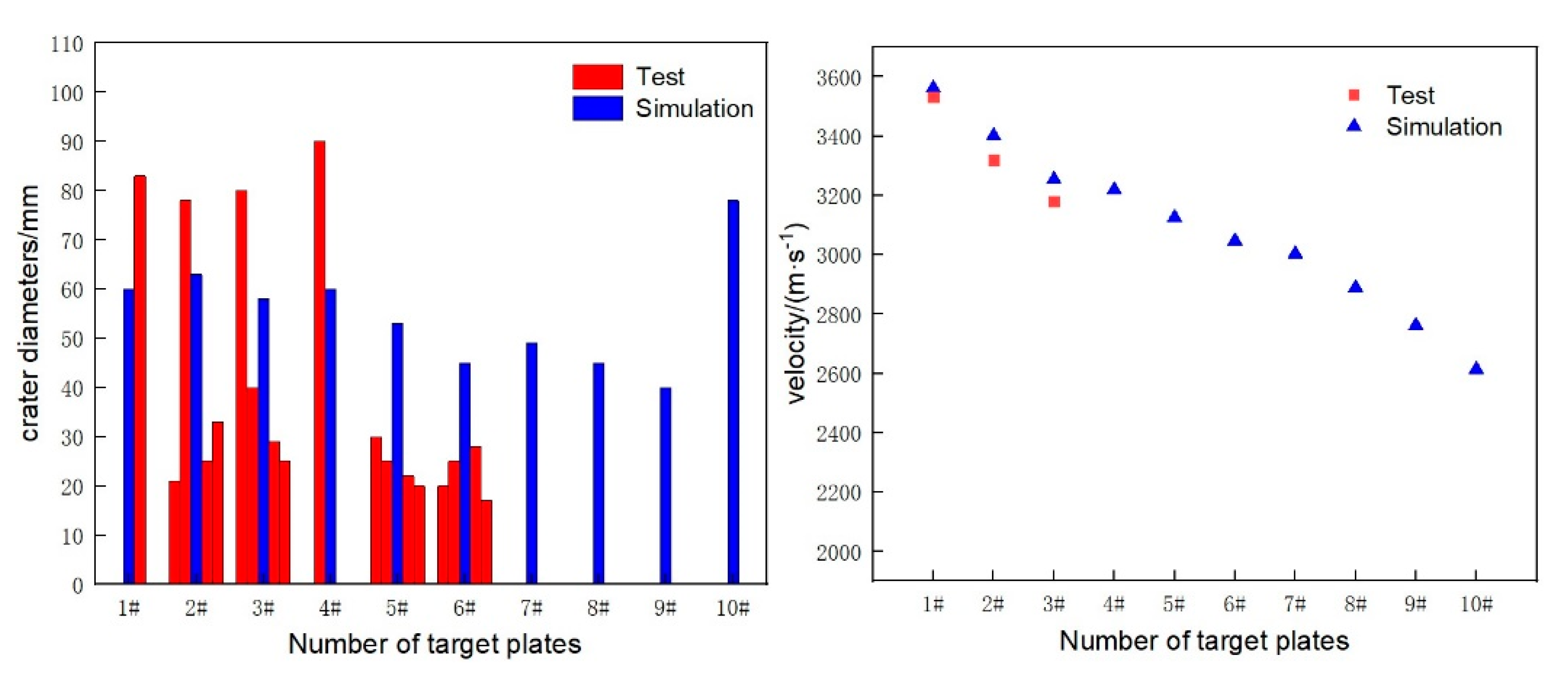
4.2. Comparison between Experimental and Simulation Results
4.3. Design Optimization of Simulation Method
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Huang, Z.X. Mechanism Study on JPC Formation; Nanjing University of Science and Technology: Nanjing, China, 2003; Volume 2–3, p. 48. [Google Scholar]
- Curtis, J.P. Axisymmetric instability model for shaped charge jets. J. Appl. Phys. 1987, 61, 4978–4985. [Google Scholar] [CrossRef]
- Romero, L.A. The instability of rapidly stretching plastic jets. J. Appl. Phys. 1989, 65, 3006–3016. [Google Scholar] [CrossRef]
- Hutchinson, J.W.; Neale, K.W. Influence of strain-rate sensitivity on necking under uniaxial tension. Acta Metall. 1977, 25, 839–846. [Google Scholar] [CrossRef]
- Shenoy, V.B.; Freund, L.B. Necking bifurcations during high strain rate extension. J. Mech. Phys. Solids 1999, 47, 2209–2233. [Google Scholar] [CrossRef]
- Walsh, J.M. Plastic instability and particulation in stretching metal jets. J. Appl. Phys. 1984, 56, 1997–2006. [Google Scholar] [CrossRef]
- Chou, P.C.; Carleone, J. The stability of shaped-charge jets. J. Appl. Phys. 1977, 48, 4187–4195. [Google Scholar] [CrossRef]
- Hennequin, E. Modelling of the Shaped Charge Jet Break-Up. Propellants Explos. Pyrotech. 1996, 21, 181–185. [Google Scholar] [CrossRef]
- Petit, J.; Jeanclaude, V.; Fressengeas, C. Breakup of copper shaped-charge jets: Experiment, numerical simulations, and analytical modeling. J. Appl. Phys. 2005, 98, 123521. [Google Scholar] [CrossRef]
- Karlsson, H.E. Computer simulation of shaped charge jet fragmentation. In Proceedings of the 20th International Symposium on Ballistics, Orlando, FL, USA, 23–27 September 2022; pp. 557–564. [Google Scholar]
- Hirsch, E. The natural spread and tumbling of the shaped charge jet segments. Propellants Explos. Pyrotech. 1981, 6, 104–111. [Google Scholar] [CrossRef]
- Rottenkolber, E.; Arnold, W. Rotation rates and transverse velocities of shaped charge jet particles caused by breakup. In Proceedings of the 15th International Symposium on Ballistics, Singapore, 15–17 December 2004; pp. 845–852. [Google Scholar]
- Kelly, R.J.; Curtis, J.P.; Cowan, K.G. An analytic model for the prediction of incoherent shaped charge jets. J. Appl. Phys. 1999, 86, 1255–1265. [Google Scholar] [CrossRef]
- Hussain, G.; Hameed, A.; Horsfall, I.; Barton, P.; Malik, A.Q. Experimental and simulation optimization analysis of the Whipple shields against shaped charge. Acta Mech. Sin. 2012, 28, 877–884. [Google Scholar] [CrossRef]
- Taylor, E.A. Simulation of hollow shaped charge jet impacts onto aluminium whipple bumpers at 11 km/s. Int. J. Impact Eng. 2001, 26, 773–784. [Google Scholar] [CrossRef]
- Svirskii, O.; Vlasova, M.; Vasetsky, V.; Toropova, T.; Nechaev, A.; Krutyakov, V. Penetration of Shaped Charge Jets at Long Standoff Distances. In Proceedings of the 21th International Symposium on Ballistics, Adelaide, Australia, 19–23 April 2004; pp. 378–382. [Google Scholar]
- Horsfall, I. The effect of spaced armour on the penetration of shaped charge warheads. In Proceedings of the 22nd International Symposium on Ballistics, Vancouver, BC, Canada, 14–18 November 2005; pp. 941–947. [Google Scholar]
- Held, M. Theoretical optimum jet diameter profile for maximum penetration. In Proceedings of the 17th International Symposium on Ballistics, Midrand, South Africa, 23–27 March 1998. [Google Scholar]
- Held, M. Penetration cutoff velocities of shaped charge jets. Propellants Explos. Pyrotech. 1988, 13, 111–119. [Google Scholar] [CrossRef]
- Haugstad, B.S. Compressibility effects in shaped charge jet penetration. J. Appl. Phys. 1981, 52, 1243–1246. [Google Scholar] [CrossRef]
- Tan, D.W.; Sun, C.W.; Zhao, J.B.; Zhang, K.M.; Xie, P.H. Experimental investigation of shaped charge with large cone angle. Chin. J. High Press. Phys. 2003, 17, 204–208. [Google Scholar]
- Daniels, A.S.; Baker, E.L.; Vuong, T.H.; Chin, C.L.; Fuchs, B.F.; DeFisher, S.E. Selectable initiation shaped charges. In Proceedings of the 20th International Symposium on Ballistics, Orlando, FL, USA, 23–27 September 2002; pp. 23–27. [Google Scholar]
- Zhang, J.; Zhi-gang, C.H.; Xiao-jun, L.I. The variable wall thickness of hemispherical liner of rod like jet formation and penetration properties. Explos. Mater. 2016, 45, 39–42. [Google Scholar] [CrossRef]







| Parameter | /g·cm−1 | A/GPa | B/GPa | R1 | R2 | W | Detonation Velocity/ m·s−1 |
|---|---|---|---|---|---|---|---|
| value | 1.717 | 6.184 × 102 | 6.9 | 4.3 | 0.87 | 0.38 | 8.35 × 103 |
| Parameter | Energy/ kJ·m−3 | Pressure/ GPa | |||||
| value | 0.09 | 29.66 | |||||
| Parameter | /g·cm−1 | C1/m·s−1 | C2/m·s−1 | S1 | S2 | Shear Modulus/ GPa | Yield Stress/ GPa |
|---|---|---|---|---|---|---|---|
| value | 8.93 | 3.94 × 103 | 0 | 1.489 | 0 | 46 | 0.09 |
| Parameter | Hardening Constant/GPa | Hardening Exponent | Strain Rate Constant | ||||
| value | 0.292 | 0.31 | 0.025 | ||||
| Parameter | /g·cm−1 | C1/m·s−1 | C2/m·s−1 | S1 | S2 | Shear Modulus/ GPa | Yield Stress/ GPa |
|---|---|---|---|---|---|---|---|
| value | 7.83 | 4.57 × 103 | 0 | 1.33 | 0 | 76.9 | 0.35 |
| Parameter | Hardening Constant/GPa | Hardening Exponent | Strain Rate Constant | ||||
| value | 0.299 | 0.26 | 0.014 | ||||
| Tip-Tail Velocity Difference/m·s−1 | Head Diameter/mm | Tail Diameter/mm | Tip Speed/m·s−1 | Total Penetration/mm | |||
|---|---|---|---|---|---|---|---|
| JPC-1 | 298.2 | 902 | 15 | 36 | 3281.3 | 160 | 570 |
| JPC-2 | 379.1 | 1574 | 7 | 34 | 3882.1 | 200 | 440 |
| Differences | 80.2 | 672 | 8 | 2 | 600.8 | 40 | 130 |
| Target No. | Thickness (mm) | Perforation or Not | Hole Size (mm) JPC-1 | Hole Size (mm) JPC-2 | ||
|---|---|---|---|---|---|---|
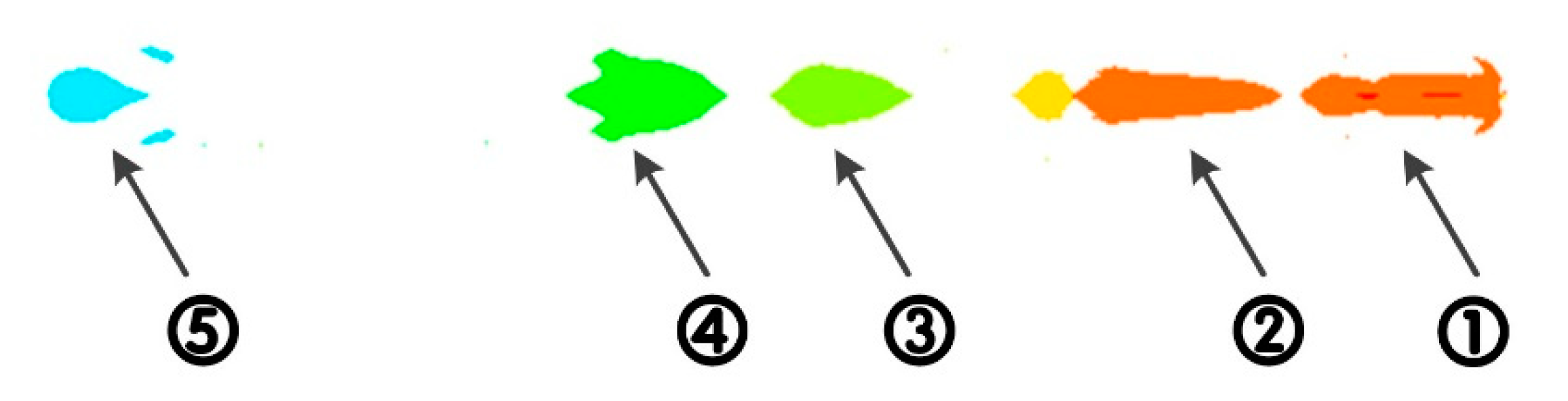
| 1# | 28 | Yes | ① | 75 × 82 | ① | 83 × 100 |
| 2# | 12 | Yes | ① ② | Φ55 Φ52 | ① ② ③ ④ | 82 × 78 Φ21 Φ25 Φ33 |
| 3# | 16 | Yes | ① | 76 × 80 | ① | 105 × 80 |
| ② | Φ40 | ② | Φ30 | |||
| ③ | Φ35 | ③ | Φ25 | |||
| ④ | Φ15 | ④ | Φ20 | |||
| 4# | 8 | Yes | ① | 70 × 80 | ① | 114 × 110 |
| ② | 45 × 70 | |||||
| ③ | Φ13 | |||||
| ④ | Φ10 | |||||
| 5# | 8 | Yes | ① | 30 × 35 | ① | 45 × 30 |
| ② | Φ30 | ② | Φ25 | |||
| ③ | Φ20 | ③ | Φ22 | |||
| ④ | Φ10 | ④ | Φ20 | |||
| 6# | 8 | Yes | ① | Φ10 | ① ② ③ ④ | 30 × 28 Φ25 Φ11 Φ10 |
| 7#–10# | 8 | No | - | - | - | - |
| Penetration Depth (JPC-1) /mm | Penetration Depth (JPC-2) /mm | |
|---|---|---|
| Steel/simulation | 421.4 | 571.8 |
| Spaced target/simulation | 160/10 layers | 200/10 layers |
| Spaced target/test | 84/6 layers | 84/6 layers |
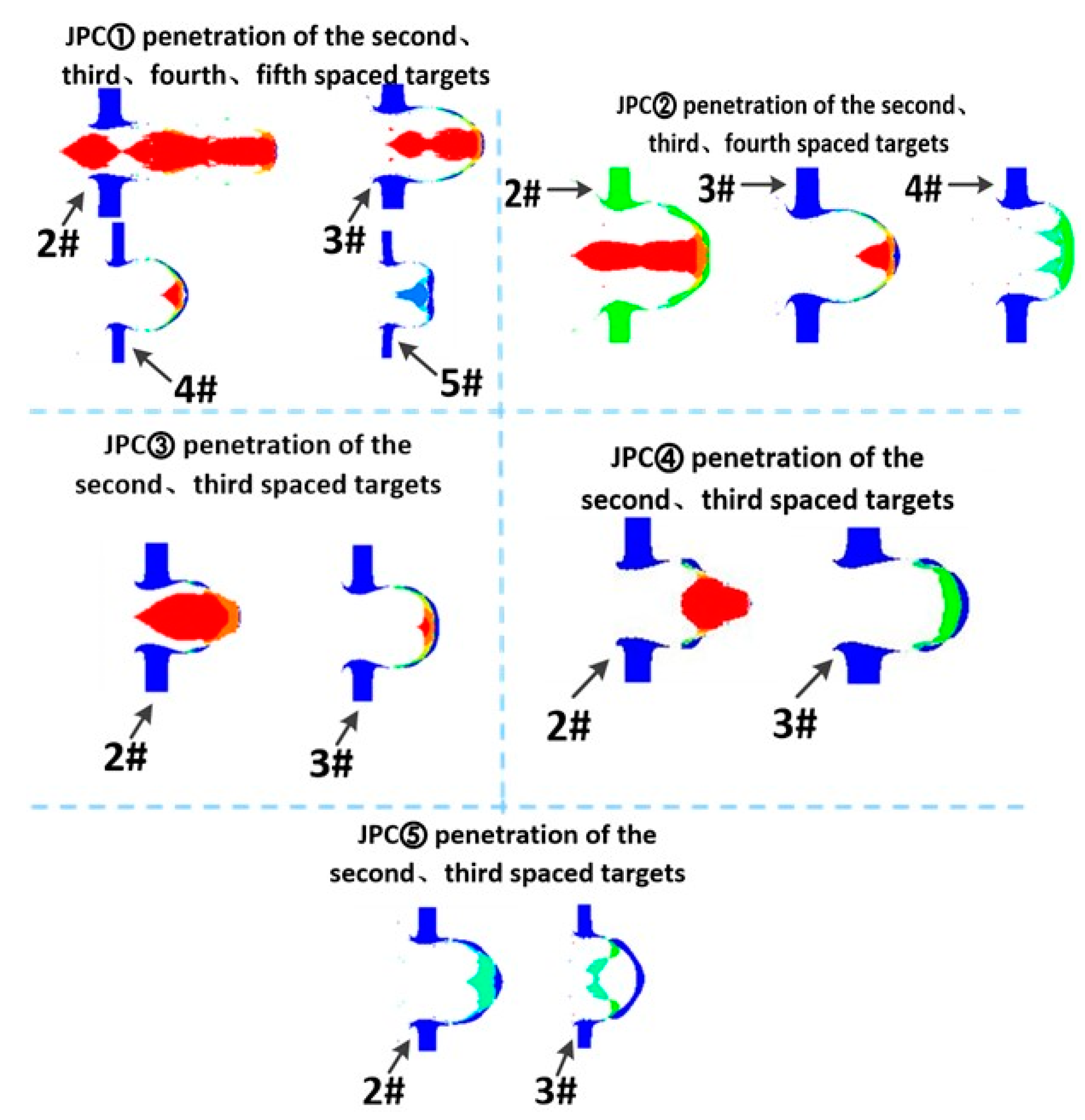
| Target No. | Hole in Each Target | ||||
|---|---|---|---|---|---|
| Segment No. | #2 | #3 | #4 | #5 | |
| ① | 49 mm | 47.6 mm | 54 mm | 47 mm | |
| ② | 50 mm | 46 mm | 52 mm | - | |
| ③ | 28.6 mm | 53.2 mm | - | - | |
| ④ | 67.2 mm | 73.2 mm | - | - | |
| ⑤ | 30 mm | 34.8 mm | - | - | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ji, L.; Jia, X.; Guo, J.; Cai, Y.; Wang, P.; Huang, Z. Simulation and Experimental Study on Jetting Projectile Charge Penetrating Multi-Layer Spaced Targets with Large Spacing. Machines 2023, 11, 526. https://doi.org/10.3390/machines11050526
Ji L, Jia X, Guo J, Cai Y, Wang P, Huang Z. Simulation and Experimental Study on Jetting Projectile Charge Penetrating Multi-Layer Spaced Targets with Large Spacing. Machines. 2023; 11(5):526. https://doi.org/10.3390/machines11050526
Chicago/Turabian StyleJi, Long, Xin Jia, Jiahui Guo, You’er Cai, Ping Wang, and Zhengxiang Huang. 2023. "Simulation and Experimental Study on Jetting Projectile Charge Penetrating Multi-Layer Spaced Targets with Large Spacing" Machines 11, no. 5: 526. https://doi.org/10.3390/machines11050526
APA StyleJi, L., Jia, X., Guo, J., Cai, Y., Wang, P., & Huang, Z. (2023). Simulation and Experimental Study on Jetting Projectile Charge Penetrating Multi-Layer Spaced Targets with Large Spacing. Machines, 11(5), 526. https://doi.org/10.3390/machines11050526






