Geometric Design of a Low-Power Arcjet Constrictor and Determination of Velocity of Air-Based Plasma by Means of Analytical and Numerical Methods
Abstract
1. Introduction
2. Materials and Methods
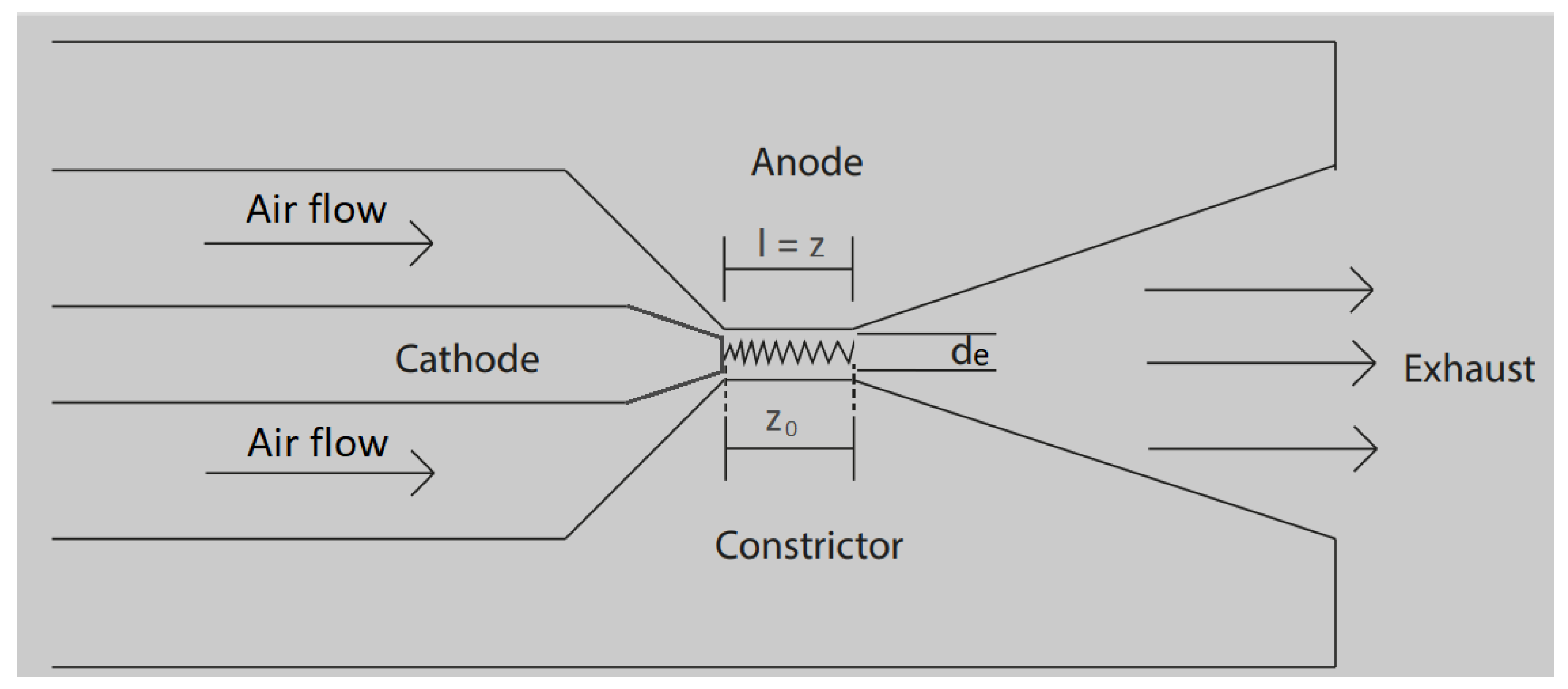
2.1. Geometric Design of the Constrictor
2.2. Assumptions for Analytical and Numerical Methods
- The airflow is assumed to be one-dimensional, laminar, steady-state, and with a constant mass flow rate.
- The electric discharge is steady.
- The heat loss due to thermal conduction is much greater compared to radiation.
- The air-based plasma is in thermodynamic equilibrium.
- The Lorentz force is negligible.
- The radial variation of the electric potential is negligible.
- The air-based plasma is in the thermodynamic equilibrium.
- The air-based plasma has an atmospheric pressure of 1.
- The gravitational effects are negligible.
- The flow is turbulent.
- Radiation loss is considered.
2.3. Analytical Method
2.4. Numerical Method
2.4.1. Governing Equations
2.4.2. Numerical Simulation Procedures
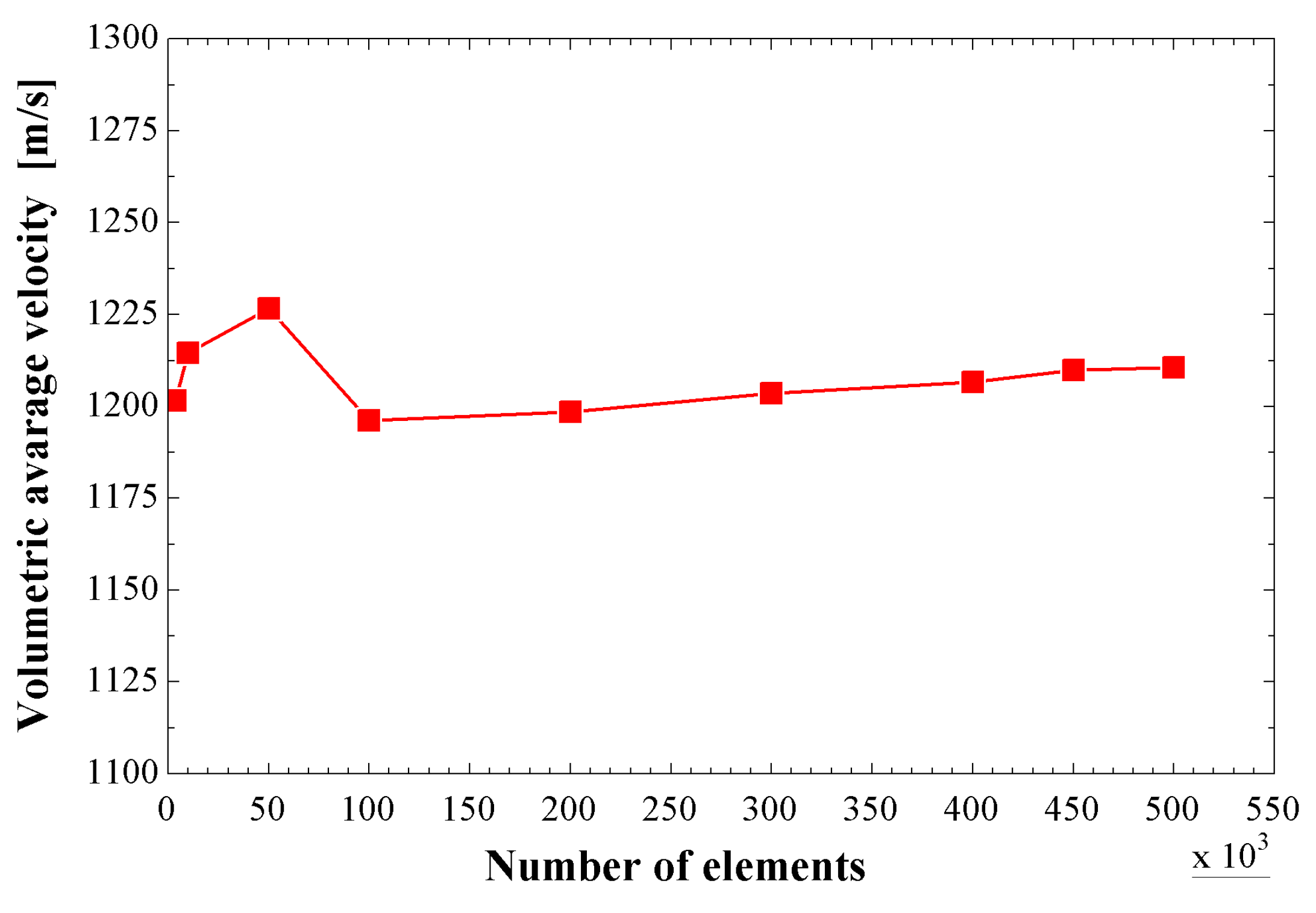
2.4.3. Computational Domain and Mesh Independence Analysis
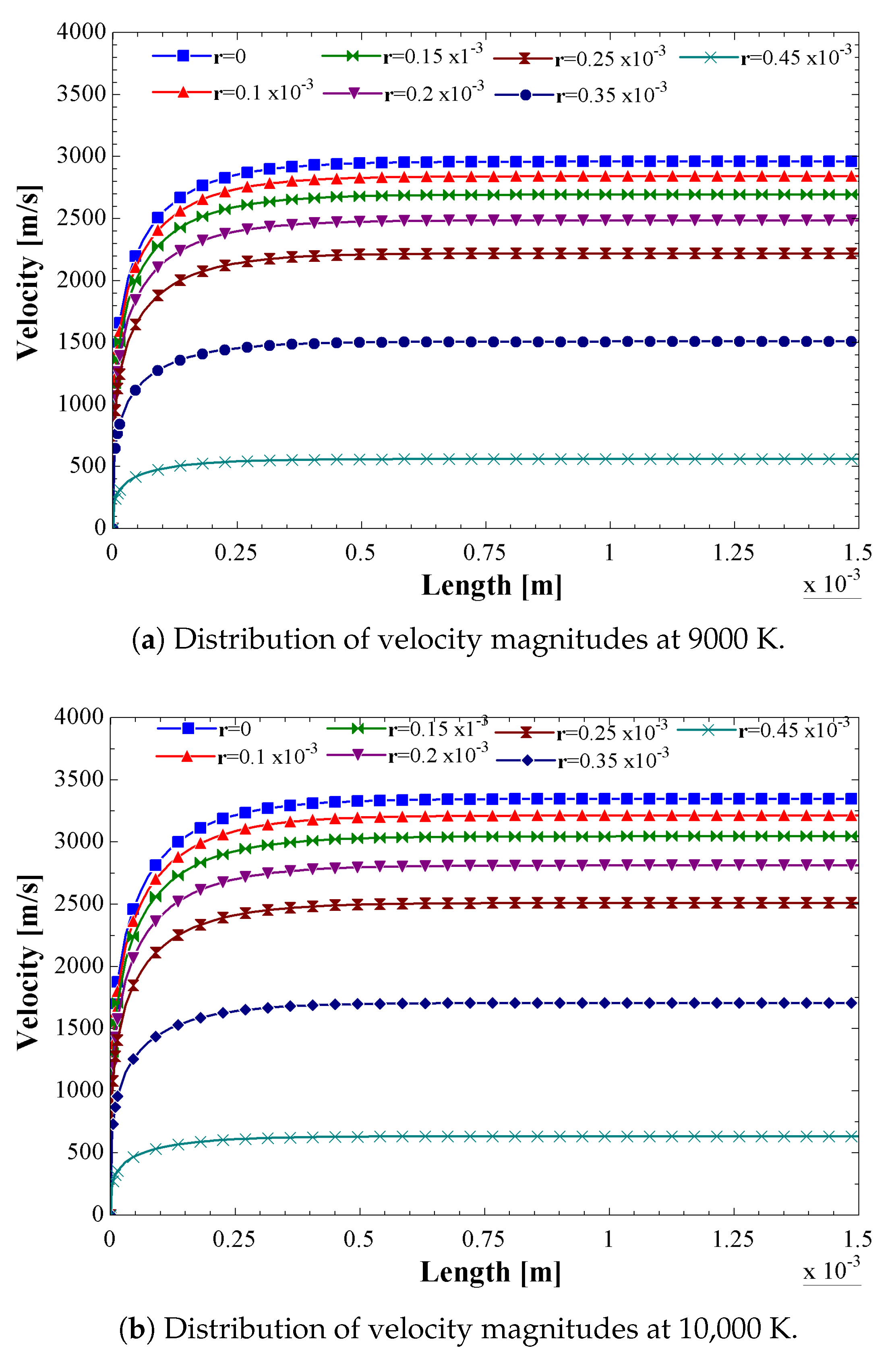
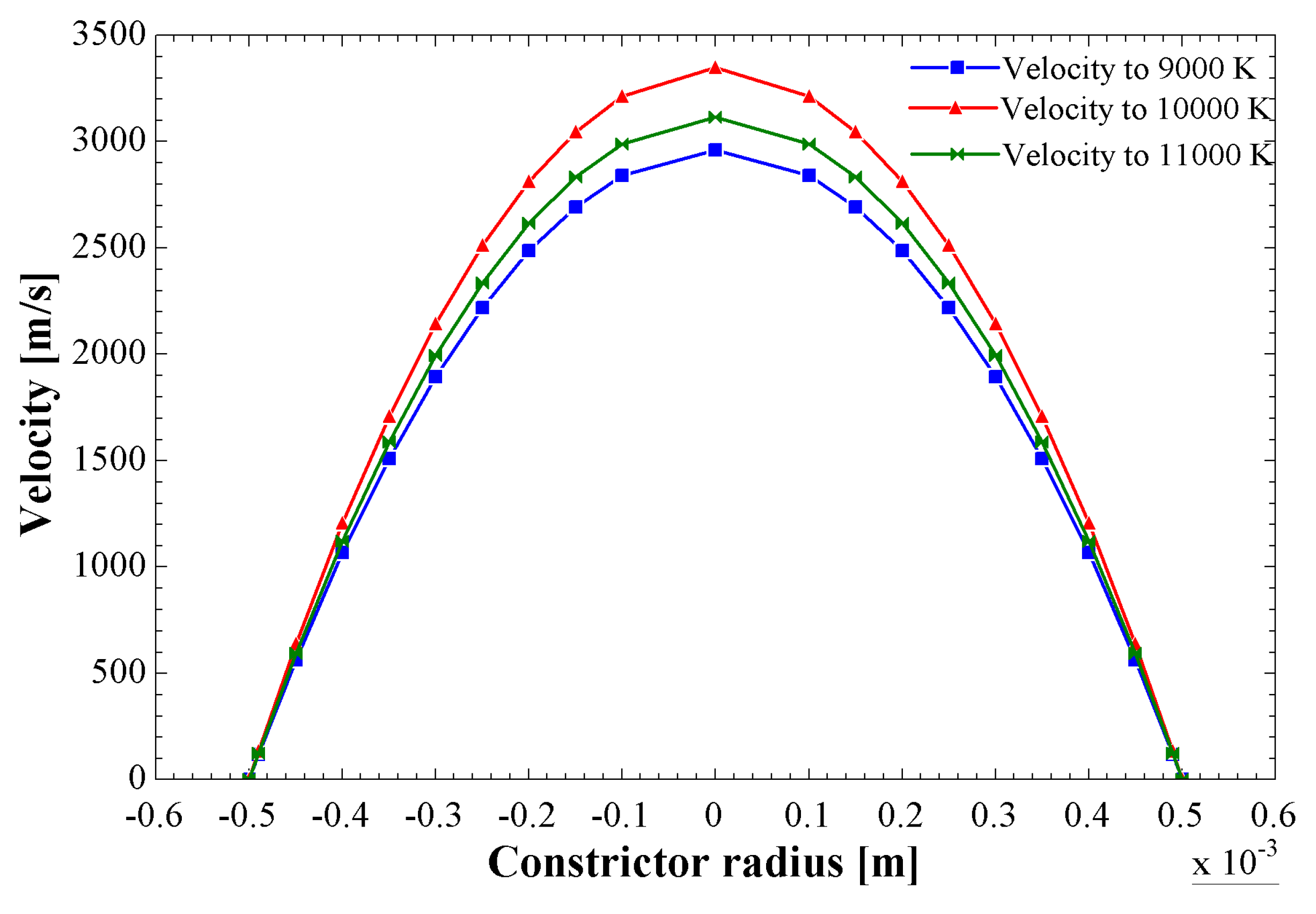
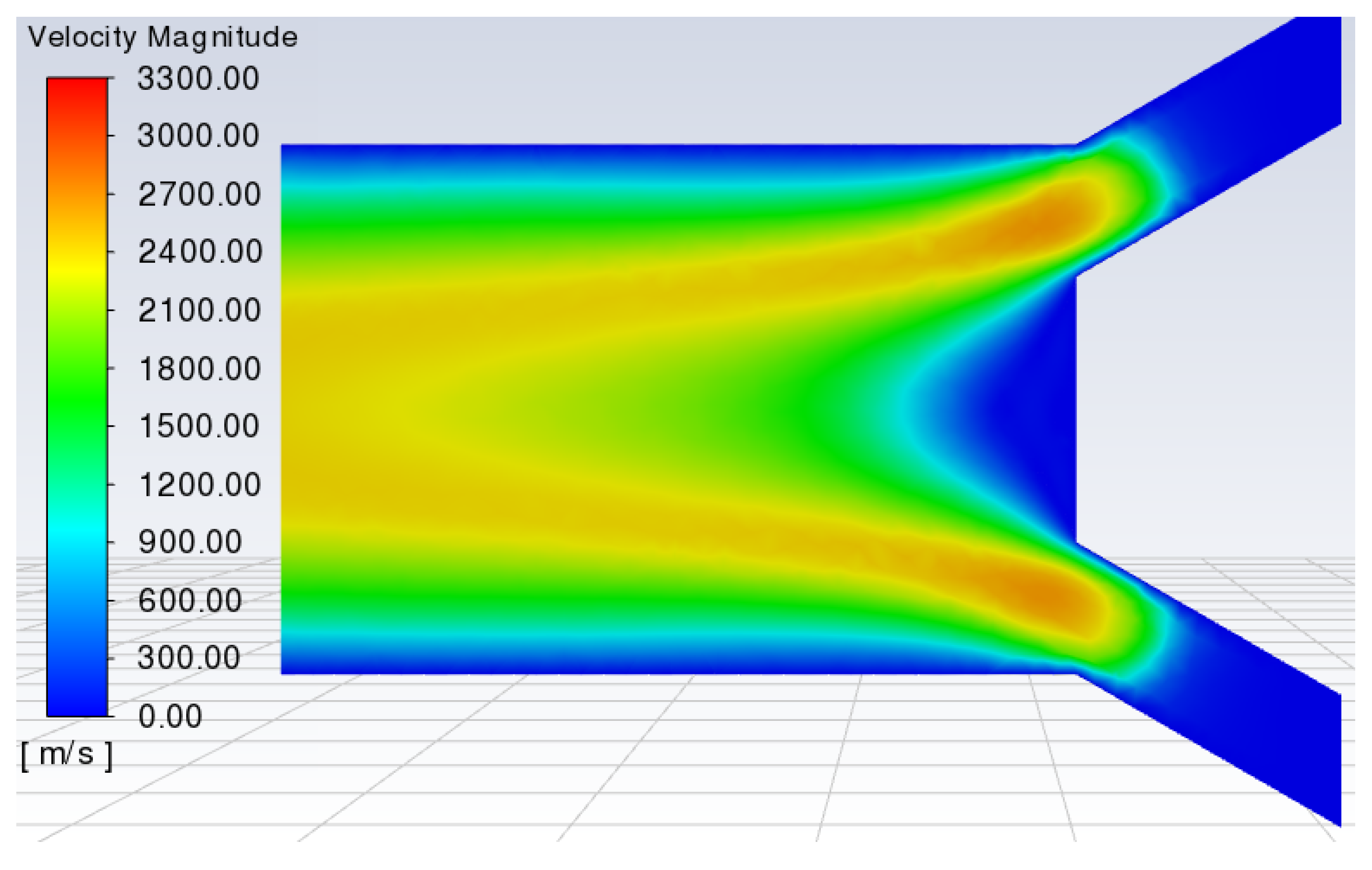
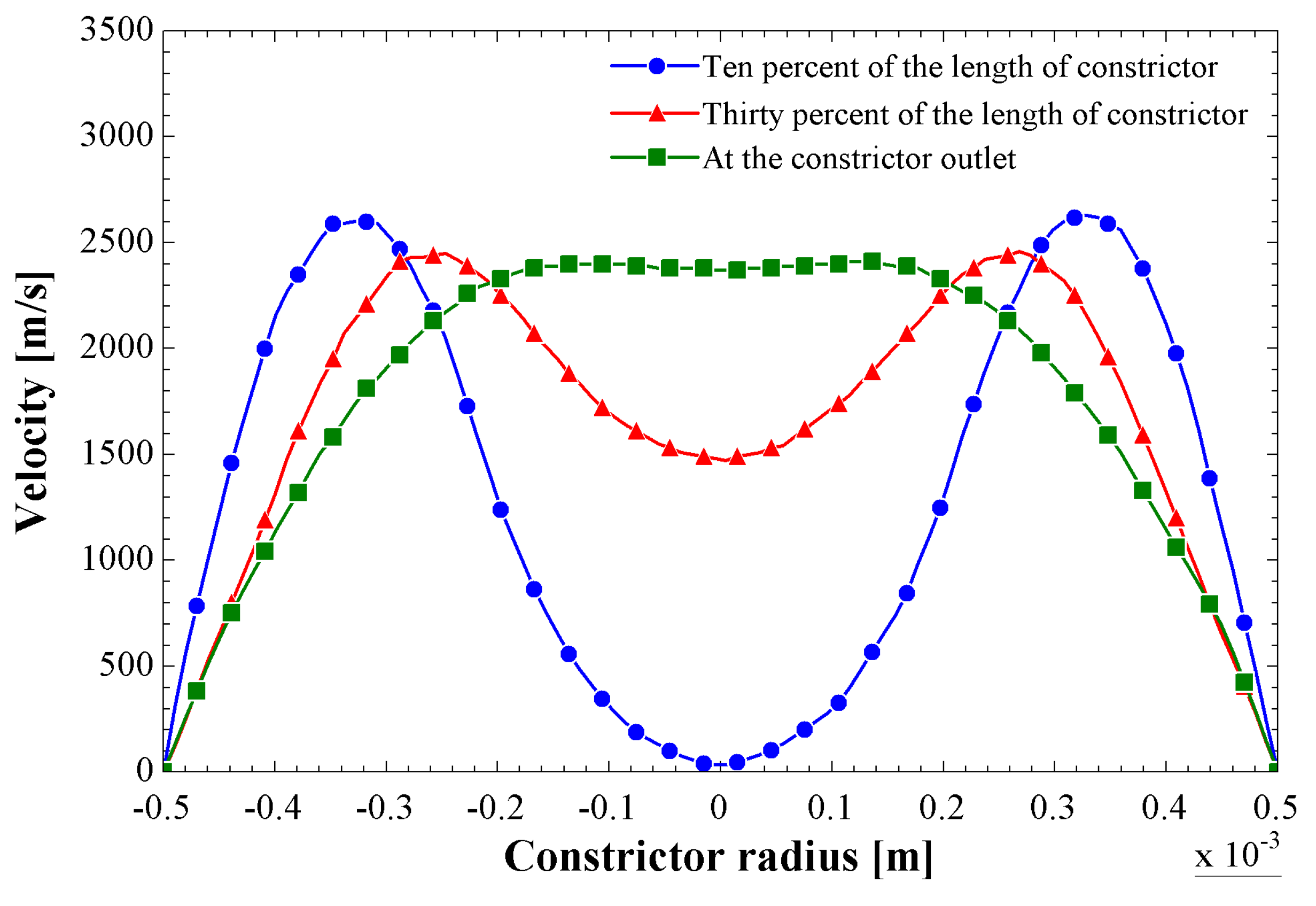
3. Results and Discussion
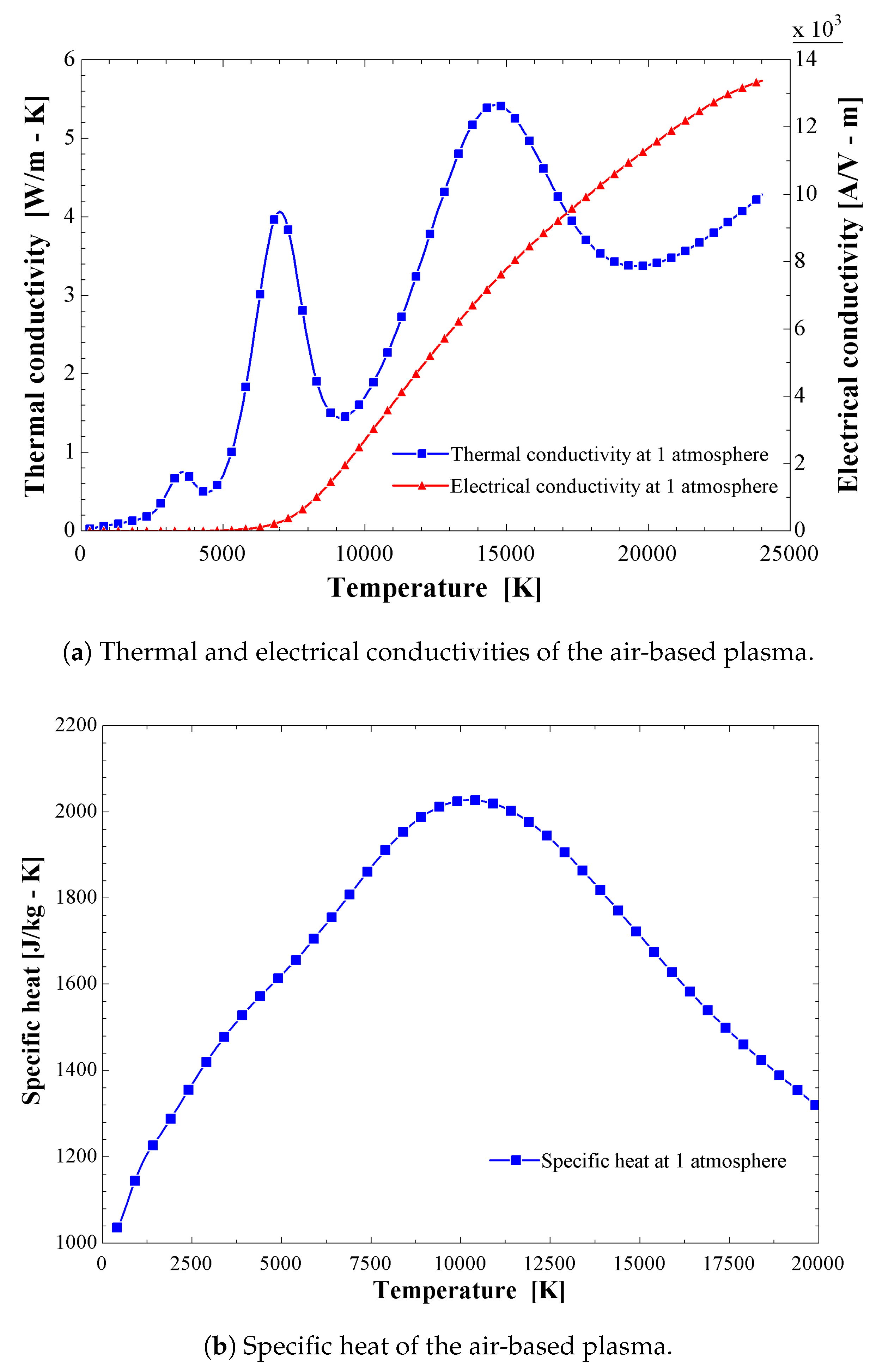
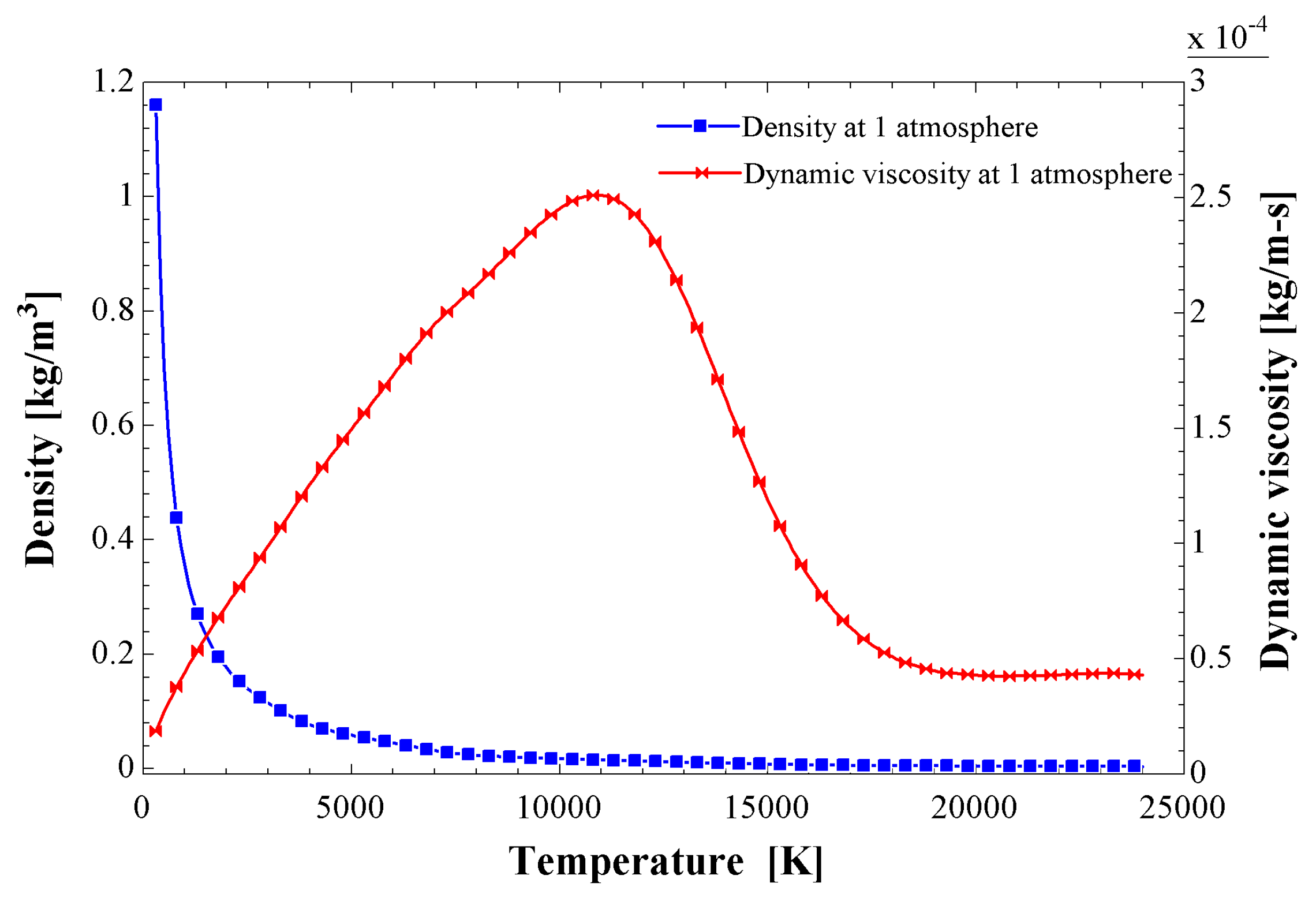
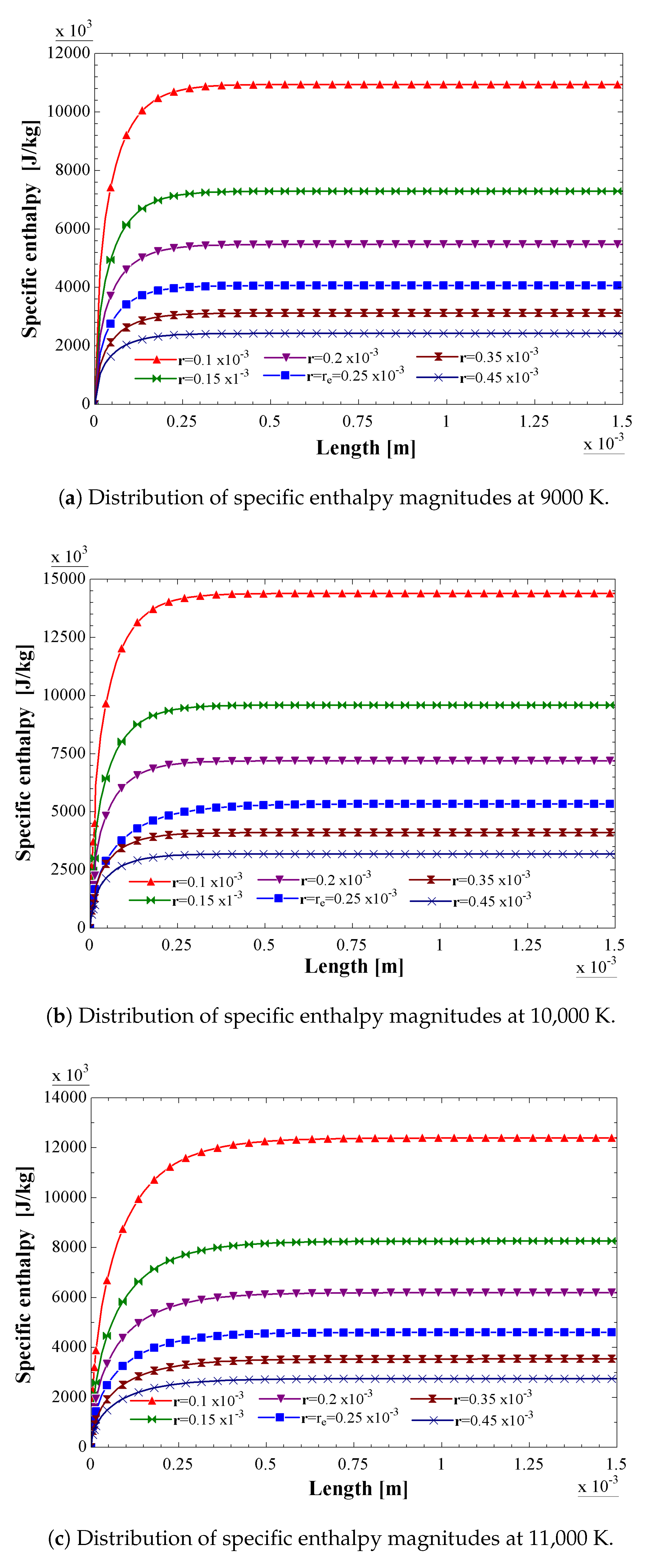
3.1. Results of the Analytical Approach
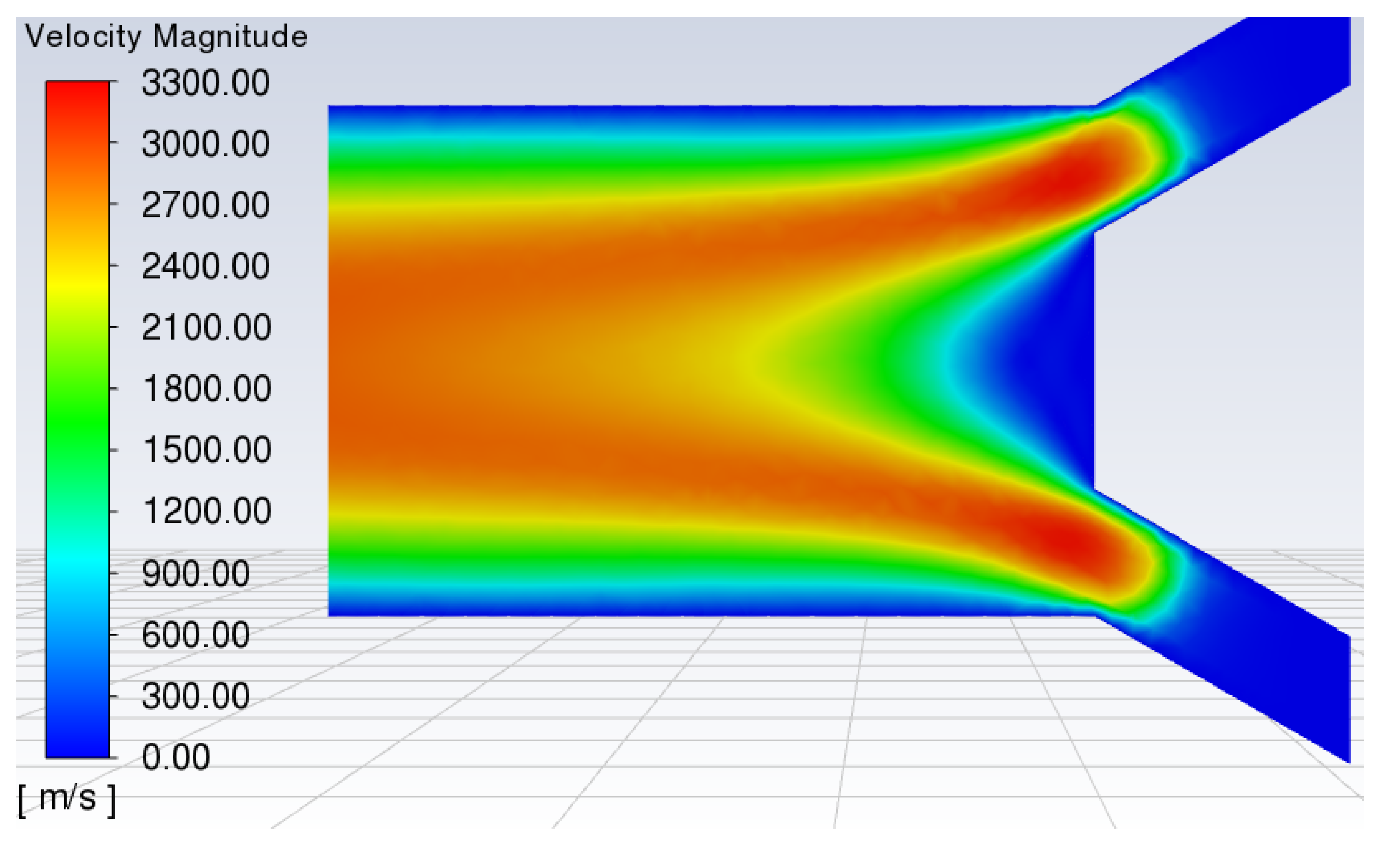
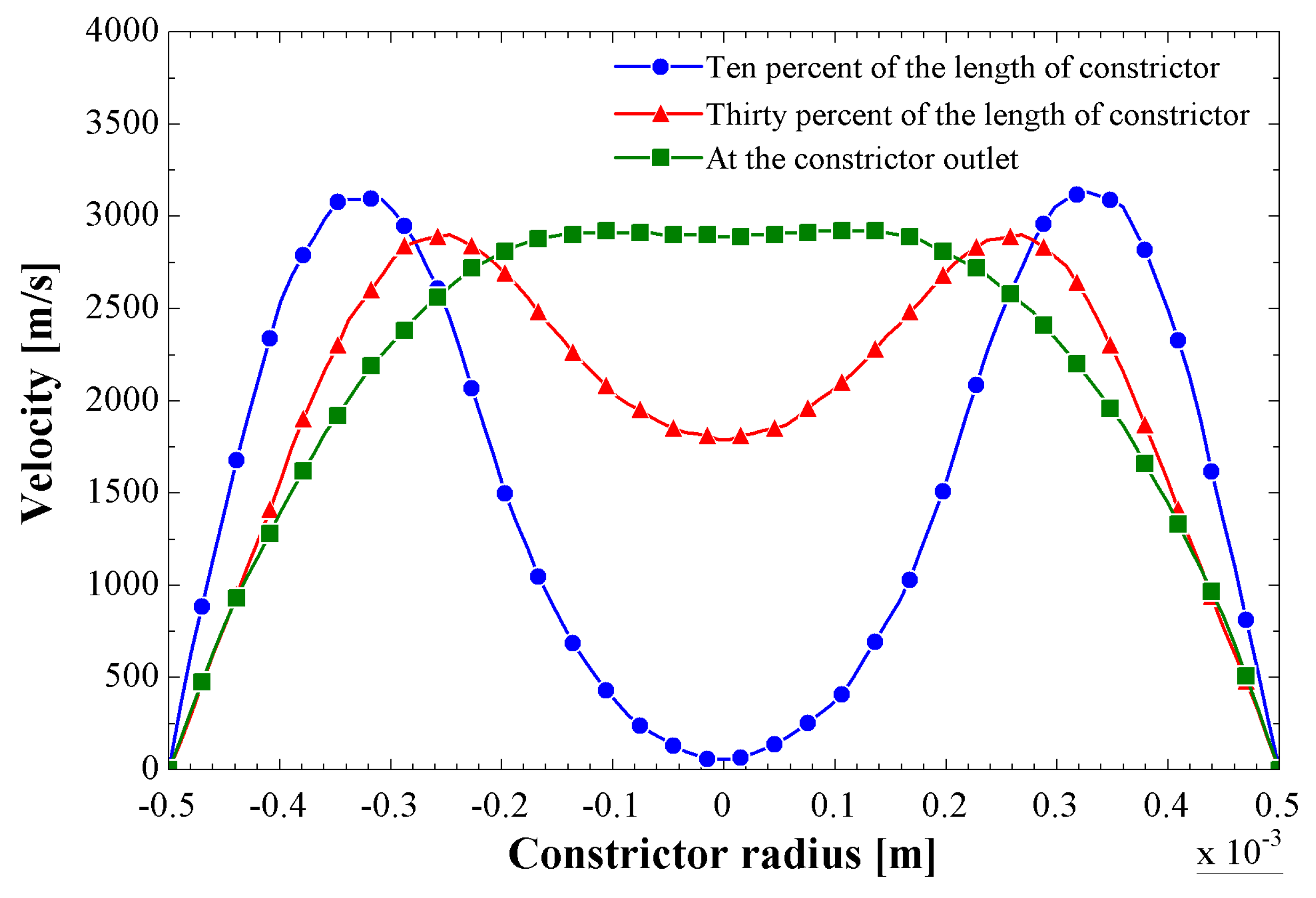
3.2. Results of the Numerical Simulation
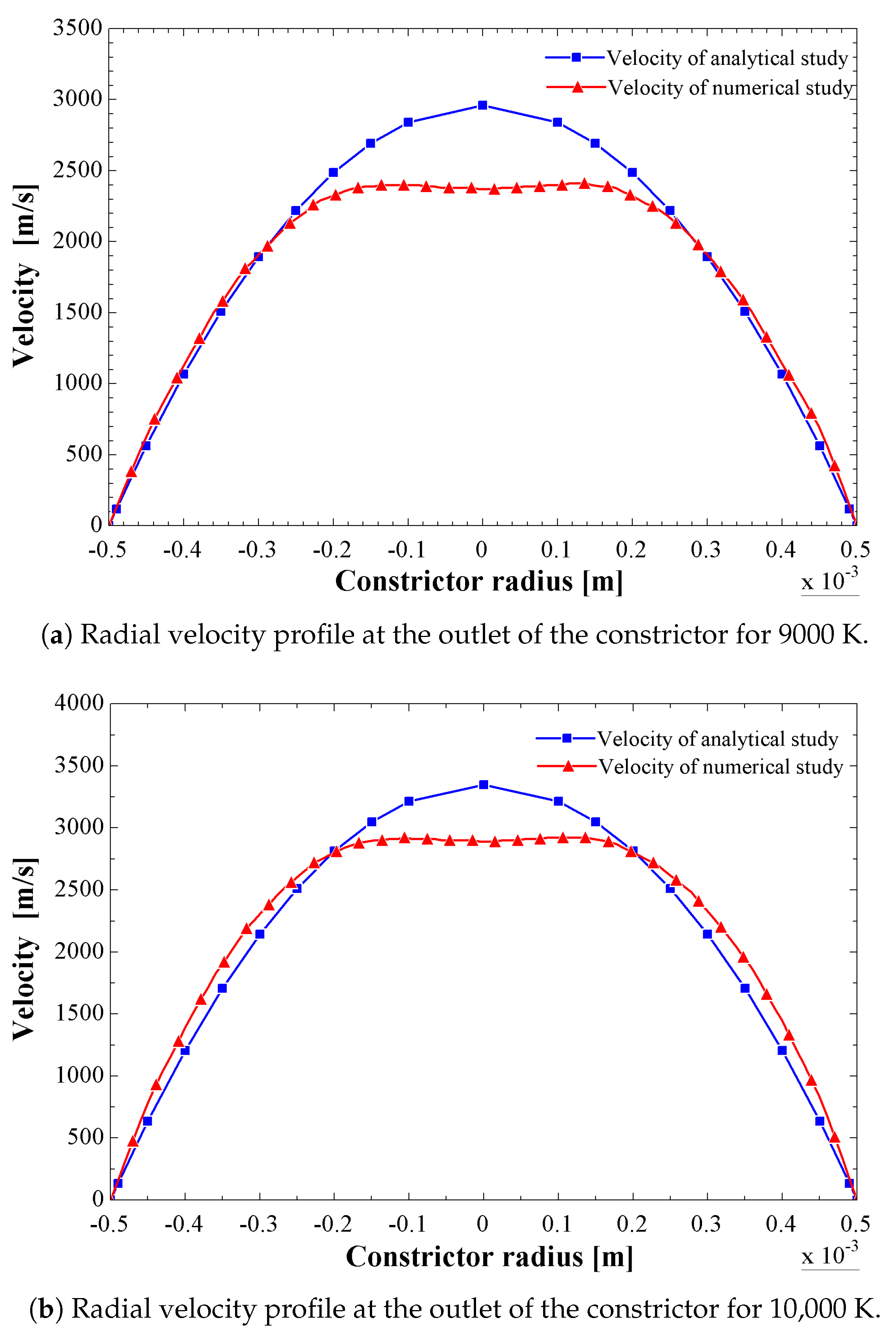
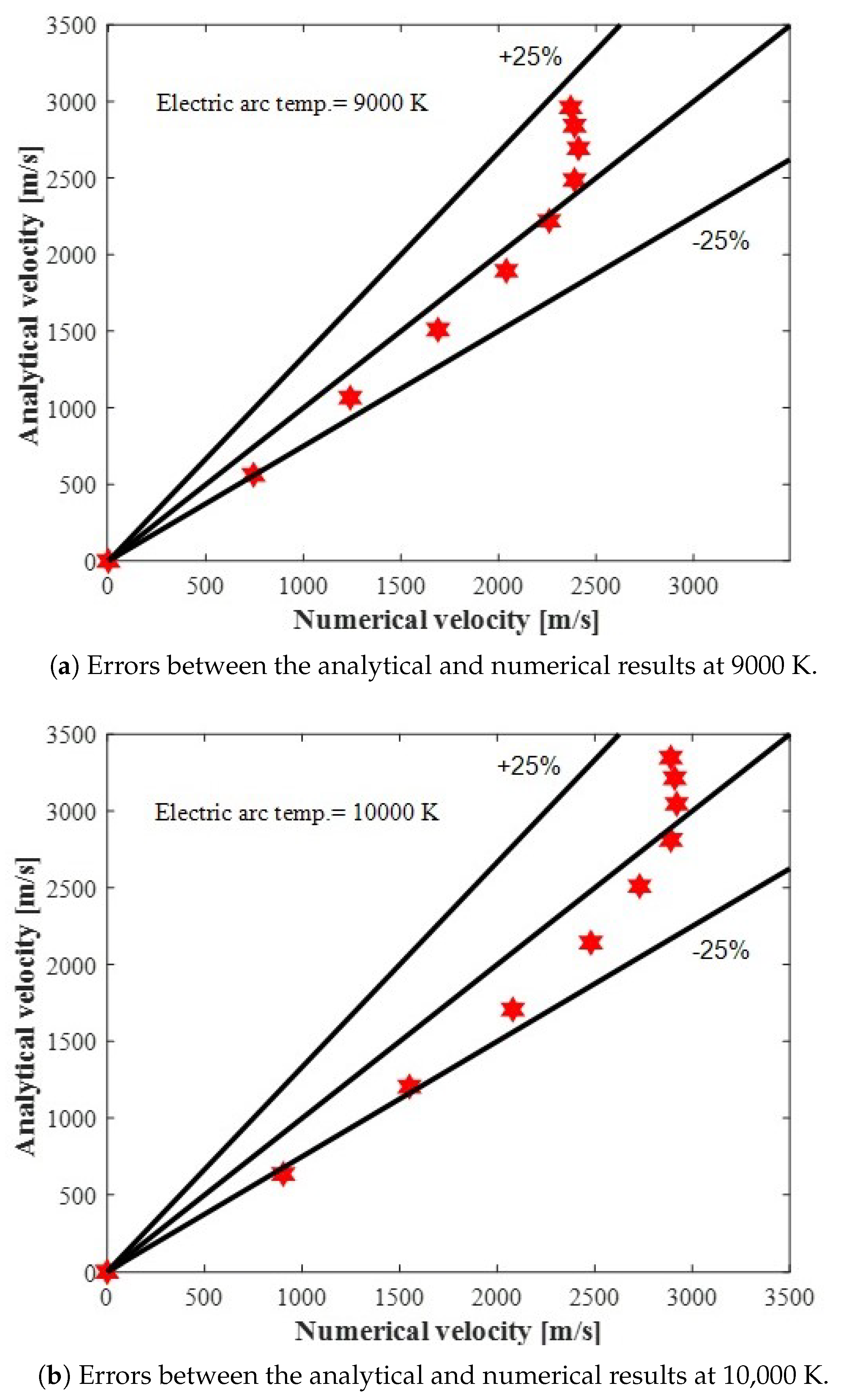
3.3. Comparison of Analytical and Numerical Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Guyot, M.; Denise, S.; Dusseau, L.; Saigné, F.; Bernard, M.; Claudé, P.; Dudeck, M. Electrostatic propulsion for satellites application to the Robusta-3 nanosatellite. IEEE Trans. Dielectr. Electr. Insul. 2014, 21, 1161–1165. [Google Scholar] [CrossRef]
- Bock, D.; Auweter-Kurtz, M.; Herdrich, G.; Kurtz, H.; Röser, H.P. An advanced ammonia propellant feed system for the thermal arcjet TALOS. In Proceedings of the 43rd AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Cincinnati, OH, USA, 8–11 July 2007; p. 5186. [Google Scholar]
- Wollenhaupt, B.; Hammer, A.; Herdrich, G.; Fasoulas, S.; Roser, H. A very low power arcjet (VELARC) for small satellite missions. In Proceedings of the 32nd International Electric Propulsion Conference, Wiesbaden, Germany, 11–15 September 2011; pp. 1988–2007. [Google Scholar]
- Skalden, J.; Herdrich, G.; Ehresmann, M.; Fasoulas, S. Development Progress of an Adaptable Deorbit System for Satellite Constellations. In Proceedings of the 36th International Electric Propulsion Conference, Vienna, Austria, 15–20 September 2019; pp. 15–20. [Google Scholar]
- National Aeronautics and Space Administration. Space Launch System Core Stage. 2021. Available online: https://www.nasa.gov/exploration/systems/sls/fs/core_stage.html (accessed on 1 January 2023).
- Kaminska, A.; Bialek, A.; Dudeck, M. Performances of an argon arcjet thruster for satellites. Rom. J. Phys. 2015, 60, 549–559. [Google Scholar]
- Goebel, D.M.; Katz, I. Fundamentals of Electric Propulsion: Ion and Hall Thrusters; John Wiley & Sons: Hoboken, NJ, USA, 2008. [Google Scholar]
- Bapat, A.; Salunkhe, P.B.; Patil, A.V. Hall-effect thrusters for deep-space missions: A review. IEEE Trans. Plasma Sci. 2022, 50, 189–202. [Google Scholar] [CrossRef]
- Sutton, G.P.; Biblarz, O. Rocket Propulsion Elements; John Wiley & Sons: Hoboken, NJ, USA, 2016. [Google Scholar]
- Kalentev, O.; Matyash, K.; Duras, J.; Lüskow, K.F.; Schneider, R.; Koch, N.; Schirra, M. Electrostatic Ion Thrusters-Towards Predictive Modeling. Contrib. Plasma Phys. 2014, 54, 235–248. [Google Scholar] [CrossRef]
- Oleson, S. Mission advantages of constant power, variable Isp electrostatic thrusters. In Proceedings of the 36th AIAA/ASME/SAE/ASEE Joint Propulsion Conference and Exhibit, Las Vegas, NV, USA, 24–28 July 2000; p. 3413. [Google Scholar]
- Mankavi, F.; Rezaeiha, A. Design and Development of a Low Power Laboratory Resistojet. In Proceedings of the Asian Joint Conference on Propulsion and Power (AJCPP2012), Xi’an, China, 1–4 March 2012. [Google Scholar]
- Cann, G.L.; Buhler, R.; Harder, R.L.; Moore, R. Basic Research on Gas Flows Through Electric Arcs-Hot Gas Containment Limits; Technical Report; Xerox Electro-Optical Systems: Pasadena, CA, USA, 1964. [Google Scholar]
- Wallner, L.E.; Czika, J., Jr. Arc-Jet Thrustor for Space Propulsion; Technical Report; National Aeronautics and Space Administration Lewis Research Center: Cleveland, OH, USA, 1965.
- Morris, J.C.; AVCO Corporation Members. Evaluation of High Temperature Gas Transport Properties. NASA Cr-575 1966, 129, 130–131. [Google Scholar]
- Kriebel, M.; Stevens, N. 30-kW class arcjet advanced technology transition demonstration (ATTD) flight experiment diagnostic package. In Proceedings of the 28th Joint Propulsion Conference and Exhibit, Nashville, TN, USA, 6–8 July 1992; p. 3561. [Google Scholar]
- King, L.A. Theoretical calculation of arc temperatures in different gases. Spectrochim. Acta 1956, 11, 152–161. [Google Scholar] [CrossRef]
- Goldenberg, H. Approximate solution of a non-linear differential equation giving the temperature distribution in the positive column of a static arc. Br. J. Appl. Phys. 1958, 10, 47–51. [Google Scholar] [CrossRef]
- Hansen, C.F. Approximations for the Thermodynamic and Transport Properties of High-Temperature Air; Number 4150; National Advisory Committee for Aeronautics: NC, USA, 1958. [Google Scholar]
- Stine, H.A.; Watson, V.R. The Theoretical Enthalpy Distribution of air in Steady Flow Along the Axis of a Direct-Current Electric Arc; National Aeronautics and Space Administration: Washington, DC, USA, 1962.
- Bauer, E. Physics of High-Temperature Air. Part 1. Basics. Final Report, October 1987–December 1988; Technical Report; Institute for Defense Analyses: Alexandria, VA, USA, 1990. [Google Scholar]
- Bauer, E. Physics of High-Temperature Air. Part. 2. Applications; Technical Report; Institute For Defense Analyses: Alexandria, VA, USA, 1990. [Google Scholar]
- Yamada, T.; Toki, K.; Kuriki, K. Behavior of Arc Column in Arcjet Constrictor; Institute of Space and Astronautical Science: Kanagawa, Japan, 2008. [Google Scholar]
- Heiermann, J.; Kurtz, M.A.; Slezion, P.; Herdrich, G. Numerical Evaluation of an Inductive Plasma Wind Tunnel Source on Structured and Unstructured Meshes; NASA Astrophysics Data System; National Aeronautics and Space Administration: Washington, DC, USA, 1988.
- Trelles, J.P.; Pfender, E.; Heberlein, J.V.R. Modelling of the arc reattachment process in plasma torches. J. Phys. D Appl. Phys. 2007, 40, 5635. [Google Scholar] [CrossRef]
- Lopez, B.; Barbosa, E.; Dudeck, M.; Izrar, B.; Kaminska, A. Modelling of a DC arc plasma source for the simulation of mars atmosphere around a spacecraft. Radiat. High Temp. Gases Atmos. Entry 2006, 629, 29. [Google Scholar]
- Gokcen, T.; Balboni, J.A.; Hartman, G.J. CFD Simulations for Arc-Jet Panel Testing Capability Development Using Semi-Elliptical Nozzles. AIAA Aviat. 2016, 2016, 3983. [Google Scholar]
- Selezneva, S.E.; Boulos, M.I.; Letourneur, K.G.Y.; Van Hest, M.F.A.M.; Van de Sanden, M.C.M.; Schram, D.C. Supersonically expanding cascaded arc plasma properties: Comparison of Ne, Ar and Xe. Plasma Sources Sci. Technol. 2003, 12, 107. [Google Scholar] [CrossRef]
- Wei, G.D.; Qi, X.; Yang, L. Modelling on dynamics properties of a stationary argon cascaded arc plasma flows. J. Appl. Phys. 2014, 21, 03350. [Google Scholar] [CrossRef]
- Ardakani, E.S. Numerical and Experimental Study of the Arc Fluctuations in a DC Plasma Torch. Ph.D. Thesis, University of Toronto, Toronto, ON, USA, 2016. [Google Scholar]
- Morel, T. Comprehensive Design of Axisymmetric Wind Tunnel Contractions. ASME J. Fluids Eng. 1975, 97, 225–233. [Google Scholar] [CrossRef]
- National Aeronautics and Space Administration. What Are SmallSats and CubeSats? 2017. Available online: https://www.nasa.gov/content/what-are-smallsats-and-cubesats (accessed on 30 November 2022).
- Cengel, Y.A.; Cimbala, J.M. Mecánica de Fluidos Fundamentos y Aplicaciones; The McGrawHill: New York, NY, USA, 2010. [Google Scholar]
- D’angola, A.; Colonna, G.; Gorse, C.; Capitelli, M. Thermodynamic and transport properties in equilibrium air plasma in a wide pressure and temperature range. Eur. Phys. J. D 2008, 46, 129–150. [Google Scholar] [CrossRef]
- Bird, R.B.; Stewart, W.E. Transport Phenomena, 2nd ed.; John Wiley & Sons Inc.: Hoboken, NJ, USA, 2002. [Google Scholar]
- Westermoen, A. Modelling of Dynamic Arc Behaviour in a Plasma Reactor; Norwegian University of Science and Technology Facuty of Natural Sciences and Technology Department of Material Sciences and Engineering: Trondheim, Norway, 2007. [Google Scholar]
- Karetta, F.; Lindmayer, M. Simulation of the gasdynamic and electromagnetic processes in low voltage switching arcs. IEEE Trans. Components Packag. Manuf. Technol. Part A 1996, 21, 35–44. [Google Scholar]
- Mutzkea, A.; Ruther, T.; Lindmayer, M. Arc behavior in low-voltage arc chambers. Eur. Phys. J. Appl. Phys. 2010, 49, 133. [Google Scholar] [CrossRef]
- Román-Roldán, N.; Yudonago, J.I.; López-Ortiz, A.; Rodríguez-Ramírez, J.; Sandoval-Torres, S. A new air recirculation system for homogeneous solar drying: Computational fluid dynamics approach. Renew. Energy 2021, 179, 1727–1741. [Google Scholar] [CrossRef]
- Ansys Fluent. ANSYS Fluent Magnetohydrodynamics (MHD) Module Manual, Release 15.0; Ansys Fluent Inc.: Canonsburg, PA, USA, 2013; Available online: https://www.scribd.com/document/284401251/ANSYS-Fluent-Magnetohydrodynamics-MHD-Module-Manual# (accessed on 2 April 2023).
- Cruz, J.C.B. Characterization of Arc Extinction in Direct Current Residential Circuit Breakers; Instituto Tecnológico y de Estudios Superiores de Monterrey: Monterrey, Mexico, 2018. [Google Scholar]
- Ansys Fluent. Fluent 14.0 User’s Guide; Ansys Fluent Inc.: Canonsburg, PA, USA, 2011. [Google Scholar]
- Boulos, M.I.; Fauchais, P.; Pfender, E. Thermal Plasma: Fundamentals and Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Trelles, J.P.; Heberlein, J.; Pfender, E. Non-equilibrium modelling of arc plasma torches. J. Phys. D Appl. Phys. 2007, 40, 5937. [Google Scholar] [CrossRef]
- Hameurlaine, K. Contribution to the Study of a Lower Power Electric Arc; Université d’Orléans: Orléans, France, 2012. [Google Scholar]
- Islas-Narvaez, E.A.; Ituna-Yudonago, J.F.; Ramos-Velasco, L.E.; Vega-Navarrete, M.A.; Garcia-Salazar, O. Design and Determination of Aerodynamic Coefficients of a Tail-Sitter Aircraft by Means of CFD Numerical Simulation. Machines 2022, 11, 17. [Google Scholar] [CrossRef]
- Horisawa, H.; Kimura, I.; Horisawa, H.; Kimura, I. Optimization of arc constrictor sizes in low power arcjet thrusters. In Proceedings of the 33rd Joint Propulsion Conference and Exhibit, Seattle, WA, USA, 6–9 July 1997; p. 3202. [Google Scholar]
- Sun, W.P.; Wei, F.Z.; Wang, H.X. Numerical Simulation of Nonequilibrium Flow in a Low-Power Hydrogen Arcjet. In Proceedings of the 33rd International Electric Propulsion Conference, Washington, DC, USA, 6–10 October 2013. [Google Scholar]
- Powell, K.; Gallimore, A.; Van Leer, B. A Numerical and Experimental Study of Arcjet Fluctuation; Technical Report; Department of Aerospace Engineering, Michigan University Ann Arbor: Ann Arbor, MI, USA, 1999. [Google Scholar]
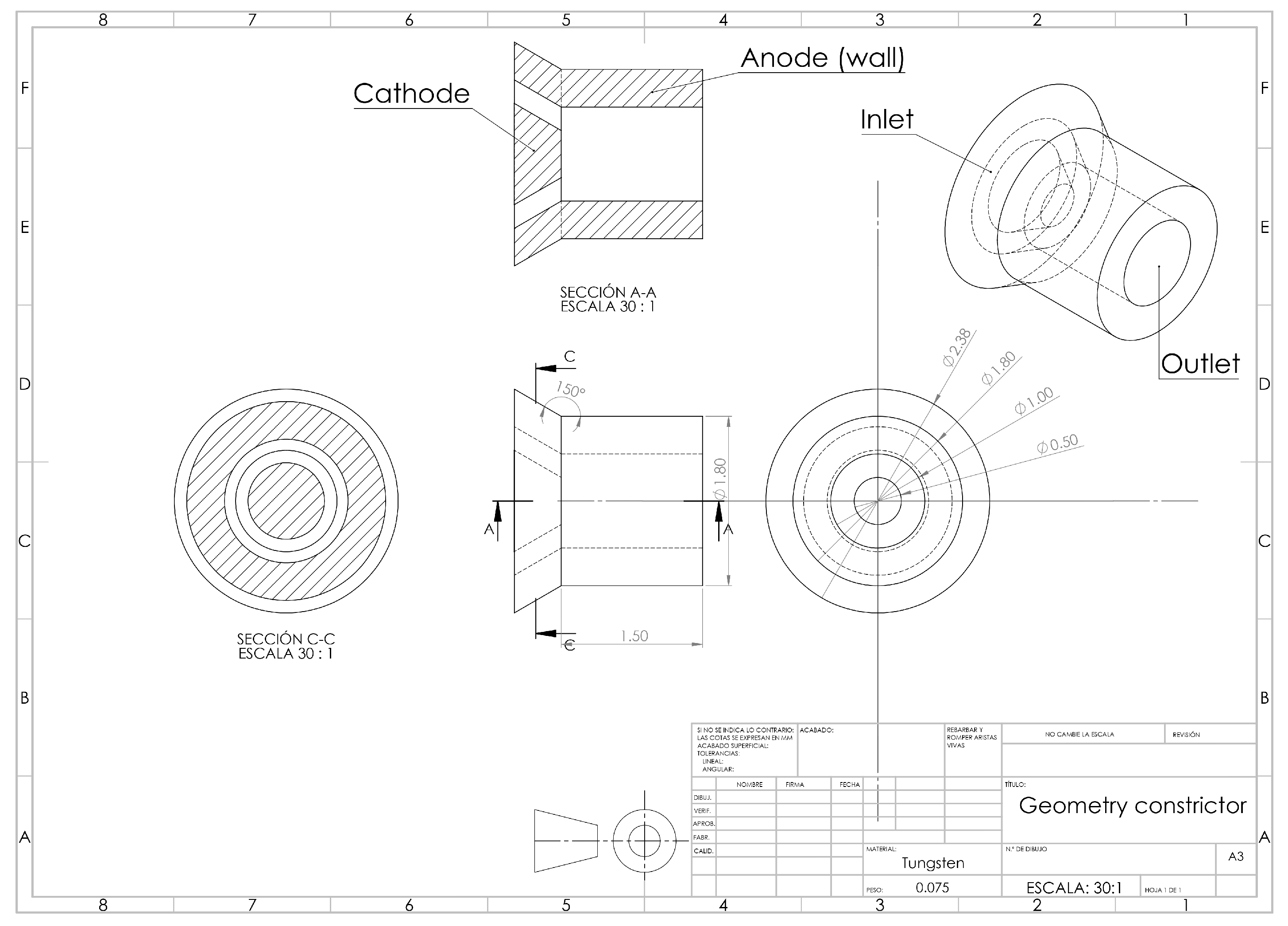

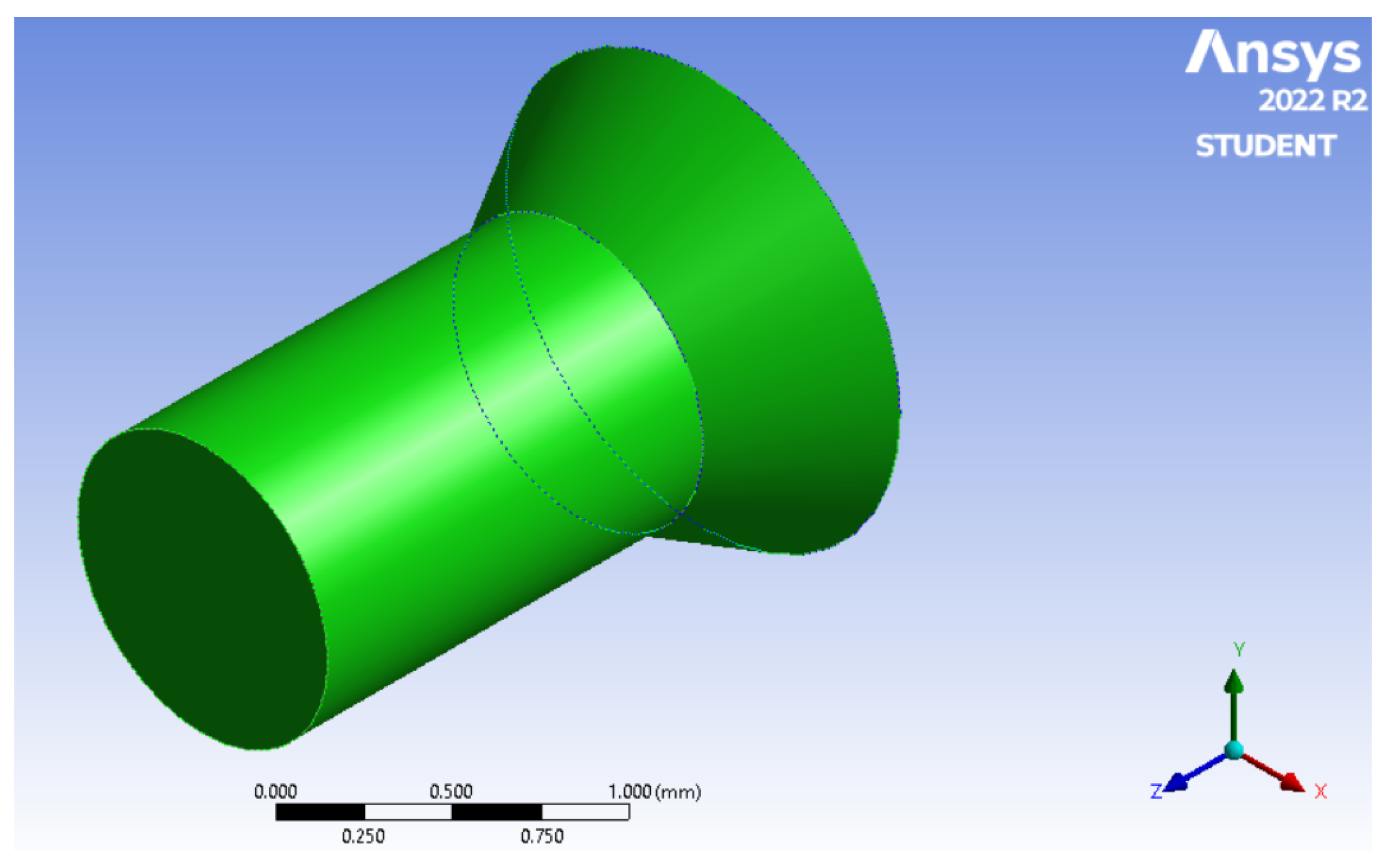
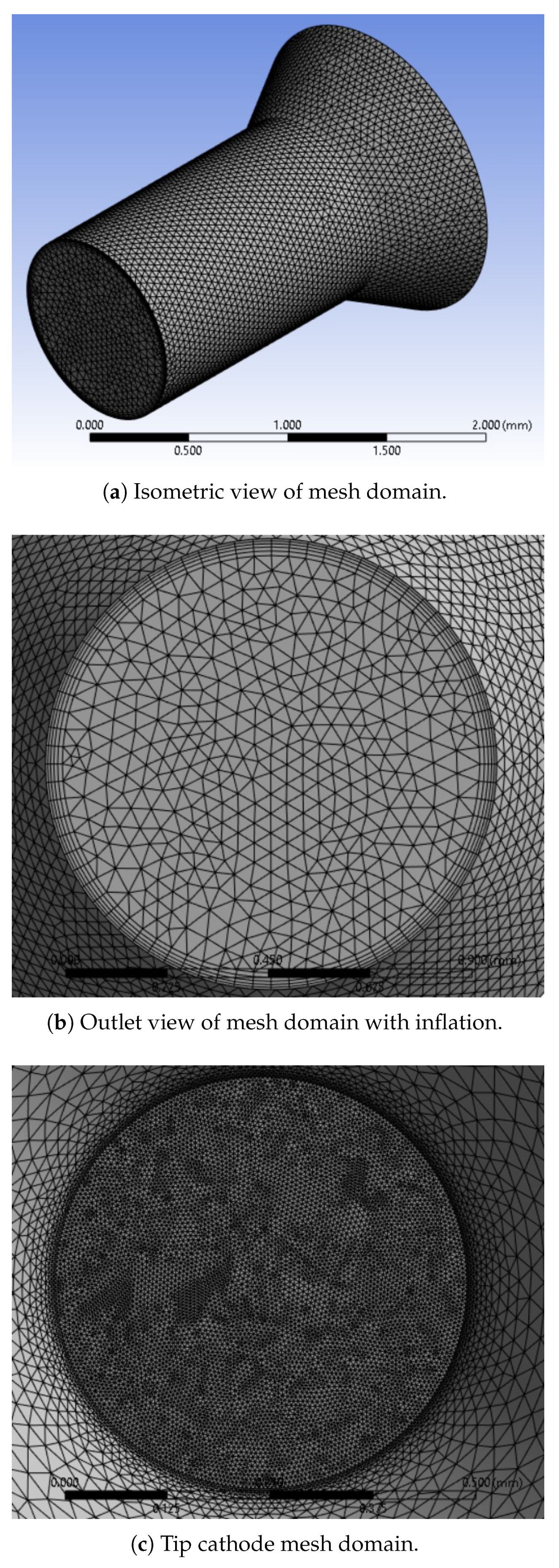

| Constrictor | Constrictor dimensions | |
| 1.5 | ||
| 1 mm | ||
| 1.5 mm | ||
| x | 0.25 mm | |
| t | 0.4 mm | |
| Inlet | Inlet dimensions | |
| 0.5 mm | ||
| 0.25 mm | ||
| Inlet | Outlet | Cathode | Cathode Tip | Anode | |
|---|---|---|---|---|---|
| P | = 101,325 Pa | = 63,284 Pa | |||
| 0 | 0 | 0 | |||
| T | 300 K | T = 1000 K | |||
| 0 | |||||
| 0 | 0 |
| Features | Mesh Size | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| M1 | M2 | M3 | M4 | M5 | M6 | M7 | M8 | M9 | |
| Nodes | 295 | 2130 | 9616 | 19,254 | 38,129 | 55,856 | 75,212 | 83,544 | 91,512 |
| Elements | 1011 | 10,227 | 50,429 | 102,093 | 204,791 | 300,551 | 405,049 | 450,898 | 495,706 |
| Mesh metric Skewness | |||||||||
| Minimum | 5.4575 | 1.3471 | 2.761 | 1.0203 | 7.5313 | 3.4219 | 1.9023 | 3.2377 | 8.7762 |
| Maximum | 0.71195 | 0.72575 | 0.79156 | 0.79982 | 0.79544 | 0.79442 | 0.79584 | 0.7915 | 0.83724 |
| Avarage | 0.23188 | 0.23615 | 0.23782 | 0.2318 | 0.22688 | 0.22492 | 0.22289 | 0.22293 | 0.21789 |
| Standard deviation | 0.1181 | 0.12525 | 0.12334 | 0.1211 | 0.11913 | 0.11825 | 0.11747 | 0.1176 | 0.11674 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Argumedo Teuffer, P.J.; Marron Ramirez, L.E.; Ituna-Yudonago, J.F.; Arizpe Carreón, P.A.; Rodríguez Roman, C.M. Geometric Design of a Low-Power Arcjet Constrictor and Determination of Velocity of Air-Based Plasma by Means of Analytical and Numerical Methods. Machines 2023, 11, 527. https://doi.org/10.3390/machines11050527
Argumedo Teuffer PJ, Marron Ramirez LE, Ituna-Yudonago JF, Arizpe Carreón PA, Rodríguez Roman CM. Geometric Design of a Low-Power Arcjet Constrictor and Determination of Velocity of Air-Based Plasma by Means of Analytical and Numerical Methods. Machines. 2023; 11(5):527. https://doi.org/10.3390/machines11050527
Chicago/Turabian StyleArgumedo Teuffer, Pedro José, Luis Enrique Marron Ramirez, Jean Fulbert Ituna-Yudonago, Pablo Alejandro Arizpe Carreón, and Carlos Manuel Rodríguez Roman. 2023. "Geometric Design of a Low-Power Arcjet Constrictor and Determination of Velocity of Air-Based Plasma by Means of Analytical and Numerical Methods" Machines 11, no. 5: 527. https://doi.org/10.3390/machines11050527
APA StyleArgumedo Teuffer, P. J., Marron Ramirez, L. E., Ituna-Yudonago, J. F., Arizpe Carreón, P. A., & Rodríguez Roman, C. M. (2023). Geometric Design of a Low-Power Arcjet Constrictor and Determination of Velocity of Air-Based Plasma by Means of Analytical and Numerical Methods. Machines, 11(5), 527. https://doi.org/10.3390/machines11050527








