Multi-Objective Optimization of Magnetorheological Mount Considering Optimal Damping Force and Maximum Adjustable Coefficient
Abstract
1. Introduction
2. Materials and Methods
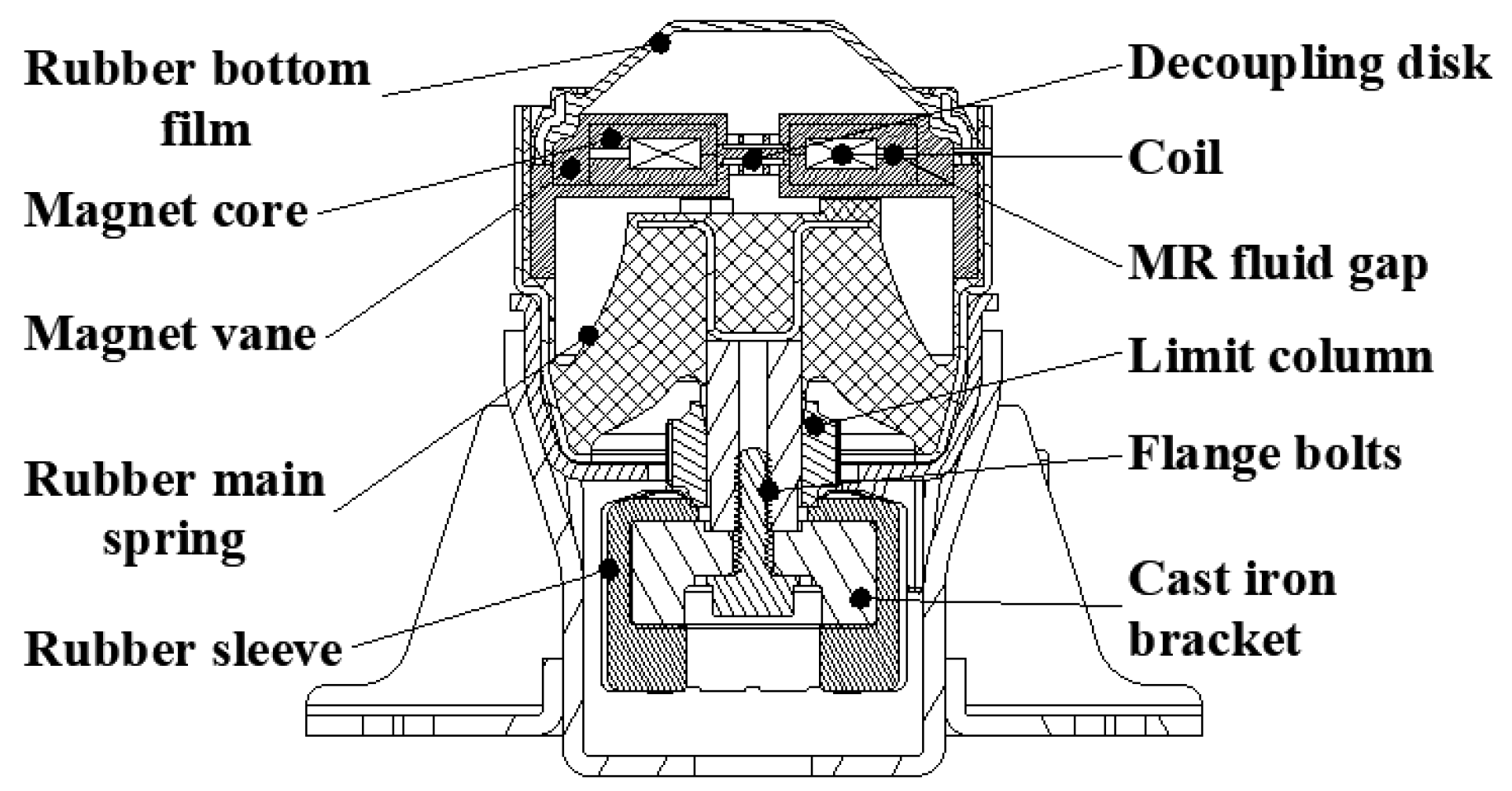
2.1. Magnetorheological Mount Structure and Working Principle
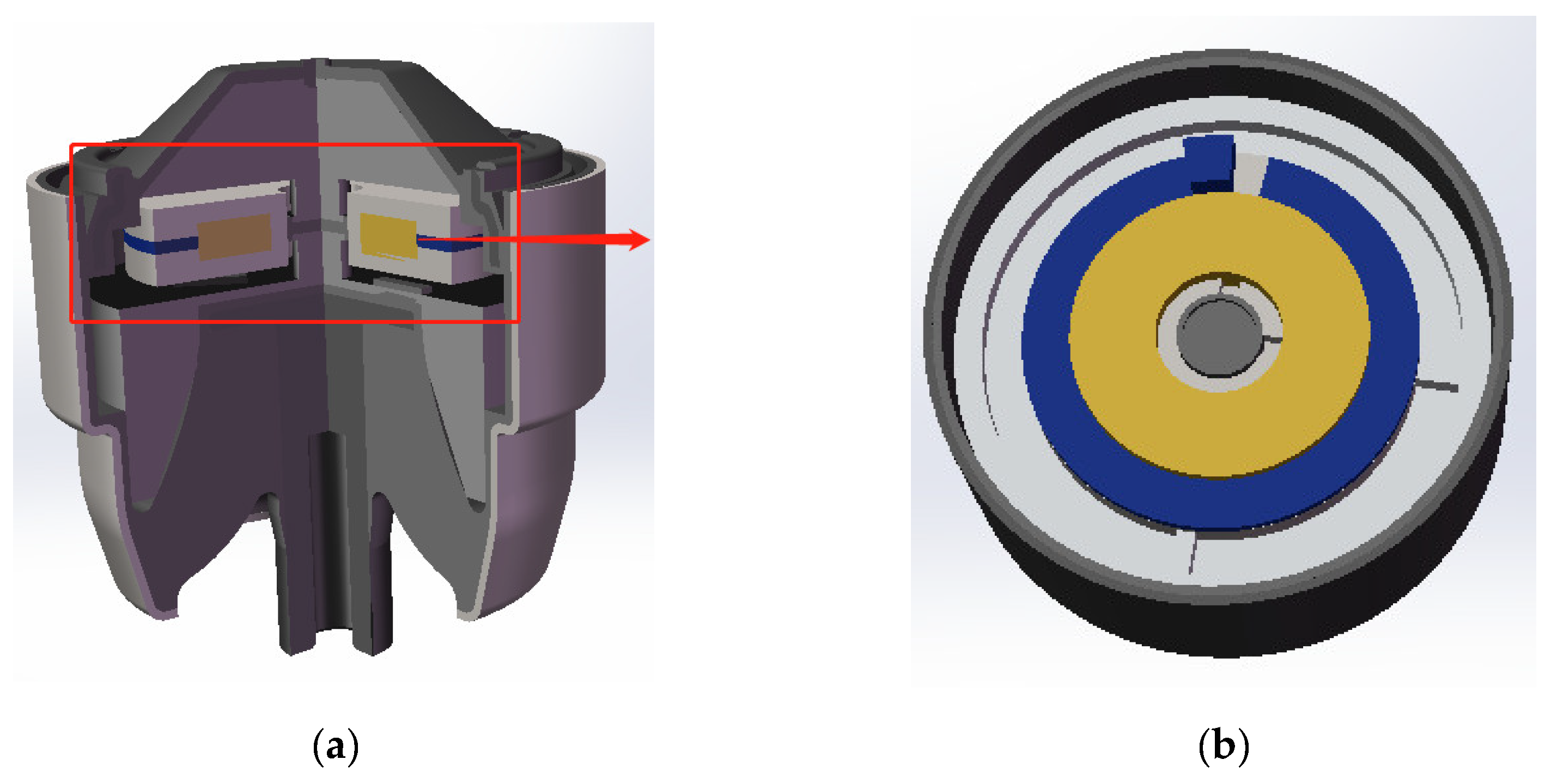
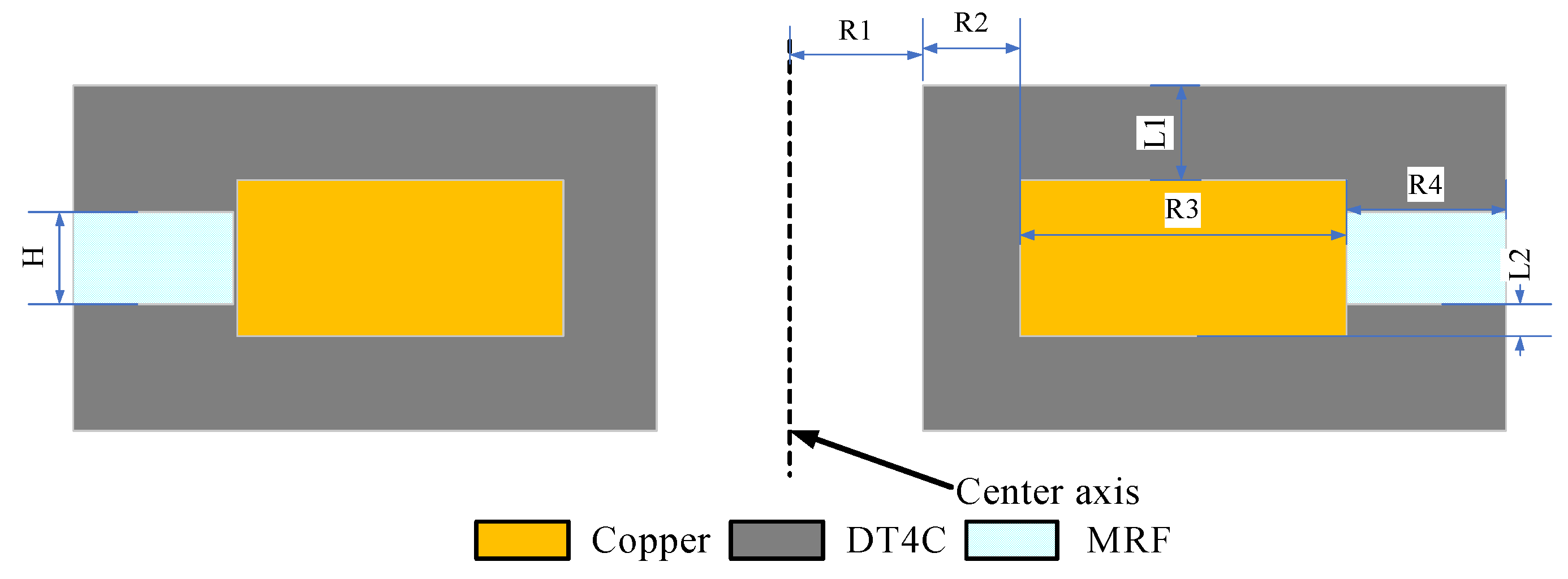
2.2. Magnetic Circuit Structure and Model Simplification
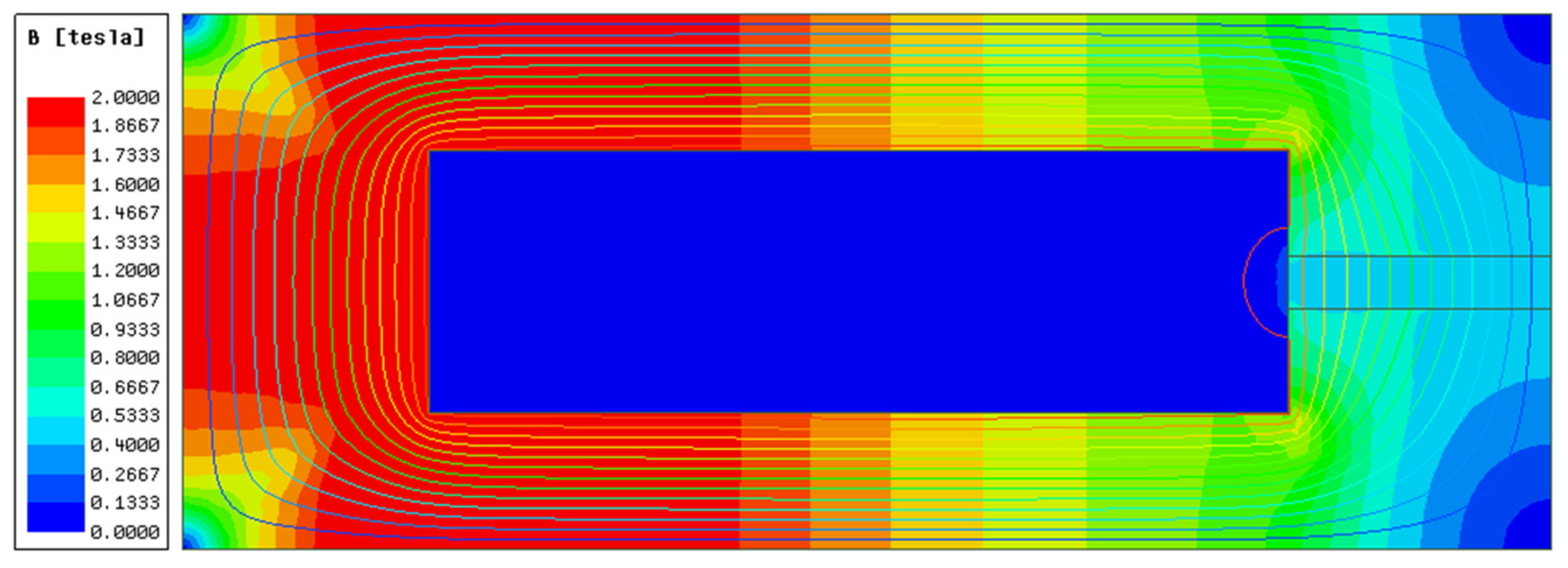
2.3. Magnetic Field Finite Element Simulation
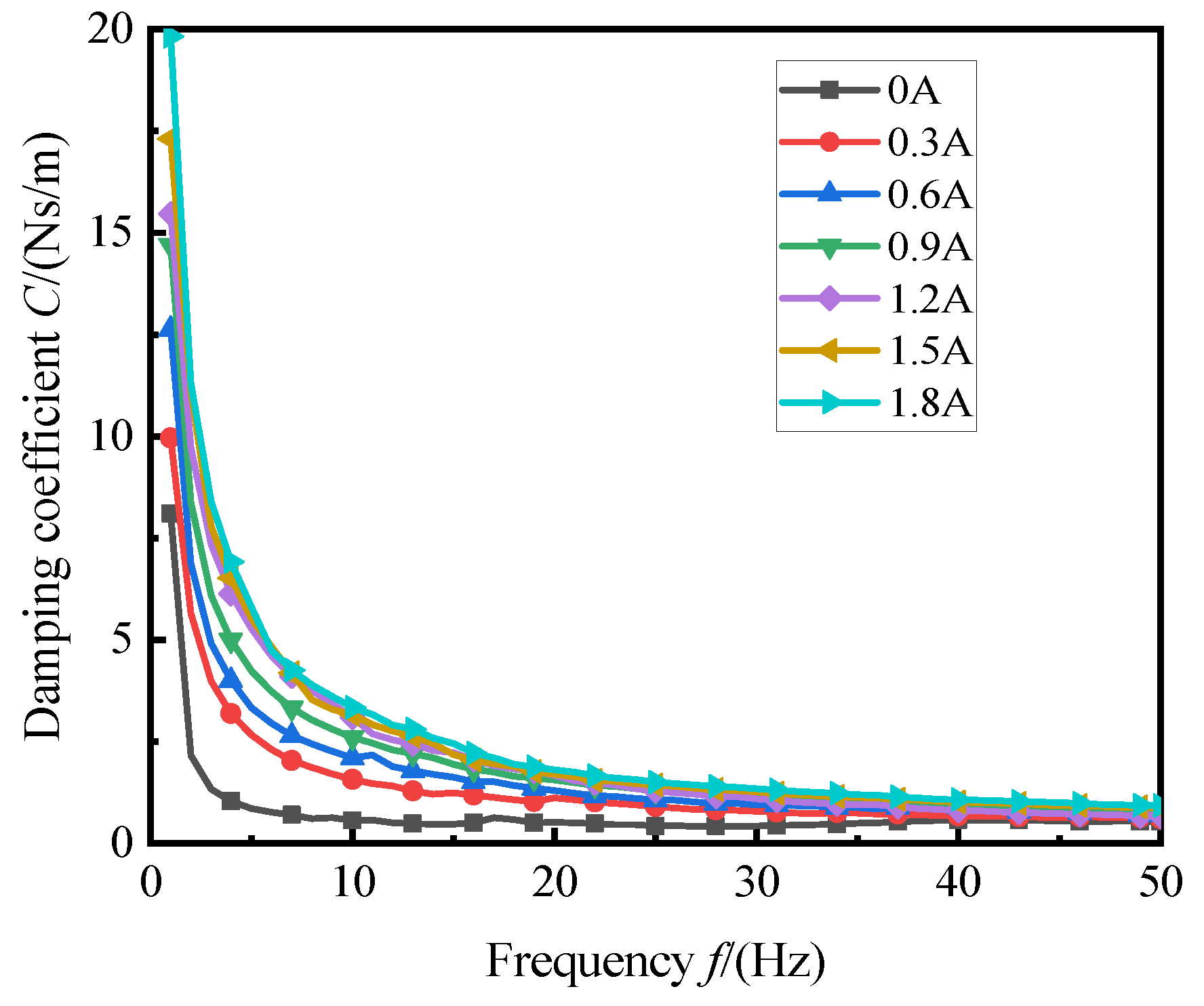
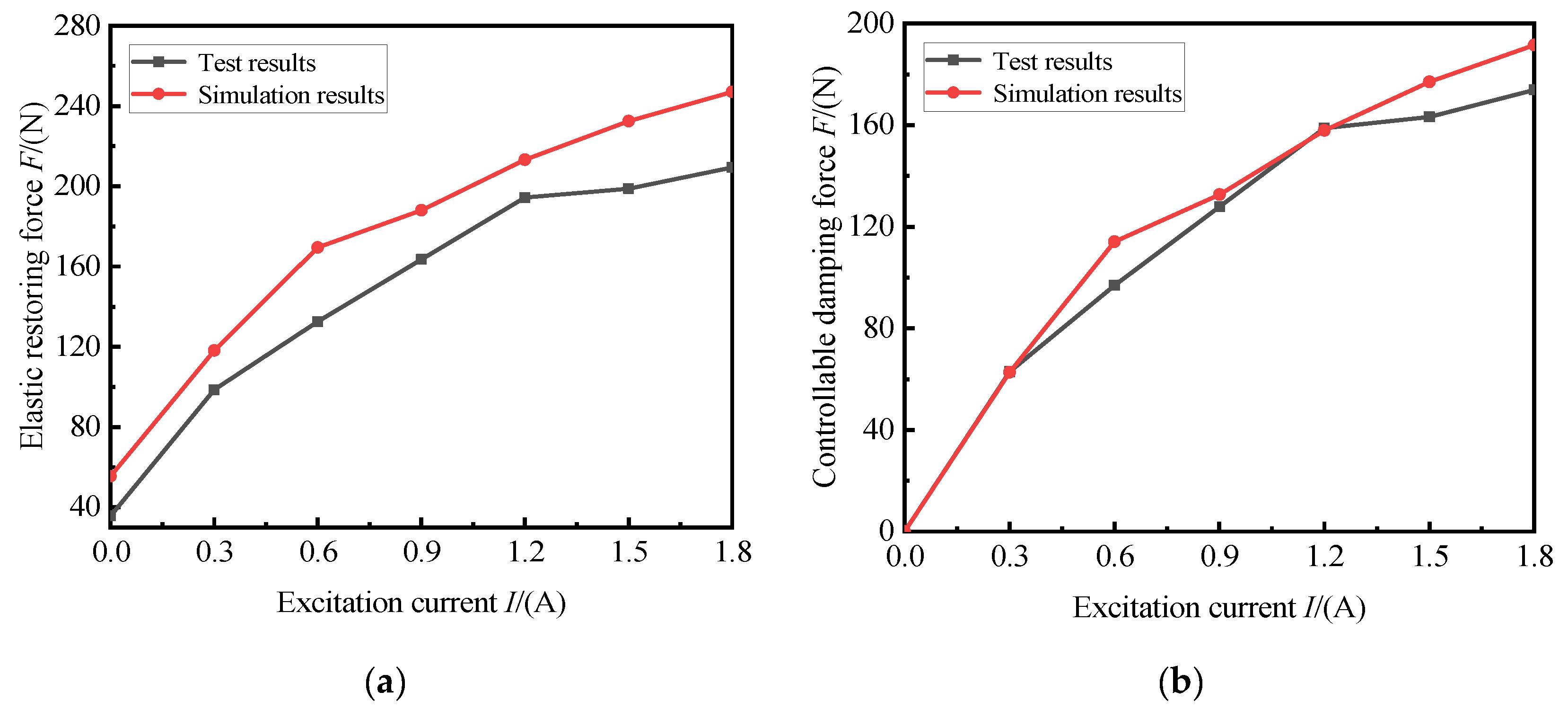
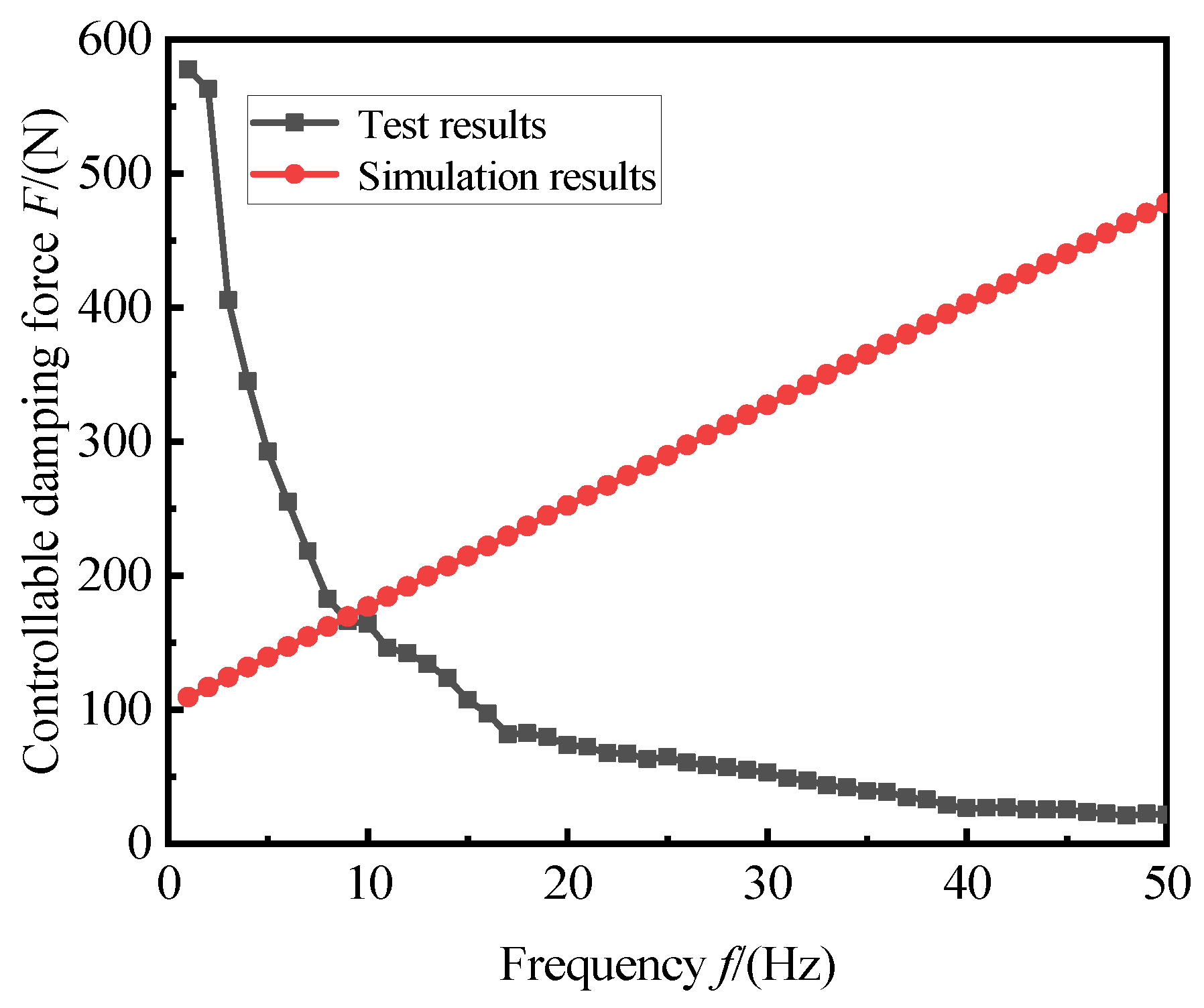
2.4. Model Verification and Correction
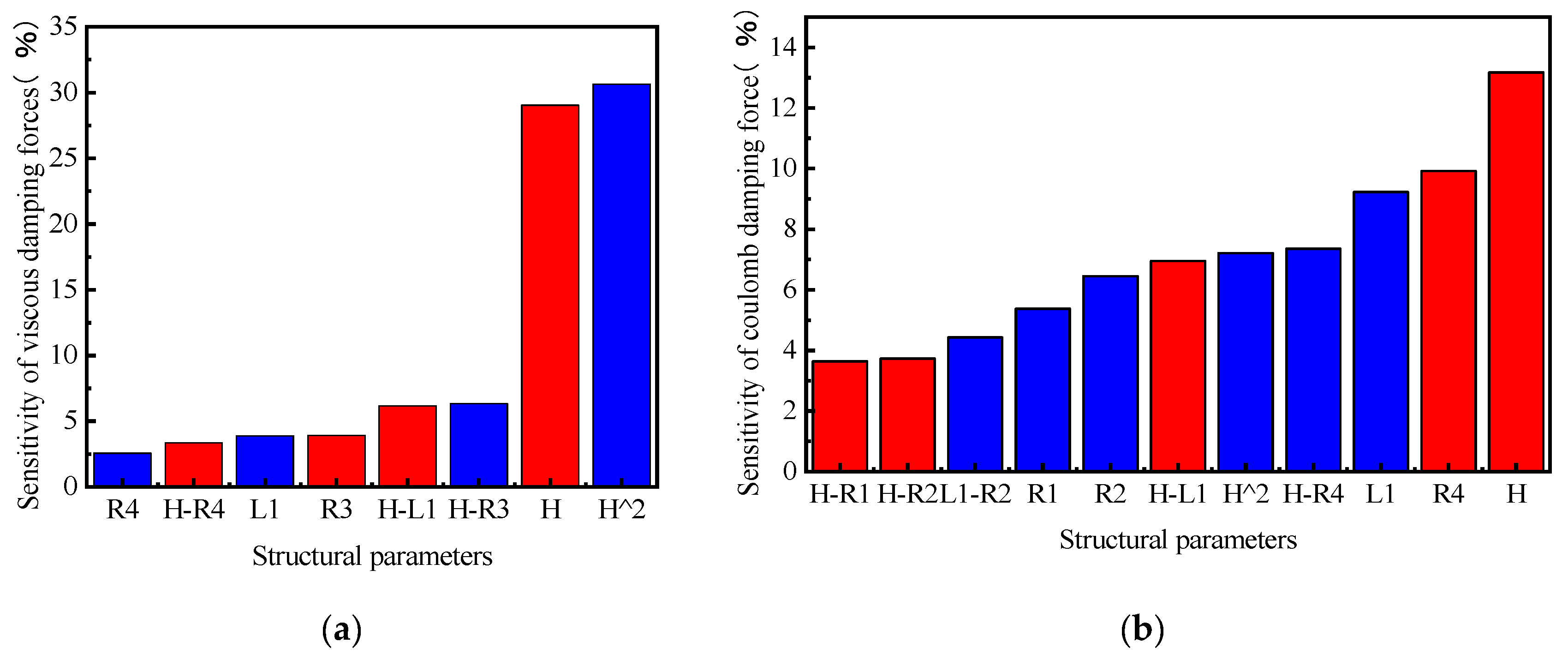
3. Analysis of Sensitivity
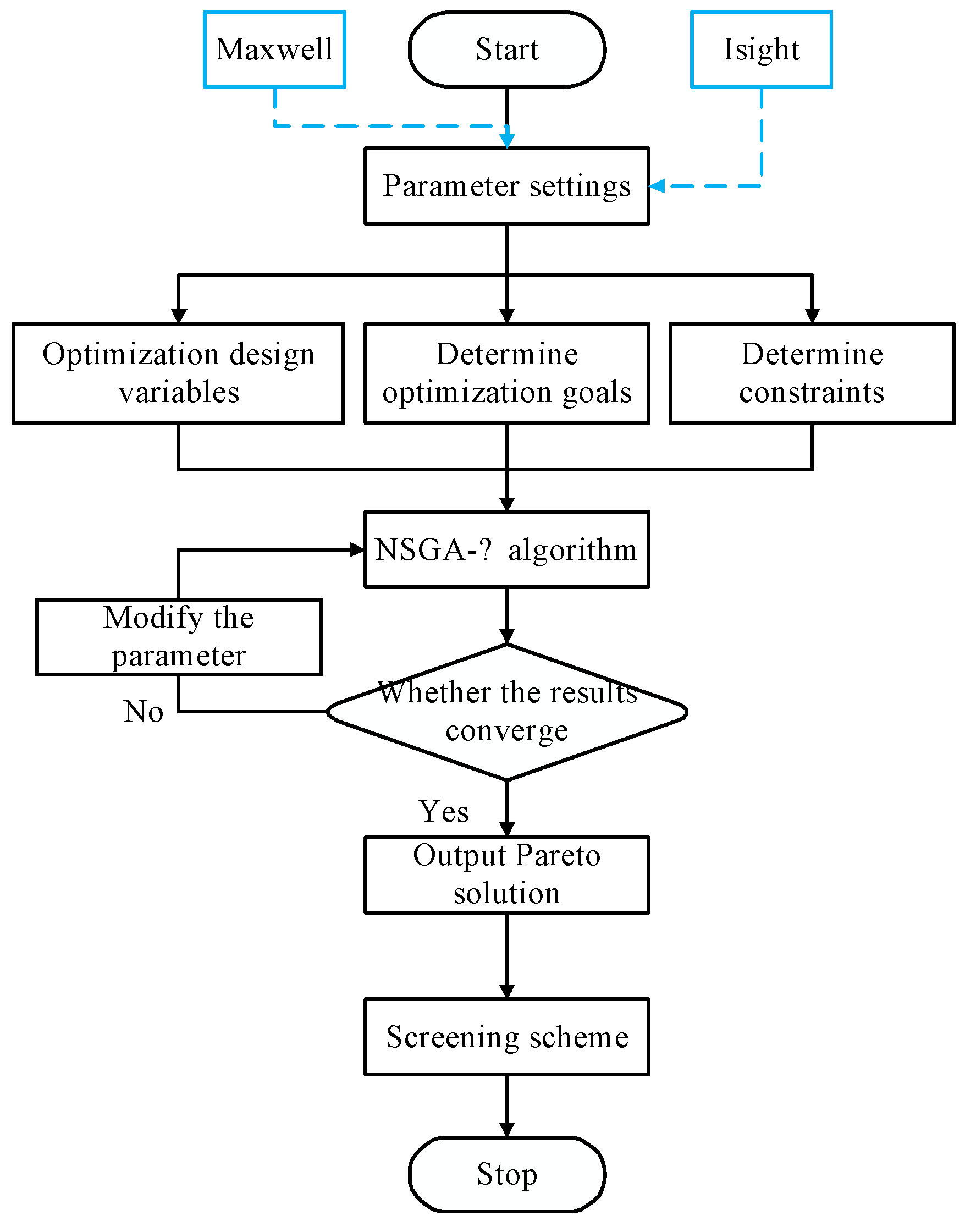
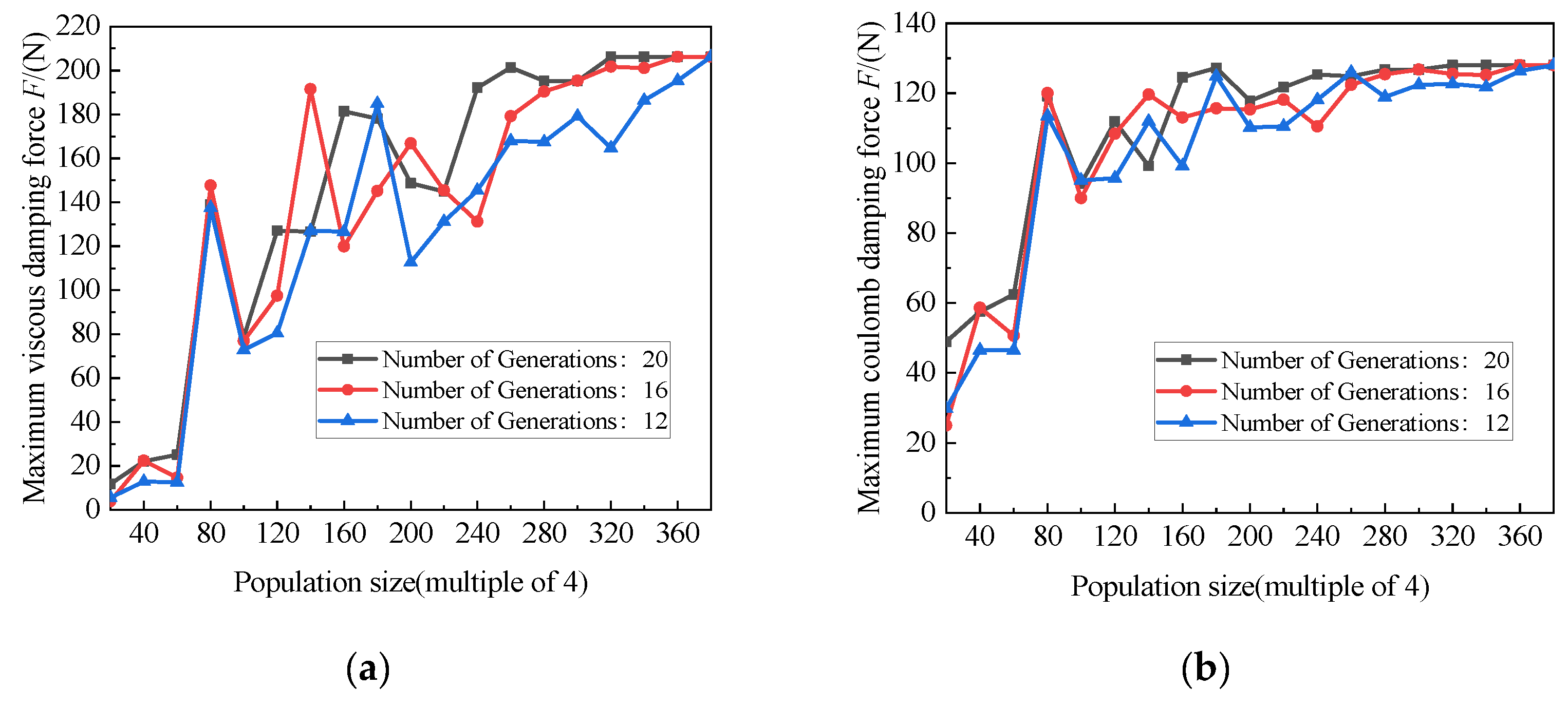
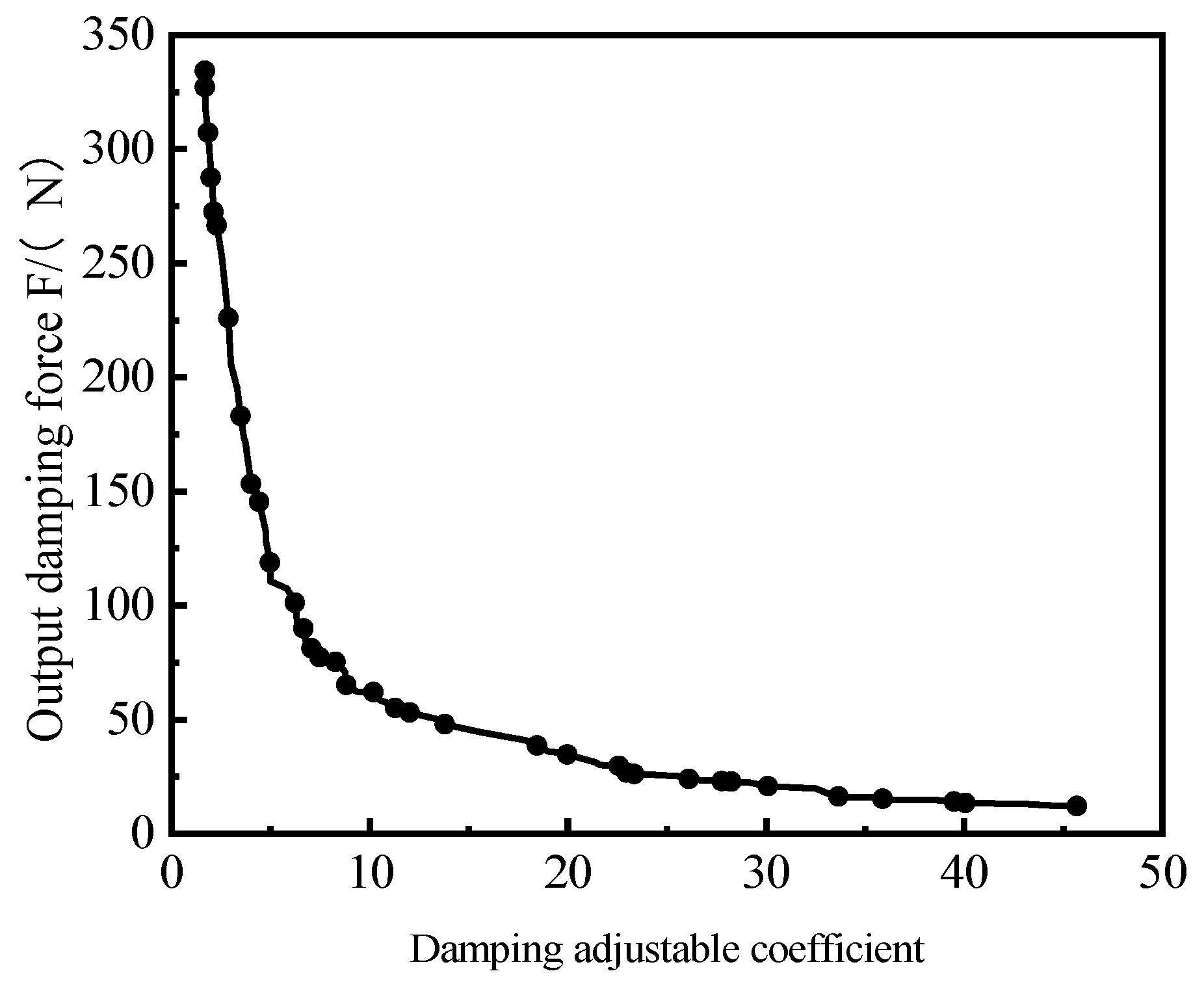
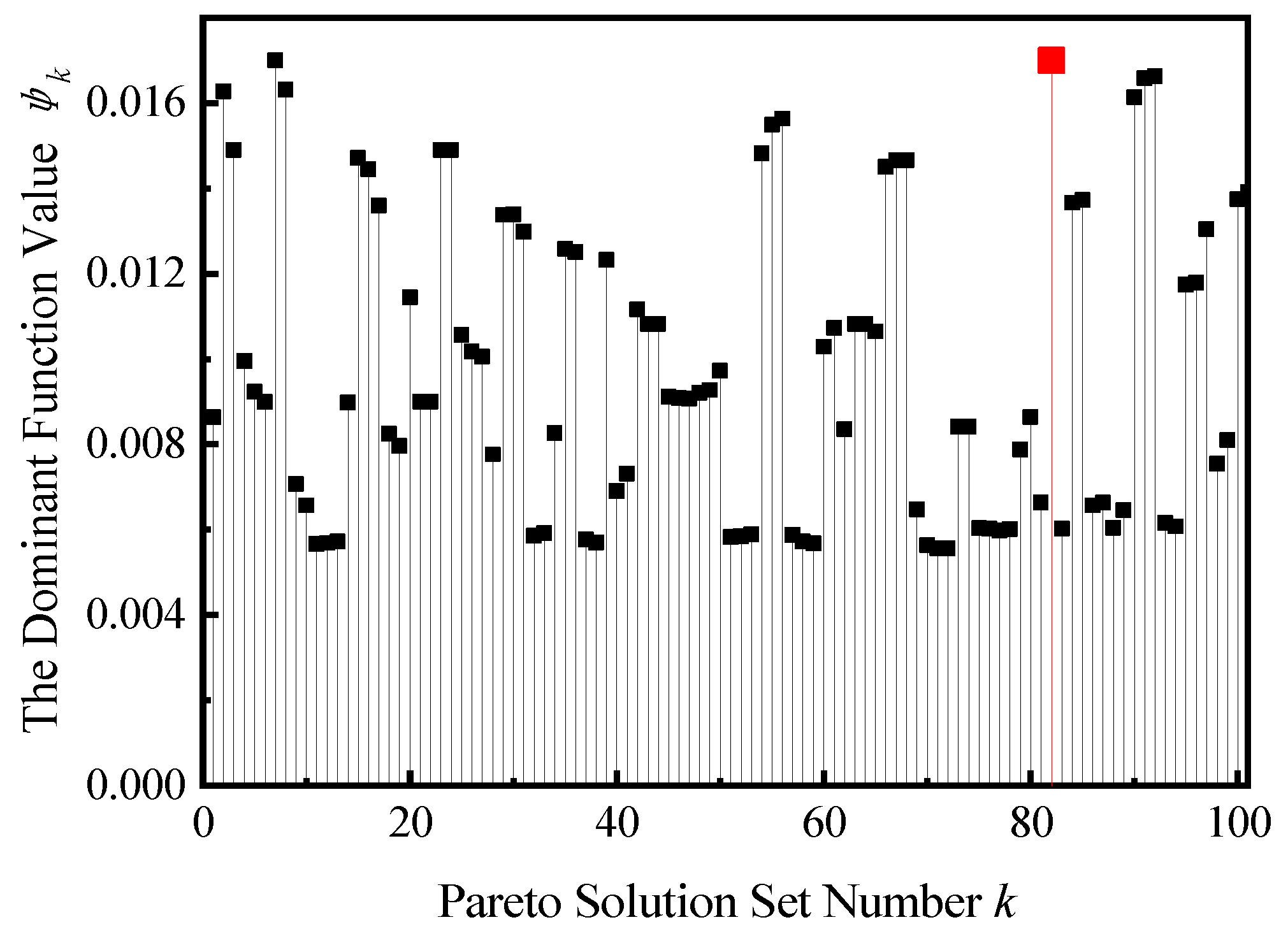
4. Multi-Objective Optimization Design of Magnetic Circuits
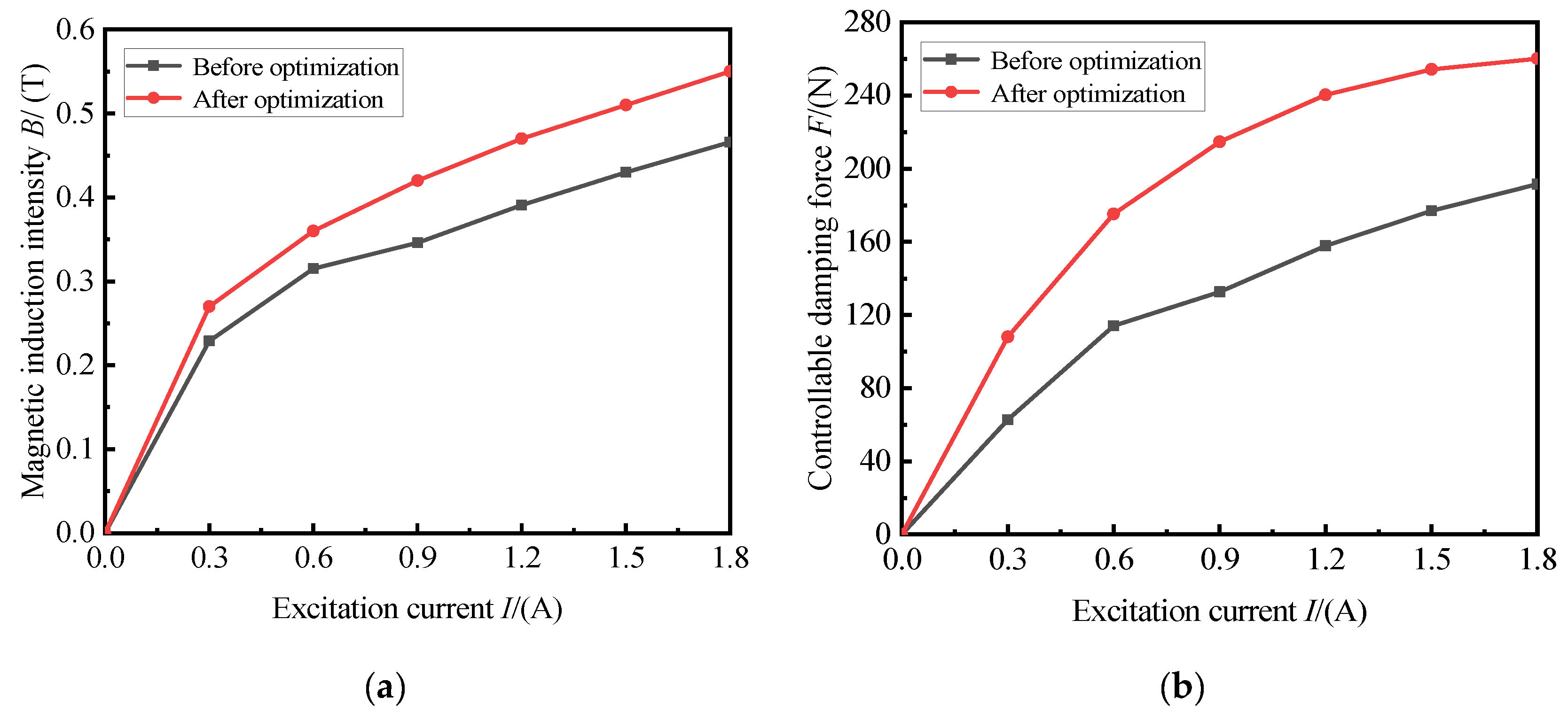
5. Comparison of Results
6. Conclusions
- Simplifying the magnetic circuit structure into a two-dimensional axisymmetric model can reduce the calculation time of the model and effectively improve the calculation speed during joint simulation optimization by ensuring accuracy. At the same time, most fluid models used today are steady-state models with certain errors, and the accuracy of the model can be further improved by fitting the extended equation. The experimental simulation comparison method described in this paper can compare the errors of theoretical and practical models from different frequency perspectives.
- Combined with the theoretical analysis and simulation verification, the core depth L2 has the least influence on the damping force. The results show that the influence of L2 on the coulomb damping force and viscous damping force is much less than 4%, which can be ignored in the structural optimization. The coulomb damping force is most correlated with the cross-sectional area and length of the inertial channel, that is, the height of the inertial channel H and the width of the inertial channel R4.
- The test results indicate that the simulation model is consistent with the change trend in the actual mount damping force, and the controllable force values are similar. After optimization, the average magnetic induction strength is increased by 18.60%, and the output damping force reaches 336.19 N. When the current is increased from 1.5 A to 1.8 A, the controllable force increased by only 2.26%, indicating that the damping performance was fully exerted at 1.5 A, which is in line with the design expectations.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Eshgarf, H.; Ahmadi Nadooshan, A.A.; Raisi, A. An Overview on Properties and Applications of Magnetorheological Fluids: Dampers, Batteries, Valves and Brakes. J. Energy Storage 2022, 50, 104648. [Google Scholar] [CrossRef]
- Pei, P.; Peng, Y.B. Constitutive Modeling of Magnetorheological Fluids: A Review. J. Magn. Magn. Mater. 2022, 550, 15. [Google Scholar] [CrossRef]
- Dassisti, M.; Brunetti, G. Introduction to Magnetorheological Fluids. Encycl. Smart Mater. 2022, 5, 187–202. [Google Scholar]
- Wu, P.; Ma, Q.H.; Zhu, J.; Liang, H.Y. The Review of the Application of Magneto-Rheological Fluid and Engineering. Math. Model. Eng. Probl. 2016, 3, 63–66. [Google Scholar] [CrossRef]
- Liu, G.Y.; Gao, F.; Liao, W.-H. Design and Optimization of a Magnetorheological Damper Based on B-Spline Curves. Mech. Syst. Signal Process. 2022, 178, 109279. [Google Scholar] [CrossRef]
- Zhu, X.; Jing, X.; Cheng, L. Magnetorheological Fluid Dampers: A Review on Structure Design and Analysis. J. Intell. Mater. Syst. Struct. 2012, 23, 839–873. [Google Scholar] [CrossRef]
- Rama Raju, K.; Vineeth Varma, D. Developments in Vibration Control of Structures and Structural Components with Magnetorheological Fluids. Curr. Sci. 2017, 112, 499–508. [Google Scholar] [CrossRef]
- Ahamed, T.I.; Sundarrajan, R.; Prasaath, G.T.; Raviraj, V. Implementation of Magneto-Rheological Dampers in Bumpers of Automobiles for Reducing Impacts During Accidents. Procedia Eng. 2014, 97, 1220–1226. [Google Scholar] [CrossRef]
- El Majdoub, K.; Ghani, D.; Giri, F.; Chaoui, F.Z. Adaptive Semi-active Suspension of Quarter-Vehicle with Magnetorheological Damper. J. Dyn. Syst. Meas. Control 2015, 137, 12. [Google Scholar] [CrossRef]
- Yang, J.; Ning, D.; Sun, S.S.; Zheng, J.; Lu, H.; Nakano, M.; Zhang, S.; Du, H.; Li, W.H. A Semi-active Suspension Using a Magnetorheological Damper with Nonlinear Negative-Stiffness Component. Mech. Syst. Signal Process. 2021, 147, 107071. [Google Scholar] [CrossRef]
- Bai, X.; Chen, P.; Qian, L.; Kan, P. Design and Analysis of a Magnetorheological Fluid Mount Featuring Uni-Directional Squeeze Mode. In Proceedings of the ASME 2015 Conference on Smart Materials, Adaptive Structures and Intelligent Systems, Colorado Springs, CO, USA, 21–23 September 2015; Volume 2, pp. 21–23. [Google Scholar]
- Lin, Z.; Wu, M. Dynamic Characterization of Controlled Multi-channel Semi-active Magnetorheological Fluid Mount. Mech. Sci. 2021, 12, 751–764. [Google Scholar] [CrossRef]
- Chung, J.U.; Phu, D.X.; Choi, S.-B. Optimization of New Magnetorheological Fluid Mount for Vibration Control of Start/stop Engine Mode. In Proceedings of the SPIE 9431, Active and Passive Smart Structures and Integrated Systems 2015, San Diego, CA, USA, 2 April 2015; p. 94311O. [Google Scholar]
- Zheng, L.; Duan, X.W.; Deng, Z.X.; Li, Y.N. Multi-objective Optimal Design of Magnetorheological Engine Mount Based on an Improved Non-dominated Sorting Genetic Algorithm. In Proceedings of the SPIE—The International Society for Optical Engineering, San Diego, CA, USA, 10–13 March 2014; Volume 9057, p. 0277786X. [Google Scholar]
- Deng, Z.; Yang, Q.; Yang, X. Optimal Design and Experimental Evaluation of Magneto-rheological Mount Applied to Start/stop Mode of Vehicle Powertrain. J. Intell. Mater. Syst. Struct. 2020, 31, 1126–1137. [Google Scholar] [CrossRef]
- Deng, Z.; Yang, Q.; Zhao, S.; Wei, H. Multi-objective Optimization of Magneto-rheological Mount Structure Based on Vehicle Vibration Control. J. Intell. Mater. Syst. Struct. 2021, 32, 1155–1166. [Google Scholar] [CrossRef]
- Deng, Z.; Cai, Q.; Li, Y.Q.; Wei, H.; Yang, Q.; Dong, S. Magnetic Circuit Design and Optimisation of Magnetorheological Mount with Tapered Channel under the Flow Mode. J. Intell. Mater. Syst. Struct. 2021, 32, 1614–1623. [Google Scholar] [CrossRef]
- Deng, Z.; Wei, X.; Li, X.; Zhao, S.; Zhu, S. Design and Multi-objective Optimization of Magnetorheological Damper Considering Vehicle Riding Comfort and Operation Stability. J. Intell. Mater. Syst. Struct. 2022, 33, 1215–1228. [Google Scholar] [CrossRef]
- Nie, S.L.; Xin, D.K.; Ji, H.; Yin, F.L. Optimization and Performance Analysis of Magnetorheological Fluid Damper Considering Different Piston Configurations. J. Intell. Mater. Syst. Struct. 2019, 30, 764–777. [Google Scholar] [CrossRef]
- Marathe, A.P.; Khot, S.M.; Nagler, J. Development of Low-Cost Optimal Magneto-Rheological Damper for Automotive Application. J. Vib. Eng. Technol. 2022, 10, 1831–1850. [Google Scholar] [CrossRef]
- Xu, F.H.; Dong, D.W.; Huang, Y.; Zhang, R.; Song, S.Z.; Zheng, D. A Comprehensive Optimal Design Method for Magnetorheological Dampers Utilized in DMU Power Package. Proc. Inst. Mech. Eng. Part L 2022, 236, 533–547. [Google Scholar] [CrossRef]
- Cheng, M.; Chen, Z.B.; Xing, J.W. Design, Analysis, and Experimental Evaluation of a Magnetorheological Damper with Meandering Magnetic Circuit. IEEE Trans. Magn. 2018, 54, 1–10. [Google Scholar] [CrossRef]
- Parlak, Z.; Engin, T.; Çallı, İ. Optimal Design of MR Damper via Finite Element Analyses of Fluid Dynamic and Magnetic Field. Mechatronics 2012, 22, 890–903. [Google Scholar] [CrossRef]
- Parlak, Z.; Engin, T.; Şahin, İ. Optimal Magnetorheological Damper Configuration Using the Taguchi Experimental Design Method. J. Mech. Des. 2013, 135, 081008. [Google Scholar] [CrossRef]
- Jiang, M.; Rui, X.; Yang, F.; Zhu, W.; Zhang, Y. Multi-objective Optimization Design for a Magnetorheological Damper. J. Intell. Mater. Syst. Struct. 2022, 33, 33–45. [Google Scholar] [CrossRef]
- Hu, G.L.; Liu, F.S.; Xie, Z.; Xu, M. Design, Analysis, and Experimental Evaluation of a Double Coil Magnetorheological Fluid Damper. Shock Vib. 2016, 2016, 1–12. [Google Scholar] [CrossRef]
- Ferdaus, M.M.; Rashid, M.M.; Hasan, M.H.; Rahman, M.A. Optimal Design of Magneto-Rheological Damper Comparing Different Configurations by Finite Element Analysis. J. Mech. Sci. Technol. 2014, 28, 3667–3677. [Google Scholar] [CrossRef]
- Dong, Z.Z.; Feng, Z.M.; Chen, Y.H.; Yu, F.; Zhang, G. Design and Multiobjective Optimization of Magnetorheological Damper Considering the Consistency of Magnetic Flux Density. Shock Vib. 2020, 2020, 12. [Google Scholar] [CrossRef]
- Zhu, S.S.; Tang, L.B.; Liu, J.G.; Tang, X.Z.; Liu, X.W. A Novel Design of Magnetorheological Damper with Annular Radial Channel. Shock Vib. 2016, 2016, 1–7. [Google Scholar] [CrossRef]
- Hu, G.; Wu, L.; Deng, Y.; Yu, L.; Luo, B. Damping Performance Analysis of Magnetorheological Damper Based on Multiphysics Coupling. Actuators 2021, 10, 176. [Google Scholar] [CrossRef]
- Liu, L.; Xu, Y.; Zhou, F.; Hu, G.; Yu, L. Performance Analysis of Magnetorheological Damper with Folded Resistance Gaps and Bending Magnetic Circuit. Actuators 2022, 11, 165. [Google Scholar] [CrossRef]
- Bai, X.-X.; Wang, D.-H.; Fu, H. Principle, Modeling, and Testing of an Annular-Radial-Duct Magnetorheological Damper. Sens. Actuators A 2013, 201, 302–309. [Google Scholar] [CrossRef]
- Deng, Z. Optimal Design and Control Strategy Research of Automotive Powertrain Magneto-Rheological Mount. Ph.D. Dissertation, Chongqing University, Chongqing, China, 2015. [Google Scholar]
- Boukerroum, S.; Hamzaoui, N. Dynamic Characterization of MR Damper and Experimental Adjustment of Numerical Model. In Applied Mechanics, Behavior of Materials, and Engineering Systems. Lecture Notes in Mechanical Engineering; Boukharouba, T., Pluvinage, G., Azouaoui, K., Eds.; Springer: Cham, Switzerland, 2017; pp. 331–343. [Google Scholar]
- Deb, K.; Pratap, A.; Agarwal, S.; Meyarivan, T. A Fast and Elitist Multiobjective Genetic Algorithm: NSGA-II. IEEE Trans. Evol. Comput. 2002, 6, 182–197. [Google Scholar] [CrossRef]
- Nebro, A.J.; Galeano-Brajones, J.; Luna, F.; Coello Coello, C.A. Is NSGA-II Ready for Large-Scale Multi-Objective Optimization? Math. Comput. Appl. 2022, 27, 103. [Google Scholar] [CrossRef]

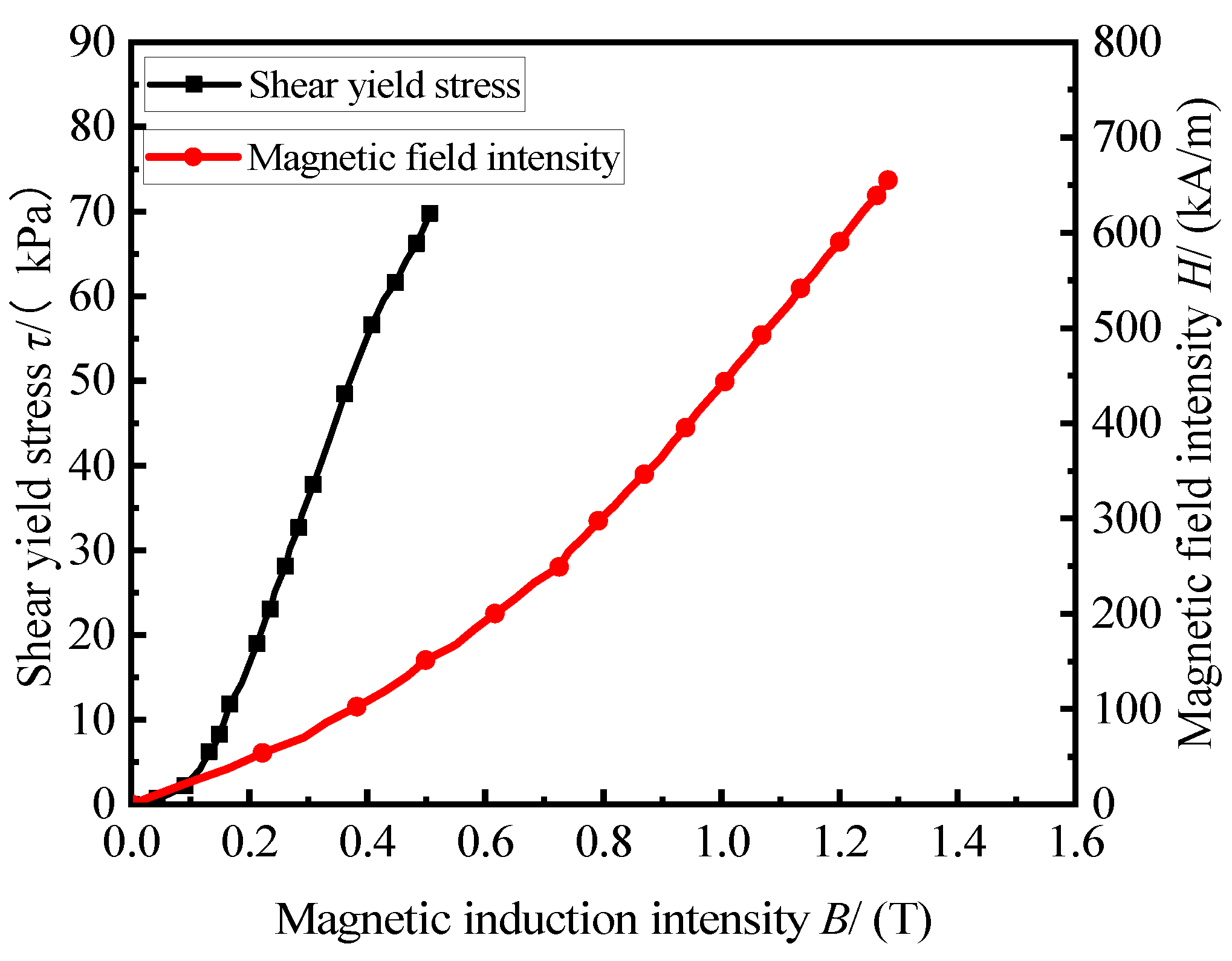
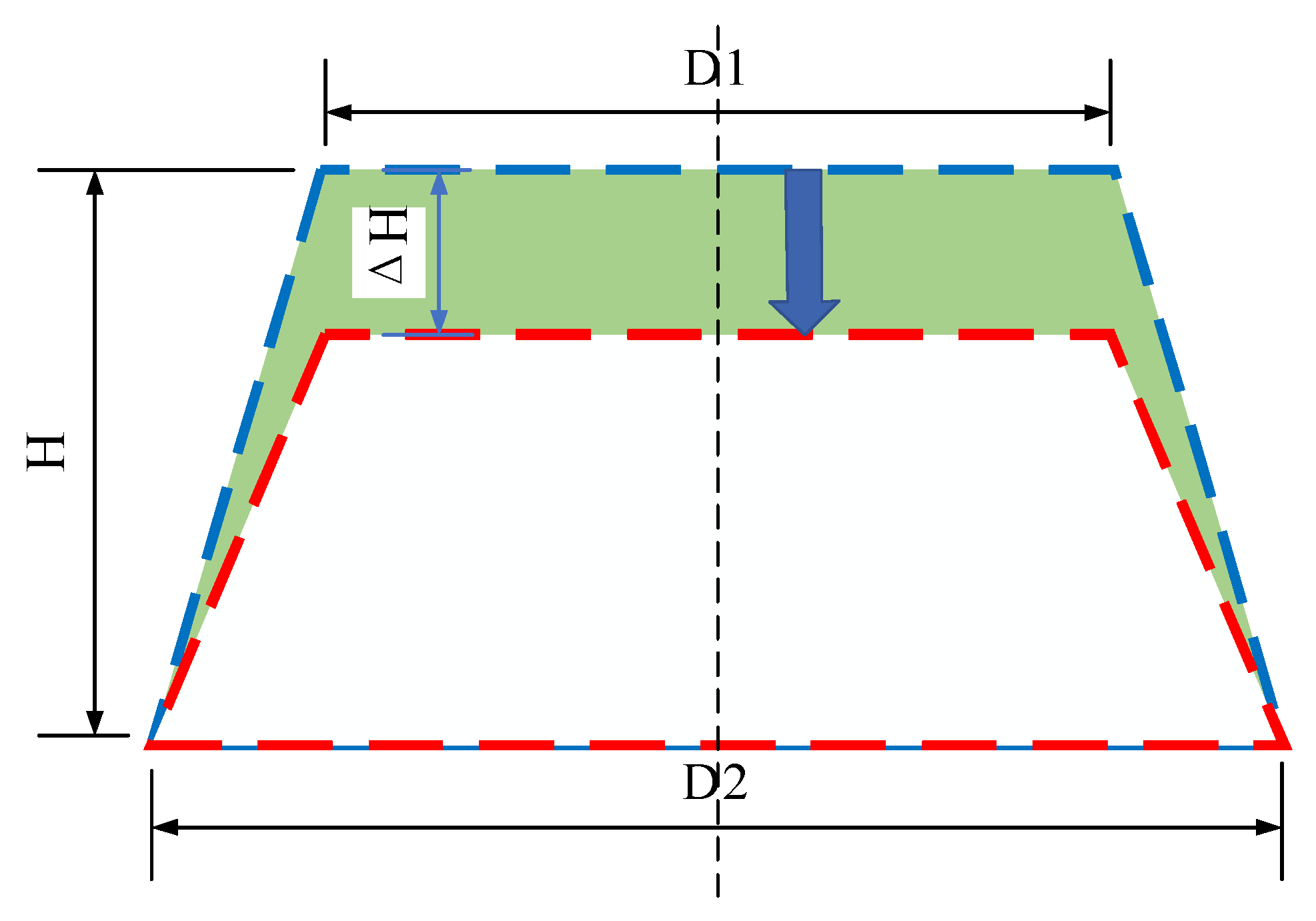
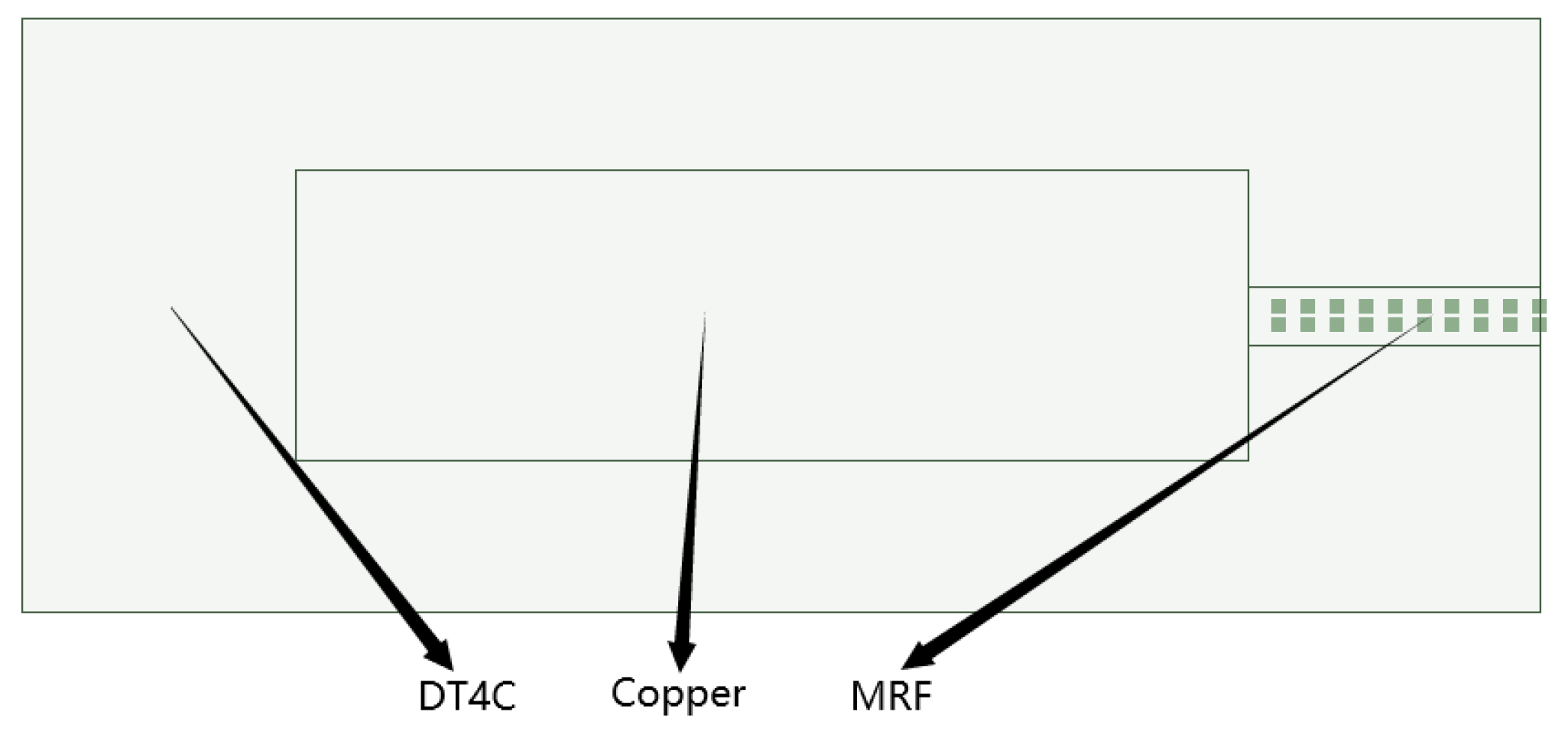
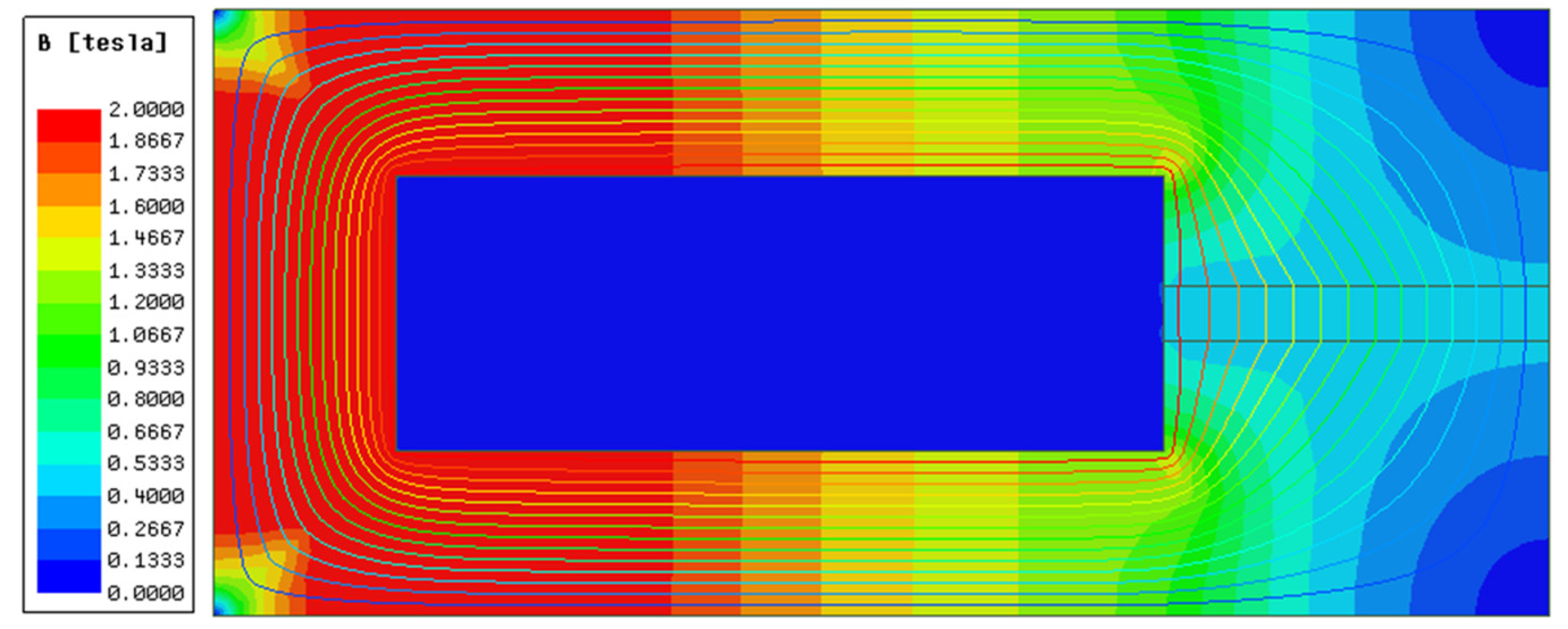

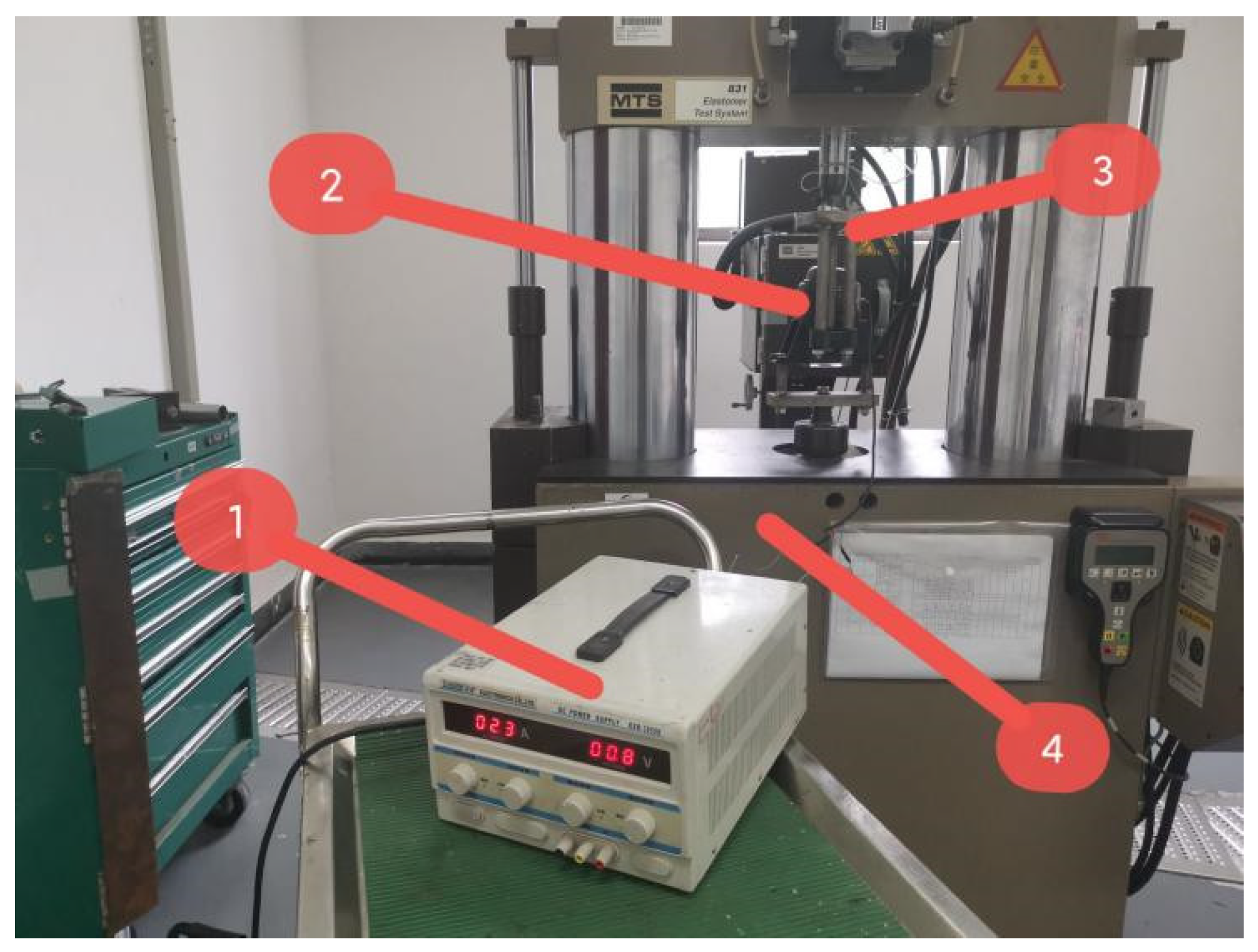




| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Fe (%vol) | 25 | Operating temperature (°C) | 40130 |
| Carrier fluid | Hydrocarbon oils | Zero magnetic field viscosity at room temperature (Pa∙s, γ = 51/s) | ≤1.0 |
| Density (room temperature) (g/cm) | 2.65 | Shear stress (kPa, B = 0.5T) | ≥40 |
| Variables | Lower Bound/(mm) | Upper Bound/(mm) |
|---|---|---|
| R1 | 5 | 12 |
| R2 | 1 | 5 |
| R3 | 10 | 18 |
| R4 | 5 | 12 |
| L1 | 1 | 5 |
| L2 | 1 | 3 |
| H | 1 | 5 |
| Optimize Variables | Lower Bound/(mm) | Upper Bound/(mm) |
|---|---|---|
| R1 | 5 | 12 |
| R2 | 1 | 5 |
| R3 | 10 | 18 |
| R4 | 5 | 12 |
| L1 | 1 | 5 |
| L2 | 2 | 2 |
| H | 1 | 5 |
| Number | H | L1 | R1 | R2 | R3 | R4 |
|---|---|---|---|---|---|---|
| 1 | 1.01 | 3.22 | 5.82 | 3.74 | 17.46 | 5.01 |
| 2 | 1.03 | 2.26 | 6.80 | 3.44 | 16.62 | 5.22 |
| 3 | 1.01 | 2.82 | 5.05 | 4.68 | 16.38 | 5.08 |
| 4 | 1.01 | 2.52 | 5.26 | 4.59 | 16.33 | 5.03 |
| 5 | 1.26 | 3.40 | 6.07 | 3.07 | 16.06 | 6.96 |
| 6 | 1.17 | 3.40 | 6.07 | 2.04 | 16.23 | 7.84 |
| 7 | 1.04 | 2.96 | 6.09 | 3.02 | 15.95 | 5.93 |
| 8 | 1.04 | 2.86 | 6.14 | 2.93 | 16.24 | 5.88 |
| 9 | 1.05 | 3.38 | 6.07 | 2.04 | 16.23 | 7.87 |
| 10 | 1.04 | 3.43 | 6.07 | 3.06 | 16.06 | 7.06 |
| 11 | 1.01 | 2.53 | 7.93 | 1.33 | 16.08 | 5.93 |
| 12 | 1.04 | 2.96 | 6.16 | 3.02 | 16.88 | 5.52 |
| 13 | 1.04 | 2.31 | 6.80 | 3.44 | 16.61 | 5.26 |
| 14 | 1.03 | 2.26 | 6.80 | 3.44 | 16.62 | 5.22 |
| 15 | 1.01 | 2.64 | 6.00 | 4.69 | 16.38 | 5.03 |
| R1 | R2 | R3 | R4 | L1 | H | |
|---|---|---|---|---|---|---|
| Before optimization | 7 | 3.3 | 14 | 7 | 3 | 1 |
| After optimization | 6.0 | 4.7 | 16.4 | 5.0 | 2.6 | 1.0 |
| Output Damping Force (N) | Controllable Force (N) | Zero Magnetic Field Damping Force (N) | Adjustable Coefficients | |
|---|---|---|---|---|
| Before optimization | 232.44 | 177.02 | 55.42 | 3.19 |
| After optimization | 336.19 | 254.37 | 81.82 | 3.11 |
| Rate of change (%) | 44.64 | 43.70 | 47.64 | −2.50 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fu, J.; Huang, C.; Shu, R.; Li, X.-Q.; Chen, M.; Chen, Z.; Chen, B. Multi-Objective Optimization of Magnetorheological Mount Considering Optimal Damping Force and Maximum Adjustable Coefficient. Machines 2023, 11, 60. https://doi.org/10.3390/machines11010060
Fu J, Huang C, Shu R, Li X-Q, Chen M, Chen Z, Chen B. Multi-Objective Optimization of Magnetorheological Mount Considering Optimal Damping Force and Maximum Adjustable Coefficient. Machines. 2023; 11(1):60. https://doi.org/10.3390/machines11010060
Chicago/Turabian StyleFu, Jianghua, Chao Huang, Ruizhi Shu, Xing-Quan Li, Ming Chen, Zheming Chen, and Bao Chen. 2023. "Multi-Objective Optimization of Magnetorheological Mount Considering Optimal Damping Force and Maximum Adjustable Coefficient" Machines 11, no. 1: 60. https://doi.org/10.3390/machines11010060
APA StyleFu, J., Huang, C., Shu, R., Li, X.-Q., Chen, M., Chen, Z., & Chen, B. (2023). Multi-Objective Optimization of Magnetorheological Mount Considering Optimal Damping Force and Maximum Adjustable Coefficient. Machines, 11(1), 60. https://doi.org/10.3390/machines11010060






