Vibro-Impact Response Analysis of Collision with Clearance: A Tutorial
Abstract
1. Introduction
- Step 1:
- Establish the dynamic equation of the nonlinear structure with local clearance.
- Step 2:
- Select the description method of clearance and the model of nonlinear impact force.
- Step 3:
- Select the solving method of the nonlinear dynamic equations.
2. Vibro-Impact Response Analysis
2.1. Nonlinear Dynamics Equations of Structures with Clearances
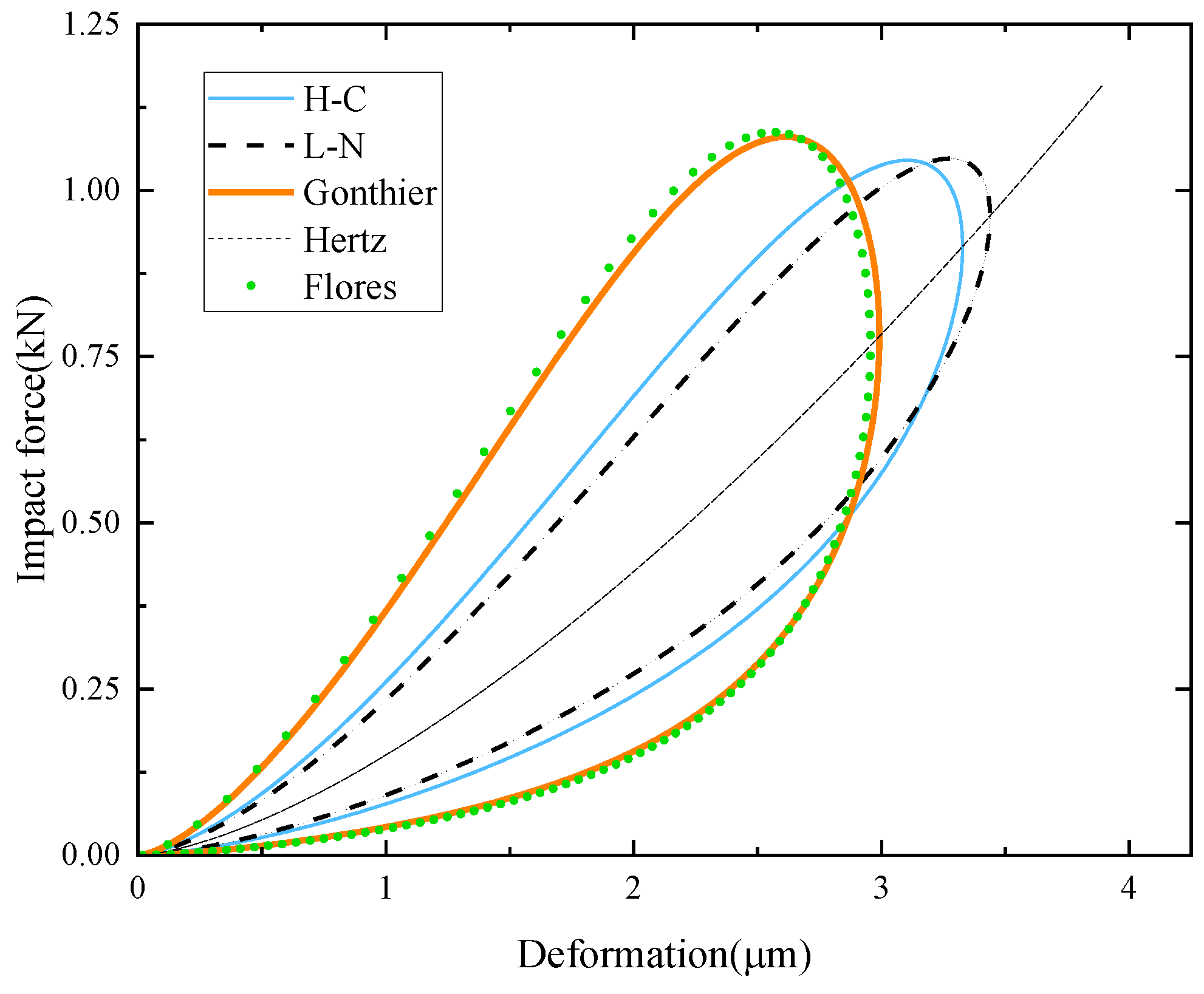
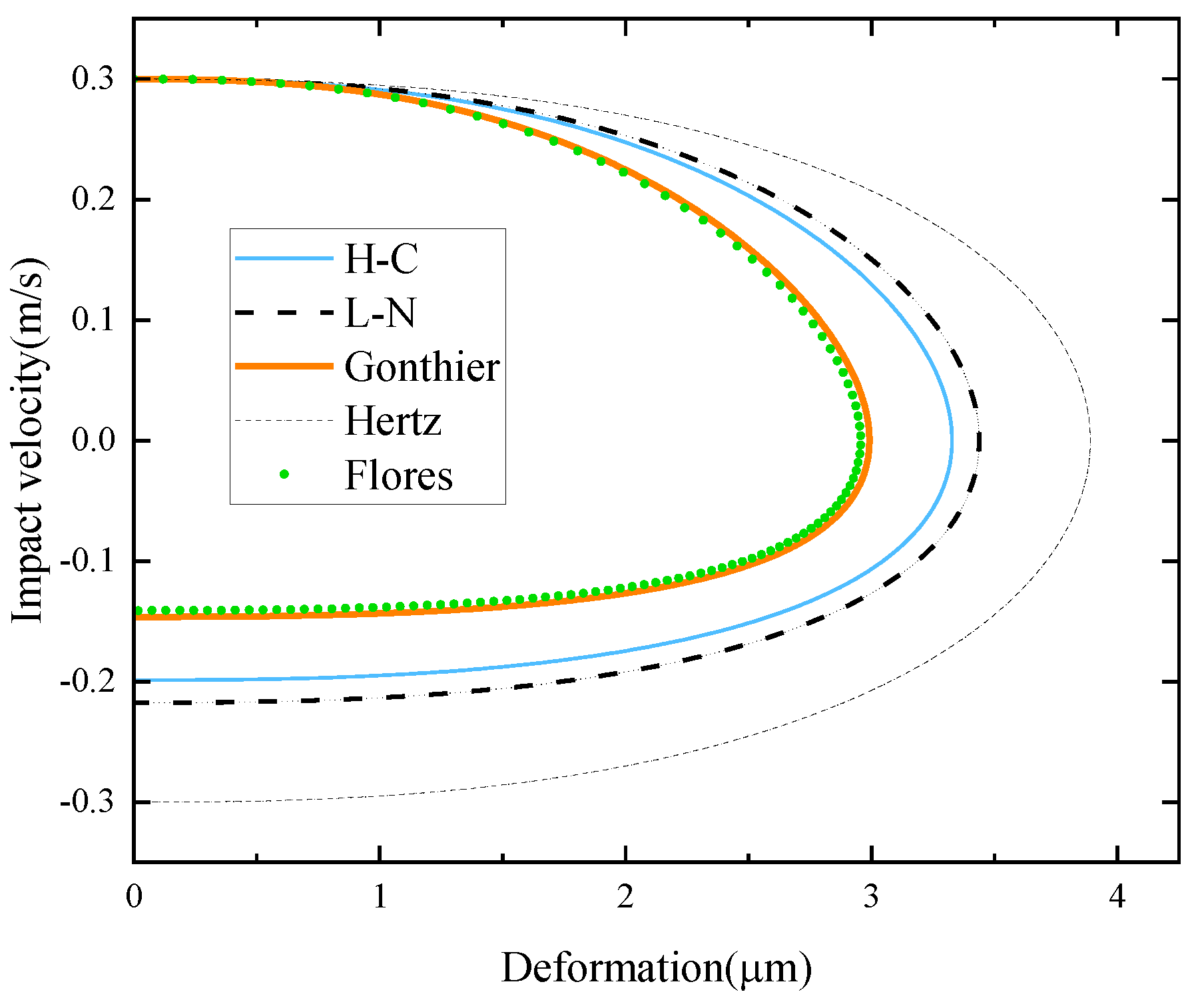
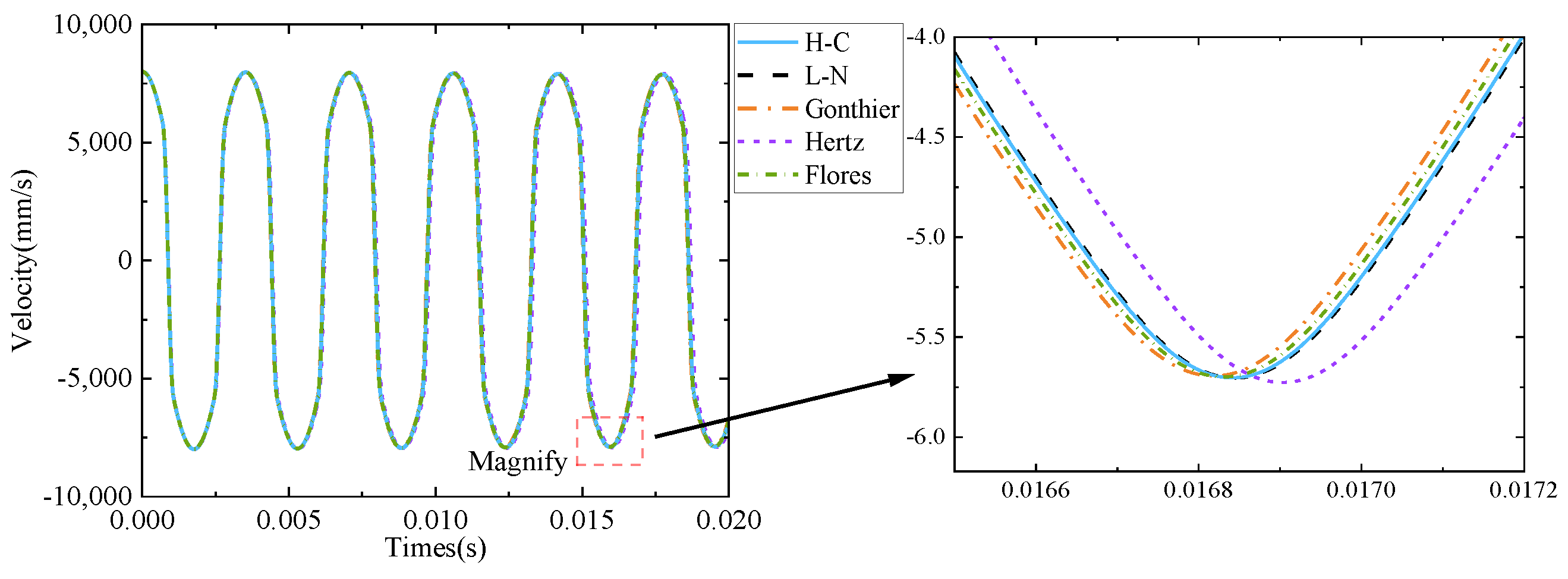
2.2. Model of Nonlinear Impact Force
- (1)
- Hertz contact force model
- (2)
- Hunt–Crossley (H-C) contact force model
- (3)
- Lankarani–Nikravesh (L–N) contact force model [35]
- (4)
- Gonthier contact force model
- (5)
- Flores contact force model [38]
2.3. Solving Method of Nonlinear Dynamic Equations
- (1)
- Newmark-β method combined with Newton-Raphson method [41]
- (2)
- Generalized α method
- (3)
- Precise Adams Multi-step method (precise integration method)
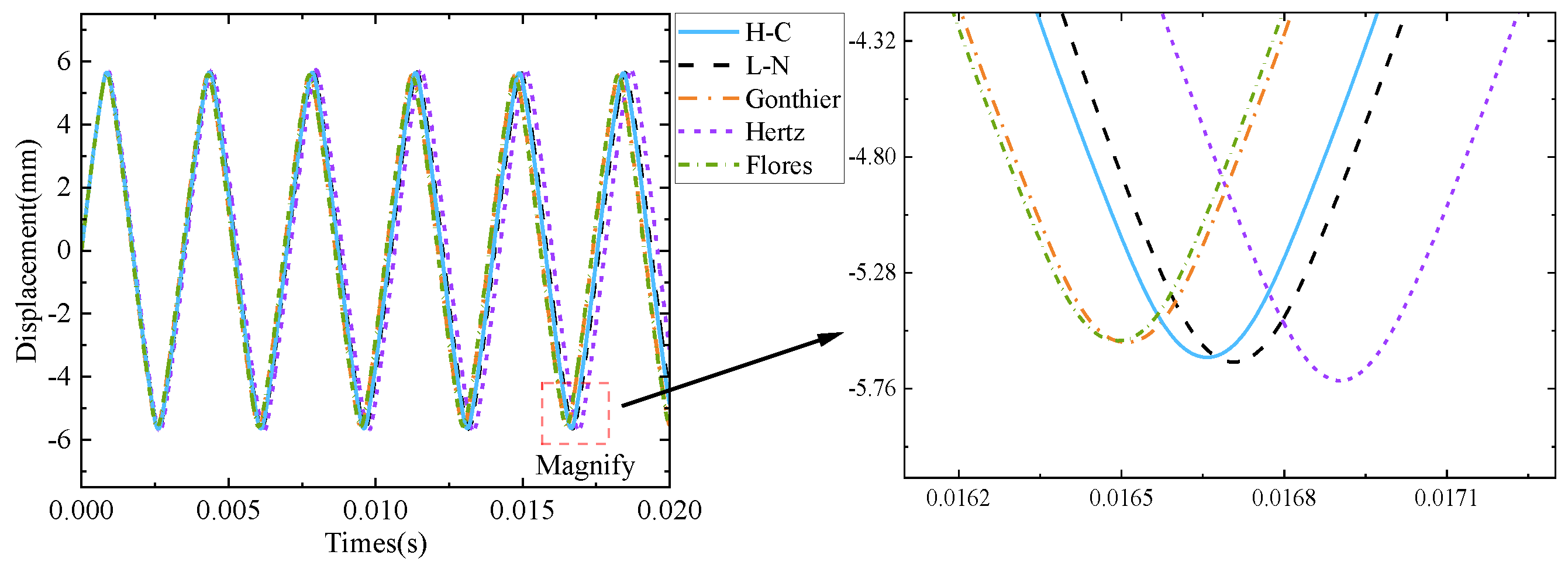
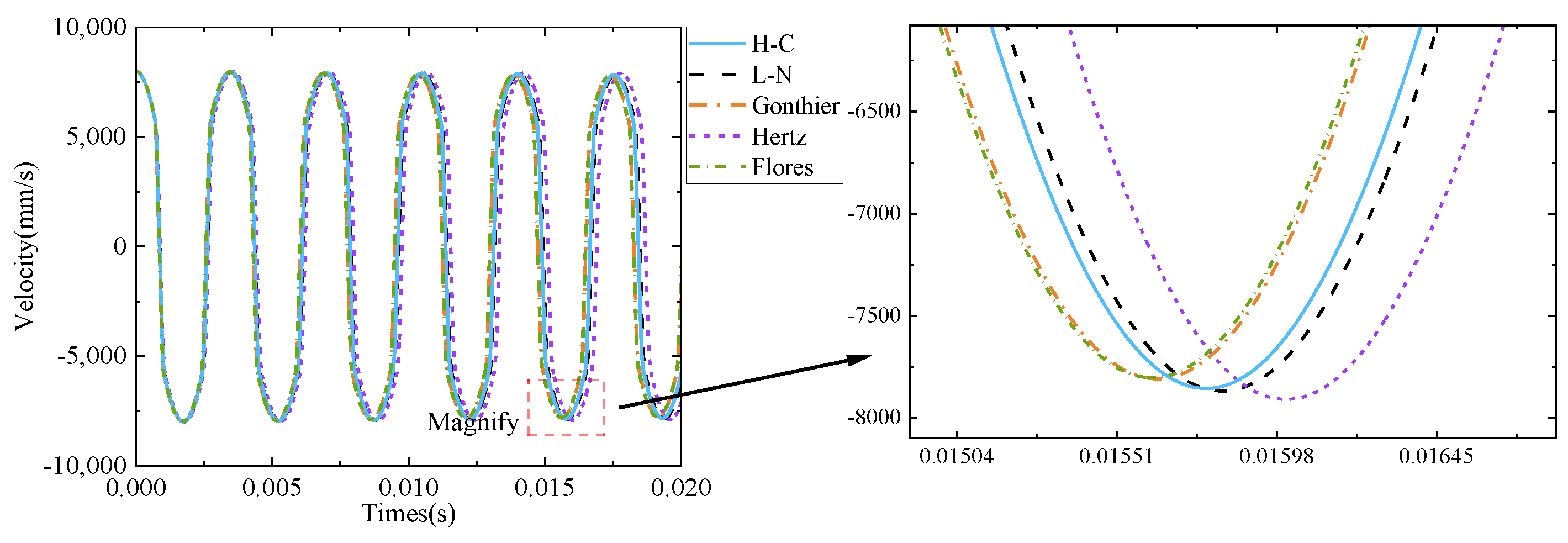
3. Nonlinear Dynamic Response Analysis of Structures with Clearance
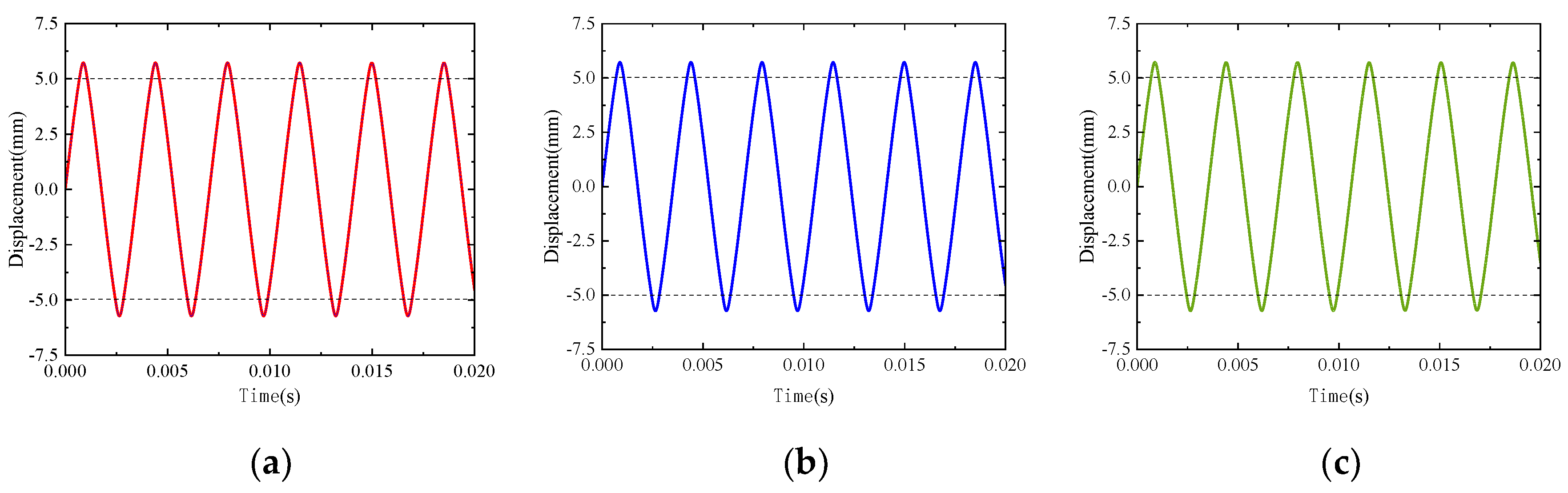
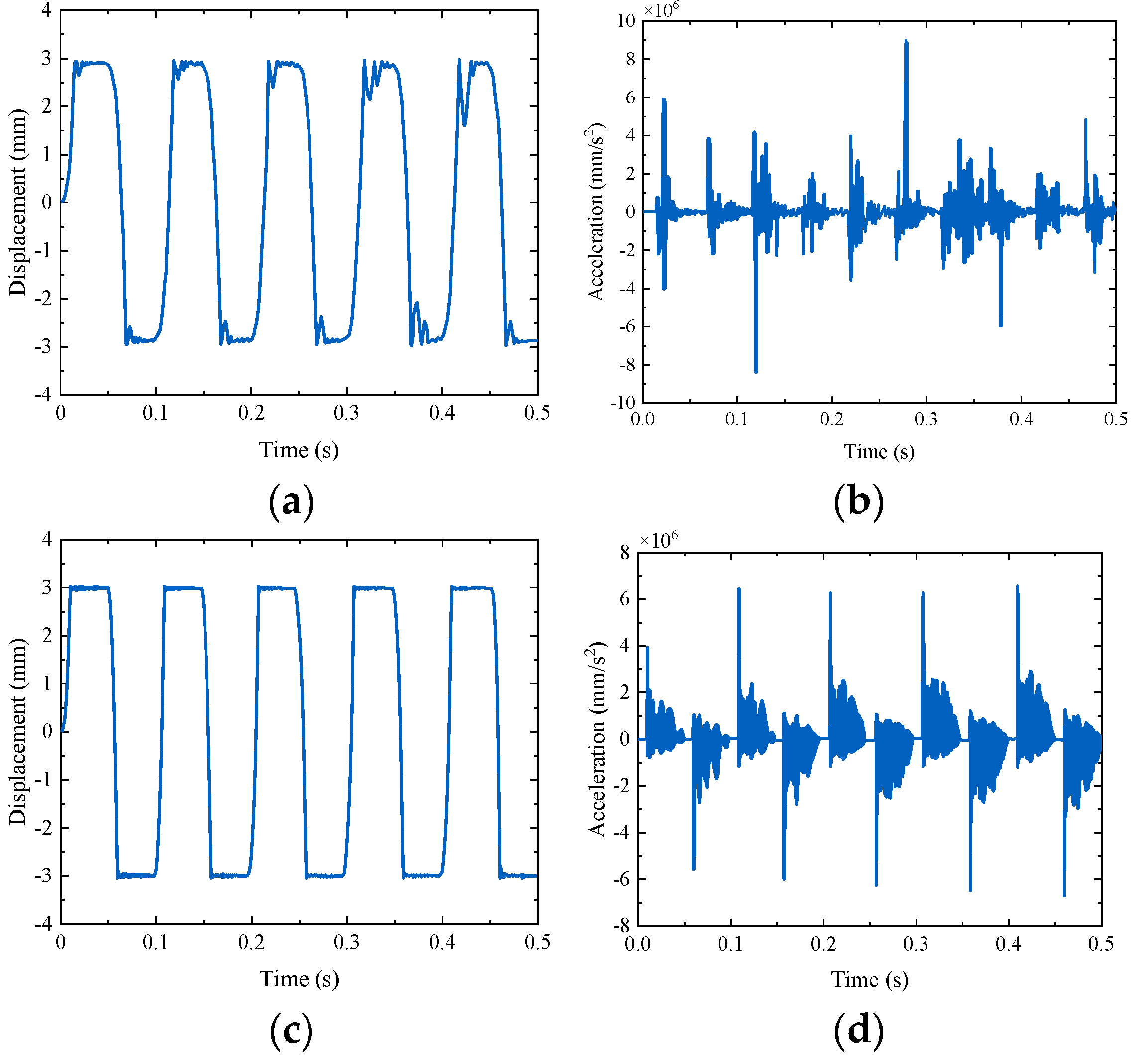
3.1. Single Degree of Freedom Model
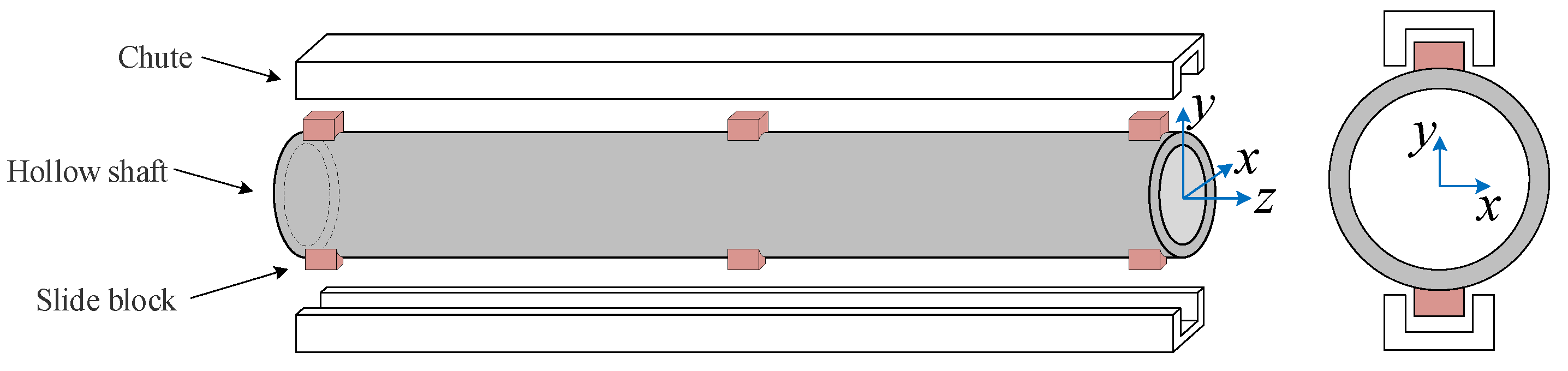
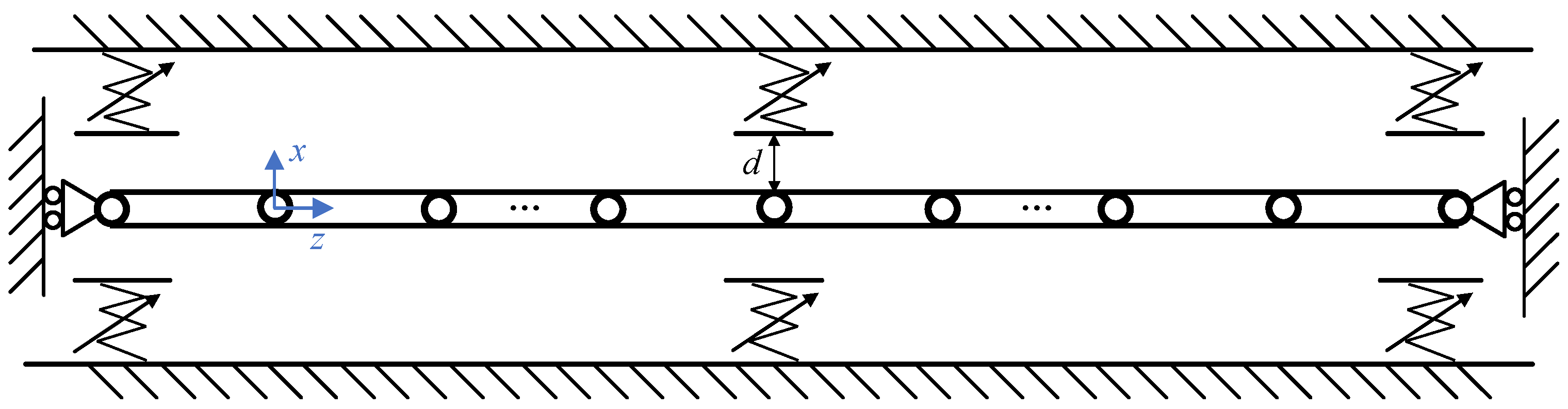
3.2. Multiple Degrees of Freedom Model
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Banerjee, A.; Chanda, A.; Das, R. Historical Origin and Recent Development on Normal Directional Impact Models for Rigid Body Contact Simulation: A Critical Review. Arch. Comput. Methods Eng. 2017, 24, 397–422. [Google Scholar] [CrossRef]
- Li, X.; Yao, Z.; Wu, R. Modeling and analysis of stick-slip motion in a linear piezoelectric ultrasonic motor considering ultrasonic oscillation effect. Int. J. Mech. Sci. 2016, 107, 215–224. [Google Scholar] [CrossRef]
- Paez Chavez, J.; Pavlovskaia, E.; Wiercigroch, M. Bifurcation analysis of a piecewise-linear impact oscillator with drift. Nonlinear Dyn. 2014, 77, 213–227. [Google Scholar] [CrossRef]
- Yaqubi, S.; Dardel, M.; Daniali, H.M.; Ghasemi, M.H. Modeling and control of crank-slider mechanism with multiple clearance joints. Multibody Syst. Dyn. 2016, 36, 143–167. [Google Scholar] [CrossRef]
- Machado, M.; Moreira, P.; Flores, P.; Lankarani, H.M. Compliant contact force models in multibody dynamics: Evolution of the Hertz contact theory. Mech. Mach. Theory 2012, 53, 99–121. [Google Scholar] [CrossRef]
- Wen, G.; Yin, S.; Xu, H.; Zhang, S.; Lv, Z. Analysis of grazing bifurcation from periodic motion to quasi-periodic motion in impact-damper systems. Chaos Solitons Fractals 2016, 83, 112–118. [Google Scholar] [CrossRef]
- Jiang, H.; Chong, A.S.E.; Ueda, Y.; Wiercigroch, M. Grazing-induced bifurcations bifurcations in impact oscillators with elastic and rigid constraints. Int. J. Mech. Sci. 2017, 127, 204–214. [Google Scholar] [CrossRef]
- Nordmark, A.B.; Piiroinen, P.T. Simulation and stability analysis of impacting systems with complete chattering. Nonlinear Dyn. 2009, 58, 85–106. [Google Scholar] [CrossRef]
- Wagg, D.J. Rising phenomena and the multi-sliding bifurcation in a two-degree of freedom impact oscillator. Chaos Solitons Fractals 2004, 22, 541–548. [Google Scholar] [CrossRef]
- Sharkawy, A.-N.; Mostfa, A.A. Neural networks’ design and training for safe human-robot cooperation. J. King Saud Univ. -Eng. Sci. 2021, 1–15. [Google Scholar]
- Wu, B.; Wu, Y.; Liu, M.; Liu, J.; Jiang, D.; Ma, S.; Yan, B.; Lu, Y. Mechanical Behavior of Human Cancellous Bone in Alveolar Bone under Uniaxial Compression and Creep Tests. Materials 2022, 15, 5912. [Google Scholar] [CrossRef] [PubMed]
- Askari, E. Mathematical models for characterizing non-Hertzian contacts. Appl. Math. Model. 2021, 90, 432–447. [Google Scholar] [CrossRef]
- Li, B.; Wang, M.S.; Gantes, C.J.; Tan, U.X. Modeling and simulation for wear prediction in planar mechanical systems with multiple clearance joints. Nonlinear Dyn. 2022, 108, 887–910. [Google Scholar] [CrossRef]
- Venanzi, S.; Parenti-Castelli, V. A new technique for clearance influence analysis in spatial mechanisms. J. Mech. Des. 2005, 127, 446–455. [Google Scholar] [CrossRef]
- Flores, P.; Machado, M.; Silva, M.T.; Martins, J.M. On the continuous contact force models for soft materials in multibody dynamics. Multibody Syst. Dyn. 2011, 25, 357–375. [Google Scholar] [CrossRef]
- Wang, G.X.; Liu, H.Z.; Deng, P.S. Dynamics Analysis of Spatial Multibody System With Spherical Joint Wear. J. Tribol. Trans. ASME 2015, 137, 021605. [Google Scholar] [CrossRef]
- Tian, Q.; Flores, P.; Lankarani, H.M. A comprehensive survey of the analytical, numerical and experimental methodologies for dynamics of multibody mechanical systems with clearance or imperfect joints. Mech. Mach. Theory 2018, 122, 1–57. [Google Scholar] [CrossRef]
- Koshy, C.S.; Flores, P.; Lankarani, H.M. Study of the effect of contact force model on the dynamic response of mechanical systems with dry clearance joints: Computational and experimental approaches. Nonlinear Dyn. 2013, 73, 325–338. [Google Scholar] [CrossRef]
- Zhang, X.; Zhang, X.; Chen, Z. Dynamic analysis of a 3-(R) under bar RR parallel mechanism with multiple clearance joints. Mech. Mach. Theory 2014, 78, 105–115. [Google Scholar] [CrossRef]
- Marques, F.; Isaac, F.; Dourado, N.; Flores, P. An enhanced formulation to model spatial revolute joints with radial and axial clearances. Mech. Mach. Theory 2017, 116, 123–144. [Google Scholar] [CrossRef]
- Skrinjar, L.; Slavic, J.; Boltezar, M. A review of continuous contact-force models in multibody dynamics. Int. J. Mech. Sci. 2018, 145, 171–187. [Google Scholar] [CrossRef]
- Ma, J.; Chen, G.; Ji, L.; Qian, L.; Dong, S. A general methodology to establish the contact force model for complex contacting surfaces. Mech. Syst. Signal Process. 2020, 140, 106678. [Google Scholar] [CrossRef]
- Zhou, J.; Xu, L.; Zhao, J.; Hang, X.; Zhou, H. Effective excitation conditions for the intense motion of the ginkgo seed-stem system during mechanical vibration harvesting. Biosyst. Eng. 2022, 215, 239–248. [Google Scholar] [CrossRef]
- Erkaya, S.; Uzmay, I. Experimental investigation of joint clearance effects on the dynamics of a slider-crank mechanism. Multibody Syst. Dyn. 2010, 24, 81–102. [Google Scholar] [CrossRef]
- Erkaya, S.; Uzmay, I. Investigation on effect of joint clearance on dynamics of four-bar mechanism. Nonlinear Dyn. 2009, 58, 179–198. [Google Scholar] [CrossRef]
- Flores, P. A parametric study on the dynamic response of planar multibody systems with multiple clearance joints. Nonlinear Dyn. 2010, 61, 633–653. [Google Scholar] [CrossRef]
- Alves, J.; Peixinho, N.; da Silva, M.T.; Flores, P.; Lankarani, H.M. A comparative study of the viscoelastic constitutive models for frictionless contact interfaces in solids. Mech. Mach. Theory 2015, 85, 172–188. [Google Scholar] [CrossRef]
- Liu, J.; Meng, X.; Jiang, C.; Han, X.; Zhang, D. Time-domain Galerkin method for dynamic load identification. Int. J. Numer. Methods Eng. 2016, 105, 620–640. [Google Scholar] [CrossRef]
- Fan, Y.; Zhao, C.; Yu, H.; Wen, B. Dynamic load identification algorithm based on Newmark-beta and self-filtering. Proc. Inst. Mech. Eng. Part C-J. Mech. Eng. Sci. 2020, 234, 96–107. [Google Scholar]
- Simsek, M. Non-linear vibration analysis of a functionally graded Timoshenko beam under action of a moving harmonic load. Compos. Struct. 2010, 92, 2532–2546. [Google Scholar] [CrossRef]
- Sun, G.; Ma, X.; Bai, Z. A Low Dispersion Precise Integration Time Domain Method Based on Wavelet Galerkin Scheme. IEEE Microw. Wirel. Compon. Lett. 2010, 20, 651–653. [Google Scholar] [CrossRef]
- Flores, P.; Ambrosio, J.; Claro, J.C.P.; Lankarani, H.M.; Koshy, C.S. A study on dynamics of mechanical systems including joints with clearance and lubrication. Mech. Mach. Theory 2006, 41, 247–261. [Google Scholar] [CrossRef]
- Guo, Y.; Mi, H.; Habibi, M. Electromechanical energy absorption, resonance frequency, and low-velocity impact analysis of the piezoelectric doubly curved system. Mech. Syst. Signal Process. 2021, 157, 107723. [Google Scholar] [CrossRef]
- Haddadi, A.; Hashtrudi-Zaad, K. Real-Time Identification of Hunt-Crossley Dynamic Models of Contact Environments. IEEE Trans. Robot. 2012, 28, 555–566. [Google Scholar] [CrossRef]
- Ma, J.; Qian, L. Modeling and simulation of planar multibody systems considering multiple revolute clearance joints. Nonlinear Dyn. 2017, 90, 1907–1940. [Google Scholar] [CrossRef]
- Chen, X.; Shuai, J.; Yu, D.; Wang, Q. Dynamics analysis of 2-DOF complex planar mechanical system with joint clearance and flexible links. Nonlinear Dyn. 2018, 93, 1009–1034. [Google Scholar] [CrossRef]
- Li, B.; Wang, S.-M.; Yuan, R.; Xue, X.-Z.; Zhi, C.-J. Dynamic characteristics of planar linear array deployable structure based on scissor-like element with joint clearance using a new mixed contact force model. Proc. Inst. Mech. Eng. Part C-J. Mech. Eng. Sci. 2016, 230, 3161–3174. [Google Scholar] [CrossRef]
- Hou, Y.-l.; Deng, Y.-j.; Zeng, D.-X. Dynamic modelling and properties analysis of 3RSR parallel mechanism considering spherical joint clearance and wear. J. Cent. South Univ. 2021, 28, 712–727. [Google Scholar] [CrossRef]
- Chen, D.; Yang, J.; Kitipornchai, S. Free and forced vibrations of shear deformable functionally graded porous beams. Int. J. Mech. Sci. 2016, 108, 14–22. [Google Scholar] [CrossRef]
- Arani, A.G.; Kiani, F.; Afshari, H. Free and forced vibration analysis of laminated functionally graded CNT-reinforced composite cylindrical panels. J. Sandw. Struct. Mater. 2021, 23, 255–278. [Google Scholar] [CrossRef]
- Cao, Z.; Fei, Q.; Jiang, D.; Zhu, R.; Jin, H. Sensitivity Analysis of Nonlinear Transient Response Based on Perturbation in the Complex Domain. J. Comput. Nonlinear Dyn. 2021, 16, 011001. [Google Scholar] [CrossRef]
- Erlicher, S.; Bonaventura, L.; Bursi, O.S. The analysis of the Generalized-alpha method for non-linear dynamic problems. Comput. Mech. 2002, 28, 83–104. [Google Scholar] [CrossRef]
- Zhang, S.Y.; Deng, Z.C. An improved precise integration method for nonlinear dynamic system. Mech. Res. Commun. 2003, 30, 33–38. [Google Scholar]








| Parameters | Definition |
|---|---|
| Fn | Nonlinear impact force |
| K | Coefficient of contact stiffness |
| δ | Elastic deformation |
| Derivative of elastic deformation | |
| Relative collision velocity |
| Model | Expression of Impact Force |
|---|---|
| Hertz contact force model | |
| Hunt–Crossley contact force model | |
| Lankarani–Nikravesh contact force model | |
| Gonthier contact force model | |
| Flores contact force model |
| Numerical Integration Algorithms | Time of Calculation/s |
|---|---|
| Newmark-β method combined with Newton–Raphson method | 1.3 |
| Generalized α method | 2.1 |
| Precise integration method | 25 |
| Model | Reference Model/Hz | Numerical Model/Hz | |
|---|---|---|---|
| Mode | |||
| First bending mode | 477 | 477 | |
| Second bending mode | 1035 | 1035 | |
| Third bending mode | 1614 | 1637 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, Y.; Tian, Y.; Li, Q.; Li, Y.; Zhang, D.; Jiang, D. Vibro-Impact Response Analysis of Collision with Clearance: A Tutorial. Machines 2022, 10, 814. https://doi.org/10.3390/machines10090814
Xu Y, Tian Y, Li Q, Li Y, Zhang D, Jiang D. Vibro-Impact Response Analysis of Collision with Clearance: A Tutorial. Machines. 2022; 10(9):814. https://doi.org/10.3390/machines10090814
Chicago/Turabian StyleXu, Yongjie, Yu Tian, Qiyu Li, Yanbin Li, Dahai Zhang, and Dong Jiang. 2022. "Vibro-Impact Response Analysis of Collision with Clearance: A Tutorial" Machines 10, no. 9: 814. https://doi.org/10.3390/machines10090814
APA StyleXu, Y., Tian, Y., Li, Q., Li, Y., Zhang, D., & Jiang, D. (2022). Vibro-Impact Response Analysis of Collision with Clearance: A Tutorial. Machines, 10(9), 814. https://doi.org/10.3390/machines10090814






