Recent Advances in Quasi-Zero Stiffness Vibration Isolation Systems: An Overview and Future Possibilities
Abstract
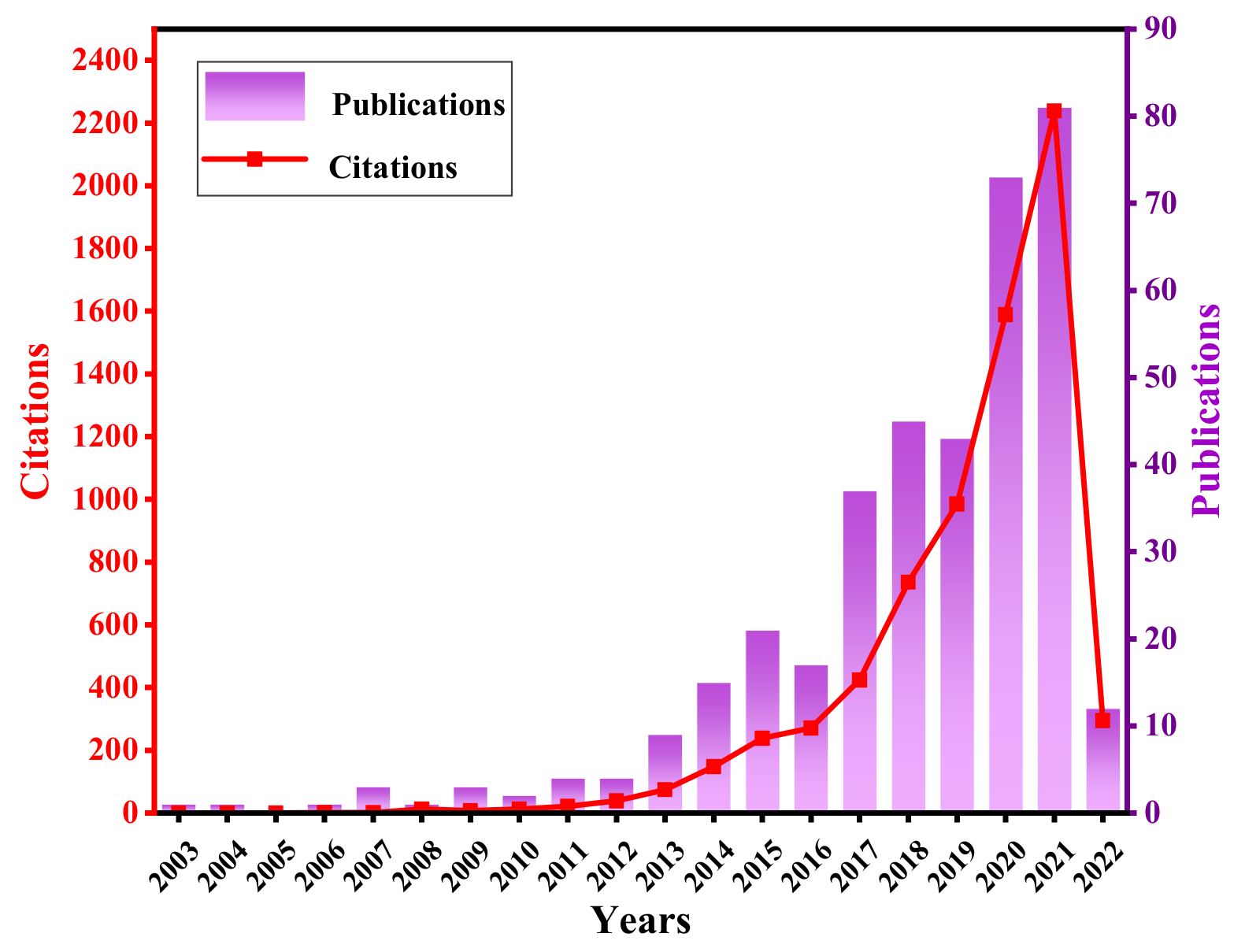
1. Introduction
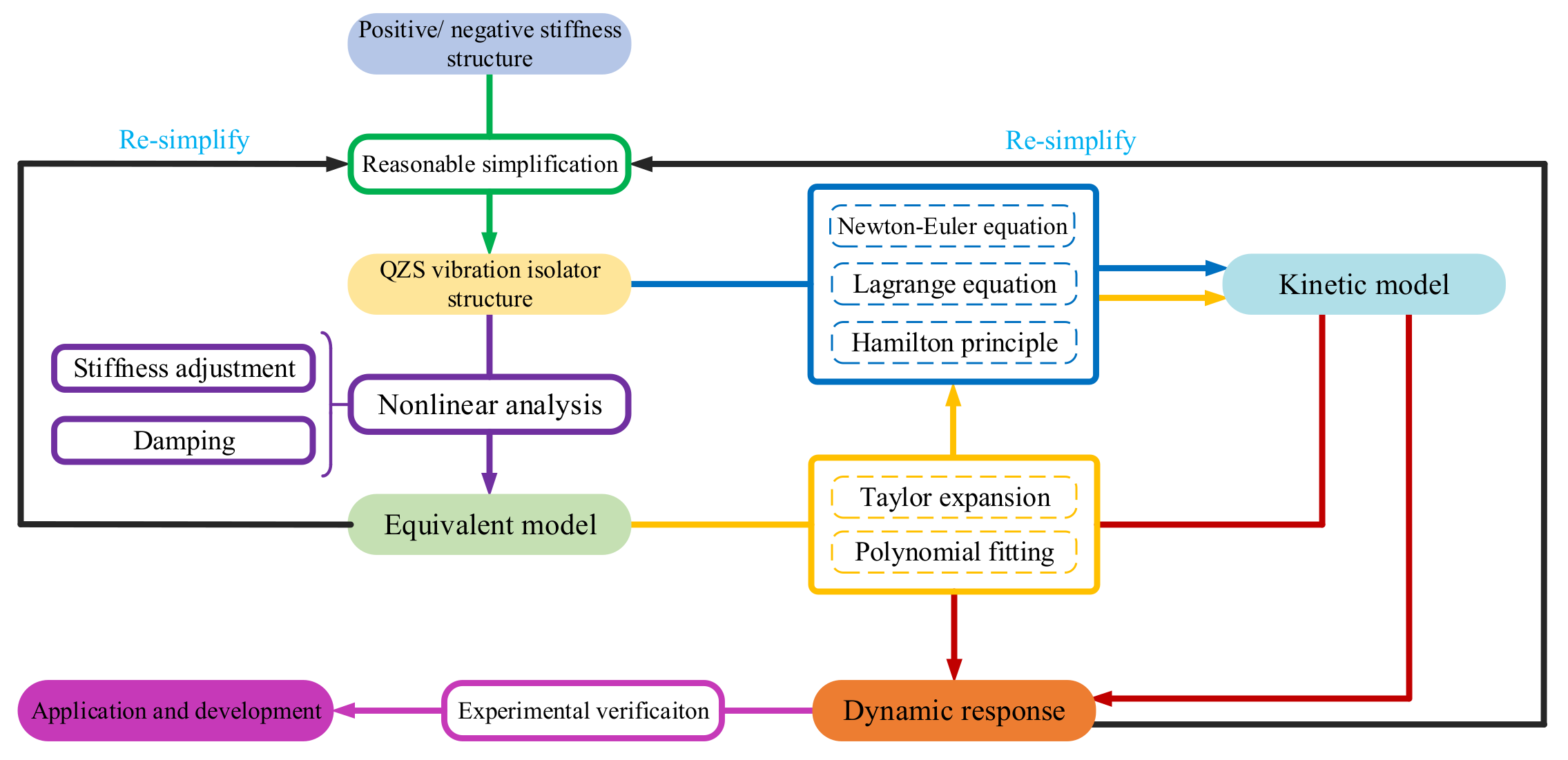
2. Basic Principle of QZS Vibration Isolation: An Overview
2.1. Concept of Quasi-Zero Stiffness
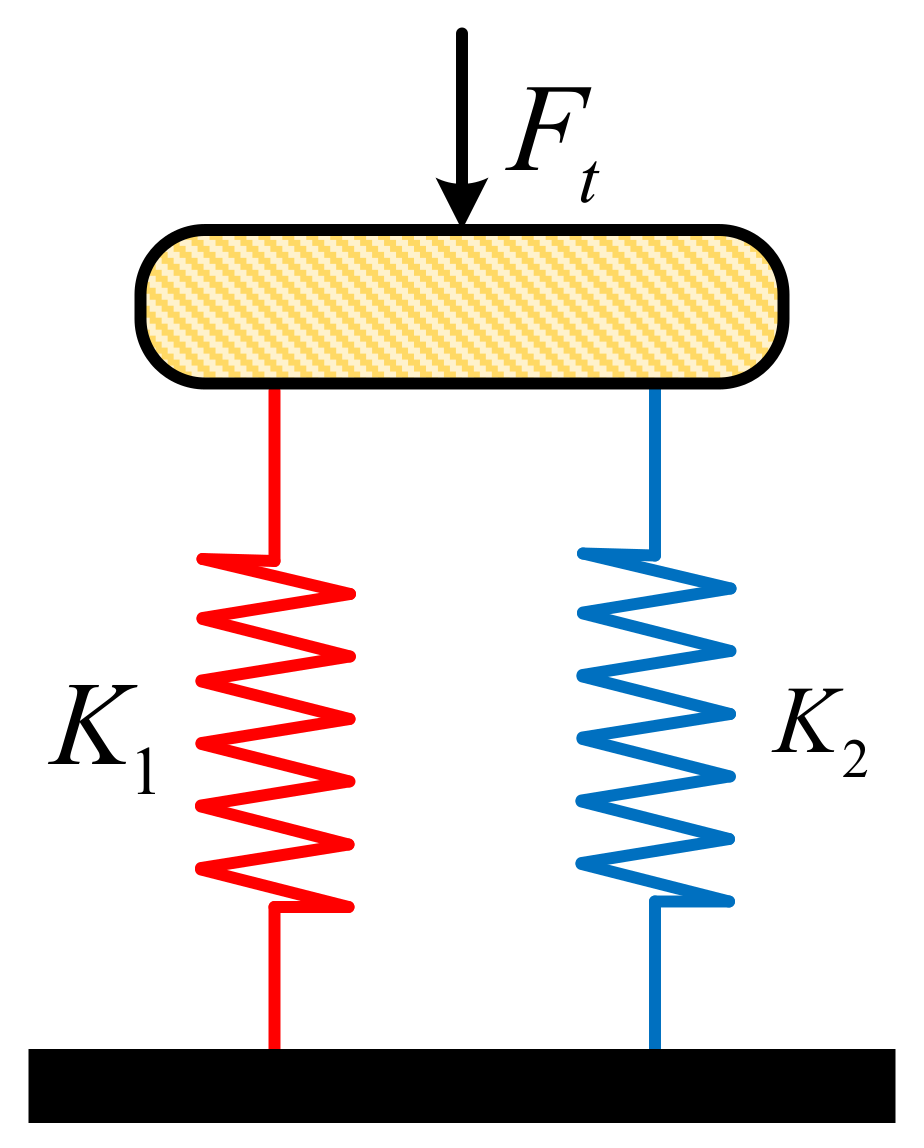
2.2. Principle of QZS Vibration Isolator
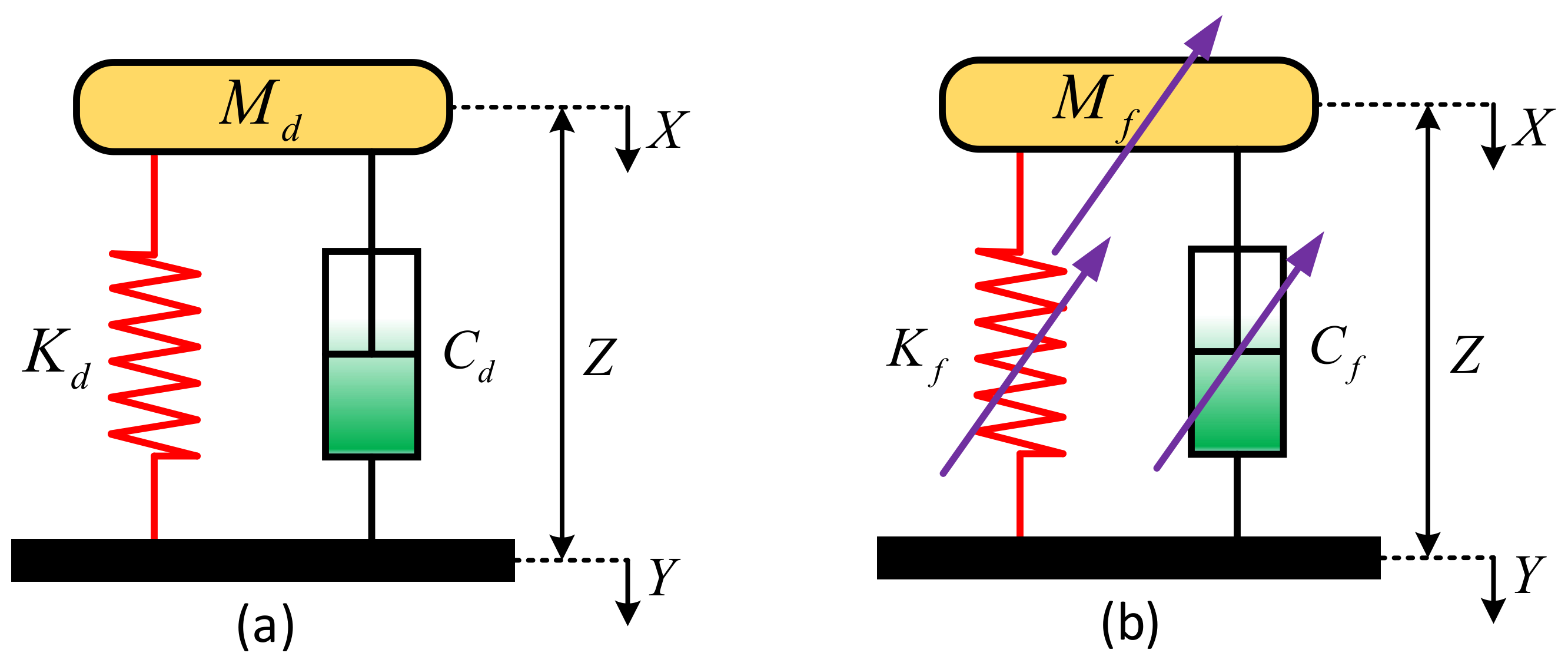
2.3. Vibration Isolation Characteristics of QZS Vibration Isolator
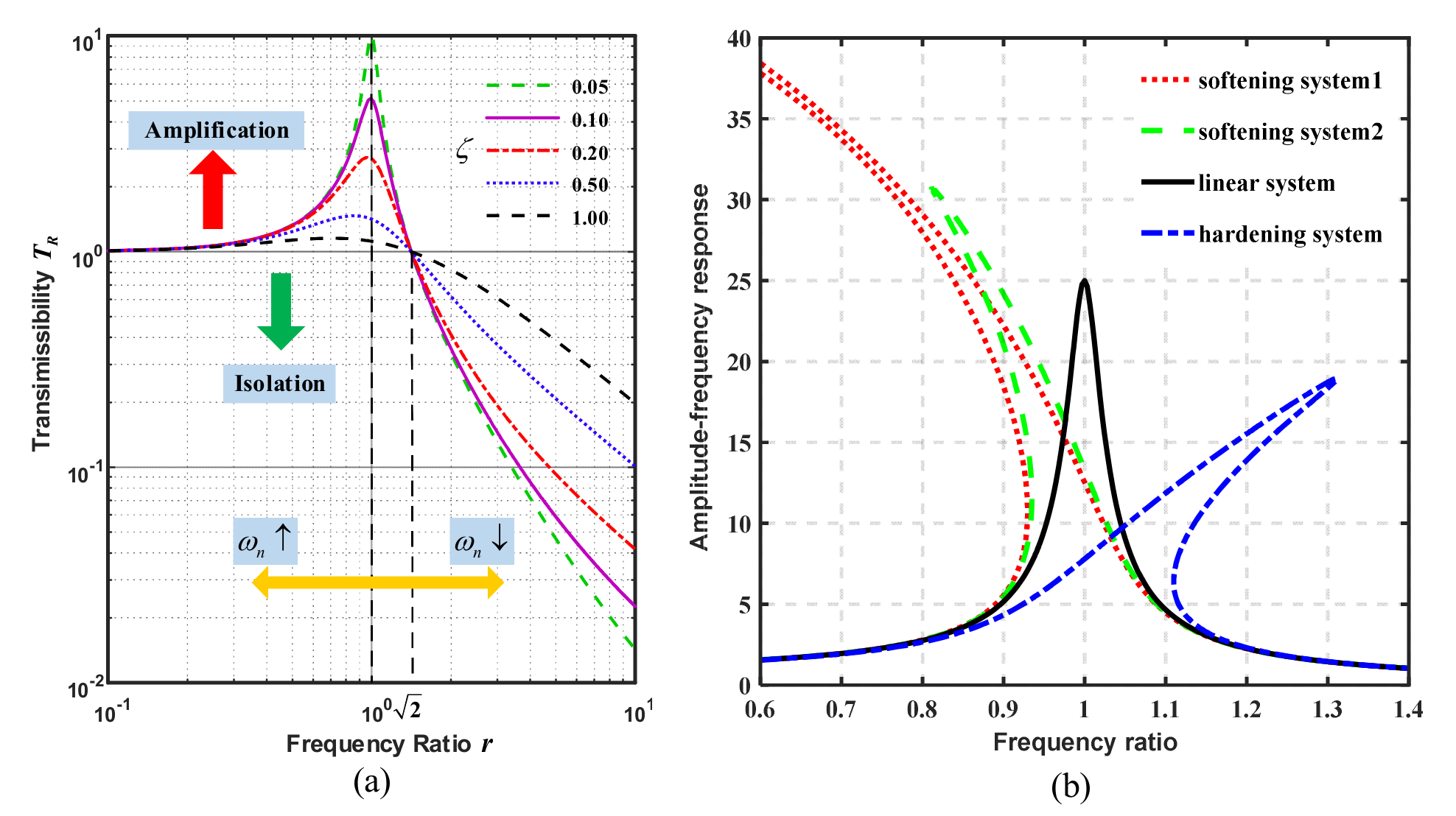
2.3.1. Vibration Transmissibility
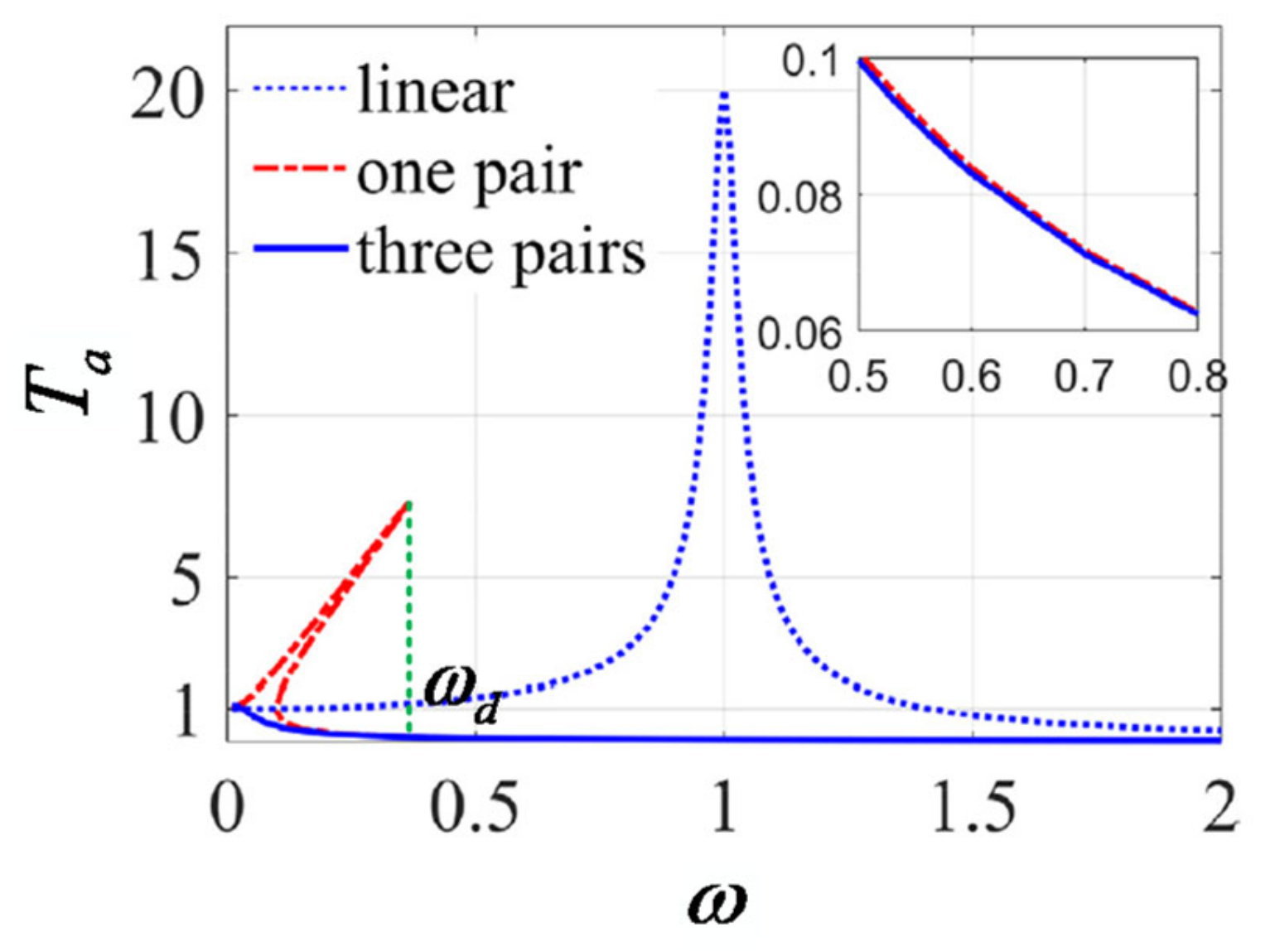
2.3.2. Amplitude-Frequency Response Curves and Frequency Jump
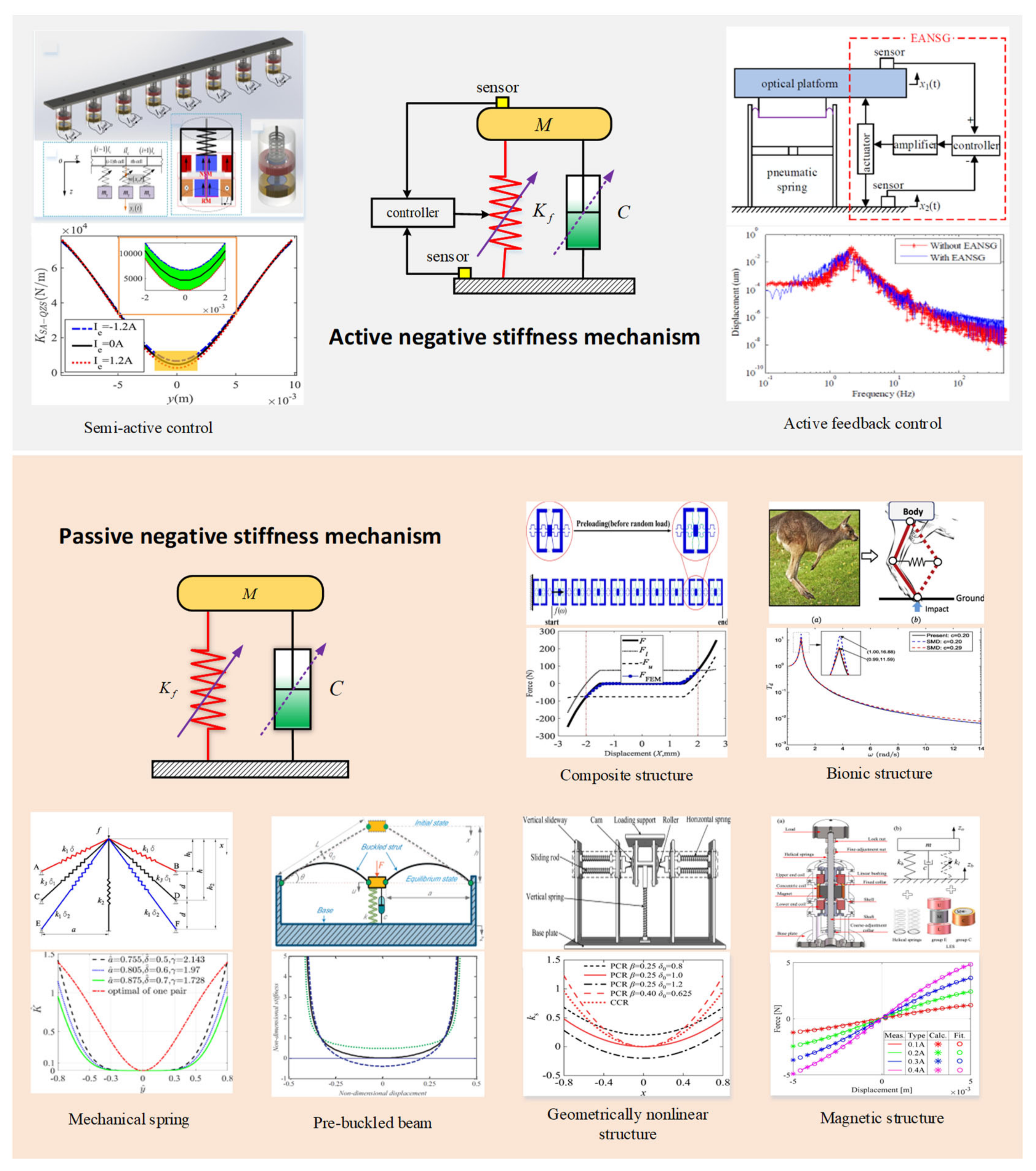
3. Design and Realization of QZS Vibration Isolators
- (1)
- Passive negative-stiffness mechanism: the design of this type of QZS vibration isolator focuses on the balanced matching of restoring force and zero stiffness. How positive- and negative-stiffness mechanisms maintain motion stability was deeply explored first, and then a QZS structure with variable stiffness was proposed. The desired QZS characteristics can be easily obtained by designing different optimal parameter combinations, and improvement design can be carried out according to the requirements for external loads and excitation range. In addition, QZS vibration isolators not only have a vibration isolation frequency bandwidth much larger than the traditional counterparts, but also have a lower transmissivity. Therefore, QZS isolators can be applied to low-frequency large-amplitude excitation or low-frequency small-amplitude excitation and perform better in vibration attenuation efficiency and attenuation range (isolating lower excitation frequencies).
- (2)
- Active negative-stiffness mechanism: multi-source vibrations are ubiquitous in mechanical equipment and building structures. It is often through the introduction of active control to ensure the adaptability and good vibration isolation performance of the vibration isolation system. After in-depth investigation of the actual QZS vibration isolation system, the influence of different physical parameters (excitation frequency, payload mass, or displacement) on the dynamic stiffness of the system can be obtained, and practical needs can be met by introducing appropriate control devices and methods.
4. Passive QZS Vibration Isolator
4.1. Mechanical Spring
4.2. Formatting of Mathematical Components
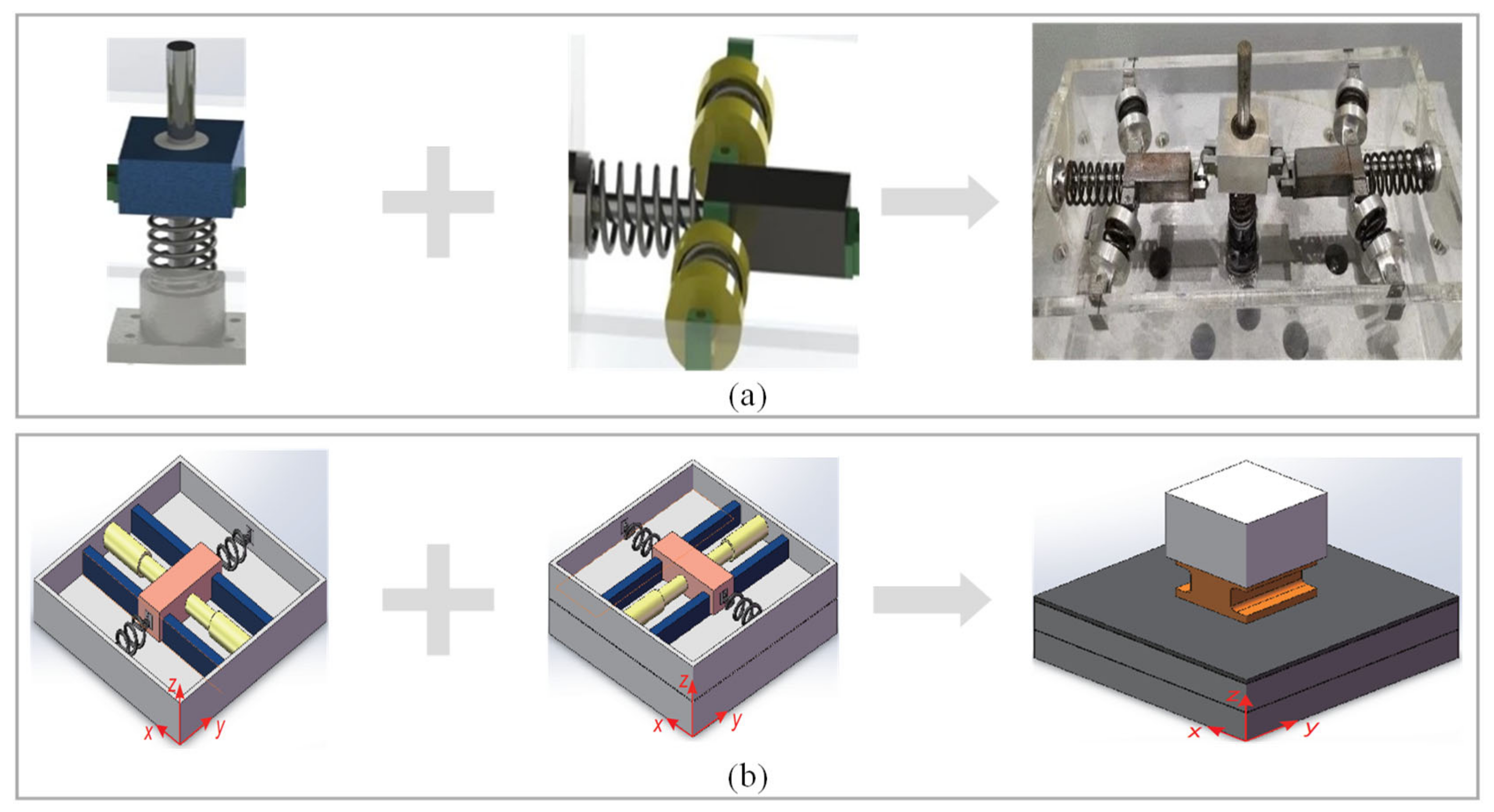
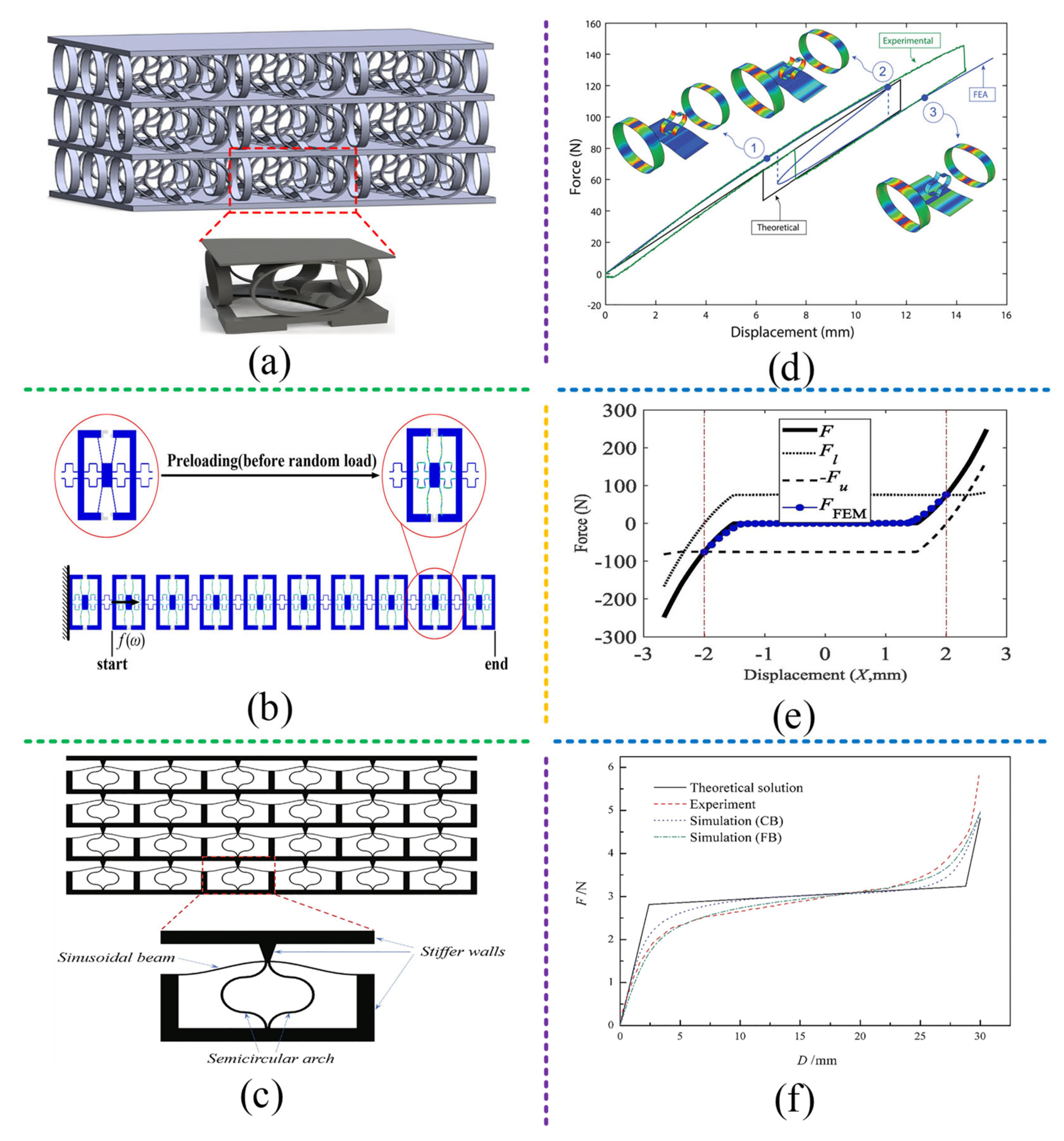
4.3. Geometrically Nonlinear Structure
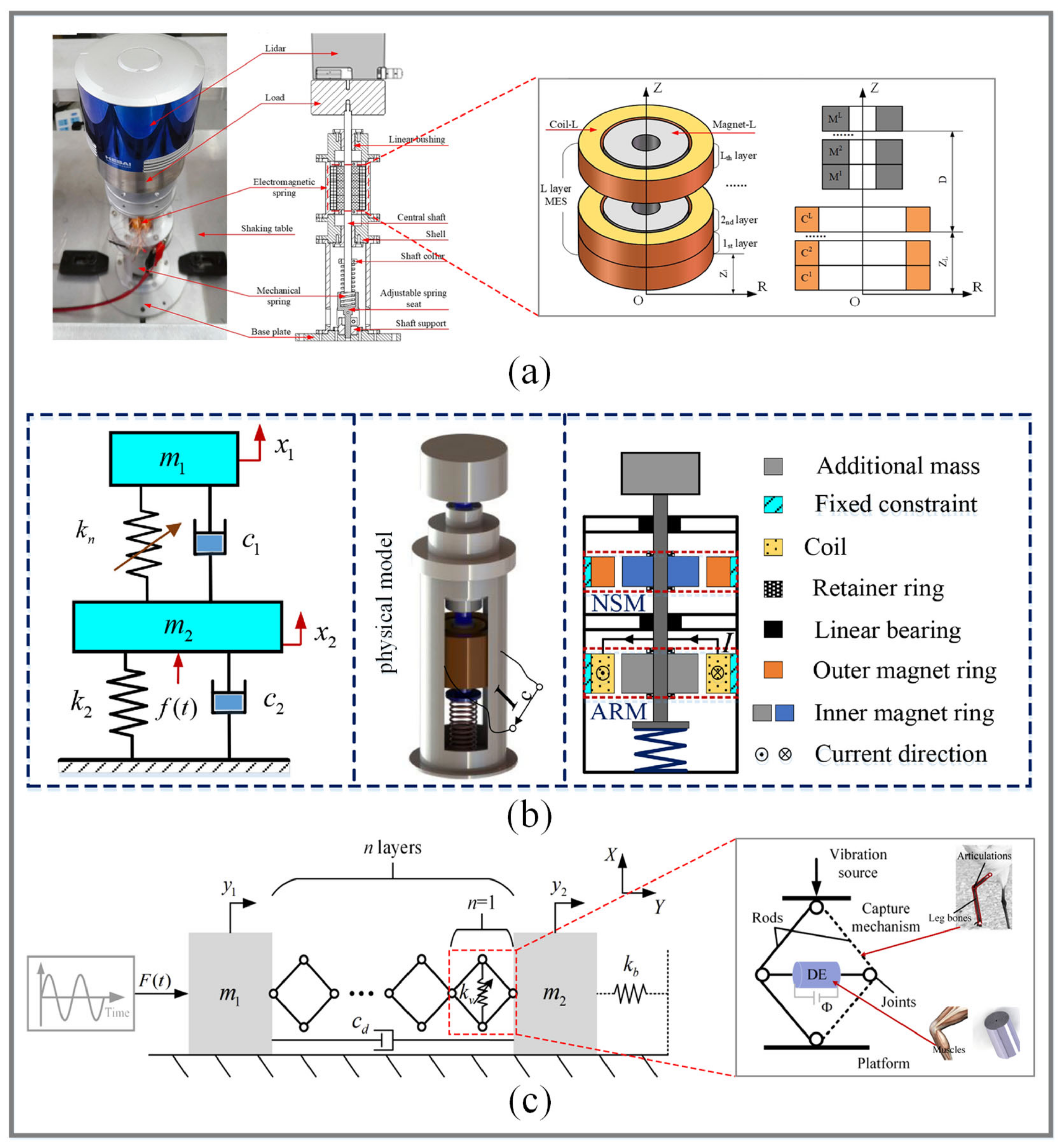
4.4. Magnetic Structure
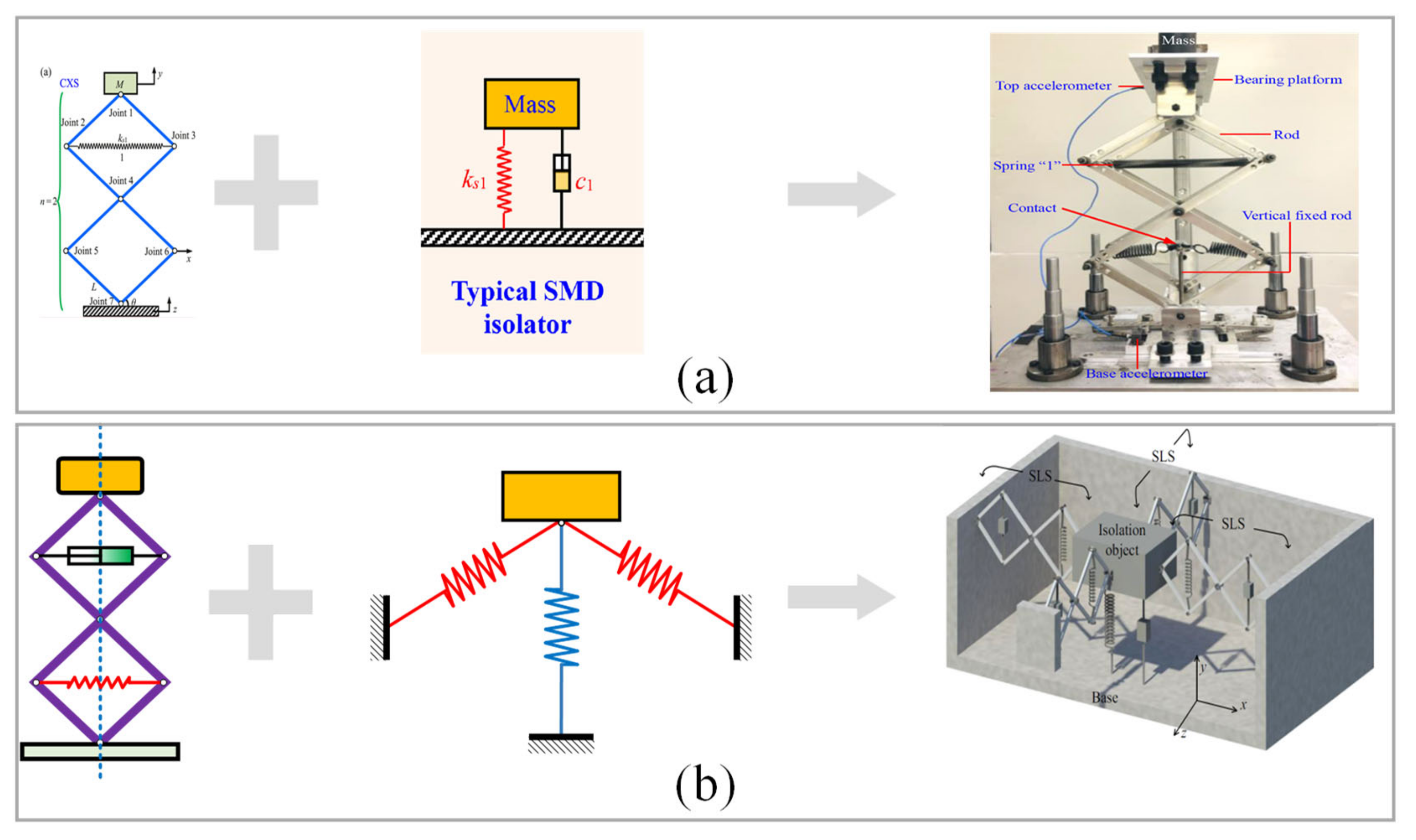
4.5. Bio-Inspired Structure
4.6. Composite Structure
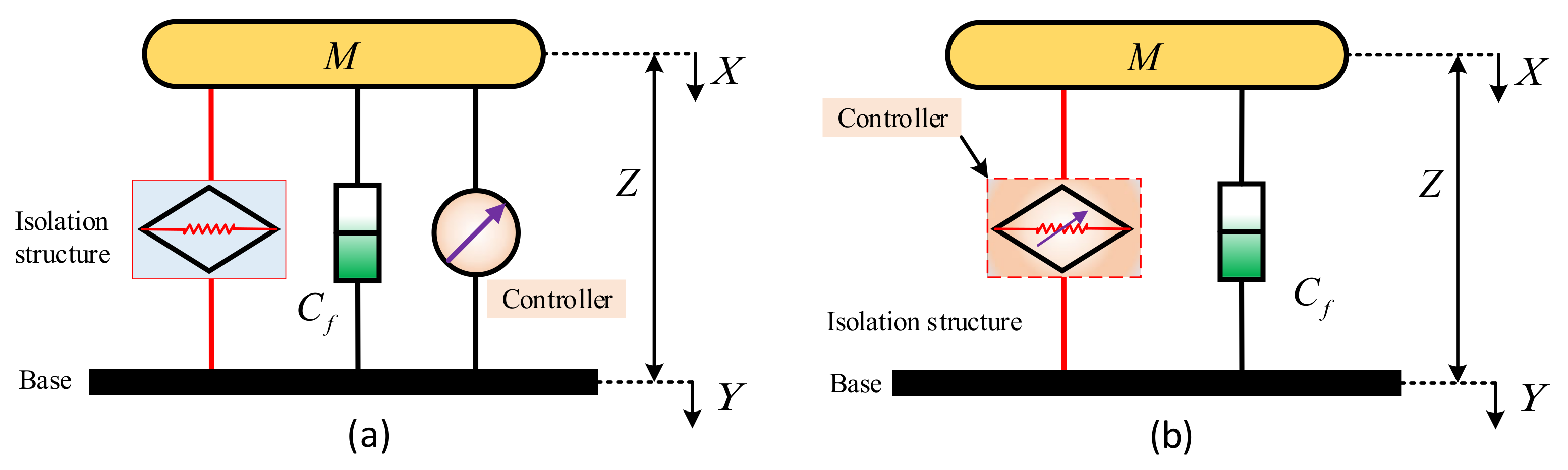
5. Active/Semi-Active QZS Isolator
6. Conclusions
- (1)
- Future directions for QZS vibration isolation may involve the optimization of existing structures and vibration isolation mechanisms for broadening the effective vibration isolation frequency band and reducing resonance peak. In particular, further study needs to be done on the modeling and realization of QZS vibration isolation systems. In the modeling process, the commonly used nonlinear degree is the nonlinear stiffness characteristics simulated by the cubic stiffness simulation system. More efforts need to be made in research on high-order stiffness to more accurately understand and predict the dynamic characteristics and vibration isolation effect of the system under high-order stiffness. In addition, most QZS vibration isolators are usually described as a single-degree-of-freedom (SDOF) system ignoring the mass of each component, which greatly affects the equivalent damping and equivalent stiffness of the system. Component mass should be taken as an important factor to further improve the accuracy of the vibration isolator model.
- (2)
- More research on the design and realization of multi-DOF and multi-stage QZS vibration isolation systems is needed. At present, SDOF QZS vibration isolation systems have been studied a lot, but due to the complexity and multidirectionality of excitation in practical engineering, multi-DOF and multistage low-frequency vibration isolation has broader application prospects. By imitating the multi-vertebra structure of a bird’s neck, a multi-stage vibration isolation method has been proposed. Compared with a single QZS system, this method expands the effective range of dynamic displacement and achieves good vibration isolation performance for low- and ultra-low-frequency vibrations. Therefore, the complex nonlinear behavior of these multi-DOF and multi-stage QZS isolators needs to be fully studied.
- (3)
- Composite structures and bio-inspired structures can further improve the vibration isolation performance and promote the diversity of QZS vibration isolators. The composite material has been designed in a special microstructure (unit cell), which has unique dynamic characteristics different from its natural form. Tunable composite materials further expand the application field. In recent years, the development of functional composite materials also provides more possibilities for variable stiffness and damping. In addition, using bio-inspired composite structures can avoid assembly clearance and mechanism wear, and thus reduce production cost and installation space. On the other hand, although composite materials designed in honeycomb structures have been found to be effective in suppressing random and harmonic excitation, and their effectiveness in suppressing impulsive and impact excitation needs to be studied in detail.
- (4)
- The combination of magnetic negative stiffness and intelligent control can further improve the applicability and stability of QZS vibration isolators. Generally, there are many mechanical negative-stiffness structures with an easy fabrication process and a simple working principle, but they have the disadvantages of nonlinearity, prominent contact friction, and limited performance. At present, for magnetic negative stiffness, specially arranged magnets are used to make the non-contact force show the negative-stiffness characteristic. This is a good way to realize QZS vibration isolation. However, more research efforts need to be made in terms of how to improve the volume density, linearity, and working range of magnetic negative-stiffness mechanisms by optimizing magnet structure and array. In addition, the active negative-stiffness mechanism makes the vibration control force show the negative-stiffness characteristic based on displacement feedback. With sufficient accuracy of sensors and actuators, the negative-stiffness characteristics will be accurately controllable, and the best QZS effect can be obtained theoretically. This is also an important research direction in this field. In conclusion, it is recommended to explore the integrated vibration isolators combining non-contact structures with intelligent control in the future.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kim, S.M.; Hong, J.R.; Yoo, H.H. Analysis and design of a torsional vibration isolator for rotating shafts. J. Mech. Sci. Technol. 2019, 33, 4627–4634. [Google Scholar] [CrossRef]
- Wiercigroch, M. Mechanical Vibrations: Theory and Application to Structural Dynamics; John Wiley & Sons Ltd Press: Chichester, UK, 2018; ISBN 978-1-118-90020-8. [Google Scholar] [CrossRef]
- Ibrahim, R.A. Recent advances in nonlinear passive vibration isolators. J. Sound Vib. 2008, 314, 371–452. [Google Scholar] [CrossRef]
- Li, H.; Li, Y.; Li, J. Negative stiffness devices for vibration isolation applications: A review. Adv. Struct. Eng. 2020, 23, 1739–1755. [Google Scholar] [CrossRef]
- Molyneux, W.G. Supports for Vibration Isolation; Her Majesty’s Stationery Office: London, UK, 1957. [Google Scholar]
- Wang, Y.C.; Lakes, R.S. Extreme stiffness systems due to negative stiffness elements. Am. J. Phys. 2004, 72, 40–50. [Google Scholar] [CrossRef]
- Bažant, Z.; Cedolin, L.; Tabbara, M. New method of analysis for slender columns. ACI Struct. J. 1991, 88, 391–401. [Google Scholar] [CrossRef]
- Zhao, F.; Ji, J.C.; Ye, K.; Luo, Q. Increase of quasi-zero stiffness region using two pairs of oblique springs. Mech. Syst. Signal Process. 2020, 144, 106975. [Google Scholar] [CrossRef]
- Zhao, F.; Ji, J.; Ye, K.; Luo, Q. An innovative quasi-zero stiffness isolator with three pairs of oblique springs. Int. J. Mech. Sci. 2021, 192, 106093. [Google Scholar] [CrossRef]
- Zhao, F.; Ji, J.; Luo, Q.; Cao, S.; Chen, L.; Du, W. An improved quasi-zero stiffness isolator with two pairs of oblique springs to increase isolation frequency band. Nonlinear Dyn. 2021, 104, 349–365. [Google Scholar] [CrossRef]
- Xu, D.; Zhang, Y.; Zhou, J.; Lou, J. On the analytical and experimental assessment of the performance of a quasi-zero-stiffness isolator. J. Vib. Control 2014, 20, 2314–2325. [Google Scholar] [CrossRef]
- Carrella, A.; Brennan, M.J.; Waters, T.P. Static analysis of a passive vibration isolator with quasi-zero-stiffness characteristic. J. Sound Vib. 2007, 301, 678–689. [Google Scholar] [CrossRef]
- Carrella, A.; Brennan, M.J.; Waters, T.P.; Lopes, V., Jr. Force and displacement transmissibility of a nonlinear isolator with high-static-low-dynamic-stiffness. Int. J. Mech. Sci. 2012, 55, 22–29. [Google Scholar] [CrossRef]
- Liu, C.; Yu, K.; Liao, B.; Hu, R. Enhanced vibration isolation performance of quasi-zero-stiffness isolator by introducing tunable nonlinear inerter. Commun. Nonlinear Sci. Numer. Simul. 2021, 95, 105654. [Google Scholar] [CrossRef]
- Gatti, G.; Shaw, A.D.; Gonçalves, P.J.P.; Brennan, M.J. On the detailed design of a quasi-zero stiffness device to assist in the realisation of a translational Lanchester damper. Mech. Syst. Signal Process. 2022, 164, 108258. [Google Scholar] [CrossRef]
- Shaw, A.D.; Gatti, G.; Gonçalves, P.J.P.; Tang, B.; Brennan, M.J. Design and test of an adjustable quasi-zero stiffness device and its use to suspend masses on a multi-modal structure. Mech. Syst. Signal Process. 2021, 152, 107354. [Google Scholar] [CrossRef]
- Liu, Y.; Ji, W.; Gu, H.; Deng, E.; Wang, X.; Song, C. Force transmissibility of a 6-DOF passive quasi-zero stiffness vibration isolation platform. J. Mech. Sci. Technol. 2021, 35, 2313–2324. [Google Scholar] [CrossRef]
- Niu, F.; Meng, L.; Wu, W.; Sun, J.; Zhang, W.; Meng, G.; Rao, Z. Design and analysis of a quasi-zero stiffness isolator using a slotted conical disk spring as negative stiffness structure. J. Vibroeng. 2014, 16, 1769–1785. [Google Scholar]
- Valeev, A.R.; Zotov, A.N.; Kharisov, S.A. Application of disk springs for manufacturing vibration isolators with quasi-zero stiffness. Chem. Petrol. Eng. 2015, 51, 194–200. [Google Scholar] [CrossRef]
- Chen, P.; Zhou, Y. Experiment and Numerical Investigations on a Vertical Isolation System with Quasi-Zero Stiffness Property. In Vibration Engineering for a Sustainable Future; Oberst, S., Halkon, B., Ji, J., Brown, T., Eds.; Springer: Cham, Switzerland, 2021; pp. 255–261. [Google Scholar] [CrossRef]
- Lan, C.C.; Yang, S.A.; Wu, Y.S. Design and experiment of a compact quasi-zero-stiffness isolator capable of a wide range of loads. J. Sound Vib. 2014, 333, 4843–4858. [Google Scholar] [CrossRef]
- Liu, C.; Yu, K. Design and experimental study of a quasi-zero-stiffness vibration isolator incorporating transverse groove springs. Arch. Civ. Mech. Eng. 2020, 20, 67. [Google Scholar] [CrossRef]
- Le, T.D.; Ahn, K.K. A vibration isolation system in low frequency excitation region using negative stiffness structure for vehicle seat. J. Sound Vib. 2011, 330, 6311–6335. [Google Scholar] [CrossRef]
- Le, T.D.; Ahn, K.K. Experimental investigation of a vibration isolation system using negative stiffness structure. Int. J. Mech. Sci. 2013, 70, 99–112. [Google Scholar] [CrossRef]
- Liu, C.; Yu, K. Superharmonic resonance of the quasi-zero-stiffness vibration isolator and its effect on the isolation performance. Nonlinear Dyn. 2020, 100, 95–117. [Google Scholar] [CrossRef]
- Ye, K.; Ji, J.C. An origami inspired quasi-zero stiffness vibration isolator using a novel truss-spring based stack Miura-ori structure. Mech. Syst. Signal Process. 2022, 165, 108383. [Google Scholar] [CrossRef]
- Han, H.; Sorokin, V.; Tang, L.; Cao, D. A nonlinear vibration isolator with quasi-zero-stiffness inspired by Miura-origami tube. Nonlinear Dyn. 2021, 105, 1313–1325. [Google Scholar] [CrossRef]
- Yang, X.; Zheng, J.; Xu, J.; Li, W.; Wang, Y.; Fan, M. Structural design and isolation characteristic analysis of new quasi-zero-stiffness. J. Vib. Eng. Technol. 2020, 8, 47–58. [Google Scholar] [CrossRef]
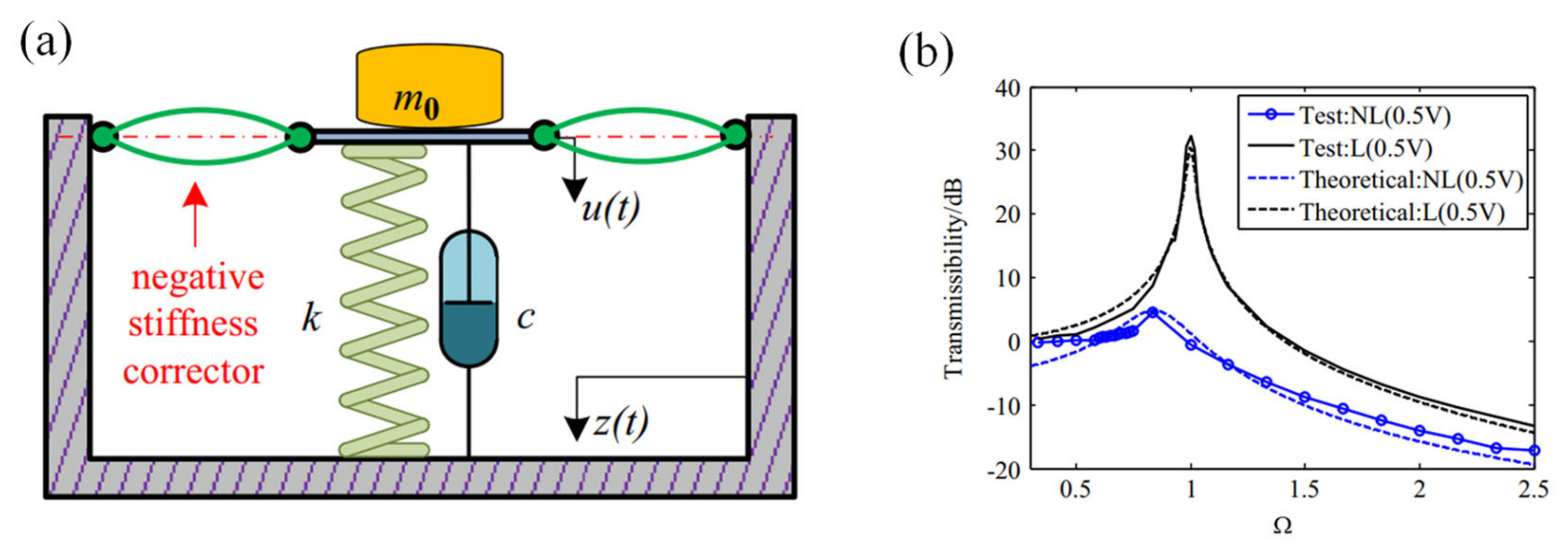
- Antoniadis, I.; Chronopoulos, D.; Spitas, V.; Koulocheris, D. Hyper-damping properties of a stiff and stable linear oscillator with a negative stiffness element. J. Sound Vib. 2015, 346, 37–52. [Google Scholar] [CrossRef]
- Wang, K.; Zhou, J.; Chang, Y.; Ouyang, H.; Xu, D.; Yang, Y. A nonlinear ultra-low-frequency vibration isolator with dual quasi-zero-stiffness mechanism. Nonlinear Dyn. 2020, 101, 755–773. [Google Scholar] [CrossRef]
- Zhu, G.; Liu, J.; Cao, Q.; Cheng, Y.; Lu, Z.; Zhu, Z. A two degree of freedom stable quasi-zero stiffness prototype and its applications in aseismic engineering. Sci. China-Technol. Sci. 2020, 63, 496–505. [Google Scholar] [CrossRef]
- Wu, T.H.; Lan, C.C. A wide-range variable stiffness mechanism for semi-active vibration systems. J. Sound Vib. 2016, 363, 18–32. [Google Scholar] [CrossRef]
- Wang, X.; Liu, H.; Chen, Y.; Gao, P. Beneficial stiffness design of a high-static-low-dynamic-stiffness vibration isolator based on static and dynamic analysis. Int. J. Mech. Sci. 2018, 142, 235–244. [Google Scholar] [CrossRef]
- Kashdan, L.; Seepersad, C.C.; Haberman, M.; Wilson, P.S. Design, fabrication, and evaluation of negative stiffness elements using SLS. Rapid Prototyp. J. 2012, 18, 194–200. [Google Scholar] [CrossRef]
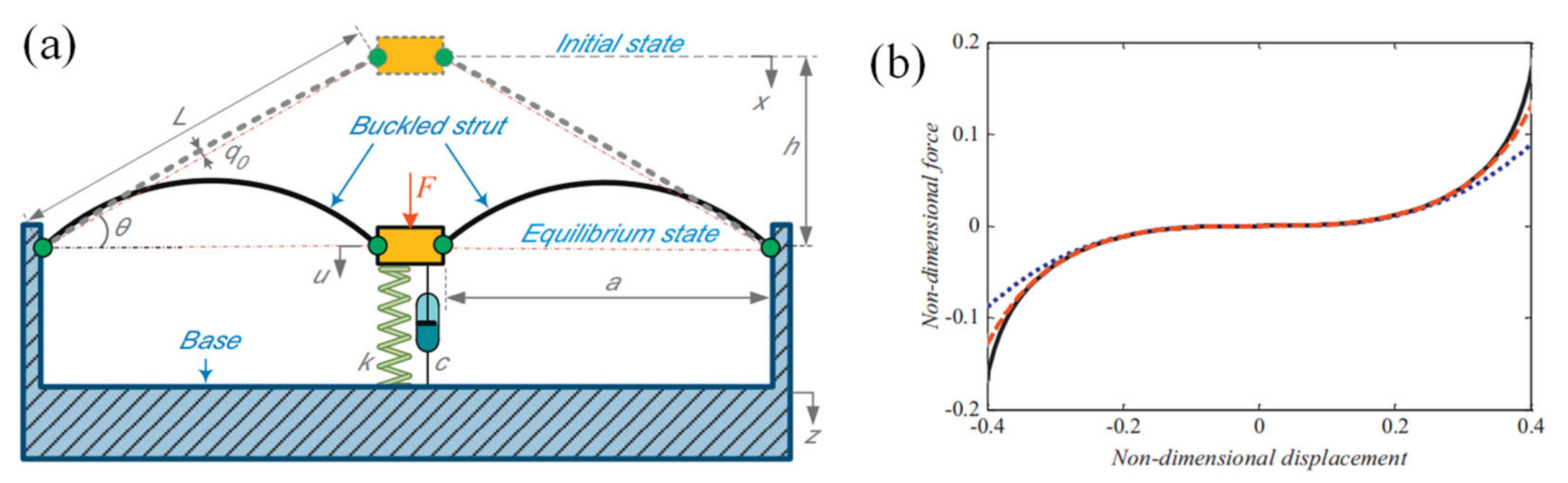
- Fulcher, B.A.; Shahan, D.W.; Haberman, M.R.; Conner Seepersad, C.; Wilson, P.S. Analytical and experimental investigation of buckled beams as negative stiffness elements for passive vibration and shock isolation systems. J. Vib. Acoust.-Trans. ASME. 2014, 136, 031009. [Google Scholar] [CrossRef]
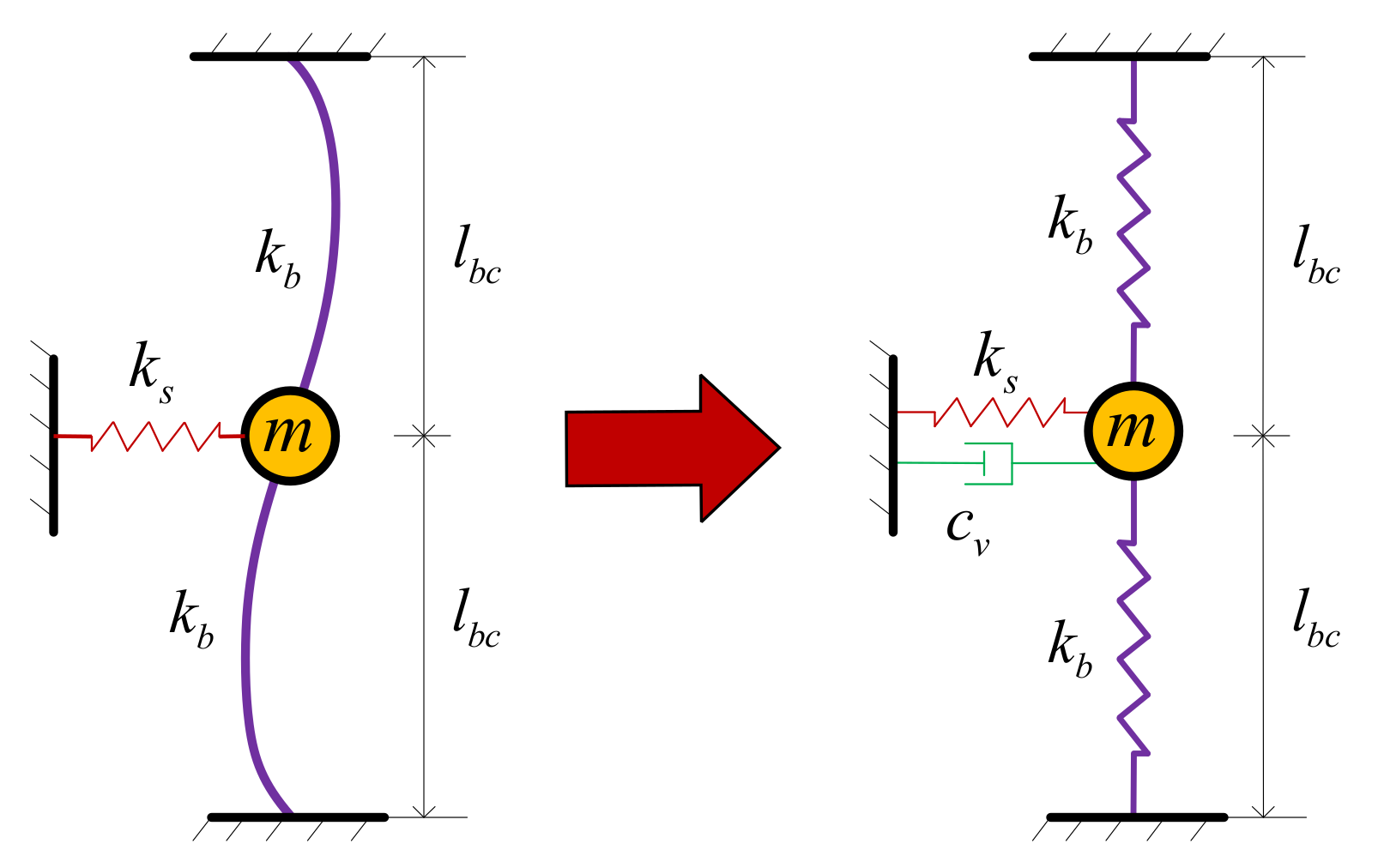
- Liu, X.; Huang, X.; Hua, H. On the characteristics of a quasi-zero stiffness isolator using Euler buckled beam as negative stiffness corrector. J. Sound Vib. 2013, 332, 3359–3376. [Google Scholar] [CrossRef]
- Liu, X.; Zhao, Q.; Zhang, Z.; Zhou, X. An experiment investigation on the effect of Coulomb friction on the displacement transmissibility of a quasi-zero stiffness isolator. J. Mech. Sci. Technol. 2019, 33, 121–127. [Google Scholar] [CrossRef]
- Huang, X.; Liu, X.; Sun, J.; Zhang, Z.; Hua, H. Vibration isolation characteristics of a nonlinear isolator using Euler buckled beam as negative stiffness corrector: A theoretical and experimental study. J. Sound Vib. 2014, 333, 1132–1148. [Google Scholar] [CrossRef]
- Kim, J.; Jeon, Y.; Um, S.; Park, U.; Kim, K.S.; Kim, S. A novel passive quasi-zero stiffness isolator for ultra-precision measurement systems. Int. J. Precis. Eng. Manuf. 2019, 20, 1573–1580. [Google Scholar] [CrossRef]
- Zou, D.; Liu, G.; Rao, Z.; Tan, T.; Zhang, W.; Liao, W.H. A device capable of customizing nonlinear forces for vibration energy harvesting, vibration isolation, and nonlinear energy sink. Mech. Syst. Signal Process. 2021, 147, 107101. [Google Scholar] [CrossRef]
- Gatti, G. An adjustable device to adaptively realise diverse nonlinear force-displacement characteristics. Mech. Syst. Signal Process. 2022, 180, 109379. [Google Scholar] [CrossRef]
- Sun, M.; Song, G.; Li, Y.; Huang, Z. Effect of negative stiffness mechanism in a vibration isolator with asymmetric and high-static-low-dynamic stiffness. Mech. Syst. Signal Process. 2019, 124, 388–407. [Google Scholar] [CrossRef]
- Liu, Y.; Ji, W.; Xu, L.; Gu, H.; Song, C. Dynamic characteristics of quasi-zero stiffness vibration isolation system for coupled dynamic vibration absorber. Arch. Appl. Mech. 2021, 91, 3799–3818. [Google Scholar] [CrossRef]
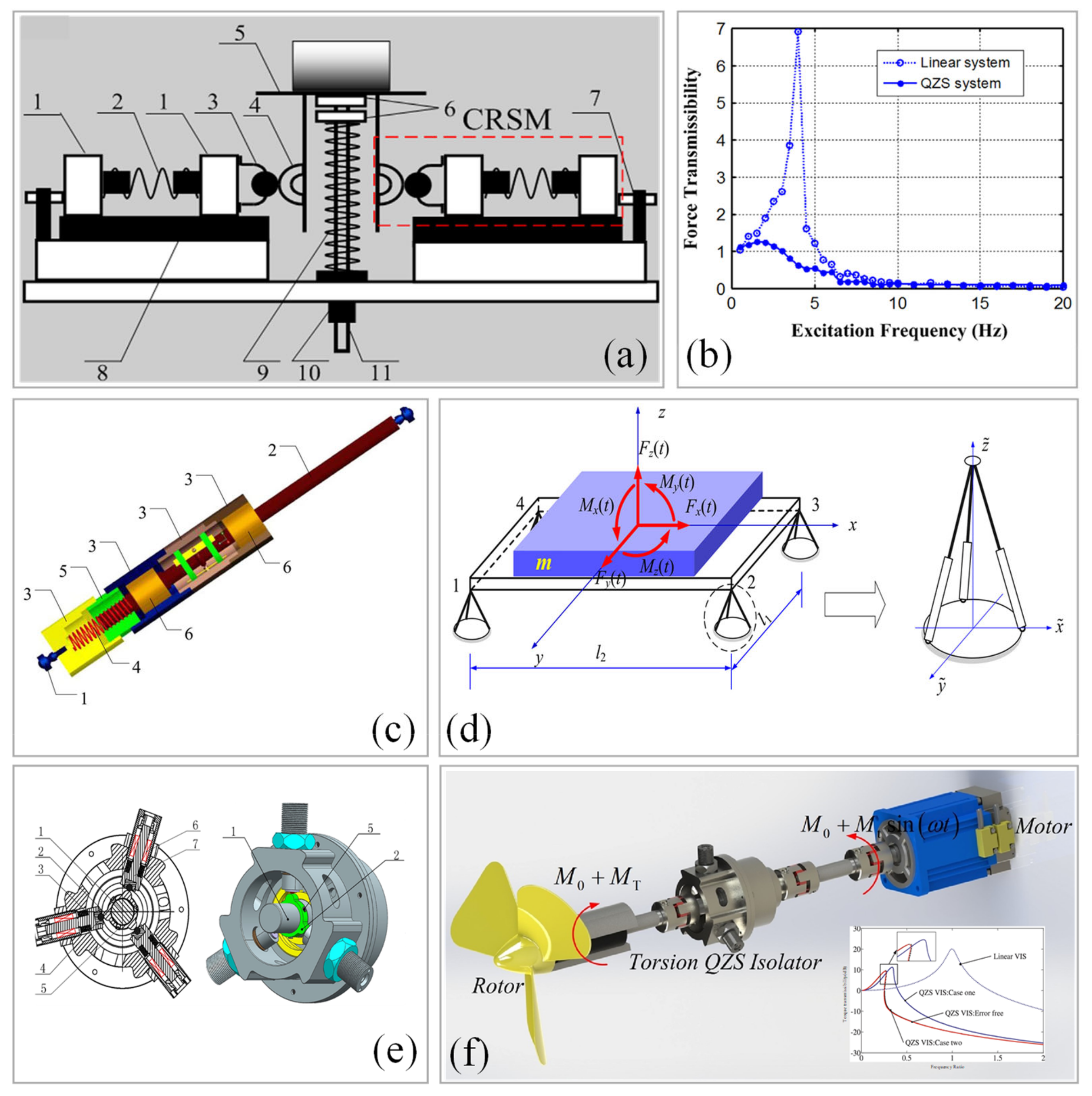
- Zhou, J.; Wang, X.; Xu, D.; Bishop, S. Nonlinear dynamic characteristics of a quasi-zero stiffness vibration isolator with cam–roller–spring mechanisms. J. Sound Vib. 2015, 346, 53–69. [Google Scholar] [CrossRef]
- Zhou, J.; Xiao, Q.; Xu, D.; Ouyang, H.; Li, Y. A novel quasi-zero-stiffness strut and its applications in six-degree-of-freedom vibration isolation platform. J. Sound Vib. 2017, 394, 59–74. [Google Scholar] [CrossRef]
- Wang, K.; Zhou, J.; Xu, D. Sensitivity analysis of parametric errors on the performance of a torsion quasi-zero-stiffness vibration isolator. Int. J. Mech. Sci. 2017, 134, 336–346. [Google Scholar] [CrossRef]
- Zhou, J.; Xu, D.; Bishop, S. A torsion quasi-zero stiffness vibration isolator. J. Sound Vib. 2015, 338, 121–133. [Google Scholar] [CrossRef]
- Ye, K.; Ji, J.C.; Brown, T. A novel integrated quasi-zero stiffness vibration isolator for coupled translational and rotational vibrations. Mech. Syst. Signal Process. 2021, 149, 107340. [Google Scholar] [CrossRef]
- Sun, Y.; Zhou, J.; Thompson, D.; Yuan, T.; Gong, D.; You, T. Design, analysis and experimental validation of high static and low dynamic stiffness mounts based on target force curves. Int. J. Non-Linear Mech. 2020, 126, 103559. [Google Scholar] [CrossRef]
- Yao, Y.; Li, H.; Li, Y.; Wang, X. Analytical and experimental investigation of a high-static-low-dynamic stiffness isolator with cam-roller-spring mechanism. Int. J. Mech. Sci. 2020, 186, 105888. [Google Scholar] [CrossRef]
- Liu, Y.; Xu, L.; Song, C.; Gu, H.; Ji, W. Dynamic characteristics of a quasi-zero stiffness vibration isolator with nonlinear stiffness and damping. Arch. Appl. Mech. 2019, 89, 1743–1759. [Google Scholar] [CrossRef]
- Zuo, S.; Wang, D.; Zhang, Y.; Luo, Q. Design and testing of a parabolic cam-roller quasi-zero-stiffness vibration isolator. Int. J. Mech. Sci. 2022, 220, 107146. [Google Scholar] [CrossRef]
- Li, M.; Cheng, W.; Xie, R. A quasi-zero-stiffness vibration isolator using a cam mechanism with user-defined profile. Int. J. Mech. Sci. 2021, 189, 105938. [Google Scholar] [CrossRef]
- Ye, K.; Ji, J.C.; Brown, T. Design of a quasi-zero stiffness isolation system for supporting different loads. J. Sound Vib. 2020, 471, 115198. [Google Scholar] [CrossRef]
- Yan, B.; Yu, N.; Wu, C. A state-of-the-art review on low-frequency nonlinear vibration isolation with electromagnetic mechanisms. Appl. Math. Mech.-Engl. Ed. 2022, 43, 1045–1062. [Google Scholar] [CrossRef]
- Wang, S.; Xin, W.; Ning, Y.; Li, B.; Hu, Y. Design, experiment, and improvement of a quasi-zero-stiffness vibration isolation system. Appl. Sci. 2020, 10, 2273. [Google Scholar] [CrossRef]
- Zheng, Y.; Zhang, X.; Luo, Y.; Yan, B.; Ma, C. Design and experiment of a high-static–low-dynamic stiffness isolator using a negative stiffness magnetic spring. J. Sound Vib. 2016, 360, 31–52. [Google Scholar] [CrossRef]
- Zheng, Y.; Zhang, X.; Luo, Y.; Zhang, Y.; Xie, S. Analytical study of a quasi-zero stiffness coupling using a torsion magnetic spring with negative stiffness. Mech. Syst. Signal Process. 2018, 100, 135–151. [Google Scholar] [CrossRef]
- Xu, J.; Yang, X.; Li, W.; Zheng, J.; Wang, Y.; Fan, M.; Lu, Y. Design of quasi-zero stiffness joint actuator and research on vibration isolation performance. J. Sound Vib. 2020, 479, 115367. [Google Scholar] [CrossRef]
- Xu, D.; Yu, Q.; Zhou, J.; Bishop, S.R. Theoretical and experimental analyses of a nonlinear magnetic vibration isolator with quasi-zero-stiffness characteristic. J. Sound Vib. 2013, 332, 3377–3389. [Google Scholar] [CrossRef]
- Wang, Q.; Zhou, J.; Wang, K.; Xu, D.; Wen, G. Design and experimental study of a compact quasi-zero-stiffness isolator using wave springs. Sci. China-Technol. Sci. 2021, 64, 2255–2271. [Google Scholar] [CrossRef]
- Wu, W.; Chen, X.; Shan, Y. Analysis and experiment of a vibration isolator using a novel magnetic spring with negative stiffness. J. Sound Vib. 2014, 333, 2958–2970. [Google Scholar] [CrossRef]
- Yan, B.; Wang, X.; Wang, Z.; Wu, C.; Zhang, W. Enhanced lever-type vibration isolator via electromagnetic shunt damping. Int. J. Mech. Sci. 2022, 218, 107070. [Google Scholar] [CrossRef]
- Yan, B.; Yu, N.; Wang, Z.; Wu, C.; Wang, S.; Zhang, W. Lever-type quasi-zero stiffness vibration isolator with magnetic spring. J. Sound Vib. 2022, 527, 116865. [Google Scholar] [CrossRef]
- Liu, C.; Zhao, R.; Yu, K.; Liao, B. In-plane quasi-zero-stiffness vibration isolator using magnetic interaction and cables: Theoretical and experimental study. Appl. Math. Model. 2021, 96, 497–522. [Google Scholar] [CrossRef]
- Shan, Y.; Wu, W.; Chen, X. Design of a miniaturized pneumatic vibration isolator with high-static-low-dynamic stiffness. J. Vib. Acoust.-Trans. ASME 2015, 137, 045001. [Google Scholar] [CrossRef]
- Yan, B.; Ma, H.; Zhao, C.; Wu, C.; Wang, K.; Wang, P. A vari-stiffness nonlinear isolator with magnetic effects: Theoretical modeling and experimental verification. Int. J. Mech. Sci. 2018, 148, 745–755. [Google Scholar] [CrossRef]
- Ma, H.; Yan, B. Nonlinear damping and mass effects of electromagnetic shunt damping for enhanced nonlinear vibration isolation. Mech. Syst. Signal Process. 2021, 146, 107010. [Google Scholar] [CrossRef]
- Yan, B.; Ma, H.; Zhang, L.; Zheng, W.; Wang, K.; Wu, C. A bistable vibration isolator with nonlinear electromagnetic shunt damping. Mech. Syst. Signal Process. 2020, 136, 106504. [Google Scholar] [CrossRef]
- Dong, G.; Zhang, X.; Xie, S.; Yan, B.; Luo, Y. Simulated and experimental studies on a high-static-low-dynamic stiffness isolator using magnetic negative stiffness spring. Mech. Syst. Signal Process. 2017, 86, 188–203. [Google Scholar] [CrossRef]
- Dong, G.; Zhang, Y.; Luo, Y.; Xie, S.; Zhang, X. Enhanced isolation performance of a high-static–low-dynamic stiffness isolator with geometric nonlinear damping. Nonlinear Dyn. 2018, 93, 2339–2356. [Google Scholar] [CrossRef]
- Dong, G.; Zhang, X.; Luo, Y.; Zhang, Y.; Xie, S. Analytical study of the low frequency multi-direction isolator with high-static-low-dynamic stiffness struts and spatial pendulum. Mech. Syst. Signal Process. 2018, 110, 521–539. [Google Scholar] [CrossRef]
- Zhou, J.; Wang, K.; Xu, D.; Ouyang, H.; Li, Y. A six degrees-of-freedom vibration isolation platform supported by a hexapod of quasi-zero-stiffness struts. J. Vib. Acoust.-Trans. ASME 2017, 139, 034502. [Google Scholar] [CrossRef]
- Zheng, Y.; Li, Q.; Yan, B.; Luo, Y.; Zhang, X. A Stewart isolator with high-static-low-dynamic stiffness struts based on negative stiffness magnetic springs. J. Sound Vib. 2018, 422, 390–408. [Google Scholar] [CrossRef]
- Yuan, S.; Sun, Y.; Wang, M.; Ding, J.; Zhao, J.; Huang, Y.; Yang, X.D. Tunable negative stiffness spring using maxwell normal stress. Int. J. Mech. Sci. 2021, 193, 106127. [Google Scholar] [CrossRef]
- Jiang, Y.; Song, C.; Ding, C.; Xu, B. Design of magnetic-air hybrid quasi-zero stiffness vibration isolation system. J. Sound Vib. 2020, 477, 115346. [Google Scholar] [CrossRef]
- Yuan, S.; Sun, Y.; Zhao, J.; Meng, K.; Wang, M.; Pu, H.; Xie, S. A tunable quasi-zero stiffness isolator based on a linear electromagnetic spring. J. Sound Vib. 2020, 482, 115449. [Google Scholar] [CrossRef]
- Sun, Y.; Zhao, J.; Wang, M.; Sun, Y.; Pu, H.; Luo, J.; Yang, Y. High-static–low-dynamic stiffness isolator with tunable electromagnetic mechanism. IEEE-ASME Trans. Mechatron. 2019, 25, 316–326. [Google Scholar] [CrossRef]
- Sun, Y.; Meng, K.; Yuan, S.; Zhao, J.; Xie, R.; Yang, Y.; Pu, H. Modeling electromagnetic force and axial-stiffness for an electromagnetic negative-stiffness spring toward vibration isolation. IEEE Trans. Magn. 2019, 55, 1–10. [Google Scholar] [CrossRef]
- Robertson, W.S.; Kidner, M.R.F.; Cazzolato, B.S.; Zander, A.C. Theoretical design parameters for a quasi-zero stiffness magnetic spring for vibration isolation. J. Sound Vib. 2009, 326, 88–103. [Google Scholar] [CrossRef]
- Li, Q.; Zhu, Y.; Xu, D.; Hu, J.; Min, W.; Pang, L. A negative stiffness vibration isolator using magnetic spring combined with rubber membrane. J. Mech. Sci. Technol. 2013, 27, 813–824. [Google Scholar] [CrossRef]
- Zhu, T.; Cazzolato, B.; Robertson, W.S.; Zander, A. Vibration isolation using six degree-of-freedom quasi-zero stiffness magnetic levitation. J. Sound Vib. 2015, 358, 48–73. [Google Scholar] [CrossRef]
- Kamaruzaman, N.A.; Robertson, W.S.; Ghayesh, M.H.; Cazzolato, B.S.; Zander, A.C. Six degree of freedom quasi-zero stiffness magnetic spring with active control: Theoretical analysis of passive versus active stability for vibration isolation. J. Sound Vib. 2021, 502, 116086. [Google Scholar] [CrossRef]
- Kamaruzaman, N.A.; Robertson, W.S.; Ghayesh, M.H.; Cazzolato, B.S.; Zander, A.C. Vibration Isolation Performance of an LQR-Stabilised Planar Quasi-zero Stiffness Magnetic Levitation System. In Vibration Engineering for a Sustainable Future; Oberst, S., Halkon, B., Ji, J., Brown, T., Eds.; Springer: Cham, Switzerland, 2021; pp. 237–243. [Google Scholar] [CrossRef]
- Dai, H.; Jing, X.; Wang, Y.; Yue, X.; Yuan, J. Post-capture vibration suppression of spacecraft via a bio-inspired isolation system. Mech. Syst. Signal Process. 2018, 105, 214–240. [Google Scholar] [CrossRef]
- Wu, Z.; Jing, X.; Bian, J.; Li, F.; Allen, R. Vibration isolation by exploring bio-inspired structural nonlinearity. Bioinspir. Biomim. 2015, 10, 056015. [Google Scholar] [CrossRef]
- Gatti, G. Optimizing elastic potential energy via geometric nonlinear stiffness. Commun. Nonlinear Sci. Numer. Simul. 2021, 103, 106035. [Google Scholar] [CrossRef]
- Chong, X.; Wu, Z.; Li, F. Vibration isolation properties of the nonlinear X-combined structure with a high-static and low-dynamic stiffness: Theory and experiment. Mech. Syst. Signal Process. 2022, 179, 109352. [Google Scholar] [CrossRef]
- Wang, Y.; Jing, X.; Guo, Y. Nonlinear analysis of a bio-inspired vertically asymmetric isolation system under different structural constraints. Nonlinear Dyn. 2019, 95, 445–464. [Google Scholar] [CrossRef]
- Yan, G.; Zou, H.X.; Wang, S.; Zhao, L.C.; Wu, Z.Y.; Zhang, W.M. Bio-inspired vibration isolation: Methodology and design. Appl. Mech. Rev. 2021, 73, 020801. [Google Scholar] [CrossRef]
- Dai, H.; Jing, X.; Sun, C.; Wang, Y.; Yue, X. Accurate modeling and analysis of a bio-inspired isolation system: With application to on-orbit capture. Mech. Syst. Signal Process. 2018, 109, 111–133. [Google Scholar] [CrossRef]
- Hu, F.; Jing, X. A 6-DOF passive vibration isolator based on Stewart structure with X-shaped legs. Nonlinear Dyn. 2018, 91, 157–185. [Google Scholar] [CrossRef]
- Chai, Y.; Jing, X.; Guo, Y. A compact X-shaped mechanism based 3-DOF anti-vibration unit with enhanced tunable QZS property. Mech. Syst. Signal Process. 2022, 168, 108651. [Google Scholar] [CrossRef]
- Sun, X.; Jing, X. A nonlinear vibration isolator achieving high-static-low-dynamic stiffness and tunable anti-resonance frequency band. Mech. Syst. Signal Process. 2016, 80, 166–188. [Google Scholar] [CrossRef]
- Yan, G.; Zou, H.X.; Wang, S.; Zhao, L.C.; Wu, Z.Y.; Zhang, W.M. Bio-inspired toe-like structure for low-frequency vibration isolation. Mech. Syst. Signal Process. 2022, 162, 108010. [Google Scholar] [CrossRef]
- Chai, Y.; Jing, X.; Chao, X. X-shaped mechanism based enhanced tunable QZS property for passive vibration isolation. Int. J. Mech. Sci. 2022, 218, 107077. [Google Scholar] [CrossRef]
- Wu, Z.; Jing, X.; Sun, B.; Li, F. A 6DOF passive vibration isolator using X-shape supporting structures. J. Sound Vib. 2016, 380, 90–111. [Google Scholar] [CrossRef]
- Dai, H.; Cao, X.; Jing, X.; Wang, X.; Yue, X. Bio-inspired anti-impact manipulator for capturing non-cooperative spacecraft: Theory and experiment. Mech. Syst. Signal Process. 2020, 142, 106785. [Google Scholar] [CrossRef]
- Yan, G.; Zou, H.X.; Wang, S.; Zhao, L.C.; Gao, Q.H.; Tan, T.; Zhang, W.M. Large stroke quasi-zero stiffness vibration isolator using three-link mechanism. J. Sound Vib. 2020, 478, 115344. [Google Scholar] [CrossRef]
- Yan, G.; Wang, S.; Zou, H.; Zhao, L.; Gao, Q.; Zhang, W. Bio-inspired polygonal skeleton structure for vibration isolation: Design, modelling, and experiment. Sci. China-Technol. Sci. 2020, 63, 2617–2630. [Google Scholar] [CrossRef]
- Sun, X.; Xu, J.; Wang, F.; Zhang, S. A novel isolation structure with flexible joints for impact and ultralow-frequency excitations. Int. J. Mech. Sci. 2018, 146, 366–376. [Google Scholar] [CrossRef]
- Zeng, R.; Wen, G.; Zhou, J.; Zhao, G. Limb-inspired bionic quasi-zero stiffness vibration isolator. Acta Mech. Sin. 2021, 37, 1152–1167. [Google Scholar] [CrossRef]
- Deng, T.; Wen, G.; Ding, H.; Lu, Z.Q.; Chen, L.Q. A bio-inspired isolator based on characteristics of quasi-zero stiffness and bird multi-layer neck. Mech. Syst. Signal Process. 2020, 145, 106967. [Google Scholar] [CrossRef]
- Jin, G.; Wang, Z.; Yang, T. Cascaded quasi-zero stiffness nonlinear low-frequency vibration isolator inspired by human spine. Appl. Math. Mech.-Engl. Ed. 2022, 43, 813–824. [Google Scholar] [CrossRef]
- Sun, X.; Qi, Z.; Xu, J. A novel multi-layer isolation structure for transverse stabilization inspired by neck structure. Acta Mech. Sin. 2022, 38, 521543. [Google Scholar] [CrossRef]
- Zeng, R.; Yin, S.; Wen, G.; Zhou, J. A non-smooth quasi-zero-stiffness isolator with displacement constraints. Int. J. Mech. Sci. 2022, 225, 107351. [Google Scholar] [CrossRef]
- Bian, J.; Jing, X. Analysis and design of a novel and compact X-structured vibration isolation mount (X-Mount) with wider quasi-zero-stiffness range. Nonlinear Dyn. 2020, 101, 2195–2222. [Google Scholar] [CrossRef]
- Sun, X.; Jing, X. Multi-direction vibration isolation with quasi-zero stiffness by employing geometrical nonlinearity. Mech. Syst. Signal Process. 2015, 62, 149–163. [Google Scholar] [CrossRef]
- Lakes, R.S. Extreme damping in compliant composites with a negative-stiffness phase. Philos. Mag. Lett. 2001, 81, 95–100. [Google Scholar] [CrossRef]
- Lakes, R.S.; Lee, T.; Bersie, A.; Wang, Y.C. Extreme damping in composite materials with negative-stiffness inclusions. Nature 2001, 410, 565–567. [Google Scholar] [CrossRef]
- Lakes, R.S.; Drugan, W.J. Dramatically stiffer elastic composite materials due to a negative stiffness phase? J. Mech. Phys. Solids 2002, 50, 979–1009. [Google Scholar] [CrossRef]
- Drugan, W.J. Elastic composite materials having a negative stiffness phase can be stable. Phys. Rev. Lett. 2007, 98, 055502. [Google Scholar] [CrossRef]
- Izard, A.G.; Alfonso, R.F.; McKnight, G.; Valdevit, L. Optimal design of a cellular material encompassing negative stiffness elements for unique combinations of stiffness and elastic hysteresis. Mater. Des. 2017, 135, 37–50. [Google Scholar] [CrossRef]
- Correa, D.M.; Klatt, T.; Cortes, S.; Haberman, M.; Kovar, D.; Seepersad, C. Negative stiffness honeycombs for recoverable shock isolation. Rapid Prototyp. J. 2015, 21, 193–200. [Google Scholar] [CrossRef]
- Virk, K.; Monti, A.; Trehard, T.; Marsh, M.; Hazra, K.; Boba, K.; Farrow, I.R. SILICOMB PEEK Kirigami cellular structures: Mechanical response and energy dissipation through zero and negative stiffness. Smart Mater. Struct. 2013, 22, 084014. [Google Scholar] [CrossRef]
- Cai, C.; Zhou, J.; Wu, L.; Wang, K.; Xu, D.; Ouyang, H. Design and numerical validation of quasi-zero-stiffness metamaterials for very low-frequency band gaps. Compos. Struct. 2020, 236, 111862. [Google Scholar] [CrossRef]
- Zhou, J.; Pan, H.; Cai, C.; Xu, D. Tunable ultralow frequency wave attenuations in one-dimensional quasi-zero-stiffness metamaterial. Int. J. Mech. Mater. Des. 2021, 17, 285–300. [Google Scholar] [CrossRef]
- Fan, H.; Yang, L.; Tian, Y.; Wang, Z. Design of metastructures with quasi-zero dynamic stiffness for vibration isolation. Compos. Struct. 2020, 243, 112244. [Google Scholar] [CrossRef]
- Ren, C.; Yang, D.; Qin, H. Mechanical performance of multidirectional buckling-based negative stiffness metamaterials: An analytical and numerical study. Materials 2018, 11, 1078. [Google Scholar] [CrossRef]
- Sun, X.; Xu, J.; Fu, J. The effect and design of time delay in feedback control for a nonlinear isolation system. Mech. Syst. Signal Process. 2017, 87, 206–217. [Google Scholar] [CrossRef]
- Sun, X.; Wang, F.; Xu, J. Dynamics and realization of a feedback-controlled nonlinear isolator with variable time delay. J. Vib. Acoust.-Trans. ASME 2019, 141, 021005. [Google Scholar] [CrossRef]
- Pu, H.; Yuan, S.; Peng, Y.; Meng, K.; Zhao, J.; Xie, R.; Chen, X. Multi-layer electromagnetic spring with tunable negative stiffness for semi-active vibration isolation. Mech. Syst. Signal Process. 2019, 121, 942–960. [Google Scholar] [CrossRef]
- Zhang, F.; Shao, S.; Tian, Z.; Xu, M.; Xie, S. Active-passive hybrid vibration isolation with magnetic negative stiffness isolator based on Maxwell normal stress. Mech. Syst. Signal Process. 2019, 123, 244–263. [Google Scholar] [CrossRef]
- Sun, X.; Xu, J.; Jing, X.; Cheng, L. Beneficial performance of a quasi-zero-stiffness vibration isolator with time-delayed active control. Int. J. Mech. Sci. 2014, 82, 32–40. [Google Scholar] [CrossRef]
- Liu, S.; Feng, L.; Zhao, D.; Shi, X.; Zhang, Y.; Jiang, J.; Chen, L. A real-time controllable electromagnetic vibration isolator based on magnetorheological elastomer with quasi-zero stiffness characteristic. Smart Mater. Struct. 2019, 28, 085037. [Google Scholar] [CrossRef]
- Xu, J.; Sun, X. A multi-directional vibration isolator based on Quasi-Zero-Stiffness structure and time-delayed active control. Int. J. Mech. Sci. 2015, 100, 126–135. [Google Scholar] [CrossRef]
- Wang, K.; Zhou, J.; Ouyang, H.; Cheng, L.; Xu, D. A semi-active metamaterial beam with electromagnetic quasi-zero-stiffness resonators for ultralow-frequency band gap tuning. Int. J. Mech. Sci. 2020, 176, 105548. [Google Scholar] [CrossRef]
- Wang, Y.; Li, S.; Cheng, C.; Su, Y. Adaptive control of a vehicle-seat-human coupled model using quasi-zero-stiffness vibration isolator as seat suspension. J. Mech. Sci. Technol. 2018, 32, 2973–2985. [Google Scholar] [CrossRef]
- Ahn, K.K. Active pneumatic vibration isolation system using negative stiffness structures for a vehicle seat. J. Sound Vib. 2014, 333, 1245–1268. [Google Scholar] [CrossRef]
- Le, T.D.; Ahn, K.K. Fuzzy sliding mode controller of a pneumatic active isolating system using negative stiffness structure. J. Mech. Sci. Technol. 2012, 26, 3873–3884. [Google Scholar] [CrossRef]
- Zhao, Y.; Cui, J.; Zhao, J.; Bian, X.; Zou, L. Improving Low Frequency Isolation Performance of Optical Platforms Using Electromagnetic Active-Negative-Stiffness Method. Appl. Sci. 2020, 10, 7342. [Google Scholar] [CrossRef]
- Pan, H.; Jing, X.; Sun, W.; Gao, H. A bioinspired dynamics-based adaptive tracking control for nonlinear suspension systems. IEEE Trans. Control Syst. Technol. 2017, 26, 903–914. [Google Scholar] [CrossRef]
- Kim, M.H.; Kim, H.Y.; Kim, H.C.; Ahn, D.; Gweon, D.G. Design and control of a 6-DOF active vibration isolation system using a Halbach magnet array. IEEE-ASME Trans. Mechatron. 2016, 21, 2185–2196. [Google Scholar] [CrossRef]
- Chang, Y.; Zhou, J.; Wang, K.; Xu, D. Theoretical and experimental investigations on semi-active quasi-zero-stiffness dynamic vibration absorber. Int. J. Mech. Sci. 2022, 214, 106892. [Google Scholar] [CrossRef]
- Zhao, Y.; Meng, G. A bio-inspired semi-active vibration isolator with variable-stiffness dielectric elastomer: Design and modeling. J. Sound Vib. 2020, 485, 115592. [Google Scholar] [CrossRef]
- Palomares, E.; Nieto, A.J.; Morales, A.L.; Chicharro, J.M.; Pintado, P. Numerical and experimental analysis of a vibration isolator equipped with a negative stiffness system. J. Sound Vib. 2018, 414, 31–42. [Google Scholar] [CrossRef]
- Alabuzhev, P.M. Vibration Protection and Measuring Systems with Quasi-Zero Stiffness; CRC Press: Boca Raton, FL, USA, 1989; ISBN 0-89116-811-7. [Google Scholar]
- Tobias, S.A. Design of small isolator units for the suppression of low-frequency vibration. Proc. Inst. Mech. Eng. Part C-J. Eng. Mech. Eng. Sci. 1959, 1, 280–292. [Google Scholar] [CrossRef]
- Ravindra, B.; Mallik, A.K. Performance of non-linear vibration isolators under harmonic excitation. J. Sound Vib. 1994, 170, 325–337. [Google Scholar] [CrossRef]
- Brennan, M.J.; Kovacic, I.; Carrella, A.; Waters, T.P. On the jump-up and jump-down frequencies of the Duffing oscillator. J. Sound Vib. 2008, 318, 1250–1261. [Google Scholar] [CrossRef]
- Jiang, G.; Jing, X.; Guo, Y. A novel bio-inspired multi-joint anti-vibration structure and its nonlinear HSLDS properties. Mech. Syst. Signal Process. 2020, 138, 106552. [Google Scholar] [CrossRef]
- Saif, M.T.A. On a tunable bistable MEMS-theory and experiment. J. Microelectromech. Syst. 2000, 9, 157–170. [Google Scholar] [CrossRef]
- Sherman, C.H.; Butler, J.L. Transducers and Arrays for Underwater Sound; Springer: New York, NY, USA, 2007; ISBN 978-0-387-32940-6. [Google Scholar]
- Kashdan, L.B. Evaluation of Negative Stiffness Elements for Enhanced Material Damping Capacity. Ph.D. Thesis, The University of Texas at Austin, Austin, TX, USA, 2010. Available online: http://hdl.handle.net/2152/ETD-UT-2010-05-1301 (accessed on 1 May 2010).
- Gao, X.; Chen, Q. Nonlinear analysis, design and vibration isolation for a bilinear system with time-delayed cubic velocity feedback. J. Sound Vib. 2014, 333, 1562–1576. [Google Scholar] [CrossRef]
- Liu, C.; Jing, X.; Daley, S.; Li, F. Recent advances in micro-vibration isolation. Mech. Syst. Signal Process. 2015, 56, 55–80. [Google Scholar] [CrossRef]
- Ledezma-Ramírez, D.F.; Tapia-González, P.E.; Ferguson, N.; Brennan, M.; Tang, B. Recent advances in shock vibration isolation: An overview and future possibilities. Appl. Mech. Rev. 2019, 71, 060802. [Google Scholar] [CrossRef]
- Zhao, G.; Ding, B.; Watchi, J.; Deraemaeker, A.; Collette, C. Experimental study on active seismic isolation using interferometric inertial sensors. Mech. Syst. Signal Process. 2020, 145, 106959. [Google Scholar] [CrossRef]



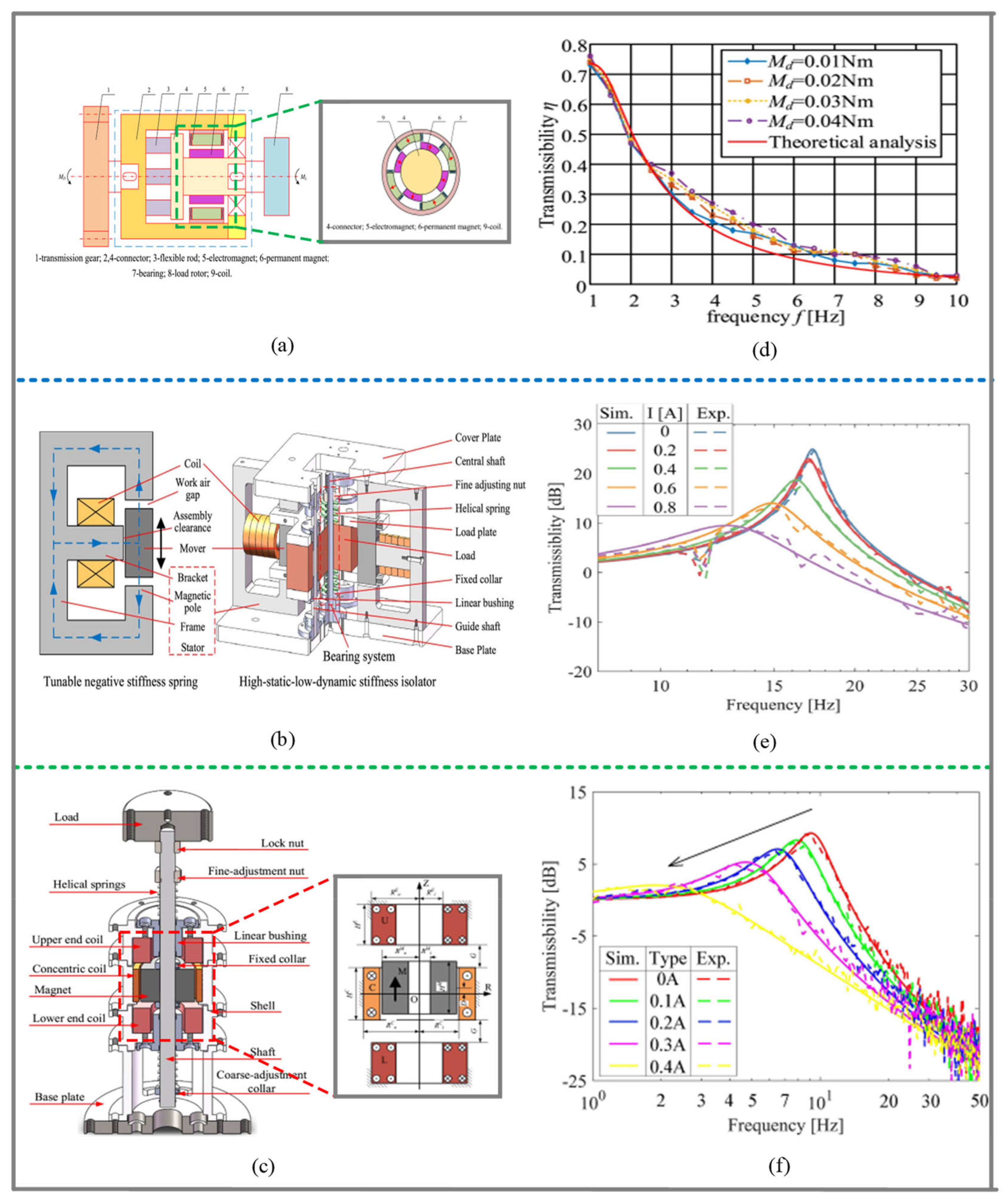
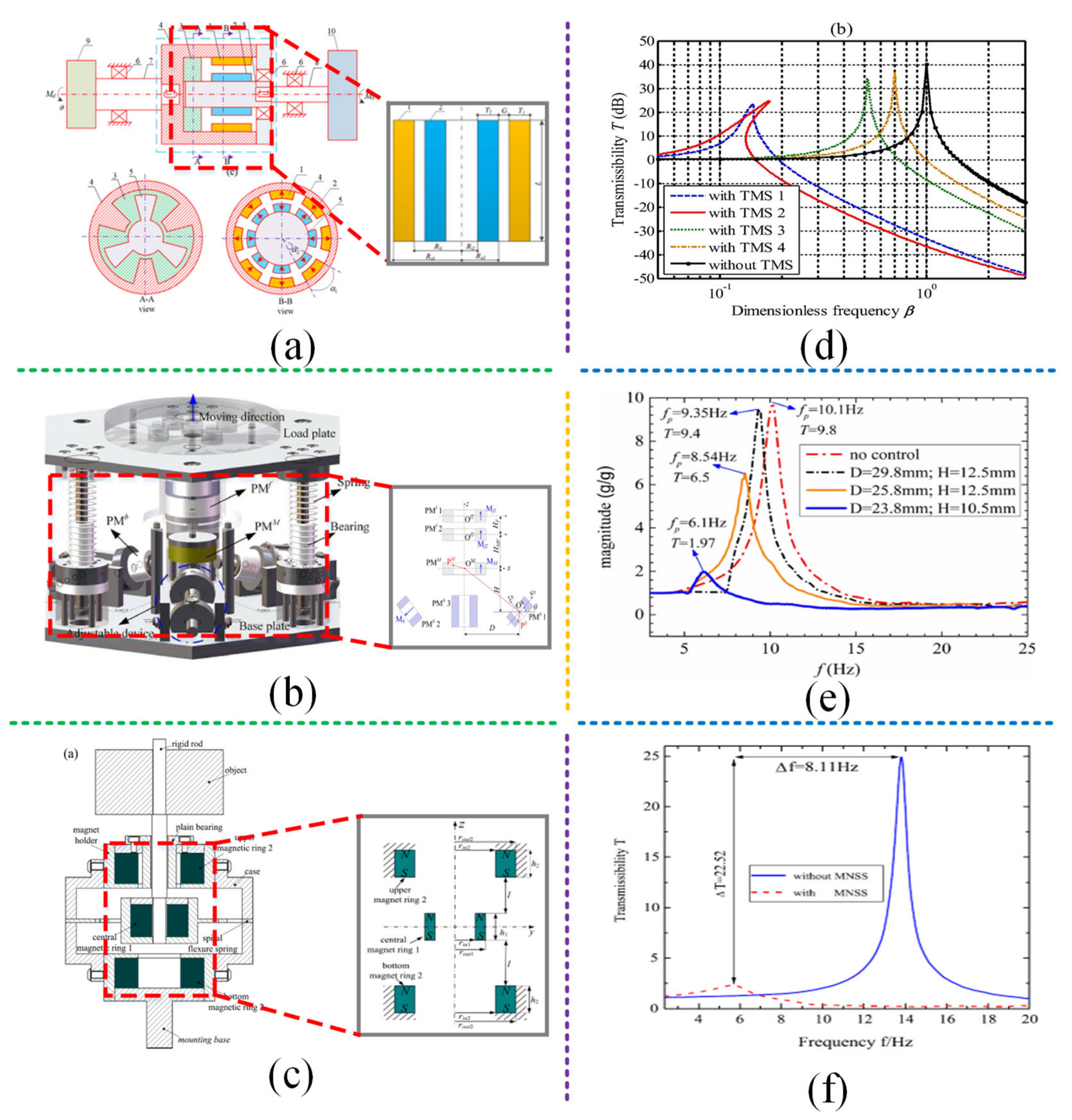
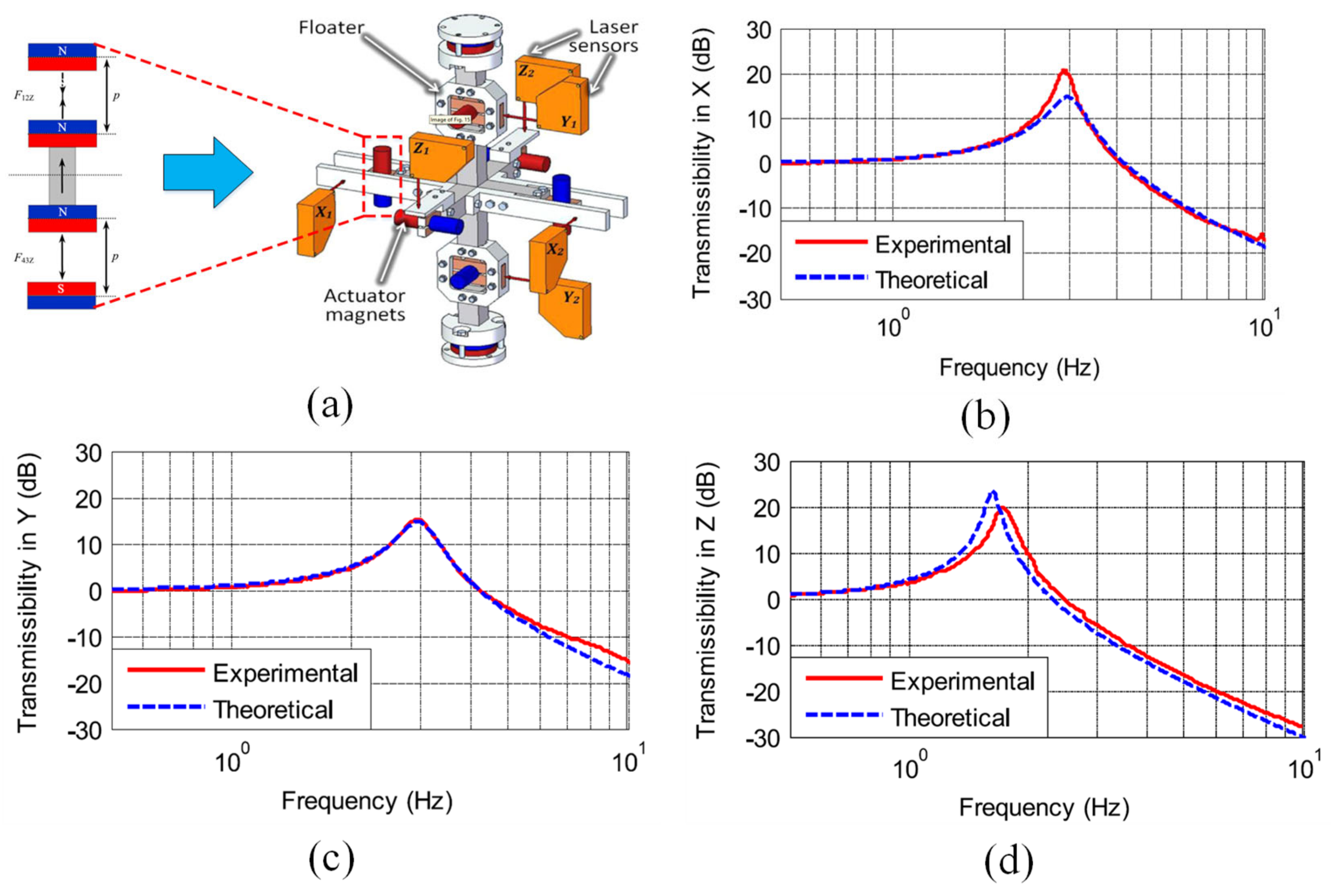

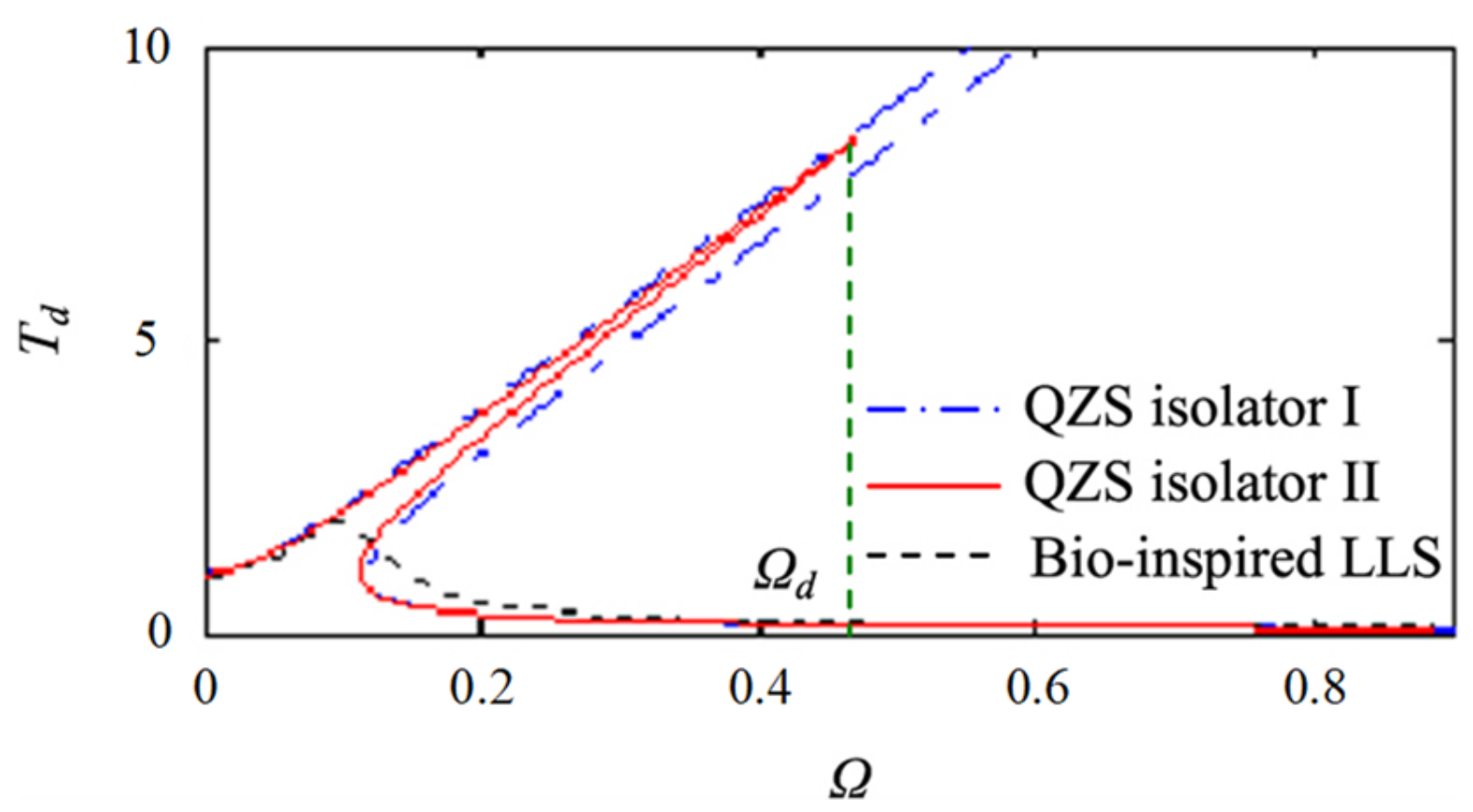

| Realization Method | Application Scenario | Source of Negative Stiffness | Reference | Vibration Isolation Performance | |
|---|---|---|---|---|---|
| Passive QZS | Resonance Frequency | Isolated Frequency Band | |||
| Low frequency vibration | Mechanical spring | [8,9,10] | ~1.0 Hz (test) | >2 Hz | |
| [21] | ~2.0 Hz (test) | >2.3 Hz | |||
| [30] | ~2.3 Hz (test) | >2.5 Hz | |||
| Prebuckled beam | [39] | 1.42 Hz (test) | >2.05 Hz | ||
| Geometrically nonlinear structure | [49] | 1.2 Hz (test) | >1.7 Hz | ||
| [50] | ~1.7 Hz (test) | >2.36 Hz | |||
| Magnetic structure | [70,71] | 5.53 Hz (test) | >5.7 Hz | ||
| [66] | 2.34 Hz (test) | >3.0 Hz | |||
| [57,58] | 5.8 Hz (test) | >7.8 Hz | |||
| Composite structure | [118] | 0.1–0.15 Hz (dimensionless) | — | ||
| [103] | 0.03–1.285 rad/s | >1.285 rad/s | |||
| Active QZS | Low frequency or ultra-low frequency vibration | Semi-active control | [122] | 2.6–8.8 Hz | >(3.8–12.6 Hz) |
| Active control | [125] | ~9 Hz (test) | >11.7 Hz | ||
| [131] | 1.37 Hz (test) | — | |||
| Design Variation | Prototype | Main Features | Performance Standard | Performance |
|---|---|---|---|---|
| Different cam structures [49,50,51,52,53] 1 | 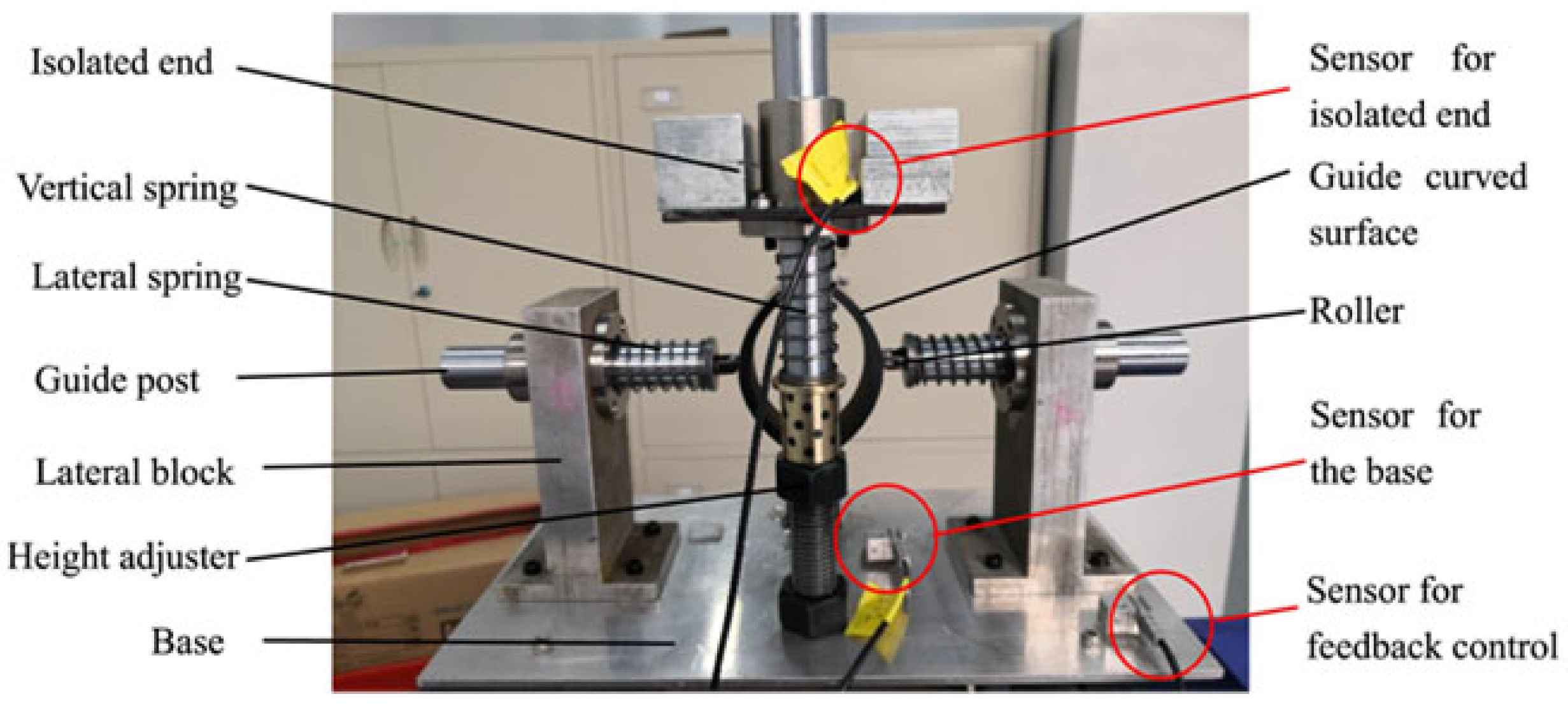 | (1) The curved surface of the guide rail support of the roller is designed based on the expected target force-deflection curve; (2) The designed carrying capacity of the isolator is 6.15 kg; (3) The negative-stiffness mechanism is composed of the guide rail support, transverse spring, and roller; (4) Two vertical springs are used as the positive-stiffness mechanism, which are arranged in parallel with the guide column. | Natural frequency, vibration transmissibility | The natural frequency is 1.2 Hz and the maximum transmissibility is 2.3; Starting from 1.7 Hz, the vibration transmissibility is less than 1, which is 2.3 Hz lower than that of the linear system. |
 | (1) A nonlinear structure with a specially designed cam profile is used; (2) The theoretical carrying capacity of the isolator is 97 N; (3) The negative-stiffness mechanism comprises the cam, two rollers, and two horizontal linear springs; (4) The positive stiffness is provided by a vertical spring and is arranged in the spring sleeve. | Time domain acceleration, amplitude response, displacement transmissibility | There is no obvious acceleration peak during frequency sweep; the displacement transmissibility of the QZS vibration isolator and the linear system is less than 1 starting from 2.36 Hz and 3.20 Hz, respectively; the displacement transmissibility at resonance frequency is 1.99, which is 20.93 lower than that of the linear system. | |
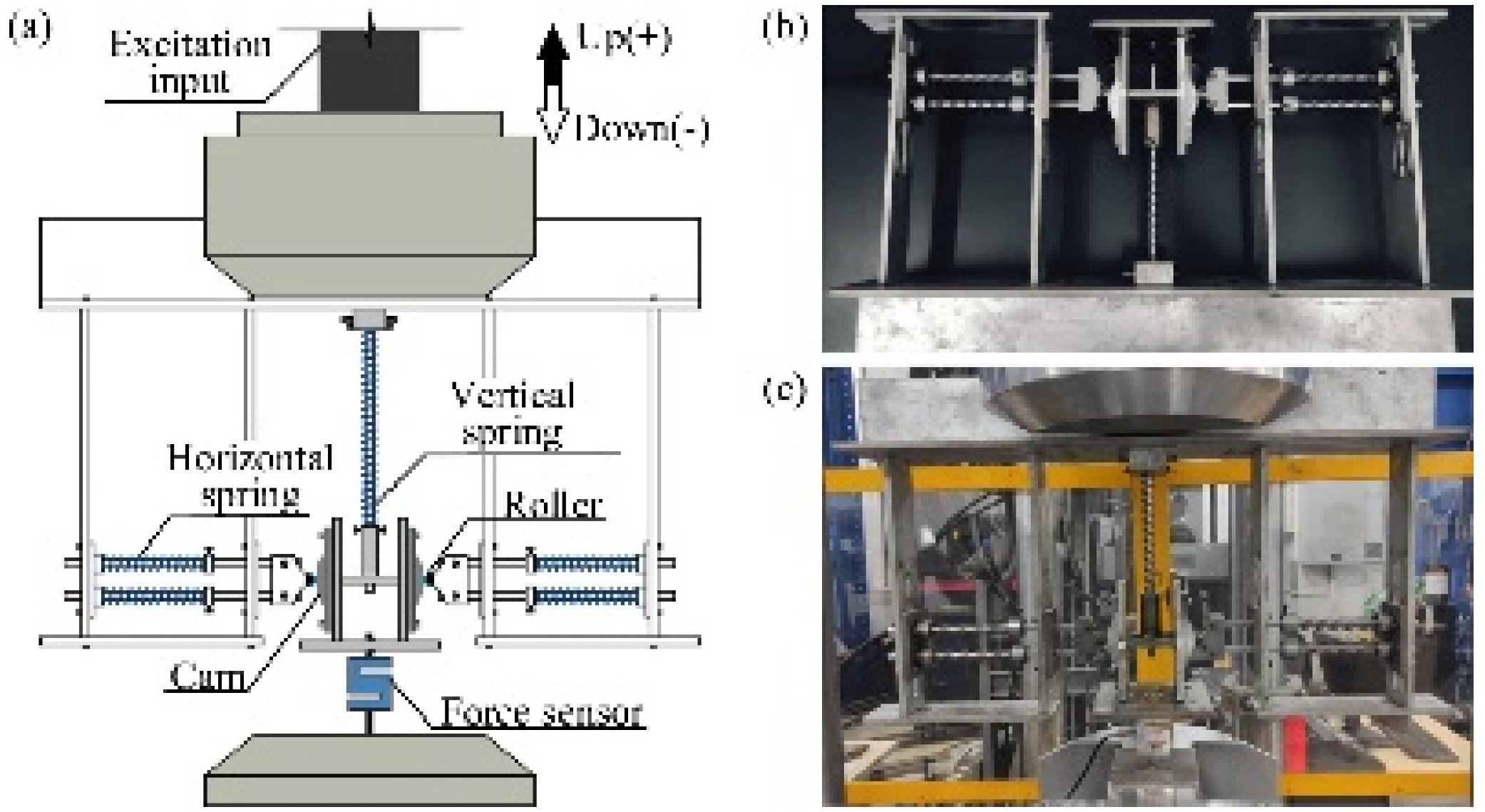 | (1) The optimal design of the parabolic-cam-roller is used; (2) The mass of isolator is 4.89 kg; (3) The negative-stiffness mechanism is composed of a cam with a parabolic profile, a roller, a pair of horizontal springs and a pair of horizontal sliding rods; (4) The positive-stiffness structure consists of a vertical spring. | Natural frequency, displacement transmissibility | The initial isolation frequencies of the isolator is 2.5 Hz; the displacement peak transmissibility of the QZS isolator is 1.014 at 2 Hz. | |
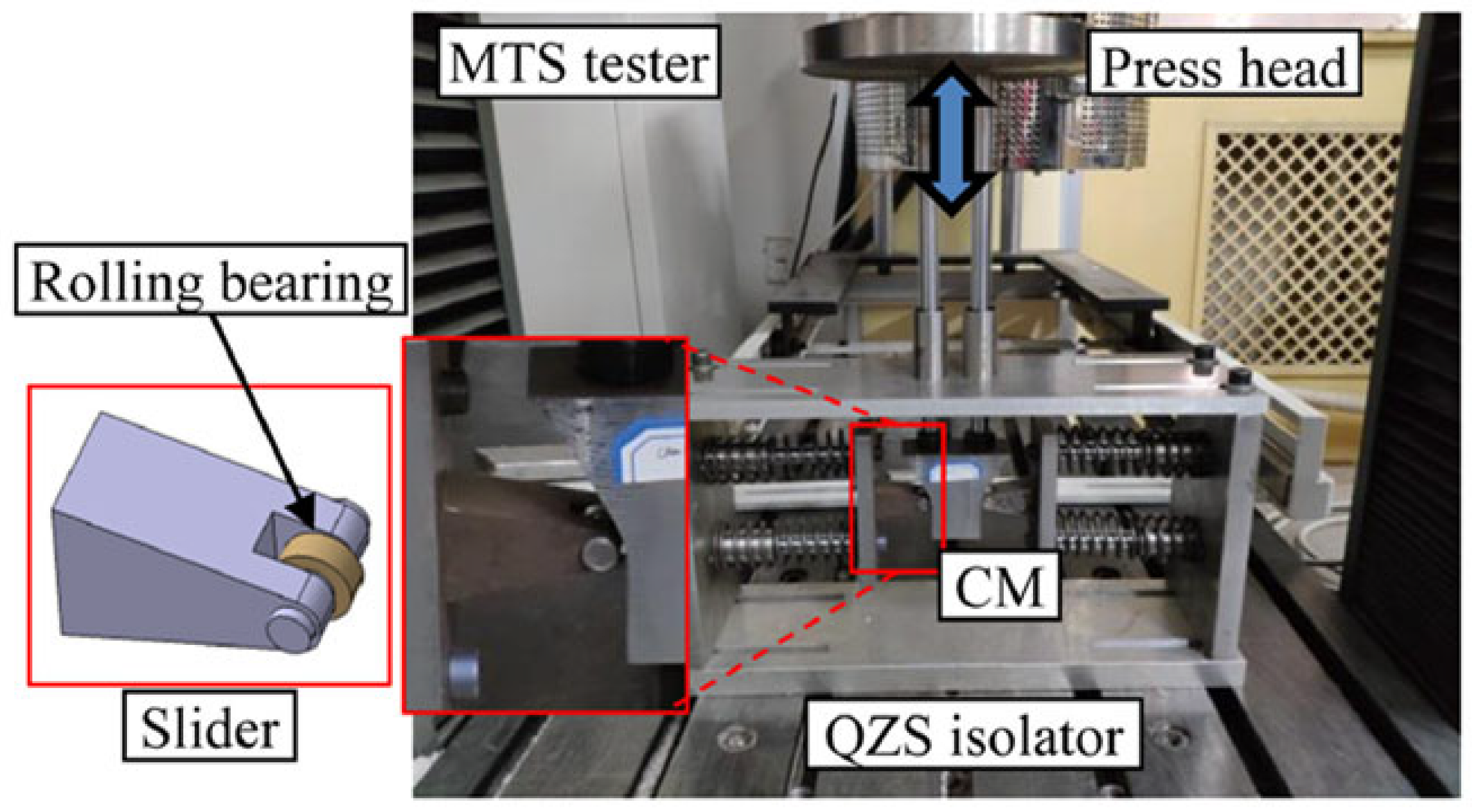 | (1) An integrated cam with a user-defined profile is used; (2) The QZS isolator is not realized by the parallel arrangement of positive- and negative-stiffness mechanisms, but through a specially designed cam mechanism; (3) The cam mechanism is composed of one cam, two rollers, and two lateral springs; (4) The vertical support force is produced during the lateral spring-supported roller pressing the cam surface. | Resonance frequency, force transmissibility, vibration transmissibility | At different excitation levels, the resonance frequency of the vibration isolator is 2.5 Hz~6.0 Hz; compared with the linear system, the maximum force transmissibility and vibration transmissibility of the proposed isolator at the resonance frequency can be reduced by about 20 dB, showing better low-frequency vibration isolation performance. | |
| Different number of cams [54] 2 |  | (1) The multi-cam nonlinear mechanism is used, and the stiffness jump phenomena at multiple-cam positions are eliminated; (2) The negative-stiffness mechanism is composed of the multiple cams and rollers, which can adapt to multiple levels of load. | Natural frequency, vibration transmissibility | When the load is 5.79 kg, the natural frequency of the isolator is 3.0 Hz, which is 1.6 Hz lower than that of the linear system; the vibration starts to be attenuated from 3.6 Hz, and the vibration transmissibility is less than 1, showing that it can provide effective vibration isolation in the low-frequency range. |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, Z.; Zhou, R.; Yang, Q. Recent Advances in Quasi-Zero Stiffness Vibration Isolation Systems: An Overview and Future Possibilities. Machines 2022, 10, 813. https://doi.org/10.3390/machines10090813
Ma Z, Zhou R, Yang Q. Recent Advances in Quasi-Zero Stiffness Vibration Isolation Systems: An Overview and Future Possibilities. Machines. 2022; 10(9):813. https://doi.org/10.3390/machines10090813
Chicago/Turabian StyleMa, Zhaozhao, Ruiping Zhou, and Qingchao Yang. 2022. "Recent Advances in Quasi-Zero Stiffness Vibration Isolation Systems: An Overview and Future Possibilities" Machines 10, no. 9: 813. https://doi.org/10.3390/machines10090813
APA StyleMa, Z., Zhou, R., & Yang, Q. (2022). Recent Advances in Quasi-Zero Stiffness Vibration Isolation Systems: An Overview and Future Possibilities. Machines, 10(9), 813. https://doi.org/10.3390/machines10090813






