Design and Mechanical Behavior of a Custom Adapter for Dimensional Stone Mining

Abstract

The paper deals with the development of a dimensional rock sawing machine to optimize the production of small-volume dimensional stone products in limestone quarries by re-thinking the use of production and transport equipment. A low-volume mining production and transportation adapter to be developed for both production and transport can reduce the number of mining machines to be used in the mine. After cutting around the block stone, the rock material can be moved with the help of the machine. Cutting tests were performed to determine the winning properties of the limestone. The first objective of the paper was to calculate the cutting forces and cutting powers and eliminate the failure possibilities and vibrations. In this study, the eigenvalue extraction used to calculate the natural frequencies and mode shapes was based on the Lanczos iteration methods using the Abaqus software to identify any potentially dangerous frequencies. By taking the load cases from modelling of the extraction of limestone and the transport of block stones, the deformation and strength properties of the adapter were also examined using FE analysis for stress/displacement analysis. Drawing the conclusion from finite element analysis, the construction of the adapter can be developed and checked. The results can be used with great confidence, so the main dimensions of the concept serve as the basis for the dimensions of the adapter.

1. Introduction

Limestone has been a popular building material for millennia. These stones were easy to mine and transport. The Celts and the Romans used this stone as well, but it became a popular building material from the 18th century. These structures are under the protection of monuments, so they can only be renovated with the original building material.

There is worldwide growing demand for the natural stone industry. Related technologies seek to find solutions to mining challenges. There are low production rates and high production costs. The economic costs and technological developments have led stone product companies to innovate new working methods and machinery, enabling increased cutting rates and lower production costs. Studies conducted efficiency analysis and examined the block production and cutting performance of cutting machines in block production [1,2]. Optimizing the mining production of small-volume dimensional stone products is also a re-thinking of the use of production and transport equipment in one. The development of the mining machine was planned to reduce the expenses of running mining businesses, which include mining operations and costs of maintenance. Research and strategies to improve the mining cycle in stone exploitation and to reduce its impact have been reported. The paper [3] by Vagnon et al. reported that advances in the assessment of characteristics and environmental impacts of natural building stones are the foundation of sustainable exploitation processes. Their study showed how to improve the quality of the exploited material through improvements in cutting technologies with the natural and induced stress states through on-site measurements and numerical modelling.

There are several methods of dimensional stone production: block production with large borehole blasting technology; cleavage of stone blocks using chemical swelling energy; use of mechanical, hydraulic and combined rock setting wedges placed in small-diameter holes; creating blocks by drilling; and rock sawing. Since the gentlest production method is rock sawing, to reduce the number of machines, we planned to solve the production challenge with a design that is capable of winning and transport. Chainsaws are used for vertical and horizontal cuts in surface and underground quarrying in low- to medium-abrasive and soft-to-medium rocks. The use of chainsaws meets the prerequisites for quarrying dimensional stones.

The determination of the mined rock environment is essential for the design of the mining adapter to be developed. For determining the cuttability of the limestones, their physical and mechanical properties can be tested with a linear cutting test rig using chisel-type cutting tools with different sideways angles. The maximum tool forces and relationships between the cutting performance of chisel tools can be determined depending on the mechanical properties of the limestones to improve the chain and eliminate the failure possibilities and vibrations. Numerous studies and papers have been conducted in this field to improve the performance of the tools, reduce the tool wear of chain cutting and increase energy efficiency. Copur [4] recommended an evaluation of the operating depth of cut and feed rate in the calculation of cutting forces. As regards the estimation of the dependence of cutting forces on the process parameter, linear cutting tests are basically used. Copur et al. [5] proposed two empirical models for the prediction of the areal net cutting rate of the chain saw machines. The first model is based on the chain saw penetration index and uses the uniaxial compressive strength of the stone, weight of the saw machine, and useful cutting depth. The other model applies the results of linear cutting experiments performed in the unrelieved cutting mode with a standard chisel tool and uses specific energy as a predictor parameter. Rostamsowlat et al. [6] investigated the influence of the rake angle on the magnitude of the intrinsic specific energy and the inclination of the force acting on the cutting face of a polycrystalline diamond compact (PDC) sharp cutter while tracing a groove on the surfaces of limestone and sandstone samples. The authors confirmed that the intrinsic specific energy increases steadily with the back rake angle and is very well correlated with the rock uniaxial compressive strength. Romoli [7] investigated the influence of rake angle by cutting force monitoring of chain saw machines.

In paper [8], numerical simulation possibilities were employed for the dynamics of cutter rock interaction between the cutting tool and the rock, rock failure, and fragmentation considering PDC cutters with a non-linear finite element method using the Ansys software AUTODYN-2D. Di Giovanni et al. [9] presented a study into the phenomenon of the tool wear of the chain cutting machines used for the exploitation of marble and concluded that when the critical moisture content is between 4% and 9% in the case studied, the added conditioning agents allow for adequate protection against tool wear action.

Korman et al. performed laboratory tests with specific chain saw tool holders and cutting tools to experiment with rock cuttability on a linear cutting machine for different values of chain and cart speed in [10]. The paper [11] deals with increasing the performance of current chain saw machines in terms of cutting rate and reducing tool wear, and examines the effect of changing the original tool lacing for shallow depth cutting.

The prototype tool to be developed for production and transport can reduce the number of mining machines used in a limestone quarry with a specific stone grade. This study addresses development issues with the prototype adapter for the given limestone grade, presenting the simplification assumptions used in important modelling questions. After identifying the need for the equipment and selecting a new adapter design, a simplified FE model was created for strength analysis and modal analysis. In this paper, the FE analysis was employed for the development and construction of the new adapter, and was carried out to identify any potentially dangerous frequencies of the structure. The purpose of the analysis was to design and choose parts for the task of limestone mining with an adapter.

2. The Mining Machine

The basic mechanics of the mining prototype are those of a construction loadall (Figure 1). Due to its high load capacity and unique design, the machine is used for many different works in many areas. It has an extremely maneuverable drive, making the machine easy to operate in confined spaces.

The vehicle has two four-wheel steering modes for working in tight spaces, and the crab steer for maneuvering close to walls. Adaptive load control automatically controls hydraulic operation to help maintain the longitudinal stability of the construction loadall. This loadall uses up to 7% less fuel than the previous ones, thereby further strengthening sustainability in the quarry. It makes the machine more eco-friendly than other machines. Its economical engine can be adapted to operate with lower-grade diesel oils, making the machines useful across different working areas.

The machine has a high-performance hydraulic system, which provides the main pump capacity of 90 + 72 L/min and a system operating pressure of 260 bar to perform its tasks. Two front stabilizers are fitted for the increased stability and lift performance of the machine.

3. Cutting Forces and Cutting Power

Determining cutting forces and cutting power is an important task during the design. The machinability properties of several types of mined rock were investigated.

3.1. Cutting Forces from Rock Cutting Test

Rock cutting tests were carried out in the laboratory. Winning experiments were performed on large samples that were brought from the mine. With these measurements, we were able to obtain real cutting parameters that could be used for development. The measurement data of cutting were collected and registered by a computer-aided measurement system. This three-component force measuring table is capable of recording the cutting and the lateral and pressing force at the same time. Figure 2 shows the basic construction of the cutting equipment installed on a longitudinal planer. In addition, an earlier study was published to show details of experimental procedures and cutting mechanisms for various materials. It characterizes the specific force and force ratio values that were initially used in the development of the cutting teeth [12]. Alternative options for stone cutting experiments were cutting disks, which Paper [13] provided with a practical anticipation model for the examination of the most important factors influencing the performance of cutting disks, i.e., the effect of cooling lubricant fluid, and the consumed electrical current.

Cuttability of the limestones was, therefore, tested with a linear cutting test rig using cutting tools. The maximum cutting forces and relationships between the cutting performance of chisel tools were determined depending on the material properties of the limestones.

On the limestone samples, 30 measurement cycles were recorded and processed. Figure 3 shows average cutting forces for each measurement cycle. The testing parameters were the cutting speed ${\mathrm{v}}_{\mathrm{cutting}}=1.818$ mm/s and the average cutting area ${\mathrm{A}}_{\mathrm{cutting}}=1.787$ mm². If we examine the numerical value of the specific cutting forces characteristic of rock cutting obtained from laboratory experiments, it can be seen that the values obtained from the measurements were within a relatively narrow range. This range was characterized by lower and upper values of $16 \mathrm{N}/{\mathrm{mm}}^2$ and $24 \mathrm{N}/{\mathrm{mm}}^2$, respectively.

3.2. Cutting Power and Power Requirements

Building on the experiences obtained in our earlier works on rock cutting, the specific cutting force was determined [12]. The used chain speeds were ${\mathrm{v}}_{\mathrm{chain}}=0.4, 0.9, 1.2$ and 2.1 m/s, which were the values specified by the chainsaw manufacturer [14]. The tooth pitch was ${\mathrm{l}}_{\mathrm{pitch}}=0.1 \mathrm{m}$. The cutting length of the adapter was ${\mathrm{l}}_{\mathrm{cutting}}=1.2 \mathrm{m}$, which was determined by the dimensions of the cutting board. Three different values were employed for the calculated chip area ${\mathrm{A}}_{\mathrm{chip}}$, namely $4$, $6$ and $8$ ${\mathrm{mm}}^2$.

The number of cutting teeth at the same time was calculated as follows:

$${\mathrm{N}}_{\mathrm{c}}=\frac{{\mathrm{l}}_{\mathrm{cutting}}}{{\mathrm{l}}_{\mathrm{pitch}}}$$

(1)

The overall cutting force is

$${\mathrm{F}}_{\mathrm{o}}={\mathrm{N}}_{\mathrm{c}}·{\mathrm{A}}_{\mathrm{chip}}·{\mathrm{F}}_{\mathrm{cutting}}$$

(2)

The measured and calculated maximum specific cutting force is a value of ${\mathrm{F}}_{\mathrm{cutting}}=24 \mathrm{N}/{\mathrm{mm}}^2$, with which the cutting power is calculated. Thereby, the cutting power is calculated as

$$\mathrm{P}={\mathrm{F}}_{\mathrm{o}}·{\mathrm{v}}_{\mathrm{chain}}$$

(3)

As the friction of the chain and the efficiency of the machine were not taken into account, based on our many years of practical experience, it was worth increasing the power by 25% to ensure safe operation as the target for the necessary power requirement.

Table 1. Cutting forces and cutting powers. The chip area is a constant ${\mathrm{A}}_{\mathrm{chip}}=4{ \mathrm{mm}}^2$.

Chain Speed [m/s]	Cutting Power [W]	Power Requirement [W]
0.4	460.8	576
0.9	1036.8	1296
1.2	1382.4	1728
2.1	2419.2	3024

,

Table 2. Cutting forces and cutting powers. The chip area is a constant ${\mathrm{A}}_{\mathrm{chip}}=6{ \mathrm{mm}}^2$.

Chain Speed [m/s]	Cutting Power [W]	Power Requirement [W]
0.4	691.2	864
0.9	1555.2	1944
1.2	2073.6	2592
2.1	3628.8	4536

and

Table 3. Cutting forces and cutting powers. The chip area is a constant ${\mathrm{A}}_{\mathrm{chip}}=8{ \mathrm{mm}}^2$.

Chain Speed [m/s]	Cutting Power [W]	Power Requirement [W]
0.4	921.6	1152
0.9	2073.6	2592
1.2	2764.8	3456
2.1	4838.4	6048

show the calculated cutting forces and cutting powers for different chain speeds.

The results for the increased energy demand, shown in Table 1, Table 2 and Table 3, ranged from 576 to 6048 W. The prototype machine could reliably provide these values.

4. Analysis of the Mechanical Behavior of the Adapter

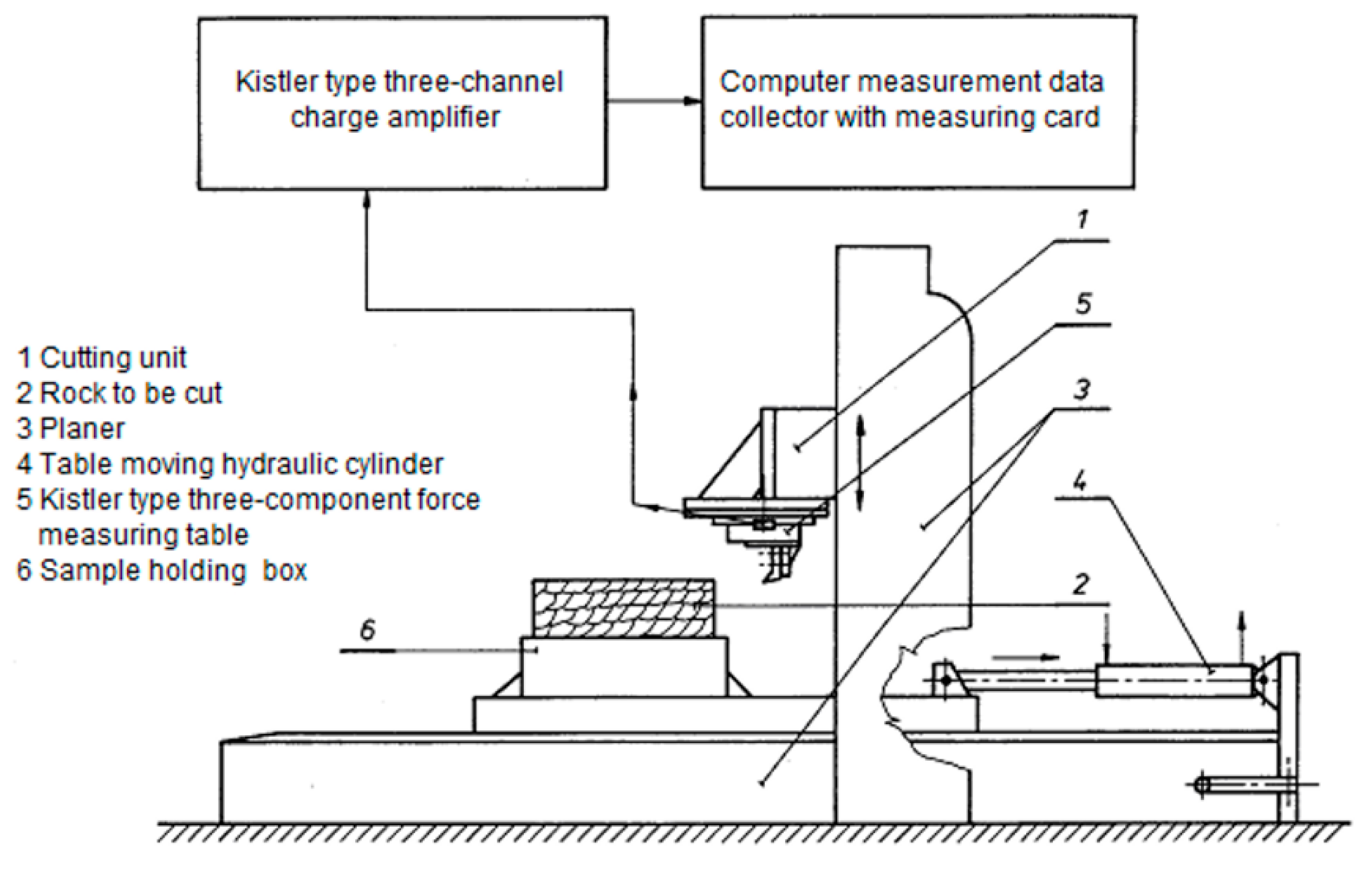
The rock sawing process results in chip formation while also creating vibrations that are transmitted to the mining adapter. The literature revealed that large chain saw machines are not used for cutting very hard stones and highly fractured deposits. In order to reduce the vibration caused by the chain, the total forces acting on the adapter must be balanced to minimize machine vibrations. The key to achieving this goal is determining the cutting force (see Section 3) and picking the proper rock chainsaw sequence for the given limestone rock quality.

4.1. Assumptions

There are four wheels in the developed chain saw adapter—one driven and freewheel sprocket and two pulleys—that operate at constant or variable speed conditions [15]. Based on Mellor [16], this part of the machine can be analyzed kinematically by investigating the working principles and design parameters of continuous belt type machines. To describe the kinematic model, a few parameters have to be defined, which leads to a complicated dynamic problem. Dynamic modelling of roller chain drives based on Hamilton’s principle, which can also be performed for chain saws based on [17], proved that chain drives must contain compliant components.

The magnitude of the chain force differs along the chain as the force from the hydraulic motor torque is dissipated in spans. Thereby, the chain tension is highest when the chain enters the drive sprocket and lowest when it leaves the drive sprocket. The applied chain derived from trade tolerated such conditions well, so that the effect is commonly negligible during model development. The adverse effects of high chain tension can be eliminated by correct installation of the chain and, therefore, were not dealt with separately here.

In contrast to the commonly used stone chain saw machines, the cutter table of the concept design is a rectangular plate with nearly equal sides, which is also a proper design from a vibration point-of-view. Finite element (FE) analysis is used to investigate the natural frequencies and mode shapes of the adapter in order to identify any potentially dangerous frequencies and eliminate failure possibilities [18]. Assuming a conceptual model of the new mining adapter design that, after consideration, can be operated with two lower hydraulic cylinders for a given stroke length and two upper extendable steel rollered booms, a simplified FE model was created for strength analysis and modal analysis. Thus, the structure of the adapter can be treated as a solid model with simplified geometry, where the forced vibrations associated with the normal operating speeds and other dynamic effects are neglected.

The type of prescribed displacement boundary conditions, when the adapter is not cutting, are fully built-in, so that in the FE model, zero displacements are given at the free ends of beams (assuming that the beam’s ends are connected to a totally rigid connector). This model simplification is based on the fact that two front stabilizers are installed to increase the stability of the machine. However, when cutting limestone, the chain is located in a slot cut by it. So, the front part of the cutting table should be considered fixed in the vertical direction, and the computed natural frequencies will be higher. The natural frequencies of the concept design, estimated by FE modal analysis, provide an opportunity to give sufficient stability against the low frequencies associated with the normal operating speed of cutting with the final version. This fact needs to be confirmed by a modal analysis of the structure. As the chain is guided along its entire length by the driving slot, any transverse moment of the chain may cause an impact.

The material is assumed to be isotropic elastic, for which Young’s modulus of $\mathrm{E} =2.1\times {10}^5 \mathrm{MPa}$, Poisson’s ratio of $\mathsf{\nu }=0.3$ and density of $\mathsf{\rho } =7.85\times {10}^{-9} \mathrm{t}/{\mathrm{mm}}^3$ are prescribed. The weight of other parts such as the chain, sprockets, pulleys, and motors can be defined as points rigidly connected to the cutting table. In the concept design, for simplicity, the cutting table is a simplified structure without cut-outs and other weight reductions. In fact, the structure weight was overestimated by simplifications, so the other parts were negligible in the FE model of the initial version. Accordingly, the geometrical model of the concept design is meshed into finite elements, which are 10-node quadratic tetrahedron elements (C3D10 in Abaqus) (see details in [19,20]). The approximate global size is specified as 20 mm, as shown in Figure 4. Thus, the sufficiently refined mesh ensures that the results from simulations are adequate.

4.2. Results of FE Analysis

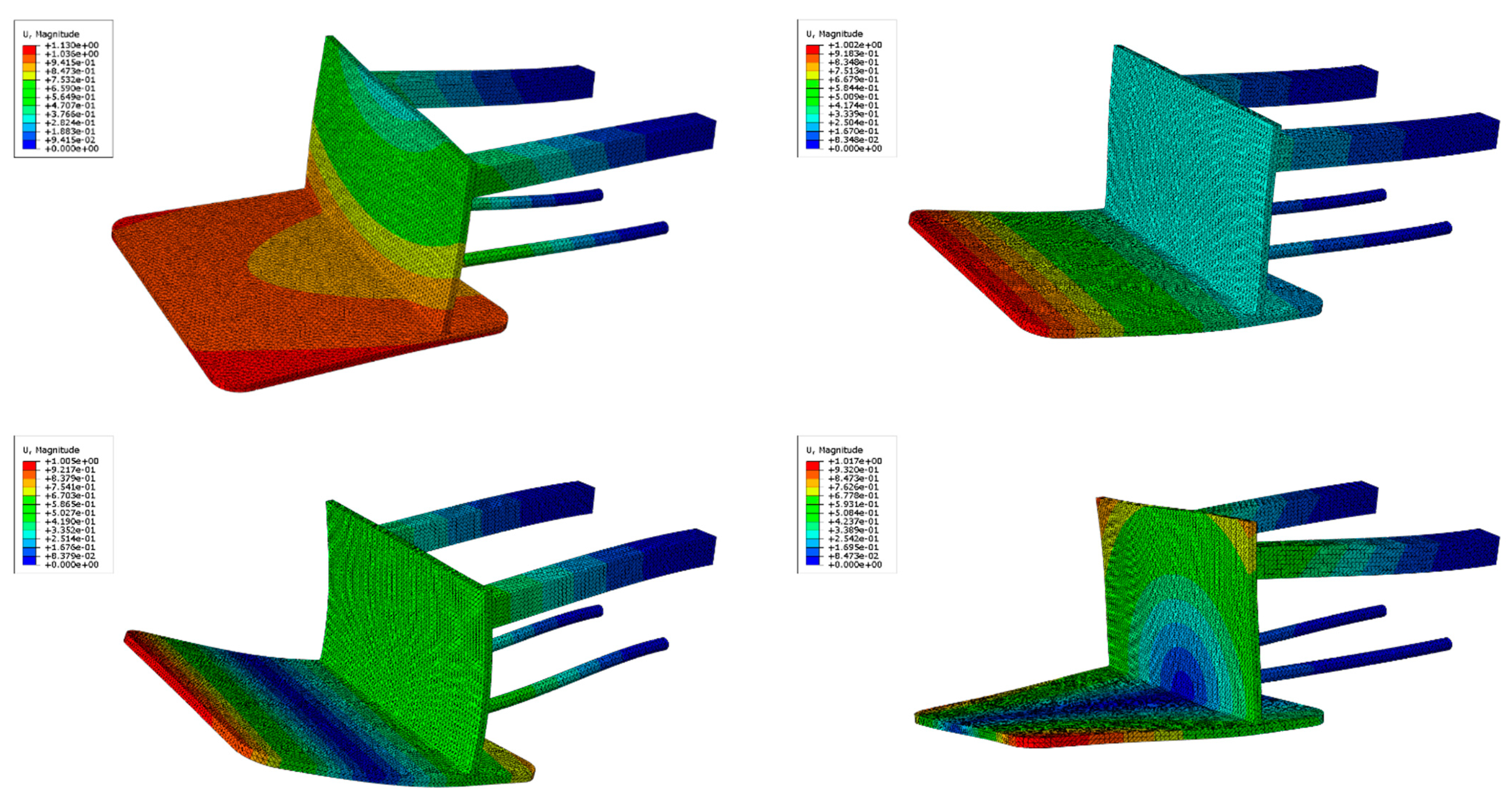
The eigenvalue extraction to calculate the natural frequencies and their mode shapes is based on Lanczos iteration methods described in [19]. The numerical results of the FE analysis for natural frequencies are summarized in

Table 4. Comparison of natural frequencies (Hz) for the adapter according to the upper and lower limits of the extension length (the stroke length is 800 mm) applied for design purposes.

Ext. Length [mm]	Mode Sequence Number
	1.	2.	3.	4.	5.	6.
1310	20.298	21.886	36.663	42.677	71.269	94.831
2110	11.644	12.792	26.161	31.149	59.254	69.386

, which contains the first six natural frequencies from No. 1. to No. 6. to identify the first potentially dangerous frequencies. The first four mode shapes for the extended length of 1310 mm are depicted in Figure 4. The mode shapes for the other cases in Table 4 are not shown separately here.

The goal is to know that the structure of the adapter is properly designed, made from proper materials, and constructed considering loading during its lifespan. The FE analysis provided the natural frequencies and shape modes so that the dynamic behavior can be further investigated, for instance, by mode superposition analysis. The major excitation frequency for various chain speeds can be given and compared with natural frequencies (resonance). According to the concept design of adapter structure, it would be 4 to 21 Hz depending on cutting speed, which can coincide with the first two modes from Table 4. So, the cutting speed must be carefully selected to avoid resonance. In order to guarantee the avoidance of resonance, weight reduction with cut-outs on the cutting table and significant structure stiffening were implemented on the upper booms (see Figure 1). The rock sawing usually starts when the stroke length is 0, so the results for an extended length of 1310 mm are relevant in practice.

The deformation and strength properties of the adapter were also investigated using FE analysis for stress/displacement analysis. The prototype is designed for a 1 m³ dimensional stone that has a density between 2000 and 2600 kg/m³ and shows maximum stress of 103 MPa and maximum displacement of 8 mm, which are small values [15].

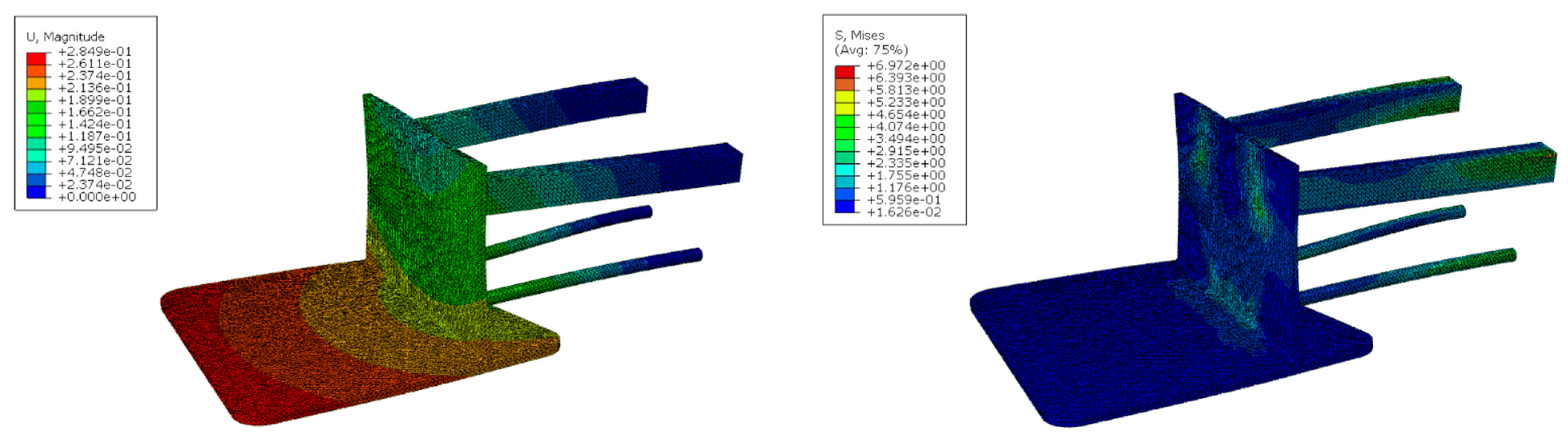
Taking the overall cutting force, given by Equation (2), as the load acting on the edge of the cutting table of the adapter in the process of cutting limestone with a chainsaw, the deformation and strength properties of the adapter were also examined using FE analysis for stress/displacement analysis. It is important to note that the effects of lateral forces are not necessarily significant here with the prototype adapter, since they are too small to induce notable detrimental effects on the adapter components.

By modeling the cutting force from the sawing process as pressure acting on the cutting table’s front edge and with the restraints the same as in Figure 4, no significant displacement effect was found, and the Mises stress in the structure remained small, as shown in

Table 5. Maximum stresses and maximum displacements from the overall cutting forces calculated at the chip surface ${\mathrm{A}}_{\mathrm{chip}}$.

${\mathsf{A}}_{\mathbf{chip}} \left[{\mathbf{mm}}^2\right]$	Overall Cutting Force [N]	Ext. Length [mm]	Max. Stress [MPa]	Max. Displacement [mm]
4	1152	1310	3.48	0.142
		2110	5.61	0.361
6	1728	1310	5.23	0.214
		2110	8.41	0.542
8	2304	1310	6.97	0.285
		2110	7.23	0.723

and Figure 5. During the design process, the maximum displacement is also an important parameter when considering the functionality of the adapter, as its hydraulic cylinders do not tolerate large displacements.

When designing the upper booms (Figure 6), the cross-sectional parameters of the beams applied in the FE model were taken into account, which showed sufficient stiffness in the loading cases considered, as shown in Table 4 and Table 5.

5. Discussion

The results obtained in this study were used to develop a prototype mining adapter (Figure 6 and Figure 7). The small volume dimensional stone mining adapter is suitable for the extraction of block stone, and primarily for the extraction of limestone. The adapter has a rotary cutting table equipped with a chainsaw. The machine is designed in such a way that it is capable of short-distance transportation of the mined rock after the cutting operation.

The rigidity of the structure is provided by the upper extendable steel rollered boom. This has been significantly strengthened to relieve the lower hydraulic cylinders from all kinds of loads during the cutting process, handling and transport, as shown in Figure 6 and Figure 7.

Based on the technology, the adapter is divided into two main units. One is a hydraulic rotating unit. It can be rotated infinitely up to 90 degrees in both directions to perform side cuts at different angles. The other is the chainsaw unit combined with a work consisting of hydraulic cylinders for feeding and rotating. There is a unique structure of the rock chainsaw’s individual elements. The teeth that make up the chain follow the chain in a specific order and form a chain sequence of 7 teeth, which reduces the chip area in this way. The set shown in Figure 8 has been developed specifically for this limestone rock quality. The cutting action of a sequence is repeated by the following sequences, which have the same cutting pattern. By correct tool lacing, special attention should also be paid to vibration aspects and adverse effects of high chain tension, as cutting vibrations increase with a small number of tools [11].

During the development period, further testing of the prototype adapter, which will be carried out in the production workshop and the limestone quarry, is unavoidable. During testing, the static and dynamic consumption of the units in the adapter are studied, even in extreme cases, to determine more accurate technical parameters for the future. Since the hydraulic cylinders are primarily designed for axial forces, an important problem can be the stress on the boom structure of the basic machine, which is caused by the cutting force generated during cutting. In the prototype structure, based on the results listed in Table 4, the boom structure is also reinforced by transverse stiffeners in order to avoid the resonance phenomenon. By considering the results of the concept plan in the prototype, basic failures and malfunctions can be avoided. Vibration problems can be significant during machine operation, so FE analyses have been carried out to avoid them. The results can be used with great confidence, so the main dimensions of the concept serve as the basis for the dimensions of the adapter. In the future, it also should be investigated with static and dynamic stress and displacement measurements during in-situ cutting.