Comparison of the Dynamic Performance of Planar 3-DOF Parallel Manipulators
Abstract
1. Introduction
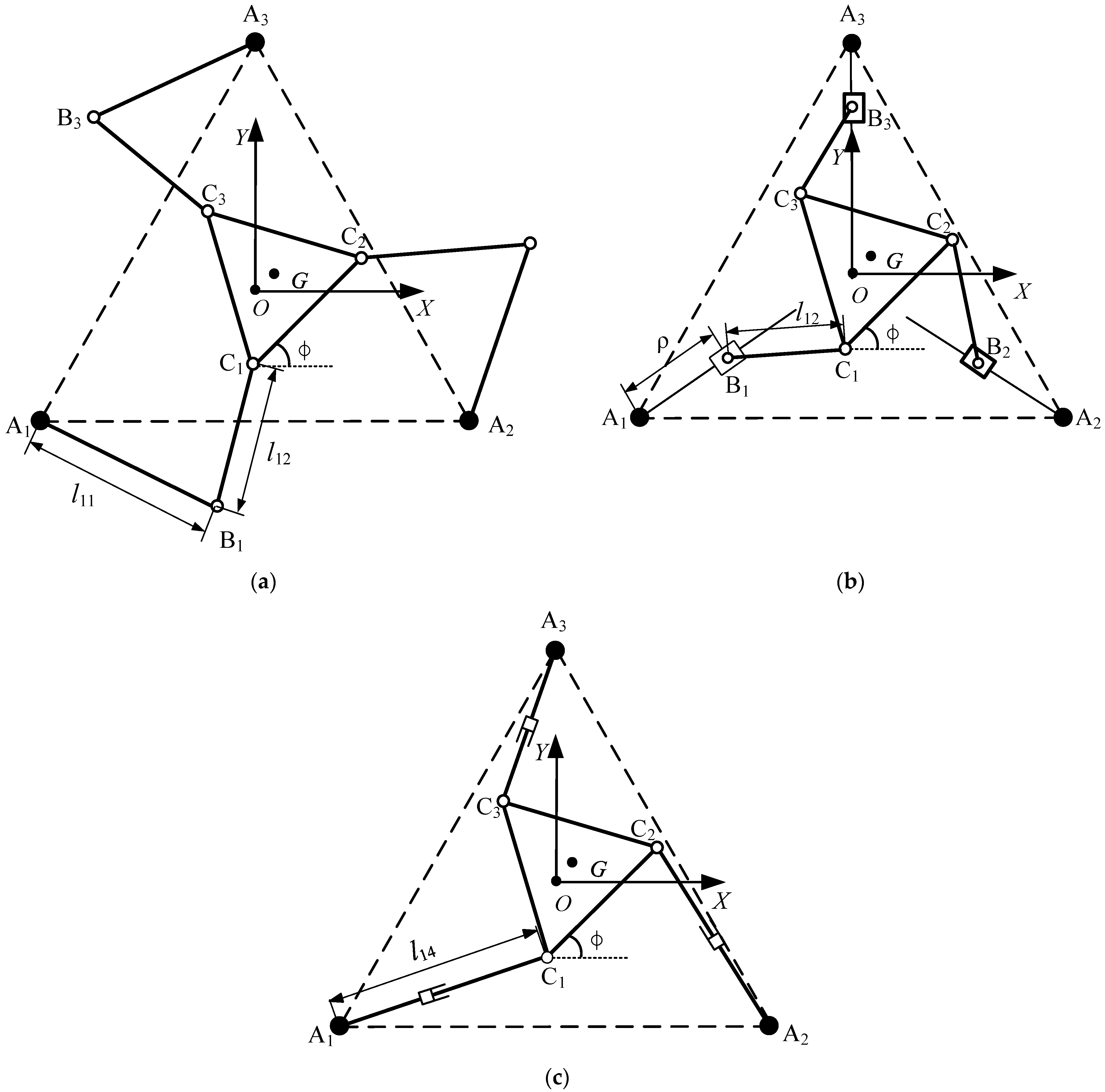
2. Coordinate System and Manipulator Architecture Description of Three PPMs
3. Dynamic Model and Simulations of Three PPMs
3.1. Discrete Time Transfer Matrix Method
- (1)
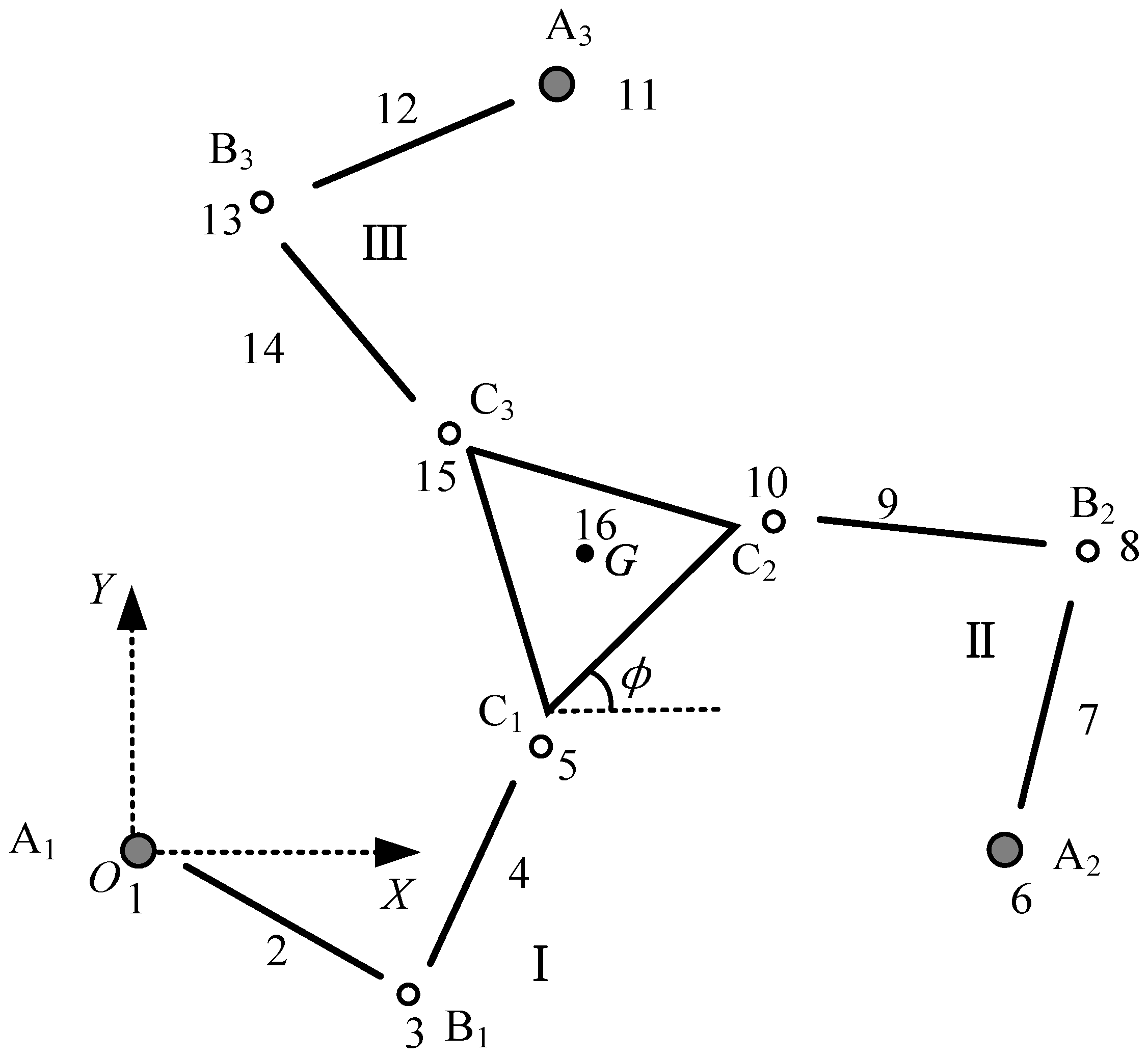
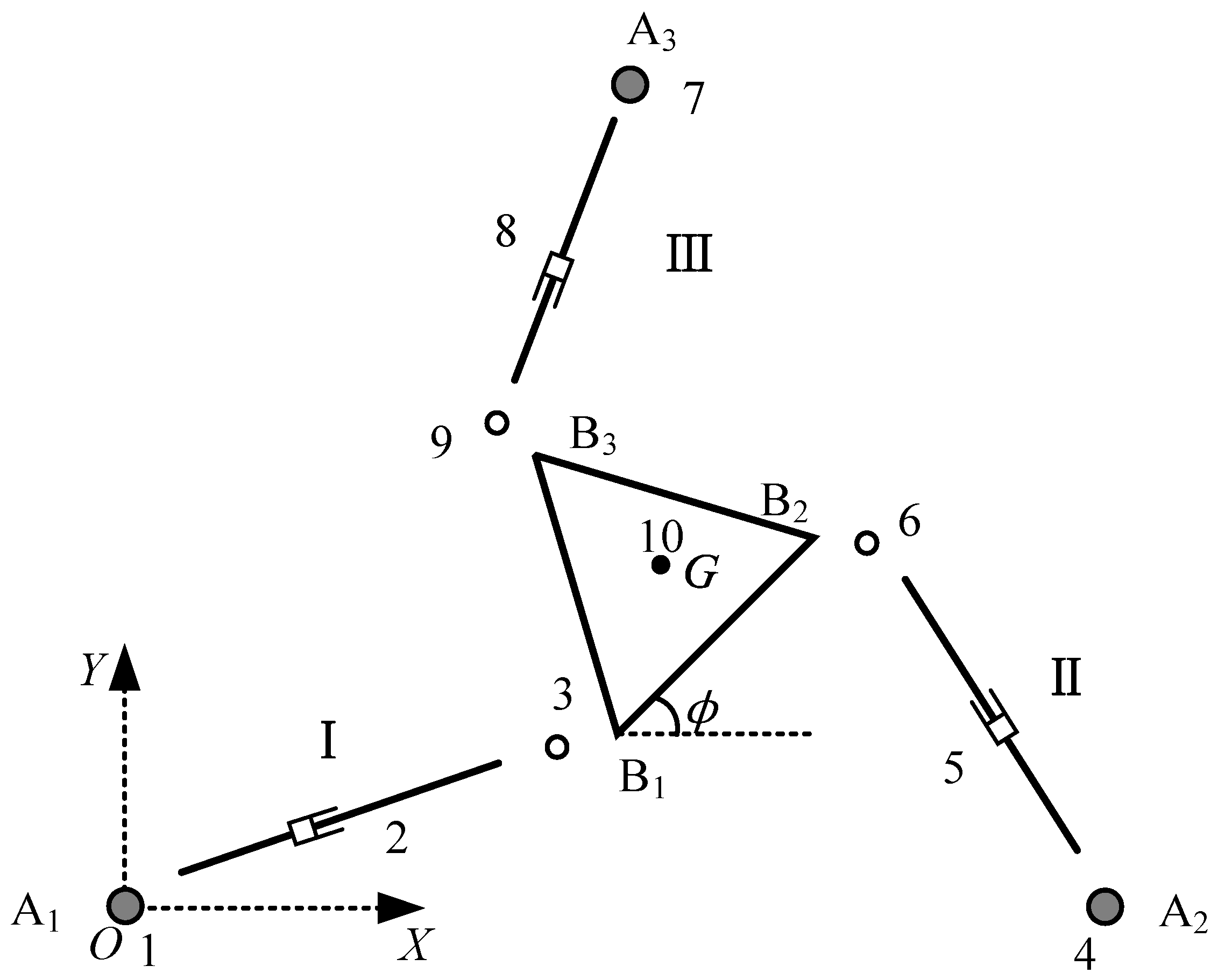
- Performing system discretization, each PPM is divided into subsystems, which can be represented by individual components, such as links, sliders and hinges;
- (2)
- Defining a state vector at both the inboard end and outboard end for each component;
- (3)
- Establishing kinematic and dynamic equations of components and linearizing the kinematic and dynamic equations of the components;
- (4)
- Deriving the component transfer matrix of each component based on its linearized kinematic and dynamic equations;
- (5)
- Assembling the component transfer matrix to obtain the transfer equation for each subsystem and then the global transfer equation with the global transfer matrix of the whole system;
- (6)
- Applying the boundary conditions to the state vectors of the system and calculating the unknown quantities.
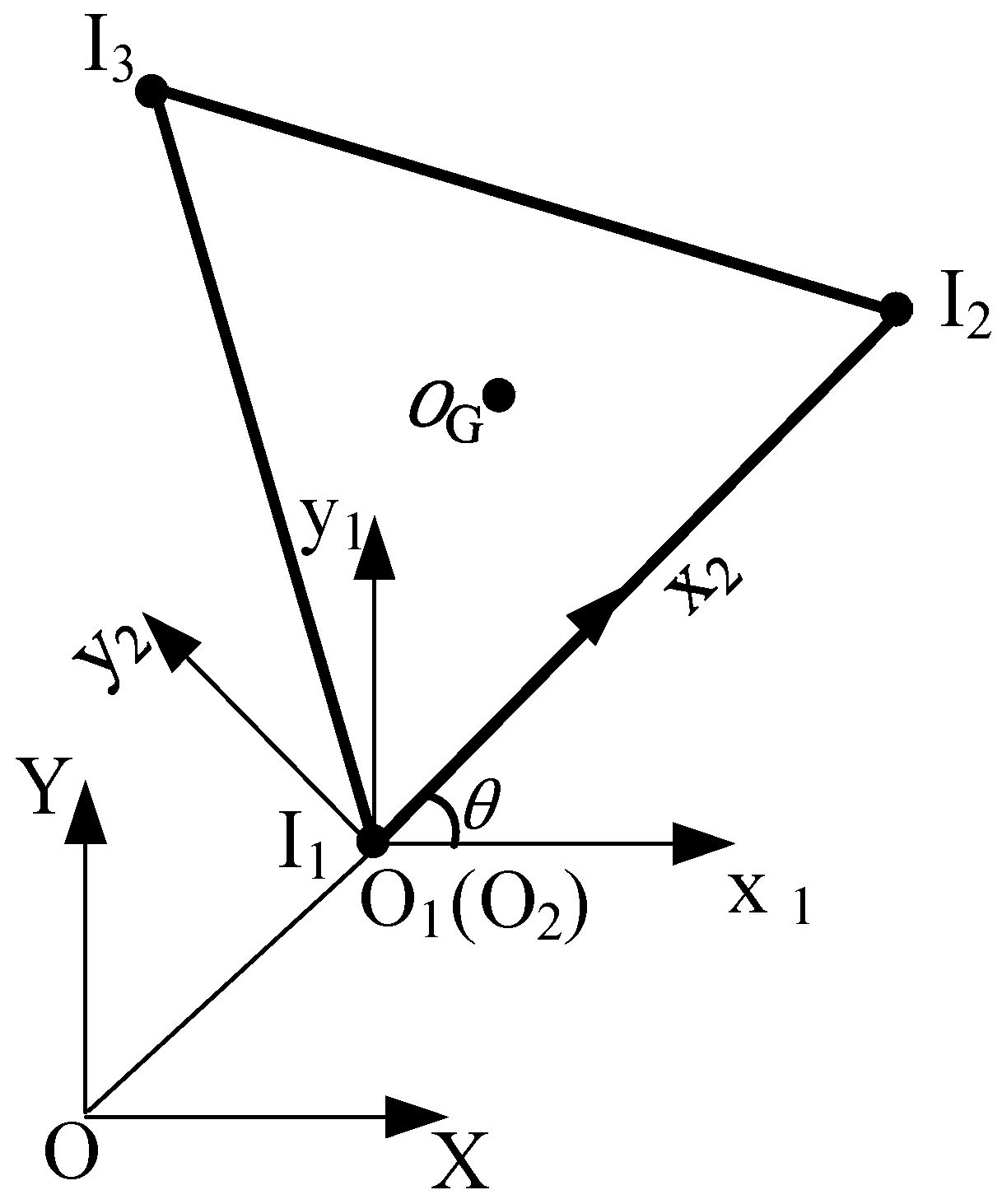
3.1.1. State Vector and Transfer Equation
3.1.2. Transfer Matrices of Components
3.2. Dynamic Model of Three PPMs with DT-TMM
3.2.1. DT-TMM Model of 3-RRR PPM
3.2.2. DT-TMM Model of 3-PRR PPM
3.2.3. DT-TMM Model of 3-RPR PPM
3.3. Dynamic Model with Virtual Work Principle
3.3.1. Jacoby Matrix and Singular Analysis
3.3.2. Virtual Work Principle
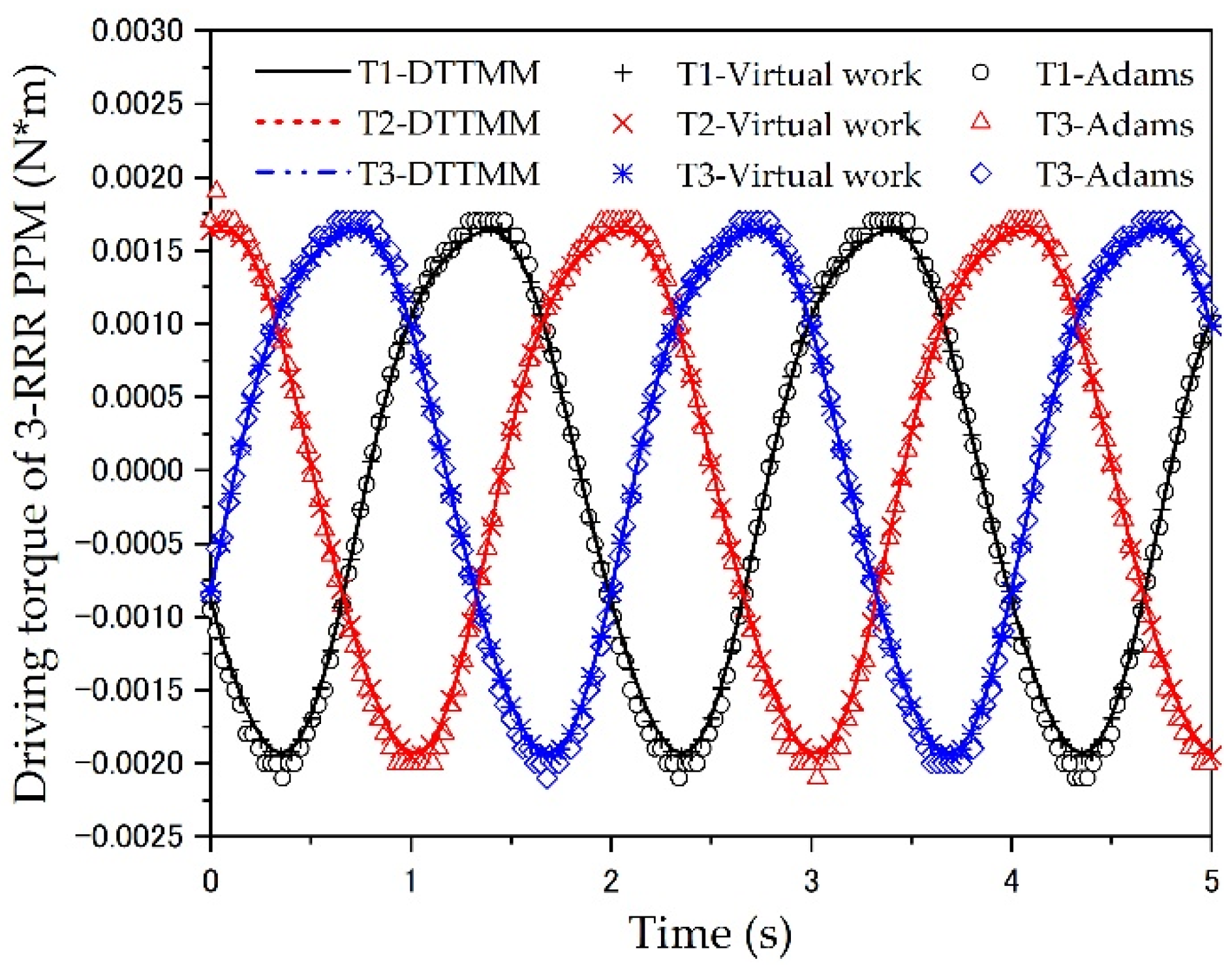
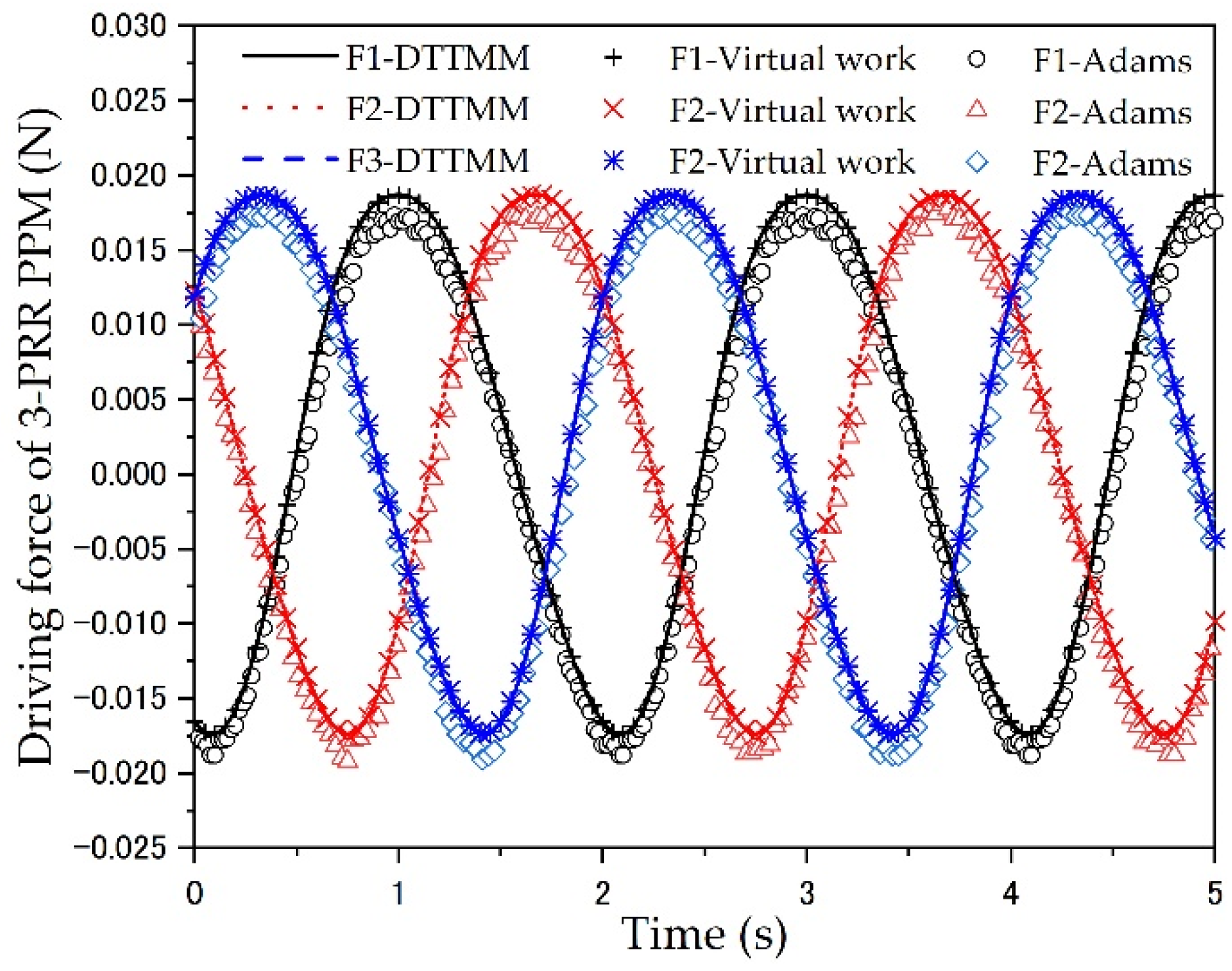
3.4. Dynamic Simulation and Verification
4. Dynamic Performance Indices
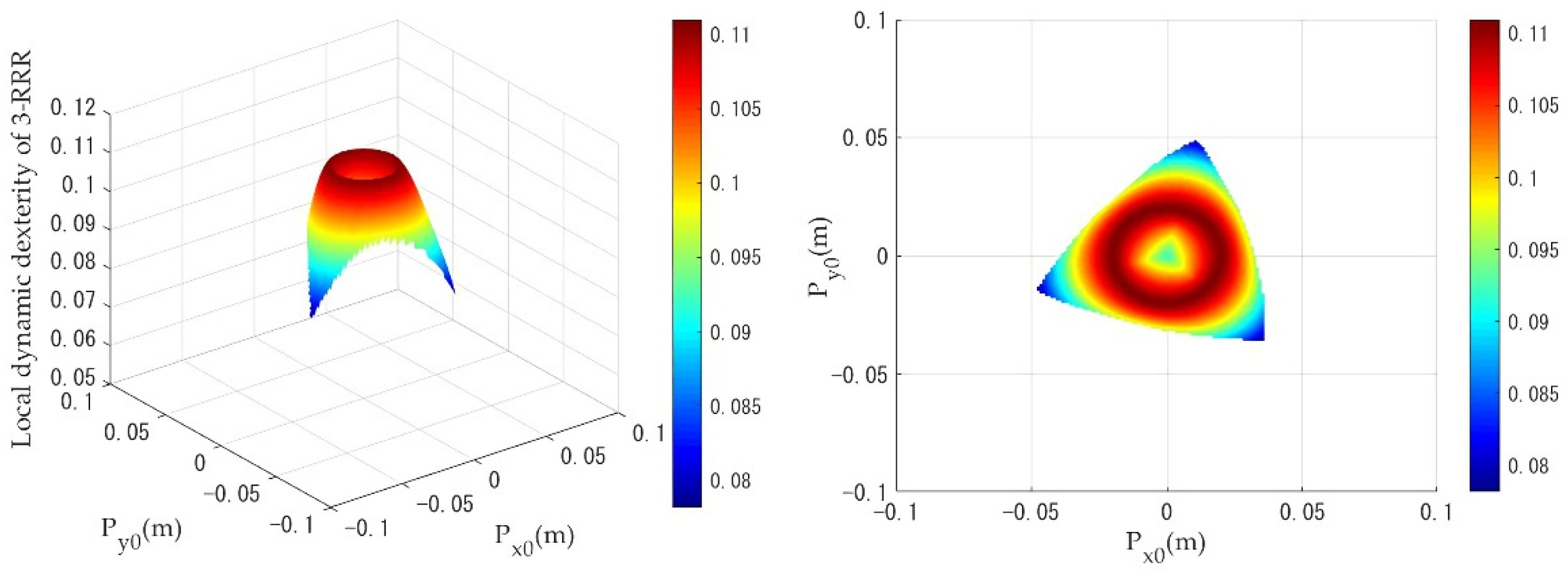
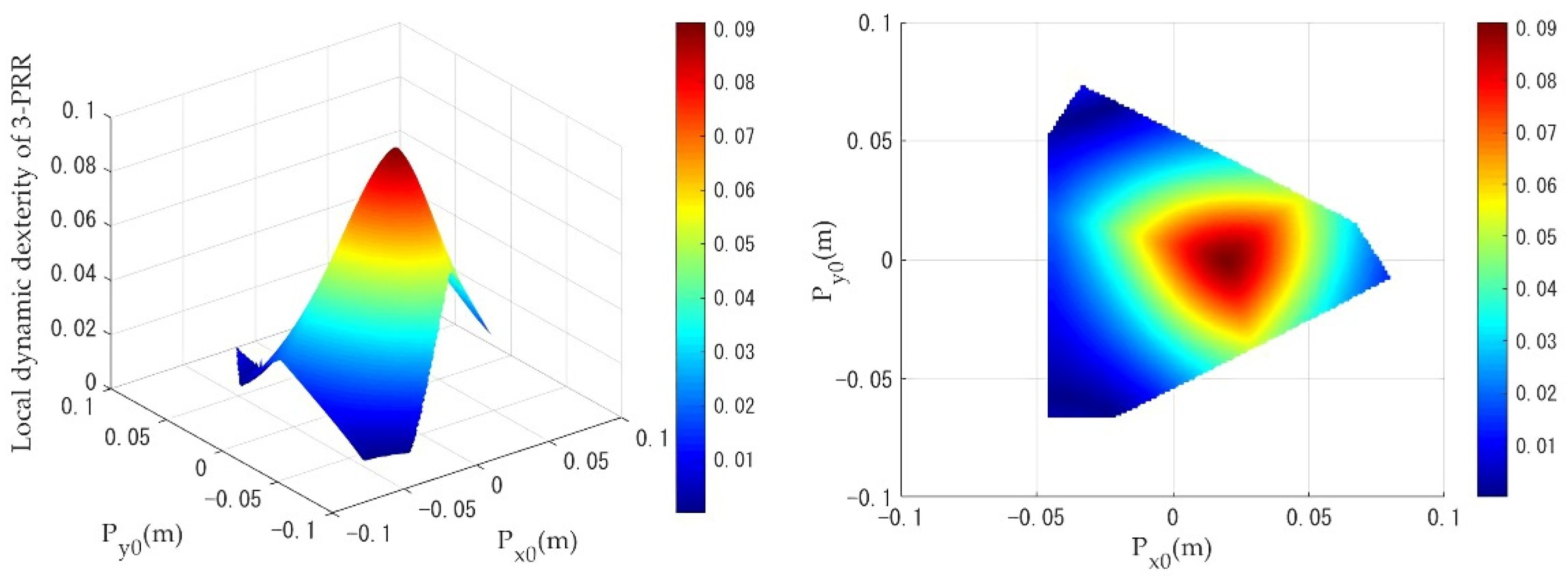
4.1. Dynamic Dexterity Index
4.2. Power Requirement
4.3. Energy Transmission Efficiency
4.4. Joint Force/Torque Margin
5. Simulation and Comparison of Dynamic Performances
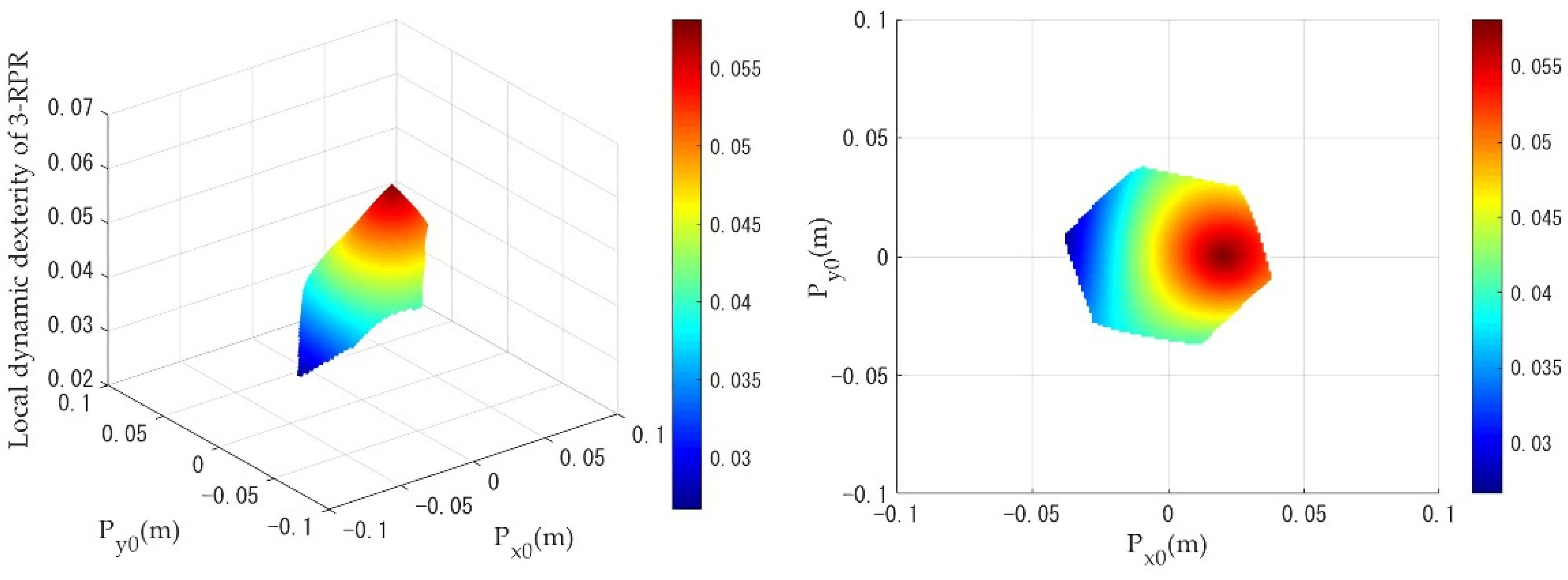
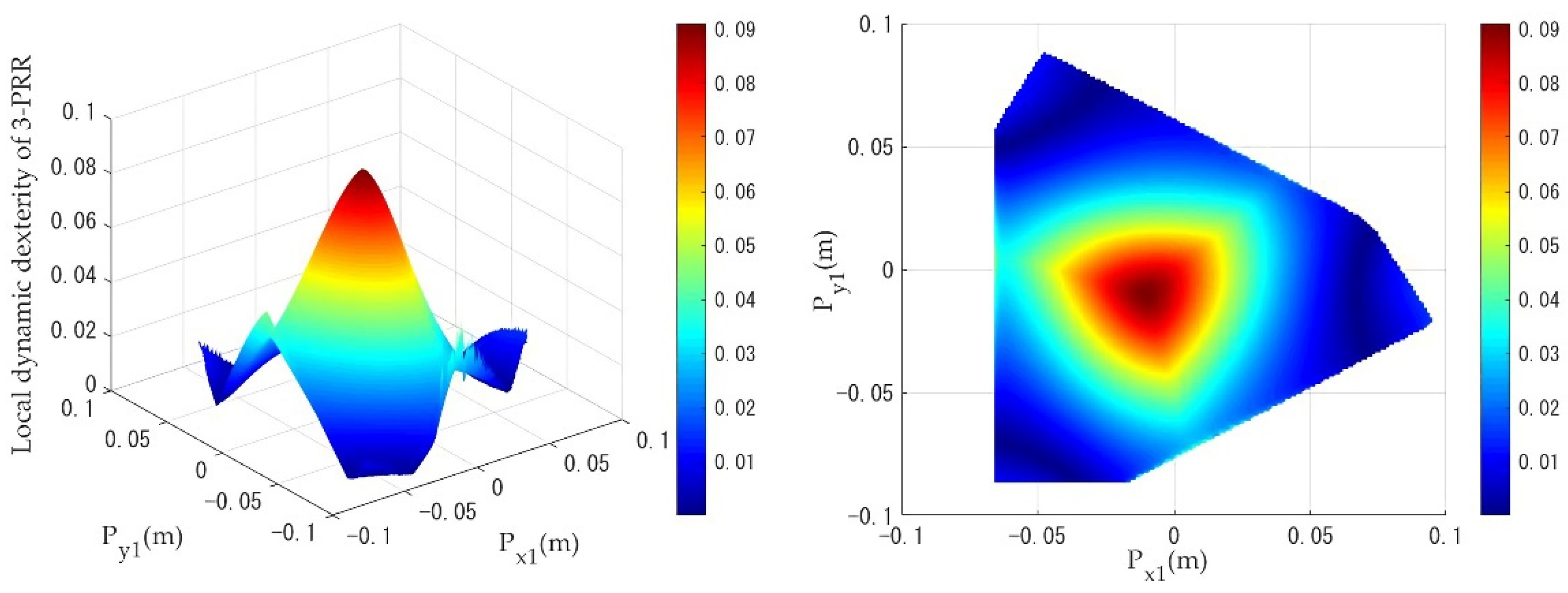
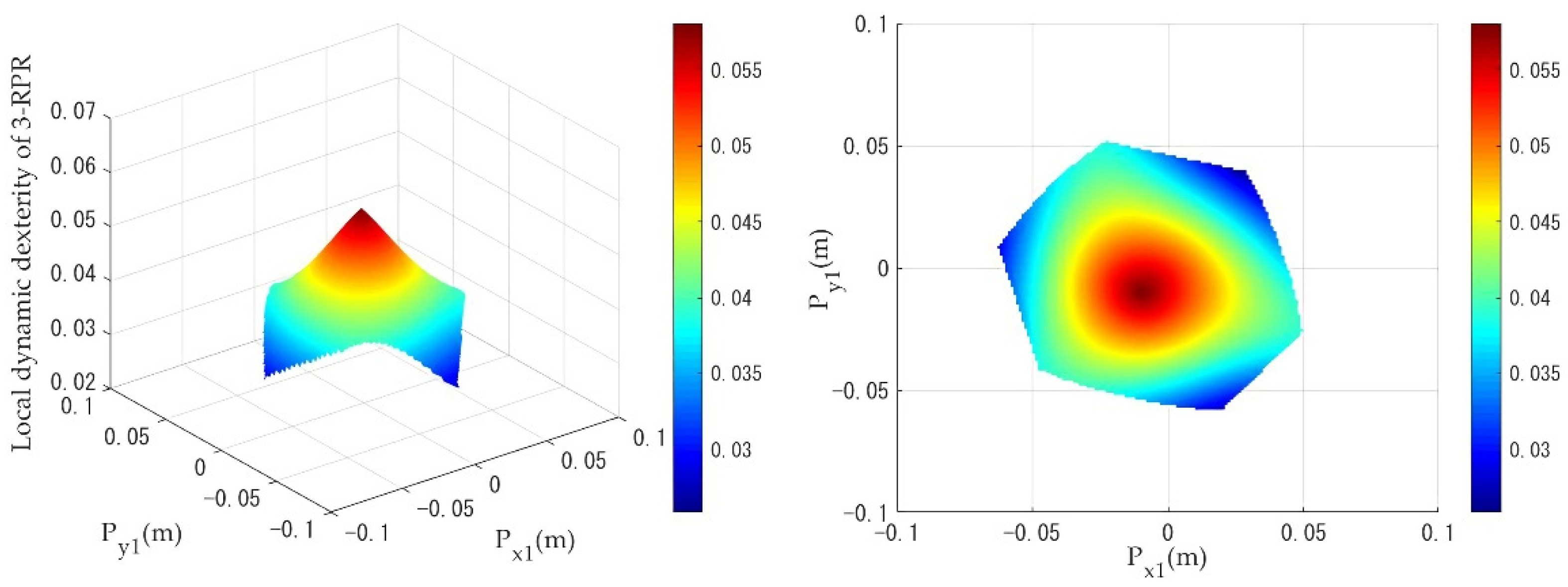
5.1. Comparative Analysis of Dynamic Dexterity
5.2. Comparative Analysis of Power Requirement
5.3. Comparative Analysis of Energy Transmission Efficiency
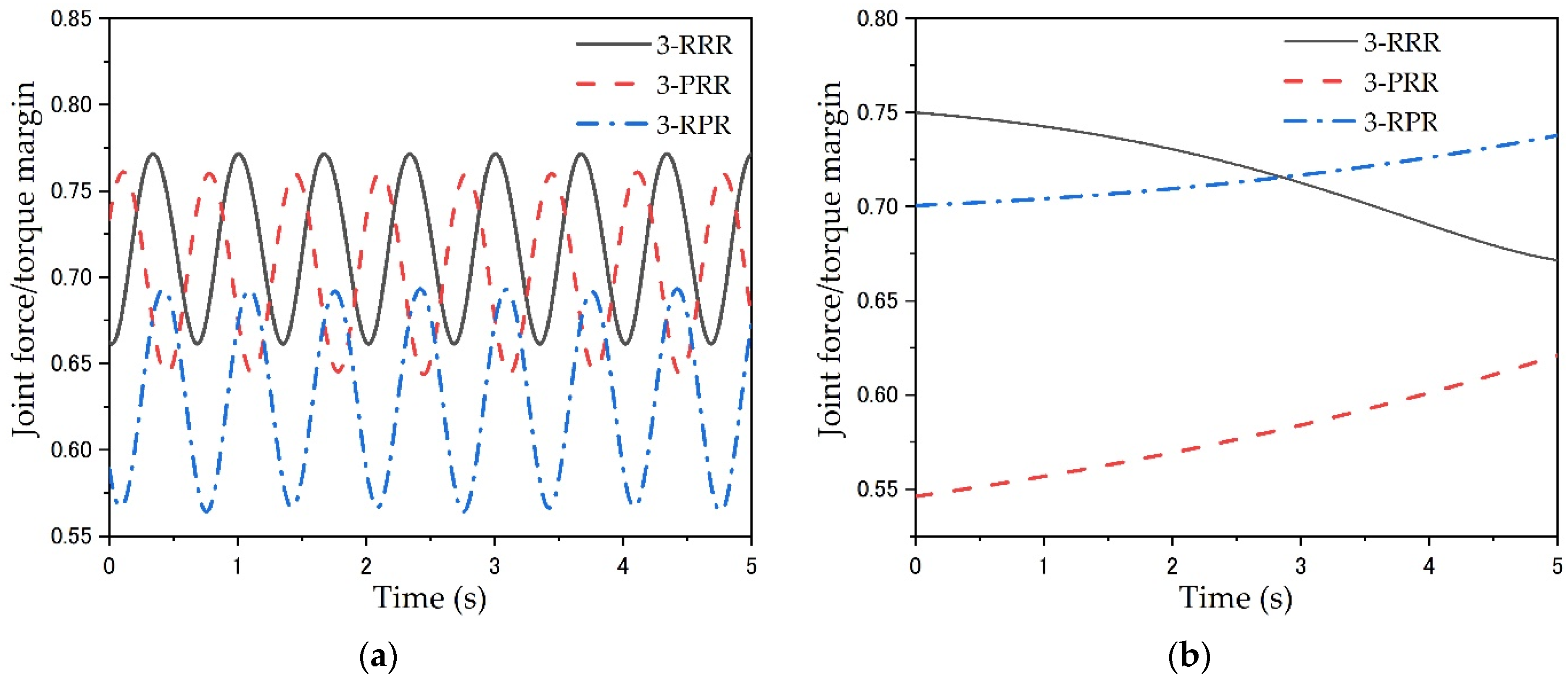
5.4. Comparative Analysis of the Joint Force/Torque Margin
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mazare, M.; Taghizadeh, M.; Najafi, M.R. Kinematic analysis and design of a 3-DOF translational parallel robot. Int. J. Autom. Comput. 2017, 14, 432–441. [Google Scholar] [CrossRef]
- Yu, R.T.; Fang, Y.F.; Guo, S. Design and kinematic performance analysis of rope driven parallel ankle rehabilitation mechanism. Robot 2015, 37, 53–62. [Google Scholar]
- Tanikawa, T.; Ukiana, M.; Morita, K.; Koseki, Y.; Fujii, K.; Arai, T. Design of 3-DOF parallel mechanism with thin plate for micro finger module in micro manipulation. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems, Lausanne, Switzerland, 30 September–4 October 2002. [Google Scholar]
- Gao, F.; Liu, X.J.; Chen, X. The relationships between the shapes of the workspaces and the link lengths of 3-DOF symmetrical planar parallel manipulators. Mech. Mach. Theory 2000, 36, 205–220. [Google Scholar] [CrossRef]
- Wu, J.; Wang, J.S.; Wang, L.P. A comparison study of two planar 2-DOF parallel mechanisms: One with 2-RRR and the other with 3-RRR structures. Robotica 2010, 28, 937–942. [Google Scholar] [CrossRef]
- Binaud, N.; Caro, S.; Wenger, P. Sensitivity comparison of planar parallel manipulators. Mech. Mach. Theory 2010, 45, 1477–1490. [Google Scholar] [CrossRef][Green Version]
- Wu, X.Y.; Yan, R.; Xiang, Z.W.; Zheng, F.Y.; Tan, R.L. Performance analysis and comparison of three planar parallel manipulators. In Proceedings of the Recent Advances in Mechanisms, Transmissions and Applications, MeTrApp 2019, Dalian, China, 9–11 October 2019. [Google Scholar]
- Kucuk, S. A dexterity comparison for 3-DOF planar parallel manipulators with two kinematic chains using genetic algorithms. Mechatronics 2009, 19, 868–877. [Google Scholar] [CrossRef]
- Li, C.R.; Wang, N.F.; Chen, K.J.; Zhang, X.M. Prescribed flexible orientation workspace and performance comparison of non-redundant 3-DOF planar parallel mechanisms. Mech. Mach. Theory 2022, 168, 104602. [Google Scholar] [CrossRef]
- Heerah, I.; Benhabib, B.; Kang, B.; Mills, J.K. Architecture selection and singularity analysis of a three-degree-of-freedom planar parallel manipulator. J. Intell. Robot. Syst. 2003, 37, 335–374. [Google Scholar] [CrossRef]
- Yoshikawa, T. Dynamic manipulability of robot manipulators. In Proceedings of the IEEE International Conference on Robotics and Automation, St. Louis, MO, USA, 25–28 March 1985. [Google Scholar]
- Asada, H. A geometrical representation of manipulator dynamics and its application to arm design. J. Dyn. Syst. Meas. Control 1983, 105, 131–142. [Google Scholar] [CrossRef]
- Asada, H. Dynamic analysis and design of robot manipulators using inertia ellipsoids. In Proceedings of the IEEE International Conference on Robotics and Automation, Atlanta, GA, USA, 13–15 March 1984. [Google Scholar]
- Ryoichi, H. Harmonic mean type manipulatability index of robotic arms. Trans. Soc. Instrum. Control. Eng. 1985, 21, 1351–1353. [Google Scholar]
- Ma, O.; Angeles, J. The concept of dynamic isotropy and its applications to inverse kinematics and trajectory planning. In Proceedings of the IEEE International Conference on Robotics and Automation, Cincinnati, OH, USA, 13–18 May 1990. [Google Scholar]
- Khezrian, R.; Abedloo, E.; Farhadmanesh, M.; Moosavian, S.A.A. Multi criteria design of a spherical 3-DOF parallel manipulator for optimal dynamic performance. In Proceedings of the 2nd RSI/ISM International Conference on Robotics and Mechatronics (ICRoM), Tehran, Iran, 15–17 October 2014. [Google Scholar]
- Li, M.; Huang, T.; Mei, J.P.; Zhao, X.M.; Chetwynd, D.G. Dynamic formulation and performance comparison of the 3-dof modules of two reconfigurable PKM-the Tricept and the TriVariant. J. Mech. Des. 2005, 127, 1129–1136. [Google Scholar] [CrossRef]
- Lu, S.; Li, Y. Dynamic dexterity evaluation of a 3-DOF 3-PUU parallel manipulator based on generalized inertia matrix. In Proceedings of the IEEE International Conference on Robotics and Biomimetics (ROBIO), Zhuhai, China, 6–9 December 2015. [Google Scholar]
- Li, Q.C.; Zhang, N.B.; Wang, F.B. New indices for optimal design of redundantly actuated parallel manipulators. J. Mech. Robot 2017, 9, 011007. [Google Scholar] [CrossRef]
- Zhang, H.Q.; Fang, H.R.; Jiang, B.S.; Wang, S.G. Dynamic performance evaluation of a redundantly actuated and over-constrained parallel manipulator. Int. J. Autom. Comput. 2019, 16, 274–285. [Google Scholar] [CrossRef]
- Wu, J.; Wang, J.S.; You, Z. A comparison study on the dynamics of planar 3-DOF 4-RRR, 3-RRR and 2-RRR parallel manipulators. Robot. Comput. Int. Manuf. 2010, 27, 150–156. [Google Scholar] [CrossRef]
- Mohanta, J.K.; Singh, Y.; Mohan, S. Kinematic and dynamic performance investigations of asymmetric (U-shape fixed base) planar parallel manipulators. Robotica 2018, 36, 1111–1143. [Google Scholar] [CrossRef]
- Singh, Y.; Santhakumar, M. Performance investigations on optimum mechanical design aspects of planar parallel manipulators. Adv. Robot. 2016, 30, 652–675. [Google Scholar] [CrossRef]
- Liu, S.Z.; Yu, Y.Q. Dynamic design of a planar 3-DOF parallel manipulator. Chin. J. Mech. Eng. 2008, 44, 47–52. [Google Scholar] [CrossRef]
- Zhang, H.D.; Zhang, X.M.; Zhang, X.C.; Mo, J. Dynamic analysis of a 3- PRR parallel mechanism by considering joint clearances. Nonlinear Dyn. 2017, 90, 405–423. [Google Scholar] [CrossRef]
- Staicu, S. Power requirement comparison in the 3-RPR planar parallel robot dynamics. Mech. Mach. Theory 2009, 44, 1045–1057. [Google Scholar] [CrossRef]
- Mei, Y.; Gao, X.X.; Song, J. Dynamics Analysis of 3-PPR Planar Parallel Mechanism Including Friction. Adv. Mater. Res. 2014, 1006, 265–270. [Google Scholar] [CrossRef]
- Rui, X.T.; He, B.; Rong, B.; Wang, G.P.; Lu, Y.Q. Discrete time transfer matrix method for dynamics of a multi-rigid-flexible-body system moving in plane. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2009, 223, 23–24. [Google Scholar] [CrossRef]
- Rui, X.T.; Wang, G.P.; Lu, Y.Q.; Yun, L.F. Transfer matrix method for linear multibody system. Multibody Syst. Dyn 2008, 19, 179–207. [Google Scholar] [CrossRef]
- Rui, X.T.; He, B.; Lu, Y.Q.; Wang, G.P. Discrete Time Transfer Matrix Method for Multibody System Dynamics. Multibody Syst. Dyn. 2005, 14, 317–344. [Google Scholar] [CrossRef]
- Rong, B.; Rui, X.T.; Tao, L.; Wang, G.P. Dynamics analysis and fuzzy anti-swing control design of overhead crane system based on Riccati discrete time transfer matrix method. Multibody Syst. Dyn. 2018, 43, 279–295. [Google Scholar] [CrossRef]
- Zhang, X.P.; Sørensen, R.; Iversen, M.R.; Li, H.J. Computationally efficient dynamic modeling of robot manipulators with multiple flexible-links using acceleration-based discrete time transfer matrix method. Robot. Comput.-Integr. Manuf. 2018, 49, 181–193. [Google Scholar] [CrossRef]
- Rui, X.T.; Wang, X. Zhou, Q.B.; Zhang, J.S. Transfer matrix method for multibody systems (Rui method) and its applications. Sci. China Technol. Sci. 2019, 62, 712–720. [Google Scholar] [CrossRef]
- Rui, X.T. Transfer Matrix Method for Multi-Body System and Application; Science Press: Beijing, China, 2008; pp. 259–299. [Google Scholar]
- Si, G.N.; Chu, M.Q.; Zhang, Z.; Li, H.J.; Zhang, X.P.; Vidoni, R. Integrating dynamics into design and motion optimization of a 3-prr planar parallel manipulator with discrete time transfer matrix method. Math. Probl. Eng. 2020, 2020, 2761508. [Google Scholar] [CrossRef]
- Wang, D.; Wang, L.P.; Wu, J.; Ye, H. An experimental study on the dynamics calibration of a 3-DOF parallel tool head. IEEE ASME Trans. Mechatron. 2019, 24, 2931–2941. [Google Scholar] [CrossRef]
- Bourbonnais, F.; Bigras, P.; Bonev, I.A. Minimum-time trajectory planning and control of a pick-and place five-bar parallel robot. IEEE ASME Trans. Mechatron. 2015, 20, 740–749. [Google Scholar] [CrossRef]
- Jiang, Q.M.; Gosselin, C.M. The maximal singularity-free workspace of planar 3RPR parallel mechanisms. In Proceedings of the IEEE International Conference on Mechatronics and Automation, Luoyang, China, 25–28 June 2006. [Google Scholar]
- Wu, J.; Wang, L.P.; You, Z. A new method for optimum design of parallel manipulator based on kinematics and dynamics. Nonlinear Dyn. 2010, 61, 717–727. [Google Scholar] [CrossRef]
- Zhan, Z.H.; Zhang, X.M.; Zhang, H.D.; Chen, G.C. Unified motion reliability analysis and comparison study of planar parallel manipulators with interval joint clearance variables. Mech. Mach. Theory 2019, 138, 58–75. [Google Scholar] [CrossRef]





| Symbols | Unit | Parameters |
|---|---|---|
| (m) | Length of the first link in the 3-RRR chain | |
| (m) | Length of the second link in the 3-RRR chain | |
| (m) | Length of the link in the 3-PRR chain | |
| (m) | Minimal length of the link in the 3-RPR chain | |
| (m) | Maximal length of the link in the 3-RPR chain | |
| (m) | Length of the mobile platform side | |
| (m) | Length of the base side | |
| (kg) | Mass of the sliders | |
| (kg) | Mass of the links in the 3-RRR and 3-PRR PPMs | |
| (kg) | Mass of the links in the 3-RPR PPM | |
| (kg) | Mass of the mobile platform | |
| (deg) | Orientation of the platform |
| Methods | 3-RRR PPM | 3-PRR PPM | 3-RPR PPM | ||||||
|---|---|---|---|---|---|---|---|---|---|
| T1 | T2 | T3 | F1 | F2 | F3 | T1 | T2 | T3 | |
| DT-TMM and ADAMS | 0.0002 | 0.0002 | 0.0002 | 0.0015 | 0.0015 | 0.0015 | 0.0003 | 0.0003 | 0.0003 |
| DT-TMM and the virtual work principle | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| PPM | (m) | |||||||
|---|---|---|---|---|---|---|---|---|
| 3-RRR | 0.3 | 0.08 | 0.098 | 0.098 | - | - | - | - |
| 3-PRR | 0.3 | 0.08 | - | 0.03–0.13 | 0.10 | - | - | |
| 3-RPR | 0.3 | 0.08 | - | - | - | - | 0.09 | 0.2 |
| PPM | Circular Trajectory | Linear Trajectory | ||
|---|---|---|---|---|
| 3-RRR | 0.1015 | 56.31% | 0.0602 | 45.53 |
| 3-PRR | 0.0592 | 73.37% | 0.0631 | 69.95 |
| 3-RPR | 0.0438 | 52.13% | 0.0452 | 46.25 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Si, G.; Chen, F.; Zhang, X. Comparison of the Dynamic Performance of Planar 3-DOF Parallel Manipulators. Machines 2022, 10, 233. https://doi.org/10.3390/machines10040233
Si G, Chen F, Zhang X. Comparison of the Dynamic Performance of Planar 3-DOF Parallel Manipulators. Machines. 2022; 10(4):233. https://doi.org/10.3390/machines10040233
Chicago/Turabian StyleSi, Guoning, Fahui Chen, and Xuping Zhang. 2022. "Comparison of the Dynamic Performance of Planar 3-DOF Parallel Manipulators" Machines 10, no. 4: 233. https://doi.org/10.3390/machines10040233
APA StyleSi, G., Chen, F., & Zhang, X. (2022). Comparison of the Dynamic Performance of Planar 3-DOF Parallel Manipulators. Machines, 10(4), 233. https://doi.org/10.3390/machines10040233







