On Extending the Applicability of Iterative Methods for Solving Systems of Nonlinear Equations
Abstract
1. Introduction
- (i)
- We obtain the convergence order of method (3) without using the Taylor series expansion.
- (ii)
- We use conditions on the operator and its Fréchet derivates up to the third order only.
- (iii)
- We provide the convergence ball which was not given in earlier studies.
- (iv)
- The analysis in [10] was conducted in the Euclidean spaces, whereas our study is in a more general commutative Banach algebra setting.
2. Preliminary Concepts
- (i)
- ,
- (ii)
- There exist , , and such that
- (C1)
- (C2)
- ;
- (C3)
- ;
- (C4)
- ;
- (C5)
- ;
- (C6)
- .
3. Main Results
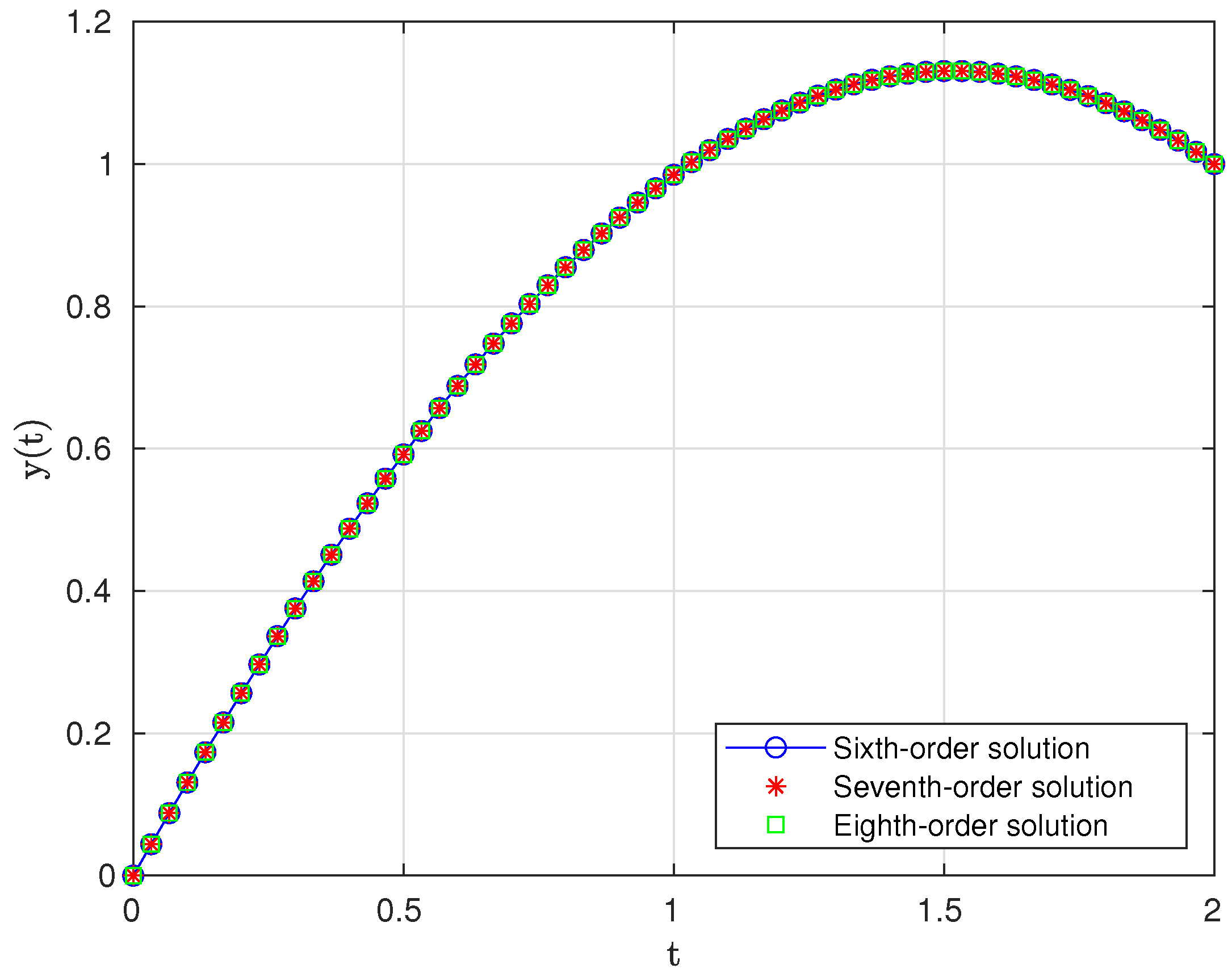
4. Numerical Examples
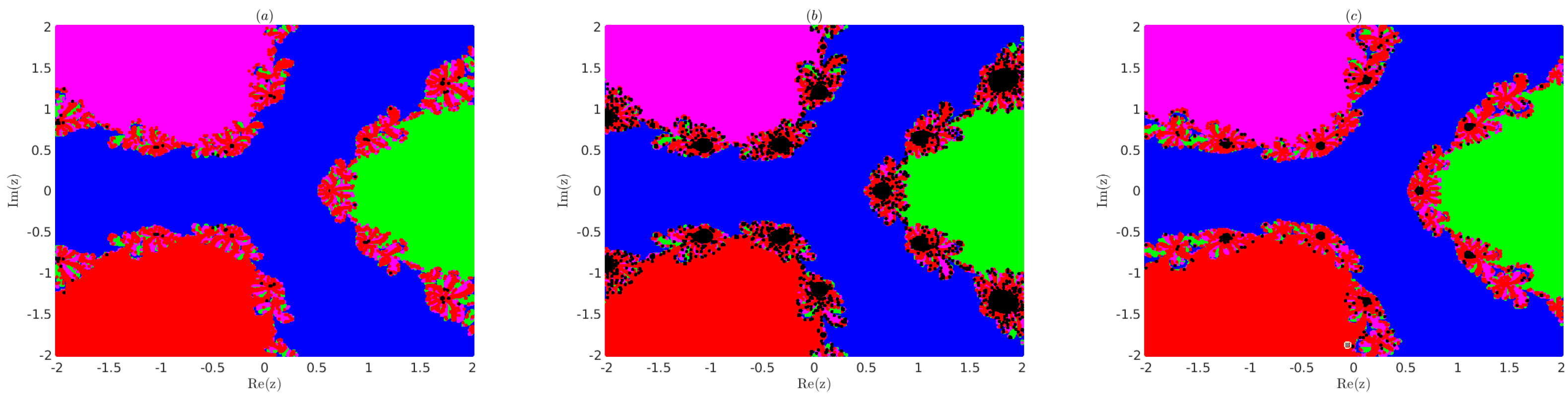
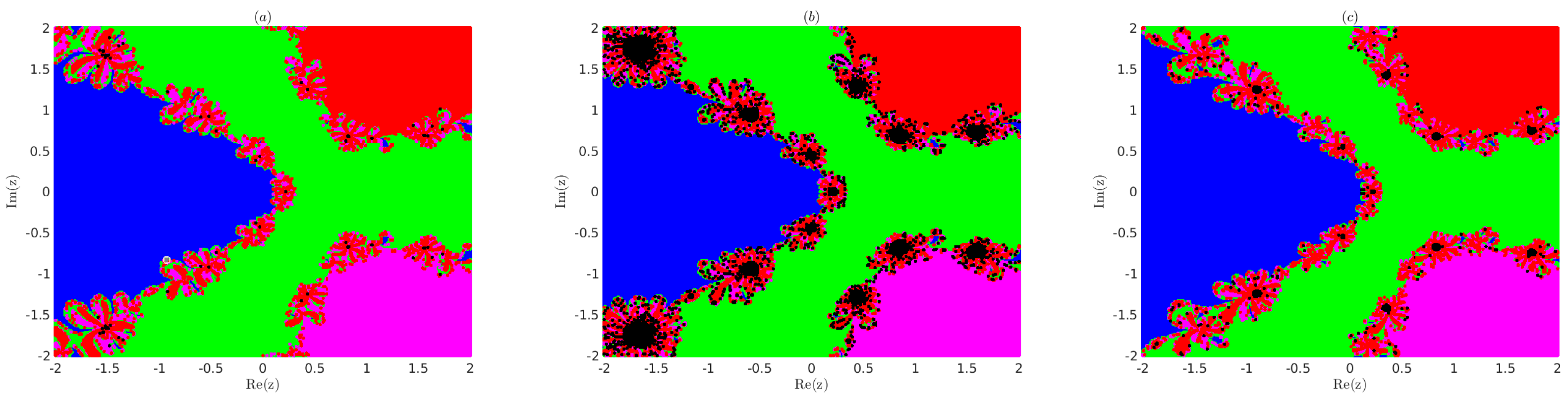
5. Basin of Attractions
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sakawa, Y. Optimal control of a certain type of linear distributed-parameter systems. IEEE Trans. Automat. Control 1966, 11, 35–41. [Google Scholar] [CrossRef]
- Hu, S.; Khavanin, M.; Zhuang, W. Integral equations arising in the kinetic theory of gases. Appl. Anal. 1989, 34, 261–266. [Google Scholar] [CrossRef]
- Cercignani, C. Nonlinear problems in the kinetic theory of gases. In Trends in Applications of Mathematics to Mechanics (Wassenaar, 1987); Springer: Berlin/Heidelberg, Germany, 1988; pp. 351–360. [Google Scholar]
- Lin, Y.; Bao, L.; Jia, X. Convergence analysis of a variant of the Newton method for solving nonlinear equations. Comput. Math. Appl. 2010, 59, 2121–2127. [Google Scholar] [CrossRef]
- Grosan, C.; Abraham, A. A New Approach for Solving Nonlinear Equations Systems. IEEE Trans. Syst. Man Cybern.-Part A Syst. Humans 2008, 38, 698–714. [Google Scholar] [CrossRef]
- Moré, J.J. A collection of nonlinear model problems. In Computational Solution of Nonlinear Systems of Equations. Lectures in Applied Mathematics; American Mathematical Society: Providence, RI, USA, 1990; Volume 26, pp. 723–762. [Google Scholar]
- Argyros, I.K. Convergence and Applications of Newton-Type Iterations; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Behl, R.; Arora, H. CMMSE: A novel scheme having seventh-order convergence for nonlinear systems. J. Comput. Appl. Math. 2022, 404, 113301. [Google Scholar] [CrossRef]
- Lotfi, T.; Bakhtiari, P.; Cordero, A.; Mahdiani, K.; Torregrosa, J.R. Some new efficient multipoint iterative methods for solving nonlinear systems of equations. Int. J. Comput. Math. 2015, 92, 1921–1934. [Google Scholar] [CrossRef]
- Cordero, A.; Leonardo-Sepúlveda, M.A.; Torregrosa, J.R.; Vassileva, M.P. Increasing in three units the order of convergence of iterative methods for solving nonlinear systems. Math. Comput. Simul. 2024, 223, 509–522. [Google Scholar] [CrossRef]
- George, S.; Bate, I.; Muniyasamy, M.; Chandhini, G.; Senapati, K. Enhancing the applicability of Chebyshev-like method. J. Complex. 2024, 83, 101854. [Google Scholar] [CrossRef]
- George, S.; Kunnarath, A.; Sadananda, R.; Jidesh, P.; Argyros, I.K. On obtaining order of convergence of Jarratt-like method without using Taylor series expansion. Comput. Appl. Math. 2024, 43, 243. [Google Scholar] [CrossRef]
- Muniyasamy, M.; Chandhini, G.; George, S.; Bate, I.; Senapati, K. On obtaining convergence order of a fourth and sixth order method of Hueso et al. without using Taylor series expansion. J. Comput. Appl. Math. 2024, 452, 116136. [Google Scholar]
- Ostowski, A.M. Solution of Equations and System of Equations; Academic Press: New York, NY, USA, 1960. [Google Scholar]
- Weerakoon, S.; Fernando, T. A variant of Newton’s method with accelerated third-order convergence. Appl. Math. Lett. 2000, 13, 87–93. [Google Scholar] [CrossRef]
- Cordero, A.; Martínez, E.; Torregrosa, J.R. Iterative methods of order four and five for systems of nonlinear equations. J. Comput. Appl. Math. 2009, 231, 541–551. [Google Scholar] [CrossRef]
- Petković, M.S.; Neta, B.; Petković, L.D.; Džunić, J. Multipoint methods for solving nonlinear equations: A survey. Appl. Math. Comput. 2014, 226, 635–660. [Google Scholar] [CrossRef]
- Argyros, I.K. The Theory and Applications of Iteration Methods, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2022. [Google Scholar]
- Cordero, A.; Gómez, E.; Torregrosa, J.R. Efficient high-order iterative methods for solving nonlinear systems and their application on heat conduction problems. Complexity 2017, 1, 6457532. [Google Scholar] [CrossRef]
- Kelley, C.T. Approximation of solutions of some quadratic integral equations in transport theory. J. Integral Equ. 1982, 4, 221–237. [Google Scholar]
- Hernández-Verón, M.A.; Martínez, E.; Singh, S. On the Chandrasekhar integral equation. Comput. Math. Methods 2021, 3, e1150. [Google Scholar] [CrossRef]
- Burridge, R.; Knopoff, L. Model and theoretical seismicity. Bull. Seismol. Soc. Amer. 1967, 57, 341–371. [Google Scholar] [CrossRef]
- van der Pol, B. The nonlinear theory of electric oscillations. Proc. IRE 1934, 22, 1051–1086. [Google Scholar] [CrossRef]
- FitzHugh, R. Impulses and Physiological States in Theoretical Models of Nerve Membrane. Biophys. J. 1961, 1, 445–466. [Google Scholar] [CrossRef]
- Hernández, M. Chebyshev’s approximation algorithms and applications. Comput. Math. Appl. 2001, 41, 433–445. [Google Scholar] [CrossRef]
- George, S.; Sadananda, R.; Padikkal, J.; Argyros, I.K. On the Order of Convergence of the Noor–Waseem Method. Mathematics 2022, 10, 4544. [Google Scholar] [CrossRef]
- Ham, Y.; Chun, C. A fifth-order iterative method for solving nonlinear equations. Appl. Math. Comput. 2007, 194, 287–290. [Google Scholar] [CrossRef]
- Grau-Sánchez, M.; Grau, À.; Noguera, M. On the computational efficiency index and some iterative methods for solving systems of nonlinear equations. J. Comput. Appl. Math. 2011, 236, 1259–1266. [Google Scholar] [CrossRef]
- Behl, R.; Cordero, A.; Motsa, S.S.; Torregrosa, J.R. Stable high-order iterative methods for solving nonlinear models. Appl. Math. Comput. 2017, 303, 70–88. [Google Scholar] [CrossRef]
- Amat, S.; Busquier, S.; Plaza, S. Review of some iterative root-finding methods from a dynamical point of view. Sci. Ser. A Math. Sci. 2004, 10, 3–35. [Google Scholar]
- Varona, J.L. Graphic and numerical comparison between iterative methods. Math. Intell. 2002, 24, 37–46. [Google Scholar] [CrossRef]
- Campos, B.; Canela, J.; Vindel, P. Dynamics of Newton-like root finding methods. Numer. Algorithms 2023, 93, 1453–1480. [Google Scholar] [CrossRef]
- Husain, A.; Nanda, M.N.; Chowdary, M.S.; Sajid, M. Fractals: An Eclectic Survey, Part-I. Fractal Fract. 2022, 6, 89. [Google Scholar] [CrossRef]
- Husain, A.; Nanda, M.N.; Chowdary, M.S.; Sajid, M. Fractals: An Eclectic Survey, Part-II. Fractal Fract. 2022, 6, 379. [Google Scholar] [CrossRef]
- Laplante, P.A.; Laplante, C. Introduction to Chaos, Fractals and Dynamical Systems; World Scientific: London, UK, 2023. [Google Scholar]
| Grids | as in (18) | as in (19) | as in (20) | |||
|---|---|---|---|---|---|---|
| 0 | 0 | 0 | ||||
| 0 | 0 | 0 | ||||
| 0 | 0 | 0 | ||||
| 0 | 0 | 0 | ||||
| 0 | 0 | 0 | ||||
| 0 | 0 | 0 | ||||
| 0 | 0 | 0 | ||||
| 0 | 0 | 0 | ||||
| Iteration | as in (18) | as in (19) | as in (20) |
|---|---|---|---|
| 1 | (, 0) | (, 0) | (, 0) |
| 2 | (, 0) | (0, , 0) | (0, 0, 0) |
| 3 | (0, 0, 0) | (0, 0, 0) | (0, 0, 0) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bate, I.; Murugan, M.; George, S.; Senapati, K.; Argyros, I.K.; Regmi, S. On Extending the Applicability of Iterative Methods for Solving Systems of Nonlinear Equations. Axioms 2024, 13, 601. https://doi.org/10.3390/axioms13090601
Bate I, Murugan M, George S, Senapati K, Argyros IK, Regmi S. On Extending the Applicability of Iterative Methods for Solving Systems of Nonlinear Equations. Axioms. 2024; 13(9):601. https://doi.org/10.3390/axioms13090601
Chicago/Turabian StyleBate, Indra, Muniyasamy Murugan, Santhosh George, Kedarnath Senapati, Ioannis K. Argyros, and Samundra Regmi. 2024. "On Extending the Applicability of Iterative Methods for Solving Systems of Nonlinear Equations" Axioms 13, no. 9: 601. https://doi.org/10.3390/axioms13090601
APA StyleBate, I., Murugan, M., George, S., Senapati, K., Argyros, I. K., & Regmi, S. (2024). On Extending the Applicability of Iterative Methods for Solving Systems of Nonlinear Equations. Axioms, 13(9), 601. https://doi.org/10.3390/axioms13090601