Fermatean Probabilistic Hesitant Fuzzy Power Bonferroni Aggregation Operators with Dual Probabilistic Information and Their Application in Green Supplier Selection
Abstract
1. Introduction
2. Preliminaries
2.1. Fermatean Fuzzy Set (FFS)
- (1)
- ;
- (2)
- ;
- (3)
- , ;
- (4)
- , if and only if .
- (1)
- ;
- (2)
- ;
- (3)
- , ;
- (4)
- , .
2.2. Fermatean Hesitant Fuzzy Set (FHFS)
2.3. Fermatean Probabilistic Hesitant Fuzzy Set (FPHFS)
- (1)
- ;
- (2)
- ;
- (3)
- .
- (1)
- ;
- (2)
- ;
- (3)
- ;
- (4)
- .
- (1)
- If , then ;
- (2)
- If , then ;
- (3)
- If , then
- (i)
- If , then ;
- (ii)
- If , then .
- (1)
- When the value of is 1, the optimistic decision maker may add the maximum membership degree and the maximum non-membership degree .
- (2)
- When the value of is 0.5, the neutral decision maker may add the membership degree of and the non-membership degree of .
- (3)
- When the value of is 0, the pessimist decision maker may add the minimum membership degree and the minimum non-membership degree .
2.4. Power Bonferroni Mean (PBM) Operator
3. Fermatean Hesitant Fuzzy Power Bonferroni Aggregation Operators
4. Fermatean Probabilistic Hesitant Fuzzy Power Bonferroni Aggregation Operators
5. Application of the FPHFPWPBM Operator in Green Supplier Selection
5.1. Decision-Making Process
5.2. Numerical Example
5.3. Sensitivity Analysis
5.4. Comparative Analysis
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| Sr.NO | Complete Word | Abbreviation |
| 1 | Multi-attribute decision-making | MADM |
| 2 | Fuzzy set | FS |
| 3 | Intuitionistic fuzzy set | IFS |
| 4 | Pythagorean fuzzy set | PFS |
| 5 | Fermatean fuzzy set | FFS |
| 6 | q-Rung orthopair fuzzy set | q-ROFS |
| 7 | Hesitant fuzzy set | HFS |
| 8 | Interval-valued hesitant fuzzy set | IVHFS |
| 9 | Intuitionistic hesitant fuzzy set | IHFS |
| 10 | Pythagorean hesitant fuzzy set | PyHFS |
| 11 | Fermatean hesitant fuzzy set | FHFS |
| 12 | Interval-valued hesitant Fermatean fuzzy set | IVHFFS |
| 13 | Probabilistic hesitant fuzzy set | PHFS |
| 14 | Probabilistic linguistic term set | PLTS |
| 15 | Probabilistic interval-valued hesitant fuzzy set | PIVHFS |
| 16 | Probabilistic interval-valued Fermatean hesitant fuzzy set | PIVFHFS |
| 17 | q-Rung orthopair probabilistic hesitant fuzzy rough set | q-ROPHFS |
| 18 | q-Rung orthopair probabilistic hesitant fuzzy rough set | q-ROPHFRS |
| 19 | Pythagorean probabilistic hesitant fuzzy set | PyPHFS |
| 20 | Fermatean probabilistic hesitant fuzzy set | FPHFS |
| 21 | Power average | PA |
| 22 | Bonferroni mean | BM |
| 23 | Power Bonferroni Mean | PBM |
| 24 | Fermatean fuzzy power Bonferroni mean | FFPBM |
| 25 | Fermatean fuzzy weighted power Bonferroni mean | FFWPBM |
| 26 | Fermatean hesitant fuzzy power Bonferroni mean | FHFPBM |
| 27 | Fermatean hesitant fuzzy weighted power Bonferroni mean | FHFWPBM |
| 28 | Fermatean hesitant fuzzy probabilistic weighted power Bonferroni mean | FHFPWPBM |
| 29 | Fermatean probabilistic hesitant fuzzy power Bonferroni mean | FPHFPBM |
| 30 | Fermatean probabilistic hesitant fuzzy weightedpower Bonferroni mean | FPHFWPBM |
| 31 | Fermatean probabilistic hesitant fuzzy probabilistic weighted power Bonferroni mean | FPHFPWPBM |
| 32 | Ordered weighted average | OWA |
| 33 | Fermatean probabilistic hesitant fuzzy average mean | FPHFAM |
| 34 | Fermatean probabilistic hesitant fuzzy weighted average | FPHFWA |
References
- Yeh, C.-H. A Problem-based Selection of Multi-attribute Decision-making Methods. Int. Trans. Oper. Res. 2022, 9, 169–181. [Google Scholar] [CrossRef]
- Dyer, J.S.; Fishburn, P.C.; Steuer, R.E.; Wallenius, J.; Zionts, S. Multiple criteria decision making, multiattribute utility theory: The next ten years. Manag. Sci. 1992, 38, 645–653. [Google Scholar] [CrossRef]
- Zadeh, L.A. Fuzzy sets. Inf. Control 1965, 8, 338–353. [Google Scholar] [CrossRef]
- Atanassov, K.T. Intuitionistic fuzzy sets. Fuzzy Sets Syst. 1986, 20, 87–96. [Google Scholar] [CrossRef]
- Zou, X.Y.; Chen, S.M.; Fan, K.Y. Multiple attribute decision making using improved intuitionistic fuzzy weighted geometric operators of intuitionistic fuzzy values. Inf. Sci. 2020, 535, 242–253. [Google Scholar] [CrossRef]
- Yang, W.; Jhang, S.T.; Shi, S.G.; Ma, Z.M. Aggregating intuitionistic fuzzy preference relations with symmetrical intuitionistic fuzzy Bonferroni mean operators in group decision making. Int. J. Fuzzy Syst. 2021, 23, 455–473. [Google Scholar] [CrossRef]
- Mahanta, J.; Panda, S. A novel distance measure for intuitionistic fuzzy sets with diverse applications. Int. J. Intell. Syst. 2021, 36, 615–627. [Google Scholar] [CrossRef]
- Singh, S.; Sharma, S.; Lalotra, S. Generalized correlation coefficients of intuitionistic fuzzy sets with application to MAGDM and clustering analysis. Int. J. Fuzzy Syst. 2020, 22, 1582–1595. [Google Scholar] [CrossRef]
- Zhang, Z. Interval-Valued Intuitionistic Hesitant Fuzzy Aggregation Operators and Their Application in Group Decision-Making. J. Appl. Math. 2013, 2013, 1–33. [Google Scholar] [CrossRef]
- Joshi, D.; Kumar, S. Interval-valued Intuitionistic HesitantFuzzy Choquet Integral Based TOPSIS Method for Multi-criteria Group Decision Making. Eur. J. Oper. Res. 2016, 248, 183–191. [Google Scholar] [CrossRef]
- Yager, R.R. Pythagorean membership grades in multicriteria decision making. IEEE Trans. Fuzzy Syst. 2013, 22, 958–965. [Google Scholar] [CrossRef]
- Haktanır, E. Interval valued pythagorean fuzzy aggregation operators based malcolm baldrige national quality award assessment. J. Intell. Fuzzy Syst. 2020, 39, 6431–6441. [Google Scholar] [CrossRef]
- Mu, Z.; Zeng, S.; Wang, P. Novel approach to multi-attribute group decision-making based on interval-valued Pythagorean fuzzy power Maclaurin symmetric mean operator. Comput. Ind. Eng. 2021, 155, 107049. [Google Scholar] [CrossRef]
- Pan, L.; Deng, Y.; Cheong, K.H. Quaternion model of Pythagorean fuzzy sets and its distance measure. Expert Syst. Appl. 2023, 213, 119222. [Google Scholar] [CrossRef]
- Ejegwa, P.A. Distance and similarity measures for Pythagorean fuzzy sets. Granul. Comput. 2020, 5, 225–238. [Google Scholar] [CrossRef]
- Kumar, K.; Chen, S.M. Group decision making based on entropy measure of Pythagorean fuzzy sets and Pythagorean fuzzy weighted arithmetic mean aggregation operator of Pythagorean fuzzy numbers. Inf. Sci. 2023, 624, 361–377. [Google Scholar] [CrossRef]
- Yanmaz, O.; Turgut, Y.; Can, E.N.; Kahraman, C. Interval-valued Pythagorean fuzzy EDAS method: An application to car selection problem. J. Intell. Fuzzy Syst. 2020, 38, 4061–4077. [Google Scholar] [CrossRef]
- Biswas, A.; Sarkar, B. Pythagorean fuzzy TOPSIS for multicriteria group decision-making with unknown weight information through entropy measure. Int. J. Intell. Syst. 2019, 34, 1108–1128. [Google Scholar] [CrossRef]
- Yager, R.R. Generalized orthopair fuzzy sets. IEEE Trans. Fuzzy Syst. 2016, 25, 1222–1230. [Google Scholar] [CrossRef]
- Liu, D.; Peng, D.; Liu, Z. The distance measures between q-rung orthopair hesitant fuzzy sets and their application in multiple criteria decision making. Int. J. Intell. Syst. 2019, 34, 2104–2121. [Google Scholar] [CrossRef]
- Khan, M.R.; Wang, H.; Ullah, K.; Karamti, H. Construction Material Selection by Using Multi-Attribute Decision Making Based on q-Rung Orthopair Fuzzy Aczel–Alsina Aggregation Operators. Appl. Sci. 2022, 12, 8537. [Google Scholar] [CrossRef]
- Khan, M.R.; Ullah, K.; Karamti, H.; Khan, Q.; Mahmood, T. Multi-attribute group decision-making based on q-rung orthopair fuzzy Aczel–Alsina power aggregation operators. Eng. Appl. Artif. Intell. 2023, 126, 106629. [Google Scholar] [CrossRef]
- Senapati, T.; Yager, R.R. Fermatean fuzzy sets. J. Ambient. Intell. Humaniz. Comput. 2020, 11, 663–674. [Google Scholar] [CrossRef]
- Seikh, M.R.; Mandal, U. Interval-valued Fermatean fuzzy Dombi aggregation operators and SWARA based PROMETHEE II method to bio-medical waste management. Expert Syst. Appl. 2023, 226, 120082. [Google Scholar] [CrossRef]
- Barokab, O.M.; Khan, A.; Khan, S.A.; Jun, Y.B.; Rushdi, A.M.A. University’s recruitment process using Fermatean fuzzy Einstein prioritized aggregation operators. J. Intell. Fuzzy Syst. 2023, 45, 3985–4008. [Google Scholar] [CrossRef]
- Rani, P.; Mishra, A.R. Fermatean fuzzy Einstein aggregation operators-based MULTIMOORA method for electric vehicle charging station selection. Expert Syst. Appl. 2021, 182, 115267. [Google Scholar] [CrossRef]
- Ganie, A.H. Multicriteria decision-making based on distance measures and knowledge measures of Fermatean fuzzy sets. Granul. Comput. 2022, 7, 979–998. [Google Scholar] [CrossRef]
- Deng, Z.; Wang, J. New distance measure for Fermatean fuzzy sets and its application. Int. J. Intell. Syst. 2022, 37, 1903–1930. [Google Scholar] [CrossRef]
- Attaullah; Rehman, N.; Khan, A.; Santos-García, G. Fermatean hesitant fuzzy rough aggregation operators and their applications in multiple criteria group decision-making. Sci. Rep. 2023, 13, 6676. [Google Scholar] [CrossRef]
- Gül, S. Fermatean fuzzy set extensions of SAW, ARAS, and VIKOR with applications in COVID-19 testing laboratory selection problem. Expert Syst. 2021, 38, e12769. [Google Scholar] [CrossRef]
- Torra, V. Hesitant fuzzy sets. Int. J. Intell. Syst. 2010, 25, 529–539. [Google Scholar] [CrossRef]
- Bahram, F. Information measures for hesitant fuzzy sets and interval-valued hesitant fuzzy sets. Inf. Sci. 2013, 240, 129–144. [Google Scholar]
- Chen, X.; Suo, C.; Li, Y. Distance measures on intuitionistic hesitant fuzzy set and its application in decision-making. Comput. Appl. Math. 2021, 40, 84. [Google Scholar] [CrossRef]
- Sajjad Ali Khan, M.; Ali, A.; Abdullah, S.; Amin, F.; Hussain, F. New extension of TOPSIS method based on Pythagorean hesitant fuzzy sets with incomplete weight information. J. Intell. Fuzzy Syst. 2018, 35, 5435–5448. [Google Scholar] [CrossRef]
- Ruan, C.Y.; Chen, X.J.; Gong, S.C.; Ali, S.; Almutairi, B. A decision-making framework based on the Fermatean hesitant fuzzy distance measure and TOPSIS. AIMS Math. 2024, 9, 2722–2755. [Google Scholar] [CrossRef]
- Lai, H.; Liao, H.; Long, Y.; Zavadskas, E.K. A hesitant Fermatean fuzzy CoCoSo method for group decision-making and an application to blockchain platform evaluation. Int. J. Fuzzy Syst. 2022, 24, 2643–2661. [Google Scholar] [CrossRef]
- Mishra, A.R.; Chen, S.M.; Rani, P. Multiattribute decision making based on Fermatean hesitant fuzzy sets and modified VIKOR method. Inf. Sci. 2022, 607, 1532–1549. [Google Scholar] [CrossRef]
- Liu, J.; Luo, S.H. Probabilistic hesitant Fermatean fuzzy extension MULTIMOORA method for evaluation of regional green restoration level. Control Decis. 2022, 37, 1–10. [Google Scholar]
- Ruan, C.Y.; Chen, X.J.; Han, L.N. Fermatean Hesitant Fuzzy Prioritized Heronian Mean Operator and Its Application in Multi-Attribute Decision Making. Comput. Mater. Contin. 2023, 75, 3203–3222. [Google Scholar] [CrossRef]
- Xu, Z.; Zhou, W. Consensus building with a group of decision makers under the hesitant probabilistic fuzzy environment. Fuzzy Optim. Decis. Mak. 2017, 16, 481–503. [Google Scholar] [CrossRef]
- Pang, Q.; Xu, Z.S.; Wang, H. Probabilistic linguistic term sets in multi-attribute group decision making. Inf. Sci. 2016, 369, 128–143. [Google Scholar] [CrossRef]
- He, Y.; Xu, Z.S.; Jiang, W.L. Probabilistic interval reference ordering sets in multi-criteria group decision making. Int. J. Uncertain. Fuzziness Knowl.-Based Syst. 2017, 25, 189–212. [Google Scholar] [CrossRef]
- Zhai, Y.; Xu, Z.; Liao, H. Measures of probabilistic interval-valued intuitionistic hesitant fuzzy sets and the application in reducing excessive medical examinations. IEEE Trans. Fuzzy Syst. 2017, 26, 1651–1670. [Google Scholar] [CrossRef]
- Ruan, C.; Chen, X. Probabilistic Interval-Valued Fermatean Hesitant Fuzzy Set and Its Application to Multi-Attribute Decision Making. Axioms 2023, 12, 979. [Google Scholar] [CrossRef]
- Ren, Y.; Yuan, X.; Zhao, X.; Yu, B. Calculation and Aggregation of Q-rung Orthopair Probabilistic Hesitant Fuzzy Information. In Proceedings of the 2021 IEEE 5th Advanced Information Technology, Electronic and Automation Control Conference (IAEAC), Chongqing, China, 12–14 March 2021. [Google Scholar]
- Chen, Z.; Shen, D.; Yu, F.; Tang, X.; Zhang, Z. Multi-attribute decision-making method based on q-rung orthopair probabilistic hesitant fuzzy schweizer-sklar power weighted hamy mean operator. PLoS ONE 2023, 18, e0266779. [Google Scholar] [CrossRef]
- Chen, Z.; Shen, D.; Ren, Y.; Yu, F.; Yuan, X. Airspace Operation Effectiveness Evaluation Based on q-Rung Orthopair Probabilistic Hesitant Fuzzy GRA and TOPSIS. Symmetry 2022, 14, 242. [Google Scholar] [CrossRef]
- Attaullah; Ashraf, S.; Rehman, N.; Khan, A. q-Rung Orthopair Probabilistic Hesitant Fuzzy Rough Aggregation Information and Their Application in Decision Making. Int. J. Fuzzy Syst. 2023, 25, 2067–2080. [CrossRef]
- Bushra, B.; Mumtaz, A.; Saleem, A.; Shahzaib, A.; Ronnason, C. Entropy Based Pythagorean Probabilistic Hesitant Fuzzy Decision Making Technique and Its Application for Fog-Haze Factor Assessment Problem. Entropy 2020, 22, 318. [Google Scholar] [CrossRef]
- Qahtan, S.; Alsattar, H.A.; Zaidan, A.A.; Deveci, M.; Pamucar, D.; Delen, D.; Pedrycz, W. Evaluation of agriculture-food 4.0 supply chain approaches using Fermatean probabilistic hesitant-fuzzy sets based decision making model. Appl. Soft Comput. 2023, 138, 110170. [Google Scholar] [CrossRef]
- Yager, R.R. The power average operator. IEEE Trans. Syst. Man Cybern. 2001, 31, 724–731. [Google Scholar] [CrossRef]
- Xu, Z.; Yager, R.R. Power-geometric operators and their use in group decision making. IEEE Trans. Fuzzy Syst. 2010, 18, 94–105. [Google Scholar]
- Zhang, Z. Hesitant fuzzy Power aggregation operators and their application to multiple attribute group decision making. Inf. Sci. 2013, 234, 150–181. [Google Scholar] [CrossRef]
- Xu, Z.S. Approaches to multiple attribute group decision making based on intuitionistic fuzzy power aggregation operators. Knowl.-Based Syst. 2011, 24, 749–760. [Google Scholar] [CrossRef]
- Xu, Z.S.; Cai, X.Q. Uncertain power average operator average operators for aggregating interval fuzzy decision and negotiation. Group Decis. Negot. 2012, 21, 381–397. [Google Scholar] [CrossRef]
- He, X.; Du, Y.X.; Liu, W.F. Pythagorean Fuzzy Power Average Operators. Fuzzy Syst. Math. 2016, 30, 116–124. [Google Scholar]
- Yager, R.R. On generalized Bonferroni mean operators for multi-criteria aggregation. Int. J. Approx. Reason. 2009, 50, 1279–1286. [Google Scholar] [CrossRef]
- Beliakov, G.; James, S.; Mordelva, J. Generalized Bonferroni mean operators in multi-criteria aggregation. Fuzzy Sets Syst. 2010, 161, 2227–2242. [Google Scholar] [CrossRef]
- Xu, Z.S. Uncertain Bonferroni means operators. Int. J. Intell. Syst. 2010, 3, 761–769. [Google Scholar]
- Xu, Z.S.; Yager, R.R. Intuitionistic fuzzy Bonferroni means. IEEE Trans. Syst. Man Cybern. B Cybern. 2011, 41, 568–578. [Google Scholar]
- He, Y.; He, Z.; Wang, G.; Chen, H. Hesitant fuzzy power Bonferroni means and their application to multiple attribute decision making. IEEE Trans. Fuzzy Syst. 2015, 23, 1655–1668. [Google Scholar] [CrossRef]
- He, Y.D.; He, Z.; Jin, C.; Chen, H.Y. Intuitionistic fuzzy power geometric Bonferroni means and their application to multiple attribute group decision making. Int. J. Uncertain. Fuzziness Knowl.-Based Syst. 2015, 23, 285–315. [Google Scholar] [CrossRef]
- Liu, P.; Li, H. Interval-Valued Intuitionistic Fuzzy Power Bonferroni Aggregation Operators and Their Application to Group Decision Making. Cogn. Comput. 2017, 9, 494–512. [Google Scholar] [CrossRef]
- Liu, P.; Liu, X. Multi-attribute group decision making methods based on linguistic intuitionistic fuzzy power Bonferroni mean operators. Complexity 2017, 2017, 1–15. [Google Scholar]
- Luo, D.; Zeng, S. Pythagorean Fuzzy Power Bonferroni Aggregation Operators and Their Application in Decision Making. Comput. Eng. Appl. 2020, 56, 58–65. [Google Scholar]
- Ruan, C.; Chen, X.; Zeng, S.; Ali, S.; Almutairi, B. Fermatean fuzzy power Bonferroni aggregation operators and their applications to multi-attribute decision-making. Soft Comput. 2024, 28, 191–203. [Google Scholar] [CrossRef]
- Qu, G.; Zhang, Z.; Qu, W.; Xu, Z. Green Supplier Selection Based on Green Practices Evaluated Using Fuzzy Approaches of TOPSIS and ELECTRE with a Case Study in a Chinese Internet Company. Int. J. Environ. Res. Public Health 2020, 17, 3268. [Google Scholar] [CrossRef]
- Konys, A. Green Supplier Selection Criteria: From a Literature Review to a Comprehensive Knowledge Base. Sustainability 2019, 11, 4208. [Google Scholar] [CrossRef]
- Qian, Y.; Hou, F. An approach for green supplier selection in the automobile manufacturing industry. Kybernetes 2016, 45, 571–588. [Google Scholar]
- Deng, X.; Hu, Y.; Deng, Y.; Mahadevan, S. Supplier selection using AHP methodology extended by D numbers. Expert Syst. Appl. 2014, 41, 156–167. [Google Scholar] [CrossRef]
- Boran, F.E.; Genç, S.; Kurt, M.; Akay, D. A multi-criteria intuitionistic fuzzy group decision making for supplier selection with TOPSIS method. Expert Syst. Appl. 2009, 36, 11363–11368. [Google Scholar] [CrossRef]
- Liang, D.; Xu, Z. The new extension of TOPSIS method for multiple criteria decision making with hesitant Pythagorean fuzzy sets. Appl. Soft Comput. 2017, 60, 167–179. [Google Scholar] [CrossRef]
- Zhu, B.; Xu, Z.; Xia, M. Dual Hesitant Fuzzy Sets. J. Appl. Math. 2012, 2012, 1–13. [Google Scholar] [CrossRef]
- Srivastava, S. Green Supply Chain Management: A State-of-The-Art Literature Review. Int. J. Manag. Rev. 2007, 9, 53–80. [Google Scholar] [CrossRef]
- Gupta, S.; Soni, U.; Kumar, G. Green supplier selection using multi-criterion decision making under fuzzy environment: A case study in automotive industry. Comput. Ind. Eng. 2019, 136, 663–680. [Google Scholar] [CrossRef]
- Wang, S.; Ji, Y.; Wahab, M.I.M.; Xu, D.; Zhou, C. A New Decision Framework of Online Multi-Attribute Reverse Auctions for Green Supplier Selection under Mixed Uncertainty. Sustainability 2022, 14, 16879. [Google Scholar] [CrossRef]
- Kumar Kar, A.; K. Pani, A. Exploring the importance of different supplier selection criteria. Manag. Res. Rev. 2014, 37, 89–105. [Google Scholar] [CrossRef]
- Shah, N.; Shah, P.; Patel, M. Pricing Decisions with Effect of Advertisement and Greening Efforts for a Greengocer. Sustainability 2022, 14, 13807. [Google Scholar] [CrossRef]
- Wang, K.-Q.; Liu, H.-C.; Liu, L.; Huang, J. Green Supplier Evaluation and Selection Using Cloud Model Theory and the QUALIFLEX Method. Sustainability 2017, 9, 688. [Google Scholar] [CrossRef]
- Yadlapalli, A.; Rahman, S.; Gunasekaran, A. Socially responsible governance mechanisms for manufacturing firms in apparel supply chains. Int. J. Prod. Econ. 2017, 196, 135–149. [Google Scholar] [CrossRef]
- Ruan, C.; Chen, X.; Yan, L. Fermatean hesitant fuzzy multi-attribute decision-making method with probabilistic information and its application. Axioms 2024, 13, 456. [Google Scholar] [CrossRef]



| <{0.6/0.4, 0.7/0.6}, {0.1/0.7, 0.3/0.3}> | <{0.5/0.8, 0.7/0.2}, {0.3/0.3, 0.4/0.7}> | <{0.5/0.5, 0.7/0.3, 0.8/0.2}, {0.4/1}> | <{0.7/0.5, 0.8/0.5}, {0.3/0.4, 0.5/0.6}> | |
| <{0.5/0.6, 0.8/0.4}, {0.2/0.5, 0.3/0.5}> | <{0.4/0.3, 0.6/0.4, 0.7/0.3}, {0.2/1}> | <{0.3/0.6, 0.6/0.4}, {0.2/0.7, 0.4/0.3}> | <{0.5/0.4, 0.8/0.6}, {0.1/0.6, 0.2/0.4}> | |
| <{0.5/0.6, 0.7/0.3, 0.9/0.1}, {0.2/1}> | <{0.7/0.5, 0.8/0.5}, {0.2/0.6, 0.3/0.4}> | <{0.6/0.3, 0.8/0.7}, {0.2/0.4, 0.3/0.6}> | <{0.7/0.6, 0.9/0.4}, {0.3/0.7, 0.4/0.3}> | |
| <{0.6/0.8, 0.8/0.2}, {0.3/0.6, 0.4/0.4}> | <{0.5/0.3, 0.6/0.7}, {0.1/0.4, 0.4/0.6}> | <{0.5/0.5, 0.7/0.5}, {0.1/0.6, 0.3/0.4}> | <{0.8/1}, {0.2/0.7, 0.5/0.2, 0.6/0.1}> |
| <{0.6/0.4, 0.7/0.6}, {0.1/0.7, 0.3/0.3}> | <{0.5/0.8, 0.7/0.2}, {0.3/0.3, 0.4/0.7}> | <{0.5/0.5, 0.7/0.3, 0.8/0.2}, {0.4/1}> | <{0.3/0.4, 0.5/0.6}, {0.7/0.5, 0.8/0.5}> | |
| <{0.5/0.6, 0.8/0.4}, {0.2/0.5, 0.3/0.5}> | <{0.4/0.3, 0.6/0.4, 0.7/0.3}, {0.2/1}> | <{0.3/0.6, 0.6/0.4}, {0.2/0.7, 0.4/0.3}> | <{0.1/0.6, 0.2/0.4}, {0.5/0.4, 0.8/0.6}> | |
| <{0.5/0.6, 0.7/0.3, 0.9/0.1}, {0.2/1}> | <{0.7/0.5, 0.8/0.5}, {0.2/0.6, 0.3/0.4}> | <{0.6/0.3, 0.8/0.7}, {0.2/0.4, 0.3/0.6}> | <{0.3/0.7, 0.4/0.3}, {0.7/0.6, 0.9/0.4}> | |
| <{0.6/0.8, 0.8/0.2}, {0.3/0.6, 0.4/0.4}> | <{0.5/0.3, 0.6/0.7}, {0.1/0.4, 0.4/0.6}> | <{0.5/0.5, 0.7/0.5}, {0.1/0.6, 0.3/0.4}> | <{0.2/0.7, 0.5/0.2, 0.6/0.1}, {0.8/1}> |
| <{0.6/0.2, 0.6/0.2, 0.7/ 0.6}, {0.1/0.7, 0.3/0.3}> | <{0.5/0.4, 0.5/0.4, 0.7/ 0.2}, {0.3/0.3, 0.4/0.7}> | <{0.5/0.5, 0.7/0.3, 0.8/ 0.2}, {0.4/0.5, 0.4/0.5}> | <{0.3/0.2, 0.3/0.2, 0.5/ 0.6}, {0.7/0.5, 0.8/0.5}> | |
| <{0.5/0.3, 0.5/0.3, 0.8/ 0.4}, {0.2/0.5, 0.3/0.5}> | <{0.4/0.3, 0.6/0.4, 0.7/ 0.3}, {0.2/0.5, 0.2/0.5}> | <{0.3/0.3, 0.3/0.3, 0.6/ 0.4}, {0.2/0.7, 0.4/0.3}> | <{0.1/0.3, 0.1/0.3, 0.2/ 0.4}, {0.5/0.6, 0.8/0.4}> | |
| <{0.5/0.6, 0.7/0.3, 0.9/ 0.1}, {0.2/0.5, 0.2/0.5}> | <{0.7/0.25, 0.7/0.25, 0.8/ 0.5}, {0.2/0.6, 0.3/0.4}> | <{0.6/0.15, 0.6/0.15, 0.8/ 0.7}, {0.2/0.4, 0.3/0.6}> | <{0.3/0.35, 0.3/0.35, 0.4/ 0.3}, {0.7/0.6, 0.9/0.4}> | |
| <{0.6/0.4, 0.6/0.4, 0.8/ 0.2}, {0.3/0.6, 0.4/0.4}> | <{0.5/0.15, 0.5/0.15, 0.6/ 0.7}, {0.1/0.4, 0.4/0.6}> | <{0.5/0.25, 0.5/0.25, 0.7/ 0.5}, {0.1/0.6, 0.3/0.4}> | <{0.2/0.7, 0.5/0.2, 0.6/ 0.1}, {0.8/0.5, 0.8/0.5}> |
| Parameter Value | S1 | S2 | S3 | S4 | Ranking |
|---|---|---|---|---|---|
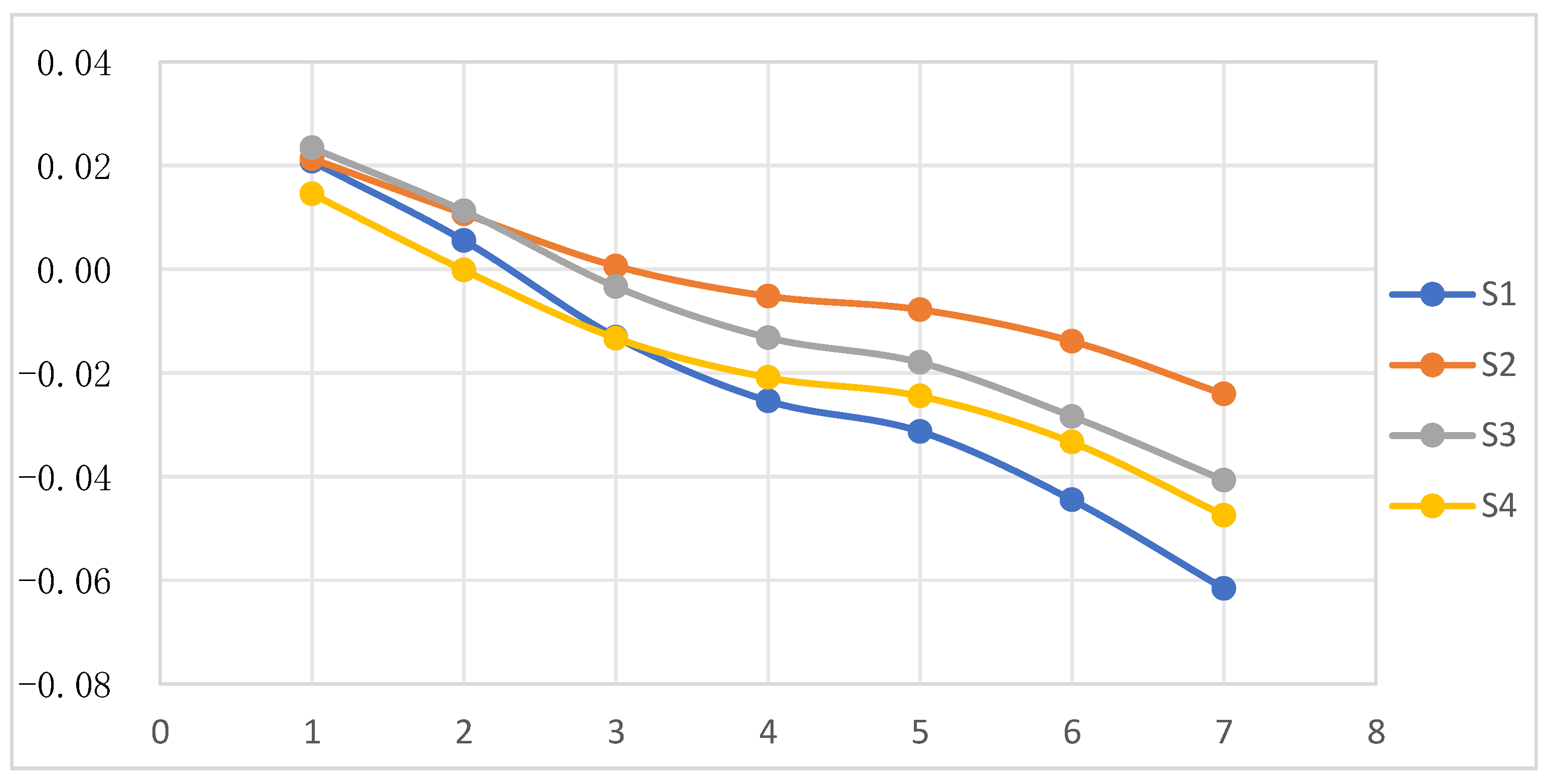
| p = q = 0.3 | 0.0208 | 0.0213 | 0.0234 | 0.0145 | |
| p = q = 0.7 | 0.0055 | 0.0107 | 0.0112 | −0.0002 | |
| p = q = 1.5 | −0.0131 | 0.0006 | −0.0034 | −0.0133 | |
| p = q = 2.4 | −0.0254 | −0.0052 | −0.0132 | −0.0209 | |
| p = q = 3 | −0.0313 | −0.0078 | −0.0180 | −0.0245 | |
| p = q = 5 | −0.0445 | −0.0139 | −0.0284 | −0.0334 | |
| p = q = 10 | −0.0616 | −0.0241 | −0.0407 | −0.0475 |
| Parameter Value | S1 | S2 | S3 | S4 | Ranking |
|---|---|---|---|---|---|
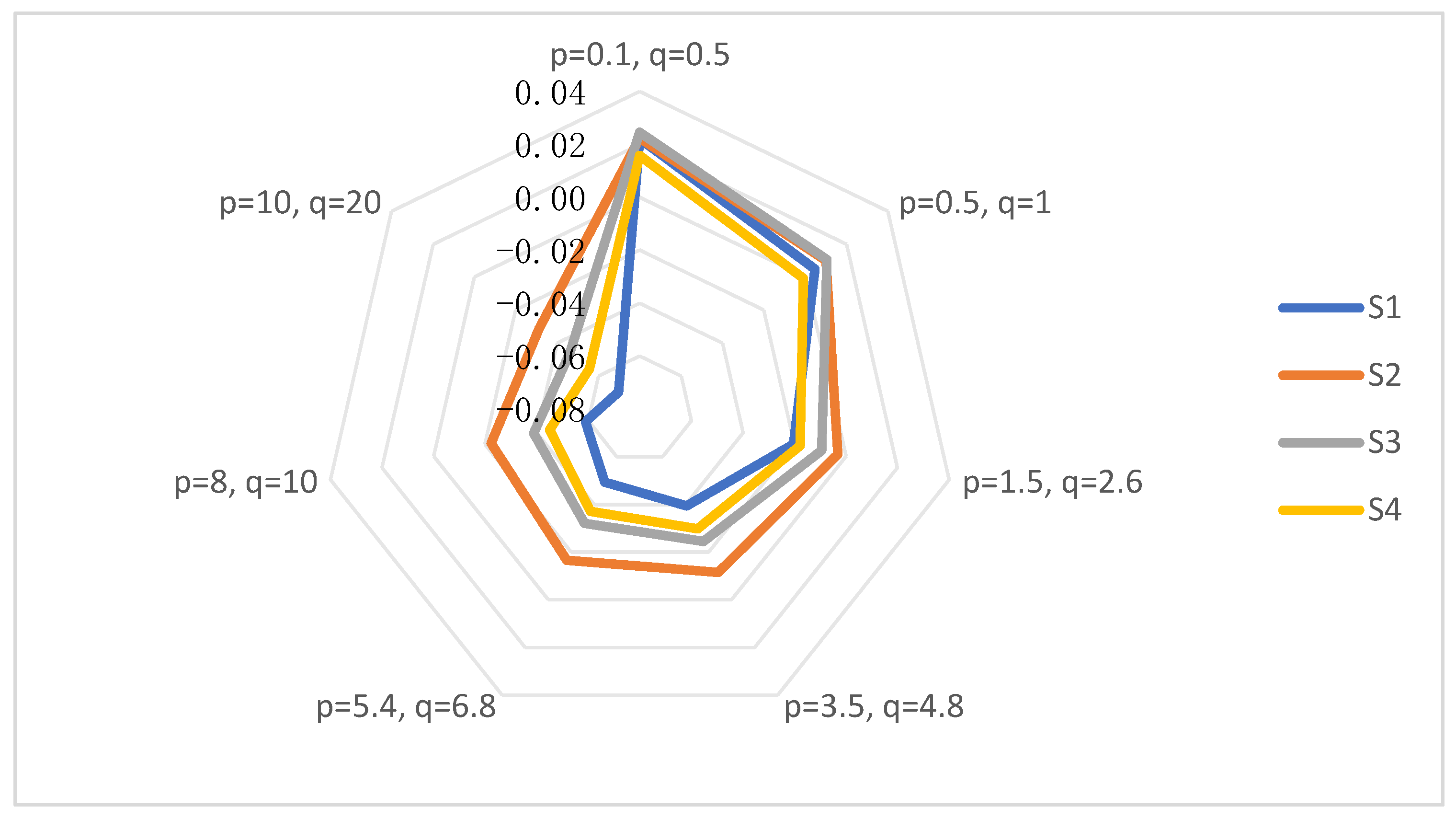
| p = 0.1, q = 0.5 | 0.0222 | 0.0224 | 0.0246 | 0.0158 | |
| p = 0.5, q = 1 | 0.0047 | 0.0100 | 0.0105 | −0.0009 | |
| p = 1.5, q = 2.6 | −0.0204 | −0.0032 | −0.0094 | −0.0178 | |
| p = 3.5, q = 4.8 | −0.0394 | −0.0115 | −0.0245 | −0.0298 | |
| p = 5.4, q = 6.8 | −0.0493 | −0.0165 | −0.0320 | −0.0370 | |
| p = 8, q = 10 | −0.0589 | −0.0223 | −0.0388 | −0.0450 | |
| p = 10, q = 20 | −0.0697 | −0.0312 | −0.0460 | −0.0557 |
| Operator | Considering Correlation of Attributes | Considering External Probabilistic Information | Considering Internal Probabilistic Information |
|---|---|---|---|
| FHFWPBM | + | − | − |
| FHFPWPBM | + | + | − |
| FPHFWPBM | + | − | + |
| FPHFPWPBM | + | + | + |
| FHFPA [81] | − | + | − |
| FHFOWA [81] | − | − | − |
| FHFPOWA [81] | − | + | − |
| GFHFPOWA [81] | − | + | − |
| FPHFAM [50] | − | − | + |
| FPHFWM [50] | − | − | + |
| Operator | S1 | S2 | S3 | S4 | Ranking |
|---|---|---|---|---|---|
| FHFWPBM | 0.0582 | 0.0607 | 0.1895 | 0.0883 | |
| FHFPWPBM | 0.0881 | 0.0710 | 0.1777 | 0.0577 | |
| FPHFWPBM | −0.0063 | 0.0035 | 0.0058 | −0.0027 | |
| FPHFPWPBM | −0.0028 | 0.0059 | 0.0047 | −0.0065 | |
| FHFPA [81] | 0.1602 | 0.0944 | 0.2716 | 0.1666 | |
| FHFOWA [81] | 0.2015 | 0.1867 | 0.3394 | 0.2094 | |
| FHFPOWA [81] | 0.1970 | 0.1526 | 0.3059 | 0.1982 | |
| GFHFPOWA [81] | −0.1203 | −0.1226 | 0.0211 | −0.0749 | |
| FPHFAM [50] | −0.0728 | −0.0427 | −0.0612 | −0.0636 | |
| FPHFWM [50] | −0.0518 | −0.0335 | −0.0483 | −0.0634 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ruan, C.; Yan, L. Fermatean Probabilistic Hesitant Fuzzy Power Bonferroni Aggregation Operators with Dual Probabilistic Information and Their Application in Green Supplier Selection. Axioms 2024, 13, 602. https://doi.org/10.3390/axioms13090602
Ruan C, Yan L. Fermatean Probabilistic Hesitant Fuzzy Power Bonferroni Aggregation Operators with Dual Probabilistic Information and Their Application in Green Supplier Selection. Axioms. 2024; 13(9):602. https://doi.org/10.3390/axioms13090602
Chicago/Turabian StyleRuan, Chuanyang, and Lin Yan. 2024. "Fermatean Probabilistic Hesitant Fuzzy Power Bonferroni Aggregation Operators with Dual Probabilistic Information and Their Application in Green Supplier Selection" Axioms 13, no. 9: 602. https://doi.org/10.3390/axioms13090602
APA StyleRuan, C., & Yan, L. (2024). Fermatean Probabilistic Hesitant Fuzzy Power Bonferroni Aggregation Operators with Dual Probabilistic Information and Their Application in Green Supplier Selection. Axioms, 13(9), 602. https://doi.org/10.3390/axioms13090602






