Real and Complex Potentials as Solutions to Planar Inverse Problem of Newtonian Dynamics
Abstract
1. Introduction
- (i)
- Potentials that satisfy the two-dimensional wave equation, i.e., = 0.
- (ii)
- Potentials that satisfy the two-dimensional Laplace equation, i.e., = 0.
- (iii)
- Separable potentials of the from , where are arbitrary functions of the -class, which satisfy the condition 0. They were used by [19].
- (iv)
- Polynomial potentials of the third degree, .
2. The Mathematical Setup
3. The 2D Wave Equation
Two Conditions on the Family of Orbits
4. The 2D Laplace Equation
Two Conditions on the Family of Orbits
- 1. “Plan A”.If the conditions (48) are satisfied for the given family of orbits (1), then we reconsider the Equation (47) and we setWe solve analytically each part of the relations (49). First, taking into account 0, we set and we obtainwith the general solutionThe differential conditions (48) are the differential conditions for the slope function , which, if they are satisfied, ensure the existence of a potential (41). On the other hand, we consider that the conditions (48) are satisfied by the slope function . Besides that, we have . Then, we obtain and we determine the function F from (52).Working in a similar way for the function , we set and, with the aid of (49), we obtain the O.D.E.or, equivalently, assuming ,The general solution of (54) isFinally, the function is found to beAs a conclusion, combining the results (52) and (56), we can find the potential function V by quadratures, i.e., . Now, we can formulate the next one.
- 2. “Plan B”.If the conditions (48) are not satisfied for the given family of orbits (1), then we refer to the Equation (47) and we use “the method of the determination of coefficients”. More precisely, since the functions are twice differentiable, we consider that the functions are polynomials of the second degree and we setwhere are const. Then, we are searching for suitable values of these parameters such that the relations (47) are satisfied.
5. Separable Potentials
6. Polynomial Potentials
- Case 1. . In addition to , this choice leads to 0, which gives the trivial solution.
- Case 2. . This choice leads to . If we set , then we obtain and finally we determine the coefficient . It is 3. As a conclusion, the potential takes the formwhich produces the monoparametric family of orbits
- Case 3. . This choice leads to . If we set , then we obtain and we find the coefficient at last. It is 3. As a conclusion, the potential iswhich produces the monoparametric family of orbits
7. Families of Straight Lines
- I. , where .
- II. , where and .
- III. .
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Szebehely, V. On the determination of the potential by satellite observations. Convegno Internazionale Sulla Rotazione Della Terra Oss. Satelliti Artif. 1974, 44, 31–35. [Google Scholar]
- Bozis, G. Generalization of Szebehely’s Equation. Celest. Mech. 1983, 29, 329–334. [Google Scholar] [CrossRef]
- Puel, F. Intrinsic formulation of the equation of Szebehely. Celest. Mech. 1984, 32, 209–216. [Google Scholar] [CrossRef]
- Bozis, G.; Tsarouhas, G. Conservative fields derived from two monoparametric families of planar orbits. Astron. Astrophys. 1985, 145, 215–220. [Google Scholar]
- Bozis, G. Szebehely’s inverse problem for finite symmetrical material concentrations. Astron. Astrophys. 1984, 134, 360–364. [Google Scholar]
- Bozis, G.; Grigoriadou, S. Families of planar orbits generated by homogeneous potentials. Celest. Mech. Dyn. Astr. 1993, 57, 461–472. [Google Scholar] [CrossRef]
- Bozis, G.; Anisiu, M.-C.; Blaga, C. Inhomogeneous potentials producing homogeneous orbits. Astron. Nach. 1997, 318, 313–318. [Google Scholar] [CrossRef]
- Bozis, G.; Stefiades, A. Geometrically similar orbits in homogeneous potentials. Inverse Probl. 1993, 9, 233–240. [Google Scholar] [CrossRef]
- Bozis, G.; Ichtiaroglou, S. Boundary Curves for Families of Planar Orbits. Celest. Mech. Dyn. Astr. 1993, 58, 371–385. [Google Scholar] [CrossRef]
- Bozis, G. The inverse problem of dynamics: Basic facts. Inverse Probl. 1995, 11, 687–708. [Google Scholar] [CrossRef]
- Anisiu, M.-C. An alternative point of view on the equations of the inverse problem of dynamics. Inverse Probl. 2004, 20, 1865–1872. [Google Scholar] [CrossRef]
- Bozis, G.; Anisiu, M.-C. A solvable version of the inverse problem of dynamics. Inverse Probl. 2005, 21, 487–497. [Google Scholar] [CrossRef]
- Grigoriadou, S.; Bozis, G.; Elmabsout, B. Solvable cases of Szebehely’s equation. Celest. Mech. Dyn. Astr. 1999, 74, 211–221. [Google Scholar] [CrossRef]
- Anisiu, M.-C.; Bozis, G.; Blaga, C. Special families of orbits in the direct problem of dynamics. Celest. Mech. Dyn. Astr. 2004, 88, 245–257. [Google Scholar] [CrossRef]
- Blaga, C.; Anisiu, M.-C.; Bozis, G. New solutions in the direct problem of dynamics. PADEU 2006, 17, 13. [Google Scholar]
- Bozis, G.; Anisiu, M.-C.; Blaga, C. A solavable version of the direct problem of dynamics. Rom. Astron. J. 2000, 10, 59–70. [Google Scholar]
- Bozis, G.; Anisiu, M.-C. Families of straight lines in planar potentials. Rom. Astron. J. 2001, 11, 27–43. [Google Scholar]
- Kotoulas, T. Monoparametric families of orbits produced by planar potentials. Axioms 2023, 12, 423. [Google Scholar] [CrossRef]
- Ichtiaroglou, S.; Meletlidou, E. On monoparametric families of orbits sufficient for integrability of planar potentials with linear or quadratic invariants. J. Phys. A. Math. Gen. 1990, 23, 3673–3679. [Google Scholar] [CrossRef]
- Ramani, A.; Dorizzi, B.; Grammaticos, B. Painlevé conjecture revisited. Phys. Rev. Let. 1982, 49, 1539–1541. [Google Scholar] [CrossRef]
- Kotoulas, T. Families of orbits produced by three-dimensional central and polynomial potentials: An application to the 3D harmonic oscillator. Axioms 2023, 12, 461. [Google Scholar] [CrossRef]
- Kotoulas, T. 3D homogeneous potentials generating two-parametric families of orbits on the outside of a material concentration. Eur. Phys. J. Plus 2023, 138, 124. [Google Scholar] [CrossRef]
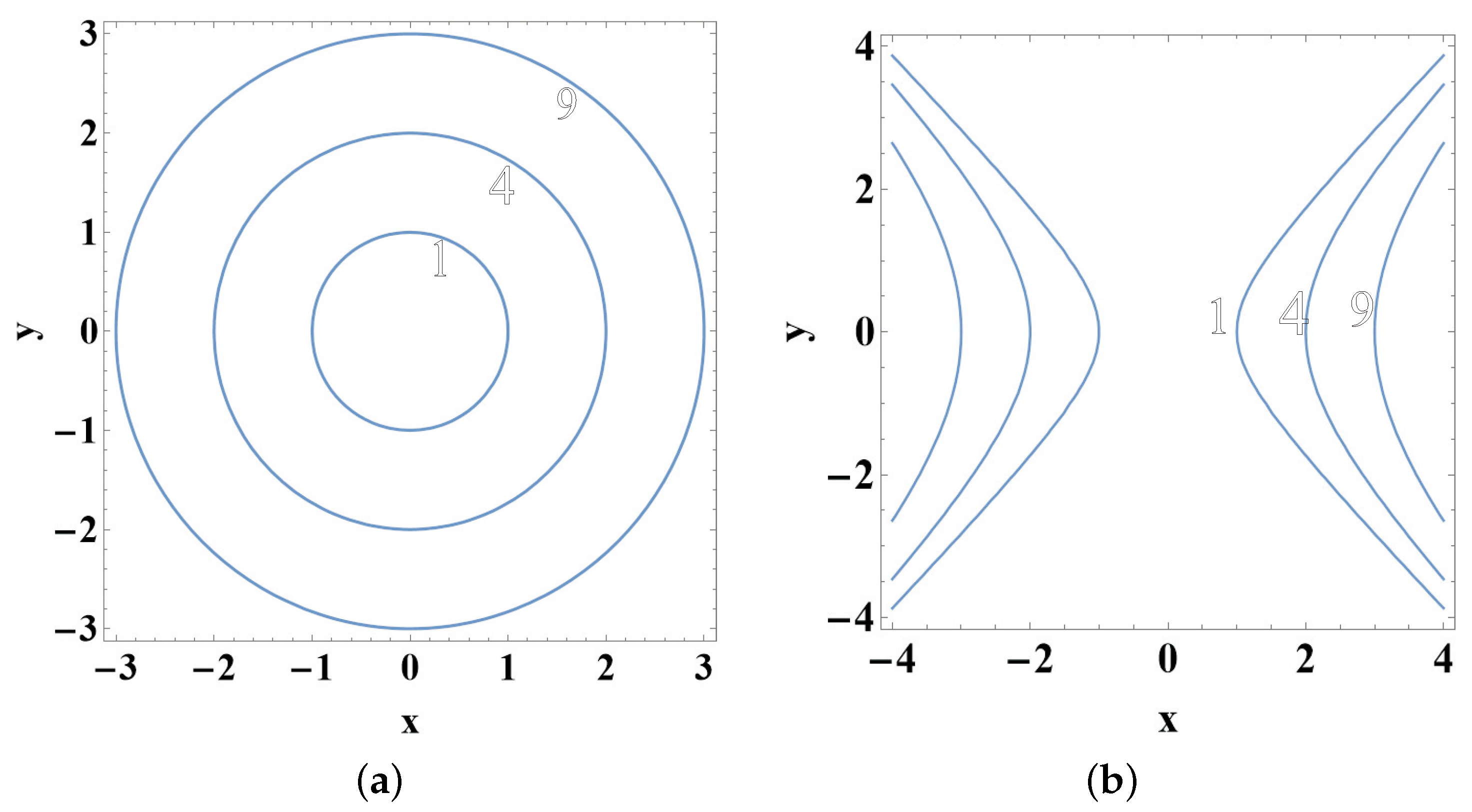
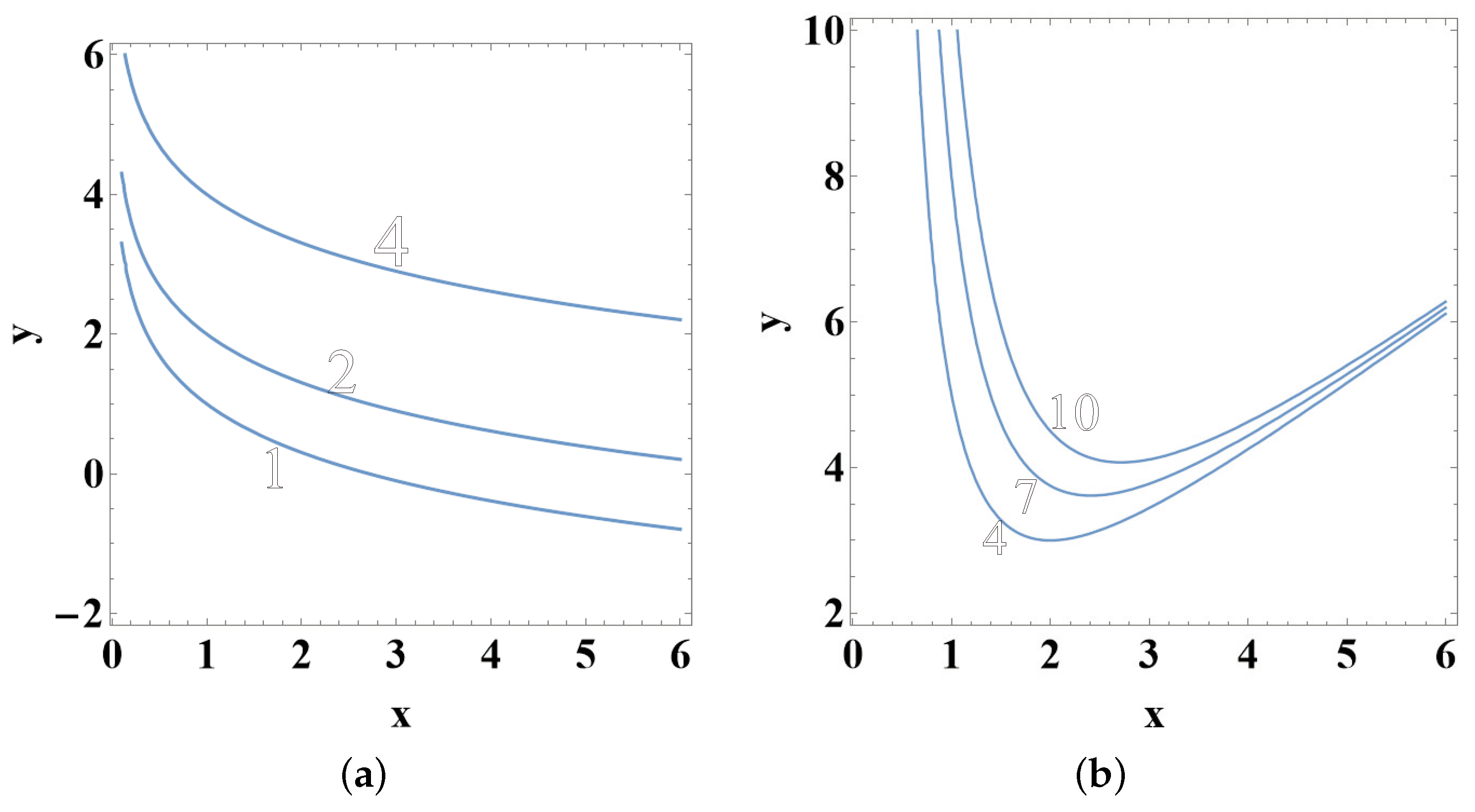
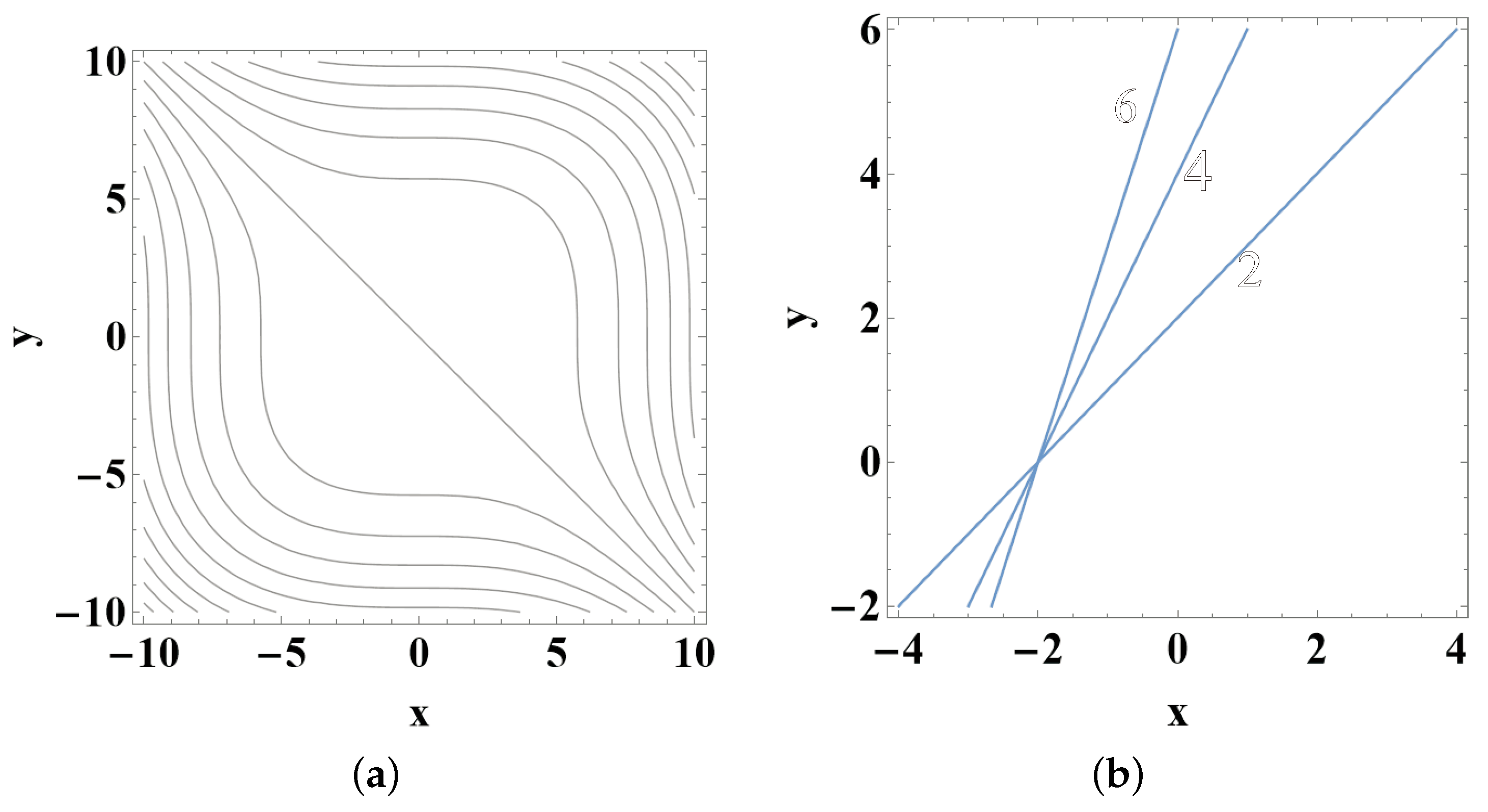
| Family of Orbits | Potential V (x, y) | Energy |
|---|---|---|
| Family of Orbits | Potential V (x, y) | Energy |
|---|---|---|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kotoulas, T. Real and Complex Potentials as Solutions to Planar Inverse Problem of Newtonian Dynamics. Axioms 2024, 13, 88. https://doi.org/10.3390/axioms13020088
Kotoulas T. Real and Complex Potentials as Solutions to Planar Inverse Problem of Newtonian Dynamics. Axioms. 2024; 13(2):88. https://doi.org/10.3390/axioms13020088
Chicago/Turabian StyleKotoulas, Thomas. 2024. "Real and Complex Potentials as Solutions to Planar Inverse Problem of Newtonian Dynamics" Axioms 13, no. 2: 88. https://doi.org/10.3390/axioms13020088
APA StyleKotoulas, T. (2024). Real and Complex Potentials as Solutions to Planar Inverse Problem of Newtonian Dynamics. Axioms, 13(2), 88. https://doi.org/10.3390/axioms13020088






