Semi-Hyers–Ulam–Rassias Stability of Some Volterra Integro-Differential Equations via Laplace Transform
Abstract
1. Introduction
2. Preliminary Notions and Results
3. Main Results
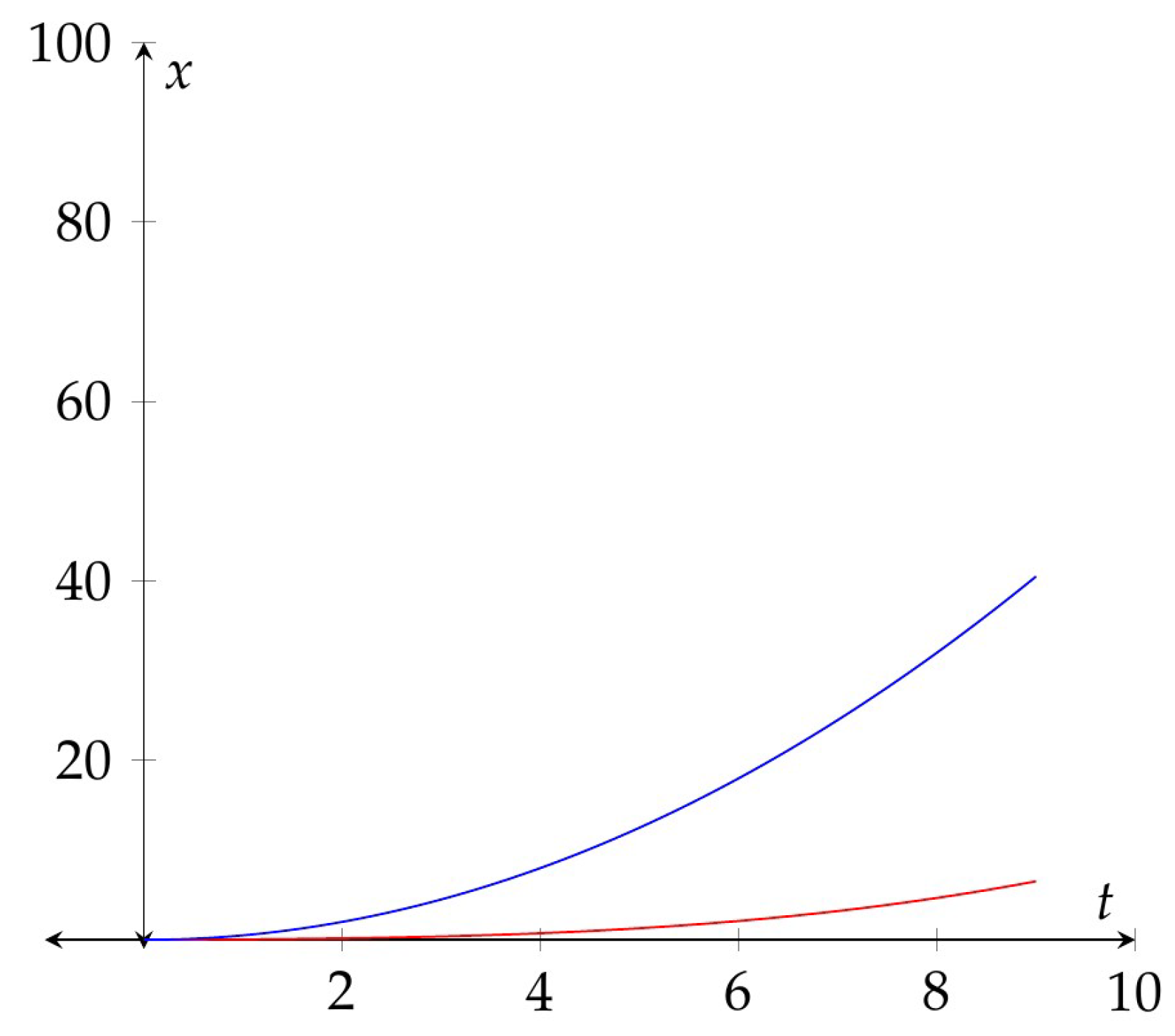
4. Study of Other Equations via Double Laplace Transform
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ulam, S.M. A Collection of Mathematical Problems; Interscience: New York, NY, USA, 1960. [Google Scholar]
- Hyers, D.H. On the stability of the linear functional equation. Proc. Natl. Acad. Sci. USA 1941, 27, 222–224. [Google Scholar] [CrossRef]
- Obloza, M. Hyers stability of the linear differential equation. Rocznik Nauk-Dydakt. Prace Mat. 1993, 13, 259–270. [Google Scholar]
- Alsina, C.; Ger, R. On some inequalities and stability results related to exponential function. J. Inequal. Appl. 1998, 2, 373–380. [Google Scholar] [CrossRef]
- Takahasi, S.E.; Takagi, H.; Miura, T.; Miyajima, S. The Hyers-Ulam stability constant of first order linear differential operators. J. Math. Anal. Appl. 2004, 296, 403–409. [Google Scholar] [CrossRef]
- Jung, S.-M. Hyers-Ulam stability of linear differential equations of first order, III. J. Math. Anal. Appl. 2005, 311, 139–146. [Google Scholar] [CrossRef]
- Cimpean, D.S.; Popa, D. On the stability of the linear differential equation of higher order with constant coefficients. Appl. Math. Comput. 2010, 217, 4141–4146. [Google Scholar] [CrossRef]
- Popa, D.; Rasa, I. Hyers-Ulam stability of the linear differential operator with non-constant coefficients. Appl. Math. Comput. 2012, 219, 1562–1568. [Google Scholar]
- Novac, A.; Otrocol, D.; Popa, D. Ulam stability of a linear difference equation in locally convex spaces. Results Math. 2021, 76, 33. [Google Scholar] [CrossRef]
- Brzdek, J.; Popa, D.; Rasa, I.; Xu, B. Ulam Stability of Operators; Elsevier: Amsterdam, The Netherlands, 2018. [Google Scholar]
- Jung, S.-M. A fixed point approach to the stability of a Volterra integral equation. Fixed Point Theory Appl. 2007, 2007, 57064. [Google Scholar] [CrossRef]
- Cadariu, L.; Radu, V. On the stability of the Cauchy functional equation: A fixed point approach. In Iteration Theory (ECIT ’02); Vol. 346 of Grazer Mathematische Berichte; Karl-Franzens-Univ. Graz: Graz, Austria, 2004; pp. 43–52. [Google Scholar]
- Castro, L.P.; Ramos, A. Hyers-Ulam-Rassias stability for a class of nonlinear Volterra integral equations. Banach J. Math. Anal. 2009, 3, 36–43. [Google Scholar]
- Castro, L.P.; Simões, A.M. Hyers-Ulam and Hyers-Ulam-Rassias stability of a class of Hammerstein integral equations. AIP Conf. Proc. 2017, 1798, 020036. [Google Scholar] [CrossRef]
- Castro, L.P.; Simões, A.M. Hyers-Ulam-Rassias stability of nonlinear integral equations through the Bielecki metric. Math. Methods Appl. Sci. 2018, 41, 7367–7383. [Google Scholar] [CrossRef]
- Castro, L.P.; Simões, A.M. Different Types of Hyers-Ulam-Rassias Stabilities for a Class of Integro-Differential Equations. Filomat 2017, 31, 5379–5390. [Google Scholar] [CrossRef]
- Simões, A.M.; Carapau, F.; Correia, P. New sufficient conditions to Ulam stabilities for a class of higher order integro-differential equations. Symmetry 2021, 13, 2068. [Google Scholar] [CrossRef]
- Ilea, V.; Otrocol, D. Existence and Uniqueness of the Solution for an Integral Equation with Supremum, via w-Distances. Symmetry 2020, 12, 1554. [Google Scholar] [CrossRef]
- Cadariu, L. The generalized Hyers-Ulam stability for a class of the Volterra nonlinear integral equations. Sci. Bull. Politeh. Univ. Timis. Trans. Math. Phys. 2011, 56, 30–38. [Google Scholar]
- Marian, D.; Ciplea, S.A.; Lungu, N. On a functional integral equation. Symmetry 2021, 13, 1321. [Google Scholar] [CrossRef]
- Gachpazan, M.; Baghani, O. Hyers–Ulam stability of nonlinear integral equation. Fixed Point Theory Appl. 2010, 2010, 927640. [Google Scholar] [CrossRef]
- Gachpazan, M.; Baghani, O. Hyers–Ulam stability of Volterra integral equation. Int. J. Nonlinear Anal. Appl. 2010, 1, 19–25. [Google Scholar]
- Morales, J.; Rojas, E. Hyers–Ulam and Hyers–Ulam–Rassias stability of nonlinear integral equations with delay. Int. J. Nonlinear Anal. Appl. 2011, 2, 1–7. [Google Scholar]
- Bahyrycz, A.; Brzdek, J.; Lesniak, Z. On approximate solutions of the generalized Volterra integral equation. Nonlinear Anal.-Real 2014, 20, 59–66. [Google Scholar] [CrossRef]
- Rus, I.A. Gronwall lemma approach to the Hyers–Ulam–Rassias stability of an integral equation. In Nonlinear Analysis and Variational Problems; Springer Optim. Appl., Springer: New York, NY, USA, 2010; Volume 35, pp. 147–152. [Google Scholar]
- Brzdek, J.; Eghbali, N. On approximate solutions of some delayed fractional differential equations. Appl. Math. Lett. 2016, 54, 31–35. [Google Scholar] [CrossRef]
- Akkouchi, M. Hyers–Ulam–Rassias stability of nonlinear Volterra integral equations via a fixed point approach. Acta Univ. Apulensis Math. Inform. 2011, 26, 257–266. [Google Scholar]
- Tunç, C.; Tunç, O.; Yao, J.-C. On the Enhanced New Qualitative Results of Nonlinear Integro-Differential Equations. Symmetry 2023, 15, 109. [Google Scholar] [CrossRef]
- Tunç, C.; Tunç, O. Solution estimates to Caputo proportional fractional derivative delay integro-differential equations. Rev. Real Acad. Cienc. Exactas Fis. Nat. Ser. A-Mat. 2023, 117, 12. [Google Scholar] [CrossRef]
- Rezaei, H.; Jung, S.-M.; Rassias, T. Laplace transform and Hyers-Ulam stability of linear differential equations. J. Math. Anal. Appl. 2013, 403, 244–251. [Google Scholar] [CrossRef]
- Alqifiary, Q.; Jung, S.-M. Laplace transform and generalized Hyers-Ulam stability of linear differential equations. Electron. J. Differ. Equ. 2014, 2014, 1–11. [Google Scholar]
- Murali, R.; Ponmana Selvan, A. Mittag-Leffler-Hyers-Ulam stability of a linear differential equation of first order using Laplace transforms. Canad. J. Appl. Math. 2020, 2, 47–59. [Google Scholar]
- Shen, Y.; Chen, W. Laplace Transform Method for the Ulam Stability of Linear Fractional Differential Equations with Constant Coefficients. Mediterr. J. Math. 2017, 14, 1–17. [Google Scholar] [CrossRef]
- Inoan, D.; Marian, D. Semi-Hyers-Ulam-Rassias stability of a Volterra integro-differential equation of order I with a convolution type kernel via Laplace transform. Symmetry 2021, 13, 2181. [Google Scholar] [CrossRef]
- Inoan, D.; Marian, D. Semi-Hyers–Ulam–Rassias Stability via Laplace Transform, for an Integro-Differential Equation of the Second Order. Mathematics 2022, 10, 1893. [Google Scholar] [CrossRef]
- Musaev, H.K. The Cauchy problem for degenerate parabolic convolution equation. TWMS J. Pure Appl. Math. 2021, 12, 278–288. [Google Scholar]
- Babolian, E.; Salimi Shamloo, A. Numerical solution of Volterra integral and integro-differential equations of convolution type by using operational matrices of piecewise constant orthogonal functions. J. Comput. Appl. Math. 2008, 214, 495–508. [Google Scholar] [CrossRef]
- Debnath, L. The Double Laplace Transform and their properties with applications to functional, integral and partial differential equations. Int. J. Appl. Comput. Math. 2016, 2, 223–241. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Inoan, D.; Marian, D. Semi-Hyers–Ulam–Rassias Stability of Some Volterra Integro-Differential Equations via Laplace Transform. Axioms 2023, 12, 279. https://doi.org/10.3390/axioms12030279
Inoan D, Marian D. Semi-Hyers–Ulam–Rassias Stability of Some Volterra Integro-Differential Equations via Laplace Transform. Axioms. 2023; 12(3):279. https://doi.org/10.3390/axioms12030279
Chicago/Turabian StyleInoan, Daniela, and Daniela Marian. 2023. "Semi-Hyers–Ulam–Rassias Stability of Some Volterra Integro-Differential Equations via Laplace Transform" Axioms 12, no. 3: 279. https://doi.org/10.3390/axioms12030279
APA StyleInoan, D., & Marian, D. (2023). Semi-Hyers–Ulam–Rassias Stability of Some Volterra Integro-Differential Equations via Laplace Transform. Axioms, 12(3), 279. https://doi.org/10.3390/axioms12030279





