Mathematical Description of the Aerodynamic Characteristics of Stationary Flows in a Vertical Conical Diffuser When Air Is Supplied through Various Tube Configurations
Abstract
1. Introduction
- -
- Gas-dynamic improvement and the development of ways to control the gas dynamics of flows in a conical diffuser remain urgent tasks for fundamental and applied science;
- -
- Recent research into the gas-dynamic characteristics of flows in conical diffusers is carried out mainly through numerical simulation;
- -
- There is a lack of reliable experimental data (and their mathematical description) on the gas-dynamic characteristics of flows in a vertical conical diffuser for mathematical model verification.
- -
- To develop an experimental set-up for studying stationary flows in a vertical conical diffuser when air is supplied through tubes with various cross sections;
- -
- To choose measuring instruments and research methods taking into account the physical features of the processes under research;
- -
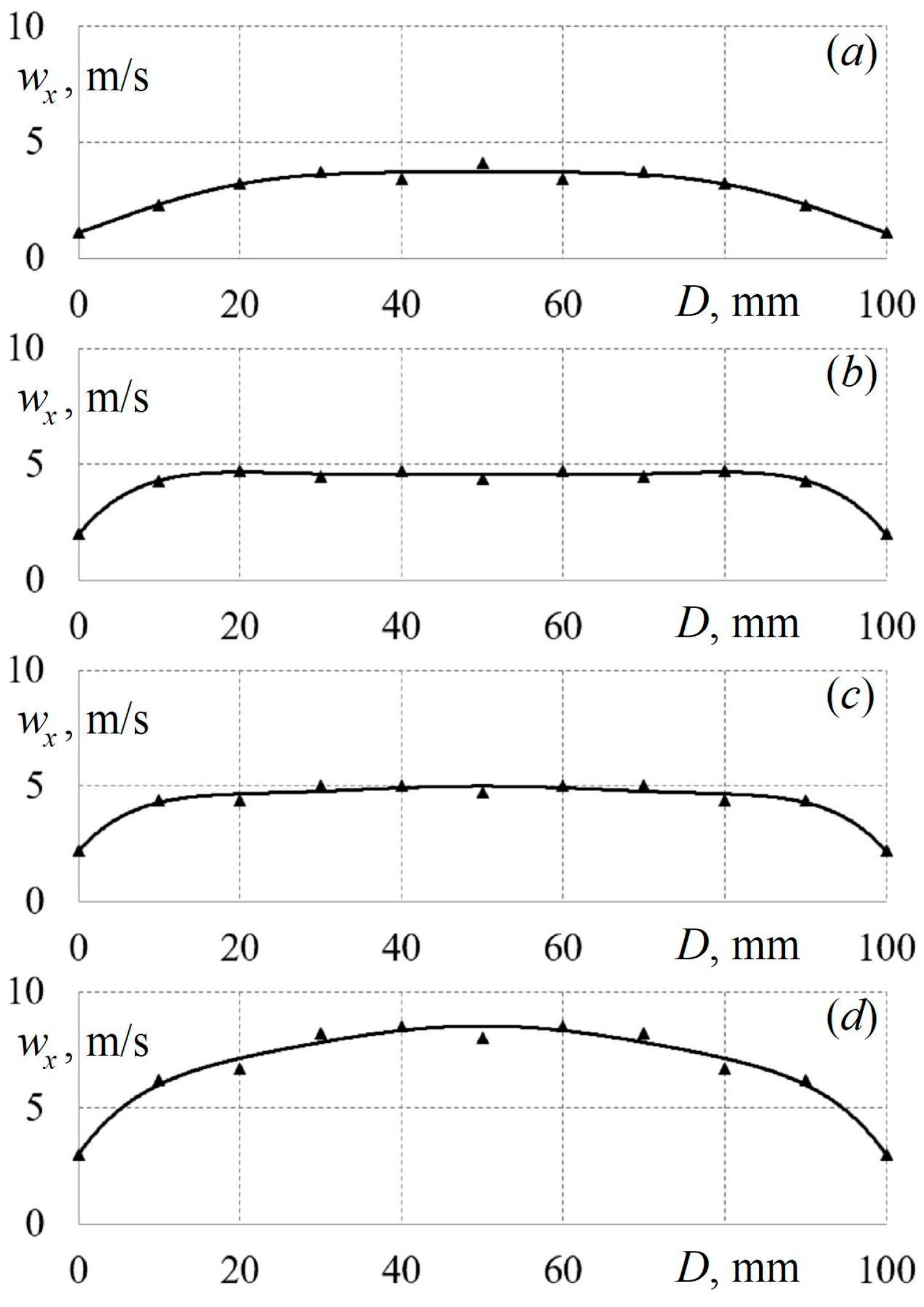
- To obtain data on the instantaneous values of the air flow velocity along the height and diameter of the diffuser’s cylindrical part for various initial conditions;
- -
- To establish the evolution of the velocity fields along the height of the diffuser’s cylindrical part for various configurations of the supply tubes;
- -
- To empirically determine the value of the drop in the average velocity along the height of the diffuser and provide a mathematical description of that process;
- -
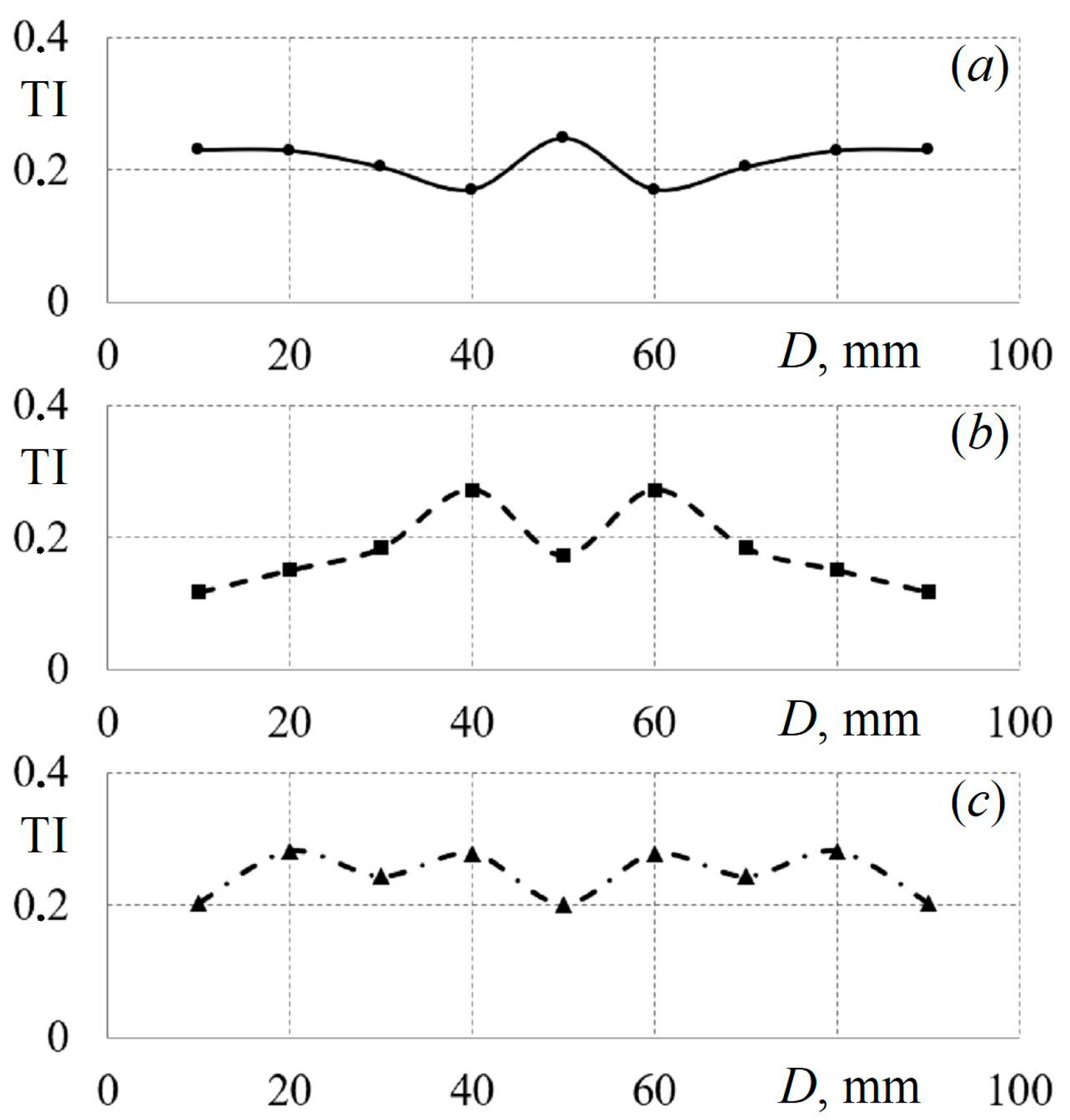
- To establish and mathematically describe the patterns of changes in the intensity of turbulence along the height of the conical diffuser under various initial conditions.
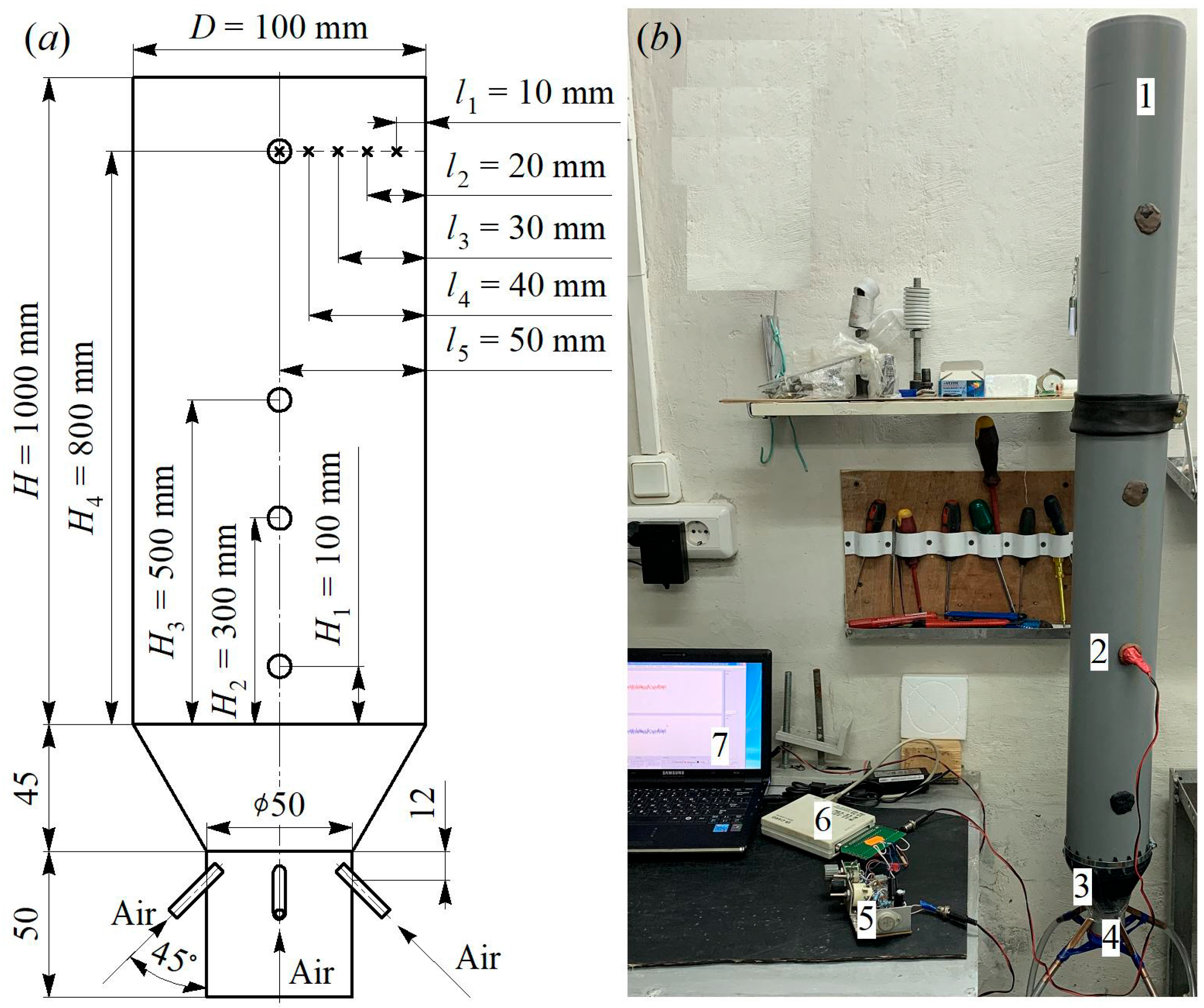
2. Description of the Experimental Measurement Facility
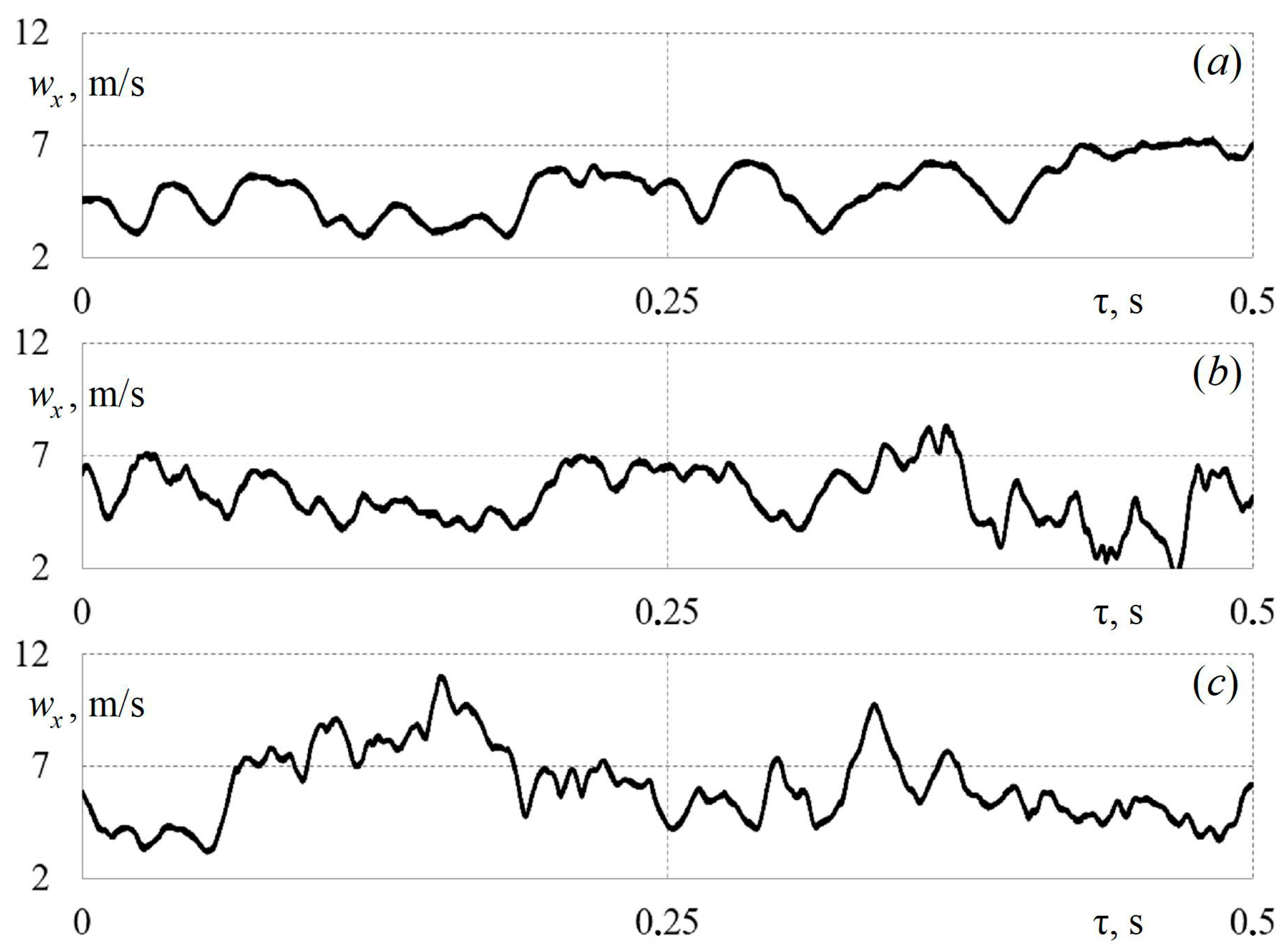
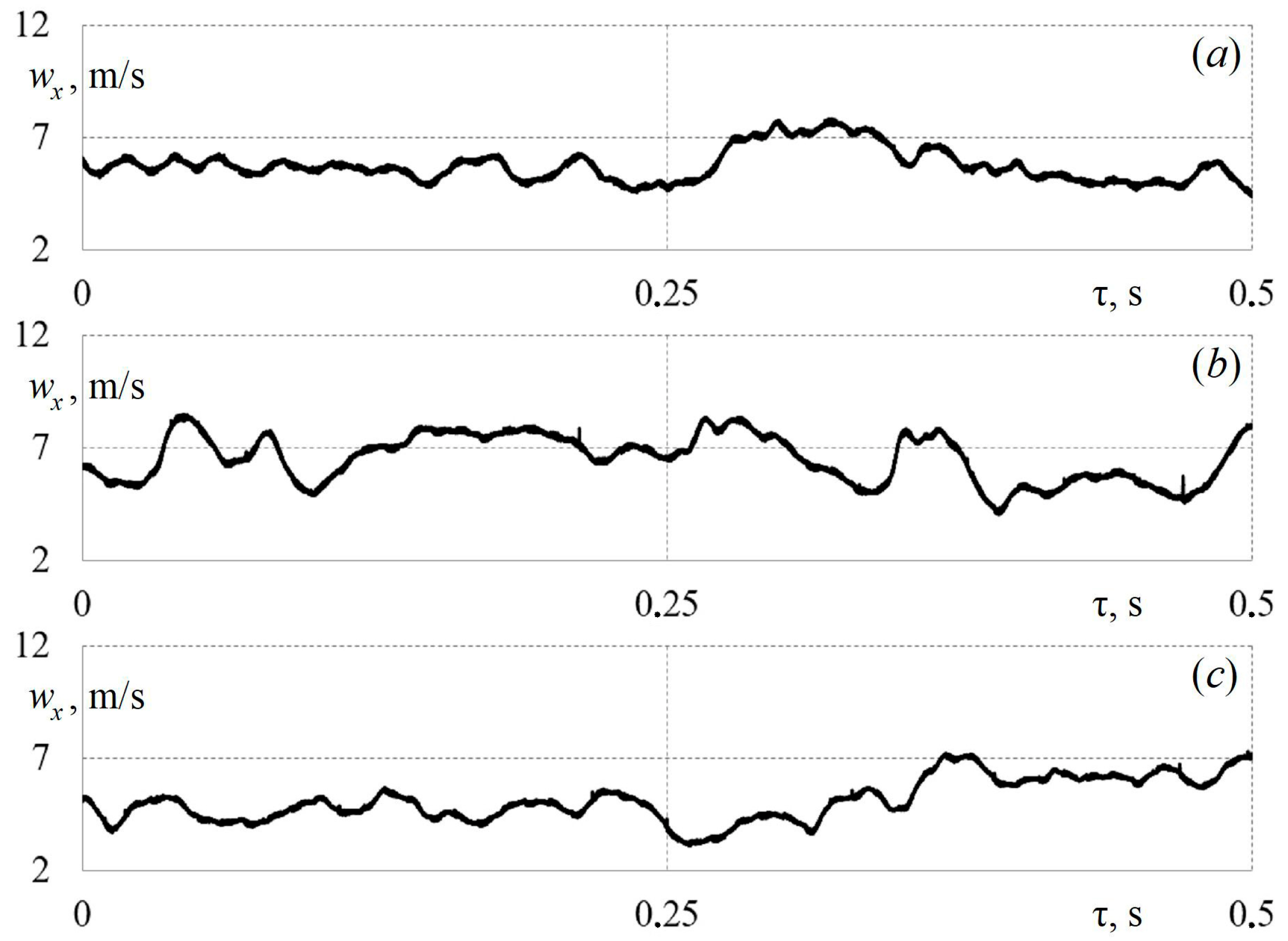
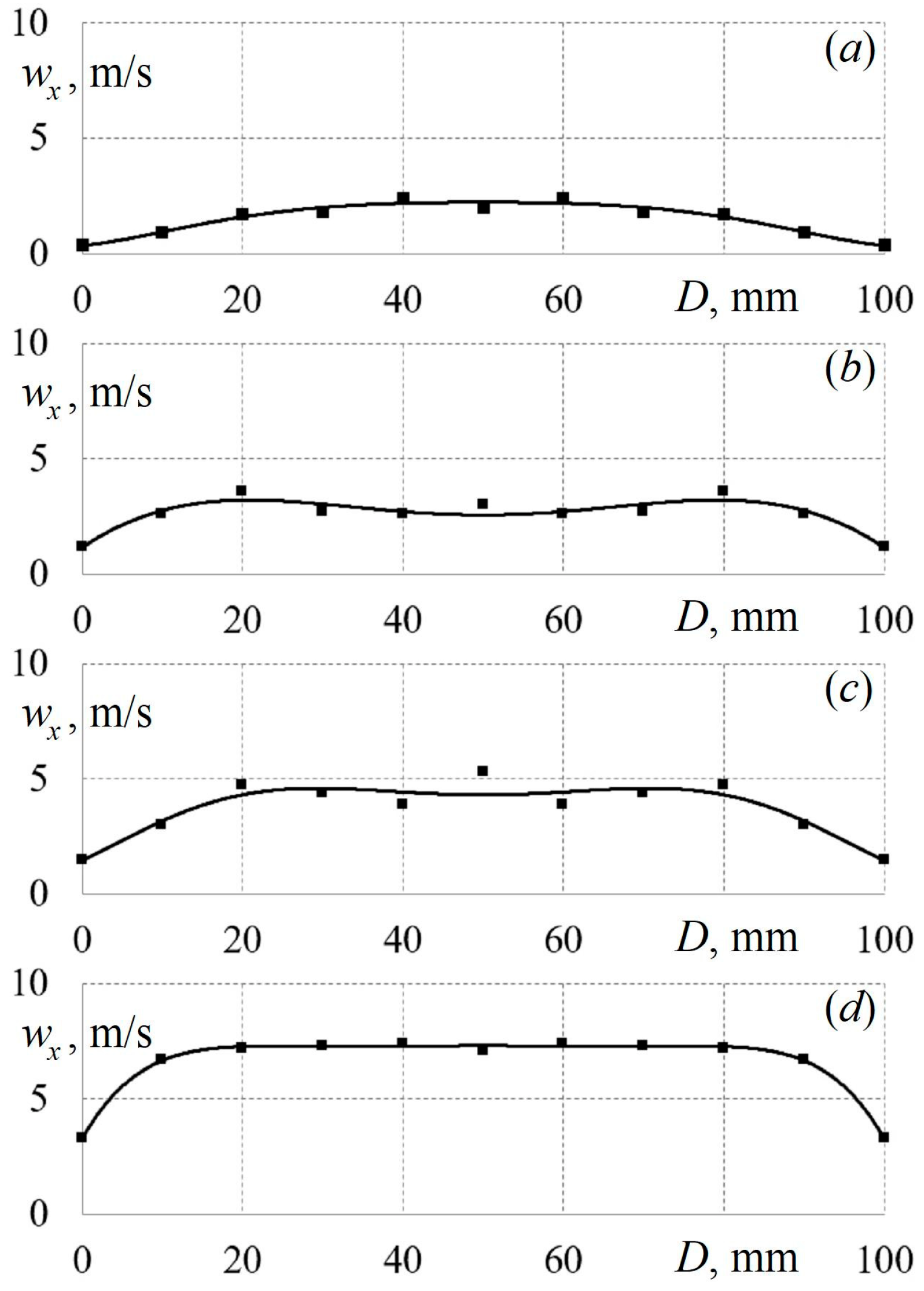
3. Results and Analysis of Experimental Findings
- -
- No clear patterns in the change in TI along the diameter of the diffuser’s cylindrical part were found;
- -
- The influence of the tubes’ cross-sectional shape on the function TI = f (D) has not been established (according to the author, the changes are random);
- -
- The amplitude of the fluctuations of turbulence intensity values relative to the average value is ±35%;
- -
- The mathematical formulation of the function TI = f (D) for the cases under study is inappropriate until the physical laws are established.
- -
- The tubes’ cross-sectional shape has a significant effect on turbulence intensity along the height of the vertical diffuser’s cylindrical part;
- -
- The dependency TI = f (H) has a pronounced maximum in the region of H = 300 mm, which is typical of all initial average flow rates and all configurations of the supply tubes;
- -
- The turbulence intensity has significantly higher values (up to 50%) when air is supplied through profiled tubes compared to round tubes, which is especially typical of a low initial w.
4. Conclusions
- Experimental data on the instantaneous values of the stationary flow velocity along the height and diameter of the diffuser’s cylindrical part for various initial conditions and when air is supplied through tubes with different configurations are obtained.
- The velocity fields along the height of the diffuser’s cylindrical part for various initial conditions when air is supplied through tubes with cross sections in the form of a circle, a square, and a triangle are determined. The evolution of the velocity fields in the upward flow direction is shown.
- The flow’s turbulence intensity TI along the height and diameter of the diffuser is calculated for various initial conditions and when air is supplied through tubes with various configurations.
- A mathematical description (exponential equations) of the change in the average flow velocity along the height of the diffuser’s cylindrical part for various initial conditions and configurations of the supply tubes is presented.
- The regularities of changes in the intensity of turbulence along the height of the diffuser for various initial conditions and tube configurations are established.
- The obtained data on the aerodynamic characteristics of flows in a conical diffuser can be useful for refining and verifying mathematical models and improving engineering calculations.
- Further research could be conducted to obtain dimensionless equations for describing the aerodynamic characteristics of flows in vertical diffusers of various designs, as well as to refine mathematical models for modelling aerodynamics.
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| wx | local air flow velocity, m/s |
| w | average flow velocity, m/s |
| τ | time, s |
| po | barometric pressure, kPa |
| t | temperature, °C |
| (d) | diameter, mm |
| l | linear dimension, mm |
| H | height, mm |
| Re | Reynolds number |
| TI | turbulence intensity |
References
- Japikse, D.; Baines, N.C. Diffuser Design Technology. Concepts ETI: Norwich, UK, 1998; 524p. [Google Scholar]
- Idelchik, I.E. Aerohydrodynamics of Technological Apparatuses (Inlet, Outlet and Distribution of the Flow over the Cross Section of the Devices); Mashinostroenie: Moscow, Russia, 1983; 351p. (In Russian) [Google Scholar]
- Emmons, H.W. Fundamentals of Gas Dynamics; Princeton University Press: Princeton, NJ, USA, 2015; 783p. [Google Scholar]
- Hirsch, C. Numerical Computation of Internal and External Flows: The Fundamentals of Computational Fluid Dynamics; Elesvier: Amsterdam, The Netherlands, 2007; 696p. [Google Scholar]
- Yavuz, M.; Sene, N. Approximate solutions of the model describing fluid flow using generalized ρ-laplace transform method and heat balance integral method. Axioms 2020, 9, 123. [Google Scholar] [CrossRef]
- Xiao, Y.; Yue, F.; Wang, X.; Zhang, X. Reliability-Based Design Optimization of Structures Considering Uncertainties of Earthquakes Based on Efficient Gaussian Process Regression Metamodeling. Axioms 2022, 11, 81. [Google Scholar] [CrossRef]
- Klein, A. Effects of inlet conditions on conical-diffuser performance. J. Fluids Eng. Trans. ASME 1981, 103, 250–257. [Google Scholar] [CrossRef]
- Jeyachandran, K.; Ganesan, V. Numerical modelling of turbulent flow through conical diffusers with uniform and wake velocity profiles at the inlet. Math. Comput. Model. 1988, 10, 87–97. [Google Scholar] [CrossRef]
- Okwuobi, P.A.C.; Azad, R.S. Turbulence in a conical diffuser with fully developed flow at entry. J. Fluid Mech. 1973, 57, 603–622. [Google Scholar] [CrossRef]
- Ferrari, A. Exact solutions for quasi-one-dimensional compressible viscous flows in conical nozzles. J. Fluid Mech. 2021, 915, 915A1-1. [Google Scholar] [CrossRef]
- Lee, J.; Jang, S.J.; Sung, H.J. Direct numerical simulations of turbulent flow in a conical diffuser. J. Turbul. 2012, 13, 1–29. [Google Scholar] [CrossRef]
- Wu, X.; Schlüter, J.; Moin, P.; Pitsch, H.; Iaccarino, G.; Ham, F. Computational study on the internal layer in a diffuser. J. Fluid Mech. 2006, 550, 391–412. [Google Scholar] [CrossRef]
- De Souza, F.J.; Silva, A.L.; Utzig, J. Four-way coupled simulations of the gas-particle flow in a diffuser. Powder Technol. 2014, 253, 496–508. [Google Scholar] [CrossRef]
- Zou, A.; Chassaing, J.-C.; Li, W.; Gu, Y.; Sauret, E. Quantified dense gas conical diffuser performance under uncertainties by flow characteristic analysis. Appl. Therm. Eng. 2019, 161, 114158. [Google Scholar] [CrossRef]
- Dong, B.; Xu, G.; Li, T.; Quan, Y.; Zhai, L.; Wen, J. Numerical prediction of velocity coefficient for a radial-inflow turbine stator using R123 as working fluid. Appl. Therm. Eng. 2018, 130, 1256–1265. [Google Scholar] [CrossRef]
- Kim, D.-Y.; Kim, Y.-T. Preliminary design and performance analysis of a radial inflow turbine for organic Rankine cycles. Appl. Therm. Eng. 2017, 120, 549–559. [Google Scholar] [CrossRef]
- From, C.S.; Sauret, E.; Armfield, S.W.; Saha, S.C.; Gu, Y.T. Turbulent dense gas flow characteristics in swirling conical diffuser. Comput. Fluids 2017, 149, 100–118. [Google Scholar] [CrossRef]
- Keep, J.A.; Head, A.J.; Jahn, I.H. Design of an efficient space constrained diffuser for supercritical CO2 turbines. J. Phys. Conf. Ser. 2017, 821, 012026. [Google Scholar] [CrossRef]
- Wang, W.-B.; Zheng, L.-X.; Lu, W.; Zhang, W.-J.; Xie, C.-X. Study on Structural Design Optimization of Ejector Expansion Chamber. J. Eng. Thermophys. 2021, 42, 309–313. [Google Scholar]
- Sierra-Pallares, J.; García del Valle, J.; Paniagua, J.M.; García, J.; Méndez-Bueno, C.; Castro, F. Shape optimization of a long-tapered R134a ejector mixing chamber. Energy 2018, 165, 422–438. [Google Scholar] [CrossRef]
- Maicke, B.A.; Bondarev, G. Quasi-one-dimensional modeling of pressure effects in supersonic nozzles. Aerosp. Sci. Technol. 2017, 70, 161–169. [Google Scholar] [CrossRef]
- Vinod, L.; Mahendra, M.A.; Mahantayya, K.H. The cfd analysis of subsonic flow around struts of airfoil and cylindrical shape attached to a conical diffuser at exhaust of a gas turbine engine. Int. J. Mech. Prod. Eng. Res. Dev. 2019, 9, 943–954. [Google Scholar]
- Moszowski, B.; Wajman, T.; Sobczak, K.; Inger, M.; Wilk, M. The analysis of distribution of the reaction mixture in ammonia oxidation reactor. Pol. J. Chem. Technol. 2019, 21, 9–12. [Google Scholar] [CrossRef]
- Huang, Y.; Coggon, M.M.; Zhao, R.; Lignell, H.; Bauer, M.U.; Flagan, R.C.; Seinfeld, J.H. The Caltech Photooxidation Flow Tube reactor: Design, fluid dynamics and characterization. Atmos. Meas. Tech. 2017, 10, 839–867. [Google Scholar] [CrossRef]
- Keshavarz, E.; Toghraie, D.; Haratian, M. Modeling industrial scale reaction furnace using computational fluid dynamics: A case study in Ilam gas treating plant. Appl. Therm. Eng. 2017, 123, 277–289. [Google Scholar] [CrossRef]
- Plotnikov, L.; Plotnikov, I.; Osipov, L.; Slednev, V.; Shurupov, V. An Indirect Method for Determining the Local Heat Transfer Coefficient of Gas Flows in Pipelines. Sensors 2022, 22, 6395. [Google Scholar] [CrossRef] [PubMed]
- Plotnikov, L.V. Thermal-mechanical characteristics of stationary and pulsating gas flows in a gas-dynamic system (in relation to the exhaust system of an engine). Therm. Sci. 2022, 26, 365–376. [Google Scholar] [CrossRef]
- Plotnikov, L.V. Unsteady gas dynamics and local heat transfer of pulsating flows in profiled channels mainly to the intake system of a reciprocating engine. Int. J. Heat Mass Transf. 2022, 195, 123144. [Google Scholar] [CrossRef]
- Brodov, Y.M.; Zhilkin, B.P.; Plotnikov, L.V. Influence of Intake/exhaust Channel Lateral Profiling on Thermomechanics of Pulsating Flows. Tech. Phys. 2018, 63, 319–324. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Plotnikov, L. Mathematical Description of the Aerodynamic Characteristics of Stationary Flows in a Vertical Conical Diffuser When Air Is Supplied through Various Tube Configurations. Axioms 2023, 12, 244. https://doi.org/10.3390/axioms12030244
Plotnikov L. Mathematical Description of the Aerodynamic Characteristics of Stationary Flows in a Vertical Conical Diffuser When Air Is Supplied through Various Tube Configurations. Axioms. 2023; 12(3):244. https://doi.org/10.3390/axioms12030244
Chicago/Turabian StylePlotnikov, Leonid. 2023. "Mathematical Description of the Aerodynamic Characteristics of Stationary Flows in a Vertical Conical Diffuser When Air Is Supplied through Various Tube Configurations" Axioms 12, no. 3: 244. https://doi.org/10.3390/axioms12030244
APA StylePlotnikov, L. (2023). Mathematical Description of the Aerodynamic Characteristics of Stationary Flows in a Vertical Conical Diffuser When Air Is Supplied through Various Tube Configurations. Axioms, 12(3), 244. https://doi.org/10.3390/axioms12030244





