The Probabilistic Dual Hesitant Fuzzy Multi-Attribute Decision-Making Method Based on Cumulative Prospect Theory and Its Application
Abstract
:1. Introduction
2. Related Concepts
2.1. Probabilistic Dual Hesitant Fuzzy Sets (PDHFSs)
2.2. Score Function of Probabilistic Dual Hesitant Fuzzy Elements
2.2.1. The Original Score Function of Probabilistic Dual-Hesitant Fuzzy Elements
- (1)
- If , then is deemed superior to , and this relation is denoted as .
- (2)
- If , then .
- (3)
- If , then:
- (1)
- If , then denoted as .
- (2)
- If , and are considered indistinguishable, and this equivalence is denoted as .
2.2.2. Comprehensive Score Function of Probabilistic Dual Hesitant Fuzzy Elements
- (1)
- If , then is deemed superior to , and this relation is denoted as .
- (2)
- If , and are considered indistinguishable, and this equivalence is denoted as .
2.3. Cumulative Prospect Theory (CPT)
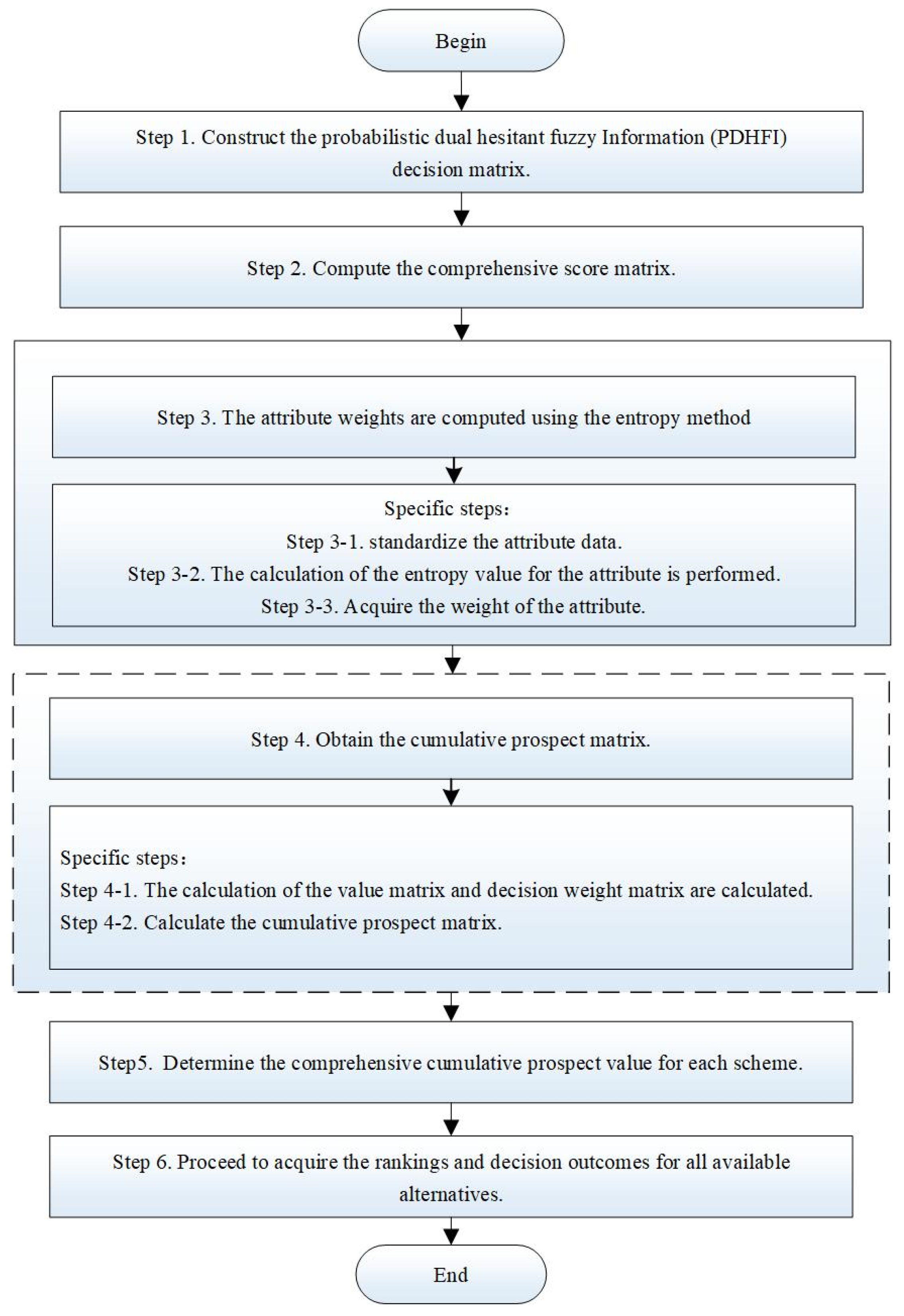
3. The Probabilistic Dual Hesitant Fuzzy Multi-Attribute Decision-Making Method Based on Cumulative Prospect Theory
4. Case Study and Methods Comparison
4.1. Case Study
4.2. Methods Comparison
4.2.1. Method A
4.2.2. Method B
4.2.3. Method C
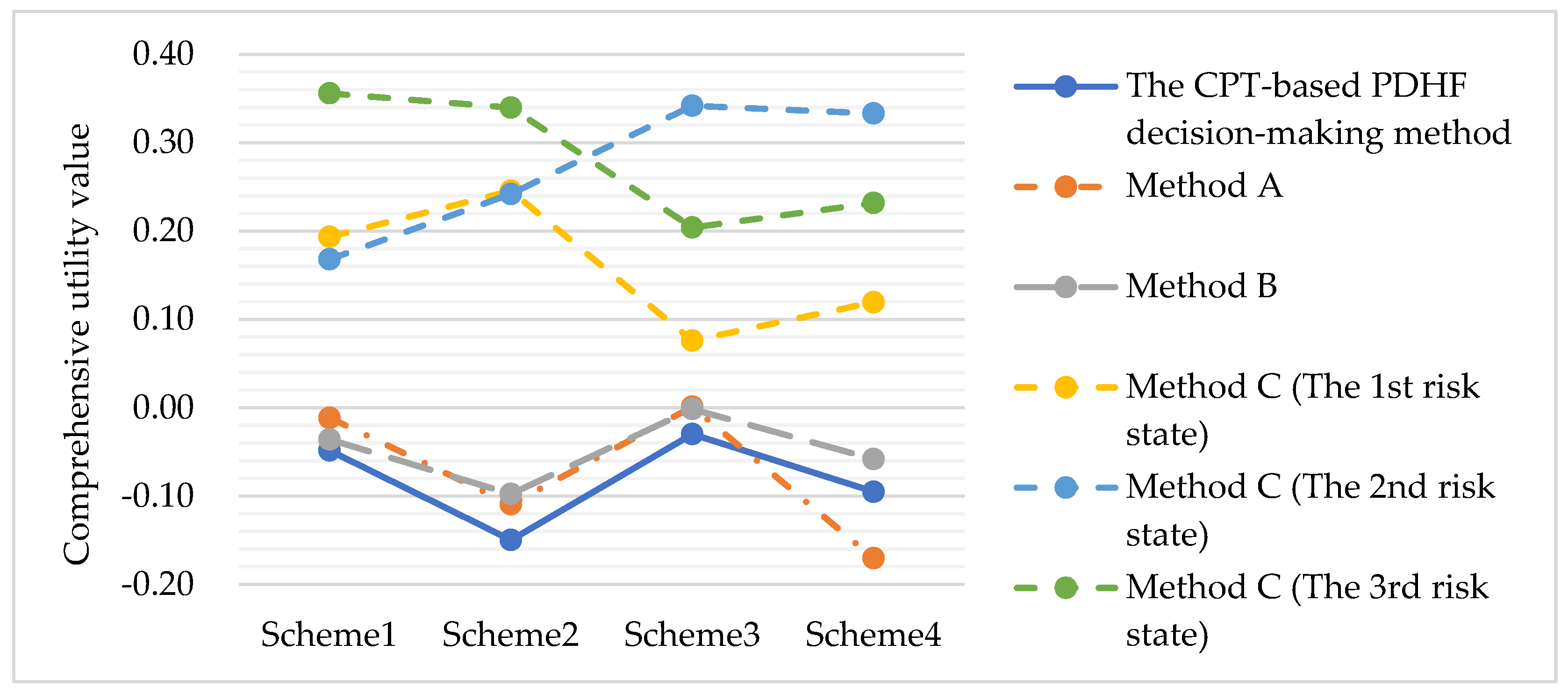
4.3. Discussion
- (1)
- To comprehensively explain DMs evaluation information, “the CPT-based PDHF decision-making method” utilizes the PDHFS format to gather the evaluation information, given the inherent ambiguity of the decision-making information. The provided information is thorough, as it encompasses both membership and non-membership details, along with their respective probabilities. Simultaneously, this method presents an improved comprehensive scoring function to more accurately represent the genuineness and thoroughness of the assessment data.
- (2)
- Within the PDHF context, the “CPT-based PDHF decision-making method” introduces a method for determining cumulative prospect value, incorporates the “bounded rationality” of the DM, and delineates their constrained psychological traits. This method effectively tackles decision-making challenges in various risk scenarios and exhibits a remarkable level of precision and discriminative capability in its decision outcomes.
- (3)
- This study comprehensively considers the subjective and objective factors, and the attribute weight can be solved using the entropy method. Compared with existing literature that requires attribute weight information to be fully known before making a decision, “the CPT-based PDHF decision-making method” has more significant advantages.
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| MADM | Multi-attribute decision-making |
| FSs | Fuzzy sets |
| DMs | Decision makers |
| HFSs | Hesitant fuzzy sets |
| DHFSs | Dual hesitant fuzzy sets |
| HTFSs | Hesitant triangular fuzzy sets |
| PHFSs | Probabilistic hesitant fuzzy sets |
| PT | Prospect theory |
| CPT | Cumulative prospect theory |
| VF | Value function |
| DWF | Decision weight function |
| PDHFI | Probabilistic dual hesitant fuzzy information |
| PDHFEs | Probabilistic dual hesitant fuzzy elements |
| HFEs | Hesitant fuzzy elements |
References
- Zadeh, L.A. Fuzzy sets. Inf. Control 1965, 8, 338. [Google Scholar] [CrossRef]
- Atanassov, K.T. Intuitionistic fuzzy-sets. Fuzzy Sets Syst. 1986, 20, 87–96. [Google Scholar] [CrossRef]
- Torra, V. Hesitant fuzzy sets. Int. J. Intell. Syst. 2010, 25, 529–539. [Google Scholar] [CrossRef]
- Zhu, B.; Xu, Z.; Xia, M. Dual hesitant fuzzy sets. J. Appl. Math. 2012, 2012, 879629. [Google Scholar] [CrossRef]
- Zhong, G.; Xu, L. Models for multiple attribute decision making method in hesitant triangular fuzzy setting. J. Intell. Fuzzy Syst. 2014, 26, 2167–2174. [Google Scholar] [CrossRef]
- Xu, Z.; Zhou, W. Consensus building with a group of decision makers under the hesitant probabilistic fuzzy environment. Fuzzy Optim. Decis. Mak. 2017, 16, 481–503. [Google Scholar] [CrossRef]
- Zhang, W.; Cao, M.; Wang, L. A two-stage large group decision-making method based on a self-confident double hierarchy interval hesitant fuzzy language. Axioms 2023, 12, 511. [Google Scholar] [CrossRef]
- Hao, Z.; Xu, Z.; Zhao, H.; Su, Z. Probabilistic dual hesitant fuzzy set and its application in risk evaluation. Knowl.-Based Syst. 2017, 127, 16–28. [Google Scholar] [CrossRef]
- Li, L.; Lei, H.; Wang, J. Q-rung probabilistic dual hesitant fuzzy sets and their application in multi-attribute decision-making. Mathematics 2020, 8, 1574. [Google Scholar] [CrossRef]
- Zhu, Y.; Zhang, W.; Hou, J. Multi-attribute group decision making algorithm with probabilistic dual hesitant fuzzy sets and PROMETHEE method. Comput. Eng. Appl. 2022, 58, 88–97. [Google Scholar] [CrossRef]
- Zhang, C.; Li, D.; Liang, J.; Wang, B. MAGDM-oriented dual hesitant fuzzy multigranulation probabilistic models based on MULTIMOORA. Int. J. Mach. Learn. Cybern. 2021, 12, 1219–1241. [Google Scholar] [CrossRef]
- Rong, Y.; Liu, Y.; Pei, Z. A novel multiple attribute decision-making approach for evaluation of emergency management schemes under picture fuzzy environment. Int. J. Mach. Learn. Cybern. 2022, 13, 633–661. [Google Scholar] [CrossRef]
- Liu, X.; Wang, Z.; Zhang, S. Probabilistic hesitant fuzzy multiple attribute decision-making based on regret theory for the evaluation of venture capital projects. Econ. Res.-Ekon. Istraz. 2020, 33, 672–697. [Google Scholar] [CrossRef]
- Seikh, M.R.; Mandal, U. Multiple attribute group decision making based on Quasirung Orthopair fuzzy sets: Application to electric vehicle charging station site selection problem. Eng. Appl. Artif. Intell. 2022, 115, 105299. [Google Scholar] [CrossRef]
- Kahneman, D.; Tversky, A. Prospect theory—Analysis of decision under risk. Econometrica 1979, 47, 263–291. [Google Scholar] [CrossRef]
- Tversky, A.; Kahneman, D. Advances in prospect-theory—Cumulative representation of uncertainty. J. Risk Uncertain. 1992, 5, 297–323. [Google Scholar] [CrossRef]
- Zhang, H.; Wei, G.; Chen, X. SF-GRA method based on cumulative prospect theory for multiple attribute group decision making and its application to emergency supplies supplier selection. Eng. Appl. Artif. Intell. 2022, 110, 104679. [Google Scholar] [CrossRef]
- Zhang, Y.; Wei, G.; Guo, Y. TODIM method based on cumulative prospect theory for multiple attribute group decision-making under 2-tuple linguistic Pythagorean fuzzy environment. Int. J. Intell. Syst. 2021, 36, 2548–2571. [Google Scholar] [CrossRef]
- Wang, X.; Wang, H.; Xu, Z. Green supplier selection based on probabilistic dual hesitant fuzzy sets: A process integrating best worst method and superiority and inferiority ranking. Appl. Intell. 2022, 52, 8279–8301. [Google Scholar] [CrossRef]
- Ren, Z.; Xu, Z.; Wang, H. The strategy selection problem on artificial intelligence with an integrated VIKOR and AHP method under probabilistic dual hesitant fuzzy information. IEEE Access 2019, 7, 103979–103999. [Google Scholar] [CrossRef]
- Garg, H.; Kaur, G. Algorithm for probabilistic dual hesitant fuzzy multi-criteria decision-making based on aggregation operators with new distance measures. Mathematics 2018, 6, 280. [Google Scholar] [CrossRef]
- Yager, R.R. On ordered weighted averaging aggregation operators in multicriteria decision-making. IEEE Trans. Syst. Man Cybern. 1988, 18, 183–190. [Google Scholar] [CrossRef]
- Ren, Z.; Xu, Z.; Wang, H. Normal Wiggly Hesitant Fuzzy Sets and Their Application to Environmental Quality Evaluation. Knowl.-Based Syst. 2018, 159, 286–297. [Google Scholar] [CrossRef]
- Tian, C.; Peng, J.; Long, Q. Extended picture fuzzy MULTIMOORA method based on prospect theory for medical institution selection. Cogn. Comput. 2022, 14, 1446–1463. [Google Scholar] [CrossRef]
- Zhu, Y.; Tian, D.; Yan, F. Effectiveness of entropy weight method in decision-making. Math. Probl. Eng. 2020, 2020, 3564835. [Google Scholar] [CrossRef]
- Wang, T.; Li, H.; Zhang, L. A three-way decision model based on cumulative prospect theory. Inf. Sci. 2020, 519, 74–92. [Google Scholar] [CrossRef]
- Yan, B.; Wang, Y.; Xia, W. Novel consensus-reaching model in the social network environment for large-group emergency decision-making: An approach to managing non-cooperative behaviors. Artif. Intell. Rev. 2023, 56, 8609–8645. [Google Scholar] [CrossRef]
- Gao, J.; Huang, X.; Guo, F. Probabilistic hesitant fuzzy multi-attribute decision-making method based on cumulative prospect theory. J. Math. Pract. Theory. 2021, 51, 45–58. [Google Scholar]
- Liu, P.; Wang, H.; Ning, B. MAGDM method based on generalized hesitant fuzzy TODIM and cumulative prospect theory and application to recruitment of university researchers. J. Intell. Fuzzy Syst. 2023, 45, 1863–1880. [Google Scholar] [CrossRef]
- Garg, H.; Kaur, G. A robust correlation coefficient for probabilistic dual hesitant fuzzy sets and its applications. Neural Comput. Appl. 2020, 32, 8847–8866. [Google Scholar] [CrossRef]

| DM | Scheme | Attribute | |||
|---|---|---|---|---|---|
| C1 | C2 | C3 | C4 | ||
| E1 | A1 | {0.2|0.2, 0.3|0.1, 0.4|0.7}, {0.6|0.2, 0.7|0.3, 0.8|0.5} | {0.3|0.4, 0.4|0.2, 0.5|0.4}, {0.5|0.1, 0.6|0.4, 0.7|0.4} | {0.8|0.7, 0.9|0.1}, {0.1|0.3, 0.2|0.3} | {0.2|0.3, 0.3|0.3, 0.4|0.4}, {0.6|0.3, 0.7|0.2, 0.8|0.5} |
| A2 | {0.7|0.6, 0.8|0.1, 0.9|0.3}, {0.1|0.3, 0.2|0.1, 0.3|0.6} | {0.6|0.7, 0.7|0.2, 0.8|0.1}, {0.2|0.4, 0.3|0.2, 0.4|0.4} | {0.2|0.4, 0.3|0.1, 0.4|0.5}, {0.6|0.2, 0.7|0.3, 0.8|0.5} | {0.1|0.2, 0.2|0.3, 0.3|0.5}, {0.7|0.1, 0.8|0.4, 0.9|0.5} | |
| A3 | {0.6|0.2, 0.7|0.2, 0.8|0.6}, {0.2|0.1, 0.3|0.2, 0.4|0.7} | {0.3|0.2, 0.4|0.2, 0.5|0.6}, {0.5|0.2, 0.6|0.3, 0.7|0.5} | {0.1|0.4, 0.2|0.2, 0.3|0.4}, {0.7|0.4, 0.8|0.3, 0.9|0.3} | {0.7|0.1, 0.8|0.3, 0.9|0.6}, {0.1|0.2, 0.2|0.2, 0.3|0.6} | |
| A4 | {0.3|0.2, 0.4|0.1, 0.5|0.7}, {0.5|0.3, 0.6|0.1, 0.7|0.6} | {0.6|0.3, 0.7|0.2, 0.8|0.5}, {0.2|0.1, 0.3|0.7, 0.4|0.2} | {0.8|0.3, 0.9|0.1, 0|0}, {0|0, 0.1|0.3, 0.2|0.2} | {0.2|0.2, 0.3|0.3, 0.4|0.5}, {0.6|0.1, 0.7|0.2, 0.8|0.7} | |
| E2 | A1 | {0.1|0.1, 0.2|0.2, 0.4|0.7}, {0.6|0.3, 0.7|0.2, 0.8|0.5} | {0.4|0.4, 0.5|0.4, 0.6|0.2}, {0.7|0.4, 0.8|0.2} | {0.2|0.3, 0.3|0.1, 0.4|0.6}, {0.6|0.2, 0.7|0.3, 0.8|0.5} | {0.1|0.2, 0.2|0.3, 0.3|0.5}, {0.7|0.1, 0.8|0.4, 0.9|0.5} |
| A2 | {0.2|0.6, 0.3|0.1, 0.4|0.3}, {0.6|0.3, 0.7|0.3, 0.8|0.4} | {0.5|0.4, 0.6|0.2, 0.7|0.4}, {0.3|0.4, 0.4|0.2, 0.5|0.4} | {0.7|0.2, 0.8|0.1, 0.9|0.7}, {0.1|0.2, 0.2|0.3, 0.3|0.5} | {0.1|0.3, 0.2|0.3, 0.3|0.4}, {0.7|0.1, 0.8|0.4, 0.9|0.5} | |
| A3 | {0.3|0.1, 0.4|0.1, 0.5|0.8}, {0.6|0.1, 0.7|0.6} | {0.4|0.3, 0.5|0.2, 0.6|0.5}, {0.4|0.4, 0.5|0.2, 0.6|0.4} | {0.2|0.4, 0.3|0.1, 0.4|0.5}, {0.6|0.2, 0.7|0.3, 0.8|0.5} | {0.1|0.2, 0.2|0.3, 0.3|0.5}, {0.7|0.1, 0.8|0.4, 0.9|0.5} | |
| A4 | {0.4|0.2, 0.5|0.1, 0.6|0.7}, {0.8|0.3, 0.9|0.2} | {0.2|0.2, 0.3|0.2, 0.4|0.6}, {0.6|0.4, 0.7|0.2, 0.8|0.4} | {0.3|0.3, 0.4|0.1, 0.5|0.6}, {0.6|0.3, 0.7|0.5} | {0.1|0.2, 0.2|0.3, 0.3|0.5}, {0.7|0.1, 0.8|0.4, 0.9|0.5} | |
| E3 | A1 | {0.3|0.4, 0.4|0.1}, {0.5|0.1, 0.6|0.1, 0.7|0.8} | {0.2|0.3, 0.3|0.2, 0.4|0.5}, {0.65|0.3, 0.75|0.2, 0.85|0.5} | {0.1|0.2, 0.2|0.1, 0.3|0.7}, {0.75|0.2, 0.85|0.3} | {0.4|0.1, 0.5|0.3, 0.6|0.6}, {0.7|0.2, 0.8|0.4} |
| A2 | {0.2|0.1, 0.3|0.1, 0.4|0.8}, {0.6|0.3, 0.7|0.1, 0.8|0.6} | {0.3|0.3, 0.4|0.2}, {0.5|0.4, 0.6|0.2, 0.7|0.4} | {0.4|0.4, 0.5|0.1, 0.6|0.5}, {0.7|0.2, 0.9|0.3} | {0.3|0.2, 0.4|0.3, 0.5|0.5}, {0.6|0.4, 0.7|0.5} | |
| A3 | {0.3|0.1, 0.4|0.1, 0.5|0.8}, {0.6|0.1, 0.7|0.6} | {0.1|0.3, 0.2|0.2, 0.3|0.5}, {0.7|0.4, 0.8|0.2, 0.9|0.4} | {0.2|0.4, 0.3|0.1, 0.4|0.5}, {0.6|0.2, 0.7|0.3, 0.8|0.5} | {0.3|0.2, 0.4|0.3, 0.5|0.5}, {0.6|0.4, 0.7|0.5} | |
| A4 | {0.7|0.1, 0.8|0.1, 0.9|0.8}, {0.1|0.3, 0.2|0.1, 0.3|0.6} | {0.4|0.3, 0.5|0.2, 0.6|0.5}, {0.8|0.4, 0.9|0.2} | {0.3|0.4, 0.4|0.1}, {0.5|0.2, 0.6|0.3, 0.7|0.5} | {0.2|0.2, 0.3|0.3, 0.4|0.5}, {0.6|0.1, 0.7|0.4, 0.8|0.5} | |
| DM | Scheme | Attribute | |||
|---|---|---|---|---|---|
| C1 | C2 | C3 | C4 | ||
| E1 | A1 | {0.2|0.4, 0.3|0.1, 0.4|0.5}, {0.6|0.1, 0.7|0.1, 0.8|0.8} | {0.6|0.2, 0.7|0.2, 0.8|0.6}, {0.25|0.3, 0.35|0.2, 0.45|0.5} | {0.5|0.1, 0.6|0.1, 0.7|0.8}, {0.35|0.2} | {0.3|0.2, 0.4|0.3, 0.5|0.5}, {0.55|0.2, 0.65|0.4, 0.75|0.4} |
| A2 | {0.6|0.1, 0.7|0.1, 0.8|0.8}, {0.2|0.1, 0.3|0.1, 0.4|0.8} | {0.1|0.2, 0.2|0.2, 0.3|0.6}, {0.75|0.3, 0.85|0.2} | {0.5|0.4, 0.6|0.1, 0.7|0.5}, {0.3|0.2, 0.4|0.3} | {0.3|0.3, 0.4|0.3, 0.5|0.4}, {0.55|0.2, 0.65|0.4, 0.75|0.4} | |
| A3 | {0.1|0.1, 0.2|0.1, 0.3|0.8}, {0.7|0.1, 0.8|0.1, 0.9|0.8} | {0.7|0.3, 0.8|0.2, 0.9|0.5}, {0.15|0.3, 0.25|0.2, 0.35|0.5} | {0.6|0.2, 0.7|0.1, 0.8|0.7}, {0.25|0.2, 0.35|0.3, 0.45|0.5} | {0.2|0.4, 0.3|0.3, 0.4|0.3}, {0.65|0.2, 0.75|0.4, 0.85|0.4} | |
| A4 | {0.3|0.4, 0.4|0.1}, {0.5|0.1, 0.6|0.1, 0.7|0.8} | {0.4|0.2, 0.5|0.2, 0.6|0.6}, {0.65|0.5} | {0.2|0.6, 0.3|0.1, 0.4|0.3}, {0.65|0.2, 0.75|0.3, 0.85|0.5} | {0.6|0.2, 0.7|0.3, 0.8|0.5}, {0.25|0.2, 0.35|0.4, 0.45|0.4} | |
| E2 | A1 | {0.2|0.1, 0.3|0.1, 0.4|0.8}, {0.8|0.2} | {0.3|0.4, 0.4|0.2, 0.5|0.4}, {0.6|0.3, 0.7|0.2, 0.8|0.5} | {0.4|0.2, 0.5|0.1, 0.6|0.7}, {0.3|0.4} | {0.1|0.3, 0.2|0.3, 0.3|0.4}, {0.6|0.1, 0.7|0.4, 0.8|0.5} |
| A2 | {0.4|0.4, 0.5|0.1, 0.6|0.5}, {0.7|0.8} | {0.3|0.2, 0.4|0.2, 0.5|0.6}, {0.6|0.5} | {0.2|0.1, 0.3|0.1, 0.4|0.8}, {0.7|0.5, 0.8|0.3} | {0.2|0.3, 0.3|0.3, 0.4|0.4}, {0.6|0.2, 0.7|0.4, 0.8|0.4} | |
| A3 | {0.3|0.3, 0.4|0.1, 0.5|0.6}, {0.6|0.8} | {0.6|0.1, 0.7|0.2, 0.8|0.7}, {0.3|0.2, 0.4|0.2, 0.5|0.6} | {0.4|0.2, 0.5|0.1, 0.6|0.7}, {0.8|0.4} | {0.8|0.4, 0.9|0.3}, {0.4|0.2, 0.5|0.4} | |
| A4 | {0.7|0.2, 0.8|0.1, 0.9|0.7}, {0.3|0.1, 0.4|0.1, 0.5|0.8} | {0.6|0.4, 0.7|0.2, 0.8|0.4}, {0.2|0.4, 0.3|0.2} | {0.9|0.3}, {0.4|0.3, 0.5|0.3, 0.6|0.4} | {0.6|0.1, 0.7|0.3, 0.8|0.6}, {0.4|0.2, 0.5|0.4} | |
| E3 | A1 | {0.5|0.1, 0.6|0.1, 0.7|0.8}, {0.2|0.1, 0.3|0.1, 0.4|0.8} | {0.4|0.2, 0.5|0.2, 0.6|0.6}, {0.8|0.2, 0.9|0.2} | {0.2|0.1, 0.3|0.1, 0.4|0.8}, {0.6|0.1} | {0.6|0.3, 0.7|0.3, 0.8|0.4}, {0.3|0.3} |
| A2 | {0.1|0.7, 0.2|0.1, 0.3|0.2}, {0.8|0.2, 0.9|0.1} | {0.2|0.4, 0.3|0.2, 0.4|0.4}, {0.6|0.4, 0.7|0.2, 0.8|0.4} | {0.3|0.6, 0.4|0.1, 0.5|0.3}, {0.7|0.3, 0.8|0.3, 0.9|0.4} | {0.4|0.6, 0.5|0.3}, {0.9|0.1} | |
| A3 | {0.5|0.1, 0.6|0.1, 0.7|0.8}, {0.2|0.8, 0.3|0.1} | {0.4|0.2, 0.5|0.2, 0.6|0.6}, {0.8|0.6} | {0.3|0.3, 0.4|0.1, 0.5|0.6}, {0.9|0.6} | {0.8|0.4, 0.9|0.3}, {0.2|0.3, 0.3|0.4, 0.4|0.3} | |
| A4 | {0.4|0.8, 0.5|0.1, 0.6|0.1}, {0.9|0.1} | {0.7|0.6, 0.8|0.2, 0.9|0.2}, {0.2|0.2, 0.3|0.4, 0.4|0.4} | {0.2|0.4, 0.3|0.1, 0.4|0.5}, {0.5|0.5, 0.6|0.3, 0.7|0.2} | {0.5|0.4, 0.6|0.3, 0.7|0.3}, {0.1|0.3, 0.2|0.4} | |
| DM | Scheme | Attribute | |||
|---|---|---|---|---|---|
| C1 | C2 | C3 | C4 | ||
| E1 | A1 | {0.1|0.1,0.2|0.1,0.3|0.8}, {0.7|0.3,0.8|0.1,0.9|0.6} | {0.3|0.2,0.4|0.2,0.5|0.6}, {0.2|0.3} | {0.4|0.1,0.5|0.1,0.6|0.8}, {0.1|0.6,0.2|0.1} | {0.2|0.3,0.3|0.3,0.4|0.4}, {0.6|0.4,0.7|0.4,0.8|0.2} |
| A2 | {0.2|0.7,0.3|0.1,0.4|0.2}, {0.6|0.2,0.7|0.1,0.8|0.7} | {0.4|0.4,0.5|0.2,0.6|0.4}, {0.4|0.4,0.5|0.2,0.6|0.4} | {0.3|0.6,0.4|0.1,0.5|0.3}, {0.5|0.3,0.6|0.3,0.7|0.4} | {0.4|0.6,0.5|0.3}, {1|0.1,1.11.2|0.9} | |
| A3 | {0.1|0.1,0.2|0.9}, {0.8|0.8,0.9|0.1,1|0.1} | {0.4|0.2}, {0.8|0.6} | {0.3|0.3,0.4|0.1,0.5|0.6}, {0.1|0.2,0.2|0.8} | {0.8|0.4,0.9|0.3}, {0.2|0.3,0.3|0.4,0.4|0.3} | |
| A4 | {0.6|0.8,0.7|0.1,0.8|0.1}, {0.2|0.1,0.3|0.2,0.4|0.7} | {0.5|0.6,0.6|0.2,0.7|0.2}, {0.3|0.2,0.4|0.4} | {0.2|0.4,0.3|0.1,0.4|0.5}, {0.6|0.5,0.7|0.3,0.8|0.2} | {0.3|0.4,0.4|0.3,0.5|0.3}, {0.8|0.4,0.9|0.6} | |
| E2 | A1 | {0.2|0.1,0.3|0.1,0.4|0.8}, {0.6|0.1,0.7|0.1,0.8|0.8} | {0.4|0.2,0.5|0.2,0.6|0.6}, {0.1|0.2,0.2|0.2} | {0.3|0.1,0.4|0.1,0.5|0.8}, {0.7|0.1,0.8|0.3} | {0.1|0.3,0.2|0.3,0.3|0.4}, {0.8|0.3,0.9|0.4} |
| A2 | {0.4|0.7,0.5|0.1,0.6|0.2}, {0.8|0.2,0.9|0.1} | {0.2|0.4,0.3|0.2,0.4|0.4}, {0.6|0.4,0.7|0.2,0.8|0.4} | {0.5|0.6,0.6|0.1,0.7|0.3}, {0.3|0.3,0.4|0.3} | {0.3|0.6,0.4|0.3}, {0.6|0.1,0.8|0.4} | |
| A3 | {0.4|0.1,0.5|0.1,0.6|0.8}, {0.7|0.8,0.8|0.1} | {0.6|0.2,0.7|0.2,0.8|0.6}, {0.2|0.6,0.3|0.3} | {0.5|0.3,0.6|0.1,0.7|0.6}, {0.3|0.6,0.4|0.2} | {0.3|0.4,0.4|0.3}, {0.7|0.3,0.8|0.4,0.9|0.3} | |
| A4 | {0.1|0.8,0.2|0.1,0.3|0.1}, {0.7|0.1} | {0.2|0.6,0.3|0.2,0.4|0.2}, {0.6|0.2,0.7|0.4,0.8|0.4} | {0.4|0.4,0.5|0.1,0.6|0.5}, {0.1|0.5,0.2|0.3} | {0.3|0.4,0.4|0.3,0.5|0.3}, {0.8|0.3,0.9|0.4} | |
| E3 | A1 | {0.2|0.4,0.3|0.1,0.4|0.5}, {0.5|0.1,0.6|0.3,0.7|0.2} | {0.4|0.3,0.5|0.2,0.6|0.5}, {0.8|0.2,0.9|0.5} | {0.3|0.6,0.4|0.1}, {0.6|0.1,0.7|0.3,0.8|0.6} | {0.6|0.3,0.7|0.3,0.8|0.4}, {0.3|0.3,0.4|0.2,0.5|0.4} |
| A2 | {0.6|0.7,0.7|0.1,0.8|0.2}, {0.1|0.2,0.2|0.1} | {0.7|0.4,0.8|0.2,0.9|0.4}, {0.6|0.4,0.7|0.2,0.8|0.4} | {0.3|0.2,0.4|0.1,0.5|0.7}, {0.3|0.3,0.4|0.3,0.5|0.4} | {0.4|0.3,0.5|0.3,0.6|0.3}, {0.1|0.1,0.3|0.5} | |
| A3 | {0.8|0.8,0.9|0.1}, {0.2|0.8,0.3|0.1} | {0.6|0.6,0.7|0.2,0.8|0.2}, {0.1|0.6,0.3|0.1,0.4|0.2} | {0.3|0.3,0.4|0.1,0.5|0.6}, {0.6|0.6,0.7|0.1} | {0.8|0.4,0.9|0.3}, {0.2|0.3,0.3|0.4,0.4|0.3} | |
| A4 | {0.2|0.3,0.3|0.1,0.4|0.6}, {0.7|0.1,0.9|0.1} | {0.7|0.6,0.8|0.2,0.9|0.2}, {0.2|0.2,0.3|0.4,0.4|0.4} | {0.3|0.2,0.4|0.1,0.5|0.7}, {0.8|0.5,0.9|0.3} | {0.6|0.4,0.7|0.3,0.8|0.3}, {0.1|0.3,0.2|0.4,0.3|0.3} | |
| The 1st Risk State | The 2nd Risk State | The 3rd Risk State | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| C1 | C2 | C3 | C4 | C1 | C2 | C3 | C4 | C1 | C2 | C3 | C4 | ||
| E1 | A1 | 0.1306 | 0.2876 | 0.2098 | 0.1295 | 0.1148 | −0.0415 | 0.1318 | 0.3087 | 0.0535 | 0.3315 | 0.4331 | 0.4177 |
| A2 | 1.6355 | 1.2186 | 0.1079 | 0.0358 | −0.0159 | −0.0178 | 0.1339 | 0.3038 | 0.1310 | 0.3490 | 0.2865 | 0.6298 | |
| A3 | −0.0312 | 0.2955 | 0.0297 | −0.2270 | 0.0496 | −0.2547 | −0.0435 | 0.1438 | −0.4044 | 0.0221 | 0.3138 | 0.3140 | |
| A4 | 0.2969 | −0.0406 | 0.0438 | 0.1313 | −0.1130 | 0.2595 | 0.1373 | −0.0415 | −0.0156 | 0.1254 | 0.1169 | 0.2713 | |
| E2 | A1 | −0.0392 | 0.2517 | 0.1381 | 0.0013 | 0.1409 | 0.3131 | 0.5235 | −0.0036 | 0.1451 | 0.4208 | 0.4028 | 0.5135 |
| A2 | 0.1220 | 0.1040 | −0.2409 | 0.0309 | 0.4006 | 0.3226 | 0.1360 | 0.1264 | 0.5312 | 0.1235 | 0.8348 | 0.4864 | |
| A3 | 0.0307 | 0.3512 | 0.1169 | 0.0358 | 0.1271 | 0.5324 | 0.0636 | 1.2012 | 0.4695 | −0.0288 | 0.1080 | 0.4401 | |
| A4 | 0.4132 | 0.1329 | −0.0026 | 0.0358 | 2.8330 | −0.0335 | 1.2124 | −0.0422 | −0.0171 | 0.1307 | 0.4041 | 0.5135 | |
| E3 | A1 | 0.2070 | 0.1415 | −0.0174 | 0.5515 | 0.1541 | 0.5324 | 0.0996 | −0.0228 | 0.1094 | 0.5099 | −0.1161 | 0.3707 |
| A2 | 0.1490 | 0.0623 | 0.3784 | 0.0021 | 0.3512 | 0.2135 | 0.3465 | 0.4507 | −0.0110 | −0.2465 | 0.2718 | 0.4183 | |
| A3 | 0.0307 | 0.2702 | 0.2969 | 0.0021 | 0.1551 | 0.4092 | 0.3248 | 0.3140 | 0.2479 | −0.0115 | 0.3238 | 0.3140 | |
| A4 | −0.2225 | 0.4009 | 0.0691 | 0.1313 | 1.7474 | −0.2603 | 0.0914 | 0.4165 | 0.3315 | −0.2603 | 0.4065 | 0.4166 | |
| The 1st Risk State | The 2nd Risk State | The 3rd Risk State | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| C1 | C2 | C3 | C4 | C1 | C2 | C3 | C4 | C1 | C2 | C3 | C4 | |
| A1 | 0.0995 | 0.2270 | 0.1102 | 0.2274 | 0.1366 | 0.2680 | 0.2516 | 0.0941 | 0.1027 | 0.4207 | 0.2399 | 0.4340 |
| A2 | 0.6355 | 0.4616 | 0.0818 | 0.0229 | 0.2453 | 0.1728 | 0.2055 | 0.2936 | 0.2170 | 0.0753 | 0.4644 | 0.5115 |
| A3 | 0.0101 | 0.3057 | 0.1478 | −0.0630 | 0.1106 | 0.2289 | 0.1150 | 0.5530 | 0.1043 | −0.0061 | 0.2485 | 0.3561 |
| A4 | 0.1625 | 0.1644 | 0.0368 | 0.0995 | 1.4891 | −0.0114 | 0.4804 | 0.1109 | 0.0996 | −0.0014 | 0.3092 | 0.4005 |
| C1 | C2 | C3 | C4 | C1 | C2 | C3 | C4 | C1 | C2 | C3 | C4 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| A1 | −0.3671 | −0.1966 | 0.0264 | 0.1946 | −0.9130 | 0.1358 | −0.0443 | −0.4702 | −0.0974 | 0.3452 | −0.2319 | 0.0150 |
| A2 | 0.4549 | 0.2124 | −0.0471 | −0.1578 | −0.6645 | 0.0146 | −0.1826 | 0.0466 | 0.1156 | −0.1521 | 0.1871 | 0.1154 |
| A3 | −0.5860 | 0.0263 | 0.0762 | −0.3855 | −0.9709 | 0.0894 | −0.4191 | 0.3365 | −0.0925 | −0.3691 | −0.2085 | −0.2151 |
| A4 | −0.2014 | −0.3617 | −0.1818 | 0.0427 | 0.9945 | −0.4877 | 0.2610 | −0.4288 | −0.1067 | −0.3572 | −0.0260 | −0.0876 |
| C1 | C2 | C3 | C4 | C1 | C2 | C3 | C4 | C1 | C2 | C3 | C4 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| A1 | 0.3077 | 0.3077 | 0.2911 | 0.2911 | 0.3470 | 0.3263 | 0.3470 | 0.3470 | 0.2267 | 0.2174 | 0.2267 | 0.2174 |
| A2 | 0.2911 | 0.2911 | 0.3077 | 0.3077 | 0.3470 | 0.3263 | 0.3470 | 0.3263 | 0.2174 | 0.2267 | 0.2174 | 0.2174 |
| A3 | 0.3077 | 0.2911 | 0.2911 | 0.3077 | 0.3470 | 0.3263 | 0.3470 | 0.3263 | 0.2267 | 0.2267 | 0.2267 | 0.2267 |
| A4 | 0.3077 | 0.3077 | 0.3077 | 0.2911 | 0.3263 | 0.3470 | 0.3263 | 0.3470 | 0.2267 | 0.2267 | 0.2267 | 0.2267 |
| A1 | A2 | A3 | A4 | |
|---|---|---|---|---|
| C1 | −0.4519 | 0.0588 | −0.0603 | −0.1032 |
| C2 | −0.0731 | 0.0321 | −0.0372 | −0.0082 |
| C3 | −0.5383 | −0.0468 | −0.1705 | −0.0576 |
| C4 | 0.2383 | −0.3615 | 0.0233 | −0.1562 |
| A1 | A2 | A3 | A4 | |
|---|---|---|---|---|
| Comprehensive cumulative prospect value | −0.0484 | −0.1496 | −0.0299 | −0.0950 |
| A1 | A2 | A3 | A4 | |
|---|---|---|---|---|
| Comprehensive utility value | −0.0115 | −0.1093 | 0.0011 | −0.1702 |
| Sort results for schemes | A3 > A1 > A2 > A4 | |||
| A1 | A2 | A3 | A4 | |
|---|---|---|---|---|
| Comprehensive score value | −0.0359 | −0.0976 | −0.0014 | −0.0582 |
| Sort results for schemes | A3 > A1 > A4 > A2 | |||
| The 1st Risk State | The 2nd Risk State | The 3rd Risk State | ||
|---|---|---|---|---|
| Score value | A1 | 0.1934 | 0.1680 | 0.3559 |
| A2 | 0.2456 | 0.2420 | 0.3397 | |
| A3 | 0.0761 | 0.3420 | 0.2040 | |
| A4 | 0.1195 | 0.3330 | 0.2318 | |
| Sort results for schemes | A2 > A1 > A4 > A3 | A3 > A4 > A2 > A1 | A1 > A2 > A4 > A3 | |
| The CPT-Based PDHF Decision-Making Method | Method A | Method B | Method C | ||||
|---|---|---|---|---|---|---|---|
| The 1st Risk State | The 2nd Risk State | The 3rd Risk State | |||||
| Comprehensive utility value | A1 | −0.0484 | −0.0115 | −0.03591 | 0.1934 | 0.1680 | 0.3559 |
| A2 | −0.1496 | −0.1093 | −0.09757 | 0.2456 | 0.2420 | 0.3397 | |
| A3 | −0.0299 | 0.0011 | −0.00142 | 0.0761 | 0.3420 | 0.2040 | |
| A4 | −0.0950 | −0.1702 | −0.05823 | 0.1195 | 0.3330 | 0.2318 | |
| Sort results for schemes | A3 > A1 > A4 > A2 | A3 > A1 > A2 > A4 | A3 > A1 > A4 > A2 | A2 > A1 > A4 > A3 | A3 > A4 > A2 > A1 | A1 > A2 > A4 > A3 | |
| Best scheme | A3 | A3 | A3 | A2 | A3 | A1 | |
| Method | Whether to Consider the Evaluation Information of the Non-Membership Part | Whether to Comprehensively Consider the Evaluation Information of Different Risk States | Whether to Consider Probability Information |
|---|---|---|---|
| The CPT-based PDHF decision-making method | √ | √ | √ |
| Method A | × | √ | √ |
| Method B | × | √ | × |
| Method C | √ | × | √ |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, W.; Zhu, Y. The Probabilistic Dual Hesitant Fuzzy Multi-Attribute Decision-Making Method Based on Cumulative Prospect Theory and Its Application. Axioms 2023, 12, 925. https://doi.org/10.3390/axioms12100925
Zhang W, Zhu Y. The Probabilistic Dual Hesitant Fuzzy Multi-Attribute Decision-Making Method Based on Cumulative Prospect Theory and Its Application. Axioms. 2023; 12(10):925. https://doi.org/10.3390/axioms12100925
Chicago/Turabian StyleZhang, Wenyu, and Yuting Zhu. 2023. "The Probabilistic Dual Hesitant Fuzzy Multi-Attribute Decision-Making Method Based on Cumulative Prospect Theory and Its Application" Axioms 12, no. 10: 925. https://doi.org/10.3390/axioms12100925
APA StyleZhang, W., & Zhu, Y. (2023). The Probabilistic Dual Hesitant Fuzzy Multi-Attribute Decision-Making Method Based on Cumulative Prospect Theory and Its Application. Axioms, 12(10), 925. https://doi.org/10.3390/axioms12100925









