Asymptotic Diffusion Analysis of Retrial Queueing System M/M/1 with Impatient Customers, Collisions and Unreliable Servers

Abstract

In this paper, a retrial queueing system of the M/M/1 type with Poisson flows of arrivals, impatient customers, collisions, and an unreliable service device is considered. To make the problem more realistic and, hence, more complicated, we include the breakdowns and repairs of the service in this research study. The retrial times of customers in the orbit, service time, impatience time of customers in the orbit, server’s lifetime (depending on whether it is idle or busy), and server recovery time are supposed to be exponentially distributed. The problem of finding the stationary probability distribution of the number of customers in orbit is solved by using the method of asymptotic diffusion analyses under the condition of a heavy load of the system and the patience of customers in orbit. Numerical results are presented that demonstrate the effectiveness of the obtained theoretical conclusions, and a comparative analysis of the method of asymptotic analysis and the method of asymptotic diffusion analysis for the considered problem is given.

1. Introduction

This paper is a continuation of [1], and it provides a new solution method and comparisons for the solution obtained in the cited paper. Let us briefly indicate the place of this work among the studies of queueing systems. To build real telecommunication systems and optimize their characteristics, queueing systems are widely studied as mathematical models. It is possible to single out a large class of queueing systems that are occupied by systems with repeated calls.

In the present study, a basic retrial queueing system (RQ-system) with an incoming Poisson flow, one server, and an infinite orbit are complicated by the presence of impatient customers in the orbit, conflicting customers in the system, and the fact that the server can fail. There is no service discipline for the customers in the orbit and customers may retry for service independently. Impatient calls in the orbit are understood as the case when a customer in the orbit can leave the orbit after a random time without service. Conflicts in the system occur in the following case: The device is busy with servicing and a customer comes into the system from outside or a customer from the orbit re-applies to the device for servicing. When a collision occurs, both calls (in service and requesting service) immediately proceed into orbit.

In many situations involving the transmission of data from different sources, uncoordinated attempts by multiple sources to use the same server can lead to a “collision” (data loss or corruption). In papers [2,3], a type of queueing system with repeated calls with collisions, called queues with double connections, was analyzed for the first time. In such models, an arriving client detects that the server (service device) is busy, aborts the service of the client being served at the moment, and both the arriving client and the served client join the orbit and the server immediately becomes idle. Furthermore, in [4], a CSMA/CD (Carrier Sense Multiple Access with Collision Detection) protocol model in the form of RQ with collisions and a constant reversal rate was proposed. The development of these results has been proposed for various models, which are reviewed in [5,6,7]. In all of the above works, the authors consider collision as the only cause of unsuccessful transmission and retransmission, which is not true in the case of real communication systems. This paper discusses other aspects that cause transmission failure and retransmission.

In many real-world situations, the server is prone to unpredictable breakdowns. Therefore, queueing systems assuming server failures can provide a realistic representation of such situations. This type of system can be found in communication networks and industrial and computer networks. RQ-systems with unreliable servers were first considered in works [8,9,10,11,12]. More details about the results can be viewed in [7]. In the present paper, the unreliability of the service device lies in the fact that it can fail after a random period of time. In this case, it takes time to repair the device, and if, at the time of the device’s failure, it was under service, the call from the device proceeds into orbit.

The main aim of this work is to develop a new solution with the help of asymptotic diffusion analysis and to find the area of its applicability.

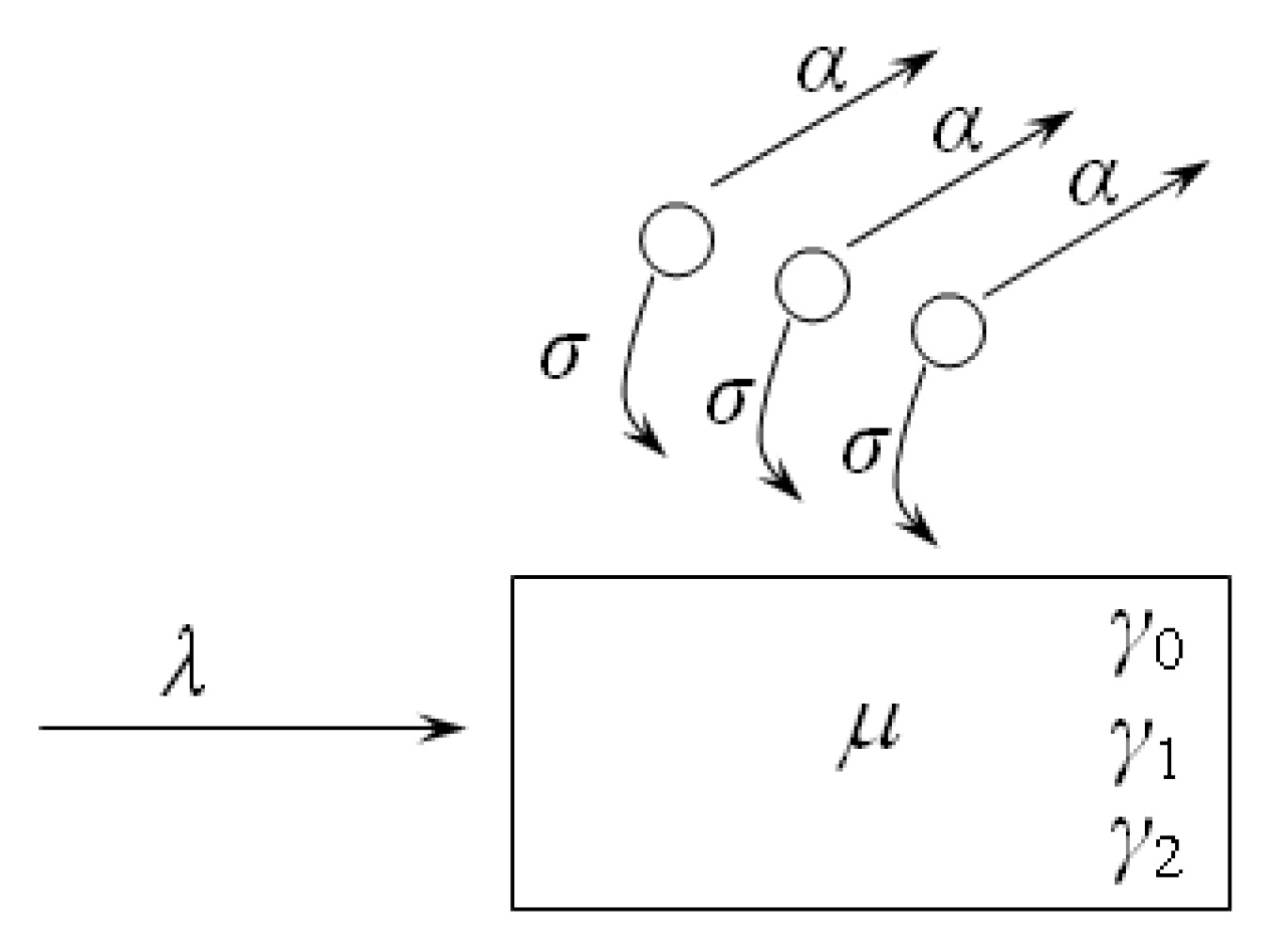
2. Description of the System and Solution Method

A detailed description of the system under consideration is presented in [1], but we present it here for the sake of completely understanding the problem.

We consider a single-server RQ-system with the Poisson arrival process with parameter $\lambda$ for the primary calls. A customer that finds the server to be idle takes it for service for an exponentially distributed random time with parameter $\mu$. If the server is busy, an arriving customer (either from the source or from the orbit) enters into a collision [1,7,13,14,15] and both proceeds into orbit.

In the orbit, each customer, called secondary call, independently of the others retries for services after a random time. The retrial time is exponentially distributed with parameter $\sigma$. If the server is busy again, the request tries to occupy the device to obtain servicing as soon as possible. If the server is idle, the secondary customer occupies it for service for an exponentially distributed random time with parameter $\mu$; that is, no difference between the service of primary and secondary calls is observed.

In addition, we assume that a customer in the orbit leaves the system without service after a random time, which has an exponential distribution with the rate $\alpha$, demonstrating the impatience property. This behaviour was investigated, for example, in papers [1,13,14,15,16,17,18,19,20,21].

We also assume that the server is unreliable; that is, the lifetime is supposed to be exponentially distributed with rate ${\gamma }_0$ if the server is idle and with parameter ${\gamma }_1$ if it is busy. When the server breaks down, it is immediately sent for repair and the recovery time is assumed to be exponentially distributed with rate ${\gamma }_2$. When the server is down, the primary sources continue the generation of customers and send them to the server. Similarly, customers may retry from the orbit relative to the server, but all arriving customers immediately proceed into the orbit. Furthermore, in this unreliable model, we suppose that interrupted requests proceed to the orbit immediately and its next service is independent of the interrupted one. Queueing systems with unreliable servers were investigated, e.g., in [1,7,15,19,22,23,24,25].

All random variables mentioned in the model’s description are assumed to be independent of each other.

Figure 1 shows the model of the RQ-system, $M/M/1$, with impatient customers, collisions, and an unreliable server.

The problem is to find the stationary distribution of the number of customers in the orbit for the described system by using the method of asymptotic diffusion analyses.

The diffusion approximation is the subject of works [26,27,28,29]. The asymptotic diffusion analysis method has already been actively developed by the Tomsk school of scientists in the field of queueing theory; see, for example, [21,30,31,32,33]. This method makes obtaining a discrete diffusion approximation possible for the probability of the distribution of the number of customers in orbit under a given asymptotic condition. In addition to the distribution of the number of customers in orbit, it is interesting to find additional characteristics of the system, for example, the ratio of calls that are in collision with the flow of primary calls or the ratio of calls leaving the orbit without service in the total flow. It should be noted that adding collisions to the model does not allow one to solve the problem analytically (or, for example, to build a recurrent algorithm for finding the desired probabilities). Therefore, we use asymptotic methods. Moreover, as our studies show (this one, and [1]), for problems with collisions of calls, the asymptotic diffusion method behaves stably in terms of the Kolmogorov distance compared to the asymptotic analysis method. We believe that this is due to the fact that by investigating models with collisions with the method of asymptotic analysis, we can obtain an asymptotic approximation of the distribution of the number of claims in orbit consisting of three parts, each of which has the form of a Gaussian distribution but with different values of the mean (see, for example, [34]).

To find the area of the applicability of the resulting approximation, we find the Kolmogorov distance as a measure of the proximity of the diffusion approximation and the distribution of the number of customers in orbit, which is the solution of a system of truncated dimensions describing the state of the system. Despite the complexity of the method, the available works, and the present study show that the method is more accurate than the method of asymptotic analysis in terms of the Kolmogorov distance.

For the numerical implementation of the theoretical results, a program code in the Mathcad 14.0 package was developed. In this work, the program code is not given, as it is in the patenting stage.

3. Investigations and Results

3.1. Theoretical Results

3.1.1. System of Kolmogorov Differential Equations

Let us introduce Markovian process $\left\{k\left(t\right),i\left(t\right)\right\}$ where $i\left(t\right)$ is the number of calls in the orbit at the moment t, $i\left(t\right)=0,1,2,3,\dots$, and $k\left(t\right)$ defines the device’s state at moment t and takes one of the following values.

$$\begin{array}{c}k\left(t\right)=\left\{\begin{array}{c}0,\mathrm{if}\mathrm{the}\mathrm{device}\mathrm{is}\mathrm{idle}\mathrm{at}\mathrm{the}\mathrm{moment}\mathrm{t},\hfill \\ 1,\mathrm{if}\mathrm{the}\mathrm{device}\mathrm{is}\mathrm{busy}\mathrm{at}\mathrm{the}\mathrm{moment}\mathrm{t},\hfill \\ 2,\mathrm{if}\mathrm{the}\mathrm{device}\mathrm{is}\mathrm{under}\mathrm{repair}\mathrm{at}\mathrm{the}\mathrm{moment}\mathrm{t}.\hfill \end{array}\right.\end{array}$$

Denote by $P_k(i,t)=P\left\{k\left(t\right)=k,i\left(t\right)=i\right\}$ the probability that, at the moment t, there are i calls in the orbit, $i=0,1,2,\dots$, and the service device is in state k.

To obtain the probability distribution $P_k(i,t)$, $k=0,1,2$, for the states of the retrial queue M/M/1 with impatient customers, collisions, and unreliable service device, as usual, we derive a system of Kolmogorov differential equations (1), which is the same as described in [1] but its solution method will be different.

$$\left\{\begin{array}{c}{\displaystyle \frac{\partial P_0\left(i,t\right)}{\partial t}}=-\left(\lambda +i\sigma +i\alpha +{\gamma }_0\right)P_0\left(i,t\right)+\left(i+1\right)\alpha P_0\left(i+1,t\right)\hfill \\ +\left(i-1\right)\sigma P_1\left(i-1,t\right)+\mu P_1\left(i,t\right)+\lambda P_1\left(i-2,t\right)+{\gamma }_2{P}_2\left(i,t\right),\hfill \\ {\displaystyle \frac{\partial P_1\left(i,t\right)}{\partial t}}=-\left(\lambda +\mu +i\sigma +i\alpha +{\gamma }_1\right)P_1\left(i,t\right)+\lambda P_0\left(i,t\right)\hfill \\ +\left(i+1\right)\sigma P_0\left(i+1,t\right)+\left(i+1\right)\alpha P_1\left(i+1,t\right),\hfill \\ {\displaystyle \frac{\partial P_2\left(i,t\right)}{\partial t}}=-\left(\lambda +i\alpha +{\gamma }_2\right)P_2\left(i,t\right)+\lambda P_2\left(i-1,t\right)\hfill \\ +\left(i+1\right)\alpha P_2\left(i+1,t\right)+{\gamma }_0{P}_0\left(i,t\right)+{\gamma }_1{P}_1\left(i-1,t\right),\hfill \end{array}\right.$$

(1)

$i=0,1,2,3,\dots$.

Introduce the partial characteristic functions

$$H_k\left(u\right)=\sum _{i=0}^{\infty }{e}^{jui}{P}_k(i,t),k=0,1,2,j=\sqrt{-1}.$$

(2)

Using (2) and $H_k^{\prime }\left(u\right)={\displaystyle \frac{d{H}_k\left(u\right)}{du}}=j{\displaystyle \sum _{i=0}^{\infty }}i{e}^{jui}{P}_k\left(i\right)$, $k=0,1,2$, we can write system (1) as follows.

$$\left\{\begin{array}{c}{\displaystyle \frac{\partial H_0(u,t)}{\partial t}}=-\left(\lambda +{\gamma }_0\right)H_0(u,t)+\left(\mu +\lambda e^{2ju}\right)H_1(u,t)+{\gamma }_2{H}_2(u,t)\hfill \\ +j\left(\sigma +\alpha -\alpha e^{-ju}\right)H_0^{\prime }(u,t)-j\sigma e^{ju}{H}_1^{\prime }(u,t),\hfill \\ {\displaystyle \frac{\partial H_1(u,t)}{\partial t}}=\lambda H_0(u,t)-\left(\lambda +\mu +{\gamma }_1\right)H_1(u,t)-j\sigma e^{-ju}{H}_0^{\prime }(u,t)\hfill \\ +j\left(\sigma +\alpha -\alpha e^{-ju}\right)H_1^{\prime }(u,t),\hfill \\ {\displaystyle \frac{\partial H_2(u,t)}{\partial t}}={\gamma }_0{H}_0(u,t)+{\gamma }_1{e}^{ju}{H}_1(u,t)-\left(\lambda +{\gamma }_2-\lambda e^{ju}\right)H_2(u,t)\hfill \\ +j\alpha \left(1-e^{-ju}\right)H_2^{\prime }(u,t),\hfill \end{array}\right.$$

(3)

Adding the equations of (3), we obtain (4):

$$\begin{array}{c}{\displaystyle \frac{\partial H(u,t)}{\partial t}}=\left(1-e^{-ju}\right)\left\{\left[\lambda \left(e^{ju}+1\right)+{\gamma }_1\right]H_1(u,t)+\lambda H_2(u,t)\right.\\ \left.+j{e}^{-ju}\left(\sigma +\alpha \right)H_0^{\prime }(u,t)+j\left(\alpha e^{-ju}-\sigma \right)H_1^{\prime }(u,t)+j\alpha e^{-ju}{H}_2^{\prime }(u,t)\right\},\end{array}$$

(4)

where $H(u,t)=H_0(u,t)+H_1(u,t)+H_2(u,t)$.

We use system (3) and Equation (4) for diffusion approximation in three stages: (1) obtaining the drift coefficient; (2) centering the process and obtaining the diffusion coefficient; (3) diffusion approximation.

3.1.2. Obtaining the Drift Coefficient

In the system of Equations (3) and (4), we make substitutions $\sigma =\epsilon$, $\alpha =q\epsilon$, $u=\epsilon w$, $\tau =\epsilon t$, $H_k(u,t)=F_k(w,\epsilon ,\tau )$, and $k=0,1,2$, where $\epsilon$ is an infinitesimal value, and we obtain (5)

$$\left\{\begin{array}{c}\epsilon {\displaystyle \frac{\partial F_0(w,\epsilon ,\tau )}{\partial \tau }}=-(\lambda +{\gamma }_0)F_0(w,\epsilon ,\tau )+(\mu +\lambda e^{2jw\epsilon })F_1(w,\epsilon ,\tau )\hfill \\ +{\gamma }_2{F}_2(w,\epsilon ,\tau )+j\left(1+q-q{e}^{-jw\epsilon }\right){\displaystyle \frac{\partial F_0(w,\epsilon ,\tau )}{\partial w}}-j{e}^{jw\epsilon }{\displaystyle \frac{\partial F_1(w,\epsilon ,\tau )}{\partial w}},\hfill \\ \epsilon {\displaystyle \frac{\partial F_1(w,\epsilon ,\tau )}{\partial \tau }}=\lambda F_0(w,\epsilon ,\tau )-(\lambda +\mu +{\gamma }_1)F_1(w,\epsilon ,\tau )\hfill \\ -j{e}^{-jw\epsilon }{\displaystyle \frac{\partial F_0(w,\epsilon ,\tau )}{\partial w}}+j\left(1+q-q{e}^{-jw\epsilon }\right){\displaystyle \frac{\partial F_1(w,\epsilon ,\tau )}{\partial w}},\hfill \\ \epsilon {\displaystyle \frac{\partial F_2(w,\epsilon ,\tau )}{\partial \tau }}={\gamma }_0{F}_0(w,\epsilon ,\tau )+{\gamma }_1{e}^{jw\epsilon }{F}_1(w,\epsilon ,\tau )\hfill \\ -(\lambda -\lambda e^{jw\epsilon }+{\gamma }_2)F_2(w,\epsilon ,\tau )+jq(1-e^{-jw\epsilon }){\displaystyle \frac{\partial F_2(w,\epsilon ,\tau )}{\partial w}},\hfill \\ \epsilon {\displaystyle \frac{\partial F(w,\epsilon ,\tau )}{\partial \tau }}=\left(e^{jw\epsilon }-1\right)\left(\left[\lambda (1+e^{jw\epsilon })+{\gamma }_1\right]F_1(w,\epsilon ,\tau )+\right.\hfill \\ +\lambda F_2(w,\epsilon ,\tau )+j(1+q)e^{-jw\epsilon }{\displaystyle \frac{\partial F_0(w,\epsilon ,\tau )}{\partial w}}-\hfill \\ \left.-j(1-q{e}^{-jw\epsilon }){\displaystyle \frac{\partial F_1(w,\epsilon ,\tau )}{\partial w}}+jq{e}^{-jw\epsilon }{\displaystyle \frac{\partial F_2(w,\epsilon ,\tau )}{\partial w}}\right).\hfill \end{array}\right.$$

(5)

Now, we transform the equations of (5) under $\epsilon \to 0$ with $F_k(w,\tau )=\underset{\epsilon \to 0}{lim}{F}_k(w,\epsilon ,\tau )$, $k=0,1,2$, and we find their solution $F_k(w,\tau )$, $k=0,1,2$, in the form (6)

$$F_k(w,\tau )=R_{k}exp\left\{jwx\left(\tau \right)\right\},k=0,1,2,$$

(6)

where $x\left(\tau \right)$ is an unknown function of time $\tau$, and $R_k\left(x\left(\tau \right)\right)=H_k\left(0\right)={\displaystyle \sum _{i=0}^{\infty }}{P}_k\left(i\right)$ is the stationary probability that i calls when in the orbit and when device is in state k.

Substituting (6) in (5), we obtain

$$\left\{\begin{array}{c}{R}_0\left(x\left(\tau \right)\right)={\displaystyle \frac{{\gamma }_2\left(\lambda +\mu +{\gamma }_1+x\left(\tau \right)\right)}{\left({\gamma }_0+{\gamma }_2\right)\left(\lambda +\mu +{\gamma }_1+x\left(\tau \right)\right)+\left({\gamma }_1+{\gamma }_2\right)\left(\lambda +x\left(\tau \right)\right)}},\hfill \\ R_1\left(x\left(\tau \right)\right)={\displaystyle \frac{{\gamma }_2\left(\lambda +x\left(\tau \right)\right)}{\left({\gamma }_0+{\gamma }_2\right)\left(\lambda +\mu +{\gamma }_1+x\left(\tau \right)\right)+\left({\gamma }_1+{\gamma }_2\right)\left(\lambda +x\left(\tau \right)\right)}},\hfill \\ R_2\left(x\left(\tau \right)\right)=1-R_0\left(x\left(\tau \right)\right)-R_1\left(x\left(\tau \right)\right).\hfill \end{array}\right.$$

(7)

Denoting $x^{\prime }\left(\tau \right)=a\left(x\left(\tau \right)\right)$, we obtain the drift coefficient from (5) and (7).

$$a\left(x\left(\tau \right)\right)=\left(2\lambda +{\gamma }_1+x\left(\tau \right)\right)R_1\left(x\left(\tau \right)\right)+\lambda R_2\left(x\left(\tau \right)\right)-x\left(\tau \right)R_0\left(x\left(\tau \right)\right)-qx\left(\tau \right).$$

(8)

3.1.3. Centering the Process and Obtaining the Diffusion Coefficient

In (3) and (4), we have

$$H_k(u,t)=exp\left\{{\displaystyle \frac{ju}{\sigma }}x\left(\sigma t\right)\right\}{H}_k^{\left(2\right)}(u,t),k=0,1,2,$$

and make substitutions $\sigma ={\epsilon }^2$, $\alpha =q{\epsilon }^2$, $u=\epsilon w$, $\tau ={\epsilon }^{2}t$, $H_k^{\left(2\right)}(u,t)=F_k^{\left(2\right)}(w,\epsilon ,\tau )$, and $k=0,1,2$ to obtain the system below

$$\left\{\begin{array}{c}jw\epsilon a\left(x\left(\tau \right)\right)F_0^{\left(2\right)}(w,\epsilon ,\tau )=-\left[\lambda +{\gamma }_0+x\left(\tau \right)+qjw\epsilon x\left(\tau \right)\right]F_0^{\left(2\right)}(w,\epsilon ,\tau )+,\hfill \\ +\left[\mu +(1+2jw\epsilon )\lambda +(1+jw\epsilon )x\left(\tau \right)\right]F_1^{\left(2\right)}(w,\epsilon ,\tau )+{\gamma }_2{F}_2^{\left(2\right)}(w,\epsilon ,\tau )+\hfill \\ +j\epsilon {\displaystyle \frac{\partial F_0^{\left(2\right)}(w,\epsilon ,\tau )}{\partial w}}-j\epsilon {\displaystyle \frac{\partial F_1^{\left(2\right)}(w,\epsilon ,\tau )}{\partial w}}+O\left({\epsilon }^2\right),\hfill \\ jw\epsilon a\left(x\left(\tau \right)\right)F_1^{\left(2\right)}(w,\epsilon ,\tau )=-\left[\lambda +\mu +{\gamma }_1+x\left(\tau \right)+qjw\epsilon x\left(\tau \right)\right]F_1^{\left(2\right)}(w,\epsilon ,\tau )\hfill \\ +\left[\lambda +(1-jw\epsilon )x\left(\tau \right)\right]F_0^{\left(2\right)}(w,\epsilon ,\tau )-j\epsilon {\displaystyle \frac{\partial F_0^{\left(2\right)}(w,\epsilon ,\tau )}{\partial w}}+j\epsilon {\displaystyle \frac{\partial F_1^{\left(2\right)}(w,\epsilon ,\tau )}{\partial w}}+O\left({\epsilon }^2\right),\hfill \\ jw\epsilon a\left(x\left(\tau \right)\right)F_2^{\left(2\right)}(w,\epsilon ,\tau )={\gamma }_0{F}_0^{\left(2\right)}(w,\epsilon ,\tau )+(1+jw\epsilon ){\gamma }_1{F}_1^{\left(2\right)}(w,\epsilon ,\tau )-\hfill \\ -\left[{\gamma }_2-\lambda jw\epsilon +qjw\epsilon x\left(\tau \right)\right]F_2^{\left(2\right)}(w,\epsilon ,\tau )+O\left({\epsilon }^2\right),\hfill \\ {\epsilon }^2{\displaystyle \frac{\partial F^{\left(2\right)}(w,\epsilon ,\tau )}{\partial \tau }}+jw\epsilon a\left(x\left(\tau \right)\right)F^{\left(2\right)}(w,\epsilon ,\tau )=\hfill \\ \left[jw\epsilon +{\displaystyle \frac{{\left(jw\epsilon \right)}^2}{2}}\right]\left\{-(1+q)x\left(\tau \right)F_0^{\left(2\right)}(w,\epsilon ,\tau )+\right.\hfill \\ \left.+\left[2\lambda +{\gamma }_1+(1-q)x\left(\tau \right)\right]F_1^{\left(2\right)}(w,\epsilon ,\tau )+(\lambda -qx\left(\tau \right))F_2^{\left(2\right)}(w,\epsilon ,\tau )\right\}+\hfill \\ +{\left(jw\epsilon \right)}^2(1+q)x\left(\tau \right)F_0^{\left(2\right)}(w,\epsilon ,\tau )+{\left(jw\epsilon \right)}^2(\lambda +qx\left(\tau \right))F_1^{\left(2\right)}(w,\epsilon ,\tau )+\hfill \\ +{\left(jw\epsilon \right)}^{2}qx\left(\tau \right)F_2^{\left(2\right)}(w,\epsilon ,\tau )+\left(jw\epsilon \right)j\epsilon (1+q){\displaystyle \frac{\partial F_0^{\left(2\right)}(w,\epsilon ,\tau )}{\partial w}}+\hfill \\ +\left(jw\epsilon \right)j\epsilon (q-1){\displaystyle \frac{\partial F_1^{\left(2\right)}(w,\epsilon ,\tau )}{\partial w}}+\left(jw\epsilon \right)j\epsilon q{\displaystyle \frac{\partial F_2^{\left(2\right)}(w,\epsilon ,\tau )}{\partial w}}+O\left({\epsilon }^3\right).\hfill \end{array}\right.$$

(9)

The solution of equations system (9) has the following form

$$\left\{\begin{array}{c}{F}_k^{\left(2\right)}(w,\epsilon ,\tau )=\mathrm{\Phi }(w,\tau )\left(R_k+jw\epsilon f_k\right)+O\left({\epsilon }^2\right),k=0,1,2,\hfill \\ R_0\left(x\left(\tau \right)\right)+R_1\left(x\left(\tau \right)\right)+R_2\left(x\left(\tau \right)\right)=1,\hfill \end{array}\right.$$

(10)

where $R_k\left(x\left(\tau \right)\right)$ and $k=0,1,2$ are defined above, $f_0,f_1,f_2$ and $\left(f_0+f_1+f_2=f\right)$ are constants, and $\mathrm{\Phi }(w,\tau )$ is a determined function.

Transformations (9) with (10) and additional condition $g_0\left(x\left(\tau \right)\right)+g_1\left(x\left(\tau \right)\right)+g_2\left(x\left(\tau \right)\right)$$=0$ lead us to

$$f_k=C{R}_k+g_k\left(x\left(\tau \right)\right)-{\phi }_k\left(x\left(\tau \right)\right){\displaystyle \frac{\partial \mathrm{\Phi }(w,\tau )/\partial w}{w\mathrm{\Phi }(w,\tau )}},k=0,1,2,$$

(11)

$$\left\{\begin{array}{c}{\phi }_k\left(x\left(\tau \right)\right)={\displaystyle \frac{\partial R_k\left(x\left(\tau \right)\right)}{\partial x\left(\tau \right)}},k=0,1,2,\hfill \\ {\phi }_0\left(x\left(\tau \right)\right)+{\phi }_1\left(x\left(\tau \right)\right)+{\phi }_2\left(x\left(\tau \right)\right)=0,\hfill \end{array}\right.$$

(12)

$$\left\{\begin{array}{c}{g}_0\left(x\left(\tau \right)\right)={\displaystyle \frac{{\Delta }_0}{\left({\gamma }_0+{\gamma }_2\right)\left(\lambda +\mu +{\gamma }_1+x\left(\tau \right)\right)+\left({\gamma }_1+{\gamma }_2\right)\left(\lambda +x\left(\tau \right)\right)}},\hfill \\ g_1\left(x\left(\tau \right)\right)={\displaystyle \frac{{\Delta }_1}{\left({\gamma }_0+{\gamma }_2\right)\left(\lambda +\mu +{\gamma }_1+x\left(\tau \right)\right)+\left({\gamma }_1+{\gamma }_2\right)\left(\lambda +x\left(\tau \right)\right)}},\hfill \\ g_2\left(x\left(\tau \right)\right)=-g_0\left(x\left(\tau \right)\right)-g_1\left(x\left(\tau \right)\right),\hfill \\ {\Delta }_0=\left({\gamma }_1+{\gamma }_2\right)\left[x\left(\tau \right)R_0\left(x\left(\tau \right)\right)+\left(a\left(x\right(\tau \left)\right)+qx\left(\tau \right)\right)R_1\left(x\left(\tau \right)\right)\right]\hfill \\ +\left(\lambda +\mu +{\gamma }_1+x\left(\tau \right)\right)\left[\left(a\left(x\right(\tau \left)\right)+qx\left(\tau \right)-\lambda \right)R_2\left(x\left(\tau \right)\right)-{\gamma }_1{R}_1\left(x\left(\tau \right)\right)\right],\hfill \\ {\Delta }_1=\left(\lambda +x\left(\tau \right)\right)\left[\left(a\left(x\right(\tau \left)\right)+qx\left(\tau \right)-\lambda \right)R_2\left(x\left(\tau \right)\right)-{\gamma }_1{R}_1\left(x\left(\tau \right)\right)\right]\hfill \\ -\left({\gamma }_0+{\gamma }_2\right)\left[x\left(\tau \right)R_0\left(x\left(\tau \right)\right)+\left(a\left(x\right(\tau \left)\right)+qx\left(\tau \right)\right)R_1\left(x\left(\tau \right)\right)\right].\hfill \end{array}\right.$$

(13)

3.1.4. Diffusion Approximation

From (9), (10) with (8), and (10)–(12), we obtain

$${\displaystyle \frac{\partial \mathrm{\Phi }(w,\tau )}{\partial \tau }}=a^{\prime }\left(x\left(\tau \right)\right)w{\displaystyle \frac{\partial \mathrm{\Phi }(w,\tau )}{\partial w}}+b\left(x\left(\tau \right)\right){\displaystyle \frac{{\left(jw\right)}^2}{2}}\mathrm{\Phi }(w,\tau ),$$

where the diffusion coefficient is obtained as follows.

$$\begin{array}{c}b\left(x\left(\tau \right)\right)=a\left(x\left(\tau \right)\right)+2(\left(2\lambda +{\gamma }_1+x\left(\tau \right)\right)g_1\left(x\left(\tau \right)\right)+\lambda g_2\left(x\left(\tau \right)\right)\\ -x\left(\tau \right)g_0\left(x\left(\tau \right)\right)+x\left(\tau \right)R_0\left(x\left(\tau \right)\right)+\lambda R_1\left(x\left(\tau \right)\right)+qx\left(\tau \right)).\end{array}$$

(14)

The Fokker–Plank equation for the probability density of a diffusion process $y\left(\tau \right)$ with drift coefficient $a^{\prime }\left(x\left(\tau \right)\right)y\left(\tau \right)$ and diffusion coefficient $b\left(x\right(\tau \left)\right)$ has the following form.

$${\displaystyle \frac{\partial P\left(y\right(\tau ),\tau )}{\partial \tau }}=-a^{\prime }\left(x\left(\tau \right)\right){\displaystyle \frac{\partial \left\{y\left(\tau \right)P\left(y\right(\tau ),\tau )\right\}}{\partial y\left(\tau \right)}}+{\displaystyle \frac{b\left(x\right(\tau \left)\right)}{2}}{\displaystyle \frac{{\partial }^{2}P(y\left(\tau \right),\tau )}{\partial y^2\left(\tau \right)}},$$

Here, $y\left(\tau \right)$ is the solution of stochastic differential Equation (15):

$$dy\left(\tau \right)=a^{\prime }\left(x\left(\tau \right)\right)y\left(\tau \right)d\tau +\sqrt{b\left(x\right(\tau \left)\right)}d\omega \left(\tau \right),$$

(15)

where $\omega \left(\tau \right)$ is the Wiener process.

We introduce diffusion process $z\left(\tau \right)=x\left(\tau \right)+\epsilon y\left(\tau \right)$.

$$dz\left(\tau \right)=a\left(z\left(\tau \right)\right)d\tau +\epsilon \sqrt{b\left(z\right(\tau \left)\right)}d\omega \left(\tau \right).$$

The Fokker–Plank equation for $\mathrm{\Pi }(z\left(\tau \right),\tau )=\partial P\left\{z\left(\tau \right)<z\right\}/\partial z$ has the following form.

$${\displaystyle \frac{\partial \mathrm{\Pi }\left(z\right(\tau ),\tau )}{\partial \tau }}=-{\displaystyle \frac{\partial \left\{a\left(z\right(\tau \left)\right)\mathrm{\Pi }\left(z\right(\tau ),\tau )\right\}}{\partial z\left(\tau \right)}}+{\displaystyle \frac{{\epsilon }^2}{2}}{\displaystyle \frac{{\partial }^2\left\{b\left(z\right(\tau \left)\right)\mathrm{\Pi }\left(z\right(\tau ),\tau )\right\}}{\partial z^2\left(\tau \right)}}.$$

(16)

The solution of Equation (16) is

$$\mathrm{\Pi }\left(z\right)={\displaystyle \frac{C}{b\left(z\right)}}exp\left\{{\displaystyle \frac{2}{\sigma }}\underset{0}{\overset{z}{\int }}{\displaystyle \frac{a\left(x\right)}{b\left(x\right)}}dx\right\},C-constant.$$

(17)

Finally, we can obtain diffusion approximation $P_{diffusion}\left(i\right)$ for stationary distribution $P\left(i\right)$ of the number of calls in the orbit:

$$P_{\mathrm{diffusion}}\left(i\right)={\displaystyle \frac{\mathrm{\Pi }\left(i\sigma \right)}{{\displaystyle \sum _{k=0}^{\infty }}\mathrm{\Pi }\left(k\sigma \right)}},$$

(18)

where $\mathrm{\Pi }\left(z\right)$ is determined in (17).

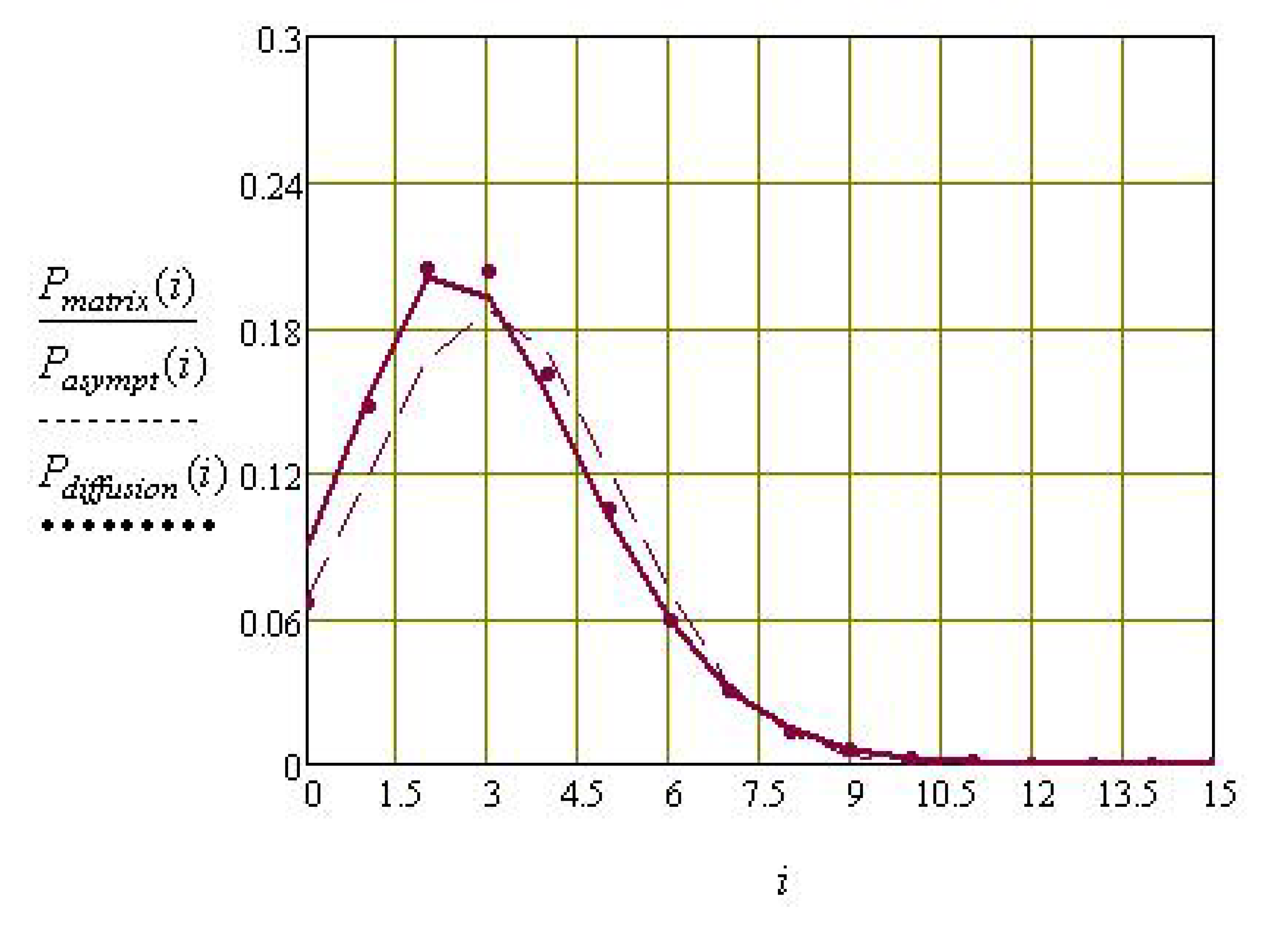
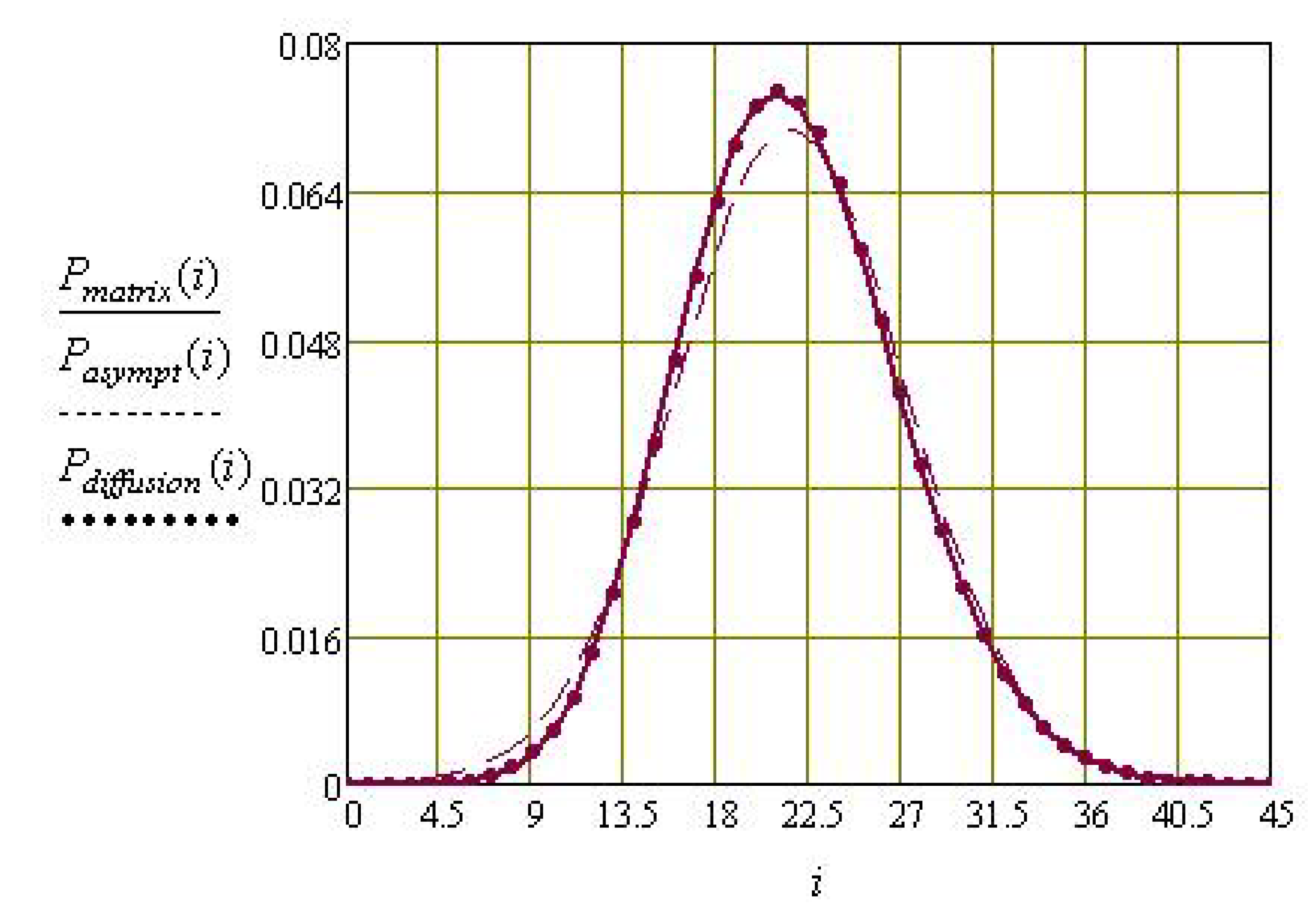
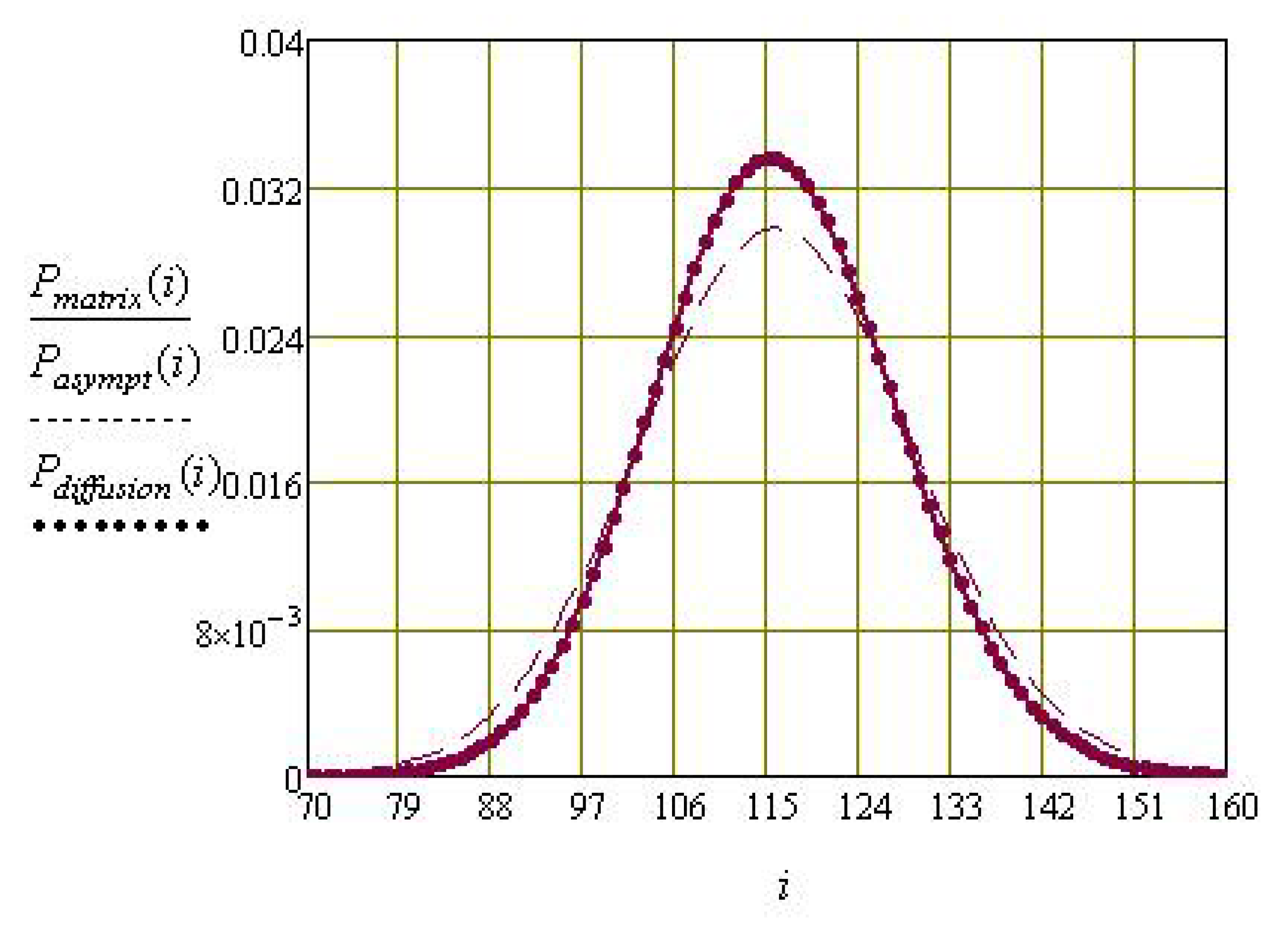
3.2. Numerical Results

To compare the pre-limit probability distribution of the number of calls in the orbit of the considered queueing system, $P_{\mathrm{matrix}}\left(i\right)$, calculated via the matrix method and its approximation $P_{\mathrm{asympt}}\left(i\right)$, and $P_{\mathrm{diffusion}}\left(i\right)$ (18), constructed by using the asymptotic analysis method published in [1] and asymptotic diffusion analysis method for different values of the system’s parameters, we use Kolmogorov distance $\Delta$ between the respective distribution functions.

$$\left\{\begin{array}{c}{\Delta }_{\mathrm{AA}}=\underset{0\le n\le N}{max}\left|\sum _{i=0}^n{P}_{\mathrm{matrix}}\left(i\right)-\sum _{i=0}^n{P}_{\mathrm{asympt}}\left(i\right)\right|,\mathrm{for}\mathrm{asymptotic}\mathrm{analysis},\hfill \\ {\Delta }_{\mathrm{ADA}}=\underset{0\le n\le N}{max}\left|\sum _{i=0}^n{P}_{\mathrm{matrix}}\left(i\right)-\sum _{i=0}^n{P}_{\mathrm{diffusion}}\left(i\right)\right|,\mathrm{for}\mathrm{asymptotic}\mathrm{diffusion}\mathrm{analysis}.\hfill \end{array}\right.$$

(19)

Table 1. Kolmogorov distance.

$\mathit{\lambda }/\mathit{\mu }$	$\mathit{\sigma }=0.1$		$\mathit{\sigma }=0.01$		$\mathit{\sigma }=0.005$
	${\mathbf{\Delta }}_{\mathbf{AA}}$	${\mathbf{\Delta }}_{\mathbf{ADA}}$	${\mathbf{\Delta }}_{\mathbf{AA}}$	${\mathbf{\Delta }}_{\mathbf{ADA}}$	${\mathbf{\Delta }}_{\mathbf{AA}}$	${\mathbf{\Delta }}_{\mathbf{ADA}}$
$0.5$	0.1610	0.0820	0.0230	0.0057	0.0160	0.0039
$0.7$	0.1170	0.0490	0.0200	0.0041	0.0160	0.0028
$0.9$	0.0920	0.0260	0.0210	0.0031	0.0200	0.0021
$1.1$	0.0750	0.0190	0.0240	0.0025	0.0220	0.0017
$1.5$	0.0550	0.0090	0.0300	0.0017	0.0290	0.0012
$2.0$	0.0460	0.0060	0.0353	0.0012	0.0350	0.0008

shows the results of calculating the Kolmogorov distances for various values of delay parameter $\sigma$ and parameter $\lambda$ when $\mu =1$, ${\gamma }_0=0.1$, ${\gamma }_1=0.2$, ${\gamma }_2=1$, and $\alpha =2\sigma$.

The values of the Kolmogorov distance from Table 1 and Figure 2, Figure 3, Figure 4 and Figure 5, which show the comparison of the pre-limit, asymptotic and asymptotic diffusion distributions for various values of the delay parameter $\sigma$, and parameter $\lambda$, allow us to draw the following conclusions:

• For a fixed system load $\lambda /\mu$, as $\sigma$ decreases, the Kolmogorov distance $\Delta$ decreases;
• At a fixed value of the delay time parameter of the call in orbit $\sigma$ and $\alpha$, with an increase in the value of system load $\lambda /\mu$, the Kolmogorov distance ${\Delta }_{\mathrm{AA}}$ decreases for $\sigma \ge 0.1$ and increases for $\sigma \le 0.01$ (this behavior of $\Delta$ is typical for systems with collisions), and the Kolmogorov distance ${\Delta }_{\mathrm{ADA}}$ decreases.

Knowing the probability distribution of the number of calls in orbit (18), we can find some other characteristics of the system, such as the following:

• $ITF=\lambda +\sigma {\displaystyle \sum _{i=0}^N}(i{P}_{\mathrm{diffusion}}\left(i\right))$ is the intensity of the total flow;
• $RC={\displaystyle \sum _{i=0}^N}(i{P}_{\mathrm{diffusion}}\left(i\right))$ is the average number of the customer’s repeating request;
• $RCPF={\displaystyle \frac{\sigma ·RC}{\lambda }}$ is the ratio of repeated calls in the primary flow;
• $RCTF={\displaystyle \frac{\sigma ·RC}{ITF}}$ is the ratio of repeated calls in the total flow;
• $NpCTF={\displaystyle \frac{q\sigma ·RC}{ITF}}$ is the ratio of calls leaving the orbit without service in the total flow;
• $CCPF={\displaystyle \frac{ITF·R_1}{\lambda }}$ is the ratio of calls that are in collision in the flow of primary calls.

As an example,

Table 2. Characteristics of the system ($\lambda =0.9$).

$\mathit{\sigma }$	$\mathit{ITF}$	$\mathit{RC}$	$\mathit{RCPF}$	$\mathit{RCTF}$	$\mathit{NpCTF}$	$\mathit{CCPF}$
$0.01$	1.202905	30.290522	0.336561	0.279790	0.673123	0.354839
$0.005$	1.202867	60.573451	0.336519	0.279764	0.673038	0.354828

shows the values of these characteristics for $\lambda =0.9$.

4. Discussion and Conclusions

The retrial queueing system of the $M/M/1$ type with impatient customers, collisions, and the unreliable server was investigated with the following methods:

• The asymptotic analysis method: see [1];
• The numerical method: see [1];
• The asymptotic diffusion analysis method: a new contribution.

As an asymptotic condition for both methods, the system was taken as a condition for a long delay of calls in orbit and a long period of patience with respect to calls in orbit. Both methods resulted in the conclusion that the number of calls in the orbit is asymptotically normal. The asymptotic diffusion analysis method is more accurate than the asymptotic analysis method under the same asymptotic condition in terms of the Kolmogorov distance.

In the future, we plan to find the sensitivity of the diffusion approximation relative to the exogenous parameters of the system and supplement this study with a simulation model for calculating the temporal characteristics of the system.