Thermo-Elasticity of Materials from Quasi-Harmonic Calculations
Abstract
1. Introduction
2. Theoretical Aspects
2.1. The Athermal Elastic Tensor
2.1.1. Calculation from Analytical Forces
2.1.2. Calculation from the Energy
2.2. The Elastic Tensor at a Finite Temperature
- The determination of the equilibrium structure of the system at temperature T;
- The calculation of the second free-energy derivatives with respect to strain.
2.2.1. The Equilibrium Structure at a Finite Temperature
2.2.2. Free Energy Derivatives with Respect to Strain
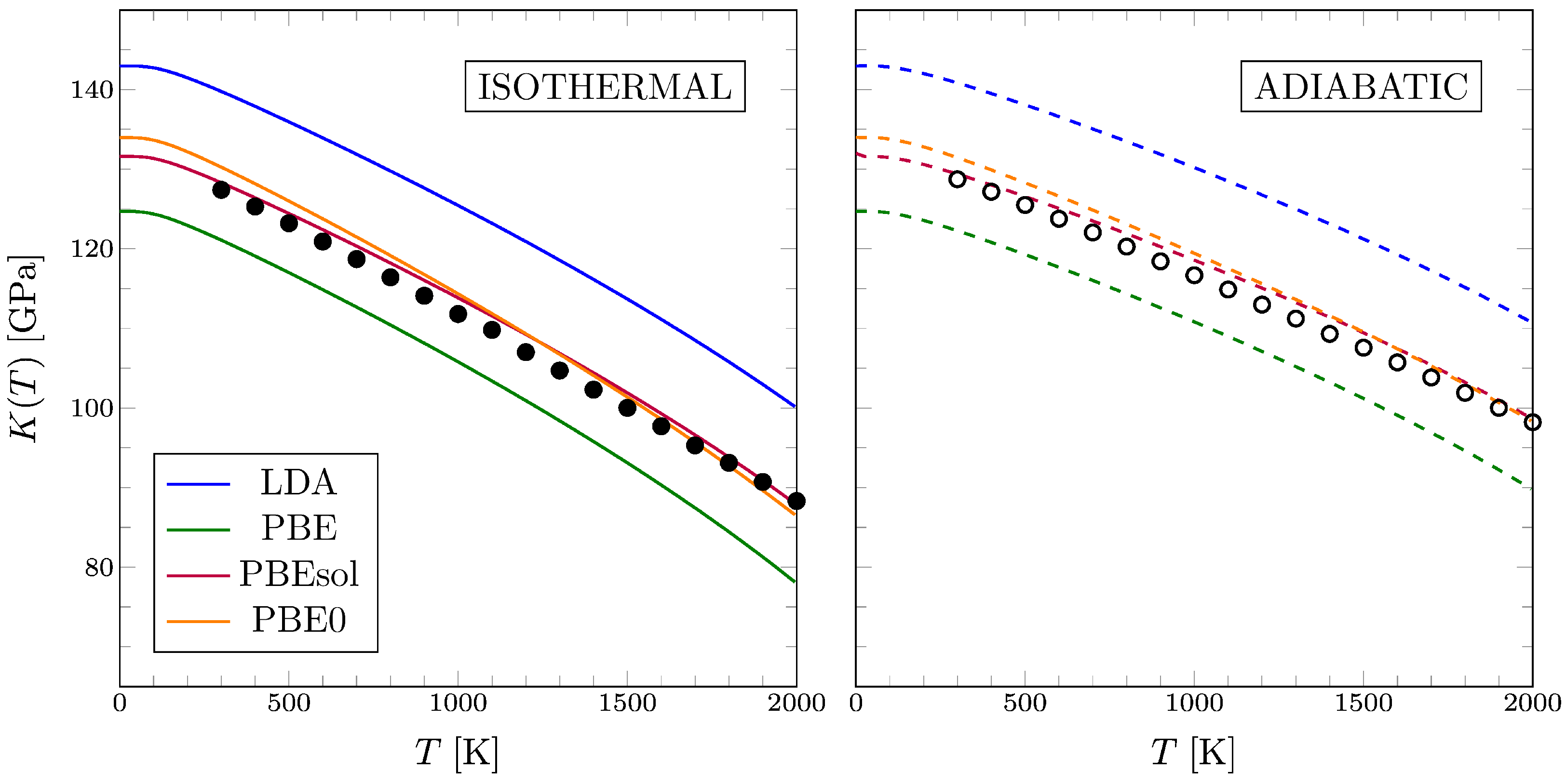
2.2.3. Adiabatic versus Isothermal Elastic Moduli
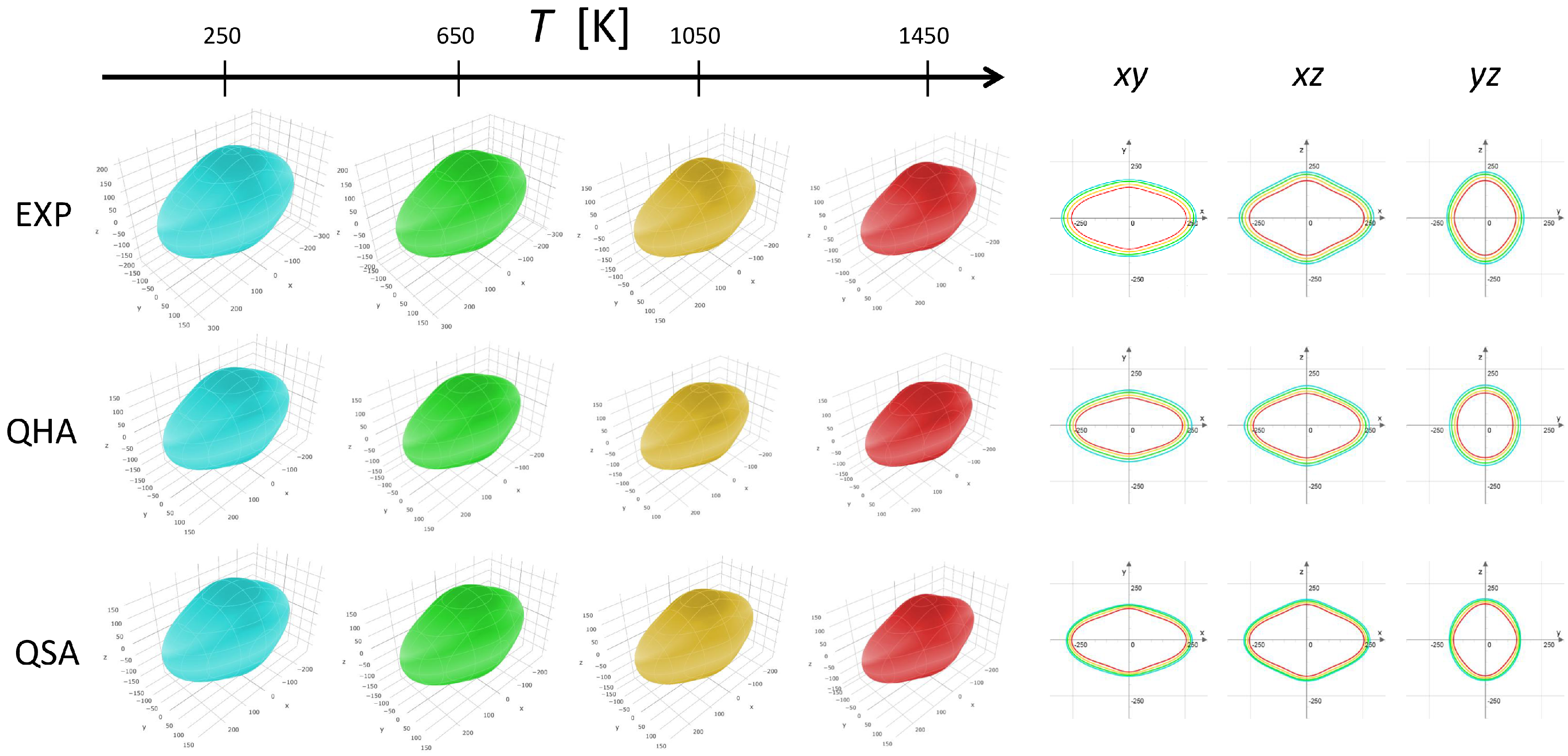
2.2.4. The Quasi-Static Approximation to Thermo-Elastic Moduli
3. The Implemented Algorithm
- A full structural relaxation of the system is performed (both atomic positions and lattice parameters are optimized). The static equilibrium structure, with volume , is obtained.
- A space group symmetry-preserving QHA calculation is performed, which provides the thermal expansion of the system. A fully-automated algorithm is implemented in the Crystal program to perform this task [22,23], where four different volumes are explored (compressed and expanded with respect to ). For each volume, a volume-constrained, lattice symmetry-preserving structural relaxation is performed and phonon frequencies computed. By minimizing at several temperatures, the relation is determined. As a result, lattice parameters as a function of temperature are obtained.
- A value of temperature T is selected. Starting from the values of the lattice parameters at this temperature obtained at the end of the previous step, a volume-constrained, lattice symmetry-preserving structural relaxation is performed to get the equilibrium structure (also in terms of atomic positions) at the desired temperature.
- A given strain shape is chosen, which will provide a linear combination of elastic stiffness constants according to Equation (16).
- The second free energy derivatives with respect to the strain are computed as discussed in Section 2.2.2. A fully-automated algorithm has been implemented in the Crystal program for this task. The starting point is represented by the optimized structure obtained at the end of step 3 above (i.e., the equilibrium structure at temperature T). The structure is deformed, in terms of the strain shape , into four strained configurations (two with positive and two with negative strain amplitude ). At each strained configuration, atomic positions are relaxed and phonon frequencies computed. The computed quasi-harmonic free energy as a function of strain amplitude is fitted to a second-order polynomial and the corresponding second-derivative determined.
4. Computational Parameters
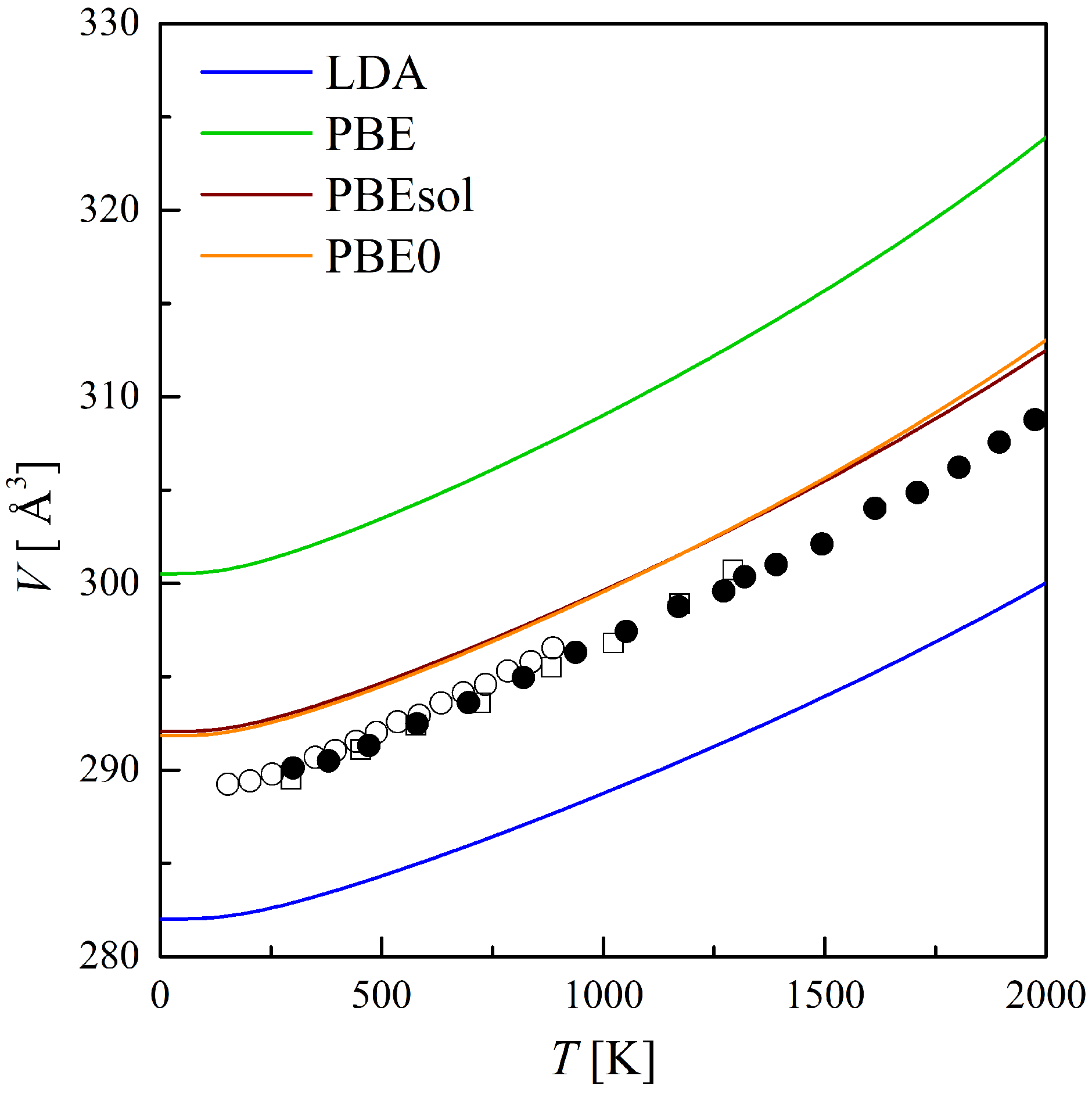
5. Results and Discussion
6. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Kuehmann, C.J.; Olson, G.B. Computational materials design and engineering. Mater. Sci. Technol. 2009, 25, 472–478. [Google Scholar] [CrossRef]
- Hafner, J.; Wolverton, C.; Ceder, G. Toward Computational Materials Design: The Impact of Density Functional Theory on Materials Research. MRS Bull. 2006, 31, 659–668. [Google Scholar] [CrossRef]
- Curtarolo, S.; Hart, G.; Nardelli, M.; Mingo, N.; Sanvito, S.; Levy, O. The high-throughput highway to computational materials design. Nat. Mater. 2013, 12, 191–201. [Google Scholar] [CrossRef] [PubMed]
- Varini, N.; Ceresoli, D.; Martin-Samos, L.; Girotto, I.; Cavazzoni, C. Enhancement of DFT-calculations at petascale: Nuclear Magnetic Resonance, Hybrid Density Functional Theory and Car-Parrinello calculations. Comput. Phys. Commun. 2013, 184, 1827–1833. [Google Scholar] [CrossRef]
- Corsetti, F. Performance Analysis of Electronic Structure Codes on HPC Systems: A Case Study of SIESTA. PLoS ONE 2014, 9, e95390. [Google Scholar] [CrossRef] [PubMed]
- Hutter, J.; Iannuzzi, M.; Schiffmann, F.; VandeVondele, J. CP2K: Atomistic simulations of condensed matter systems. WIREs Comput. Mol. Sci. 2014, 4, 15–25. [Google Scholar] [CrossRef]
- Maniopoulou, A.; Davidson, E.R.; Grau-Crespo, R.; Walsh, A.; Bush, I.J.; Catlow, C.R.A.; Woodley, S.M. Introducing k-point parallelism into VASP. Comput. Phys. Commun. 2012, 183, 1696–1701. [Google Scholar] [CrossRef]
- Kendall, R.A.; Aprá, E.; Bernholdt, D.E.; Bylaska, E.J.; Dupuis, M.; Fann, G.I.; Harrison, R.J.; Ju, J.; Nichols, J.A.; Nieplocha, J.; et al. High performance computational chemistry: An overview of NWChem a distributed parallel application. Comput. Phys. Commun. 2000, 128, 260–283. [Google Scholar] [CrossRef]
- Orlando, R.; Delle Piane, M.; Bush, I.J.; Ugliengo, P.; Ferrabone, M.; Dovesi, R. A new massively parallel version of CRYSTAL for large systems on high performance computing architectures. J. Comput. Chem. 2012, 33, 2276–2284. [Google Scholar] [CrossRef]
- Bush, I.J.; Tomic, S.; Searle, B.G.; Mallia, G.; Bailey, C.L.; Montanari, B.; Bernasconi, L.; Carr, J.M.; Harrison, N.M. Parallel implementation of the ab initio CRYSTAL program: Electronic structure calculations for periodic systems. Proc. R. Soc. A Math. Phys. Eng. Sci. 2011, 467, 2112. [Google Scholar] [CrossRef]
- Wen, S.; Nanda, K.; Huang, Y.; Beran, G.J.O. Practical quantum mechanics-based fragment methods for predicting molecular crystal properties. Phys. Chem. Chem. Phys. 2012, 14, 7578–7590. [Google Scholar] [CrossRef] [PubMed]
- Moellmann, J.; Grimme, S. DFT-D3 Study of Some Molecular Crystals. J. Phys. Chem. C 2014, 118, 7615–7621. [Google Scholar] [CrossRef]
- Bucko, T.; Hafner, J.; Lebegue, S.; Angyan, J.G. Improved Description of the Structure of Molecular and Layered Crystals: Ab Initio DFT Calculations with van der Waals Corrections. J. Phys. Chem. A 2010, 114, 11814–11824. [Google Scholar] [CrossRef] [PubMed]
- Tkatchenko, A.; DiStasio, R.A.; Car, R.; Scheffler, M. Accurate and Efficient Method for Many-Body van der Waals Interactions. Phys. Rev. Lett. 2012, 108, 236402. [Google Scholar] [CrossRef] [PubMed]
- Civalleri, B.; Zicovich-Wilson, C.; Valenzano, L.; Ugliengo, P. B3LYP augmented with an empirical dispersion term (B3LYP-D*) as applied to molecular crystals. CrystEngComm 2008, 10, 405. [Google Scholar] [CrossRef]
- Neumann, M.A.; Perrin, M.A. Energy Ranking of Molecular Crystals Using Density Functional Theory Calculations and an Empirical van der Waals Correction. J. Phys. Chem. B 2005, 109, 15531–15541. [Google Scholar] [CrossRef] [PubMed]
- Dovesi, R.; Orlando, R.; Erba, A.; Zicovich-Wilson, C.M.; Civalleri, B.; Casassa, S.; Maschio, L.; Ferrabone, M.; De La Pierre, M.; D’Arco, P.H.; et al. CRYSTAL14: A Program for the Ab initio Investigation of Crystalline Solids. Int. J. Quantum Chem. 2014, 114, 1287–1317. [Google Scholar] [CrossRef]
- Dovesi, R.; Erba, A.; Orlando, R.; Zicovich-Wilson, C.M.; Civalleri, B.; Maschio, L.; Rérat, M.; Casassa, S.; Baima, J.; Salustro, S.; et al. Quantum-Mechanical Condensed Matter Simulations with CRYSTAL. WIREs Comput. Mol. Sci. 2018, 8, e1360. [Google Scholar] [CrossRef]
- Erba, A.; Baima, J.; Bush, I.; Orlando, R.; Dovesi, R. Large Scale Condensed Matter DFT Simulations: Performance and Capabilities of the Crystal Code. J. Chem. Theory Comput. 2017, 13, 5019–5027. [Google Scholar] [CrossRef]
- Grimme, S.; Antony, J.; Ehrlich, S.; Krieg, H. A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 2010, 132, 154104. [Google Scholar] [CrossRef]
- Brandenburg, J.; Grimme, S. Dispersion Corrected Hartree-Fock and Density Functional Theory for Organic Crystal Structure Prediction. In Prediction and Calculation of Crystal Structures; Atahan-Evrenk, S., Aspuru-Guzik, A., Eds.; Topics in Current Chemistry; Springer: Berlin/Heidelberg, Germany, 2014; Volume 345, pp. 1–23. [Google Scholar]
- Erba, A. On combining temperature and pressure effects on structural properties of crystals with standard ab initio techniques. J. Chem. Phys. 2014, 141, 124115. [Google Scholar] [CrossRef] [PubMed]
- Erba, A.; Shahrokhi, M.; Moradian, R.; Dovesi, R. On How Differently the Quasi-harmonic Approximation Works for Two Isostructural Crystals: Thermal Properties of MgO and CaO. J. Chem. Phys. 2015, 142, 044114. [Google Scholar] [CrossRef] [PubMed]
- Erba, A.; Maul, J.; Demichelis, R.; Dovesi, R. Assessing Thermochemical Properties of Materials through Ab initio Quantum-mechanical Methods: The Case of α-Al2O3. Phys. Chem. Chem. Phys. 2015, 17, 11670–11677. [Google Scholar] [CrossRef] [PubMed]
- Erba, A.; Maul, J.; De La Pierre, M.; Dovesi, R. Structural and Elastic Anisotropy of Crystals at High Pressure and Temperature from Quantum-mechanical Methods: The Case of Mg2SiO4 Forsterite. J. Chem. Phys. 2015, 142, 204502. [Google Scholar] [CrossRef] [PubMed]
- Allen, R.E.; De Wette, F.W. Calculation of Dynamical Surface Properties of Noble-Gas Crystals. I. The Quasiharmonic Approximation. Phys. Rev. 1969, 179, 873–886. [Google Scholar] [CrossRef]
- Boyer, L.L. Calculation of Thermal Expansion, Compressiblity, an Melting in Alkali Halides: NaCl and KCl. Phys. Rev. Lett. 1979, 42, 584. [Google Scholar] [CrossRef]
- Davies, G. Effective elastic moduli under hydrostatic stress. Quasi-harmonic theory. J. Phys. Chem. Solids 1974, 35, 1513–1520. [Google Scholar] [CrossRef]
- Karki, B.B.; Wentzcovitch, R.M.; de Gironcoli, S.; Baroni, S. High-pressure lattice dynamics and thermoelasticity of MgO. Phys. Rev. B 2000, 61, 8793–8800. [Google Scholar] [CrossRef]
- Wu, Z.; Wentzcovitch, R.M. Quasiharmonic thermal elasticity of crystals: An analytical approach. Phys. Rev. B 2011, 83, 184115. [Google Scholar] [CrossRef]
- Karki, B.B.; Wentzcovitch, R.M.; de Gironcoli, S.; Baroni, S. First-Principles Determination of Elastic Anisotropy and Wave Velocities of MgO at Lower Mantle Conditions. Science 1999, 286, 1705–1707. [Google Scholar] [CrossRef]
- Isaak, D.G.; Anderson, O.L.; Goto, T.; Suzuki, I. Elasticity of single-crystal forsterite measured to 1700 K. J. Geophys. Res. Solid Earth 1989, 94, 5895–5906. [Google Scholar] [CrossRef]
- Doll, K. Analytical stress tensor and pressure calculations with the CRYSTAL code. Mol. Phys. 2010, 108, 223–227. [Google Scholar] [CrossRef]
- Nye, J.F. Physical Properties of Crystals; Oxford University Press: Oxford, UK, 1957. [Google Scholar]
- Doll, K.; Dovesi, R.; Orlando, R. Analytical Hartree-Fock gradients with respect to the cell parameter for systems periodic in three dimensions. Theor. Chem. Acc. 2004, 112, 394–402. [Google Scholar] [CrossRef]
- Doll, K.; Dovesi, R.; Orlando, R. Analytical Hartree-Fock gradients with respect to the cell parameter: Systems periodic in one and two dimensions. Theor. Chem. Acc. 2006, 115, 354–360. [Google Scholar] [CrossRef]
- Perger, W.F.; Criswell, J.; Civalleri, B.; Dovesi, R. Ab-initio calculation of elastic constants of crystalline systems with the CRYSTAL code. Comput. Phys. Commun. 2009, 180, 1753–1759. [Google Scholar] [CrossRef]
- Erba, A.; Mahmoud, A.; Orlando, R.; Dovesi, R. Elastic Properties of Six Silicate Garnet End-members from Accurate Ab initio Simulations. Phys. Chem. Miner. 2014, 41, 151–160. [Google Scholar] [CrossRef]
- Erba, A. The Internal-Strain Tensor of Crystals for Nuclear-relaxed Elastic and Piezoelectric Constants: On the Full Exploitation of its Symmetry Features. Phys. Chem. Chem. Phys. 2016, 18, 13984–13992. [Google Scholar] [CrossRef]
- Erba, A.; Caglioti, D.; Zicovich-Wilson, C.M.; Dovesi, R. Nuclear-relaxed Elastic and Piezoelectric Constants of Materials: Computational Aspects of Two Quantum-mechanical Approaches. J. Comput. Chem. 2017, 38, 257–264. [Google Scholar] [CrossRef]
- Ashcroft, N.W.; Mermin, N.D. Solid State Physics; Saunders College: Philadelphia, PA, USA, 1976. [Google Scholar]
- Baroni, S.; Giannozzi, P.; Isaev, E. Density-Functional Perturbation Theory for Quasi-Harmonic Calculations. Rev. Miner. Geochem. 2010, 71, 39–57. [Google Scholar] [CrossRef]
- Erba, A.; Maul, J.; Civalleri, B. Thermal Properties of Molecular Crystals through Dispersion-corrected Quasi-harmonic Ab initio Calculations: The Case of Urea. Chem. Commun. 2016, 52, 1820–1823. [Google Scholar] [CrossRef]
- Erba, A.; Maul, J.; Itou, M.; Dovesi, R.; Sakurai, Y. Anharmonic Thermal Oscillations of the Electron Momentum Distribution in Lithium Fluoride. Phys. Rev. Lett. 2015, 115, 117402. [Google Scholar] [CrossRef] [PubMed]
- Maul, J.; Santos, I.M.G.; Sambrano, J.R.; Erba, A. Thermal properties of the orthorhombic CaSnO3 perovskite under pressure from ab initio quasi-harmonic calculations. Theor. Chem. Acc. 2016, 135, 1–9. [Google Scholar] [CrossRef]
- Ruggiero, M.T.; Zeitler, J.; Erba, A. Intermolecular Anharmonicity in Molecular Crystals: Interplay between Experimental Low-Frequency Dynamics and Quantum Quasi-Harmonic Simulations of Solid Purine. Chem. Commun. 2017, 53, 3781–3784. [Google Scholar] [CrossRef] [PubMed]
- Brandenburg, J.G.; Potticary, J.; Sparkes, H.A.; Price, S.L.; Hall, S.R. Thermal Expansion of Carbamazepine: Systematic Crystallographic Measurements Challenge Quantum Chemical Calculations. J. Phys. Chem. Lett. 2017, 8, 4319–4324. [Google Scholar] [CrossRef] [PubMed]
- Belousov, R.I.; Filatov, S.K. Algorithm for calculating the thermal expansion tensor and constructing the thermal expansion diagram for crystals. Glass Phys. Chem. 2007, 33, 271–275. [Google Scholar] [CrossRef]
- Paufler, P.; Weber, T. On the determination of linear expansion coefficients of triclinic crystals using X-ray diffraction. Eur. J. Mineral. 1999, 11, 721. [Google Scholar] [CrossRef]
- Fortes, A.D.; Wood, I.G.; Knight, K.S. The crystal structure and thermal expansion tensor of MgSO4–11D2O(meridianiite) determined by neutron powder diffraction. Phys. Chem. Miner. 2008, 35, 207–221. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, J.J.; Zhang, H.; Manga, V.R.; Shang, S.L.; Chen, L.Q.; Liu, Z.K. A first-principles approach to finite temperature elastic constants. J. Phys. Condens. Matter 2010, 22, 225404. [Google Scholar] [CrossRef] [PubMed]
- Kádas, K.; Vitos, L.; Ahuja, R.; Johansson, B.; Kollár, J. Temperature-dependent elastic properties of α-beryllium from first principles. Phys. Rev. B 2007, 76, 235109. [Google Scholar] [CrossRef]
- Shang, S.L.; Zhang, H.; Wang, Y.; Liu, Z.K. Temperature-dependent elastic stiffness constants of α- and θ-Al2O3 from first-principles calculations. J. Phys. Condens. Matter 2010, 22, 375403. [Google Scholar] [CrossRef]
- De La Pierre, M.; Orlando, R.; Maschio, L.; Doll, K.; Ugliengo, P.; Dovesi, R. Performance of six functionals (LDA, PBE, PBESOL, B3LYP, PBE0 and WC1LYP) in the simulation of vibrational and dielectric properties of crystalline compounds. The case of forsterite Mg2SiO4. J. Comp. Chem. 2011, 32, 1775–1784. [Google Scholar] [CrossRef] [PubMed]
- Bouhifd, M.A.; Andrault, D.; Fiquet, G.; Richet, P. Thermal expansion of forsterite up to the melting point. Geophys. Res. Lett. 1996, 23, 1143–1146. [Google Scholar] [CrossRef]
- Ye, Y.; Schwering, R.A.; Smyth, J.R. Effects of hydration on thermal expansion of forsterite, wadsleyite, and ringwoodite at ambient pressure. Am. Mineral. 2009, 94, 899–904. [Google Scholar] [CrossRef]
- Hazen, R.M. Effects of temperature and pressure on the crystal structure of forsterite. Am. Mineral. 1976, 61, 1280–1293. [Google Scholar]
- Anderson, O.L.; Isaak, D.; Oda, H. High-temperature elastic constant data on minerals relevant to geophysics. Rev. Geophys. 1992, 30, 57–90. [Google Scholar] [CrossRef]
- Howarth, A.; Liu, Y.; Li, P.; Li, Z.; Wang, T.; Hupp, J.; Farha, O. Chemical, thermal and mechanical stabilities of metal-organic frameworks. Nat. Rev. Mater. 2016, 1, 15018. [Google Scholar] [CrossRef]
- Marmier, A.; Lethbridge, Z.A.; Walton, R.I.; Smith, C.W.; Parker, S.C.; Evans, K.E. ElAM: A computer program for the analysis and representation of anisotropic elastic properties. Comput. Phys. Commun. 2010, 181, 2102–2115. [Google Scholar] [CrossRef]

© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Destefanis, M.; Ravoux, C.; Cossard, A.; Erba, A. Thermo-Elasticity of Materials from Quasi-Harmonic Calculations. Minerals 2019, 9, 16. https://doi.org/10.3390/min9010016
Destefanis M, Ravoux C, Cossard A, Erba A. Thermo-Elasticity of Materials from Quasi-Harmonic Calculations. Minerals. 2019; 9(1):16. https://doi.org/10.3390/min9010016
Chicago/Turabian StyleDestefanis, Maurizio, Corentin Ravoux, Alessandro Cossard, and Alessandro Erba. 2019. "Thermo-Elasticity of Materials from Quasi-Harmonic Calculations" Minerals 9, no. 1: 16. https://doi.org/10.3390/min9010016
APA StyleDestefanis, M., Ravoux, C., Cossard, A., & Erba, A. (2019). Thermo-Elasticity of Materials from Quasi-Harmonic Calculations. Minerals, 9(1), 16. https://doi.org/10.3390/min9010016




