Enhancement of Potential Field Source Boundaries Using the Hyperbolic Domain (Gudermannian Function)
Abstract
:1. Introduction
2. Hyperbolic Domain (Gudermannian Function) Filters
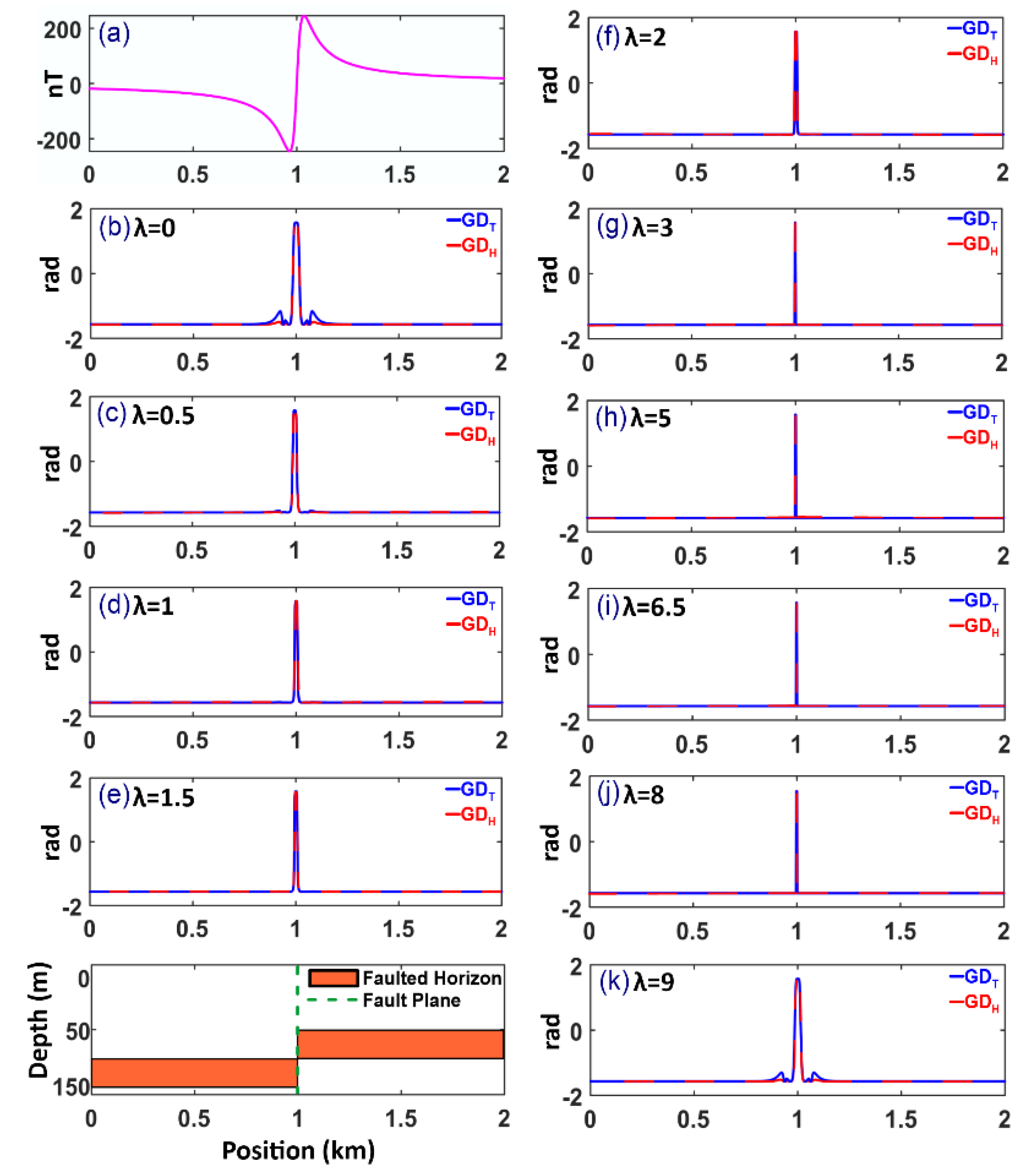
3. Evaluation of Parameter
4. Application to Simulated Profile Data
5. Application to Synthetic Data
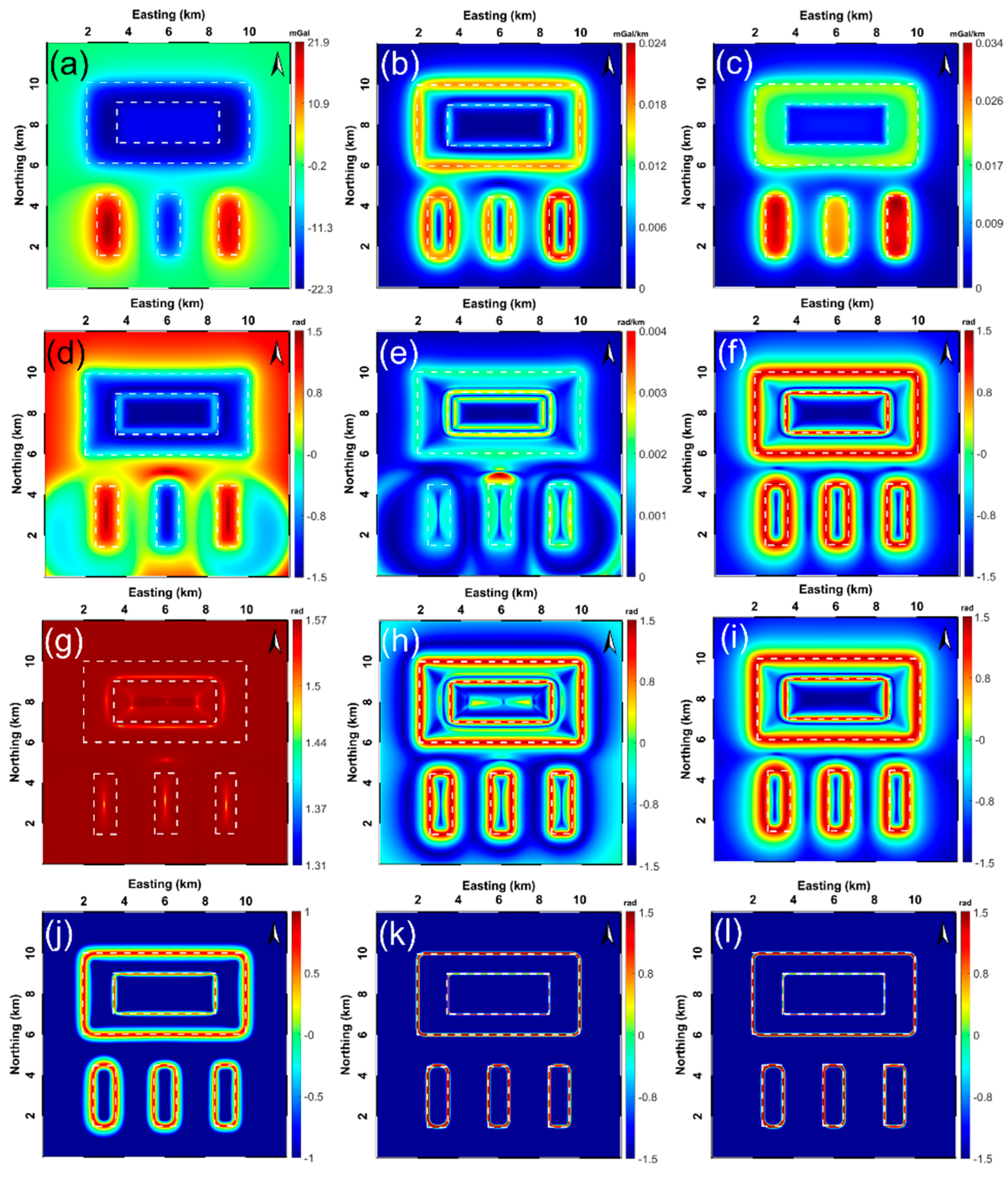
5.1. The Gravity Model
5.2. The Gravity Model with Noise Contamination
5.3. The Geomagnetic Model
5.4. The Geomagnetic Anomaly with Noise Corruption
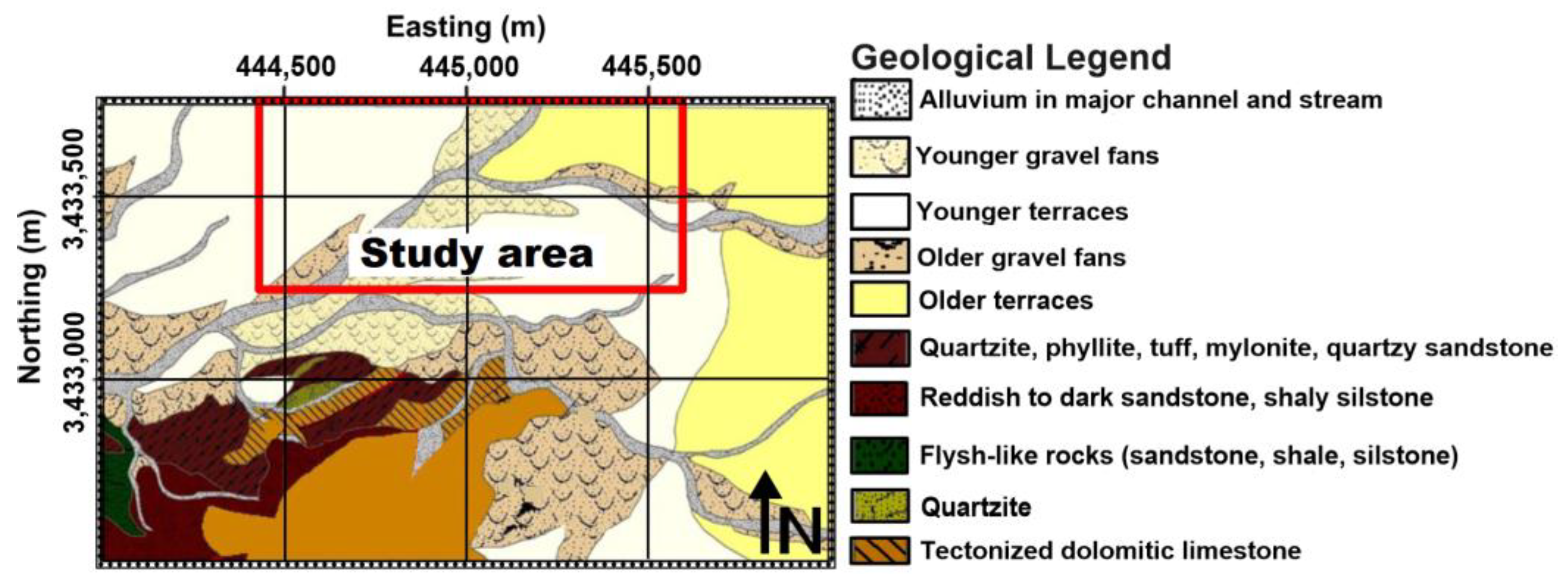
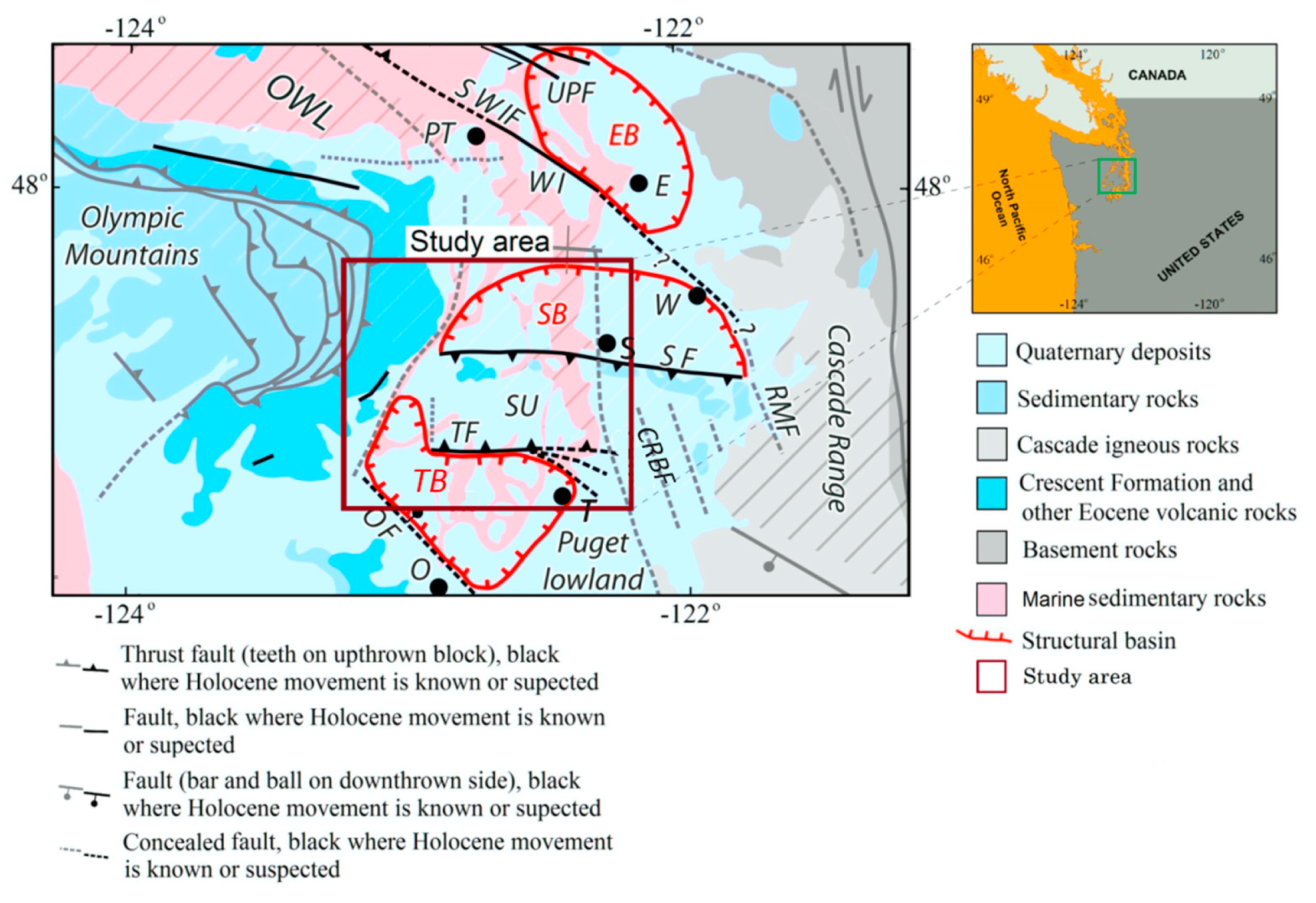
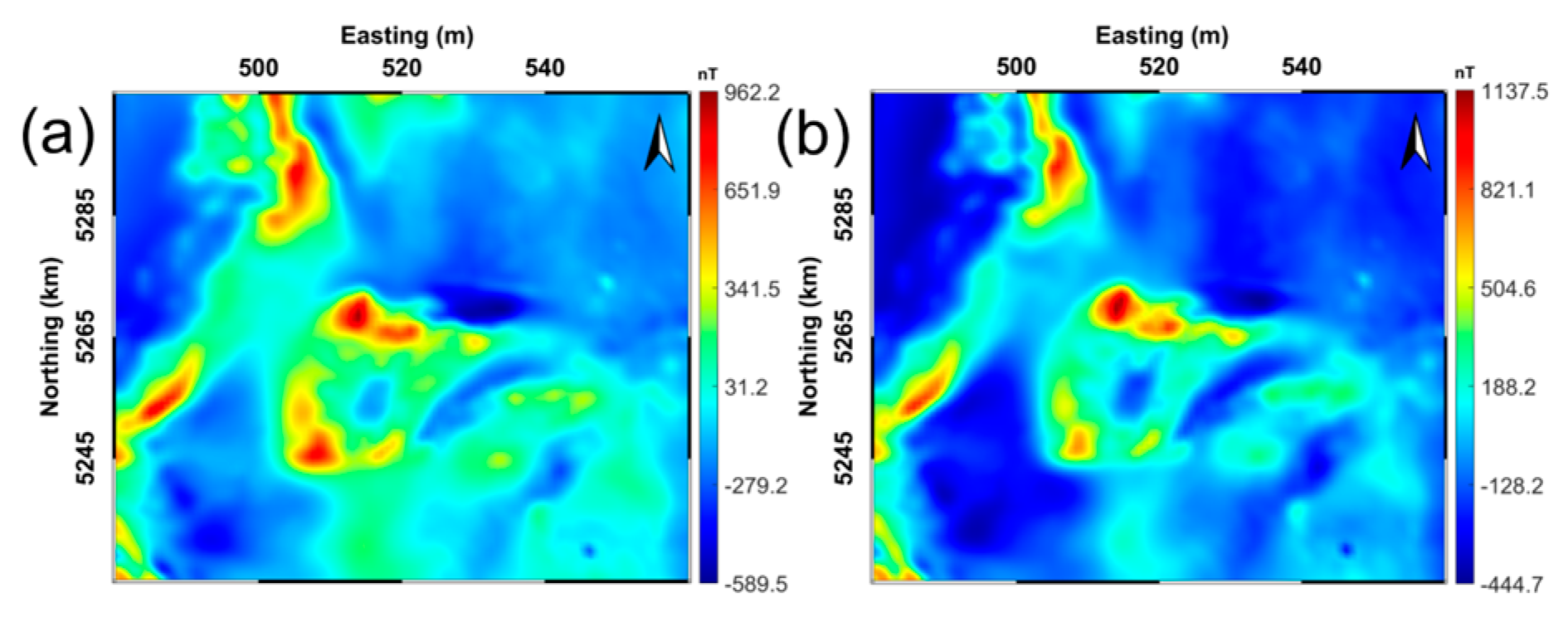
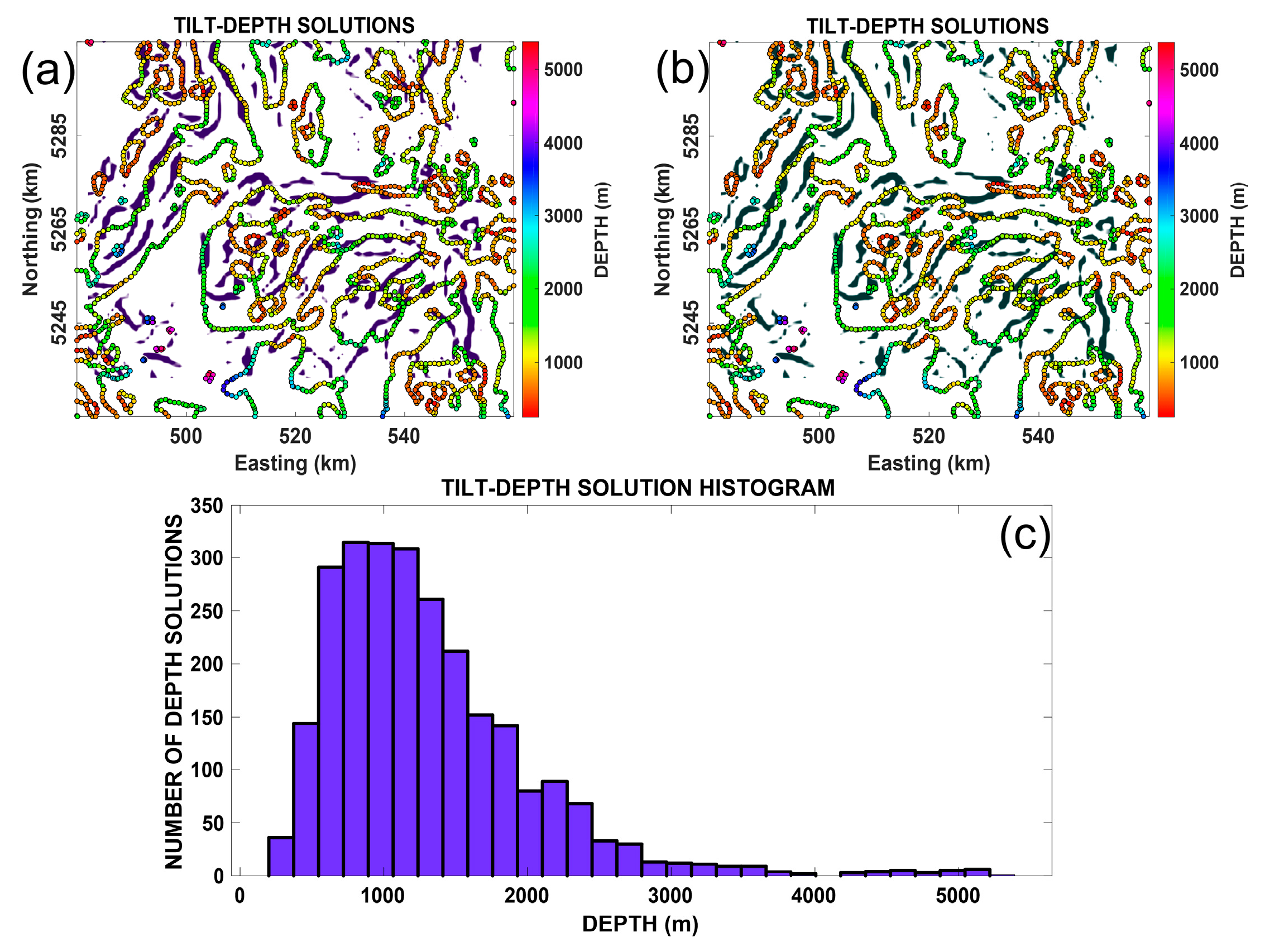
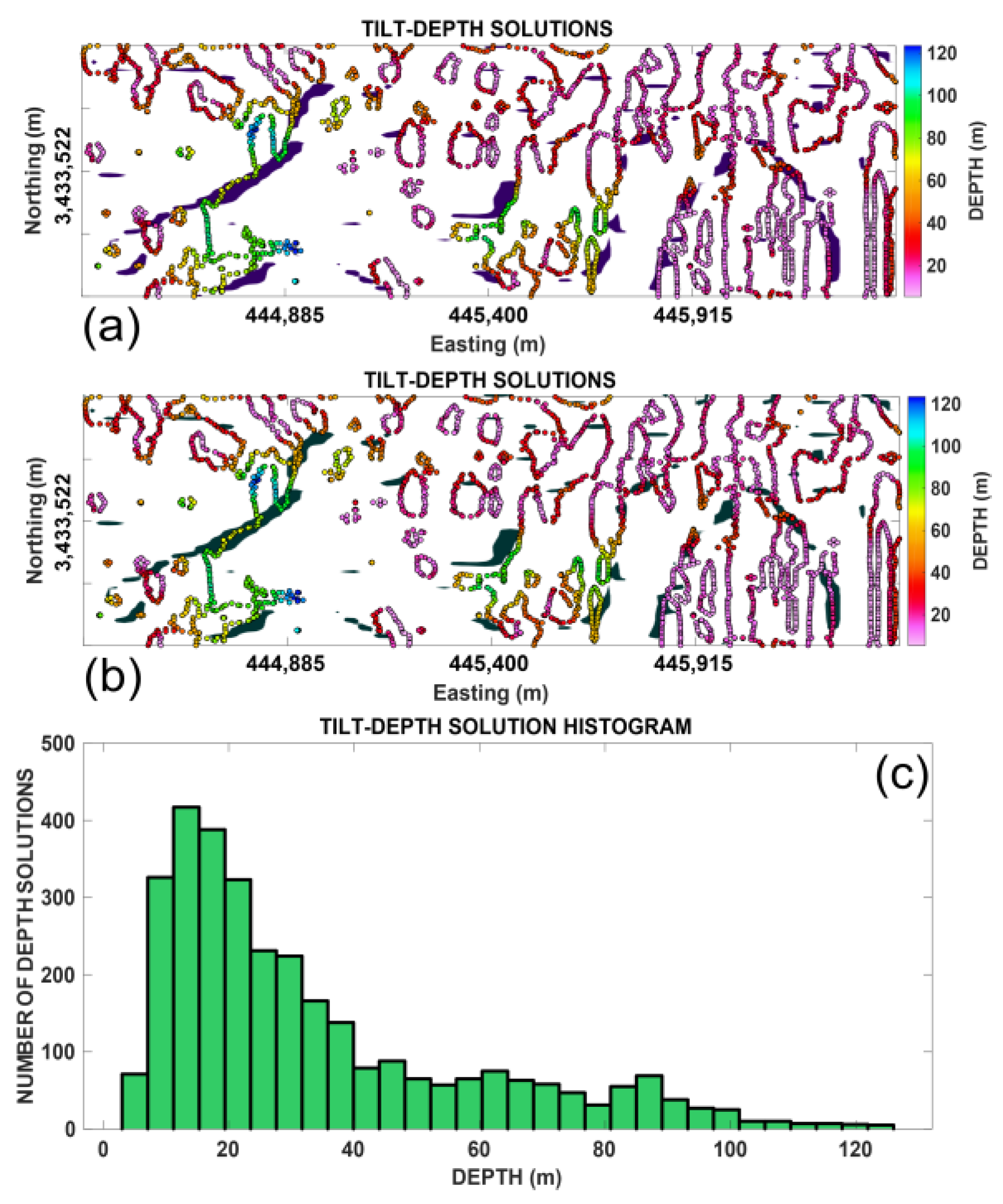
6. Application to Field Aeromagnetic Data
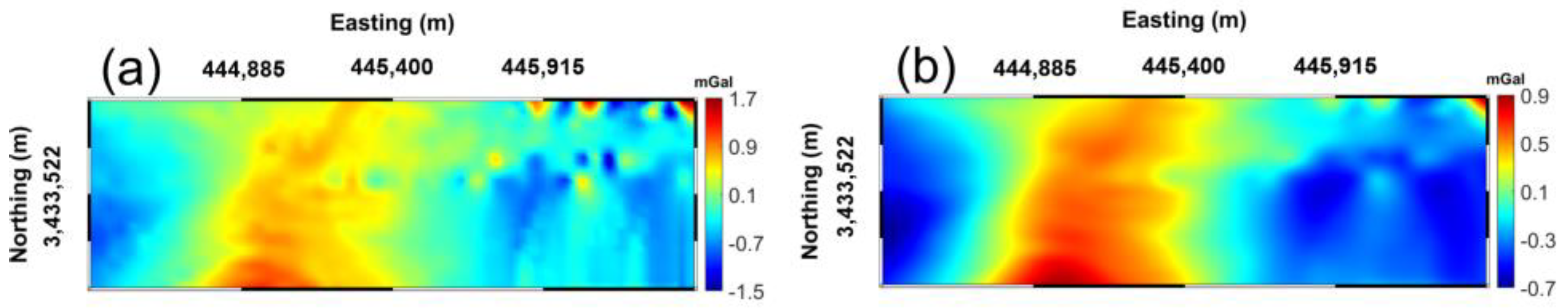
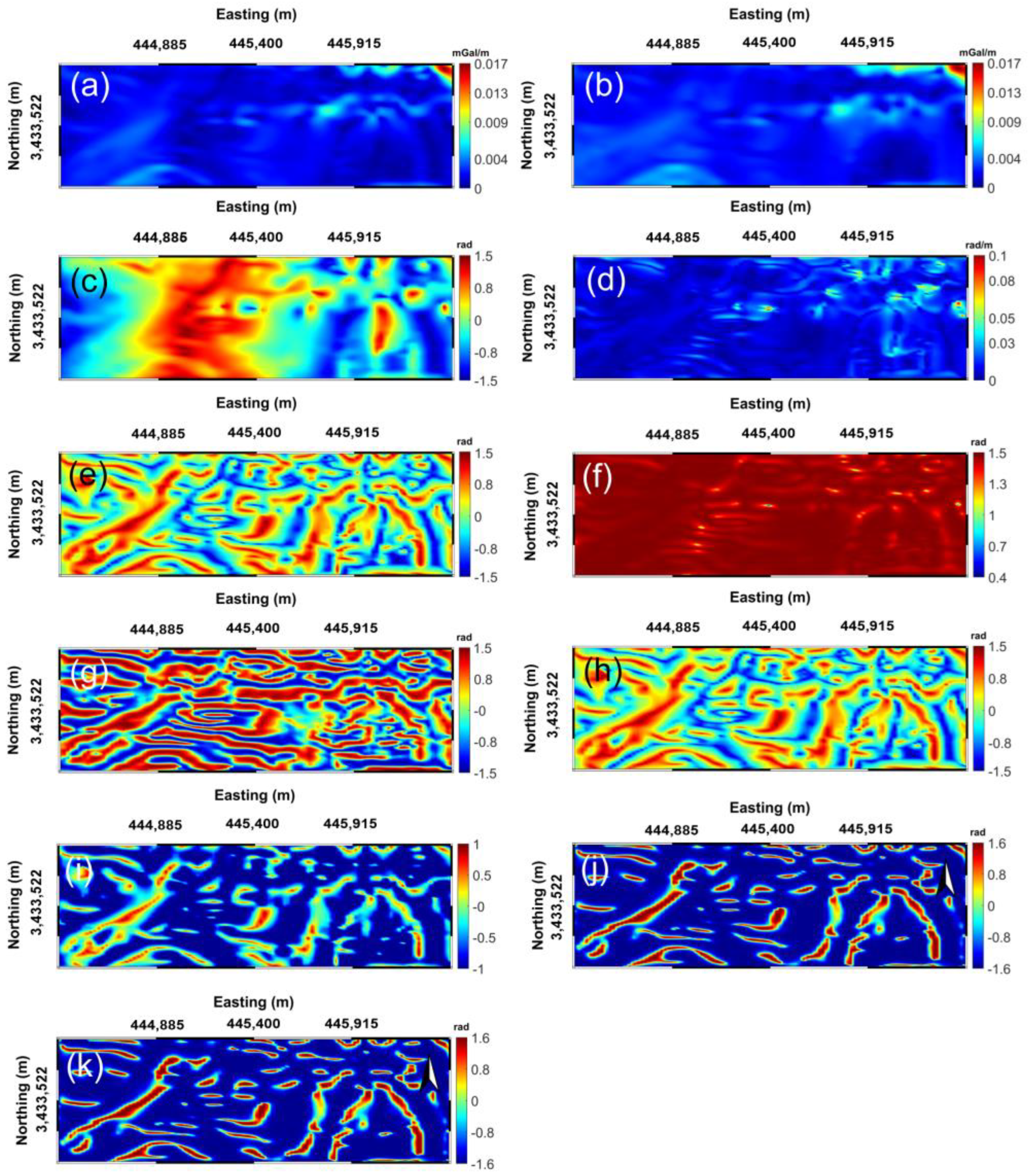
7. Application to Field Gravity Data
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cooper, G.R.J.; Cowan, D.R. Enhancing potential field data using filters based on the local phase. Comput. Geosci. 2006, 32, 1585–1591. [Google Scholar] [CrossRef]
- Sun, Y.; Yang, W.; Zeng, X.; Zhang, Z. Edge enhancement of potential field data using spectral moments. Geophysics 2016, 81, G1–G11. [Google Scholar] [CrossRef]
- Dwivedi, D.; Chamoli, A. Source edge detection of potential field data using wavelet decomposition. Pure Appl. Geophys. 2021, 178, 919–938. [Google Scholar] [CrossRef]
- Pham, L.T.; Oksum, E.; Do, T.D. Edge enhancement of potential field data using the logistic function and the total horizontal gradient. Acta Geod. Geophys. 2019, 54, 143–155. [Google Scholar] [CrossRef]
- Pham, L.T.; Van Vu, T.; Le Thi, S.; Thi Trinh, P. Enhancement of Potential Field Source Boundaries Using an Improved Logistic Filter. Pure Appl. Geophys. 2020, 177, 5237–5249. [Google Scholar] [CrossRef]
- Pham, L.T.; Oksum, E.; Le, D.V.; Ferreira, F.J.F.; Le, S.T. Edge detection of potential field sources using the soft sign function. Geocarto Int. 2021, 37, 4255–4268. [Google Scholar] [CrossRef]
- Prasad, K.N.D.; Pham, L.T.; Singh, A.P. Structural mapping of potential field sources using BHG filter. Geocarto Int. 2022, 37, 11253–11280. [Google Scholar] [CrossRef]
- Alvandi, A.; Toktay, H.D.; Pham, L.T. Capability of improved Logistics filter in determining lateral boundaries and edges of gravity and magnetic anomalies Tuzgolu, Area Turkey. Iran. J. Min. Eng. 2022, 17, 57–72. (In Persian) [Google Scholar] [CrossRef]
- Ibraheem, I.M.; Tezkan, B.; Ghazala, H.; Othman, A.A. A New Edge Enhancement Filter for the Interpretation of Magnetic Field Data. Pure Appl. Geophys. 2023, 180, 2223–2240. [Google Scholar] [CrossRef]
- Alvandi, A.; Ardestani, V.E. Edge detection of potential field anomalies using the Gompertz function as a high-resolution edge enhancement filter. Bull. Geophys. Oceanogr. 2023, 64, 279–300. [Google Scholar] [CrossRef]
- Chen, A.G.; Zhou, T.F.; Liu, D.J.; Zhang, S. Application of an enhanced theta-based filter for potential field edge detection: A case study of the luzong ore district. Chin. J. Geophys. 2017, 60, 203–218. [Google Scholar]
- Weihermann, J.D.; Ferreira, F.J.F.; Oliveira, S.P.; Cury, L.F.; de Souza, J. Magnetic interpretation of the Paranaguá Terrane, southern Brazil by signum transform. J. Appl. Geophys. 2018, 154, 116–127. [Google Scholar] [CrossRef]
- Nasuti, Y.; Nasuti, A. NTilt as an improved enhanced tilt derivative filter for edge detection of potential field anomalies. Geophys. J. Int. 2018, 214, 36–45. [Google Scholar] [CrossRef]
- Nasuti, Y.; Nasuti, A.; Moghadas, D. STA: A novel approach for enhancing and edge detection of potential field data. Pure Appl. Geophys. 2019, 176, 827–841. [Google Scholar] [CrossRef]
- Eldosouky, A.M. Aeromagnetic data for mapping geologic contacts at Samr El-Qaa area, North Eastern Desert. Egypt. Arab J. Geosci. 2019, 12, 2. [Google Scholar] [CrossRef]
- Cordell, L.; Grauch, V.J.S. Mapping Basement Magnetization Zones from Aeromagnetic Data in the San Juan Basin. In The Utility of Regional Gravity and Magnetic Anomaly Maps; SEG Publication: Houston, TX, USA, 1985; pp. 181–197. [Google Scholar] [CrossRef]
- Roest, W.R.J.; Verhoef, J.; Pilkington, M. Magnetic interpretation using the 3-D analytic signal. Geophysics 1992, 57, 116–125. [Google Scholar] [CrossRef]
- Oksum, E.; Le, D.V.; Vu, M.D.; Nguyen, T.H.T.; Pham, L.T. A novel approach based on the fast-sigmoid function for interpretation of potential field data. Bull. Geophys. Oceanogr. 2021, 62, 543–556. [Google Scholar]
- Miller, H.G.; Singh, V. Potential field tilt—A new concept for location of potential field sources. J. Appl. Geophys. 1994, 32, 213–217. [Google Scholar] [CrossRef]
- Verduzco, B.; Fairhead, J.D.; Green, C.M.; MacKenzie, C. New insights into magnetic derivatives for structural mapping. Lead. Edge 2004, 23, 116–119. [Google Scholar] [CrossRef]
- Alvandi, A.; Ghanati, R. Using magnetic data for estimating the location of lateral boundaries and the depth of the shallow salt dome of Aji-Chai, East Azerbaijan Province, Iran. Int. J. Min. Geo-Eng. 2023, 57, 251–258. [Google Scholar] [CrossRef]
- Alvandi, A.; Toktay, H.D.; Ardestani, V.E. Edge detection of geological structures based on a logistic function: A case study for gravity data of the Western Carpathians. Int. J. Min. Geo-Eng. 2023, 57, 267–274. [Google Scholar] [CrossRef]
- Othman, A.A.; Ibraheem, I.M. Origin of El-Maghara Anticlines, North Sinai Peninsula, Egypt: Insights from Gravity Data Interpretation Using Edge Detection Filters. Arab. J. Sci. Eng. 2023. [Google Scholar] [CrossRef]
- Ferreira, F.J.F.; de Souza, J.; Bongiolo, A.B.S.; de Castro, L.G. Enhancement of the total horizontal gradient of magnetic anomalies using the tilt angle. Geophysics 2013, 78, J33–J41. [Google Scholar] [CrossRef]
- Ma, G. Edge detection of potential field data using improved local phase filter. Explor. Geophys. 2013, 44, 36–41. [Google Scholar] [CrossRef]
- Zhang, X.; Yu, P.; Tang, R.; Xiang, Y.; Zhao, C.J. Edge enhancement of potential field data using an enhanced tilt angle. Explor. Geophys. 2014, 46, 276–283. [Google Scholar] [CrossRef]
- Eshaghzadeh, A.; Dehghanpour, A.; Kalantari, R.A. Application of the tilt angle of the balanced total horizontal derivative filter for the interpretation of potential field data. Boll. Geofis. Teor. Appl. 2018, 59, 161–178. [Google Scholar]
- Cooper, G.R.J. Balancing images of potential field data. Geophysics 2009, 74, L17–L20. [Google Scholar] [CrossRef]
- Gudermann, C. Grundriss der Analytischen Sphärik; DuMont-Schauberg: Köln, Germany, 1830. (In German) [Google Scholar]
- Zwillinger, D. CRC Standard Mathematical Tables and Formulae; Chapman and Hall/CRC: Boca Raton, FL, USA, 2002. [Google Scholar] [CrossRef]
- Romakina, L.N. The inverse Gudermannian in the hyperbolic geometry. Integral Transform. Spec. Funct. 2018, 29, 384–401. [Google Scholar] [CrossRef]
- Sherrod, B.L.; Blakely, R.J.; Weaver, C.S.; Kelsey, H.M.; Barnett, E.; Liberty, L.; Meagher, K.L.; Pape, K. Finding concealed active faults: Extending the southern Whidbey Island fault across the Puget Lowland, Washington. J. Geophys. Res. 2008, 113, B05313. [Google Scholar] [CrossRef]
- Johnson, S.Y.; Potter, C.J.; Miller, J.J.; Armentrout, J.M.; Finn, C.; Weaver, C.S. The southern Whidbey Island fault: An active structure in the Puget Lowland, Washington. Geol. Soc. Am. Bull. 1996, 108, 334–354. [Google Scholar] [CrossRef]
- Saltus, R.W.; Blakely, R.J.; Haeussler, P.J.; Wells, R.E. Utility of aeromagnetic studies for mapping of potentially active faults in two forearc basins: Puget Sound, Washington, and Cook Inlet, Alaska. Earth Planets Space 2005, 57, 781–793. [Google Scholar] [CrossRef]
- Blakely, R.J.; Wells, R.E.; Weaver, C.S. Puget Sound Aeromagnetic Maps and Data. U.S. Geological Survey Open-File Report. 1999; pp. 99–514. Available online: https://pubs.usgs.gov/of/1999/of99-514, (accessed on 15 March 2020).
- Blakely, R.J.; Sherrod, B.L.; Hughes, J.F.; Anderson, M.L.; Wells, R.E.; Weaver, C.S. Saddle Mountain fault deformation zone, Olympic Peninsula, Washington: Western boundary of the Seattle uplift. Geosphere 2009, 5, 105–125. [Google Scholar] [CrossRef]
- Alvandi, A.; Toktay, H.D.; Nasri, S. Application of direct source parameter imaging (direct local wave number) technique to the 2-D gravity anomalies for depth determination of some geological structures, for depth determination of some geological structures. Acta Geophys. 2022, 70, 659–667. [Google Scholar] [CrossRef]
- Salem, A.; Williams, S.; Fairhead, J.D.; Ravat, D.J.; Smith, R. Tilt-depth method: A simple depth estimation method using first-order magnetic derivatives. Lead. Edge 2007, 26, 1502–1505. [Google Scholar] [CrossRef]
- Joulidehsar, F.; Moradzadeh, A.; Doulati Ardejani, F. An Improved 3D Joint Inversion Method of Potential Field Data Using Cross-Gradient Constraint and LSQR Method. Pure Appl. Geophys. 2018, 175, 4389–4409. [Google Scholar] [CrossRef]
- Madankav. Report of Geophysical Investigation of Jalal Abad Iron Mine. Madankav Company: Tehran, Iran, 2013. (In Persian) [Google Scholar]
- Oruç, B. Edge detection and depth estimation using a tilt angle map from gravity gradient data of the Kozaklı-Central Anatolia Region, Turkey. Pure Appl. Geophys. 2010, 168, 1769–1780. [Google Scholar] [CrossRef]

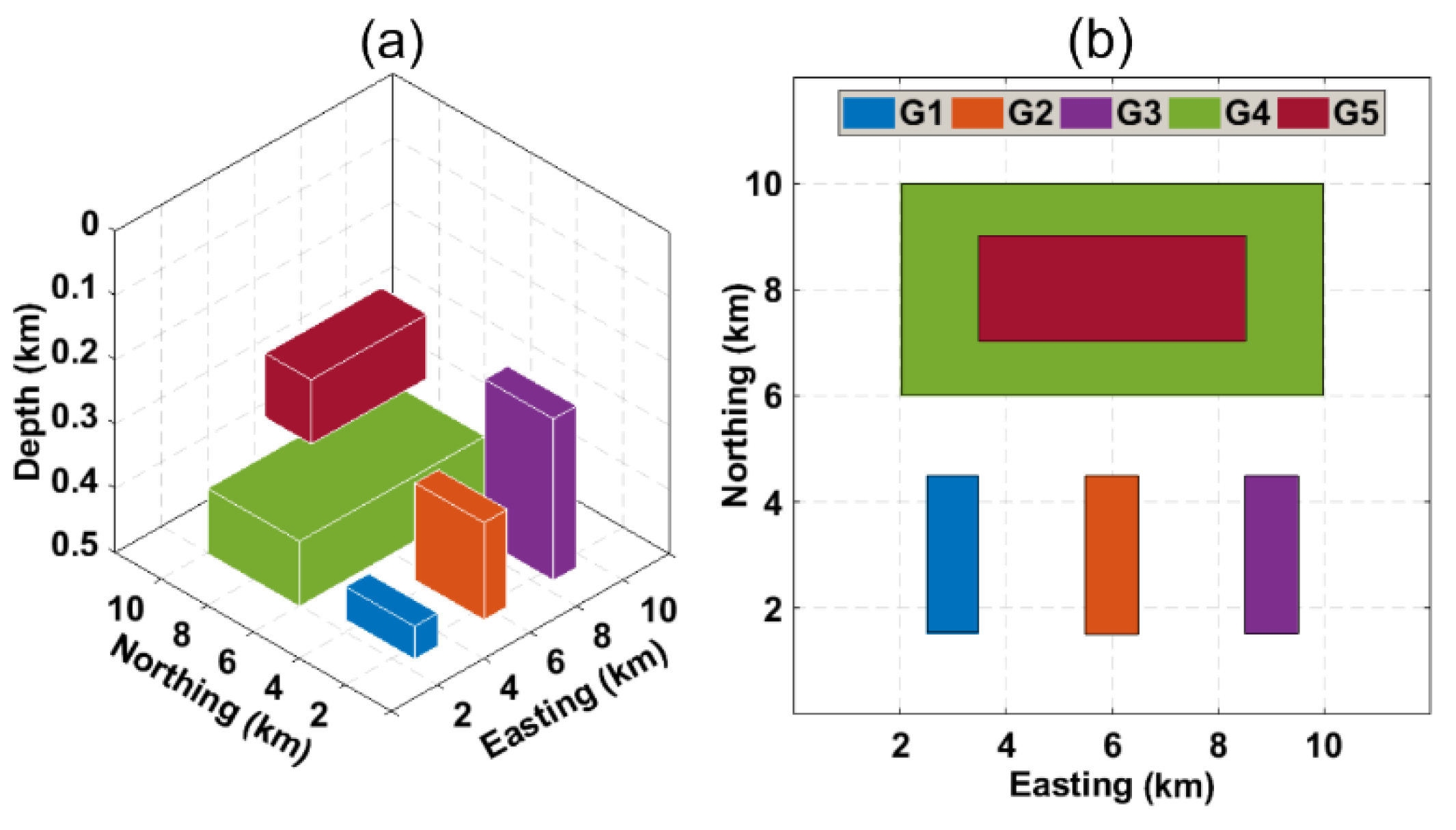
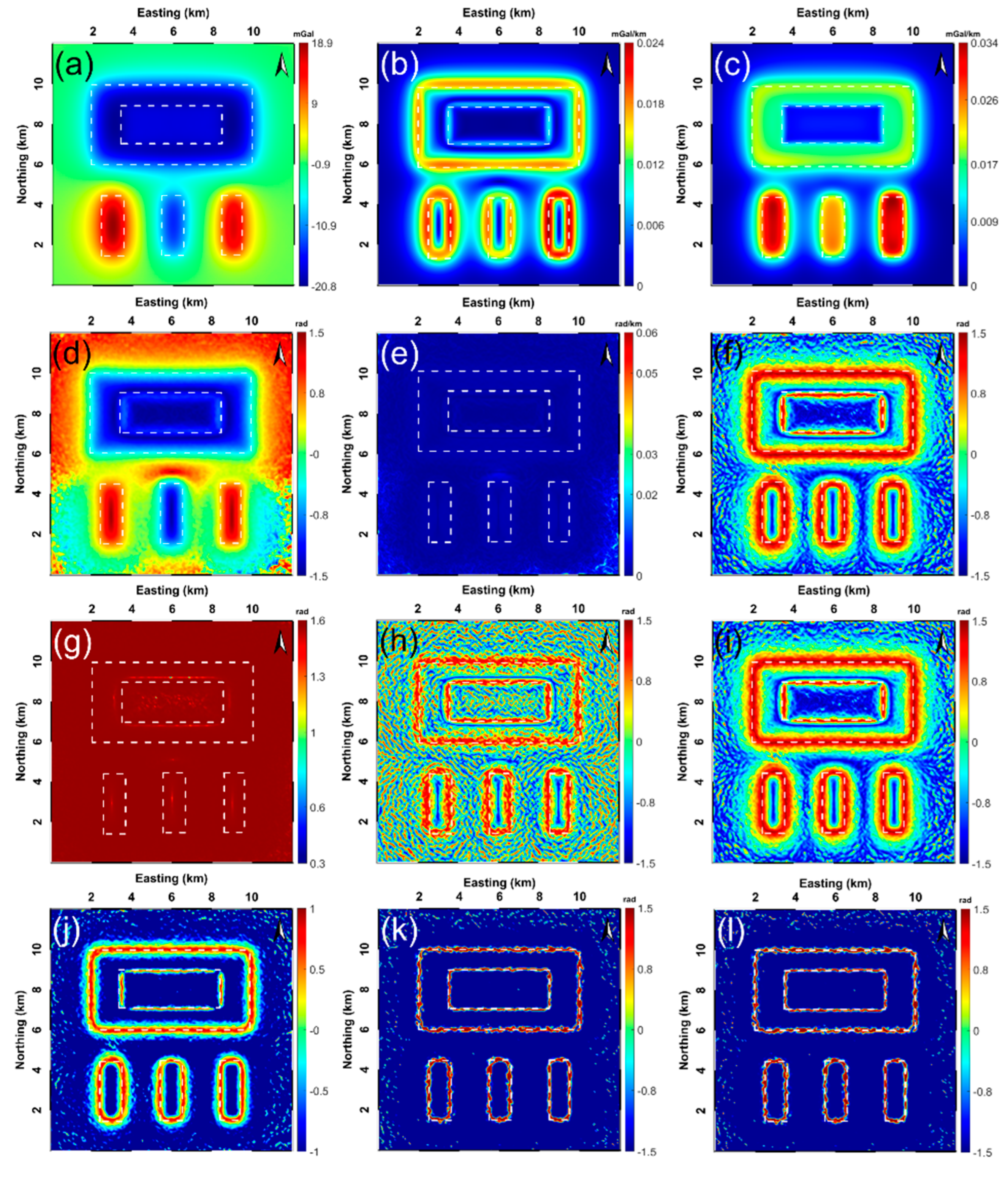

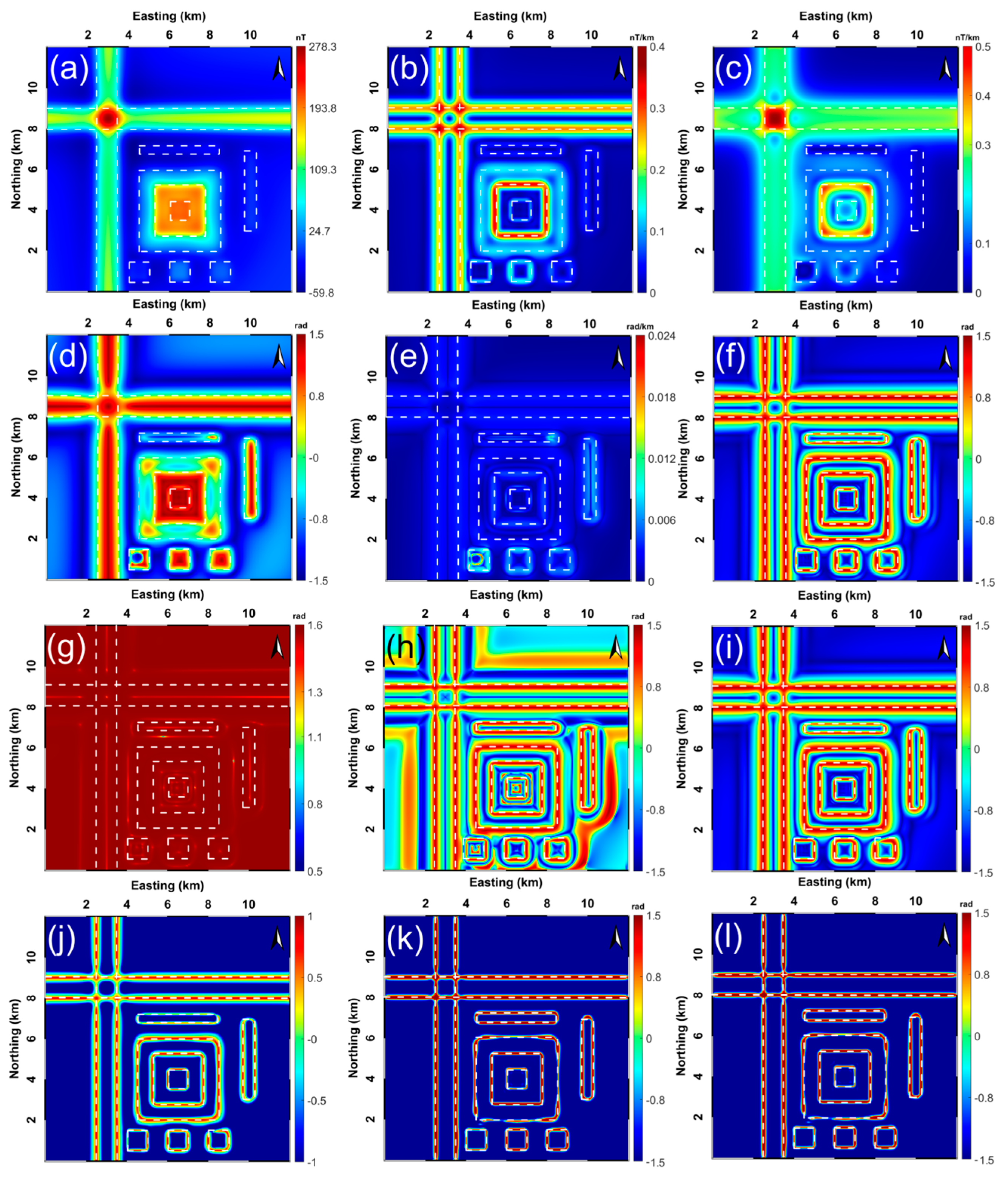
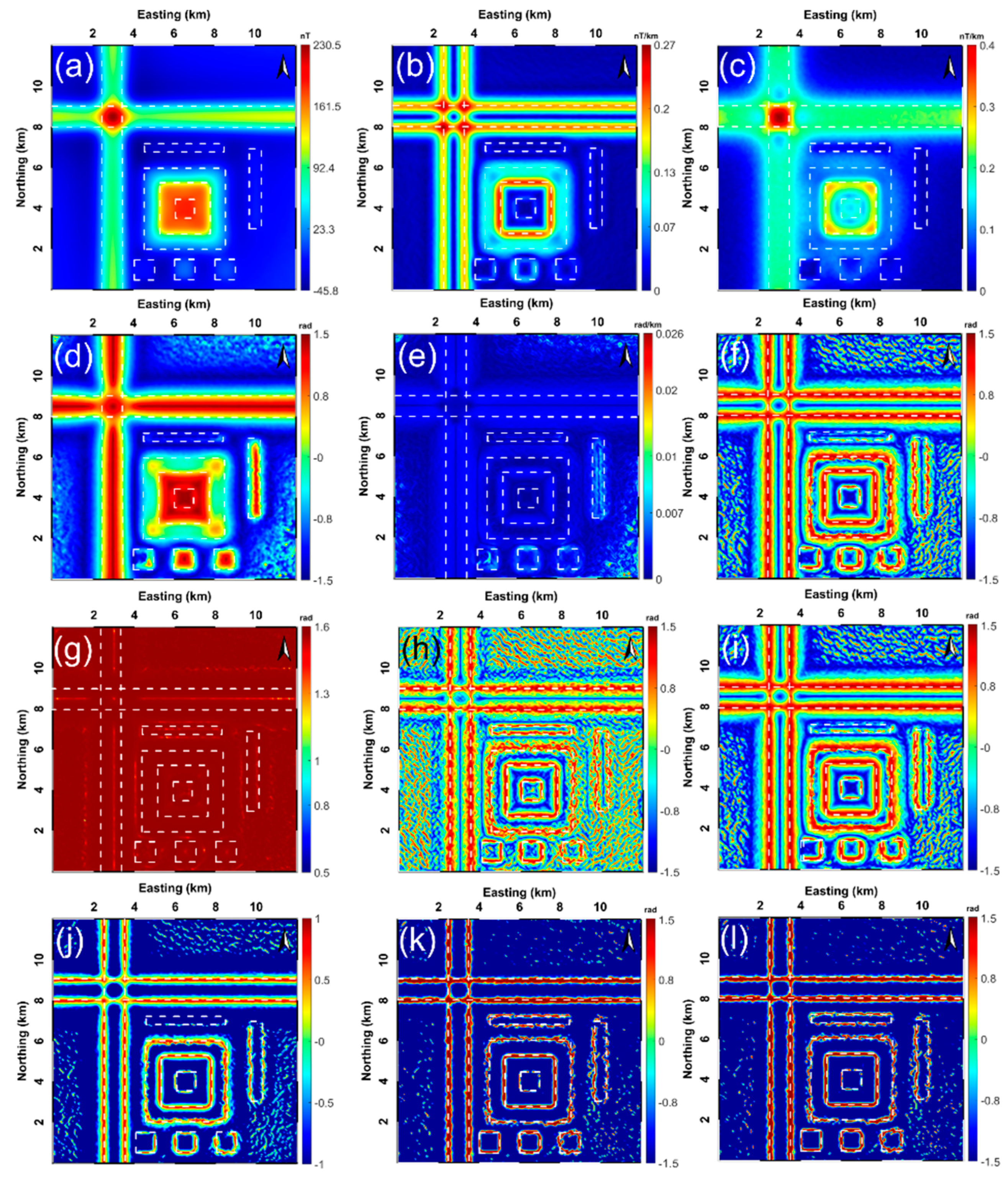

| Source/Label | G1 | G2 | G3 | G4 | G5 |
|---|---|---|---|---|---|
| x-Coordinate of the Geometric Center (m) | 3000 | 6000 | 9000 | 6000 | 6000 |
| y-Coordinate of the Geometric Center (m) | 3000 | 3000 | 3000 | 8000 | 8000 |
| Prism Width (m) | 1000 | 1000 | 1000 | 8000 | 5000 |
| Prism Length (m) | 3000 | 3000 | 3000 | 4000 | 2000 |
| Depth of the Top (m) | 450 | 350 | 250 | 400 | 200 |
| Depth of the Bottom (m) | 950 | 850 | 750 | 700 | 300 |
| Density Contrast (kg/m3) | 3000 | −2000 | 2000 | −2500 | 2000 |
| Source/Label | M1 | M2 | M3 | M4 | M5 | M6 | M7 | M8 | M9 | M10 |
|---|---|---|---|---|---|---|---|---|---|---|
| x-Coordinate of the Center (m) | 3000 | 5000 | 6500 | 6500 | 6500 | 4500 | 6500 | 8500 | 6500 | 10,000 |
| y-Coordinate of the Center (m) | 5000 | 8500 | 4000 | 4000 | 4000 | 1000 | 1000 | 1000 | 7000 | 5000 |
| Prism Width (m) | 1000 | 1000 | 4000 | 2500 | 1000 | 1000 | 1000 | 1000 | 500 | 500 |
| Prism Length (m) | 20,000 | 20,000 | 4000 | 2500 | 1000 | 1000 | 1000 | 1000 | 4000 | 4000 |
| Depth of the Top (m) | 450 | 450 | 600 | 300 | 200 | 200 | 300 | 400 | 400 | 500 |
| Depth of the Bottom (m) | 950 | 950 | 1100 | 500 | 220 | 220 | 350 | 450 | 450 | 550 |
| Strike Azimuth (°) | 0 | 90 | 0 | 0 | 0 | 0 | 0 | 0 | 90 | 0 |
| Magnetic Susceptibility (SI) | 0.021 | 0.023 | 0.019 | 0.025 | 0.027 | 0.03 | 0.032 | −0.024 | 0.025 | −0.026 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alvandi, A.; Su, K.; Ai, H.; Ardestani, V.E.; Lyu, C. Enhancement of Potential Field Source Boundaries Using the Hyperbolic Domain (Gudermannian Function). Minerals 2023, 13, 1312. https://doi.org/10.3390/min13101312
Alvandi A, Su K, Ai H, Ardestani VE, Lyu C. Enhancement of Potential Field Source Boundaries Using the Hyperbolic Domain (Gudermannian Function). Minerals. 2023; 13(10):1312. https://doi.org/10.3390/min13101312
Chicago/Turabian StyleAlvandi, Ahmad, Kejia Su, Hanbing Ai, Vahid E. Ardestani, and Chuan Lyu. 2023. "Enhancement of Potential Field Source Boundaries Using the Hyperbolic Domain (Gudermannian Function)" Minerals 13, no. 10: 1312. https://doi.org/10.3390/min13101312
APA StyleAlvandi, A., Su, K., Ai, H., Ardestani, V. E., & Lyu, C. (2023). Enhancement of Potential Field Source Boundaries Using the Hyperbolic Domain (Gudermannian Function). Minerals, 13(10), 1312. https://doi.org/10.3390/min13101312