A Review of Particle Shape Effects on Material Properties for Various Engineering Applications: From Macro to Nanoscale
Abstract
1. Introduction
2. Shape Parameters
3. Measurement Techniques Used for the Shape Characterization of Particles
3.1. Classification Charts
3.2. Two Dimensional Measurements
3.2.1. Microscope Techniques
Optical Microscope Technique
Stereo-Microscopical Analysis
SEM-Based Techniques
3.3. Three Dimensional Measurements
3.4. Permaran Technique
3.5. Brunauer, Emmett, and Teller (BET) Technique
3.6. Image-Based Techniques
3.6.1. Static Image Analysis (SIA)
3.6.2. Dynamic Image Analysis (DIA)
3.6.3. Automated Image Analysis
3.6.4. Advanced Techniques with Sophisticated Equipment
4. Effect of Particle Shape on Industrial Applications of Various Materials
4.1. Aggregate Particles
4.1.1. Ballast Aggregate Particles in Railway Roads
4.1.2. Aggregates Particles in Concrete and Asphalt
4.2. Proppant Particles in Hydraulic Fracturing for Oil and Gas

4.3. Glass Bead Particles in Highway Road Paints
4.4. Viscosity and Rheological Properties of Particles in Suspensions
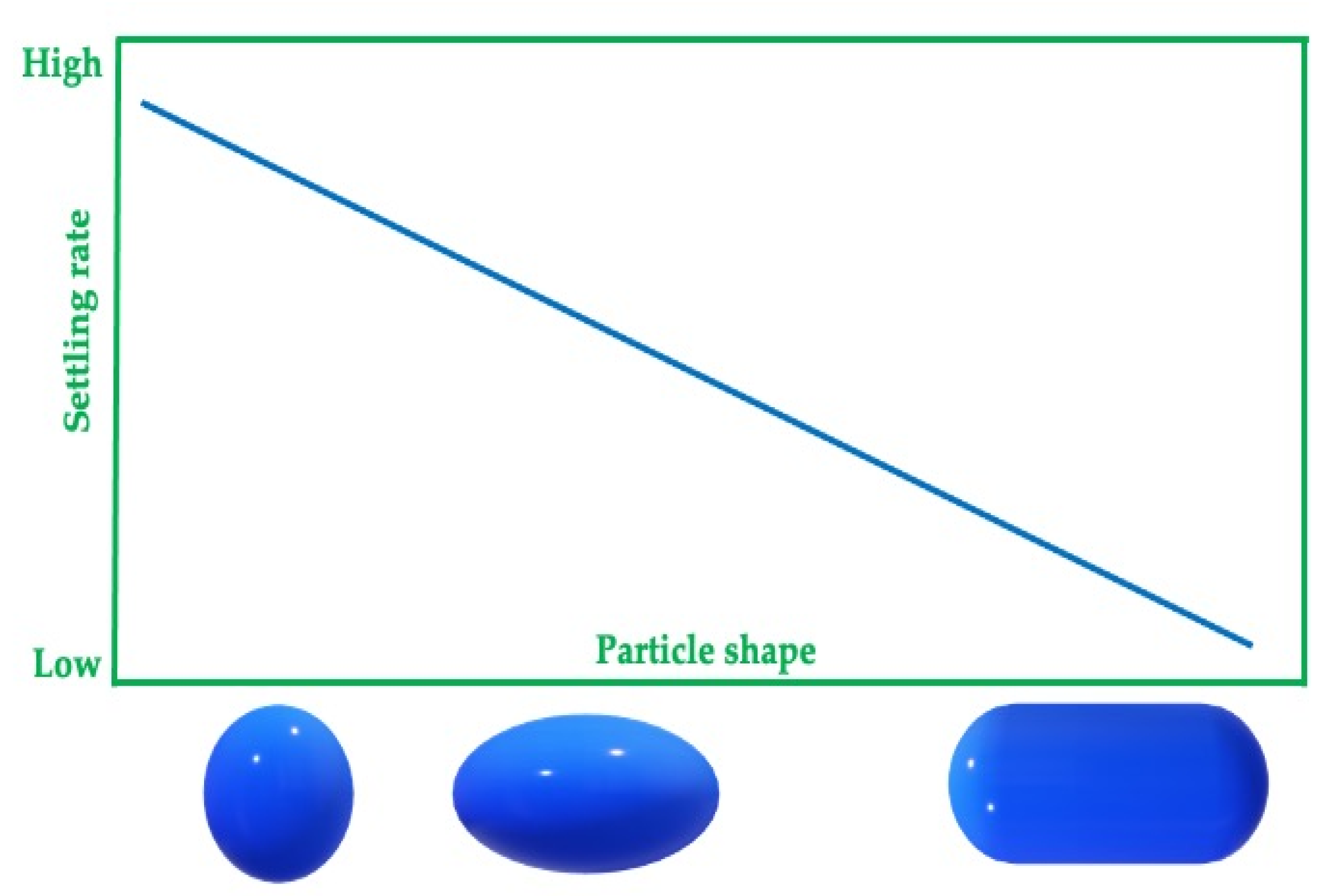
4.5. Settling Properties of Particles in Gravity Sedimentation
4.6. Mechanical Properties of Bulk Solids in Conveying
4.6.1. Density Properties Related to the Packing of Particles
4.6.2. Properties of Flowability and Angle of Repose
4.6.3. Pharmaceutical Powders in the Compaction Process
4.6.4. Discharge Rates of Particles in Storage Systems
4.7. Mechanical Properties of Soil Particles
4.8. Metal Additive Particles
4.9. Bulk Powder Properties of Particles in Powder Metallurgy
4.9.1. Thermal Spraying
4.9.2. Gas Atomization
4.9.3. Sintering Behavior
4.9.4. Optical Properties
4.10. Mineral Particles in Ore Processing
4.10.1. Communition (Crushing and Grinding)
4.10.2. Classification
4.10.3. Separation of Valuable Minerals
Magnetic Separation
Electrostatic Separation
Gravity Separation
Flotation
Entrainment in Flotation
4.11. Mineral Filler Particles Used in Various Industries
4.11.1. Rubbers and Plastics
4.11.2. Pigments and Cosmetics
4.11.3. Paints and Coatings
4.11.4. Ceramics
4.11.5. Abrasives and Explosives
4.11.6. Paper
4.12. Graphite Particles Used in the Battery Anode Material
4.13. Microparticles in Magneto-Rheological Fluids
4.14. Nanoparticles
4.14.1. Nanoparticles’ Super-Paramagnetic Properties
4.14.2. Nanoparticles in Ferrofluids
4.14.3. Nanoparticles Used in Pharmaceutical Manufacturing
4.14.4. Pharmaceuticals Particles in the Dissolution Process of Drugs
4.14.5. Nanoparticles Used in Drug Delivery
4.14.6. Nanoparticles Used in Disease Diagnosis and Therapy
4.14.7. Nanoparticles for Advanced Immunotherapy
4.14.8. Nanoparticles Used in Catalysis
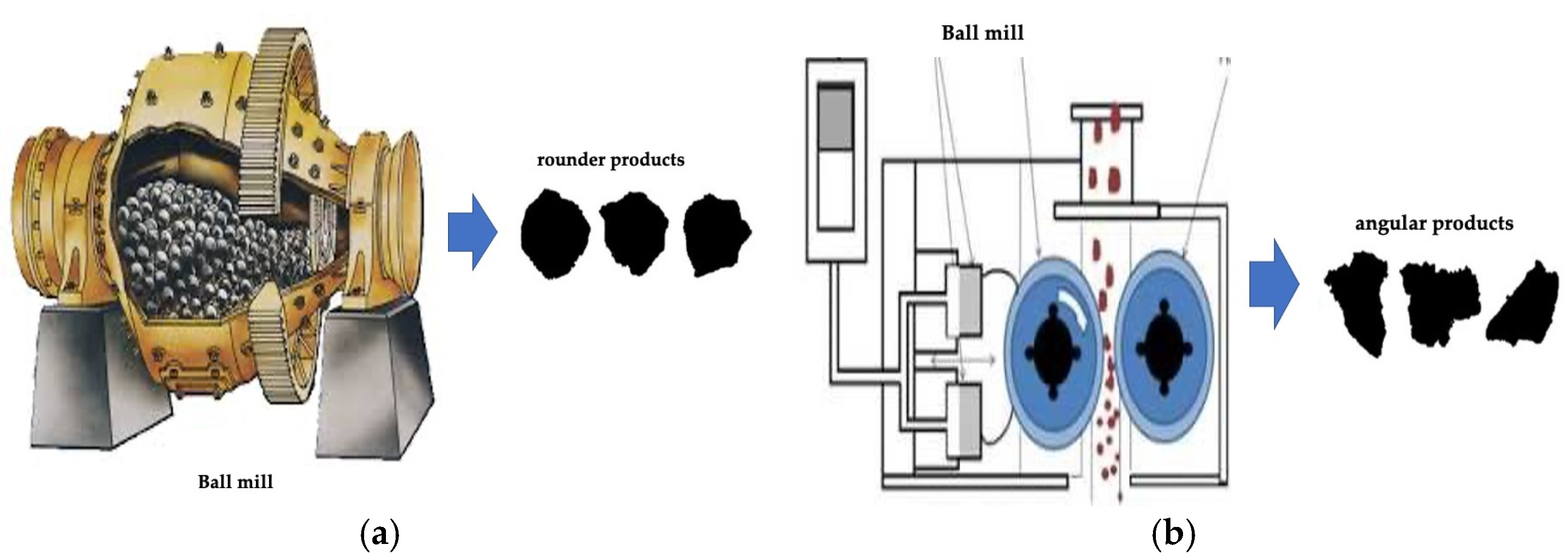
5. Tuning the Shape of Particles by Mechanical Grinding

6. Modeling
7. Conclusions
8. Future Recommendations
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- BS 2955:1993; British Standards 2955; Glossary of Terms Relating to Powders. British Standards Institution: London, UK, 1993.
- McGraw Hill’s Dictionary of Scientific and Technical Terms, 6th ed.; McGraw-Hill: New York, NY, USA, 2003.
- Meloy, T.P. Particle Shape Characterization: Recent Developments (Review). In Testing and Characterization of Powders and Fine Particles; Beddow, J.K., Meloy, T.P., Eds.; Heyden and Son: London, UK, 1980; Chapter 1. [Google Scholar]
- Rhodes, M. Introduction to Particle Technology, 2nd ed.; John Wiley & Sons Ltd.: Chichester, UK, 2008. [Google Scholar]
- Xu, R. Particle Characterization: Light Scattering Methods; Kluwer Academic publishers: Hingham, MA, USA, 2000. [Google Scholar]
- Allen, T. Powder Sampling and Size Determination; Elsevier Science: New York, NY, USA, 2003. [Google Scholar]
- Gani, A.; Mhaskar, P.; Christofides, P.D. Handling sensor malfunctions in control of particulate processes. Chem. Eng. Sci. 2008, 63, 1217–1229. [Google Scholar] [CrossRef]
- Ortega-Rivas, E. Unit Operations of Particulate Solids: Theory and Practice; CRC Press: Boca Raton, FL, USA, 2012; pp. 3–13. [Google Scholar]
- Park, A.-H.A.; Zhu, C.; Fan, L.-S. Particle Technology. In Kirk-Othmer Encyclopedia of Chemical Technology, 5th ed.; Ley, C., Ed.; John Wiley & Sons, Inc.: New York, NY, USA, 2007; pp. 1–41. [Google Scholar]
- Richardson, J.F.; Harker, J.H.; Backhurst, J.R. Coulson and Richardson’s Chemical Engineering: Particle Technology and Separation Processes, 5th ed.; Butterworth-Heinemann: Oxford, UK, 2002; Volume 2. [Google Scholar]
- Shinahara, K. Fundamental and Rheological Properties of Powders. In Handbook of Powder Science and Technology, 2nd ed.; Fayed, M.E., Otten, L., Eds.; Chapman and Hall: New York, NY, USA, 1997; Chapter 4; p. 96. [Google Scholar]
- Davies, R. Particle size measurement: Experimental techniques. In Handbook of Powder Science and Technology; Fayen, M.E., Otten, L., Eds.; Van Nostrand Reinhold: New York, NY, USA, 1984. [Google Scholar]
- Müller, F.; Polke, R.; Schafer, M.; Scholz, N. Particle system characterization and modelling. Part. Part. Syst. Charact. 2001, 18, 248–253. [Google Scholar] [CrossRef]
- Barbosa-Canovas, G.V.; Ortega-Rivas, E.; Juliano, P.; Yan, H. Food Powders, Physical Properties, Processing, and Functionality; Kluwer Academic/Plenum Publishers: New York, NY, USA, 2005; pp. 19–20. [Google Scholar]
- Singh, P.; Ramakrishnan, P. Powder Characterization by Particle Shape Assessment. KONA Powder Part. J. 1996, 14, 16–30. [Google Scholar] [CrossRef]
- Seville, J.P.K.; Wu, C.-Y. Particle Technology and Engineering: An Engineer’s Guide to Particles and Powders: Fundamentals and Computational Approaches, 1st ed.; Butterworth-Heinemann: Amsterdam, The Netherlands, 2016; pp. 1–294. [Google Scholar]
- Vehring, R. Pharmaceutical Particle Engineering via Spray Drying. Pharm. Res. 2008, 25, 999–1022. [Google Scholar] [CrossRef] [PubMed]
- Neikov, O.D.; Lotsko, D.V.; Gopienko, V.G. Powder Characterization and Testing. In Handbook of Non-Ferrous Metal Powders: Technologies and Applications, 1st ed.; Neikov, O.D., Naboychenko, S.S., Dowson, G., Eds.; Elsevier: Oxford, UK, 2009; Chapter 1; pp. 7–44. [Google Scholar]
- Ciullo, P.A. The Industrial Minerals. In Industrial Minerals and Their Uses: A Handbook and Formulary; Noyes Publications: Westwood, MA, USA, 1996; Chapter 2; pp. 17–82. [Google Scholar]
- Lobato, E.M.C. Determination of Surface Free Energies and Aspect Ratio of Talc. Master’s Thesis, Virginia Polytechnic Institute and State University, Blacksburg, VA, USA, 2004. [Google Scholar]
- Vanderbilt, R.T. Paints and Coatings, A Guide to Filler Properties and Uses, Technical Data, No. 703. Vanderbilt Minerals. 2013. Available online: https://www.vanderbiltminerals.com/resources/VR_703_Paint_Filler_Minerals_Reference_Web.pdf (accessed on 23 November 2022).
- Gupta, C.K. Chemical Metallurgy: Principles and Practice; WILEY-VCH Verlag GmbH & Co.: Weinheim, Germany, 2003; Chapter 2; pp. 119–121. [Google Scholar]
- Malvern Instruments Limited. A Basic Guide to Particle Characterization, Inform White Paper; Malvern Instruments Limited: Worcestershire, UK, 2012; pp. 1–26. Available online: https://www.atascientific.com.au/wp-content/uploads/2017/02/MRK1806-01-basic-guide-to-particle-characterisation-1.pdf (accessed on 26 December 2022).
- Chen, J.; Li, R.; Mo, P.-Q.; Zhou, G.; Cai, S.; Chen, D. A modified method for morphology quantification and generation of 2D granular particles. Granul. Matter 2022, 24, 16. [Google Scholar] [CrossRef]
- Bergeron, M.; Laurin, P.; Tawashi, R. Effect of particle morphology in selecting pharmaceutical excipients. Drug Dev. Ind. Pharm. 1986, 12, 915–926. [Google Scholar] [CrossRef]
- Murphy, K.A.; Dahmen, K.A.; Jaeger, H.M. Transforming Mesoscale Granular Plasticity Through Particle Shape. Phys. Rev. X 2019, 9, 011014. Available online: https://link.aps.org/doi/10.1103/PhysRevX.9.011014 (accessed on 26 December 2022).
- Horiba Instruments, Inc. A Guidebook to Particle Size Analysis. 2012. Available online: https://ats-scientific.com/uploads/PSA_Guidebook.pdf (accessed on 25 November 2022).
- Lim, M.S.; Wijeyesekera, D.C.; Zainorabidin, A.; Bakar, I. The Effects of Particle Morphology (Shape and Sizes) Characteristics on its Engineering Behaviour and Sustainable Engineering Performance of Sand. Int. J. Integr. Eng. 2013, 4, 4. Available online: https://publisher.uthm.edu.my/ojs/index.php/ijie/article/view/582 (accessed on 23 November 2022).
- Wadell, H. Volume, shape, and roundness of rock particles. J. Geol. 1932, 40, 443–451. Available online: https://www.jstor.org/stable/30058012 (accessed on 23 November 2022). [CrossRef]
- Bagheri, G.H.; Bonadonna, C.; Manzella, I.; Vonlanthen, P. On the characterization of size and shape of irregular particles. Powder Technol. 2015, 270, 141–153. [Google Scholar] [CrossRef]
- De Vicente, J.; Vereda, F.; Segovia-Gutiérrez, J.P.; Morales, M.d.-P.; Hidalgo-Álvarez, R. Effect of particle shape in magnetorheology. J. Rheol. 2010, 54, 1337. [Google Scholar] [CrossRef]
- Durney, T.E.; Meloy, T.P. Particle shape effects due to crushing method and size. Int. J. Min. Process. 1986, 16, 109–123. [Google Scholar] [CrossRef]
- Crompton, C. Particle Shape: An Important Parameter in Pharmaceutical Manufacturing. 2005. Available online: http://www.samedanltd.com/index.php?mod=magazine&page=pdfsignup&id=456&pid=456&issid=55 (accessed on 23 November 2022).
- Vision Analytical Inc. Why Particle Shape Is Important. 2022. Available online: https://particleshape.com/why-particle-shape-is-important (accessed on 23 November 2022).
- Gantenbein, D.; Schoelkopf, J.; Matthews, G.P.; Gane, P.A.C. Determining the size distribution-defined aspect ratio of rod-like particles. Appl. Clay Sci. 2011, 53, 538–543. [Google Scholar] [CrossRef]
- Manek, R.V.; Builders, P.F.; Kolling, W.M.; Emeje, M.; Kunle, O.O. Physicochemical and binder properties of starch obtained from Cyperus esculentus. AAPS PharmSciTech 2012, 13, 379–388. [Google Scholar] [CrossRef] [PubMed]
- Microtrac Retsch GmbH. White Paper, Particle Characterization of Pharmaceutical Products by Dynamic Image Analysis. 2022, pp. 1–8. Available online: https://www.microtrac.com/dltmp/www/5e396c09-83b0-4cd8-a505-7f30c3c9c754-52db18a3716c/wp_pharma_0118_en.pdf (accessed on 23 November 2022).
- Retsch Technology. Dynamic Image Analysis. The sample. Verder Sci. Cust. Mag. 2022, 42, 2. Available online: http://www.mep.metrohm.com.au/wp-content/uploads/2015/07/the_sample_vol.42.pdf (accessed on 23 November 2022).
- Ma, H.; Bolster, C.; Johnson, W.P.; Li, K.; Pazmino, E.; Camacho, K.M.; Anselmo, A.C.; Mitragotri, S. Coupled influences of particle shape, surface property and flow hydrodynamics on rod-shaped colloid transport in porous media. J. Colloid Interface Sci. 2020, 577, 471–480. [Google Scholar] [CrossRef] [PubMed]
- Cho, G.C.; Dodds, J.; Santamarina, J.C. Particle shape effects on packing density, stiffness, and strength: Natural and crushed sands. J. Geotech. Geoenviron. 2006, 132, 591–602. [Google Scholar] [CrossRef]
- Little, L. The Development and Demonstration of a Practical Methodology for Fine Particle Shape Characterisation in Minerals Processing. Ph.D. Thesis, University of Cape Town, Cape Town, South Africa, 2016. [Google Scholar]
- Arasan, S.; Hasiloglu, A.S.; Akbulut, S. Shape particle of natural and crushed aggregate using image analysis. Int. J. Civ. Eng. 2010, 1, 221–233. [Google Scholar]
- Mora, C.F.; Kwan, A.K.H. Sphericity, shape factor, and convexity measurement of coarse aggregate for concrete using digital image processing. Cem. Concr. Res. 2000, 30, 351–358. [Google Scholar] [CrossRef]
- Santamarina, J.C.; Cho, G.C. Soil behaviour: The role of particle shape. Advances in geotechnical engineering: The Skempton conference. In Proceedings of the Three Day Conference on Advances in Geotechnical Engineering, Organised by the Institution of Civil Engineers and Held at the Royal Geographical Society, London, UK, 29–31 March 2004; pp. 604–617. [Google Scholar]
- Jindal, A.B. The effect of particle shape on cellular interaction and drug delivery applications of micro- and nanoparticles. Int. J. Pharm. 2017, 532, 450–465. [Google Scholar] [CrossRef]
- Almeida-Prieto, S.; Blanco-Mendez, J.; Otero-Espinar, F.J. Microscopic image analysis techniques for the morphological characterization of pharmaceutical particles: Influence of the software, and the factor algorithms used in the shape factor estimation. Eur. J. Pharm. Biopharm. 2007, 67, 766–776. [Google Scholar] [CrossRef]
- Yu, W.; Hancock, B.C. Evaluation of dynamic image analysis for characterizing pharmaceutical excipient particles. Int. J. Pharm. 2008, 361, 150–157. [Google Scholar] [CrossRef]
- Nouri, A.; Sola, A. Metal particle shape: A practical perspective. Met. Powder Rep. 2018, 73, 276–282. [Google Scholar] [CrossRef]
- Youd, T.L. Factors controlling the maximum and minimum densities of sands. In Evaluation of Relative Density and Its Role in Geotechnical Projects Involving Cohesionless Soils; Selig, E.T., Ladd, R.S., Eds.; ASTM Special Technical Publication 523; ASTM: West Conshohocken, PA, USA, 1973; pp. 98–112. [Google Scholar]
- Mitchell, J.K.; Soga, K. Fundamentals of Soil Behaviour, 3rd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2005. [Google Scholar]
- Lee, C.; Suh, H.S.; Yoon, B.; Yun, T.S. Particle shape effect on thermal conductivity and shear wave velocity in sands. Acta Geotech. 2017, 12, 615–625. [Google Scholar] [CrossRef]
- Edelstein, A.S.; Cammarata, R.C. Nanomaterials: Synthesis, Properties and Applications; IOP: Bristol, UK, 1998. [Google Scholar]
- Rao, C.N.R.; Cheetham, A.K. Science and technology of nanomaterials: Current status and future prospects. J. Mater. Chem. 2001, 11, 2887–2894. [Google Scholar] [CrossRef]
- Sanyal, M.K.; Mukhopadhyay, M.K.; Mukherjee, M.; Datta, A.; Basu, J.K.; Penfold, J. Role of molecular self-assembling in Langmuir-Blodgett film growth. Phys. Rev. B 2002, 65, 033409. [Google Scholar] [CrossRef]
- The Oxford English Dictionary, 2nd ed; Simpson, J., Weiner, E., Eds.; Clarendon Press: Oxford, UK, 1989. [Google Scholar]
- ISO 9276-6:2008; Representation of Results of Particle Size Analysis-Part 6: Descriptive and Quantitative Representation of Particle Shape and Morphology. ISO: Geneva, Switzerland, 2008.
- Podczeck, F. Particle Shape Analysis, Encyclopedia of Pharmaceutical Science and Technology; Taylor and Francis: New York, NY, USA, 2013; pp. 2465–2481. [Google Scholar]
- Neal, F.B.; Russ, J.C. Measuring Shape; CRC Press: Boca Raton, FL, USA, 2012. [Google Scholar]
- Zavala, J.M.R. Particle Shape Quantities and Influence on Geotechnical Properties-A Review. Ph.D. Thesis, Luleå University of Technology, Luleå, Sweden, 2012. [Google Scholar]
- Barrett, P.J. The shape of rock particles, a critical review. Sedimentology 1980, 27, 291–303. [Google Scholar] [CrossRef]
- Blott, S.J.; Pye, K. Particle shape: A review and new methods of characterization and classification. Sedimentology 2008, 55, 31–63. [Google Scholar] [CrossRef]
- Pabst, W.; Berthold, C.; Gregorova, E. Size and shape characterization of oblate and prolate particles. J. Eur. Ceram. Soc. 2007, 27, 1759–1762. [Google Scholar] [CrossRef]
- Suhr, B.; Six, K. Simple particle shapes for DEM simulations of railway ballast: Influence of shape descriptors on packing behaviour. Granul. Matter 2020, 22, 43. [Google Scholar] [CrossRef]
- Wang, Y.; Ma, G.; Mei, J.; Zou, Y.; Zhang, D.; Zhou, W.; Cao, X. Machine learning reveals the influences of grain morphology on grain crushing strength. Acta Geotech. 2021, 16, 3617–3630. [Google Scholar] [CrossRef]
- Wadell, H. Sphericity and roundness of rock particles. J. Geol. 1933, 41, 310–331. [Google Scholar] [CrossRef]
- Wadell, H. Volume, shape and roundness of quartz particles. J. Geol. 1935, 43, 250. [Google Scholar] [CrossRef]
- Zheng, J.; Hryciw, R.D. Traditional soil particle sphericity, roundness and surface roughness by computational geometry. Geotechnique 2015, 65, 494–506. [Google Scholar] [CrossRef]
- Su, J.; Li, P.; Wei, X.; Sun, S.; Zhu, L.; Dong, C. Analysis of interface interaction of aggregate-asphalt system and its effect on shear-slip behavior of asphalt mixture. Constr. Build. Mater. 2020, 264, 120680. [Google Scholar] [CrossRef]
- Bullard, J.W.; Garboczi, E.J. Defining shape measures for 3d star-shaped particles: Sphericity, roundness, and dimensions. Powder Technol. 2013, 249, 241–252. [Google Scholar] [CrossRef]
- Zhao, B.; Wang, J. 3d quantitative shape analysis on form, roundness, and compactness with μCT. Powder Technol. 2016, 291, 262–275. [Google Scholar] [CrossRef]
- Nie, Z.; Liang, Z.; Wang, X. A three-dimensional particle roundness evaluation method. Granul. Matter 2018, 20, 32. [Google Scholar] [CrossRef]
- Nie, Z.; Wang, X.; Liang, Z.; Gong, J. Quantitative analysis of the three-dimensional roundness of granular particles. Powder Technol. 2018, 336, 584–593. [Google Scholar] [CrossRef]
- Zhou, B.; Wang, J.; Wang, H. Three-dimensional sphericity, roundness and fractal dimension of sand particles. Geotechnique 2018, 68, 18–30. [Google Scholar] [CrossRef]
- Masad, E.; Saadeh, S.; Al-Rousan, T.; Garboczi, E.; Little, D. Computations of particle surface characteristics using optical and x-ray ct images. Comput. Mater. Sci. 2005, 34, 406–424. [Google Scholar] [CrossRef]
- Pan, T.; Tutumluer, E.; Anochie-Boateng, J. Aggregate morphology affecting resilient behavior of unbound granular materials. In Proceedings of the 85th Annual Meeting of Transportation Research Board, Washington, DC, USA, 22–26 January 2006. [Google Scholar]
- Al-Rousan, T.; Masad, E.; Tutumluer, E.; Pan, T. Evaluation of image analysis techniques for quantifying aggregate shape characteristics. Constr. Build. Mater. 2007, 21, 978–990. [Google Scholar] [CrossRef]
- Xiao, J.; Zhang, D.; Wei, K.; Luo, Z. Shakedown behaviors of railway ballast under cyclic loading. Constr. Build. Mater. 2017, 155, 1206–1214. [Google Scholar] [CrossRef]
- Lee, J.R.J.; Smith, M.L.; Smith, L.N.; Midha, P.S. A mathematical morphology approach to image based 3d particle shape analysis. Mach. Vis. Appl. 2005, 16, 282–288. [Google Scholar] [CrossRef]
- Garboczi, E.; Liu, X.; Taylor, M. The 3-d shape of blasted and crushed rocks: From 20 μm to 38 mm. Powder Technol. 2012, 229, 84–89. [Google Scholar] [CrossRef]
- Yang, X.; Chen, S.; You, Z. 3d voxel-based approach to quantify aggregate angularity and surface texture. J. Mater. Civ. Eng. 2017, 29, 04017031. [Google Scholar] [CrossRef]
- Oja, M.; Tuunila, R. The influence of comminution method to particle shape. In Developments in Mineral Processing; Massacci, P., Ed.; Elsevier: Amsterdam, The Netherlands, 2000; Volume 13, pp. C4.64–C4.70. [Google Scholar] [CrossRef]
- Meloy, T.P.; Durney, T.E.; Eppler, D.T. Sophisticated shape analysis of green pellets from a balling Drum. In Proceedings of the XIII International Mineral Processing Congress, Warsaw, Poland, 4–9 June 1979. [Google Scholar]
- Krumbein, W.C. Measurement and geological significance of shape and roundness of sedimentary particles. J. Sediment. Petrol. 1941, 11, 64–72. [Google Scholar] [CrossRef]
- Powers, M.C. A new roundness scale for sedimentary particles. J. Sediment. Res. 1953, 23, 117–119. [Google Scholar] [CrossRef]
- Krumbein, W.C.; Sloss, L.L. Stratigraphy and Sedimentation; Freeman: San Francisco, CA, USA, 1951; Volume 114, pp. 335–336. [Google Scholar]
- Hryciw, R.D.; Zheng, J.; Shetler, K. Comparison of particle roundness and sphericity by traditional chart and computer methods. J. Geotech. Geoenviron. Eng. 2016, 142, 04016038. [Google Scholar] [CrossRef]
- Krumbein, W.C.; Sloss, L.L. Stratigraphy and Sedimentation; W. H. Freeman and Company: San Francisco, CA, USA, 1963. [Google Scholar]
- Hawkins, A.E. The Shape of Powder-particle Outlines; Research Studies Press Ltd.: Taunton, UK, 1993. [Google Scholar]
- Miller, N.A.; Henderson, J.J. Quantifying Sand Particle Shape Complexity using a Dynamic, Digital Imaging Technique. Agron. J. 2010, 102, 1407–1414. [Google Scholar] [CrossRef]
- Yan, W.M.; Su, D. Inferring 3D particle size and shape characteristics from projected 2D images: Lessons learned from ellipsoids. Comput. Geotech. 2018, 104, 281–287. [Google Scholar] [CrossRef]
- Vangla, P.; Roy, N.; Gali, M.L. Image based shape characterization of granular materials and its effect on kinematics of particle motion. Granul. Matter 2018, 20, 6. [Google Scholar] [CrossRef]
- Tutumluer, E.; Huang, H.; Hashash, Y.M.A.; Ghaboussi, J. Imaging based discrete element modeling of granular assemblies. AIP Conf. Proc. 2008, 973, 544–549. [Google Scholar] [CrossRef]
- Nyembwe, A.M.; Cromarty, R.D.; Garbers-Craig, A.M. Relationship Between Iron Ore Granulation Mechanisms, Granule Shapes, and Sinter Bed Permeability. Miner. Process. Extr. Metall. Rev. 2017, 38, 388–402. [Google Scholar] [CrossRef]
- Pons, M.-N.; Vivier, H.; Delcour, V.; Authelin, J.-R.; Paille’res-Hubert, L. Morphological analysis of pharmaceutical powders. Powder Technol. 2002, 128, 276–286. [Google Scholar] [CrossRef]
- Marinoni, N.; Pavese, A.; Foi, M.; Trombino, L. Characterisation of mortar morphology in thin sections by digital image processing. Cem. Concr. Res. 2005, 35, 1613–1619. [Google Scholar] [CrossRef]
- Attota, R.K.; Weck, P.; Kramar, J.A.; Bunday, B.; Vartanian, V. Feasibility study on 3-D shape analysis of high-aspect-ratio features using through-focus scanning optical microscopy. Opt. Express 2016, 24, 16574–16585. [Google Scholar] [CrossRef]
- Zingerman, J.P.; Mehta, S.C.; Salter, J.M.; Radebaugh, G.W. Validation of a Computerized Image-Analysis System for Particle-Size Determination-Pharmaceutical Applications. Int. J. Pharm. 1992, 88, 303–312. [Google Scholar] [CrossRef]
- Brewer, E.; Ramsland, A. Particle-Size Determination by Automated Microscopic Imaging Analysis with Comparison to Laser Diffraction. J. Pharm. Sci. 1995, 84, 499–501. [Google Scholar] [CrossRef]
- Ballerini, L.; Franke-Stenport, V.; Borgefors, G.; Johansson, C.B. Comparison of Histomorphometrical Data obtained with Two Different Image Analysis Methods. J. Mater. Sci. Mater. Med. 2007, 18, 1471–1479. [Google Scholar] [CrossRef]
- Cox, M.R.; Budhu, M. A practical approach to grain shape quantification. Eng. Geol. 2008, 96, 1–16. [Google Scholar] [CrossRef]
- Wawrzenczyk, J.; Molendowska, A. The Use of a Special Stereoscopic Microscope Attachment for the Sieve Analysis of Aggregate in Concrete. Appl. Sci. 2019, 9, 1853. [Google Scholar] [CrossRef]
- Patchigolla, K.; Wilkinson, D. Crystal Shape Characterisation of Dry Samples using Microscopic and Dynamic Image Analysis. Part. Part. Syst. Charact. 2009, 26, 171–178. [Google Scholar] [CrossRef]
- Babick, F. Characterisation of Colloidal Suspensions. In Suspensions of Colloidal Particles and Aggregates; Particle Technology Series Vol. 20; Springer: Cham, Switzerland, 2016; Chapter 2; p. 17. [Google Scholar] [CrossRef]
- Rs’ Science. 2022. Available online: https://rsscience.com/stereo-microscope/ (accessed on 23 November 2022).
- Davey, R. What are Stereo Microscopes Used For? News-Medical. 17 January 2020. Available online: https://www.news-medical.net/life-sciences/What-are-Stereo-Microscopes-Used-For.aspx (accessed on 23 November 2022).
- Vissotto, F.Z.; Giarola, R.C.; Jorge, L.C.; Makita, G.T.; Cardozo, G.M.B.Q.; Rodrigues, M.I.; Menegalli, F.C. Morphological characterization with image analysis of cocoa beverage powder agglomerated with steam. Food Sci. Technol. 2014, 34, 649–656. [Google Scholar] [CrossRef]
- Ulusoy, U.; Atagun, O.N. Particle shape characterization of shaking table streams in a Turkish chromite concentration plant by using dynamic imaging and microscopical techniques. Part. Sci. Technol. 2022, in press. [Google Scholar] [CrossRef]
- Mills, O.P.; Rose, W.I. Shape and surface area measurements using scanning electron microscope stereo-pair images of volcanic ash particles. Geosphere 2010, 6, 805–811. [Google Scholar] [CrossRef]
- Critchley, L. Editorial future, Azo Materials, Electron Microscopy—How It Works, 22 April 2019. Available online: https://www.azom.com/article.aspx?ArticleID=17915 (accessed on 24 September 2022).
- Black, C.A.; Evans, D.D.; White, J.L.; Ensminger, L.E.; Clark, F.E. Methods of Soil Analysis; Part 1; Physical and Mineralogical Properties, Including Statistics of Measurement and Sampling, 9.1; American Society of Agronomy, Inc.: Madison, WI, USA, 1965; p. 770. [Google Scholar] [CrossRef]
- Blatt, H. Sedimentary Petrology; W. H. Freeman and Company: New York, NY, USA, 1982; p. 564. [Google Scholar]
- De Pellegrin, D.V.; Stachiowak, G.W. Assessing the role of particle shape and scale in abrasion using ‘sharpness analysis’; Part I. Technique development. Wear 2002, 253, 1016–1025. [Google Scholar] [CrossRef]
- Ulusoy, U. Effect of different grinding on critical surface tension of wetting. Unpublished Ph.D. Thesis, Cumhuriyet University, Sivas, Turkey, 2003. [Google Scholar]
- Forssberg, E.; Zhai, H. Shape and surface properties of particles liberated by autogenous grinding. Scand. J. Metall. 1985, 14, 25–32. [Google Scholar]
- Beyer, W.H. Handbook of Mathematical Sciences; CRC Press: Boca Raton, FL, USA, 1978. [Google Scholar]
- Heywood, H. The Scope of Particle Size Analysis and Standardization. The Institution of Chemical Engineers. In Proceedings of Symposium on Particle Size Analysis, February 4, 1947; Supplement to Transactions. Inst. Chem. Eng. 1947, 25, 14–24. [Google Scholar]
- Hausner, H.H. Characterization of the powder particle shape. Planseeber. Pulvermetall. 1966, 14, 75–84. [Google Scholar]
- Serra, J. Image Analysis and Mathematical Morphology; Academic Press: New York, NY, USA, 1982. [Google Scholar]
- Ulusoy, U.; Yekeler, M.; Hiçyılmaz, C. Determination of the shape, morphological and wettability properties of Quartz and their correlations. Miner. Eng. 2003, 16, 951–964. [Google Scholar] [CrossRef]
- Hentschel, M.L.; Page, N.W. Selection of descriptors for particle shape characterization. Part. Syst. Charact. 2003, 20, 25–83. [Google Scholar] [CrossRef]
- Altuhafi, F.N.; O’Sullivan, C.; Cavarretta, I. Analysis of an image-based method to quantify the size and shape of sand particles. J. Geotech. Geoenviron. Eng. 2013, 139, 1290–1307. [Google Scholar] [CrossRef]
- Xiao, Y.; Long, L.H.; Matthew, E.T.; Zhou, H.; Liu, H.L.; Stuedlein, A.W. Effect of particle shape on stress-dilatancy responses of medium-dense sands. J. Geotech. Geoenviron. Eng. 2019, 145, 04018105. [Google Scholar] [CrossRef]
- Zheng, J.; Sun, Q.; Zheng, H.; Wei, D.; Li, Z.; Gao, L. Three-dimensional particle shape characterizations from half particle geometries. Powder Technol. 2020, 367, 122–132. [Google Scholar] [CrossRef]
- Stachowiak, G.W.; Podsiadlo, P. Characterization and classification of wear particles and surfaces. Wear 2001, 249, 194–200. [Google Scholar] [CrossRef]
- Pourghahramani, P.; Forssberg, F. Review of Applied Particle Shape Descriptors and Produced Particle Shapes in Grinding Environments. Part I: Particle Shape Descriptors. Miner. Process. Extr. Metall. Rev. 2005, 26, 145–166. [Google Scholar] [CrossRef]
- Zheng, J.; Hryciw, R.D. Soil particle size and shape distributions by stereophotography and image analysis. Geotech. Test. J. 2017, 40, 317–328. [Google Scholar] [CrossRef]
- Hicyilmaz, C.; Ulusoy, U.; Bilgen, S.; Yekeler, M. Flotation responses of morphological properties of particles measured with a 3-dimensional approach. Int. J. Miner. Process. 2005, 75, 229–236. [Google Scholar] [CrossRef]
- Hicyilmaz, C.; Ulusoy, U.; Bilgen, S.; Yekeler, M.; Akdogan, G. Response of rough and acute surfaces of pyrite with 3-D approach to the flotation. J. Min. Sci. 2006, 42, 393–402. [Google Scholar] [CrossRef]
- Ulusoy, U.; Hicyilmaz, C.; Yekeler, M.; Bilgen, S.; Akdogan, G. Review of Morphological Attributes with the New 3-D Approach: Role of Roughness and Acuteness of Barite and Pyrite Particles on the Floatability. In Proceedings of the XXIV International Mineral Processing Congress, Beijing, China, 24–28 September 2008; Wang, D.Z., Sun, C.Y., Wang, F.L., Zhang, L.C., Han, L., Eds.; Science Press: Beijing, China; pp. 300–309. [Google Scholar]
- Anonymous. Permaran Operating Instructions; Outokumpu, Oy: Tapiola, Finland, 1970. [Google Scholar]
- Brunauer, S.; Emmett, P.H.; Teller, E. Adsorption of Gases in Multimolecular Layers. J. Am. Chem. Soc. 1938, 60, 309–319. [Google Scholar] [CrossRef]
- Horwell, C.J.; Sparks, R.S.J.; Brewer, T.S.; Llewellin, E.W.; Williamson, B.J. Characterization of respirable volcanic ash from the Soufriere Hills volcano, Montserrat, with implications for human health hazards. Bull. Volcanol. 2003, 65, 346–362. [Google Scholar] [CrossRef]
- Hohenberger, W. Fillers and reinforcements. In Plastics Additives Handbook; Zweifel, H., Ed.; Hanser Publishers: Munich, Germany, 2001; pp. 901–948. [Google Scholar]
- Moore, A.G. Automatic scanning and computer process for the quantitative analysis of micrographs and equivalent subjects. In Pictorial Pattern Recognition; Cheng, G.C., Ledley, R.S., Pollock, D.K., Rosenfeld, A., Eds.; Thompson Book Co.: Washington, DC, USA, 1968; pp. 275–326. [Google Scholar]
- Persson, A.L. Image analysis of shape and size of fine aggregates. Eng. Geol. 1998, 50, 177–186. [Google Scholar] [CrossRef]
- Wang, Z.; Sohn, I.L. Effect of the Al2O3/SiO2 mass ratio on the crystallization behavior of CaO-SiO2-MgO-Al2O3 slags using confocal laser scanning microscopy. Ceram. Int. 2018, 44, 19268–19277. [Google Scholar] [CrossRef]
- Xing, B.; Fan, W.; Zhuang, C.; Qian, C.; Lv, X. Effects of the morphological characteristics of mineral powder fillers on the rheological properties of asphalt mastics at high and medium temperatures. Powder. Technol. 2019, 348, 33–42. [Google Scholar] [CrossRef]
- Gonzalez, R.C.; Woods, R.E. Digital Image Processing; Addison-Wesley: Reading, MA, USA, 1993; pp. 418–420. [Google Scholar]
- Li, C.; Xu, C.; Gui, C.; Fox, M.D. Level set evolution without re-initialization: A new variational formulation. In Proceedings of the 2005 IEEE Computer Society Conference on Computer Vision and Pattern Recognition (CVPR’05), San Diego, CA, USA, 20–25 June 2005; Volume 1, pp. 430–436. [Google Scholar] [CrossRef]
- Wang, W.; Ji, T. Adaptive analysis method for particles image. Multimed. Tools Appl. 2019, 78, 15317–15333. [Google Scholar] [CrossRef]
- Ulusoy, U.; Igathinathane, C. Particle size distribution modeling of milled coals by dynamic image analysis and mechanical sieving. Fuel Process. Technol. 2016, 143, 100–109. [Google Scholar] [CrossRef]
- Ulusoy, U. Application of ANOVA to image analysis results for talc particles produced by different milling. Powder Technol. 2008, 188, 133–138. [Google Scholar] [CrossRef]
- Pei, J.; Bi, Y.; Zhang, J.; Li, R.; Liu, G. Impacts of aggregate geometrical features on the rheological properties of asphalt mixtures during compaction and service stage. Constr. Build. Mater. 2016, 126, 165–171. [Google Scholar] [CrossRef]
- Yue, Z.Q.; Morin, I. Digital image processing for aggregate orientation in asphalt concrete mixtures. Can. J. Civ. Eng. 1996, 23, 480–489. [Google Scholar] [CrossRef]
- Rajan, B.; Singh, D. Understanding influence of crushers on shape characteristics of fine aggregates based on digital image and conventional techniques. Constr. Build. Mater. 2017, 150, 833–843. [Google Scholar] [CrossRef]
- Liu, Y.; Huang, Y.; Sun, W.; Nair, H.; Lane, D.S.; Wang, L. Effect of coarse aggregate morphology on the mechanical properties of stone matrix asphalt. Constr. Build. Mater. 2017, 152, 48–56. [Google Scholar] [CrossRef]
- Westermann, J. Trends in Particle Size Analysis: Quality Control in the Fertiliser Industry with Dynamic Image Analysis; Retsch Technology GmbH: Hertfordshire, UK, 2014; Available online: https://www.labmate-online.com/article/laboratory-products/3/microtrac-retsch-gmbh/trends-in-particle-size-analysis-quality-control-in-the-fertiliser-industry-with-dynamic-image-analysis/1662 (accessed on 23 November 2022).
- Rabinski, G.; Thomas, D. Dynamic digital image analysis: Emerging technology for particle characterization. Water Sci Technol. 2014, 50, 19–26. [Google Scholar] [CrossRef]
- Westermann, J. Particle Characterization with Dynamic Image Analysis; Retsch Technology GmbH: Haan, Germany, 2022; Available online: https://cdn.technologynetworks.com/TN/Resources/PDF/tr_whitepaper_pharma_0813_en.pdf (accessed on 23 November 2022).
- Tysmans, D.; Claeys, P.; Deriemaeker, L.; Maes, D.; Finsy, R.; Molle, M.V. Size and Shape Analysis of Sedimentary Grains by Automated Dynamic Image Analysis. Part. Part. Syst. Charact. 2006, 23, 381–387. [Google Scholar] [CrossRef]
- Ulusoy, U.; Igathinathane, C. Dynamic image based shape analysis of hard and lignite coal particles ground by laboratory ball and gyro mills. Fuel Process. Technol. 2014, 126, 350–358. [Google Scholar] [CrossRef]
- Fernlund, J.M.R. Image analysis method for determining 3-D shape of coarse aggregate. Cem. Concr. Res. 2005, 35, 1629–1637. [Google Scholar] [CrossRef]
- Evans, C.L.; Wightman, E.M.; Manlapig, E.V.; Coulter, B.L. Application of process mineralogy as a tool in sustainable processing. Miner. Eng. 2011, 24, 1242–1248. [Google Scholar] [CrossRef]
- Rule, C.; Schouwstra, R.P. Process mineralogy delivering significant value at Anglo Platinum concentrator operations. In Proceedings of the 10th International Congress for Applied Mineralogy; Broekmans, M.A.T.M., Ed.; Springer: Heidelberg, Germany, 2011; pp. 1–5. [Google Scholar] [CrossRef]
- Little, L.; Wiese, J.; Becker, M.; Mainza, A.; Ross, V. Investigating the effects of particle shape on chromite entrainment at a platinum concentrator. Miner. Eng. 2016, 96–97, 46–52. [Google Scholar] [CrossRef]
- Little, L.; Becker, M.; Wiese, J.; Yorath, G.; Mainza, A.N.; Tonzetic, I. Shape characterisation: Can different devices produce comparable data for particulate samples? In Proceedings of the XXVIIIth International Mineral Processing Congress, Quebec City, QC, Canada, 11–15 September 2016. [Google Scholar]
- Fennel, M.; Guevara, J.; Canchaya, S.; Velarde, G.; Baum, W.; Gottlieb, P. QEMSCAN Mineral Analysis for Ore Characterisation and Plant Support at Cerro Verde. In Proceedings of the 27th Mining Convention, Technical Papers Metallurgical Processes, Arequipa, Peru, 12–15 September 2005; Available online: https://sampling-ok.com/files/QemscanMineralAnalysisforOreCharacterization.pdf (accessed on 26 November 2022).
- Kuo, C.-Y.; Freeman, R.B. Image analysis evaluation of aggregates for asphalt concrete mixtures. Transp. Res. Rec. 1998, 1615, 65–71. [Google Scholar] [CrossRef]
- Prowell, B.D.; Weingart, R.L. Precision of flat and elongated particle tests: ASTM 4791 and VDG 40 video grader. In Proceedings of the 78th Annual Meeting of the Transportation Research Board, Transportation Research Board, Washington, DC, USA, 10–14 January 1999. [Google Scholar]
- Rao, C.; Tutumluer, E. Determination of Volume of Aggregates: New Image-Analysis Approach. J. Transp. Res. Rec. 2000, 1721, 73–80. [Google Scholar] [CrossRef]
- Anochie-Boateng, J.K.; Komba, J.J.; Mvelase, G.M. Three-dimensional laser scanning technique to quantify aggregate and ballast shape properties. Constr. Build. Mater. 2013, 43, 389–398. [Google Scholar] [CrossRef]
- Jia, X.; Liu, R.; Ren, H.; Han, Y.; Ouyang, J.; Zheng, H.; Peng, C.; Zheng, J. Particle shape characterizations for energetic materials by computational geometry and stereology method. SN Appl. Sci. 2022, 4, 147. [Google Scholar] [CrossRef]
- Garboczi, E.J. Three-dimensional mathematical analysis of particle shape using X-ray tomography and spherical harmonics: Application to aggregates used in concrete. Cem. Concr. Res. 2002, 32, 1621–1638. [Google Scholar] [CrossRef]
- Whiting, J.G.; Garboczi, E.J.; Tondare, V.N.; Scott, J.H.J.; Donmez, M.A.; Moylan, S.P. A comparison of particle size distribution and morphology data acquired using lab-based and commercially available techniques: Application to stainless steel powder. Powder Technol. 2022, 396, 648–662. [Google Scholar] [CrossRef]
- Slotwinski, J.A.; Garboczi, E.J.; Stutzman, P.E.; Ferraris, C.F.; Watson, S.S.; Peltz, M.A. Characterization of metal powders used for additive manufacturing. J. Res. Natl. Inst. Stand. Technol. 2014, 119, 460. [Google Scholar] [CrossRef]
- Garboczi, E.J.; Bullard, J.W. 3D analytical mathematical models of random star-shape particles via a combination of X-ray computed microtomography and spherical harmonic analysis. Adv. Powder Technol. 2017, 28, 325–339. [Google Scholar] [CrossRef]
- Flannery, B.P.; Deckman, H.W.; Roberge, W.G.; D’Amico, K.L. Three- dimensional X-ray microtomography. Science 1987, 237, 1439–1443. [Google Scholar] [CrossRef]
- Bhuiyan, I.U. Characterization of Iron Ore Green Pellets by Scanning Electron Microscopy and X-ray Microtomography. Ph.D. Thesis, Luleå University of Technology, Luleå, Sweden, 2011. [Google Scholar]
- Shatokha, V.; Korobeynikov, I.; Maire, E.; Adrien, J. Application of 3D X-ray tomography to investigation of structure of sinter mixture granules. Ironmak. Steelmak. 2009, 36, 416–420. [Google Scholar] [CrossRef]
- Lenarno, F.; Tolppanen, P. 3D Characterization of coarse aggregates. Eng. Geol. 2002, 15, 17–30. [Google Scholar] [CrossRef]
- Kim, H.; Haas, C.T.; Rauch, A.F.; Browne, C. 3D image segmentation of aggregates from laser profiling. Comput. Aided Civ. Infrastruct. Eng. 2003, 18, 254–263. [Google Scholar] [CrossRef]
- Pan, T.; Tutumluer, E. Imaging-based direct measurement of aggregate surface area and its application in asphalt mixture design. Int. J. Pavement Eng. 2010, 11, 415–428. [Google Scholar] [CrossRef]
- Tolppanen, P.; Illerstrom, A.; Stephansson, O. 3-D Laser analysis of size, shape and roughness of railway ballast: Research in progress. In Proceedings of the 7th Annual Symposium, ICAR, Austin, TX, USA, 19–21 April 1999. [Google Scholar]
- Hayakawa, Y.; Oguchi, T. Evaluation of gravel sphericity and roundness based on surface-area measurement with a laser scanner. Comput. Geosci. 2005, 3, 735–741. [Google Scholar] [CrossRef]
- Raja, P.M.V.; Barron, A.R. Transmission Electron Microscopy. In Physical Methods in Chemistry and Nano Science (Barron); Rice University: Houston, TX, USA, 28 August 2022; Chapter 8.2; Available online: https://chem.libretexts.org/Bookshelves/Analytical_Chemistry/Physical_Methods_in_Chemistry_and_Nano_Science_(Barron)/08%3A_Structure_at_the_Nano_Scale/8.02%3A_Transmission_Electron_Microscopy (accessed on 23 November 2022).
- Kim, Y.; Ma, J.; Lim, S.Y.; Song, J.Y.; Yun, T.S. Determination of shape parameters of sands: A deep learning approach. Acta Geotech. 2022, 17, 1521–1531. [Google Scholar] [CrossRef]
- Endoh, S. Particle Shape Characterization. In Powder Technology. Fundamentals of Particles, Powder Beds, and Particle Generation; Masuda, H., Higashitani, K., Yoshida, H., Eds.; CRC Press: Boca Raton, FL, USA, 2007; Chapter 1.3; pp. 33–47. [Google Scholar] [CrossRef]
- Miwa, S. Funryutai Kogaku; Asakura Shoten: Tokyo, Japan, 1972; p. 65. (In Japanese) [Google Scholar]
- Boler, H.; Qian, Y.; Tutumluer, E. Influence of Size and Shape Properties of Railroad Ballast on Aggregate Packing: Statistical Analysis. Transp. Res. Rec. 2014, 2448, 94–104. [Google Scholar] [CrossRef]
- Tutumluer, E.; Huang, H.; Hashash, Y.; Ghaboussi, J. Aggregate shape effects on ballast tamping and railroad track lateral stability. In Proceedings of the AREMA 2006 Annual Conference, AREMA, Louisville, KY, USA, 17–20 September 2006; Available online: https://www.researchgate.net/profile/Erol-Tutumluer/publication/237116793_Aggregate_Shape_Effects_on_Ballast_Tamping_and_Railroad_Track_Lateral_Stability/links/0046353691a2c498c1000000/Aggregate-Shape-Effects-on-Ballast-Tamping-and-Railroad-Track-Lateral-Stability.pdf (accessed on 23 November 2022).
- Siswosoebrotho, B.I.; Ginting, K.; Soedirdjo, T.L. Workability and resilient modulus of asphalt concrete mixtures containing flaky aggregates shape. J. East Asia Soc. Transp. Stud. 2005, 6, 1302–1312. [Google Scholar] [CrossRef]
- Cao, Z.; Masanet, E.; Tiwari, A.; Akolawala, S. Decarbonizing Concrete: Deep decarbonization Pathways for the Cement and Concrete Cycle in the United States, India, and China; Industrial Sustainability Analysis Laboratory, Northwestern University: Evanston, IL, USA, 2021. [Google Scholar]
- Belaïd, F. How does concrete and cement industry transformation contribute to mitigating climate change challenges? RCR Adv. 2022, 15, 200084. [Google Scholar] [CrossRef]
- Hudson, B. Modification to the Fine Aggregate Angularity Test. In Proceedings of the 7th Annual International Center for Aggregates Research Symposium, ICAR, Austin, TX, USA, 19–21 April 1999. [Google Scholar]
- Zielinski, Z. Korelacja Parametrów Technologicznych Mechanicznego Kruszeniai Przesiewania Materiałów Skalnych Stosowanych w Budownictwie Drogowym; Wydawnictwo Uczelniane Politechniki Szczecin skiej: Szczecin, Poland, 1983; p. 228. [Google Scholar]
- Neville, A.M. Własciwosci Betonu; Polski Cement: Kraków, Poland, 2000. [Google Scholar]
- Tumidajski, T.; Naziemiec, Z. Wpływ warunków procesu kruszenia na kształt ziaren kruszyw mineralnych. In IV Konferencja, Kruszywa Mineralne-Surowce-Rynek-Technologie-Jakosc; Szklarska, P., Ed.; Politechnika Wrocławska: Wrocław, Poland, 2004. [Google Scholar]
- Naziemiec, Z.; Gawenda, T. Ocena efektów rozdrabniania surowców mineral nychwróz nych urza dzeniach krusza cych. In VI Konferencja, Kruszywa Mineralne-Surowce-Rynek-Technologie-Jakosc; Szklarska, P., Ed.; OWPW: Wrocław, Poland, 2006; pp. 83–94. [Google Scholar]
- Malewski, J. Kształt ziaren w produktach kruszenia. Kruszywa 2014, 3, 52–55. [Google Scholar]
- Gawenda, T. Zasady Doboru Kruszarek Oraz Układów Technologicznych w Produkcji Kruszyw Łamanych; AGH University of Science and Technology Press: Cracow, Poland, 2015; pp. 1–232. [Google Scholar]
- Gawenda, T. Innowacyjne technologie produkcji kruszyw o ziarnach foremnych. Min. Sci. 2020, 22, 45–59. Available online: http://www.miningscience.pwr.edu.pl/pdf-59952-3147?filename=INNOVATIVE%20TECHNOLOGIES.pdf (accessed on 26 November 2022).
- Jamkar, S.S.; Rao, C.B.K. Index of Aggregate Particle Shape and Texture of coarse aggregate as a parameter for concrete mix proportioning. Cem. Concr. Res. 2004, 34, 2021–2027. [Google Scholar] [CrossRef]
- Erdogan, S.T. Determination of aggregate shape properties using X-ray tomographic methods and the effect of shape on concrete rheology. Ph.D. Dissertation, University of Texas at Austin, Austin, TX, USA, 2005. [Google Scholar]
- Erdogan, S.T.; Quiroga, P.N.; Fowler, D.W.; Saleh, H.A.; Livingston, R.A.; Garboczi, E.J.; Ketcham, P.M.; Hagedorn, J.G.; Satterfield, S.G. Three- dimensional shape analysis of coarse aggregates: New techniques for and preliminary results on several different coarse aggregates and reference rocks. Cem. Concr. Res. 2006, 36, 1619–1627. [Google Scholar] [CrossRef]
- Quiroga, P.N.; Fowler, D.W. The Effects of Aggregate Characteristics on the Performance of Portland Cement Concrete; Report ICAR 104-1F. Project Number 104; International Center for Aggregates Research, University of Texas: Austin, TX, USA, 2003. [Google Scholar]
- Polat, R.; Yadollahi, M.M.; Sagsoz, A.E.; Arasan, S. The Correlation Between Aggregate Shape and Compressive Strength of Concrete: Digital Image Processing Approach. Int. J. Struct. Civ. Eng. Res. 2013, 2, 62–80. Available online: http://www.ijscer.com/uploadfile/2015/0429/20150429074945165.pdf (accessed on 23 November 2022).
- Ozol, M.A. Test and Properties of Concrete Aggregates, Shape, Surface Texture, Surface Area, and Coatings; STP169B-EB; ASTM International: West Conshohocken, PA, USA, 1978; Chapter 35; pp. 584–628. [Google Scholar] [CrossRef]
- Kwan, A.K.H.; Mora, C.F.; Chan, H.C. Particle shape analysis of coarse aggregate using digital image processing. Cem. Concr. Res. 1999, 29, 1403–1410. [Google Scholar] [CrossRef]
- Sims, I.; Brown, B. Concrete Aggregates. In Lea’s Chemistry of Cement and Concrete, 4th ed.; Hewlett, P.C., Ed.; Butterworth-Heinemann: Woburn, MA, USA, 1998; Chapter 16; pp. 907–1015. ISBN 9780750662567. [Google Scholar] [CrossRef]
- Ostrowski, K.; Sadowski, Ł.; Stefaniuk, D.; Wałach, D.; Gawenda, T.; Oleksik, K.; Usydus, I. The effect of the morphology of coarse aggregate on the properties of self-compacting high-performance fibre-reinforced concreto. Materials 2018, 11, 1372. [Google Scholar] [CrossRef] [PubMed]
- Saramak, A.; Naziemiec, Z. Determination of dust emission level for various crushing devices. Min. Sci. 2019, 26, 45–54. [Google Scholar] [CrossRef]
- Xing, B.; Du, Y.; Fang, C.; Sun, H.; Lyu, Y.; Fan, W. Particle morphology of mineral filler and its effects on the asphalt binder-filler interfacial interaction. Constr. Build. Mater. 2022, 321, 126292. [Google Scholar] [CrossRef]
- Ramlan, A.S.; Zin, R.M.; Bakar, N.F.A.; Othman, N.H. Recent progress on proppant laboratory testing method: Characterisation, conductivity, transportation, and erosivity. J. Pet. Sci. Eng. 2021, 205, 108871. [Google Scholar] [CrossRef]
- Alotaibi, M.A.; Miskimins, J.L. Slickwater proppant transport in complex fractures: New experimental findings & scalable correlation. In Proceedings of the SPE Annual Technical Conference and Exhibition. Society of Petroleum Engineers, Houston, TX, USA, 28 September 2015. [Google Scholar] [CrossRef]
- Baldini, M.; Carlevaro, C.M.; Pugnaloni, L.A.; Sanchez, M. Numerical simulation of proppant transport in a planar fracture. A study of perforation placement and injection strategy. Int. J. Multiph. Flow. 2018, 109, 207–218. [Google Scholar] [CrossRef]
- Liang, F.; Sayed, M.; Al-Muntasheri, G.A.; Chang, F.F.; Li, L. A comprehensive review on proppant technologies. Petroleum 2016, 2, 26–39. [Google Scholar] [CrossRef]
- Hellmann, J.R.; Scheetz, B.E.; Luscher, W.G.; Hartwich, D.G.; Koseski, R.P. Engineering ceramics for stimulation of unconventional energy resources. Am. Ceram. Soc. Bull. 2009, 93, 28–35. [Google Scholar]
- Montgomery, C.T.; Smith, M.B. Hydraulic fracturing: History of an enduring technology. J. Pet. Technol. 2010, 62, 26–40. [Google Scholar] [CrossRef]
- Hydraulic Fracturing 101, Washington State University, December 2017. Available online: http://ses.wsu.edu/wp-content/uploads/2017/12/Hydraulic-Fracturing-101.pdf (accessed on 23 November 2022).
- Alkhasov, S. Commercializing a Resin-Coated Proppant. Ph.D. Thesis, Case Western Reserve University, Cleveland, OH, USA, 2014. Available online: https://etd.ohiolink.edu/apexprod/rws_etd/send_file/send?accession=case1404838630&disposition=inline (accessed on 26 November 2022).
- Schmidt, K.K. Propublica, FRACKING, What Is Hydraulic Fracturing? 13 November 2008. Available online: https://www.propublica.org/article/hydraulic-fracturing-national (accessed on 23 November 2022).
- Gallagher, D. Hierarchy of oily conductivity. J. Pet. Technol. 2011, 63, 18–20. [Google Scholar] [CrossRef]
- Veatch, R.W. Overview of current hydraulic fracturing design and treatment technology—Part 2. J. Pet. Technol. 1983, 35, 853–864. [Google Scholar] [CrossRef]
- Economides, M.J.; Nolte, K.G. Reservoir Stimulation, 3rd ed.; Wiley and Sons Ltd.: West Sussex, UK, 2000. [Google Scholar]
- Reinicke, A.; Rybacki, E.; Stanchits, S.; Huenges, E.; Dresen, G. Hydraulic fracturing stimulation techniques and formation damage mechanisms-implications from laboratory testing of tight sandstone-proppant systems. Geochemistry 2010, 70, 107–117. [Google Scholar] [CrossRef]
- Alseamr, N.A.H. A Theoretical Simulation of the Settling of Proppants in a Hydraulic Fracturing Process. Master’s Thesis, Virginia Commonwealth University, Richmond, VA, USA, 2016. [Google Scholar]
- Liang, F.; Sayed, M.; Al-Muntasheri, G.; Chang, F.F. Overview of existing proppant technologies and challenges. In Proceedings of the SPE Middle East Oil & Gas Show and Conference Manama, Manama, Bahrain, 8 March 2015. [Google Scholar]
- Scott, G.D. Packing of spheres. Nature 1960, 188, 908–909. [Google Scholar] [CrossRef]
- Liu, G.; Thompson, K.E. Influence of computational domain boundaries on internal structure in low-porosity sphere packing. Powder Technol. 2000, 113, 185–196. [Google Scholar] [CrossRef]
- Roblee, L.H.S.; Baird, R.M.; Tierney, J.W. Radial porosity variations in packed beds. AIChE J. 1958, 4, 460–464. [Google Scholar] [CrossRef]
- Zhang, W.; Thompson, K.E.; Reed, A.H.; Beenken, L. Relationship between packing structure and porosity in fixed beds of equilateral cylindrical particles. Chem. Eng. Sci. 2006, 61, 8060–8074. [Google Scholar] [CrossRef]
- Alary, J.A.; Parias, T. Method of Manufacturing and Using Rod-Shaped Proppants and Anti-Flowback Additives. U.S. Patent No. 8,562,900 B2, 22 October 2013. [Google Scholar]
- McDaniel, G.; Abbott, J.; Mueller, F.; Mokhtar, A.; Pavlova, S.; Nevvonen, O.; Parias, T.; Alary, J.A. Changing the Shape of Fracturing: New Proppant Improves Fracture Conductivity. In Proceedings of the SPE Annual Technical Conference and Exhibition, Florence, Italy, 19 September 2010. [Google Scholar] [CrossRef]
- Phoowarang, J. A numerical model investigation of the packing characteristics of different shaped proppants for use with hydraulic fracturing. Master’s Thesis, Department of Earth Science and Engineering Imperial College London, London, UK, 2012. [Google Scholar]
- Osuji, N.I.; Zhang, J.; Tagne, M.D. Numerical simulation on the effect of particle shape on mechanical response of proppants in horizontal fractures. Comput. Part. Mech. 2022, 9, 513–523. [Google Scholar] [CrossRef]
- Mehmood, F.; Liao, J.; Hou, M.Z.; Zahoor, M.K.; Xiong, Y. Optimization of hydraulic fracturing with rod-shaped proppants for improved recovery in tight gas reservoirs. Geomech. Geophys. Geo Energy Geo Resour. 2022, 8, 38. [Google Scholar] [CrossRef]
- Pocock, B.W.; Rhodes, C.C. Principles of glass-bead reflectorization. Highw. Res. Board Bull. 1952, 57, 32–48. [Google Scholar]
- Babic, D.; Burghardt, T.E.; Babic, D. Application and characteristics of waterborne road marking paint. Int. J. Traffic Transp. Eng. 2015, 5, 150–169. [Google Scholar] [CrossRef] [PubMed]
- Schnell, T.; Zwahlen, H. Driver preview distances at night based on driver eye scanning recordings as a function of pavement marking retroreflectivities. Transp. Res. Record 1999, 1692, 129–141. [Google Scholar] [CrossRef]
- Burghardt, T.E.; Pashkevich, A.; Babic, D.; Mosbock, H.; Babic, D.; Zakowska, L. Microplastics and road markings: The role of glass beads and loss estimation. Transp. Res. Part D 2022, 102, 103123. [Google Scholar] [CrossRef]
- European Standard EN 1436; Road Marking Materials-Road Marking Performance for Road Users and Test Methods. European Committee for Standardization: Brussels, Belgium, 2018.
- Plainis, S.; Murray, I.; Pallikaris, I. Road traffic casualties: Understanding the night-time death toll. Inj. Prev. 2006, 12, 125–138. [Google Scholar] [CrossRef]
- Wenzel, K.M.; Burghardt, T.E.; Pashkevich, A.; Buckermann, W.A. Glass Beads for Road Markings: Surface Damage and Retroreflection Decay Study. Appl. Sci. 2022, 12, 2258. [Google Scholar] [CrossRef]
- Hagerty, M.M.; Hite, D.R.; Ullrich, C.R.; Hagerty, D.J. One-dimensional high-pressure compression of granular media. J. Geotech. Eng. ASCE 1993, 119, 1–18. [Google Scholar] [CrossRef]
- ALLGlass Limited, Scotland. 2022. Available online: https://www.geveko-markings.com/fileadmin/root/common/pdf/brochures/UCme_R__Brochure_GB.pdf (accessed on 23 November 2022).
- King, D.A.; Doi, M.; Eiser, E. Particle shapes leading to Newtonian dilute suspensions. Phys. Rev. E 2020, 102, 032615. [Google Scholar] [CrossRef]
- Pabst, W.; Gregorova, E.; Berthold, C. Particle shape and suspension rheology of short-fiber systems. J. Eur. Ceram. Soc. 2006, 26, 149–160. [Google Scholar] [CrossRef]
- Baltar, C.A.M.; da Luz, A.B.; Baltar, L.M.; de Oliveira, C.H.; Bezerra, F.J. Influence of morphology and surface charge on the suitability of palygorskite as drilling fluid. Appl. Clay Sci. 2009, 42, 597–600. [Google Scholar] [CrossRef]
- Yin, H.; Mo, D.; Chen, D. Orientation behaviour of attapulgite nanoparticles in poly(acrylonitrile)/attapulgite solutions by rheological analysis. J. Polym. Sci. B 2009, 47, 945–954. [Google Scholar] [CrossRef]
- Cilas. Particle Size and Shape Analysis in Pharmaceutical Industry. 2022. Available online: http://www.bruben.com.ar/pdf/Aplicaciones/015-pharma%20industry.pdf (accessed on 23 November 2022).
- Ten Brinke, A.J.W.; Bailey, L.; Lekkerkerker, H.N.W.; Maitland, G.C. Rheology modification in mixed shape colloidal dispersions. Part II: Mixtures. Soft Matter 2008, 4, 337–348. [Google Scholar] [CrossRef]
- Burba, J.L.; Holman, W.E.; Crabb, C.R. Laboratory and Field Evaluation of Novel Inorganic Drilling Fluid Additive; Paper IADC/SPE 17198, IADC/SPE Drilling Conference; Society of Petroleum Engineers: Houston, TX, USA, 1988; pp. 179–186. [Google Scholar] [CrossRef]
- Van der Kooij, F.; Lekkerkerker, H.N.W.; Boek, E.S. Process Fluid. World Pat. WO 03/014252, 2003. [Google Scholar]
- Ten Brinke, A.J.W.; Bailey, L.; Lekkerkerker, H.N.W.; Maitland, G.C. Rheology modification in mixed shape colloidal dispersions. Part I: Pure components. Soft Matter 2007, 3, 1145–1162. [Google Scholar] [CrossRef]
- Wypych, G. Fillers—Origin, Chemical Composition, Properties, and Morphology. In Handbook of Fillers, 5th ed.; ChemTec Publishing: Toronto, ON, Canada, 2021; Chapter 2; pp. 13–302. [Google Scholar] [CrossRef]
- Atkinson, J.F.; Chakraborti, R.K.; VanBenschoten, J.E. Effects of Floc Size and Shape in Particle Aggregation. In Flocculation in Natural and Engineered Environmental Systems, 1st ed.; CRC Press: Boca Raton, FL, USA, 2004; Chapter 5; pp. 95–120. [Google Scholar]
- De Haan, A.B. Solid-Liquid Separation. In Process Technology: An Introduction; Walter de Gruyter GmbH: Berlin, Germany; Boston, MA, USA, 2015; pp. 197–228. [Google Scholar]
- Svarovsky, L. Characterization of Particles Suspended in Liquids, Solid-Liquid Separation, 2nd ed.; Svarovsky, L., Ed.; Butterworths-Heinemann Ltd.: Oxford, UK, 1981; pp. 8–32. [Google Scholar]
- Trunk, R.; Bretl, C.; Thäter, G.; Nirschl, H.; Dorn, M.; Krause, M.J. A Study on Shape-Dependent Settling of Single Particles with Equal Volume Using Surface Resolved Simulations. Computation 2021, 9, 40. [Google Scholar] [CrossRef]
- Komar, P.D.; Reimers, C. Grain Shape Effects on Settling Rates. J. Geol. 1978, 86, 2. [Google Scholar] [CrossRef]
- Yokojima, S.; Takashima, R.; Asada, H.; Miyahara, T. Impacts of particle shape on sedimentation of particles. Eur. J. Mech. B Fluids 2021, 89, 323–331. [Google Scholar] [CrossRef]
- Jakub, H.; David, Z.; Lucie, J.; Jiri, Z.; Jan, N. Effect of Particle Shape and Size on the compressibility and bulk properties of powder. In Proceedings of the Metal 2016, Brno, Czech Republic, 25–27 May 2016. [Google Scholar]
- Bettersize Instruments Ltd. Powder Characteristics Guidebook. 2022. Available online: https://www.bettersizeinstruments.com/uploads/file/powder-characteristics-guidebook.pdf (accessed on 23 November 2022).
- Wouterse, A.; Williams, S.R.; Philipse, A.P. Effect of particle shape on the density and microstructure of random packings. J. Phys. Condens. Matter. 2007, 19, 406215. [Google Scholar] [CrossRef]
- Zhao, S.; Zhang, N.; Zhou, X.; Zhang, L. Particle shape effects on fabric of granular random packing. Powder Technol. 2017, 310, 175–186. [Google Scholar] [CrossRef]
- Ng, T.T.; Zhou, W.; Ma, G.; Chang, X.L. Macroscopic and microscopic behaviors of binary mixtures of different particle shapes and particle sizes. Int. J. Solids Struct. 2018, 135, 74–84. [Google Scholar] [CrossRef]
- Nouri, A.; Wen, C. Surfactants in Mechanical Alloying/Milling: A Catch-22 Situation. Crit. Rev. Solid State Mater. Sci. 2014, 39, 81–108. [Google Scholar] [CrossRef]
- Nazari, K.A.; Nouri, A.; Hilditch, T. Effects of milling time on powder packing characteristics and compressive mechanical properties of sintered Ti-10Nb-3Mo alloy. Mater. Lett. 2015, 140, 55–58. [Google Scholar] [CrossRef]
- Nazari, K.A.; Nouri, A.; Hilditch, T. Compressibility of a Ti-based alloy with varying amounts of surfactant prepared by high-energy ball milling. Powder Technol. 2015, 279, 33–41. [Google Scholar] [CrossRef]
- Allen, T. Particle Size Measurement, 5th ed.; Chapmann & Hall: Washington, DC, USA, 1997. [Google Scholar]
- Peleg, M. Flowability of food powders and methods for its evaluation-a review. J. Food Process Eng. 1977, 1, 303–328. [Google Scholar] [CrossRef]
- Chen, X.D. Mathematical analysis of powder discharge through longitudinal slits in a slowly rotating drum: Objective measurements of powder flowability. J. Food Eng. 1994, 21, 421–437. [Google Scholar] [CrossRef]
- Heaney, D.F. Handbook of Metal Injection Molding; Woodhead Publishing: Cambridge, UK, 2012. [Google Scholar]
- Bennett, J.P.; Smith, J.D. Fundamentals of Refractory Technology (Ceramic Transaction Series); American Chemical Society: Washington, DC, USA, 2001; Volume 25. [Google Scholar]
- Malvern Panalytical. Exploring the Role and Measurement of Particle Size and Shape in Metal Additive Manufacturing, PN12776. 2021. Available online: https://www.malvernpanalytical.com/br/learn/knowledge-center/whitepapers/wp20210727particlesizeshapemetalam (accessed on 23 November 2022).
- McGlinchey, D. Characterization of Bulk Solids; Blackwell Publishing Ltd.: Oxford, UK, 2005. [Google Scholar]
- Sukumaran, B.; Ashmawy, A.K. Influence of inherent particle characteristics on hopper flow rate. Powder Technol. 2003, 138, 46–50. [Google Scholar] [CrossRef]
- Podczeck, F.; Mia, Y. The influence of particle size and shape on the angle of internal friction and the flow factor of unlubricated and lubricated powders. Int. J. Pharm. 1996, 144, 187–194. [Google Scholar] [CrossRef]
- Orumwense, F.F.O.; Okorie, B.A.; Okeakpu, E.O.; Obiora, E.N.; Onyeji, L.I. Sintered copper–graphite powder compacts forindustrial applications. Powder Metall. 2001, 44, 62–66. [Google Scholar] [CrossRef]
- pharmacy180.com. 2022. Available online: https://www.pharmacy180.com/article/particle-shape-and-size---analyses-of-powders-2826/ (accessed on 13 March 2022).
- Kuno, H.; Hayashi, Y. Measurement of apparent density of powder. In Proceedings of the Fujihara Memorial Faculty of Engineering Keio University; Koganei: Tokyo, Japan, 1953; Volume 6, No. 22; pp. 59(6)–64(11). [Google Scholar]
- Hayashi, T.; Sasano, M.; Tsutsumi, Y.; Kawakita, K.; Ikeda, C. Segregation in the flow of powder-particles in tapping. J. Soc. Mater. Sci. Jpn. 1970, 19, 574–578. [Google Scholar] [CrossRef]
- Yubuta, K.; Gotoh, K.; Masuda, H. Size segregation of polydispersed particles caused by virtical tapping. J. Soc. Powder Technol. Jpn. 1995, 32, 8996. [Google Scholar] [CrossRef]
- Miyajima, T.; Yamamoto, K.; Sugimoto, M. Effect of particle shape on packing properties during tapping. Adv. Powder Technol. 2001, 12, 117–134. [Google Scholar] [CrossRef]
- Davies, R. A Simple Feature Based Representation of Particle Shape. Powder Technol. 1975, 12, 111–124. [Google Scholar] [CrossRef]
- German, R.M. Particle Packing Characteristics; Metal Powder Industries Federation: Princeton, NJ, USA, 1989. [Google Scholar]
- Poquillon, D.; Lemaitre, J.; Baco-Carles, V.; Tailhades, P.; Lacaze, J. Cold compaction of iron powders—Relations between powder morphology and mechanical properties: Part I: Powder preparation and compaction. Powder Technol. 2002, 126, 65–74. [Google Scholar] [CrossRef]
- Mamtani, K. Effect of particle shape on hopper discharge rate. Master’s Thesis, Graduate School of the University of Florida, Gainesville, FL, USA, 2011. [Google Scholar]
- Brown, R.L.; Richards, J.C. Exploratory study of the flow of granules through apertures. Trans. Inst. Chem. Eng. 1959, 37, 108–119. [Google Scholar]
- Cleary, P.W.; Sawley, M.L. DEM modelling of industrial granular flows: 3D case studies and the effect of particle shape on hopper discharge. Appl. Math. Model. 2002, 26, 89–111. [Google Scholar] [CrossRef]
- Jin, B.; Tao, H.; Zhong, W. Flow behaviors of non-spherical granules in rectangular hopper. Chin. J. Chem. Eng. 2010, 18, 931–939. [Google Scholar] [CrossRef]
- Langston, P.A.; Al-Awamleh, M.A.; Fraige, F.Y.; Asmar, B.N. Distinct element modelling of non-spherical frictionless particle flow. Chem. Eng. Sci. 2004, 59, 425–435. [Google Scholar] [CrossRef]
- Boateng, J.A.; Erol, T. Sustainable use of oil sands for geotechnical construction and oad building. J. ASTM Int. 2012, 9, 2. [Google Scholar] [CrossRef]
- Mota, M.; Teixeira, J.A.; Bowen, W.R.; Yelshin, A. Binary spherical particle mixed beds porosity and permeability relationship measurement. Trans. Filtration Soc. 2001, 1, 101–106. Available online: https://hdl.handle.net/1822/1403 (accessed on 26 December 2022).
- Witt, K.J.; Brauns, J. Permeability-Anisotropy due to particle shape. J. Geotech. Eng. 1983, 109, 1181–1187. [Google Scholar] [CrossRef]
- Göktepe, A.B.; Sezer, A. Effect of particle shape on density and permeability of sands. Proc. Inst. Civ. Eng. Geotech. Eng. 2010, 163, 307–320. [Google Scholar] [CrossRef]
- Pestan, J.M.; Whittle, A.J. Compression model for cohesionless soils. Geotechnique 1995, 45, 611–631. [Google Scholar] [CrossRef]
- Rouse, P.C.; Fannin, R.J.; Shuttle, D.A. Influence of roundness on the void ratio and strength of uniform sand. Geotechnique 2008, 58, 227–231. [Google Scholar] [CrossRef]
- Shin, H.; Santamarina, J.C. Role of particle angularity on the mechanical behavior of granular mixtures. J. Geotech. Geoenviron. Eng. 2013, 139, 353–355. [Google Scholar] [CrossRef]
- Altuhafi, F.N.; Coop, M.R.; Georgiannou, V.N. Effect of Particle Shape on the Mechanical Behavior of Natural Sands. J. Geotech. Geoenviron. Eng. 2016, 142, 04016071. [Google Scholar] [CrossRef]
- Bareither, C.A.; Edil, T.B.; Benson, C.H.; Mickelson, D.M. Geological and physical factors affecting the friction angle of compacted sands. J. Geotech. Geoenviron. Eng. 2008, 134, 1476–1489. [Google Scholar] [CrossRef]
- Kozicki, J.; Tejchman, J.; Mroz, Z. Effect of grain roughness on strength, volume changes, elastic and dissipated energies during quasi-static homogeneous triaxial compression using DEM. Granul. Matter. 2012, 14, 457–468. [Google Scholar] [CrossRef]
- Alshibli, K.A.; Druckrey, A.M.; Al-Raoush, R.I.; Weiskittel, T.; Lavrik, N.V. Quantifying morphology of sands using 3D imaging. J. Mater. Civ. Eng. 2015, 27, 04014275. [Google Scholar] [CrossRef]
- Suh, H.S.; Kim, K.Y.; Lee, J.; Yun, T.S. Quantification of bulk form and angularity of particle with correlation of shear strength and packing density in sands. Eng. Geol. 2017, 220, 256–265. [Google Scholar] [CrossRef]
- Yang, J.; Luo, X.D. Exploring the relationship between critical state and particle shape for granular materials. J. Mech. Phys. Solids 2015, 84, 196–213. [Google Scholar] [CrossRef]
- Adams, J. Particle Size and Shape Effects in Materials Science: Examples from Polymer and Paper Systems. Clay Miner. 1993, 28, 509–530. [Google Scholar] [CrossRef]
- Güden, M.; Çelik, E.; Hizal, A.; Altindis, M.; Çetiner, S. Effects of compaction pressure and particle shape on the porosity and compression mechanical properties of sintered Ti6Al4V powder compacts for hard tissue implantation. J. Biomed. Mater. Res. B 2008, 85, 547–555. [Google Scholar] [CrossRef]
- Nakayama, N.; Horita, M.; Sakagami, S.; Miki, H.; Miyazaki, T.; Takeishi, H. Effect of powder shape and size on mechanical properties of Al thin plate formed by compression shearing method at room temperature. Procedia Eng. 2014, 81, 1163–1168. [Google Scholar] [CrossRef]
- Ghadr, A.; Assadi-Langroudi, A. Effect of grain size and shape on undrained behaviour of sands. Int. J. Geosynth. Ground Eng. 2019, 5, 18. [Google Scholar] [CrossRef]
- Wohlers Report, 3D Printing and Additive Manufacturing State of the Industry; Annual Worldwide Progress Report; Wohlers Associates, ASTM International: Denver, CO, USA, 2020.
- Westbeek, S.; van Dommelen, J.A.W.; Remmers, J.J.C.; Geers, M.G.D. Influence of particle shape in the additive manufacturing process for ceramics. Comput. Math. Appl. 2019, 78, 2360–2376. [Google Scholar] [CrossRef]
- Thermofisher Scientific, Explorer 4 Additive, Qualify & Quantify your Metal Powders for Additive Manufacturing. 2018. Available online: https://assets.thermofisher.com/TFS-Assets/MSD/brochures/Explorer-4-Additive-Brochure.pdf (accessed on 23 November 2022).
- Sutton, A.T.; Kriewall, C.S.; Leu, M.C.; Newkirk, J.W. Powders for additive manufacturing processes: Characterization techniques and effects on part properties. In Proceedings of the 27th Annual International Solid Freeform Fabrication Symposium—An Additive Manufacturing Conference, Solid Freeform Fabrication 2016, Austin, TX, USA, 8–10 August 2016; Bourell, D.L., Crawford, R.H., Seepersad, C.C., Beaman, J.J., Fish, S., Marcus, H., Eds.; The University of Texas in Austin: Austin, TX, USA, 2016; pp. 1004–1030. [Google Scholar]
- Cacace, S.; Semeraro, Q. Influence of the atomization medium on the properties of stainless steel SLM parts. Addit. Manuf. 2020, 36, 101509. [Google Scholar] [CrossRef]
- Lemoisson, F.; Froyen, L. Understanding and improving powder metallurgical processes. In Fundamentals of Metallurgy; Seetharaman, S., Ed.; Woodhead Publishing Limited: Cambridge, UK, 2005; pp. 471–502. [Google Scholar]
- Mikli, V.; Kaerdi, H.; Kulu, P.; Besterci, M. Characterization of powder particle morphology. Proc. Est. Acad. Sci. Eng. 2001, 7, 22–34. [Google Scholar] [CrossRef]
- Fauchais, P.; Montavon, G.; Bertrand, G. From Powders to Thermally Sprayed Coatings. J. Therm. Spray Technol. 2010, 19, 56–80. [Google Scholar] [CrossRef]
- Wong, W.; Vo, P.; Irissou, E.; Ryabinin, A.N.; Legoux, J.G.; Yue, S. Effect of Particle Morphology and Size Distribution on Cold-Sprayed Pure Titanium Coatings. J. Thermal Spray Technol. 2013, 22, 1140–1153. [Google Scholar] [CrossRef]
- Skotnicova, K. Praskova Metalurgie. Studijni Opory: Ucebni Text, Scenare, Testy, 1st ed.; VSB—Technicka univerzita Ostrava: Ostrava, Czech Republic, 2013. [Google Scholar]
- Fang, Q.; Kang, Z. An investigation on morphology and structure of Cu-Cr alloy powders prepared by mechanical milling and alloying. Powder Technol. 2015, 270, 104–111. [Google Scholar] [CrossRef]
- Angelo, P.C.; Subramanian, R. Powder Metallurgy: Science, Technology and Applications; PHI Learning Pvt: New Delhi, India, 2008. [Google Scholar]
- Chikosha, S.; Shabalala, T.C.; Chikwanda, H.K. Effect of particle morphology and size on roll compaction of Ti-based powders. Powder Technol. 2014, 264, 310–319. [Google Scholar] [CrossRef]
- Abreu, C.R.A.; Tavares, F.W.; Castier, M. Influence of particle shape on the packing and on the segregation of spherocylinders via Monte Carlo simulations. Powder Technol. 2003, 134, 167–180. [Google Scholar] [CrossRef]
- Greco, A.; Maffezzoli, A. Powder-shape analysis and sintering behavior of high-density polyethylene powders for rotational molding. J. Appl. Polym. Sci. 2004, 92, 449–460. [Google Scholar] [CrossRef]
- Schlechtriemen, N.; Knitter, R.; Haulelt, J.; Binder, J.R. Impact of powder morphology on quality of low-pressure injection moulded reaction-bonded net shape oxide ceramics. J. Eur. Ceram. Soc. 2013, 33, 709–715. [Google Scholar] [CrossRef]
- Lampman, S. Compressibility and Compactibility of metal powders. In ASM Handbook, Powder Metal Technologies and Applications, 10th ed.; ASM International, 9639 Kinsman Road Materials Park: Novelty, OH, USA, 1998; Volume 7, pp. 704–716. [Google Scholar] [CrossRef]
- Nikolakakis, I.; Pilpel, N. Effects of particle shape and size on the tensile strengths of powders. Powder Technol. 1988, 56, 95–103. [Google Scholar] [CrossRef]
- Hatim, Z.; Michrafy, A.; Elassfouri, M.; Abida, F. Stoichiometry and particle morphology effects on the aptitude to compaction of apatitic structure powders. Powder Technol. 2009, 190, 210–214. [Google Scholar] [CrossRef]
- Carter, R.M.; Yan, Y. Measurement of particle shape using digital imaging techniques. J. Phys. Conf. Ser. 2005, 15, 177–182. [Google Scholar] [CrossRef]
- Pulit, J.; Banach, M.; Kowalski, Z. Does appearance matter? Impact of particle shape on nanosilver characteristics. Chemik 2011, 65, 445–456. [Google Scholar]
- Herman, H. Powders for Thermal Spray Technology. KONA Powder Part. J. 1991, 9, 187–199. [Google Scholar] [CrossRef]
- Hinkley, J.; Waters, A.G.; O’Dea, D.; Litster, J.D. Voidage of ferrous sinter beds: New measurement technique and dependence on feed characteristics. Int. J. Miner. Process. 1994, 41, 53–69. [Google Scholar] [CrossRef]
- Klar, E.; Samal, P.K. Powder Metallurgy Stainless Steels: Processing, Microstructures, and Properties; ASM International: Materials Park, OH, USA, 2007; pp. 23–38. [Google Scholar]
- Miyake, K.; Hirata, Y.; Shimonosono, T.; Sameshima, S. The Effect of Particle Shape on Sintering Behavior and Compressive Strength of Porous Alumina. Materials 2018, 11, 1137. [Google Scholar] [CrossRef]
- Alves, T.E.P.; Kolodziej, C.; Burda, C.; Franco, A. Effect of particle shape and size on the morphology and optical properties of zinc oxide synthesized by the polyol method. Mater. Des. 2018, 146, 125–133. [Google Scholar] [CrossRef]
- Kumar, N.; Bhardwaj, N.K.; Chakrabarti, S.K. Influence of Particle Size Distribution of Calcium Carbonate Pigments on Coated Paper Whiteness. J. Coat. Technol. Res. 2011, 8, 613–618. [Google Scholar] [CrossRef]
- Nguyen, A.V.; Schulze, H.J. Colloidal Science of Flotation; Marcel Dekker: New York, NY, USA, 2004. [Google Scholar]
- Kashiwaya, K.; Noumachi, T.; Hiroyoshi, N.; Ito, M.; Tsunekawa, M. Effect of particle shape on hydrocyclone classification. Powder Technol. 2012, 226, 147–156. [Google Scholar] [CrossRef]
- Wills, B.A.; Napier-Munn, T. Wills’ Mineral Processing Technology, 7th ed.; Butterworth-Heinemann: Oxford, UK, 2006. [Google Scholar]
- Pourghahramani, P.; Forssberg, F. Review of Applied Particle Shape Descriptors and Produced Particle Shapes in Grinding Environments. Part II: The Influence of communition on the Particle Shape. Miner. Process. Extr. Metall. Rev. 2005, 26, 167–186. [Google Scholar] [CrossRef]
- Meng, M.; Xiao, Y.; Duan, X.; Sun, Z.; Du, L.; Fan, H.; Liu, H. Crushing strength of artificial single-particle considering the effect of particle morphology. Acta Geotech. 2022, 17, 3909–3926. [Google Scholar] [CrossRef]
- Xiao, Y.; Jiang, Y.; Tan, P.; Kong, K.; Ali, J.; Mustafina, R.; Zhu, H.; Cai, D. Investigating Morphology and Breakage Evolution Characteristics of Railroad Ballasts over Distinct Supports Subjected to Impact Loading. Materials 2022, 15, 6295. [Google Scholar] [CrossRef] [PubMed]
- Krawczykowski, D. Application of a vision systems for assessment of particle size and shape for mineral crushing products. IOP Conf. Ser. Mater. Sci. Eng. 2018, 427, 1–5. [Google Scholar] [CrossRef]
- Yan, W.M. Fabric evolution in a numerical direct shear test. Comput. Geotech. 2009, 36, 597–603. [Google Scholar] [CrossRef]
- Zhu, F.; Zhao, J. Interplays between particle shape and particle breakage in confined continuous crushing of granular media. Powder Technol. 2021, 378, 455–467. [Google Scholar] [CrossRef]
- Nakayima, Y.N.; Whiten, W.J.; White, M.R. Method for measurement of particle shape distribution by sieves. Trans. Inst. Min. Met. Sec. C 1978, 87, C194–C203. [Google Scholar]
- Beddow, J.K. Particulate Science and Technology; Chemical Publishing, Co. Inc: New York, NY, USA, 1980. [Google Scholar]
- Krumbein, W.C. Settling velocity of flume behavior of nonspherical particles. Trans. Am. Geophys. Union 1942, 41, 621–633. [Google Scholar] [CrossRef]
- Jonasz, M. Nonsphericity of suspended marine particles and its influence on light scattering. Limnol. Oceanogr. 1987, 32, 1059–1065. [Google Scholar] [CrossRef]
- Zhu, G.; Liow, J.-L. Experimental study of particle separation and the fishhook effect in a mini-hydrocyclone. Chem. Eng. Sci. 2014, 111, 94–105. [Google Scholar] [CrossRef]
- Abdollahzadeh, L.; Habibian, M.; Etezazian, R.; Naseri, S. Study of particle’s shape factor, inlet velocity and feed concentration on mini-hydrocyclone classification and fishhook effect. Powder Technol. 2015, 283, 294–301. [Google Scholar] [CrossRef]
- Niazi, S.; Habibian, M.; Rahimi, M. A Comparative Study on the Separation of Different-Shape Particles Using a Mini-Hydrocyclone. Chem. Eng. Technol. 2017, 40, 1–11. [Google Scholar] [CrossRef]
- Pita, F.; Castilho, A. Influence of shape and size of the particles on jigging separation of plastics mixture. Waste Manag. 2016, 48, 89–94. [Google Scholar] [CrossRef] [PubMed]
- Pita, F.; Castilho, A. Separation of plastics by froth flotation. The role of size, shape and density of the particles. Waste Manag. 2017, 60, 91–99. [Google Scholar] [CrossRef] [PubMed]
- Richard, G.; Touhami, S.; Zeghloul, T.; Dascalescu, L. Optimization of metals and plastics recovery from electric cable wastes using a plate-type electrostatic separator. Waste Manag. 2017, 60, 112–122. [Google Scholar] [CrossRef]
- Phengsaart, T.; Ito, M.; Hamaya, N.; Tabelin, C.B.; Hiroyoshi, N. Improvement of jig efficiency by shape separation, and a novel method to estimate the separation efficiency of metal wires in crushed electronic wastes using bending behavior and “entanglement factor”. Miner. Eng. 2018, 129, 54–62. [Google Scholar] [CrossRef]
- Tripathy, S.K.; Suresh, N. Influence of particle size on dry high-intensity magnetic separation of paramagnetic mineral. Adv. Powder Technol. 2017, 28, 1092–1102. [Google Scholar] [CrossRef]
- Zong, Q.X.; Fu, L.Z.; Bo, L. Variables and Applications on Dry Magnetic Separator. E3S Web Conf. 2018, 53, 02019. [Google Scholar] [CrossRef]
- Lileg, K.; Schnizer, B. Influence of particle shape on forces in magnetic separators. IEEE Trans. Magn. 1989, 25, 4292–4297. [Google Scholar] [CrossRef]
- Galipeau, E.; Castañeda, P.P. The effect of particle shape and distribution on the macroscopic behavior of magnetoelastic composites. Int. J. Solids Struct. 2012, 49, 1–17. [Google Scholar] [CrossRef]
- Gómez-Ramírez, A.; López-López, M.T.; Durán, J.D.G.; González-Caballero, F. Influence of particle shape on the magnetic and magnetorheological properties of nanoparticle suspensions. Soft Matter 2009, 5, 3888–3895. [Google Scholar] [CrossRef]
- Haldar, S.K. Mineral Processing. In Mineral Exploration: Principles and Applications, 2nd ed.; Elsevier: Amsterdam, The Netherlands, 2018; Chapter 13; p. 378. [Google Scholar]
- Zhang, S.; Forssberg, E. Optimization of electrodynamic separation for metals recovery from electronic scrap. Resour. Conserv. Recycl. 1998, 22, 143–162. [Google Scholar] [CrossRef]
- Lee, H.-Y.; Oh, J.-K. A study on the shredding of end-of-life vehicles and materials separation. Geochem. Eng. 2003, 6, 100–105. [Google Scholar] [CrossRef]
- Veit, H.M.; Diehl, T.R.; Salami, A.P.; Rodrigues, J.S.; Bernardes, A.M.; Teno’rio, J.A.S. Utilization of magnetic and electrostatic separation in the recycling of printed circuit boards scrap. Waste Manag. 2005, 25, 67–74. [Google Scholar] [CrossRef]
- Wu, J.; Li, J.; Xu, Z. Electrostatic separation for recovering metals and nonmetals from waste printed circuit board: Problems and improvements. Environ. Sci. Technol. 2008, 42, 5272–5276. [Google Scholar] [CrossRef] [PubMed]
- Tilmatine, A.; Medles, K.; Bendimerad, S.-E.; Boukholda, F.; Dascalescu, L. Electrostatic separators of particles: Application to plastic/metal, metal/metal and plastic/plastic mixtures. Waste Manag. 2009, 29, 228–232. [Google Scholar] [CrossRef]
- Tripathy, S.K.; Ramamurthy, Y.; Kumar, C.R. Modeling of high-tension roll separator for separation of titanium bearing minerals. Powder Technol. 2010, 201, 181–186. [Google Scholar] [CrossRef]
- Lu, H.; Jia, L.; Jie, G.; Zhenming, X. Movement behavior in electrostatic separation: Recycling of metal materials from waste printed circuit board. J. Mater. Process. Technol. 2008, 197, 101–108. [Google Scholar] [CrossRef]
- Li, J.; Lu, H.; Xu, Z.; Zhou, Y. A model for computing the trajectories of the conducting particles from waste printed circuit boards in corona electrostatic separators. J. Hazard. Mater. 2008, 151, 52–57. [Google Scholar] [CrossRef]
- Li, J.; Xu, Z.; Zhou, Y. Theoretic model and computer simulation of separating mixture metal particles from waste printed circuit board by electrostatic separator. J. Hazard. Mater. 2008, 153, 1308–1313. [Google Scholar] [CrossRef] [PubMed]
- Wu, J.; Li, J.; Xu, Z. An improved model for computing the trajectories of conductive particles in roll-type electrostatic separator for recycling metals from WEEE. J. Hazard. Mater. 2009, 167, 489–493. [Google Scholar] [CrossRef] [PubMed]
- Guven, O.; Celik, M.S. Interplay of particle shape and surface roughness to reach maximum flotation efficiencies depending on collector concentration. Miner. Process. Extr. Metall. Rev. 2016, 37, 412–417. [Google Scholar] [CrossRef]
- Richards, R.L.; Locke, S.B. Textbook of Ore Dressing, 3rd ed.; McGraw-Hill: New York, NY, USA, 1940. [Google Scholar]
- Sivamohan, R. A study of gravity concentration with emphasis on surface phenomena. Ph.D. Thesis, Lulea University of Technology, Lulea, Sweden, 1985. [Google Scholar]
- Brits, B.R. Effect of particle size in gravity separation processes at Palabora, South Africa. In Proceedings of African Mining ’91; Springer: Dordrecht, The Netherlands, 1991; pp. 159–167. [Google Scholar] [CrossRef]
- Aplan, F.F. Gravity concentration. In Principles of Mineral Processing; Fuerstenau, M.C., Kenneth, N.H., Eds.; SME: Littleton, CO, USA, 2003; Chapter 6; pp. 185–219. [Google Scholar]
- Pryor, E.J. Mineral Processing, 3rd ed.; Elsevier: London, UK, 1965. [Google Scholar]
- Ofori-Sarpong, G.; Amankwah, R.K. Comminution environment and gold particle morphology: Effects on gravity concentration. Miner. Eng. 2011, 24, 590–592. [Google Scholar] [CrossRef]
- Kademli, M.; Gulsoy, O.Y. The role of particle size and solid contents of feed on mica-feldspar separation in gravity concentration. Physicochem. Probl. Miner. Process. 2012, 48, 645–654. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhang, Y.; Bao, S.; Liu, T.; Bian, Y.; Liu, X.; Jiang, M. Separation factor of shaking table for vanadium pre-concentration from stone coal. Sep. Purif. Technol. 2013, 115, 92–99. [Google Scholar] [CrossRef]
- Brożek, M.; Surowiak, A. Effect of particle shape on jig separation efficiency. Physicochem. Probl. Miner. Process. 2007, 41, 397–413. [Google Scholar]
- Langen, M.; Bender, M.; Christiani, J.; Hoberg, H.; Eickhoff, A.; Gödecker, D.; Koch, U.; Zimmermann, M. KAKTUS: A new plant design for processing of plastic packaging waste—Experiences of pilot scale operation. In Proceedings of the XX International Mineral Processing Congress, Aachen, Germany, 21—26 September 1997. [Google Scholar]
- Ferrara, G.; Bevilacqua, P.; Lorenzi, L.D.; Zanin, M. The influence of particle shape on the dynamic dense medium separation of plastics. Int. J. Mineral Process. 2000, 59, 225–235. [Google Scholar] [CrossRef]
- Klimpel, R.R. Introduction to Solid-Solid Separation of Fine Particles by Froth Flotation; NSF Engineering Resource Center for Particle Science & Technology, University of Florida: Gainesville, FL, USA, 1998. [Google Scholar]
- Revuelta, M.B. Mineral Resources: From Exploration to Sustainability Assessment; Springer Textbooks: Cham, Switzerland, 2018; pp. 19–38. [Google Scholar] [CrossRef]
- Harris, C.C. Flotation Machines. In Flotation; Fuerstenau, M.C., Ed.; AIME: New York, NY, USA, 1975; Chapter 27; pp. 753–815. [Google Scholar]
- Laurila, H.; Karesvuori, J.; Tiili, O. Strategies for instrumentation and control of flotation circuits. In Mineral Processing Plant Design, Practise and Control; SME: Chicago, IL, USA, 2002; pp. 2174–2195. [Google Scholar]
- Pattanaik, A.; Venugopal, R. Investigation of adsorption mechanism of reagents (surfactants) system and its applicability in iron ore flotation-An overview. Colloids Interface Sci. Commun. 2018, 25, 41–65. [Google Scholar] [CrossRef]
- Bhambhani, T. The Effect of Particle Size and Shape on Transport through Confined Channels in three-phase Froths. Ph.D. Thesis, Columbia University, New York, NY, USA, 2019. [Google Scholar]
- Huh, C.; Mason, S.G. The flotation of axisymmetric particles at horizontal liquid interfaces. J. Colloid Interface Sci. 1974, 2, 271–289. [Google Scholar] [CrossRef]
- Chau, T.T.; Bruckard, W.J.; Koh, P.T.L.; Nguyen, A.V. A review of factors that affect contact angle and implications for flotation practice. Adv. Colloid Interface Sci. 2009, 150, 106–115. [Google Scholar] [CrossRef] [PubMed]
- Koh, P.T.L.; Hao, F.P.; Smith, L.K.; Chau, T.T.; Bruckard, W.J. The effect of particle shape and hydrophobicity in flotation. Int. J. Miner. Process. 2009, 93, 128–134. [Google Scholar] [CrossRef]
- Vizcarra, T.G.; Harmer, S.L.; Wightman, E.M.; Johnson, N.W.; Manlapig, E.V. The influence of particle shape properties and associated surface chemistry on the flotation kinetics of chalcopyrite. Miner. Eng. 2011, 24, 807–816. [Google Scholar] [CrossRef]
- Verrelli, D.I.; Bruckard, W.J.; Koh, P.T.L.; Schwarz, M.P.; Follink, B. Particle shape effects in flotation. Part 1: Microscale experimental observations. Miner. Eng. 2014, 58, 80–89. [Google Scholar] [CrossRef]
- Ma, G.; Xia, W.; Xie, G. Effect of particle shape on the flotation kinetics of fine coking coal. J. Clean. Prod. 2018, 195, 470–475. [Google Scholar] [CrossRef]
- Wen, B.; Xia, W. Effect of particle shape on coal flotation. Energy Source Part A 2017, 1339, 1390–1394. [Google Scholar] [CrossRef]
- Li, C.; Gao, Z. Effect of grinding media on the surface property and flotation behavior of scheelite particles. Powder Technol. 2017, 322, 386–392. [Google Scholar] [CrossRef]
- Li, C.; Gao, Z. Tune surface physicochemical property of fluorite particles by regulating the exposure degree of crystal surfaces. Miner. Eng. 2018, 128, 123–132. [Google Scholar] [CrossRef]
- Gao, Z.; Sun, W.; Hu, Y. New insights into the dodecylamine adsorption on scheelite and calcite: An adsorption model. Miner. Eng. 2015, 79, 54–61. [Google Scholar] [CrossRef]
- Zhu, G.; Wang, Y.; Liu, X.; Yu, F.; Lu, D. The cleavage and surface properties of wet and dry ground spodumene and their flotation behavior. Appl. Surf. Sci. 2015, 357, 333–339. [Google Scholar] [CrossRef]
- Gao, Z.; Xie, L.; Cui, X.; Hu, Y.; Sun, W.; Zeng, H. Probing anisotropic surface properties and surface forces of fluorite crystals. Langmuir 2018, 34, 2511–2521. [Google Scholar] [CrossRef] [PubMed]
- Gao, Z.; Fan, R.; Ralston, J.; Sun, W.; Hu, Y. Surface broken bonds: An efficient way to assess the surface behaviour of fluorite. Miner. Eng. 2019, 130, 15–23. [Google Scholar] [CrossRef]
- Ally, J.; Kappl, M.; Butt, H.-J. Adhesion of Particles with Sharp Edges to Air–Liquid Interfaces. Langmuir 2012, 28, 11042–11047. [Google Scholar] [CrossRef]
- Ulusoy, U.; Hiçyılmaz, C.; Yekeler, M. Role of shape properties of calcite and barite particles on apparent hydrophobicity. Chem. Eng. Process. 2004, 843, 1047–1053. [Google Scholar] [CrossRef]
- Verrelli, D.I.; Koh, P.T.L.; Nguyen, A.V. Particle-bubble interaction and attachment in flotation. Chem. Eng. Sci. 2011, 2366, 5910–5921. [Google Scholar] [CrossRef]
- Wotruba, H.; Hoberg, H.; Schneider, F.U. Investigation on the separation of microlithe and zircon. The influence of particle shape on floatability. In Proceedings of XVII International Mineral Processing Congress; Schubert, H., Ed.; Bergakademie Freiberg: Dresden, Germany, 1991; Volume 4, p. 83. [Google Scholar]
- Vaziri Hassas, B.; Caliskan, H.; Guven, O.; Karakas, F.; Cinar, M.; Celik, M.S. Effect of roughness and shape factor on flotation characteristics of glass beads. Colloids Surf. A 2016, 492, 88–99. [Google Scholar] [CrossRef]
- Wang, L.; Peng, Y.; Runge, K. Entrainment in froth flotation: The degree of entrainment and its contributing factors. Powder Technol. 2016, 288, 202–211. [Google Scholar] [CrossRef]
- Yu, X.; Ma, L.; Cao, M.; Liu, Q. Slime coatings in froth flotation: A review. Miner. Eng. 2017, 114, 26–36. [Google Scholar] [CrossRef]
- Smith, P.G.; Warren, L.J. Entrainment of particles into flotation froths. Miner. Process. Extr. Metall. Rev. 1989, 5, 123–145. [Google Scholar] [CrossRef]
- Wang, L.; Peng, Y.; Runge, K.; Bradshaw, D. A review of entrainment: Mechanisms, contributing factors and modelling in flotation. Miner. Eng. 2015, 70, 77–91. [Google Scholar] [CrossRef]
- Ata, S.; Ahmed, N. Particle shape effects on entrainment in flotation. In Proceedings of the 26th Australasian Chemical Engineering Conf. (Chemeca ’98), 28–30 September 1998; Institute of Engineers: Port Douglas, QLD, Australia, 1998. [Google Scholar]
- Wiese, J.; Becker, M.; Yorath, G.; O’connor, C. An investigation into the relationship between particle shape and entrainment. Miner. Eng. 2015, 83, 211–216. [Google Scholar] [CrossRef]
- Yang, B.; Yin, W.; Zhu, Z.; Wang, D.; Han, H.; Fu, Y.; Sun, H.; Chu, F.; Yao, J. A new model for the degree of entrainment in froth flotation based on mineral particle characteristics. Powder Technol. 2019, 354, 358–368. [Google Scholar] [CrossRef]
- Xia, W. Role of particle shape in the floatability of mineral particle: An overview of recent advances. Powder Technol. 2017, 317, 104–116. [Google Scholar] [CrossRef]
- Wiese, J.G.; O’Connor, C.T. An investigation into the relative role of particle size, particle shape and froth behaviour on the entrainment of chromite. Int. J. Miner. Process. 2016, 156, 127–133. [Google Scholar] [CrossRef]
- U.S. Geological Survey. Mineral Commodity Summaries; U.S. Geological Survey: Reston, VA, USA, 2021. [CrossRef]
- Severinghaus, N., Jr. Fillers, filters, and absorbents. In Industrial Minerals and Rocks, 4th ed.; Stanley, J., Ed.; AIME: New York, NY, USA, 1975; pp. 235–249. [Google Scholar]
- Guillet, G.R.; Kriens, J. Ontario and the Mineral Filler Industry; Industrial Mineral Background Paper 5 (IMBP 5); Ontario Ministry of Natural Resources, Mineral Resources Branch: Toronto, ON, Canada, 1984; pp. 1–175. Available online: http://www.geologyontario.mndmf.gov.on.ca/mndmfiles/pub/data/imaging/IMBP005/IMBP005.pdf (accessed on 23 November 2022).
- Hubbe, M.; Gill, R.A. Fillers for Papermaking: A Review of their Properties, Usage Practices, and their Mechanistic Role. Bioresources 2016, 11, 2886–2963. Available online: https://bioresources.cnr.ncsu.edu/BioRes_11/BioRes_11_1_2886_Review_Hubbe_Gill_Fillers_Papermaking_Props_Usage_Prac_Mechanistic_Role_8676.pdf (accessed on 23 November 2022). [CrossRef]
- Purcell, R.J.; Parker, D.C. Purified Attapulgite Clay. U.S. Patent 6,444,601, 3 September 2002. [Google Scholar]
- Vanderbilt Company Inc. Non-Black Fillers for Rubber. 2022. Available online: https://www.vanderbiltworldwide.com/public/assets/uploads/documents/technical/Non-Black_Fillers_Web.pdf (accessed on 23 November 2022).
- Roshanaei, H.; Khodkar, F.; Alimardani, M. Contribution of filler–filler interaction and filler aspect ratio in rubber reinforcement by silica and mica. Iran. Polym. J. 2020, 29, 901–909. [Google Scholar] [CrossRef]
- Geologyscience. 2018. Available online: https://geologyscience.com/minerals/calcite (accessed on 23 November 2022).
- Cilas. Particle Size and Shape Analysis in Paint, Ink and Pigment Industry. 2022. Available online: http://www.bruben.com.ar/pdf/Aplicaciones/020-pigmentos%20paint%20industry.pdf (accessed on 23 November 2022).
- Holt, C.B. The shape of particles produced by comminution. A review. Powder Technol. 1981, 28, 59–63. [Google Scholar] [CrossRef]
- Husband, J.C.; Preston, J.S.; Gate, L.F.; Storert, A.; Creaton, P. The influence of pigment particle shape on the in-plane tensile strength properties of kaolin-based coating layers. Tappi J. 2006, 5, 3–8. Available online: https://imisrise.tappi.org//download.aspx?key=06DEC03 (accessed on 23 November 2022).
- Hsieh, M.Y.; Guo, Y.L.; Shiao, J.S.C.; Sheu, H.M. Morphology of glass fibers in electronics workers with fiberglass dermatitis—A scanning electron microscopy study. Int. J. Dermatol. 2001, 40, 258–261. [Google Scholar] [CrossRef]
- Lohmander, S. Aspect ratios of pigment particles determined by different methods. Nordic Pulp Pap. Res. J. 2000, 15, 221–230. [Google Scholar] [CrossRef]
- Koleske, J.V. Extender pigments. In Paint and Coating Testing Manual, 14th ed.; Of the Gardner-Sward Handbook, Chapter 24; ASTM Manual Series: MNL 17; American Society for Testing and Materials: Philadelphia, PA, USA, 1995; p. 219. [Google Scholar]
- Gill, R.; Scott, W. The Relative Effects of Different Calcium Carbonate Filler Pigments on Optical Properties. Tappi J. 1987, 70, 93–99. [Google Scholar]
- Gill, R. The Behavior of On-Site Synthesized Precipitated Calcium Carbonates and Other Calcium Carbonate Fillers on Paper Properties. Nordic Pulp Pap. Res. J. 1989, 4, 120–127. [Google Scholar] [CrossRef]
- Weigl, J.; Ritter, E. Importance of Particle Size, Shape, and Distribution of Fillers in Supercalendered Paper Manufacture. Wochenbl. Papierfabr. 1995, 123, 739–747. [Google Scholar]
- Bown, R. Physical and Chemical Aspects of the Use of Fillers in Paper. In Paper Chemistry, 2nd ed.; Roberts, J.C., Ed.; Springer: Dordrecht, The Netherlands, 1996; Chapter 11; pp. 194–230. [Google Scholar] [CrossRef]
- Bown, R. Particle Size, Shape, and Structure of Paper Fillers and Their Effect on Paper Properties. Pap. Technol. 1998, 39, 44–48. [Google Scholar]
- Hubbe, M.A. Filler Particle Shape vs. Paper Properties—A Review. In Proceedings of the TAPPI 2004 Spring Tech. Conf., Atlanta, GA, USA, 2–5 May 2004; pp. 3–7. [Google Scholar]
- Armand, M.; Tarascon, J.-M. Building better batteries. Nature 2008, 451, 652–657. [Google Scholar] [CrossRef]
- Yoshio, M.; Brodd, R.J.; Kozawa, A. Lithium-ion Batteries: Science and Technologies; Springer: New York, NY, USA, 2009. [Google Scholar] [CrossRef]
- Tarascon, J.-M. Is lithium the new gold? Nat. Chem. 2010, 2, 510. [Google Scholar] [CrossRef]
- Zheng, H.; Kim, M.-S. Performance of modified graphite as anode material for lithium-ion secondary battery. Carbon Lett. 2011, 12, 243–248. [Google Scholar] [CrossRef]
- Dai, K.; Wang, Z.; Ai, G.; Zhao, H.; Yuan, W.; Song, X.; Battaglia, V.; Sun, C.; Wu, K.; Liu, G. The transformation of graphite electrode materials in lithium-ion batteries after cycling. J. Power Sources 2015, 298, 349–354. [Google Scholar] [CrossRef]
- Chung, G.C.; Kim, H.J.; Yu, S.I.; Jun, S.H.; Choi, J.W.; Kim, M.H. Origin of graphite exfoliation an investigation of the important role of solvent cointercalation. J. Electrochem. Soc. 2000, 147, 4391–4398. [Google Scholar] [CrossRef]
- Endo, M.; Kim, C.; Nishimura, K.; Fujino, T.; Miyashita, K. Recent development of carbon materials for Li ion batteries. Carbon 2000, 38, 183–197. [Google Scholar] [CrossRef]
- Shim, J.; Striebel, K.A. Cycling performance of low-cost lithium ion batteries with natural graphite and LiFePO4. J. Power Sources 2003, 121, 955–958. [Google Scholar] [CrossRef]
- Buchmann, I. Lithium-Ion Batteries: Fundamentals and Safety, Encyclopedia of Inorganic and Bioinorganic Chemistry; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2015. [Google Scholar] [CrossRef]
- Bhutada, G. Electrification, The Key Minerals in an EV Battery, 2 May 2022. Available online: https://elements.visualcapitalist.com/the-key-minerals-in-an-ev-battery/ (accessed on 23 November 2022).
- Van Zyl, W. Analysis of Graphite for Lithium Ion Batteries, Thermo Fisher Scientific. 2021. Available online: https://www.thermofisher.com/blog/materials/analysis-of-graphite-for-lithium-ion-batteries/ (accessed on 23 November 2022).
- Qiu, X. Investigating the Particle Size and Shape Influences on Anode Energy Density of Lithium-Ion Batteries, Application Notes; Bettersize Instruments Ltd.: Liaoning, China, 2022; Available online: https://www.bettersizeinstruments.com/uploads/file/investigating-the-particle-size-and-shape-influences-on-anode-energy-density-of-lithium-ion-batteries.pdf (accessed on 23 November 2022).
- Oetzel, C. Use of acoustic methods and current flow measurement in battery research. In 3P Instruments Particle World, 22nd ed.; Klank, D., Ed.; 3P Instruments GmbH & Co. KG: Odelzhausen, Germany, 2021; pp. 9–14. [Google Scholar]
- Teng, D.; Li, P.; Yuan, N.; Lyu, J.; Chen, J.; Lin, L.; Chen, H. Process parameters optimization of natural graphite spheroidization. China Powder Sci. Technol. 2021, 27, 4. [Google Scholar]
- Zhang, H.L.; Li, F.; Liu, C.; Tan, J.; Cheng, H.M. New Insight into Solid Electrolyte Interphase with Use of a Focused Ion Beam. J. Phys. Chem. B 2005, 109, 22205–22211. [Google Scholar] [CrossRef]
- Wang, H.; Yoshio, M. Carbon-coated natural graphite prepared by thermal vapor decomposition process, a candidate anode material for lithium-ion battery. J. Power Sources 2001, 93, 123–129. [Google Scholar] [CrossRef]
- Guo, K.K.; Pan, Q.M.; Fan, S.B. Poly(acrylonitrile) encapsulated graphite as anode materials for lithium ion batteries. J. Power Sources 2002, 111, 350–356. [Google Scholar] [CrossRef]
- Hongyu, W.; Kenji, F.; Masaki, Y.; Takeshi, A.; Zempachi, O. Measurement of carbon amount in carbon-coated graphite by thermal analysis. Chem. Lett. 2002, 31, 238. [Google Scholar] [CrossRef]
- Pan, Q.M.; Guo, K.K.; Wang, L.Z.; Fang, S. Ionic conductive copoly- mer encapsulated graphite as an anode material for lithium ion batteries. Solid State Ion. 2002, 149, 193–200. [Google Scholar] [CrossRef]
- Wu, Y.P.; Jiang, C.; Wan, C.; Holze, R. Anode materials for lithium ion batteries by oxidative treatment of common natural graphite. Solid State Ion. 2003, 156, 283–290. [Google Scholar] [CrossRef]
- Guoping, W.; Bolan, Z.; Min, Y.; Xiaoluo, X.; Meizheng, Q.; Zuolong, Y. A modified graphite anode with high initial efficiency and excellent cycle life expectation. Solid State Ion. 2005, 176, 905–909. [Google Scholar] [CrossRef]
- Yoshio, M.; Wang, H.Y.; Fukuda, K. Spherical carbon-coated natural graphite as a lithium-ion battery anode material. Angew. Chem. 2003, 42, 4203–4206. [Google Scholar] [CrossRef]
- Yoshio, M.; Wang, H.; Fukuda, K.; Umeno, T.; Abe, T.; Ogumi, Z. Improvement of natural graphite as a lithium-ion battery anode material, from raw flake to carbon-coated sphere. J. Mater. Chem. 2004, 14, 1754–1758. [Google Scholar] [CrossRef]
- Zaghib, K.; Song, X.; Guerfi, A.; Kostecki, R.; Kinoshita, K. Effect of particle morphology on lithium intercalation rates in natural graphite. J. Power Sources 2003, 124, 505–512. [Google Scholar] [CrossRef]
- Susan-Resiga, D.; Barvinschi, P. Nano-microstructured Magnetorheological Fluids and Engineering Applications. The Romanian Journal of Technical Sciences. Appl. Mech. 2020, 65, 89–122. [Google Scholar]
- Rankin, P.J.; Ginder, J.M.; Klingenberg, D.J. Electro- and magnetorheology. Curr. Opin. Colloid Interface Sci. 1998, 3, 373–381. [Google Scholar] [CrossRef]
- Klingenberg, D.J. Magnetorheology: Applications and challenges. AIChE J. 2001, 47, 246–249. [Google Scholar] [CrossRef]
- Bossis, G.; Volkova, O.; Lacis, S.; Meunier, A. Magnetorheology: Fluids, structures and rheology. In Ferrofluids. Lecture Notes in Physics; Odenbach, S., Ed.; Springer-Verlag: Berlin, Germany, 2002; Volume 594, pp. 202–230. [Google Scholar] [CrossRef]
- Vekas, L. Ferrofluids and magnetorheological fluids. Adv. Sci. Technol. 2008, 54, 127–136. [Google Scholar] [CrossRef]
- De Vicente, J.; Klingenberg, D.J.; Hidalgo-Alvarez, R. Magnetorheological fluids: A review. Soft Matter 2011, 7, 3701–3710. [Google Scholar] [CrossRef]
- Rabinow, J. Magnetic Fluid Torque and Force Transmitting Device. U.S. Patent 2575360A, 20 November 1951. [Google Scholar]
- Carlson, J.D.; Jolly, M.R. MR Fluid, foam and elastomer devices. Mechatronics 2000, 10, 555–569. [Google Scholar] [CrossRef]
- Lita, M.; Popa, C.N.; Velescu, C.; Vekas, L. Investigations of a Magnetorheological Fluid Damper. IEEE Trans. Magn. 2004, 40, 469–472. [Google Scholar] [CrossRef]
- Park, B.J.; Fang, F.F.; Choi, H.J. Magnetorheology: Materials and application. Soft Matter 2010, 6, 5246–5253. [Google Scholar] [CrossRef]
- Friedman, A.J.; Dyke, S.J. Development and Experimental Validation of a New Control Strategy Considering Device Dynamics for Large Scale MR Dampers Using Real Time Hybrid Simulation; Report Intelligent Infrastructure Systems Laboratory-003; Digital Environment for Enabling Data-Driven Science (DEEDS), National Science Foundation: Alexandria, VA, USA, 2013. Available online: https://datacenterhub.org/resources/7022 (accessed on 26 December 2022).
- Portillo, M.A.; Lozada, P.S.A.; Figueroa, I.A.; Suarez, M.A.; Delgado, A.V.C.; Iglesias, G.R. Synergy between magnetorheological fluids and aluminum foams: Prospective alternative for seismic damping. J. Intell. Mater. Syst. Struct. 2016, 27, 872–879. [Google Scholar] [CrossRef]
- Ahamed, R.; Choi, S.B.; Ferdaus, M.M. A state of art on magnetorheological materials and their potential applications. J. Intell. Mater. Syst. Struct. 2018, 29, 2051–2095. [Google Scholar] [CrossRef]
- Oh, J.-S.; Shul, C.W.; Kim, T.-H.; Lee, T.-H.; Son, S.-W.; Choi, S.-B. Dynamic Analysis of Sphere-Like Iron Particles Based Magnetorheological Damper for Waveform-Generating Test System. Int. J. Mol. Sci. 2020, 21, 1149. [Google Scholar] [CrossRef] [PubMed]
- Read, D.H.; Martin, J.E. Field-Structured Chemiresistors. Adv. Funct. Mater. 2010, 20, 1577–1584. [Google Scholar] [CrossRef]
- Carlson, J.D.; Sproston, J.L. Controllable Fluids in 2000—Status of ER and MR Fluid Technology. In Proceedings of the Actuator 2000-7th Int. Conf on New Actuators, 19–21 June 2000; Messe Bremen: Bremen, Germany, 2000; pp. 126–130. [Google Scholar]
- Liu, J.; Flores, G.A.; Sheng, R. In-vitro investigation of blood embolization in cancer treatment using magnetorheological fluids. J. Magn. Magn. Mater. 2001, 225, 209–217. [Google Scholar] [CrossRef]
- Kordonski, W.I.; Golini, D. Fundamentals of Magnetorheological Fluid Utilization in High Precision Finishing. In Proceedings of the 7th International Conference on Electro-rheological Fluids and Magneto-rheological Suspensions, Honolulu, HI, USA, 19–23 July 1999; World Scientific: Singapore, 2000; pp. 682–692. [Google Scholar] [CrossRef]
- Jha, S.; Jain, V.K. Design and development of the magnetorheological abrasive flow finishing (MRAFF) process. Int. J. Mach. Tool. Manuf. 2004, 44, 1019–1029. [Google Scholar] [CrossRef]
- Kordonski, W.I.; Shorey, A.B.; Tricard, M. Magnetorheological Jet (MR JetTMJetTM) Finishing Technology. J. Fluids Eng. 2006, 128, 20–26. [Google Scholar] [CrossRef]
- Donado, F.; Carrillo, J.L.; Mendoza, M.E. Sound propagation in magneto-rheological suspensions. J. Condens. Matter Phys. 2002, 14, 2153–2157. [Google Scholar] [CrossRef]
- Reinecke, B.N.; Shan, J.W.; Suabedissen, K.K.; Cherkasova, A.S. On the anisotropic thermal conductivity of magnetorheological suspensions. J. Appl. Phys. 2008, 104, 023507. [Google Scholar] [CrossRef]
- Yıldırım, G.; Genc, S. Experimental study on heat transfer of the magnetorheological fluids. Smart Mater. Struct. 2013, 22, 085001. [Google Scholar] [CrossRef]
- Taketomi, S.U.S. Optical apparatus using anomalously strong magneto-birefringence of magnetic. fluid. Patent 4812767, 14 March 1989. [Google Scholar]
- Taketomi, S.; Ozaki, Y.; Kawasaki, K.; Yuasa, S.; Miyajima, H. Transparent magnetic Fluid: Preparation of YIG Ultrafine Particles. J. Magn. Magn. Mater. 1993, 122, 6–9. [Google Scholar] [CrossRef]
- Kanu, R.C.; Shaw, M.T. Studies of ER fluids featuring rodlike particles. Int. J. Mod. Phys. B 1996, 10, 2925–2932. [Google Scholar] [CrossRef]
- Kanu, R.C.; Shaw, M.T. Enhanced electrorheological fluids using anisotropic particles. J. Rheol. 1998, 42, 657–670. [Google Scholar] [CrossRef]
- Satoh, A. Rheological properties and orientational distributions of dilute ferromagnetic spherocylinder particle dispersions. J. Colloid Interface Sci. 2001, 234, 425–433. [Google Scholar] [CrossRef] [PubMed]
- Watanabe, T.; Aoshima, M.; Satoh, A. Rheological properties and particle behaviours of a nondilute colloidal dispersion composed of ferromagnetic spherocylinder particles subjected to a simple shear flow: Analysis by means of a mean-field approximation for the two typical external magnetic field directions. J. Colloid Interface Sci. 2006, 302, 347–355. [Google Scholar] [CrossRef]
- Chin, B.D.; Park, J.H.; Kwon, M.H.; Park, O.O. Rheological properties and dispersion stability of magnetorheological (MR) suspensions. Rheol. Acta 2001, 40, 211–219. [Google Scholar] [CrossRef]
- De Vicente, J.; Gutierrez, J.P.S.; Reyes, E.A.; Vereda, F.; Alvarez, R.H. Dynamic rheology of sphere- and rod-based magnetorheological fluids. J. Chem. Phys. 2009, 131, 194902–194910. [Google Scholar] [CrossRef]
- Webb, P.A.; Orr, C. Analytical Methods in Fine Particle Technology; Chapter 1; Micromeritics Instrument Corp: Norcross, GA, USA, 1997. [Google Scholar]
- Service, R.F. Materials and biology. Nanotechnology takes aim at cancer. Science 2005, 310, 1132–1134. [Google Scholar] [CrossRef]
- Borm, P.J.A.; Robbins, D.; Haubold, S.; Kuhlbush, T.; Fissan, H.; Donaldson, K.; Schins, R.; Stone, V.; Kreyling, W.; Lademann, J.; et al. The potential risks of nanomaterials: A review carried out for ECETOC. Part. Fibre Toxicol. 2006, 3, 11. [Google Scholar] [CrossRef]
- Boyd, R.D.; Cuenat, A. New analysis procedure for fast and reliable size measurement of nanoparticles from atomic force microscopy images. J. Nanopart. Res. 2011, 13, 105–113. [Google Scholar] [CrossRef]
- Stark, W.J.; Stoessel, P.R.; Wohlleben, W.; Hafner, A. Industrial applications of nanoparticles. Chem. Soc. Rev. 2015, 44, 5793–5805. [Google Scholar] [CrossRef] [PubMed]
- Saqib, S.; Faryad, S.; Afridi, M.I.; Arshad, B.; Younas, M.; Naeem, M.; Zaman, W.; Ullah, F.; Nisar, M.; Ali, S.; et al. Bimetallic Assembled Silver Nanoparticles Impregnated in Aspergillus fumigatus Extract Damage the Bacterial Membrane Surface and Release Cellular Contents. Coatings 2022, 12, 1505. [Google Scholar] [CrossRef]
- Saqib, S.; Nazeer, A.; Ali, M.; Zaman, W.; Younas, M.; Shahzad, A.; Sunera, N.M. Catalytic potential of endophytes facilitates synthesis of biometallic zinc oxide nanoparticles for agricultural application. Biometals 2022, 35, 967–985. [Google Scholar] [CrossRef] [PubMed]
- Asghar, M.; Habib, S.; Zaman, W.; Hussain, S.; Ali, H.; Saqib, S. Synthesis and characterization of microbial mediated cadmium oxide nanoparticles. Microsc. Res. Tech. 2020, 83, 1574–1584. [Google Scholar] [CrossRef]
- Jeevanandam, J.; Barhoum, A.; Chan, Y.S.; Dufresne, A.; Danquah, M.K. Review on nanoparticles and nanostructured materials: History, sources, toxicity and regulations. Beilstein J. Nanotechnol. 2018, 9, 1050–1074. [Google Scholar] [CrossRef] [PubMed]
- Vippola, M.; Valkonen, M.; Sarlin, E.; Honkanen, M.; Huttunen, H. Insight to Nanoparticle Size Analysis—Novel and Convenient Image Analysis Method Versus Conventional Techniques. Nanoscale Res. Lett. 2016, 11, 169. [Google Scholar] [CrossRef]
- Lasic, D.D.; Papahadjopoulos, D. Liposomes revisited. Science 1995, 267, 1275–1276. [Google Scholar] [CrossRef] [PubMed]
- Yezhelyev, M.V.; Gao, X.; Xing, Y.; Al-Hajj, A.; Nie, S.; O’Regan, R.M. Emerging use of nanoparticles in diagnosis and treatment of breast cancer. Lancet Oncol. 2006, 7, 657–667. [Google Scholar] [CrossRef] [PubMed]
- Huang, X.; El-Sayed, I.H.; Qian, W.; El-Sayed, M.A. Cancer cell imaging and photothermal therapy in the near-infrared region by using gold nanorods. J. Am. Chem. Soc. 2006, 128, 2115–2120. [Google Scholar] [CrossRef]
- Sardar, R.; Shumaker-Parry, J.S. Asymmetrically functionalized gold nanoparticles organized in one- dimensional chains. Nano Lett. 2008, 8, 731–736. [Google Scholar] [CrossRef]
- Park, J.H.; von Maltzahn, G.; Zhang, L.L.; Schwartz, M.P.; Ruoslahti, E.; Bhatia, S.N.; Sailor, M.J. Magnetic iron oxide nanoworms for tumor targeting and imaging. Adv. Mater. 2008, 20, 1630–1635. [Google Scholar] [CrossRef] [PubMed]
- Park, J.H.; von Maltzahn, G.; Zhang, L.; Derfus, A.M.; Simberg, D.; Harris, T.J.; Ruoslahti, E.; Bhatia, S.N.; Sailor, M.J. Systematic surface engineering of magnetic nanoworms for in vivo tumor targeting. Small 2009, 5, 694–700. [Google Scholar] [CrossRef]
- Toy, R.; Hayden, E.; Shoup, C.; Baskaran, H.; Karathanasis, E. The effects of particle size, density and shape on margination of nanoparticles in microcirculation. Nanotechnology 2011, 22, 115101. [Google Scholar] [CrossRef] [PubMed]
- Tadic, M.; Kralj, S.; Kopanja, L. Synthesis, particle shape characterization, magnetic properties and surface T modification of superparamagnetic iron oxide nanochains. Mater. Character. 2019, 148, 123–133. [Google Scholar] [CrossRef]
- Grau-Crespo, R.; Al-Baitai, A.Y.; Saadoune, I.; De Leeuw, N.H. Vacancy ordering and electronic structure of γ-Fe2O3 (maghemite): A theoretical investigation. J. Phys. Condens. Matter. 2010, 22, 255401. [Google Scholar] [CrossRef]
- Nedelcu, G.G.; Nastro, A.; Filippelli, L.; Cazacu, M.; Iacob, M.; Rossi, C.O.; Popa, A.; Toloman, D.; Dobromir, M.; Iacomi, F. Structural characterization of copolymer embedded magnetic nanoparticles. Appl. Surf. Sci. 2015, 352, 109–116. [Google Scholar] [CrossRef]
- Saravanan, A.; Ramasamy, R.P. Investigation of polymer dynamics in chitosan- maghemite nanocomposites: A potential green superparamagnetic material. J. Polym. Res. 2016, 23, 104. [Google Scholar] [CrossRef]
- Waifalkar, P.P.; Parit, S.B.; Chougale, A.D.; Sahoo, S.C.; Patil, P.S.; Patil, P.B. Immobilization of invertase on chitosan coated γ-Fe2O3 magnetic nanoparticles to facilitate magnetic separation. J. Colloid Interface Sci. 2016, 482, 159–164. [Google Scholar] [CrossRef]
- Zulfiqar, S.; Khan, R.; Rahman, M.U.; Iqbal, Z. Variation of structural, dielectric and magnetic properties of PVP coated γ-Fe2O3 nanoparticles. J. Mater. Sci. Mater. Electron. 2016, 27, 12490–12498. [Google Scholar] [CrossRef]
- Rajput, S.; Singh, L.P.; Pittman, C.U.; Mohan, D. Lead (Pb2+) and copper (Cu2+) remediation from water using superparamagnetic maghemite (γ-Fe2O3) nano-particles synthesized by flame spray pyrolysis (FSP). J. Colloid Interface Sci. 2017, 492, 176–190. [Google Scholar] [CrossRef]
- Stier, M.; Neumann, A.; Philippi-Kobs, A.; Oepen, H.P.; Thorwart, M. Implications of a temperature-dependent magnetic anisotropy for superparamagnetic switching. J. Magn. Magn. Mater. 2018, 447, 96–100. [Google Scholar] [CrossRef]
- Wang, H.; Chen, Q.-W.; Sun, L.-X.; Qi, H.; Yang, X.; Zhou, S.; Xiong, J. Magnetic-field- induced formation of one-dimensional magnetite nanochains. Langmuir 2009, 25, 7135–7139. [Google Scholar] [CrossRef]
- Hassan, M.; Fetecau, C.; Majeed, A.; Zeeshan, A. Effects of iron nanoparticles’ shape on convective flow of ferrofluid under highly oscillating magnetic field over stretchable rotating disk. J. Magn. Magn. Mater. 2018, 465, 531–539. [Google Scholar] [CrossRef]
- Ellahi, R.; Hassan, M.; Zeeshan, A. The shape effects of nanoparticles suspended in HFE-7100 over wedge with entropy generation and mixed convection. Appl. Nanosci. 2016, 6, 641–651. [Google Scholar] [CrossRef]
- Ellahi, R.; Tariq, M.H.; Hassan, M.; Vafai, K. On boundary layer nano-ferroliquid flow under the influence of low oscillating stretchable rotating disk. J. Mol. Liq. 2017, 229, 339–345. [Google Scholar] [CrossRef]
- Keith, A.; Bouza, P. The Impact of Dissolution on Particle Size and Particle Shape of Multi-Component Drug Delivery Systems. Micromeritics Pharmaceutical Services. Available online: https://www.micromeritics.com/Repository/Files/The_Impact_of_Dissolution_on_Particle_Size_and_Particle_Shape_of_Multi-Component_Drug_Delivery_Systems.pdf (accessed on 23 November 2022).
- Tanguy, D.; Marchal, P. Relations between the properties of particles and their process of manufacture. Chem. Eng. Res. Des. 1996, 74, 715–722. [Google Scholar]
- Allen, K.; Davey, R.J. Controlling polymorphism via nucleation: Glycine crystallising in microemulsions. In Proceedings of the 14th International Symposium on Industrial Crystallization, Cambridge, UK, 12–16 September 1999. [Google Scholar]
- Alander, E. The influence of solvent composition on crystal agglomeration of paracetamol. Licentiate Thesis, Royal Institute of Technology, Stockholm, Sweden, 2001. [Google Scholar]
- Finnie, S.D.; Ristic, R.I.; Sherwood, J.N.; Zikic, A.M. Morphological and growth rate distributions of small self-nucleated paracetamol crystals grown from pure aqueous solutions. J. Cryst. Growth 1999, 207, 308–318. [Google Scholar] [CrossRef]
- Lee, H.Y.; Monnier, O. The control of crystal morphology of pharmaceutical products. In Proceedings of the 14th International Symposium on Industrial Crystallization, Cambridge, UK, 12–16 September 1999. [Google Scholar]
- Bhamidi, V.; Hanson, B.L.; Edmundson, A.; Skrzypczak-Jankun, E.; Schall, C. Influence of a Homologous Protein Impurity on Lysozyme Crystal Growth. J. Cryst. Growth 1999, 204, 542–552. [Google Scholar] [CrossRef]
- Graves, B.; Weiss, H. Encapsulation techniques. In Encyclopedia of Food Science and Technology; Hui, Y.H., Ed.; John Wiley & Sons, Inc.: New York, NY, USA, 1992; Volume 2, pp. 697–703. [Google Scholar]
- DeZarn, T.J. Food ingredients encapsulation. In Encapsulation and Controlled Release of Food Ingredients; Risch, S.J., Reineccius, G.A., Eds.; American Chemical Society: Washington, DC, USA, 1995; Chapter 7; pp. 75–86. [Google Scholar]
- Vision Analytical Inc. 2022. Available online: https://particleshape.com/wp-content/uploads/2018/01/Brochure-redesign-Peter-3-updateD_3-3.pdf (accessed on 23 November 2022).
- Chen, J.; Sarma, B.; Evans, J.M.B.; Myerson, A.S. Pharmaceutical Crystallization. Cryst. Growth Des. 2011, 11, 887–895. [Google Scholar] [CrossRef]
- Decuzzi, P.; Godin, B.; Tanaka, T.; Lee, S.Y.; Chiappini, C.; Liu, X.; Ferrari, M. Size and shape effects in the biodistribution of intravascularly injected particles. J. Control. Release 2010, 141, 320–327. [Google Scholar] [CrossRef]
- Doshi, N.; Prabhakarpandian, B.; Rea-Ramsey, A.; Pant, K.; Sundaram, S.; Mitragotri, S. Flow and adhesion of drug carriers in blood vessels depend on their shape: A study using model synthetic microvascular networks. J. Control. Release 2010, 146, 196–200. [Google Scholar] [CrossRef]
- Liu, Q.; Lazouskaya, V.; He, Q.; Jin, Y. Effect of particle shape on colloid retention and release in saturated porous media. J. Environ. Qual. 2010, 39, 500–508. [Google Scholar] [CrossRef]
- Yoo, J.-W.; Irvine, D.J.; Discher, D.E.; Mitragotri, S. Bio-inspired, bioengineered and biomimetic drug delivery carriers. Nat. Rev. Drug Discov. 2011, 10, 521–535. [Google Scholar] [CrossRef] [PubMed]
- Adriani, G.; de Tullio, M.D.; Ferrari, M.; Hussain, F.; Pascazio, G.; Liu, X.; Decuzzi, P. The preferential targeting of the diseased microvasculature by disk-like particles. Biomaterials 2012, 33, 5504–5513. [Google Scholar] [CrossRef] [PubMed]
- Mosharraf, M.; Nystrom, C. The effect of particle size and shape and the surface specific dissolution rate of microsized practically insoluble drugs. Int. J. Pharm. 1995, 122, 35–47. [Google Scholar] [CrossRef]
- Hsu, S.-Y.; Wu, C.-W. Effects of particle shape in mass-diffusion-controlled dissolution process. Int. Commun. Heat Mass Transf. 2021, 125, 105321. [Google Scholar] [CrossRef]
- Benival, D.M.; Devarajan, P.V. Lipomer of doxorubicin hydrochloride for enhanced oral bioavailability. Int. J. Pharm. 2012, 423, 554–561. [Google Scholar] [CrossRef]
- Alibolandi, M.; Abnous, K.; Sadeghi, F.; Hosseinkhani, H.; Ramezani, M.; Hadizadeh, F. Folate receptor-targeted multimodal polymersomes for delivery of quantum dots and doxorubicin to breast adenocarcinoma: In vitro and in vivo evaluation. Int. J. Pharm. 2016, 500, 162–178. [Google Scholar] [CrossRef]
- Olson, J.A.; Schwartz, J.A.; Hahka, D.; Nguyen, N.; Bunch, T.; Jensen, G.M.; Adler-Moore, J.P. Toxicity and efficacy differences between liposomal amphotericin B formulations in uninfected and Aspergillus fumigatus infected mice. Med. Mycol. 2015, 53, 107–118. [Google Scholar] [CrossRef]
- Varamini, P.; Toth, I. Recent advances in oral delivery of peptide hormones. Expert Opin. Drug Deliv. 2016, 13, 1–16. [Google Scholar] [CrossRef]
- He, C.; Yin, L.; Song, Y.; Tang, C.; Yin, C. Optimization of multifunctional chitosan-siRNA nanoparticles for oral delivery applications, targeting TNF-α silencing in rats. Acta Biomater. 2015, 17, 98–106. [Google Scholar] [CrossRef]
- He, X.; Li, L.; Su, H.; Zhou, D.; Song, H.; Wang, L.; Jiang, X. Poly(ethylene glycol)-block-poly(ε-caprolactone)-and phospholipid-based stealth nanoparticles with enhanced therapeutic efficacy on murine breast cancer by improved intracellular drug delivery. Int. J. Nanomed. 2015, 10, 1791–1804. [Google Scholar] [CrossRef]
- Yang, H.; Tang, A.; Ouyang, J.; Li, M.; Mann, S. From natural attapulgite to mesoporous materials: Methodology, characterisation and structural evolution. J. Phys. Chem. B 2010, 114, 2390–2398. [Google Scholar] [CrossRef]
- Gomez-Flores, A.; Bradford, S.A.; Hwang, G.; Choi, S.; Tong, M.; Kim, H. Shape and orientation of bare silica particles influence their deposition under intermediate ionic strength: A study with QCM–D and DLVO theory. Colloids Surf. A Physicochem. Eng. Asp. 2020, 599, 124921. [Google Scholar] [CrossRef]
- Rolland, J.P.; Maynor, B.W.; Euliss, L.E.; Exner, A.E.; Denison, G.M.; DeSimone, J.M. Direct fabrication and harvesting of monodisperse, shape- specific nanobio-materials. J. Am. Chem. Soc. 2005, 127, 10096–10100. [Google Scholar] [CrossRef] [PubMed]
- Müller, K.; Fedosov, D.; Gompper, G. Margination of micro- and nano-particles in blood flow and its effect on drug delivery. Sci. Rep. 2014, 4, 4871. [Google Scholar] [CrossRef]
- Ferrari, M. Cancer nanotechnology: Opportunities and challenges. Nat. Rev. Cancer. 2005, 5, 161–171. [Google Scholar] [CrossRef] [PubMed]
- Jain, R.K.; Stylianopoulos, T. Delivering nanomedicine to solid tumors. Nat. Rev. Clin. Oncol. 2010, 7, 653–664. [Google Scholar] [CrossRef]
- Thompson, A.J.; Mastria, E.M.; Eniola-Adefeso, O. The margination propensity of ellipsoidal micro/nanoparticles to the endothelium in human blood flow. Biomaterials 2013, 34, 5863–5871. [Google Scholar] [CrossRef]
- Charoenphol, P.; Huang, R.B.; Eniola-Adefeso, O. Potential role of size and hemodynamics in the efficacy of vascular-targeted spherical drug carriers. Biomaterials 2010, 31, 1392–1402. [Google Scholar] [CrossRef]
- Charoenphol, P.; Mocherla, S.; Bouis, D.; Namdee, K.; Pinsky, D.J.; Eniola-Adefeso, O. Targeting therapeutics to the vascular wall in atherosclerosisecarrier size matters. Atherosclerosis 2011, 217, 364–370. [Google Scholar] [CrossRef]
- Champion, J.A.; Mitragotri, S. Shape induced inhibition of phagocytosis of polymer particles. Pharm. Res. 2009, 26, 244–249. [Google Scholar] [CrossRef] [PubMed]
- Geng, Y.; Dalhaimer, P.; Cai, S.; Tsai, R.; Tewari, M.; Minko, T.; Discher, D.E. Shape effects of filaments versus spherical particles in flow and drug delivery. Nat. Nanotechnol. 2007, 2, 249–255. [Google Scholar] [CrossRef] [PubMed]
- Muro, S.; Garnacho, C.; Champion, J.A.; Leferovich, J.; Gajewski, C.; Schuchman, E.H.; Mitragotri, S.; Muzykantov, V.R. Control of endothelial targeting and intracellular delivery of therapeutic enzymes by modulating the size and shape of ICAM-1-targeted carriers. Mol. Ther. 2008, 16, 1450–1458. [Google Scholar] [CrossRef]
- Gavze, E.; Shapiro, M. Motion of inertial spheroidal particles in a shear flow near a solid wall with special application to aerosol transport in microgravity. J. Fluid. Mech. 1998, 371, 59–79. [Google Scholar] [CrossRef]
- Lee, S.Y.; Ferrari, M.; Decuzzi, P. Shaping nano-/micro-particles for enhanced vascular interaction in laminar flows. Nanotechnology 2009, 20, 495101. [Google Scholar] [CrossRef] [PubMed]
- Decuzzi, P.; Ferrari, M. The adhesive strength of non-spherical particles mediated by specific interactions. Biomaterials 2006, 27, 5307–5314. [Google Scholar] [CrossRef]
- Gómez, L.J.V.; Magaña, Y.T.; Bravo, J.M.C.; Graziano, R.V.; Cai, S. Cellular Responses to Nanomaterials with Biomedical Applications. J. Nanomater. 2022, 3, 9823140. [Google Scholar] [CrossRef]
- Chowdhury, M.M.H.; Kubra, K.; Kanwar, R.K.; Kanwar, J.R. Nanoparticles Advancing Cancer Immunotherapy. In Biomedical Applications of Graphene and 2D Nanomaterials: Micro and Nano Technologies; Nurunnabi, M.D., McCarthy, J.R., Eds.; Elsevier: Amsterdam, The Netherlands, 2019; Chapter 13; pp. 283–304. [Google Scholar] [CrossRef]
- Toy, R.; Peiris, P.M.; Ghaghada, K.B.; Karathanasis, E. Shaping cancer nanomedicine: The effect of particle shape on the in vivo journey of nanoparticles. Nanomedicine 2014, 9, 121–134. [Google Scholar] [CrossRef] [PubMed]
- Agarwal, R.; Jurney, P.; Raythatha, M.; Singh, V.; Sreenivasan, S.; Shi, L.; Roy, K. Effect of shape, size, and aspect ratio on nanoparticle penetration and distribution inside solid tissues using 3D spheroid models. Adv. Healthc. Mater. 2015, 4, 2269–2280. [Google Scholar] [CrossRef]
- Augustine, R.; Hasan, A. Cellular response to nanobiomaterials. In Handbook of Biomaterials Biocompatibility; Mozafari, M., Ed.; Woodhead Publishing: Cambridge, UK, 2020; Chapter 21; pp. 473–504. [Google Scholar] [CrossRef]
- Sartin, M.M.; Yu, Z.; Chen, W.; He, F.; Sun, Z.; Chen, Y.-X.; Huang, W. Effect of Particle Shape and Electrolyte Cation on CO Adsorption to Copper Oxide Nanoparticle Electrocatalysts. J. Phys. Chem. C 2018, 122, 26489–26498. [Google Scholar] [CrossRef]
- Gentile, F.; Chiappini, C.; Fine, D.; Bhavane, R.C.; Peluccio, M.S.; Cheng, M.M.; Liu, X.; Ferrari, M.; Decuzzi, P. The effect of shape on the margination dynamics of non-neutrally buoyant particles in two- dimensional shear flows. J. Biomech. 2008, 41, 2312–2318. [Google Scholar] [CrossRef]
- Ottemann, B.M.; Helmink, A.J.; Zhang, W.; Mukadam, I.; Woldstad, C.; Hilaire, J.R.; Liu, Y.; McMillan, J.E.M.; Edagwa, B.J.; Mosley, R.L.; et al. Bioimaging predictors of rilpivirine biodistribution and antiretroviral activities. Biomaterials 2018, 185, 174–193. [Google Scholar] [CrossRef]
- Huang, X.; Teng, X.; Chen, D.; Tang, F.; He, J. The effect of the shape of mesoporous silica nanoparticles on cellular uptake and cell function. Biomaterials 2010, 31, 438–448. [Google Scholar] [CrossRef]
- Dasgupta, S.; Auth, T.; Gompper, G. Shape and orientation matter for the cellular uptake of nonspherical particles. Nano Lett. 2014, 14, 687–693. [Google Scholar] [CrossRef]
- Graf, C.; Nordmeyer, D.; Sengstock, C.; Ahlberg, S.; Diendorf, J.; Raabe, J.; Epple, M.; Koller, M.; Lademann, J.; Vogt, A.; et al. Shape-Dependent Dissolution and Cellular Uptake of Silver Nanoparticles. Langmuir 2018, 34, 1506–1519. [Google Scholar] [CrossRef]
- Gratton, S.E.A.; Ropp, P.A.; Pohlhaus, P.D.; Luft, J.C.; Madden, V.J.; Napier, M.E.; DeSimone, J.M. The effect of particle design on cellular internalization pathways. Proc. Natl. Acad. Sci. USA 2008, 105, 11613–11618. [Google Scholar] [CrossRef]
- Kolhar, P.; Anselmo, A.C.; Gupta, V.; Pant, K.; Prabhakarpandian, B.; Ruoslahti, E.; Mitragotri, S. Using shape effects to target antibody-coated nanoparticles to lung and brain endothelium. Proc. Natl. Acad. Sci. USA 2013, 110, 10753–10758. [Google Scholar] [CrossRef]
- Salatin, S.; Dizaj, S.M.; Khosroushahi, A.Y. Effect of the surface modification, size, and shape on cellular uptake of nanoparticles. Cell Biol. Int. 2015, 39, 881–890. [Google Scholar] [CrossRef]
- Teleanu, D.M.; Chircov, C.; Grumezescu, A.M.; Volceanov, A.; Teleanu, R.I. Impact of Nanoparticles on Brain Health: An Up to Date Overview. J. Clin. Med. 2018, 7, 490. [Google Scholar] [CrossRef]
- Grimaldi, A.M.; Incoronato, M.; Salvatore, M.; Soricelli, A. Nanoparticle-based strategies for cancer immunotherapy and immunodiagnostics. Nanomedicine 2017, 12, 2349–2365. [Google Scholar] [CrossRef]
- Barua, S.; Yoo, J.-W.; Kolhar, P.; Wakankar, A.; Gokarn, Y.R.; Mitragotri, S. Particle shape enhances specificity of antibody-displaying nanoparticles. Proc. Natl. Acad. Sci. USA 2013, 110, 3270–3275. [Google Scholar] [CrossRef]
- Melchionna, M.; Fornasiero, P.; Cargnello, M. Opportunities and Challenges in the Synthesis, Characterization, and Catalytic Properties of Controlled Nanostructures. In Studies in Surface Science and Catalysis; Fornasiero, P., Cargnello, M., Eds.; Elsevier: Amsterdam, The Netherlands, 2017; Volume 177, Chapter 1; pp. 1–56. [Google Scholar] [CrossRef]
- Lee, I.; Delbecq, F.; Morales, R.; Albiter, M.A.; Zaera, F. Tuning selectivity in catalysis by controlling particle shape. Nat. Mater. 2009, 8, 132–138. [Google Scholar] [CrossRef]
- Taylor, H.S. A theory of the catalytic surface. Proc. R. Soc. A 1925, 108, 105–111. [Google Scholar] [CrossRef]
- Sinfelt, J.H. Specificity in catalytic hydrogenolysis by metals. Adv. Catal. 1973, 23, 91–119. [Google Scholar] [CrossRef]
- Clarke, J.K.A.; Rooney, J.J. Stereochemical approach to mechanisms of hydrocarbon reactions on metal catalysts. Adv. Catal. 1976, 25, 125–183. [Google Scholar] [CrossRef]
- Bennett, C.O.; Che, M. Some geometric aspects of structure sensitivity. J. Catal. 1989, 120, 293–302. [Google Scholar] [CrossRef]
- Ertl, G. Surface science and catalysis-studies on the mechanism of ammonia synthesis: The P. H. Emmett Award address. Catal. Rev. Sci. Eng. 1980, 21, 201–223. [Google Scholar] [CrossRef]
- Somorjai, G.A.; Materer, N. Surface structures in ammonia synthesis. Top. Catal. 1994, 1, 215–231. [Google Scholar] [CrossRef]
- Babich, I.V.; Moulijn, J.A. Science and technology of novel processes for deep desulfurization of oil refinery streams: A review. Fuel 2003, 82, 607–631. [Google Scholar] [CrossRef]
- Shi, Y.; Li, Z.; Yang, C.; Yang, Z.; Lv, Z.; Peng, C.; Su, B.-L.; Yuan, W.; Zhou, X.; Duan, X. Catalyst particle shapes and pore structure engineering for hydrodesulfurization and hydrodenitrogenation reactions. Front. Chem. Sci. Eng. 2022, 16, 897–908. [Google Scholar] [CrossRef]
- Kolitcheff, S.; Jolimate, E.; Hugon, A.; Verstraete, J.; Rivallan, M.; Carrette, P.L.; Couenne, F.; Tayakout-Fayolle, M. Tortuosity and mass transfer limitations in industrial hydrotreating catalysts: Effect of particle shape and size distribution. Catal. Sci. Technol. 2018, 8, 4537–4549. [Google Scholar] [CrossRef]
- Hagemeyer, R.W. Pigments for Paper: A Project of the Coating Pigments Committee of Tappi’s Coating & Graphic Arts Division; TAPPI Press: Atlanta, GA, USA, 1997. [Google Scholar]
- Domingo, C.; Loste, E.; Gómez-Morales, J.; García-Carmona, J.; Fraile, J. Calcite Precipitation by a High-Pressure CO2 Carbonation Route. J. Supercrit. Fluids 2006, 36, 202–215. [Google Scholar] [CrossRef]
- Alen, R. Papermaking Chemistry, 2nd ed.; Finnish Paper Engineers’ Association: Helsinki, Finland, 2007. [Google Scholar]
- VTT Products and Production; Knowpap Versio 11.0; VTT Products and Production: Espoo, Finland, 2009.
- Pinto, G.; Jimenez-Martin, A. Conducting aluminum-filled nylon 6 composites. Polym. Compos. 2001, 22, 65–70. [Google Scholar] [CrossRef]
- Rose, H.E. Particle shape and surface area. In Powders in Industry; Society of Chemical Industry: London, UK, 1961; pp. 130–149. [Google Scholar]
- Kaya, E.; Hogg, R.; Kumar, S. Particle Shape Modification in Comminution. KONA Powder Part. J. 2002, 20, 188–195. [Google Scholar] [CrossRef]
- Abazarpoor, A.; Halali, M.; Hejazi, R.; Saghaeian, M.; Zadeh, V.S. Investigation of iron ore particle size and shape on green pellet quality. Can. Metall. Q. 2020, 59, 2. [Google Scholar] [CrossRef]
- Grasse, A.M. Roll Crusher for Producing Cubical Shaped Fragments. U.S. Patent 2696 949A, 14 December 1954. [Google Scholar]
- Papacharalambous, H.G. Method of Producing Spherical Particles. U.S. Patent 3,160,997A, 15 December 1964. [Google Scholar]
- Dehghani, F.; Rahimi, M.; Rezai, B. Influence of particle shape on the flotation of magnetite, alone and in the presence of quartz particles. J. South. Afr. Insti. Min. Metall. 2012, 113, 905–911. [Google Scholar]
- Little, L.; Mainza, A.N.; Becker, M.; Wiese, J. Fine grinding: How mill type affects particle shape characteristics and mineral liberation. Miner. Eng. 2017, 111, 148–157. [Google Scholar] [CrossRef]
- Gao, Z.; Li, C. Tuning the Morphology and Surface Property of Mineral Particles by Grinding Media. In Abrasive Technology: Characteristics and Applications; Rudawska, A., Ed.; IntechOpen: London, UK, 2018. [Google Scholar] [CrossRef]
- Guven, O.; Karakas, F.; Kodrazi, N.; Celik, M.S. Dependence of morphology on anionic flotation of alumina. Int. J. Miner. Process. 2016, 156, 69–74. [Google Scholar] [CrossRef]
- Ulusoy, U.; Bayar, G. Prediction of average shape values of Quartz particles by vibrating disc and ball milling using dynamic image analysis based on established time-dependent shape models. Part. Sci. Technol. 2022, 40, 870–886. [Google Scholar] [CrossRef]
- Dumm, T.F.; Hogg, R. Characterization of particle shape. In Proceedings of International Symposium Respirable Dust in the Mineral Industries; SME: Littleton, CO, USA, 1990; pp. 283–288. [Google Scholar]

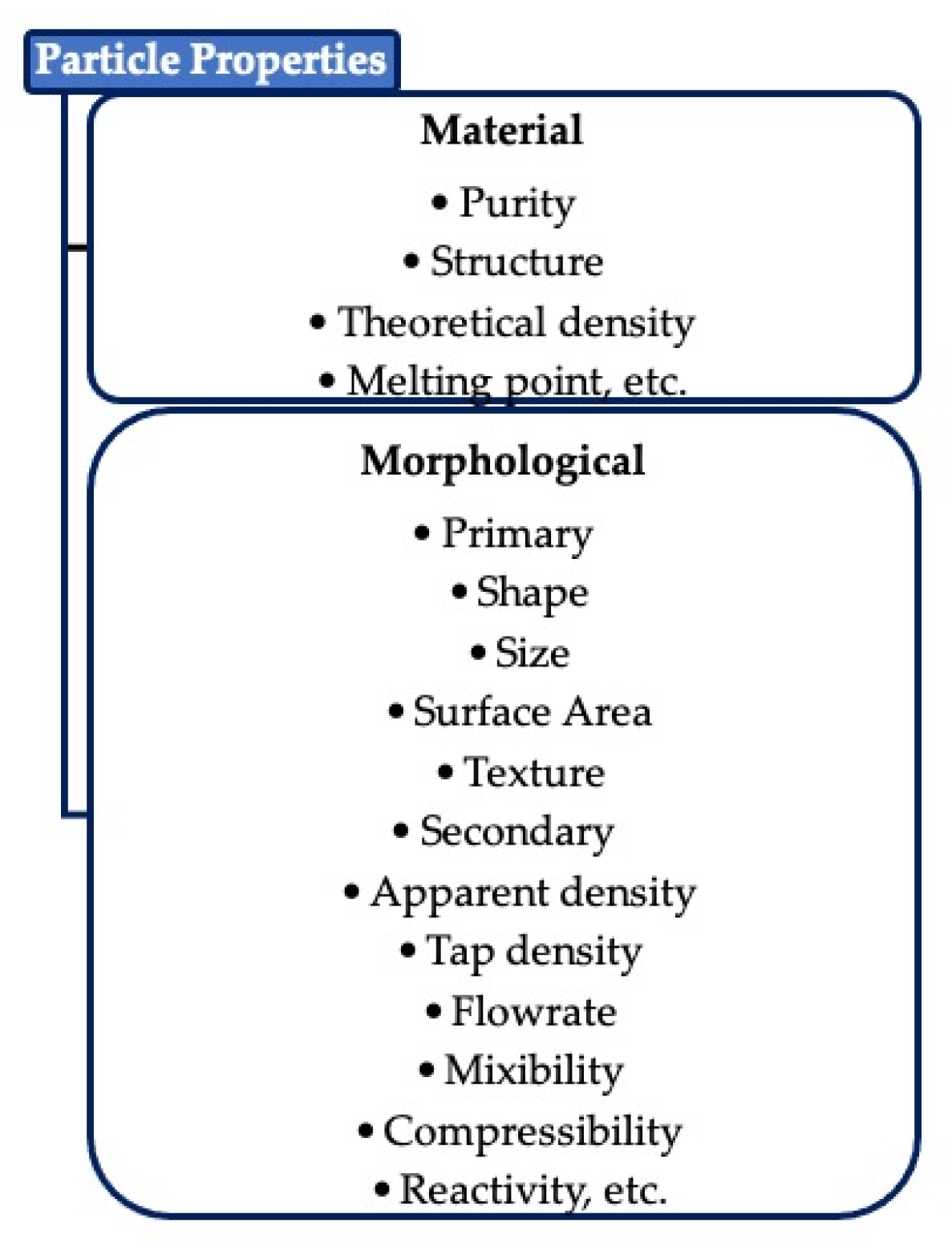
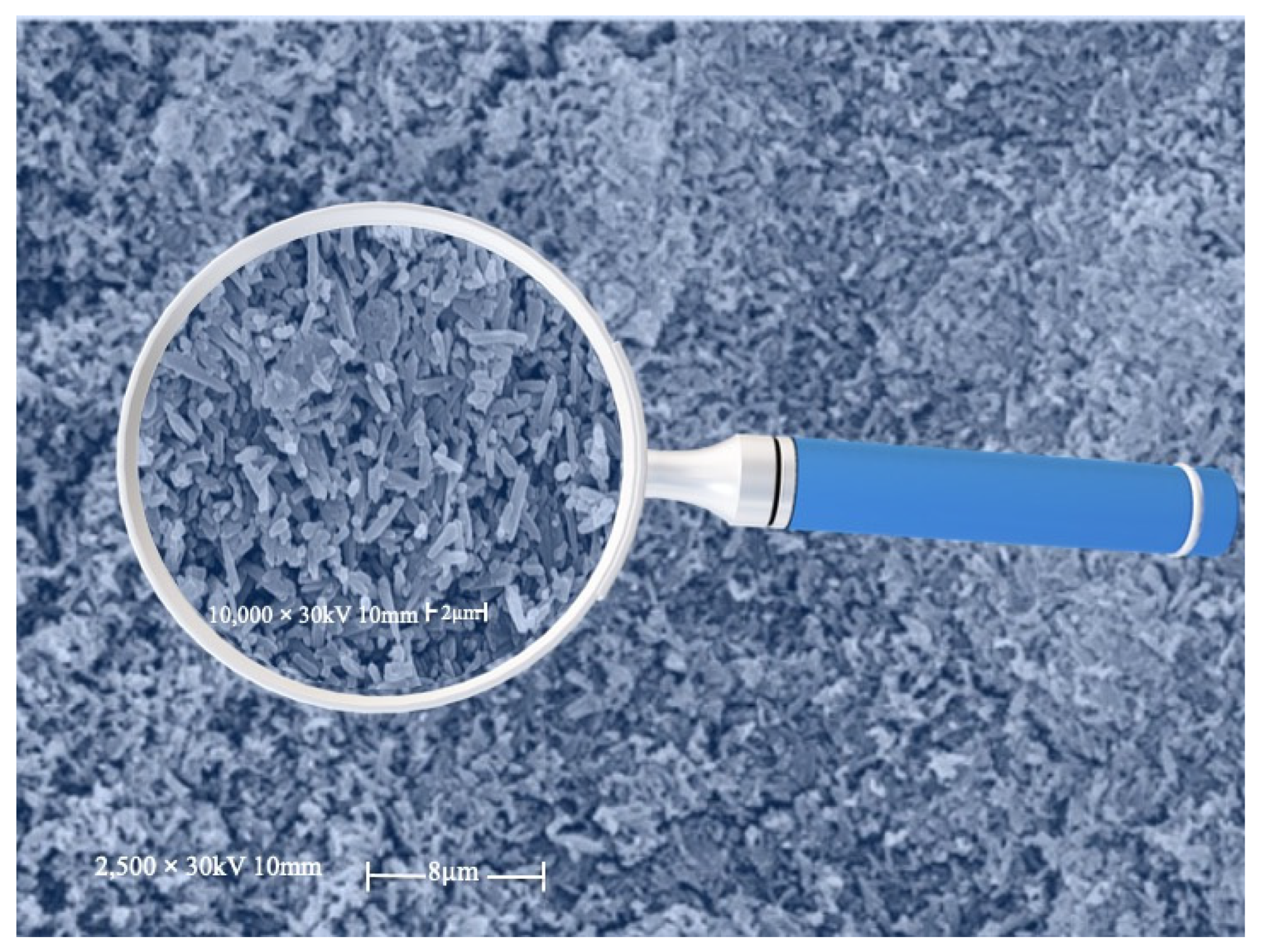

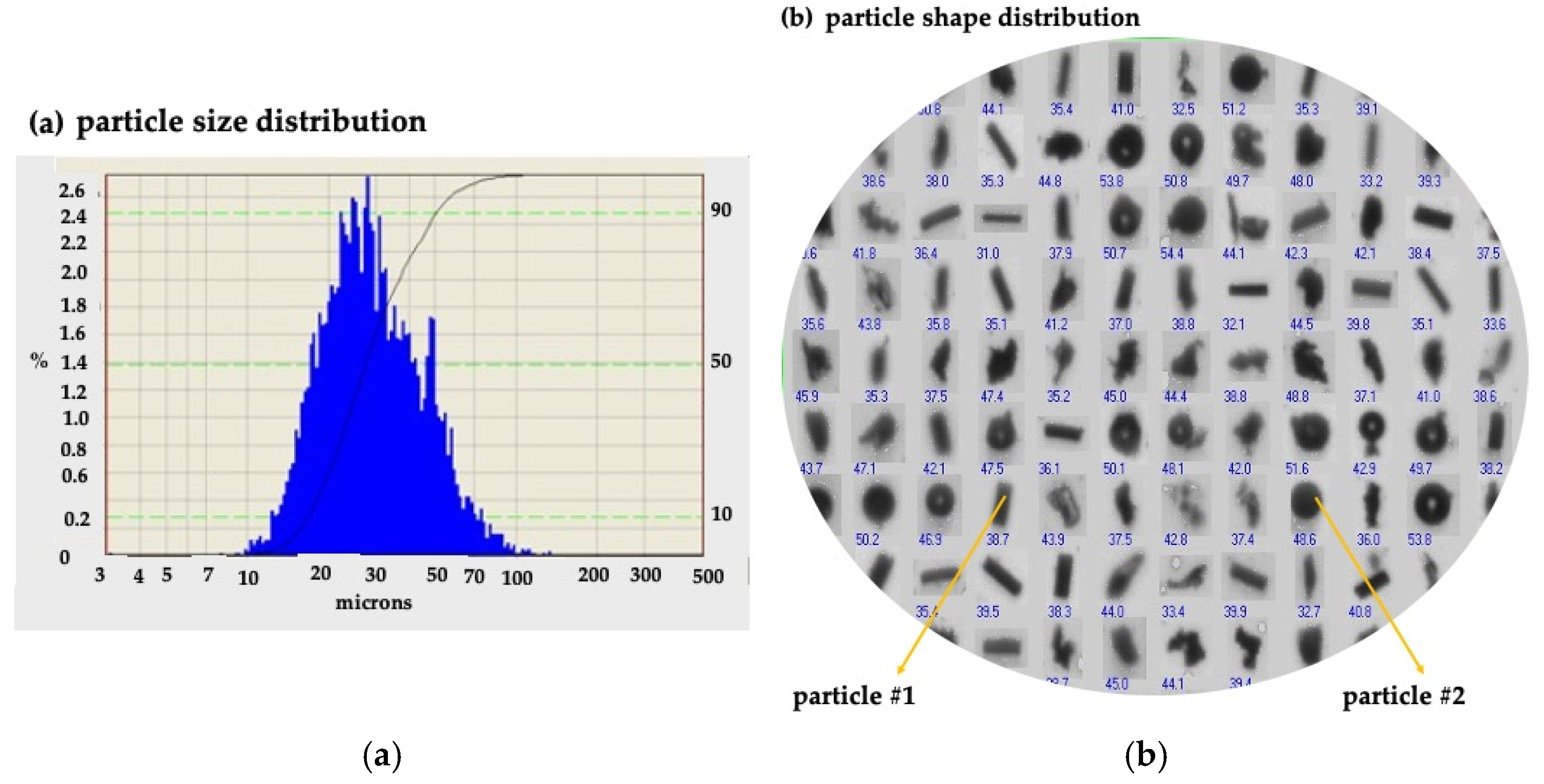
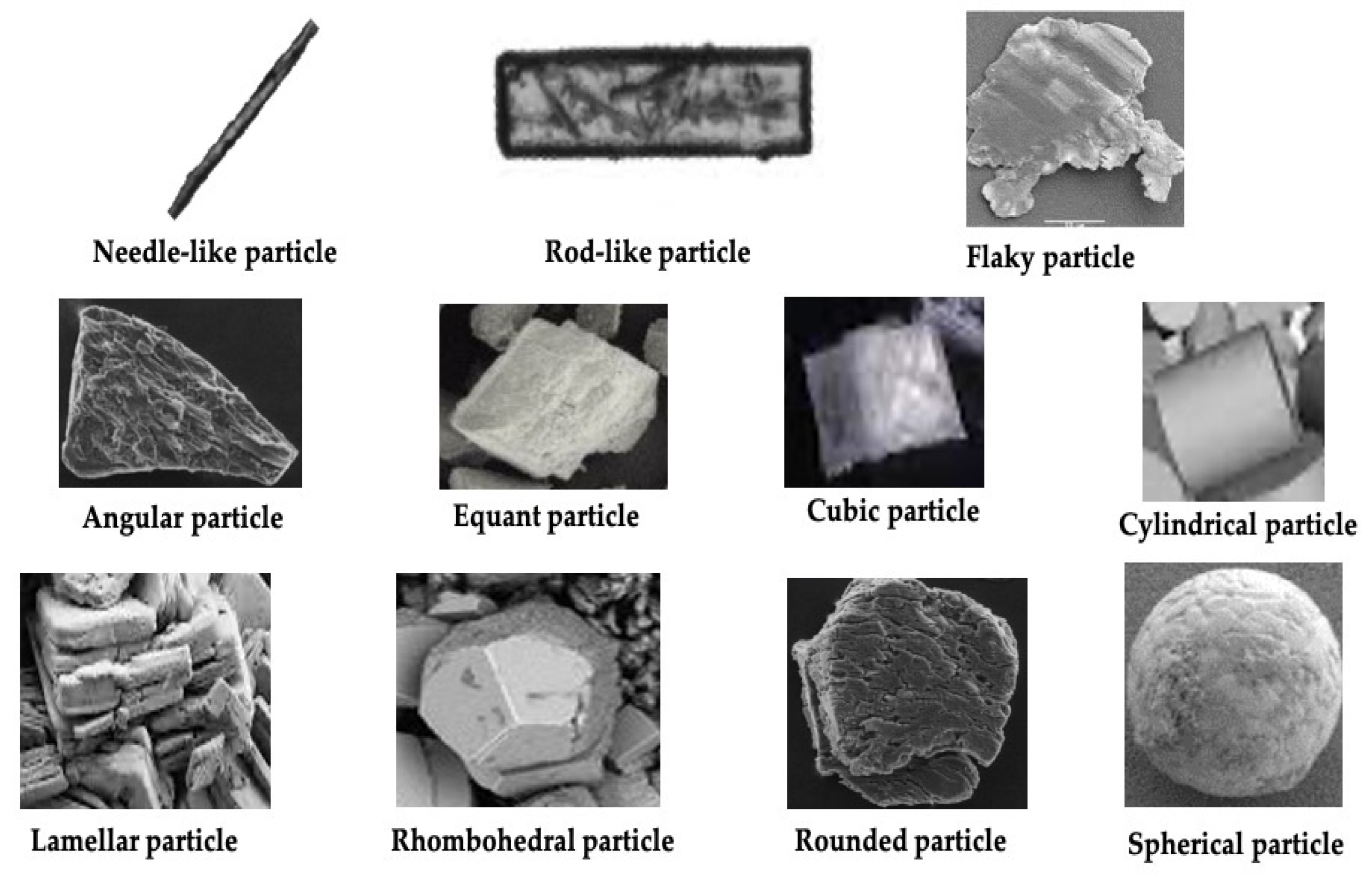
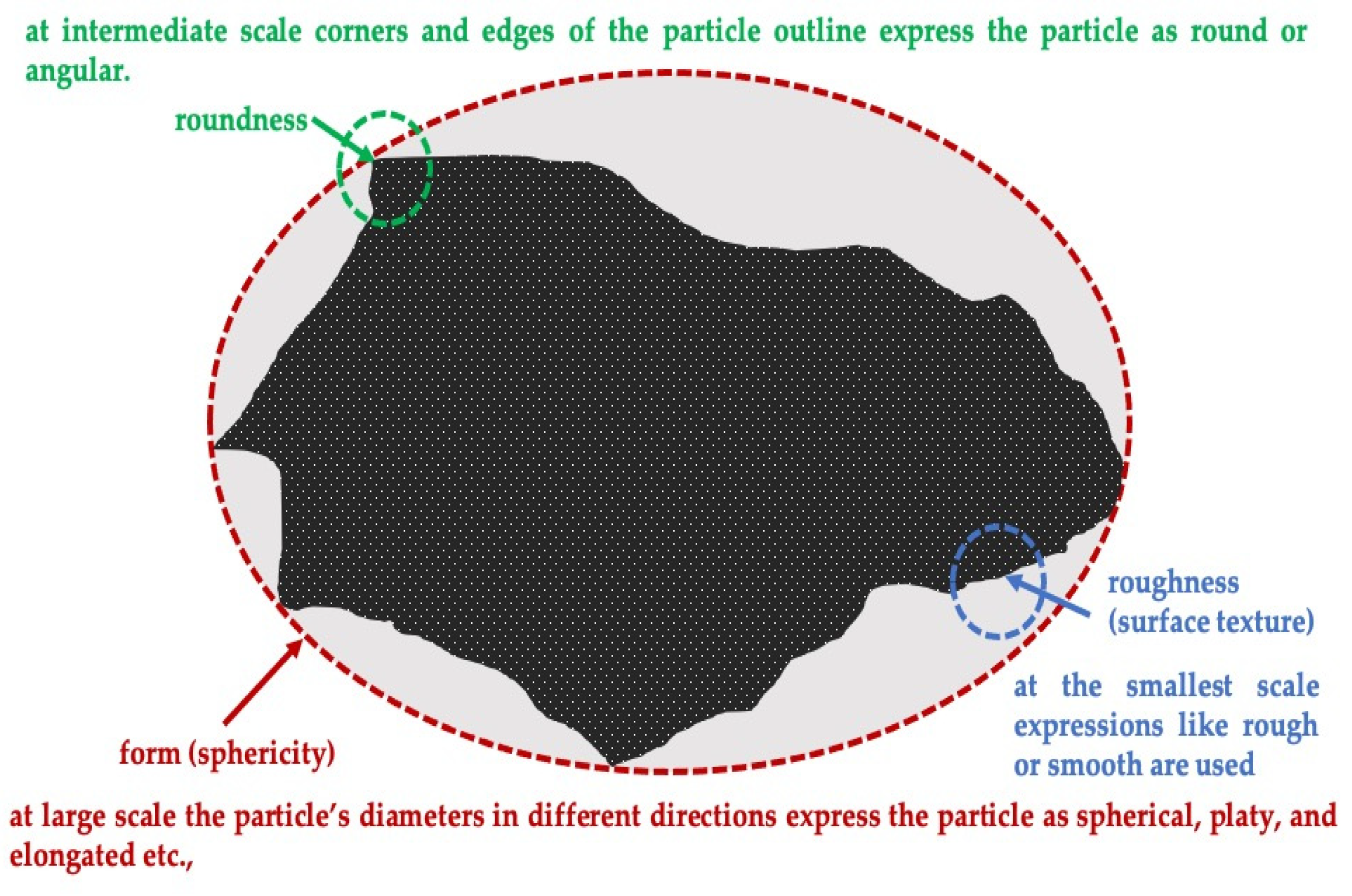
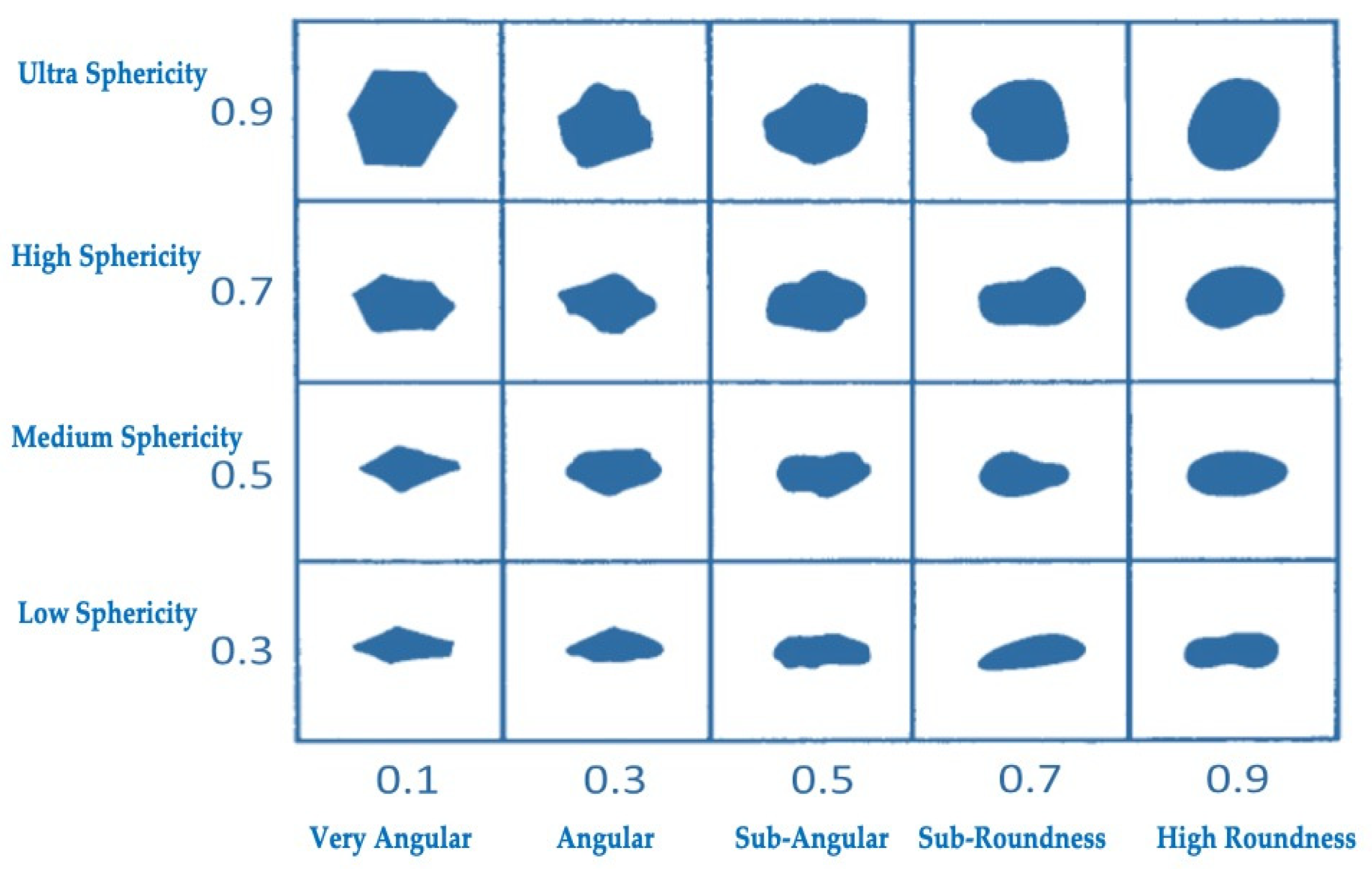

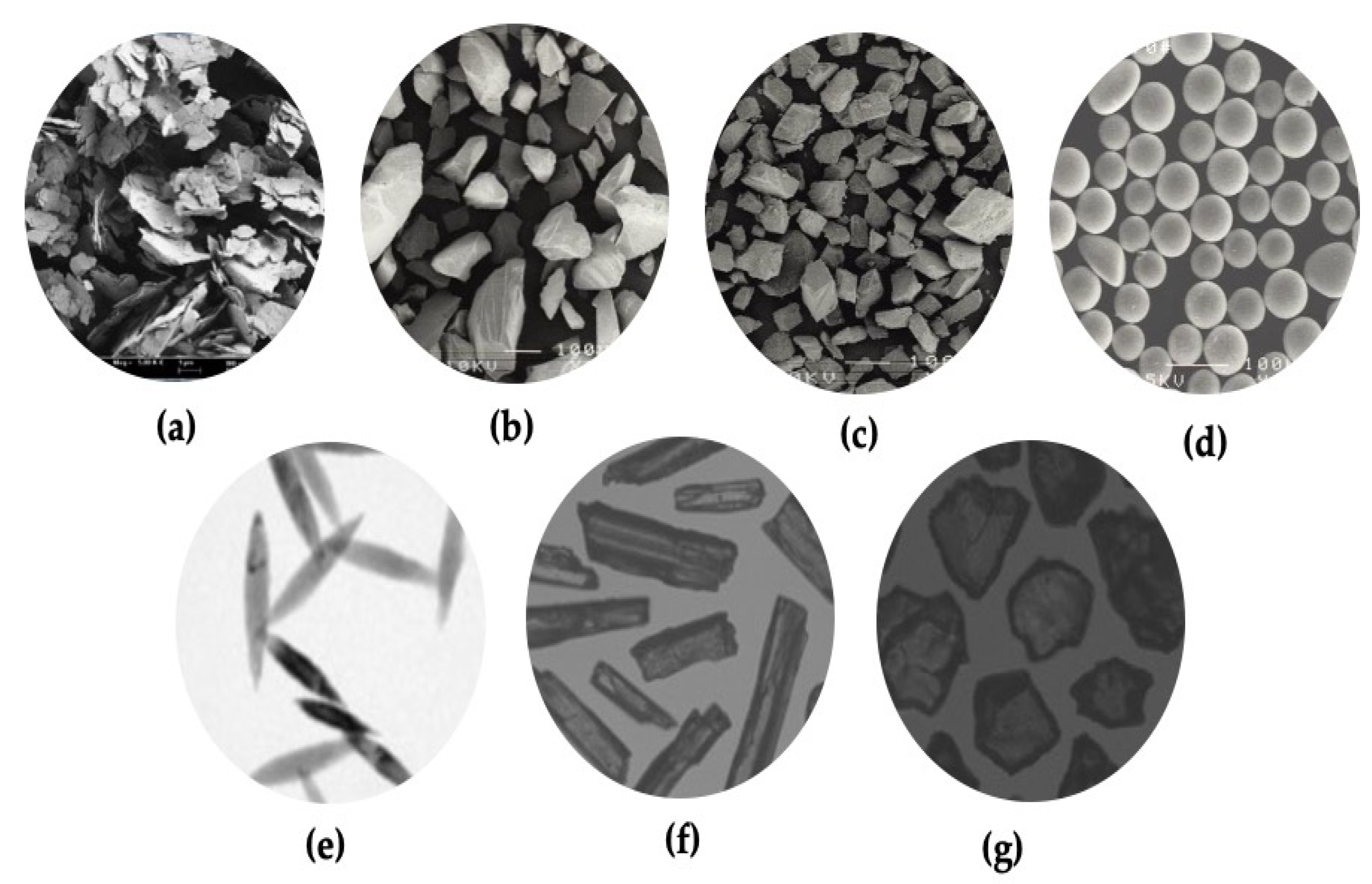
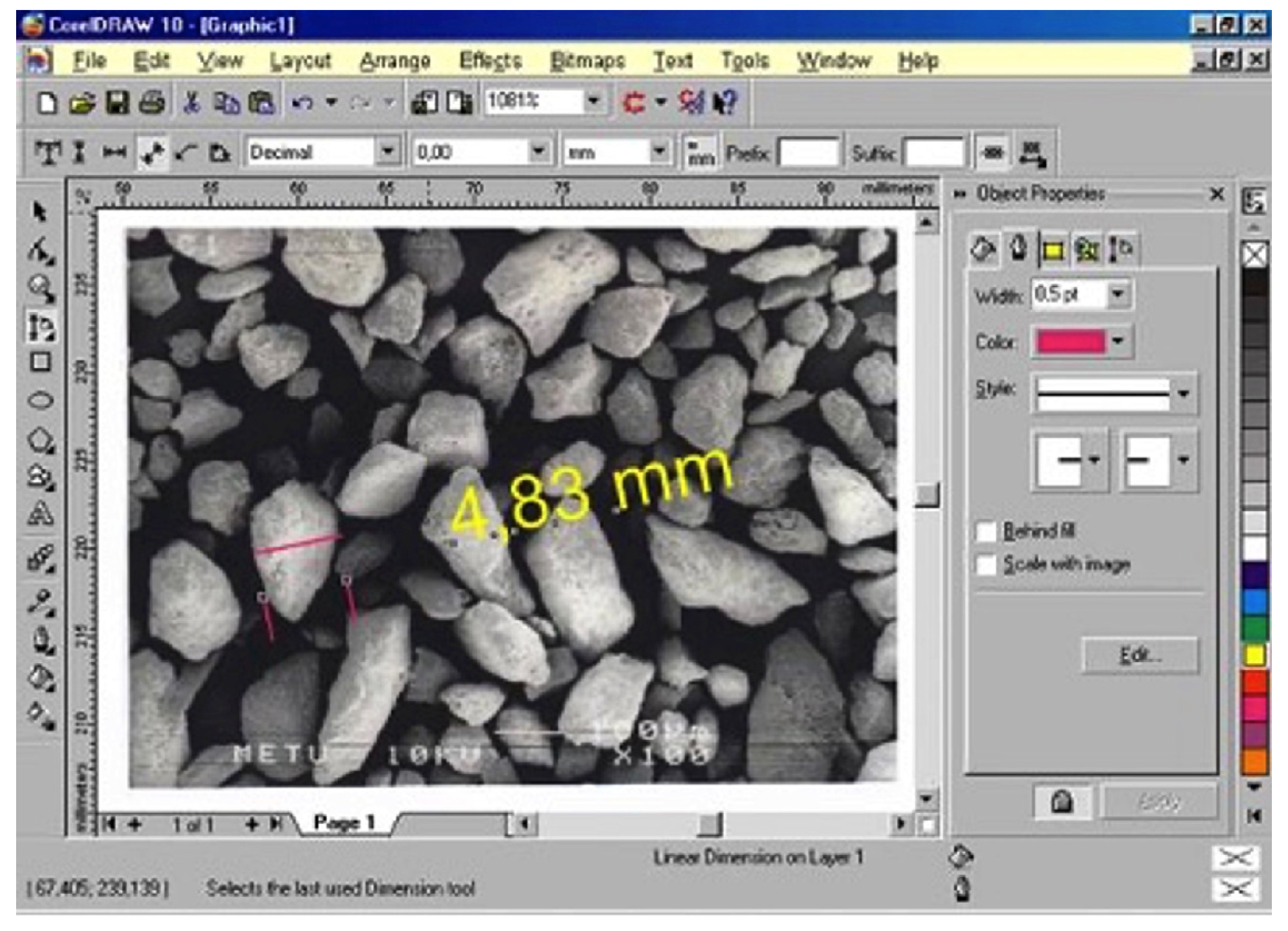

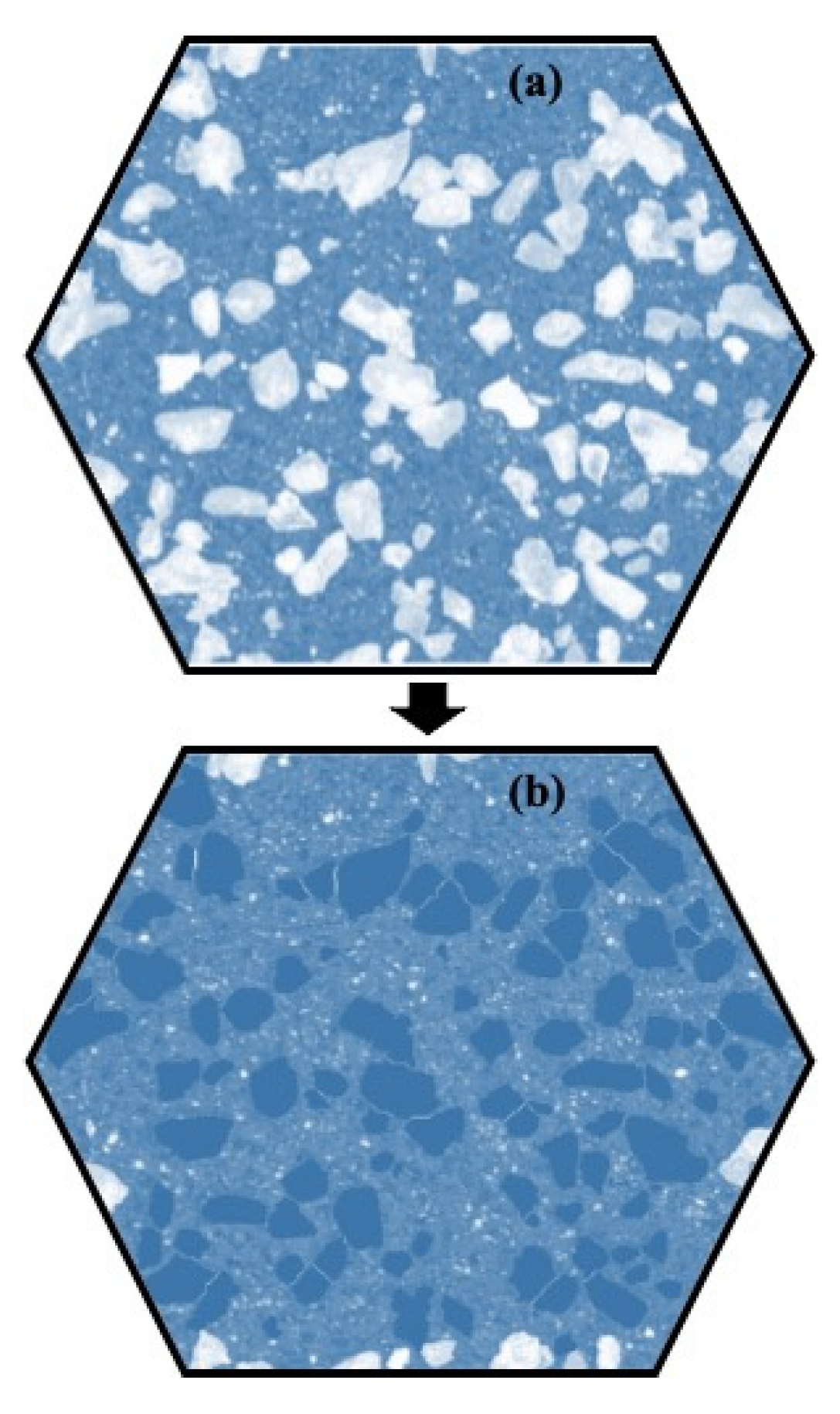
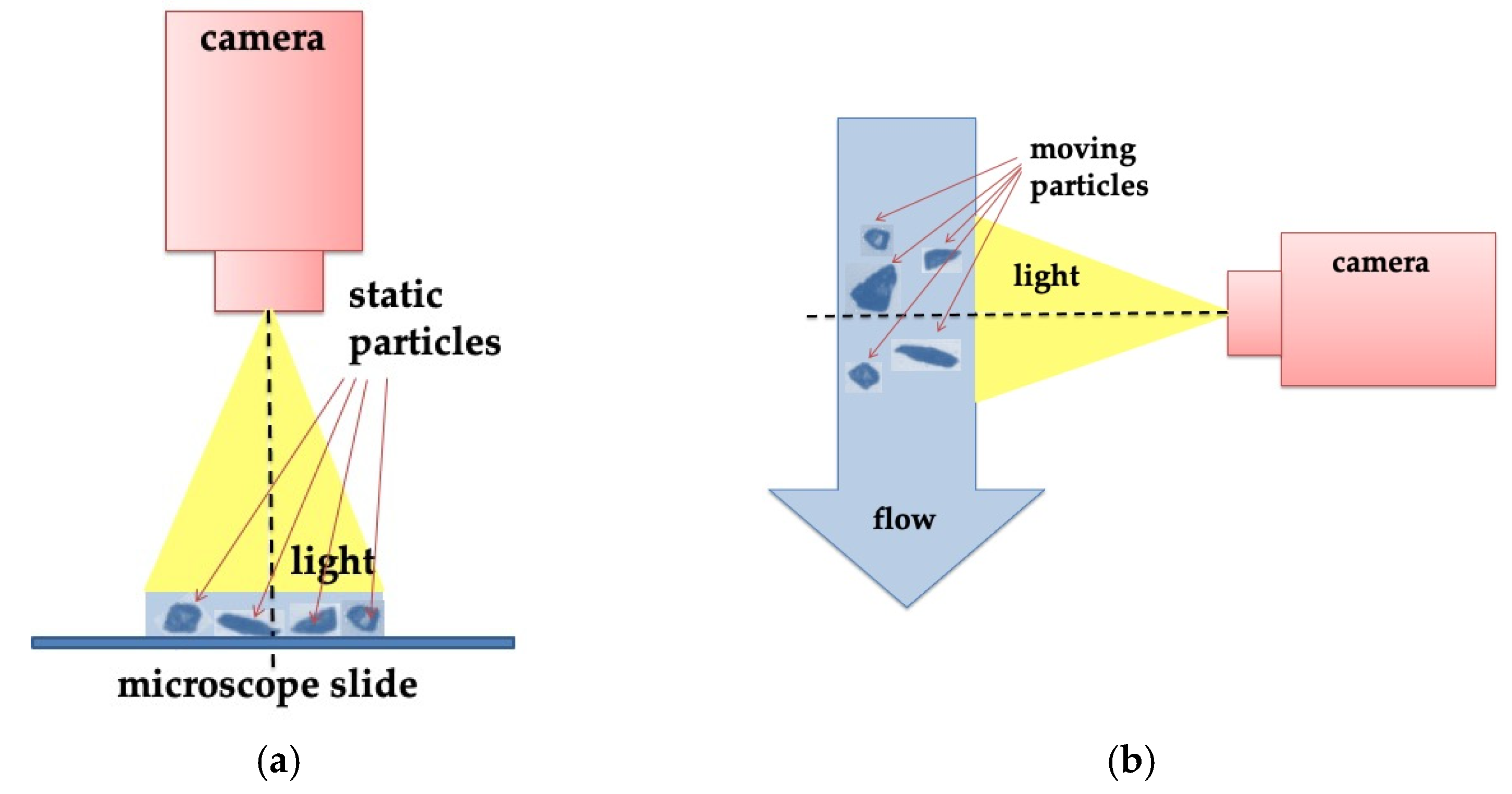

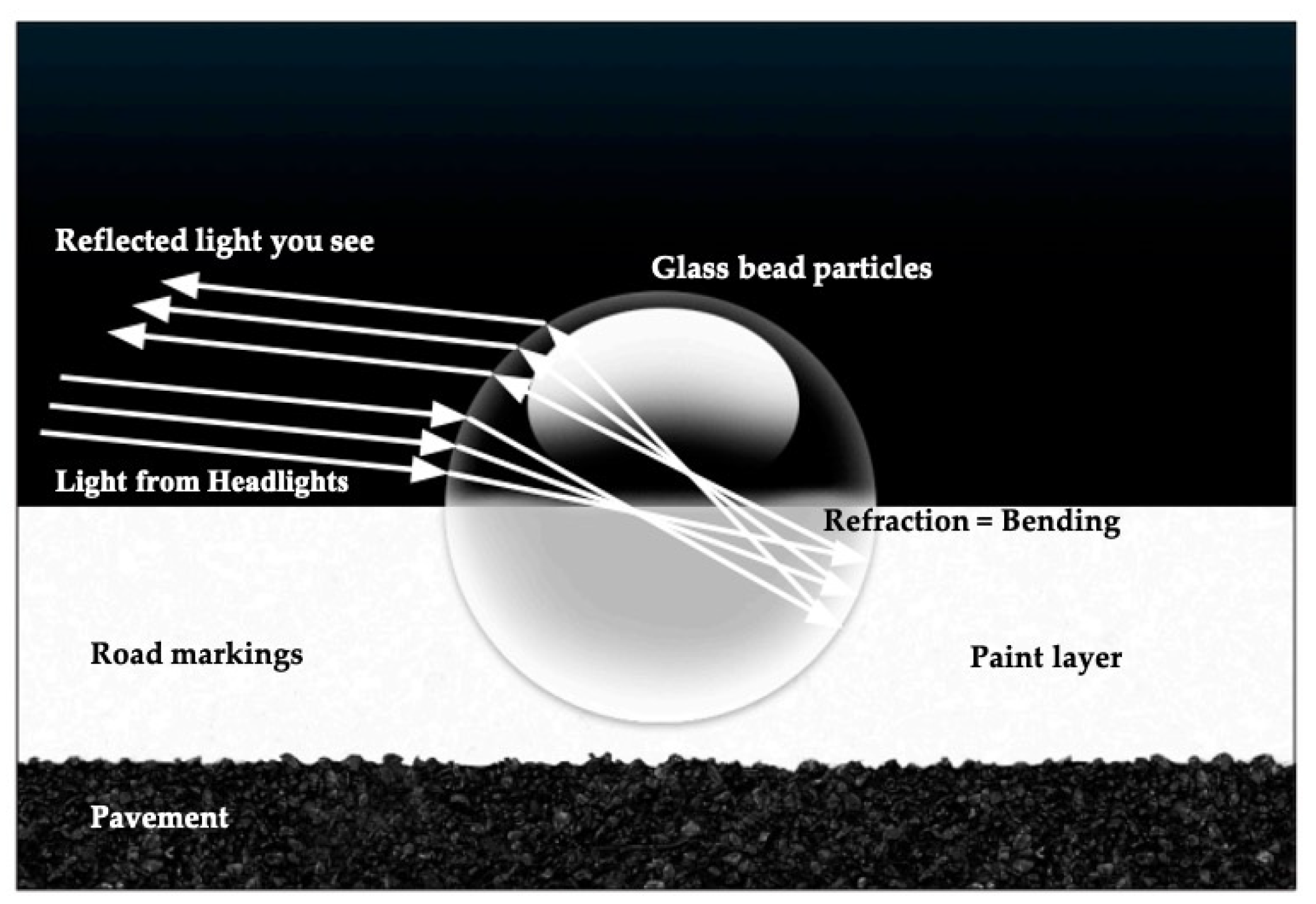
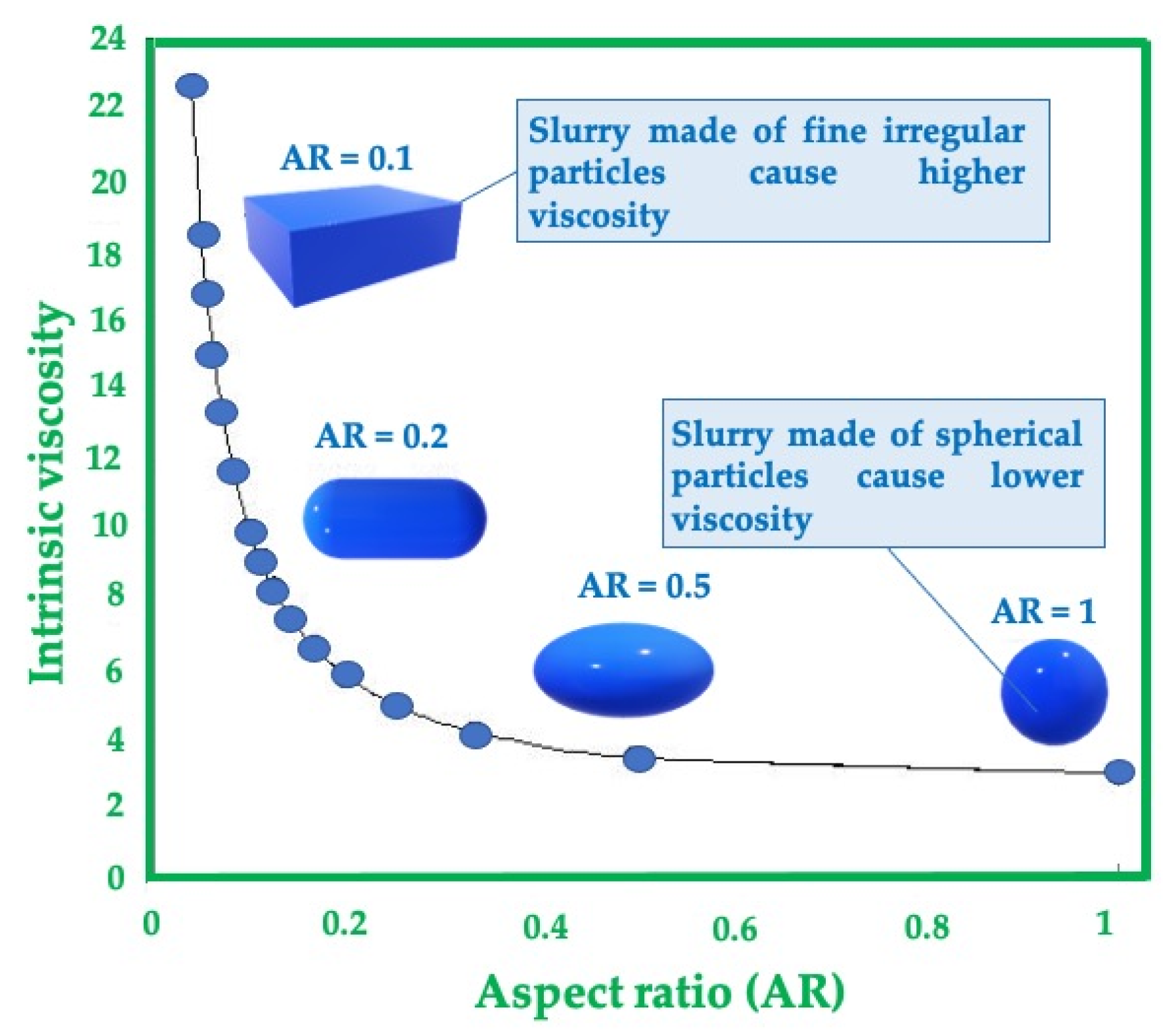
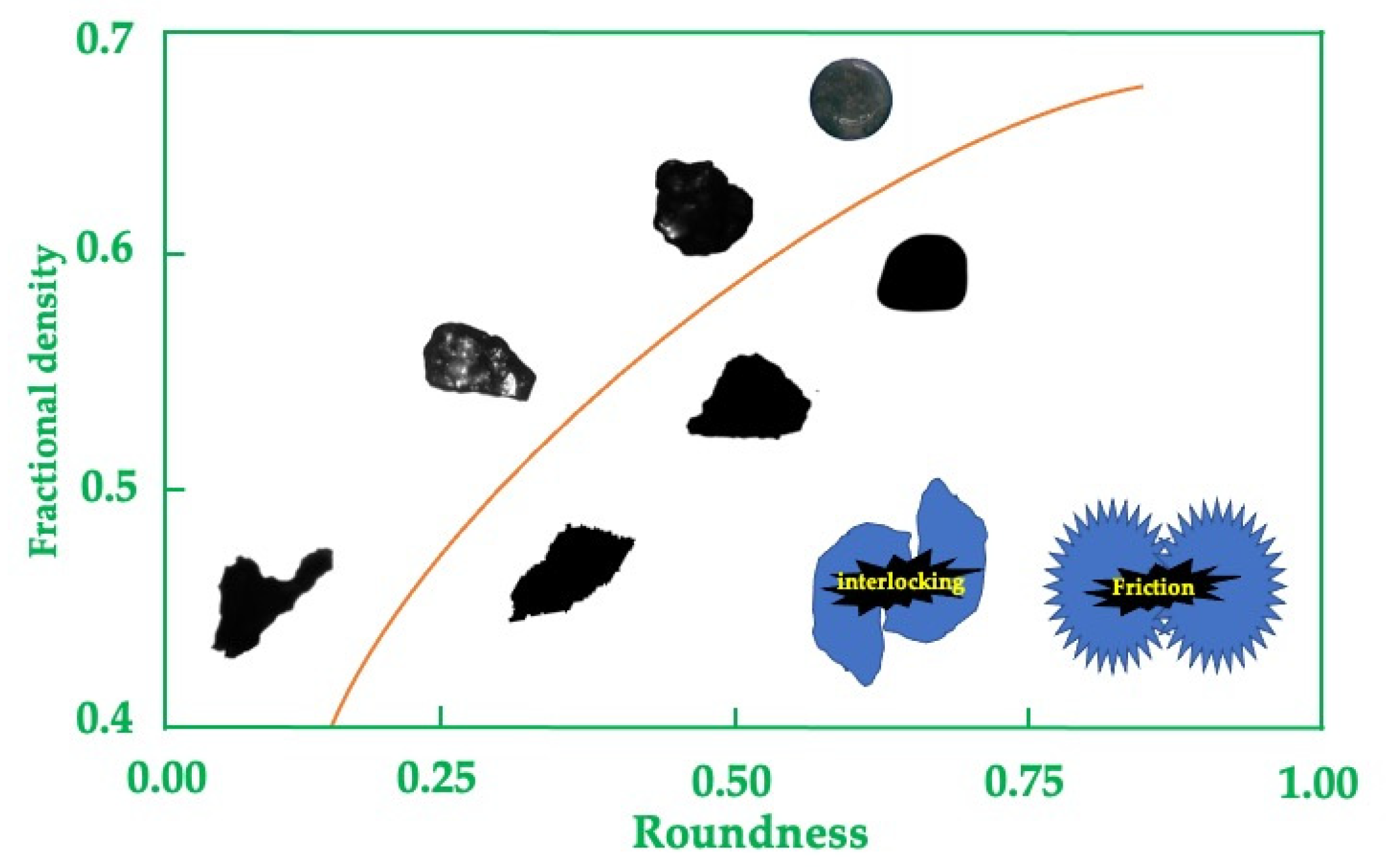
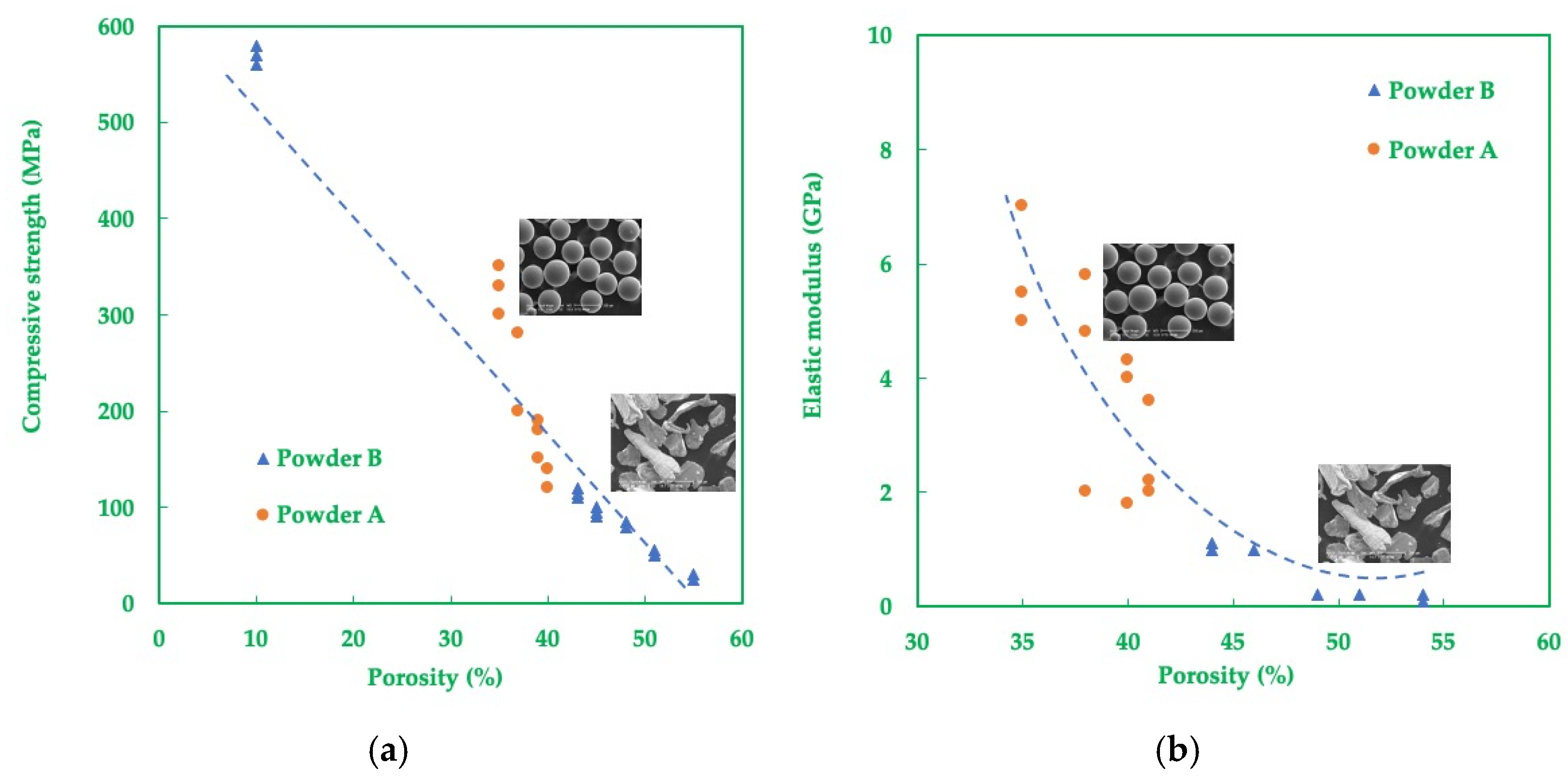
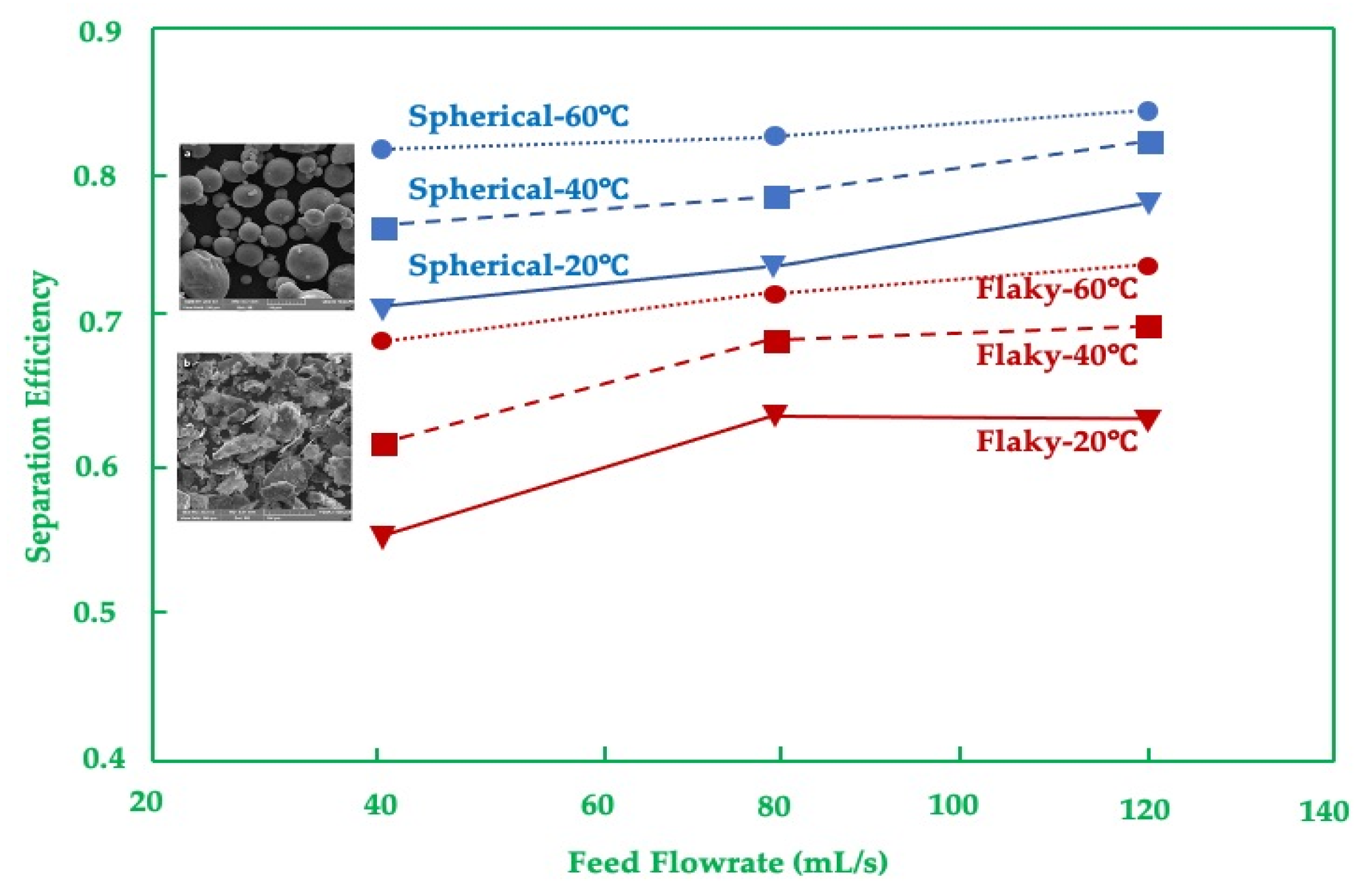
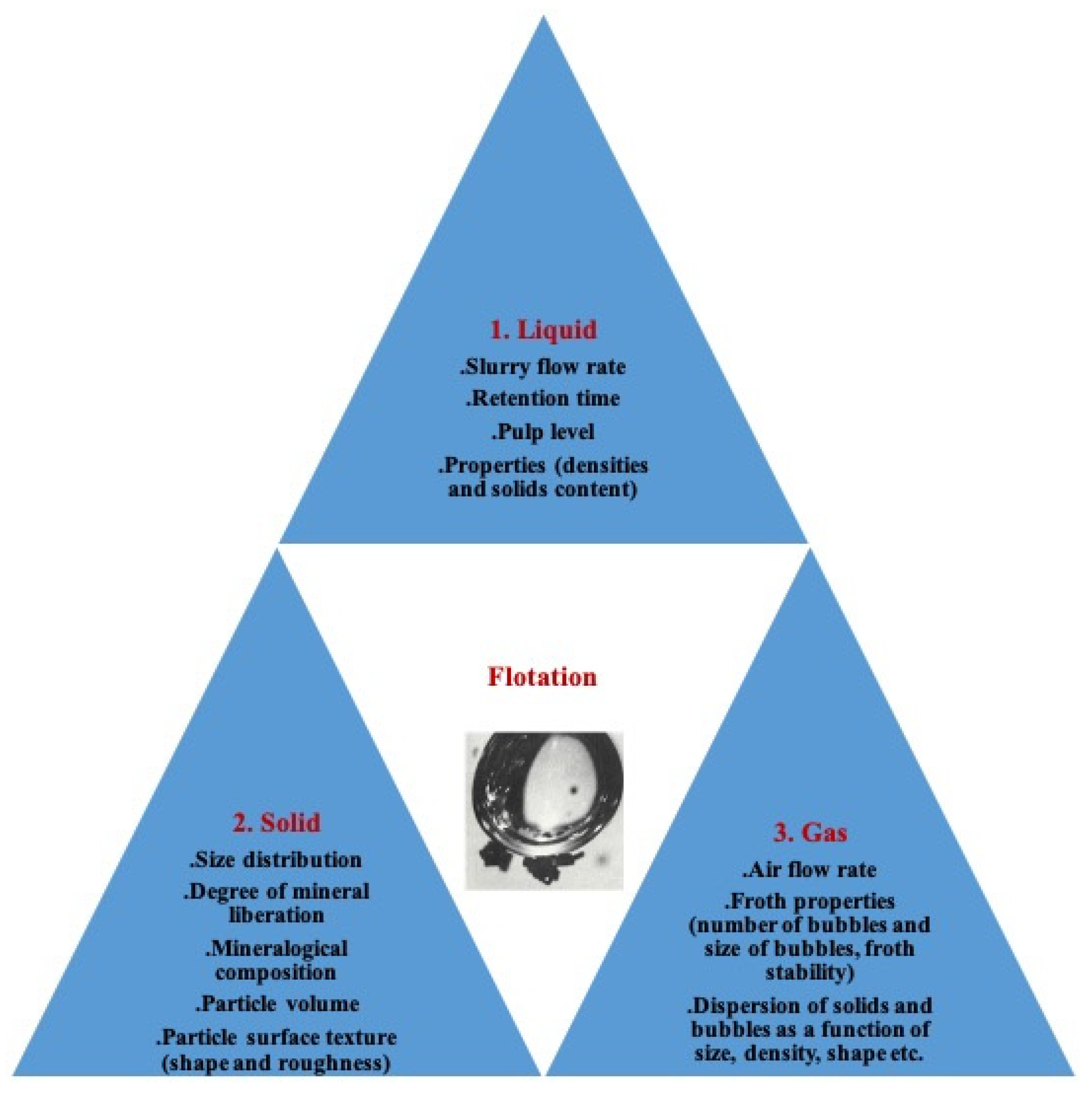
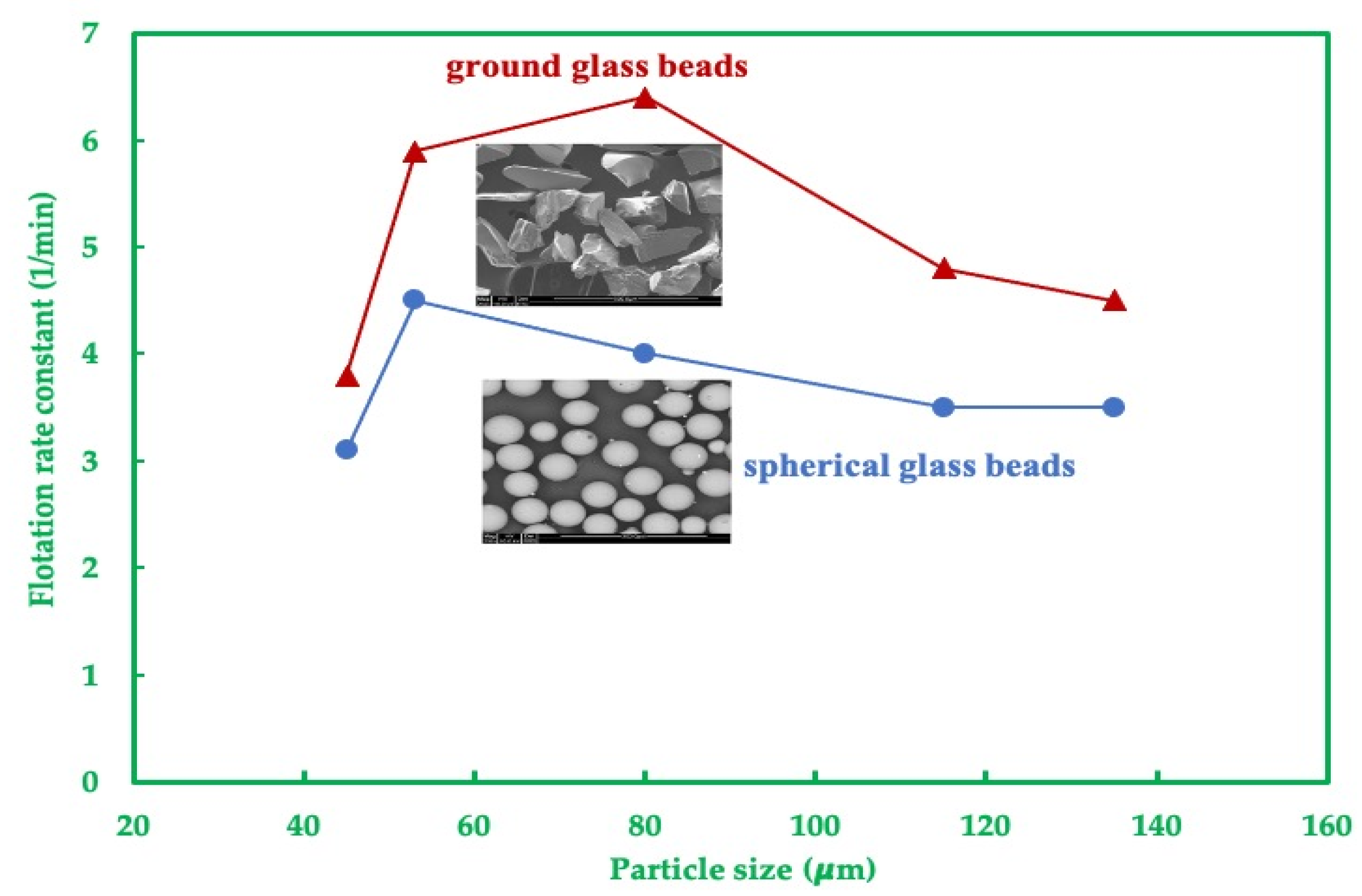
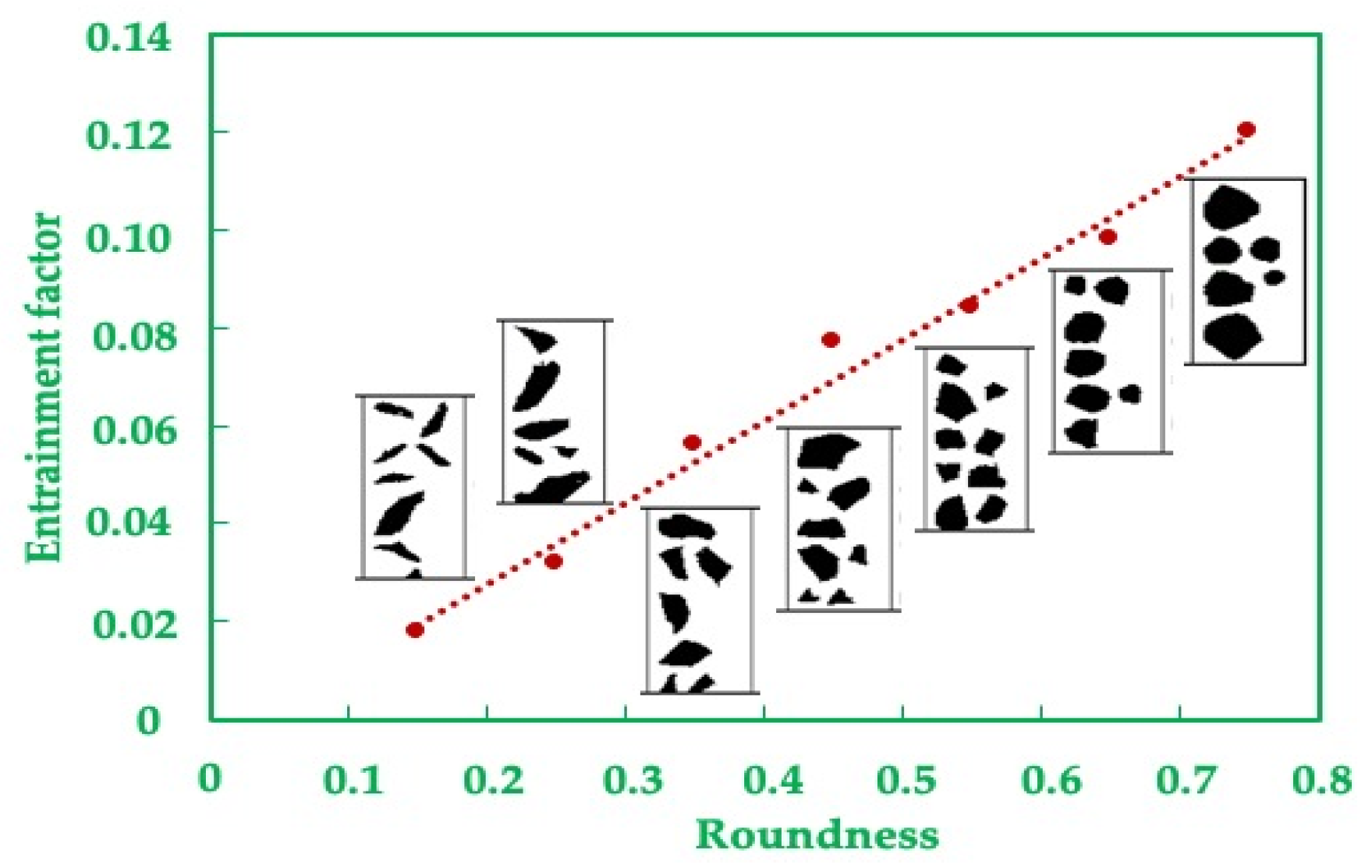


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ulusoy, U. A Review of Particle Shape Effects on Material Properties for Various Engineering Applications: From Macro to Nanoscale. Minerals 2023, 13, 91. https://doi.org/10.3390/min13010091
Ulusoy U. A Review of Particle Shape Effects on Material Properties for Various Engineering Applications: From Macro to Nanoscale. Minerals. 2023; 13(1):91. https://doi.org/10.3390/min13010091
Chicago/Turabian StyleUlusoy, Ugur. 2023. "A Review of Particle Shape Effects on Material Properties for Various Engineering Applications: From Macro to Nanoscale" Minerals 13, no. 1: 91. https://doi.org/10.3390/min13010091
APA StyleUlusoy, U. (2023). A Review of Particle Shape Effects on Material Properties for Various Engineering Applications: From Macro to Nanoscale. Minerals, 13(1), 91. https://doi.org/10.3390/min13010091