Assessment of the Self-Compaction Effect in Filtered Tailings Disposal under Unsaturated Condition
Abstract
:1. Introduction
2. Materials and Methods
2.1. Geotechnical Characterization of the Material
2.2. Acid Drainage Potential
2.3. Experimental Program
2.4. Conventions Considered for the Presentation of the Results
3. Results and Discussions
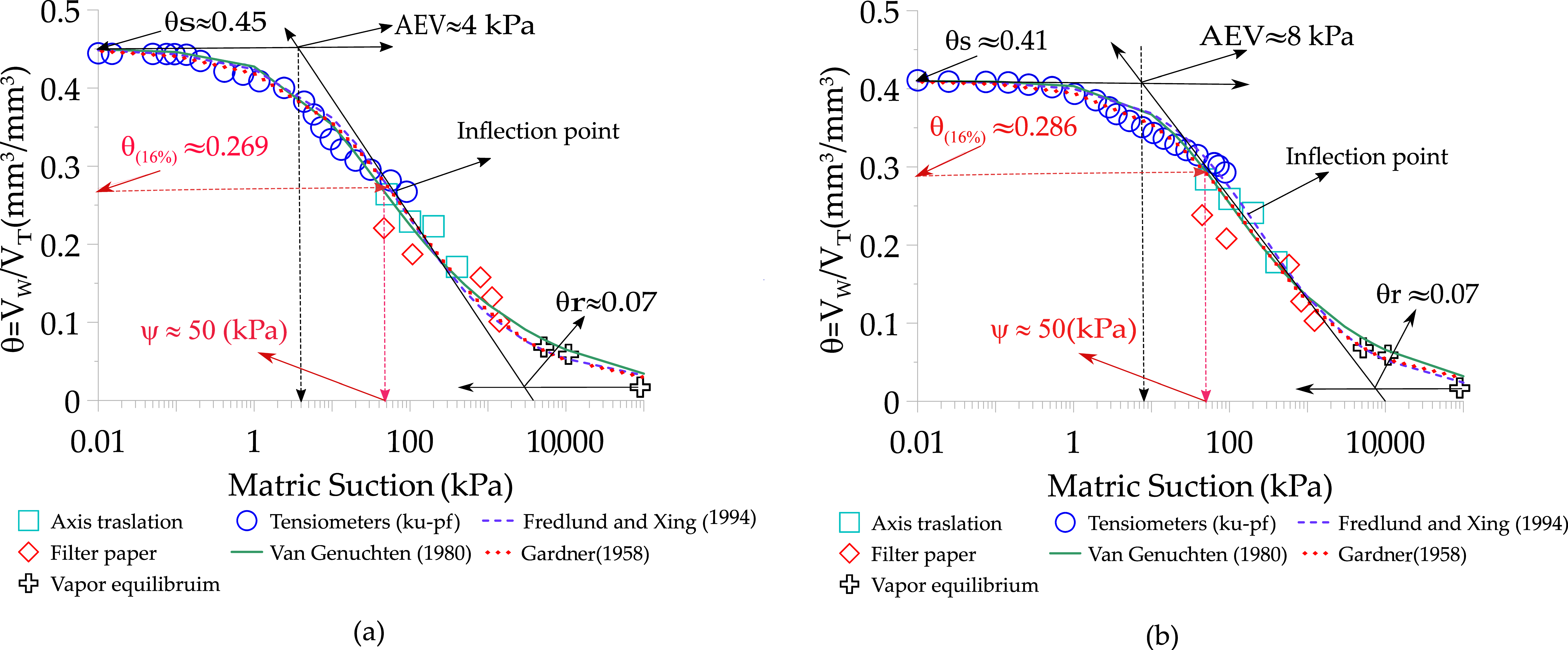
3.1. SWCC of Studies Densities
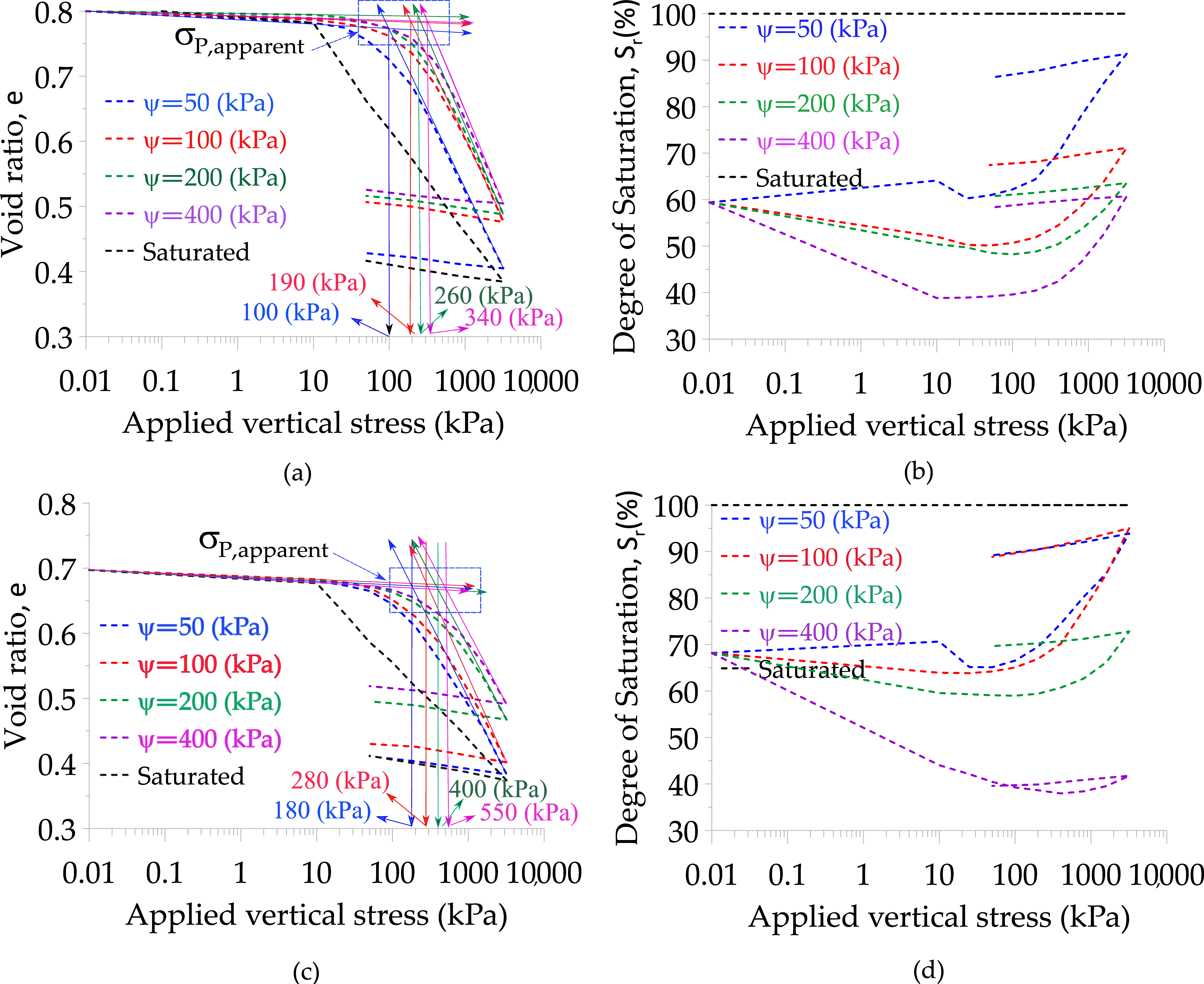
3.2. Hydromechanical Behaviour of the Material at a Constant Matric Suction in the 1D Consolidation Test
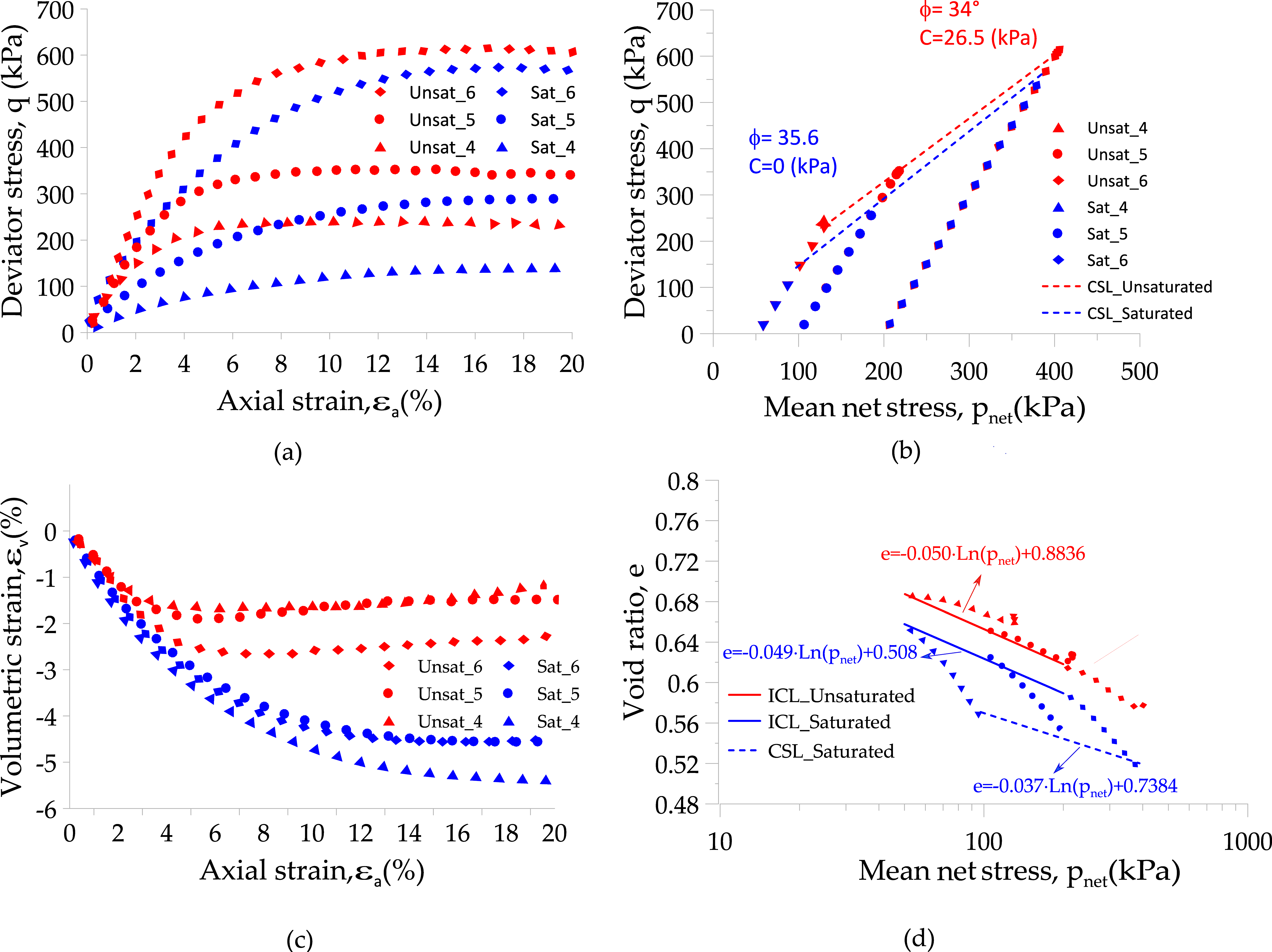
3.3. Hydromechanical Behaviour of Material at a Constant Matric Suction in Drained Triaxial Tests
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Simms, P. 2013 colloquium of the Canadian geotechnical society: Geotechnical and geoenvironmental behaviour of high-density tailings. Can. Geotech. J. 2016, 54, 455–468. [Google Scholar] [CrossRef] [Green Version]
- Lara Montani, J.; Pornillos, E.; Munoz, H. Geotechnical-Geochemical and operational considerations for the application ofdry stacking tailings deposits—State ofthe art. In Proceedings of the 16th International Seminar on Paste and Thickened Tailings, Belo Horizonte, Brazil, 17–19 June 2013; pp. 249–260. [Google Scholar]
- Weatherwx, T.W.; Kipara, A. Paste operations training at Barrick’s Bulyanhulu Gold Mine. In Proceedings of the 13th International Conference on Paste and Thickened Tailings, Toronto, ON, Canada, 3–6 May 2010; pp. 41–50. [Google Scholar]
- Davies, M.P.; Rice, S. An alternative to conventional tailing management—“Dry stack” filtered tailings. In Proceedings of the 8th International Conference on Tailings and Mine Waste’01, Fort Collins, CO, USA, 15–18 January 2001; pp. 411–420. [Google Scholar]
- Daliri, F.; Simms, P.; Sivathayalan, S. Shear and dewatering behaviour of densified gold tailings in a laboratory simulation of multi-layer deposition. Can. Geotech. J. 2016, 53, 1246–1257. [Google Scholar] [CrossRef]
- Oldecop, L.; Rodari, G. Unsaturated Soil Mechanics in Mining. In Proceedings of the Pan Am Unsaturated Soils, Dallas, TX, USA, 12–15 November 2017; pp. 257–280. [Google Scholar]
- Ulrich, B.; Coffin, J. Considerations for Tailings Facility Design and Operation Using Filtered Tailings Summary of Significant Operating Filtered Tailings Operations. In Proceedings of the 16th International Seminar on Paste and Thickened Tailings, Belo Horizonte, Brazil, 17–20 June 2013; pp. 201–210. [Google Scholar]
- Wilson, G.W.; Robertson, A.M. The Value of Failure. Geotech. News 2015, 33, 24–28. [Google Scholar]
- Ulrich, B. Practical thoughts regarding filtered tailings. In Proceedings of the 22th International Conference on Tailings and Mine Waste, Cape Town, South Africa, 8–10 May 2019; pp. 71–79. [Google Scholar]
- East, D.; Fernandez, R. Managing Water to Minimize Risk in Tailings Storage Facility Design, Construction, and Operation. Mine Water Environ. 2021, 40, 36–41. [Google Scholar] [CrossRef]
- Fredlund, D.G.; Houston, S.L. Interpretation of soil-water characteristic curves when volume change occurs as soil suction is changed. In Proceedings of the 1st Pan-American Conference on Unsaturated Soils, Cartagena de Indias, Colombia, 20–22 February 2013; pp. 15–31. [Google Scholar]
- Bussière, B. Colloquium 2004: Hydrogeotechnical properties of hard rock tailings from metal mines and emerging geoenvironmental disposal approaches. Can. Geotech. J. 2007, 44, 1019–1052. [Google Scholar] [CrossRef]
- Sánchez, J.A.; Beltra, L.N.; Trujillo-Vela, M.G.; Larrahondo, J.M. Flows of Liquefied Filtered Tailings: Laboratory-Scale Physical and Numerical Modeling. Int. J. Civ. Eng. 2020, 18, 393–404. [Google Scholar] [CrossRef]
- Crystal, C.; Hore, C.; Ezama, I. Filter-Pressed Dry Stacking: Design Considerations Based on Practical Experience. In Proceedings of the 22th International Conference on Tailings and Mine Waste, Keystone, CO, USA, 30 September–2 October 2018; pp. 209–219. [Google Scholar]
- Hore, C.; Luppnow, D. Karara Iron Ore TSF—Design considerations for a unique large scale dry stack facility. In Proceedings of the 18th International Conference on Tailings and Mine Waste, Keystone, CO, USA, 5–8 October 2014; pp. 293–304. [Google Scholar]
- Robertson, P.K.; Da Fonseca, A.V.; Ulrich, B.; Coffin, J. Characterization of unsaturated mine waste: A case history. Can. Geotech. J. 2017, 54, 1752–1761. [Google Scholar] [CrossRef] [Green Version]
- Whatnall, O.; Barber, K.; Robinson, P. Tailings Filtration Using Viper Filtration Technology—A Case Study. Min. Metall. Explor. 2021, 38, 1297–1303. [Google Scholar] [CrossRef]
- Borja, R.N.; Bareither, C.A. Shear Behavior of Waste Rock and Filtered Tailings Mixtures. In Proceedings of the Geo-Congress 2020: Geotechnical Earthquake Engineering and Special Topics, Minneapolis, MI, USA, 25–28 February 2020; pp. 872–880. [Google Scholar]
- Gorakhki, M.R.H.; Bareither, C.A.; Scalia, J.; Aparicio, M. Hydrologic and Environmental Behavior of Geo Waste and Waste Rock in Field Experimental Piles. Min. Metall. Explor. 2021, 38, 1339–1354. [Google Scholar] [CrossRef]
- Ulrich, B.; Coffin, J. Combined tailings and mine waste. In Proceedings of the Tailings and Mine Waste, Vancouver, BC, Canada, 26–28 October 2015; pp. 83–92. [Google Scholar]
- Lee, K.; Sills, G. The consolidation of a soil stratum, including self-weight effects and large strains. Int. J. Numer. Anal. Methods Geomech. 1981, 5, 405–428. [Google Scholar] [CrossRef]
- ASTM Standard D854-14; ASTM Standard Test Methods for Specific Gravity of Soil Solids by Water Pycnometer. ASTM Int.: West Conshohocken, PA, USA, 2014.
- ASTM Standard D2487-17e1; ASTM Standard Practice for Classification of Coils for Engineering Purposes (Unified Soil Classification System). ASTM Int.: West Conshohocken, PA, USA, 2017.
- ASTM Standard D4318-17e1; ASTM Standard Test Methods for Liquid Limit, Plastic Limit, and Plasticity Index of Soils. ASTM Int.: West Conshohocken, PA, USA, 2017.
- ASTM Standard D1557-12; ASTM Standard Test Methods for Laboratory Compaction Characteristics of Soil Using Modified Effort. ASTM Int.: West Conshohocken, PA, USA, 2012.
- ASTM Standard D698-12; ASTM Standard Test Methods for Laboratory Compaction Characteristics of Soil Using Standard Effort. ASTM Int.: West Conshohocken, PA, USA, 2012.
- Dold, B. Evolution of acid mine drainage formation in sulphidic mine tailings. Minerals 2014, 4, 621–641. [Google Scholar] [CrossRef] [Green Version]
- Murray, J.; Nordstrom, D.K.; Dold, B.; Kirschbaum, A. Seasonal fluctuations and geochemical modeling of acid mine drainage in the semi-arid Puna region: The Pan de Azúcar Pb–Ag–Zn mine, Argentina. J. South. Am. Earth Sci. 2021, 109, 103197. [Google Scholar] [CrossRef]
- Akcil, A.; Koldas, S. Acid Mine Drainage (AMD): Causes, treatment and case studies. J. Clean. Prod. 2006, 14, 1139–1145. [Google Scholar] [CrossRef]
- Daliri, F.; Paul, S.; Siva, S. A Comparison of Different Laboratory Techniques to Simulate Stress and Moisture History of Hard Rock Mine Tailings. In Proceedings of the Tailings and Mine Waste 2011 Conference, Vancouver, BC, Canada, 6–9 November 2011; pp. 163–175. [Google Scholar]
- Daliri, F.; Kim, H.; Simms, P.; Sivathayalan, S. Influence of desiccation and overconsolidation on monotonic and cyclic shear response of thickened gold tailings. In Proceedings of the 14th Pan-American Conference on Soil Mechanics and Geotechnical Engineering, Toronto, ON, Canada, 2–6 October 2011; pp. 1–8. [Google Scholar]
- Simms, P.; Grabinsky, M.; Zhan, G. Modelling evaporation of paste tailings from the Bulyanhulu mine. Can. Geotech. J. 2007, 44, 1417–1432. [Google Scholar] [CrossRef]
- Hilf, J.W. An Investigation of Pore-Water in Compacted Cohesive Soils. Technical Memo. No. 654. Ph.D. Thesis, Bureau of Reclamation, Design and Construction Division, U.S. Department of the Interior, Denver, CO, USA, 1956. [Google Scholar]
- ASTM Standard D5298-16; ASTM Standard Test Method for Measurement of Soil Potential (Suction) Using Filter Paper. ASTM Int.: West Conshohocken, PA, USA, 2016. [CrossRef]
- Edlefsen, N.E.; Alfred, B.C.A. Thermodynamics of soil moisture. Hilgardia 1943, 15, 31–298. [Google Scholar] [CrossRef] [Green Version]
- Hoyos, L.R.; Pérez-Ruiz, D.D.; Puppala, A.J. Modeling Unsaturated Soil Response under Suction-Controlled True Triaxial Stress Paths. Int. J. Geomech. 2012, 12, 292–308. [Google Scholar] [CrossRef]
- Schumacher, V.; Comin, A.; Sarricolea, P.; Meseguer-ruiz, O. Comparison between observations and gridded data sets over complex terrain in the Chilean Andes: Precipitation and temperature. Int. J. Climatol. 2020, 40, 5266–5288. [Google Scholar] [CrossRef]
- Fredlund, D.; Morgenstern, N.; Widger, R. The shear strength of unsaturated soils. Can. Geotech. J. 1978, 15, 313–321. [Google Scholar] [CrossRef]
- Wang, Q.; Pufahl, D.; Fredlund, D. A study of critical state on an unsaturated silty soil. Can. Geotech. J. 2002, 39, 213–218. [Google Scholar] [CrossRef]
- Adams, B.A.; Wulfsohn, D.; Fredlund, D.G. Air volume change measurement in unsaturated soil testing using a digital pressure-volume controller. Geotech. Test. J. 1996, 19, 12–21. [Google Scholar] [CrossRef]
- Gardner, W. Some steady-state solutions of the unsaturated moisture flow equation with application to evaporation from a water table. Soil Sci. 1958, 85, 228–232. [Google Scholar] [CrossRef]
- Van Genuchten, M.T. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef] [Green Version]
- Fredlund, D.G.; Xing, A. Equations for the soil-water characteristic curve. Can. Geotech. J. 1994, 31, 521–532. [Google Scholar] [CrossRef]
- Vanapalli, S.K.; Fredlund, D.G.; Pufahl, D.E.; Clifton, A.W. Model for the prediction of shear strength with respect to soil suction. Can. Geotech. J. 1996, 33, 379–392. [Google Scholar] [CrossRef]
- Fredlund, D.G.; Rajardo, H.; Frdlund, M.D. Unsaturated Soil Mechanics in Engineering Practice; Wiley Online Library: Hoboken, NJ, USA, 2012; ISBN 978111813359. [Google Scholar]
- Heshmati, A.A.; Motahari, M.R. Modeling the Dependency of Suction Stress Characteristic Curve on Void Ratio in Unsaturated Soils. KSCE J. Civ. Eng. 2015, 19, 91–97. [Google Scholar] [CrossRef]
- Gao, Y.; Sun, D. Soil-water retention behavior of compacted soil with different densities over a wide suction range and its prediction. Comput. Geotech. 2017, 91, 17–26. [Google Scholar] [CrossRef]
- Cai, G.; Zhou, A.; Sheng, D. Permeability function for unsaturated soils with different initial densities. Can. Geotech. J. 2014, 51, 1456–1467. [Google Scholar] [CrossRef]
- Roy, S.; Rajesh, S. Test apparatus for rapid determination of soil-water retention curve under isotropic loading condition. Geotech. Test. J. 2021, 44, 255–273. [Google Scholar] [CrossRef]
- Islam, T.; Kodikara, J. Interpretation of the loading—Wetting behaviour of compacted. Can. Geotech. J. 2016, 805, 783–805. [Google Scholar] [CrossRef] [Green Version]
- Zou, W.; Han, Z.; Vanapalli, S.K.; Zhang, J.; Zhao, G. Predicting volumetric behavior of compacted clays during compression. Appl. Clay Sci. 2018, 156, 116–125. [Google Scholar] [CrossRef]
- Burton, G.; Pineda, J.; Sheng, D.; Airey, D.; Zhang, F. Exploring one-dimensional compression of compacted clay under constant degree of saturation paths. Geotechnique 2016, 66, 435–440. [Google Scholar] [CrossRef]
- Kimoto, S.; Oka, F.; Fukutani, J.; Yabuki, T.; Nakashima, K. Monotonic and cyclic behavior of unsaturated sandy soil under drained and fully undrained conditions. Soils Found. 2011, 51, 663–681. [Google Scholar] [CrossRef] [Green Version]









| * Tons (Mt) | Facility | Region | Mineral | Conditions | Approval Date |
|---|---|---|---|---|---|
| 14.8 | El Peñón | II | Gold | Inactive | 01-2003 |
| 129.6 | La Coipa | III | Gold, silver | Inactive | 02-2005 |
| 49.8 | Ampliación El Peñón | II | Gold, silver | Active | 12-2010 |
| 138.2 | Mantos Blancos | II | Copper | Active | 07-2018 |
| 1.2 | Tambo de Oro | IV | Gold | Active | 03-2018 |
| 2.8 | Filtrados 2 | III | Copper, molybdenum | In construction | 04-2019 |
| 3.3 | El Toqui | XI | Zinc, gold | Inactive | 06-2011 |
| 0.73 | Guanaco | II | Copper | Active | 08-2016 |
| 1.9 | Convertidor Teniente | III | Copper, molybdenum | Active | 12-2016 |
| 39.4 | Planta San José | III | Copper | In construction | 03-2016 |
| 21 | Manto Verde | II | Copper | Active | ** 02-2021 |
| 6.8 | Faena Tambillos | IV | Copper | Active | 07-2018 |
| 3.7 | Escondida | II | Copper | Inactive | 11-2016 |
| 1.1 | Diego de Almeyda | III | Copper | Inactive | 10-2017 |
| 24.5 | Kozan | III | Copper | In construction | 10-2019 |
| 22.2 | Salares Norte | III | Gold, silver | In construction | 04-2020 |
| Property | Value | Testing Procedure |
|---|---|---|
| Gs | 2.97 | ASTM D854-14 [22] |
| D10, D30, D50, D60 (μm) | 3, 15, 65, 112 | ASTM D2487-17e1 [23] |
| Cu, Cc | 37.3, 1 | ASTM D2487-17e1 [23] |
| LL, PL, PI | 18, 15, 3 | ASTM D4318-17e1 [24] |
| γd,max (kN/m3)S.P *, M.P ** | 20.3, 21.95 | ASTM 2012 D698-12; D1557-12 [25,26] |
| Clasification (USCS) | ML | ASTM D2487-17e1 [23] |
| pH | Leached Iron (%) | Leached Copper (%) |
|---|---|---|
| 2 | 0.012 | 0.08 |
| 10 | <0.00004 | <0.00005 |
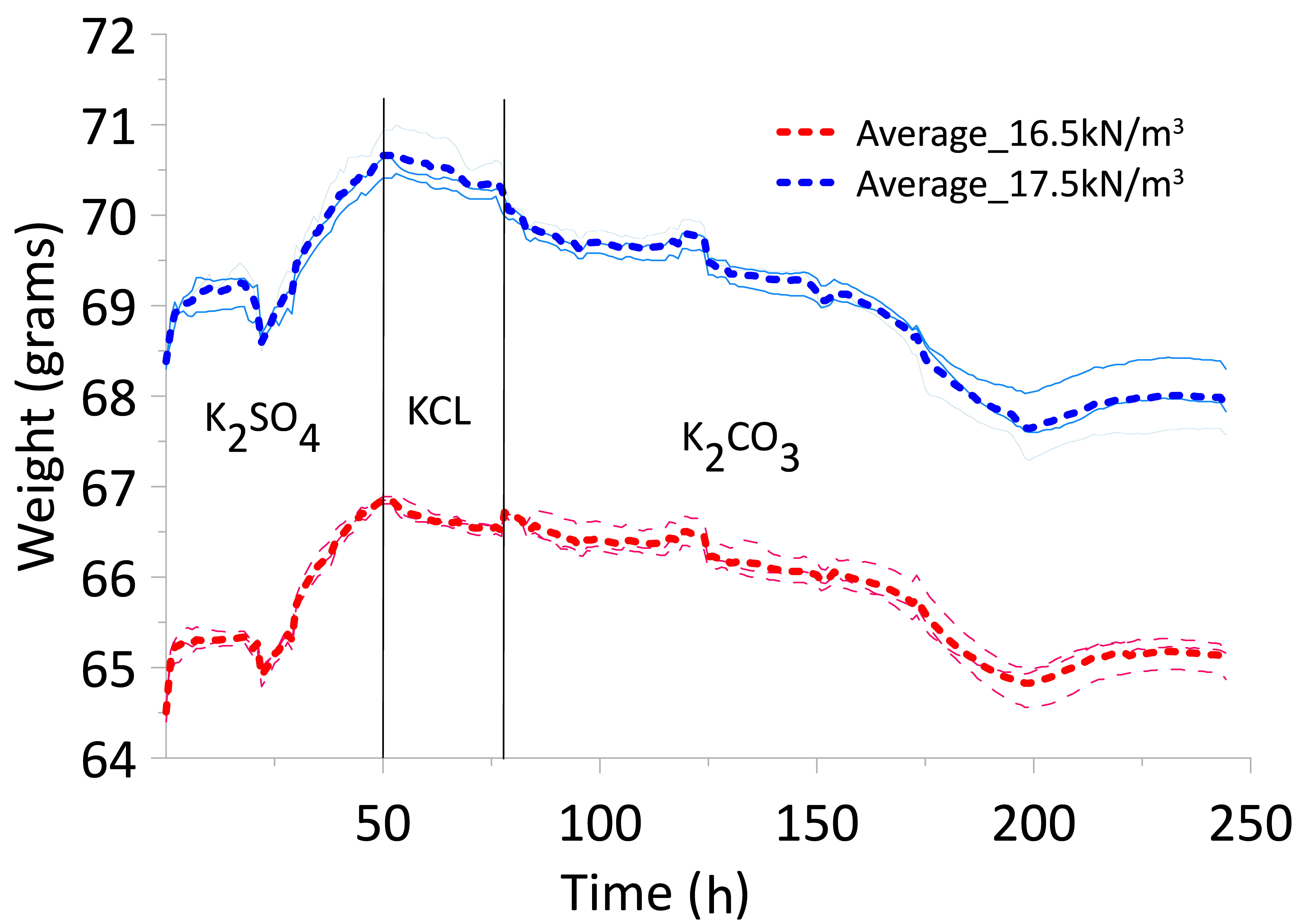
| Salt | RH (%) Reached | T (°C) Equilibrium | Total Suction (MPa) |
|---|---|---|---|
| K2SO4 | 96.2% | 20.2 | 4.1 |
| KCl | 92.3% | 19.1 | 23.5 |
| K2CO3 | 51.2% | 17.6 | 110.1 |
| Test | Sample ID | Cell Pressure (kPa) | Back Pressure (kPa) | Air Pressure (kPa) | γd (kN/m3) |
|---|---|---|---|---|---|
| 1D Consolidation | - | 0 | 0 | 0 | 16.5 |
| - | 150 | 100 | 150 | 16.5 | |
| - | 200 | 100 | 200 | 16.5 | |
| - | 300 | 100 | 300 | 16.5 | |
| - | 500 | 100 | 500 | 16.5 | |
| - | 0 | 0 | 0 | 17.5 | |
| - | 150 | 100 | 150 | 17.5 | |
| - | 200 | 100 | 200 | 17.5 | |
| - | 300 | 100 | 300 | 17.5 | |
| - | 500 | 100 | 500 | 17.5 | |
| CID | Sat_1 | 450 | 400 | 0 | 16.5 |
| Sat_2 | 500 | 400 | 0 | 16.5 | |
| Sat_3 | 600 | 400 | 0 | 16.5 | |
| Unsat_1 | 110 | 10 | 60 | 16.5 | |
| Unsat_2 | 160 | 10 | 60 | 16.5 | |
| Unsat_3 | 260 | 10 | 60 | 16.5 | |
| Sat_4 | 1040 | 990 | 0 | 17.5 | |
| Sat_5 | 1040 | 940 | 0 | 17.5 | |
| Sat_6 | 1040 | 840 | 0 | 17.5 | |
| Unsat_4 | 110 | 10 | 60 | 17.5 | |
| Unsat_5 | 160 | 10 | 60 | 17.5 | |
| Unsat_6 | 260 | 10 | 60 | 17.5 |
| Model | Equation | Variable | γd = 16.5 kN/m3 | γd = 17.5 kN/m3 |
|---|---|---|---|---|
| Gardner (1958) [41] | θs | 0.45 | 0.41 | |
| θr | 0.01 | 0.01 | ||
| α | 100 | 200 | ||
| n | 0.55 | 0.6 | ||
| Van Genuchten (1980) [42] | θs | 0.45 | 0.41 | |
| θr | 0.01 | 0.01 | ||
| 8 | 18 | |||
| n | 1.28 | 1.34 | ||
| m | 0.24 | 0.24 | ||
| Fredlund and Xing (1994) [43] | θs | 0.45 | 0.41 | |
| θr | 0.01 | 0.01 | ||
| α | 60 | 200 | ||
| n | 0.66 | 0.66 | ||
| m | 2 | 2.4 |
| γd (kN/m3) | ψ (kPa) | CC | CS |
|---|---|---|---|
| 16.5 | 0 | 0.152 | 0.018 |
| 50 | 0.235 | 0.016 | |
| 100 | 0.230 | 0.017 | |
| 200 | 0.226 | 0.016 | |
| 400 | 0.224 | 0.016 | |
| 17.5 | 0 | 0.120 | 0.021 |
| 50 | 0.231 | 0.015 | |
| 100 | 0.226 | 0.016 | |
| 200 | 0.209 | 0.016 | |
| 400 | 0.202 | 0.015 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gallardo Sepúlveda, R.; Sáez Robert, E.; Camacho-Tauta, J. Assessment of the Self-Compaction Effect in Filtered Tailings Disposal under Unsaturated Condition. Minerals 2022, 12, 422. https://doi.org/10.3390/min12040422
Gallardo Sepúlveda R, Sáez Robert E, Camacho-Tauta J. Assessment of the Self-Compaction Effect in Filtered Tailings Disposal under Unsaturated Condition. Minerals. 2022; 12(4):422. https://doi.org/10.3390/min12040422
Chicago/Turabian StyleGallardo Sepúlveda, Ricardo, Esteban Sáez Robert, and Javier Camacho-Tauta. 2022. "Assessment of the Self-Compaction Effect in Filtered Tailings Disposal under Unsaturated Condition" Minerals 12, no. 4: 422. https://doi.org/10.3390/min12040422
APA StyleGallardo Sepúlveda, R., Sáez Robert, E., & Camacho-Tauta, J. (2022). Assessment of the Self-Compaction Effect in Filtered Tailings Disposal under Unsaturated Condition. Minerals, 12(4), 422. https://doi.org/10.3390/min12040422






