Infrared Thermography: A Method to Visualise and Analyse Sulphide Oxidation
Abstract
1. Introduction
2. Materials and Methods
2.1. Samples and Sample Processing
2.2. Mineralogical and Geochemical Analyses
2.3. Infrared Thermography Method
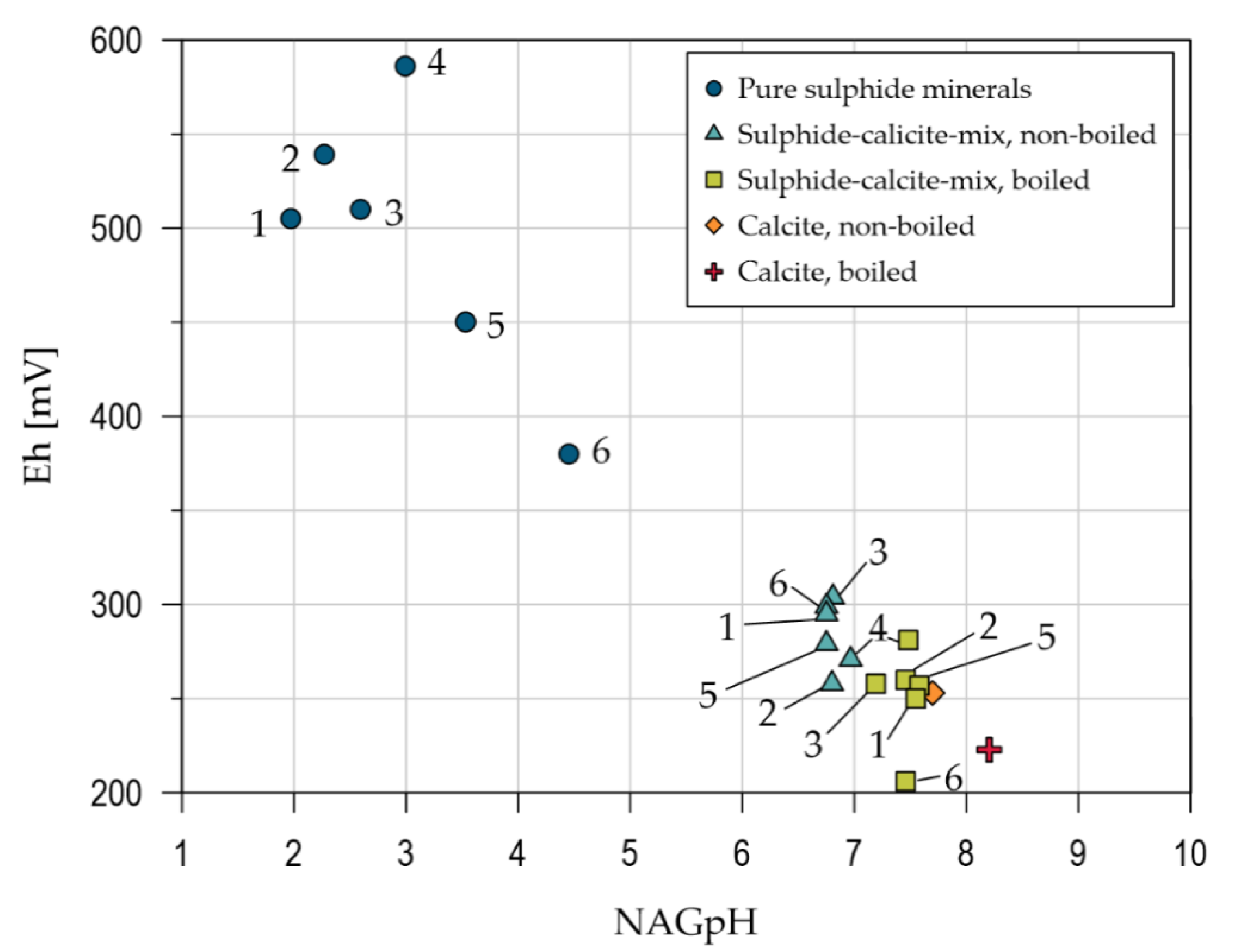
3. Results
3.1. Sample Characteristics
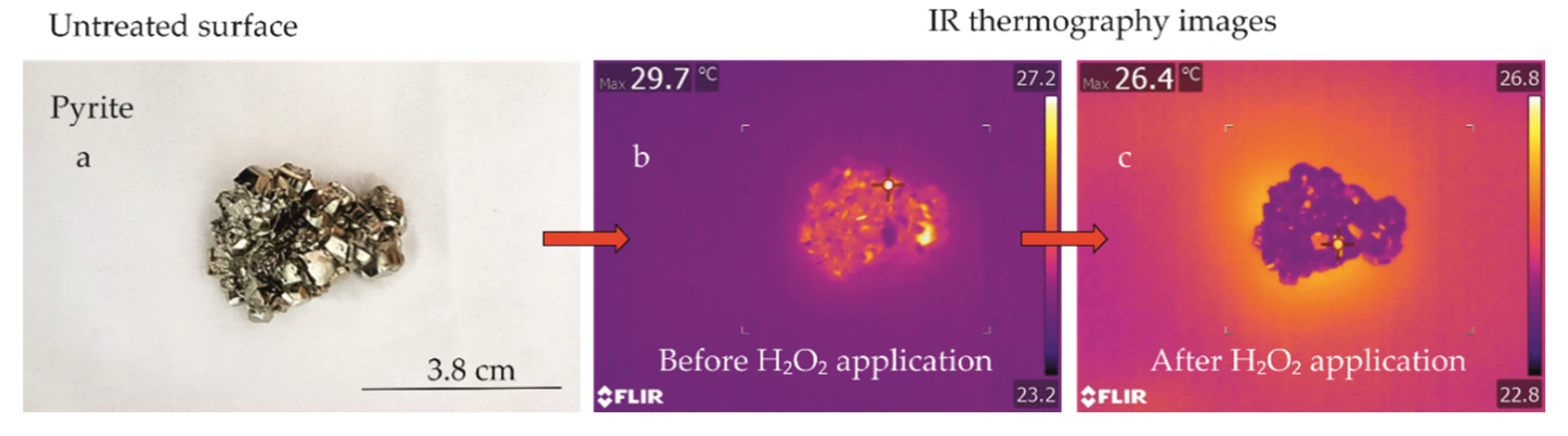
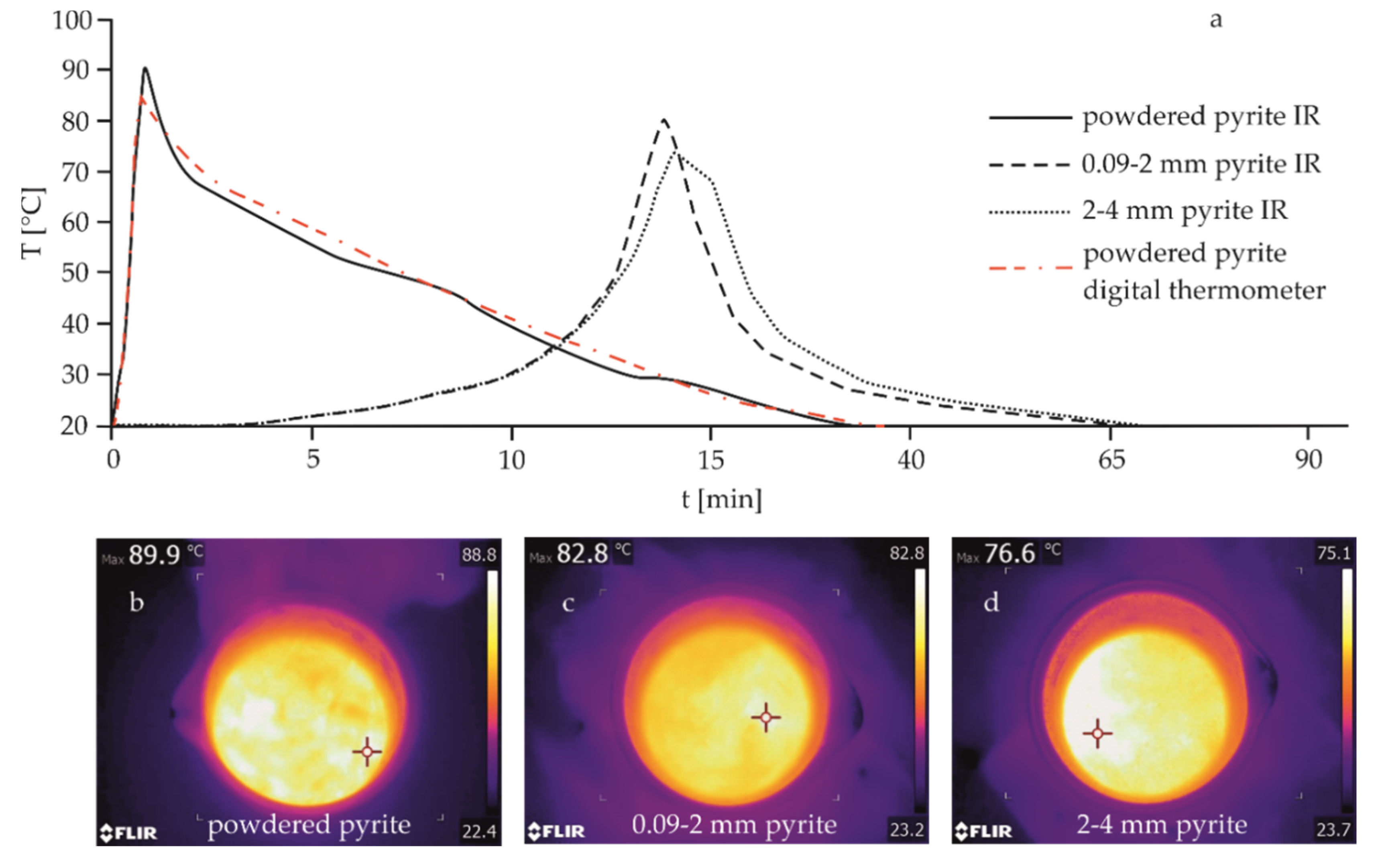
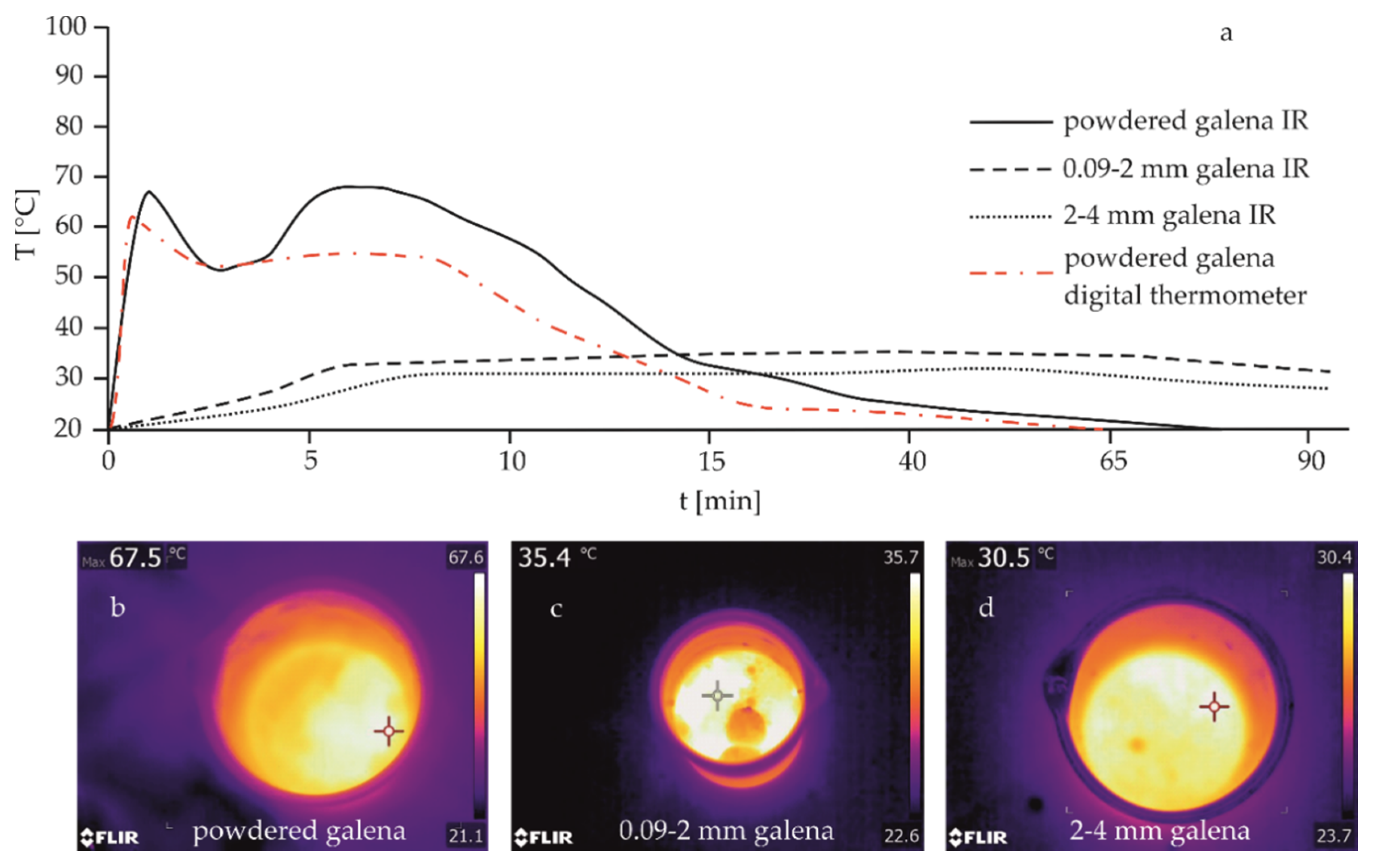
3.2. Infrared Thermography
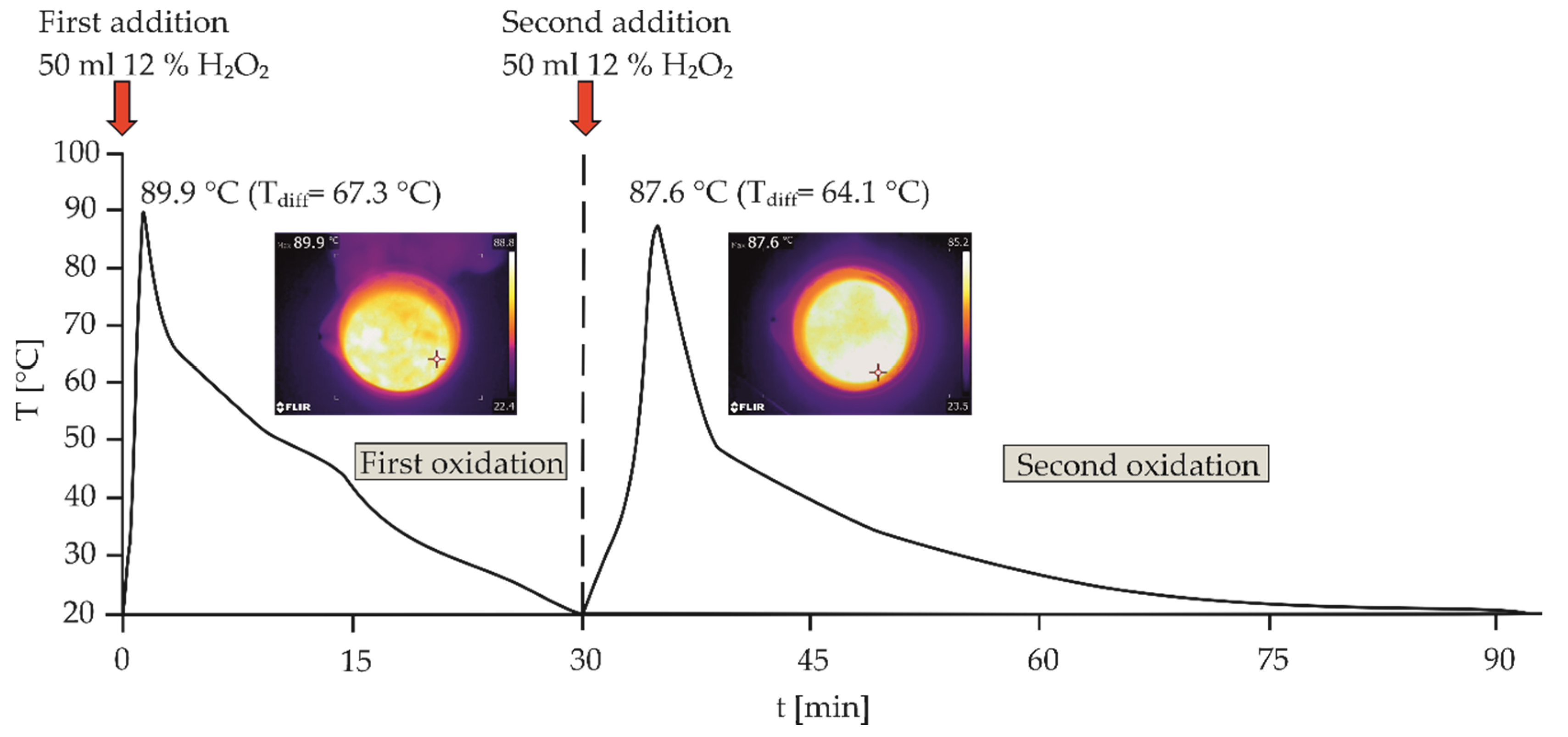
3.2.1. Pilot Study
3.2.2. Thermography Method
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Lottermoser, B.G. Preface. In Environmental Indicators in Metal Mining; Lottermoser, B.G., Ed.; Springer International Publishing: Berlin/Heidelberg, Germany, 2017; pp. v–viii. ISBN 978-3-319-42729-4. [Google Scholar]
- Parbhakar-Fox, A.; Lottermoser, B.G. A critical review of acid rock drainage prediction methods and practices. Miner. Eng. 2015, 82, 107–124. [Google Scholar] [CrossRef]
- Vink, B.W. Stability relations of antimony and arsenic compounds in the light of revised and extended Eh-pH diagrams. Chem. Geol. 1996, 130, 21–30. [Google Scholar] [CrossRef]
- Ashley, P.M.; Craw, D.; Graham, B.P.; Chappell, D.A. Environmental mobility of antimony around mesothermal stibnite deposits, New South Wales, Australia and southern New Zealand. J. Geochem. Explor. 2003, 77, 1–14. [Google Scholar] [CrossRef]
- Wilson, S.C.; Lockwood, P.V.; Ashley, P.M.; Tighe, M. The chemistry and behaviour of antimony in the soil environment with comparisons to arsenic: A critical review. Environ. Pollut. 2010, 158, 1169–1181. [Google Scholar] [CrossRef]
- INAP. Prediction tools. In Global Acid Rock Drainage Guide (GARD). Available online: www.gardguide.com/index.php?title=Main_Page (accessed on 2 October 2020).
- Hageman, P.L. U.S. Geological Survey Field Leach Test for Assessing Water Reactivity and Leaching Potential of Mine Wastes, Soils, and Other Geologic and Environmental Materials; Techniques and Methods; U.S. Department of the Interior, U.S. Geological Survey: Reston, VA, USA, 2007; ISBN 978-1-4113-1798-7.
- Ackerman, L.; Rohovec, J.; Šebek, O. Determination of total sulfur in fifteen geological materials using inductively coupled plasma-optical emission spectrometry (ICP-OES) and combustion/infrared spectrometry. Geostand. Geoanal. Res. 2012, 36, 407–414. [Google Scholar] [CrossRef]
- Okai, T.; Terashima, S.; Imai, N. Determination of total sulfur in thirty one geochemical reference materials using an inductively coupled plasma-atomic emission spectrometer fitted with a semiconductor photodiode detector. Geostand. Geoanal. Res. 2001, 25, 133–136. [Google Scholar] [CrossRef]
- Terashima, S. Determination of total carbon and sulfur in fifty-two geochemical reference samples by combustion and infrared absorption spectrometry. Geostand. Geoanal. Res. 1988, 12, 249–252. [Google Scholar] [CrossRef]
- Jackson, L.L.; Roof, S.R. Determination of the forms of carbon in geologic materials. Geostand. Newslett. 1992, 16, 317–323. [Google Scholar] [CrossRef]
- Paktunc, A.D. Mineralogical constraints on the determination of neutralising potential and prediction of acid mine drainage. Environ. Geol. 1999, 39, 103–112. [Google Scholar] [CrossRef]
- Dold, B. Acid rock drainage prediction: A critical review. J. Geochem. Explor. 2017, 172, 120–132. [Google Scholar] [CrossRef]
- Coastec Research Inc. A Manual of Chemical Evaluation Procedures for the Prediction of Acid Generation from Mine Wastes; Energy, Mines and Resources, Canada, MEND Report 1.16.1 (b); Coastec Research Inc.: Vancouver, BC, Canada, 1991; p. 77. [Google Scholar]
- Lapakko, K.A. Evaluation of neutralization potential determinations for metal mine waste and a proposed alternative. Am. Soc. Min. Reclam. 1994, 129–137. [Google Scholar] [CrossRef]
- Price, W.A.; Errington, J.C. Guidelines for metal leaching and acid rock drainage at minesites in British Columbia. In Proceedings of the Fourth International Conference on Acid Rock Drainage, Vancouver, BC, Canada, 31 May–6 June 1997; Volume 1, pp. 15–30. [Google Scholar]
- Lawrence, R.W.; Wang, Y. Determination of neutralization potential in the prediction of acid rock drainage. In Proceedings of the Fourth International Conference on Acid Rock Drainage, Vancouver, BC, Canada, 31 May–6 June 1997; pp. 449–464. [Google Scholar]
- Karlsson, T.; Räisänen, M.L.; Lehtonen, M.; Alakangas, L. Comparison of static and mineralogical ARD prediction methods in the Nordic environment. Environ. Monit. Assess. 2018, 190, 1–29. [Google Scholar] [CrossRef] [PubMed]
- Weber, P.A.; Thomas, J.E.; Skinner, W.M.; Smart, R.S.C. Calculated acid base balance for H2O2 oxidation of carbonate-poor pyritic mine-rock. Canad. Mineral. 2005, 43, 1193–1203. [Google Scholar] [CrossRef]
- Weber, P.A.; Thomas, J.E.; Skinner, W.M.; Smart, R.S.C. A methodology to determine the acid-neutralization capacity of rock samples. Canad. Mineral. 2005, 43, 1183–1192. [Google Scholar] [CrossRef]
- Stewart, W.A.; Miller, S.D.; Smart, R. Advances in acid rock drainage (ARD) characterisation of mine wastes. In Proceedings of the 7th ICARD, St. Louis, MO, USA, 26–30 March 2006; pp. 2098–2119. [Google Scholar]
- Deopker, R.D.; O’Connor, W.K. Column leach study 1: Heavy metal dissolution characteristics from selected copper mine tailings. Mine Water Environ. 1991, 10, 57–71. [Google Scholar] [CrossRef]
- Noble, T.L.; Lottermoser, B.G.; Parbhakar-Fox, A. Evaluation of pH testing methods for sulfidic mine waste. Mine Water Environ. 2016, 35, 318–331. [Google Scholar] [CrossRef]
- Oh, C.; Ji, S.; Yim, G.; Cheong, Y. Evaluation of net acid generation pH as a single indicator for acid forming potential of rocks using geochemical properties. Environ. Monit. Assess. 2017, 189, 165. [Google Scholar] [CrossRef]
- Smart, R.; Skinner, W.M.; Gerson, A.R.; Thomas, J.E.; Sobieraj, H.; Schumann, R.; Weisener, C.G.; Weber, P.A.; Miller, S.D.; Stewart, W.A. ARD Test Handbook: Project P387, A Prediction & Kinetic Control of Acid Mine Drainage; AIMIRA International Ltd.: Melbourne, Australia, 2002. [Google Scholar]
- Reimers, G.W.; Franke, D.F. Effect of Additives on Pyrite Oxidation; Report of investigations; United States Bureau of Mines: Washington, DC, USA, 1991; p. 13.
- Banks, D.; Skarphagen, H.; Wiltshire, R.; Jessop, C. Heat pumps as a tool for energy recovery from mining wastes. In Energy, Waste and the Environment: A Geochemical Perspective; Gieré, R., Stille, P., Eds.; The Geological Society: London, UK, 2004; pp. 499–514. [Google Scholar]
- Shamsuddin, M. Roasting of Sulfide Minerals. In Physical Chemistry of Metallurgical Processes; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2016; pp. 39–69. ISBN 978-1-119-07832-6. [Google Scholar]
- Miller, S.D.; Jeffery, J.J.; Donohue, T.A. Development in predicting and managament of acid forming mine watses in Australia and Southeast Asia. In Proceedings of the International Land Reclamation and Mine Drainage Conference and the Third International Conference on the Abatement of Acidic Drainage, Pittsburgh, PA, USA, 24–29 April 1994; pp. 177–184. [Google Scholar]
- Miller, S.D.; Jeffery, J.J.; Murray, G.S.C. Identification and management of acid generating mine wastes-procedures and practices in south-east Asia and the Pacific regions. Int. J. Mine Water 1990, 9, 57–67. [Google Scholar] [CrossRef]
- Miller, S.D.; Jeffery, J.J.; Wong, J.W.C. Use and misuse of the acid-base account for AMD prediction. In Proceedings of the Conference on the Abattement of Acidic Drainage, Montreal, QC, Canada, 16–18 September 1991; pp. 489–506. [Google Scholar]
- Sapsford, D.J.; Bowell, R.J.; Dey, M.; Williams, M.C.; Williams, K.P. A comparison of kinetic NAG tests with static and humidity cell tests for the prediction of ARD. In Proceedings of the 10th International Mine Water Association Congress, Karlovy Vary, Czech Republic, 2–5 June 2008; pp. 1–4. [Google Scholar]
- Weber, P.A.; Hughes, J.B.; Conner, L.B.; Lindsay, P.; Smart, S.C.R. Short-term acid rock drainage characteristics determined by paste pH and kinetic NAG testing: Cypress prospect, New Zealand. In Proceedings of the 7th ICARD, St. Louis, MO, USA, 26–30 March 2006; pp. 2289–2310. [Google Scholar]
- Henke, S.; Karstädt, D.; Möllmann, K.P.; Pinno, F.; Vollmer, M. Identification and suppression of thermal reflections in infrared thermal imaging. Proc. Inframation 2004, 5, 287–298. [Google Scholar]
- Noble, T.L.; Lottermoser, B.G. Modified abrasion pH and NAGpH testing of minerals. In Environmental Indicators in Metal Mining; Lottermoser, B., Ed.; Springer International Publishing: Berlin/Heidelberg, Germany, 2017; pp. 211–220. ISBN 978-3-319-42729-4. [Google Scholar]
- Kononov, V.A. Infrared Thermography of Loose Hangingwalls; CSIR: Pretoria, South Africa, 2002; p. 102. [Google Scholar]
- Vraetz, T.; Baltes, R.; Fietz, N.; Nienhaus, K. Acoustic emission technology and thermal imaging for material flow characterization in mining. Min. Rep. 2017, 153, 461–467. [Google Scholar]
- Zhai, X.; Wu, S.; Wang, K.; Drebenstedt, C.; Zhao, J. Environment influences and extinguish technology of spontaneous combustion of coal gangue heap of Baijigou coal mine in China. Energy Procedia 2017, 136, 66–72. [Google Scholar] [CrossRef]
- Fierro, V.; Miranda, J.L.; Romero, C.; Andrés, J.M.; Pierrot, A.; Gómez-Landesa, E.; Arriaga, A.; Schmal, D. Use of infrared thermography for the evaluation of heat losses during coal storage. Fuel Process. Technol. 1999, 60, 213–229. [Google Scholar] [CrossRef]
- Carpentier, O.; Defer, D.; Antczak, E.; Duthoit, B. The use of infrared thermographic and GPS topographic surveys to monitor spontaneous combustion of coal tips. Appl. Therm. Eng. 2005, 25, 2677–2686. [Google Scholar] [CrossRef]
- Liu, H.; Wu, C. A new approach to detect fire source underground mine for preventing spontaneous combustion of sulfide ores. Procedia Eng. 2010, 7, 318–326. [Google Scholar] [CrossRef]
- Mishra, R.K.; Bahuguna, P.P.; Singh, V.K. Detection of coal mine fire in jharia coal field using Landsat-7 ETM+ data. Int. J. Coal Geol. 2011, 86, 73–78. [Google Scholar] [CrossRef]
- Hu, Z.; Xia, Q. An integrated methodology for monitoring spontaneous combustion of coal waste dumps based on surface temperature detection. Appl. Therm. Eng. 2017, 122, 27–38. [Google Scholar] [CrossRef]
- Tomescu, C.; Prodan, M.; Vatavu, N.; Chiuzan, E. Monitoring the work environment using thermal imaging cameras in order to prevent the self-ignition of coal. Environ. Eng. Manag. J. 2017, 16, 1389–1393. [Google Scholar] [CrossRef]
- Joshi, P.K.; Punia, A. Thermal infrared imaging to identify surface mines. Mine Water Environ. 2019, 38, 700–704. [Google Scholar] [CrossRef]
- Liu, H.; Wu, C.; Shi, Y. Locating method of fire source for spontaneous combustion of sulfide ores. J. Cent. South Univ. Technol. 2011, 18, 1034–1040. [Google Scholar] [CrossRef]
- Ghosh, A.; Sharma, A.K.; Nayak, B.; Sagar, S.P. Infrared thermography: An approach for iron ore gradation. Miner. Eng. 2014, 62, 85–90. [Google Scholar] [CrossRef]
- Shannon, H.R.; Sigda, J.M.; Van Dam, R.L.; Hendrickx, J.M.H.; McLemore, V.T. Thermal camera imaging of rock piles at the questa molybdenum mine, questa, New Mexico. JASMR 2005, 2005, 1015–1028. [Google Scholar] [CrossRef]
- Stylianou, M.; Tsiftes, K.; Gavriel, I.; Kostarelos, K.; Demetriou, C.; Papaioannou, A. Environmental impacts of abandoned sulphide mines -the example of mathiatis mine in cyprus. In Proceedings of the SYMBIOSIS International Conference, Athens, Greece, 19–21 June 2014; p. 7. [Google Scholar]
- Garrels, R.M.; Thompson, M.E. Oxidation of pyrite by iron sulfate solutions. Amer. J. Sci. 1960, 258, 57–67. [Google Scholar]
- Singer, P.C.; Stumm, W. Acidic mine drainage: The rate-determining step. Science 1970, 167, 1121–1123. [Google Scholar] [CrossRef] [PubMed]
- Haynes, W.M. CRC Handbook of Chemistry and Physics: A Ready-Reference Book of Chemical and Physical Data, 97th ed.; CRC Press: Boca Raton, FL, USA, 2017; ISBN 978-1-4987-5428-6. [Google Scholar]
- Lefebvre, R.; Gelinas, P.; Isabel, D. Heat Transfer during Acid Mine Drainage Production in a Waste Rock Dump, La Mine Doyon (Québec); University Laval: Québec QC, Canada, 1997; p. 106. [Google Scholar]
- Zhang, Y.; Li, Q.; Liu, X.; Xu, B.; Yang, Y.; Jiang, T. A thermodynamic analysis on the roasting of pyrite. Minerals 2019, 9, 220. [Google Scholar] [CrossRef]
- Dunn, J.G. The oxidation of sulphide minerals. Thermochim. Acta 1997, 300, 127–139. [Google Scholar] [CrossRef]
- Pérez-Tello, M.; Sohn, H.Y.; Löttiger, J. Determination of the oxidation characteristics of solid copper matte particles by differential scanning calorimetry and thermogravimetric analysis. Min. Metall. Explor. 1999, 16, 1–7. [Google Scholar] [CrossRef]
- Wang, H.; Pring, A.; Xie, Y.; Ngothai, Y.; O’neill, B. Phase evolution and kinetics of the oxidation of monosulfide solid solution under isothermal conditions. Thermochim. Acta 2005, 427, 13–25. [Google Scholar] [CrossRef]
- Deore, S. Oxide melt solution calorimetry of sulfides: Enthalpy of formation of sphalerite, galena, greenockite, and hawleyite. Am. Mineral. 2006, 91, 400–403. [Google Scholar] [CrossRef]
- Pérez-Fontes, S.E.; Pérez-Tello, M.; Prieto-López, L.O.; Brown, F.; Castillón-Barraza, F. Thermoanalytical study on the oxidation of sulfide minerals at high temperatures. Min. Metall. Explor. 2007, 24, 275–283. [Google Scholar] [CrossRef]
- Bylina, I.; Trevani, L.; Mojumdar, S.C.; Tremaine, P.; Papangelakis, V.G. Measurement of reaction enthalpy during pressure oxidation of sulphide minerals. J. Therm. Anal. Calorim. 2009, 96, 117–124. [Google Scholar] [CrossRef]
- Iliyas, A.; Hawboldt, K.; Khan, F. Thermal stability investigation of sulfide minerals in DSC. J. Hazard. Mater. 2010, 178, 814–822. [Google Scholar] [CrossRef]
- Iliyas, A.; Hawboldt, K.; Khan, F. Kinetics and safety analysis of sulfide mineral self-heating: Part 1. Effect of mineralogy. J. Therm. Anal. Calorim. 2011, 106, 53–61. [Google Scholar] [CrossRef]
- Bylina, I.V.; Mojumdar, S.C.; Papangelakis, V.G. Effect of storage time on the pressure oxidation enthalpy of pyrite. J. Therm. Anal. Calorim. 2012, 108, 829–835. [Google Scholar] [CrossRef]
- Yang, F.; Wu, C.; Li, Z. Spontaneous combustion tendency of fresh and pre-oxidized sulfide ores. J. Cent. South Univ. 2014, 21, 715–719. [Google Scholar] [CrossRef]
- Liu, H.; Xiang, C.; Hong, R.; Song, Y.; Jin, K.; Zhu, K.; Yang, C.; Lv, C. Thermal behavior and kinetics of sulfide concentrates. Therm. Sci. 2019, 23, 2801–2811. [Google Scholar] [CrossRef]
- Teong, S.P.; Li, X.; Zhang, Y. Hydrogen peroxide as an oxidant in biomass-to-chemical processes of industrial interest. Green. Chem. 2019, 21, 5753–5780. [Google Scholar] [CrossRef]
- Golchoubian, H.; Hosseinpoor, F. Effective oxidation of sulfides to sulfoxides with hydrogen peroxide under transition-metal-free conditions. Molecules 2007, 12, 304–311. [Google Scholar] [CrossRef]
- Lin, S.-S.; Gurol, M.D. Catalytic Decomposition of hydrogen peroxide on iron oxide: Kinetics, mechanism, and implications. Environ. Sci. Technol. 1998, 32, 1417–1423. [Google Scholar] [CrossRef]
- De Laat, J.; Gallard, H. Catalytic decomposition of hydrogen peroxide by Fe(III) in homogeneous aqueous solution: Mechanism and kinetic modeling. Environ. Sci. Technol. 1999, 33, 2726–2732. [Google Scholar] [CrossRef]
- Huang, H.-H.; Lu, M.-C.; Chen, J.-N. Catalytic decomposition of hydrogen peroxide and 2-chlorophenol with iron oxides. Water Res. 2001, 35, 2291–2299. [Google Scholar] [CrossRef]
- Hermanek, M.; Zboril, R.; Medrik, I.; Pechousek, J.; Gregor, C. Catalytic efficiency of iron(III) oxides in decomposition of hydrogen peroxide: Competition between the surface area and crystallinity of nanoparticles. J. Am. Chem. Soc. 2007, 129, 10929–10936. [Google Scholar] [CrossRef] [PubMed]
- Stewart, W.A.; Miller, S.D.; Smart, R.; Gerson, A.R.; Thomas, J.E.; Skinner, W.M.; Levay, G.; Schumann, R. Evaluation of the net acid generation (NAG) test for Assessing the acid generation capacity of sulphide minerals. In Proceedings of the 6th ICARD, Queensland, Australia, 14–17 July 2003; pp. 617–625. [Google Scholar]
- Evangelou, V.P. Pyrite Oxidation and its Control: Solution Chemistry, Surface Chemistry, Acid Mine Drainage (AMD), Molecular Oxidation Mechanisms, Microbial Role, Kinetics, Control, Ameliorates and Limitations, Microencapsulation, 1st ed.; CRC Press: Boca Raton, FL, USA, 1995; ISBN 978-0-203-74164-1. [Google Scholar]
- Elberling, B. Environmental controls of the seasonal variation in oxygen uptake in sulfidic tailings deposited in a permafrost-affected area. Water Resour. Res. 2001, 37, 99–107. [Google Scholar] [CrossRef]
- Elberling, B. Temperature and oxygen control on pyrite oxidation in frozen mine tailings. Cold Reg. Sci. Technol. 2005, 41, 121–133. [Google Scholar] [CrossRef]
- Antonijević, M.M.; Dimitrijević, M.; Janković, Z. Leaching of pyrite with hydrogen peroxide in sulphuric acid. Hydrometallurgy 1997, 46, 71–83. [Google Scholar] [CrossRef]
- Chirita, P. Pyrite oxidation by hydrogen peroxide in phosphoric acid solutions. Eur. J. Miner. Process. Environ. 2004, 4, 203–209. [Google Scholar]
- Liu, W.; Xu, L.; Li, X.; Shen, C.; Rashid, S.; Wen, Y.; Liu, W.; Wu, X. High-dispersive FeS 2 on graphene oxide for effective degradation of 4-chlorophenol. RSC Adv. 2015, 5, 2449–2456. [Google Scholar] [CrossRef]
- Lefticariu, L.; Schimmelmann, A.; Pratt, L.M.; Ripley, E.M. Oxygen isotope partitioning during oxidation of pyrite by H2O2 and its dependence on temperature. Geochim. Cosmochim. Acta 2007, 71, 5072–5088. [Google Scholar] [CrossRef]
- Dold, B.; Fontboté, L. A mineralogical and geochemical study of element mobility in sulfide mine tailings of Fe oxide Cu–Au deposits from the Punta del Cobre belt, northern Chile. Chem. Geol. 2002, 189, 135–163. [Google Scholar] [CrossRef]
- Vaughan, D.J.; Becker, U.; Wright, K. Sulphide mineral surfaces: Theory and experiment. Int. J. Miner. Process. 1997, 51, 1–14. [Google Scholar] [CrossRef]
- Rimstidt, J.D.; Chermak, J.A.; Gagen, P.M. Rates of reaction of galena, sphalerite, chalcopyrite, and arsenopyrite with Fe(III) in acidic solutions. In Environmental Geochemistry of Sulfide Oxidation; ACS Symposium Series; Alpers, C.N., Blowes, D.W., Eds.; American Chemical Society: Washington, DC, USA, 1993; Volume 550, pp. 2–13. ISBN 978-0-8412-2772-9. [Google Scholar]
- Chopard, A.; Benzaazoua, M.; Plante, B.; Bouzahzah, H.; Marion, P. Kinetic tests to evaluate the relative oxidation rates of various sulfides and sulfosalts. In Proceedings of the 10th ICARD, Santiago, Chile, 21–24 April 2015; pp. 1–10. [Google Scholar]
- Mok, W.M.; Wai, C.M. Mobilization of arsenic in contaminated river waters. In Arsenic in the Environment. Part 1 Cycling and Characterization; Nriagu, J.O., Ed.; John Wiley Interscience: New York, NY, USA, 1994; pp. 99–108. [Google Scholar]
- Dold, B. Basic concepts in environmental geochemistry of sulfidic mine-waste management. In Waste Management; Kumar, S., Ed.; IntechOpen: Rijeka, Croatia, 2010; pp. 173–198. ISBN 978-953-7619-84-8. [Google Scholar]
- Hoefer Chemie GmbH Safety Data Sheet According to Regulation (EC) No 1907/2006. 2012, 1–10. Available online: https://www.hoefer-chemie.de/upload/SDB/Wasserstoffperoxid11.9.pdf (accessed on 2 October 2020).
- Steger, H.F. Oxidation of sulfide minerals. Chem. Geol. 1982, 35, 281–295. [Google Scholar] [CrossRef]
- Lottermoser, B. Acid producing reactions. In Mine Wastes; Lottermoser, B.G., Ed.; Springer: Berlin/Heidelberg, Germany, 2010; Volume 3, pp. 46–60. ISBN 978-3-642-12418-1. [Google Scholar]
- Yazıcı, E.Y.; Deveci, H. Factors Affecting decomposition of hydrogen peroxide. In Proceedings of the XIIth international mineral processing symposium, Cappadocia-Nevsehir, Turkey, 6–8 October 2010; pp. 609–616. [Google Scholar]
- Lengke, M.F.; Tempel, R.N. Geochemical modeling of arsenic sulfide oxidation kinetics in a mining environment. Geochim. Cosmochim. Acta 2005, 69, 341–356. [Google Scholar] [CrossRef]
- Evangelou, V.P.; Zhang, Y.L. A review: Pyrite oxidation mechanisms and acid mine drainage prevention. Crit. Rev. Environ. Sci. Technol. 1995, 25, 141–199. [Google Scholar] [CrossRef]
- Rosso, K.M. Reactivity of sulfide mineral surfaces. Rev. Mineral. Geochem. 2006, 61, 557–607. [Google Scholar] [CrossRef]
- Barnes, A.; Bowell, R.J.; Warrender, R.; Sapsford, D.J.; Sexsmith, K.; Charles, J.; Declercq, J.; Santonastaso, M.; Dey, M. Comparison between long-Term humidity cell testing and static net acid generation (NAG) tests: Potential for NAG use in preliminary mine site water quality predictions. In Proceedings of the 10th ICARD, Santiago, Chile, 21–24 April 2015; pp. 1–10. [Google Scholar]
- Weber, P.A.; Stewart, W.A.; Skinner, W.M.; Weisener, C.G.; Thomas, J.E.; Smart, R.S.C. Geochemical effects of oxidation products and framboidal pyrite oxidation in acid mine drainage prediction techniques. Appl. Geochem. 2004, 19, 1953–1974. [Google Scholar] [CrossRef]
- Kwong, Y.T.J. Prediction and Prevention of Acid Rock Drainage from a Geological and Mineralogical Perspective; MEND Project 1.32.1. National Hydrology Research Institute: Saskatoon, SK, Canada, 1993. [Google Scholar]

| Parameter | Description |
|---|---|
| Tstart | Initial temperature of the sample material |
| T0 | First reaction temperature; recorded immediately after the addition of 50 mL of 12% H2O2 |
| Tx | Reaction temperature of the indicated minute |
| Tmax | Highest recorded temperature |
| Tdiff | Absolute temperature increase; difference between the maximum temperature (Tmax) and the initial temperature (Tstart) |
| tmax | Time taken to reach the maximum temperature (Tmax) |
| ttotal | Total reaction time |
| Sample | Material | Content (wt%) |
|---|---|---|
| 1 | Chalcopyrite (CuFeS2) | 100 |
| 2 | Galena (PbS) | 100 |
| 3 | Sphalerite (ZnS) | 91 |
| Galena (PbS) | 8 | |
| Quartz (SiO2) | 1 | |
| 4 | Pyrite (FeS2) | 85 |
| Rhomboclase (H5Fe3+O2(SO4)2·2(H2O) | 12 | |
| Szomolnokite (Fe2+SO4·H2O) | 3 | |
| 5 | Stibnite (Sb2S3) | 81 |
| Quartz (SiO2) | 19 | |
| 6 | Arsenopyrite (FeS2) | 69 |
| Szomolnokite (Fe2+SO4·H2O) | 20 | |
| Siderite (FeCO3) | 5 | |
| Quartz (SiO2) | 6 | |
| 7 | Calcite (CaCO3) | 100 |
| 8 | Quartz (SiO2) | 100 |
| Sample | Fe (wt%) | Cu (ppm) | Pb (ppm) | Zn (ppm) | As (ppm) | Sb (ppm) | Other Trace Elements (≤1000 ppm) |
|---|---|---|---|---|---|---|---|
| Pyrite | 42.13 | 143 | 132 | 71 | 280 | 79 | Co |
| Chalcoyprite | 20.20 | 347,800 | <8 | 2020 | <3 | 131 | Ag, Co |
| Arsenopyrite | 20.62 | 174 | <4 | 89 | 402,700 | 266 | Co, Cr, Sn, W |
| Stibnite | 0.05 | 77 | 74 | <3 | 138 | 508,000 | Cd |
| Sphalerite | 7.62 | 736 | 61,700 | 502,300 | 3240 | 180 | Ag, Cd, Cr, Mn (16,000 ppm), Mo, Ni, |
| Galena | <0.002 | 284 | 556,300 | 1067 | 33,360 | 990 | Cr, Ni, W |
| Mineral | Fraction Size (mm) | Tmax (°C) | tmax (min) | Tdiff (°C) | ttotal (min) | Corresponding Figure |
|---|---|---|---|---|---|---|
| Pyrite | powdered | 89.9 | 1 | 69.8 | 30 | Figure 3a |
| 0.09–2 | 82.8 | 13 | 62.8 | 65 | Figure 3b | |
| 2–4 | 76.6 | 14 | 56.4 | 70 | Figure 3c | |
| Galena | powdered | 67.5 | 1 | 47.6 | 75 | Figure 4a |
| 0.09–2 | 35.4 | 20 | 15.2 | >90 | Figure 4b | |
| 2–4 | 30.5 | 45 | 10.3 | >90 | Figure 4c | |
| Chalcopyrite | powdered | 89.6 | 2 | 69.6 | 50 | Supplementary Figure S4a |
| 0.09–2 | 66.2 | 75 | 45.9 | >90 | Supplementary Figure S4b | |
| 2–4 | 21.5 | >90 | 1.5 | >90 | Supplementary Figure S4c | |
| Arsenopyrite | powdered | 83.3 | 1 | 63.0 | 45 | Supplementary Figure S5a |
| 0.09–2 | 24.4 | >90 | 4.3 | >90 | Supplementary Figure S5b | |
| 2–4 | 20.9 | >90 | 0.6 | >90 | Supplementary Figure S5c | |
| Stibnite | powdered | 87.9 | 1 | 67.8 | 70 | Supplementary Figure S6a |
| 0.09–2 | 24.3 | >90 | 4.4 | >90 | Supplementary Figure S6b | |
| 2–4 | 21.1 | >90 | 1.1 | >90 | Supplementary Figure S6c | |
| Sphalerite | powdered | 87.9 | 2 | 67.8 | 55 | Supplementary Figure S7a |
| 0.09–2 | 28.1 | >90 | 7.9 | >90 | Supplementary Figure S7b | |
| 2–4 | 23.7 | >90 | 3.4 | >90 | Supplementary Figure S7c |
| Mineral | Fraction Size | Integral ∫ (K∙s) |
|---|---|---|
| Pyrite | powdered | 258 × 102 |
| Chalcopyrite | powdered | 228 × 102 |
| Arsenopyrite | powdered | 231 × 102 |
| Stibnite | powdered | 314 × 102 |
| Galena | powdered | 349 × 102 |
| Sphalerite | powdered | 272 × 102 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Knobloch, M.; Lottermoser, B.G. Infrared Thermography: A Method to Visualise and Analyse Sulphide Oxidation. Minerals 2020, 10, 933. https://doi.org/10.3390/min10110933
Knobloch M, Lottermoser BG. Infrared Thermography: A Method to Visualise and Analyse Sulphide Oxidation. Minerals. 2020; 10(11):933. https://doi.org/10.3390/min10110933
Chicago/Turabian StyleKnobloch, Marjan, and Bernd G. Lottermoser. 2020. "Infrared Thermography: A Method to Visualise and Analyse Sulphide Oxidation" Minerals 10, no. 11: 933. https://doi.org/10.3390/min10110933
APA StyleKnobloch, M., & Lottermoser, B. G. (2020). Infrared Thermography: A Method to Visualise and Analyse Sulphide Oxidation. Minerals, 10(11), 933. https://doi.org/10.3390/min10110933





